Hemispherical and Flat Head Cylindrical Specimen Taylor Impact
-
摘要: 为研究不同头型对泰勒（Taylor）撞击载荷的影响，在材料静动态力学性能实验和Taylor撞击实验的基础上进行数值模拟，分析试件在撞击过程中的接触力、速度和外形尺寸等参量的变化历程。结果表明：在相同的撞击速度下，半球头试件的塑性变形程度更大；半球头试件的撞击载荷上升沿变缓，载荷脉冲时间增长，而两种试件的载荷平台段幅值相当。根据实验和数值模拟结果，进一步分析了霍普金森杆测试Taylor撞击载荷历程的适用性。Abstract: In order to understand the impact loading of Taylor impact process with head shape changes, numerical simulation studies were carried out on the basis of static and dynamic mechanical performance experiments and Taylor impact experiments, and the contact force, velocity and dimensions of the specimen during the impact process were studied. The change history of the parameters is analyzed. The results show that: under the same impact speed, the plastic deformation of the hemispherical head specimen is greater; the impact load of the hemispherical head specimen has a slower rising edge and the load pulse time increases, and the amplitude of the load platform section of the two specimens is almost the same. According to the results of experiments and numerical simulations, the applicability of Hopkinson bar measuring Taylor impact load history was further analyzed and discussed.
-
Key words:
- Taylor impact /
- hemispherical head /
- impact load /
- strain measuring
-
战斗部爆炸后，破片的空间分布是确定破片杀伤作用场的一个重要指标。传统战斗部起爆后形成的破片场在弹轴方向上以一定的锥角向空中飞散，在环向上则为均匀分布，在一定弹目交汇条件下，仅有10%的破片能够对目标产生有效破坏[1-2]。为了提升战斗部在轴向上对目标的毁伤能力和毁伤效率，使破片在轴向上平行飞散甚至聚焦飞散成为一种技术途径，研究破片轴向飞散控制及预测的方法显得十分重要[3]。
战斗部的装药结构、壳体和炸药材料、端部约束、引爆方式等均会对破片飞散特性产生影响，国内外许多学者都对此类课题展开了相应研究。Taylor[4]最早提出了长柱壳装药结构下抛板速度和轴向飞散角之间的半经验公式，得到了科学界和工程界的广泛应用。许多学者对Taylor公式进行修正和改进，其中Shapiro提出的非柱壳结构装药的破片飞散角公式被广泛应用于工程实践中[5]。Held[6]运用Taylor公式给出了偏心起爆下战斗部弹体截面内破片径向速度的Gurney方程。国内众多学者也在相关领域展开了充分探索，为破片战斗部破片飞散控制的工程应用提供了重要依据。在轴向聚焦战斗部的设计应用方面，李晋庆等[7-8]讨论了聚焦型破片战斗部的聚焦曲线工程设计，给出了可用于工程设计的计算方法和工程计算实例，并通过靶场实验研究了不同起爆方式对战斗部聚焦性能的影响。黄广炎等[9]分析了一端起爆下破片微元的飞散机理，结合破片初速的端部修正，得到了一种基于微元柱的破片飞散方向沿轴线分布的计算方法。他们采用光学凹面镜反射原理对战斗部壳体进行设计，得到轴向聚焦战斗部的一种设计方法，并进行了实验验证[10]。Chen等[11]通过对战斗部壳体进行设计，运用大量仿真研究了不同结构的平行式战斗部的破片飞散特性，为平行式破片战斗部设计提供了思路。
现有研究表明，破片飞散控制可有效提高破片在目标区域的密度，从而提高战斗部的杀伤威力和毁伤效率。轴向聚焦式战斗部作为其中的典型代表，能够对目标进行“切割式”毁伤。本研究在前人工作的基础上，以圆柱形轴向聚焦式战斗部为研究对象，运用仿真程序对战斗部破片的飞散特性进行研究，从而实现战斗部结构和性能的优化。
1. 战斗部壳体设计
战斗部壳体受爆轰波驱动作用向空中飞散，为了对破片的空间分布进行预测和控制，达到优化战斗部毁伤性能的目的，常常需要预测破片的初始飞散速度和飞散角度。在工程实践中，Shapiro公式被广泛应用于战斗部破片飞散角的计算。如图 1(a)所示，在过战斗部轴线的平面内，壳体由一定长度的微元组成，DBA为其中一段，O为起爆点，AA′、BB′为爆轰波波阵面，波速为De，在Δt时间内壳体微元AB运动到CB位置，壳体破片初始速度为v0，壳体初始法线方向为n。爆轰波方向、初始法线方向、破片初始速度方向与弹轴的夹角分别为φ1、φ2和φ3，破片初始速度方向与初始法线方向的夹角为θs，显然θs=φ1－φ3。
计算破片飞散角的Shapiro公式[5]为
tanθs=v04Decos(θs+φ2) (1) 破片初始速度v0的计算公式[12]为
v0=√2Em/mc+0.5 (2) 式中：m为壳体和破片的总质量，mc为装药质量, √2E为炸药的Gurney常数[5]。
基于Shapiro公式，采用微元法根据破片在靶板上的落点分布对战斗部壳体母线进行设计。设计思路如图 1(b)所示，在过战斗部轴线的二维平面内将战斗部壳体划分成固定长度的微元，其长度设为h，取z轴方向为战斗部轴线方向，x轴为过战斗部中心与z轴相垂直的方向。
设战斗部的直径为L，高度为H，弹靶距离为M，靶板上破片分布区域的长度为N，起爆点为O。将壳体微元从上到下依次编号为1、2、3…。对于1号壳体微元，其上端点的坐标为(L/2，H/2)，记为(X1，Z1)，在靶板上的落点坐标为(M，N/2)，由几何三角关系可知
φ2=arctanLH (3) φ3=π2+arctan(H−N)/2M−N/2=π2+arctanH−N2M−L (4) 将(3)式和(4)式代入(1)式，并结合φ1=φ3+θs可得
tan(φ1−arctanH−N2M−L)=−v04Decos(φ1−arctanLH) (5) 破片战斗部的壳体结构主要包括内、外壳体，称重壳，破片等。记内、外壳体厚度为h1，破片层厚度为h2，承重壳厚度为h3，则微元1处的爆炸载荷系数β1的表达式为
β1=mcm=ρ1(X1−2h1−h2)22ρ2h1(2X1−h1−h2)+ρ2h3(X1+d)+0.524ρ3[2h2(X1−h1)−h22] (6) 式中：ρ1为炸药密度；ρ2为内、外壳体密度；ρ3为破片密度；d为承重壳与外壳的间距，即辅药厚度。
获得以上参数后，通过对(5)式进行迭代计算得到角度φ1的数值解。于是微元1的下端点坐标为(X1－hsinφ1，Z1+hcosφ1)，此坐标也是微元2的上端点坐标，微元2的落点位置为(M，N/2-N/H)。按照上述方法进行递推计算，可得到所有壳体微元的端点坐标，对所有端点坐标进行曲线拟合便可得到战斗部壳体母线的解析表达式。
选择RDX为主装药，装药直径130 mm，高130 mm，Gurney常数为2 834 m/s，对战斗部壳体进行设计，得到聚焦式、平行式和发散式3种不同结构的战斗部, 如表 1所示。
表 1 3种战斗部的壳体母线设计参数Table 1. Shell parameters of 3 kinds of warheadsNo. Warhead mode Detonation mode M/m Fragment distribution Fitting radius/mm 1# Focusing Symmetrical point 3.5 Focusing 711 2# Parallel Symmetrical point 3.5 Uniform distribution (130 mm in axis direction) 1 076 3# Emanative Symmetrical point 3.5 Uniform distribution (300 mm in axis direction) 1 700 预制破片的直径为8 mm，故取壳体微元长度为8 mm，对微元端点坐标进行求解，结果如图 2所示。为了方便建立战斗部模型以及后期实验加工设计，对微元端点进行二次圆弧拟合，即将壳体母线简化拟合成一段圆弧，得到的拟合圆弧与微元端点重合度较好，说明圆弧拟合能够较为真实地反映壳体母线的形状特征，可以使用圆弧建立战斗部模型。
2. 数值计算模型
2.1 有限元模型
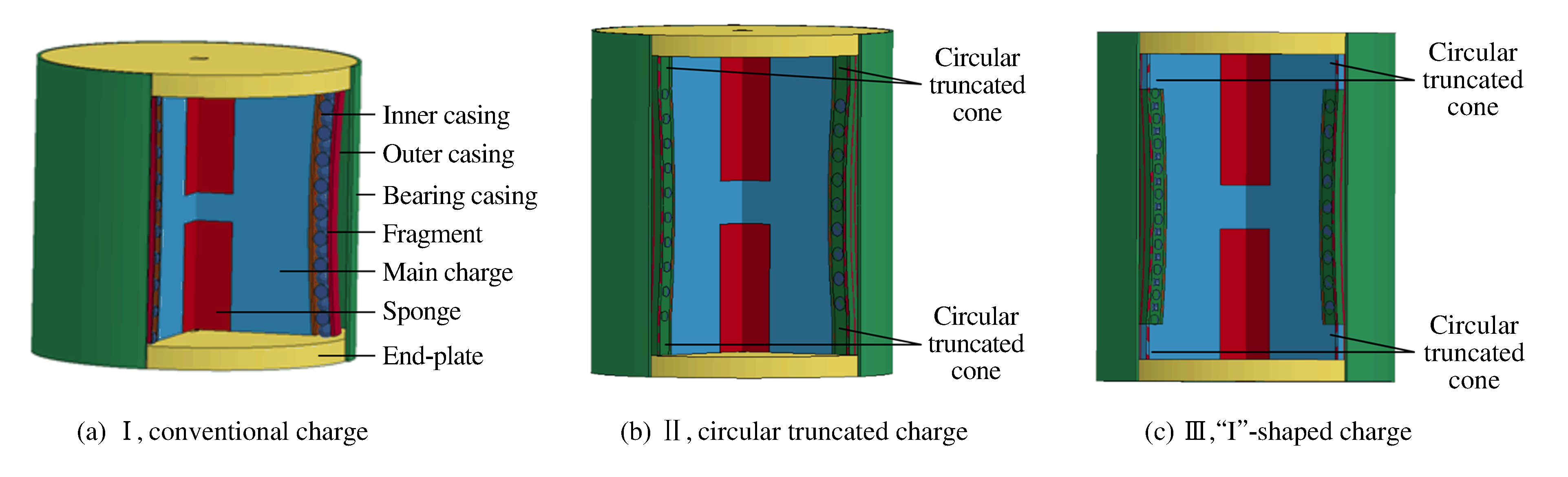
传统的轴向聚焦式战斗部模型如图 3(a)所示，记为Ⅰ型战斗部。使用ALE方法建模，炸药、海绵、环氧树脂和空气采用Euler网格，内壳、外壳、承重壳、破片和端盖采用Lagrange网格。战斗部中心有一个海绵填充的中心孔，用于放置起爆的扩爆药柱，扩爆药柱简化为和主装药相同的炸药类型。主装药起爆后，其能量通过ALE耦合算法传递给壳体和破片，驱动破片向外飞散形成破片场。
在设计壳体母线的过程中忽略了战斗部端部效应的影响，但实际情况是爆轰波会在战斗部端部反射稀疏波，导致破片飞散角增大, 从而影响破片轴向飞散控制的效率。为了消除端部效应的影响，设计了如图 3(b)和图 3(c)所示的装药结构，其中：Ⅱ型战斗部在Ⅰ型战斗部的基础上添加了高20 mm、无破片的圆台结构；Ⅲ型战斗部则对该圆台结构进行改进，将主装药填充到圆台结构中，高度仍然为20 mm，形成了“工”字形的装药结构。
2.2 材料模型及状态方程
战斗部模型由炸药、空气、壳体、破片等组成，各部分材料参数如表 2所示。为了有效实现对战斗部爆炸过程的仿真，对壳体和端盖材料使用了应变失效准则[13]：失效应变为0.4，即当单元应变大于0.4时单元失效，材料发生断裂。
表 2 各部分材料及材料参数Table 2. Parameter of different materialsComponent Material Density/(g·cm-3) Material model Equation of state Explosive RDX 1.75 High_Explosive_Burn JWL Null Air 0.001 29 Null Linear_Polynomial Epoxy Epoxy 1.198 Null Grüneisen Sponge Sponge 0.315 Null Grüneisen Casing 45 steel 7.85 Elastic_Plastic_Hydro_Spall Grüneisen End-plate LY12 2.73 Elastic_Plastic_Hydro_Spall Grüneisen Fragment screw 45 steel 7.89 Plastic_Kinematic 3. 仿真结果及分析
3.1 3种装药结构战斗部的破片飞散特性
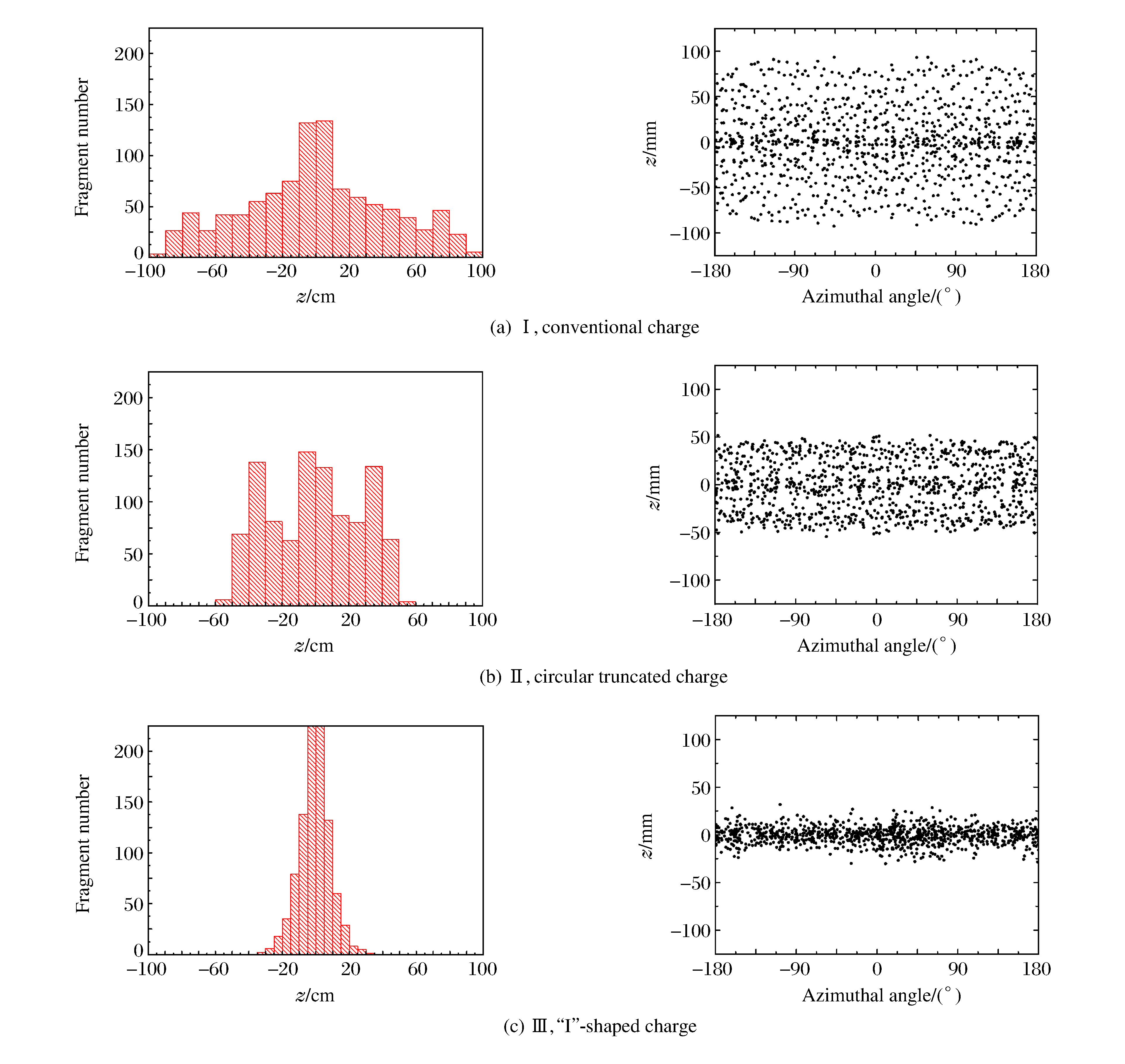
选择聚焦型壳体参数，对3种不同装药结构战斗部的破片飞散过程进行仿真计算，并利用破片搜索软件[14]对破片场进行搜索，得到破片在3.5 m远处靶板上的分布，如图 4所示。观察破片在z轴(即战斗部轴向)上的分布发现，Ⅱ型战斗部破片在轴向上明显比Ⅰ型战斗部集中。在3.5 m远的靶板上：Ⅰ型战斗部破片分布在(-100 cm, 100 cm)区间上，并且仅在(-10 cm, 10 cm)区间上分布较为集中，占总破片数的26.41%；Ⅱ型战斗部破片集中分布在(-50 cm, 50 cm)区间上，占总破片数的99.01%，远大于Ⅰ型在此区间内的72.10%；Ⅲ型战斗部破片主要分布在(-10 cm, 10 cm)区间上，占总破片数的76.84%，远大于Ⅰ型和Ⅱ型战斗部的数据。这说明圆台结构，特别是Ⅲ型战斗部结构能够最大限度地消除端部效应的影响，达到破片聚焦的目的。
图 4 不同装药结构战斗部在3.5 m远处靶板上的破片分布Figure 4. Distribution of warhead fragments with different charge structures on the 3.5 m-away target图 5给出了Ⅰ型和Ⅲ型装药结构在爆炸过程中的压力等值面变化。在12 μs时刻，两种装药结构中的炸药爆轰完毕，爆轰波作用在破片上并推动破片向外飞散。在20 μs时刻：由于端部稀疏波的作用，Ⅰ型装药结构的压力等值面在端部开始发生凹陷，端部破片附近逐渐形成低压力区域，内外压力差使破片获得向外的轴向速度；而Ⅲ型装药结构的稀疏波只影响了圆台部分爆轰产物的压力等值面，破片端面仍然保持一个较高的压力值。在24和30 μs时刻：在稀疏波作用的持续影响下，Ⅰ型装药结构的压力等值面凹陷越来越大，端部低压区域越来越大，受端部低压区影响的破片越来越多，破片的轴向飞散速度也逐渐增大；而Ⅲ型装药结构的压力等值面仍然包裹着破片，破片仍然处在较为均匀的压力驱动下，轴向飞散速度变化较小。在40 μs时刻：Ⅰ型装药结构的高压等值面的宽度变得很窄，导致破片在轴向上进一步发散，此时破片在轴向上呈圆弧状排布(破片发散的标志)；而Ⅲ型装药结构的高压等值面仍然较宽，破片排布和初始时刻相比没有太大变化，破片保持较好的轴向聚焦飞散。
图 5 Ⅰ型和Ⅲ型装药结构在爆炸过程中的压力等值面变化Figure 5. Isoface of pressure in type Ⅰ and type Ⅲ charges3.2 不同圆台高度战斗部的破片飞散特性
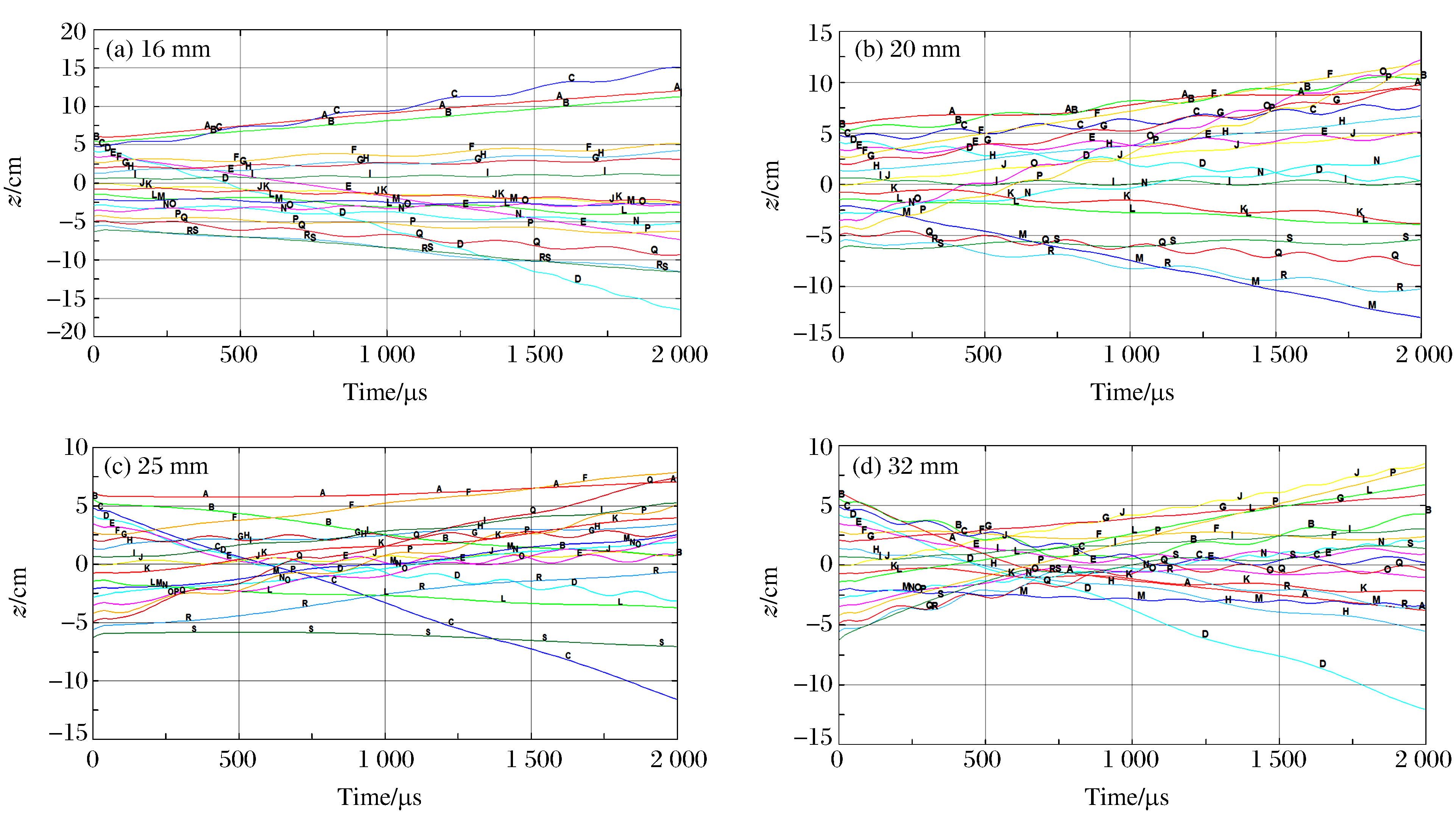
为了研究Ⅲ型——“工”字形装药结构的圆台高度对破片飞散的影响，为聚焦型壳体选取16、20、25和32 mm 4种不同高度的圆台结构，对战斗部破片飞散特性进行仿真，得到破片在3.5 m远处靶板上的轴向分布，如图 6所示。图 6中每个点的数据表示以该点为中心、宽5 cm区间内的破片数，对破片数量进行高斯拟合，得到不同圆台高度下战斗部破片分布的拟合曲线。
图 6 不同圆台高度战斗部在3.5 m远处靶板上的破片分布统计Figure 6. Distribution of fragments on the 3.5 m-awaytarget with different platform heights由图 6的拟合曲线可知，4种圆台高度下，战斗部破片在轴向上的分布均较为集中，但具体分布不同。16 mm圆台战斗部的破片分布相对分散，在(-25 cm, 25 cm)区间内有85.19%的破片；随着圆台高度的增加，破片分布越来越集中，20和25 mm圆台战斗部在(-15 cm, 15 cm)区间内的破片数分别达到总数的89.66%和87.01%；当圆台高度为32 mm时，破片分布的集中程度反而下降。观察高斯拟合曲线发现，20和25 mm圆台战斗部的破片聚焦效果最好，其中25 mm圆台战斗部更好，但优势并不大。综合聚焦效果和战斗部有效长度两方面因素，确定Ⅲ型战斗部的合理圆台高度为20 mm。
取4种战斗部壳体母线上的一列破片，记录它们在2 000 μs内的飞散轨迹，如图 7所示。图 7(a)中，几乎所有破片在轴向上都呈发散状向外飞散，因此破片在靶板上的分布较为分散；图 7(b)和图 7(c)中，大部分破片平行向外飞散，另一部分则向内汇聚飞散，故破片在靶板上分布很集中；在图 7(d)中，几乎所有破片都向内汇聚飞散，但由于汇聚点在靶板之前，破片汇聚后又呈发散状继续飞散，因此破片在靶板上的汇聚效果反而不如20和25 mm圆台战斗部。由此可见，即使再增加圆台高度，破片在靶板上的分布也不会更集中，反而会更分散。
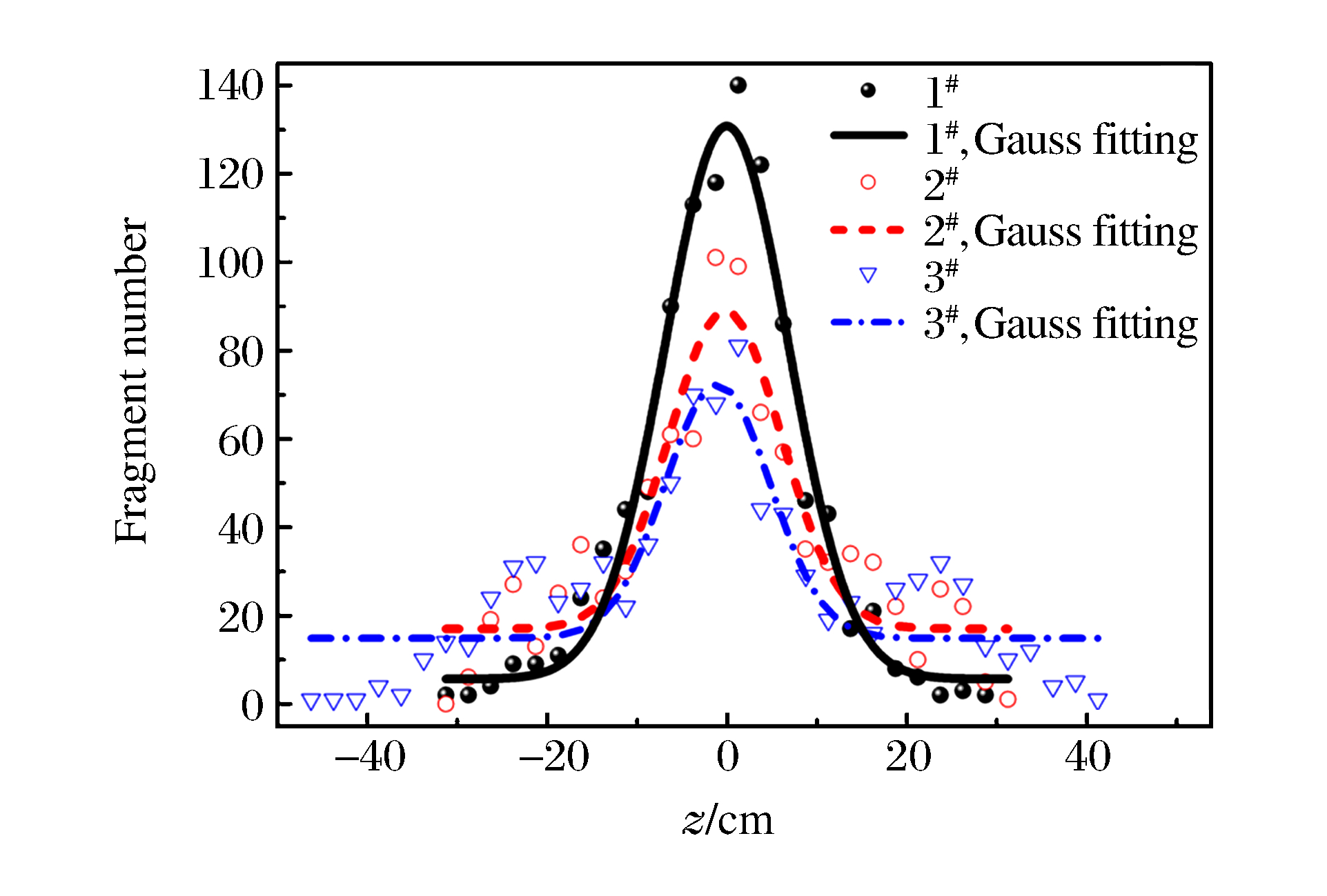
图 7 不同圆台高度战斗部的破片飞散轨迹(A~S表示不同节点)Figure 7. Trace of fragments with different platform heights (Different nodes represented by A-S)3.3 不同母线曲率战斗部的破片飞散特性
选取Ⅲ型战斗部装药结构，圆台高度定为20 mm，对表 1中不同曲率半径的1#、2#和3#壳体进行仿真，破片在3.5 m远处靶上的轴向分布如图 8所示，其中每个数据点表示以该点为中心、宽2.5 cm区间内的破片数量。观察破片分布的高斯拟合曲线可知，聚焦式、平行式和发散式战斗部的破片集中程度依次减小，证明通过设计壳体曲线控制破片飞散是可行的。定义破片轴向分布的目标区域为该类型战斗部设计时要求破片落向的区域，不同战斗部在目标区域内的破片分布情况列于表 3。1#、2#和3#战斗部在目标区域内的破片数分别达到了总破片数的25.64%、44.40%和59.22%，分布是普通圆柱形战斗部在相应区域内的破片数百分比的2.44、2.23和1.48倍，且破片在目标区域内分布较为均匀，说明通过Shapiro公式对壳体进行曲线设计从而控制破片飞散的方法切实有效。以上结果表明，在不改变战斗部大小的情况下，通过设计壳体母线曲率，可以实现战斗部在目标区域内的破片数增益，从而达到高效毁伤的目的。
图 8 不同壳体母线的战斗部在3.5 m远靶板上的破片分布Figure 8. Distribution of fragments on the 3.5 m-awaytarget with different generatrixes表 3 目标区域内破片统计Table 3. Fragments in specific target areaNo. Area/cm Fragment number Percent/% Gain 1# (-2.5，2.5) 258 25.64 1.44 2# (-6.5, 6.5) 396 44.40 1.23 3# (-15.0，15.0) 517 59.22 0.48 4. 结论
(1) 基于Shapiro公式设计了实现破片不同程度聚焦的战斗部壳体曲线，并利用LS-DYNA计算了破片飞散特性，验证了战斗部壳体曲线设计的合理性。
(2) 研究了战斗部装药结构对破片飞散结果的影响，提出“工”字形圆台装药结构，并对其进行仿真验证，确定了“工”字形圆台的合理高度为20 mm。
(3) 当“工”字形圆台的高度为20 mm时，聚焦式、平行式和发散式3种不同聚焦程度的战斗部壳体型面均能使破片在各自目标区域内的破片数量实现较大增益，分别达到了1.44、1.23和0.48。
-
图 2 3A21铝合金静、动态变形条件下的应力-应变曲线
Figure 2. Quasi-static and dynamic engineering stress-strain curve of 3A21 aluminum alloy
图 3 不同撞速实验后两种试件的典型形貌
Figure 3. Typical morphology of two specimens after different impact velocity experiments
图 4 两种形状试件初始外形和最终变形比较
Figure 4. The initial shape and the final dimensions of the two kinds of specimen
图 8 试件最终长度和最大直径的比较
Figure 8. Comparison of the final length andmaximum diameter of the specimen
图 10 试件长度和接触面直径随时间的变化
Figure 10. Variation of specimen length andcontact surface diameter with time
图 11 沿轴线距撞击面不同位置的节点速度（150 m/s）
Figure 11. Node velocity at different positions along the axis from the impact plane (150 m/s)
图 12 半球头试件的最终长度、接触面直径和最大直径
Figure 12. The final length, contact surface diameter and maximum diameter of the hemispherical head specimen
图 13 半球头试件撞击靶杆的载荷曲线
Figure 13. Load curves of the hemispherical head specimen hitting target bar
图 14 半球头试件长度和接触面直径随时间的变化历程
Figure 14. Change course of the hemispherical head specimenlength and contact surface diameter with time
图 15 半球头试件沿轴线距撞击面不同距离节点的速度
Figure 15. Node velocity at different positions along the axis from the impact plane
图 16 两种头型试件的最大等效塑性应变
Figure 16. The maximum effective plastic strain of specimens with two types of head
图 17 撞击速度150 m/s时两种头型试件的载荷历程
Figure 17. Load history of specimens with two types of head under 150 m/s
图 18 两种头型试件端面中心单元的应力三轴度
Figure 18. The stress triaxiality of the end face centerelement of the specimen with two types of head
图 19 两种试件靶杆载荷实验与模拟结果比较
Figure 19. Comparison of the load test and simulation of target rod
图 20 靶杆表面测得的接触力比较
Figure 20. Comparison of the contact force measured on the surface of the target rod
表 1 材料参数
Table 1. Material parameters
Material ρ/(g·cm−3) E/GPa μ ˙εs/s−1 cp/[J·(kg·℃)−1] A/MPa 3A21 2.73 70 0.32 0.001 880 90 7A04 2.85 72 0.31 Material B/MPa C n m Tm/℃ Tt/℃ 3A21 216 0.01 0.31 0.8 600 25 
下载: 导出CSV
-
[1] FIELD J E, WALLEY S M, PROUD W G, et al. Review of experimental techniques for high rate deformation and shock studies [J]. International Journal of Impact Engineering, 2004, 30(7): 725–775. doi: 10.1016/j.ijimpeng.2004.03.005 [2] TAYLOR G I. The use of flat-ended projectiles for determining dynamic yield stress I. theoretical considerations [J]. Proceedings of the Royal Society of London. Series A, Mathematical and Physical Sciences, 1948, 194(1038): 289–299. doi: 10.1098/rspa.1948.0081 [3] LEE E H, TUPPER S J. Analysis of plastic deformation in a steel cylinder striking a rigid target [J]. Journal of Applied Mechanics, 1954, 21(1): 63–70. [4] HAWKYARD J B. A theory for the mushrooming of flat-ended projectiles impinging on a flat rigid anvil, using energy considerations [J]. International Journal of Mechanical Sciences, 1969, 11(3): 313–333. doi: 10.1016/0020-7403(69)90049-6 [5] 钱伟长. 穿甲力学[M]. 北京: 国防工业出版社, 1984. [6] JONES S E, GILLIS P P, FOSTER J C JR, et al. A one-dimensional, two-phase flow model for taylor impact specimens [J]. Journal of Engineering Materials and Technology, 1991, 113(2): 228–235. doi: 10.1115/1.2903397 [7] JONES S E, MAUDLIN P J, FOSTER J C JR. An engineering analysis of plastic wave propagation in the Taylor test [J]. International Journal of Impact Engineering, 1997, 19(2): 95–106. doi: 10.1016/S0734-743X(96)00020-6 [8] JONES S E, DRINKARD J A, RULE W K. An elementary theory for the Taylor impact test [J]. International Journal of Impact Engineering, 1998, 21(1/2): 1–13. doi: 10.1016/S0734-743X(97)00036-5 [9] ZHANG W, XIAO X K, WEI G, et al. Evaluation of five fracture models in Taylor impact fracture [J]. AIP Conference Proceedings, 2012, 1426(1): 1125–1128. doi: 10.1063/1.3686477 [10] ROHR I, NAHME H, THOMA K. Material characterization and constitutive modelling of ductile high strength steel for a wide range of strain rates [J]. International Journal of Impact Engineering, 2005, 31(4): 401–433. doi: 10.1016/j.ijimpeng.2004.02.005 [11] KLEISER G, REVIL-BAUDARD B, PASILIAO C L. High strain-rate plastic deformation of molybdenum: experimental investigation, constitutive modeling and validation using impact tests [J]. International Journal of Impact Engineering, 2016, 96: 116–128. doi: 10.1016/j.ijimpeng.2016.05.019 [12] ABED F, JANKOWIAK T, RUSINEK A. Verification of a Thermoviscoplastic constitutive relation for brass material using Taylor’s test [J]. Journal of Engineering Materials and Technology, 2015, 137(4): 041005. doi: 10.1115/1.4030804 [13] MA S, ZHANG X, QIU X M. Comparison study of MPM and SPH in modeling hypervelocity impact problems [J]. International Journal of Impact Engineering, 2009, 36(2): 272–282. doi: 10.1016/j.ijimpeng.2008.07.001 [14] TURGUTLU A, AL-HASASNI S T S, AKYURT M. Impact deformation of polymeric projectiles [J]. International Journal of Impact Engineering, 1996, 18(2): 119–127. doi: 10.1016/0734-743X(95)00033-7 [15] 胡文军. 聚合物弹丸的碰撞行为研究 [D]. 重庆: 重庆大学, 2008.HU W J. Study on impact behavior of polymeric projectiles [D]. Chongqing: Chongqing University, 2008. [16] LOPATNIKOV S L, GAMA B A, HAQUE M J, et al. Dynamics of metal foam deformation during Taylor cylinder–Hopkinson bar impact experiment [J]. Composite Structures, 2003, 61(1/2): 61–71. doi: 10.1016/S0263-8223(03)00039-4 [17] RADFORD D D, DESHPANDE V S, FLECK N A. The use of metal foam projectiles to simulate shock loading on a structure [J]. International Journal of Impact Engineering, 2005, 31(9): 1152–1171. doi: 10.1016/j.ijimpeng.2004.07.012 [18] 《工程材料实用手册》编辑委员会. 工程材料实用手册: 第3卷-铝合金 镁合金[M]. 北京: 中国标准出版社, 2002. [19] 朱珏. 混凝土类材料冲击本构特性的SHPB技术及Lagrange反解法的研究[D]. 合肥: 中国科学技术大学, 2006.ZHU J. On SHPB Technique and Lagrangian analysis used for studying the impact response of concrete-like materials [D]. Hefei: University of Science and Technology of China, 2006. [20] 刘孝敏, 胡时胜. 大直径SHPB弥散效应的二维数值分析 [J]. 实验力学, 2000, 15(4): 371–376. doi: 10.3969/j.issn.1001-4888.2000.04.003LIU X M, HU S S. Two-dimensional numerical analysis for the dispersion of stress waves in large-diameter-SHPB [J]. Journal of Experimental Mechanics, 2000, 15(4): 371–376. doi: 10.3969/j.issn.1001-4888.2000.04.003 -
下载:
下载:
点击查看大图
计量
- 文章访问数: 4079
- HTML全文浏览量: 1272
- PDF下载量: 30
