In-Plane Biaxial Impact Response of Re-Entrant Auxetic Honeycomb
-
摘要: 利用有限元模拟方法研究了内凹负泊松比蜂窝结构的面内双轴冲击响应。用节点扰动方法建立了具有不同规则度的内凹负泊松比蜂窝结构,并将其在不同冲击速度下的变形模态、应力-应变曲线和能量耗散能力与规则蜂窝进行了对比分析。结果表明,冲击速度是内凹蜂窝结构变形模态最主要的影响因素。此外,在双轴冲击下,由于不规则度的引入,延长了应力-应变曲线的平台阶段,抑制了结构的各向异性程度,从而使结构的变形特征从局部密实转向整体密实。在能量吸收能力方面,结构的不规则性导致了密实化阶段的滞后,因此在相同的压缩程度下,其塑性耗散能低于规则模型。Abstract: The in-plane biaxial impact response of a re-entrant auxetic honeycomb structure is studied by finite element simulation. A re-entrant auxetic honeycomb structure with different regularities is established by using the node perturbation method, and its deformation modes, stress-strain curves and energy dissipation capacity under different impact velocities are compared with the regular honeycomb structure. The results show that the impact velocity is the most important factor affecting the deformation mode of the honeycomb structure. In addition, due to the influence of irregularity, the plateau stage of stress-strain curve is prolonged and the degree of anisotropy of the structure is inhibited under biaxial impact, resulting that the deformation characteristics of the structure change from local compactness to overall compactness. In terms of energy absorption capacity, the irregularity of the structure leads to the lag of the compaction stage, so its plastic energy dissipation is lower than that of the regular model under the same compression degree.
-
Key words:
- re-entrant auxetic honeycomb /
- biaxial impact /
- irregularity /
- deformation mode /
- energy absorption
-
负泊松比蜂窝结构又称拉胀结构,因具有许多常规结构不具备的力学特性[1],而成为研究热点。蜂窝材料具有较高的相对刚度、强度和高效的能量吸收能力,在抗剪切、抗屈曲、提高硬度以及抗疲劳等方面拥有独特的优越性[2-3],在一些应用领域中发挥着关键作用,如汽车、航空、军事、医学领域[4]。多孔结构的力学性能主要取决于细观上的拓扑结构。近年来,通过改变细观结构,人们发现负泊松比结构具有很多特殊优势,因而被广泛应用[5]。
马芳武等[6]研究了一种内凹三角形负泊松比结构,通过改变内凹角度,分析了冲击端和固定端的平台应力和能量吸收能力,并与内凹六边形进行了对比。Zhang等[7]分析了内凹六边形蜂窝在两个正交方向上的后继屈服拉伸行为,同时考虑结构的塑性影响以及孔壁的非线性行为分析模型,提出了单胞结构的塑性铰变形机制,得到了单胞结构的应力-应变曲线。Li等[8-9]对内凹蜂窝结构进行分级、强化,并将正弦曲线引入内凹蜂窝结构,得到了新的改进模型,进而分析了结构的泊松比和能量吸收变化。邓小林等[10]研究了全参数化的正弦曲线蜂窝结构,以不同振幅、不同厚度建立模型,研究了蜂窝结构在不同冲击速度下的动力响应,发现正弦曲线蜂窝较常规六边形蜂窝有更好的能量吸收效果。崔世堂等[11]利用有限元模拟方法研究了负泊松比蜂窝结构面内冲击动力学特性,发现平台应力和结构的比吸能随冲击速度的增大而增高,随胞元扩展角的增大而降低。陈鹏等[12]研究了具有零泊松比特征的半凹角蜂窝结构,并将其与正六边形蜂窝和内凹负泊松比蜂窝在面内冲击荷载作用下的抗冲击性能进行对比分析,数值结果表明,半凹角蜂窝的抗冲击性能介于正六边形蜂窝和内凹蜂窝之间。Hu等[13]通过理论分析和数值模拟,研究了内凹角度和壁长对内凹负泊松比蜂窝在大变形下的单轴动态冲击性能的影响,推导出冲击过程中平均冲击应力的经验公式。Zhang等[14]通过有限元模拟,研究了内凹蜂窝x方向的平面内动态冲击行为,发现内凹蜂窝的面内动态性能不仅与冲击速度和边缘厚度有关,还受蜂窝壁角的影响。Li等[15]通过单轴和双轴压缩模拟以及理论分析,研究了正六边形蜂窝结构的面内压缩动态力学性能,分析了双轴压缩的变形模式,结果表明:相比单轴冲击,双轴冲击下在x和y方向的真实应力增强,能量吸收能力也得到了提高,且完全致密化阶段比单轴压缩阶段更平滑。此外,Li等[16]研究了六边形、内凹、混合3种蜂窝模型在单、双轴冲击下的面内动态力学性能,结果表明:正交双轴冲击下,六边形蜂窝表现出3种变形模式,内凹和混合型蜂窝没有明显的过渡模式,由于负泊松比效应的影响,内凹蜂窝具有较差的耗能能力。
值得注意的是,自然界中的蜂窝结构和人造蜂窝结构在细观上总存在一定的缺陷,从而引起结构的不规则性,力学性能也会发生一定的变化。Ajdari等[17]通过数值模拟研究了正六边形和不规则二维蜂窝的平面内动态冲击问题,分析了孔壁缺失和空间扰动形成的结构微观不规则性对力学性能的影响。Alkhader等[18]用函数定义六边形蜂窝、随机Voronoi泡沫以及正方形和三角形拓扑结构等多种二维拓扑结构的不规则程度,以研究其单轴压缩响应,结果表明,相对于以弯曲为主的结构,以拉伸为主的结构有表现出灾难性屈服后软化反应的趋势,而不规则性则会导致更多的弯曲现象。Liu等[19]对内凹蜂窝材料的面内动态冲击过程进行了数值模拟,并在此基础上定义了内凹蜂窝结构的不规则性,分析发现,在准静态下不规则的内凹蜂窝比规则的正六边形蜂窝能吸收更多的能量,但这种情况在高速撞击下逆转。Zheng等[20]通过数值模拟研究了坐标扰动和Voronoi随机模型两种不规则模型与正六边形蜂窝在不同冲击速度下的变形模式和平台冲击力,得到不规则性结构更具复杂性的结论。Zhu等[21]研究了孔的不规则性对二维随机泡沫弹性性能的影响,构造了不规则度不同的周期性随机结构,并通过数值模拟确定了其有效弹性性能,结果表明,二维随机泡沫体形状越不规则,有效弹性模量和剪切模量越大,在一定的压比相对密度下,体积模量越小。
综上所述,实际中蜂窝结构往往是不规则的,且易受双轴冲击载荷作用。而关于不规则结构在双轴冲击下的研究较少,为此本工作将针对不规则内凹负泊松比蜂窝结构在双轴冲击下的面内冲击响应,分析规则度和冲击速度对结构变形影响的规律。
1. 计算模型
1.1 不规则内凹蜂窝结构有限元模型的建立
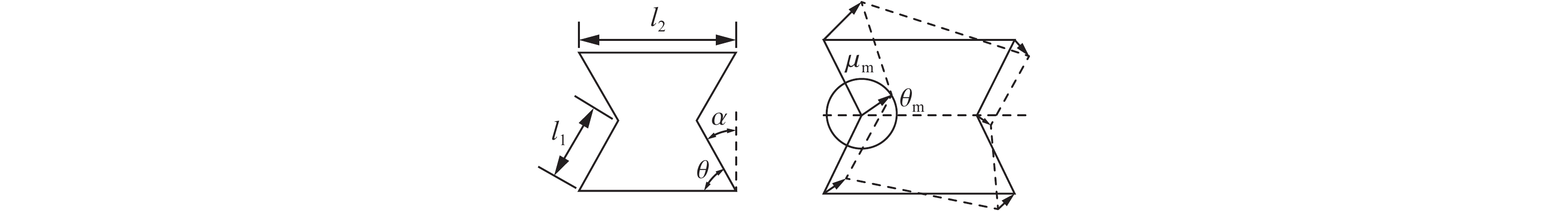
采用如图1所示的节点扰动方法来建立不规则内凹蜂窝的有限元模型。
如图1所示,将规则的内凹六边形蜂窝结构的每个节点按照式(1)中的方法进行随机扰动
{yi=y0+Δysinθmxi=x0+Δxcosθm (1) 式中:
θm 为角度随机值(0∘⩽θm⩽360∘ ),x0 、y0 为节点的原始坐标,xi 、yi 为扰动后的节点坐标。为了保证随机扰动之后模型的棱壁不会重叠,需要对Δx 、Δy 进行如式(2)的限制{μ=Δx2+Δy20⩽μ⩽μm (2) 式中:
μ 为节点扰动的随机长度;μm 为扰动的最大长度,0⩽μm⩽l1/2 。内凹负泊松比蜂窝结构的不规则度可以定义为K=2μml1 (3) 式中:
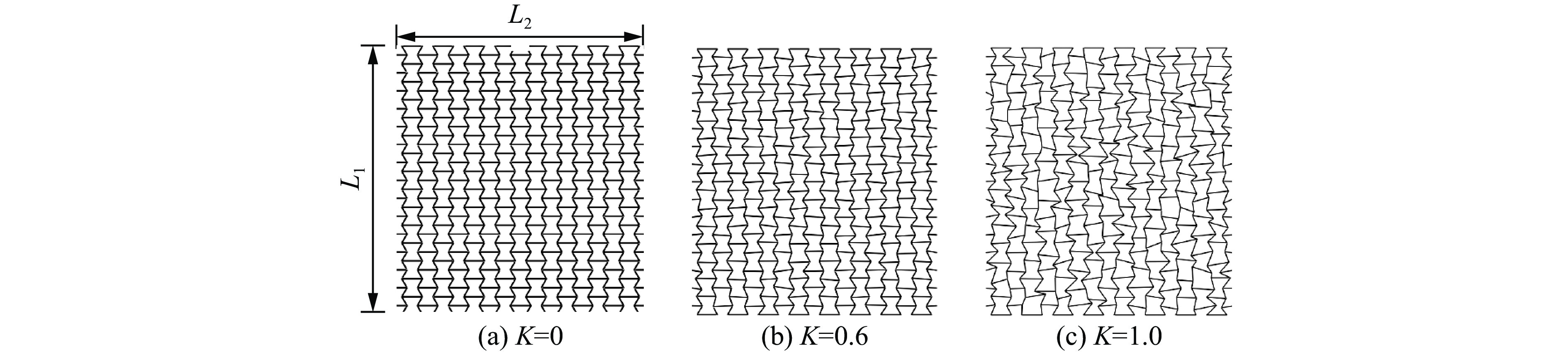
l1 为规则蜂窝结构的最短棱壁长度。假设蜂窝结构所有棱壁的厚度均相同,则可通过改变棱壁的厚度来调节蜂窝结构的相对密度。本研究采用15%的相对密度进行分析,图2显示了部分模型。
图2中内凹蜂窝结构的相对密度
Δρ 可以表示为Δρ=ρ∗ρs=N∑i=1li×tL1×L2 (4) 此外,对于规则的内凹负泊松比蜂窝,其相对密度
Δρr 也可以表示为Δρr=12tl1(l2/l1+2)cosα(l2/l1+sinα) (5) 式中:
ρ∗ 为模型的密度,ρs 为基体材料的密度,li 为各个孔壁的长度,t 为孔壁的厚度,N 为孔壁的数量,L1 、L2 为整个蜂窝结构的长度和宽度,l2为规则蜂窝结构的最长棱壁长度。1.2 有限元模型
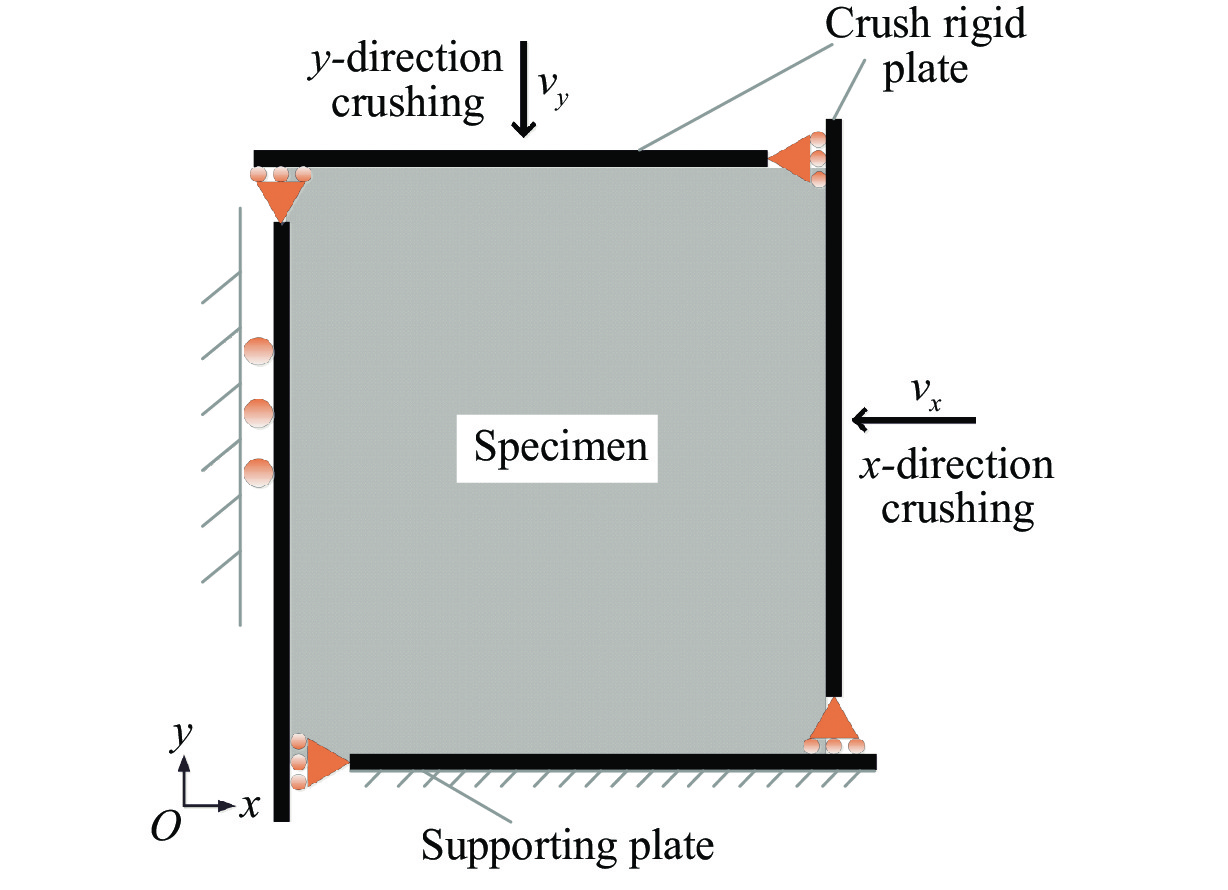
采用ABAQUS/EXPLICIT软件进行分析。模型的边界条件设置:在两个正交方向上,将模型置于两块刚性板之间、底部刚性板之上,底部和左端的刚性板作为固定端, 顶部和右端作为冲击端,冲击速度为3~100 m/s,同时约束内凹蜂窝结构的面内自由度,如图3所示。建立的内凹蜂窝结构的主要参数为L1 = 129.9 mm, L2 = 120.0 mm, l1 = 5 mm, l2 = 10 mm,
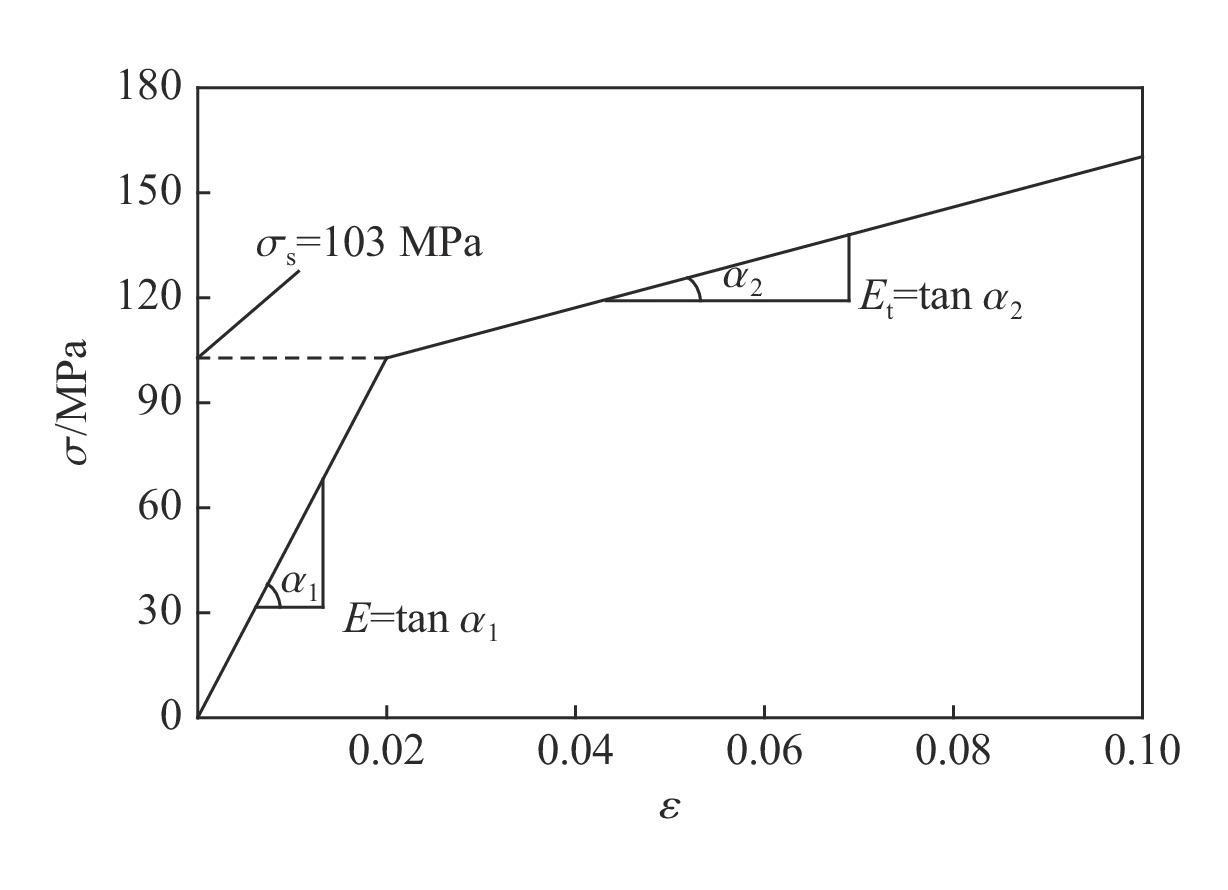
θ = 60°。由于蜂窝铝具有高强度和高刚度的良好力学性能,本研究采用铝合金作为基体材料,主要参数为:密度ρ = 2700 kg/m3,弹性模量E = 72 GPa,泊松比为0.33,屈服强度σy = 103 MPa,并采用线性强化模型,图4为结构基体材料的本构关系,其中Et为切线模量,σs 为线性强化模型的屈服强度。蜂窝细胞数量为15 × 15,可保证材料不受尺寸效应的影响。所有单元均采用4节点壳单元进行网格划分,网格单元尺寸为0.5 mm,节点数为28660,网格数为 19 540,建立无摩擦和通用接触。2. 结果与讨论
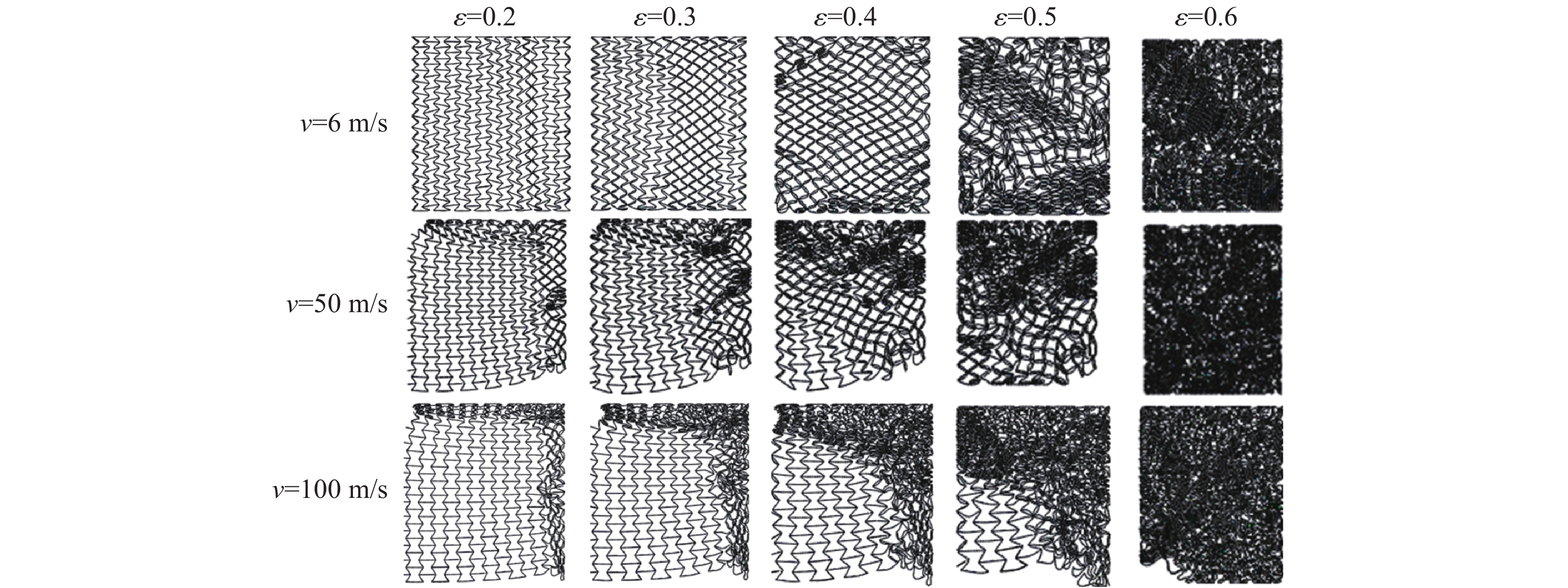
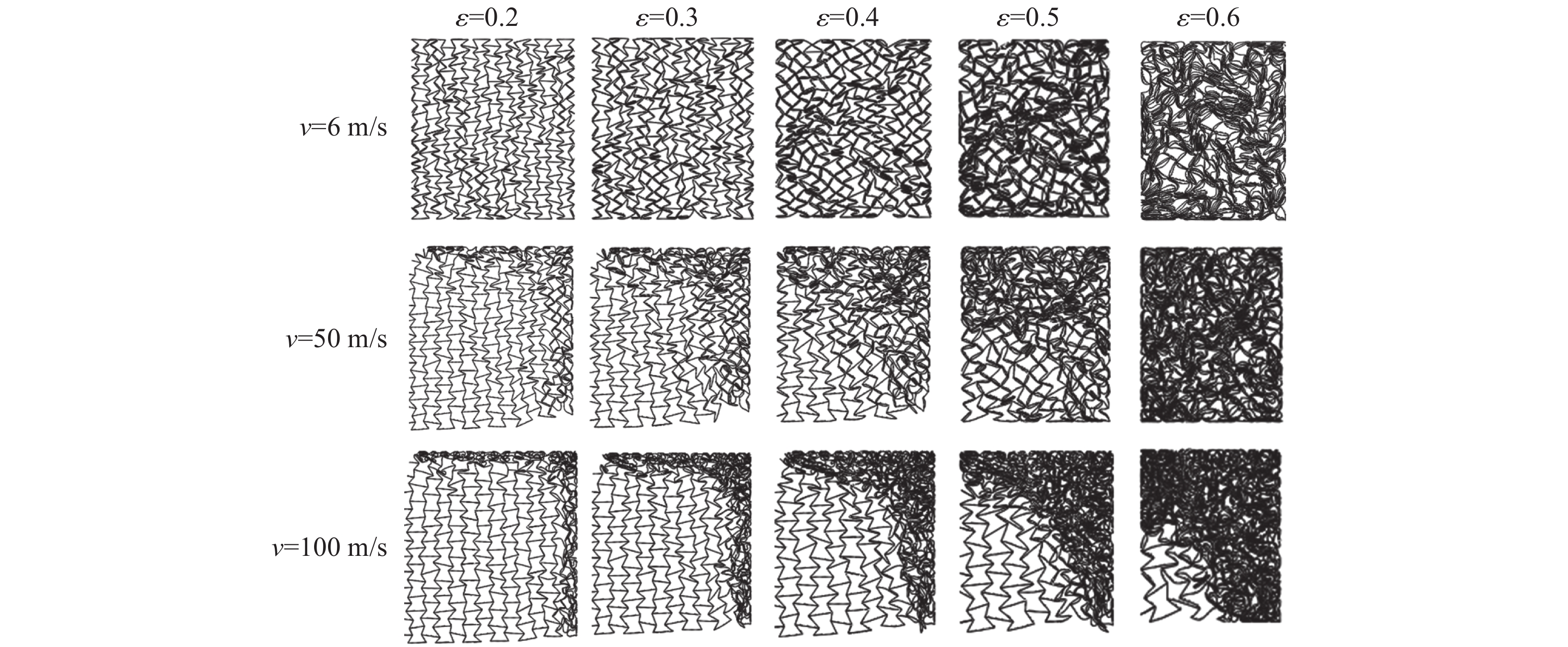
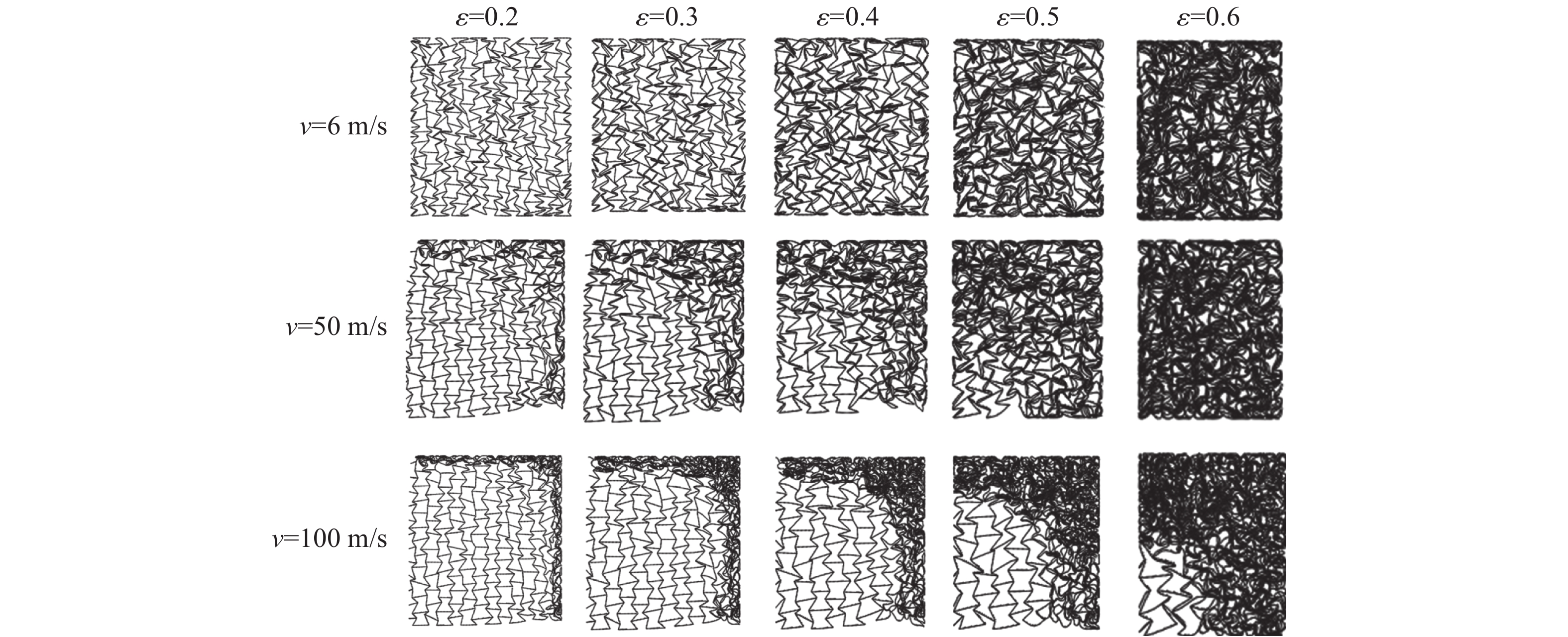
2.1 双轴冲击下的变形模式
为了对双轴冲击条件进行分类,采用与双轴冲击有关的参数
λ ,表达式为λ=vx/vy ,其中vx 、vy 分别为x 、y 方向的冲击速度。这里只讨论λ=1 的情况,显然λ=1 时为等双轴冲击。首先研究了不同规则度(K = 0, 0.6, 1.0)的内凹负泊松比在不同冲击速度(6、50和100 m/s)下的变形模式。图5、图6和图7给出了内凹蜂窝结构的结构变形情况。需要说明的是,为更好地展示变形结果,每隔约10%的应变截取一张变形模态图,同时为了清晰、规律地显示图像,所有图形都设置了相同的大小。从图5可以看出,对于规则的内凹蜂窝,在等双轴低速冲击过程中,结构首先在交叉处棱壁堆积,从而使内部先形成四边形,结构整体的变形在近端和远端都较均匀。这与文献[15]中内凹蜂窝的变形是一致的,也验证了本模型的有效性。持续的压缩使孔壁进一步堆积形成局部致密化,结构的致密过程主要是局部致密。由于负泊松比效应的影响,材料在一个方向受压时,其另一个正交方向会出现颈缩。因此,在双向冲击受压的情况下,结构会更早进入完全密实阶段。随着冲击速度的增大,结构从冲击端(上部和右端)开始密实,而固定端几乎没有变形。随着应变增加,致密向固定端传递,直至完全进入密实化。从图5中第2行和第3行图像还可以看出,随着冲击速度的增大,蜂窝结构的下端会产生部分“翘起”现象,这是由于负泊松比效应的影响会导致结构颈缩,且结构与固定端端部是无绑定约束,从而造成这类现象。
与规则蜂窝不同的是,不规则蜂窝结构在低速冲击下,其内部不会形成较为规则的四边形。这是由于不规则度的存在使结构棱壁处的堆叠也变得不规则。此外,从图5~图7中
ε=0.5 列可以看出,由于不规则度的引入,结构的变形模式由局部密实转变为整体密实,从而使内凹蜂窝结构在相同压缩程度下,密实化程度明显降低。在高速冲击下(v = 100 m/s),从图5~图7中可以看出,不规则程度越高,冲击端的致密程度越大。这是因为高速冲击下,结构在冲击端的密实主要是棱壁的弯曲折叠过程,随着不规则度的增加,棱壁的弯曲折叠受到的约束增大,向固定端传递的速度也会降低,所以不规则蜂窝结构的密实过程会更长,而在冲击端密实程度也会更高。此外,从图6、图7中ε=0.6 、v = 100 m/s对应的变形情况可以看出,固定端还有尚未变形进入密实的孔,说明不规则蜂窝结构具有较长的平台阶段,能够承受更大的压缩变形。2.2 不同冲击速度下的应力-应变曲线
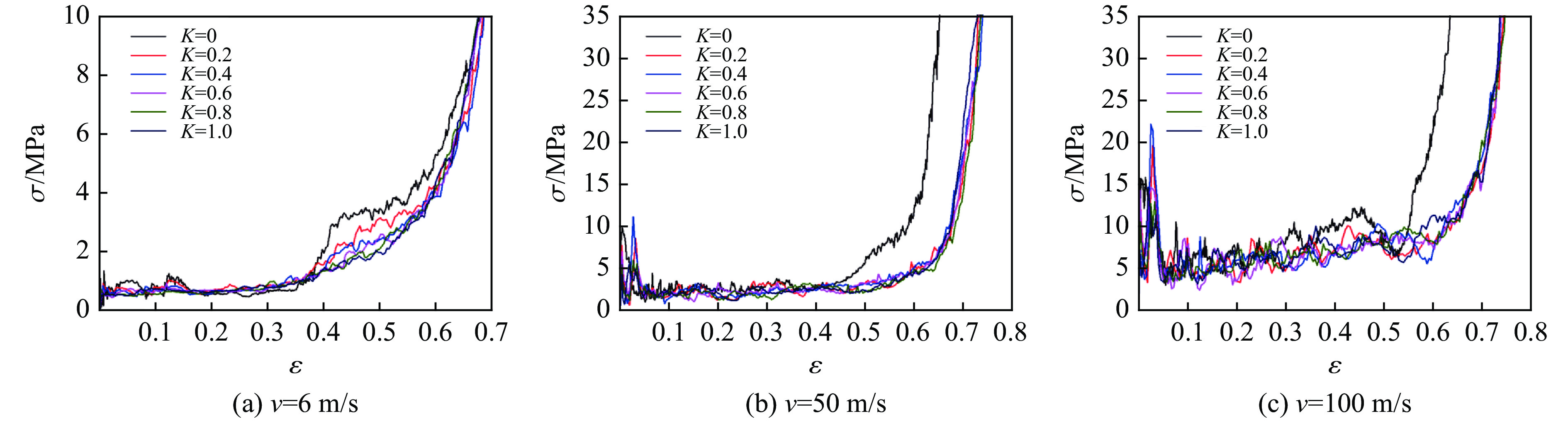
图8和图9给出了蜂窝结构在双轴冲击下两个正交方向冲击端的名义应力-应变曲线,其中名义应力
σ 通过冲击端的反力除以对应截面的原始面积获得,名义应变ε 通过冲击位移除以对应的原长获得。从图中可以看出,内凹蜂窝结构在不同方向上的σ-ε 曲线均表现出典型多孔材料在受压时所具有的弹性阶段、平台阶段和密实阶段3部分。从图8和图9中v = 6 m/s时的曲线可以看出,对于K = 0时的应力-应变曲线,在应变接近0.4处,结构变形的平台阶段均出现一个上升的阶梯,并且x方向最明显。结合2.1节关于变形模态的分析,认为这主要是由于在等低速双轴冲击下内凹蜂窝结构变形主要经历两种棱壁堆叠过程,即堆叠形成四边形以及四边形的进一步弯曲堆叠。由于第1步的堆叠,棱壁基本不会屈曲,主要是旋转折叠,因此这一平台阶段的应力水平较低;第2步的堆叠主要是棱壁的屈曲折叠,所以此阶段的应力水平较高。从图8和图9中也可以看出,K = 0时,结构会更早进入密实化阶段,而不规则度的引入使结构拥有较长的平台阶段,密实化阶段出现滞后现象,此现象与2.1节中变形模态的分析结果是一致的。随着冲击速度的增大,平台阶段的应力升高,说明结构的能量吸收能力随着冲击速度的增大而增强。
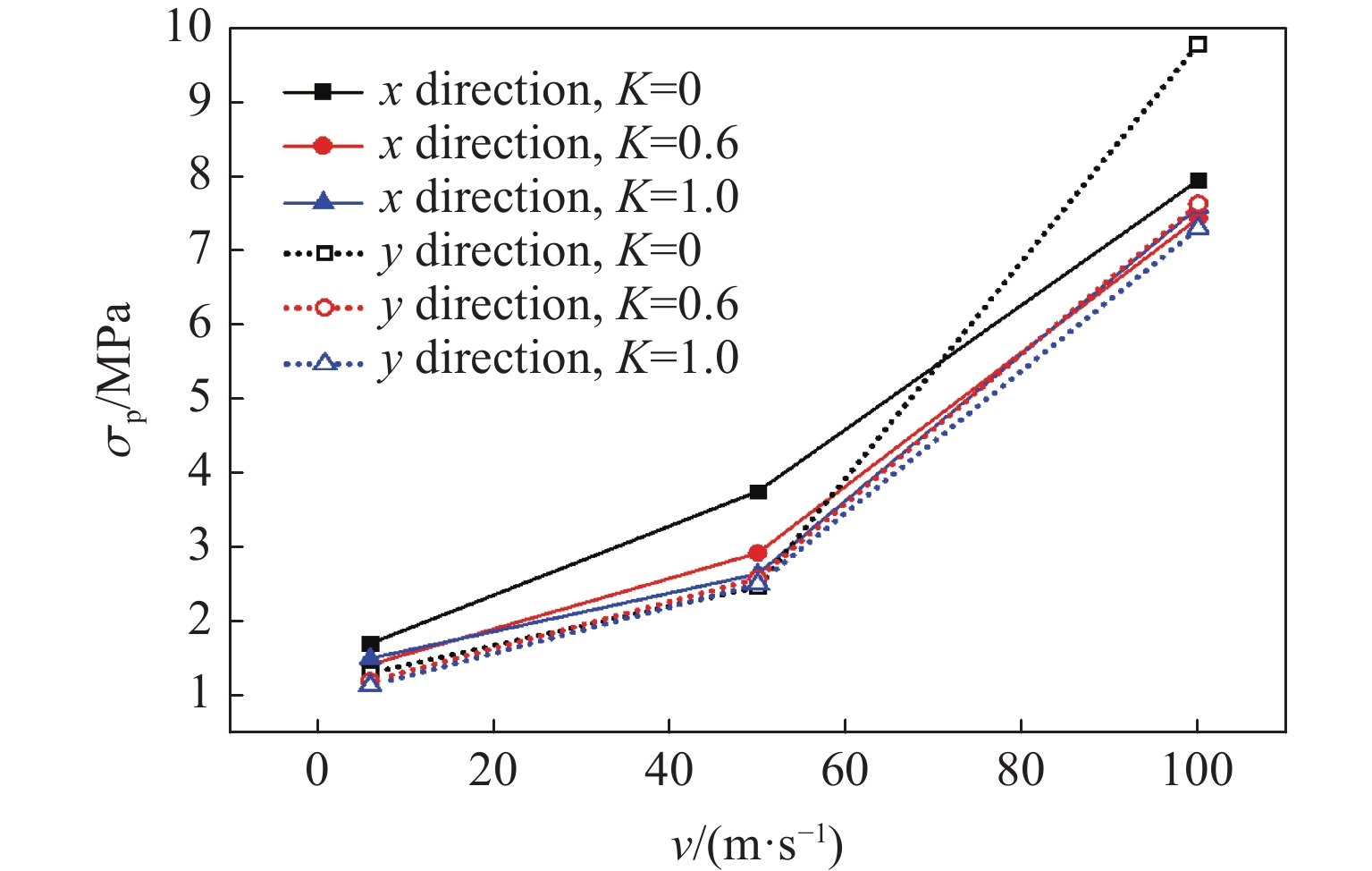
蜂窝结构的平台应力一般表示为
σp=1εd−ε0∫εdε0σ(ε)dε (6) 式中:
σp 为平台应力;ε0 为对应初始应力峰值的名义应变;εd 为锁定应变,为蜂窝结构密实化阶段所对应的应变;σ(ε) 为名义应力-应变曲线。图10给出了不规则度不同的内凹蜂窝结构在两个正交方向上不同冲击速度下的平台应力变化趋势。从图10可以看到:随着冲击速度的增大,两个方向上的平台应力值都会上升;对于K = 0的规则蜂窝结构,其在两个方向上的平台应力相差较大,这是结构的各向异性所导致的。引入不规则度时,在高速冲击下两个方向上的平台应力变化大小及趋势都较接近,说明结构的各向异性降低,这一点从2.1节内凹蜂窝结构的变形模态中也可以看出。
2.3 内凹蜂窝结构的能量吸收能力
在动态冲击过程中,能量主要由材料的塑性变形消耗。采用比塑性耗散能表征单位质量的能量吸收能力,表达式为
W=EPEDM (7) 式中:
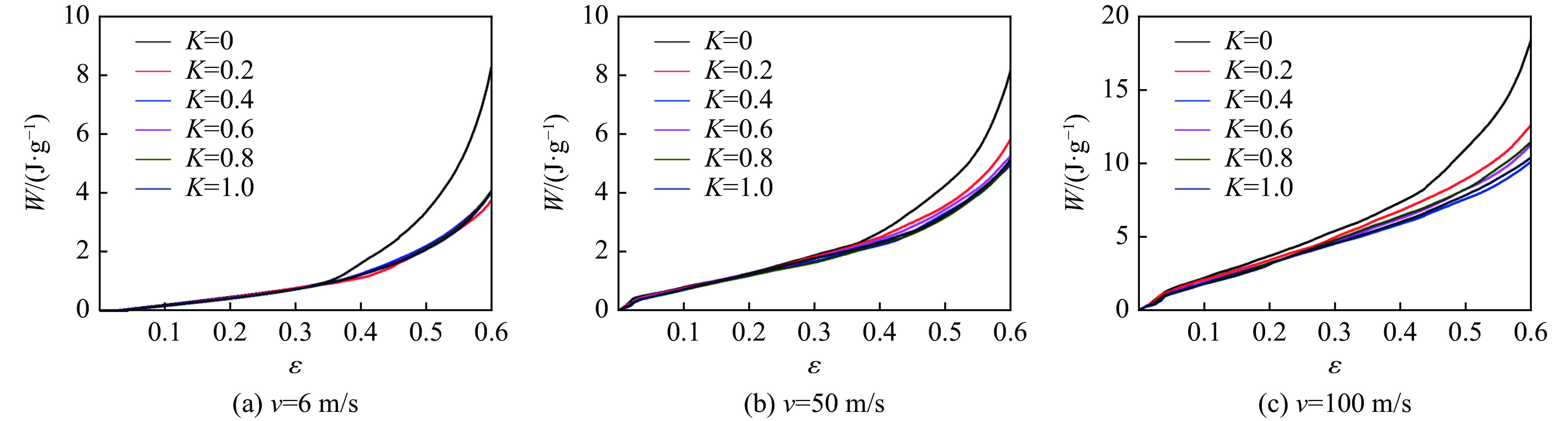
EPED 为塑性耗散能,可以从有限元分析软件中直接获得;M为结构的质量。图11给出了内凹蜂窝结构在6、50和100 m/s 3种不同冲击速度下的比塑性能量耗散与
y 方向冲击应变的关系。从图11中可以看出,当应变较低时,比塑性耗散能W 上升较缓慢,且所有曲线基本重合。这表明在早期,不规则度对内凹蜂窝结构的影响较小。随着压缩程度的增加,W 增加的速率变大,且K = 0时,W 增加得最快,表明结构开始进入密实阶段,这是由结构的负泊松比效应引起的。对于不规则蜂窝结构,曲线上升得较缓慢,表明不规则度的引入使结构的平台阶段延长,结构具有更强的能量吸收能力。3. 结 论
采用有限元方法研究了具有不同不规则度内凹负泊松比结构的面内双轴冲击响应,得到了以下结论。
(1)内凹蜂窝结构的变形受冲击速度的影响。随着冲击速度的提高,蜂窝结构的变形逐渐转向逐层致密,受结构负泊松比效应的影响,在等高速双轴压缩时,结构的固定端会有局部“翘起”现象。此外,由于不规则度的引入,在低速冲击下,结构的密实化过程从局部致密转变为整体致密,从而导致在相同的压缩程度下,结构的密实化程度降低。
(2)随着冲击速度的增大,平台阶段的应力上升,能量吸收能力更强,比塑性耗散能也上升。不规则度的引入延长了平台阶段,降低了结构的各向异性程度,从而提高了结构的能量吸收能力。
-
-
[1] LAKES R. Foam structures with a negative Poisson’s ratio [J]. Science, 1987, 235(4792): 1038–1040. doi: 10.1126/science.235.4792.1038 [2] ALDERSON A, EVANS K E. Microstructural modelling of auxetic microporous polymers [J]. Journal of Materials Science, 1995, 30(13): 3319–3332. doi: 10.1007/BF00349875 [3] MILLER W, SMITH C W, EVANS K E. Honeycomb cores with enhanced buckling strength [J]. Composite Structures, 2011, 93(3): 1072–1077. doi: 10.1016/j.compstruct.2010.09.021 [4] LAKES R. Deformation mechanisms in negative Poisson’s ratio materials: structural aspects [J]. Journal of Materials Science, 1991, 26(9): 2287–2292. doi: 10.1007/BF01130170 [5] 任鑫, 张相玉, 谢亿民. 负泊松比材料和结构的研究进展 [J]. 力学学报, 2019, 51(3): 656–689. doi: 10.6052/0459-1879-18-381REN X, ZHANG X Y, XIE Y M. Research progress in auxetic materials and structures [J]. Chinese Journal of Theoretical and Applied Mechanics, 2019, 51(3): 656–689. doi: 10.6052/0459-1879-18-381 [6] 马芳武, 梁鸿宇, 赵颖, 等. 内凹三角形负泊松比材料的面内冲击动力学性能 [J]. 振动与冲击, 2019, 38(17): 81–87.MA F W, LIANG H Y, ZHAO Y, et al. In-plane impact dynamic performance of re-entrant triangle material with negative Poisson’s ratio [J]. Journal of Vibration and Shock, 2019, 38(17): 81–87. [7] ZHANG J J, LU G X, RUAN D, et al. Tensile behavior of an auxetic structure: analytical modeling and finite element analysis [J]. International Journal of Mechanical Science, 2018, 136: 143–154. doi: 10.1016/j.ijmecsci.2017.12.029 [8] LI D, YIN J H, DONG L, et al. Strong re-entrant cellular structures with negative Poisson’s ratio [J]. Journal of Materials Science, 2017, 53(5): 3493–3499. [9] HOU J H, LI D, DONG L. Mechanical behaviors of hierarchical cellular structures with negative Poisson’s ratio [J]. Journal of Materials Science, 2018, 53(14): 10209–10216. doi: 10.1007/s10853-018-2298-0 [10] 邓小林, 刘旺玉. 一种负泊松比正弦曲线蜂窝结构的面内冲击动力学分析 [J]. 振动与冲击, 2017, 36(13): 103–109.DENG X L, LIU W Y. In-plane impact dynamic analysis for a sinusoidal curved honeycomb structure with negative Poisson’s ratio [J]. Journal of Vibration and Shock, 2017, 36(13): 103–109. [11] 崔世堂, 王波, 张科. 负泊松比蜂窝面内动态压缩行为与吸能特性研究 [J]. 应用力学学报, 2017, 34(5): 919–924.CUI S T, WANG B, ZHANG K. Mechanical behavior and energy absorption of honeycomb with negative Poisson’s ratio under in-plane dynamic compression [J]. Chinese Journal of Applied Mechanics, 2017, 34(5): 919–924. [12] 陈鹏, 侯秀慧, 张凯. 面内冲击荷载下半凹角蜂窝的抗冲击特性 [J]. 高压物理学报, 2019, 33(6): 064104. doi: 10.11858/gywlxb.20190759CHEN P, HOU X H, ZHANG K. Impact resistance of semi re-entrant honeycombs under in-plane dynamic crushing [J]. Chinese Journal of High Pressure Physics, 2019, 33(6): 064104. doi: 10.11858/gywlxb.20190759 [13] HU L L, ZHOU M Z, DENG H. Dynamic crushing response of auxetic honeycombs under large deformation: theoretical analysis and numerical simulation [J]. Thin Walled Structures, 2018, 131: 373–384. [14] ZHANG X C, DING H M, AN L Q, et al. Numerical investigation on dynamic crushing behavior of auxetic honeycombs with various cell-wall angles [J]. Advances in Mechanical Engineering, 2015, 7(2): 679678. doi: 10.1155/2014/679678 [15] LI Z, WANG T, JIANG Y, et al. Design-oriented crushing analysis of hexagonal honeycomb core under in-plane compression [J]. Composite Structures, 2017, 187: 429–438. [16] LI Z, GAO Q, YANG S, et al. Comparative study of the in-plane uniaxial and biaxial crushing of hexagonal, re-entrant, and mixed honeycombs [J]. Journal of Sandwich Structures and Materials, 2019, 21(6): 1991–2013. doi: 10.1177/1099636218755294 [17] AJDARI A, NAYEB-HASHEMI H, VAZIRI A. Dynamic crushing and energy absorption of regular, irregular and functionally graded cellular structures [J]. International Journal of Solids & Structures, 2011, 48(3/4): 506–516. [18] ALKHADER M, VURAL M. Mechanical response of cellular solids: role of cellular topology and microstructural irregularity [J]. International Journal of Engineering Science, 2008, 46(10): 1035–1051. doi: 10.1016/j.ijengsci.2008.03.012 [19] LIU W, WANG N, LUO T, et al. In-plane dynamic crushing of re-entrant auxetic cellular structure [J]. Materials & Design, 2016, 100: 84–91. [20] ZHENG Z, YU J, LI J. Dynamic crushing of 2D cellular structures: a finite element study [J]. International Journal of Impact Engineering, 2005, 32(1/4): 650–664. [21] ZHU H X, HOBDELL J R, WINDLE A H. Effects of cell irregularity on the elastic properties of 2D Voronoi honeycombs [J]. Journal of the Mechanics & Physics of Solids, 2001, 49(4): 857–870. -








 下载:
下载:

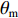
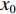

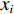
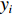
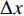
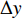
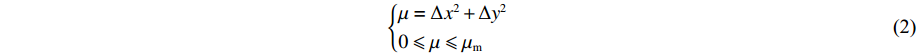


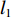

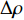
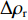
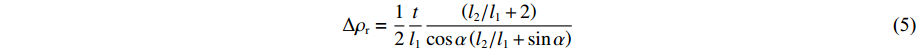
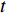
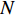
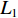
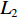
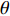
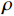
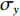
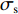


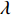
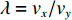
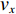
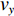
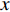
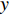
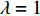
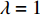



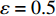
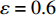
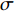
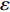
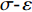


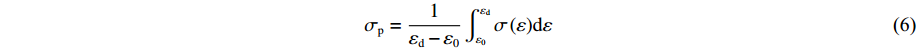
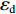
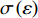


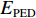

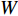

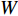


 下载:
下载: