Research on the Ballistic Performance of Cement Mortar
-
摘要: 对于水泥砂浆的抗弹性能研究,目前很少考虑靶体所处的应力状态,为此基于自研的真三轴静载混凝土侵彻实验装置和水泥砂浆抗弹性能实验结果,讨论了水泥砂浆在不同应力状态下的开坑深度和开坑阻力。应用侵彻深度的经验公式和基于HJC模型的有限元数值计算方法,对比分析了水泥砂浆侵彻实验,结果表明,对于低速冲击过程,采用UMIST公式和HJC模型的数值分析对开坑深度的预测较为有效。应力状态对开坑深度有明显的影响,即随着侧限增加,水泥砂浆的三轴强度提高,弹丸的开坑深度减小。应用基于HJC模型的数值分析方法,研究了弹丸开坑过程中弹体内的加速度波形和y轴支撑杆上的波形,结果表明:弹丸开坑过程对两种波形都有影响,其中y轴支撑杆上的波形可以更好地反映开坑过程。虽然数值模拟结果与实验波形的趋势基本一致,但是应力幅值有一定的差异,说明基于HJC模型的数值分析对开坑阻力的计算能力尚待提高。Abstract: The stress state of the target was seldom taken into account in the investigation of the ballistic performance of cement mortar. Based on the self-developed penetration experimental system of concrete under true-triaxial confinement and the experimental results of the anti-bullet performance of cement mortar, the depth and resistance of opening pit under different stress states were discussed in the present paper. The empirical formula of penetration depth and finite element method (FEM) based on HJC model were used to analyze the penetration behaviors of cement mortar results. The results showed that under the lower velocity impact, the UMIST formula and the HJC model were both effective in the prediction of pit depth. At the same time, the stress state had an obvious influence on pit depth. With the increase of the lateral limit, the cement mortar strength increases and the pit depth of the projectile decreases. The acceleration wave in bullet and the wave in the y-axis support rod were calculated by FEM based on HJC model. The results showed that the process of projectile opening pit would be recorded by these two waveforms, and the wave structure in the y-axis support rod would be more significantly. Although the tendency of the simulation results was basically consistent with the experimental waves, there was difference in the stress amplitude to some degree, which also indicated that the calculation method of the pit opening resistance based on HJC model needed to be improved.
-
Key words:
- ballistic performance /
- pit depth /
- pit resistance /
- cement mortar /
- true-triaxial stress state
-
混凝土结构在爆炸作用下一般处于复杂的应力状态。混凝土材料具有复杂的细观结构,是一种典型的结构材料,表现出强烈的应变率相关和加载路径相关特性[1-3]。这使得应力状态和冲击速度对此类材料的开坑与破坏行为产生非常重要的影响,相关研究对揭示混凝土结构的防护机理具有重要的参考意义。为了简化问题,本研究将讨论应力状态对水泥砂浆抗弹性能的影响,主要集中于侵彻阻力和开坑深度两方面。
目前,有很多关于弹丸侵彻混凝土靶体过程中侵彻阻力和开坑深度的研究[4-6],相关理论和数值分析的综述文献很充分[7-9]。然而,关于应力状态影响的研究工作并不多,并且主要通过圆形或多边形钢管对混凝土等靶材进行约束来实现[10-11]。例如:甄明等[12]开展了12.7 mm卵形弹侵彻圆形钢管(外径140 mm,厚度3.5 mm)约束的混凝土靶体实验,结果表明约束后靶体的抗弹性能明显提高;蒙朝美等[13]研究了不同形状钢管对靶体抗侵彻能力和靶体破坏模式的影响。钢管这类约束确实改变了靶体在侵彻过程中的应力状态,并且随着侵彻过程的进行,钢管的约束作用逐渐增强,防护性能得到很大的提升。但是,靶体的初始状态和侵彻过程中约束体的状态是未知的,能否真实反映实际侵彻过程不是很好评估;同时,在初始开坑阶段,靶体变形较小,钢管的约束作用很难发挥出来。因此,这类约束可以增强靶体的抗侵彻能力,但无法进行靶体在应力状态下的抗弹性能研究。本工作将基于新研制的真三轴应力状态下混凝土侵彻实验装置[14],在前期水泥砂浆侵彻性能初步研究[15]的基础上,结合数值分析方法,讨论应力状态对水泥砂浆抗弹性能的影响。
1. 实验装置与数值分析模型
1.1 真三轴静载下侵彻实验装置
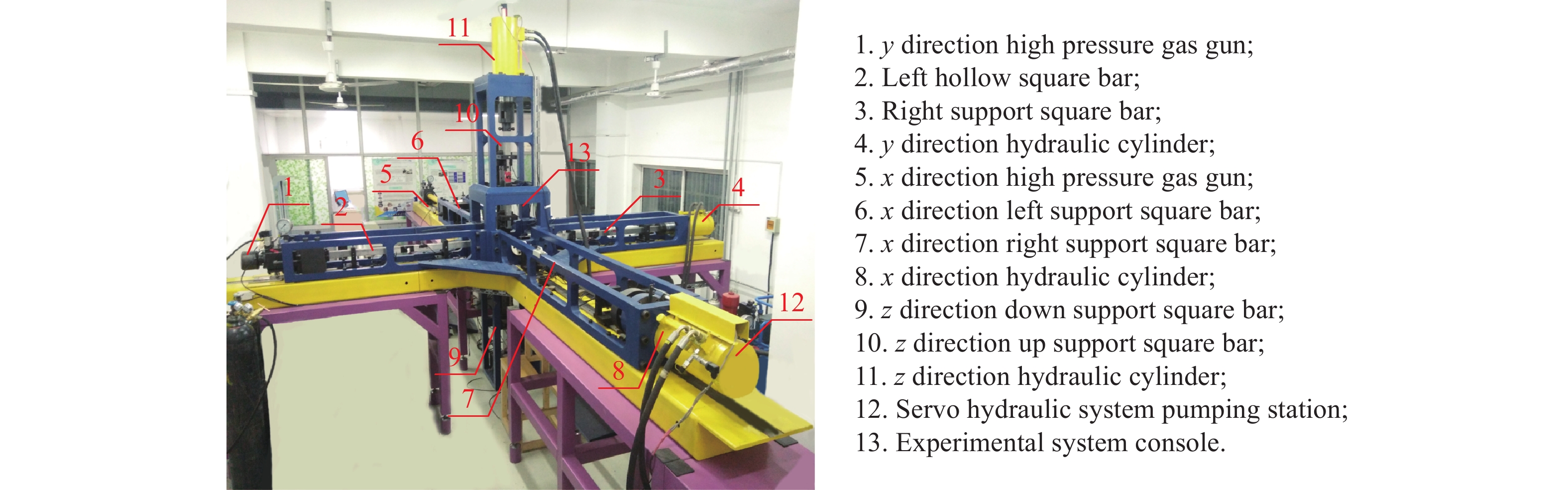
本实验采用边长为50 mm的立方体试件。实验装置实物如图1所示。该实验系统包括两部分:一部分是真三轴静载施加系统,分别由3个方向的液压缸(4、8、11)和对应的反力支架组成,可对立方体试件施加三向不等的压应力;另一部分是弹丸发射和信号测试系统,主要由侵彻方向(y方向)的高压气炮(1)、子弹入射的中空方杆(2)、支撑方杆(3)、水平x方向(垂直于侵彻方向)的左支撑方杆(6)和右支撑方杆(7),以及z方向的下左支撑方杆(9)和上左支撑方杆(10)等组成。
实验的第1阶段:在立方体试件的3个方向分别施加预定的静载
$\sigma_x $ 、$\sigma_y $ 和$\sigma_z $ 。实验的第2阶段:y方向的高压气体驱动子弹,从y方向中空方杆中的弹道加速射出,撞击试件。记录子弹速度和6根杆上的波动信号,由此可得到侵彻过程中立方体试件6个面的动态响应。实验结束后,对靶体的开坑深度等破坏情况进行拍摄和测量,采用三维光学扫描仪对侵彻实验的试件进行三维扫描成像,评估侵彻效果。水泥砂浆和混凝土的实验结果参见文献[14-15]。1.2 数值分析
真三轴静载侵彻实验系统的数值模型如图2所示,其中:6根方杆的截面尺寸均为50 mm × 50 mm,x、y、z方向各有两根长度相同的杆,杆长分别为2.0、1.5、1.0 m;y方向的入射杆中心有直径为6 mm的弹道。
采用30CrMnSi合金结构钢平头弹,其直径为5 mm,长度为20 mm。靶体材料为M10水泥砂浆,采用S型砂浆的HJC本构模型[16]描述,具体参数如表1所示,其中:
${\rho _0}$ 为密度,$G$ 为剪切模量,AHJC为特征化黏性强度系数,BHJC表示特征化压力硬化指数,CHJC为应变率影响参数,NHJC为压力硬化指数,$f{'}$ 为单轴抗压强度,T为拉伸强度,${\dot \varepsilon _0}$ 为参考应变率,${\varepsilon _{{\rm{f}},\min }}$ 为材料断裂时的最小塑性应变,Smax为最大特征化等效应力,pc为压垮时的静水压力,${\mu _{\rm{c}}}$ 为压垮时的体积应变,${p_{\rm{l}}}$ 为弹性极限下的静水压力,${\mu _{\rm{l}}}$ 为弹性极限下的体积应变,k1、k2、k3为压力常数,D1、D2为损伤常数。弹丸材料采用JC本构模型[17]描述,具体参数见表2,其中:${T_0}$ 为参考温度,c为材料比热容,AJC为材料在室温条件下的静态屈服强度,BJC为材料应变硬化模量,nJC为应变硬化指数,CJC为应变率常数,Tm为材料熔点,d1、d2、d3、d4、d5均为材料失效参数。6根方形钢杆采用线弹性模型描述,密度为7850 kg/m3,弹性模量E = 210 GPa,泊松比$\;\mu $ = 0.3。表 1 水泥砂浆的HJC本构模型参数Table 1. Parameters of HJC model for cement mortar${\;\rho {_0} }$/(kg·m−3) $G$/GPa AHJC/GPa BHJC/GPa CHJC NHJC $f{'} $/MPa 1844 1.32 0.66 1.335 0.0018 0.845 14.4 T/MPa ${\dot \varepsilon{_0} }$/s−1 $\varepsilon $f,min Smax ${p{\rm{_c}} }$/MPa ${\;\mu {\rm{_c}} }$ ${p{\rm{_l} } }$/GPa 2.0 1 0.01 80.24 13.8 0.0075 1.096 ${\;\mu {\rm{_l} } }$ k1/GPa k2/GPa k3/GPa D1 D2 0.15 85 −171 208 0.006629 1.0 表 2 弹丸JC本构模型参数Table 2. Parameters of JC model of projectile${\rho{_0} }$/(kg·m−3) G/GPa T0/K c/(J·kg−1·K−1) AJC/MPa BJC/MPa nJC 7830 77 293 477 792 510 0.26 CJC Tm/K d1 d2 d3 d4 d5 0.014 1793 0.05 3.44 −2.12 0.002 0.61 2. 水泥砂浆中的开坑深度
2.1 主要经验公式
(1)ACE公式
ACE公式是由美国陆军工程兵提出的混凝土侵彻深度经验公式,表达式为[18]
Xd=1.8628Dd0.215f0.5c(v0304.8)1.5+0.5 (1) 式中:X为弹体侵彻深度(m),D为弹丸的口径密度(kg/m3),d为弹丸直径(m),fc为混凝土靶的无侧限抗压强度(Pa),v0为弹丸初始速度(m/s)。
(2)修正NDRC公式
美国国防委员会提出了刚性弹体侵彻理论,并在此基础上,通过分析大量实验数据,提出适用于动能弹侵彻混凝土靶的半经验公式[19]
G(Xd)=KNDd0.2(v01000)1.8 (2) 式中:
$G\left( {{X}/{d}} \right) = {{(X/2d)2X/d⩽2(X/d)−1X/d>2}$ ;K为混凝土穿透系数,是混凝土强度的函数;N为弹头形状系数。Kennedy[20]提出混凝土的穿透系数K与混凝土无侧限抗压强度fc的平方根成正比,即
$K = {{180} / {{{{f^{0.5}_{\rm c}}}}}}$ 。代入式(2),并用国际单位制表示为G(Xd)=3.8×10−5NMv1.80f0.5cd2.8 (3) 式中:
$M$ 为弹丸的质量(kg),fc的单位为Pa。(3)Barr公式
Barr[21]针对低速实验结果,对NDRC公式进行了修正,公式如下
G(Xd)=3.8×10−5NMv1.80f0.5cd2.8 (4) 其中:
$G\left( {{X}/{d}} \right) = {{0.55(X/d)−(X/d)2X/d⩽0.22(X/2d)2+0.06050.22<X/d<2(X/d)−0.9395X/d⩾2}$ 。(4)Young公式
美国Sandia国家实验室提出了预测天然土材料和混凝土侵彻的经验公式,通常称为Young公式[22],其形式如下
H={8.0×10−3SKcN(MA)0.7ln(1+2.15v20×10−4)v0<61m/s1.8×10−5SKcN(MA)0.7ln(v0−30.5)v0⩾61m/s (5) 式中:M的单位为kg;Kc为缩尺效应,当M < 182 kg时,Kc = 0.46M0.15,当M ≥ 182 kg时,Kc = 1;A为弹丸的最大横截面积(m2);S为阻力参数。对于岩石,
$S = 2.7{({f_{\rm{c}}}/Q)^{ - 0.3}}$ ,Q为岩石质量指标,对于混凝土,$S = 0.085{K_{\rm{e}}}{t_{\rm{c}}}{T_{\rm{c}}}(11 - P){(35/{f_{\rm{c}}})^{0.3}}$ ,其中:fc的单位为MPa;${K_{\rm{e}}} = {(F/{W_{\rm{l}}})^{0.3}}$ ,Wl为靶体宽度与弹体直径之比,对于钢筋混凝土,F = 20,对于素混凝土,F = 30,如果Wl > F,取Ke = 1;P为混凝土体积配筋率;tc为混凝土浇筑时间,以年为单位,若浇筑满一年,则取tc = 1;Tc为靶体厚度h与弹体直径d的比值,在0.5~6之间,在缺少数据无法计算的情况下,可取0.9。(5)UMIST公式
Reid等[23]对大量混凝土冲击试验进行了分析和总结,考虑了应变率效应对混凝土强度的影响,提出了预测弹丸低速侵彻钢筋混凝土靶板的侵彻深度公式,即
Xd=2π⋅NMv200.72σtd3 (6) 式中:
${\sigma _{\rm{t}}}$ 为混凝土的动态强度(Pa),${\sigma _{\rm{t}}} = 4.2{f_{\rm{c}}} + 135.0 + (0.014{f_{\rm{c}}} + 0.450){v_0}$ ,其中fc的单位为MPa。对于平头弹,N = 0.72;对于球头弹,N = 0.84;对于钝头弹,N = 1.00;对于尖卵形弹,N = 1.14。(6)Chen和Li的无量纲侵彻深度公式
Chen和Li[5, 24]引入了两个无量纲量,即撞击函数I和弹头形状函数N,将Forrestal半经验公式无量纲化,并应用滑移场理论将公式的适用性推广到浅层和中等深度的侵彻,提出开坑深度与弹头形状的关系。无量纲侵彻深度公式如下
Xd={√1+kπ/4N1+I/N4kπIXd⩽k2πNln(1+I/N1+kπ/4N)+kXd>k (7) 式中:
$N = {\lambda / {{N^*_1}}}$ ,$\lambda=M/\rho{\rm{_c}}d^3,\;\rho\rm{_c}$ 为靶体密度,$0 < {N^*_1} \leqslant 1$ ,且${N^*_1} = \displaystyle\frac{8}{{{d^2}}}\int_0^h {\displaystyle\frac{{y{{y'}^3}}}{{1 + {{y'}^2}}}} {\rm{d}}x$ ,${N^*_1}$ 越小,弹丸头部越尖,对于平头弹,${N^*_1} = 1$ ;$I = {{{I^*}} / S_{\rm c}}$ ,其中${I^*} = Mv_0^2/{d^3}{f_{\rm{c}}}$ ,Sc是与靶体无侧限抗压强度相关的经验常数,$S_{\rm c} = 82.6f_{\rm{c}}^{ - 0.544}$ ;k为塑性滑移区深度与弹体直径之比,对于任意弹头形状,k = 0.707 + H/d,其中H为子弹头部高度,对于平头弹,k = 0.707。2.2 开坑深度对比
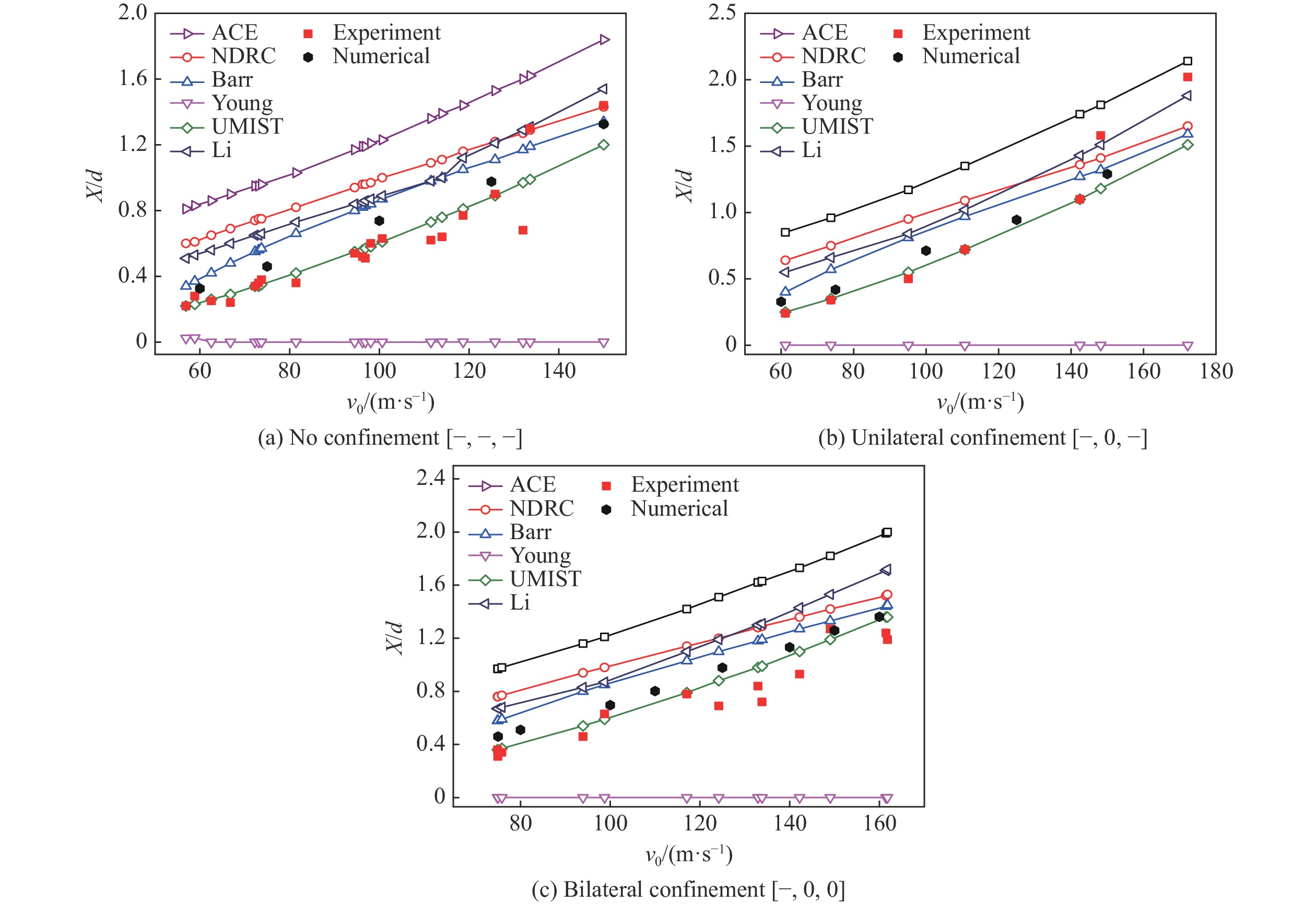
弹体为平头实心弹,质量为3.08 g,直径为5 mm,长度为20 mm。靶体为边长50 mm的立方体,平均密度为1844 kg/m3,无侧限抗压强度fc为14.4 MPa。采用上述经验公式进行计算,与实验测试结果和有限元分析结果进行对比,如图3所示,图3中方括号内的横线表示自由面,0表示施加侧限。整体上看,随着冲击速度的增大,无量纲深度基本单调增大;6种经验公式的计算结果存在较大差异,与实验结果的吻合程度各不相同。
在无侧限、单向侧限、双向侧限应力状态下,UMIST公式(式(6))的计算结果在冲击速度较低时与实验结果吻合很好。在低速冲击条件下使用该公式预测不同应力状态下的开坑结果比较有效。但是,当冲击速度逐渐增大时,该公式的预测结果低于实验结果。这是因为UMIST公式主要是基于大量平头弹低速侵彻实验结果总结得到,适用范围为:50 mm < d < 600 mm,35 kg < M < 2500 kg,0 < X/d < 2.5,3 m/s< v0 < 66.2 m/s。
有限元计算结果与实验结果也非常接近,表明HJC模型对于开坑深度的计算是非常有效的。
ACE公式(式(1))是基于弹体直径为12.7~155.0 mm、混凝土靶板厚度h与弹径d之比(h/d)为3~18的冲击实验得到的,与本研究中无侧限、单向侧限、双向侧限应力状态下的水泥砂浆侵彻实验情况差别较大,因此ACE公式的计算结果明显高于实验值。修正NDRC公式(式(3))是在Kennedy的补充修正基础上得到的,实验所用的弹体直径也比较大,但是冲击速度不是很高。对比结果表明:在冲击速度较低时,修正NDRC公式的计算结果与实验结果的差距较大;随着冲击速度的增大,两者的差距逐渐缩小,说明此时该公式具有较好的适用性。Barr公式(式(4))主要针对低速范围进行了修正,其计算值低于修正NDRC公式的计算结果,而且在较低的冲击速度下,该公式的计算值比修正NDRC公式的计算值低得多,更接近实验结果。Li公式(式(7))中的撞击函数是由Forrestal等根据实验结果拟合得到的,然后基于Forrestal半经验公式进行无量纲化,其计算结果与修正NDRC公式的计算结果相交,即当冲击速度较低时,该公式更接近实验结果,而当冲击速度较高时,该公式的计算结果与实验结果偏离更多。由于实验所用弹体的质量较小,超出了Young公式(式(5))的适用范围,因此Young公式的计算结果远低于实验结果,几乎趋于零。
图3所示的对比结果也表明,随着实验过程中试件侧限程度的增加,这些公式的计算结果与实验结果的符合程度更好。其原因在于,这些经验公式的获得虽然没有强调应力状态,但是基本上是基于大尺寸靶的实验,在弹丸冲击开坑过程中靶体实际上处于一定程度的侧限约束。
2.3 应力状态对开坑深度拟合结果的影响
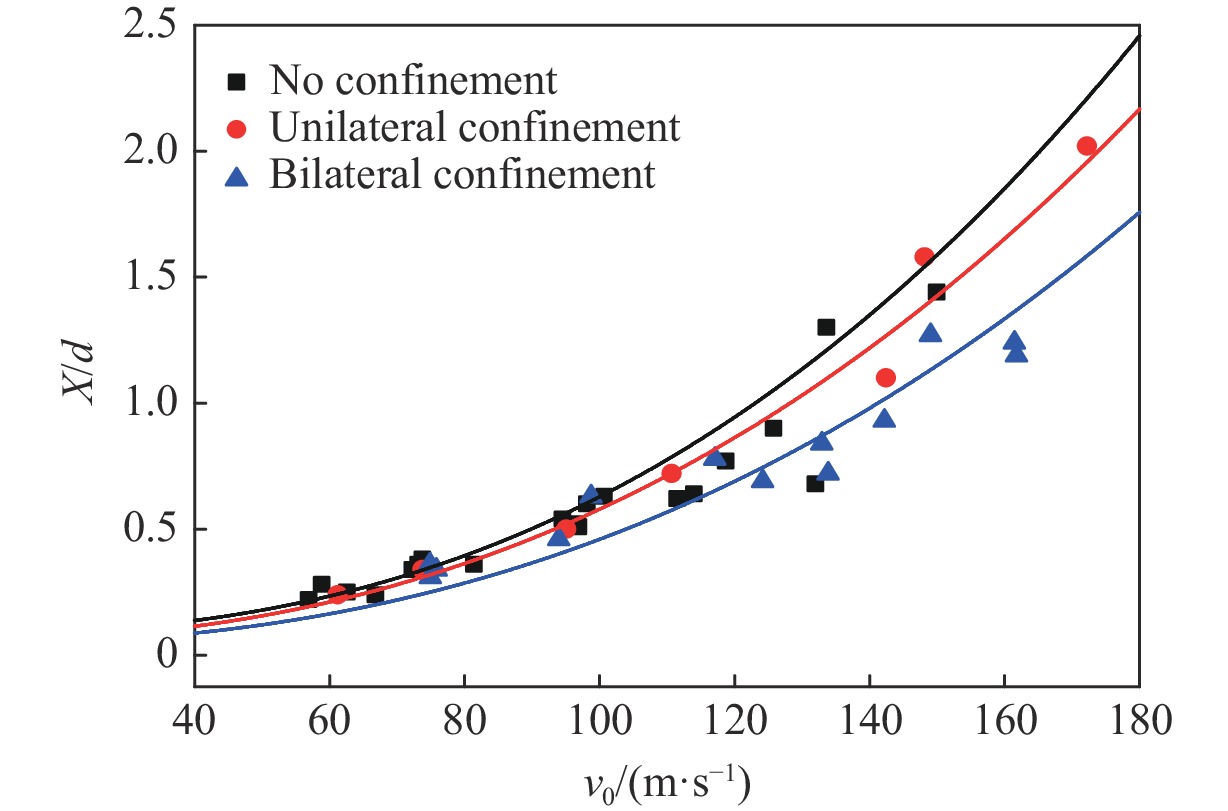
为讨论应力状态对开坑深度拟合结果的影响,采用形式相对简单的修正NDRC公式,并对其形式进行适当修改,即
Xd=CNNMvn0f∗0.5d2.8 (8) 式中:C和n是由实验确定的经验常数;NN为弹头形状因子,对于平头弹,取NN = 0.72;考虑到不同的初始应力状态,将原来的单轴抗压强度扩展为靶体的动态强度
${f^*} $ ,${f^*} $ 与应力状态有关,是一种三轴应力状态下的强度(MPa)。拟合结果如图4所示,其中:n为2.0,即无量纲开坑深度与弹丸初始动能相关;C为4.2,较修正NDRC公式中的C值略大,与水泥砂浆材料强度有关;对于拟合强度
${f^*} $ ,双向侧限时最高,达到25.8 MPa,无侧限时最低,为14.7 MPa,单向侧限时为17.6 MPa。无侧限时的拟合强度与M10水泥砂浆试样的单轴压缩强度相当,表明初始应力状态对开坑过程有较大的影响。需要注意的是,式(8)等号右边是含量纲的,因此,此拟合结果对于其他尺寸的弹丸开坑须慎重使用。下面采用无量纲形式的拟合。
开坑深度主要受以下参数控制
X=f(ρp,Ep,Yp,μp;v0,d,L;ρt,ft,Et,μt) (9) 式中:
${\rho _{\rm{t}}}$ 、Et、${\mu _{\rm{t}}}$ 、ft分别为靶材的密度、杨氏模量、泊松比和无侧限抗压强度,v0、d、L、${\rho _{\rm{p}}}$ 、Ep、${\mu _{\rm{p}}}$ 、Yp分别为子弹的初始速度、直径、长度、密度、杨氏模量、泊松比和屈服强度。以
${\rho _{\rm{t}}}$ 、d和ft作为基本量,则式(9)的无量纲形式为Xd=f(ρpρt,Epft,Ypft,μp;v0√ft/ρt,Ld;Etft,μt) (10) 由于开坑过程主要与惯性作用(密度
$\;\rho $ )、靶体材料的强度(屈服强度ft)及材料的压缩特性(v0/Ct)相关,因此开坑过程的初步分析可集中于对这3个量的讨论。引入水泥砂浆靶材的声速Ct,${C_{\rm{t}}} = \sqrt {{E_{\rm{t}}}/{\rho _{\rm{t}}}} $ ,则无量纲开坑深度的表达式为Xd=f(ρpρt,v0√ft/ρt,v0Ct) (11) 将泊松比等其他材料参数代入待定系数,则式(11)可初步表达为
Xd=k0(ρpρt)m1(v0√ft/ρt)m2(v0Ct)m3 (12) 拟合实验数据,结果如图5和表3所示。可见,水泥砂浆的开坑过程主要受强度特性影响,并且随着侧限的增强,影响增强。无侧限条件下,惯性效应和压缩特性对开坑的影响基本相同;随着侧限的增强,压缩特性对开坑过程的影响增强,惯性特性减弱。因此,应力状态对水泥砂浆的侵彻开坑过程有非常大的影响。
表 3 无量纲侵彻深度公式参数Table 3. Formula parameters of dimensionless penetration depthStress state k0 m1 m2 m3 No confinement 0.90 0.70 1.21 0.60 Unilateral confinement 1.02 0.55 1.25 0.65 Bilateral confinement 1.05 0.40 1.30 0.68 3. 水泥砂浆中的侵彻阻力
3.1 侵彻过程测试
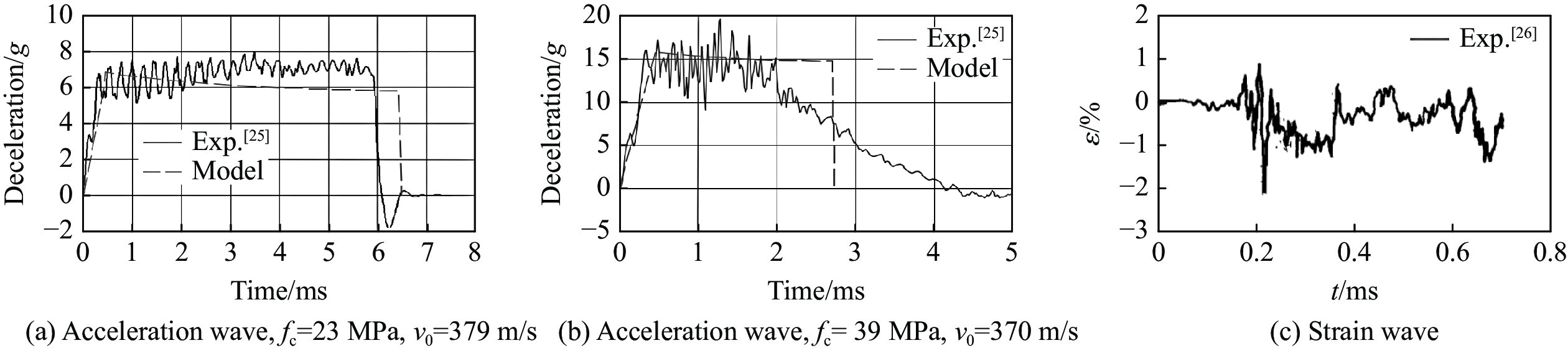
Forrestal等[25]采用直径为76.2 mm、质量为13 kg的4340钢卵形弹对混凝土进行了冲击速度为140~460 m/s的侵彻实验,通过在弹丸内部安装单通道加速度数据记录器,实时记录弹丸的加速度波形。当混凝土无侧限抗压强度为23 MPa时,预测与测量结果一致,如图6(a)所示;而当抗压强度为39 MPa时,在上升时间和峰值平台响应上预测与测量结果一致,在侵彻即将结束时两者有所偏差,如图6(b)所示。王琳等[26]提出了一种采用应变片直接测量弹体变形的方法,对混凝土靶进行了速度范围为150~300 m/s的正侵彻实验,记录了弹体的应变时程曲线,如图6(c)所示。这些实验成功获得了侵彻过程中弹体的响应,为计算提供了很好的依据。
徐松林等[14]、陈丽娜等[15]应用真三轴混凝土侵彻实验装置,从另一个角度获得了弹丸侵彻过程中混凝土的响应曲线,即通过与试件底端接触的钢杆传递并记录侵彻过程中试件内的波形。图7所示波形包含了多个过程:未开坑情况下(速度56.90 m/s),弹丸与试件弹性碰撞;开坑很浅(速度73.11~113.97 m/s)时,弹丸回弹;弹丸稳态侵入(速度150.00 m/s)时,形成较完整的开坑。
以上两类实验分别从子弹内部的加速度响应和穿过试件的波形来分析侵彻阻力的发展过程。加速度波形对开坑过程有一定的反映,其脉宽和幅值与未开坑情况对比有一定的变化。但是,由于还要考虑子弹高速冲击时的结构响应、子弹头部与试样的局部接触等因素的影响,因此加速度波形的变化与开坑过程的对应关系不是很直接,其定性的意义更强一些。对比而言,y轴支撑杆上的信息传递的是试件开坑过程的直接响应,其应力波形变化对开坑过程的反映更敏感,更能揭示开坑过程的特征。本测试结果可为相关抗弹性能的计算提供多种边界条件;同时,也可以结合加速度波形,形成更科学的抗弹性能计算和分析评估方法。由此可见,研究侵彻过程时,需要将二者有机结合。下面将结合数值分析对此过程进行讨论。
3.2 冲击速度对开坑过程的影响
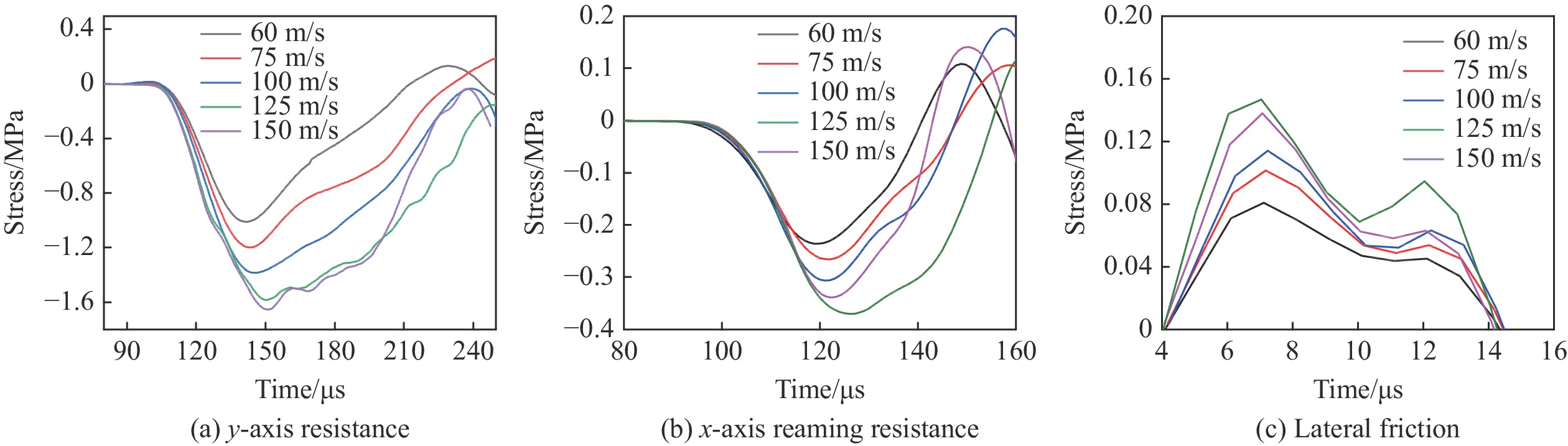
采用数值分析方法模拟了不同弹速下水泥砂浆的抗弹情况。图8显示了应力状态为三向侧限时不同弹速下各方向杆计算得到的结果。端部阻力、侧面扩孔阻力及侧面摩擦力幅值均随弹速的增加而增加;随着冲击速度的增加,开坑效应越来越明显,与图7所示形态一致。侧面摩擦脉宽远小于端部阻力与侧面扩孔力脉宽,表明侧面摩擦在开坑前期起主要作用。数值模拟结果所呈现的趋势与实验结果[15]基本一致。
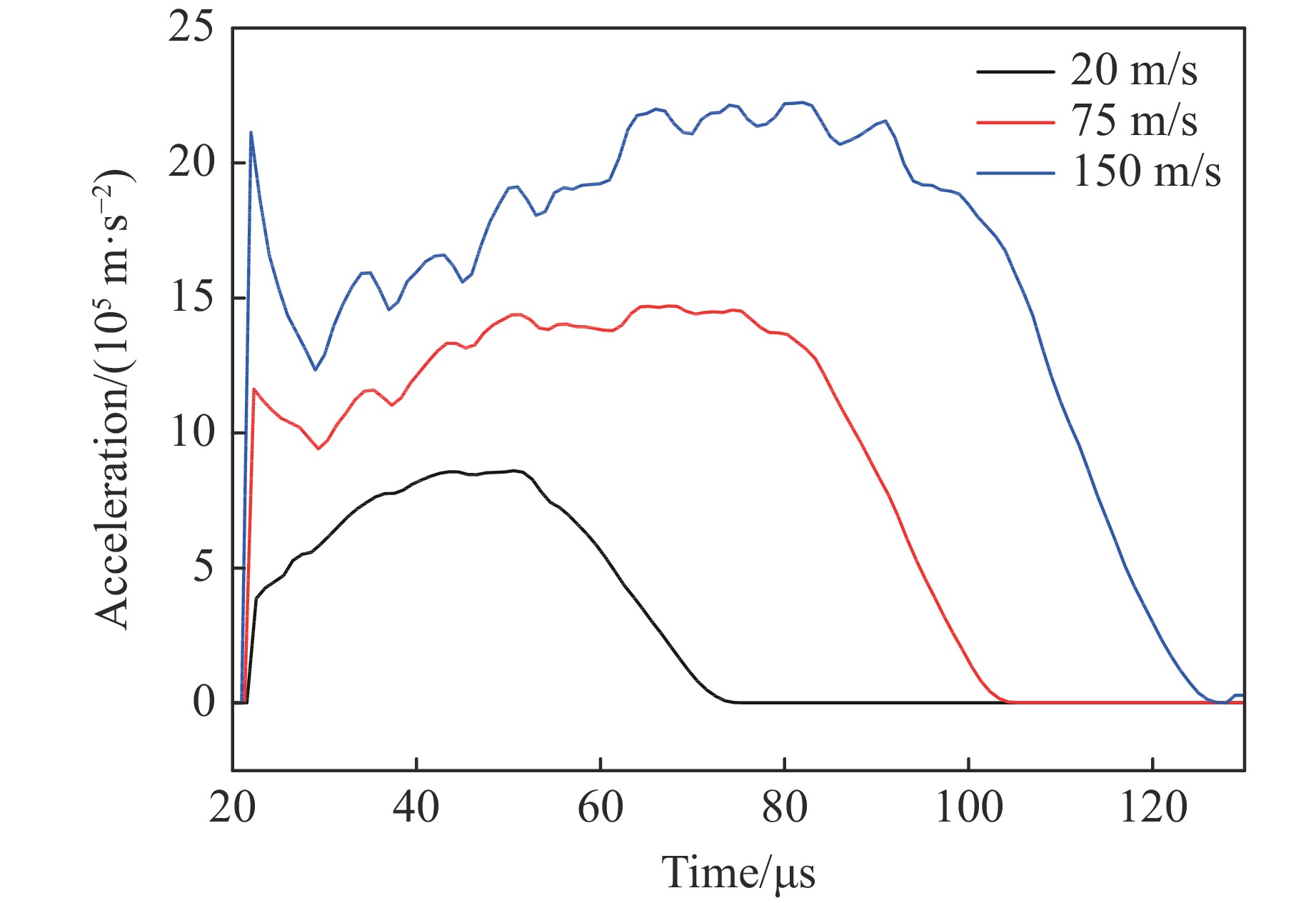
平头弹速度为20、75和150 m/s时,分别实现了弹丸与试件弹性碰撞、开坑较浅及完整开坑3种工况。图9显示了三向侧限时3种速度下弹丸的加速度时程曲线。随着弹丸速度的增加,弹丸端部加速度发展历程出现一定差异,即加速度脉宽逐渐增加。计算结果与图6(a)和图6(b)所示的测试结果有较大差异,计算加速度曲线没有明显的平台段。其原因在于:Forrestal等[25]的实验中,加速度记录点位于弹丸内部,与撞击端有一定的距离;本计算中,加速度记录点在弹丸内部并接近靶材的撞击端。在撞击端附近,加速度波形能很好地反映开坑过程,然而受加速度测试元件埋设工艺所限,实验中只能进行离撞击端较远处的加速度响应信息采集。在低速冲击下,弹丸以开坑为主。以20 m/s的速度碰撞靶体时,靶体处于弹性状态,弹丸压缩靶体后回弹,靶体没有损伤,加速度波形没有波动;随着弹丸速度增加,弹丸碰撞靶体后,靶体开始破损,加速度曲线出现波动,波动幅度随靶体破损程度的增加而增加。
计算结果表明:子弹内的加速度波形和y轴支撑杆上记录的波形都可以很好地反映弹丸开坑过程,理想的加速度波形需要通过靠近撞击端的测点记录。
3.3 应力状态对开坑过程的影响
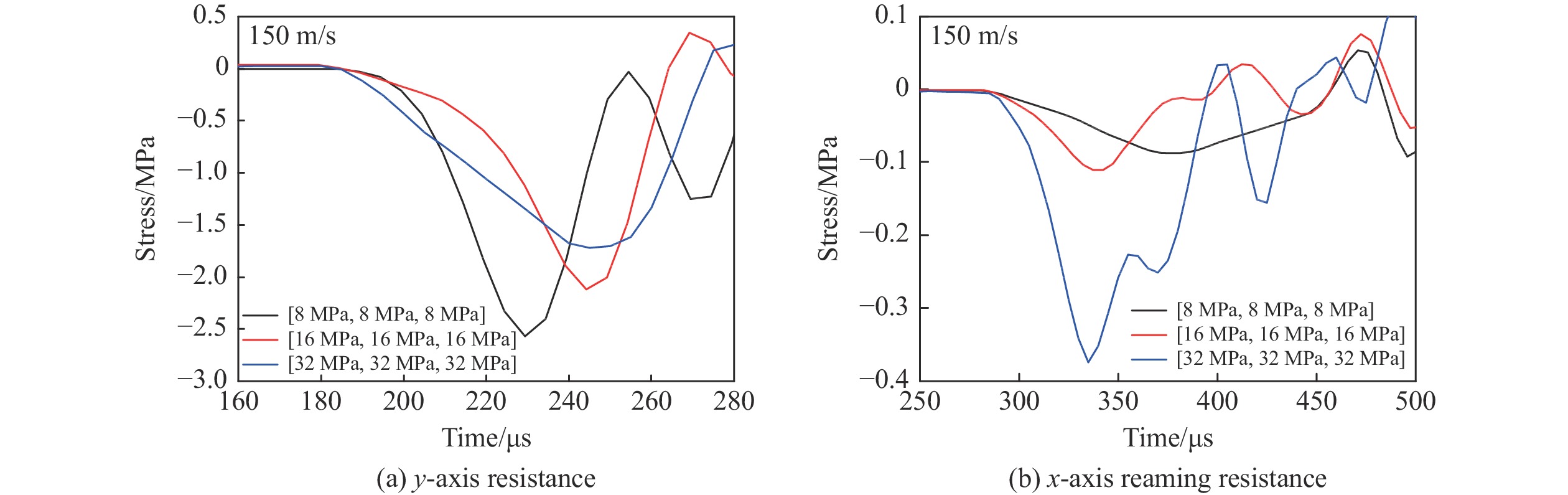
改变靶体初始应力状态,模拟相同弹速下不同应力状态的弹丸开坑过程。图10显示了冲击速度为150 m/s时3种应力状态下各杆上应力计算结果。当靶体围压从8 MPa增加至32 MPa时,y方向端部阻力的应力峰值随着围压的增加而减小,脉宽随围压的增加而增加;应力值的变化趋势与实验结果[15]一致,但脉宽变化存在一定差异。图10(b)所示的侧面膨胀力信号随着围压的增加而明显增强,波形呈现出明显差异,表明初始应力状态对开坑过程有较大影响,进行深部抗弹研究时需要给予足够的重视。
4. 讨 论
综合上述数值分析结果,可以看出:(1)HJC模型计算的开坑深度从整体上看比较有效,其数值比实验结果略高;(2)HJC模型计算的端部阻力与实验测试结果虽然在趋势上比较一致,但是在数值上比实验结果高很多。其原因比较复杂。
冲击作用下水泥砂浆具有静水压效应和应变率效应,两种效应是耦合的,如何分离尚没有很好的方法。HJC 模型中仅考虑了应变率效应的影响,即在冲击过程中通过引入率敏感项
$\left( {1 + C_{\rm {HJC}}\ln {{\dot \varepsilon }^ * }} \right)$ 来考虑动态强度的增加。张磊等[27]的研究表明,混凝土材料的率效应具有分段特性,即存在多个CHJC值。HJC模型中,状态方程分为3个阶段,这3个阶段的静水压效应不同,而分段的应变率敏感项则是对不同阶段的静水压效应的逐步考虑。本研究所采用的HJC模型参数参考Meyer的实验结果,开坑深度的计算比较符合实验结果,但是由于没有采用分段式率效应表达,因此使用适用于较高冲击速度的CHJC值是造成HJC模型计算结果中坑深略高的原因。HJC模型中,第1阶段的静水压
$p = K\mu $ ,第2阶段的静水压$p = {p_{\rm{c}}} + {{(\mu - {\mu _{\rm{c}}})({p_{\rm{l}}} - {p_{\rm{c}}})}}/({{{\mu _{\rm{p}}} - {\mu _{\rm{c}}}}}) $ ,第3阶段的静水压$p = {k_1}\bar \mu + {k_2}{\bar \mu ^2} + {k_3}{\bar \mu ^3}$ ,$\;\bar {\mu}$ 为修正的体积应变。这种表达对于中等和较高的应力水平较好,而对于较低的应力水平则会产生较大的误差。在前期较低的应力水平下,这类关系曲线的体积变形随静水压的增加快速增长;但是,实际上当对试件施加三向围压时,由于侧限的影响,试件没有明显的压缩过程,即压缩过程中试样体积变形较小,而试样静水压力值迅速增加,因此试样所受的静水压力值随着体积变形的增加快速增加。这是HJC模型在保证坑深计算较好的情况下,却得到较低应力水平的原因。要在较低冲击速度下同时得到较好的坑深和应力模拟结果,需要将各阶段的应变率效应和静水压效应等参数纳入模拟过程,把不同阶段的材料特性完整呈现出来,建立一个较系统的新的模型参数。5. 结 论
基于真三轴应力状态下水泥砂浆材料侵彻性能的实验结果,进行了经验公式计算、无量纲公式计算、有限元数值模拟对比研究,得到如下主要结论:
(1)应用基于HJC模型的有限元方法分析开坑深度和开坑阻力,计算得到的开坑深度与实验结果基本一致,但是应力与实验结果差别较大,表明HJC模型能有效模拟不同应力状态下的开坑深度,但是却只能定性描述开坑阻力;
(2)不同侵彻经验公式对侵彻结果的预测存在一定的差异,其中UMIST经验公式对低速侵彻实验深度的预测较为有效;
(3)虽然子弹加速度波形和y轴支撑杆上记录的波形都能反映弹丸开坑过程,但是y轴支撑杆上表现的开坑特征更明显,数值模拟结果中杆上的应力波形与实验结果趋势一致,应力峰值均随着弹丸速度的增加而增加。
-
表 1 水泥砂浆的HJC本构模型参数
Table 1. Parameters of HJC model for cement mortar
${\;\rho {_0} }$/(kg·m−3) $G$/GPa AHJC/GPa BHJC/GPa CHJC NHJC $f{'} $/MPa 1844 1.32 0.66 1.335 0.0018 0.845 14.4 T/MPa ${\dot \varepsilon{_0} }$/s−1 $\varepsilon $f,min Smax ${p{\rm{_c}} }$/MPa ${\;\mu {\rm{_c}} }$ ${p{\rm{_l} } }$/GPa 2.0 1 0.01 80.24 13.8 0.0075 1.096 ${\;\mu {\rm{_l} } }$ k1/GPa k2/GPa k3/GPa D1 D2 0.15 85 −171 208 0.006629 1.0 表 2 弹丸JC本构模型参数
Table 2. Parameters of JC model of projectile
${\rho{_0} }$/(kg·m−3) G/GPa T0/K c/(J·kg−1·K−1) AJC/MPa BJC/MPa nJC 7830 77 293 477 792 510 0.26 CJC Tm/K d1 d2 d3 d4 d5 0.014 1793 0.05 3.44 −2.12 0.002 0.61 表 3 无量纲侵彻深度公式参数
Table 3. Formula parameters of dimensionless penetration depth
Stress state k0 m1 m2 m3 No confinement 0.90 0.70 1.21 0.60 Unilateral confinement 1.02 0.55 1.25 0.65 Bilateral confinement 1.05 0.40 1.30 0.68 -
[1] 徐松林, 王鹏飞, 赵坚, 等. 基于三维Hopkinson杆的混凝土动态力学性能研究 [J]. 爆炸与冲击, 2017, 37(2): 180–185. doi: 10.11883/1001-1455(2017)02-0180-06XU S L, WANG P F, ZHAO J, et al. Dynamic behavior of concrete under static triaxial loading using 3D-Hopkinson bar [J]. Explosion and Shock Waves, 2017, 37(2): 180–185. doi: 10.11883/1001-1455(2017)02-0180-06 [2] 徐松林, 王鹏飞, 单俊芳, 等. 真三轴静载作用下混凝土的动态力学性能研究 [J]. 振动与冲击, 2018, 37(15): 59–67. doi: 10.13465/j.cnki.jvs.2018.15.008XU S L, WANG P F, SHAN J F, et al. Dynamic behavior of concrete under static tri-axial loadings [J]. Journal of Vibration and Shock, 2018, 37(15): 59–67. doi: 10.13465/j.cnki.jvs.2018.15.008 [3] XU S L, SHAN J F, ZHANG L, et al. Dynamic compression behaviors of concrete under true triaxial confinement: an experimental technique [J]. Mechanics of Materials, 2020, 140: 103220. doi: 10.1016/j.mechmat.2019.103220 [4] FORRESTAL M J, ALTMAN B S, CARGILE J D, et al. An empirical equation for penetration depth of ogive-nose projectiles into concrete targets [J]. International Journal of Impact Engineering, 1994, 15(4): 395–405. doi: 10.1016/0734-743X(94)80024-4 [5] CHEN X W, LI Q M. Deep penetration of a non-deformable projectile with different geometrical characteristics [J]. International Journal of Impact Engineering, 2002, 27(6): 619–637. doi: 10.1016/S0734-743X(02)00005-2 [6] CHEN X W, LI J C. Analysis on the resistive force in penetration of a rigid projectile [J]. Defence Technology, 2014, 10(3): 285–293. doi: 10.1016/j.dt.2014.06.007 [7] 沈河涛. 弹丸侵彻混凝土介质效应的研究[D]. 北京: 北京理工大学, 1996.SHEN H T. Study on the effect of projectile penetrating concrete medium [D]. Beijing: Beijing Institute of Technology, 1996. [8] BACKMAN M E, GOLDSMITH W. The mechanics of penetration of projectiles into targets [J]. International Journal of Engineering Science, 1978, 16(1): 1–99. doi: 10.1016/0020-7225(78)90002-2 [9] 薛建锋, 沈培辉, 王晓鸣. 弹体侵彻混凝土开坑阶段阻力的计算 [J]. 高压物理学报, 2016, 30(6): 499–504. doi: 10.11858/gywlxb.2016.06.010XUE J F, SHEN P H, WANG X M. Resistance during cratering for projectile penetrating into concrete target [J]. Chinese Journal of High Pressure Physics, 2016, 30(6): 499–504. doi: 10.11858/gywlxb.2016.06.010 [10] 蒋志刚, 甄明, 刘飞, 等. 钢管约束混凝土抗侵彻机理的数值模拟 [J]. 振动与冲击, 2015, 34(11): 1–6. doi: 10.13465/j.cnki.jvs.2015.11.001JIANG Z G, ZHEN M, LIU F, et al. Simulation of anti-penetration mechanism of steel tube confined concrete [J]. Journal of Vibration and Shock, 2015, 34(11): 1–6. doi: 10.13465/j.cnki.jvs.2015.11.001 [11] 朱翔, 陆新征, 杜永峰, 等. 外包钢管加固RC柱抗冲击试验研究 [J]. 工程力学, 2016, 33(6): 23–33. doi: 10.6052/j.issn.1000-4750.2014.11.0991ZHU X, LU X Z, DU Y F, et al. Experimental study on impact resistance of reinforced conceret columns strengthened with steel jackets [J]. Engineering Mechanics, 2016, 33(6): 23–33. doi: 10.6052/j.issn.1000-4750.2014.11.0991 [12] 甄明, 蒋志刚, 万帆, 等. 钢管约束混凝土抗侵彻性能试验 [J]. 国防科技大学学报, 2015, 37(3): 121–127. doi: 10.11887/j.cn.201503020ZHEN M, JIANG Z G, WAN F, et al. Steeltube confined concrete targets penetration experiments [J]. Journal of National University of Defense Technology, 2015, 37(3): 121–127. doi: 10.11887/j.cn.201503020 [13] 蒙朝美, 宋殿义, 蒋志刚, 等. 多边形钢管约束混凝土靶抗侵彻性能试验研究 [J]. 振动与冲击, 2018, 37(13): 14–19. doi: 10.13465/j.cnki.jvs.2018.13.003MENG C M, SONG D Y, JIANG Z G, et al. Tests for anti-penetration performance of polygonal steel tube-confined concrete targets [J]. Journal of Vibration and Shock, 2018, 37(13): 14–19. doi: 10.13465/j.cnki.jvs.2018.13.003 [14] 徐松林, 单俊芳, 王鹏飞, 等. 三轴应力状态下混凝土的侵彻性能研究 [J]. 爆炸与冲击, 2019, 39(7): 071101. doi: 10.11883/bzycj-2019-0034XU S L, SHAN J F, WANG P F, et al. Penetration performance of concrete under triaxial stress [J]. Explosion and Shock Waves, 2019, 39(7): 071101. doi: 10.11883/bzycj-2019-0034 [15] 陈丽娜, 单俊芳, 周李姜, 等. 应力状态对水泥砂浆侵彻性能的影响 [J]. 振动与冲击, 2020, 39(15): 32–40. doi: 10.13465/j.cnki.jvs.2020.15.005CHEN L N, SHAN J F, ZHOU L J, et al. Effects of stress state on penetration performance of cement mortar [J]. Journal of Vibration and Shock, 2020, 39(15): 32–40. doi: 10.13465/j.cnki.jvs.2020.15.005 [16] MEYER C S. Development of geomaterial parameters for numerical simulations using the Holmquist-Johnson-Cook constitutive model for concrete: ARL-TR-5556 [R]. Orlando: Army Research Laboratory, 2011. [17] JOHNSON G R, COOK W H. Fracture characteristics of three metals subjected to various strains, strain rates, temperatures and pressures [J]. Engineering Fracture Mechanics, 1985, 21(1): 31–48. doi: 10.1016/0013-7944(85)90052-9 [18] Army Corps of Engineers. Fundamentals of protective design: AT1207821 [R]. Army Corps of Engineers, 1946. [19] National Defense Research Committee. Effects of impact and explosion: summery technical report of division 2 [R]. Washington DC: National Defense Research Committee, 1946. [20] KENNEDY R P. A review of procedures for the analysis and design of concrete structures to resist missile impact effects [J]. Nuclear Engineering and Design, 1976, 37(2): 183–203. doi: 10.1016/0029-5493(76)90015-7 [21] BARR P. Guidelines for the design and assessment of concrete structures subjected to impact [R]. London, UK: UK Atomic Energy Authority, Safety and Reliability Directorate, 1990. [22] YOUNG C W. Penetration equations: SAND 97-2426 [R]. Albuquerque, NM, US: Sandia National Laboratories, 1997. [23] REID S R, WEN H M. Predicting penetration, cone cracking, scabbing and perforation of reinforced concrete targets struck by flat-faced projectiles: UMIST Report ME/AM/02.01/TE/G/018507/Z [R]. Manchester: University of Manchester Institute of Science and Technology, 2001. [24] LI Q M, CHEN X W. Dimensionless formulae for penetration depth of concrete target impacted by a non-deformable projectile [J]. International Journal of Impact Engineering, 2003, 28(1): 93–116. doi: 10.1016/S0734-743X(02)00037-4 [25] FORRESTAL M J, FREW D J, HICKERSON J P, et al. Penetration of concrete targets with deceleration-time measurements [J]. International Journal of Impact Engineering, 2003, 28(5): 479–497. doi: 10.1016/S0734-743X(02)00108-2 [26] 王琳, 王富耻, 王鲁, 等. 空心弹体垂直侵彻混凝土靶板的应变测试研究 [J]. 北京理工大学学报, 2002, 22(4): 453–456. doi: 10.3969/j.issn.1001-0645.2002.04.014WANG L, WANG F C, WANG L, et al. Strain measurement in hollow projectiles impacting concrete targets [J]. Journal of Beijing Institute of Technology, 2002, 22(4): 453–456. doi: 10.3969/j.issn.1001-0645.2002.04.014 [27] 张磊, 任新见, 孔德锋. 钢筋混凝土HJC模型的研究和改进[C]//第四届全国工程安全与防护学术会议. 洛阳, 2014: 134−138.ZHANG L, REN X J, KONG D F. Research and improvement of HJC model of steel reinforced concrete [C]//Proceedings of the 4th National Conference of Engineering Safety and Protection. Luoyang, 2014: 134−138. -








 下载:
下载:
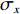
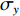
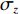


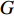

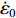
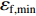
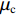
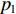
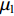

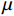
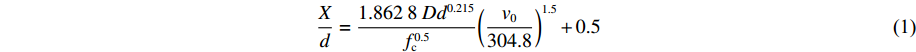
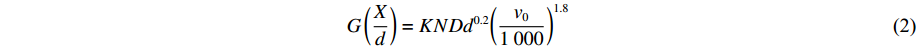
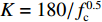
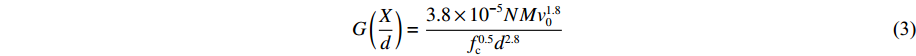
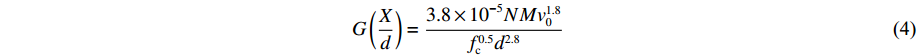
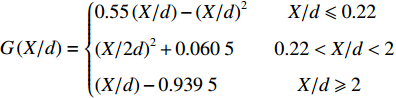
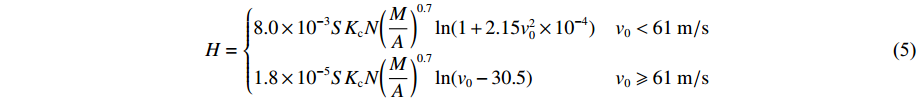
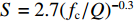
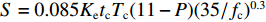
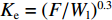
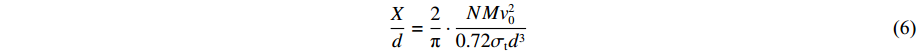
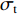
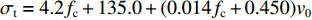
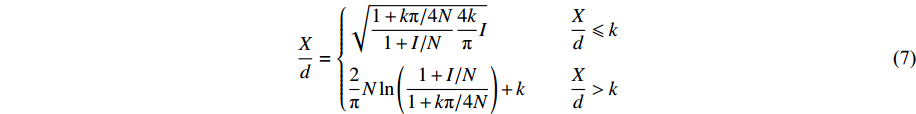
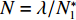

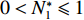
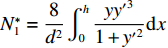
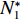
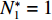


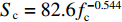

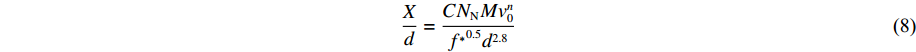
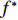

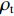
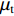
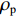

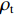
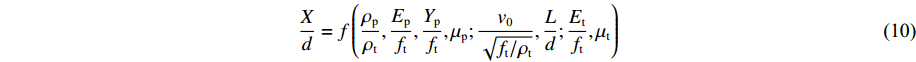
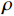
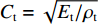
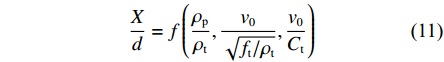
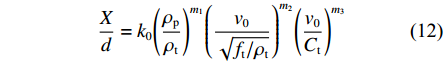






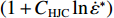
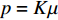
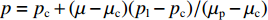
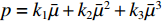
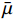

 下载:
下载: