Numerical Simulation of Quasi-Static Compression and Energy Absorption of Bionic BCC Structure
-
摘要: 晶格点阵结构因具有质量轻、吸能性好等优点,被广泛应用于航空、航天、军工等领域。研究了仿生体心立方(BCC)结构的吸能性,并探讨了截面形貌对BCC晶格结构吸能性的影响。基于毛竹的宏观结构和细观结构,设计了3种不同的BCC仿竹晶格点阵结构,对3种结构及原始BCC晶格结构进行了轴向准静态压缩数值模拟。结果表明:静载下仿竹BCC结构的吸能性和比吸能均比原始BCC结构提高了25%以上,但3种仿竹BCC结构的吸能性、比吸能相差不大;仿竹BCC结构的相对密度对其吸能性和比吸能的影响较大;在压缩过程中,仿生结构的韧性截面有效保证了塌陷稳定性,这是该结构吸能稳定的重要原因。Abstract: The lattice structure is widely used in aerospace, military, and other fields due to its lightweight and excellent energy absorption. This article mainly studies the energy absorption of the bionic BCC (body-centered cubic) structure and discusses its influence by the cross-sectional morphology. In this paper, three different BCC bionic bamboo lattice structures are designed based on the macro-structure and meso-structure of Phyllostachys pubescens. Additionally, the axial compression numerical simulation is carried out on the bionic bamboo lattice structures and original BCC lattice structure, respectively. The results show that both the energy absorption and specific energy absorption of the bionic bamboo lattice structures under quasi-static load are improved by more than 25% compared with the original BCC structure. However, the energy absorption and specific energy absorption of the three bionic bamboo lattice structures are similar. It is also indicated that the relative density of the structure has great influence on its energy absorption and specific energy absorption. During the compression process of the bionic BCC structure, there are wrinkles and collapses inside, which might be an important reason for the stable energy absorption of the bionic structure.
-
晶格结构因具有轻量化、高强度、高刚度以及出色的吸能性等特点,广泛应用于航空航天、汽车制造、军工等领域。近年来,国内外学者对体心立方(BCC)晶格结构开展研究并取得了一定成果。如Cao等[1]和Bai等[2]提出了变截面BCC晶格结构,发现与原结构相比,变截面晶格结构具有更好的力学性能和吸能性;Li[3]对商用3D打印的316L不锈钢微晶格结构进行了准静态压缩实验研究和数值模拟,发现塑性应变发生在铰结点附近;Tsopanos等[4]研究了微米级3D打印不锈钢材料的BCC晶格结构,指出激光曝光时间和激光功率将影响晶格结构的力学性能;Jin等[5]通过实验和数值计算,发现不同相对密度的BCC晶格结构在支柱连接处先破坏,揭示了可用幂函数描述具有不同相对密度晶格结构的力学性能。
近年来,采用仿生元素进行结构设计被广泛应用。如郝美荣[6]从菠萝纤维获得灵感,设计了点阵圆筒结构,并测试其平压性能;Tsang等[7]研究了仿生管结构的吸能性;Zou等[8]研究了管内具有多个仿生元素仿生管的仿生结构;Liu等[9]设计了碳纤维增强塑料(CFRP)和Al方管,并进行了准静态压缩试验,研究其破坏过程、耐撞性能及相应的能量吸收机制;Tao等[10]进行了准静态压缩方形分层蜂窝(SHHs)和常规方形蜂窝(RSH)结构的平面准静态压缩试验,探讨子结构数目对变形模式、力学性能和能量吸收的影响;魏灿刚[11]对仿竹结构薄壁管进行了耐撞性设计和能量吸收研究。
本研究将通过仿竹元素与拓扑结构相结合的方法改进BCC结构,数值模拟其轴向准静态压缩过程,研究仿竹结构与原始BCC结构的吸能性和比吸能,以及分析仿生结构在准静态压缩过程中的变形模式。
1. 仿生结构的设计方法
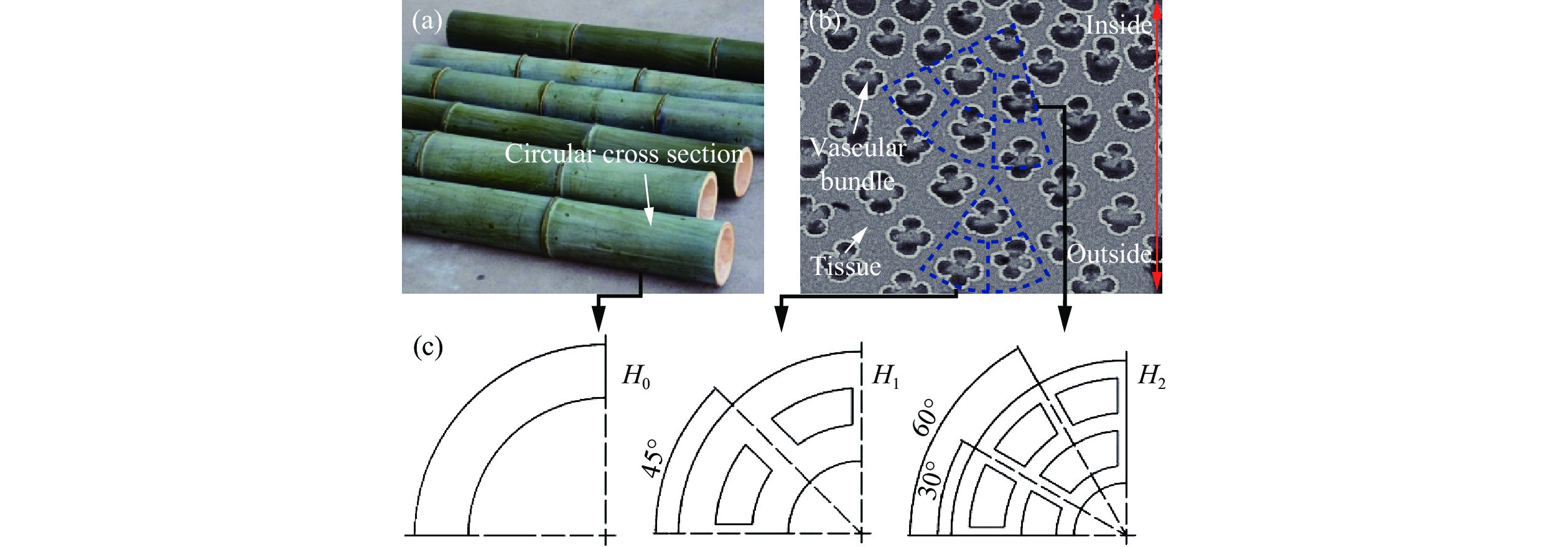
如图1(a)所示,宏观下毛竹具有中空结构,其竹壁由维管束和竹体组织构成,维管束从外侧向内侧逐渐增多,竹体组织密度逐渐降低。由图1(b)的组织细观结构可见,其内部包裹的维管束对结构起到缓冲和提高韧性的作用,主要表现在:(1)组织变形、挤压或塌陷过程中能够吸收外界能量;(2)在横向载荷作用下,竹体组织可以保护维管束,防止其拉伸、弯曲和扭转。根据宏观环形截面和细观组织分布的孔隙结构特征,设计了图1(c)所示的3种截面形貌。
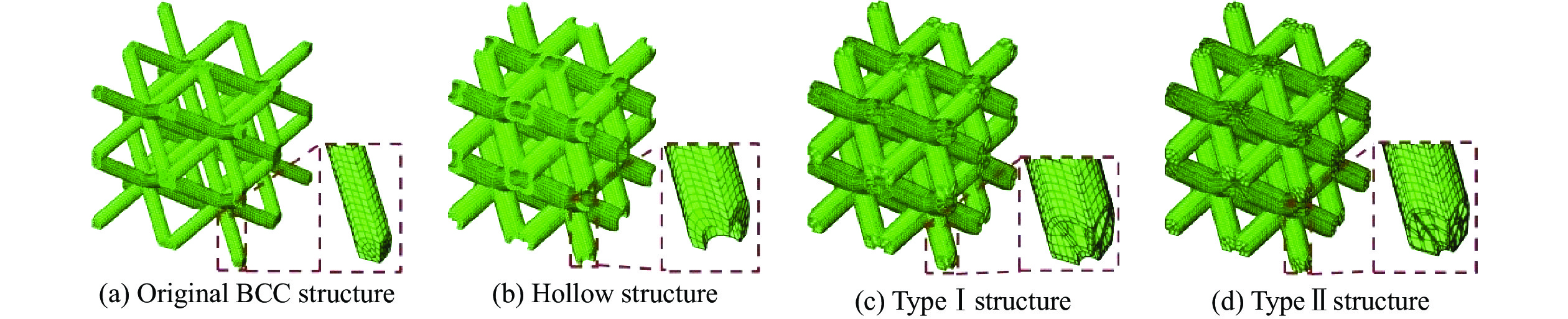
图2(a)为原始BCC实心结构。图2(b)、图2(c)和图2(d)分别为图1(c)中H0、H1和H2支杆截面对应的仿竹BCC结构,为便于命名,简称空心、Ⅰ型和Ⅱ型结构,具有仿竹截面的这3种BCC结构统称为仿生晶格。晶格边长为10 mm,截面最大直径不超过3 mm,如图1(c)所示,厚度
Hi(i=1,2,3) 是3个独立的尺寸参数,结构设计过程中,始终保持晶胞内部结构厚度一致,并由单胞以2 × 2 × 2方正形式堆叠而成,结构的相对密度为ˉρ=V∗/V (1) 式中:
V 为方块块体体积,V∗ 为单胞体积。原始BCC晶胞单胞体积为V∗=π ld2/4 (2) 式中:l为支柱长度,
d 为BCC晶胞支柱截面直径。BCC单胞的支柱长度l=√3a2/2 (3) 式中:a为晶格边长。
对原始BCC结构、仿竹空心结构、仿竹Ⅰ型结构和仿竹Ⅱ型结构分别设定13%、15%、19%和22% 4种相对密度,仿生晶格结构单个晶胞体积
V∗i=lSi (4) 式中:Si为接触净面积;i=1,2,3。使用ANSYS-spaceclaim(SCDM)建模软件计算结构的接触净面积
Si ,误差在0.1%以内,详细描述晶格结构的物理特性参数,见表1。表 1 晶格结构的物理参数Table 1. Physical parameters of the lattice structureLattice
structureRelative
density/%Diameter/mm Cross-sectional
area/mm2Thickness/mm Minimum
thickness/mmOriginal structure 13 1.66 15 1.81 19 2.08 22 2.25 Hollow
structure13 26.56 0.35 15 30.88 0.41 19 38.56 0.54 22 43.58 0.64 Type Ⅰ
structure13 24.07 0.06 15 27.80 0.07 19 36.64 0.10 22 41.92 0.12 Type Ⅱ
structure13 23.84 0.10 15 28.22 0.13 19 36.13 0.17 22 41.41 0.20 2. 建立有限元模型
2.1 准静态压缩分析
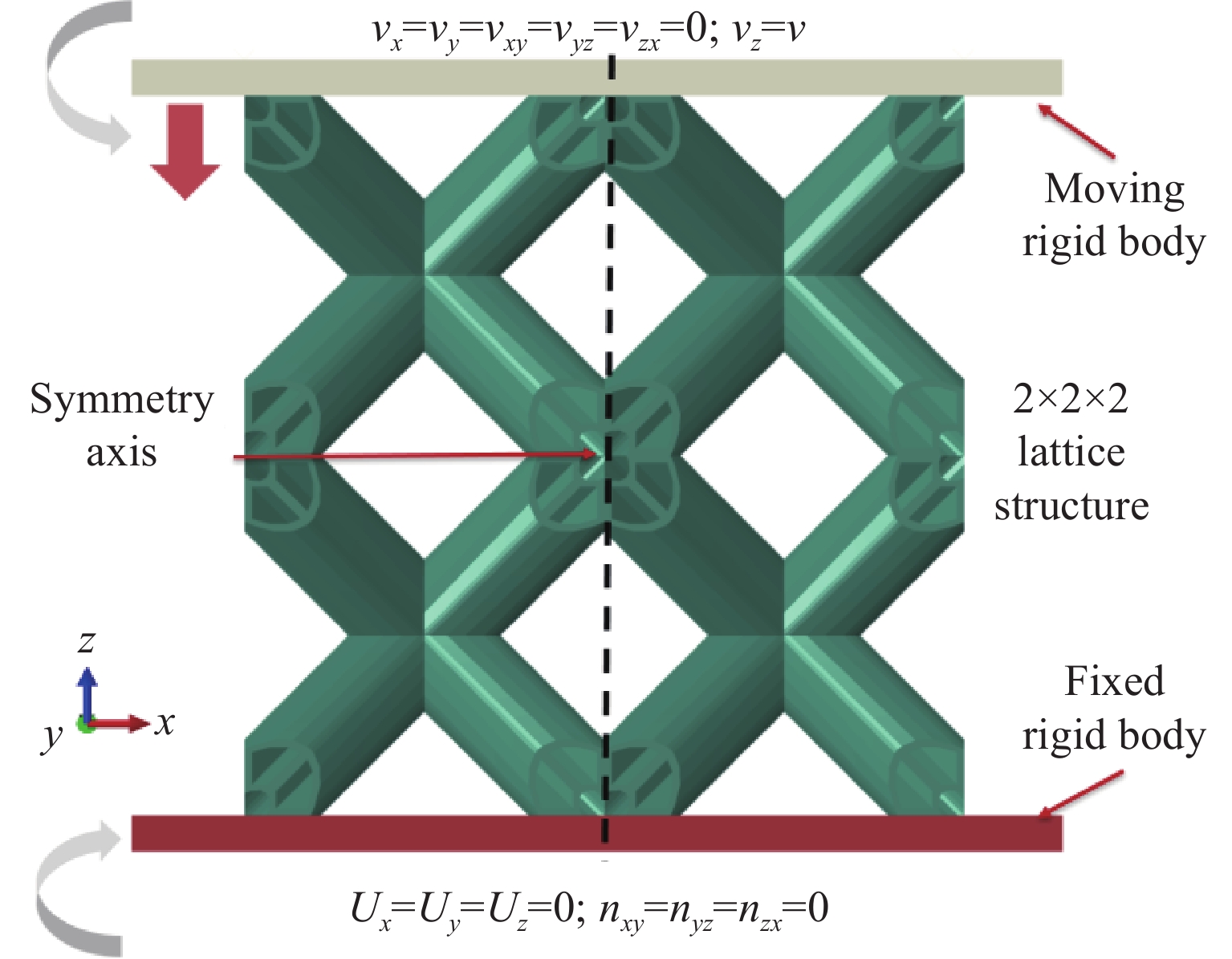
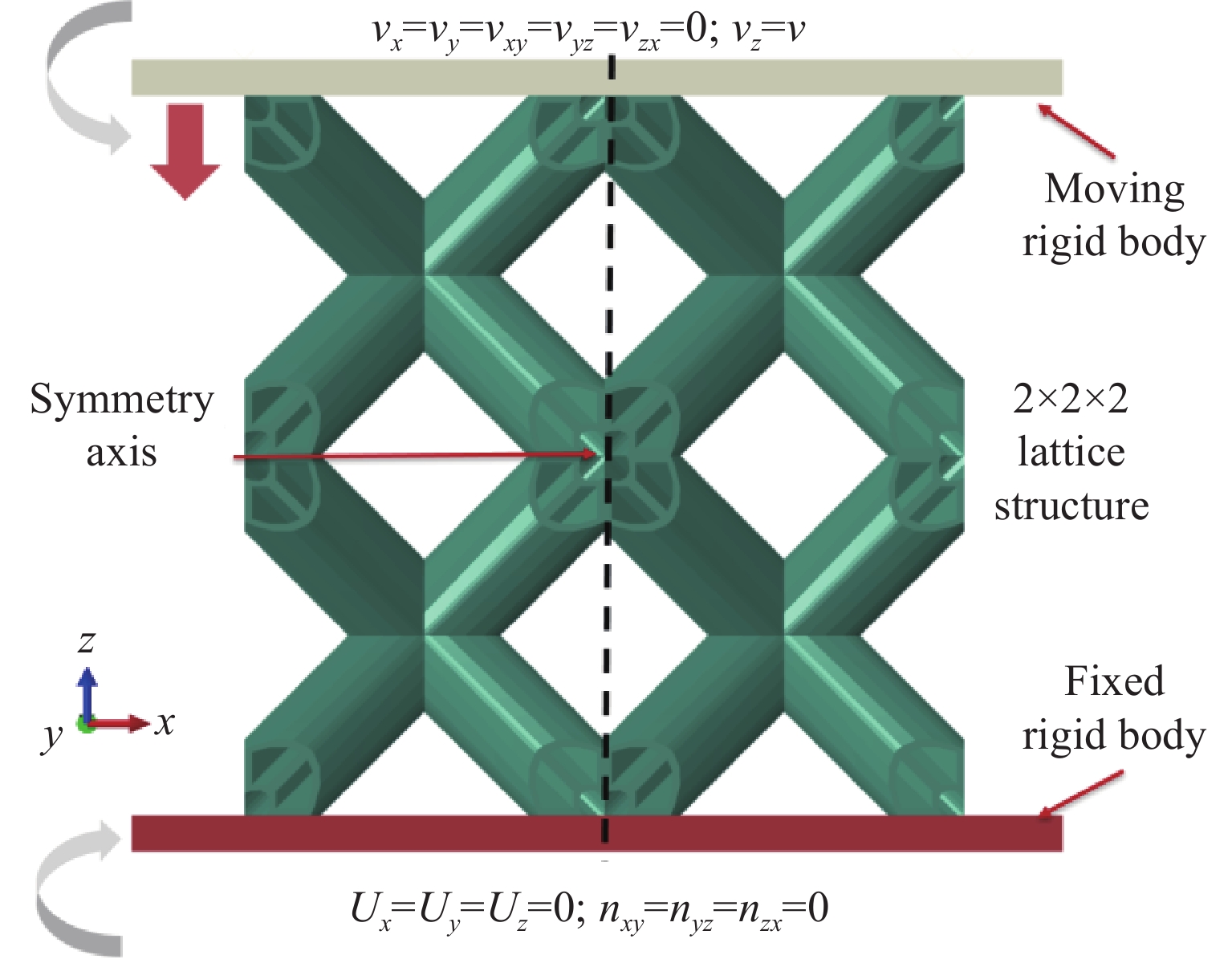
如图3所示,使用Abaqus-6.14-4版商用软件建立有限元模型,模拟晶格结构的准静态轴向压缩试验。晶格结构放置于移动和固定刚板之间,移动刚板以速度v向固定刚板移动。固定刚板设置为完全固定,这里Ux、Uy、Uz为固定刚板在x、y、z 3个方向的位移,nxy、nyz、nzx为3个旋转自由度,并且刚板在运动和受力过程中保持形状和大小不变。刚板速度满足
v=˙εL (5) 式中:
L 为结构压缩前的初始高度,˙ε 为结构压缩应变率。压缩应变e满足e=Δh/L (6) 式中:
Δh 为结构被压缩的距离。分别对4种相对密度的4种结构进行相同条件的压缩模拟,最终获取轴向压缩状态下的力-位移响应结果。晶格结构为选区激光熔化制造(SLM)的不锈钢316L,其密度为7980 kg/m3,弹性模量为80.78 GPa,屈服应力为636.57 MPa,泊松比为0.3。刚体与晶格结构的接触面设置为通用接触方式,假设接触切线方向无摩擦,接触法线方向设置为硬接触,由于结构压缩过程为非线性过程,考虑结构受压时的大变形行为。
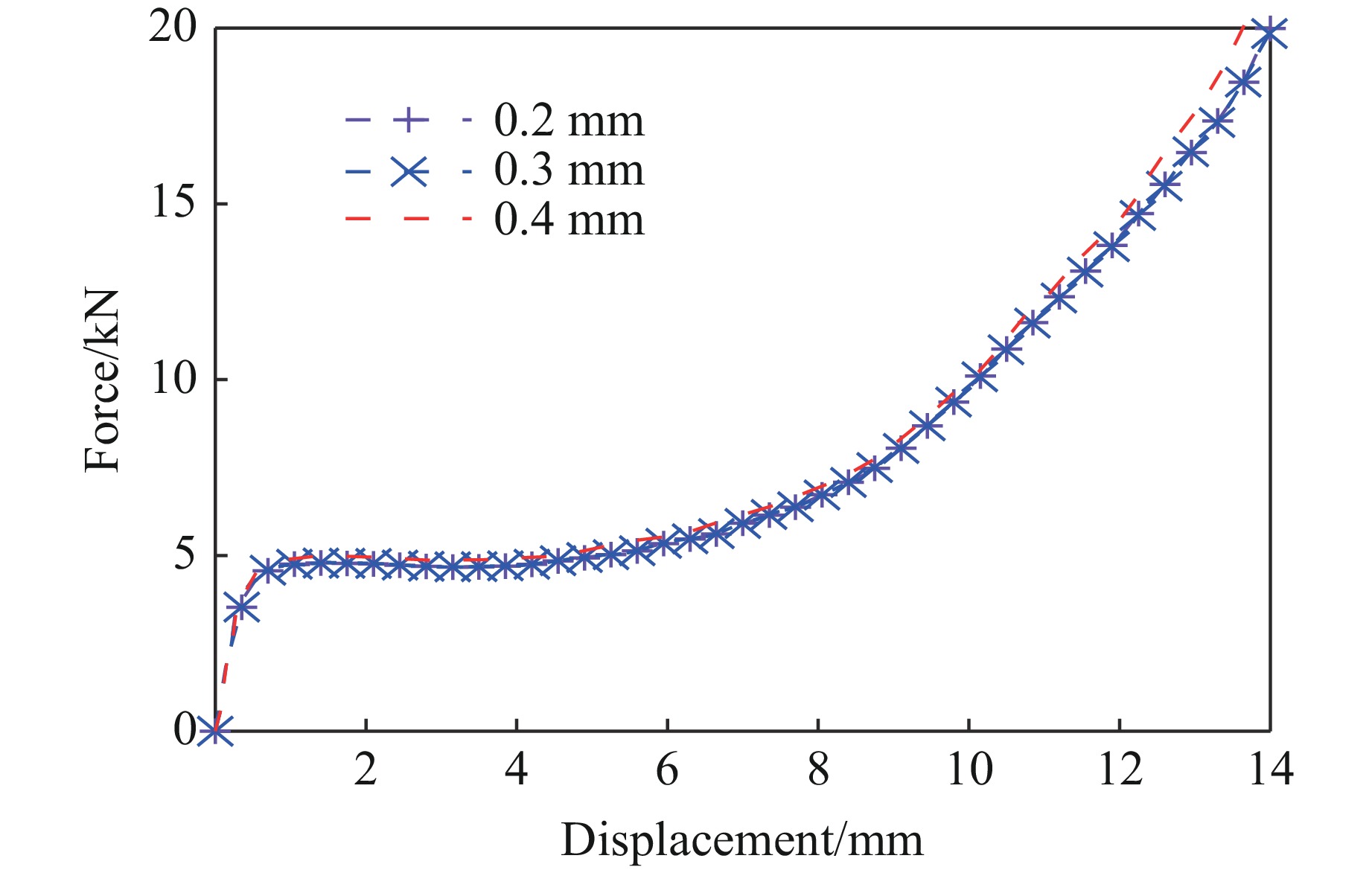
考虑到晶格结构具有对称性,取1/4晶格结构,采用六面体单元(C3D8R和C3D6)进行数值模拟,有限元网格如图4所示。在两个对称面上设置对称约束,假定材料对速率不敏感,对仿生空心结构进行网格灵敏度分析,将晶格结构分别划分为0.2、0.3和0.4 mm的网格单元,如图5所示。数值模拟结果表明,相对密度为15%的结构在模拟压缩时的力-位移响应结果一致。考虑模型的计算效率,选取最经济的网格尺寸0.4 mm。
2.2 模拟结果有效性验证
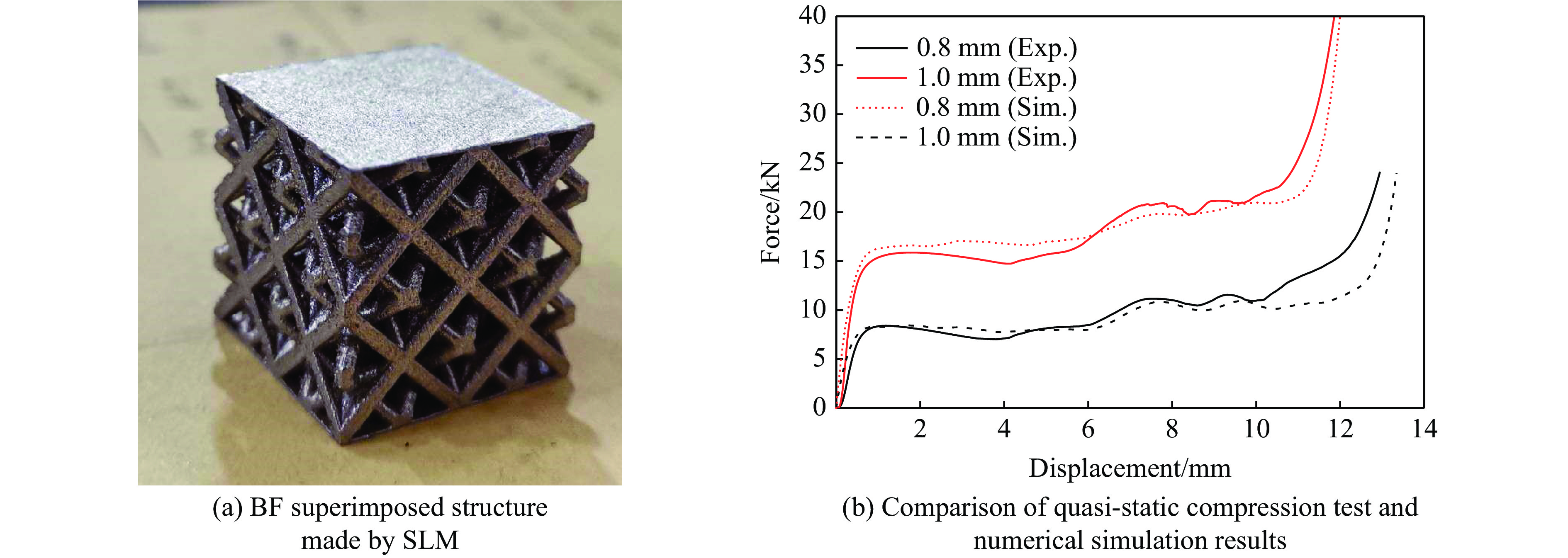
为了验证仿生结构准静态压缩数值模拟结果的有效性和正确性,以BCC和面心立方(Face center cube,FCC)为基础晶胞,如图6(a)所示,叠加设计了BF叠加晶胞结构,使用SLM制造了两种支杆直径(d = 0.8 mm和d = 1.0 mm)的叠加结构,在常温下,使用万能材料试验机对两种尺寸的试样进行准静态压缩实验,同时对整个压缩过程进行数值模拟。如图6(b)所示,准静态压缩实验获得的力-位移曲线与数值模拟结果高度一致,验证了本研究有限元模型的可靠性。
3. 结果和讨论
3.1 能量吸收评估
以恒定速度加载得到反作用力和压缩位移的响应结果,根据式(6)和式(7)将实验获得的力-位移曲线转换为应力-应变曲线
σ=F/Ai (7) 式中:
σ 为应变,Ai 为晶格结构与移动刚体初始接触面积,F 为结构被压缩过程中的反作用力。结构吸收能量EA为压缩过程中结构在应力-应变曲线下的积分[12]EA=∫e00σde (8) 式中:
e0 为能量吸收截止应变0.4。单位质量结构吸收能量ESA表示能量的吸收效率[13]ESA=EA/m (9) 式中:m为结构的总质量。
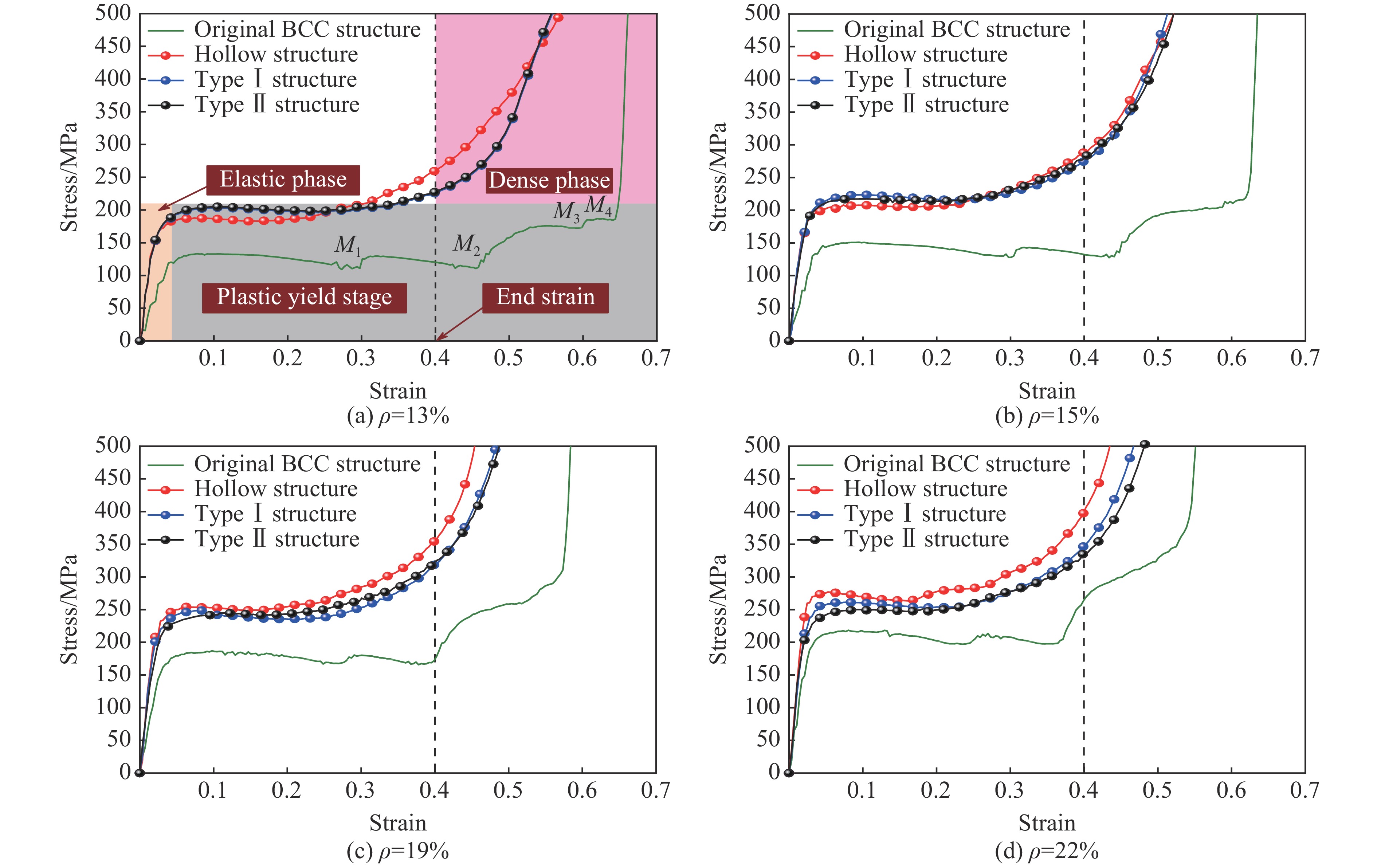
晶格结构的变形曲线可以划分为3个阶段:小应变范围的弹性变形阶段、塑性屈服平台阶段和压缩致密阶段。如图7(a)所示,原始BCC结构在塑性屈服平台阶段出现了4段压缩不稳定波动,M1、M2、M3和M4表示在不同应变下的层压现象点,几乎不随相对密度(p)增大而改变,但处于该阶段时,屈服应力不断增加。仿生结构在屈服阶段较为稳定,屈服平台持续稳定在屈服应力值。空心结构中屈服应力随着相对密度增加达到最大值,屈服区间出现了轻微波动。Ⅰ型和Ⅱ型结构在压缩过程中均保持塑性屈服趋势,即满足吸能稳定性要求,随着相对密度增大,Ⅰ型结构的屈服应力略高于Ⅱ型结构。
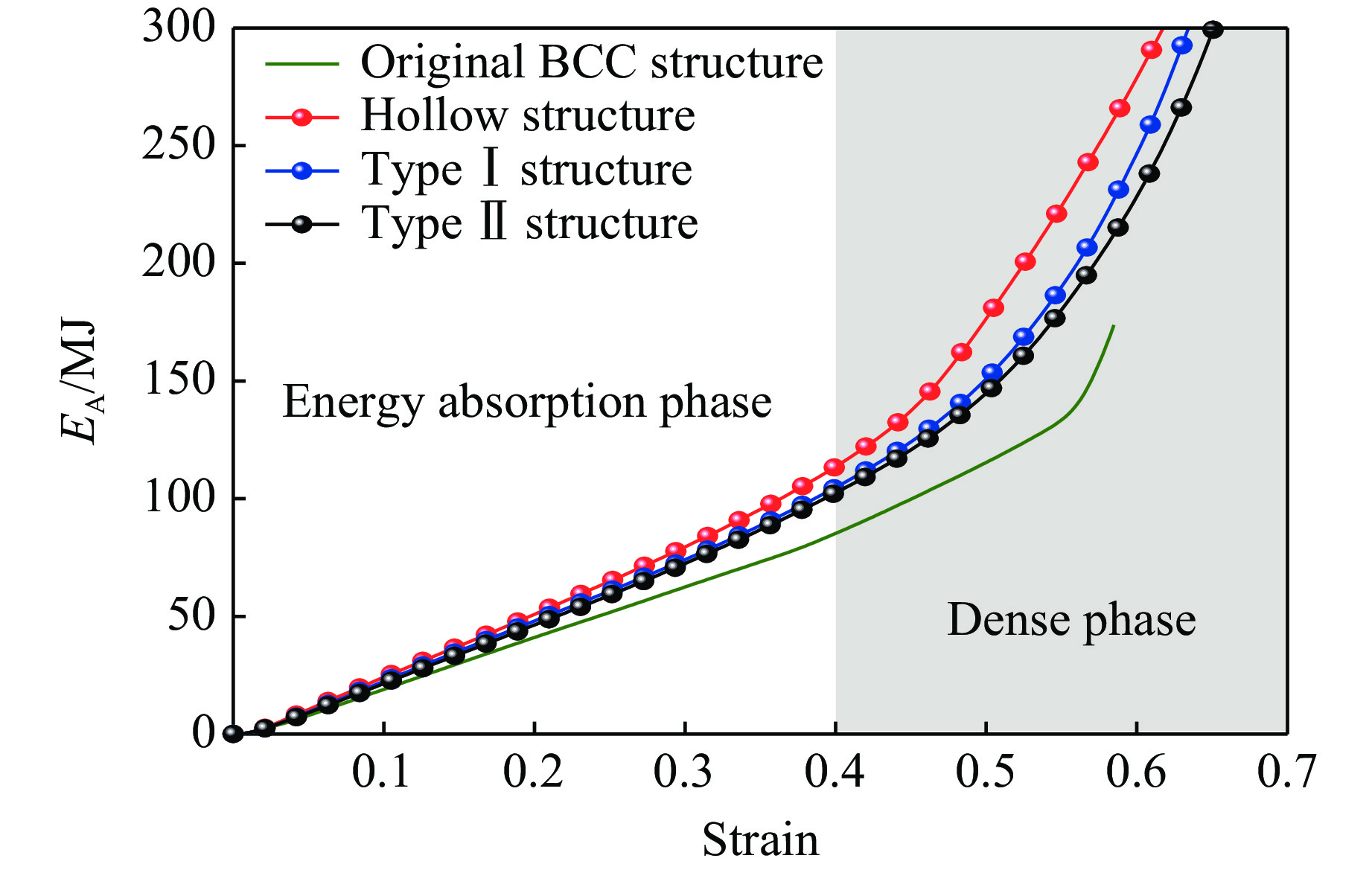
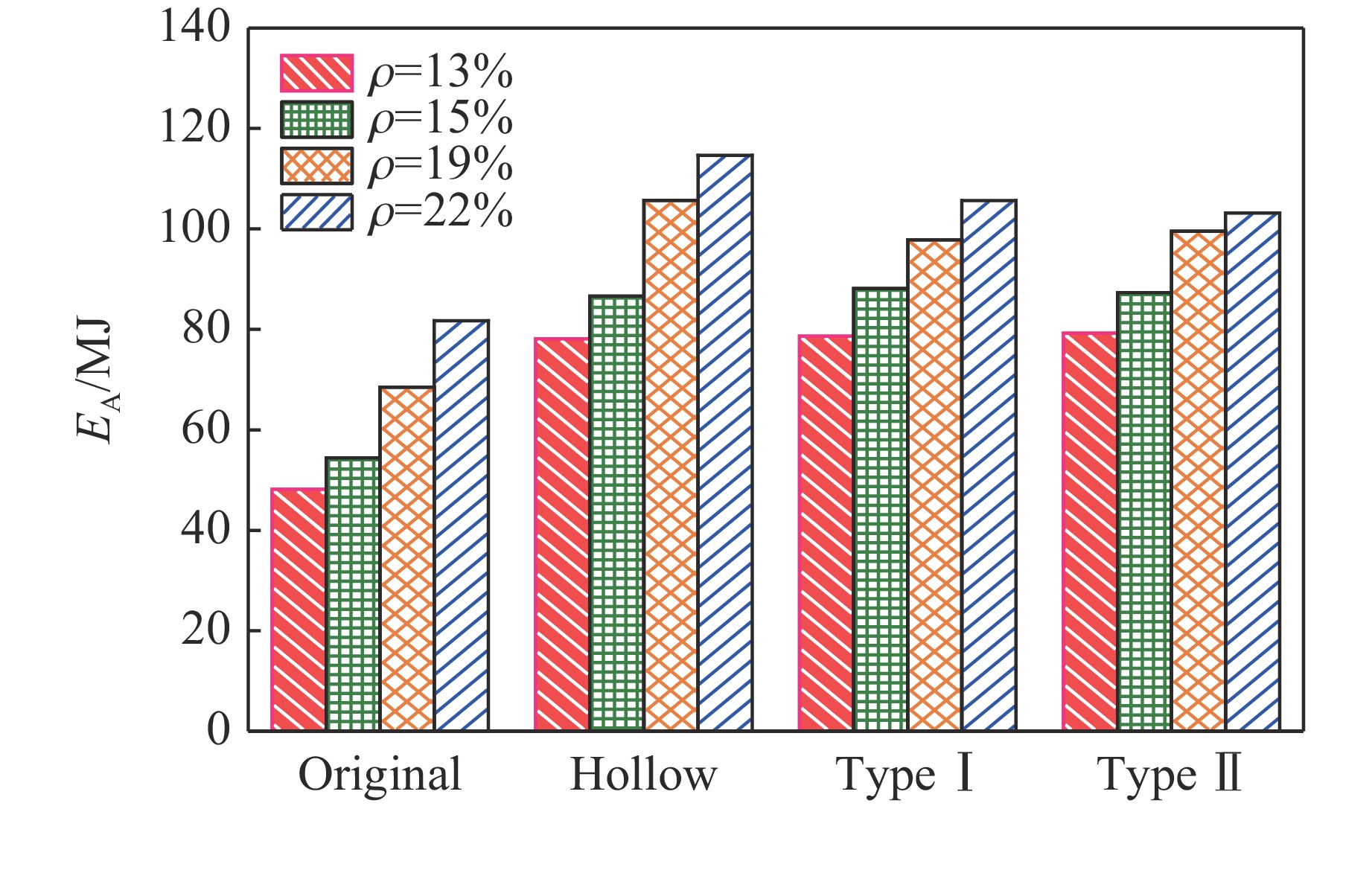
如图8所示,使用梯形求积公式计算晶格结构在相对密度为22%时应力-应变曲线累积面积,即能量吸收。晶格结构的吸能曲线呈单调递增趋势。在吸能阶段,空心结构的吸能效果最好,其次为Ⅰ型、Ⅱ型晶格结构。在致密阶段,吸能曲线的斜率增大,当结构不断被压缩致密时,各层晶格逐渐压实,此时的结构已由基体材料决定,不再属于晶格结构的稳定吸能范围。
4种结构的能量吸收及比吸能数据见表2。与原始BCC结构相比,由于仿生晶格结构在结构刚度和屈服强度等方面得到提升,当应变
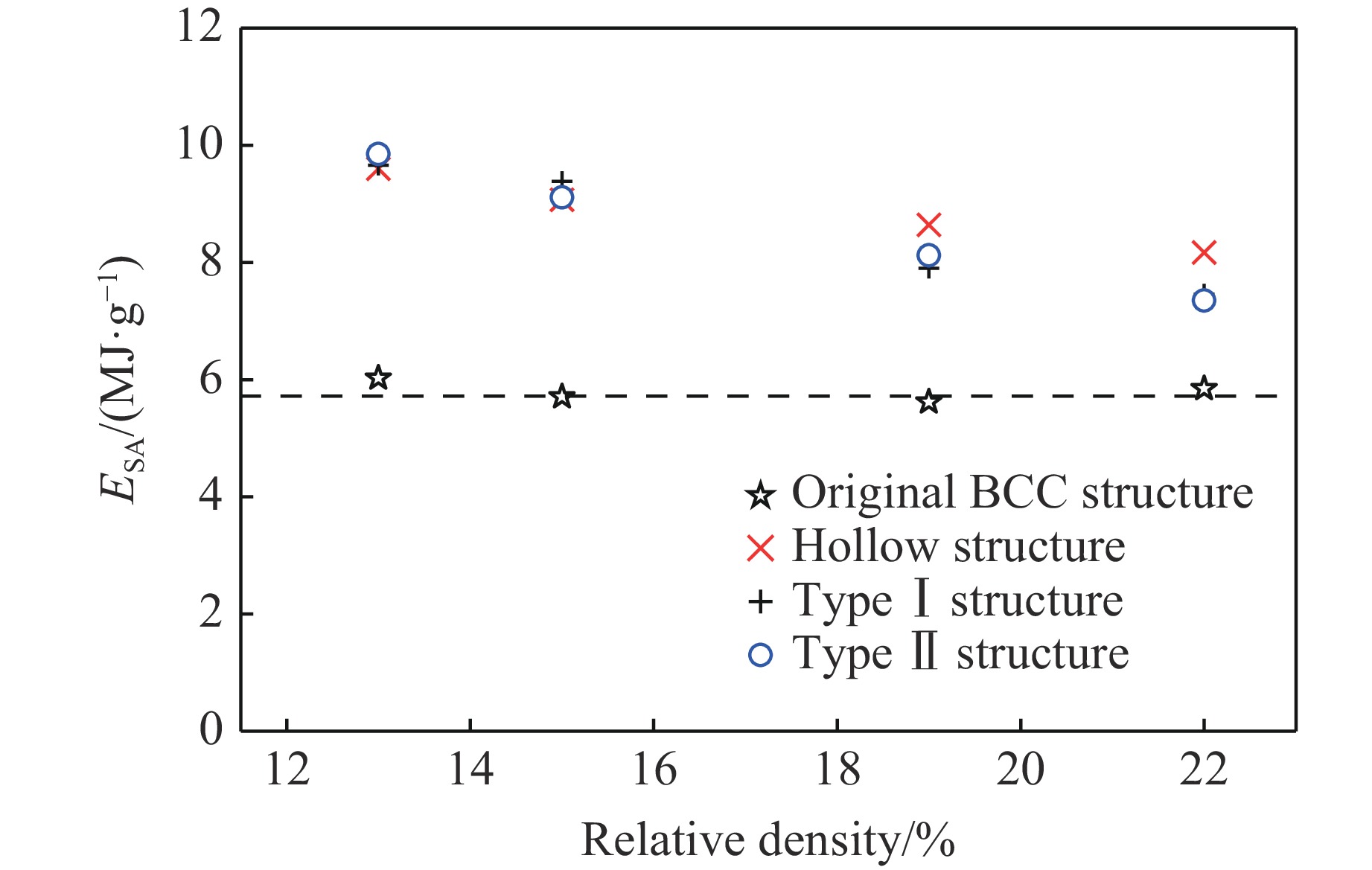
ε 在0~0.4范围内时,仿生晶格结构的能量吸收增加了26.23%~62.33%;相对密度为13%时,仿生晶格的吸能性相差不大;相对密度增加到22%时,空心结构的能量吸收达到最大值114.65 MJ。如图9所示,随着相对密度增大,4种结构的能量吸收均得到提高,空心结构增幅最大,达到36.52 MJ。如图10所示,在该应变范围内,仿生晶格结构的比吸能增加了25.64%~59.20%,随着相对密度增大,原始BCC结构的比吸能恒定在平均值5.8,而仿生晶格结构的比吸能呈现下降趋势,这是由于仿生晶格结构的相对密度增加,虽然初始屈服应力继续增长,但是塑性屈服平台范围逐渐缩小,并迅速向致密化过渡。表 2 晶格结构在0 < ε < 0.4范围内的能量吸收和比吸能Table 2. Energy absorption and specific energy absorption of lattice structure at 0 < ε < 0.4Lattice structure Mass/g EA/MJ ESA/(MJ·g–1) Original structure 7.98 48.13 6.03 9.53 54.39 5.71 12.21 68.51 5.62 13.98 81.72 5.85 Hollow structure 8.14 78.13 9.60 9.55 86.58 9.07 12.23 105.68 8.64 14.04 114.65 8.17 Type I structure 8.14 78.62 9.66 9.40 88.14 9.38 Type I structure 12.39 97.83 7.90 14.16 105.64 7.46 Type Ⅱ structure 8.04 79.21 9.85 9.58 87.29 9.11 12.26 99.52 8.12 14.04 103.16 7.35 3.2 变形模式评估
图11(a)展示了Ⅰ型晶格结构在俯视和正视角度下的Mises等效应力云图,S为应力分量。准静态压缩下的仿生晶格结构与原始BCC的变形具有共同的特点,都存在以拉伸和弯曲为主导的两种变形形式,不同的是,仿生晶格结构的支柱铰接处与支柱内部还发生皱折和塌陷现象。从图11(b)所示的等效塑性应变(PEEQ)云图中可以看到,塑性屈服主要集中在支柱的铰接处,压缩过程中支柱的铰接处首先发生屈服,当压缩位移继续增大,为Ⅰ型晶格的变形过程提供了塌陷空间,仿生晶格结构的韧性截面有效地保持了吸能稳定性。
4. 结 论
对仿生晶格结构的轴向准静态压缩进行了数值模拟,给出了4种相对密度下结构的应力-应变曲线,并用EA和ESA等指标对其进行评估,与原始BCC结构进行对比,得到如下结论。
(1)与原始BCC结构相比,仿竹BCC结构的吸能性、比吸能明显提高,且随着相对密度的增加,吸能性的提高值从62.33%下降至26.23%,比吸能提高值从25.64%升至59.20%。
(2)3种仿竹BCC结构的吸能性、比吸能相差不大。相对密度低时Ⅱ型结构略好,相对密度高时空心结构略好。
(3)原始BCC结构的比吸能随相对密度的变化略有波动,而仿生晶格结构的比吸能随着相对密度的增加逐渐减少。
(4)在准静态压缩下,原始BCC结构出现了屈服波动,而仿生晶格结构中主要是以弯曲和拉伸为主导的变形形式,其内部有折皱和塌陷现象,这是仿生结构吸能稳定的重要原因。
-
表 1 晶格结构的物理参数
Table 1. Physical parameters of the lattice structure
Lattice
structureRelative
density/%Diameter/mm Cross-sectional
area/mm2Thickness/mm Minimum
thickness/mmOriginal structure 13 1.66 15 1.81 19 2.08 22 2.25 Hollow
structure13 26.56 0.35 15 30.88 0.41 19 38.56 0.54 22 43.58 0.64 Type Ⅰ
structure13 24.07 0.06 15 27.80 0.07 19 36.64 0.10 22 41.92 0.12 Type Ⅱ
structure13 23.84 0.10 15 28.22 0.13 19 36.13 0.17 22 41.41 0.20 表 2 晶格结构在0 < ε < 0.4范围内的能量吸收和比吸能
Table 2. Energy absorption and specific energy absorption of lattice structure at 0 < ε < 0.4
Lattice structure Mass/g EA/MJ ESA/(MJ·g–1) Original structure 7.98 48.13 6.03 9.53 54.39 5.71 12.21 68.51 5.62 13.98 81.72 5.85 Hollow structure 8.14 78.13 9.60 9.55 86.58 9.07 12.23 105.68 8.64 14.04 114.65 8.17 Type I structure 8.14 78.62 9.66 9.40 88.14 9.38 Type I structure 12.39 97.83 7.90 14.16 105.64 7.46 Type Ⅱ structure 8.04 79.21 9.85 9.58 87.29 9.11 12.26 99.52 8.12 14.04 103.16 7.35 -
[1] CAO X F, DUAN S Y, LIANG J, et al. Mechanical properties of an improved 3D-printed rhombic dodecahedron stainless steel lattice structure of variable cross section [J]. International Journal of Mechanical Sciences, 2018, 145: 53–63. doi: 10.1016/j.ijmecsci.2018.07.006 [2] BAI L, YI C Y, CHEN X H, et al. Effective design of the graded strut of BCC lattice structure for improving mechanical properties [J]. Materials, 2019, 12: 2192. doi: 10.3390/ma12132192 [3] LI P F. Simulating the dynamic deformation behaviour of selective laser melted stainless steel microlattice structures [J]. 2016. [4] TSOPANOS S, MINES R A W, MCKOWN S, et al. The influence of processing parameters on the mechanical properties of selectively laser melted stainless steel microlattice structures [J]. Journal of Manufacturing Science and Engineering, 2010, 132(4): 041011. doi: 10.1115/1.4001743 [5] JIN N, WANG F C, WANG Y W, et al. Failure and energy absorption characteristics of four lattice structures under dynamic loading [J]. Materials & Design, 2019, 169: 107655. doi: 10.1016/j.matdes.2019.107655 [6] 郝美荣. 菠萝叶纤维增强点阵圆筒结构的平压性能研究 [D]. 哈尔滨: 东北林业大学, 2017.HAO M R. Compression performance of pineapple leaf fiber reinforced lattice cylinder [D]. Harbin: Northeast Forestry University, 2017. [7] TSANG H H, RAZA S. Impact energy absorption of bio-inspired tubular sections with structural hierarchy [J]. Composite Structures, 2018, 195: 199–210. doi: 10.1016/j.compstruct.2018.04.057 [8] ZOU M, XU S C, WEI C G, et al. A bionic method for the crashworthiness design of thin-walled structures inspired by bamboo [J]. Thin-Walled Structures, 2016, 101: 222–230. doi: 10.1016/j.tws.2015.12.023 [9] LIU Q, MA J B, HE Z H, et al. Energy absorption of bio-inspired multi-cell CFRP and aluminum square tubes [J]. Composites Part B: Engineering, 2017, 121: 134–144. doi: 10.1016/j.compositesb.2017.03.034 [10] TAO Y, LI W G, WEI K, et al. Mechanical properties and energy absorption of 3D printed square hierarchical honeycombs under in-plane axial compression [J]. Composites Part B: Engineering, 2019, 176: 107219. doi: 10.1016/j.compositesb.2019.107219 [11] 魏灿刚. 仿竹结构薄壁管的耐撞性设计和分析 [D]. 长春: 吉林大学, 2014.WEI C G. Crashworthiness bionic design and analysis of thin-walled tube inspired by bamboo structure [D]. Changchun: Jilin University, 2014. [12] HABIB F N, IOVENITTI P, MASOOD S H, et al. Fabrication of polymeric lattice structures for optimum energy absorption using Multi Jet Fusion technology [J]. Materials & Design, 2018, 155: 86–98. doi: 10.1016/j.matdes.2018.05.059 [13] MUELLER J, SHEA K. Stepwise graded struts for maximizing energy absorption in lattices [J]. Extreme Mechanics Letters, 2018, 25: 7–15. doi: 10.1016/j.eml.2018.10.006 -








 下载:
下载:
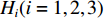

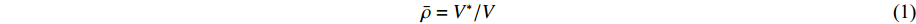
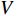
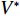

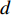
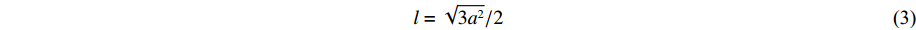
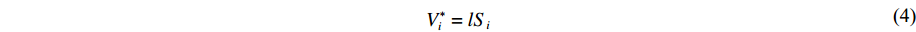
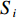

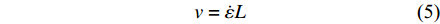
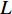
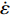
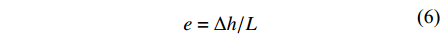
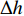



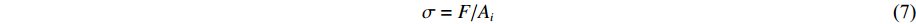
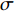

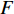

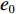
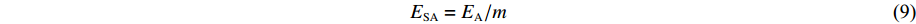


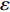




 下载:
下载: