Dispersion and Dissipation Relations of One-Dimensional Viscoelastic Phononic Crystals
-
摘要: 基于运动方程和广义麦克斯韦本构,推导了一维黏弹性局域共振型和布拉格散射型声子晶体的色散与耗散关系。结果表明:对于时间谐波传播问题,这两种声子晶体的色散关系中均不存在禁带,波的衰减完全依赖于黏性耗散和周期性调制,且周期性调制会增强这种耗散作用;相反,对于自由波传播问题,两种声子晶体的色散关系中均存在禁带,但在禁带之外,波的衰减仍需借助于黏性耗散和周期性调制。研究结果对于由高分子材料构成的层状复合材料中的应力波传播研究具有一定的意义。Abstract: Based on equations of motion and the generalized Maxwell constitutive model, this paper derives the dispersion and dissipation relations of one-dimensional viscoelastic local resonance and Bragg scattering type phononic crystals. The results show that, for the time-harmonic propagation, band gap does not exist in the dispersion relation and the attenuation of wave solely relies on viscous dissipation and periodic modulation, which will enhance the dissipation; on the contrary, for the free wave propagation, there is a band gap in the dispersion relation, but beyond the band gap, the attenuation of wave is still dependent on viscous dissipation and periodic modulation. These results are valuable for the study on stress wave propagation in layered composite materials made of polymers.
-
层状复合材料具有诸多有趣和有用的波动特性,因此在相关领域受到普遍重视。在冲击波物理领域,对层状复合材料中的应力波传播特性研究至少有半个多世纪的历史,人们发现通过改变各层材料的属性、厚度、界面结合强度和加载条件等,可以在层状结构中产生十分丰富的应力波现象。例如:Lundergan等[1]研究了应力波在10层环氧树脂和10层不锈钢交替组成的结构中的色散现象;Oved等[2]研究了有机玻璃和钢交替组成的多层结构中的应力波共振现象;Benson等[3]研究了铜/铝、钛/铝多层结构中的应力波异常衰减现象;Zhuang等[4]研究了聚碳酸酯分别与铝、不锈钢及玻璃交替组成的多层结构中的应力波,发现应力波速度低于其组成材料;Franco Navarro等[5]研究了铝/钨层状材料中的应力波传播,发现通过改变加载脉冲的宽度与结构内在特征时间的比值,可以产生3种不同类型的应力波,即振荡型冲击波、一串孤立波、单一孤立波。由于具有对应力波的衰减作用及其他功能(如提升断裂韧性),某些层状复合结构常用作冲击波衰减器和复合装甲[6-8]。
在振动和声学领域,声子晶体[9-10]和声学超材料[10-11]研究于20世纪末21世纪初陆续兴起,层状复合材料属于其中的一维情形。狭义而言,声子晶体和声学超材料有所不同,前者的关键特性之一是由布拉格散射(Bragg scattering,BS)形成频率禁带从而禁止某频率范围的波通过,后者的关键特性是由局域共振(Local resonance,LR)机制产生负密度、负模量、负折射等负参数效应[12-13]。但在文献中,两种名称常常混用,即声子晶体也可以称为声学超材料,而声学超材料如果具有周期性结构也可以称为声子晶体。声子晶体和声学超材料的波动特性使其在减振降噪中具有重要的应用价值。为方便起见,本研究统一使用声子晶体的名称,但区分LR和BS两种类型。
本研究将报道我们在一维黏弹性声子晶体色散与耗散关系研究中的初步进展。黏弹性是层状复合结构中常用的基础材料(即高分子材料)的基本力学属性,但是从现有文献上看,无论在冲击波物理相关领域还是在振动和声学领域,黏弹性对层状复合结构波动特性的影响都没有得到充分的研究。在声子晶体研究中,大多数都不考虑黏弹性,或者仅用十分简化的黏弹性本构进行描述[9-10]。直到近年来才将可真实反映高分子材料黏弹性的广义麦克斯韦本构[14]逐渐应用于声子晶体的相关研究,如利用平面波展开法研究二维黏弹性声子晶体的禁带和耗散特性[15-17]。
从高强度应力波防护到减振降噪,一维黏弹性声子晶体的色散与耗散关系都值得深入研究。正如本研究将展示的:一方面,黏弹性改变了纯弹性声子晶体的波动衰减特性,甚至改变了其禁带的存在性;另一方面,声子晶体的周期性结构也改变了无结构均质黏弹性材料的波动衰减特性(总的来看是促进了衰减)。
具体而言,本研究将首先建立离散形式的一维黏弹性LR和BS型声子晶体模型,其中离散形式作为连续介质的简化近似[18-21],黏弹性由广义麦克斯韦本构模型表示,进而从运动方程出发,推导求解模型的色散与耗散关系。本研究结果有助于进一步推动基于高分子材料的声子晶体研究,也可为冲击波物理相关领域层状复合结构中的应力波研究提供参考。
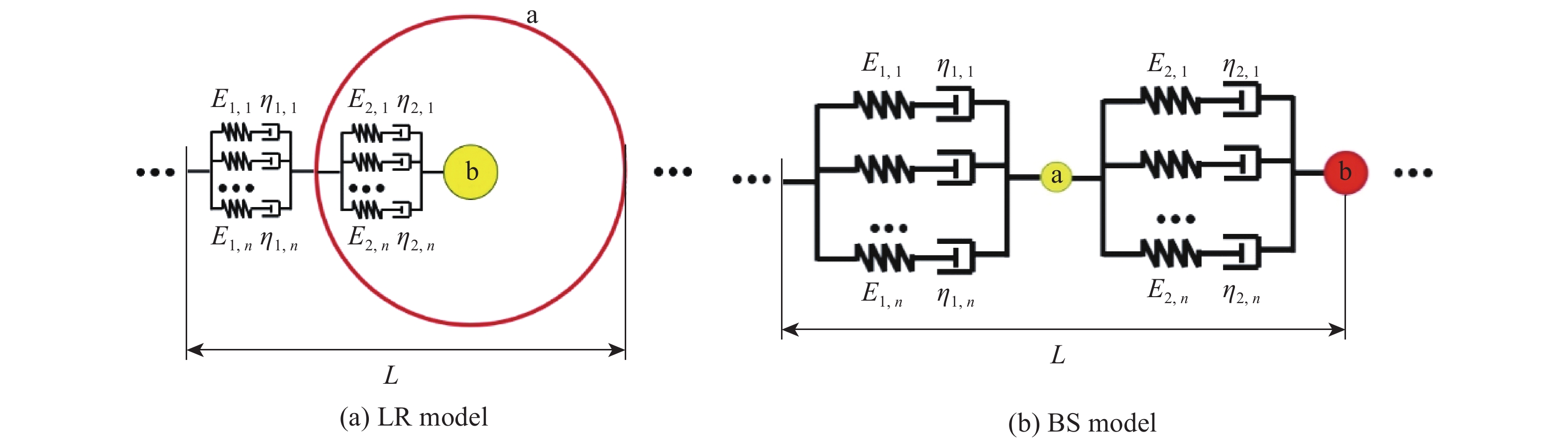
1. 离散形式的一维黏弹性声子晶体模型
黏弹性材料在任意时刻的行为都与其经历的全部历史有关,其应力-应变关系由遗传积分表示[14]
σ(t)=∫t−∞E(t−s)∂ε∂sds (1) 式中:
σ 为应力,ε 为应变,E(t)为模量,s为时间,t为当前时刻。E(t)的具体形式取决于本构模型,对于广义麦克斯韦本构模型[14],E(t)可表示为E(t)=N∑l=1Elexp(−tτl) (2) 式中:El、
η l、τ l分别表示第l个麦克斯韦单元的弹性模量、黏性系数和松弛时间,τ l =η l/El;N为麦克斯韦单元的数目。离散形式的一维黏弹性LR模型和BS模型分别是由图1(a)、图1(b)所示的单元周期性重复连接而成的一维无限长链。在有的文献[10]中,二者分别被称作Mass in Mass Structure(LR型)和Mass and Mass Structure(BS型),这是从它们各自的质量块与连接体的不同排列形式来作的形象区分。从原理上说,BS结构是利用周期变化的材料特性与波的相互作用,而LR结构则是利用单个散射体(振子)与波的相互作用。设模型中单元的长度为L,质量块的质量为ma和mb。各质量块之间链接的模量由式(2)给出。设接触面积为1,则质量块的受力等于应力
σ ,由式(1)和式(2)联立确定。2. 复波数实频率假定下的色散与耗散关系
2.1 LR模型的色散与耗散关系
考虑到模型的周期性,取第n个单元,对其中质量块ma和mb进行受力分析,得到如下两个方程
Fan(t)=2L∫t−∞E1(t−s)(˙uan+1+˙uan−1−2˙uan)ds+2L∫t−∞E2(t−s)(˙ubn−˙uan)ds (3) Fbn(t)=2L∫t−∞E2(t−s)(˙uan−˙ubn)ds (4) 式中:u为相对位移,
˙u 为位移u对时间s的微分。代入牛顿第二定律,得到两个质量块的运动方程
L2ma¨uan=N∑l=1E1,lexp(−t/τ1,l)∫t−∞exp(s/τ1,l)(˙uan+1+˙uan−1−2˙uan)ds+N∑l=1E2,lexp(−t/τ2,l)∫t−∞exp(s/τ2,l)(˙ubn−˙uan)ds (5) L2mb¨ubn=N∑l=1E2,lexp(−t/τ2,l)∫t−∞exp(s/τ2,l)(˙uan−˙ubn)ds (6) 式中:上标a、b区分质量块,下标区分单元。
为了得到色散与耗散关系,选取简谐形式的试探解
uan=Uaexp[i(ωt−knL)] (7) ubn=Ubexp[i(ωt−knL)] (8) 式中:U为振幅,
ω 为频率,k为波数,L为单元长度。将式(7)、式(8)代入式(5)和式(6),得到关于频率ω 与波数k的方程−L2maUaω2=iωUa[exp(−ikL)+exp(ikL)−2]N∑l=1E1,lτ1,l1+iωτ1,l+iω(Ub−Ua)N∑l=1E2,lτ2,l1+iωτ2,l (9) −L2mbUbω2=iω(Ua−Ub)N∑l=1E2,lτ2,l1+iωτ2,l (10) 消去Ua和Ub,最终得到方程
−L2maω2=iω[exp(−ikL)+exp(ikL)−2]N∑l=1E1,lτ1,l1+iωτ1,l+iωL2mbω2N∑l=1E2,lτ2,l1+iωτ2,l−L2mbω2+iωN∑l=1E2,lτ2,l1+iωτ2,l (11) 可以看出,若使式(11)成立,波数k和频率
ω 中需至少有一个为复数。若同为复数,则方程数目(分离式(11)得到的实部和虚部,2个方程)与未知数数目(两个复数的实部和虚部,4个未知数)不匹配,难以求解讨论。故以下仅将波数k视作复数,频率ω 视作实数,或者反过来,将频率ω 视作复数,波数k视作实数。这里先采取第一种假定。令k =
α + iβ ,其中α 称为真实波数,β 是与耗散有关的衰减系数。代入式(11)中,利用欧拉公式,分离方程两边的实部和虚部得到{C1(eβL+e−βL)cos(αL)−D1(eβL−e−βL)sin(αL)=ξD1(eβL+e−βL)cos(αL)+C1(eβL−e−βL)sin(αL)=ψ (12) 式中:
A=L2maω2 ,B=L2mbω2 ,C1(2)=N∑l=1E1(2),lτ1(2),lω1+ω2τ21(2),l ,D1(2)=N∑l=1E1(2),lτ21(2),lω21+ω2τ21(2),l ,ξ=B2C2(D2−B)2+C22+2C1 ,ψ=2D1−A−B(D22+C22−BD2)(D2−B)2+C22 。由方程组(12)可以得到
{sin(αL)=PRxx2−1cos(αL)=QRxx2+1 (13) 式中:
P=C1ψ−D1ξ ,Q=C1ξ+D1ψ ,R=C21+D21 ,x=eβL 。由式(13)得到
x2=Qtan(αL)+PQtan(αL)−P (14) 将式(14)代入式(13)中的第1个方程,得到
4R2sin4(αL)+(Q2+P2−4R2)sin2(αL)−P2=0 (15) 求解这个关于
sin2(αL) 的一元二次方程,得到sin2(αL)=−(Q2+P2−4R2)+√(Q2+P2−4R2)2+16R2P28R2 (16) 这里由于
sin2(αL) 的非负性,舍去了负值解。将式(16)代入式(13)中的第1个方程,得到sinh2(βL)=P24R2sin2(αL) (17) 至此,就得到了在黏弹性LR模型中真实波数
α 与频率ω 的关系,即色散关系式(16),以及衰减系数β 与频率ω 的关系,即耗散关系式(17)。2.2 BS模型的色散与耗散关系
类似地,考虑到模型的周期性,选取第n个单元进行分析,得到单元中质量块ma和mb的运动方程
L2ma¨uan=N∑l=1E2,lexp(−tτ2,l)∫t−∞exp(sτ2,l)(˙ubn−˙uan)ds−N∑l=1E1,lexp(−tτ1,l)∫t−∞exp(sτ1,l)(˙uan−˙ubn−1)ds (18) L2mb¨ubn=N∑l=1E2,lexp(−tτ2,l)∫t−∞exp(sτ2,l)(˙uan−˙ubn)ds+N∑l=1E1,lexp(−tτ1,l)∫t−∞exp(sτ1,l)(˙uan+1−˙ubn)ds (19) 将试探解式(7)和式(8)代入上述运动方程,得到
−L2maUaω2=iω(Ub−Ua)N∑l=1E2,lτ2,l1+iωτ2,l−iω[Ua−Ubexp(ikL)]N∑l=1E1,lτ1,l1+iωτ1,l (20) −L2mbUbω2=iω(Ua−Ub)N∑l=1E2,lτ2,l1+iωτ2,l+iω[Uaexp(−ikL)−Ub]N∑l=1E1,lτ1,l1+iωτ1,l (21) 消去Ua和Ub,最终得到方程
N∑l=1iωE1,lτ1,l1+iωτ1,lN∑l=1iωE2,lτ2,l1+iωτ2,l[exp(ikL)+exp(−ikL)−2]+L2mbω2(N∑l=1iωE1,lτ1,l1+iωτ1,l+N∑l=1iωE2,lτ2,l1+iωτ2,l)=−L2maω2(−L2mbω2+N∑l=1iωE1,lτ1,l1+iωτ1,l+N∑l=1iωE2,lτ2,l1+iωτ2,l) (22) 与式(11)类似,若使式(22)成立,可将波数k视作复数,频率
ω 视作实数,或者反过来,将频率ω 视作复数,波数k视作实数。这里同样先采取第一种假定。令
k=α+iβ ,代入式(22)并分离方程两边的实部和虚部,得到方程组{(C1D2+C2D1)(eβL+e−βL)cos(αL)−(D1D2−C1C2)(eβL−e−βL)sin(αL)=ξ(D1D2−C1C2)(eβL+e−βL)cos(αL)+(C1D2+C2D1)(eβL−e−βL)sin(αL)=ψ (23) 式中:
A=L2maω2 ,B=L2mbω2 ,C1(2)=N∑l=1E1(2),lτ1(2),lω1+ω2τ21(2),l ,D1(2)=N∑l=1E1(2),lτ21(2),lω21+ω2τ21(2),l ,ξ=2(C1D2+C2D1)−(A+B)·(C1+C2) ,ψ=2(D1D2−C1C2)−(A+B)(D1+D2)+AB 。求解得到与式(13)形式相同的结果,区别在于这里P=(C1D2+C2D1)ψ−(D1D2−C1C2)ξ ,Q=(D1D2−C1C2)ψ+(C1D2+C2D1)ξ ,R=(D1D2−C1C2)2+(C1D2+C2D1)2 ,x=eβL 。可以发现,继续求解后,最终得到形式上与式(16)和式(17)完全相同的色散与耗散关系式。2.3 关于不存在禁带的判断
色散关系式(16)的右端显然非负,令其大于1,即
−(Q2+P2−4R2)+√(Q2+P2−4R2)2+16R2P28R2>1 (24) 可推导得到
R2Q2<0 ,这在ω 为实数时显然不成立,因此证得0≤ sin2(α L) ≤ 1。于是可以发现,无论频率
ω 为何值,均满足sin2(α L)有解的条件,换言之,总有真实波数α 与之对应。于是可以确定LR和BS两种模型的色散关系中均不存在禁带。值得注意的是,对于纯弹性LR与BS结构而言,色散关系中是存在禁带的。为了探究上述色散关系在黏弹性退化至纯弹性(松弛时间
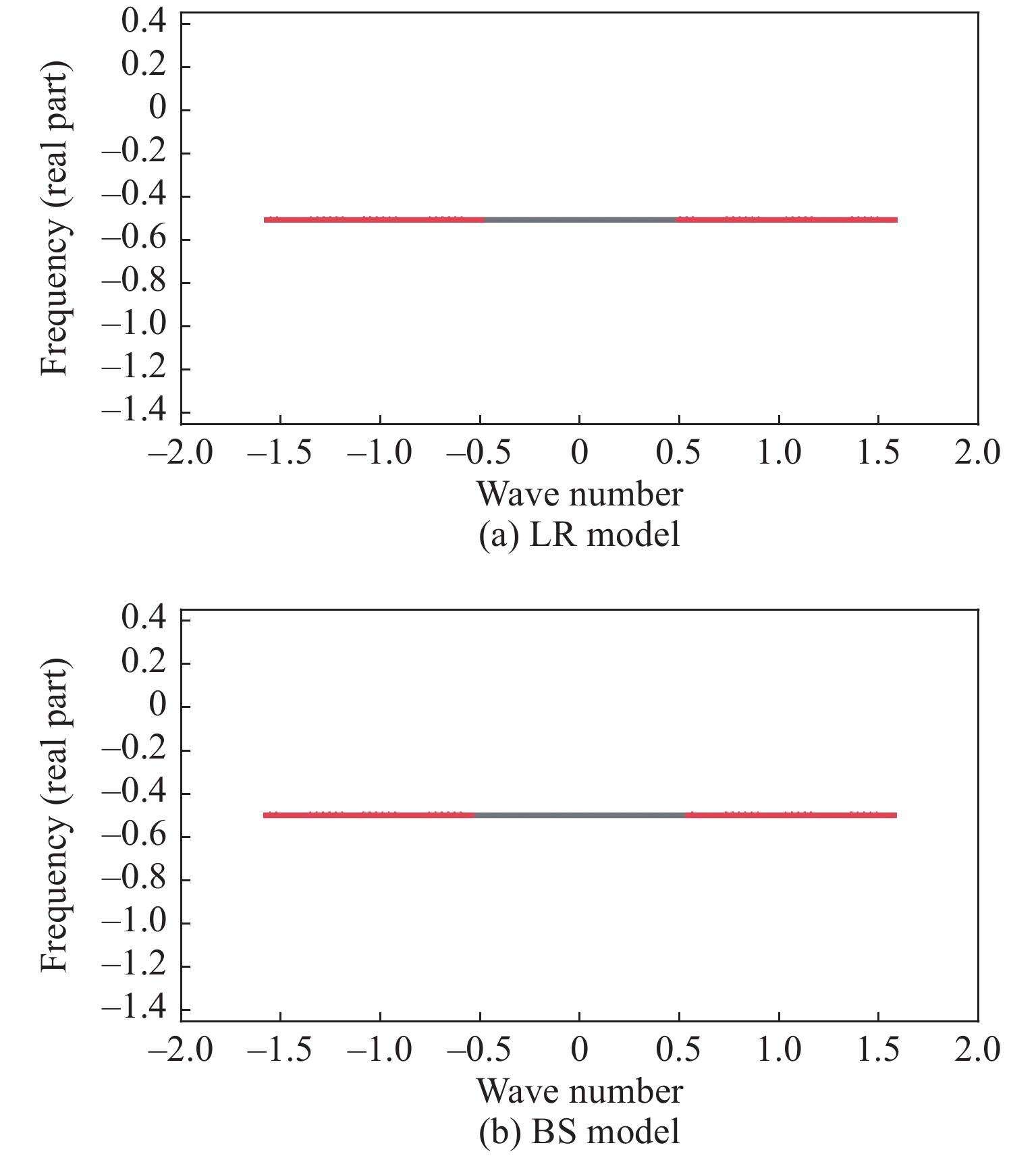
τ 趋于无穷大)时是否存在禁带,绘制了如图2所示的不同松弛时间下的色散关系图,其中:N = 1,ma = 1,mb = 2,L = 2,E1 = E2 = 1。从图2可以看出,对两种结构而言,色散关系中确实不存在禁带,即对每个频率取值都可以找到与之对应的非零真实波数
α ,色散关系难以退化至纯弹性情形下的“禁带”模型。将式(11)和式(22)与弹性情形下LR、BS结构的方程[22-23]对比,可以发现,波数k仅出现在自然指数项中,且形式与弹性情形下的方程一致,而弹性情形下的弹性模量E与黏弹性情形下的“等效模量”Eeff相对应。Eeff=N∑l=1iωElτl1+iωτl (25) 当松弛时间
τ 趋于无穷大时,有Eeff=E ,意味着式(11)和式(22)在τ 趋于无穷大时可以退化至纯弹性情形。但是,若将k视作复数,原先的方程被分离成实部和虚部两个方程进行后续的求解,就破坏了方程的形式,而造成复数波数假定下的色散关系无法退化至纯弹性情形。2.4 周期性调制的衰减作用
由于在复数波数实数频率假定下,上述BS和LR模型的色散关系中不存在禁带,所以波的衰减将完全由黏性耗散和周期性调制引起。如果将单原子链视作均匀材料的离散化,那么将质量块交替排列的BS模型与之对比,可以看出由质量差异排列导致的周期性调制所带来的影响。
由耗散关系式(17)可以得到
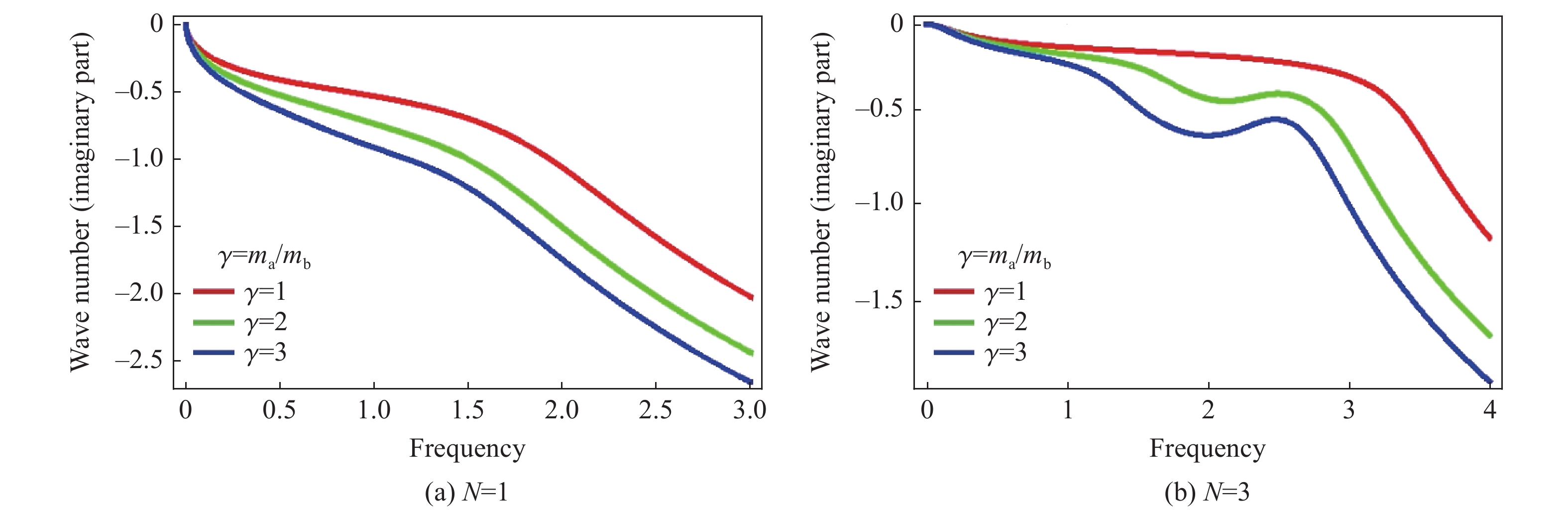
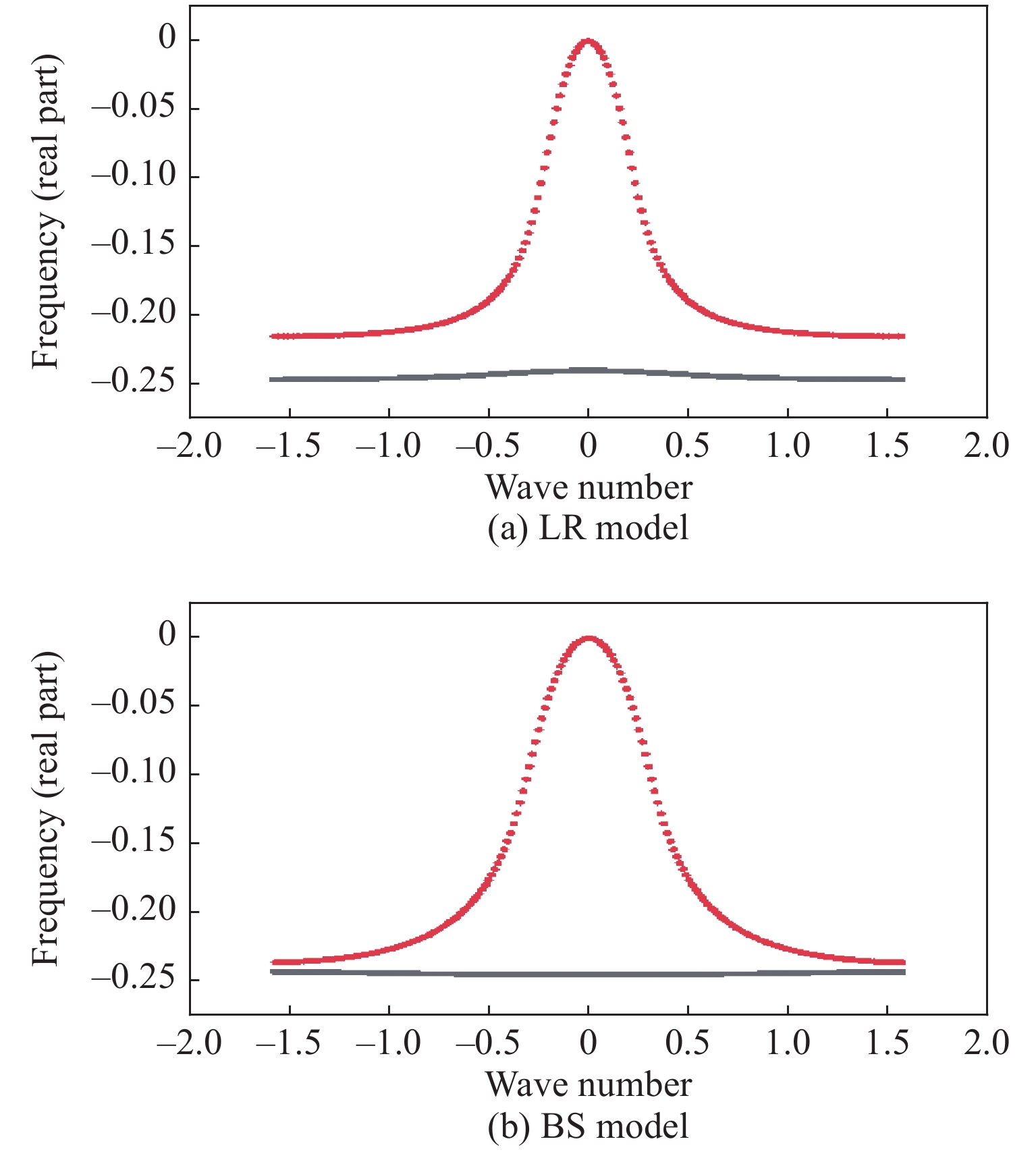
e2βL=2R2sin2(αL)+P2−√4R2P2sin2(αL)+P42R2sin2(αL) (26) 将式(16)代入,绘制出耗散曲线(
β -ω 关系),如图3所示。各项参数为:E1 = E2 = 1,τ 1 =τ 2 = 1,L = 2。可以看出,质量比越大,耗散系数(其绝对值)越大,耗散越明显,因此可以认为,虽然此时周期性结构中没有禁带,但周期性调制增强了对波的衰减作用。类似地,可以得到LR结构的耗散曲线,如图4所示。图4中也反映了与BS结构的对比情况。可以看出,LR结构产生的耗散作用只在某一频率区间内大于BS结构。比较不同质量比下的耗散曲线,可以看出,质量比越大,耗散作用越明显,这一点与BS模型一致。
综上,虽然不存在禁带,但黏性产生的耗散作用仍会导致波的衰减,且对于两种模型而言,周期性质量差异的调制会增强这种耗散作用。
3. 复频率实波数假定下的色散与耗散关系
下面采取复频率实波数假定。选取如下试探解形式
ua,b=Ua,bexp(λt−inkL) (27) 式中:波数k为实数;令
λ =α + iβ ,其中β 为真实频率,α 是与耗散有关的衰减系数。3.1 LR模型的色散与耗散关系
将式(27)代入运动方程式(5)和式(6)中,得到
L2maUaλ2=λUa[exp(−ikL)+exp(ikL)−2]N∑l=1E1,lτ1,l1+λτ1,l+λ(Ub−Ua)N∑l=1E2,lτ2,l1+λτ2,l (28) L2mbUbλ2=λ(Ua−Ub)N∑l=1E2,lτ2,l1+λτ2,l (29) 消去Ua和Ub,得到
L2maλ2=2λ[cos(kL)−1]N∑l=1E1,lτ1,l1+λτ1,l+λ−L2mbλ2N∑l=1E2,lτ2,l1+λτ2,lL2mbλ2+λN∑l=1E2,lτ2,l1+λτ2,l (30) 求得
λ 后分离实部和虚部,虚部β 关于k的表达式便是色散关系式,实部α 关于k的表达式是耗散关系式。这是一个关于λ 的高次方程。阿贝尔曾证明,高于四次的一般方程没有求根公式,因此只能得到N = 1时λ 的解析解,而对于N > 1的情形,由于λ 的次幂大于4,故无法得到解析解。但原则上可以通过数值计算方式得到给定k情形下λ 的解,从而绘制出色散图和耗散图。3.2 BS模型的色散与耗散关系
将试探解式(27)代入运动方程式(18)和式(19)中,得到
L2maUaλ2=λ(Ub−Ua)N∑l=1E2,lτ2,l1+λτ2,l−λ[Ua−Ubexp(ikL)]N∑l=1E1,lτ1,l1+λτ1,l (31) L2mbUbλ2=λ(Ua−Ub)N∑l=1E2,lτ2,l1+λτ2,l+λ[Uaexp(−ikL)−Ub]N∑l=1E1,lτ1,l1+λτ1,l (32) 消去Ua和Ub,得到
2N∑l=1λE1,lτ1,l1+λτ1,lN∑l=1λE2,lτ2,l1+λτ2,l[cos(kL)−1]−L2mbλ2(N∑l=1λE1,lτ1,l1+λτ1,l+N∑l=1λE2,lτ2,l1+λτ2,l)=L2maλ2(L2mbλ2+N∑l=1λE1,lτ1,l1+λτ1,l+N∑l=1λE2,lτ2,l1+λτ2,l) (33) 求得
λ 后分离实部和虚部,得到色散与耗散关系式。同样地,这个关于λ 的方程仅在N = 1时存在解析解,当N > 1时由于λ 的次幂大于4而无法得到解析解。但原则上依然可以通过数值计算方式求得某一给定k情形下的λ ,即可画出色散图和耗散图。3.3 N = 1和N = 3时色散和耗散关系的进一步讨论
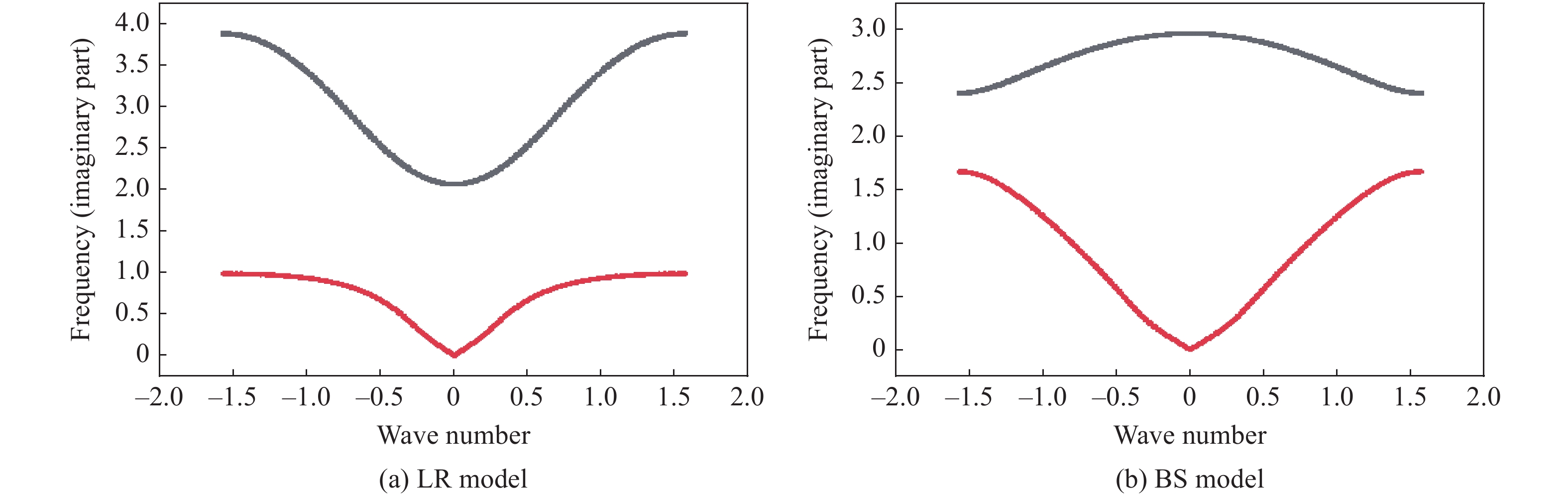
为了进一步探究色散与耗散关系,本研究具体探讨N = 1和N = 3两种情形。前者对应方程有解析解,即可以得到色散和耗散关系的解析表达式;后者则需要通过数值求解得到散点图。以下情形均舍去了频率虚部为零的解,即不考虑真实频率为零的情况。
在N = 1的情形下,为了简化式(30)和式(33)且不失一般性,可令ma = 1,mb = 2,E1 = 1,E2 = 2,
τ 1 =τ 2 = 1,L = 2。对于LR模型,代入式(30),整理得到
λ4+2λ3+[6−2cos(2k)]λ2+[5−2cos(2k)]λ−2[cos(2k)−1]=0 (34) 求解这个四次方程,得到4个解析解
{λ1,2=12(−1±√−9+4cos(2k)−2√19−10cos(2k))λ3,4=12(−1±√−9+4cos(2k)+2√19−10cos(2k)) (35) 分离这4个解的虚部,在
k∈[−π/L,π/L] 区间画出色散曲线,如图5(a)所示。对于BS模型,式(33)可以化简为
2λ4+4λ3+11λ2+9λ−4[cos(2k)−1]=0 (36) 求解这个四次方程,得到4个解析解
{λ1,2=12(−1±√−8−√49+32cos(2k))λ3,4=12(−1±√−8+√49+32cos(2k)) (37) 分离这4个解的虚部,在
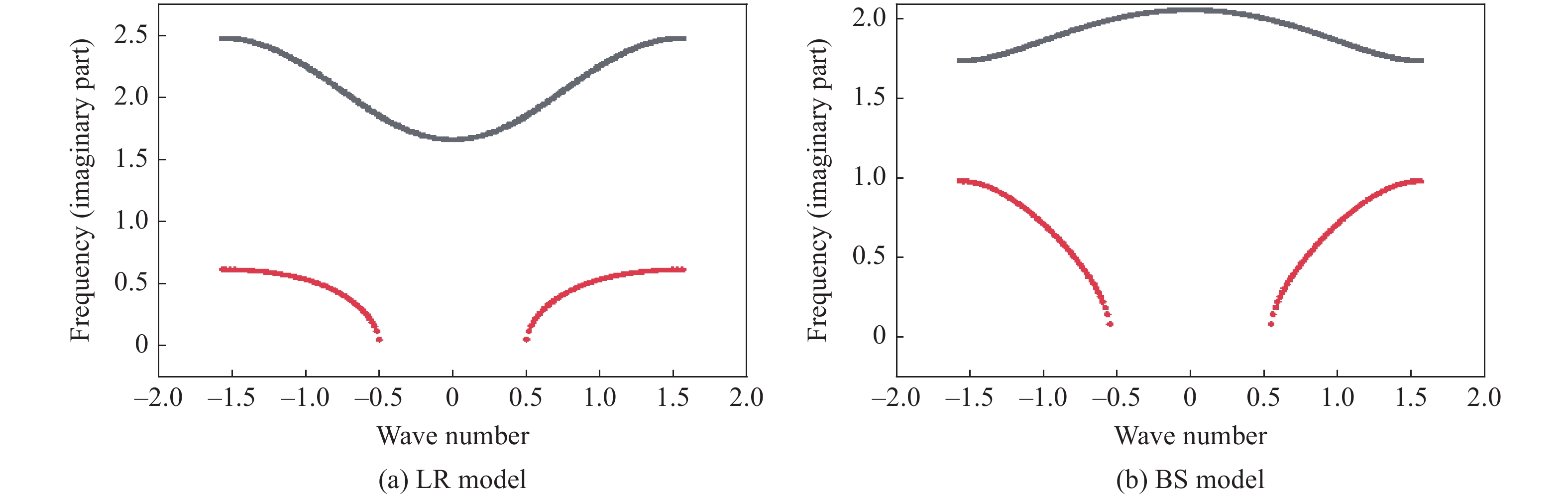
k∈[−π/L,π/L] 区间画出色散曲线,如图5(b)所示。可以看出,这种耗散由时间的系数体现,即振幅随时间衰减的情形下,两种模型是存在禁带的,在禁带范围内,对真实频率
β 而言没有与之对应的波数k。为了探究当
λ 的方程为更高次时是否依然存在禁带,研究N = 3的情形。如前所述,此时的方程由于幂次大于4而没有解析解,只能通过数值计算方式得到某一给定波数k对应方程的数值解,再分离出虚部β 。当给定的k足够多时,可以得到足够多的数据组(k,β ),从而得到色散关系图。对于LR模型,为了简化方程,不妨假定连接两质量块ma和mb的两个广义麦克斯韦模型相同,令ma = 1,mb = 2,E1,1 = E2,1 = 1,E1,2 = E2,2 = 1,E1,3 = E2,3 = 1,
τ 1,1 =τ 2,1→∞,τ 1,2 =τ 2,2 = 1,τ 1,3 =τ 2,3 = 2,L = 2。此时的模量为E1(t)=E2(t)=3∑l=1E1,lexp(−tτ1,l)=1+exp(−t)+exp(−t2) (38) 将上述参数代入式(30)并化简,得到
(2λ4+3λ3+λ2)(4λ4+6λ3+20λ2+18λ+3)=2[cos(2k)−1](6λ2+6λ+1)(4λ4+6λ3+8λ2+6λ+1) (39) 这是关于
λ 的八次方程。首先在[−π/L,π/L] 区间内取多个实数k,数值求解得到各自对应的方程的解,分离出虚部β ,得到的色散关系如图6(a)所示。对BS模型作相同的假定,将参数代入式(33)并化简,得到
λ4(1+λ)2(1+2λ)2=[cos(2k)−1](6λ2+6λ+1)2−3λ2(1+λ)(1+2λ)(6λ2+6λ+1) (40) 得到的色散关系如图6(b)所示。
从图6可以看到,当N = 3时禁带依然存在。尽管无法穷举或严格证明,我们似乎可以谨慎地推断N取任意值时都存在禁带(实际使用广义麦克斯韦模型时,N一般不超过20)。
值得注意的是,这里的色散关系与纯弹性情形类似[22-23],这是因为将i
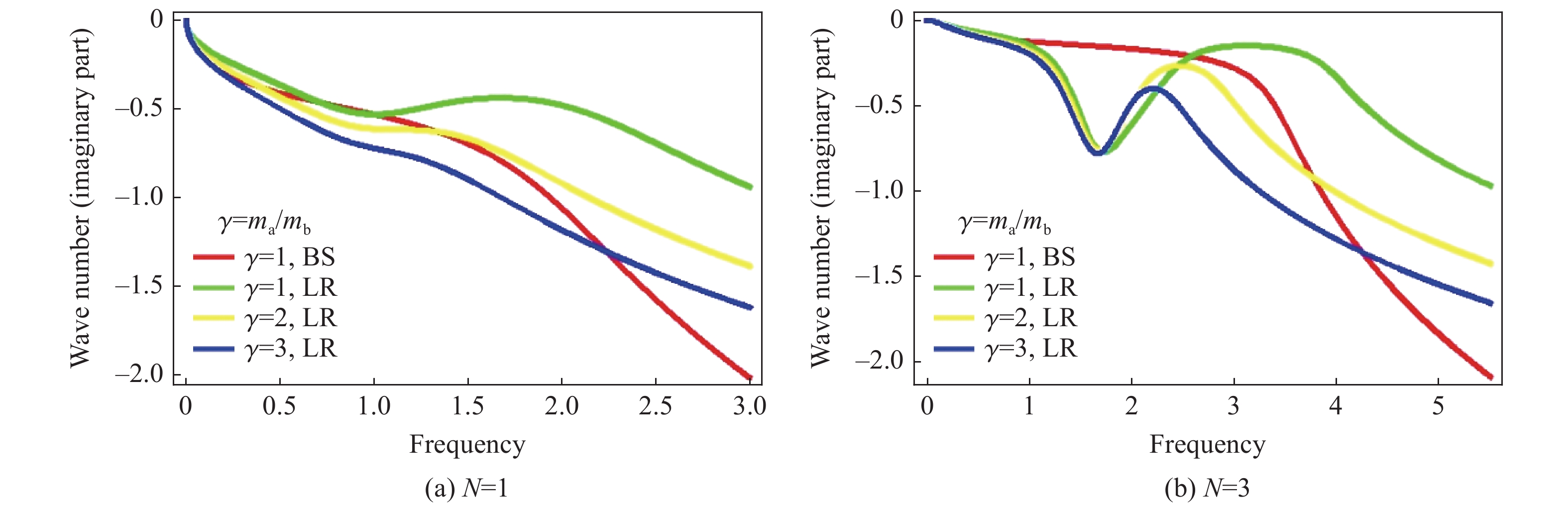
ω 视作一个复数λ ,式(11)和式(22)成为关于λ 的高次方程,即式(30)和式(33)。与复数波数假定不同,这里不需要分离实虚部求解,原方程的形式得到了保留,且这个高次方程在松弛时间趋于无穷大时会退化为关于λ 的四次方程,其形式与弹性情形下关于ω 的四次方程一致。因此也可以预见,复数频率假定下的色散关系可以退化至弹性情形。对式(34)、式(36)、式(39)、式(40)分离出解的实部,便得到耗散关系,如图7和图8所示。可以看出,N对耗散曲线形状的影响较大。当N相同时,LR和BS两种结构的耗散曲线相似,说明耗散作用受到结构的影响不大,应主要由黏性引起。
4. 结 论
研究了一维广义麦克斯韦黏弹性情形下LR和BS型声子晶体的色散与耗散关系。在求解运动方程时,采用了波数为复数、频率为实数,以及频率为复数、波数为实数的两种不同假定,两种假定给出了禁带存在与否的相反结果。
对于复波数假定,推导所得的LR和BS型声子晶体的色散关系在形式上一致,都不存在禁带,两者的耗散关系在形式上也是一致的。对于复频率假定,LR和BS型声子晶体均得到关于时间系数
λ 的一个高次方程,求解该方程并分离虚部和实部,得到色散和耗散关系,此时色散关系存在禁带,且二者的耗散关系类似。鉴于复波数假定时黏性造成波幅随空间衰减,该假定适合于时间谐波传播问题[24-25];复频率假定时,黏性造成波幅随时间衰减,该假定适合于自由波传播问题[24-25]。因此可以确定:在广义麦克斯韦黏弹性情形下,对于LR和BS两种声子晶体中的时间谐波传播问题,禁带不能发挥作用,波的衰减完全依赖于黏性耗散和周期性调制,且周期性调制会增强耗散作用;相反,对于这两种声子晶体中的自由波传播问题,禁带能够发挥作用,但在禁带之外,波动的衰减仍需借助于黏性耗散和周期性调制。
本研究不失普遍性的定性认识对于减振降噪和冲击波防护研究具有一定的参考价值。在实际应用中,还需进一步研究连续介质形式的一维声子晶体(或层状复合结构),使用真实材料参数,针对波动或振动能谱中能量集中的频率范围,优化设计结构,以期最大程度地发挥禁带、黏性耗散和周期性调制对波动或振动衰减的作用。
-
[1] LUNDERGAN C D, DRUMHELLER D S. Propagation of stress waves in a laminated plate composite [J]. Journal of Applied Physics, 1971, 42(2): 669–675. doi: 10.1063/1.1660078 [2] OVED Y, LUTTWAK G E, ROSENBERG Z. Shock wave propagation in layered composites [J]. Journal of Composite Materials, 1978, 12(1): 84–96. doi: 10.1177/002199837801200107 [3] BENSON D J, NESTERENKO V F. Anomalous decay of shock impulses in laminated composites [J]. Journal of Applied Physics, 2001, 89(7): 3622–3626. doi: 10.1063/1.1329146 [4] ZHUANG S M, RAVICHANDRAN G, GRADY D E. An experimental investigation of shock wave propagation in periodically layered composites [J]. Journal of the Mechanics and Physics of Solids, 2003, 51(2): 245–265. doi: 10.1016/S0022-5096(02)00100-X [5] FRANCO NAVARRO P, BENSON D J, NESTERENKO V F. Nature of short, high-amplitude compressive stress pulses in a periodic dissipative laminate [J]. Physical Review E, 2015, 92(6): 062917. doi: 10.1103/PhysRevE.92.062917 [6] YADAV S, RAVICHANDRAN G. Penetration resistance of laminated ceramic/polymer structures [J]. International Journal of Impact Engineering, 2003, 28(5): 557–574. doi: 10.1016/S0734-743X(02)00122-7 [7] GRUJICIC M, BELL W C, PANDURANGAN B. Design and material selection guidelines and strategies for transparent armor systems [J]. Materials & Design, 2012, 34: 808–819. doi: 10.1016/j.matdes.2011.07.007 [8] MINES R A W. A one-dimensional stress wave analysis of a lightweight composite armour [J]. Composite Structures, 2004, 64(1): 55–62. doi: 10.1016/S0263-8223(03)00213-7 [9] HUSSEIN M I, FRAZIER M J. Band structure of phononic crystals with general damping [J]. Journal of Applied Physics, 2010, 108(9): 093506. doi: 10.1063/1.3498806 [10] HUSSEIN M I, FRAZIER M J. Metadamping: an emergent phenomenon in dissipative metamaterials [J]. Journal of Sound and Vibration, 2013, 332(20): 4767–4774. doi: 10.1016/j.jsv.2013.04.041 [11] LI J, CHAN C T. Double-negative acoustic metamaterial [J]. Physical Review E, 2004, 70(5): 055602. doi: 10.1103/PhysRevE.70.055602 [12] LIU Z Y, ZHANG X X, MAO Y W, et al. Locally resonant sonic materials [J]. Science, 2000, 289(5485): 1734–1736. doi: 10.1126/science.289.5485.1734 [13] DING Y Q, LIU Z Y, QIU C Y, et al. Metamaterial with simultaneously negative bulk modulus and mass density [J]. Physical Review Letters, 2007, 99(9): 093904. doi: 10.1103/PhysRevLett.99.093904 [14] 王礼立. 应力波基础[M]. 2版. 北京: 国防工业出版社, 2005.WANG L L. Foundation of stress waves [M]. 2nd ed. Beijing: National Defense Industry Press, 2005. [15] ZHU X Y, ZHONG S, ZHAO H D. Band gap structures for viscoelastic phononic crystals based on numerical and experimental investigation [J]. Applied Acoustics, 2016, 106: 93–104. doi: 10.1016/j.apacoust.2016.01.007 [16] LEWIŃSKA M A, KOUZNETSOVA V G, VAN DOMMELEN J A W, et al. The attenuation performance of locally resonant acoustic metamaterials based on generalised viscoelastic modelling [J]. International Journal of Solids and Structures, 2017, 126/127: 163–174. doi: 10.1016/j.ijsolstr.2017.08.003 [17] GUO F X, GUO H, SUN P, et al. Study on band gap properties of two-dimensional phononic crystals based on generalized viscoelastic modeling [J]. Modern Physics Letters B, 2019, 33(32): 1950403. doi: 10.1142/S0217984919504037 [18] YAO S S, ZHOU X M, HU G K. Experimental study on negative effective mass in a 1D mass-spring system [J]. New Journal of Physics, 2008, 10(4): 043020. doi: 10.1088/1367-2630/10/4/043020 [19] TAN K T, HUANG H H, SUN C T. Optimizing the band gap of effective mass negativity in acoustic metamaterials [J]. Applied Physics Letters, 2012, 101(24): 241902. doi: 10.1063/1.4770370 [20] MEAD D J. A general theory of harmonic wave propagation in linear periodic systems with multiple coupling [J]. Journal of Sound and Vibration, 1973, 27(2): 235–260. doi: 10.1016/0022-460X(73)90064-3 [21] LAZAROV B S, THOMSEN J J. Using high-frequency vibrations and non-linear inclusions to create metamaterials with adjustable effective properties [J]. International Journal of Non-Linear Mechanics, 2009, 44(1): 90–97. doi: 10.1016/j.ijnonlinmec.2008.09.001 [22] HUANG H H, SUN C T, HUANG G L. On the negative effective mass density in acoustic metamaterials [J]. International Journal of Engineering Science, 2009, 47(4): 610–617. doi: 10.1016/j.ijengsci.2008.12.007 [23] 阎守胜. 固体物理基础[M]. 北京: 北京大学出版社, 2000.YAN S S. Fundamentals of solid state physics [M]. Beijing: Peking University Press, 2000. [24] HUSSEIN M I, LEAMY M J, RUZZENE M. Dynamics of phononic materials and structures: historical origins, recent progress, and future outlook [J]. Applied Mechanics Reviews, 2014, 66(4): 040802. doi: 10.1115/1.4026911 [25] FRAZIER M J, HUSSEIN M I. Viscous-to-viscoelastic transition in phononic crystal and metamaterial band structures [J]. The Journal of the Acoustical Society of America, 2015, 138(5): 3169–3180. doi: 10.1121/1.4934845 -








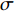
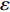
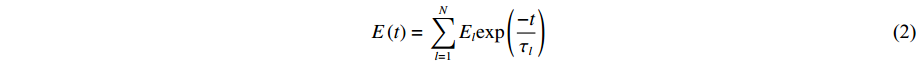
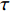
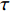
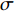

 下载:
下载:
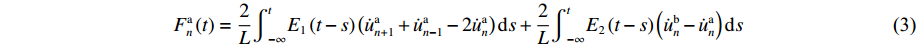
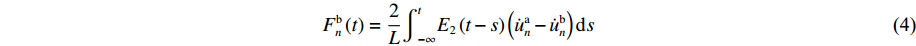
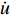
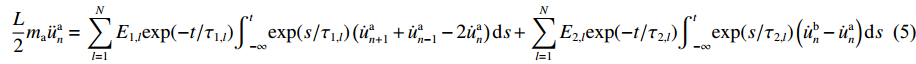

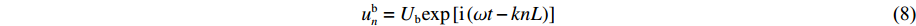
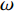
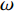
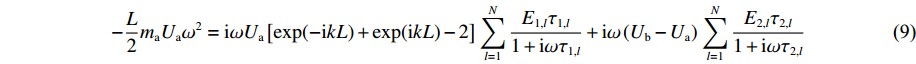
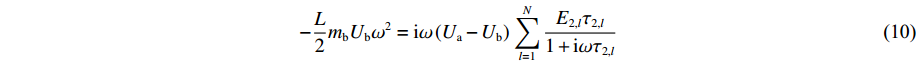
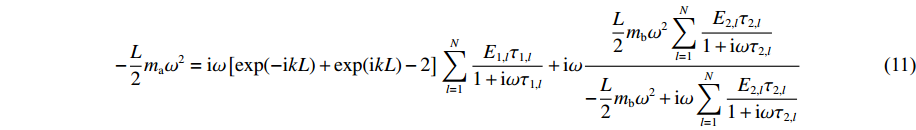
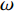
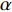

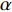
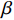
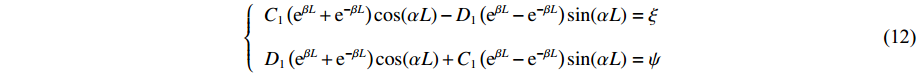
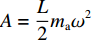
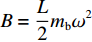

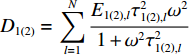
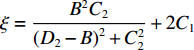
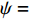
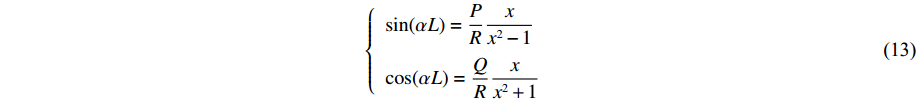
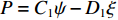
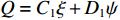
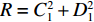
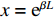
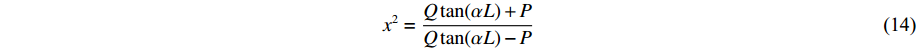
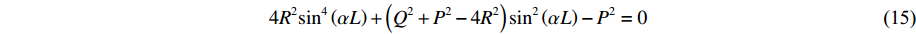
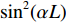
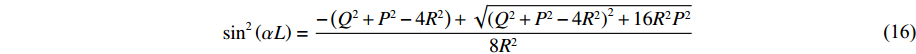

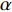
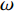
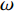
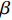
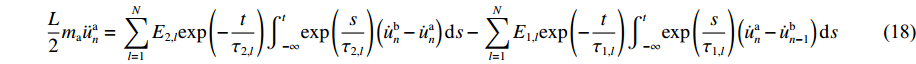
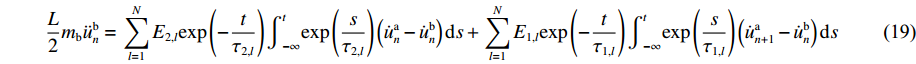
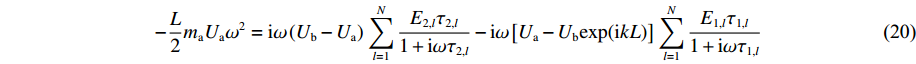
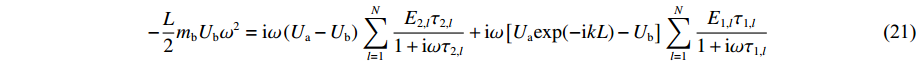
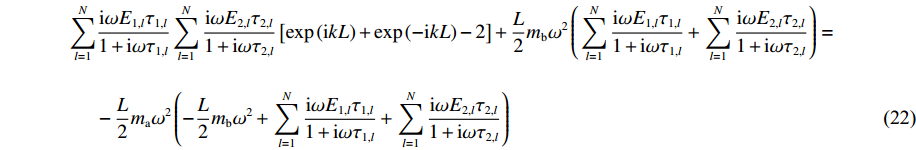

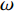

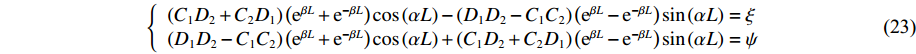
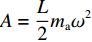


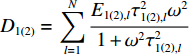

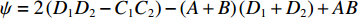
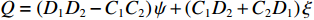
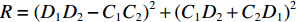

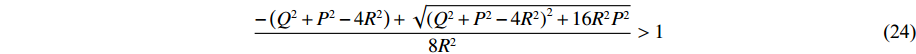

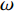
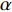
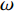
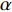

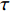



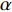
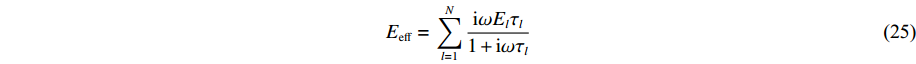
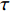
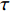
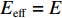

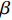
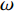
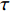
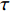


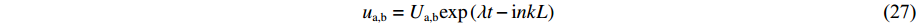
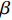
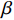
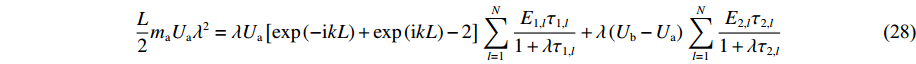
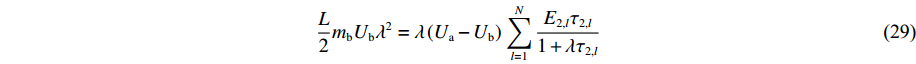
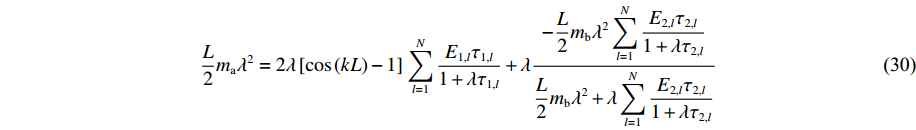

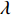
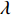
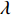

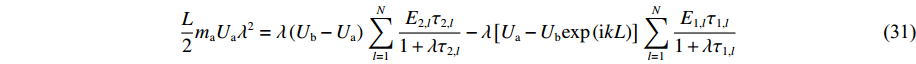

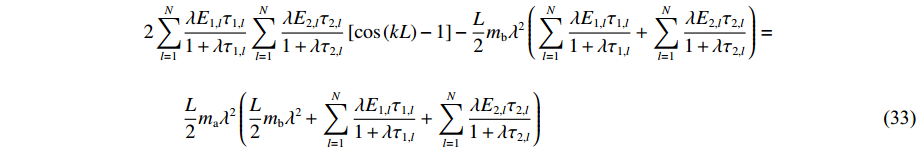
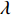

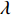
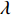
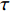
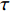
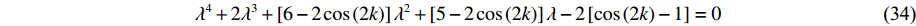

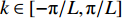

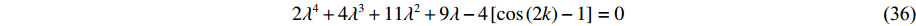
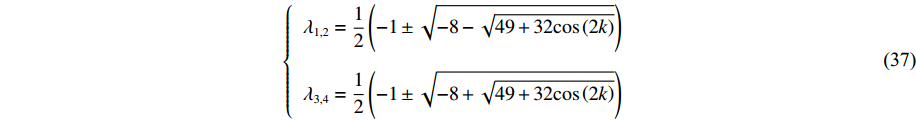
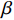
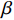
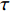
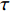
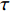
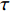
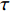
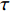
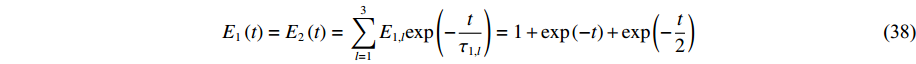
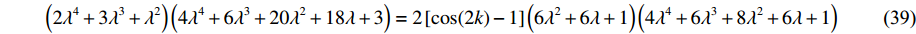
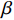

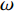
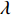
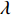



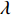

 下载:
下载: