Damage Boundary of Crystal Oscillator under Shock Environment
-
摘要: 贴片式石英晶体振荡器广泛应用于各类电子和通信设备系统中。针对晶振在冲击环境中容易出现结构破坏而导致系统工作异常的问题,通过分析单自由度系统在不同频率冲击载荷作用下的响应特点,建立了结构的应力响应水平与相关冲击响应谱谱值之间的联系,获得了较已有结论更合理的损伤边界形式。根据典型晶振结构易损组件的力学特性建立对应的简化分析模型,得到了贴片晶振在大频率范围内的结构损伤边界。利用有限元仿真软件,对晶振结构在0.5~30 kHz频率范围内冲击载荷下的响应进行仿真分析,验证了该损伤边界的有效性。这也为以贴片晶振为代表的微小元器件在冲击环境下的可靠性研究提供了一种可行的方法。Abstract: The surface mounted devices (SMD) crystal oscillator is widely used in various electrical and communication equipment or systems. The crystal oscillator is prone to structural damage under shock environment, which may results in abnormal operation of the system. The relationship between the level of structural stress response and the value of related shock response spectrum (SRS) is established and a more reasonable damage boundary form is obtained by analyzing the response characteristics of the single-degree-of-freedom (SDOF) system under shock loads with different frequencies. Based on the mechanical characteristics of the vulnerable component of a typical crystal oscillator, the corresponding simplified analytical model is established, and its structural damage boundary in a large frequency range is obtained. The finite element simulation software is used to simulate the response of crystal oscillator structure under shock loads within the frequency range of 0.5–30 kHz to verify the effectiveness of the structural damage boundary. This paper also provides a feasible method for the reliability study of various micro-components represented by SMD crystal oscillator under shock environment.
-
作为各种电子装备中最典型的机电耦合器件之一,石英晶体振荡器是设备中不可或缺的高稳定频率源,是整个电子系统的关键元件,广泛地应用于各种导航、通信、测量等仪器设备中。随着科技发展与需求的增长,工业部门要求晶体振荡器(晶振)在更严苛的环境中也能够稳定可靠地工作,而晶振因其结构与材料的特点恰恰对振动冲击环境极其敏感[1-3],过量的冲击振动会引起晶振输出频率偏移,甚至导致组件发生物理破坏而失效[4-5]。在一些航空航天装备中,由各种因素导致的冲击环境是很难避免的。如Moening[6]统计分析了1963~1985年间因振动和冲击导致的航天飞行故障案例,发现由火工品爆炸冲击引起的运载火箭飞行失效比例非常高,在88起故障案例中有41起由此引起,并且其中的70%最终造成巨大损失。虽然航天器上火工品的爆炸冲击一般不会引起主结构的变形或损坏,但是对晶体、陶瓷、玻璃外壳等脆性材料而言却足够严酷,有可能导致其结构碎裂而失效。
相关研究表明,在高加速度冲击下,由于芯片内部材料与可动组件的脆弱性[7]、缓冲材料性能不足[8]等因素,一些电子元件很容易发生结构损伤而失效。为保证这类元件在工作中的安全,人们一方面研究元件在冲击环境下的响应特性与恰当的隔振缓冲装置[9-10],减弱冲击环境对元件的作用;另一方面总结冲击环境测试评估方法与规范[11-12],通过冲击环境测试确认元件的可靠性。为了评估冲击环境的严酷程度,Gaberson等[13-14]指出伪速度冲击响应谱较加速度谱更有优势,并利用半正弦载荷模拟了多种类型爆炸冲击环境。Irvine[15]总结了电子器件在冲击环境下的失效理论和试验研究结果,给出了多种结构下材料的力学常数与失效阈值。Li等[16]根据单自由度系统的响应特点,分析了结构的损伤边界形式。上述研究为电子产品在冲击环境下的可靠性评估提供了一种可行的途径。本研究借鉴单自由度系统研究思路,改进文献[16]中损伤边界在低频段的临界参数选取方式,结合贴片晶振的典型结构,分析其易损组件的结构特点,通过施加与实际冲击信号更接近的正弦衰减信号来研究结构的动力学响应,以获得各频率的临界载荷与临界冲击谱,通过真实测得的冲击载荷验证改进后损伤边界的有效性。
1. 贴片晶振在冲击环境下的失效模式
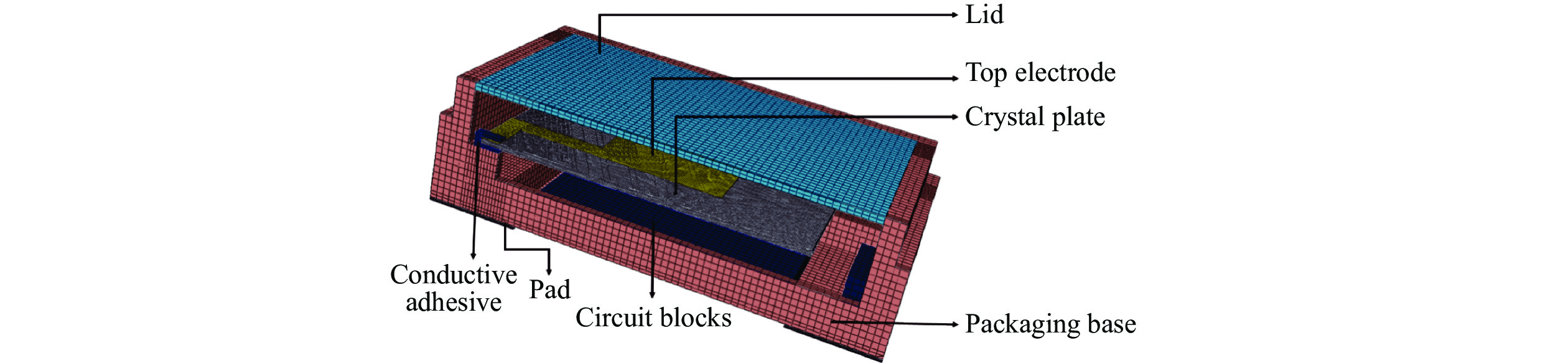
贴片晶振是利用石英晶体的压电效应制成的一种电子器件,可为系统提供高稳定的频率源。它主要由石英晶片、基座、上盖板、导电胶、电极镀层以及内部电路构成,如图1所示。其中,石英晶片是一片按一定方位角从石英晶体上切下的薄片,在晶片的两面涂敷电极,通过导电胶固定在基座上,是晶振的核心组件。
在冲击环境下,电子器件的失效一般可分为结构失效和性能失效,其中结构失效又可根据失效机理分为材料破坏(材料的应力、应变超出其容许极限)和大位移失效(如大位移导致各组件间设计外的接触、碰撞等)。如图2所示,晶振在受到加速度冲击作用时,内部依靠导电胶支承的晶片可能会由于端部应力过大而发生断裂[4-5],从而导致晶振乃至整个系统的不可逆性失效。这也是晶振在冲击环境中经常发生的损伤模式。另外,在极端温度条件下,冲击载荷会导致导电胶破坏或脱胶,此种情况不在本研究讨论范围。
2. 基于冲击响应谱的结构损伤边界
2.1 冲击响应谱中的结构应力损伤准则
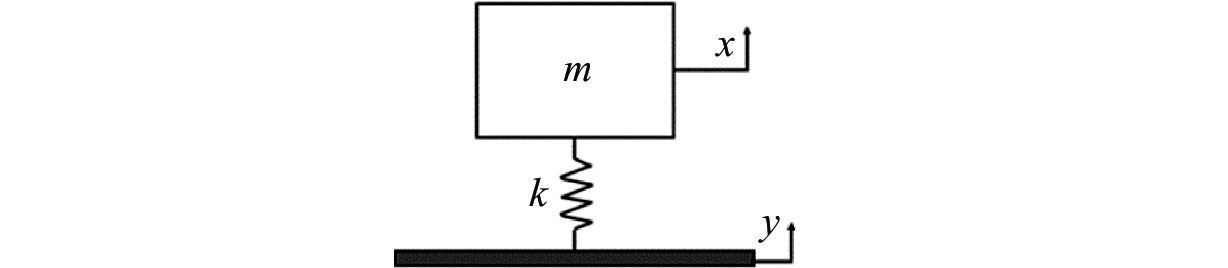
在考核器件和设备在冲击环境下的可靠性时,一般用冲击响应谱[17]表征环境的严酷度。它用载荷作用在一系列不同频率单自由度系统上的效果,即结构对冲击载荷的响应来描述冲击环境。当一个单自由度质量弹簧系统受到给定冲击激励时,其响应峰值为其固有频率的函数。由此函数绘成的图形即为冲击响应谱。按照所选用的单自由度系统响应参数,冲击响应谱可分为绝对加速度谱、伪速度谱、相对位移谱等。
对于如图3所示的无阻尼单自由度系统,受到基础加速度激励
¨y(t) 时,若记z=x−y 为振子与基础的相对位移,ω=√k/m 为系统无阻尼固有圆频率(k、m分别为无阻尼单自由度系统的刚度与质量),则其运动方程可写为¨z+ω2z=−¨y (1) 解得振子的相对位移、相对速度、绝对加速度分别为
z(t)=−1ω∫t0¨y(τ)sinω(t−τ)dτ (2) ˙z(t)=−∫t0¨y(τ)cosω(t−τ)dτ (3) ¨x(t)=ω∫t0¨y(τ)sinω(t−τ)dτ (4) 对于一系列这样的单自由度系统,所得的绝对加速度谱(a)、伪速度谱(vp)与相对位移谱(dr)与其所选取的响应参数分别为
{a(f)=max(|¨x(t)|)vp(f)=max(|ωz(t)|)dr(f)=max(|z(t)|) (5) 式中:
f=ω/2π 为单自由度系统的固有频率。它们之间有以下关系a(f)2πf=vp(f)=2πfdr(f) (6) 根据结构的应力损伤准则,当结构某处的材料应力大于其临界应力时,可以认为结构发生损坏,无法再满足正常工作需求。考虑如图3所示的单自由度系统。Li等[16]对不同频率载荷作用下结构的应力响应特点进行了分析。当冲击载荷频率显著低于结构频率时,相当于考察一个质量块通过刚度很大的弹簧连接件施加加速度激励时的响应。这种情况下质量块的运动与激励几乎一致,作用于质量块的力主要由质量块运动导致的惯性力产生。由于惯性力与质量块的加速度一一对应,且质量块的最大加速度与激励载荷的最大加速度近似,因而作用于质量块的力的大小可以用式(4)中的绝对加速度表征,在冲击响应谱上表现为绝对加速度谱的谱值大小。结构中的最大应力(
σmax )为σmax=mS¨xmax=mSa (7) 式中:S为弹簧连接件的连接面积。当冲击载荷频率显著高于结构频率时,相当于考察一个大质量块通过刚度很小的弹簧连接件施加加速度激励时的响应。这种情况下质量块对激励的响应很小,而弹簧连接件的变形较大,作用于质量块的力主要由弹簧连接件变形导致的弹力引起。弹力的大小可以用式(2)中的相对位移表征,在冲击响应谱上表现为相对位移谱的谱值大小。结构中的最大应力为
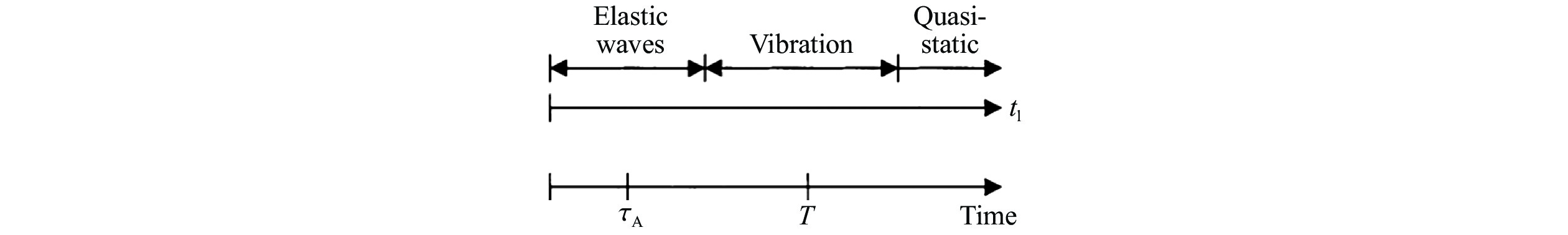
σmax=kSzmax=kSdr (8) 另一方面,在实际的微小结构中,冲击下的响应特点由3个时间尺度及其相互关系决定[18],即弹性波的渡越时间
τA 、结构的振动周期T和冲击载荷的持续时间tl 。当冲击持续时间tl 大于弹性波渡越时间τA 及结构振动周期T时,可用准静态理论来分析结构在冲击下的响应;当冲击持续时间tl 小于弹性波的渡越时间τA 及结构振动周期T时,需要考虑应力波在结构中的传播,用应力波理论来分析结构响应。因此,当冲击载荷频率大于结构频率时(即冲击载荷特征时间与结构振动周期有
tl<T ),应用应力波理论来分析结构响应更恰当。在材料的弹性范围内,根据一维弹性波理论[19],材料的应力响应σ 和质点与基础之间的相对速度v 之间的关系为σ=ρcv (9) 式中:
ρ 为材料的质量密度,c 为材料中的弹性波速。在冲击载荷频率与结构频率相当的范围内(即图4中的振动区),考虑到不同的结构形式对应力波在结构中传播的影响,引入与结构形状有关的形状系数κ ,则结构中某处的最大应力响应与最大相对速度vmax的关系可写为[13, 20]σmax=κρcvmax (10) 定义结构某点的最大伪速度响应与最大相对速度响应的比值为加载因子
λ λ=vpvmax=max(|ωz(t)|)max(|˙z(t)|) (11) 则式(10)可改写为
σmax=κρcvp/λ (12) 由于式(9)是式(10)的特殊形式(
κ=1 ),因此式(12)适用于图4中的整个非准静态区(即当冲击载荷频率大于或等于结构频率时)。在式(7)、式(8)及式(12)中,若已知结构的临界应力σc ,则可以在冲击响应谱图上确定各临界谱的谱值ac、drc及vpcac=Smσc (13) drc=Skσc (14) vpc=λκρcσc (15) 根据以上讨论,当冲击载荷频率fp小于结构频率f时,临界谱值由式(13)给出;当冲击载荷频率大于或等于结构频率时,临界谱值由式(14)与式(15)的较小值给出,即
B(f)={ac2πffp<fmin(2πfdrc,vpc)fp⩾f (16) 式(16)对Li等[16]提出的冲击响应谱损伤边界作了进一步改进。需要指出的是,在工程实际中,结构频率与冲击载荷频率并不是一个可以直接比较的值,并且结构件也不是单自由度的,而是具有多阶固有频率,同时冲击载荷信号也包含各种频率成分,其主导频率往往是一个频率范围。为了突出主要矛盾,以上提到的结构频率视为结构被冲击载荷所激起有效质量最大的模态所对应的频率,冲击载荷频率为其主导频率范围的上界。
2.2 结构的模态分析与简化
结合贴片晶振的典型结构,利用ABAQUS软件建立仿真模型,如图5所示,各组件的尺寸见表1。
表 1 模型尺寸Table 1. Geometrical dimensions of the modelStructure module Length/mm Width/mm Height/mm Structure module Length/mm Width/mm Height/mm Crystal plate 5.0 3.2 0.08 Pad 1.4 1.1 0.05 Conductive adhesive 0.4 0.4 0.20 Lid 6.0 4.0 0.10 Electrode 2.0 1.5 0.02 Circuit block 1 4.0 2.2 0.30 Packaging base 7.0 5.0 1.80 Circuit block 2 0.3 1.2 0.50 为了获得更高的数值精度,对晶片组件及封装结构中相关区域的网格进行细化。对于晶片网格数与总单元数分别为51200、109352的模型,若将单元数增加一倍,则相同条件下模型应力与结构基频的相对偏差均小于1%,可以认为,该有限元模型是足够准确的。为模拟材料的阻尼作用,在ABAQUS中设定线性体黏度参数为0.06,二次体黏度参数为1.2。
由于常用导电胶的玻璃态转化温度在100 ℃左右,室温下处于玻璃态,因此仿真过程中选用线弹性模型进行模拟。石英晶体的抗压屈服应力为1 GPa,而抗拉强度受拉伸形式及样品形状的影响较大,其值为40~70 MPa。以下计算中,抗拉强度取临界值40 MPa,即当晶片某处应力大于该临界值时,视为结构发生破坏而导致晶振失效。有限元模型的材料参数如表2所示。
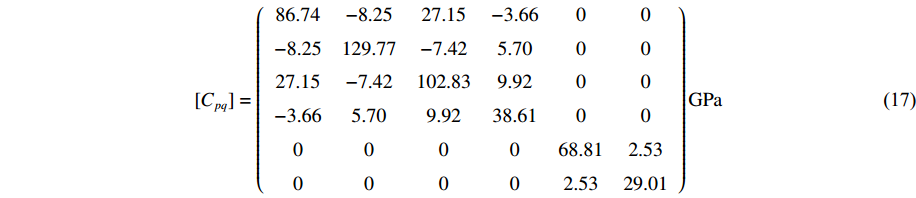
表 2 有限元模型中的材料参数Table 2. Material parameters in finite element modelModule Material Elastic modulus/GPa Density/(g·cm−3) Poisson’s ratio Tensile strength/MPa Crystal plate Quartz [Cpq][21] 2.65 40 Integrated circuit Silicon 13.0 2.33 0.28 Electrode Silver 73.2 10.53 0.38 Packaging base Phenolic resin 2.0–2.9 1.25–1.30 0.35–0.38 Lid Packfong 100.8 8.70 0.37 Conductive adhesive Epoxy polymer 2.9 2.52 0.34 Pad SnAgCu solder 41.6 8.74 0.40 对于表2中没有确定值的参数,在有限元建模过程中均取其取值范围的中间值。石英晶体的弹性常数由以下矩阵给定
[Cpq]=(86.74−8.2527.15−3.6600−8.25129.77−7.425.700027.15−7.42102.839.9200−3.665.709.9238.6100000068.812.5300002.5329.01)GPa (17) 考虑到实际试验中载荷的频率范围以及可能的失效部件,在0.5~15 kHz频率范围内对晶片组件进行模态分析,考察其动态特性,分析晶片及相关组件的模态时,将导电胶与基座相连的表面全约束。表3列出了晶片及相关组件的前15阶模态以及各阶模态在法向(垂直于晶片所在平面的方向)激起的有效质量。若将第1阶模态激起的有效质量作为参考标准,各阶模态的有效质量与该标准的比值设为比例系数P,可见在晶片的法向,第1、3、5阶模态所激起的有效质量明显较大,而且这3阶激起的有效质量占总体的94.87%。可以认为,当该结构受到法向冲击载荷时,2.585、16.898、42.546 kHz这3阶频率的模态叠加基本反映了结构响应,并且第1阶模态的有效质量远大于其他模态,因此认为响应中结构的主要频率f1 = 2.585 kHz。
表 3 晶片的各阶模态频率Table 3. Modal frequencies of the crystal plateModal
orderNatural
frequency/kHzEffective mass in
normal direction/kgP Modal
orderNatural
frequency/kHzEffective mass in
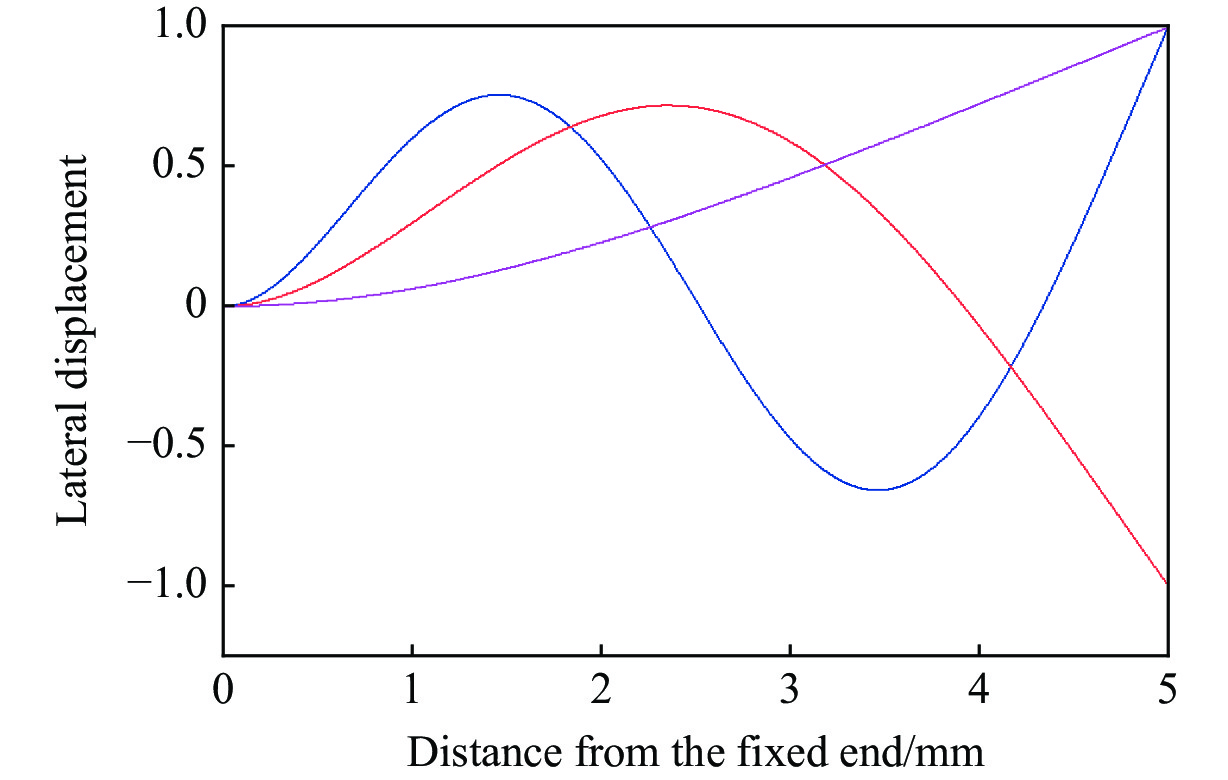
normal direction/kgP 1 2.585 3.26288 × 10−6 1.00000 9 73.438 1.13510 × 10−8 0.00348 2 12.479 7.97027 × 10−9 0.00244 10 89.044 4.91526 × 10−8 0.01506 3 16.898 8.92118 × 10−7 0.27341 11 106.386 3.74328 × 10−9 0.00115 4 36.781 1.54332 × 10−8 0.00473 12 114.336 1.38521 × 10−10 0.00004 5 42.546 3.24468 × 10−7 0.09944 13 120.211 2.83863 × 10−9 0.00087 6 56.597 4.45802 × 10−11 0.00001 14 127.139 2.82303 × 10−8 0.00865 7 61.552 5.64709 × 10−9 0.00173 15 145.952 2.23781 × 10−10 0.00007 8 69.982 1.17592 × 10−7 0.03604 图6显示了第1、3、5阶模态的平均横向振型的相对幅值(以最大位移为参考值1)。可以看到,第1、3、5阶模态均为横向弯曲模态,与悬臂梁的前3阶振型类似。为分析晶振结构在冲击环境下的损伤边界,在后续的理论分析中将晶片组件简化为全支承悬臂梁结构,即忽略基座构件和导电胶,直接将载荷施加于全支承晶体板的固定端。需要注意的是,石英晶体板与基座实际上是通过两角点处的导电胶连接的,如图1(侧视图)和图2(俯视图)所示。若将结构视为长l = 5.0 mm、宽b = 3.2 mm、厚h = 0.08 mm的悬臂梁,容易求得其前3阶频率分别为2.777、17.405、48.793 kHz,与晶片结构法向占优势的前3阶模态十分接近,相应的振型也具有良好的一致性。可以认为,结构在法向载荷作用下所激起的响应可以近似用悬臂梁模型描述。
2.3 晶振结构的损伤边界计算
当冲击载荷主导频率的上界低于晶片组件(简化为悬臂梁结构)的一阶频率时,认为梁中的应力主要由梁结构随载荷运动所导致的惯性力产生,结构的损伤边界由冲击载荷的加速度谱控制。将悬臂梁固支端受到的横向加速度载荷近似为恒定加速度作用下梁所受的惯性力(见图7)。单位长度梁结构所受的惯性力F为
F=ρbhah (18) 式中:
ah 为原横向载荷的加速度幅值,ρ为梁材料的密度。此时梁上的最大应力响应在固支端发生,且在这种弯曲状态下梁结构最外层纤维的应力是最大的,即σ=Mh2I=ρbh2l2ah4I (19) 式中:
M 为梁的最大弯矩,I 为梁截面关于中性轴的惯性矩。若材料发生损伤的临界应力为σc ,则有悬臂梁结构在低频载荷作用下的损伤边界ac=4Iρbh2l2σc (20) 当冲击载荷主导频率的上界高于梁结构的一阶频率时,梁中的最大应力主要由梁受冲击部位相对位移导致的结构最大应力以及应力波在结构中的传播产生的最大应力来决定,因此结构的损伤边界由相对位移谱与伪速度谱中的较小值控制。若设
drc 线与ac 线在伪速度冲击谱中相交于频率f0 处,由式(6)可得2πf0drc=ac/2πf0 (21) 结合式(7)、式(8),可求得交点频率
f0=√k/m2π 恰好与结构频率相等。对于受横向载荷的悬臂梁结构,两者交点处对应的圆频率为ω1=(β1l)2√EIρbhl4 (22) 式中:
β1l 是与悬臂梁频率阶数相关的常数(β1l=1.875 ),E为梁材料的弹性模量。结合式(20)和式(22),可得结构的临界相对位移谱值drc=acω21=4l2(β1l)4hEσc (23) 结构的临界伪速度谱值由式(15)求得,悬臂梁结构受横向载荷的形状系数[16]
κ=r/η ,η=√I/bh ,其中r=h/2 为梁外层纤维距中性轴的距离,则临界伪速度谱值为vpc=2√I/(bh)ρh√E/ρλσc=2σc√IEρbh3 (24) 当载荷频率大于或等于结构频率时,
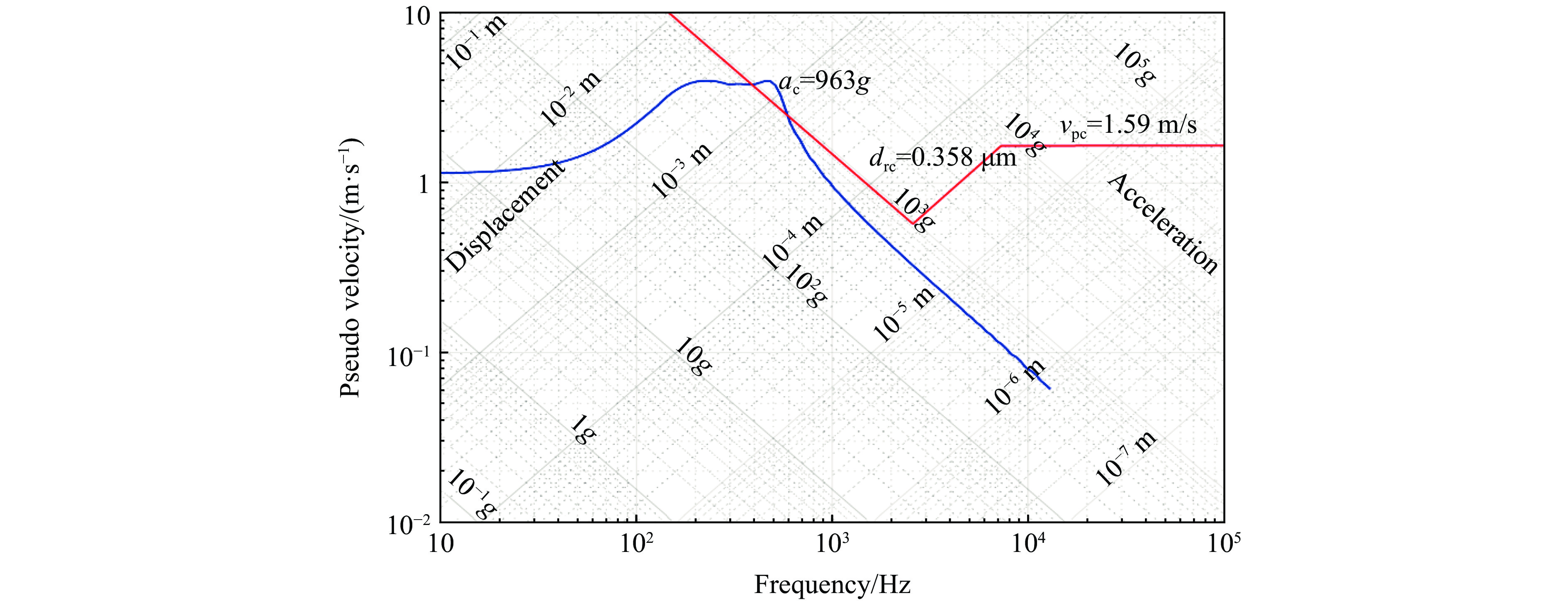
λ = 1(见附录A)。根据表2中石英晶体的材料参数,当简化为悬臂梁模型进行分析时,其弹性模量取3个主方向的平均值,由式(20)、式(23)、式(24)求得其损伤边界的各临界参数为ac=963g ,drc=35.8μm ,vpc=1.59m/s 。3. 晶振结构冲击响应的有限元仿真验证
为了验证第2节中简化分析得到的损伤边界对于晶振结构的有效性,如图8所示,通过在晶振底部4个焊盘处施加垂直向上的冲击载荷,利用有限元模型计算晶振结构在冲击环境下的动力学响应,使用ABAQUS/Explicit求解器进行冲击动力学分析,以得到临界冲击载荷及相应的临界冲击响应谱。
为了验证求得的损伤边界在各频率载荷作用下的有效性,首先在晶振焊盘处施加与冲击载荷相近的正弦衰减信号(见图9)
as(t)=a0⋅sin(ωpt)⋅exp(−ωpt10π)(0⩽t⩽80πωp) (25) 对在0.5~30.0 kHz频率范围内的几组载荷,逐步增加其幅值,当晶片的最大应力响应达到其临界值时,判定结构发生失效,记录失效发生的时间,并标记从开始加载至结构破坏这一过程中的载荷信号为临界载荷,其冲击响应谱即为该频率载荷下的临界冲击响应谱。
依次施加不同频率的正弦衰减信号,得到相应的临界载荷,在图10上画出各临界冲击谱谱线。可以看到,其基本与依据悬臂梁模型推导得到的损伤边界相吻合。当载荷频率低于结构频率时,损伤边界受等加速度谱线控制;当载荷频率高于结构频率时,损伤边界受等相对位移谱线与等伪速度谱线中的较小值控制。可以注意到各临界谱谱线所形成的最低点对应的频率
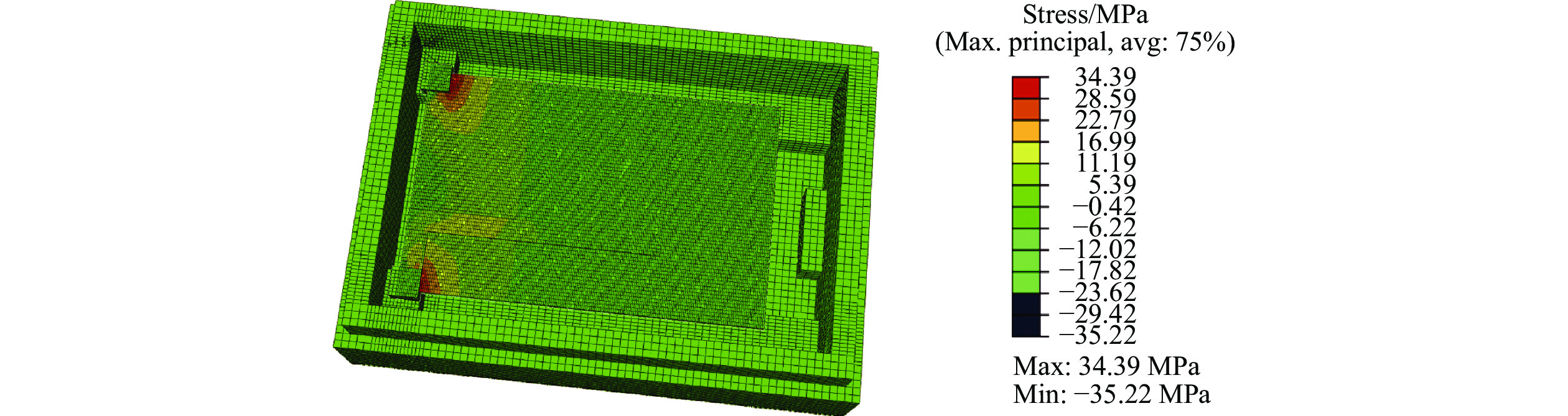
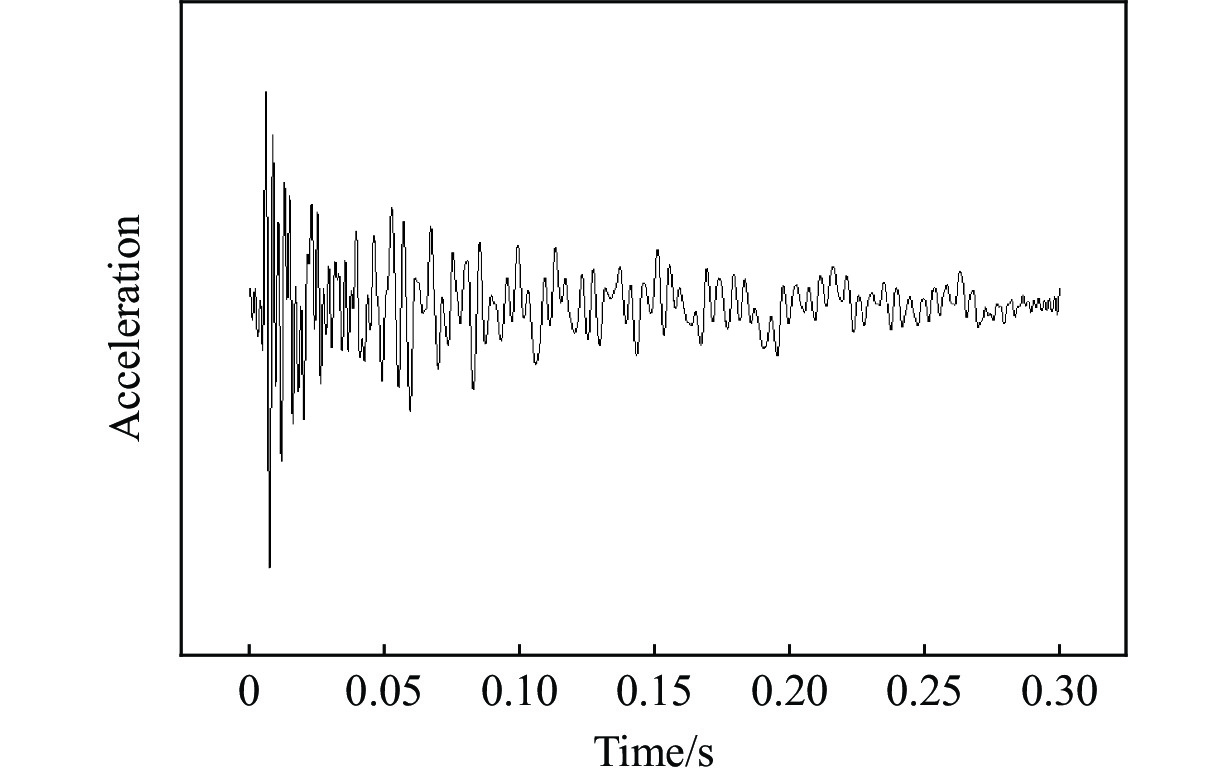
f′0 比损伤边界的交点频率f0 稍小,该现象与悬臂梁模型的一阶频率为2.777 kHz而晶片组件的一阶频率为2.585 kHz的观察相吻合。由于有限元模型中晶片组件的支承方式并非全边固支,而是通过与导电胶连接的面区域支承,因此损伤边界与临界冲击谱之间仍存在一些差异。图11为晶振结构受横向冲击作用时的应力云图,晶片组件最大应力出现在与导电胶、基座连接区的附近。由于上述仿真分析所施加的冲击载荷均只包含一种频率成分,与实际工程中的冲击载荷存在一定差异,为了验证所得的损伤边界是否适用于实际冲击环境,选用一组实测冲击载荷信号来验证损伤边界的有效性。图12为一组实测冲击信号的加速度时程曲线,在晶振底部的焊盘处施加该冲击载荷,逐步调整载荷幅值,使晶片达到的最大应力响应恰好等于其临界应力,并标记达到最大应力的时间,记该时刻之前加载的冲击信号为临界冲击载荷,得到的临界冲击响应谱如图13所示。可见,其与损伤边界较好地吻合,可以认为该损伤边界在包含多个频率分量的冲击载荷作用下依然可以适用。需要指出的是,晶片结构在整个载荷时程的初期,即7.25 ms时,已达到最大值,因而所得的临界载荷的冲击响应谱并非图12中载荷的冲击响应谱。
4. 结 论
借鉴单自由度系统在不同频率下响应特点的分析,根据结构的应力损伤准则,在伪速度冲击响应谱中获得了改进的损伤边界参数,并结合晶振模型验证了其有效性,得到以下结论。
(1)当冲击载荷主导频率上界低于结构主要频率时,结构的损伤边界由等加速度谱线控制;当冲击载荷主导频率上界高于结构主要频率时,结构的损伤边界由等相对位移谱线和等伪速度谱线中的较小值控制。
(2)当晶振受到垂直于晶片平面的冲击载荷时,晶片的动力学响应与悬臂梁结构类似,可以用简单的悬臂梁模型近似地分析晶片的损伤破坏机理。
(3)通过对晶振结构的有限元分析,得到了其在冲击载荷作用下大频率范围的损伤边界,验证了损伤边界各参数选取的有效性。这为以晶振为代表的一些微小高频元器件的力学失效分析以及冲击环境适应性设计提供了参考。
-
表 1 模型尺寸
Table 1. Geometrical dimensions of the model
Structure module Length/mm Width/mm Height/mm Structure module Length/mm Width/mm Height/mm Crystal plate 5.0 3.2 0.08 Pad 1.4 1.1 0.05 Conductive adhesive 0.4 0.4 0.20 Lid 6.0 4.0 0.10 Electrode 2.0 1.5 0.02 Circuit block 1 4.0 2.2 0.30 Packaging base 7.0 5.0 1.80 Circuit block 2 0.3 1.2 0.50 表 2 有限元模型中的材料参数
Table 2. Material parameters in finite element model
Module Material Elastic modulus/GPa Density/(g·cm−3) Poisson’s ratio Tensile strength/MPa Crystal plate Quartz [Cpq][21] 2.65 40 Integrated circuit Silicon 13.0 2.33 0.28 Electrode Silver 73.2 10.53 0.38 Packaging base Phenolic resin 2.0–2.9 1.25–1.30 0.35–0.38 Lid Packfong 100.8 8.70 0.37 Conductive adhesive Epoxy polymer 2.9 2.52 0.34 Pad SnAgCu solder 41.6 8.74 0.40 表 3 晶片的各阶模态频率
Table 3. Modal frequencies of the crystal plate
Modal
orderNatural
frequency/kHzEffective mass in
normal direction/kgP Modal
orderNatural
frequency/kHzEffective mass in
normal direction/kgP 1 2.585 3.26288 × 10−6 1.00000 9 73.438 1.13510 × 10−8 0.00348 2 12.479 7.97027 × 10−9 0.00244 10 89.044 4.91526 × 10−8 0.01506 3 16.898 8.92118 × 10−7 0.27341 11 106.386 3.74328 × 10−9 0.00115 4 36.781 1.54332 × 10−8 0.00473 12 114.336 1.38521 × 10−10 0.00004 5 42.546 3.24468 × 10−7 0.09944 13 120.211 2.83863 × 10−9 0.00087 6 56.597 4.45802 × 10−11 0.00001 14 127.139 2.82303 × 10−8 0.00865 7 61.552 5.64709 × 10−9 0.00173 15 145.952 2.23781 × 10−10 0.00007 8 69.982 1.17592 × 10−7 0.03604 -
[1] VIG J R, AUDOIN C, CUTLER L S, et al. Acceleration, vibration and shock effects-IEEE standards project P1193 [C]//Proceedings of 1992 IEEE Frequency Control Symposium. Hershey: IEEE, 1992: 763−781. DOI: 10.1109/FREQ.1992.269960. [2] FILLER R L. The acceleration sensitivity of quartz crystaloscillators: a review [J]. IEEE Transactions on Ultrasonics, Ferroelectrics, and Frequency Control, 1988, 35(3): 297–305. doi: 10.1109/58.20450 [3] 冷国俊, 陈睿, 何著, 等. 晶振加速度敏感性矢量计算及耦合机理研究 [J]. 应用数学和力学, 2014, 35(Suppl 1): 65–69.LENG G J, CHEN R, HE Z, et al. Research on crystal acceleration sensitivity vectorcalculation and coupling mechanism [J]. Applied Mathematics and Mechanics, 2014, 35(Suppl 1): 65–69. [4] 邱士起, 牛少华, 高世桥. 冲击载荷下石英晶体振荡器失效机理分析 [J]. 兵工学报, 2016, 37(Suppl 2): 96–100.QIU S Q, NIU S H, GAO S Q. Failure mechanism analysis of quartz crystal oscillators under impact load [J]. Acta Armamentarii, 2016, 37(Suppl 2): 96–100. [5] 李乐, 祖静, 徐鹏. EXO3晶振与KSS晶振在高过载下的失效特性分析 [J]. 中国测试技术, 2007, 33(3): 88–90. doi: 10.3969/j.issn.1674-5124.2007.03.029LI L, ZU J, XU P. Failure mechanism of crystal oscillator EXO3 and KSS under high shock [J]. China Measurement Technology, 2007, 33(3): 88–90. doi: 10.3969/j.issn.1674-5124.2007.03.029 [6] MOENINGC J. Pyrotechnic shock flight failures [C]//Proceedings of the 31st Annual Technical Meeting of the Institute of Environmental Sciences. Las Vegas, 1985: 4−5. [7] 赵小龙, 马铁华, 范锦彪. 弹载常用芯片在高g值冲击下的失效分析 [J]. 仪器仪表学报, 2013, 34(10): 2358–2364.ZHAO X L, MA T H, FAN J B. Failure analysis of common missile-borne chip in high g shock [J]. Chinese Journal of Scientific Instrument, 2013, 34(10): 2358–2364. [8] 鲍爱达, 陈员娥, 李长龙, 等. 弹载加速度记录仪在冲击环境下的失效研究 [J]. 振动与冲击, 2013, 32(13): 182–186, 196. doi: 10.3969/j.issn.1000-3835.2013.13.034BAO A D, CHEN Y E, LI C L, et al. Failure study on a missile accelerometer recorder under shock environment [J]. Journal of Vibration and Shock, 2013, 32(13): 182–186, 196. doi: 10.3969/j.issn.1000-3835.2013.13.034 [9] 杨磊, 秦玉浩, 蒋韦, 等. 星载高稳晶振的减振设计 [J]. 空间电子技术, 2016, 13(5): 9–13. doi: 10.3969/j.issn.1674-7135.2016.05.003YANG L, QIN Y H, JIANG W, et al. Vibration attenuation design of OCXO for aerospace applications [J]. Space Electronic Technology, 2016, 13(5): 9–13. doi: 10.3969/j.issn.1674-7135.2016.05.003 [10] 徐鹏. 高g值加速度作用下晶振的失效机理分析 [J]. 中北大学学报(自然科学版), 2010, 31(4): 424–428. doi: 10.3969/j.issn.1673-3193.2010.04.022XU P. Failure mechanical analysis of crystal oscillator under high g acceleration [J]. Journal of North University of China (Natural Science Edition), 2010, 31(4): 424–428. doi: 10.3969/j.issn.1673-3193.2010.04.022 [11] 刘晨, 张欢, 朱剑涛, 等. 航天器电子产品抗火工冲击环境设计方法 [J]. 航天器工程, 2018, 27(3): 45–51. doi: 10.3969/j.issn.1673-8748.2018.03.007LIU C, ZHANG H, ZHU J T, et al. Design to resist pyroshock environment for space electronic unit [J]. Spacecraft Engineering, 2018, 27(3): 45–51. doi: 10.3969/j.issn.1673-8748.2018.03.007 [12] 马爱军, 石蒙, 刘洪英, 等. 应用谐振装置在电动振动台上实现高量级冲击响应谱的仿真研究 [J]. 航天器环境工程, 2011, 28(5): 427–430. doi: 10.3969/j.issn.1673-1379.2011.05.004MA A J, SHI M, LIU H Y, et al. Simulations of high level shock response spectrum test using resonant fixtureon an electrodynamics shaker [J]. Spacecraft Environment Engineering, 2011, 28(5): 427–430. doi: 10.3969/j.issn.1673-1379.2011.05.004 [13] GABERSON H A. Pseudo velocity shock spectrum rules for analysis of mechanical shock [C]//Proceedings of IMAC XXV. Orlando: Society of Experimental Mechanics, 2007: 367−402. [14] GABERSON H A. Half sine shock tests to assure machinery survival in explosive environments [EB/OL]. (2013–04–15) [2020–04–26]. http://www.vibrationdata.com/tutorials2/HSS_test.pdf. [15] IRVINE T. Shock severity limits for electronic component [EB/OL]. (2014–06–27)[2020–04–26]. http://vibrationdata.com/tutorials2/shock_severity_electronics.pdf. [16] LI B W, LI Q M. Damage boundary of structural components under shock environment [J]. International Journal of Impact Engineering, 2018, 118: 67–77. doi: 10.1016/j.ijimpeng.2018.04.002 [17] ALEXANDER J E. Shock response spectrum: a primer [J]. Sound & Vibration, 2015, 43(6): 6–14. [18] SRIKAR V T, SENTURIA S D. The reliability of microelectromechanical systems (MEMS) in shock environments [J]. Journal of Microelectromechanical Systems, 2002, 11(3): 206–214. doi: 10.1109/JMEMS.2002.1007399 [19] 王礼立. 应力波基础[M]. 2版. 北京: 国防工业出版社, 2005: 12–20.WANG L L. Foundation of stress waves [M]. 2nd ed. Beijing: National Defense Industry Press, 2005: 12–20. [20] GABERSON H A. Shock severity estimation [J]. Sound & Vibration, 2012, 46(1): 12–20. [21] 李迪. 石英晶体圆板谐振器的有限元分析及实验研究 [D]. 南京: 南京航空航天大学, 2017: 14–15.LI D. The finite element analysis and experiment research of circular quartz crystal resonator [D]. Nanjing: Nanjing University of Aeronautics and Astronautics, 2017: 14−15. -








 下载:
下载:

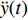

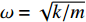

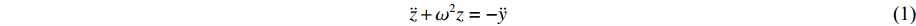
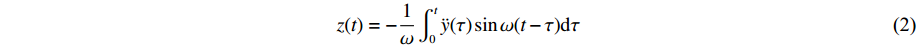

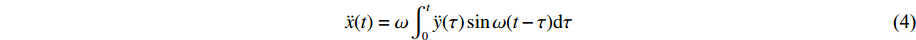
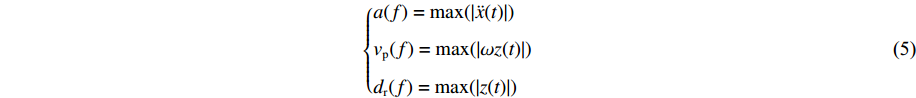
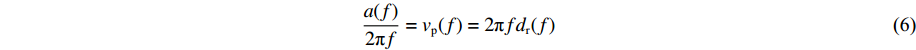
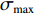
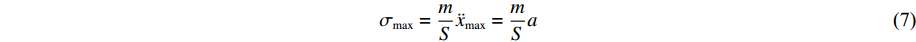
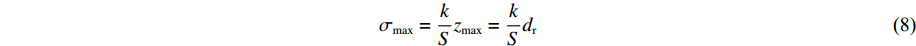
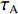
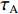
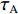
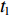
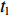
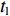
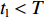
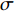
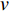

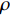
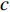
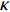

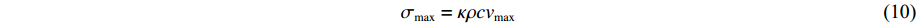
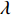
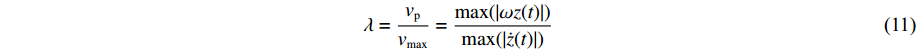
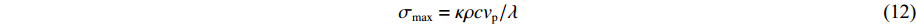
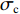

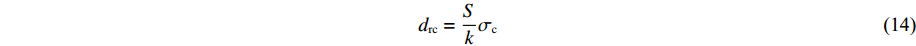
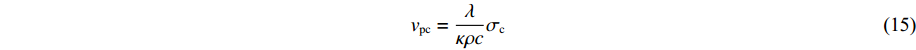



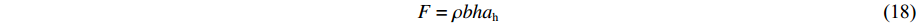
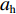
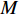
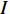
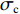
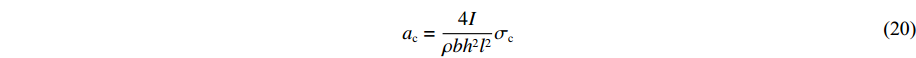

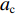
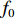
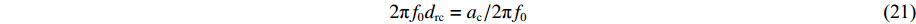

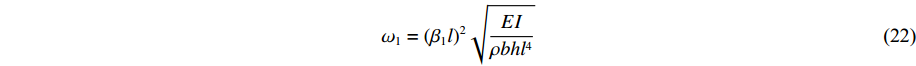
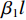
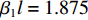
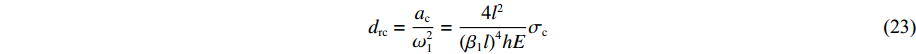
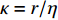
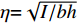
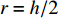
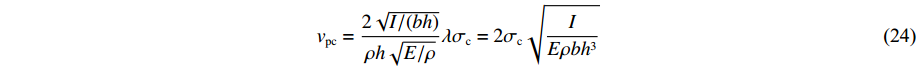
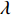
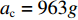
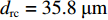



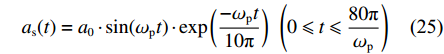
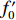
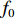





 下载:
下载: