Failure of Square Plate under the Influence of Boundary Conditions Subjected to Shock Loading
-
摘要: 冲击载荷作用下边界条件对方板的毁伤破坏具有很大影响。利用落锤试验机开展了不同边界支撑下固支方板的冲击试验，为获取固支方板边界撕裂的典型破坏模式，专门设计加工了与固支方板尺寸相当的冲击锤头和可改变倒角的方板支撑框架。研究结果表明：（1）冲击载荷作用下，固支方板呈现出塑性大变形、单边撕裂、双边撕裂等典型破坏模式，倒角越小，方板越容易撕裂；（2）边界支撑对固支方板中心位移、整体变形轮廓影响较小，但对方板的撕裂长度、临界撕裂阈值存在较大影响；（3）不同边界支撑主要改变方板边界处的剪切应变，边界支撑倒角越小，剪切效果越明显，方板边界临界撕裂应变位于[0.191，0.241]区间。Abstract: Boundary condition exerts great influence on the failure mode of square plate under intensive loading. In this paper, a series of experiments of clamped square plates with different boundary chamfer radius were performed in the drop hammer impacting machine, in which the drop hammer was specially designed nearly same as the square plate in dimension and the supporting framework could offer various boundary conditions. The results show that: (1) the failure mode of large plastic deformation, one-side tearing and double-side tearing can be observed, and the square plates are more easily damaged under smaller boundary chamfer radius. (2) The boundary chamfer radius has a minor effect on the plate center displacement and deformation profile, but a major influence on the boundary tearing length and crack forming process. (3) The shear effect on the boundary region of clamped square plate would decrease with increasing boundary chamfer radius, and it is more easier for the plate with smaller boundary chamfer radius to get torn under the same intense impulsive loading. The critical failure strain of square plate is among [0.191, 0.241].
-
Key words:
- boundary chamfer /
- deformation/failure mode /
- square plate /
- drop hammer /
- stress state
-
浅部矿物资源的日渐枯竭使资源开采工作向地下更深处发展。矿物采掘工作往往需要多次爆破，加之大型机械设备的连续钻探施作[1-3]，以及随深度增加而增大的地应力，导致巷道围岩遭受频繁的爆破冲击和机械振动，围岩体的破坏程度不断加剧，岩爆发生的概率也大大增加，严重威胁煤炭开采人员和机械设备的安全，开采进度难以保证。
分离式霍普金森压杆（split Hopkinson pressure bar，SHPB）是目前国内外众多学者研究深部岩石动态力学性能和破坏机制的主要工具。金解放等[4-6]通过改造的SHPB动静组合加载装置，对长径比为1.0的砂岩进行了动静组合循环冲击试验，发现冲击试样呈现锥体的破坏模式，表明砂岩的单位体积吸收能随循环冲击次数的增加而增大，致使岩石内部损伤加剧、强度降低，他们还将岩石的动态应力-应变曲线划分为5个发展阶段，即压密阶段、弹性阶段、内部裂纹扩展的加载阶段、第1卸载阶段和第2卸载阶段。王志亮等[7]在岩石的单轴循环冲击试验中，利用静态应力-应变曲线，推导出静态裂纹起裂应力，进而得出动态裂纹起裂应力。田诺成等[8]对不同轴压下的花岗岩进行等幅循环冲击，发现在相同的入射波应力循环冲击下，循环冲击次数随轴压的增大先增加后减少，即轴压使循环冲击荷载作用下的岩石动力学特性呈先强化后劣化的变化趋势，结合声发射技术，发现强度强化是由于预加轴压小于岩石的起裂应力，反之则会造成岩石强度劣化。Li等[9]利用大直径（75 mm）压杆对无轴压和无围压下的花岗岩试样进行循环冲击试验，发现当动载应力不超过岩石静态强度的60%时，循环冲击基本不会对岩石造成明显损伤，该结论在文献[5]中也得到证实。唐礼忠等[10]研究岩石在预加轴压与循环冲击共同作用时发现，岩石的应力-应变曲线在不同轴压下呈现回弹与不回弹两种特性（定义为局部回弹），且岩石的破碎块度随轴压的增大而增大。
很多理论和试验研究均表明，在静载与动载的共同作用下岩石的力学性能和破坏模式相较于静载条件下有所不同。目前，已有部分学者对砂岩开展了一维或三维动静组合加载下的循环冲击试验[11-13]，但鲜有涉及一维动静组合循环加载下的岩石力学性能和能量耗散，以及冲击气压对岩石动态力学性能的影响等问题。对于深部巷道开挖时的围岩体，矿柱在承受一个方向上的静荷载（地应力）时还要承受外部较大且较频繁的动载扰动，明显的应力集中极易造成矿柱失稳。为此，本研究主要探讨砂岩在同一方向的动静组合循环加载下的力学性能、能量耗散及破坏模式，这对地下工程建设的安全评价具有重要的现实意义。
1. 砂岩的SHPB试验
1.1 试样制备
砂岩岩样取自鹤煤三矿井下800 m巷道工作面，其完整性和均质性较好。依据国际岩石力学学会（ISRM）推荐的试样尺寸[11, 14]，将砂岩加工成长径比为0.5（直径为50 mm，长度为25 mm）的圆柱试块。为了确保试验结果的精确度，精细打磨试件，使试件两个端面的不平行度小于0.02 mm，不垂直度小于0.001 rad。取样所选砂岩为天然湿度状态，测得7个试块的单轴抗压强度分别为89.31、123.75、117.47、97.78、120.36、125.94、138.36 MPa，根据离散性要求，舍去最大值和最小值，求得试样的平均单轴抗压强度（fcu）为116.94 MPa，其他静态物理力学参数见表1，其中：ft为抗拉强度，E为弹性模量，μ为泊松比，v为纵波波速。
表 1 砂岩的静态物理力学参数Table 1. Static physical and mechanical parameters of sandstonefcu/MPa ft/MPa E/GPa $\,\mu $ v/(m·s−1) 116.94 5.30 31.25 0.26 3923 1.2 试验系统及原理
采用河南理工大学冲击实验室改进的SHPB试验装置（见图1）：压杆系统由子弹、入射杆、透射杆和吸收杆等部分组成，其中子弹为直径37 mm、长度 400 mm的合金冲头，入射杆为直径50 mm、长度2400 mm的变截面合金钢，透射杆为直径50 mm、长度1200 mm的合金钢，吸收杆为直径37 mm的铝质杆，合金钢杆中的纵波波速为5190 m/s，弹性模量为210 GPa；氮气为动力源，通过调节气压控制器改变子弹冲击速度，子弹速度由红外测速仪测量；试验数据由江苏东华生产的DH8302超动态应变放大仪采集。该试验系统可进行常规的单轴、一维和三维冲击试验，亦可进行轴压和围压的手动或数控设定。
图2为SHPB试验装置示意图。子弹在冲击气压下以一定速度撞击入射杆，入射杆中产生入射波传递给试样，由于试块与试样的波阻抗存在较大差异，使得部分入射波经试样传给透射杆转化为透射波，一部分经试样反射回入射杆转化为反射波，另有少数被试样吸收，转化为吸收波，用于岩样裂缝的产生与发展。其中，入射波
${\varepsilon _{\rm i}}(t)$ 和反射波${\varepsilon _{\rm r}}(t)$ 均由粘贴在入射杆表面的应变片A1测得，而透射波${\varepsilon _{\rm t}}(t)$ 由应变片A2测得。由于试样厚度远小于应力波波长，因此在“应力均匀性假设”的前提下，可认为试样中的应力均匀无衰减，根据一维应力波理论，由关系式${\varepsilon _{\rm i}}(t) + {\varepsilon _{\rm r}}(t) = {\varepsilon _{\rm t}}(t)$ ，将“三波法”简化为“二波法”[15]，推导出试样的动态应力$ {\sigma _{\text{s}}}(t) $ 、应变$ {\varepsilon _{\text{s}}}(t) $ 、应变率$ {\dot \varepsilon _{\text{s}}}(t) $ 分别为σs(t)=E0A0Asεt(t) (1) εs(t)=−2c0Ls∫t0εr(t)dt (2) ˙εs(t)=−2c0Lsεr(t) (3) 式中：
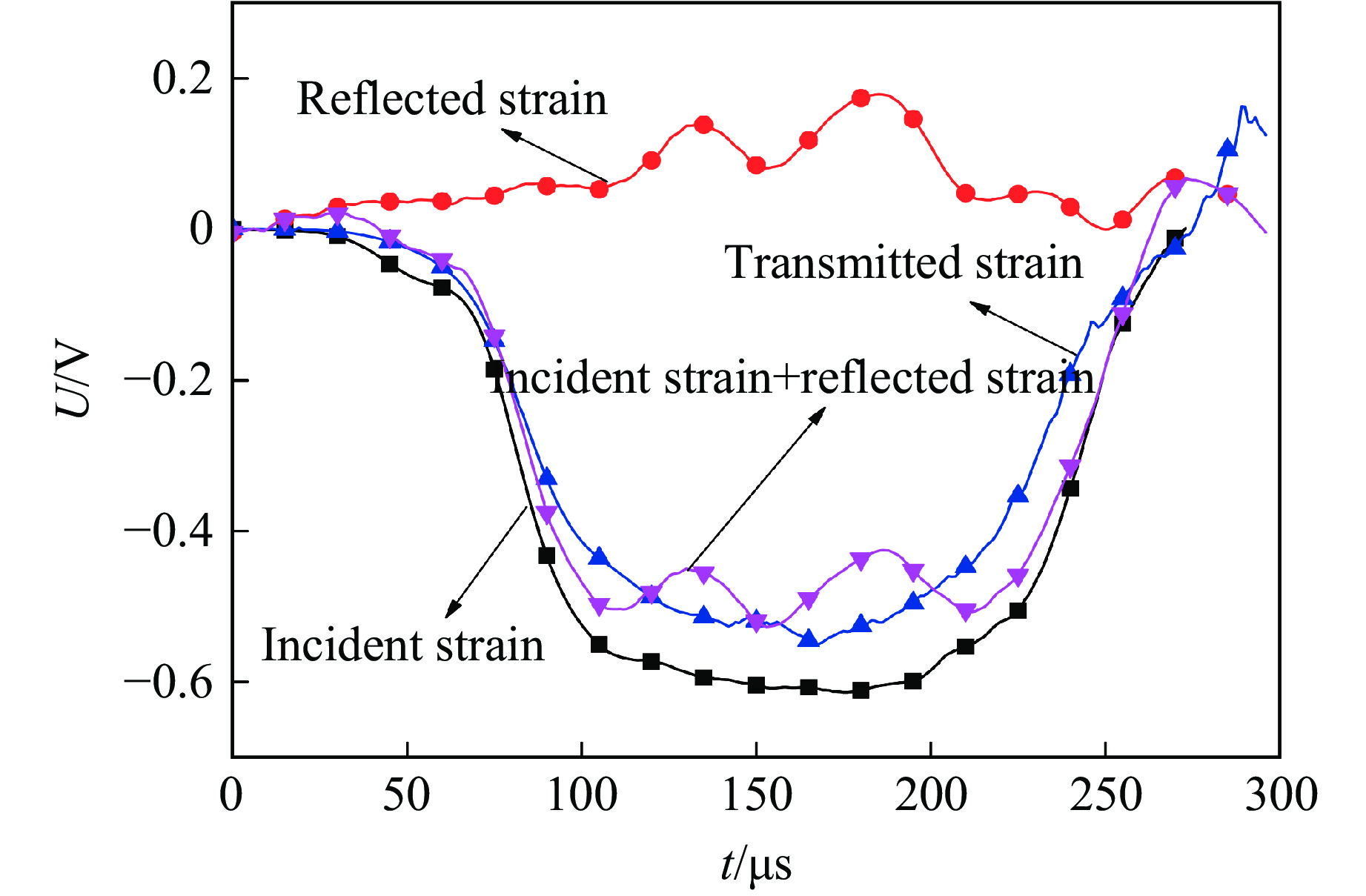
$ {A_0} $ 、$ {c_0} $ 和$ {E_0} $ 分别为弹性杆的横截面面积（mm2）、纵波波速（m/s）和弹性模量（GPa），${A_{\rm s}}$ 和${L_{\rm s}}$ 分别为试样的横截面面积（mm2）和长度（mm）。选取最具代表性的砂岩试样应力测量原始数据，得到图3（其中U为电压），对入射波和反射波进行叠加，可见其波形与透射波基本重合，表明试样左右两端受力基本相等，满足应力均衡条件[16]。
1.3 试验方法
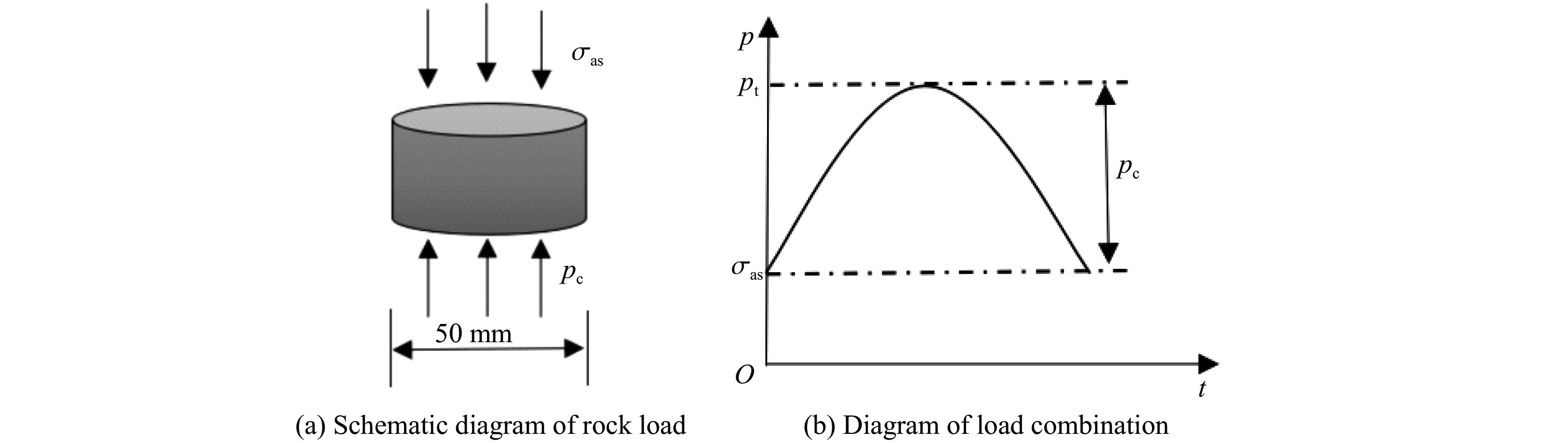
为探究砂岩在一维静压下的循环冲击动态力学特性，基于试验装置的加载限度，将轴压分别设置为 5、15 和 20 MPa，对应于砂岩单轴抗压强度的 4%、13%和 17%，每组试验对3个试样进行冲击。试验前，为减少压杆与试样之间的摩擦力，保证良好接触，在试样与压杆接触端面涂抹少量黄油用作耦合剂；取边长15 mm、厚0.3 mm的正方形H62黄铜片作为波形整形器贴于入射杆靠近子弹的端面；由同一细直钢杆将子弹送入发射腔内同一位置，尽可能保证相同气压下入射波的峰值相同或相近。一维动静组合岩石加载模型见图4(a)，采用等截面积加载方式对试样进行循环冲击，加载的恒应变率半正弦波应力脉冲[9, 11, 17] 如图4(b)所示 （其中：pt为总载荷；
$\sigma $ as为预加轴压；pc为冲击气压，即动载荷），冲击完成后对试样拍照并观察，直至试样产生宏观裂纹并完全破碎。试样的物理参数及加载方案见表2，其中：L为试样长度，D为试样直径，$\,\rho $ s为试样密度。
图 4 一维动静组合加载模型示意图Figure 4. Sketch of model under one-dimensional coupled static and dynamic loads表 2 代表性试样的基本物理参数及加载方案Table 2. Basic parameters of the representative samples and the loading schemeSample No. L/mm D/mm $\,\rho $s/(kg·m−3) $\sigma $as/MPa pc/MPa S3-1
S3-2
S3-3
S3-4
S3-5
S3-6
S3-724.97
25.26
25.38
25.26
25.35
25.04
24.9449.56
49.60
49.53
49.84
49.90
49.88
49.652591
2571
2567
2561
2580
2564
26285
15
20
15
20
15
200.8
0.8
0.8
1.0
1.0
1.2
1.22. SHPB试验结果及分析
2.1 试验结果
记录每块岩样的累计冲击次数，并绘制动态应力-应变曲线，相同加载及冲击类型下进行多次试验，选取最具代表性的试验数据进行分析。表3列出了试样的累计冲击次数（n）。对于试样S3-7，因试验数据采集不及时造成数据缺失，无法对其各项动态力学性能进行分析评价，但其冲击破坏形态及循环冲击次数等数据仍具有有效性。
表 3 代表性试样的总循环冲击次数Table 3. Total number of the cyclic impacts of the representative samplesS3-1 S3-2 S3-3 S3-4 S3-5 S3-6 S3-7 15 10 8 11 9 6 2 2.2 应力-应变曲线特征分析
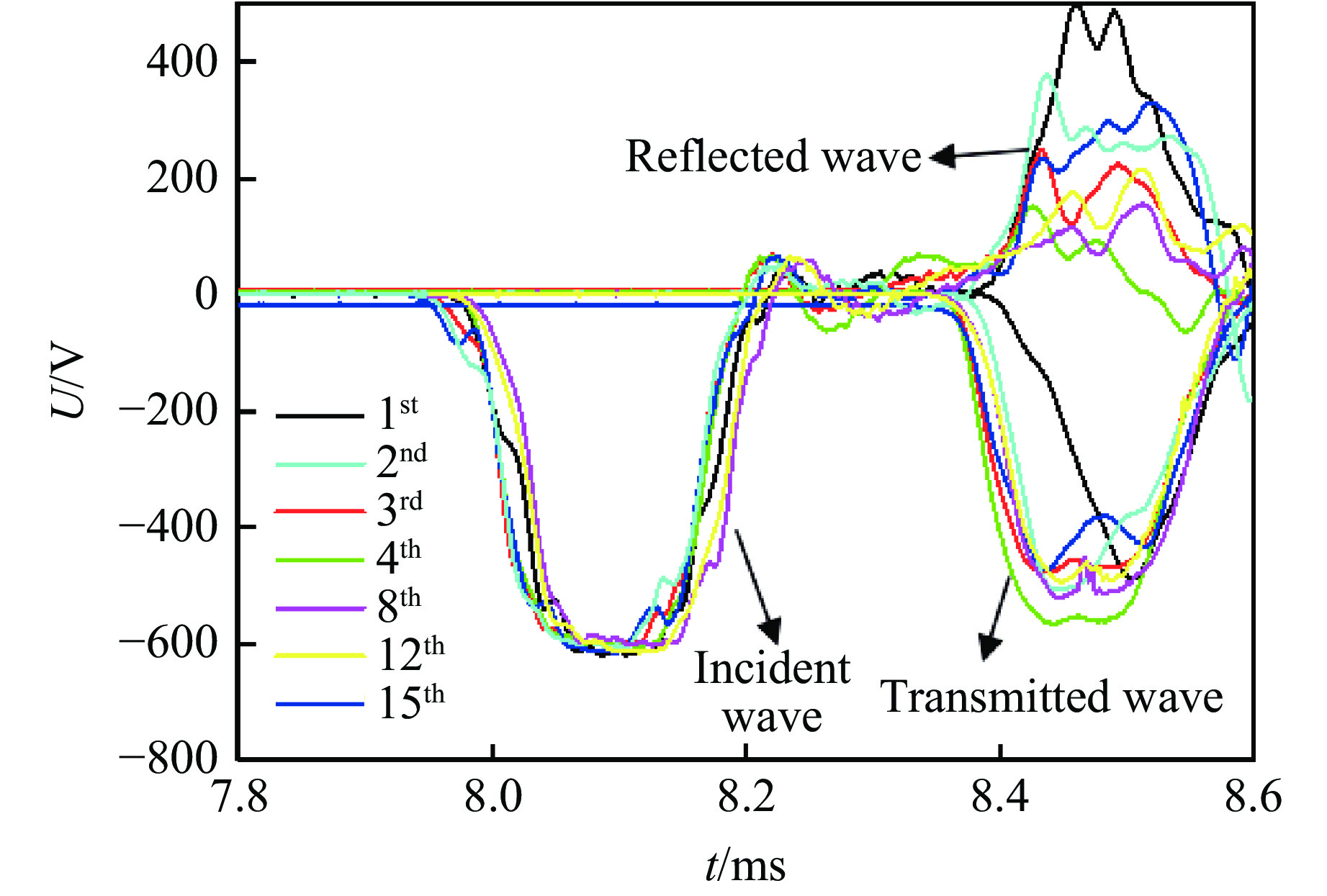
图5显示了循环冲击下试样S3-1的原始波形。可以看出，在轴压与冲击气压不变的情况下，每次冲击的入射波基本重合，说明试样每次受到的冲击荷载基本相同。首次冲击的反射波峰值最大，透射波峰值最小；随着冲击次数的增加，反射波和透射波曲线整体下移；而后，随着冲击的持续进行，反射波与透射波曲线又整体上移，直至试样发生宏观破碎。
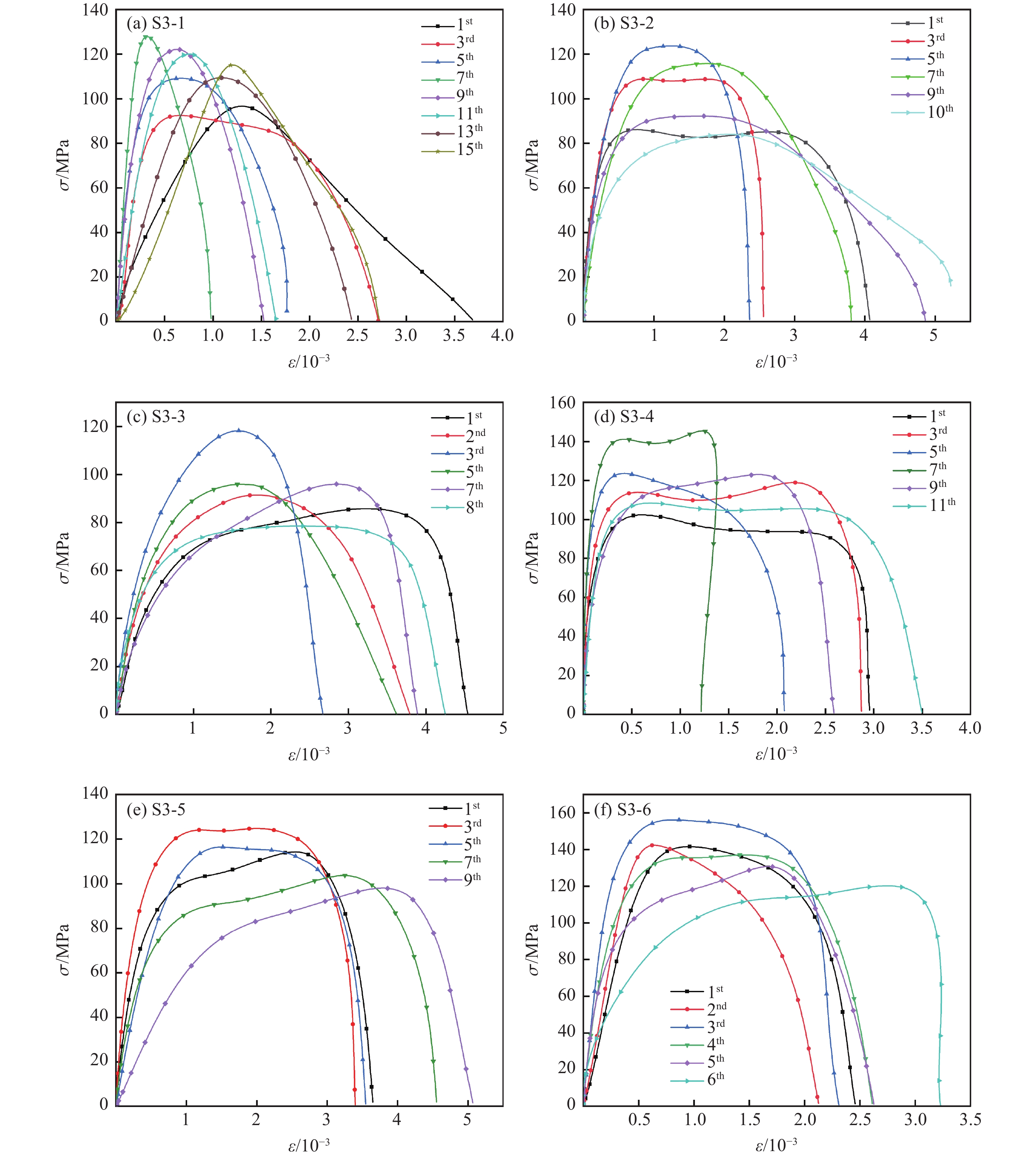
图6为砂岩试样在循环冲击作用下的动态应力-应变曲线。可以看出：所有曲线在应力峰值前的变化趋势呈现一致性，即应力随应变呈递增关系；应力峰值前，曲线斜率逐渐减小，没有压密阶段，仅有弹性阶段和非线性变形阶段。应力峰值后，应力-应变曲线表现出两种变形特征：一是极少数的局部回弹现象，如试样S3-4的第7次冲击，可能的原因是轴压的施加使试样内部提前储存一定的弹性能，导致试样在经历多次冲击没有完全破碎的情况下内部积攒的弹性力大于外部冲击力，而在此瞬间，试样上的质点速度可能高于入射杆端面的质点速度，使得试样在以上两种因素的综合作用下于卸载阶段出现曲线局部回弹现象；二是无局部回弹，即与一般单轴压缩试验得到的应力-应变曲线变化趋势一致，该现象可能是由于试样内部的弹性能过早释放，造成卸载阶段的弹性力始终小于外部加载应力。
图 6 砂岩试样在循环冲击荷载下的应力-应变曲线Figure 6. Stress-strain curves of sandstone subjected to cyclic impact为进一步说明图5中冲击波形曲线的变化特征，对图6所示的砂岩试样在循环冲击作用下的动态应力-应变曲线变化特征进行分析。定义曲线峰值应力（
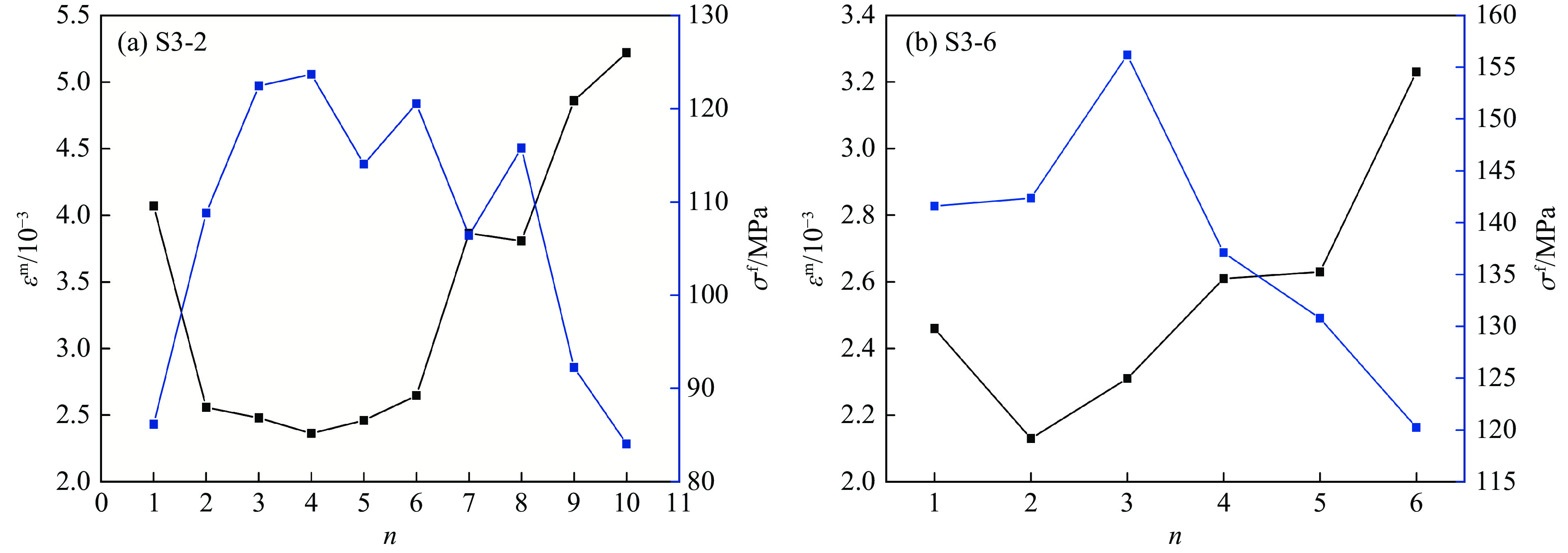
$\sigma $ f）对应的应变为峰值应变（$\varepsilon $ f），应变的最大值为最大应变（$\varepsilon $ m）。在试块冲击破坏的整个过程中，动态应力-应变曲线整体出现回弹现象（定义为整体回弹），即动态峰值应力总体呈现先上升后下降的变化趋势，而最大应变则刚好相反（见图7）。在加载初期，由于试块内部具有较多孔隙及细小裂纹，大部分入射波在孔隙或裂纹表面发生反射，小部分发生透射，因此造成反射波峰值较大，透射波峰值较小，导致动态峰值应力逐渐增大，峰值应变逐渐减小，最大应变也相应减小。这说明在前几次冲击过程中，砂岩内部众多微小孔隙（裂隙）在荷载作用下不断变小甚至“愈合”，使得大部分入射波穿过试样，造成试块抵抗外界动态荷载的能力逐渐增强，即动态应力-应变曲线整体回弹。随着冲击的继续进行，砂岩的动态强度呈减小趋势，而峰值应变却增大，说明砂岩试块在持续的损伤软化作用下，内部开始产生细小裂纹，越来越多的入射波发生反射，且随着冲击次数的增加，裂纹不断发展，直至产生宏观破坏。
图 7 峰值应力及最大应变与冲击次数的关系Figure 7. Variations of peak stress and maximum strain with impact times2.3 砂岩的动态应力、应变及应变率的变化规律
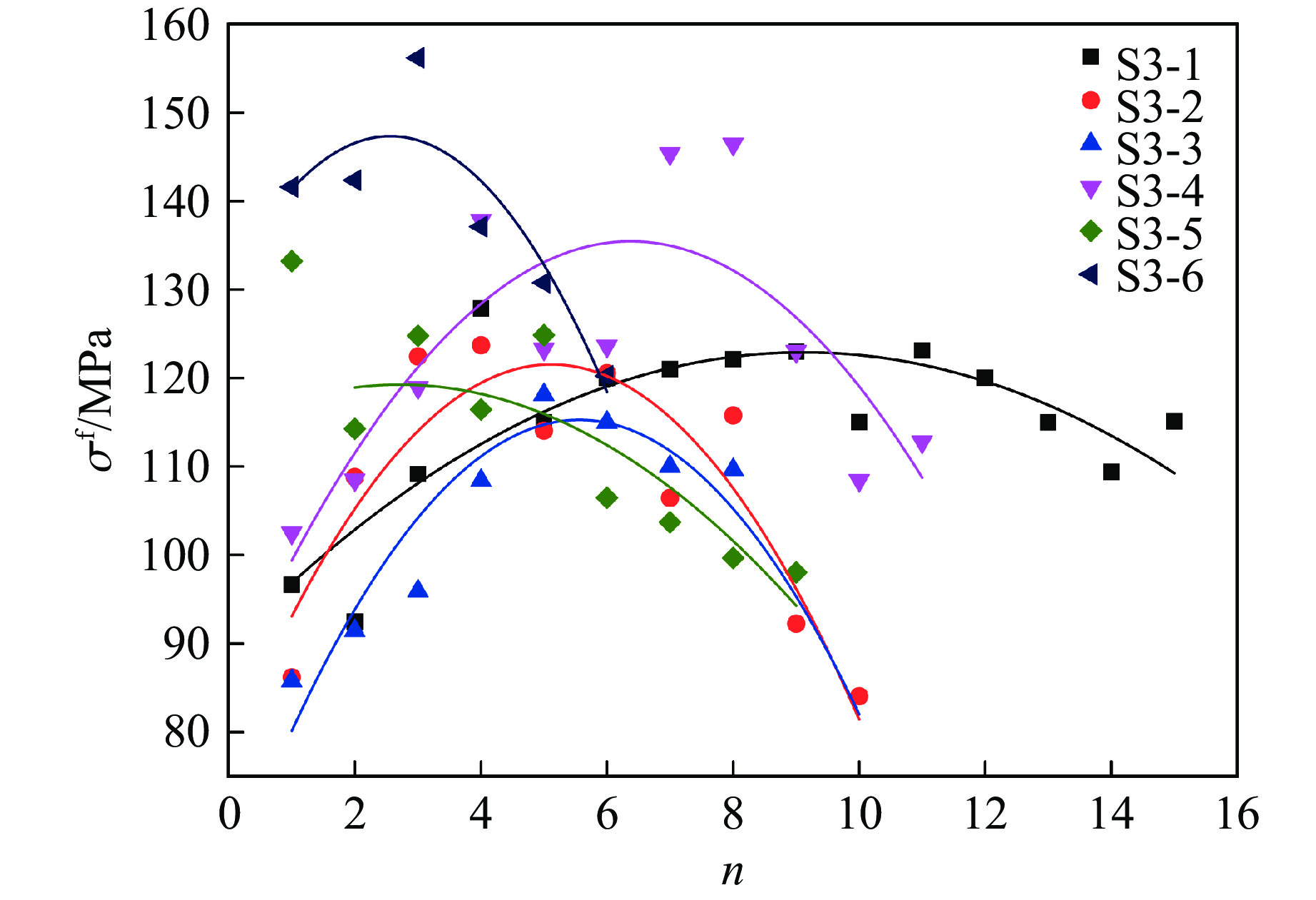
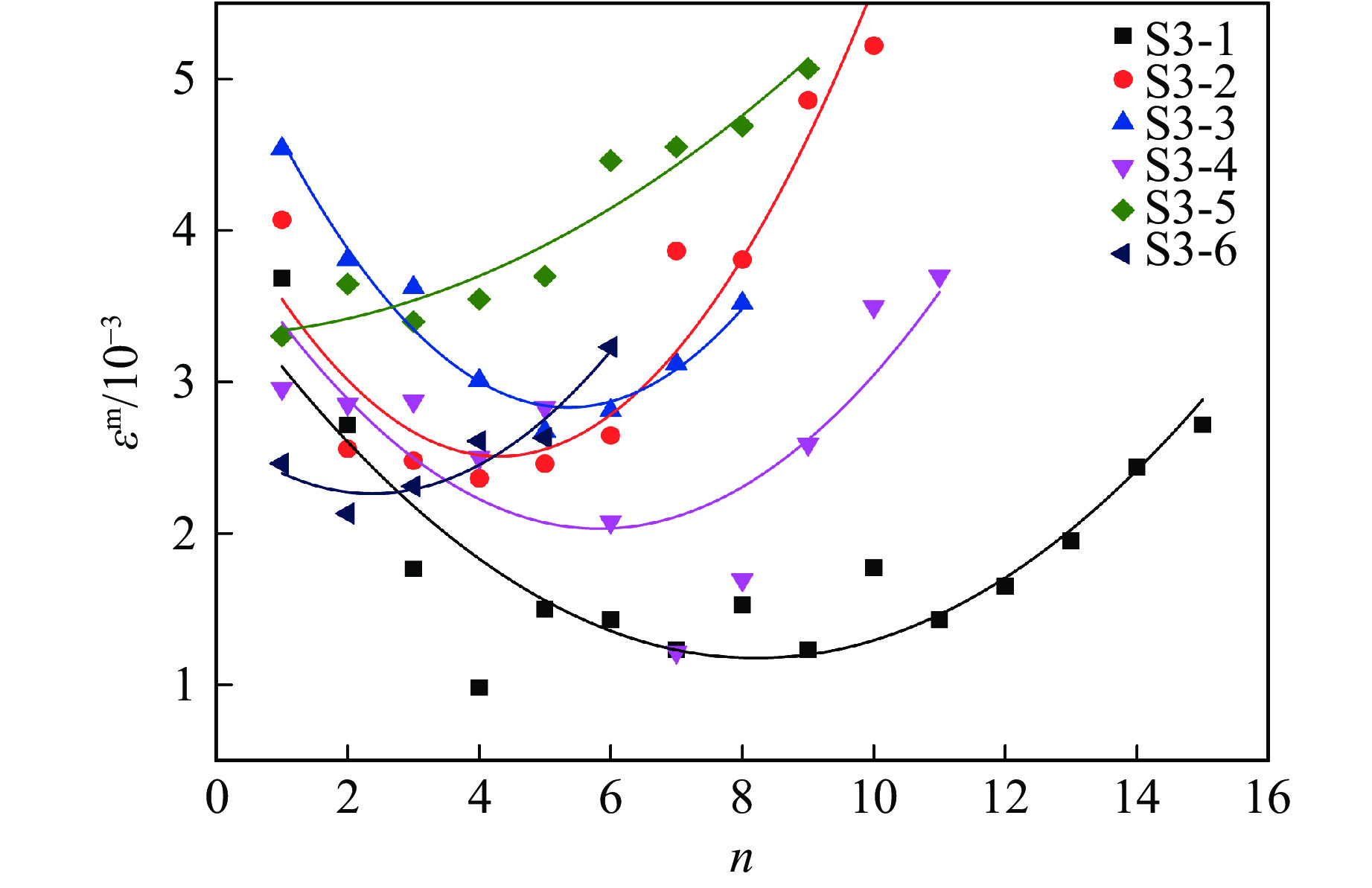
图8显示了各试样每次冲击的峰值应力与循环冲击次数的二次抛物曲线拟合关系，拟合曲线的最高点为冲击回弹转折点。对比试样S3-2和S3-3可以发现，在相同的冲击气压下，峰值应力曲线随轴压的增大而整体下移，试样S3-4和S3-5也有相同的变化趋势，表明峰值应力随轴压的增大而减小，此现象说明轴压对岩石的承载能力起到削弱效果，在一定程度上验证了文献[18-20]得出的“轴压弱化岩石承载能力”这一结论。另外，回弹转折点所对应的循环冲击次数随轴压的增大而减小，即回弹转折点前移（试样S3-1和S3-3、试样S3-4和S3-5）。在相同的轴压（试样S3-2、S3-4和S3-6，以及试样S3-3和S3-5)下，随着冲击气压的增大，峰值应力曲线整体上移，表明峰值应力随冲击气压的增大而增大，而回弹转折点对应的循环冲击次数随冲击气压的增大而减小。同样地，对比图9中试样S3-2和S3-3可以发现，在相同的冲击气压下，最大应变曲线随轴压的增大而整体上移，试样S3-4和S3-5也有相同的变化趋势，表明最大应变随轴压的增大而增大，说明轴压对岩石的承载能力起到削弱效果。另外，回弹转折点所对应的循环冲击次数随轴压的增大而减小，即回弹转折点前移（试样S3-1和S3-3、试样S3-4和S3-5）。在相同的轴压下，随着冲击气压的增大，试样S3-3和S3-5、试样S3-4和S3-6的最大应变曲线具有上移的变化趋势，表明最大应变随冲击气压的增大而增大，而回弹转折点对应的循环冲击次数随冲击气压的增大而减小。
图 9 最大应变与循环冲击次数的关系Figure 9. Relationship between the maximum strain and cyclic impact times普遍而言，多数砂岩试样在经历1～5次冲击后承载能力才会显著下降，说明动态冲击次数不超过某一阈值时，冲击并不会对岩石内部造成实质性损伤，甚至还会提高其动态强度。
以试样S3-2、S3-4、S3-5及S3-6为例，绘制平均应变率随循环冲击次数的关系曲线，如图10所示。在一维应力波理论下，仅考虑试样与入射杆及透射杆之间应力波的一次透反射，有如下关系式[17]
图 10 平均应变率与循环冲击次数的关系Figure 10. Relationship between average strain rate and cyclic impact timesF=ρscs−ρ0c0ρscs+ρ0c0−1<F<0 (4) εr(t)=Fεi(t) (5) εt(t)=(1−F2)εi(t) (6) 式中：
$ {\,\rho _0}{c_0} $ 、${\,\rho _{\rm s}}{c_{\rm s}}$ 分别为压杆和试样的波阻抗，F为试件与入射杆之间的反射波系数。分析认为，在砂岩试样的前几次冲击过程中，由于压密作用，岩石内部微粒错动，内部孔隙与裂缝闭合，微裂纹的闭合量总体上大于冲击造成的损伤量[5]。在以压密作用为主导的情况下，试样变得更加致密，造成试样的波阻抗
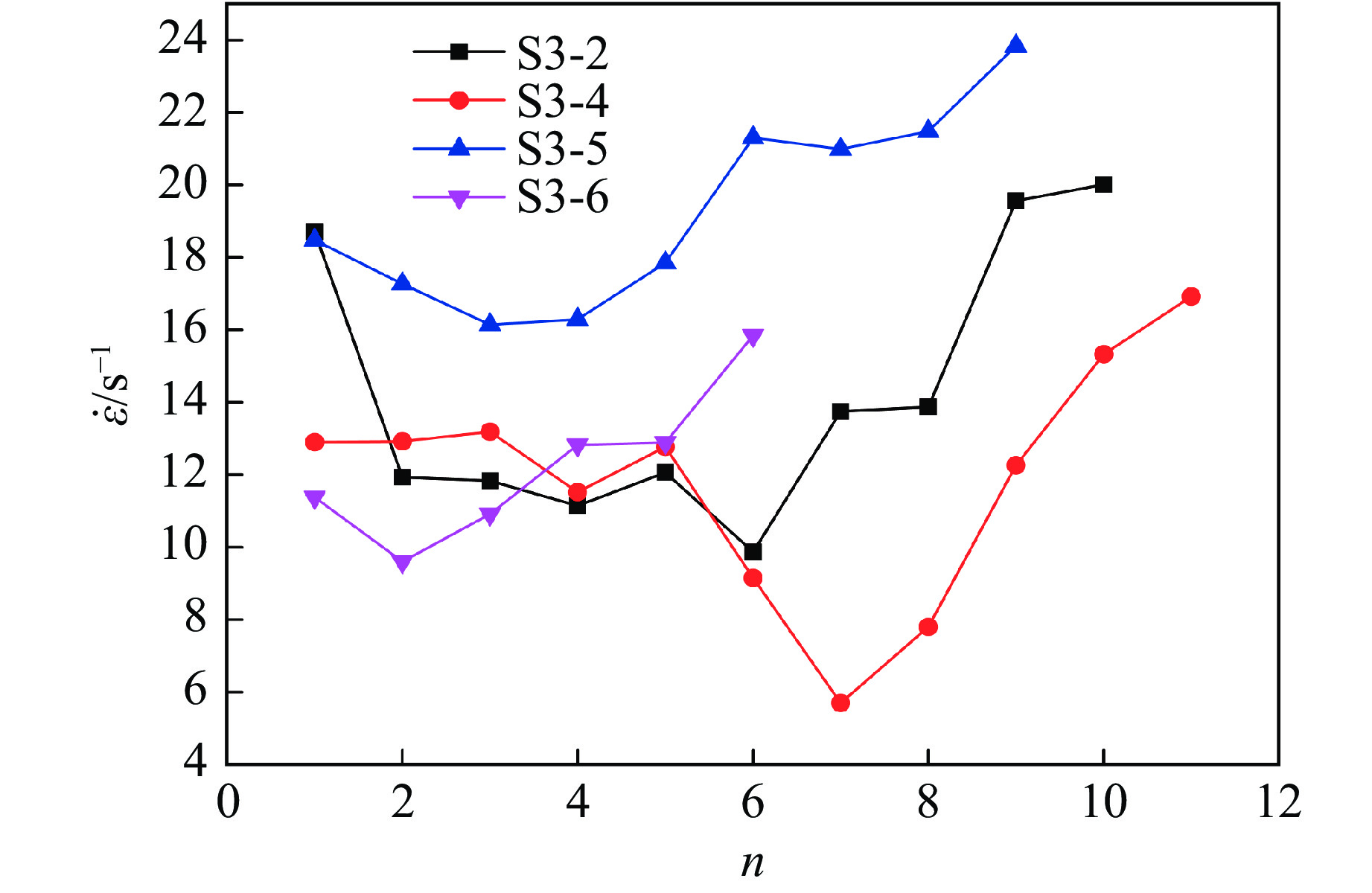
${\,\rho _{\rm s}}{c_{\rm s}}$ 逐渐增大，反射系数F也逐渐增大，当入射波${\varepsilon _{\rm i}}(t)$ 相同时，反射波${\varepsilon _{\rm r}}(t)$ 逐渐减小。同时，由式(3)可知，平均应变率$ {\dot \varepsilon _{\text{s}}}(t) $ 与反射波${\varepsilon _{\rm r}}(t)$ 成正比，因此，多数情况下（试样S3-2、S3-4和S3-5），在前几次冲击过程中，试样的平均应变率逐渐减小，且减小幅度呈逐渐减小趋势，而后趋于稳定，说明压密效果显著，试样的力学性能得以增强。当试样经历几次冲击作用后，其内部裂隙达到最大闭合率，动态强度达到最大，继续冲击加载后，岩石内部开始衍生裂纹，损伤软化效果逐渐加剧，此时试样内部由冲击造成的损伤量大于微裂纹的闭合量，岩石的波阻抗减小。同样地，反射系数F减小，引起反射波${\varepsilon _{\rm r}}(t)$ 逐渐增强，试样的平均应变率随冲击次数的增加而增大，表明岩石内部正在进行裂缝的生成与扩展，力学性能降低，从而形成了如图10所示的平均应变率先减小后增大的变化趋势。对比试样S3-2、S3-4、S3-6可以发现，在相同的轴压（15 MPa）、不同的气压（0.8、1.0、1.2 MPa）下，首次冲击的平均应变率分别为18.7、12.9、11.38 s−1，而后随着冲击气压的增大，砂岩的平均应变率总体呈先减小后增大的趋势。
2.4 冲击气压和轴压对累计循环冲击次数的影响
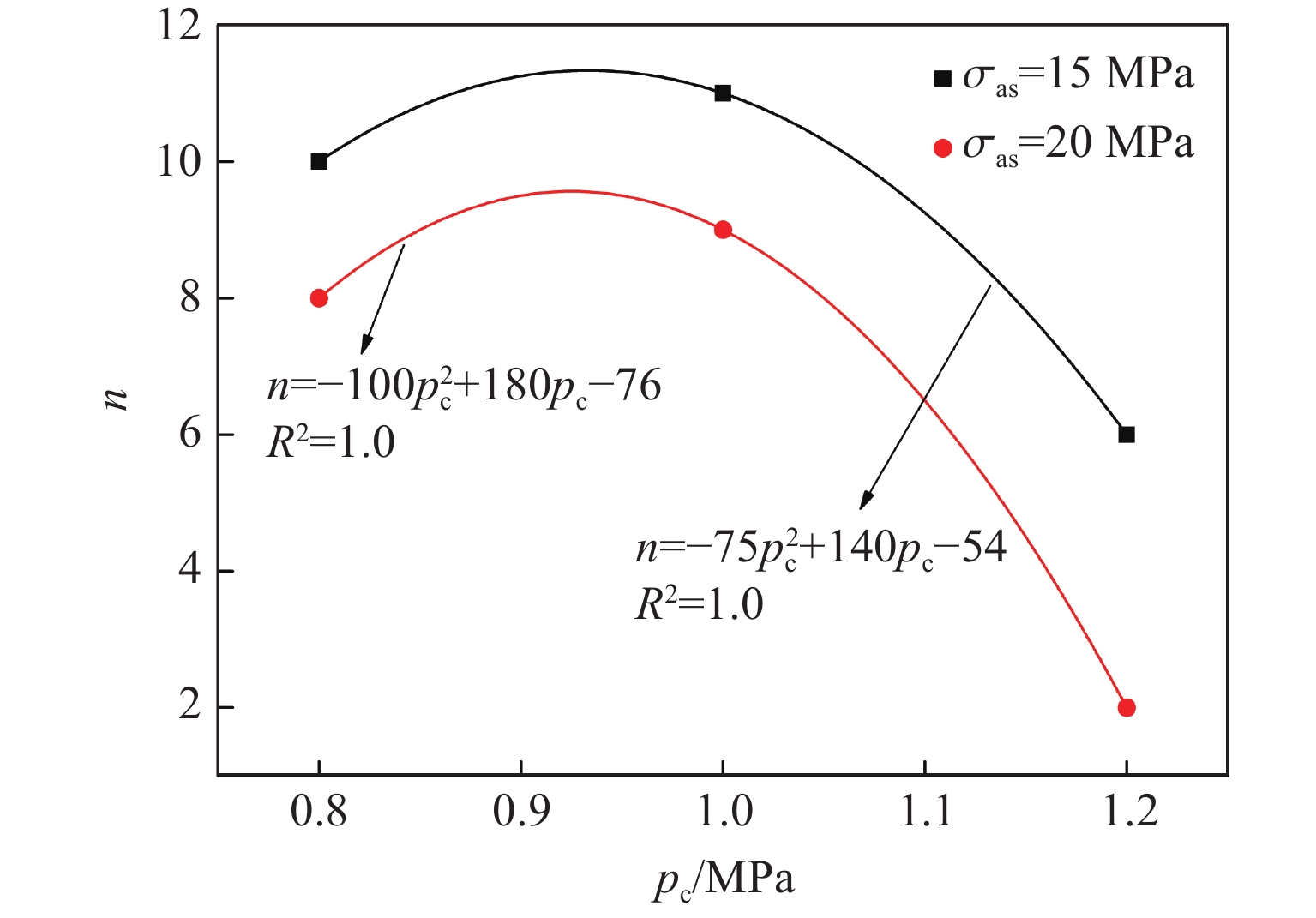
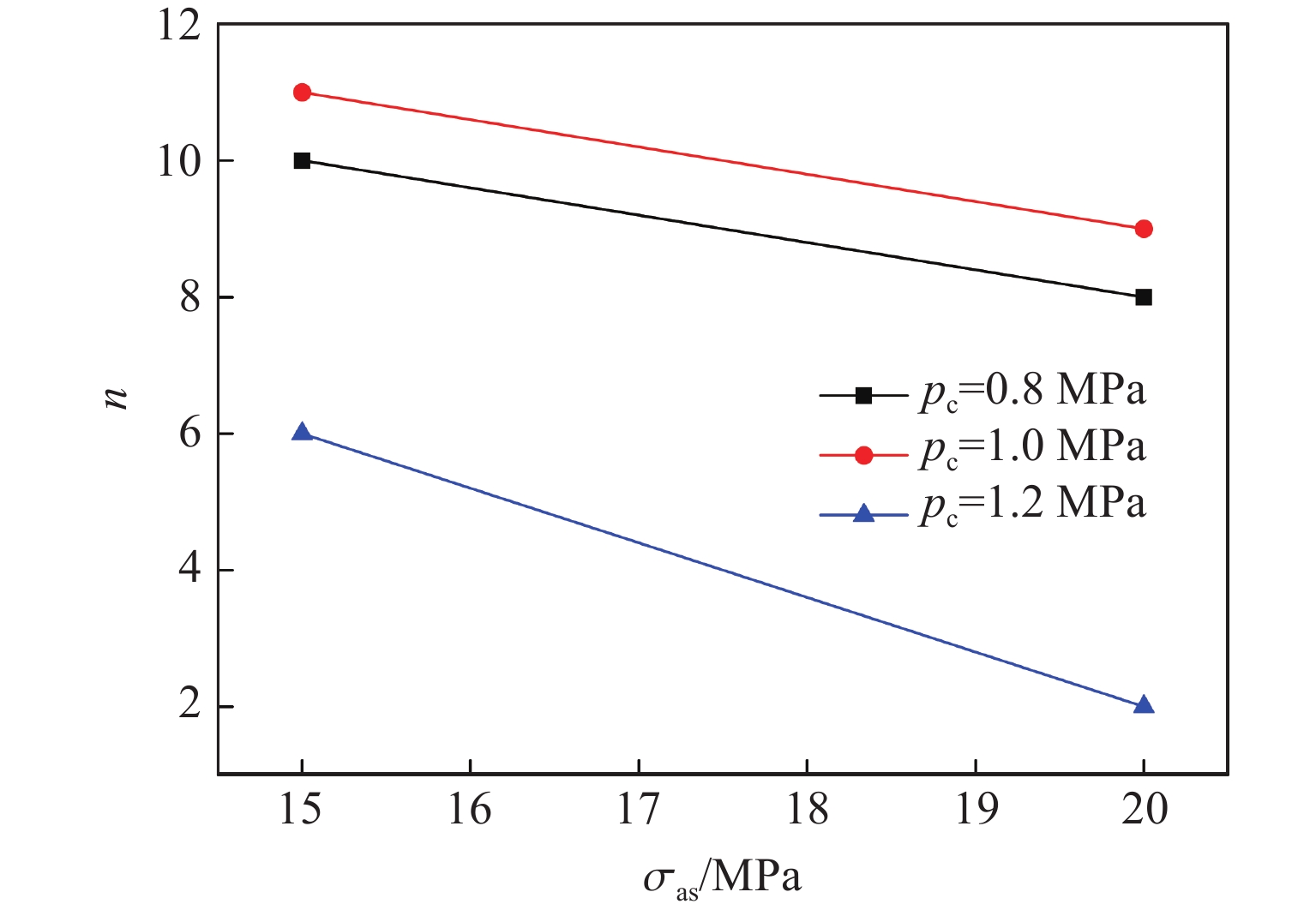
图11显示了砂岩承受的累计循环冲击次数与冲击气压的变化关系。当轴压为15、20 MPa时，对不同冲击气压下试样的累计循环冲击次数进行一元二次多项式回归，二者呈上凸的二次抛物线关系：轴压不变时，砂岩承受的累计冲击次数随冲击气压的增大呈先增大后减小的变化趋势；而当冲击气压不变时，砂岩的累计循环冲击次数随轴压的增大而减小，与唐礼忠等[10]得出的结论一致。同样地，在图12所示的不同冲击气压下轴压对砂岩累计循环冲击次数的影响中可以看出，累计循环冲击次数随轴压的增大而减小；而轴压不变时，累计冲击次数随冲击气压的增大先增大后减小。由此可以大致预测砂岩在爆破冲击下的失稳条件，为深部岩石巷道开挖提供理论依据。
图 12 冲击次数与预加轴压的关系Figure 12. Relationship between impact times and preloading axial pressure2.5 单位体积耗能规律
根据SHPB一维试验原理，由入射波
${\sigma _{\rm i}}(t)$ 、反射波${\sigma _{\rm r}}(t)$ 和透射波${\sigma _{\rm t}}(t)$ 及能量守恒定律，通过下式对能量进行求解Ei=A0C0ρs∫t0σ2i(t)dt (7) Er=A0C0ρs∫t0σ2r(t)dt (8) Et=A0C0ρs∫t0σ2t(t)dt (9) EA=Ei−Er−Et (10) eV=EAVs (11) 式中：
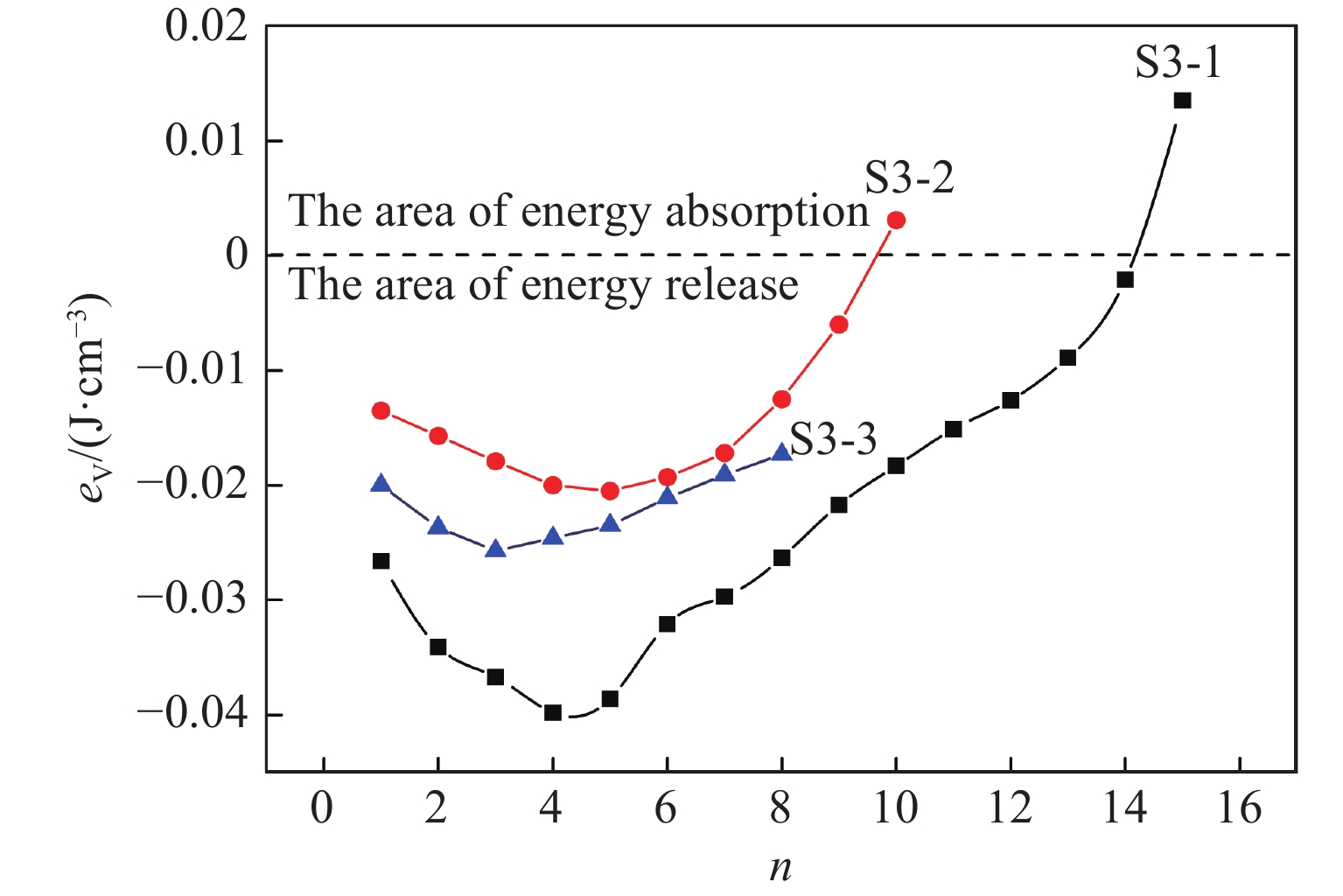
${E_{\rm {i}}}$ 、${E_{\rm {r}}}$ 、${E_{\rm {t}}}$ 及${E_{\rm {A}}}$ 分别为入射能、反射能、透射能和吸收能，J；${\,\rho _{\rm {s}}}$ 为试样密度，kg/m3；$ {e_V} $ 为单位体积耗能，J/cm3；${V_{\rm {s}}}$ 为试样体积，cm3。图13为相同冲击气压、不同轴压下试样的单位体积耗能与循环冲击次数的关系。当轴压为5 MPa时，随着冲击次数的增加，砂岩由释能区向吸能区方向发展；整个循环冲击过程中，释放的能量先增大后减小，随后向吸收能量过渡。分析认为，前几次冲击时，试样内部微裂纹逐渐闭合，随着冲击次数的增加，微裂纹闭合速度减缓，释放的能量逐渐减小，并达到释放能量峰值；随着冲击的持续进行，原本已经闭合以及将要闭合的微裂纹开始劣化，加之新微裂纹产生，不断消耗试样储存的弹性能，且消耗弹性能的速度随着裂纹扩展速度的加快而加快，最终导致试样破坏；当试样释放的能量接近零时，预加轴压产生的弹性能无法满足试样破碎而弹射的弹性能，试样吸收能量补充弹性能以使碎岩弹出。对于预加轴压分别为15、20 MPa的试样S3-2和S3-3，单位体积耗能与循环冲击次数曲线的变化趋势与试样S3-1具有一致性，但就试样S3-3而言，直到循环冲击结束，始终未出现由释能区向吸能区的过渡现象，原因在于试样破碎所消耗的弹性能较少，无法使碎石发生弹射现象。由砂岩的单位体积耗能规律可知，地下深部矿柱失稳大概率是在静载与动载的相互作用下导致的，当静压一定时，动载的频繁扰动是主要诱导因素。
图 13 单位体积耗能与循环冲击次数的关系Figure 13. Relationship between energy consumption per volume and cyclic impact times2.6 砂岩动态变形特性及破坏机理
砂岩试样在整个循环冲击直至破坏的过程中，大致经历3个发展阶段：压密阶段、内部裂纹发展阶段和加速破坏阶段[21]。(1) 压密阶段：此阶段为前几次冲击过程中岩石内部持续演化过程，由于砂岩实体材料内部微粒较大，孔隙较多，在预加轴压和冲击荷载的作用下，微粒与微粒之间发生错动，通过局部变形调整使孔隙填塞（无法完全填塞），在此阶段试样结构更加致密均匀，力学性能有所改善，抵抗外界冲击荷载的能力逐渐增强。(2) 内部裂纹发展阶段：当试块经历多次冲击后，内部裂隙达到最小，强度达到最大，当继续冲击，伴随着损伤微裂纹的发展，轴向裂纹发展尤为明显，此阶段岩石承受动态荷载的能力逐渐减弱，且趋势不断加快。(3) 加速破坏阶段：岩石内部裂纹发展到一定程度，破坏面沿着试块轴向劈裂加速进行，最终产生宏观破碎形态，试样基本失去承载能力。
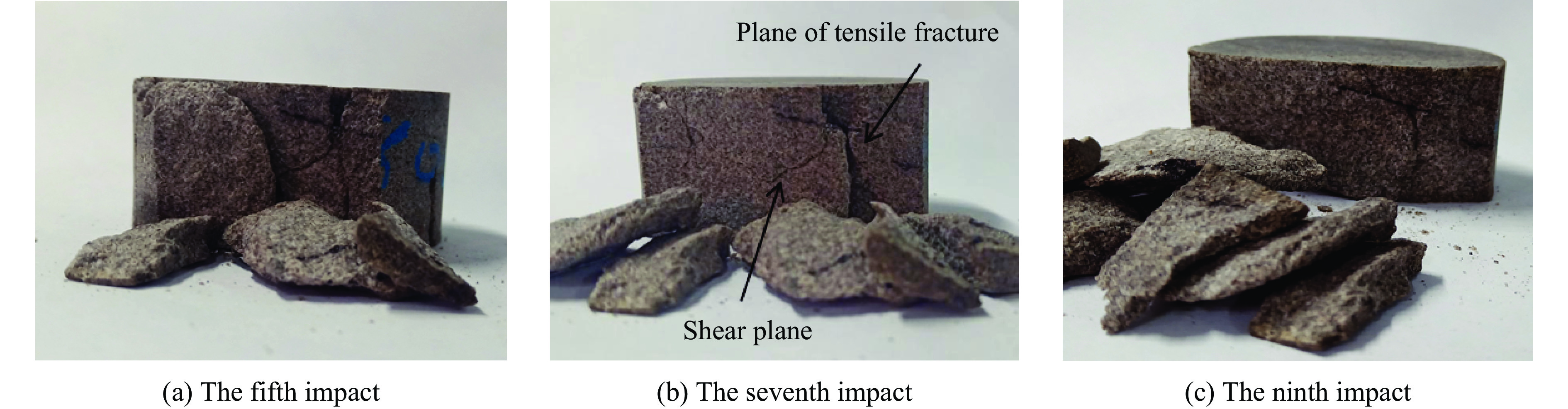
图14显示了试样在循环冲击作用下典型的破坏形态。在单轴单次或循环冲击试验中，黄油的耦合效果很好，有效地减小了端部效应带来的影响，使得试样的破坏模式为沿纵向面的剥离破坏。对于预加轴向静压的一维循环冲击加载，轴压将黄油挤出，试样与各端面之间的黄油所剩无几，端部效应并无明显改善。另外，在轴压加载过程中，试样内部原本的横向裂纹被挤压，轴向产生微裂纹，导致试样尺寸沿横向变大且不均匀，沿纵向变小（变化甚微）；由于入/透射杆与试样的材质不同，试样横向尺寸的变化将产生较大的摩擦力，试样横向外侧变形被限制，从而成为端部效应的又一助长因素（由于轴压经透射杆持续施加于试样，因此该效应只对透射界面端部有较大的影响）。由此可以发现，破坏面是沿着试样轴向倾斜发展的，即试样入射面破坏较大，透射面破坏相对较小。
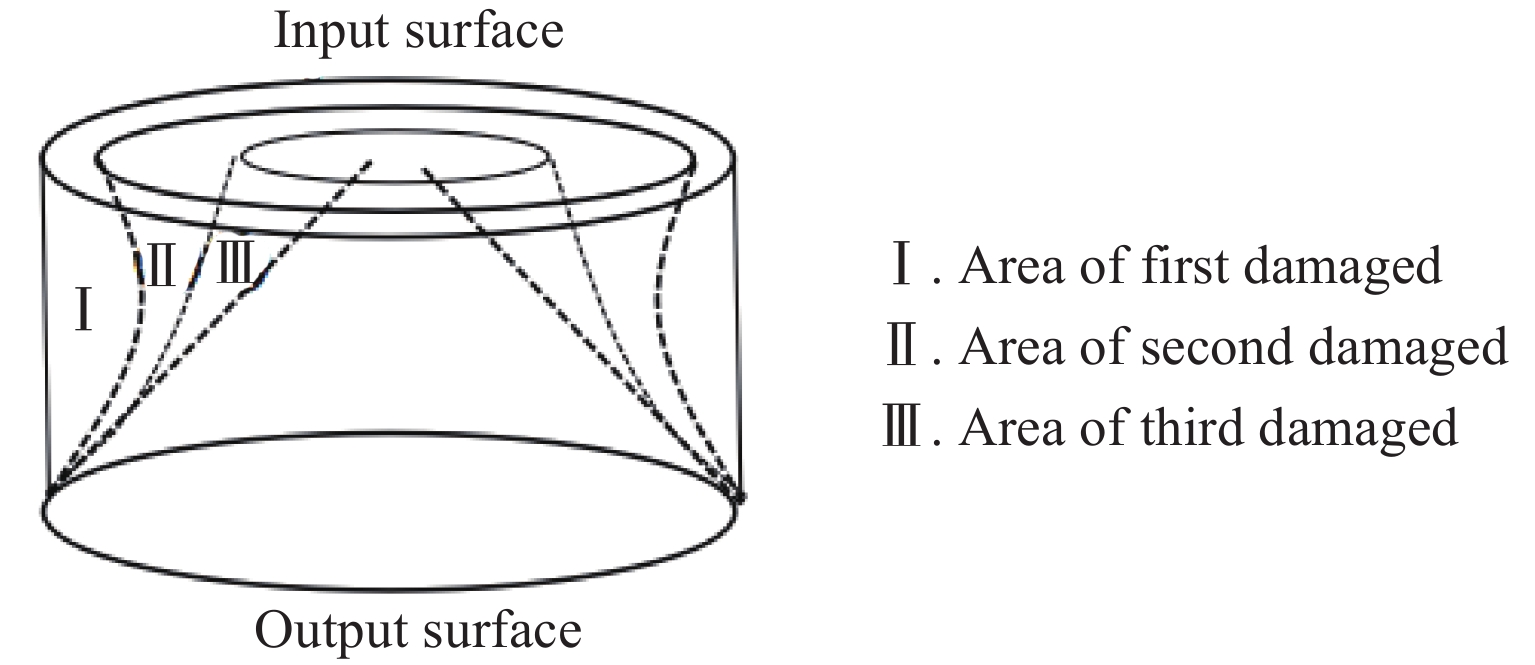
图 14 循环冲击作用下砂岩的破坏形态Figure 14. Failure morphology of sandstone under cyclic impact loads当入射杆的应力波到达入射界面时，入射杆与试样材质的差异以及试样内部孔隙、裂缝的随机分布使入射波发生透射和反射，反射波到达入射界面以及透射波到达透射界面又会进行下一阶段的反射和透射，如此循环往复，对试样造成动态疲劳破坏。试样被冲击压缩时，与空气接触的圆周面因位于临空面而不受横向约束，越往试样内部，横向约束越大，导致横向变形越发困难，从而形成了如图15所示的试样破坏。图15中共轭双曲破坏面形成的主要原因是端部的约束效应，另一个原因则是临空面的无约束作用，当最外侧（图15中区域Ⅰ）破碎剥落时（前一次破坏为后一次破坏提供补偿空间），由于应力波的速度远大于裂纹的张裂速度，导致试样内侧部分因快速压缩而与外侧将要剥落部分发生摩擦，即形成剪切力。因此，砂岩最终的宏观破碎是由张裂破坏与剪切破坏共同导致的。
3. 结 论
通过对硬岩巷道砂岩开展一维动静组合循环冲击加载试验，分析了砂岩的应力-应变曲线特征、冲击气压和预加轴压对循环冲击次数的影响、能量耗散规律以及砂岩的破坏机制，得出以下主要结论。
(1) 循环冲击过程中，砂岩的应力-应变曲线出现整体回弹现象，即随着冲击次数的增加，砂岩的峰值应力先增大后减小，而峰值应变、最大应变以及平均应变率则呈现相反的变化趋势。
(2) 在相同的冲击气压下，砂岩的峰值应力及承受的累计循环冲击次数随轴压的增大而减小；在相同的预加轴压下，砂岩的峰值应力随冲击气压的增大而增大，但承受的累计冲击次数却随冲击气压的增大先增大后减小。
(3) 根据应力-应变曲线和平均应变率随循环冲击次数的变化规律，可将砂岩的整个循环冲击过程分为压密阶段、内部裂纹发展阶段以及加速破坏阶段，对应于释放能先增后减以及向吸收能过渡的发展趋势。
(4) 一维动静组合循环冲击试验证明了轴压的施加对微元体的力学性能起到劣化作用，砂岩体会发生由张裂和剪切共同作用的宏观破坏模式。
-
图 5 落锤高度为400 mm时边界倒角对固支方板毁伤的影响
Figure 5. Dynamic behavior of square plates under boundary conditions with 400 mm hammer height
图 6 落锤高度为500 mm时边界倒角对固支方板毁伤的影响
Figure 6. Dynamic behavior of square plates under boundary conditions with 500 mm hammer height
图 7 落锤高度为700 mm时边界倒角对固支方板毁伤的影响
Figure 7. Dynamic behavior of square plates under boundary conditions with 700 mm hammer height
图 8 不同边界倒角半径和落锤高度对固支方板中心位移的影响
Figure 8. Center displacement of square plates under different boundary chamfer radius and hammer height
图 9 落锤高度为500 mm时边界倒角半径对固支方板中心位移的影响曲线
Figure 9. Center displacement curves of plates under different boundary chamfer radius with 500 mm hammer height
图 10 落锤高度为700 mm时边界倒角半径对固支方板中心位移的影响曲线
Figure 10. Center displacement curves of plates under different boundary chamfer radius with 700 mm hammer height
图 11 边界倒角半径对固支方板裂缝总长度的影响
Figure 11. Total crack length of square plates underdifferent boundary chamfer radius
图 12 边界倒角半径对固支方板长裂缝长度的影响
Figure 12. Longest crack length of square plates underdifferent boundary chamfer radius
图 13 落锤高度为400 mm时不同倒角半径固支方板的仿真计算结果
Figure 13. Simulation results of square plates under different boundary chamfer radius with 400 mm hammer height
图 14 不同倒角下固支方板最大塑性应变点的应力状态
Figure 14. Stress triaxility of the max PEEQ element of square plates under different boundary chamfer radius
表 1 试验工况及试验结果
Table 1. Experiment cases and experiment results
Experiment
caseSpecimen No. R/mm h/mm v/(m·s−1) M/kg E/kJ Experiment results D/mm Failure mode 1 S6-1 6 400 2.80 2 024 7.934 41.2 One-side tearing 2 S6-2 6 500 3.13 2 024 9.917 45.7 Double-side tearing 3 S6-3 6 600 3.42 2 024 11.901 52.0 One-side tearing 4 S6-4 6 700 3.70 2 024 13.884 56.4 Double-side tearing 5 S9-1 9 400 2.80 2 024 7.934 39.3 Plastic deformation 6 S9-2 9 500 3.13 2 024 9.917 45.9 One-side tearing 7 S9-3 9 600 3.42 2 024 11.901 48.6 Double-side tearing 8 S9-4 9 700 3.70 2 024 13.884 55.8 Double-side tearing 9 S12-1 12 400 2.80 2 024 7.934 39.8 Plastic deformation 10 S12-2 12 500 3.13 2 024 9.917 44.6 One-side tearing 11 S12-3 12 600 3.42 2 024 11.901 51.1 One-side tearing 12 S12-4 12 700 3.70 2 024 13.884 56.9 One-side tearing 
下载: 导出CSV
表 2 不同工况下固支方板的裂缝长度
Table 2. Crack length of square plates under different experiment cases
Experiment
caseSpecimen
No.R/mm h/mm Failure mode Crack length/mm Crack 1 Crack 2 1 S6-1 6 400 One-side tearing 207 2 S6-2 6 500 Double-side tearing 216 125 3 S6-3 6 600 One-side tearing 290 4 S6-4 6 700 Double-side tearing 294 184 5 S9-1 9 400 Plastic deformation 6 S9-2 9 500 One-side tearing 195 7 S9-3 9 600 Double-side tearing 250 213 8 S9-4 9 700 Double-side tearing 184 132 9 S12-1 12 400 Plastic deformation 10 S12-2 12 500 One-side tearing 170 11 S12-3 12 600 One-side tearing 230 12 S12-4 12 700 One-side tearing 280
下载: 导出CSV
表 3 最大塑性应变单元结果对比
Table 3. Comparison of the max PEEQ results
Experiment
caseR/mm h/mm Plastic deformation Stress triaxility PEEQ $\varepsilon_{11} $ $\varepsilon_{33} $ $\varepsilon_{13} $ 1 6 400 0.241 –0.137 0.139 0.268 [0.5, 0.6] 5 9 400 0.191 –0.133 0.130 0.219 [0.5, 0.6] 9 12 400 0.185 –0.141 0.133 0.210 [0.5, 0.6]
下载: 导出CSV
-
[1] NURICK G N, SHAVE G C. The deformation and tearing of thin square plates subjected to impulsive loads—an experimental study [J]. International Journal of Impact Engineering, 1996, 18(1): 99–116. doi: 10.1016/0734-743X(95)00018-2 [2] RAMAJEYATHILAGAM K, VENDHAN C P. Deformation and rupture of thin rectangular plates subjected to underwater shock [J]. International Journal of Impact Engineering, 2004, 30(6): 699–719. doi: 10.1016/j.ijimpeng.2003.01.001 [3] NURICK G N, RADFORD A M. Deformation and tearing of clamped circular plates subjected to localised central blastloads [C]//REDDY B D. Recent developments in computational and applied mechanics. A Volume in Honour of John B Martin, 1997: 276–301. [4] RAJENDRAN R, NARASIMHAN K. Damage prediction of clamped circular plates subjected to contact underwater explosion [J]. International Journal of Impact Engineering, 2001, 25(4): 373–386. doi: 10.1016/S0734-743X(00)00051-8 [5] JACOB N, YUEN S C K, NURICK G N, et al. Scaling aspects of quadrangular plates subjected to localised blast loads—experiments and predictions [J]. International Journal of Impact Engineering, 2004, 30(8/9): 1179–1208. [6] LONGÈRE P, GEFFROY-GRÈRE A-G, LEBLÉ B, et al. Ship structure steel plate failure under near-filed air-blast loading: numerical simulations vs experiment [J]. International Journal of Impact Engineering, 2013, 62: 88–98. [7] 李典, 郑羽, 陈长海, 等. 空爆载荷下舰船典型结构损伤研究进展 [J]. 船舶力学, 2020, 24(4): 543–557. doi: 10.3969/j.issn.1007-7294.2020.04.015LI D, ZHENG Y, CHEN C H, et al. Review on damage of typical ship protective structures under explosion load [J]. Journal of Ship Mechanics, 2020, 24(4): 543–557. doi: 10.3969/j.issn.1007-7294.2020.04.015 [8] JACOB N, NURICK G N, LANGDON G S. The effect of stand-off distance on the failure of fully clamped circular mild steel plates subjected to blast loads [J]. Engineering Structure, 2007, 29(10): 2723–2736. doi: 10.1016/j.engstruct.2007.01.021 [9] TEELING-SMITH R G, NURICK G N. The deformation and tearing of thin circular plates subjected to impulsive loads [J]. International Journal of Impact Engineering, 1991, 11(1): 77–91. doi: 10.1016/0734-743X(91)90032-B [10] SHEN W Q, JONES N. Dynamic response and failure of fully clamped circular plates under impulsive loading [J]. International Journal of Impact Engineering, 1993, 13(2): 259–278. doi: 10.1016/0734-743X(93)90096-P [11] BONORCHIS D, NURICK G N. The influence of boundary conditions on the loading of rectangular plates subjected to localised blast loading—importance in numerical simulations [J]. International Journal of Impact Engineering, 2009, 36(1): 40–52. doi: 10.1016/j.ijimpeng.2008.03.003 [12] NURICK G N, GELMAN M E, MARSHELL N S. Tearing of blast loaded plates with clamped boundary conditions [J]. International Journal of Impact Engineering, 1996, 18(7/8): 803–827. [13] MENKES S B, OPAT H J. Broken beams—tearing and shear failures in explosively loaded clamped beams [J]. Experimental Mechanics, 1973, 13(11): 480–486. doi: 10.1007/BF02322734 [14] BAO Y B, WIERZBICKI T. On fracture locus in the equivalent strain and stress triaxiality space [J]. International Journal of Mechanical Sciences, 2004, 46(1): 81–98. doi: 10.1016/j.ijmecsci.2004.02.006 [15] TENG X, WIERZBICKI T. Evaluation of six fracture models in high velocity perforation [J]. Engineering Fracture Mechanics, 2006, 73(12): 1653–1678. doi: 10.1016/j.engfracmech.2006.01.009 [16] BAO Y B, WIERZBICKI T. On the cut-off value of negative triaxiality for fracture [J]. Engineering Fracture Mechanics, 2005, 72(7): 1049–1069. doi: 10.1016/j.engfracmech.2004.07.011 -
下载:
下载:
点击查看大图
计量
- 文章访问数: 5662
- HTML全文浏览量: 2810
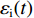
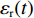
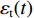
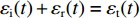
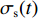
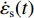
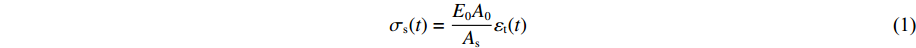
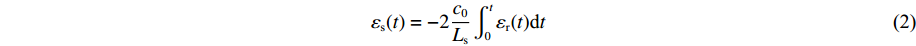
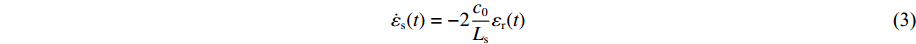
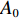
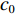
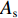
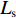
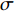
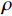
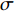
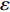
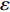
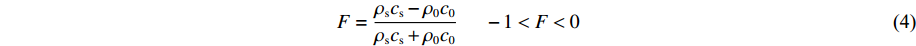
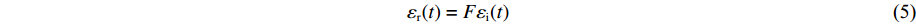
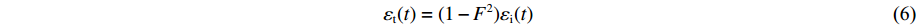
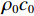
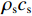
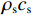
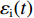
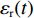
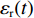
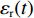
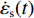
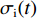
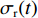
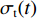
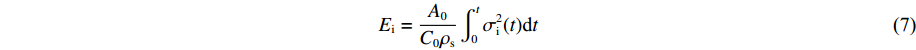
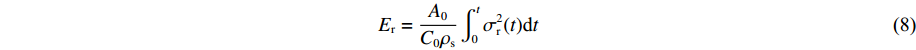
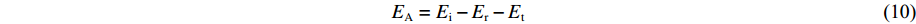
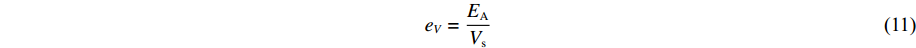
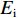
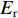
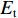
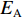
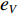
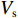
- PDF下载量: 43
