Dynamic Responses of Nare-Like Voronoi Structure under Impact Loading
-
摘要: 贝壳珍珠层复合结构是一种有效的抗压结构系统,微观上具有Voronoi随机结构,具有良好的力学特性。为了研究仿贝壳珍珠层Voronoi结构在冲击载荷下的动态力学响应,建立了一种铝/乙烯基复合材料的三维Voronoi模型。首先,应用随机Voronoi技术建立仿贝壳珍珠层Voronoi随机模型,然后在随机多边形铝片之间引入黏结层,模拟黏结和分层过程,从最大变形、损伤分布和耗散能量等方面探讨Voronoi片板模型在弹丸冲击荷载作用下的力学性能,并与规则片板模型进行对比分析。结果显示:Voronoi结构更有利于冲击能量的扩散与吸收,减小应力集中,更好地发挥能量共享机制;而规则模型的冲击损伤主要集中在弹丸冲击点附近区域。最后讨论了黏结层厚度和分块尺寸对Voronoi模型力学性能的影响,结果表明:分块尺寸对Voronoi模型抗冲击性能的影响很小;黏结层对损伤耗散能和塑性能的影响很明显,黏结层越薄,模型的抗冲击性能越好。Abstract: As an effective structure system for resisting the compression, the nacre composite structure has random Voronoi structure at a microscopic level and good mechanical performance. In this study, a three-dimensional Voronoi model consisting of aluminum/vinyl composite structure was presented to investigate the dynamic responses of nare-like Voronoi model under impact loading. Firstly the stochastic Voronoi model was built using the Voronoi technology, and then the adhesive layers were introduced between random polygonal aluminium sheets to simulate the bonding and delaminating processes. Via the maximum deformation, damage distribution and dissipation energy, the mechanical performances of Voronoi plate model were evaluated and compared with those of the regular plate model. The results showed that the Voronoi model is in favor of energy spreading and absorbing, reducing the stress concentration and making the energy sharing mechanism work better. But the impact damage of the regular plate model is concentrated near the impacting point of the bullet. Finally the influences of adhesive thickness and block size on mechanical performances were discussed. It was indicated that the block size has little effect on the impact resistance of the Voronoi model while the adhesive thickness exerts significant influence on the damage dissipation energy and plastic energy. It was found that the thinner is the adhesive thickness, the better is the impact resistance performance of the model.
-
Key words:
- nacre /
- Voronoi structure /
- dynamic response /
- impact loading /
- finite element analysis
-
仿贝壳珍珠层复合材料作为新型复合材料,有着优异的力学性能,不仅具有很高的强度,而且具有很好的韧性,近年来引起了学术界的不断关注[1-6]。很多发达国家非常重视贝壳结构材料和仿生材料研究,如美国等国家设置了专门的经费来研究贝壳生物材料的仿生设计和性能,用于装甲防弹衣和防爆装置。贝壳珍珠层复合材料的优异力学性能与其微观结构密切联系,为此研究人员对珍珠质的微观结构特征(体积分数、片剂长宽比、重叠长度等)进行了深入分析,试图将其与模型的力学性能联系起来[7-12]。Dutta等[13]研究了珍珠层中裂纹的萌生规律,评估了重叠长度对裂纹尖端驱动力的影响。Kotha等[14]的研究显示,低纵横比的文石片可以制造出具有高韧性的复合材料。Barthelat等[15]发现,珍珠层没有实现稳定状态的裂纹扩展,并将其归因于片层拔出增韧机制。其他学者也发现珍珠层内部和外部韧化机制阻止了裂纹的扩展[16-21]。
Barthelat等[15]通过观察发现,在每层贝壳珍珠层中,平板的排列与Voronoi图相似,从一个红色鲍鱼标本的光学图像中可以看到每个贝壳层压板都有矿物片的随机分布,并与其他珍珠层成键。基于这些光学图像,他们生成了一个由两层贝壳的平板结构组成的几何模型,用于有限元分析。自1907年Shamos和Hoey提出分治算法的最初定义和描述之后,Voronoi图便成为众多学科的中心主题之一。Voronoi图所具有的自然描述性和操纵能力,使其获得了广泛应用[22-24]。尽管Voronoi图对科学和工程中的各种应用具有重大的潜在影响,但是在很多领域包括仿生结构领域,Voronoi结构对材料力学性能的影响还未得到透彻的理解,为此本工作将探讨Voronoi结构的随机性对仿贝壳珍珠层结构力学性能的影响。
为了研究仿贝壳珍珠层Voronoi随机模型结构的动态力学响应,首先建立一种铝/乙烯基复合材料结构的三维Voronoi模型,然后对模型在弹丸冲击载荷下的动态力学性能进行有限元模拟分析,最后讨论黏结层厚度和Voronoi模型分块尺寸对模型抗冲击力学性能的影响。
1. Voronoi随机模型生成
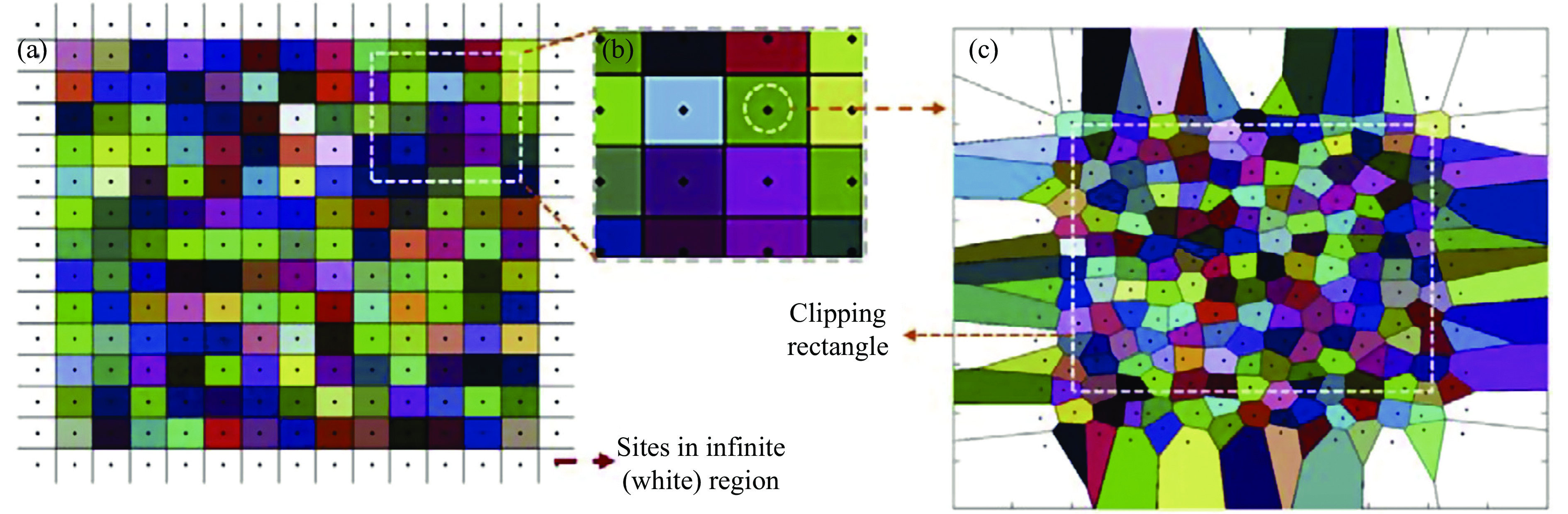
利用文献[25]给出的随机Voronoi技术生成Voronoi随机模型。图1描述了仿贝壳珍珠层随机Voronoi结构的生成技术。图1(a)显示了由网格组成的Voronoi初始构型,每个网格内都包含1个站点;站点可以在圆内随机移动,如图1(b)所示,站点位置
(x,y) 由极坐标控制方程决定 图 1 (a) Voronoi图的初始网格构型;(b)每个站点都在一个圆圈区域内随机移动;(c)新的Voronoi图是从新的站点系统中生成,通过矩形裁剪,形成有限区域的随机Voronoi图[25]Figure 1. (a) Initial grid formation of Voronoi diagram; (b) each site moves randomly within a circle region; (c) a new Voronoi diagram is generated from the new site system, and by a rectangle cut, a finite Voronoi diagram is generated[25]
图 1 (a) Voronoi图的初始网格构型;(b)每个站点都在一个圆圈区域内随机移动;(c)新的Voronoi图是从新的站点系统中生成,通过矩形裁剪,形成有限区域的随机Voronoi图[25]Figure 1. (a) Initial grid formation of Voronoi diagram; (b) each site moves randomly within a circle region; (c) a new Voronoi diagram is generated from the new site system, and by a rectangle cut, a finite Voronoi diagram is generated[25]x=x0+rcosθ,y=y0+rsinθ (1) 式中:
(x0,y0) 为网格内站点的参考位置;r 为(0,R) 之间的随机值,R 为圆的半径;θ 为(0,2π) 之间的随机值。通过站点的随机移动,最终形成新的随机Voronoi图,如图1(c)所示。新生成的Voronoi图由一个无限大的区域组成,采用矩形切割使新生成的Voronoi图限定在有限区域内,丢弃有限区域外的站点和多边形网格,最终形成仿贝壳珍珠层随机Voronoi模型。2. Voronoi模型有限元分析
2.1 几何建模
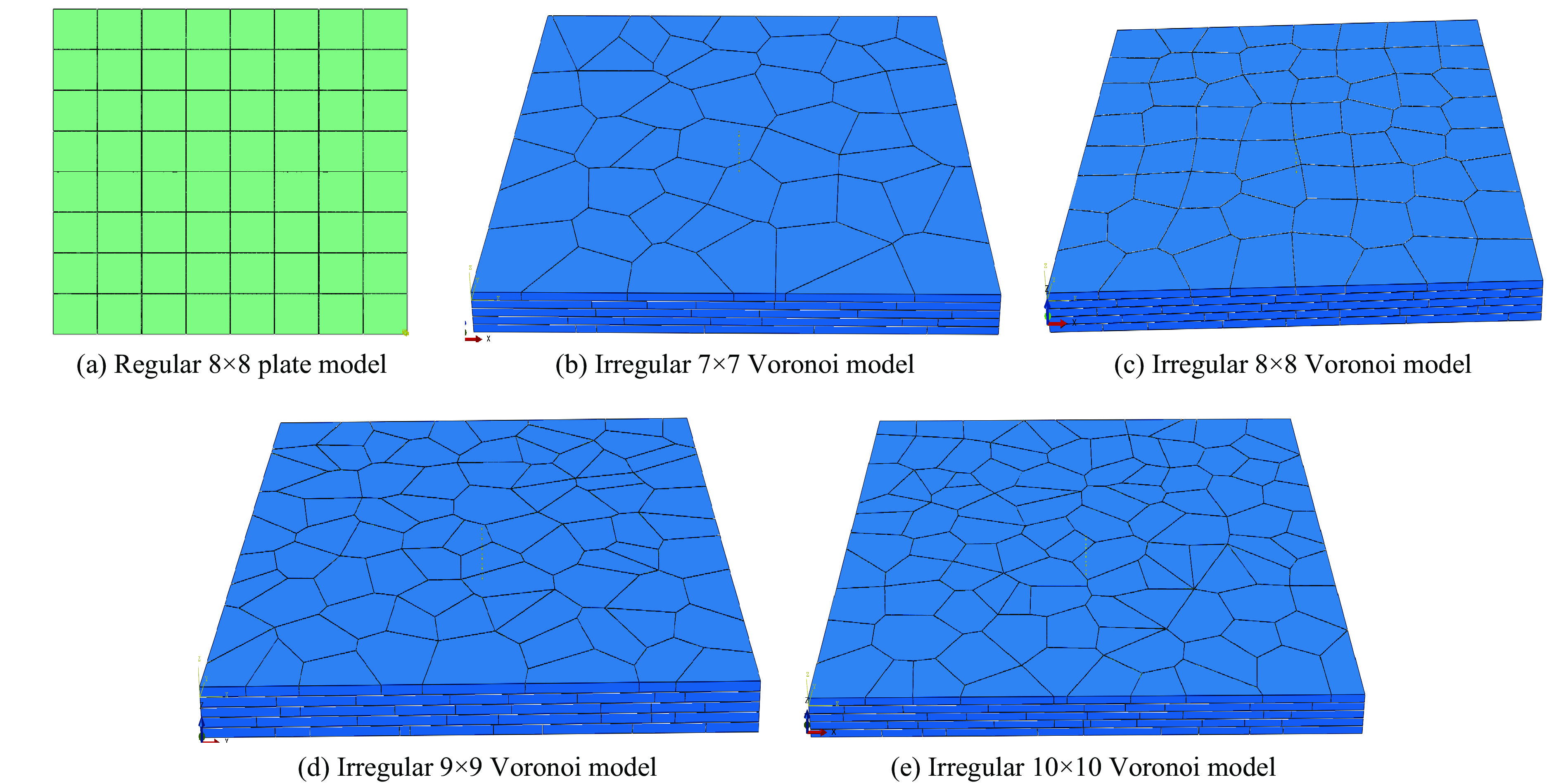
图2给出了规则片板单元模型以及4种不同分块尺寸的不规则Voronoi片板模型,5种模型的总体几何尺寸相同,均为240 mm × 240 mm × 15 mm,模型总层数均为5层。图2(a)为规则片板模型,每个规则片板的几何尺寸为30 mm × 30 mm × 3 mm,每层由8 × 8共64个片板组成,图2(b)~图2(e)分别给出了7 × 7、8 × 8、9 × 9和10 × 10分块的Voronoi不规则模型,每种模型均包括5层不同的随机单层结构,每层厚度为3 mm。
为了模拟仿贝壳珍珠层片层之间受冲击破坏时的脱黏现象,采用了内聚力Cohesive模型。通过合理的参数选择,内聚力Cohesive模型能够部分描述贝壳珍珠层内部层与层之间的变形和失效现象[2]。在片板之间以及板层之间插入Cohesive黏结层,考虑3种黏结层厚度0.1、0.2和0.3 mm,讨论黏结层厚度对模型冲击损伤的影响。
内聚力Cohesive模型的牵引分离定律涉及黏性牵引应力矢量
T={tn,ts,tt} ,其中下标n、s和t分别表示一个法向和两个切向分量。这些变量之间满足双线性二次黏聚律[25](tnt0n)2+(tst0s)2+(ttt0t)2=1 (2) 式中:
t0n 、t0s 、t0t 分别表示变形垂直于界面以及在第一、第二剪切方向上的最大应力。Cohesive黏结层刚度退化速率满足
(GnG0n)2+(GsG0s)2+(GtG0t)2=1 (3) 式中:
Gn 、Gs 、Gt 分别为正向和两个切向的断裂能,G0n 、G0s 、G0t 分别为正向和两个切向引起破坏所需的最大断裂能。2.2 材料参数
仿贝壳珍珠层三维Voronoi结构模型包括两种材料模型,其中片层采用铝AA5083-H116,片层之间Cohesive黏性层使用乙烯树脂材料。表1和表2列出了两种材料参数[19, 25],其中
ρ 为密度,ν 为泊松比,E为弹性模量,Es和Et为两个切向弹性模量。表 1 铝片的材料参数Table 1. Parameters of aluminum plateMaterial ρ/(kg·m−3) ν E/GPa Aluminum 2750 0.3 72 表 2 Cohesive模型的材料参数Table 2. Parameters of Cohesive modelt0n/MPa t0s/MPa t0t/MPa G0n/(kJ·m−2) G0s/(kJ·m−2) G0t/(kJ·m−2) ρ/(kg·m−3) Es/GPa Et/GPa 80 80 80 1 1 1 1 850 4 1.5 在大应变情况下,铝合金的本构关系可采用Johnson-Cook模型描述
σ=(A+Bεn)(1+Cln˙ε˙ε0)[1−(T−TrT−Tm)m] (4) 式中:
σ 为等效应力,A 为材料在常温准静态下的屈服强度,B 为应变强化系数,n 为强化指数,ε 为等效塑性应变,˙ε 为塑性应变率,˙ε0 为初始应变率,C 为应变率敏感系数,m 为温度软化系数,Tm 为材料的熔点,Tr 为参考温度(取常温),T 为当前温度。表3列出了Johnson-Cook模型参数[25]。2.3 加载条件与边界条件
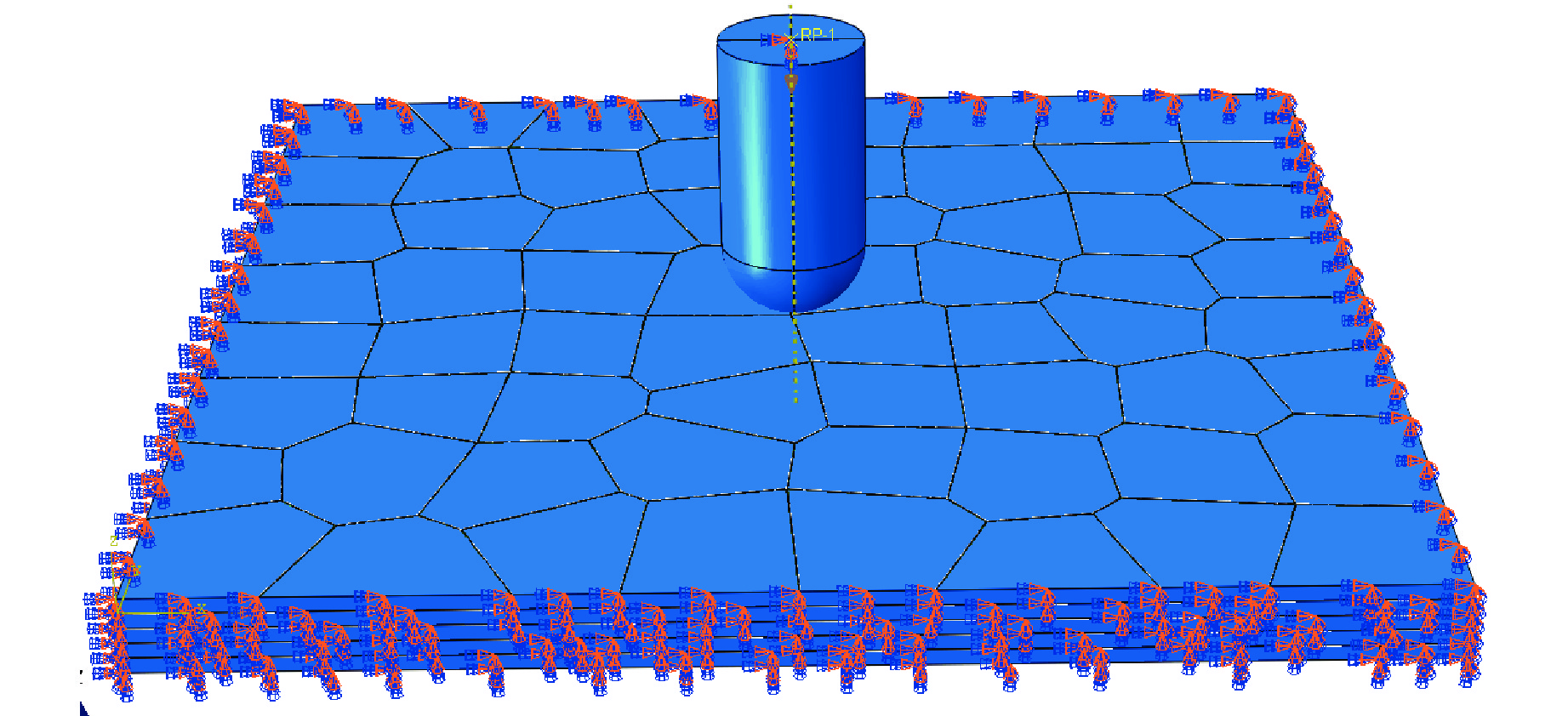
图3显示了模型的边界条件和加载条件。弹丸以18 m/s的初速度冲击Voronoi模型,弹丸速度属于中低速范围。弹丸模型上半部分是一个半径15 mm、长45 mm的圆柱体,下半部分是一个半径为15 mm的半球体,总长为60 mm,刚体属性。冲击载荷下仿贝壳珍珠层三维Voronoi模型的边界条件为4个侧边均完全固定,弹丸与复合结构模型的接触为通用接触,弹丸作用在复合结构模型中心。
黏结层网格类型采用COH3D8,铝片网格类型采用C3D8R,黏结层网格大小为1 mm,铝片网格大小为2 mm。在该网格密度下,模型的网格单元总数达到167230。节点总数为567001时,最大应力值保持稳定,网格的收敛性较好。
3. 模拟结果分析
3.1 有限元分析
在珍珠层结构中,片板滑动机制被认为是激活内在和外在韧化机制的关键因素,可以阻止裂纹扩展。该机制分别引起内聚力和残余塑性应变,从而闭合裂纹。由于弹丸冲击载荷方向垂直于Voronoi板模型,冲击载荷破坏的主要形式是黏性层剥离,因此片板滑动引起的增韧机制在这种特定的冲击加载问题中不占主导地位。在冲击载荷下,损伤和变形耗散的能量比摩擦接触要多得多,Voronoi模型对珍珠层结构负载分配和能量吸收机制的影响是所要考虑的主要因素。本研究首先分析冲击载荷下模型的动态响应,在此基础上考察不规则Voronoi片板等几何因素对动态力学特性和能量分配的影响。
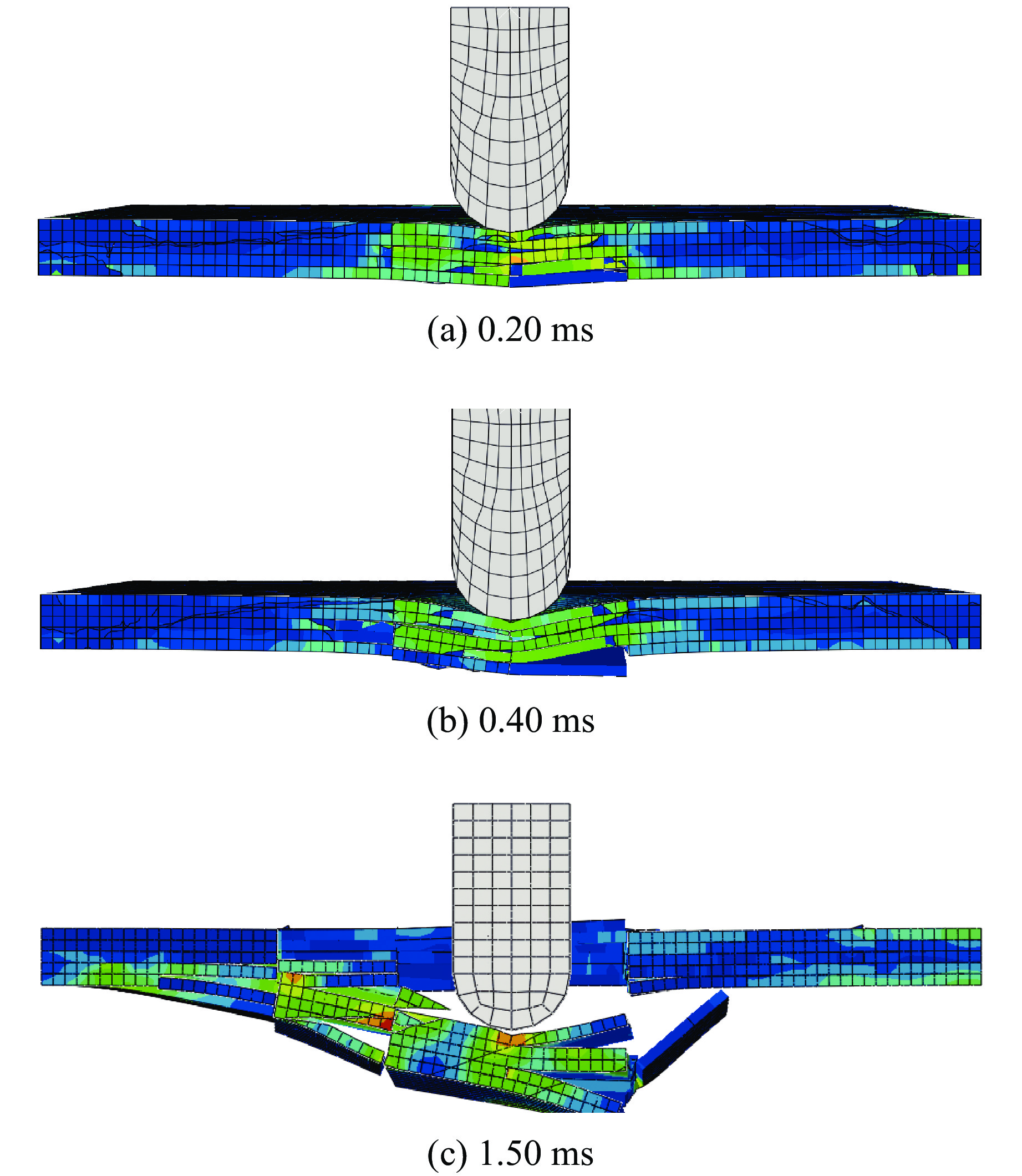
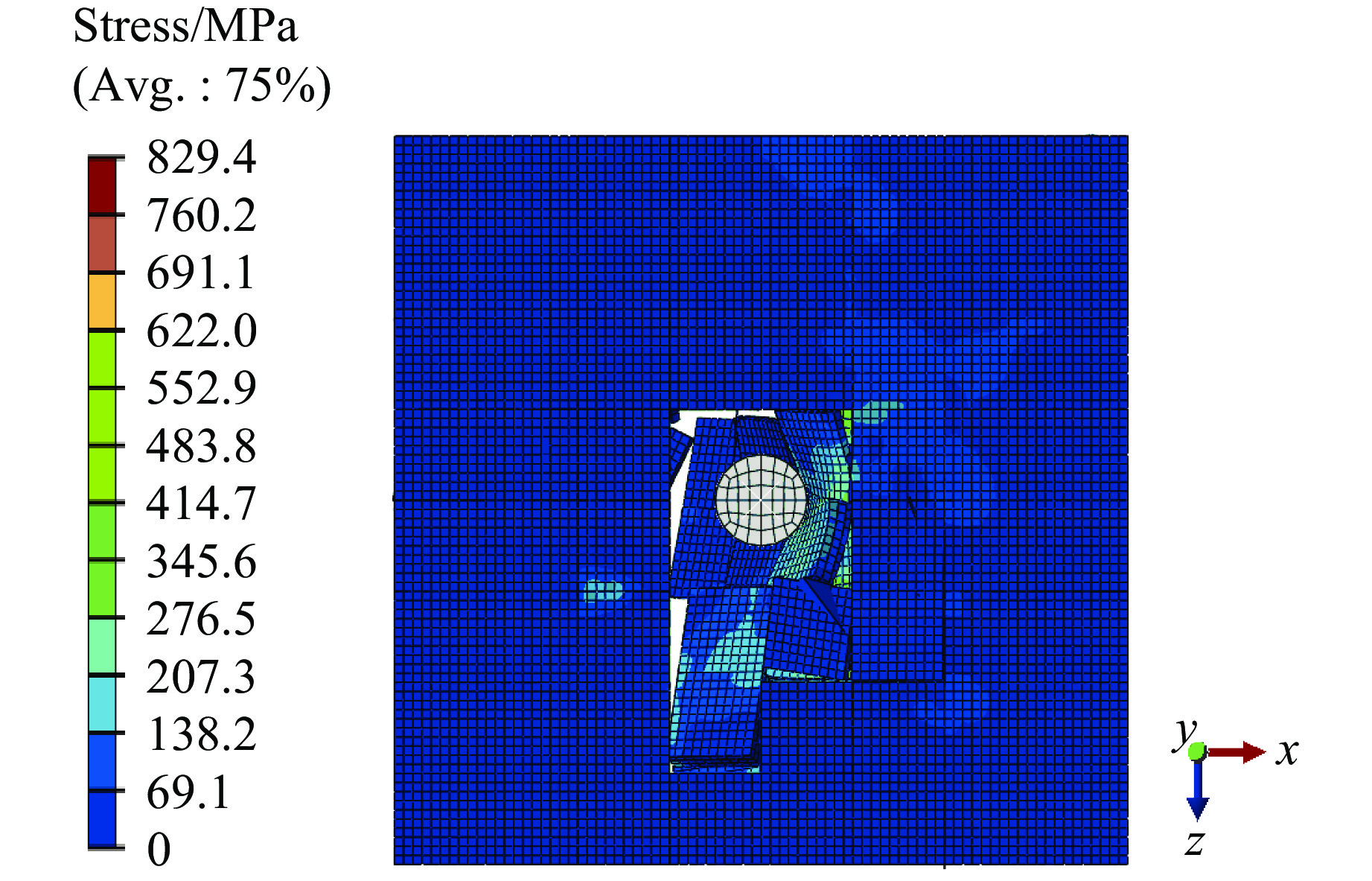
图4和图5分别为不同时刻规则片板模型与Voronoi片板模型受弹丸冲击时的应力云图剖视图。通过对比可以发现:在规则模型中,应力主要集中在弹丸冲击点及附近区域,远离冲击点区域的应力很小;在Voronoi模型中,应力分布区域更大,受力更加均匀。规则模型受冲击后很快就被冲破;而Voronoi模型的冲击模拟结果显示,其最大应力载荷小于规则模型,最终弹丸并未完全贯穿模型。
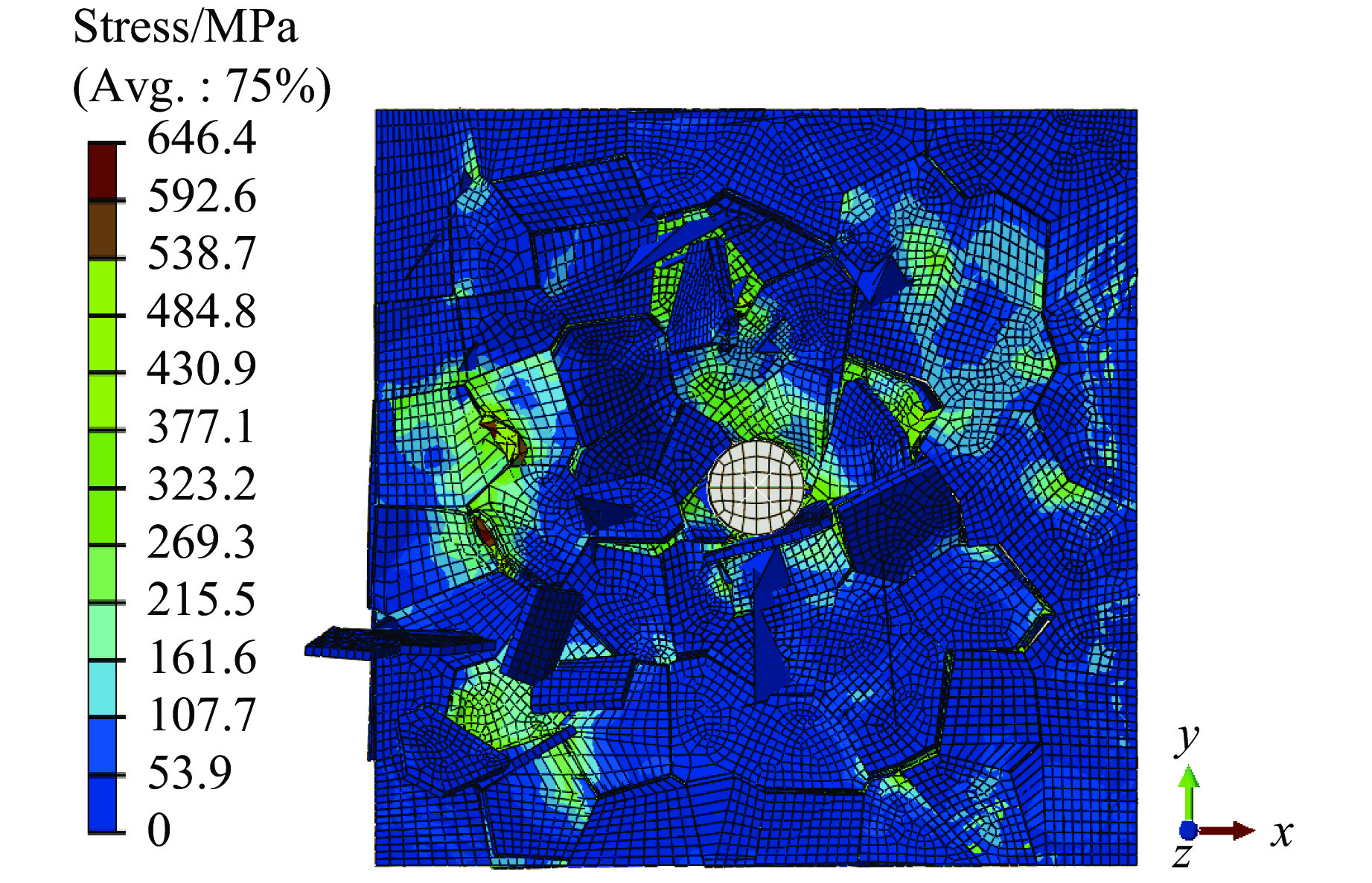
图6和图7分别为规则片板模型和Voronoi片板模型受弹丸冲击3.00 ms时应力云图的俯视图。从受弹丸冲击破坏情况来看:规则模型中脱黏现象主要集中在弹丸冲击点附近区域;而Voronoi模型的冲击影响区域更大,基本遍布整个模型。对于Voronoi模型,冲击区域发生变形时,其余片板受挤压后也发生了脱黏现象,吸收更多的冲击能量,从而有利于冲击能量的扩散与吸收,使模型的更多部分承担冲击负载,即增加承载区域,减小应力集中,更好地发挥能量共享机制。因此Voronoi片板模型抵抗冲击荷载的能力明显优于规则片板模型。
3.2 黏结层厚度和分块尺寸对冲击吸能的影响
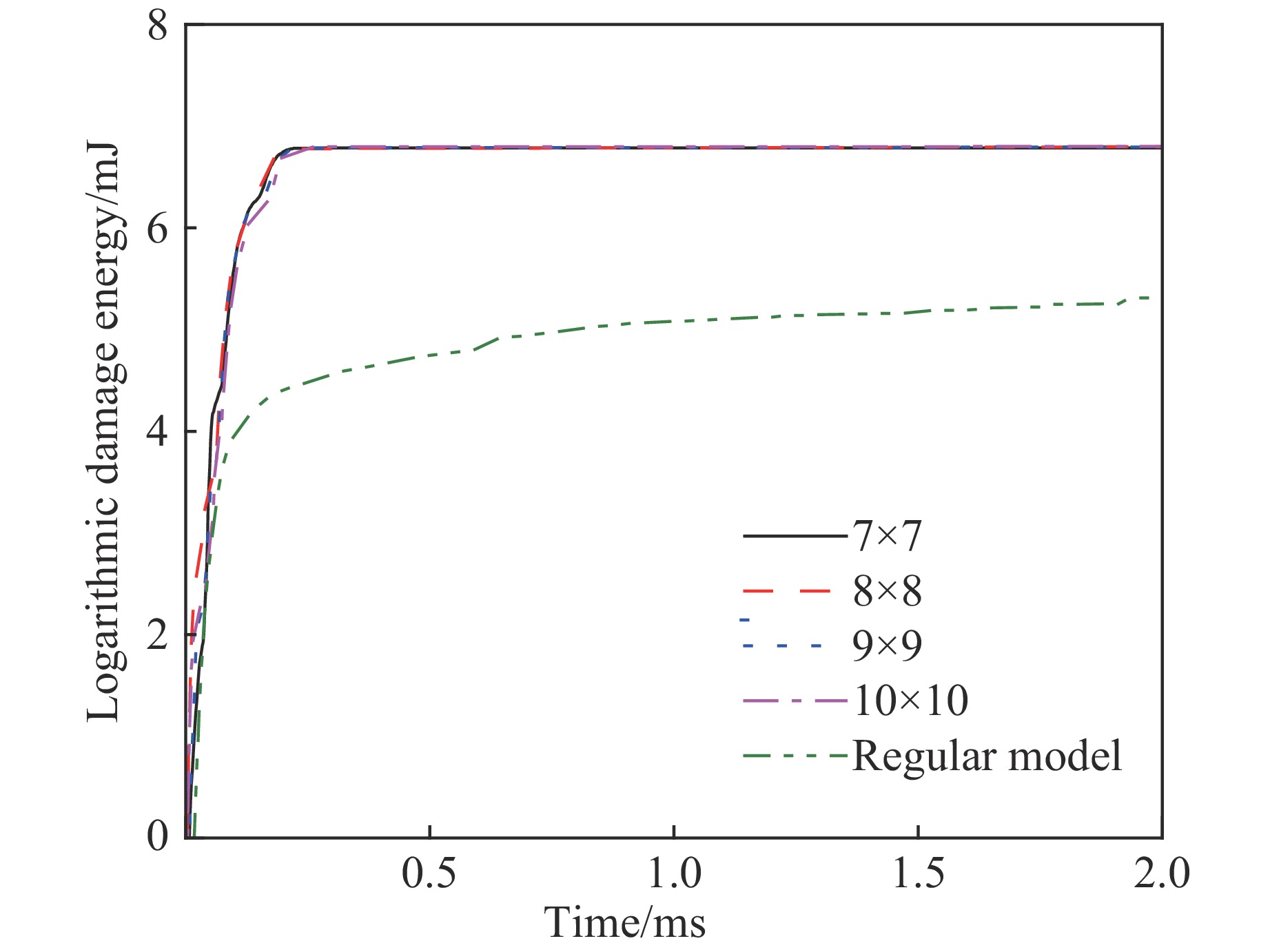
图8和图9分别给出了规则模型和不同分块Voronoi模型的损伤耗散能和塑性耗散能对比。在模型总尺寸相同的情况下,Voronoi模型的损伤耗散能远远高于规则片板模型,而塑性耗散能则小于规则片板模型,说明在冲击载荷作用下Voronoi模型抵抗冲击的能力优于规则片板模型。不同分块Voronoi模型的损伤耗散能和塑性耗散能差别不大,分块尺寸对Voronoi模型抗冲击性能的影响很小。
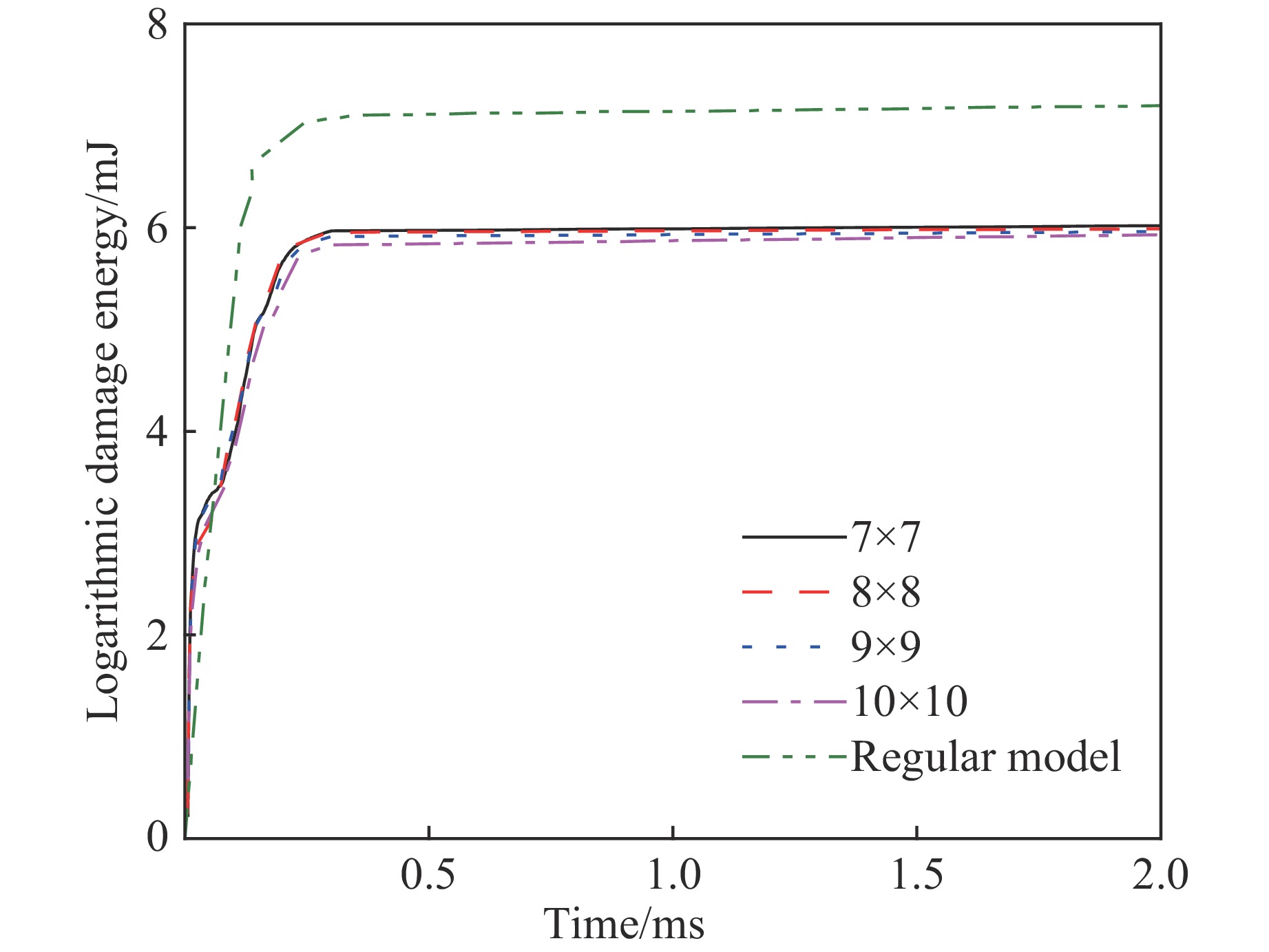
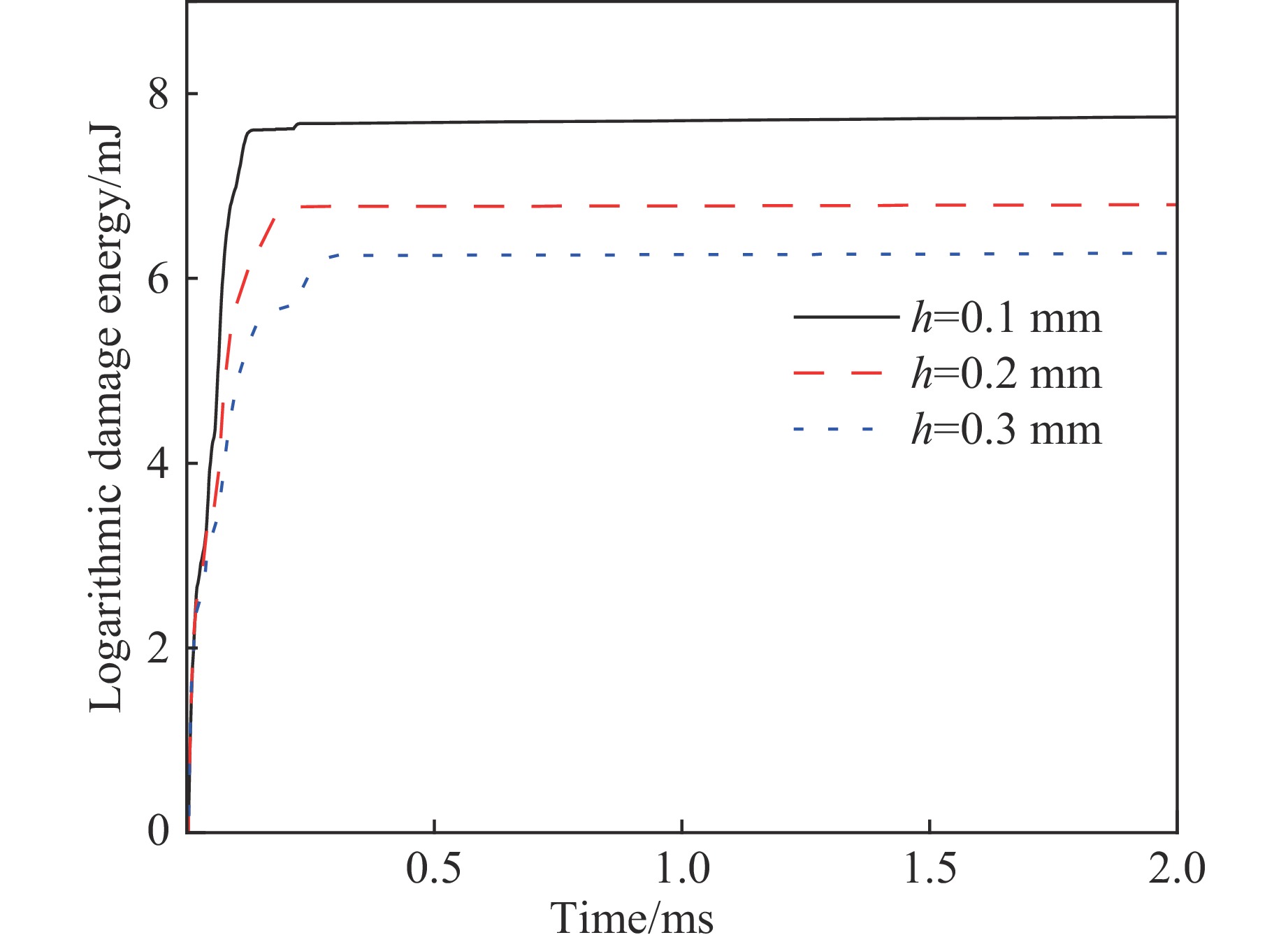
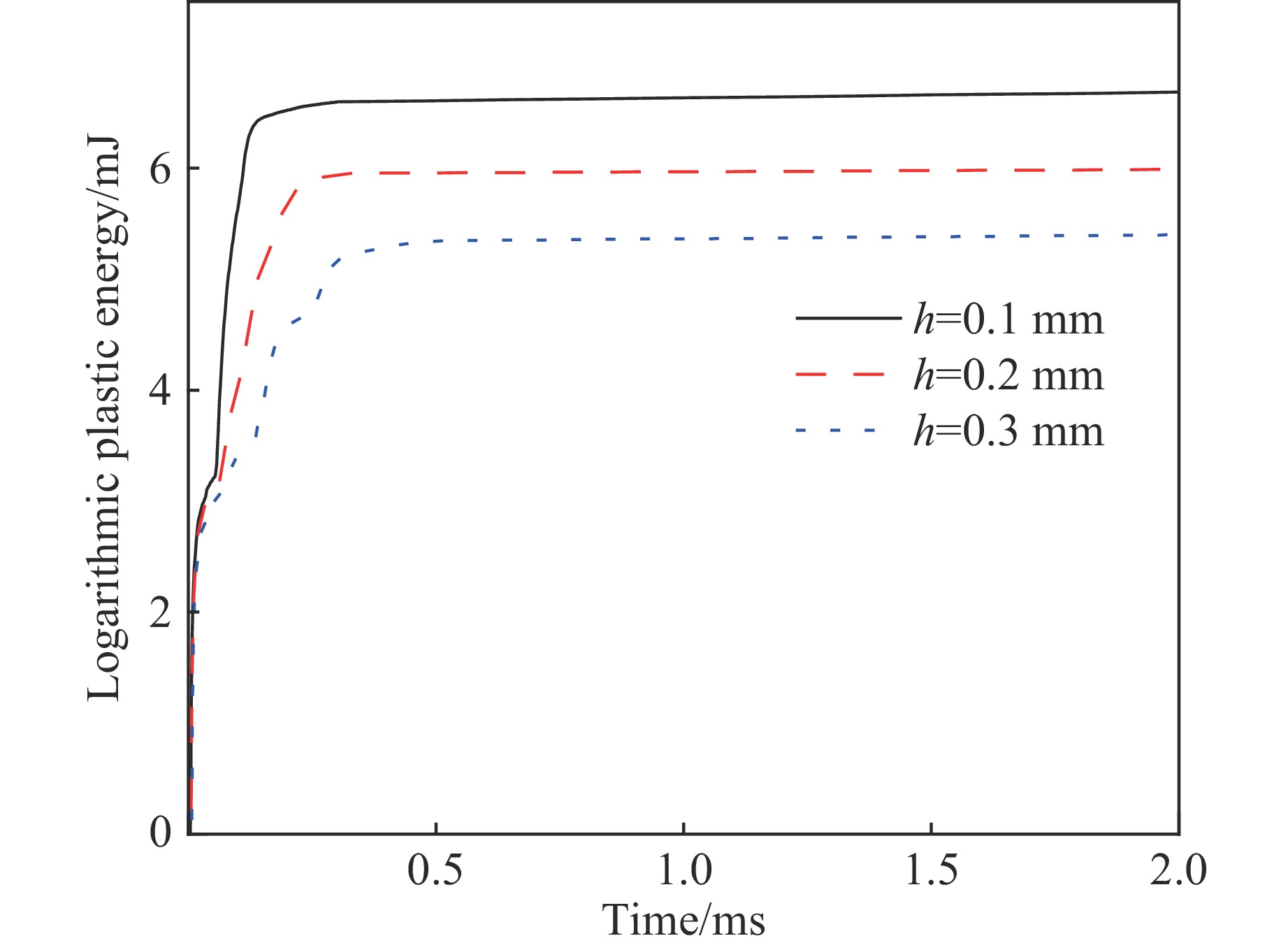
图10和图11分别给出了不同黏结层厚度(h)的Voronoi模型的损伤耗散能和塑性耗散能。可以看出,黏结层对损伤耗散能和塑性耗散能的影响很明显。黏结层越薄,模型整体吸能越大,越薄的黏结层使模型具有更高的抗弯刚度,抗冲击性能越强。由此可见,Voronoi模型的不规则性是仿贝壳珍珠层复合结构模型抗冲击性能的影响因素,对贝壳结构韧性的提升发挥着重要作用。
4. 结 论
通过有限元数值模拟研究了仿贝壳珍珠层Voronoi模型在弹丸冲击载荷下的动态力学响应,得到如下主要结论。
(1)从冲击破坏受损情况来看,不规则Voronoi模型的冲击影响区域比规则模型更大,基本遍布整个模型。对于Voronoi模型,当冲击区域发生变形时,其余片板受挤压后发生脱黏现象,从而吸收更多的冲击能量,有利于冲击能量的扩散与吸收,让模型的更多部分承担冲击负载,即增加承载区域,并且减小应力集中,更好地发挥共享机制。规则模型的脱黏现象主要集中在弹丸冲击点及其附近区域。
(2)在模型总尺寸相同的情况下,Voronoi片板模型的损伤耗散能远远高于规则片板模型,而塑性耗散能则小于规则模型。在冲击载荷作用下,不规则Voronoi片板模型抵抗冲击的能力优于规则片板模型。
(3)分块尺寸对Voronoi模型抗冲击性能的影响很小,而黏结层对损伤耗散能和塑性耗散能的影响很明显,黏结层越薄,模型整体吸能越大,抗冲击性能越强。
鉴于目前制备具有Voronoi结构的金属/高分子材料复合实验模型具有一定困难,因此未对模拟结果进行实验验证。随着3D打印技术的进一步发展,可采用金属/高分子材料混合3D打印技术制备仿贝壳珍珠层Voronoi实验模型,届时即可对铝/乙烯基复合三维Voronoi模型的数值模拟工作进行验证,进而开展更深入的研究。
-
图 1 (a) Voronoi图的初始网格构型;(b)每个站点都在一个圆圈区域内随机移动;(c)新的Voronoi图是从新的站点系统中生成,通过矩形裁剪,形成有限区域的随机Voronoi图[25]
Figure 1. (a) Initial grid formation of Voronoi diagram; (b) each site moves randomly within a circle region; (c) a new Voronoi diagram is generated from the new site system, and by a rectangle cut, a finite Voronoi diagram is generated[25]
表 1 铝片的材料参数
Table 1. Parameters of aluminum plate
Material ρ/(kg·m−3) ν E/GPa Aluminum 2750 0.3 72 表 2 Cohesive模型的材料参数
Table 2. Parameters of Cohesive model
t0n/MPa t0s/MPa t0t/MPa G0n/(kJ·m−2) G0s/(kJ·m−2) G0t/(kJ·m−2) ρ/(kg·m−3) Es/GPa Et/GPa 80 80 80 1 1 1 1 850 4 1.5 -
[1] CURREY J D. Mechanical properties of mother of pearl in tension [J]. Proceedings of the Royal Society B: Biological Sciences, 1977, 196(1125): 443–463. doi: 10.1098/rspb.1977.0050 [2] BARTHELAT F, TANG H, ZAVATTIERI P D, et al. On the mechanics of mother-of-pearl: a key feature in the material hierarchical structure [J]. Journal of the Mechanics and Physics of Solids, 2007, 55(2): 306–337. doi: 10.1016/j.jmps.2006.07.007 [3] IMBALZANO G, TRAN P, NGO T D, et al. A numerical study of auxetic composite panels under blast loadings [J]. Composite Structures, 2016, 135: 339–352. doi: 10.1016/j.compstruct.2015.09.038 [4] NGUYEN Q T, NGO T D, TRAN P, et al. Influences of clay and manufacturing on fire resistance of organoclay/thermoset nanocomposites [J]. Composites Part A: Applied Science and Manufacturing, 2015, 74: 26–37. doi: 10.1016/j.compositesa.2015.03.014 [5] PRO J W, LIM R K, PETZOLD L R, et al. GPU-based simulations of fracture in idealized brick and mortar composites [J]. Journal of the Mechanics and Physics of Solids, 2015, 80: 68–85. doi: 10.1016/j.jmps.2015.03.011 [6] ZHANG N, YANG S F, XIONG L M, et al. Nanoscale toughening mechanism of nacre tablet [J]. Journal of the Mechanical Behavior of Biomedical Materials, 2016, 53: 200–209. doi: 10.1016/j.jmbbm.2015.08.020 [7] SARIKAYA M. An introduction to biomimetics: a structural viewpoint [J]. Microscopy Research and Technique, 1994, 27(5): 360–375. doi: 10.1002/jemt.1070270503 [8] GAO H J, JI B H, JÄGER I L, et al. Materials become insensitive to flaws at nanoscale: lessons from nature [J]. Proceedings of the National Academy of Sciences of the United States of America, 2003, 100(10): 5597–5600. doi: 10.1073/pnas.0631609100 [9] SONG F, SOH A K, BAI Y L. Structural and mechanical properties of the organic matrix layers of nacre [J]. Biomaterials, 2003, 24(20): 3623–3631. doi: 10.1016/S0142-9612(03)00215-1 [10] BARTHELAT F. Nacre from mollusk shells: a model for high-performance structural materials [J]. Bioinspiration & Biomimetics, 2010, 5(3): 035001. doi: 10.1088/1748-3182/5/3/035001 [11] TRAN P, NGO T D, MENDIS P. Bio-inspired composite structures subjected to underwater impulsive loading [J]. Computational Materials Science, 2014, 82: 134–139. doi: 10.1016/j.commatsci.2013.09.033 [12] FLORES-JOHNSON E A, SHEN L M, GUIAMATSIA I, et al. A numerical study of bioinspired nacre-like composite plates under blast loading [J]. Composite Structures, 2015, 126: 329–336. doi: 10.1016/j.compstruct.2015.02.083 [13] DUTTA A, TEKALUR S A. Crack tortuousity in the nacreous layer-topological dependence and biomimetic design guideline [J]. International Journal of Solids and Structures, 2014, 51(2): 325–335. doi: 10.1016/j.ijsolstr.2013.10.006 [14] KOTHA S P, LI Y, GUZELSU N. Micromechanical model of nacre tested in tension [J]. Journal of Materials Science, 2001, 36(8): 2001–2007. doi: 10.1023/A:1017526830874 [15] BARTHELAT F, RABIEI R. Toughness amplification in natural composites [J]. Journal of the Mechanics and Physics of Solids, 2011, 59(4): 829–840. doi: 10.1016/j.jmps.2011.01.001 [16] SONG F, BAI Y L. Effects of nanostructures on the fracture strength of the interfaces in nacre [J]. Journal of Materials Research, 2003, 18(8): 1741–1744. doi: 10.1557/JMR.2003.0239 [17] RABIEI R, BEKAH S, BARTHELAT F. Failure mode transition in nacre and bone-like materials [J]. Acta Biomaterialia, 2010, 6(10): 4081–4089. doi: 10.1016/j.actbio.2010.04.008 [18] SHAO Y, ZHAO H P, FENG X Q, et al. Discontinuous crack-bridging model for fracture toughness analysis of nacre [J]. Journal of the Mechanics and Physics of Solids, 2012, 60(8): 1400–1419. doi: 10.1016/j.jmps.2012.04.011 [19] GHAZLAN A, NGO T, VAN LE T, et al. Blast performance of a bio-mimetic panel based on the structure of nacre: a numerical study [J]. Composite Structures, 2020, 234: 111691. doi: 10.1016/j.compstruct.2019.111691 [20] KO K, JIN S, LEE S E, et al. Impact resistance of nacre-like composites diversely patterned by 3D printing [J]. Composite Structures, 2020, 238: 111951. doi: 10.1016/j.compstruct.2020.111951 [21] WU K J, ZHENG Z J, ZHANG S S, et al. Interfacial strength-controlled energy dissipation mechanism and optimization in impact-resistant nacreous structure [J]. Materials & Design, 2019, 163: 107532. doi: 10.1016/j.matdes.2018.12.004 [22] 刘金义, 刘爽. Voronoi图应用综述 [J]. 工程图学学报, 2004, 25(2): 125–132. doi: 10.3969/j.issn.1003-0158.2004.02.023LIU J Y, LIU S. A survey on applications of Voronoi diagrams [J]. Journal of Engineering Graphics, 2004, 25(2): 125–132. doi: 10.3969/j.issn.1003-0158.2004.02.023 [23] BARGMANN S, KLUSEMANN B, MARKMANN J, et al. Generation of 3D representative volume elements for heterogeneous materials: a review [J]. Progress in Materials Science, 2018, 96: 322–384. doi: 10.1016/j.pmatsci.2018.02.003 [24] BAHMANI A, LI G, WILLETT T L, et al. Generating realistic representative microstructure of biomimetic composite materials for computational assessment of mechanical properties [J]. Materials Today: Proceedings, 2019, 7: 373–381. doi: 10.1016/j.matpr.2018.11.098 [25] GHAZLAN A, NGO T D, TRAN P. Three-dimensional Voronoi model of a nacre-mimetic composite structure under impulsive loading [J]. Composite Structures, 2016, 153: 278–296. doi: 10.1016/j.compstruct.2016.06.020 -







 下载:
下载:
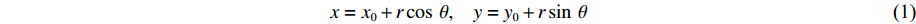
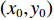
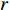
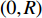
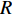
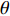
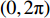

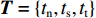
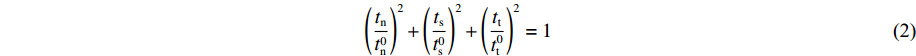
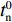
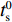
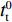
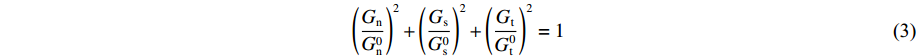
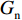
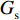
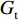
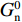
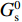
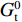
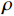

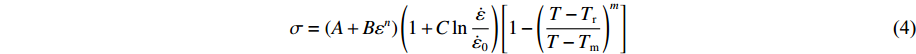
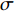
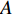
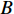

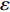
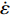
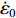

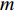
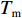












 下载:
下载: