Johnson-Cook Failure Model Parameters of Tantalum-Tungsten Alloy for Rod-Shaped EFP
-
摘要: 针对目前数值仿真不能有效预测钽钨合金药型罩聚能装药杆式爆炸成型弹丸(Explosive formed projectile,EFP)的爆炸成型和断裂问题,开展了钽钨合金材料在不同应力、应变率以及温度条件下的力学性能实验,通过实验数据拟合得到了材料的Johnson-Cook失效模型参数。基于LS-DYNA嵌入该套模型参数开展了典型球缺钽钨药型罩EFP的成型仿真,通过同结构聚能装药静爆脉冲X射线摄影实验对仿真形成的EFP形状和速度计算结果进行对比验证。结果表明:将实测Johnson-Cook失效模型参数应用于杆式EFP成型的数值仿真时,各项成型参数的计算结果(形状、速度等)与实验结果的相对误差均小于9%,实现了对杆式EFP成型及断裂的准确预测。
-
关键词:
- 爆炸成型弹丸 /
- 钽钨合金 /
- Johnson-Cook失效模型
Abstract: Due to the limitation of current numerical simulation model in predicting the fracture of rod-shaped tantalum-tungsten (Ta-W) alloy explosive formed projectile (EFP) during the forming process, the tests of the mechanical properties of Ta-W alloy specimen under different stress, strain rate and temperature conditions were carried out to obtain the parameters of Johnson-Cook failure model. The forming process of Ta-W EFP with typical charge structure was simulated by LS-DYNA software using the Johnson-Cook failure model and adaptive algorithm. X-ray experiment was carried out to verify the effectiveness of the numerical simulation. When the failure model was used in the numerical simulation of rod-shaped EFP molding, the prediction of EFP fracture was better, and the errors between the simulation results and the experiment results were less than 9%. The results revealed that the formation and fracture of rod-shaped EFP can be accurately predicted by the failure model. -
爆炸成型弹丸(Explosive formed projectile, EFP)是一种利用装药聚能效应形成的高速弹丸,在智能灵巧反装甲弹药中具有广泛应用[1-2]。钽及其合金因具有高密度、高延展性等特点成为主选罩材之一,研究者不断探索通过提高EFP的长径比来提高侵彻威力。随着数值仿真技术的飞速发展,这种使用方便、直观高效的方法逐渐成为当下EFP研究的主要手段。数值仿真的准确性主要依赖于计算方法、材料模型及其参数等因素。目前关于EFP成型的数值模拟主要采用Lagrange、ALE和Lagrange/Euler混合等计算方法,而药型罩一般采用Lagrange网格表征,对于其大变形引起的计算步长过小问题则采用无物理意义的侵蚀算法处理,即当微元几何应变超过某定值,将该变形网格从计算中删除。药型罩材料本构模型一般采用考虑材料应变率的Johnson-Cook(JC)、Zerilli-Armstrong等模型,通常未考虑使用材料的失效模型。如Kim等[3]、郭腾飞等[4]、樊雪飞等[5]和朱志鹏等[6]分别采用未加失效的本构模型研究了聚能装药结构参数对钽EFP成型的影响。上述方法对球形或小长径比EFP成型形态预测得较为可信,但对于寻求进一步提高其侵彻能力的大长径比杆式EFP则显得无能为力,存在误判EFP断裂而得出错误成型形态的情况,这已成为制约大长径比EFP数值模拟预测的瓶颈。针对这一问题,已有相关学者针对成熟罩材展开研究,如丁力等[7]引入JC失效模型预测了紫铜罩杆式EFP的成型,而对于钽及其合金EFP的这类问题研究较少。
近年来不少学者也开展了钽及其合金的材料表征,研究主要集中在材料的本构关系[8-12],对材料断裂失效表征研究却鲜有报道。在钽及其合金的JC模型研究方面,彭建祥[8]、郭伟国[9]、Gao等[11]进行了相关力学性能试验,根据试验结果拟合了材料JC本构模型,但并未对材料的JC失效模型进行研究。
为了实现采用数值模拟方法精准预测钽EFP的成型,指导EFP装药结构设计,针对一种典型钽钨合金材料,开展不同应力、温度和应变率下的力学性能测试,根据试验测试数据拟合得到JC失效模型参数;通过对比典型球缺钽钨药型罩EFP成型的数值模拟结果与拍摄的脉冲X射线摄影照片,验证数值模拟的有效性。研究结果可为钽钨EFP战斗部的设计提供技术支撑。
1. 钽钨合金JC失效模型参数测定
1.1 JC失效模型表征
材料的失效是受到多种因素影响的复杂物理现象。针对金属材料在较大压力、应变率和温度条件下的失效,Johnson等[13]提出了考虑应力三轴度、温度及应变率效应的JC失效模型。模型采用累积损伤准则,定义损伤参数
D=∑Δεeqεf (1) 式中:
$\Delta {\varepsilon \rm_{eq}}$ 为一个计算循环的等效塑性应变增量,${\varepsilon _{\rm{f}}}$ 为当前计算循环的应力、应变率和温度下材料的失效应变。当损伤参数D=1时,材料失效。失效应变的表达形式如下εf=[D1+D2exp(D3σ∗)](1+D4ln˙ε∗eq)(1+D5T∗) (2) 式中:D1、D2、D3、D4、D5为材料参数;
${\sigma ^*}$ 为应力三轴度;$\dot \varepsilon \rm_{eq}^*$ 为无量纲等效应变率,$\dot \varepsilon \rm_{eq}^* = {\dot \varepsilon \rm_{eq}}/{\dot \varepsilon _0}$ ,${\dot \varepsilon \rm_{eq}}$ 为实际应变率,${\dot \varepsilon _0}$ 为参考应变率;${T^*}$ 为无量纲温度,${T^*} = \left( {T - {T\rm_r}} \right)/\left( {{T\rm_m} - {T\rm_r}} \right)$ ,T为瞬时温度,${T\rm_r}$ 为参考温度,${T\rm_m}$ 为材料熔化温度。式(2)等号右边的3个部分分别对应应力、应变率和温度对失效应变的影响。三者为乘积关系,相互独立,依次单独变化某一因素,即可拟合出相应材料参数。
1.2 材料力学性能实验
钽及其合金材料因其高密度和优异的力学性能,在聚能装药药型罩中具有应用潜能。本研究选用钽钨合金,密度为16.65 g/cm3,采用电子束熔炼及锻造挤压加工工艺。表1显示了钽钨合金主要化学成分(质量分数)。实验试件均加工自同一批次钽钨合金棒材。为了标定钽钨合金的JC失效模型,开展了3个系列的材料力学性能实验:缺口试件拉伸实验、光滑试件室温拉伸实验和高温拉伸实验。
表 1 钽钨合金化学成分Table 1. Chemical composition of tantalum tungsten alloy(%) Nb W Mo N O Si 0.006 6 2.830 0 0.001 0 0.001 5 0.007 1 0.001 0 1.2.1 应力三轴度对失效应变的影响
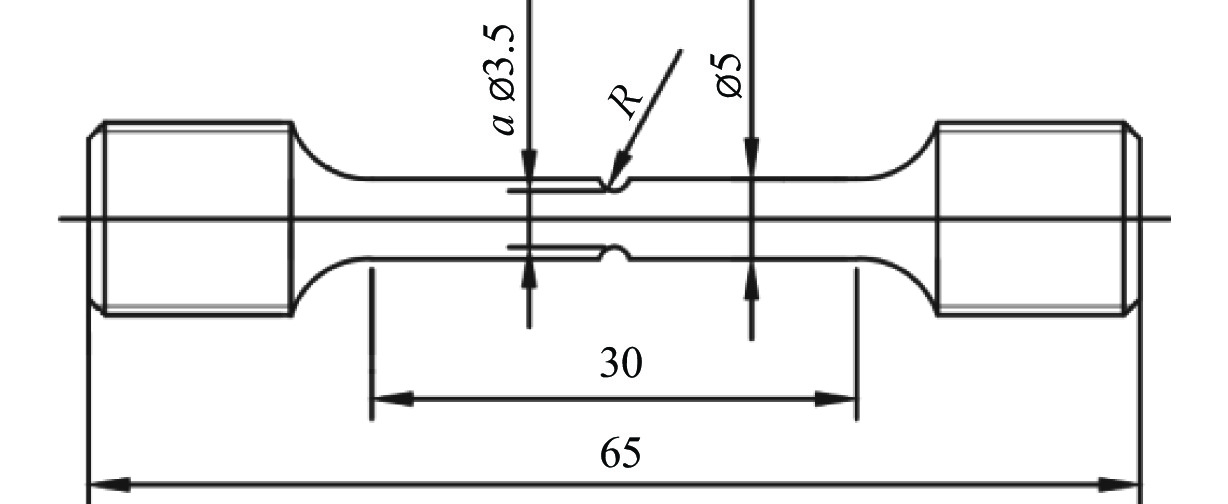
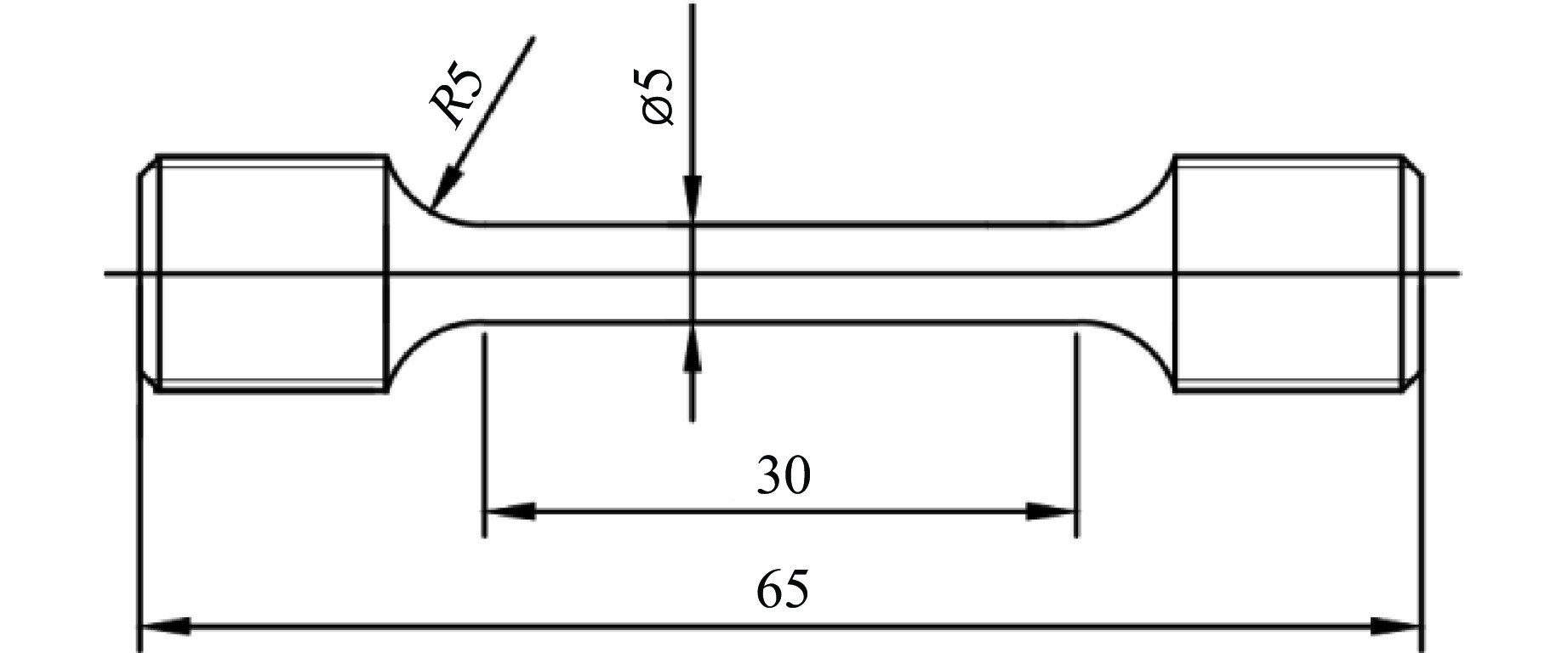
通过对不同缺口试件的单向拉伸实验来获取不同应力三轴度下钽钨合金的失效应变。缺口试件尺寸如图1所示。根据Bridgman[14]的分析,可近似取缺口拉伸试件的应力三轴度
σ∗=13+ln(1+a2R) (3) 式中:a为试件缺口处横截面的半径,R为缺口曲率半径。
为了获取较宽的应力三轴度范围,加工了4种缺口试件,缺口半径R分别为1.0、1.5、3.0和6.0 mm,同时也加工了不含缺口的光滑圆柱试件,其R可视为无穷大。
钽钨合金EFP成型过程中的断裂主要是由拉伸导致,因此在进行应力三轴度对失效应变影响的材料实验时,未考虑压缩断裂的情况。
在常温下,使用INSTRON万能材料实验机对各试件进行参考应变率下的单向拉伸实验。该实验以及随后的拉伸实验结果中断裂试件的失效应变
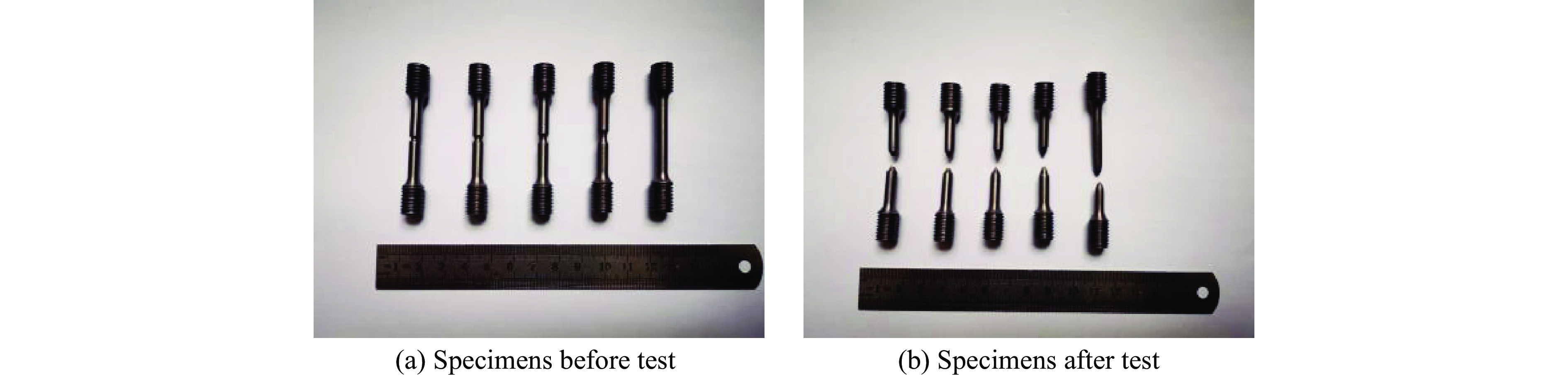
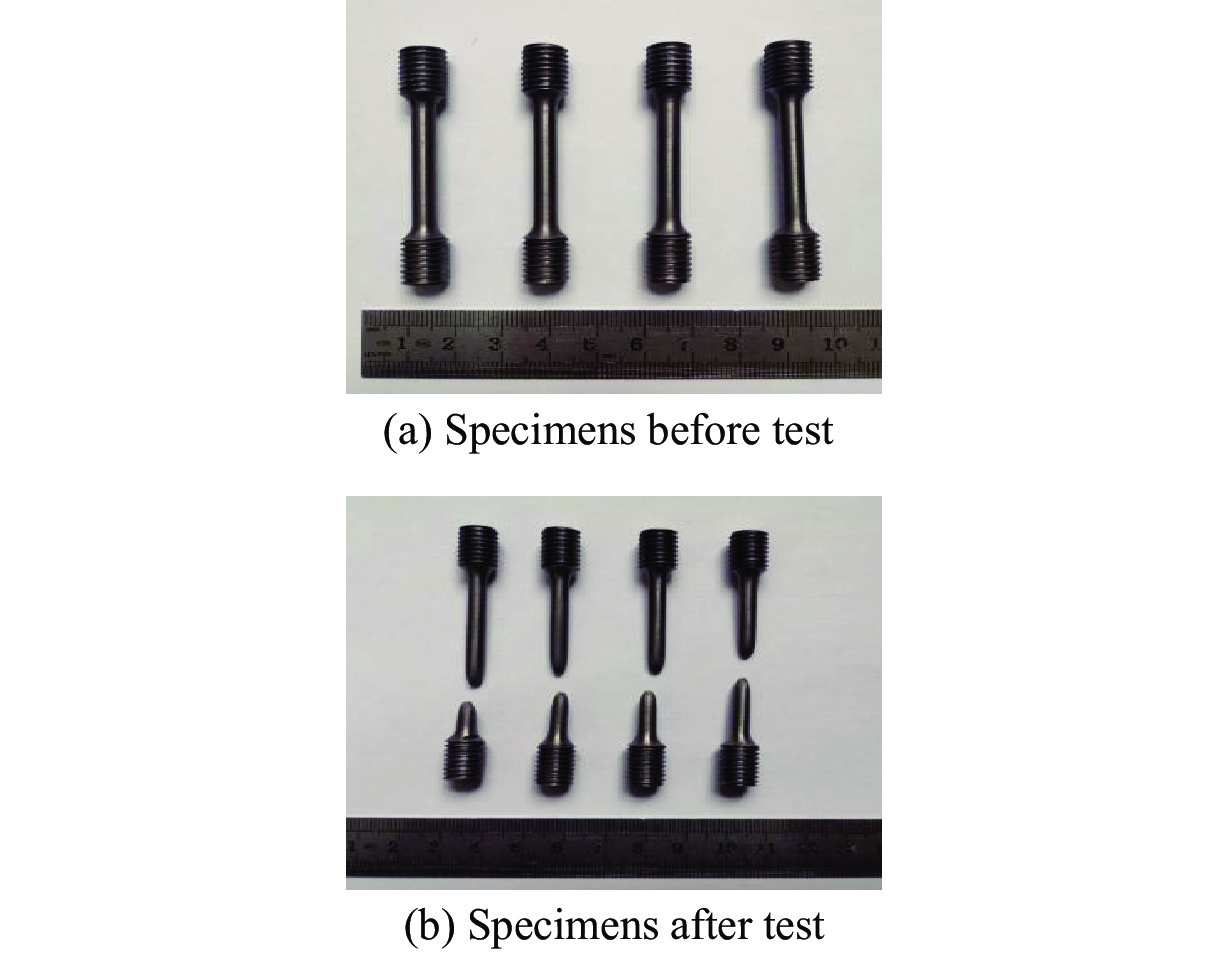
$\varepsilon\rm_f $ 采用下式计算[15]εf=ln(A0/Af) (4) 式中:A0为试件初始横截面积,Af为断裂后断口区域横截面积。图2为试件拉伸实验前后照片。
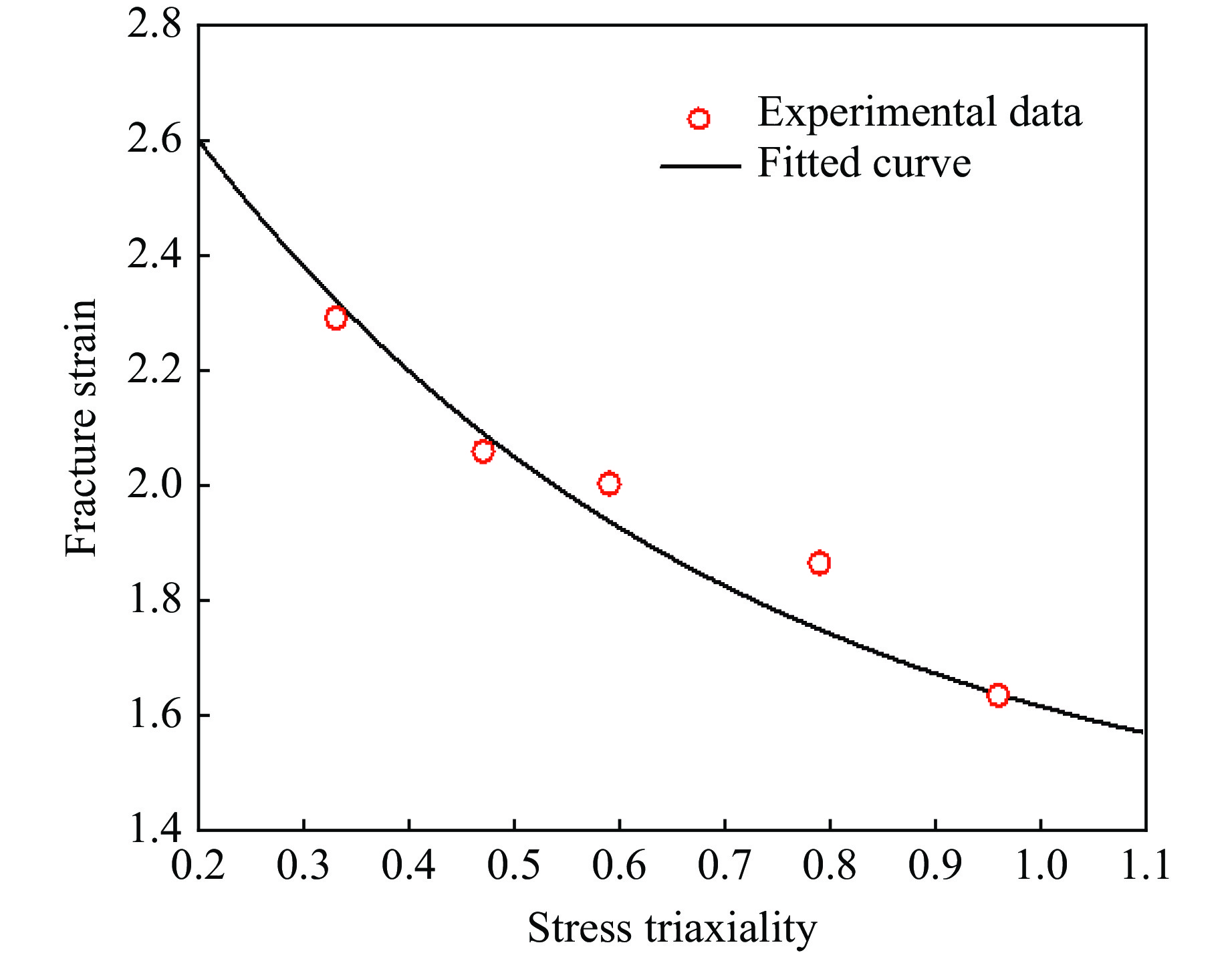
缺口试件拉伸实验中,失效应变随应力三轴度的变化如图3所示。从图3可以看出,钽钨合金的失效应变随应力三轴度的增加而减小。在参考应变率和室温条件下,JC失效模型可简化为
${\varepsilon _{\rm{f}}} = {D_1} + {D_2}\exp \left( {{D_3}{\sigma ^*}} \right)$ 。采用非线性最小二乘法,通过JC失效模型对图3中的数据进行拟合,得到失效参数D1=1.355,D2=1.833,D3= −1.930,拟合曲线与测试数据点对比见图3。1.2.2 应变率对失效应变的影响
采用光滑圆柱试件,在室温下使用材料拉伸实验机进行不同拉伸速率的单向拉伸实验,获得钽钨合金材料在应变率为10−4~10−1 s−1范围内的失效应变,图4为圆柱试件夹持状态。拉伸实验机的拉伸速率通过引伸计进行控制。光滑圆柱试件尺寸见图5,试件拉伸实验前后照片见图6。
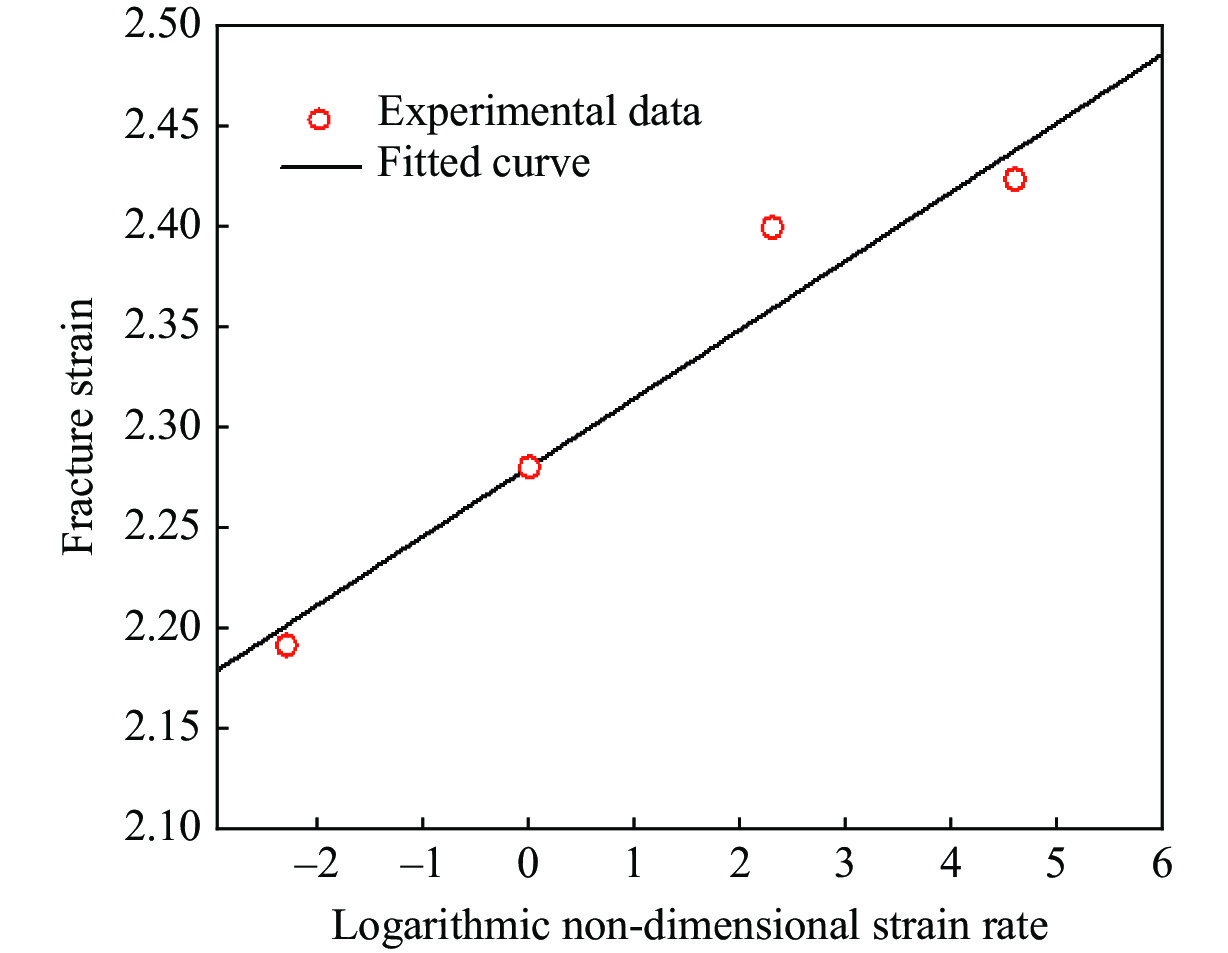
以
${\dot \varepsilon _0}$ =10−3 s−1作为参考应变率,失效应变随无量纲应变率的对数的变化如图7所示。在室温条件下,JC失效模型简化为${\varepsilon _{\rm{f}}} = K\left( {1 + {D_4}\ln \dot \varepsilon \rm_{eq}^*} \right)$ ,其中K可由已知的D1、D2、D3以及光滑圆柱试件的${\sigma ^*}$ 求得。通过JC失效模型公式对图中数据进行拟合,得到失效参数D4=0.015。1.2.3 温度对失效应变的影响
在参考应变率下进行不同温度下光滑圆柱试件单向拉伸实验,可以获得温度影响常数D5,实验选取的温度为室温、300 ℃、500 ℃。采用电加热方式,并使用热电偶进行测温。实验中试件升温至规定温度并稳定15 min后进行拉伸。拉伸实验前后试件照片如图8所示。
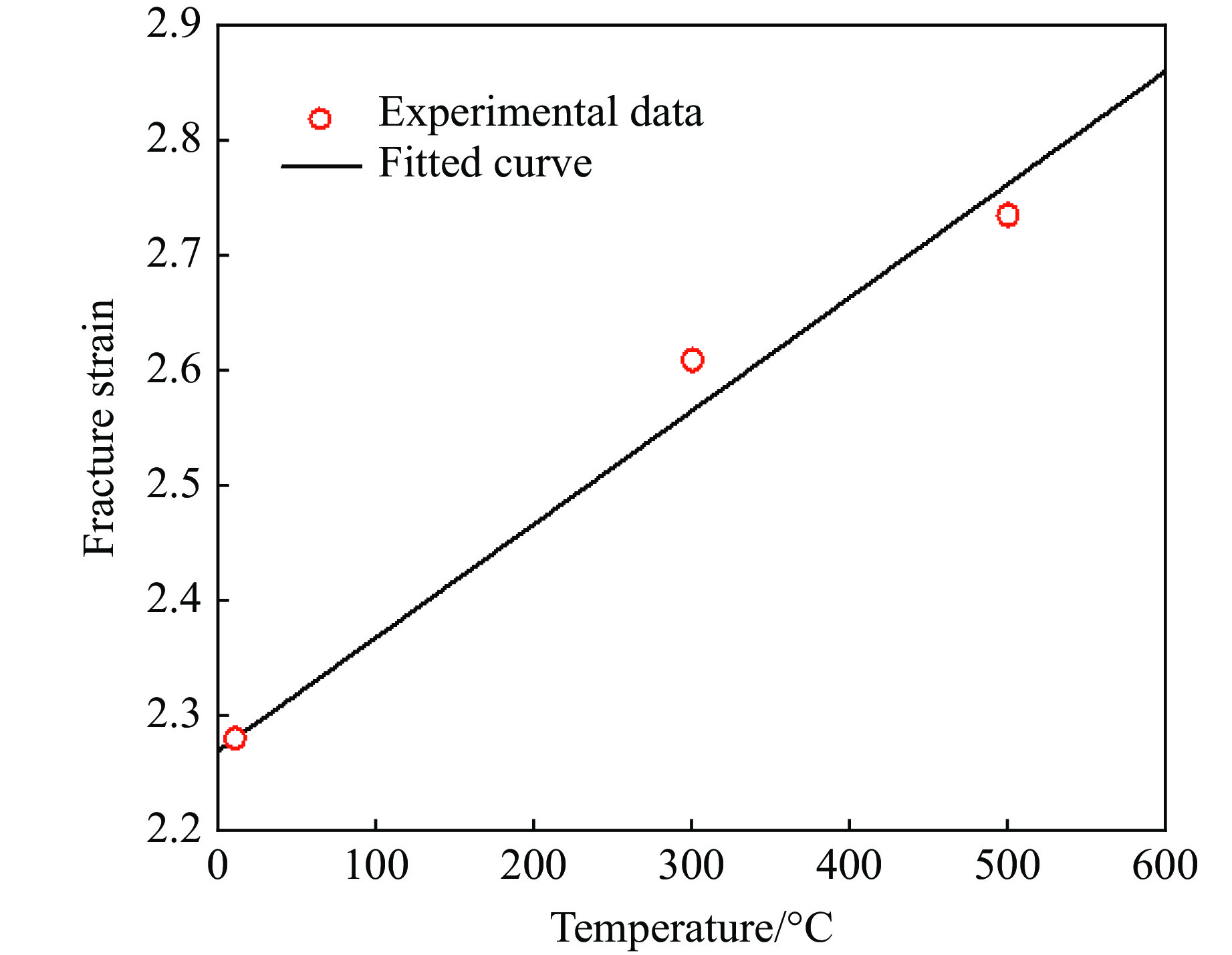
图9给出了试件的失效应变随温度的变化。随着温度上升,钽钨合金的延性提高,失效应变增大。在该实验条件下,JC失效模型的形式可简化为
${\varepsilon _{\rm{f}}} = K\left( {1 + {D_5}{T^*}} \right)$ 。通过简化的JC失效模型对图9中的数据进行拟合,得到失效参数D5=1.868。2. 钽钨合金失效模型参数验证
基于实验测得的钽钨合金的JC失效模型参数,能否实现对钽钨合金EFP成型及断裂的有效预测,需要进行数值模拟和对比实验的验证。
2.1 EFP成型数值计算模型
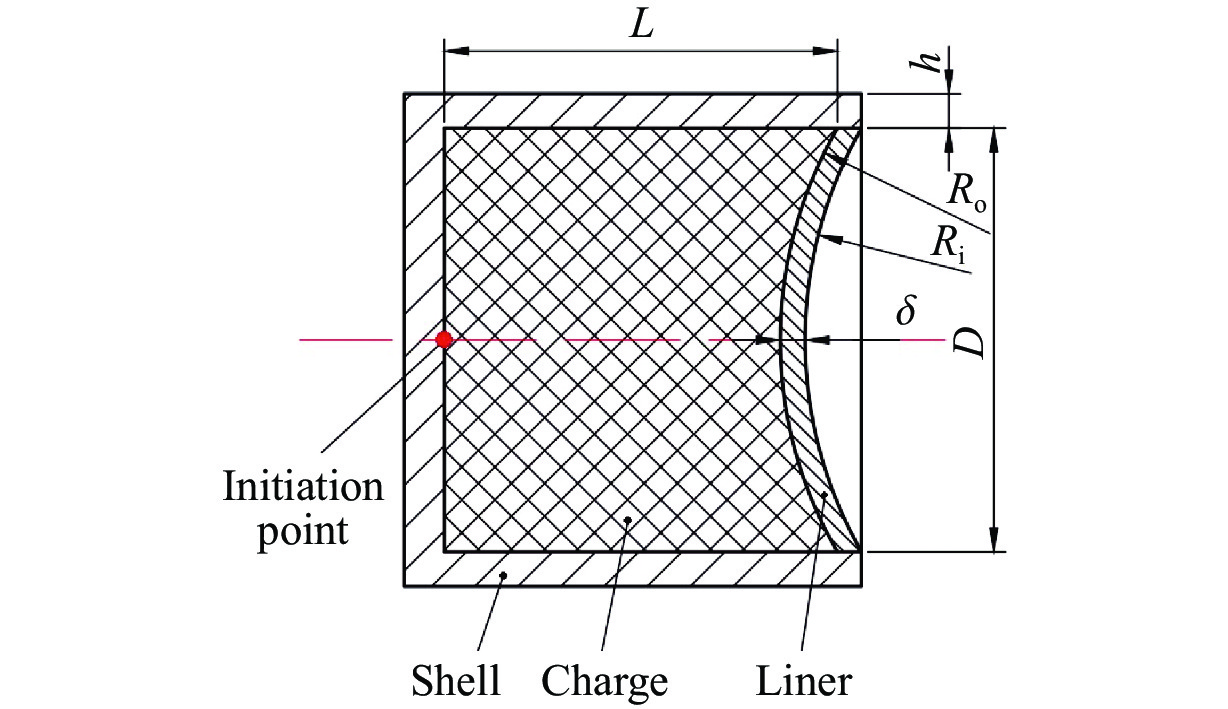
为验证钽钨合金JC失效模型参数模拟EFP成型的有效性,针对性地设计了两种预期能形成不同长径比的杆式EFP聚能装药结构,两种结构的唯一差异在于药型罩的厚度。图10为EFP装药结构的几何简化模型。装药类型为JH-2炸药,装药直径D=56 mm,装药长度L=48.5 mm;壳体材料为45钢,壁厚h=5 mm;药型罩材料为钽钨合金,采用球缺变壁厚结构,外壁曲率Ro=58.55 mm,内壁曲率Ri=57.70 mm,两种结构的药型罩中心厚度
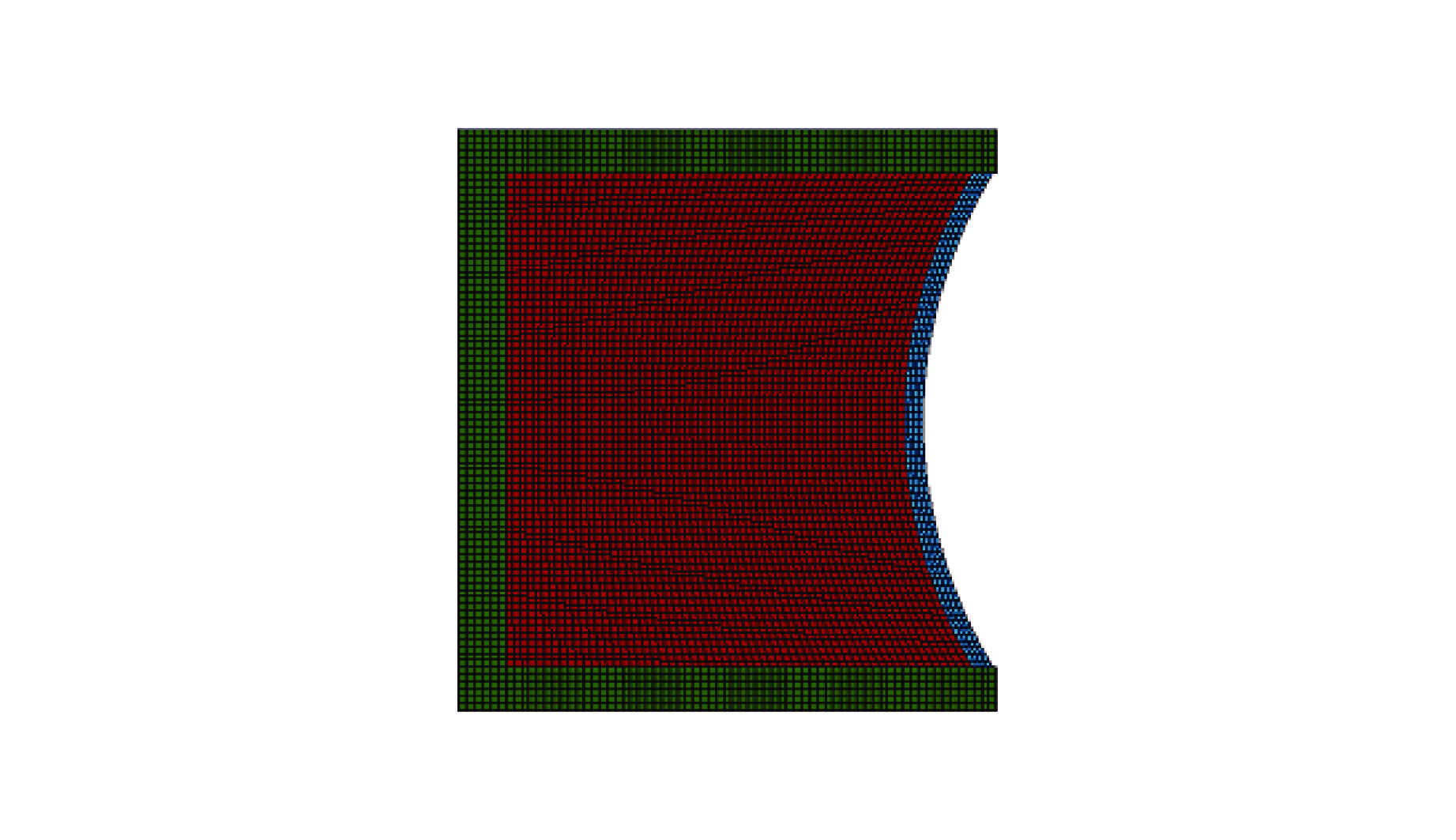
$\delta $ 分别取1.5和2.0 mm。采用LS-DYNA非线性动力学软件Lagrange 算法对两种结构的EFP成型过程进行数值模拟。图11为计算网格模型,各部件均使用二维14号Shell单元进行划分。按照是否嵌入失效模型分两组开展数值模拟,按序记为组Ⅰ、组Ⅱ。组Ⅰ中钽钨合金材料不应用失效模型,组Ⅱ中引入本研究测得的JC失效模型。各部件选用的材料模型参数如表2所示,其中ρ为密度,45钢、JH-2的材料模型参数引自文献[6]。钽钨合金的JC本构模型参数(A、B、n、C、m)引自文献[16],如表3所示。
表 2 数值模拟中采用的材料模型参数Table 2. Material models used in the numerical simulationComponent Material $\,\rho $/(g∙cm−3) Equation of state Constitutive relation Failure model Liner Ta-W 16.65 Grüneisen Johnson-Cook None (Ⅰ) Johnson-Cook (Ⅱ) Shell 45 steel 7.83 Grüneisen Johnson-Cook None Charge JH-2 1.71 JWL High-Explosive-Burn None 2.2 数值计算结果及实验验证
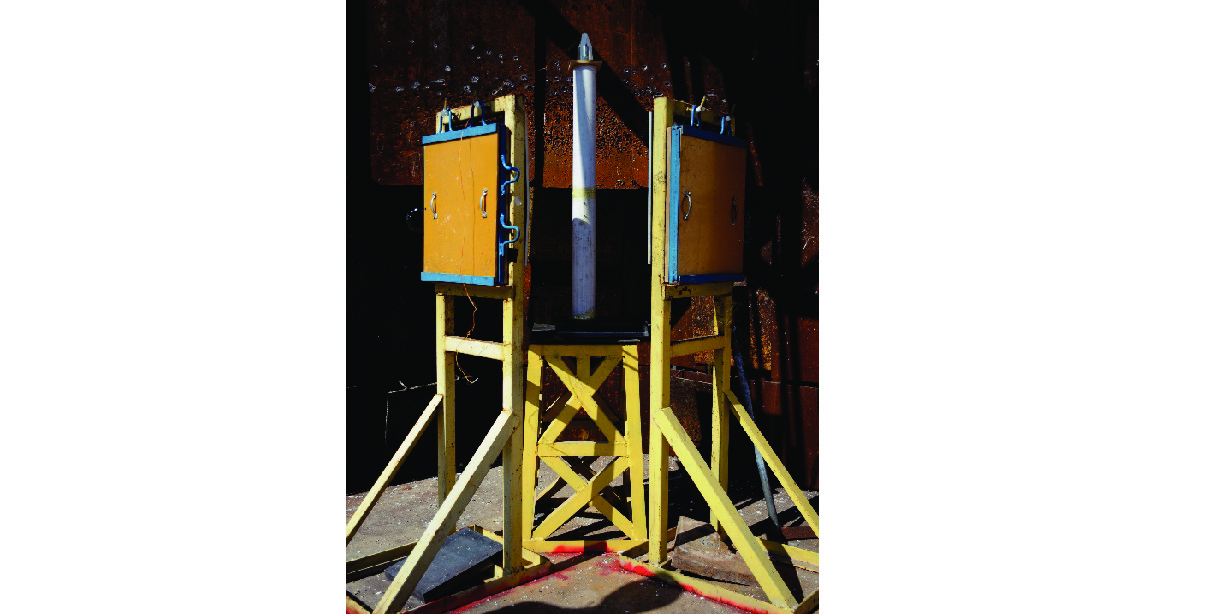
采用与数值模拟计算一致的聚能装药结构以及材料,开展了EFP战斗部静爆脉冲X射线实验,图12、图13分别显示了EFP实验中的战斗部部件以及脉冲X射线摄影实验现场布置。
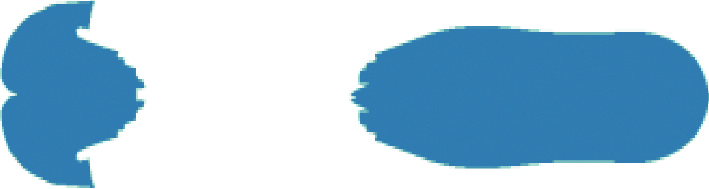
表4为不同药型罩壁厚条件下EFP的成型仿真计算结果与脉冲X射线实验拍摄到的EFP形态对比。当药形罩壁厚
$\delta $ =2.0 mm时,选取EFP的成型时刻为300 μs;当药形罩壁厚$\delta $ =1.5 mm时,选取EFP的成型时刻为270 μs。表 4 数值模拟与实验得到的EFP成型形态对比Table 4. Comparisons of EFP forming states in numerical simulation and experiment$\delta $/mm Simulation Ⅰ
(Without failure model)Simulation Ⅱ
(With failure model)X-ray imaging
experimentForming time/
μs2.0 


300 1.5 


270 当药型罩壁厚
$\delta $ =2.0 mm时,X射线成像图中药型罩形成了形态完整、具有一定长径比的EFP。对于该EFP的数值模拟,组Ⅰ、组Ⅱ的计算结果一致,JC失效模型的嵌入对计算结果并无明显影响,且二者的计算结果与实验较为吻合,各项成型参数的相对误差均小于5%,数值模拟与实验得到的EFP成型参数如表5所示。表 5 数值模拟与实验得到的EFP成型参数对比Table 5. Comparisons of EFP forming results in numerical simulation and experiment$\delta $/mm Method Velocity/(m·s–1) Length/mm Diameter/mm 2.0 Simulation Ⅰ 1718 41.6 11.4 Simulation Ⅱ 1718 41.6 11.4 Experiment 1770 41.3 10.8 1.5 Simulation Ⅰ 1968 51.5 8.9 Simulation Ⅱ 2021 36.1+14.7 10.4 Experiment 2120 33.2+15.6 10.1 当药型罩壁厚
$\delta $ =1.5 mm时,X射线成像图中药型罩形成的EFP总长径比因壁厚减小而有所增大,但是在尾部发生了断裂。对于该EFP的数值模拟,组Ⅰ未能模拟出EFP的断裂,而是得到了具有较高长径比的完整EFP,与实验结果并不相符,若以此为依据指导EFP战斗部设计则会造成误判;组Ⅱ则较为准确地预测了EFP的断裂,得到的EFP成型形态与实验结果较为吻合,各项成型参数的相对误差均小于9%。可以认为,相较于无失效模型的组Ⅰ,嵌入本研究测得的JC失效模型的组Ⅱ较为准确地预测了不同长径比钽钨合金杆式EFP的成型和断裂。
3. 结 论
(1)针对典型钽钨合金材料开展了不同缺口尺寸试件在不同应力、应变率和温度影响下的力学性能实验,测得了失效应变,并根据实验测试数据拟合得到了该钽钨合金的JC失效模型参数。
(2)设计了两种壁厚钽钨合金球缺形药型罩的聚能装药结构,开展了带JC失效模型与不带JC失效模型的EFP成型数值模拟及静爆脉冲X射线对比实验。对于较小长径比的EFP模拟,JC失效模型的嵌入对计算结果并无明显影响。当EFP的长径比增大到一定程度发生颈缩或断裂时,就会凸显失效模型的作用。JC失效模型在数值模拟中的嵌入应用,可以有效解决现有数值模拟无法精确表征长杆EFP的断裂问题。
-
表 1 钽钨合金化学成分
Table 1. Chemical composition of tantalum tungsten alloy
(%) Nb W Mo N O Si 0.006 6 2.830 0 0.001 0 0.001 5 0.007 1 0.001 0 表 2 数值模拟中采用的材料模型参数
Table 2. Material models used in the numerical simulation
Component Material $\,\rho $/(g∙cm−3) Equation of state Constitutive relation Failure model Liner Ta-W 16.65 Grüneisen Johnson-Cook None (Ⅰ) Johnson-Cook (Ⅱ) Shell 45 steel 7.83 Grüneisen Johnson-Cook None Charge JH-2 1.71 JWL High-Explosive-Burn None 表 3 钽钨合金的Johnson-Cook本构模型参数[16]
Table 3. Material parameters of Johnson-Cook constitutive model for Ta-W[16]
A/MPa B/MPa n C m 211 381 0.75 0.068 0.38 表 4 数值模拟与实验得到的EFP成型形态对比
Table 4. Comparisons of EFP forming states in numerical simulation and experiment
$\delta $/mm Simulation Ⅰ
(Without failure model)Simulation Ⅱ
(With failure model)X-ray imaging
experimentForming time/
μs2.0 


300 1.5 

270 表 5 数值模拟与实验得到的EFP成型参数对比
Table 5. Comparisons of EFP forming results in numerical simulation and experiment
$\delta $/mm Method Velocity/(m·s–1) Length/mm Diameter/mm 2.0 Simulation Ⅰ 1718 41.6 11.4 Simulation Ⅱ 1718 41.6 11.4 Experiment 1770 41.3 10.8 1.5 Simulation Ⅰ 1968 51.5 8.9 Simulation Ⅱ 2021 36.1+14.7 10.4 Experiment 2120 33.2+15.6 10.1 -
[1] 隋树元, 王树山. 终点效应学[M]. 北京: 国防工业出版社, 2000: 233–237.SUI S Y, WANG S S. Terminal effects [M]. Beijing: National Defense Industry Press, 2000: 233–237. [2] 杨绍卿. 灵巧弹药工程[M]. 北京: 国防工业出版社, 2010: 1–7.YANG S Q. Smart munition engineering [M]. Beijing: National Defense Industry Press, 2010: 1–7. [3] KIM H J, YI Y S, PARK L J. Analysis of forming characteristics of Ta EFP according to material model [J]. The European Physical Journal Conferences, 2015, 94: 04060. doi: 10.1051/epjconf/20159404060 [4] 郭腾飞, 李伟兵, 李文彬, 等. 钽罩结构参数对EFP成型及侵彻性能的控制 [J]. 高压物理学报, 2018, 32(3): 96–103.GUO T F, LI W B, LI W B, et al. Controlling effect of tantalum liner's structural parameters on EFP formation and penetration performance [J]. Chinese Journal of High Pressure Physics, 2018, 32(3): 96–103. [5] 樊雪飞, 李伟兵, 王晓鸣, 等. 爆轰驱动钽药型罩形成双模毁伤元仿真与试验研究 [J]. 兵工学报, 2017, 38(10): 1918–1925.FAN X F, LI W B, WANG X M, et al. Simulation and experimental study of tantalum liner to form dual-mode damage element by detonation [J]. Acta Armamentarii, 2017, 38(10): 1918–1925. [6] 朱志鹏, 门建兵, 蒋建伟, 等. 大长径比钽爆炸成型弹丸控制研究 [J]. 兵工学报, 2018, 39(Suppl 1): 29–36.ZHU Z P, MEN J B, JIANG J W, et al. Forming control of tantalum EFP with large aspect ratio [J]. Acta Armamentarii, 2018, 39(Suppl 1): 29–36. [7] 丁力, 蒋建伟, 门建兵, 等. 爆炸成型弹丸成型过程中的断裂数值模拟及机理分析 [J]. 兵工学报, 2017, 38(3): 417–423.DING L, JIANG J W, MEN J B, et al. Numerical simulation and mechanism analysis of EFP’s fracture in forming process [J]. Acta Armamentarii, 2017, 38(3): 417–423. [8] 彭建祥. 钽的本构关系研究 [D]. 绵阳: 中国工程物理研究院, 2001.PENG J X. Constitutive behavior of tantalum [D]. Mianyang: Chinese Academy of Engineering Physics, 2001. [9] 郭伟国. 锻造钽的性能及动态流动本构关系 [J]. 稀有金属材料与工程, 2007, 36(1): 23–27.GUO W G. The characteristics of forged tantalum and dynamic constitutive modelling [J]. Rare Metal Materials and Engineering, 2007, 36(1): 23–27. [10] 闫洪霞, 高重阳. BCC金属物理型动态本构关系及在钽中的应用 [J]. 兵工学报, 2010, 31(Suppl 1): 149–153.YAN H X, GAO C Y. A physically-based constitutivemodel for BCC metals and its application in tantalum [J]. Acta Armamentarii, 2010, 31(Suppl 1): 149–153. [11] GAO F, ZHANG X F, AHMAD S, et al. Dynamic behavior and constitutive model for two tantalum-tungsten alloys under elevated strain rates [J]. Rare Metal Materials & Engineering, 2017, 46(10): 2753–2762. [12] KIM J B, SHIN H. Comparison of plasticity models for tantalum and a modification of the PTW model for wide ranges of strain, strain rate, and temperature [J]. International Journal of Impact Engineering, 2009, 36(5): 746–753. doi: 10.1016/j.ijimpeng.2008.11.003 [13] JOHNSON G R, COOK W H. Fracture characteristics of three metals subjected to various strains, strain rates, temperatures and pressures [J]. Engineering Fracture Mechanics, 1985, 21(1): 31–48. doi: 10.1016/0013-7944(85)90052-9 [14] BRIDGMAN P W. Studies in large plastic flow and fracture: with special emphasis on the effects of hydrostatic pressure [M]. New York: McGraw-Hill, 1952. [15] 林莉, 支旭东, 范锋, 等. Q235B钢Johnson-Cook模型参数的确定 [J]. 振动与冲击, 2014, 33(9): 153–158, 172.LIN L, ZHI X D, FAN F, et al. Determination of parameters of Johnson-Cook models of Q235B steel [J]. Journal of Vibration and Shock, 2014, 33(9): 153–158, 172. [16] 朱志鹏. 反ERA铜钽双EFP设计技术研究 [D]. 北京: 北京理工大学, 2019: 33–37.ZHU Z P. Research on design technology of anti-ERA tantalum-copper double EFP [D]. Beijing: Beijing Institute of Technology, 2019: 33−37. -








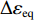
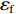
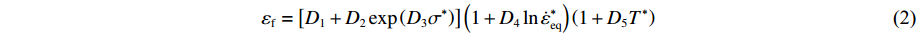
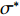


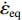
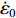


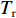

 下载:
下载:

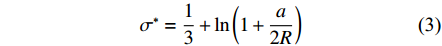
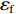
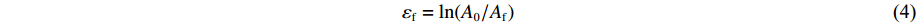

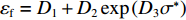





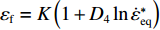
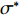


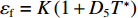

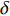




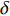
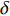
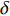
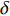

 下载:
下载: