Variation of Stress Distribution in Metal Fracture Process under Compressive, Torsional, and Tensile Loading
-
摘要: 为更好地拟合断裂准则（JC、BW、MMC）参数，借助数值模拟手段，对Q345B和921A钢的压缩、扭转、拉伸试件的断裂过程进行了模拟，以应力三轴度、Lode参数作为衡量应力状态变化的重要指标，分析了两种金属材料断裂过程中试件典型位置的变化过程及断裂时刻试件的径向分布趋势，对比分析了Q345B和921A钢的不同类型试件在不同应力三轴度、不同Lode参数表征下的结果。计算结果表明：（1）除扭转试件外，压缩、拉伸试件在断裂过程中的应力状态不断发生变化，试件断裂时刻端口剖面的应力状态分布也不一致；（2）试件断裂过程是一个应力状态不断变化的过程，采用平均应力三轴度、平均Lode参数进行描述更加妥当；（3）对于同一尺寸的拉伸试件，不同金属材料断裂过程中的平均应力三轴度不尽相同。研究成果可为后续断裂准则（JC、BW、MMC）参数的拟合及材料断裂力学性能试验的开展提供指导。Abstract: In order to accurately fit the failure criteria in JC failure model, BW failure model, and MMC failure model, numerical simulations for metal materials Q345B and 921A under various loading conditions of compression, torsion, tension were performed in this work. The variation of stresses, indicated by stress triaxiality and Lode parameter, was investigated during the fracture progress. The results indicated: (1) exclusive of torsional loading, the stress distribution varied in the cracking plane as the crack growth; (2) the average stress triaxiality and Lode parameter are more suitable for describing the stress status; (3) for specimens having the same size, the value of average stress triaxiality was dependent on metal properties. This work would provide useful knowledge for obtaining the failure criterion from material failure experiments.
-
Key words:
- failure experiments /
- stress triaxiality /
- Lode parameter /
- stress status
-
目前，人们对炸药爆轰驱动下金属飞片的运动规律开展了大量工作，如研究不同炸药的影响[1-2]、引入多种测试方法[3-4]等。基于光学多普勒效应的激光干涉测速系统(Doppler Pin System，DPS)具有高精度、非接触连续测量等特征，近年来被较多地应用在爆轰实验中[5-6]，以测试真实速度及速度变化，从而获得金属飞片从静止加速到超高速过程中速度的连续变化。越来越多的实验研究表明[7-8]，配合间隙及裱糊垫层的存在对飞片早期动力学行为产生明显影响，而装配过程中难免要引入间隙或垫层，因此阐明装配间隙或垫层对金属飞片运动特征的影响具有重要意义。
本研究拟在两类装置上开展装配垫层或间隙下炸药爆轰驱动金属飞片实验：一是在金属飞片与炸药之间装配垫层或间隙，二是在两层金属飞片之间装配垫层或间隙。通过分析DPS测得的飞片自由面速度历史，阐释两者之间的差异。
1. 实验方法
1.1 实验装置
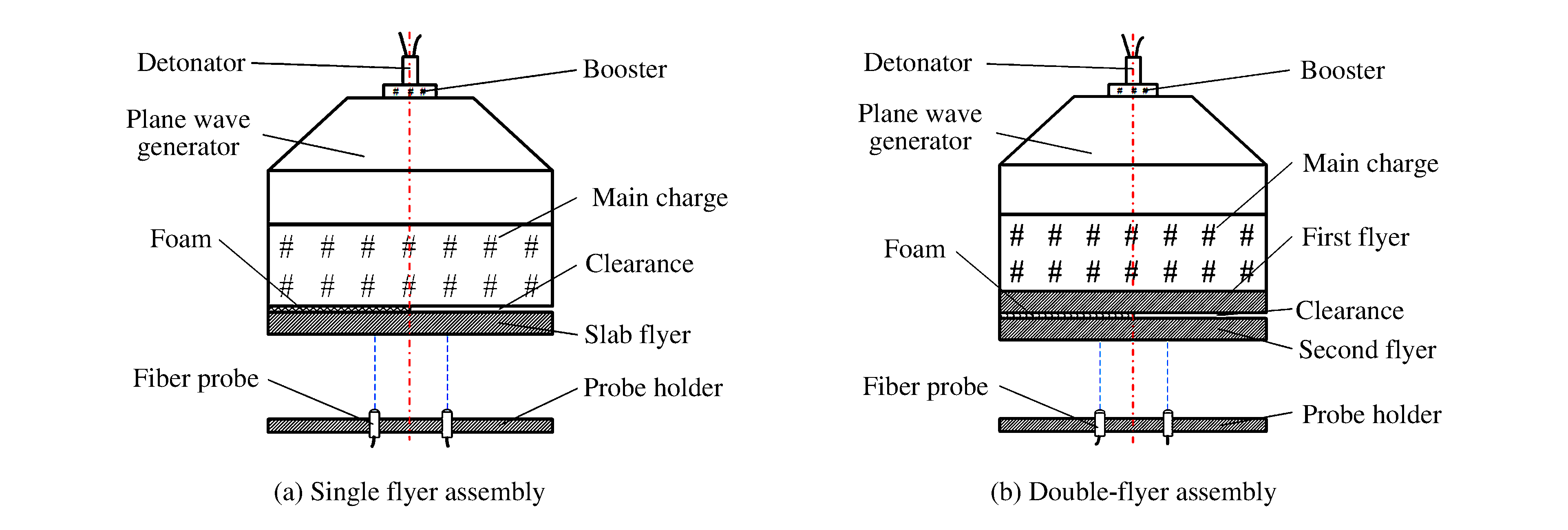
为研究装配垫层和间隙对爆轰加载下金属飞片运动特征的影响规律，设计了两套实验装置(炸药爆轰驱动单/双层飞片实验装置)，如图 1所示。两套实验装置均采用雷管起爆平面波透镜，同步传至柱形炸药，产生平面爆轰波，驱动金属飞片运动。平面波透镜的尺寸为Ø100 mm×37°；柱形主炸药为RHT-901，尺寸为Ø200 mm×20 mm。图 1(a)显示炸药爆轰驱动单层飞片实验装置，其中：飞片材料为45钢，厚度为3.5 mm；在金属飞片与柱形炸药之间，一半区域裱糊泡沫垫层，另一半区域为空气间隙；泡沫垫层和空气间隙的理论设计值为0.50 mm。该实验用于研究炸药与金属飞片之间的装配垫层和间隙对金属飞片运动特征的影响规律。图 1(b)显示炸药爆轰驱动双层飞片实验装置，其中：两层飞片材料均为45钢，厚度均为3.5 mm；在两层飞片之间，一半区域裱糊泡沫垫层，另一半区域为空气间隙；泡沫垫层和空气间隙的理论设计值也为0.50 mm；飞片与炸药直接紧密接触。该实验用于研究两层飞片之间的装配垫层和间隙对金属飞片运动特征的影响规律。
在实验装置装配过程中严格控制装配精度，通过定位销钉限制各结构之间的相对位置，保证雷管、传爆药柱、柱形炸药、泡沫垫层、金属飞片、探头定位板等满足同轴度要求。装配完成后，对泡沫垫层厚度和空气间隙进行测量，单层飞片实验装置中泡沫厚度和空气间隙为0.49 mm，双层飞片实验装置中泡沫厚度和空气间隙为0.48 mm。
1.2 测试方法
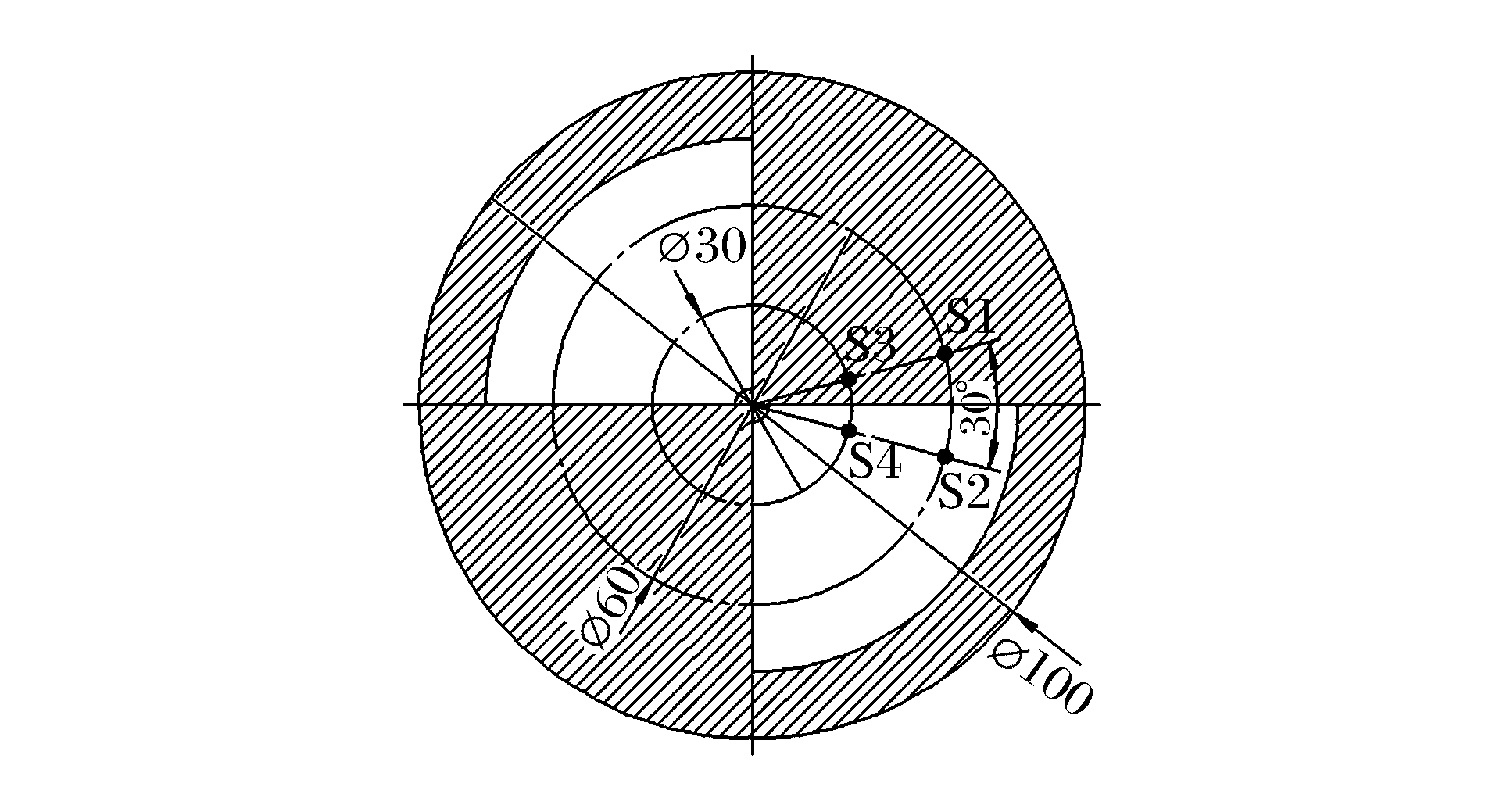
采用DPS测量金属飞片自由面速度历史。两发实验装置的测点布局相同，如图 2所示，共布置4个测点。其中测点S1和S2位于以雷管轴线为圆心的Ø60 mm圆周上，分别位于泡沫垫层区域和间隙区域，用于对比研究泡沫垫层和间隙对飞片运动规律的影响；测点S3和S4位于以雷管轴线为圆心的Ø30 mm圆周上，一方面作为测点S1和S2的复测，另一方面可与测点S1和S2的结果进行对比，分析不同半径处飞片的运动规律(确定边侧稀疏波对飞片的影响时刻)。为尽量减小装配和炸药密度不均等带来的误差，4个测点均位于泡沫垫层与空气间隙的分界面附近。
2. 结果与讨论
2.1 炸药爆轰驱动单层金属飞片测试结果分析
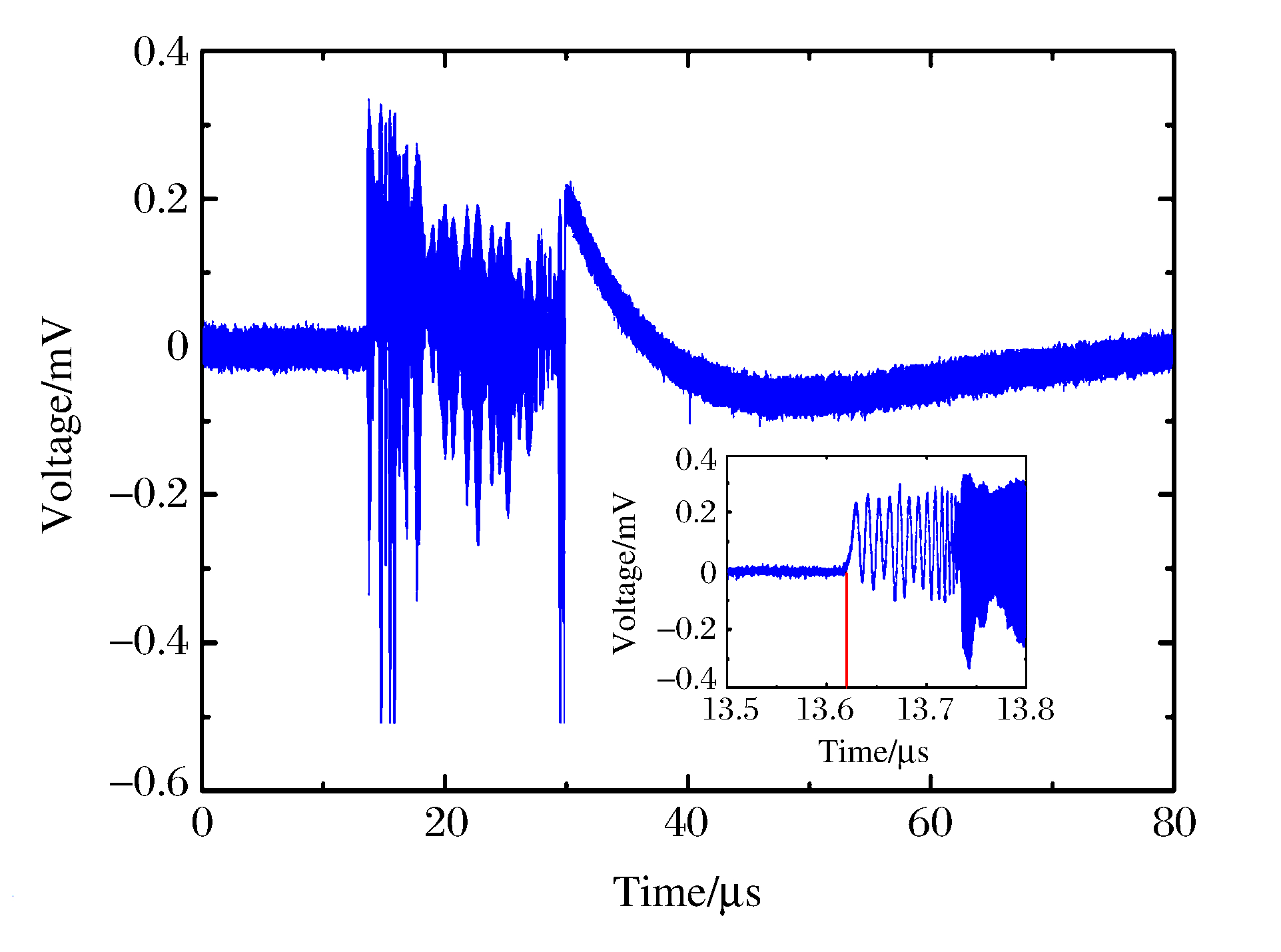
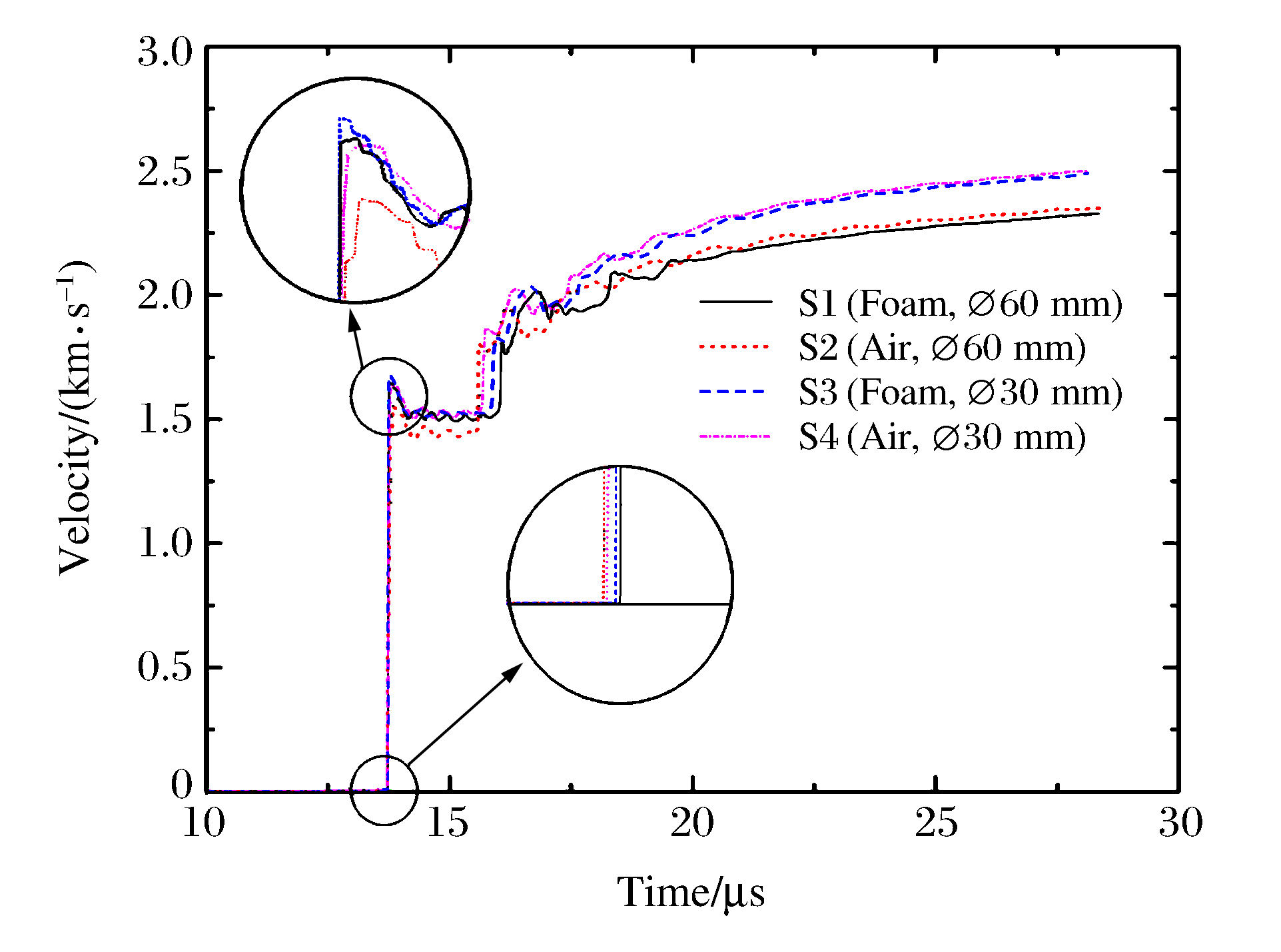
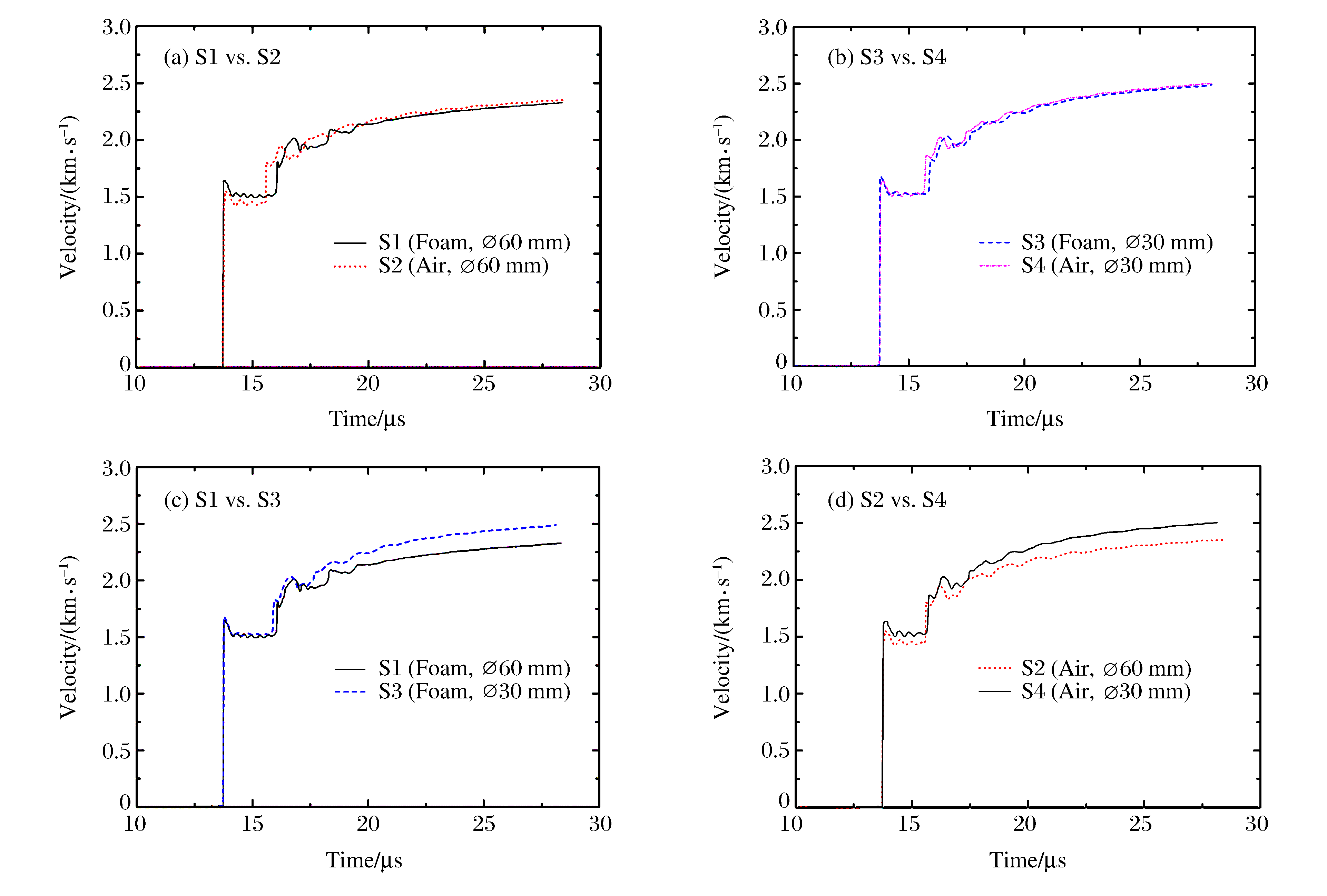
DPS测得的典型原始波形如图 3所示。对原始波形进行傅里叶变换便可得到频谱图，进而获得速度历史，如图 4所示。可见，4个测点的速度曲线的整体特征相似，但在细节上存在一些差异，主要包括速度曲线的起跳时刻、起跳幅值和末速度。在速度起跳时刻，原始波形发生两个明显变化：(1)原始波形的幅值突然增强，即干涉光的光强突然增大；(2)干涉光的频率发生突变，如图 3中插图所示。利用该特性可从原始波形上准确获取各测点速度起跳时刻。测速曲线的起跳时刻(t0)和起跳幅值(v1)列于表 1，同时表 1还列出了25 μs时刻的飞片速度(v2)，以便比较不同测点处金属飞片的后期飞行速度。
表 1 炸药爆轰驱动单层飞片实验结果Table 1. Experimental results of single flyer under detonation loadingProbe Location of test point t0/μs v1/(m·s-1) v2/(m·s-1) S1 Foam cushion, Ø60 mm 13.619 1 640 2 277 S2 Air clearance, Ø60 mm 13.589 1 447 2 303 S3 Foam cushion, Ø30 mm 13.631 1 679 2 434 S4 Air clearance, Ø30 mm 13.605 1 510 2 450 测点S1和测点S2位于相同半径处，对比测点S1与测点S2的速度历史(见图 5(a))可知：对应于泡沫区域的飞片自由面速度起跳时刻晚于空气间隙区域，起跳时刻差值约为30 ns；对应于泡沫区域的测点速度起跳幅值高于空气间隙区域，前者约为后者的1.13倍；爆轰加载后期(以t=25 μs为例)，对应于泡沫区域的金属飞片自由面速度小于空气间隙区域，前者约为后者的0.99倍。对比图 5(b)中测点S3和测点S4的速度曲线也可以得到类似结果。
图 5 单层飞片组装中各测点的速度曲线对比Figure 5. Comparison of velocity profiles at different test points in single flyer assembly速度起跳时刻的差异与爆轰产物或冲击波在间隙或泡沫垫层中的传播速度相关。在空气间隙区域，炸药爆轰后，爆轰产物高速飞行穿过空气间隙至飞片表面，驱动飞片运动，因此爆轰产物通过装配间隙的时间取决于其运动速度；在泡沫垫层区域，炸药爆轰后爆轰产物直接加载泡沫，冲击波通过泡沫传播至飞片表面，驱动飞片运动，因此冲击波通过泡沫垫层的时间取决于其在泡沫中的传播速度。测点S2的速度起跳时刻早于测点S1，可以推测出爆轰产物在0~0.5 mm厚空气间隙中的平均速度高于冲击波在泡沫垫层中的传播速度，造成对应于空气间隙区域的金属飞片较早承受加载。
速度起跳幅值的差异与爆轰产物或冲击波对金属的加载强度相关。在空气间隙区域，爆轰产物先向空气间隙卸载扩散，而后再作用于飞片；在泡沫垫层区域，爆轰产物作用于泡沫垫层形成冲击波，冲击波传播到泡沫垫层与飞片接触面，透射波加载飞片高速运动。空气间隙和泡沫垫层相当于在炸药与金属飞片之间增加一层缓冲层，测点S2的速度起跳幅值低于测点S1，说明空气间隙对爆轰产物压力的卸载作用大于泡沫垫层，缓冲作用更强。
末速度幅值的差异与炸药做功能力和飞片质量的比值相关。在炸药做功能力相同的条件下，被加载物质的质量越高，末速度越低。对应于泡沫垫层区域的飞片飞行速度低于空气间隙区域，是由于同一圆周半径处爆轰波的加载能量相等，而泡沫垫层增加了被加载物质的总质量。另一方面，二者的末速度差值极小，其原因在于增加的泡沫垫层质量相对于金属飞片质量很小。
测点S1和测点S3都对应于泡沫垫层区域，对比图 5(c)中测点S1与测点S3的速度历史可知：金属飞片内、外侧的速度起跳时刻近乎一致；外围Ø60 mm圆周上测点S1的速度起跳幅值和末速度均低于内侧Ø30 mm圆周上的测点S3，这是边侧稀疏效应的影响；自t=17.3 μs起两条速度曲线开始呈现出不同的走势，可以判定该时刻即为边侧稀疏波对飞片的影响时刻。同理，对比图 5(d)中测点S2和测点S4的速度曲线也可以得到类似结果。
2.2 炸药爆轰驱动双层金属飞片测试结果分析
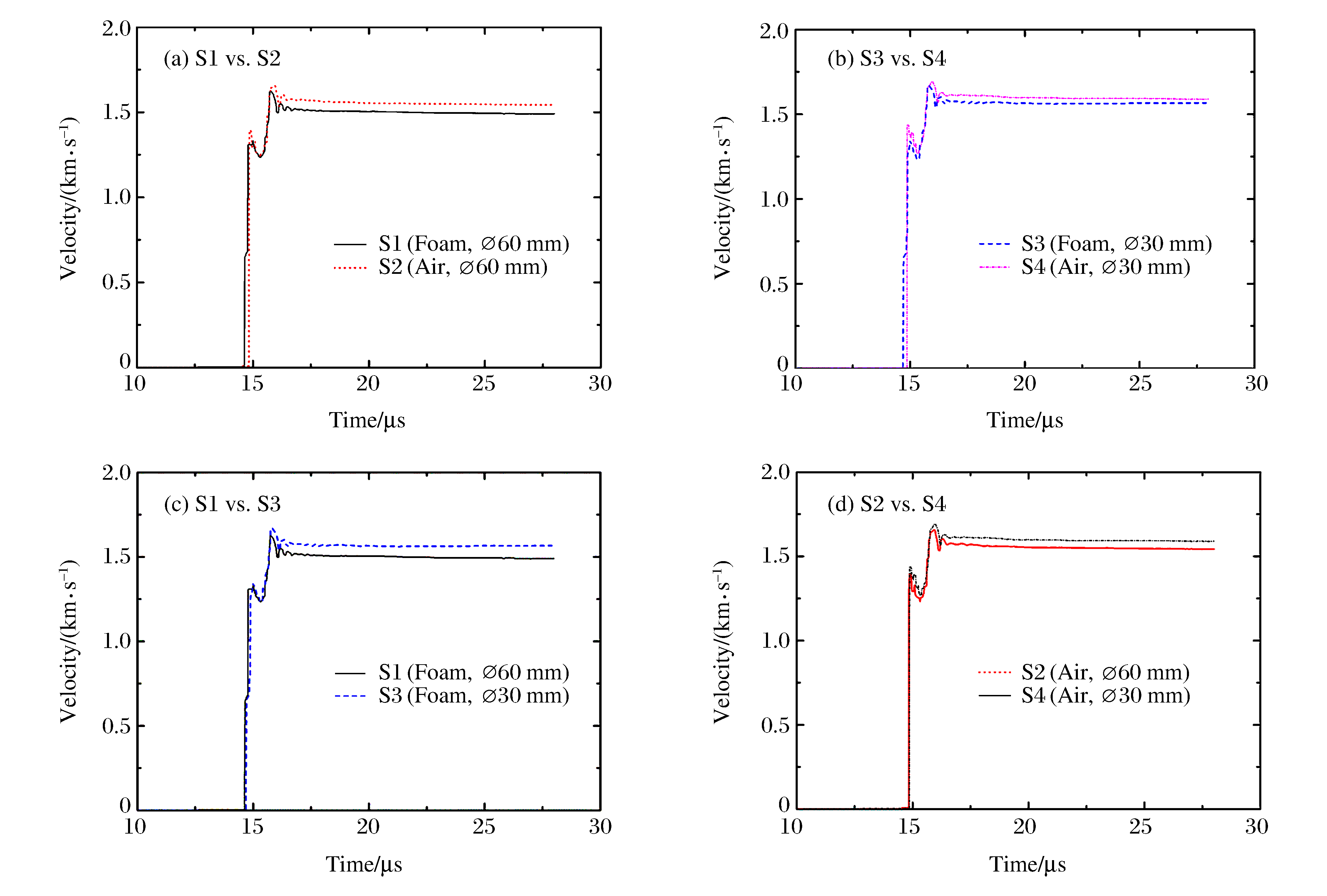
炸药爆轰驱动双层金属飞片实验获得的DPS测速结果如图 6所示，各测速曲线的起跳时刻、起跳幅值和25 μs时的速度列于表 2。
表 2 炸药爆轰驱动双层飞片实验结果Table 2. Experimental results of double-flyer under detonation loadingProbe Location of test point t0/μs v1/(m·s-1) v2/(m·s-1) S1 Foam cushion, Ø60 mm 14.483 1 307 1 494 S2 Air clearance, Ø60 mm 14.679 1 394 1 547 S3 Foam cushion, Ø30 mm 14.542 1 340 1 566 S4 Air clearance, Ø30 mm 14.721 1 439 1 593 比较图 7(a)中测点S1与测点S2的速度历史可知：对应于泡沫区域的测点速度起跳时刻早于空气间隙区域，起跳时刻差值约为200 ns；对应于泡沫区域的测点速度起跳幅值低于空气间隙区域，前者约为后者的0.94倍；爆轰加载后期(以t=25 μs为例)，对应于泡沫区域测点的速度幅值小于空气间隙区域，前者约为后者的0.97倍。对比图 7(b)中测点S3和测点S4的速度曲线也可以得到类似结果。
图 7 双层飞片组装中各测点的速度曲线对比Figure 7. Comparison of velocity profiles at different test points in double-flyer assembly当炸药与第1层飞片直接接触时，炸药爆炸产生的爆轰波传入第1层飞片并驱动其高速运动。在空气间隙区域，第1层飞片高速穿过空气间隙，撞击第2层飞片，驱动第2层飞片运动；在泡沫垫层区域，第1层飞片直接加载泡沫，应力波穿过泡沫后驱动第2层飞片。对应于泡沫垫层区域的速度起跳时刻较空气间隙区域早，说明应力波在泡沫中的传播速度高于第1层飞片在空气间隙中的飞行速度。
在空气间隙区域，由于间隙路程很短，第1层金属飞片穿过该区域时速度变化不大，而后第1层金属飞片撞击第2层飞片，第2层飞片的自由面速度相比于第1层飞片稍有降低，主要是由于冲击波传播衰减所致；而在泡沫垫层区域，泡沫相当于缓冲层，降低了应力波到达第2层飞片表面时的加载压力，造成泡沫垫层处飞片速度起跳幅值较空气间隙处低。在后续加载过程中，与炸药爆轰驱动单层金属飞片的测试结果类似，泡沫垫层处金属飞片的末速度幅值也低于空气间隙处。
对比图 7(c)中测点S1与测点S3的速度历史可知：金属飞片内、外侧的速度起跳时刻几乎一致；受边侧稀疏效应的影响，外围Ø60 mm圆周上测点S1的速度起跳幅值和末速度均低于内侧Ø30 mm圆周上的测点S3；t=16.2 μs为边侧稀疏波对飞片的影响时刻。同理，对比图 7(d)中测点S2和测点S4的速度曲线也可以得到类似结果。
2.3 空气中近RHT-901装药处冲击波速度估算
在炸药爆轰驱动单层金属飞片实验中，泡沫垫层厚度和空气间隙很小，仅为0.5 mm，可以假定在炸药爆轰驱动双层金属飞片实验中第1层飞片自由面的起跳速度近似等于(实际上稍大于)炸药驱动单层金属飞片自由面的速度起跳幅值，即约为1 679 m/s(取最大值)。根据两发实验中泡沫垫层与空气间隙区域的速度起跳时刻差异以及泡沫和空气间隙厚度，可以计算出应力波在泡沫垫层中的传播速度约为4 879 m/s，在0~0.5 mm区间爆轰产物向空气中飞散的平均速度约为6 765 m/s，则爆轰产物向空气中飞散的初始速度应大于6 765 m/s。大量实验研究表明[9]，爆轰产物向空气中飞散时的初始速度接近炸药的爆轰速度(RHT-901炸药的爆轰速度为7.86 km/s[10])，该结果进一步验证了2.1节和2.2节关于速度起跳时刻差异解释的正确性。
3. 结论
对炸药爆轰驱动金属飞片开展了较为精密的实验研究，结果表明装配间隙和垫层对飞片运动特征(包括起跳时间、速度起跳幅值等)产生明显影响，具体结论如下：
(1) 在炸药与金属飞片之间装配垫层或间隙时，相比于空气间隙区域，泡沫垫层区域金属飞片的速度起跳时刻较晚(约30 ns)，速度起跳幅值较高(约1.13倍)，末速度较低；
(2) 在两层金属飞片之间装配垫层或间隙时，相比于空气间隙区域，泡沫垫层区域金属飞片的速度起跳时刻较早(约200 ns)，速度起跳幅值较低(约0.94倍)，末速度较低；
(3) 在0~0.5 mm区间，RHT-901爆轰产物向空气中飞散的平均速度高于应力波在泡沫垫层中的传播速度。
-
图 1 光滑圆棒拉伸试件尺寸（单位：mm）
Figure 1. Dimensions of round smooth tension specimen (Unit: mm)
图 2 缺口圆棒拉伸试件尺寸（单位：mm）
Figure 2. Dimensions of notched round tension specimen (Unit: mm)
图 6 试验和有限元计算得到的载荷-位移曲线对比（Q345B钢）
Figure 6. Comparisons of the displacement-load curves between experimental results and simulation results（Q345B steel）
图 7 试验和有限元计算得到的载荷-位移曲线对比（921A钢）
Figure 7. Comparisons of the displacement-load curves between experimental results and simulation results（921A steel）
图 8 压缩试件受力过程中应力三轴度和Lode参数变化（921A钢）
Figure 8. Changes of stress triaxiality and Lode value in the loading process of compression specimen (921A steel)
图 9 扭转试件受力过程中应力三轴度和Lode参数变化（921A钢）
Figure 9. Changes of stress triaxiality and Lode value in the loading process of torsion specimen (921A steel)
图 10 光滑拉伸试件受力过程中应力三轴度和Lode参数变化（921A钢）
Figure 10. Changes of stress triaxiality and Lode value in the loading process of smooth tension specimen (921A steel)
图 11 8 mm缺口拉伸试件受力过程中应力三轴度和Lode参数变化（921A钢）
Figure 11. Changes of stress triaxiality and Lode value in the loading process of tension specimen with a 8 mm notch (921A steel)
图 12 断裂时刻压缩试件应力三轴度和Lode参数的径向分布（Q345B钢）
Figure 12. Radial distribution of stress triaxiality and Lode value of the compressive specimen at final failure（Q345B steel）
图 13 断裂时刻扭转试件应力三轴度和Lode参数的径向分布（Q345B钢）
Figure 13. Radial distribution of stress triaxiality and Lode value of the torsion specimenat final failure（Q345B steel）
图 14 断裂时刻光滑拉伸试件应力三轴度和Lode参数的径向分布（Q345B钢）
Figure 14. Radial distribution of stress triaxiality and Lode value of the smooth tension specimen at final failure（Q345B steel）
图 15 断裂时刻带有2 mm缺口的拉伸试件的应力三轴度和Lode参数径向分布（Q345B钢）
Figure 15. Radial distribution of stress triaxiality and Lode value of the tensile specimen with a 2 mm notch at final failure（Q345B steel）
图 16 不同试件断裂时刻应力三轴度、平均应力三轴度增加百分比的对比
Figure 16. Increases of the stress triaxiality and Lode parameter for specimens at final failure
表 1 JC强度模型参数
Table 1. Parameters of JC strength model
Material A/MPa B/MPa n C ˙ε/s−1 Q345B steel 360 700 0.547 0.046 0.002 921A steel 700 706 0.580 0.013 0.002 
下载: 导出CSV
表 2 各类试件应力三轴度不同表征方法对比
Table 2. Comparison of the stress triaxiality among different specimens
Specimen type Stress triaxiality (Q345B steel) Stress triaxiality (921A steel) Initial Fracture Average Initial Fracture Average Compression −0.333 0.179 −0.120 −0.333 0.156 −0.116 Torsion 0 0 0 0 0 0 Smooth tension 0.333 0.841 0.562 0.333 0.987 0.668 With a 18 mm notch 0.413 0.867 0.663 0.413 1.075 0.825 With a 8 mm notch 0.505 0.915 0.752 0.505 1.133 0.919 With a 6 mm notch 0.556 0.947 0.805 0.556 1.160 0.973 With a 2 mm notch 0.893 1.123 1.085 0.893 1.451 1.400
下载: 导出CSV
表 3 各类试件Lode参数不同表征方法对比
Table 3. Comparison of the Lode parameter among different specimens
Specimen type Lode (Q345B steel) Lode (921A steel) Initial Fracture Average Initial Fracture Average Compression 1.00 −0.73 0.24 1.00 −0.22 0.45 Torsion 0 0 0 0 0 0 Smooth tension −1.00 −1.00 −1.00 −1.00 −1.00 −1.00 With a 18 mm notch −1.00 −1.00 −1.00 −1.00 −1.00 −1.00 With a 8 mm notch −1.00 −1.00 −1.00 −1.00 −1.00 −1.00 With a 6 mm notch −1.00 −1.00 −1.00 −1.00 −1.00 −1.00 With a 2 mm notch −1.00 −1.00 −1.00 −1.00 −1.00 −1.00
下载: 导出CSV
表 4 拟合JC参数取值
Table 4. JC failure model constant
Material D1 D2 D3 Q345B steel 1.816 −0.353 1.056 921A steel 2.475 −0.661 0.650
下载: 导出CSV
-
[1] JOHNSON G R, COOK W H. A constitutive model and data for metals subjected to large strains, high strain rates and high temperatures [C]//Proceeding of the 7th International Symposium on Ballistic. The Hague, Netherlands, 1983: 541–547. [2] BAO Y B, WIERZBICKI T. A comparative study on various ductile crack formation criteria [J]. Journal of Engineering Material and Technology, 2004, 126: 314–324. doi: 10.1115/1.1755244 [3] BAO Y B, WIERZBICKI T. Application of extended Mohr-Coulomb criterion to ductile fracture [J]. International Journal of Fracture, 2010, 161(1): 1–20. doi: 10.1007/s10704-009-9422-8 [4] BORVIK T, HOPPERSTAD O S. A computational model of viscoplasticity and ductile damage for impact and penetration [J]. European Journal of Mechanics Solids, 2001, 20(5): 685–712. doi: 10.1016/S0997-7538(01)01157-3 [5] GUPTA N K, IQBAL M A. Experiment and numerical studies on the behavior of thin aluminum plates subjected to impact by blunt and hemispherical-nosed projectile [J]. International Journal of Impact Engineering, 2006, 32(12): 1921–1944. doi: 10.1016/j.ijimpeng.2005.06.007 [6] 肖新科, 王要沛, 王爽, 等. 应力状态在球形弹丸撞击6061-T6铝薄靶弹道行为数值预报中的作用 [J]. 振动与冲击, 2015, 34(22): 87–91.XIAO X K, WANG Y P, WANG S, et al. Effect of stress state on the numerical prediction of ballistic resistance of thin 6061-T6 aluminum alloy targets against sphere projectile impacts [J]. Journal of Vibration and Shock, 2015, 34(22): 87–91. [7] 肖新科, 王要沛, 张伟. 应力状态在2024-T351 Taylor杆断裂行为数值预报中的作用 [J]. 北京理工大学学报, 2016, 36(1): 157–161.XIAO X K, WANG Y P, ZHANG W. Effect of stress state on the numerical prediction of the fracture behavior of 2064-T351 aluminium alloy Taylor rods [J]. Transactions of Beijing Institute of Technology, 2016, 36(1): 157–161. [8] BORVIK T, HOPPERSTAD O S. Numerical simulation of plugging failure in ballistic penentrtion [J]. International Journal of Solids and Structures, 2001, 38(25): 6241–6264. [9] BAO Y B, WIERZBICKI T. On fracture locus in the equivalent strain and stress triaxiality space [J]. International Journal of Mechanical Sciences, 2004, 46(12): 81–98. [10] GILIOLI A, WIERZBICKI T. Predicting ballistic impact failure of aluminium 6061-T6 with the rate-independent Bao-Wierzbicki fracture model [J]. International Journal of Impact Engineering, 2015, 76(15): 207–220. [11] TENG X, WIERZBICKI T. Evaluation of six fracture models in high velocity perforation [J]. Engineering Fracture Mechanics, 2006, 73(12): 1653–1678. [12] 李营. 液舱防爆炸破片侵彻作用机理研究 [D]. 武汉: 武汉理工大学, 2014.LI Y. Fragment resistant mechanism research of safety liquid cabin [D]. Wuhan: Wuhan University of Technology, 2014. [13] 孟利平. 应变率和应力三轴度对船用钢变形和断裂的影响研究 [D]. 无锡: 中国船舶科学研究中心, 2016.MENG L P. Influence of strain rate and stress triaxiality on the deformation and fracture behavior of ship hull steel [D]. Wuxi: China Ship Scientific Research Center, 2016. [14] BAO Y B, WIERZBICKI T. On fracture locus in the equivalent strain and stress triaxiality space [J]. International Journal of Mechanical Sciences, 2004, 46(1): 81–98. doi: 10.1016/j.ijmecsci.2004.02.006 [15] BAO Y B, WIERZBICKI T. On the cut-off value of negative triaxiality for fracture [J]. Engineering Fracture Mechanics, 2005, 72(7): 1049–1069. doi: 10.1016/j.engfracmech.2004.07.011 -
下载:
下载:
点击查看大图
计量
- 文章访问数: 6357
- HTML全文浏览量: 3247
- PDF下载量: 53
