Dynamic Response of Hollow Tempered Laminated Glass
-
摘要: 利用DHR-9401型落锤冲击试验机,结合最小碎裂能方法,研究了中空钢化夹层玻璃的厚度、结构配置对其抗冲击性能的影响,并从冲击力载荷峰值、能量吸收率、应变等方面对影响效应进行评估。实验结果表明:玻璃作为日常生活中的承载结构,其厚度和结构配置对性能有很大影响,在控制试样总厚度相同或不同的前提下,随着冲击层厚度的增加,中空钢化夹层玻璃的抗冲击性能明显提高;在试样总厚度不同的前提下,随着内层玻璃厚度的增加,中空钢化夹层玻璃的承载能力显著提高。Abstract: In order to study the influence of thickness and structure configuration of hollow tempered laminated glass on its impact resistance, DHR-9401 drop hammer impact testing machine combined with the method of minimum fragmentation energy was used in this experiment, and the impact effect was evaluated from the impact load peak, energy absorption rate and strain. The experimental results show that the glass as a bearing structure in daily life, its thickness and configuration have a great influence on its performance. Under the condition that the total thickness of the sample is the same or different, with the increase of the thickness of the impact layer, the impact resistance of the hollow tempered laminated glass is obviously improved. Under the premise that the total thickness of the sample is different, with the increase of the thickness of the inner glass, the bearing capacity of the hollow tempered laminated glass is improved obviously.
-
稀土六硼化物LaB6具有较低的逸出功和低蒸发率等优异的电子发射性能,同时还具有熔点高、化学稳定性强、硬度高等特点,因此自Lafferty发现LaB6的热发射性能以来一直是电子材料及器件领域的研究热点[1-3]。人们对LaB6晶体材料的制备和表征及其多元硼化物开展了较多的研究工作,例如:张宁等[4]通过悬浮区熔法制备了高质量LaB6单晶体;包黎红等[5]制备了La0.6Ce0.4B6材料,硬度达到2.31 GPa,1873 K下的最高发射电流密度达40.7 A/cm2;刘洪亮等[6]通过测试发现,LaB6晶体材料(100)晶面具有最佳的发射性能,最大发射电流密度在1773 K时可达40.4 A/cm2。然而,高致密度的LaB6晶体材料的烧结制备较为困难(致密度较低,80%~92%),所得材料的力学性能较差[7]。当前,关于LaB6晶体材料力学性能的理论研究报道较少,为此本研究基于密度泛函理论,结合Birch-Murnaghan物态方程,系统研究LaB6晶体材料的弹性性质及其他力学性能,以期为LaB6的深入研究和应用提供参考。
1. 计算方法与过程
计算中,将芯电子及核视为原子核,用Vanderbilt超软势近似其对外层电子的作用,外层电子设为La(5s25p65d16s2)、B(2s2 2p1),电子波函数采用平面波基矢组[8]。电子交换关联项采用广义梯度近似法(General Gradient Approximation,GGA)中的PBE(Perdew Burke Ernzerhof)泛函近似。首先对晶格结构进行充分弛豫,在此过程中固定晶体的对称性,允许原子在3个方向上弛豫。考虑到La d电子的在位库仑相互作用,计算时将其作用项设置为2.5 eV。计算弹性常数时,每个原子的能量收敛精度设置为2×10–6 eV,最大受力收敛精度设置为0.06 eV/nm;应力-应变计算中,原子最大位移的收敛精度设置为2×10–5 nm;电子结构计算中,电子平面波矢组基矢截止能量设为240 eV,布里渊区k点的自动生成采用Monkhorst-Pack法,k点网格密度为4×4×4。
根据胡克定律,固体材料在弹性形变范围内所受的应力与应变之间符合
S=Cε(1) (1) 式中:S为应力,
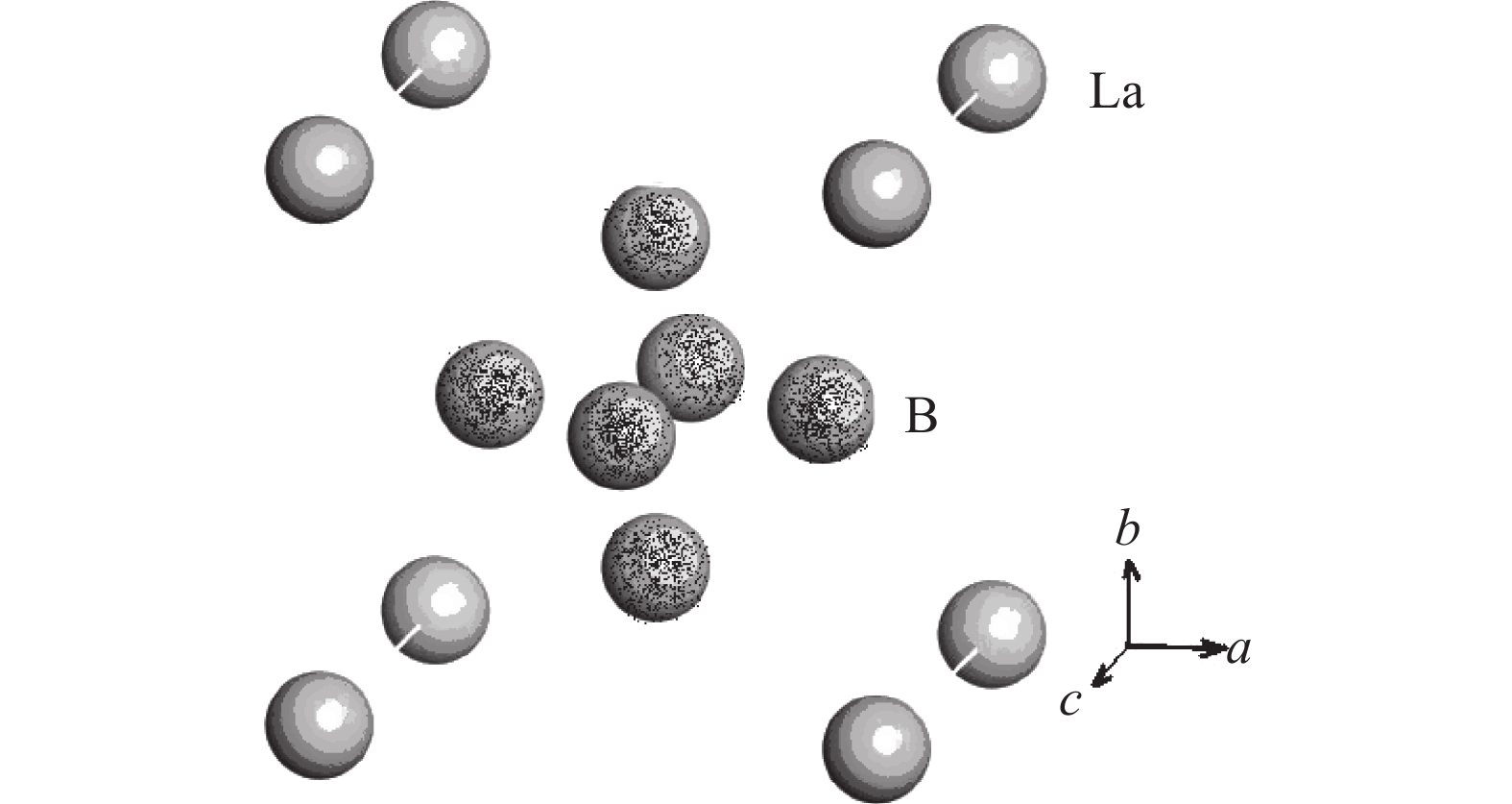
C 为弹性常数,ε 为应变。广义上晶体材料中的应力和应变都是二阶张量,因此弹性常数c 为六阶张量,其中独立分量数为36个,即[S1S2S3S4S5S6]=[C11C12C13C14C15C16C21C22C23C24C25C26C31C32C33C34C35C36C41C42C43C44C45C46C51C52C53C54C55C56C61C62C63C64C65C66][ε1ε2ε3ε4ε5ε6](2) (2) 由于晶体具有结构对称性(见图1),因此弹性常数张量矩阵可化为一个具有21个独立分量的矩阵[8],考虑到LaB6为立方晶系,空间群为
Pmˉ3m ,因此其弹性常数张量矩阵具有3个独立分量,即C11 、C44 、C12 。采用密度泛函理论和B-M物态方程研究LaB6的弹性常数,基于Voigt方法、Reuss方法和Hill方法[9]对体弹性模量和剪切弹性模量进行计算分析,采用压缩各向因子衡量弹性的各向异性,采用Tian等[10]的维氏硬度方法分析硬度,并采用泊松比和各向异性因子对其延脆性进行分析[10-11]。2. 结果与讨论
计算分析得到的LaB6晶体材料的晶格参数列于表1。从表1中可以看出,初始结构经过充分弛豫之后,计算得到的晶格参数与实验值之间的相对误差均小于3%,说明计算分析过程所用参数较为合理。
表 1 LaB6晶体材料的晶格参数Table 1. Structural parameters of LaB6 crystalline materialMethod a/nm b/nm c/nm α/(°) β/(°) γ/(°) Experiment 0.415 49 0.415 49 0.415 49 90 90 90 Calculation 0.420 205 0.420 205 0.420 205 90 90 90 通过计算得到LaB6晶体材料的弹性常数参数C11、C12和C44分别为436.92、22.37和47.64 GPa,
C11 较大,表明在此主轴应力方向上LaB6晶体具有较大的弹性常数和体弹性模量。根据立方晶体的力学稳定性判定公式[12],经过计算分析得出LaB6晶体的弹性常数参数满足C11>0,C44>0,C11>|C12|,C11+2C12>0 (3) 表明本研究所用晶格结构为力学稳定的晶体结构。
分别采用Voigt法、Reuss法和Hill法,根据以下公式,计算LaB6晶体材料的体弹性模量和剪切弹性模量
BV=(C11+2C12)/3 (4) GV=(C11−C12+3C44)/5 (5) BR=(C11+2C12)/3 (6) GR=5(C11−C12)C44/[4C44+3(C11−C12)] (7) BH=(BR+BV)/2 (8) GH=(GR+GV)/2 (9) 式中:B为体弹性模量,G为剪切弹性模量,下标V、R、H分别表示Voigt法、Reuss法和Hill法。计算结果列于表2,可见LaB6晶体具有较大的体弹性模量。由于LaB6晶体在a、b、c方向上具有各向同性,因此采用Voigt方法和Reuss方法计算所得体弹性模量具有相同的数值。
表 2 LaB6晶体材料的体弹性模量和剪切弹性模量Table 2. Bulk modulus and shear modulus of LaB6 crystalline materialBV/GPa BR/GPa BH/GPa GV/GPa GR/GPa GH/GPa 160.55 160.55 160.55 111.49 68.85 90.17 杨氏模量E可用于衡量固体材料的刚度,其值越大,刚性越强。泊松比
γ 可用于衡量固体材料抵抗切应变的能力,数值一般在–1~0.5之间,值越大,延性越好,一般认为γ <1/3时材料呈脆性,γ >1/3时呈韧性。体弹性模量与剪切弹性模量的比值λ可用于衡量固体材料的脆性和延展性,其分界值一般是1.75,小于此值时材料明显呈脆性,大于此值时则呈延性。弹性各向异性因子A在0~1之间,A=0表明材料具有各向同性,A=1表明具有最大的各向异性。根据微观原子结合键强度理论计算出的硬度适合分析含有d态电子体系的抗剪性,且其值越高,表明抵抗变形的能力越强[11]。采用以下公式计算LaB6晶体材料的杨氏模量E、泊松比
γ 、体剪弹性模量比λ(λ=B/G)、体弹性模量各向异性因子AB、剪切弹性模量各向异性因子AG和硬度H。E=9BG/(3B+G) (10) γ=(3B−2G)/(6B+2G) (11) AB=(BV−BR)/(BV+BR) (12) AG=(GV−GR)/(GV+GR) (13) H=0.92λ−1.137G0.708 (14) λ=B/G (15) 表3列出了计算分析结果,可以看出:LaB6晶体材料的杨氏模量为227.85 GPa,远大于一些金属的杨氏模量,与钢材的杨氏模量接近,表明LaB6不易发生弹性形变,刚性较强;其泊松比为0.26,表明LaB6具有一定的脆性;体剪弹性模量上限值的比值λ为1.44,小于1.75,与泊松比计算分析结果吻合;体弹性模量各向异性因子AB=0,剪切弹性模量各向异性因子AG=0.24,表明LaB6的体弹性具有各向同性,而剪切弹性具有一定的各向异性;理论硬度H达到11.56 GPa,表明LaB6抵抗剪切形变的能力较强。
表 3 LaB6晶体材料的力学性能参数和弹性波速Table 3. Mechanical parameters and elastic velocities of LaB6 crystalline materialE/GPa γ λ AB AG H/GPa vl/(km·s–1) vt/(km·s–1) vm/(km·s–1) 227.85 0.26 1.44 0 0.24 11.56 7.72 4.38 4.87 采用以下公式[13-14]计算LaB6晶体材料的纵波弹性波速vl、剪切弹性波速vt和平均弹性波速vm
vl=√(B+43G)1ρ,vt=√Gρ,vm=[13(2v3t+1v3l)]−13 (16) 计算结果如表3所示。LaB6晶体材料的3支弹性波中,有1支纵波和2支横波。从表3可以看出,LaB6晶体的纵波弹性波速(7.72 km/s)较大,剪切弹性波速(4.38 km/s)相对较小,平均波速达到4.87 km/s。在LaB6晶体材料的长波极限,声学波的纵波支速度较大,横波支速度相对较小,表明原子相对运动的振动波速较大。
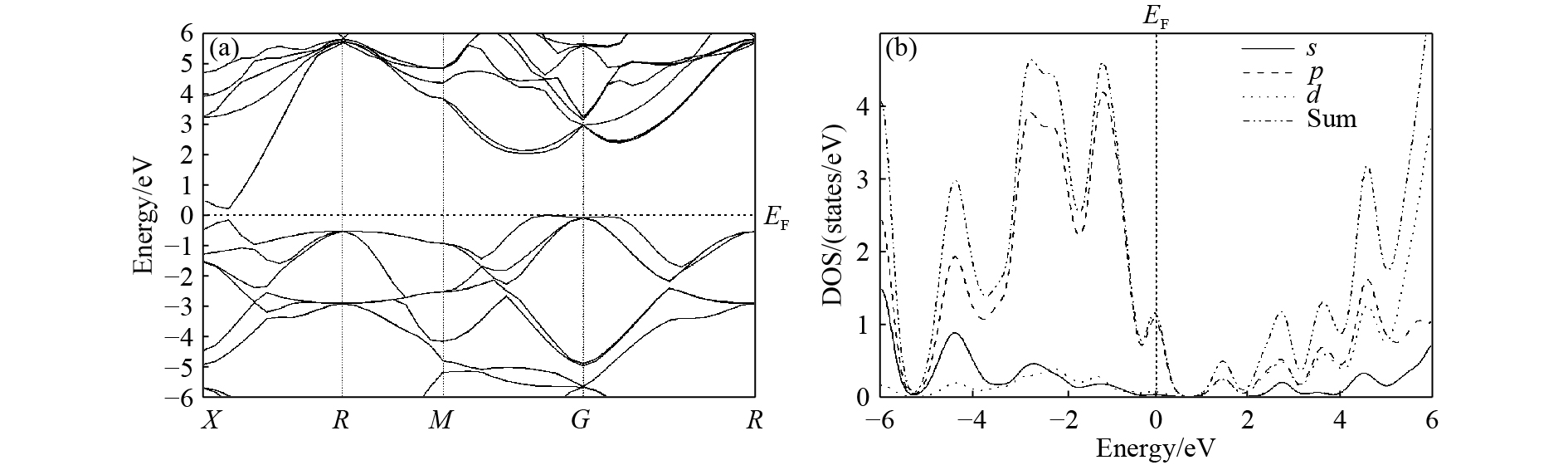
计算得到的LaB6晶体材料的能带结构和分态密度如图2所示。从图2中可以看出,LaB6具有较窄的带隙,带隙宽度为0.20 eV,费米能级穿过价带,表明LaB6呈金属性。从分态密度图可以看出,曲线具有多个峰值,表明LaB6内部电子具有较强的局域性,这也是稀土La化合物特有的性质。结合能带结构和分态密度可以看出,LaB6材料价带顶的能带由p、d和s电子形成,导带底的能带由p和d电子形成,其中p态电子对价带顶和导带底的形成起最重要的作用。计算分析得到的LaB6晶体材料各轨道的电荷转移分布情况如表4所示。可见,La的s轨道和p轨道的电子转移至d轨道和B原子上,B得到La的电子,其s轨道的电子转移至p轨道,因此La呈2.53价而B呈–0.42价,表明La和B之间具有较强的共价键成分。La和B的这种结合也表明LaB6具有较高的体弹性模量、杨氏模量和硬度,同时其离子键成分使其具有一定的脆性。
表 4 LaB6晶体材料的电荷转移Table 4. Charge distributions of LaB6 crystalline materialAtom Charge distribution/e s orbital p orbital d orbital Total charge B 0.88 2.54 0.00 –0.42 La 1.50 5.46 1.51 2.53 3. 结 论
基于密度泛函理论和Birch-Murnaghan物态方程,系统分析了LaB6晶体材料的弹性常数参数、体弹性模量、剪切弹性模量和力学性能。结果表明:LaB6晶体材料具有较大的
C11 ,表明在此主轴应力方向上具有较大的弹性常数;LaB6晶体具有较大的体弹性模量,并且体弹性模量呈各向同性,而剪切弹性模量呈各向异性;LaB6晶体的杨氏模量为227.85 GPa,泊松比为0.26,体剪弹性模量比值λ达到1.44,表明其脆性较强,不易发生弹性形变;LaB6晶体的硬度达到11.56 GPa,平均弹性波速达4.87 km/s;LaB6晶体呈金属性,带隙宽度为0.20 eV,内部电子具有较强的局域性,La和B之间具有较强的共价键成分。 -
表 1 方案1结构配置
Table 1. Structure configuration of scheme 1
Group No. dt/mm Serial No. d/mm ds/mm Glass PVB Glass Air Glass PVB Glass 1 41.04 D4(1) 6 1.52 8 6 8 1.52 10 6 41.04 D1 8 1.52 8 6 8 1.52 8 8 41.04 D4(2) 10 1.52 8 6 8 1.52 6 10 2 49.04 D8(1) 8 1.52 10 6 10 1.52 12 8 49.04 D7 10 1.52 10 6 10 1.52 10 10 49.04 D8(2) 12 1.52 10 6 10 1.52 8 12 表 2 方案2结构配置
Table 2. Structure configuration of scheme 2
Group No. dt/mm Serial No. d/mm ds/mm Glass PVB Glass Air Glass PVB Glass 3 41.04 D1 8 1.52 8 6 8 1.52 8 8 45.04 D6 10 1.52 8 6 8 1.52 10 10 49.04 D10 12 1.52 8 6 8 1.52 12 12 4 41.04 D2 6 1.52 10 6 10 1.52 6 6 45.04 D5 8 1.52 10 6 10 1.52 8 8 49.04 D7 10 1.52 10 6 10 1.52 10 10 表 3 方案3结构配置
Table 3. Structure configuration of scheme 3
Group No. dt/mm Serial No. d/mm ds/mm Glass PVB Glass Air Glass PVB Glass 5 41.04 D1 8 1.52 8 6 8 1.52 8 8 45.04 D5 8 1.52 10 6 10 1.52 8 8 49.04 D9 8 1.52 12 6 12 1.52 8 8 6 41.04 D3 10 1.52 6 6 6 1.52 10 10 45.04 D6 10 1.52 8 6 8 1.52 10 10 49.04 D7 10 1.52 10 6 10 1.52 10 10 -
[1] LI M X, LU G J, HU Z R, et al. Research on fire endurance of tempered glass based on infrared imaging technology [J]. Procedia Engineering, 2014, 84: 553–557. doi: 10.1016/j.proeng.2014.10.467 [2] ZHANG X H, HAO H, WANG Z Q. Experimental investigation of monolithic tempered glass fragment characteristics subjected to blast loads [J]. Engineering Structures, 2014, 75: 259–275. doi: 10.1016/j.engstruct.2014.06.014 [3] THOMPSON D, BROWN S, MALLONEE S, et al. Fatal and non-fatal injuries among US air force personnel resulting from the terrorist bombing of the Khobar Towers [J]. Journal of Trauma Injury Infection and Critical Care, 2004, 57(2): 208–215. doi: 10.1097/01.TA.0000142672.99660.80 [4] 马志宏. 化学钢化玻璃 [J]. 中国玻璃, 2012(4): 45–47.MA Z H. Chemically toughened glass [J]. China Glass, 2012(4): 45–47. [5] 郑立新. 中空玻璃在我国的应用与发展 [J]. 科技创新导报, 2008(13): 7. doi: 10.3969/j.issn.1674-098X.2008.13.005ZHENG L X. Application and development of insulating glass in China [J]. Science and Technology Innovation Herald, 2008(13): 7. doi: 10.3969/j.issn.1674-098X.2008.13.005 [6] CASTORI G, SPERANZINI E. Structural analysis of failure behavior of laminated glass [J]. Composites Part B: Engineering, 2017, 125: 89–99. doi: 10.1016/j.compositesb.2017.05.062 [7] CHEN X D, CHAN A H C, YANG J. FEM/DEM modelling of hard body impact on the laminated glass [J]. Applied Mechanics and Materials, 2014, 553: 786–791. doi: 10.4028/www.scientific.net/AMM.553.786 [8] 郑金峰. 公共建筑节能设计标准与玻璃幕墙 [J]. 建设科技, 2005(20): 37–38. doi: 10.3969/j.issn.1671-3915.2005.20.015ZHENG J F. Design standards for energy saving in public buildings and glass curtain wall [J]. Construction Science and Technology, 2005(20): 37–38. doi: 10.3969/j.issn.1671-3915.2005.20.015 [9] 安二峰, 李磊, 杨军. 典型玻璃材料冲击力学性能研究 [J]. 北京理工大学学报, 2010, 30(2): 127–130.AN E F, LI L, YANG J. A study on the impact properties of typical glassy materials [J]. Transactions of Beijing Institute of Technology, 2010, 30(2): 127–130. [10] 刘永华, 祖成奎, 包亦望, 等. 无碱铝硅酸盐钢化玻璃的安全性评价 [J]. 硅酸盐通报, 2011, 30(6): 1264–1267.LIU Y H, ZU C K, BAO Y W, et al. Safety evaluation of alkali-free alumina silicate glass [J]. Bulletin of the Chinese Ceramic Society, 2011, 30(6): 1264–1267. [11] MOHAGHEGHIAN I, WANG Y, JIANG L, et al. Quasi-static bending and low velocity impact performance of monolithic and laminated glass windows employing chemically strengthened glass [J]. European Journal of Mechanics A/Solids, 2017, 63: 165–186. doi: 10.1016/j.euromechsol.2017.01.006 [12] CHEN J J, XU J, YAO X F, et al. Experimental investigation on the radial and circular crack propagation of PVB laminated glass subject to dynamic out-of-plane loading [J]. Engineering Fracture Mechanics, 2013, 112/113: 26–40. doi: 10.1016/j.engfracmech.2013.09.010 [13] 韩阳. 冲击载荷下中空钢化和中空钢化夹层玻璃动态响应研究 [D]. 太原: 太原理工大学, 2019.HAN Y. Dynamic response of hollow toughened glass and hollow toughened laminated glass under impact loading [D]. Taiyuan: Taiyuan University of Technology, 2019. [14] 凌洁, 陈峻. 中国中央电视台(CCTV)新台址工程玻璃幕墙设计 [J]. 上海建设科技, 2006(2): 38–40. doi: 10.3969/j.issn.1005-6637.2006.02.012LING J, CHEN J. Curtain wall glass design of new constructed China central television station project [J]. Shanghai Construction Science & Technology, 2006(2): 38–40. doi: 10.3969/j.issn.1005-6637.2006.02.012 [15] 张杨梅, 王星尔, 杨健, 等. 多层SGP夹层玻璃受刚体冲击作用的实验研究 [J]. 无机材料学报, 2018, 33(10): 1110–1118. doi: 10.15541/jim20170595ZHANG Y M, WANG X E, YANG J, et al. Experimental study of multiple layered SGP laminated glass under hard body impact [J]. Journal of Inorganic Materials, 2018, 33(10): 1110–1118. doi: 10.15541/jim20170595 [16] CHOCRON S, DANNEMANN K A, WALKER J D, et al. Static and dynamic confined compression of borosilicate glass [C]// DYMAT 2009-9th International Conference on the Mechanical and Physical Behaviour of Materials under Dynamic Loading. France: EDP Sciences, 2009: 67–72. [17] TANG T, ZHANG W G, YIN H F, et al. Crushing analysis of thin-walled beams with various section geometries under lateral impact [J]. Thin-Walled Structures, 2016, 102(1): 43–57. [18] 李胜杰. 爆炸载荷下夹层玻璃的动态响应及裂纹扩展的研究 [D]. 太原: 太原理工大学, 2015.LI S J. Study on the dynamic response and cracks propagation of laminated glass subjected to blast load [D]. Taiyuan: Taiyuan University of Technology, 2015. [19] SINGH R P, KAVATURU M, SHUKLA A. Initiation, propagation and arrest of an interface crack subjected to controlled stress wave loading [J]. International Journal of Fracture, 1997, 83(3): 291–304. doi: 10.1023/A:1007358901588 [20] XU J, LI Y B, CHEN X, et al. Characteristics of windshield cracking upon low-speed impact: numerical simulation based on the extended finite element method [J]. Computational Materials Science, 2010, 48(3): 582–588. doi: 10.1016/j.commatsci.2010.02.026 [21] BOUMBIMBA R M, COULIBALY M, KHABOUCHI A, et al. Glass fibers reinforced acrylic thermoplastic resin-based tri-block copolymer composites: low velocity impact response at various temperatures [J]. Composite Structures, 2017, 160: 939–951. doi: 10.1016/j.compstruct.2016.10.127 [22] 熊杰, 唐菊, 顾伯洪, 等. 层压复合材料靶板的动态点冲击响应 [J]. 纺织学报, 2003(1): 32–35. doi: 10.3321/j.issn:0253-9721.2003.01.010XIONG J, TANG J, GU B H, et al. The response to point impact on target board from laminated composite [J]. Journal of Textile Research, 2003(1): 32–35. doi: 10.3321/j.issn:0253-9721.2003.01.010 [23] 张晓颖, 李胜杰, 李志强. 爆炸载荷作用下夹层玻璃动态响应的数值模拟 [J]. 兵工学报, 2018, 39(7): 1379–1388. doi: 10.3969/j.issn.1000-1093.2018.07.016ZHANG X Y, LI S J, LI Z Q. Numerical simulation of dynamic response of laminated glass subjected to blast load [J]. Acta Armamentarii, 2018, 39(7): 1379–1388. doi: 10.3969/j.issn.1000-1093.2018.07.016 [24] 王礼立, 朱兆祥. 应力波基础 [M]. 2版. 北京: 国防工业出版社, 2005: 41–42.WANG L L, ZHU Z X. Foundation of stress wave [M]. 2nd ed. Beijing: National Defense Industry Press, 2005: 41–42. -









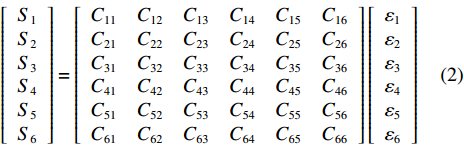
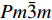
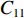
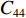
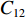

 下载:
下载:
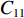
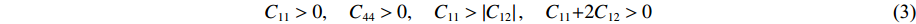
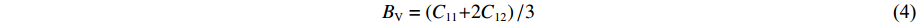
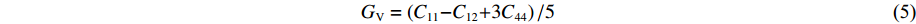
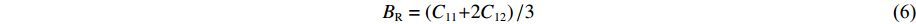
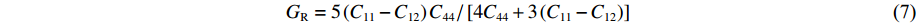
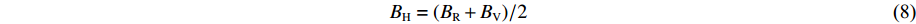
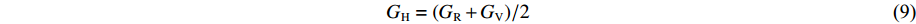
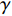
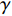
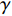
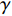
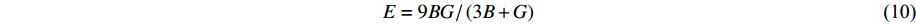
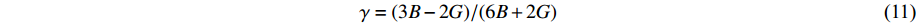
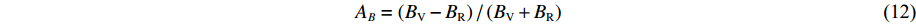

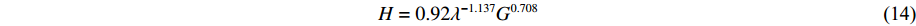
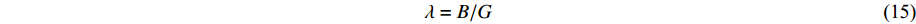
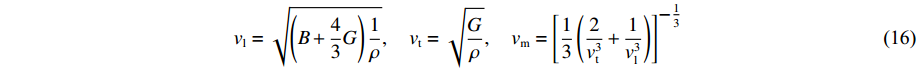

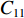

 下载:
下载: