High Pressure Raman Spectroscopy and X-ray Diffraction of CuS2
-
摘要: 利用金刚石压腔在高温高压条件下合成了黄铁矿结构CuS2,结合显微拉曼光谱和同步辐射X射线衍射实验,发现黄铁矿结构CuS2在低于30 GPa的压力范围内保持稳定,无结构相变发生。高压拉曼光谱研究结果显示,CuS2的所有拉曼模频率随压力升高呈连续单调线性增加。对X射线衍射实验获得的CuS2体积随压力变化关系进行Birch-Murnaghan状态方程拟合,得到零压晶胞体积V0 = 193.8(5)Å3,体积模量K0 = 99(2)GPa,K0'=4(固定)。运用第一性原理理论计算,得到CuS2的拉曼模频率和晶胞体积随压力变化关系均与实验观测结果保持一致。与其他黄铁矿结构过渡金属二硫化物MS2(M = Mn,Fe,Co,Ni)对比,发现MS6配位八面体大小(M—S键长)主导晶胞的体积大小和晶体的压缩性,并推测CuS2中Cu离子可能以+2价形式存在。研究结果弥补了黄铁矿结构CuS2高压拉曼光谱和X射线衍射研究的缺失,证实其在高温高压下的结构稳定性,对全面认识CuS2的物理化学性质及黄铁矿结构物质的统一规律具有重要价值,对于探索Cu在地球深部的价态和赋存形式也具有指示意义。Abstract: Pyrite structure CuS2 was synthesized in diamond anvil cell at high pressures and high temperatures. Using Raman spectroscopy and synchrotron X-ray diffraction, the pyrite-type CuS2 was found to be stable in 0-30 GPa without any phase transition. Raman spectroscopy show that all observed Raman frequencies increasemonotonously with increasing pressures. Fitting experimental pressure and volume data of X-ray diffraction with Birch-Murnaghan equation of state, gives V0 = 193.8(5) Å3, K0 = 99(2) GPa and K0' = 4 (fix). The dependencies of Raman frequencies and unit-cell volumes with pressures are coincident with the results of first-principles calculation. The results of calculation properly depict that of experiments. Compared with other pyrite structure transition-metal disulfides MS2(M = Mn, Fe, Co, Ni), the length of M—S dominates the unit-cell volume and compressibility of MS2, and the Cu cation tends to be +2 valance in the CuS2. This study makes up for the lack of high-pressure Raman and XRD research of CuS2, and confirms structural stability of pyrite-type CuS2 at high pressures and high temperatures. The results are important for comprehending the physical and chemical properties of CuS2 and realizing the unified law of pyrite structure materials. It’s also meaningful in discussion of the valance and distribution of copper in deep Earth.
-
Key words:
- pyrite structure /
- CuS2 /
- Raman spectroscopy /
- X-ray diffraction /
- high pressure
-
The field of antiferromagnetic (AFM) spintronics has recently garnered attention due to its ultrafast dynamics, zero net magnetization, and stability against external magnetic fields, which hold promise for future computing power[1–4]. In these materials, information can be encoded by manipulating the antiferromagnetic order via domain wall motion[5], optical excitation[6], spin-orbit torques[3], and staggered local relativistic fields[7]. Among the different categories of antiferromagnet, metallic antiferromagnet are particularly unique as they provide an ideal platform for fundamental studies and applications due to the strong interplay between electrons, spin, phonons, and photons[8–9]. However, metallic antiferromagnets are rare, and the known materials display AFM order only well below room temperature (RT), which limits their practical applications. For instance, the transition-metal oxides Nb12O29[10], Ca3Ru2O7[11], CaCrO3[12], and LaNiO3[13] exhibit Néel transition temperature TN of 12, 56, 90, and 157 K, respectively. Although RuO2 exhibits AFM order at around RT (TN ~ 300 K), the magnetic moment is as small as 0.05µB (µB represents Bohr magneton) at about 300 K. Therefore, exploring materials with RT magnetic order is of utmost importance for practical magnetic applications[1].
Recently, research on the magnetic properties of double-perovskite oxides A2BB'O6, where B and B' are ordered 3d and 4d/5d transition metal ions, has demonstrated the emergence of homogeneous and long-range magnetic order due to periodic intrinsic magnetic ions and magnetic exchange interactions[2–9]. These materials exhibit multiple functions resulting from multiple physical interactions, such as spin, orbit, dipole, and lattice. For example, such interactions give rise to magnetoelectric coupling based multiferroics[14–15], half-metallicity[16], colossal magnetoresistance[16], and ferromagnetic (FM) semiconductor and insulator[17] properties in RT perovskite-oxides magnets.
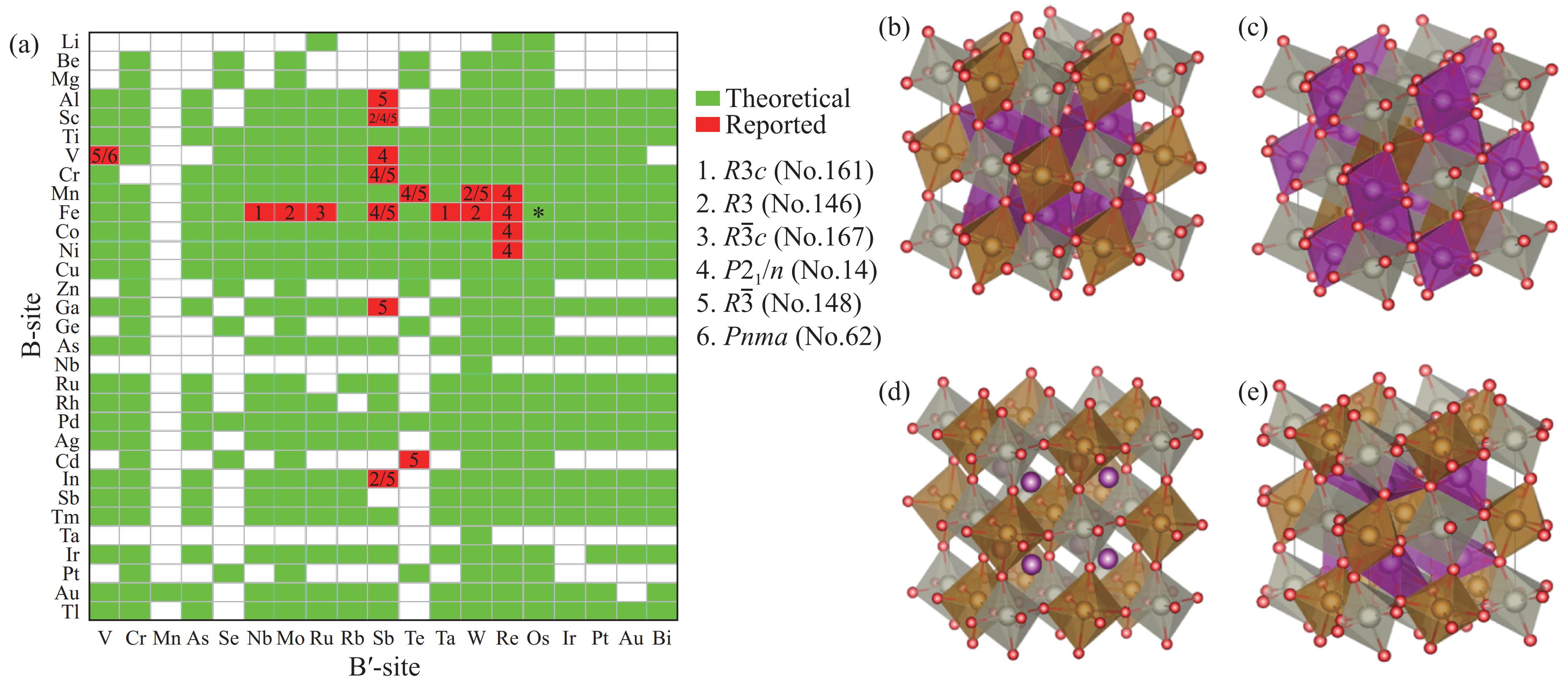
Theoretical analysis of A2BB'O6 materials with divalent A-site alkali earth cations reveals that the maximum number of unpaired 3d and 4d/5d electrons can reach 3d5 (
t32ge2g in high spin) or 4d3/5d3 (t32ge0g ) combination over the B- and B'-sites, such as in A2Fe3+B'5+O6 (B' = Ru, Os) and A2Mn2+B'6+O6 (B' = Rh, Ir) under charge balance. For example, the Ca2FeOsO6 material exhibits electrically insulated behavior and undergoes a FM transition at about 320 K driven by lattice distortion and chemical pressure[6, 18–19]. In contrast, spin frustration arising from the disordered alignment of Fe3+/Ru5+ in A2FeRuO6 (Pbnm symmetry)[20–21] may result in spin-glass or AFM behavior[22]. Notably, the Fe/Ru ordered double perovskite Sr2FeRuO6 is stabilized in film form by hetero-interface strain (chemical pressure) on SrTiO3 substrate, achieving a Curie temperature (TC) of 390 K[23]. To date, there are no reports on A2Mn2+Rh6+O6 or A2Mn2+Ir6+O6.The emerging research on exotic perovskites under high-pressure and high-temperature (HPHT) conditions has led to the investigation of transition metal cations at the A-site, resulting in enhanced magnetic interactions when compared to conventional analogs[14–15, 17, 24–34]. The occupancy of the A-site by high-spin d5-Mn2+ cations can significantly increase the number of unpaired d electrons, thereby promoting strong magnetic interactions in Mn2BB'O6[35]. Theoretically, there are 411 possible combinations of Mn2BB'O6 in Mn2B1+B'7+O6, Mn2B2+B'6+O6, and Mn2B3+B'5+O6, as shown in Fig. 1. Mn2B4+B'4+O6 is unlikely to form ordered double perovskites and is ignored here. Up to now, only 20 compounds have been experimentally validated to crystallize in Mg3TeO6-type (R
¯3 )[36–37], Ni3TeO6-type (R3)[38–39], β-Li3VF6-type (C2/c)[40–42], and GdFeO3-type (P21/n)-type[43–44] structures, depending on the chemical and synthesis parameters (see Fig. 1). Figure 1. (a) Possible combinations of Mn2BB′O6 in the chemical space of B and B′ cations under charge balance (The possible, reported, and inexistent compounds are displayed in green, red, and blank, respectively. The numbers marked for the 20 known compounds denote the space group types listed on the right side.); (b)–(e) the crystal structures of some of the known compounds, including Mg3TeO6-type (R
Figure 1. (a) Possible combinations of Mn2BB′O6 in the chemical space of B and B′ cations under charge balance (The possible, reported, and inexistent compounds are displayed in green, red, and blank, respectively. The numbers marked for the 20 known compounds denote the space group types listed on the right side.); (b)–(e) the crystal structures of some of the known compounds, including Mg3TeO6-type (R¯3 )[36–37], Ni3TeO6-type (R3)[38–39], β-Li3VF6-type (C2/c)[40–42], and GdFeO3-type (P21/n)[43–44](To ensure that our calculations are compatible with different magnetic configurations and atomic distortions, we have useda 40-atom super-cell, which can be considered as a (2, 2, 2) multiple of the ideal single perovskite structure alongthe three axes of Cartesian coordinates[50–51]. (b)–(e) also show the examples of collinear magnetic structure thatwe considered structure optimization with (b) FM, (c) Type Ⅰ AFM, (d) Type Ⅱ AFM and (e) Type Ⅲ AFM.)Taking the number of unpaired d electrons (nd), chemical valences and ionic radius into account, it is noteworthy that eight transition-metal-only (TMO) double perovskites with nd between 14 and 18 exhibit robust magnetoelectric properties, which provides motivation for further exploration in this category. More than 70 TMO double perovskites can be formed in Mn2BB′O6 by ordered combination of 3d and 4d/5d ions over B- and B′-sites under charge balance, offering a vast space to explore new multifunctional materials. However, the normally arranged atoms will have a certain displacement and deformation due to the influence of strong magnetism[45], making TMO double perovskites’ symmetry further reduced than that of conventional nonmagnetic perovskites. As a result, tolerance factor (t)[46–47] and octahedral factor (µ)[48–49] as geometric descriptors for conventional perovskites with large A-cations are not longer suitable for the TMO double perovskites. Therefore, discovering these materials mainly relies on empirical intuition and costly trial-and-error in HPHT synthesis.
Recently, the successful prediction and precise synthesis of A3TeO6 have provided insight into the screening of new materials oriented towards structure and function, which can help avoid the costly trial-and-error process involved in preparing magnetically exotic perovskites under HPHT[52–53]. These studies have used first-principles density functional theory (DFT) calculations based on the Murnaghan equation of state for solids[54] to show that the phase stability between competing polymorphs can be estimated using the phase diagram of the relative enthalpy (∆H), the total energy (E) and the pressure (p). The reported DFT-based workflow, which adopts a restricted collinear magnetic structure, has been shown to provide considerable accuracy in predicting the polymorph and corresponding synthesis parameters (such as pressure). However, for TMO double perovskites with 4d/5d ions at the B′-site, the variation in the energy scale of possible interactions, such as magnetic interactions, spin-orbit coupling (SOC), Dzyaloshinskii-Moriya (DM) interaction, Hubbard U and Hund’s interactions, cause a challenge for calculating these materials reliably and efficiently using ab initio many-body methods[45]. This remains an open challenge in providing reliable and timely predictions for this strongly correlated perovskites family[55–56]. Conversely, validation calculations based on experimentally reported TMO double perovskite-related compounds are expected to facilitate the understanding of dominant factors in inverse prediction and rational design.
In this article, we will firstly conduct test calculations on the known transition metal oxide Mn2BB'O6, establish calculation models, and then these models will be used to predict the pressure-dependent polymorph evolution and synthesis parameters of the TMO double perovskite Mn2FeOsO6, which contains a maximum nd of 18. Additionally, we will propose several magnetoelectric properties of Mn2FeOsO6. Due to the high cationic disordering in corundum-type, Mn2FeRuO6 is beyond the scope of this work, shown experimentally[25].
1. Symmetry Analysis
For double perovskite materials with the chemical formula A2BB'O6, the ideal crystal structure is usually doubled in all three crystallographic directions compared to a single perovskite ABO3, resulting in a space group of Fm
¯3 m[32]. However, this only occurs when the size of the A-site cation is much larger than that of the B-site cation, i. e. with tolerance factor t around 1[57]. When the size of the A-site cation is comparable to that of the B-site cation, i. e. with tolerance factor t less than or equal to 0.85, the BO6 octahedra will tilt to optimize the A-cation bonding[58]. According to Glazer’s tilt rule, there can be 12 tilt systems in A2BB'O6 perovskites, as determined by Howard et al[59–60]. As mentioned in our previous article[53], exotic double perovskites with transition metals and t≤0.85 may adopt one of six possible structures identified by database screening, including distorted GdFeO3-type double perovskite (P21/n), B-site ordered LiSbO3 derivatives (Pnn2), Mg3TeO6 (R¯3 )[36–37], corundum derivatives (LiNbO3-type R3c, ilmenite R¯3 , and ordered ilmenite or Ni3TeO6-type R3)[38–39], bixbyite (Ia¯3 ), and β-Li3VF6 (C2/c)[40–42]. Based on chemical and geometrical considerations, the size and the charge differences between the A-site and B-site cations studied in this paper do not energetically favor the formation of bixbyite (Ia¯3 ) and LiSbO3 derivatives (Pnn2). Therefore, we only consider the remaining four structures (R3, R¯3 , C2/c and P21/n).Stretching the ideal face-centered cubic structure Fm
¯3 m along the <111> direction results in the R¯3 structure, where the BO6 octahedra tilts and the space group changes to a–a–a– in Glazer tilt systems. However, in practice, the orders of B and B' coordination can destroy the space-reversal symmetry, leading to the R3 polymorphs. The reported R3c structure is unlikely to happen for rock-salt double perovskite structures.Meanwhile, the C2/c and P21/n structures can be obtained by sliding the ideal Fm
¯3 m structure along the <110> direction and considering the tilt of the BO6 octahedra. In the case of small A-cations, the A cation is unlikely to maintain the ideal position, leading to the distortion of the lattice and the formation of the P21/n structure with a+b–b– in Glazer tilt systems. Both C2/c and P21/n configurations are commonly observed in double perovskite structures.The single perovskite structure is also the most abundant in Pnma (a+b–b–) and R
¯3 c (a0b–b–) configurations[48, 61] since the tilt direction of BO6 octahedra in the adjacent two layers of perovskite structure tends to be opposite. In the Glazer symbol, therefore, compared with in-phase BO6 rotations, such as Im3 (a+a+a+) and Pmmn (a–b+b+), the anti-phase BO6 rotations are more common. When it comes to double perovskites A2BB'O6, two typical progress of octahedral tilts with decreasing t has been found as[62–63]Fmˉ3m(a0a0a0)→Rˉ3(a−a−a−)→R3(a−a−a−)Fmˉ3m(a0a0a0)→C2/c(a0b−b−)→P21/n(a+b−b−) (1) We note that in material calculations, A-site cations are always repelled or attracted by B-site cations due to the influence of magnetism and Jahn-Teller effect, making it difficult to maintain a square arrangement in a plane. Therefore, the common tetragonal lattice (I4/m (a0a0c–) or P4/mnc (a0a0c+)) in perovskite is hard to appear under the condition that both A- and B-sites of double-perovskite are transition metal ions. In fact, experimental research on this type of materials has also confirmed our conclusion, i. e. there is no report on tetragonal lattice double-perovskite oxides with A-site alkali earth cations, B and B′ ordered transition metal ions so far, as shown in Fig. 1(a).
In this work, we investigated the effect of magnetism on the lattice distortion of double perovskite structures, in addition to the BO6 octahedral tilt induced by the small A-cation. Previous studies have also reported the influence of magnetism on the lattice distortion phenomenon[22–23, 45]. It is important to note that the nearest-neighbor (NN) and next nearest-neighbor (NNN) interactions can have similar strengths. In the ideal double perovskite structure, there are many possible magnetic configurations. However, experimental observations have shown that only four magnetic configurations are mostly observed. There are three types of AFM order, namely, type-Ⅰ, type-Ⅱ, and type-Ⅲ, depending on the relative magnitudes of NN and NNN interactions. For type-Ⅰ order, the spins are anti-parallel between the [100] planes and parallel within the [100] plane. Type Ⅱ order occurs when the NN interactions are weaker than the NNN interactions, with the spins arranged anti-parallel between the [111] planes and parallel within the [111] plane. Type-Ⅲ order is an intermediate form in which the NN interactions are relatively strong, while the NNN interactions are still significant.
In addition to these four configurations, there are other disordered magnetic phases such as magnetic frustration and spin-glass-type behavior. However, these phases are less relevant to the present study, therefore, we limit our DFT calculation and analysis of structural optimization to the four aforementioned configurations. When analyzing the magnetic properties of materials, we consider as many magnetic configurations as possible to obtain more reliable magnetic properties.
Since most experimental synthesis of transition-metal double perovskites require HPHT conditions[64–66], we considered the synthesis pressure as a crucial parameter. To investigate the effect of pressure on the stability of double perovskite structures, we calculated the energies of all four lattice configurations and four magnetic configurations for each material under varying pressure conditions.
2. Model and Methods
To simulate the application of relatively large pressure, we started with a relatively small initial volume for each polymorph with a specific set of ingredients. We then gradually increased the simulating pressure by reducing the lattice to determine the most stable structure type at each given pressure. After that, we obtained the total energy (E) and volume (V) curve of each compound, and fit it with the Brich-Murnaghan function
E(V)=−916B0[(4−B′0)V30V2−(14−3B′0)V7/7330V4/433+(16−3B′0)V5/5330V2/233]+E0 (2) where B0,
B′0 , V0 and E0 are fitting parameters. We finally obtained the relative energy-pressure (E-p) relation to determine the phase transition of each compoundp(V)=1.5B0[(V0V)7/733−(V0V)5/533] (3) Our DFT calculations were performed using the Vienna ab initio simulation package (VASP)[67–70] with the projector augmented wave (PAW) method. We used the spin-polarized generalized gradient approximation (GGA) and the Perdew Burke-Ernzerhof (PBE) function to treat the electron exchange correlation potential[71]. The plane wave kinetic energy cutoff was set to 400 eV.
To ensure our calculations are compatible with different magnetic configurations and atomic distortions, we used a 40-atom supercell, which can be considered as a (2, 2, 2) multiple of the ideal single perovskite structure along the three axes of Cartesian coordinate[50–51]. The Γ point only in VASP was used to accelerate the crystal structure optimization until the force on each atom is less than 0.001 eV/Å. We built initial structure models for calculations by elemental substitutions of the crystallographic information files of known compounds with elemental substitutions.
To investigate the effect of pressure on the stability of double perovskite structures, we applied isotropic pressure during the calculations. In DFT calculations, we have considered various factors, such as collinear magnetic structure, SOC, DFT+U (UFe = 4.6 eV, UMn = 4 eV, UOs = 2.0 eV), and non-collinear magnetic structure to determine which factors would have undeniable impact on the structure.
For Mn2FeOsO6, its magnetic properties can be described by the Heisenberg Hamiltonian
H=−∑i,jJijSi⋅Sj−D(Si×Sj)−∑iΔs(Szi)2 (4) where the symbol Si (Siz) represents the spin operator at the lattice site i (along the z direction), Jij refers to the Heisenberg exchange between Si and Sj, D refers to the DM interaction, and
Δ s represents the single-ion Ising anisotropy.There are 8 magnetic atoms in a supercell, and it is needed to consider 64 types of magnetic exchange interactions between these magnetic ions. If we ignore magnetic exchange interaction J between identical magnetic ions (i. e. Jii = 0) and the directional dependence between different magnetic ions (i. e. Jij = Jji), there are 28 distinct values left. Therefore, we need to calculate these 28 different magnetic exchange interaction values individually.
We calculated 64 different magnetic configurations among the total 28 = 256 configurations in the magnetic unit cell containing 8 magnetic ions. Because the magnetic anisotropy is basically negligible, we used collinear magnetic DFT calculations to save the resources requirement. These 64 magnetic configurations are enough to obtain 28 unknown J. In order to simplify the calculation, we used the linear matrix method to calculate J one by one using the minimum dichotomy method
˜J=˜E/(˜S⊗˜S) (5) where
˜J is a 1×28 matrix contained 28 different magnetic exchange interaction values,˜E is a 64×1 matrix contained 64 different energies obtained by DFT calculations, and˜S⊗˜S represents the direct product between magnetic moments of the magnetic ions.To study the spin dynamic behaviours, we applied a linear spin wave theory by using SpinW package to compute the excitation spectrum[72]
I(k,ω)∝∑α,β(δα,β−˜kα˜kβ)Sα,β(k,ω) (6) where
k is normalized wave vector, ω is frequency of the excitation spectrum,Sα,β (α, β = x, y, z) is the dynamic structure factor,˜kα, ˜kβ are components of the normalized wave vectork ,δα,β is the Dirac delta function which takes the value of 1 only when α=β, otherwise it takes 0.3. Validation Calculations on Known Two Double Perovskites
Table 1 provides the details of the synthesis conditions, structural symmetry, electron configurations, and physical properties of TMO double perovskites A2BB'O6 where B- and B′-site cations is transition-metal atoms. The Re-based compounds crystallize in a distorted GdFeO3-type monoclinic structure (P21/n), while Mo-based and W-based compounds are more likely to adopt rhombohedral symmetry (R3). Previous studies have shown that DFT caculations in consideration of only collinear magnetic structures can yield reasonable and acceptable overall trends of synthesis condition and E-p phase diagrams for TMO double perovskites[52–53].
Table 1. Structural and electronic properties of the 7 experimentally realized TMO double perovskite-related compounds, along with the predicted Mn2FeOsO6. Herend ≥14 provide certain displacement and deformation due to the influence of strong magnetism, resulting in low symmetry (R3 and P21/n)Compound Space group nd Synthesis conditions Physical properties Magnetic structure Ref. Mn2FeMoO6 R3 16 1350 ℃, 8 GPa Multiferroic,
TC = 337 KType-Ⅱ AFM [17] Mn2FeWO6 R3 14 1400 ℃, 8 GPa Second-Harmonic generation,
TN = 70 KType-Ⅰ AFM [24] Mn2MnWO6 R3 15 1400 ℃, 8 GPa TN = 58 K Type-Ⅰ AFM [15] Mn2CoReO6 P21/n 14 1300 ℃, 8 GPa Semiconductor, TN = 94 K Type-Ⅱ AFM [26] Mn2FeReO6 P21/n 17 1350 ℃, 5/11 GPa Half-metal, TC = 520 K Type-Ⅲ AFM [16, 29–30] Mn2MnReO6 P21/n 17 1400 ℃, 5/8 GPa Semiconductor, TN = 50 K Type-Ⅱ AFM [29, 31] Mn2NiReO6 P21/n 17 1573 ℃, 8 GPa Semiconductor, TN = 80 K Type-Ⅱ AFM [73] Mn2FeOsO6 P21/n 18 5 GPa Metal, TN = 680 K Type-Ⅲ AFM This work We investigated the effect of other possible properties, such as DFT+U (UFe = 4.6 eV, UMn = 4 eV, UOs = 2.0 eV), and the role of non-collinear magnetic structure, on the energy-volume (E-V) results, but found that these interactions do not impact the results significantly. Therefore, we concluded that these additional interactions could be ignored in structural optimization, and we only adopted collinear magnetic structures in the structural optimization calculations to save computational resources.
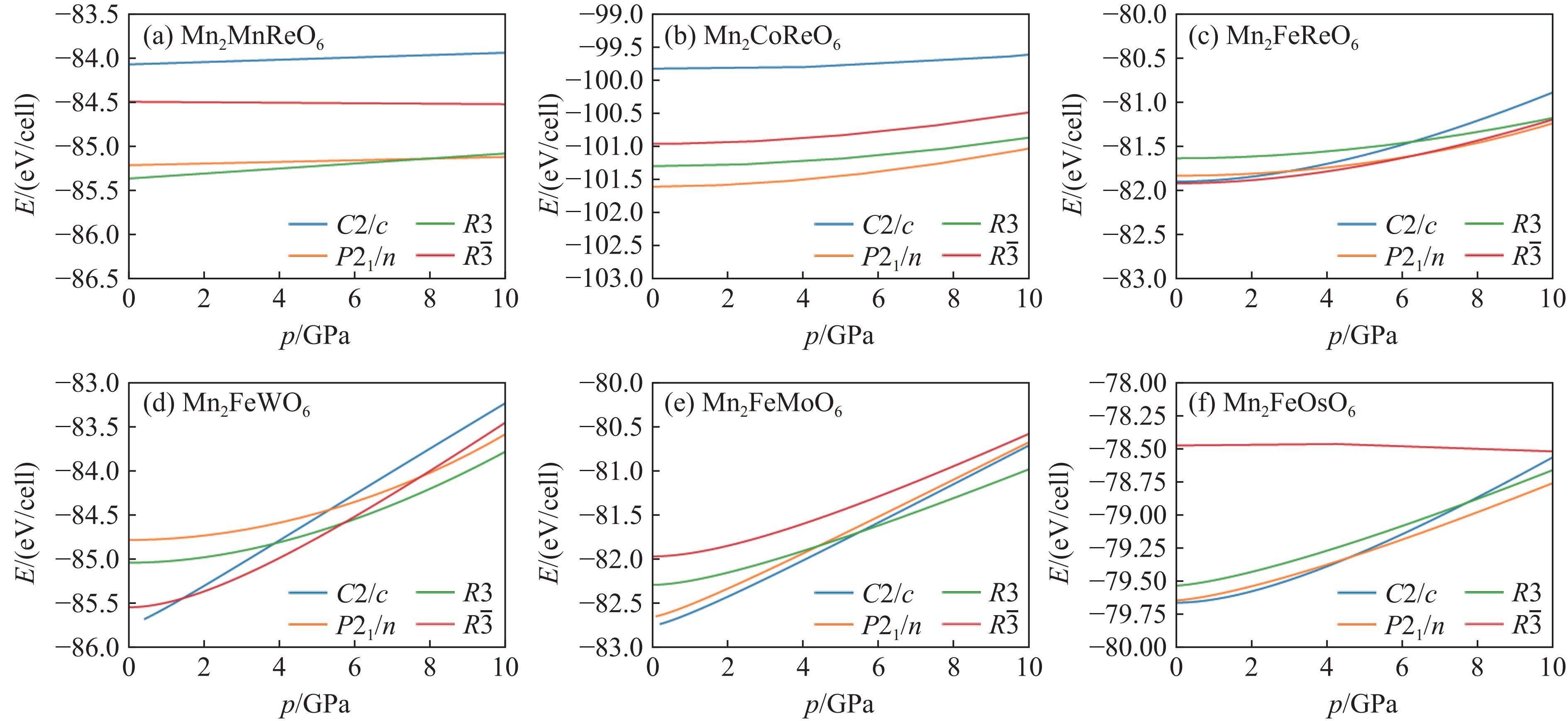
To be clear, we started with Mn2MnReO6 with the known P21/n structure synthesized in previous studies[16, 29–30]. To evaluate the effect of magnetism on the crystal structure, we calculated the E-V curve using the Brich-Murnaghan function. It turns out that for collinear magnetic structures, the magnetism contribution to the energy difference is on the order of 0.1 eV/atom, accordingly, having an effect on the lattice structure. However, the E-p curve calculated using Eq. (3) shows that, the R3 structure is the most stable one when p≤10 GPa, with a minimum energy difference of less than 0.1 eV per cell without considering the magnetic structure. This small energy difference results in inconsistency between the calculated R3 output and the experimental P21/n result. Therefore, we must consider the magnetic structure in TMO double perovskites. We conjectured that the magnetic exchange between magnetic ions in Mn2MnReO6 would affect the crystal structure, and the magnetic contribution of atoms should be considered in the DFT calculations of structure optimization. After considering magnetic structure, the type-Ⅱ AFM configuration has the lowest energy of the four collinear magnetic structures considered, which is a good validation of previous experiment results[29–31].
In the present study, we investigated the polymorphic phase transitions of five double perovskite compounds, namely Mn2MnReO6, Mn2CoReO6, Mn2FeReO6, Mn2FeMoO6, and Mn2FeWO6. In our calculations, we have considered the four different magnetic configurations and obtained their respective E-V relations (see Fig. 2(a)–Fig.2(e)). However, due to the existence of metastable magnetic structures, the total magnetic moment after DFT convergence is found to be inconsistent with the calculations of a single magnetic configuration. The E-V curves also exhibit abnormal points and protrusions, rendering the fitting of these curves with the Brich-Murnaghan function inaccurate. To address this issue, we selected the magnetic structure with the lowest energy under each V point of the four magnetic configurations as the source of E-V curve. By uniformly fitting these E-V curves with the lowest energy, we obtained appropriate parameters to produce a sufficiently reliable E-p curve.
Upon adding magnetism, we observed that although the influence of magnetism on each structure is different, the total energy of the system is reduced by about 1 eV per cell. For Mn2MnReO6, such a small influence of magnetic exchange energy is able to reverse the two energy levels of R3 and P21/n structures under the external pressure around 7 GPa, beyond which the P21/n structure is more stable and in good agreement with the experimental results[29, 31]. Notably, 5 GPa is needed to prepare P21/n Mn2MnReO6 in the experiment[29], and the gap of 2 GPa between theory and experiment may be caused by the 1400 ℃ treatment and can be considered as within the theoretical error range.
We then applied this method to the related double perovskite compounds that had been experimentally prepared. In our calculations, the lowest synthesis energy configuration of Mn2CoReO6 is always the P21/n structure, which roughly echoes the experimental results[26]. For Mn2FeReO6, the synthesis energies of the four structures exhibit little differences in the pressure range considered. The energy of R
¯3 overlaps that of P21/n at 5 GPa, being consistent with the synthetic conditions in the experiment[16]. The situations of Mn2FeMoO6 and Mn2FeWO6 are similar. Both compounds show the R3 structure under high pressure, and the synthesis conditions are about 6 GPa. We also checked the calculations without considering magnetic conditions and found that they do not match experiments. Validation calculations on these five synthesized double perovskite materials prove that our proposed method for computing polymorph-dependent phase diagrams is reliable.4. Magnetism of Mn2FeOsO6
In this section, we present predictions on the structure and the properties of Mn2FeOsO6, a compound that has not yet been experimentally synthesized. Our DFT calculations demonstrate that the C2/c structure is relatively stable under atmospheric pressure, as illustrated in Fig. 2(f). However, under a pressure greater than 5 GPa, the P21/n structure with type-Ⅲ AFM configuration becomes the most stable.
In addition, our calculations on the possible magnetic structures of Mn2FeOsO6 revealed a standard AFM configuration, where the magnetism of each magnetic ion is reversed. Specifically, in a 40-atom supercell, four spins of the eight Mn atoms are opposite to the other four, and two spins of the four Fe atoms are also opposite to the other two. We have also confirmed that the magnetic anisotropy is relatively small, as the energy difference per cell caused by magnetism in the lattice vectors a, b, and c (Fig. 3(a)) directions is less than 0.1 meV.
 Figure 3. DFT results of Mn2FeOsO6 in consideration of SOC, DFT+U (UFe = 4.6 eV, UMn = 4 eV, UOs = 2.0 eV) and non-collinear magnetism: (a) the predicted atomic and magnetic structures, with a distorted GdFeO3-type monoclinic structure (P21/n) as for Mn2FeReO6[16, 29–30] (The arrow on each atom represents the spin orientation and the size of spin value. Every spin is almostlaying at <001> direction, forming a relatively ideal AFM configuration.); (b) energy band structure near Fermi level andspin-resolved density of states (DOS) (Mn2FeOsO6 has no bandgap, forming as conductor. There is a suspectedformation of Dirac points between wavevectors B-D, marked by red rectangle and arrow. The DOS of spin-upand spin-down are basically the same, verifying the AFM configuration of Mn2FeOsO6.)
Figure 3. DFT results of Mn2FeOsO6 in consideration of SOC, DFT+U (UFe = 4.6 eV, UMn = 4 eV, UOs = 2.0 eV) and non-collinear magnetism: (a) the predicted atomic and magnetic structures, with a distorted GdFeO3-type monoclinic structure (P21/n) as for Mn2FeReO6[16, 29–30] (The arrow on each atom represents the spin orientation and the size of spin value. Every spin is almostlaying at <001> direction, forming a relatively ideal AFM configuration.); (b) energy band structure near Fermi level andspin-resolved density of states (DOS) (Mn2FeOsO6 has no bandgap, forming as conductor. There is a suspectedformation of Dirac points between wavevectors B-D, marked by red rectangle and arrow. The DOS of spin-upand spin-down are basically the same, verifying the AFM configuration of Mn2FeOsO6.)We then proceeded to approximate the magnetic properties of Mn2FeOsO6. Our calculations showed that the total magnetic anisotropy energy (MAE) in a single magnetic unit cell is negligible (
Δ s<0.1 meV) in consideration of SOC, and the spins of magnetic atoms exhibit almost collinear configuration with only a degree difference of less than 5°, as depicted in Fig. 3(a). Therefore, in the estimation of magnetic properties, we neglected the MAE, as well as DM interaction, and only considered Heisenberg exchange in Eq. (4).As illustrated in Fig. 3, Mn2FeOsO6 contains 8 magnetic ions in a single magnetic unit cell. Among them, the four Mn ions possess a local magnetic moment of approximately 4.5µB, and the two Fe ions possess a local magnetic moment of approximately 4µB, while the two Os ions possess a local magnetic moment of approximately 1µB. Although basically Os ions is not magnetic, they exhibit magnetism induced by the surrounding strong magnetic Mn and Fe ions.
In our magnetic exchange model for Mn2FeOsO6, we calculated 28 different J due to the irregular configuration of the magnetic ions in the P21/n structure. Here, we present a few examples to illustrate the numerical values of these J . The AFM exchanges (J > 0) between the NN Mn ions (J13≈J24) and NNN ones are approximately equal to the values reported in [74]. However, due to the low symmetry of P21/n, there are two different distances between NN Mn ions, resulting in slightly different FM exchanges (J23<J14) induced by indirect Ruderman-Kittel-Kasuya-Yosida (RKKY) exchanges. Thus, our group of magnetic exchange models, which contains 8 different magnetic ions, is considered to be reliable.
According to the aforementioned magnetic structure model, we constructed the corresponding exchange interactions between magnetic ions, as shown in Table 2. The result of spin dynamic behaviours of Mn2FeOsO6 is shown in Fig. 4(b). Note that we have ignored the DM interaction and single-ion Ising MAE in the Hamiltonian. An obvious value of intensity is shown at AFM order wave vector QAFM = (0, 0, 1). As the NN interactions are on the order of 10 meV, the maximum of the magnon dispersion reaches 600 meV. Interestingly, the 8 bands along the Z−D momentum path all show characteristics of flat band.
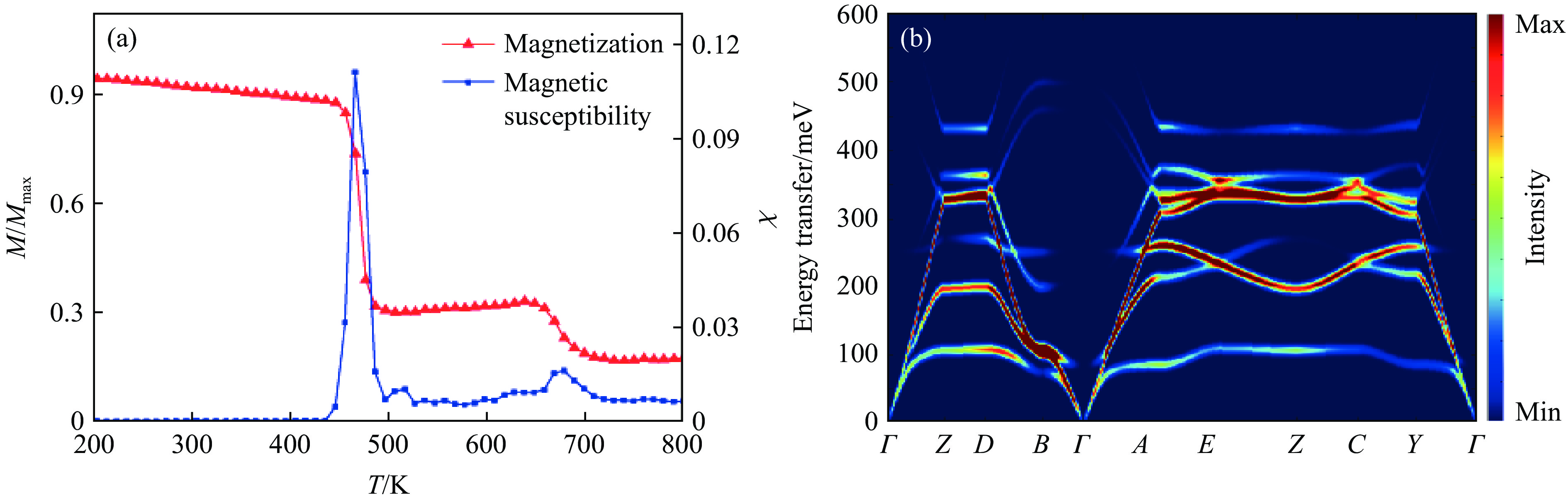
Table 2. Magnetic exchangeJij between magnetic ions within a magnetic unit cell of Mn2FeOsO6i j Jij/meV i j Jij/meV i j Jij/meV i j Jij/meV Mn1 Mn2 0.97 Mn2 Mn3 −19.20 Mn3 Fe1 1.01 Mn4 Os2 −15.77 Mn1 Mn3 40.48 Mn2 Mn4 39.88 Mn3 Fe2 13.49 Fe1 Fe2 −12.01 Mn1 Mn4 −20.07 Mn2 Fe1 15.06 Mn3 Os1 −16.74 Fe1 Os1 35.37 Mn1 Fe1 −14.33 Mn2 Fe2 18.32 Mn3 Os2 15.65 Fe1 Os2 35.37 Mn1 Fe2 −16.58 Mn2 Os1 12.26 Mn4 Fe1 −1.26 Fe2 Os1 9.14 Mn1 Os1 −12.72 Mn2 Os2 25.43 Mn4 Fe2 −13.27 Fe2 Os2 −33.49 Mn1 Os2 −27.35 Mn3 Mn4 28.61 Mn4 Os1 17.05 Os1 Os2 −6.61  Figure 4. Magnetism of Mn2FeOsO6: (a) calculated magnetization and magnetic susceptibility via classical Monte Carlo simulation (The red line represents the theoretical total magnetic moment M divided by saturation magnetic moment Mmax, while the blue line represents the relative magnetic susceptibility χ, both indicating the phase transition positions around temparature T about 480 K.); (b) simulated excitation spectrum using SpinW package (The vertical axis represents the energy of the excitation spectrum,and the colors correspond to the intensity of the excitation spectrum.)
Figure 4. Magnetism of Mn2FeOsO6: (a) calculated magnetization and magnetic susceptibility via classical Monte Carlo simulation (The red line represents the theoretical total magnetic moment M divided by saturation magnetic moment Mmax, while the blue line represents the relative magnetic susceptibility χ, both indicating the phase transition positions around temparature T about 480 K.); (b) simulated excitation spectrum using SpinW package (The vertical axis represents the energy of the excitation spectrum,and the colors correspond to the intensity of the excitation spectrum.)In Fig. 4(a), the magnetization and magnetic susceptibility of Mn2FeOsO6 were calculated using classical Monte Carlo simulations with Metropolis algorithm. It was observed that the magnetic susceptibility curve has two peaks, indicating the possible existence of two different AFM TN in this system, consistent with previous experiments[29]. We propose that this phenomenon is due to two sets of AFM atoms at A and B sub-lattices. The higher AFM TN is directly dependent on the Mn ion at A-sites. The estimated TN is above 680 K, which is higher than the TN (or TC ) observed in related experiments. It is noteworthy that the estimated transition temperature is relatively conservative. The actual transition temperature would be further increased if the MAE resulting from shape and DM interaction is considered.
Additionally, the electronic band structure of Mn2FeOsO6 was computed, shown in Fig. 3(b). Due to the low lattice symmetry, the energy bands are complex and disordered. However, in contrast to other TMO double perovskites, Mn2FeOsO6 exhibits metallic properties, with a high DOS near the Fermi surface. The DOS indicates that the energy band near the Fermi level is primarily contributed by the 3d electrons of the magnetic ions. Furthermore, the system presents an AFM configuration, where the spin up and down DOS are consistent, and the total magnetization equals zero. Thus, Mn2FeOsO6 is an ideal AFM metallic material.
Finally, the excitation spectrum mode of the above spin model was computed using the spin-wave method. The excitation mode, shown in Fig. 4(b), reveal clear AFM imprints in the <100> direction, consisting of two sets of different AFM excitation spectra, supporting the previously estimated AFM configuration obtained by our Monte Carlo method.
5. Conclusions
In conclusion, we extend a method that can determine the complex magnetism configuration and synthesis condition of double perovskite materials at the same time consuming relatively low computational resources. Our method is shown to provide satisfactory results for several known double perovskite compounds.
We apply this method to investigate the promising material Mn2FeOsO6, which has not yet been experimentally synthesized. Our analysis reveals that this material has a P21/n structural configuration with a high density of states near the Fermi level, making it an ideal AFM metal with a total magnetic moment of zero. Using a set of matrix operations, we are able to obtain the complex magnetic exchange interaction of the P21/n structure, and identify two sets of AFM TN. Our findings are further verified by classical Monte Carlo simulations and spin wave excitation spectrum analysis.
Overall, we theoretically identify an ideal AFM metal with extremely high AFM TN, which has promising applications in the field of spintronics. Our method is an effective approach to study complex magnetism in double perovskite materials and can be extended to investigate other related materials.
-
图 2 不同条件下4组实验反应前后的特征拉曼光谱(LH和RH分别代表激光加温和电阻丝加温,4组实验中加温前所测拉曼信号均为单质硫的高压相,加温后产物为黄铁矿结构CuS2)
Figure 2. Raman spectra of the four experiments at different conditions before and after reaction (LH and RH represent laser heating and resistance heating, respectively. Before heating, all the Raman peaks in four experiments belong to the high-pressure phase of elemental sulfur. After heating, the reaction products are pyrite structure CuS2.)
图 4 实验和理论计算CuS2的拉曼频率随压力变化关系(黑色圆形和菱形分别对应Exp4合成的CuS2在常温加压和卸压过程的结果,三角形表示Exp2合成CuS2卸压过程的数据,星形为理论计算值,实线和虚线分别为实验和计算数据线性拟合结果)
Figure 4. Experimental and theory calculated pressure dependence of Raman vibrational modes of CuS2(Black spheres and diamonds correspond to the results of compression process and decompression process of CuS2 synthesised at Exp4,respectively. Black triangles are data of decompression process of CuS2 synthesised at Exp2. The stars represent theory calculated points. Solid line and dotted line are linnear fitted with all experimental and calculated data, respectively.)
图 6 在加压(a)和卸压(b)过程中CuS2在不同压力下的X射线衍射图谱(下三角指示产物中的杂质峰,黄铁矿结构CuS2和传压介质KCl的衍射峰位在图中用短棒标出)
Figure 6. X-ray powder diffraction patterns at different pressures on compression (a) and decompression (b)(The inverted triangles indicate impurity peaks. The diffraction peaks of pyrite structure CuS2 and pressure transmitting medium KCl are represented with vertical bars.)
图 7 理论计算和衍射实验的CuS2及其它过渡金属二硫化物MS2(M = Mn,Fe,Co,Ni,Cu)的体积-压力变化关系(黑色圆形和黑色三角形分别对应X射线衍射实验和第一性原理理论计算结果。黑色实线和黑色虚线是对实验和计算数据进行Birch-Murnaghan 状态方程拟合结果。蓝色、绿色、紫色、棕色和红色实线分别为文献给出的黄铁矿结构MnS2、FeS2、CoS2、NiS2和CuS2状态方程结果。)
Figure 7. Pressure-volume relationship of experimental and calculated CuS2 and other transition metal disulfides MS2(M = Mn,Fe,Co,Ni,Cu) (The black circles and triangles represent the experimental and observed data of the CuS2, respectively. The solid black line and dotted black line are the Birch-Murnaghan equation of state fit respectively with listed parameters. The blue, green, purple, yellow, and red line shows the equation of state of the pyrite structure MnS2, FeS2, CoS2, NiS2, and CuS2 in references.)
表 1 黄铁矿结构CuS2的拉曼频率随压力变化及格临爱森参数(γ)
Table 1. Pressure dependences of Raman modes and the Grüneisen parameters (γ) of pyrite-type CuS2
Symmetry classification ω0/cm−1 (dω/dp)/(cm−1·GPa−1) γ Method This work Ref.[38] Eg + Tg(1) 213 207 2.45 1.14 Exp. Tg(2) 266 264 3.06 1.14 Ag + Tg(3) 512 512 1.75 0.34 Tg(1) 206 2.33 0.97 Calc. Eg 207 2.31 0.96 Tg(2) 257 3.11 1.04 Tg(3) 495 1.80 0.31 Ag 499 1.41 0.24 Note: The K0(Exp.) = 99 and K0(Calc.) = 85.6 are used respectively to calculate the Grüneisen parameters. They are obtained from the fitted Birch-Murnaghan EOS in this work. 表 2 黄铁矿结构CuS2与其他黄铁矿结构过渡金属二硫化物的零压体积模量和晶胞体积对比
Table 2. Comparison of zero-pressure bulk modulus (K0) and unit formula volume (V0) of CuS2 with that of other pyrite structure transition-metal disulfides
Compositions Pressure range/GPa V0/Å3 K0/GPa K0′ Reference MnS2 0−11.7 225.74(0) 65.9(3) 5.1(2) Ref.[14] FeS2 0−80 159.00(7) 140.2(15) 5.52(19) Ref.[41] CoS2 0−20 169.68(1) 94(2) 6.9(5) Ref.[42] NiS2 0−150 178.32 102.1 4.6 Ref.[43] CuS2 0−7 194.10(10) 98.8(6) 4(fix) Ref.[27] CuS2 0−29.6 193.8(5) 99(2) 4(fix) This study (Exp.) CuS2 0−30 196.5(2) 85.6(7) 4(fix) This study (Calc.) Note: All MS2(M = Mn,Fe,Co,Ni,Cu) are pyrite structure (Pa3) in p-range. All the V0 are uniformly transformed to same units for comparison purposes. -
[1] BROSTIGEN G, KJEKSHUS A. Redetermined crystal structure of FeS2 (Pyrite) [J]. Acta Chemica Scandinavica, 1969, 23(6): 2186–2188. doi: 10.3891/acta.chem.scand.23-2186 [2] BITHER T A, BOUCHARD R, CLOUD W, et al. Transition metal pyrite dichalcogenides. High-pressure synthesis and correlation of properties [J]. Inorganic Chemistry, 1968, 7(11): 2208–2220. [3] NOWACK E, SCHWARZENBACH D, HAHN T. Charge densities in CoS2 and NiS2 (pyrite structure) [J]. Acta Crystallographica Section B: Structural Science, 1991, 47(5): 650–659. doi: 10.1107/S0108768191004871 [4] MAKOVICKY E. Crystal structures of sulfides and other chalcogenides [J]. Reviews in Mineralogy and Geochemistry, 2006, 61(1): 7–125. doi: 10.2138/rmg.2006.61.2 [5] TEMPLETON D H, DAUBEN C H. The crystal structure of sodium superoxide [J]. Journal of the American Chemical Society, 1950, 72(5): 2251–2254. doi: 10.1021/ja01161a103 [6] KJEKSHUS A, RAKKE T. Preparation and properties of magnesium, copper, zinc and cadmium dichalcogenides [J]. Acta Chemica Scandinavica A, 1979, 33(8): 617–620. doi: 10.3891/acta.chem.scand.33a-0617 [7] KUWAYAMA Y, HIROSE K, SATA N, et al. The pyrite-type high-pressure form of silica [J]. Science, 2005, 309(5736): 923–925. doi: 10.1126/science.1114879 [8] SHIRAKO Y, WANG X, TSUJIMOTO Y, et al. Synthesis, crystal structure, and electronic properties of high-pressure PdF2-type oxides MO2(M= Ru, Rh, Os, Ir, Pt) [J]. Inorganic Chemistry, 2014, 53(21): 11616–11625. doi: 10.1021/ic501770g [9] YU R, ZHAN Q, DE JONGHE L C. Crystal structures of and displacive transitions in OsN2, IrN2, RuN2, and RhN2 [J]. Angewandte Chemie International Edition, 2007, 46(7): 1136–1140. doi: 10.1002/anie.200604151 [10] HU Q, KIM D Y, YANG W, et al. FeO2 and FeOOH under deep lower-mantle conditions and Earth’s oxygen-hydrogen cycles [J]. Nature, 2016, 534(7606): 241–244. doi: 10.1038/nature18018 [11] LIU J, HU Q Y, BI W L, et al. Altered chemistry of oxygen and iron under deep Earth conditions [J]. Nature Communications, 2019, 10(1): 153. doi: 10.1038/s41467-018-08071-3 [12] KLEPPE A K, JEPHCOAT A P. High-pressure Raman spectroscopic studies of FeS2 pyrite [J]. Mineralogical Magazine, 2004, 68(3): 433–441. doi: 10.1180/0026461046830196 [13] HARRAN I, CHEN Y Z, WANG H Y, et al. High-pressure induced phase transition of FeS2: electronic, mechanical and thermoelectric properties [J]. Journal of Alloys and Compounds, 2017, 710: 267–273. doi: 10.1016/j.jallcom.2017.03.256 [14] KIMBER S A J, SALAMAT A, EVANS S R, et al. Giant pressure-induced volume collapse in the pyrite mineral MnS2 [J]. Proceedings of the National Academy of Sciences of the United States of America, 2014, 111(14): 5106–5110. doi: 10.1073/pnas.1318543111 [15] FUJII T, TANAKA K, MARUMO F, et al. Structural behaviour of NiS2 up to 54 kbar [J]. Mineralogical Journal, 1987, 13(7): 448–454. doi: 10.2465/minerj.13.448 [16] ELGHAZALI M A, NAUMOV P G, MU Q, et al. Pressure-induced metallization, transition to the pyrite-type structure, and superconductivity in palladium disulfide PdS2 [J]. Physical Review B, 2019, 100(1): 014507. doi: 10.1103/PhysRevB.100.014507 [17] HUANG S X, WU X, QIN S. Ultrahigh-pressure phase transitions in FeS2 and FeO2: implications for super-earths' deep interior [J]. Journal of Geophysical Research, 2018, 123(1): 277–284. doi: 10.1002/2017JB014766 [18] BITHER T A, PREWITT C T, GILLSON J L, et al. New transition metal dichalcogenides formed at high pressure [J]. Solid State Communications, 1966, 4(10): 533–535. doi: 10.1016/0038-1098(66)90419-4 [19] MUNSON R A. The synthesis of copper disulfide [J]. Inorganic Chemistry, 1966, 5(7): 1296–1297. doi: 10.1021/ic50041a055 [20] BAYLISS P. Crystal chemistry and crystallography of some minerals within the pyrite group [J]. American Mineralogist, 1989, 74(9/10): 1168–1176. [21] UEDA H, NOHARA M, KITAZAWA K, et al. Copper pyrites CuS2 and CuSe2 as anion conductors [J]. Physical Review B, 2002, 65(15): 155104. doi: 10.1103/PhysRevB.65.155104 [22] KAKIHANA M, MATSUDA T D, HIGASHINAKA R, et al. Superconducting and fermi surface properties of pyrite-type compounds CuS2 and CuSe2 [J]. Journal of the Physical Society of Japan, 2019, 88(1): 014702. doi: 10.7566/JPSJ.88.014702 [23] KING H E, PREWITT C T. Structure and symmetry of CuS2 (pyrite structure) [J]. American Mineralogist, 1979, 64(11/12): 1265–1271. [24] MOSSELMANS J F W, PATTRICK R A D, VAN DER LAAN G, et al. X-ray absorption near-edge spectra of transition metal disulfides FeS2 (pyrite and marcasite), CoS2, NiS2 and CuS2, and their isomorphs FeAsS and CoAsS [J]. Physics and Chemistry of Minerals, 1995, 22(5): 311–317. doi: 10.1007/BF00202771 [25] FOLMER J C W, JELLINEK F, CALIS G H M. The electronic structure of pyrites, particularly CuS2 and Fe1- xCuxSe2: an XPS and Mössbauer study [J]. Journal of Solid State Chemistry, 1988, 72(1): 137–144. doi: 10.1016/0022-4596(88)90017-5 [26] TOSSELL J A, VAUGHAN D J, BURDETT J K. Pyrite, marcasite, and arsenopyrite type minerals: crystal chemical and structural principles [J]. Physics and Chemistry of Minerals, 1981, 7(4): 177–184. doi: 10.1007/BF00307263 [27] HÜPEN H, WILL G, HÖFFNER C, et al. X-ray diffraction of CuS2 under high pressure [J]. Materials Science Forum, 1991, 79/80/81/82: 697–702. doi: 10.4028/www.scientific.net/MSF.79-82.697 [28] NIWA K, TERABE T, SUZUKI K, et al. High-pressure stability and ambient metastability of marcasite-type rhodium pernitride [J]. Journal of Applied Physics, 2016, 119(6): 065901. doi: 10.1063/1.4941436 [29] TSE J S, KLUG D D, UEHARA K, et al. Elastic properties of potential superhard phases of RuO2 [J]. Physical Review B, 2000, 61(15): 10029–10034. doi: 10.1103/PhysRevB.61.10029 [30] MAO H K, XU J, BELL P M. Calibration of the ruby pressure gauge to 800 kbar under quasi-hydrostatic conditions [J]. Journal of Geophysical Research, 1986, 91(B5): 4673–4676. doi: 10.1029/JB091iB05p04673 [31] DATCHI F, LETOULLEC R, LOUBEYRE P. Improved calibration of the SrB4O7: Sm2+ optical pressure gauge: advantages at very high pressures and high temperatures [J]. Journal of Applied Physics, 1997, 81(8): 3333–3339. doi: 10.1063/1.365025 [32] HAMMERSLEY A P, SVENSSON S O, HANFLAND M, et al. Two-dimensional detector software: from real detector to idealised image or two-theta scan [J]. High Pressure Research, 1996, 14(4/5/6): 235–248. doi: 10.1080/08957959608201408 [33] GONZALEZ-PLATAS J, ALVARO M, NESTOLA F, et al. EosFit7-GUI: a new graphical user interface for equation of state calculations, analyses and teaching [J]. Journal of Applied Crystallography, 2016, 49(4): 1377–1382. doi: 10.1107/S1600576716008050 [34] TOBY B H, VON DREELE R B. GSAS-II: the genesis of a modern open-source all purpose crystallography software package [J]. Journal of Applied Crystallography, 2013, 46(2): 544–549. doi: 10.1107/S0021889813003531 [35] PERDEW J P, BURKE K, ERNZERHOF M. Generalized gradient approximation made simple [J]. Physical Review Letters, 1996, 77(18): 3865–3868. doi: 10.1103/PhysRevLett.77.3865 [36] ECKERT B, SCHUMACHER R, JODL H J, et al. Pressure and photo-induced phase transitions in sulphur investigated by Raman spectroscopy [J]. High Pressure Research, 2000, 17(2): 113–146. doi: 10.1080/08957950008200934 [37] PEIRIS S M, SWEENEY J S, CAMPBELL A J, et al. Pressure-induced amorphization of covellite, CuS [J]. The Journal of Chemical Physics, 1996, 104(1): 11–16. doi: 10.1063/1.470870 [38] ANASTASSAKIS E, PERRY C H. Light scattering and ir measurements in XS2 pryite-type compounds [J]. The Journal of Chemical Physics, 1976, 64(9): 3604–3609. doi: 10.1063/1.432711 [39] VOGT H, CHATTOPADHYAY T, STOLZ H J. Complete first-order Raman spectra of the pyrite structure compounds FeS2, MnS2 and SiP2 [J]. Journal of Physics and Chemistry of Solids, 1983, 44(9): 869–873. doi: 10.1016/0022-3697(83)90124-5 [40] SOURISSEAU C, CAVAGNAT R, FOUASSIER M. The vibrational properties and valence force fields of FeS2, RuS2 pyrites and FeS2 marcasite [J]. Journal of Physics and Chemistry of Solids, 1991, 52(3): 537–544. doi: 10.1016/0022-3697(91)90188-6 [41] THOMPSON E C, CHIDESTER B A, FISCHER R A, et al. Equation of state of pyrite to 80 GPa and 2 400 K [J]. American Mineralogist, 2016, 101(5): 1046–1051. doi: 10.2138/am-2016-5527 [42] BRAZHKIN V V, DZHAVADOV L N, EL'KIN F S. Study of the compressibility of FeSi, MnSi, and CoS2 transition-metal compounds at high pressures [J]. JETP Letters, 2016, 104(2): 99–104. doi: 10.1134/S0021364016140083 [43] YUY G, ROSS N L. Prediction of high-pressure polymorphism in NiS2 at megabar pressures [J]. Journal of Physics: Condensed Matter, 2010, 22(23): 235401. doi: 10.1088/0953-8984/22/23/235401 -







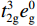
 DownLoad:
DownLoad:

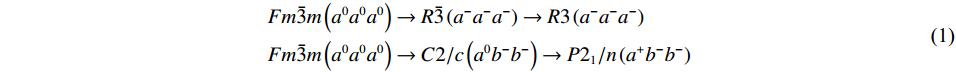
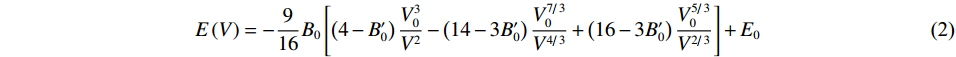
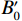
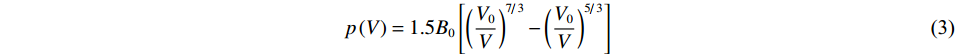
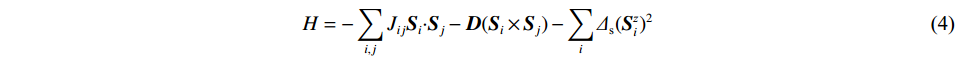

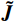
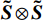
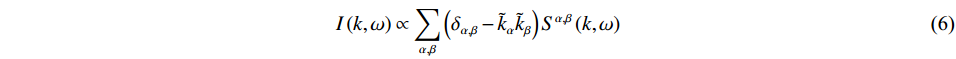
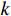
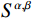
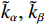

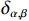

 DownLoad:
DownLoad:


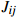

 下载:
下载: