Crystal Plasticity Finite Element Theoretical Models and Applications for High Temperature, High Pressure and High Strain-Rate Dynamic Process
-
摘要: 对于高温、高压、高应变速率加载条件下的材料冲击变形行为,动态晶体塑性模型能够直接反映晶体中塑性滑移的各向异性及其对温度、压力和微观组织结构的依赖性,因而广泛应用于材料的动态冲击力学响应、微观结构演化以及动态损伤破坏的模拟。本文综述了高压冲击下动态晶体塑性有限元的理论模型,主要包括变形运动学、包含状态方程的超弹性本构模型和晶体塑性本构模型,涉及位错滑移、相变、孪生等塑性变形机制,以及层裂、绝热剪切带等动态破坏方式。Abstract: For shock deformation behavior of materials under high temperature, high pressure and high strain-rate loading conditions, dynamic crystal plasticity models can directly reflect the anisotropy of plastic slip and its dependence of temperature, pressure and microstructure in crystals. In consequence, dynamic crystal plasticity models are widely used in simulations of material impact dynamic response, microstructure evolution and dynamic damage. Theoretical models of dynamic crystal plasticity under high pressure shock loading conditions were reviewed in this paper, mainly including: deformation kinetics, hyperelastic constitutive models incorporating equations of state, and crystal plasticity constitutive models. This paper also covers plastic deformation mechanisms, including dislocation slip, phase transition and twinning; as well as dynamic damage, including spalling and adiabatic shear band.
-
海床已成为一个全新的冲突领域,近海底爆炸可以对海底光缆、海底管道等设施造成严重的破坏,其破坏过程涉及近海底反射、多相流掺混、结构与流体的耦合作用,开展近海底爆炸冲击波时空演化规律研究对于水中兵器研制及海底设施抗爆抗冲击设计具有重要的意义。
目前,国内外对近海底水下爆炸研究已经取得了一定成果。基于Kelvin的冲击理论,Blake等[1]得出了判断自由面附近气泡射流方向和气泡运动方向的方法,该方法对近壁面也同样适用。张永坤[2]对沉底水雷在水下爆炸作用下的毁伤情况进行了研究,总结了水雷在不同情况下被毁伤的特点,为提高水下灭雷武器的作战能力奠定了理论基础。邵建军等[3]基于相似理论,研究了海底对爆炸的影响,发现海底底质变化显著影响水下爆炸的冲击波能和气泡能。杨莉等[4–7]在泥底、砂底及石底3种条件下进行了沉底爆炸试验,发现在3种底质条件下,气泡后期溃灭形态存在差异,最大峰值压力通常出现在装药靠近水底面一侧的斜上方,对于爆距较小的水底面测点,反射波追上入射波会形成马赫波。黄潇等[8]采用镜像法模拟海底边界的影响,发现自由场爆炸气泡比近海底爆炸气泡对潜艇施加的总纵弯矩更大。姚熊亮等[9]基于光滑粒子流体动力学(smooth particle hydrodynamics,SPH)方法模拟沉底水下爆炸,研究了水底底质厚度和炸药当量对冲击波压力的影响。邵宗战等[10]提出了沉底水雷海上爆炸威力的测量方法,给出了冲击波峰值压力的拟合方法及爆炸能量计算方法。前人在近海底水下爆炸冲击波载荷分析方面已取得了一些成果,然而,针对不同底质条件下近海底水下爆炸冲击波载荷时空分布规律的定量研究尚不充分。
本研究拟采用耦合欧拉-拉格朗日(coupled Eulerian-Lagrangian,CEL)方法建立近海底水下爆炸模型,探讨不同底质条件下近海底爆炸冲击波的时空分布规律,以期为近海底设施的抗爆抗冲击结构设计提供支撑。
1. 数值模型与数值验证
1.1 状态方程
本研究采用TNT炸药,爆轰产物通过JWL方程[11]描述
p=A(1−ωR1v)e−R1v+B(1−ωR2v)e−R2v+ωev (1) 式中:A、B、R1、R2及
ω 为与炸药状态有关的常数,v为爆轰产物的相对比容,e为炸药单位质量所蕴含的内能。TNT炸药的材料参数及JWL状态方程参数如表1[12]所示,其中:ρ为密度,D为爆速。水采用Mie-Grüneisen状态方程描述,表达式[11]为
p=pH(1−Γ0η/2)+Γ0ρ0Em (2) 式中:pH为Hugoniot压力;ρ0为初始密度;Em为单位质量内能;Γ0为Grüneisen常数;η为名义体积压缩应变,
η=1−ρ0/ρ 。pH的表达式[11]为pH=ρ0C20η(1−Sη)2 (3) us=C0+Sup (4) 式中:us为冲击速度,up为粒子速度,S和C0为us-up曲线参数。水的状态方程参数列于表2[12]。
在数值模型中,设空气为理想气体,其状态方程[11]为
p+pa=(γ−1)ρEm (5) Em=cV(θ−θz) (6) 式中:γ为绝热指数,pa为外界压力,cV为比定容热容,
θ 为当前温度,θz 为绝对零度。空气的状态方程参数如表3[12]所示。1.2 数值模型
为研究近海底水下爆炸冲击波的传播规律,建立了数值模型,欧拉域尺寸为15.0 m×6.5 m×15.0 m,模拟50 kg TNT在50 m水深处的近海底爆炸,TNT底部距海底0.05 m。将整个欧拉域的边界条件设置为流出无反射,海底底质采用模型1或模型2描述。模型1是一种较软的土壤模型,参考了Ambrosini等[13]和Luccioni等[14]的研究结果,具体参数见表4[13–14],其中:E为杨氏模量,ν为泊松比,φ为内摩擦角,c为黏聚力。模型2为刚性固壁,通过在欧拉域中的海底底质区域放置拉格朗日刚体实现。
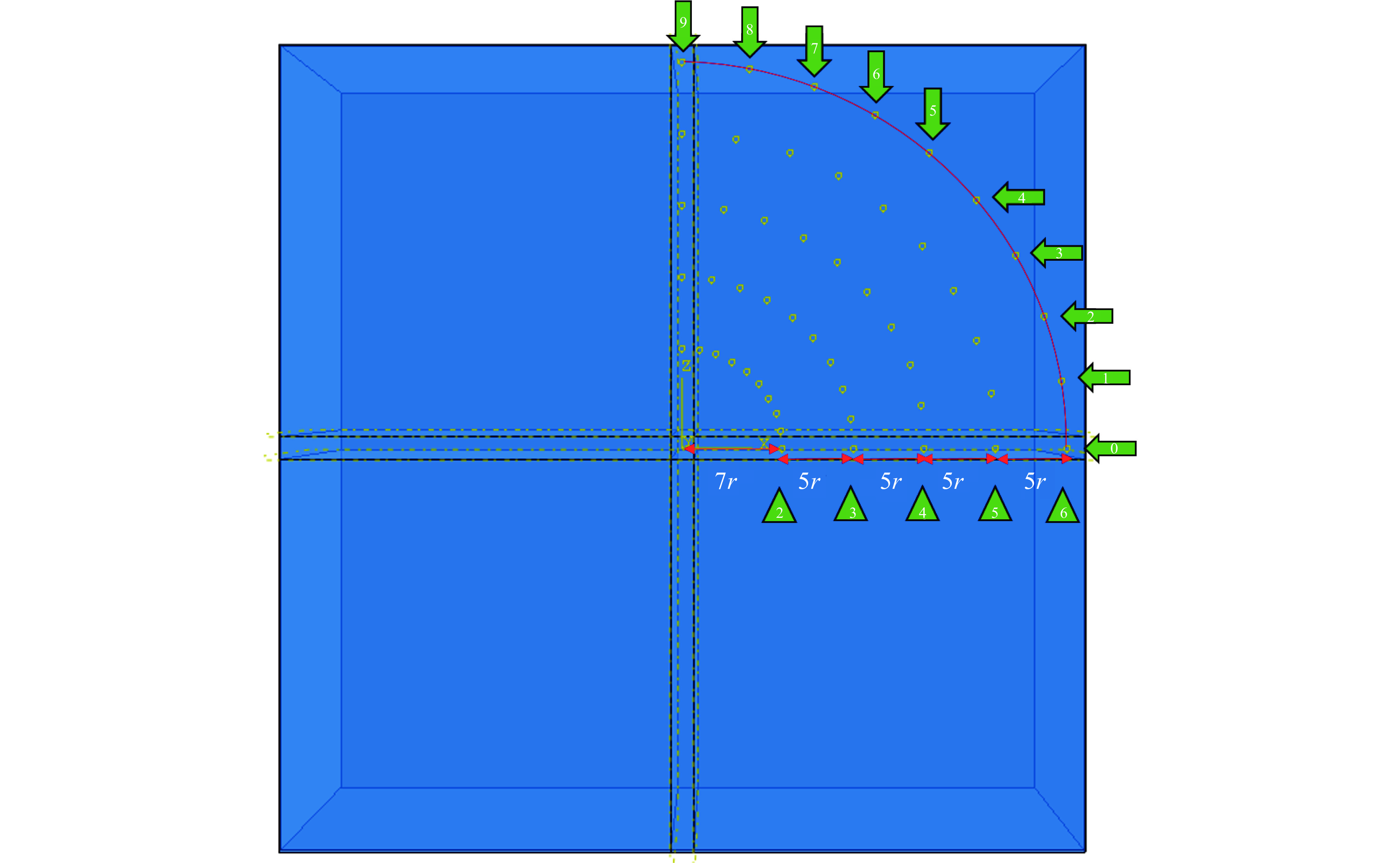
为了更准确地获得同一位置的冲击波压力,在模型的不同位置设置测点,测点布局如图1所示。设装药半径为r,测点到爆心的距离为R,测点与水平方向的夹角为测点角度α。在距离爆心分别为7r、12r、17r、22r、27r,测点角度分别为0°、10°、20°、30°、40°、50°、60°、70°、80°、90°处布置测点,共计50个测点。为方便后续分析,用测点距爆心的距离(爆距)以及测点角度命名测点,爆距7r、12r、17r、22r、27r用数字2~6表示,测点角度0°~90°用数字0~9表示,例如:将爆距为7r、测点角度为0°的测点命名为2-0。
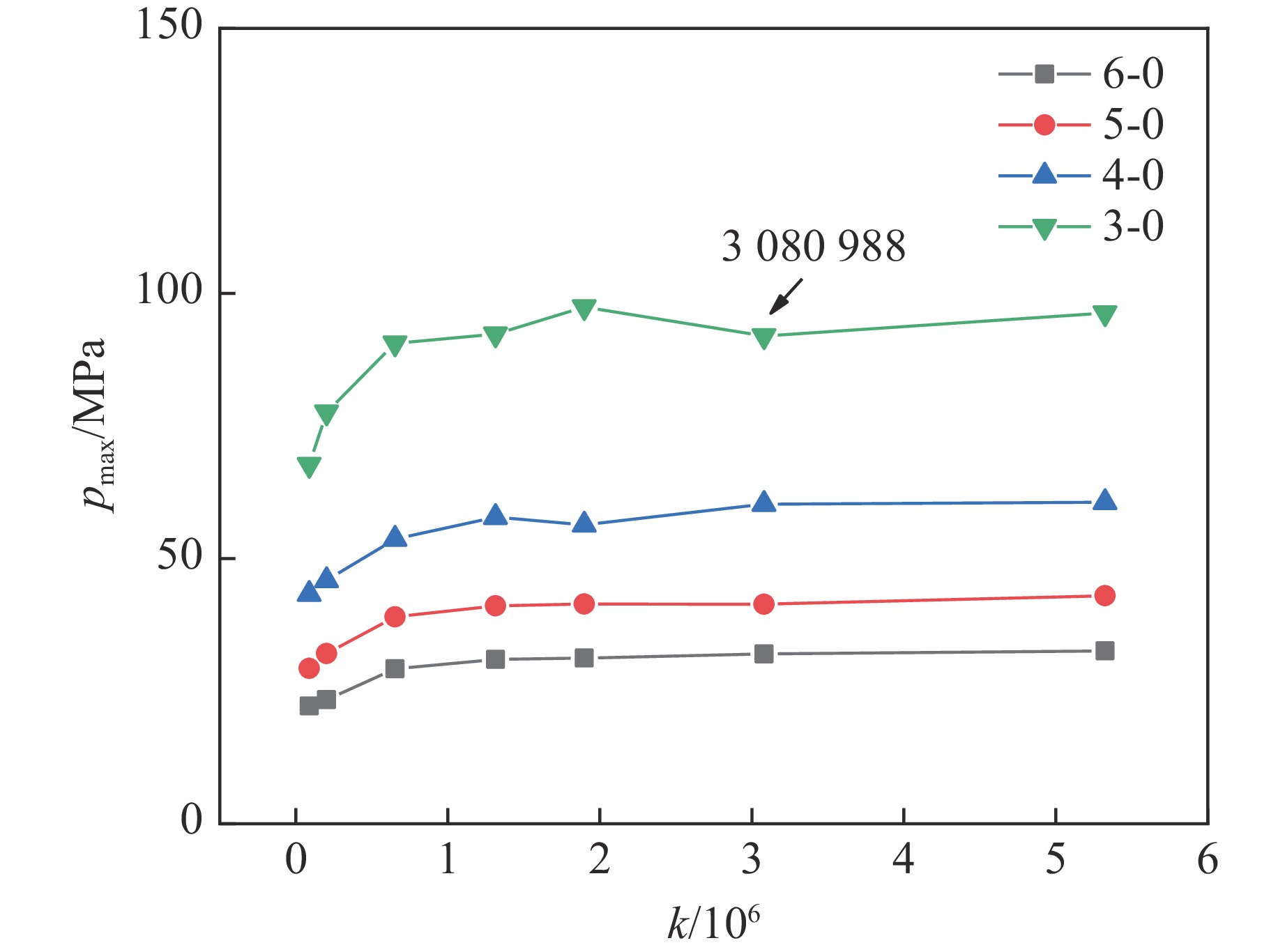
为验证结果的可靠性,对测点3-0、4-0、5-0、6-0测得的冲击波峰值压力进行收敛性分析,得到冲击波峰值压力pmax随网格数量k的变化曲线,如图2所示。当网格数量为3080988时,测点测得的冲击波峰值压力基本收敛。为此,选取网格数量为5324000来研究近海底水下爆炸冲击波的载荷特性。
1.3 数值验证
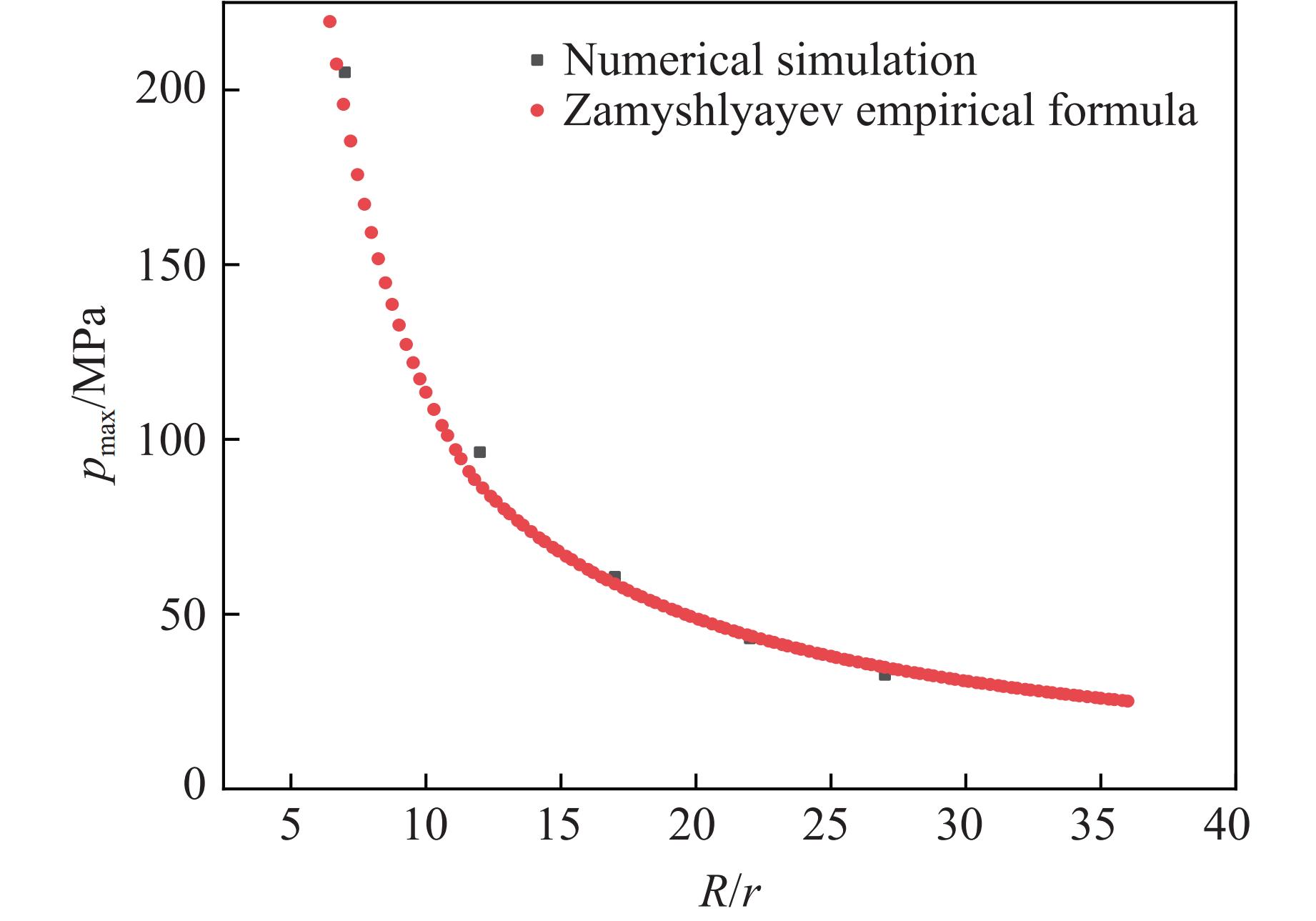
为验证模型的准确性,选取测点2-0、3-0、4-0、5-0、6-0在无海底底质时测得的峰值压力与Zamyshlyayev经验公式计算结果进行对比,如图3和表5所示。可以看出,数值模拟结果与经验公式拟合结果符合得较好,说明该模型能够用于近海底水下爆炸冲击波研究。
表 5 数值模拟与经验公式结果对比Table 5. Comparison of numerical simulation and empirical formula resultsR/r Peak pressure/MPa Error/% Simulation Empirical formula 7 205.03 193.72 5.84 12 96.32 86.90 10.84 17 60.65 58.62 3.45 22 43.04 43.81 1.75 27 32.58 37.91 14.07 1.4 工况设置
为研究水深(H)及海底底质对近海底水下爆炸冲击波载荷特性的影响,仅改变海底底质及静水压力,其他条件保持不变,进行数值模拟,计算工况如表6所示,其中:工况1和工况2用于研究自由场与近海底冲击波压力的差异,工况2和工况3用于研究底质条件对近海底爆炸冲击波载荷特性的影响,工况2、工况4和工况5用于研究水深对近海底水下爆炸冲击波载荷特性的影响。
表 6 数值模拟工况设置Table 6. Settings of simulation casesCase H/m Seafloor sediment Explosive environment 1 50 Nothing Free field 2 50 Model 1 Near the seabed 3 50 Model 2 Near the seabed 4 100 Model 1 Near the seabed 5 150 Model 1 Near the seabed 2. 结果讨论与分析
2.1 自由场和近海底爆炸冲击波特性对比
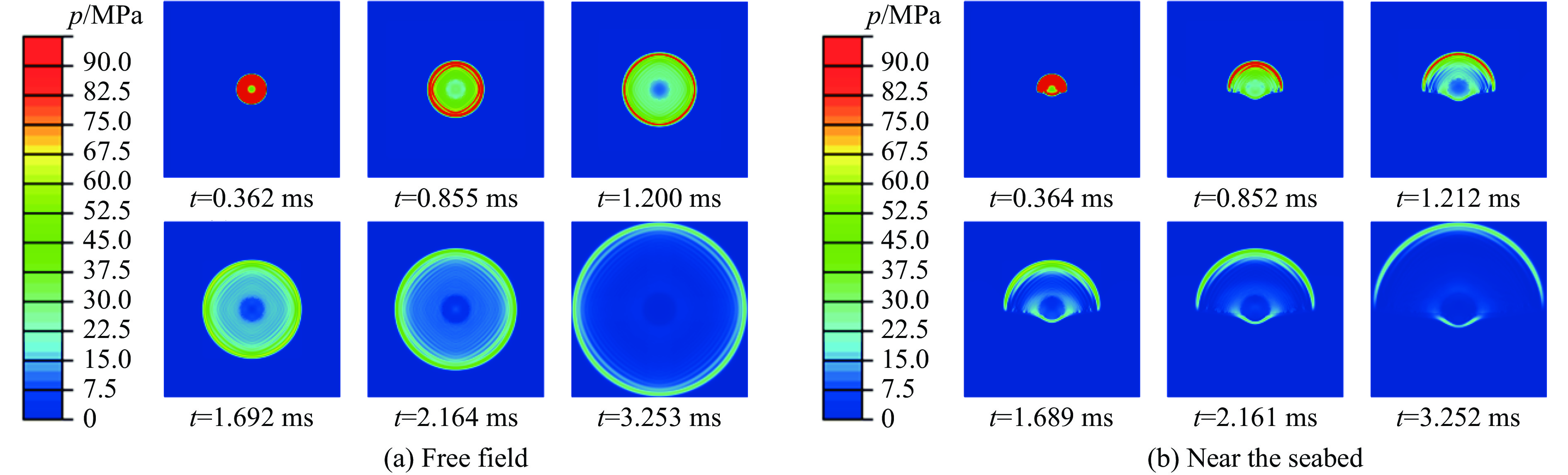
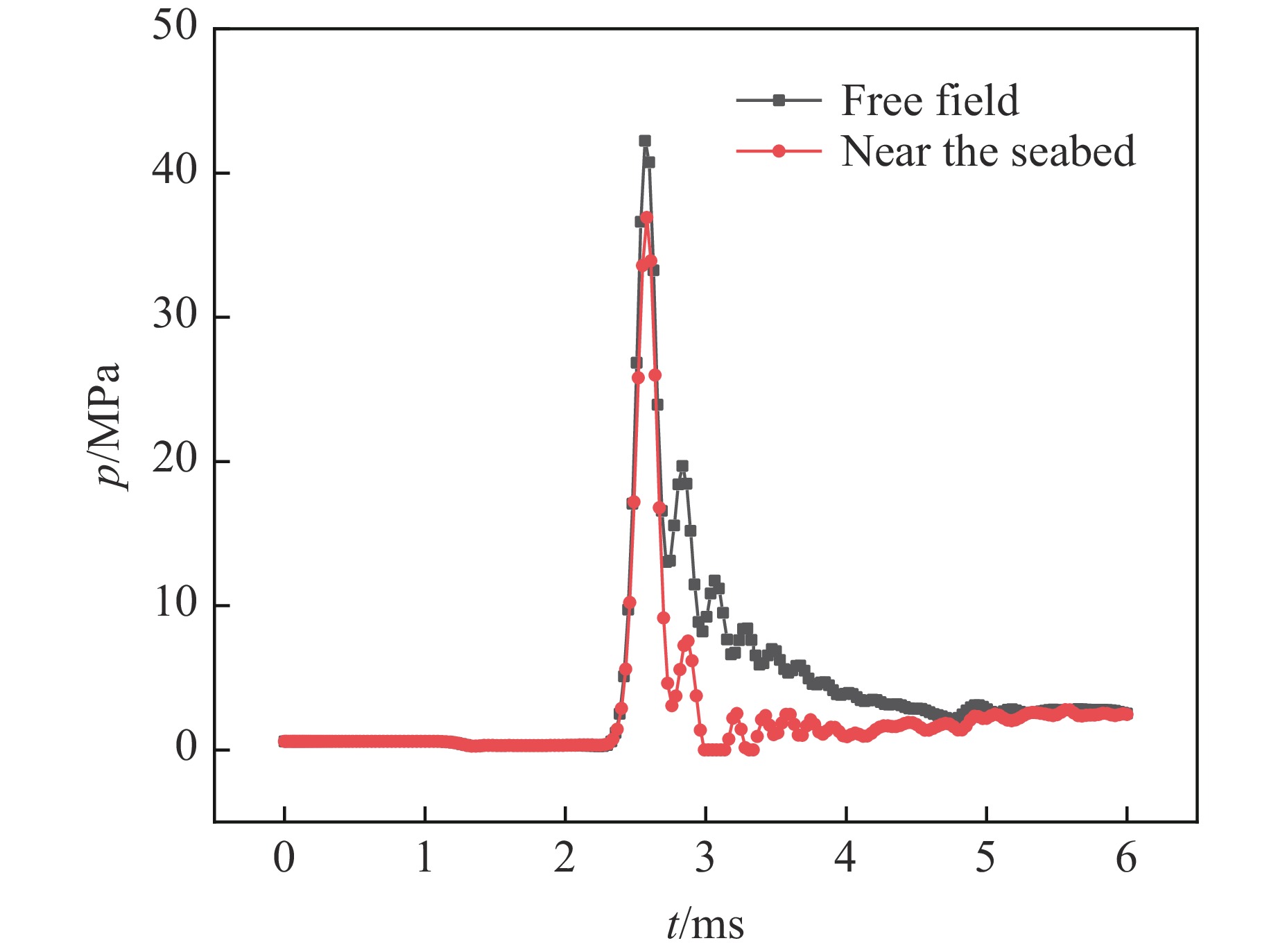
图4为工况1和工况2下不同时刻的冲击波压力云图,图5为测点5-3在工况1和工况2下测得的爆炸冲击波压力时程曲线。可以看出,自由场水下爆炸与近海底水下爆炸存在明显差别,受近海底条件的影响,近海底水下爆炸冲击波形状不是规则的圆形,冲击波波面上的压力峰值并非处处相等,而是越靠近海底区域,冲击波压力越小,测点5-3在近海底条件下测得的冲击波峰值压力及后续的脉动压力均小于自由场条件下测得的压力。由于水的冲击阻抗大于海底底质模型1的冲击阻抗,因此,水中冲击波在近海底的反射波为稀疏波,稀疏波追上冲击波波面将会导致冲击波压力降低;另外,海底底质模型1是一种较软且易变形的底质,冲击波作用在海底底质上,不仅会发生反射,还会发生透射,部分能量传递到海底底质中,使海底底质发生形变,冲击波能量也会发生损耗。因此,在近海底条件下,冲击波峰值压力和气泡脉动压力均小于自由场下的压力,理论与数值模拟结果相符。
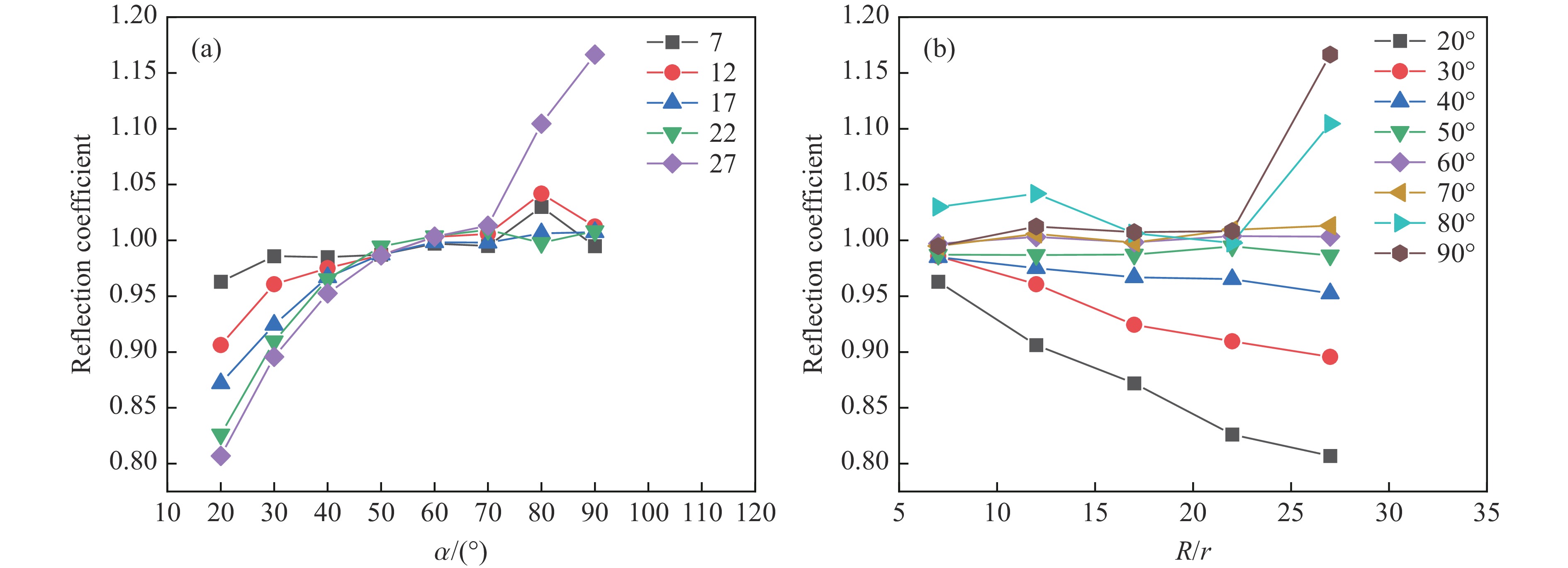
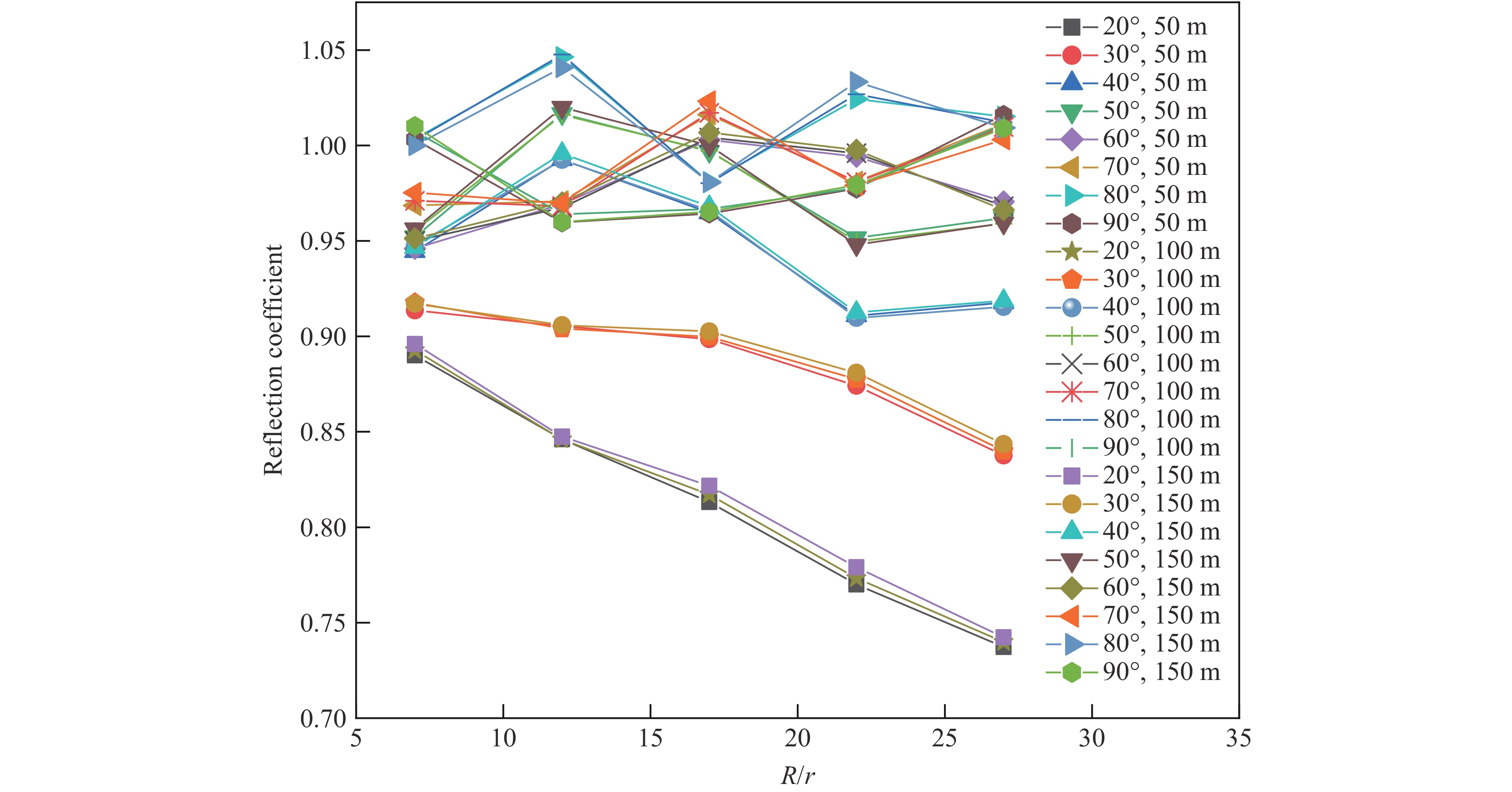
近海底水下爆炸时,靠近海底区域处的冲击波压力明显偏小,即不同测点角度(α)条件下的冲击波峰值压力存在差异。定义测点测得的冲击波峰值压力与自由场时的冲击波峰值压力之比为该测点的反射系数。计算所有测点的反射系数,结果如图6所示。当爆距比一定时,随着α的增大,反射系数也逐渐增大,即海底吸能现象和稀疏波的影响逐渐减小;α在0°~10°区间时,海底的吸能现象过于剧烈,故不对该角度范围进行深入研究;当α处于20°~30°区间时,测点的反射系数随爆距比增加呈减小趋势,即近海底反射的影响随爆距比的增大而增强;当α > 40°时,海底吸能现象不明显。
为了更细致地研究近海底反射规律,将自由场与近海底工况下测点2-2~测点2-9、测点3-2~测点3-9、测点4-2~测点4-9的冲击波峰值压力进行对比,结果如表7所示,可以看出:当α处于20°~30°时,近海底工况下,冲击波峰值压力是自由场工况下冲击波峰值压力的81%~91%;随着α的增大,测点距海底越来越远,近海底反射现象也越来越弱,近海底反射影响逐渐消失。
表 7 自由场与近海底测得的冲击波峰值压力对比Table 7. Comparison of peak pressures of free-field and near-seabed underwater explosion shock waveR/r α/(°) pmax/MPa Reflection
coefficientR/r α/(°) pmax/MPa Reflection
coefficientNear seabed Free field Near seabed Free field 7 20 179.29 201.44 0.89 12 60 92.73 95.67 0.97 7 30 186.21 203.80 0.91 12 70 95.00 97.86 0.97 7 40 193.43 204.80 0.94 12 80 98.33 93.98 1.05 7 50 194.98 204.80 0.95 12 90 92.42 96.30 0.96 7 60 192.78 203.80 0.95 17 20 47.78 58.76 0.81 7 70 195.10 201.43 0.97 17 30 53.24 59.25 0.90 7 80 202.46 201.74 1.00 17 40 56.52 58.58 0.96 7 90 196.41 195.79 1.00 17 50 58.40 58.58 1.00 12 20 82.82 97.87 0.85 17 60 59.44 59.27 1.00 12 30 86.62 95.67 0.91 17 70 61.07 60.10 1.02 12 40 93.96 94.66 0.99 17 80 60.00 61.17 0.98 12 50 96.23 94.65 1.02 17 90 57.77 59.91 0.96 2.2 海底底质对近海底爆炸冲击波载荷特性的影响
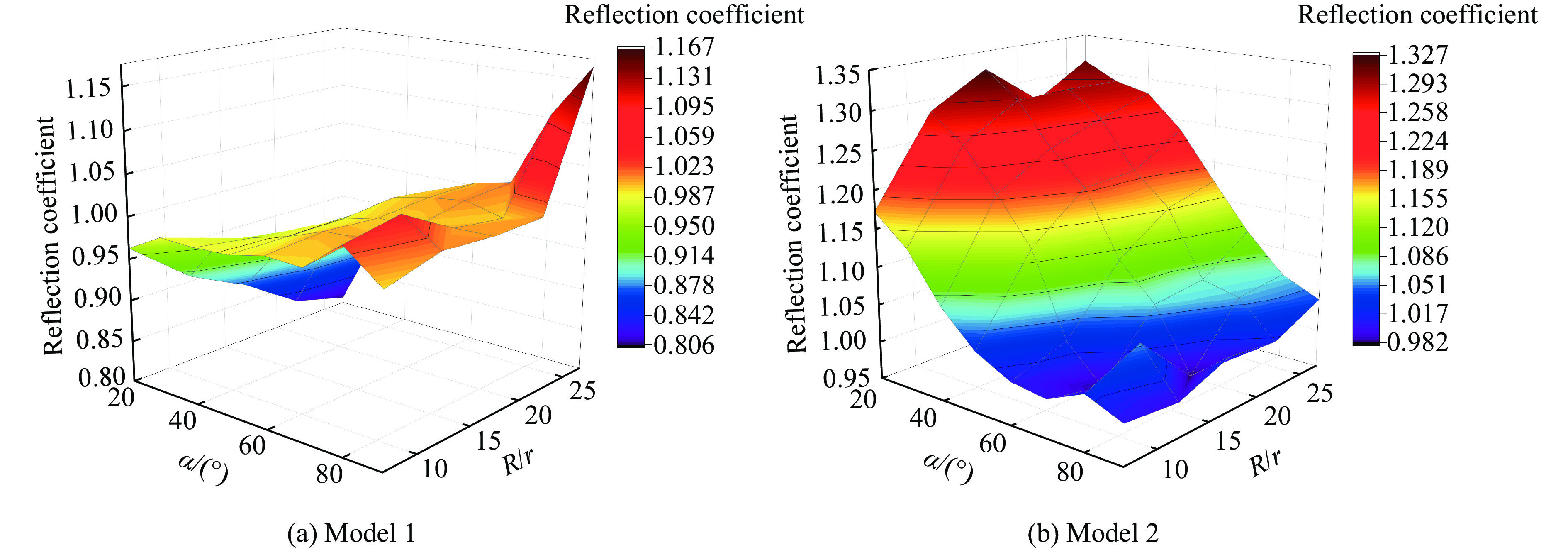
图7显示了工况2和工况3下相同测点测得的近海底冲击波反射系数对比。可以发现,2种底质条件下冲击波传播规律存在明显差异:当底质为模型1时,由于近海底反射波为稀疏波,稀疏波追上冲击波波面将导致冲击波峰值压力变小,且海底底质模型1较软,吸能作用较强,因此,绝大部分测点的反射系数小于1;当海底底质为模型2时,受刚固边界的影响,近海底反射波为压缩波,压缩波追上冲击波波面会导致冲击波峰值压力增大,且海底底质模型2的吸能作用较弱,绝大部分测点的反射系数大于1。无论底质是模型1还是模型2,反射系数异常均发生在爆距比为7~15、α在60°~90°范围内。
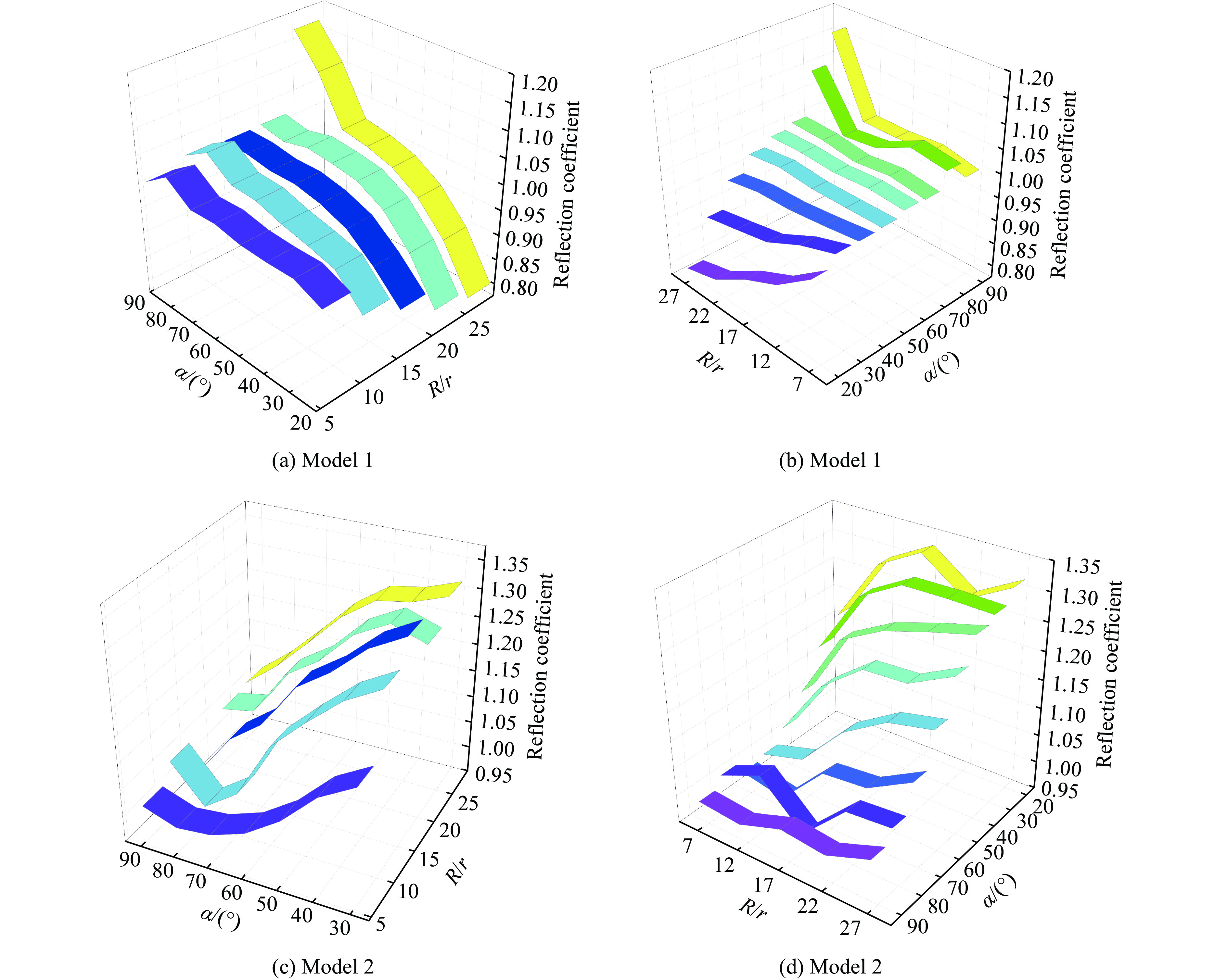
为更清楚地研究不同底质条件对反射系数的影响,将不同底质条件下反射系数随测点角度和爆距比的变化绘制成图8。由图8(a)可知,当海底底质为模型1、爆距比保持不变时,反射系数随测点角度的增大呈增大趋势。由图8(c)可知,当海底底质为模型2、爆距比一定时,反射系数随测点角度的增大呈减小趋势。
当海底底质为模型1时,海底底质较软,海底反射稀疏波,对TNT爆炸冲击波的吸收较强,因此,近海底冲击波峰值压力减小,反射系数小于1是常态。图8(a)显示,当爆距比一定时,测点角度越大,反射系数就越大,因而可以推断,海底底质对不同测点角度处反射系数的影响随测点角度的增大而减弱。当海底底质为模型2时,海底底质较硬,海底反射压缩波,对冲击波的吸收很弱,因此,近海底冲击波峰值压力更大,反射系数大于1是常态。图8(c)显示,当爆距比一定时,测点角度越大,反射系数越小,同样可以得到海底底质对不同测点角度处反射系数的影响随测点角度增大而减弱的结论。
从图8(b)可以看出,当测点角度处于20°~40°区间时,不同测点测得的近海底冲击波反射系数随着爆距比的增大而减小;而图8(d)显示,当测点角度处于20°~60°区间时,不同测点测得的近海底冲击波反射系数随着爆距比的增大而增大。由于2种底质对近海底冲击波反射系数的影响截然相反,因此,综合图8(b)和图8(d)可以看出,测点角度在一定范围内时,海底底质对反射系数的影响随着测点爆距比的增大而增强。当测点角度超出该范围时,该现象减弱甚至消失。综合图8(a)~图8(d)可以发现,虽然海底底质发生了变化,不同海底底质对冲击波的影响效果不同,但是测点反射系数受显著影响的区域均集中在20°~50°范围。
为研究近海底水下爆炸反射波对冲击波的影响是否与起爆深度有关,将底质条件为模型1时不同水深处各测点的反射系数进行对比,结果如图9所示。从图9可以看出,随着水深的增加,同一测点处的反射系数基本一致,说明水深并不能显著影响反射系数,即水深的变化并不会对近海底反射现象造成显著影响,也不会对海底底质的吸能作用造成显著影响,近海底反射稀疏波对冲击波的削减作用并不会随着静水压力的变化而发生显著变化。
3. 结 论
基于CEL方法建立了近海底水下爆炸数值模型,对近海底爆炸冲击波的时空分布规律进行了研究,探究了海底底质和水深对近海底爆炸冲击波载荷特性的影响规律,得到如下结论。
(1) 近海底水下爆炸冲击波载荷特性与自由场明显不同。当测点角度为20°~30°时,近海底反射系数为0.81~0.91;随着测点角度进一步增大,近海底反射的影响逐渐减弱;当测点角度达到80°~90°时,近海底反射的影响基本消失。
(2) 在一定的角度范围内,近海底反射的影响随着爆距比的增大而增强,超出该角度范围后,该现象基本消失。改变底质时,近海底反射的影响使冲击波峰值压力增强或减弱,并且影响区域基本一致。水深对近海底水下爆炸反射系数无显著影响。
(3) 海底底质材料属性不同时,其对爆炸冲击波的吸收作用也存在差异,近海底水下爆炸反射波的种类也不一致。当海底底质较硬时,海底底质对冲击波的吸收作用相对较弱,反射波为压缩波,致使测点角度在20°~50°范围内的冲击波峰值压力增大,当测点角度在20°~50°范围内且测点角度一定时,反射系数随爆距比的增大而增大;当海底底质较软时,海底底质对冲击波的吸收作用较强,近海底反射波为稀疏波,致使20°~50°角度范围内冲击波峰值压力减小,当测点角度在20°~50°范围内且测点角度一定时,反射系数随爆距比的增大而减小。海底底质对反射系数的影响区域主要集中在20°~50°测点范围内,超出该范围时,海底底质对反射系数的影响随着测点角度的增大而逐渐消失,海底底质对反射系数的影响随爆距比的增大而增强的现象也随测点角度的增大而逐渐消失。
-
图 7 位错平均运动与热激活运动以及拖曳运动的对比[27]
Figure 7. Comparison of the average dislocation velocity with the velocities of thermally-activated and drag-dominated dislocation motions[27]
图 12 铅合金动态晶体塑性有限元模拟结果:(a)层裂形核时的压力,(b)层裂形核时的弹性能密度,(c)经250 m/s冲击加载层裂面附近的等效应力;(d)经350 m/s冲击加载层裂面附近的等效应力[24]
Figure 12. Dynamic crystal plasticity finite element simulation results of lead alloy: (a) pressure of spalling nucleation; (b) elastic energy density of spalling nucleation; (c) equivalent stress near the spalling surface under 250 m/s shock loading; (d) equivalent stress near the spalling surface under 350 m/s shock loading[24]
A1 运动学符号说明
A1. Symbol description of kinematics
Symbols Description F(Fe, Fp, Fθ ) Deformation gradient including elastic, plastic and thermal components L(Le, Lp, Lθ ) Velocity gradient including elastic, plastic and thermal components Re Rotation tensor Ue Right stretch tensor α Thermal expansion coefficient tensor A2 热力学符号说明
A2. Symbol description of thermodynamics
Symbols Description Symbols Description Dint Intrinsic dissipation of the system K0 Bulk modulus at zero pressure ψ Helmholtz free energy K′ Pressure derivative of bulk modulus s Entropy of the system TD Debye temperature T Temperature R Molar gas constant KT Isothermal bulk modulus Mmol Molar mass of the material cV Heat capacity at constant volume kB Boltzmann constant Γ Grüneisen coefficient XTN Variables related to the lattice thermal vibration qn Internal variables for microscopic defects such
as dislocations in materialsXTE Variables related to the electron activation A3 塑性本构符号说明
A3. Symbol description of plastic constitution
Symbols Description Symbols Description λ α Mean spacing between obstacles ραfor Forest dislocation density τα Resolved shear stress tαr The drag-dominated mean transit time between obstacles Qα Activation energy B Viscous drag coefficient gα Slip resistance ˙ραnuc The nucleation rate gαath Athermal slip resistance ˙ραhom The homogeneous nucleation rate hαβ Hardening coefficient ˙ραhet The heterogeneous nucleation rate ρα Total dislocation density ˙ραmult The multiplication rate ραm Mobile dislocation density ˙ραtrap The trapping rate ραi Immobile dislocation density ˙ραann The annihilation rate bα Burgers vector da Capture distance of annihilation vα Velocity of mobile dislocations tαw The thermal activation-dominated waiting time at a barrier ˙γα Slip rate on slip system α A4 超弹性本构符号说明
A4. Symbol description of hyper-elastic constitution
Symbols Description Symbols Description I Second-order unit tensor ^Ee Isochoric strain in expanded configuration Ee Elastic Green–Lagrange strain ^^Ee Isochoric strain in configuration I Ce Elastic right Cauchy-Green tensor ¯Ee Volumetric strain in configuration I ^Fe Isochoric part of elastic deformation S Second Piola–Kirchhoff stress ¯Fe Volumetric expansion A5 相变、孪晶与动态破坏符号说明
A5. Symbol description of phase transformation, twining and damage
Symbols Description Symbols Description Ftr Deformation gradient of phase transformation Sβtw Twin resistance of twin system vp Volume fraction of the parent phase ρdeb Dislocationdebris density vt Volume fraction of the new phase t dmfp Dislocation mean free path related to the volume fraction of twin vN Volume fraction of all new phases φ Void volume fraction ft Driving force of phase transformation Fd Volumetricpartofplastic deformation gradient in porous crystal plastic model f β Volume fraction of twin Yr Resistance of damage evolution γtw Characteristic shear strain of twining -
[1] BODNER S R, PARTOM Y. Constitutive equations for elastic-viscoplastic strain-hardening materials [J]. Journal of Applied Mechanics, 1975, 42(2): 385–389. doi: 10.1115/1.3423586 [2] JOHNSON G R. A constitutive model and data for materials subjected to large strains, high strain rates, and high temperatures [J]. Proceeding of the 7th International Symposium on Ballistics, 1983: 541–547. [3] ZERILLI F J, ARMSTRONG R W. Dislocation-mechanics-based constitutive relations for material dynamics calculations [J]. Journal of Applied Physics, 1987, 61(5): 1816–1825. doi: 10.1063/1.338024 [4] STEINBERG D J, COCHRAN S G, GUINAN M W. A constitutive model for metals applicable at high-strain rate [J]. Journal of Applied Physics, 1980, 51(3): 1498–1504. doi: 10.1063/1.327799 [5] GUPTA Y M, DUVALL G E, FOWLES G R. Dislocation mechanisms for stress relaxation in shocked LiF [J]. Journal of Applied Physics, 1975, 46(2): 532–546. doi: 10.1063/1.321678 [6] ASAY J R, FOWLES G R, DURALL G E, et al. Effects of point defects on elastic precursor decay in LiF [J]. Journal of Applied Physics, 1972, 43(5): 2132–2145. doi: 10.1063/1.1661464 [7] WOLFER W G. Phonon drag on dislocations at high pressures: UCRL-ID-136221 [R]. Office of Scientific and Technical Information (OSTI), 1999. [8] CAWKWELL M J, RAMOS K J, HOOKS D E, et al. Homogeneous dislocation nucleation in cyclotrimethylene trinitramine under shock loading [J]. Journal of Applied Physics, 2010, 107(6): 063512. doi: 10.1063/1.3305630 [9] SHEHADEH M A, BRINGA E M, ZBIB H M, et al. Simulation of shock-induced plasticity including homogeneous and heterogeneous dislocation nucleations [J]. Applied Physics Letters, 2006, 89(17): 171918. doi: 10.1063/1.2364853 [10] CERRETA E K, ESCOBEDO J P, RIGG P A, et al. The influence of phase and substructural evolution during dynamic loading on subsequent mechanical properties of zirconium [J]. Acta Materialia, 2013, 61(20): 7712–7719. doi: 10.1016/j.actamat.2013.09.009 [11] BOURNE N K, MILLETT J C F, CHEN M, et al. On the Hugoniot elastic limit in polycrystalline alumina [J]. Journal of Applied Physics, 2007, 102(7): 073514. doi: 10.1063/1.2787154 [12] MEYERS M A, GREGORI F, KAD B K, et al. Laser-induced shock compression of monocrystalline copper: characterization and analysis [J]. Acta Materialia, 2003, 51(5): 1211–1228. doi: 10.1016/S1359-6454(02)00420-2 [13] RAHUL, DE S. A phase-field model for shock-induced α-γ phase transition of RDX [J]. International Journal of Plasticity, 2017, 88: 140–158. doi: 10.1016/j.ijplas.2016.10.006 [14] ADDESSIO F L, LUSCHER D J, CAWKWELL M J, et al. A single-crystal model for the high-strain rate deformation of cyclotrimethylene trinitramine including phase transformations and plastic slip [J]. Journal of Applied Physics, 2017, 121(18): 185902. doi: 10.1063/1.4983009 [15] WINEY J M, GUPTA Y M. Shock wave compression of hexagonal-close-packed metal single crystals: time-dependent, anisotropic elastic-plastic response of beryllium [J]. Journal of Applied Physics, 2014, 116(3): 033505. doi: 10.1063/1.4889886 [16] KADAU K, GERMANN T C, LOMDAHL P S, et al. Shock waves in polycrystalline iron [J]. Physical Review Letters, 2007, 98(13): 135701. doi: 10.1103/PhysRevLett.98.135701 [17] KANEL G I. Spall fracture: methodological aspects, mechanisms and governing factors [J]. International Journal of Fracture, 2010, 163(1/2): 173–191. [18] CHEN X, ASAY J R, DWIVEDI S K, et al. Spall behavior of aluminum with varying microstructures [J]. Journal of Applied Physics, 2006, 99(2): 023528. doi: 10.1063/1.2165409 [19] ROTERS F, EISENLOHR P, HANTCHERLI L, et al. Overview of constitutive laws, kinematics, homogenization and multiscale methods in crystal plasticity finite-element modeling: theory, experiments, applications [J]. Acta Materialia, 2010, 58(4): 1152–1211. doi: 10.1016/j.actamat.2009.10.058 [20] 郑松林. 晶体塑性有限元在材料动态响应研究中的应用进展 [J]. 高压物理学报, 2019, 33(3): 030108. doi: 10.11858/gywlxb.20190725ZHENG S L. Advances in the study of dynamic response of crystalline materials by crystal plasticity finite element modeling [J]. Chinese Journal of High Pressure Physics, 2019, 33(3): 030108. doi: 10.11858/gywlxb.20190725 [21] HILL R, RICE J R. Constitutive analysis of elastic-plastic crystals at arbitrary strain [J]. Journal of the Mechanics and Physics of Solids, 1972, 20(6): 401–413. doi: 10.1016/0022-5096(72)90017-8 [22] ASARO R J. Micromechanics of crystals and polycrystals [M]//Advances in Applied Mechanics. Elsevier, 1983: 1–115. [23] CLAYTON J D. Dynamic plasticity and fracture in high density polycrystals: constitutive modeling and numerical simulation [J]. Journal of the Mechanics and Physics of Solids, 2005, 53(2): 261–301. doi: 10.1016/j.jmps.2004.06.009 [24] VOGLER T, CLAYTON J. Heterogeneous deformation and spall of an extruded tungsten alloy: plate impact experiments and crystal plasticity modeling [J]. Journal of the Mechanics and Physics of Solids, 2008, 56(2): 297–335. doi: 10.1016/j.jmps.2007.06.013 [25] HANSEN B L, BEYERLEIN I J, BRONKHORST C A, et al. A dislocation-based multi-rate single crystal plasticity model [J]. International Journal of Plasticity, 2013, 44: 129–146. doi: 10.1016/j.ijplas.2012.12.006 [26] DE S, ZAMIRI A R, RAHUL. A fully anisotropic single crystal model for high strain rate loading conditions with an application to α-RDX [J]. Journal of the Mechanics and Physics of Solids, 2014, 64: 287–301. doi: 10.1016/j.jmps.2013.10.012 [27] SHAHBA A, GHOSH S. Crystal plasticity FE modeling of Ti alloys for a range of strain-rates. Part Ⅰ: a unified constitutive model and flow rule [J]. International Journal of Plasticity, 2016, 87: 48–68. doi: 10.1016/j.ijplas.2016.09.002 [28] BELYTSCHKO T, LIU W K, MORAN B, et al. Nonlinear finite elements for continua and structures [M]. John Wiley & Sons, 2013. [29] BECKER R. Effects of crystal plasticity on materials loaded at high pressures and strain rates [J]. International Journal of Plasticity, 2004, 20(11): 1983–2006. doi: 10.1016/j.ijplas.2003.09.002 [30] PI A G, HUANG F L, WU Y Q, et al. Anisotropic constitutive model and numerical simulations for crystalline energetic material under shock loading [J]. Mathematics and Mechanics of Solids, 2014, 19(6): 640–658. doi: 10.1177/1081286513482322 [31] LUSCHER D J, BRONKHORST C A, ALLEMAN C N, et al. A model for finite-deformation nonlinear thermomechanical response of single crystal copper under shock conditions [J]. Journal of the Mechanics and Physics of Solids, 2013, 61(9): 1877–1894. doi: 10.1016/j.jmps.2013.05.002 [32] LUSCHER D J, MAYEUR J R, MOURAD H M, et al. Coupling continuum dislocation transport with crystal plasticity for application to shock loading conditions [J]. International Journal of Plasticity, 2016, 76: 111–129. doi: 10.1016/j.ijplas.2015.07.007 [33] DOS SANTOS T, ROSA P A R, MAGHOUS S, et al. A simplified approach to high strain rate effects in cold deformation of polycrystalline FCC metals: constitutive formulation and model calibration [J]. International Journal of Plasticity, 2016, 82: 76–96. doi: 10.1016/j.ijplas.2016.02.003 [34] STAINIER L, ORTIZ M. Study and validation of a variational theory of thermo-mechanical coupling in finite visco-plasticity [J]. International Journal of Solids and Structures, 2010, 47(5): 705–715. doi: 10.1016/j.ijsolstr.2009.11.012 [35] VON NEUMANN J, RICHTMYER R D. A method for the numerical calculation of hydrodynamic shocks [J]. Journal of Applied Physics, 1950, 21(3): 232–237. doi: 10.1063/1.1699639 [36] VINET P, SMITH J R, FERRANTE J, et al. Temperature effects on the universal equation of state of solids [J]. Physical Review B, 1987, 35(4): 1945. doi: 10.1103/PhysRevB.35.1945 [37] 李欣竹. 金属物态方程的讨论 [D]. 绵阳: 中国工程物理研究院, 2003.LI X Z. Discussion for the semi-empiric equation of state of metals [D]. Mianyang: China Academy of Engineering Physics, 2003. [38] CAWKWELL M J, LUSCHER D J, ADDESSIO F L, et al. Equations of state for the α and γ polymorphs of cyclotrimethylene trinitramine [J]. Journal of Applied Physics, 2016, 119(18): 185106. doi: 10.1063/1.4948673 [39] LUSCHER D J, ADDESSIO F L, CAWKWELL M J, et al. A dislocation density-based continuum model of the anisotropic shock response of single crystal α-cyclotrimethylene trinitramine [J]. Journal of the Mechanics and Physics of Solids, 2017, 98: 63–86. doi: 10.1016/j.jmps.2016.09.005 [40] 尚福林, 王子昆. 塑性力学基础 [M]. 西安: 西安交通大学出版社, 2011.SHANG F L, WANG Z K. Fundamentals of of plasticity [M]. Xi’an: Xi’an Jiaotong University Press, 2011. [41] HUTCHINSON J W. Bounds and self-consistent estimates for creep of polycrystalline materials [J]. Proceedings of the Royal Society A: Mathematical, Physical and Engineering Sciences, 1976, 348(1652): 101–127. [42] LI H W, YANG H, SUN Z C. A robust integration algorithm for implementing rate dependent crystal plasticity into explicit finite element method [J]. International Journal of Plasticity, 2008, 24(2): 267–288. doi: 10.1016/j.ijplas.2007.03.014 [43] KHAN A S, LIU J, YOON J W, et al. Strain rate effect of high purity aluminum single crystals: experiments and simulations [J]. International Journal of Plasticity, 2015, 67: 39–52. doi: 10.1016/j.ijplas.2014.10.002 [44] ZHANG K, HOPPERSTAD O, HOLMEDAL B, et al. A robust and efficient substepping scheme for the explicit numerical integration of a rate-dependent crystal plasticity model [J]. International Journal for Numerical Methods in Engineering, 2014, 99(4): 239–262. doi: 10.1002/nme.4671 [45] LIM H, JONG BONG H, CHEN S R, et al. Developing anisotropic yield models of polycrystalline tantalum using crystal plasticity finite element simulations [J]. Materials Science and Engineering A, 2018, 730: 50–56. doi: 10.1016/j.msea.2018.05.096 [46] BOBBILI R, MADHU V. Crystal plasticity modeling of a near alpha titanium alloy under dynamic compression [J]. Journal of Alloys and Compounds, 2018, 759: 85–92. doi: 10.1016/j.jallcom.2018.05.167 [47] KOCKS U F, ARGON A S, ASHBY M F. Thermodynamics and kinetics of slip [J]. Progress in Materials Science, 1975, 19: 141–145. [48] LI J F, ROMERO I, SEGURADO J. Development of a thermo-mechanically coupled crystal plasticity modeling framework: application to polycrystalline homogenization [J]. International Journal of Plasticity, 2019, 119: 313–330. doi: 10.1016/j.ijplas.2019.04.008 [49] BRONKHORST C A, GRAY G T III, ADDESSIO F L, et al. Publisher's note: “Response and representation of ductile damage under varying shock loading conditions in tantalum”[J. Appl. Phys. 119, 085103(2016)] [J]. Journal of Applied Physics, 2016, 119(10): 109901. [50] CHANDRA S, SAMAL M K, KAPOOR R, et al. Deformation behavior of Nickel-based superalloy Su-263: experimental characterization and crystal plasticity finite element modeling [J]. Materials Science and Engineering A, 2018, 735: 19–30. doi: 10.1016/j.msea.2018.08.022 [51] 王礼立, 胡时胜, 杨黎明. 材料动力学 [M]. 合肥: 中国科学技术大学出版社, 2017.WANG L L, HU S S, YANG L M. Thermodynamics and kinetics of materials [M]. Hefei: Press of University of Science and Technology of China, 2017. [52] MEYERS M A. 材料的动力学行为 [M]. 张庆明, 译. 北京: 国防工业出版社, 2006.MEYERS M A. Dynamic behavior of materials [M]. Translated by ZHANG Q M. Beijing: National Defense Industry Press, 2006. [53] 黄克智, 肖纪美. 材料的损伤断裂机理和宏微观力学理论 [M]. 北京: 清华大学出版社, 1999.HUANG K Z, XIAO J M. Damage and fracture mechanisms of materials and macro-micro-mechanics [M]. Beijing: Tsinghua University Press, 1999. [54] BITTENCOURT E. Dynamic explicit solution for higher-order crystal plasticity theories [J]. International Journal of Plasticity, 2014, 53: 1–16. doi: 10.1016/j.ijplas.2013.06.010 [55] PEIRCE D, ASARO R J, NEEDLEMAN A. An analysis of nonuniform and localized deformation in ductile single crystals [J]. Acta Metallurgica, 1982, 30(6): 1087–1119. doi: 10.1016/0001-6160(82)90005-0 [56] ACHARYA A, BEAUDOIN A J. Grain-size effect in viscoplastic polycrystals at moderate strains [J]. Journal of the Mechanics and Physics of Solids, 2000, 48(10): 2213–2230. doi: 10.1016/S0022-5096(00)00013-2 [57] CLAYTON J D. Modeling dynamic plasticity and spall fracture in high density polycrystalline alloys [J]. International Journal of Solids and Structures, 2005, 42(16/17): 4613–4640. [58] CLAYTON J D. Plasticity and spall in high density polycrystals: modeling and simulation [C]//AIP Conference Proceedings, Baltimore, Maryland (USA). AIP, 2006. [59] TAJALLI S A, MOVAHHEDY M R, AKBARI J. Simulation of orthogonal micro-cutting of FCC materials based on rate-dependent crystal plasticity finite element model [J]. Computational Materials Science, 2014, 86: 79–87. doi: 10.1016/j.commatsci.2014.01.016 [60] LI J G, LI Y L, HUANG C X, et al. On adiabatic shear localization in nanostructured face-centered cubic alloys with different stacking fault energies [J]. Acta Materialia, 2017, 141: 163–182. doi: 10.1016/j.actamat.2017.09.022 [61] LI J G, LI Y L, SUO T, et al. Numerical simulations of adiabatic shear localization in textured FCC metal based on crystal plasticity finite element method [J]. Materials Science and Engineering A, 2018, 737: 348–363. doi: 10.1016/j.msea.2018.08.105 [62] JOHNSTON W G, GILMAN J J. Dislocation velocities, dislocation densities, and plastic flow in lithium fluoride crystals [J]. Journal of Applied Physics, 1959, 30(2): 129–144. doi: 10.1063/1.1735121 [63] GILMAN J J. The plastic resistance of crystals [J]. Australian Journal of Physics, 1960, 13(2): 327. doi: 10.1071/PH600327a [64] GILMAN J J. Micromechanics of flow in solids [M]. McGraw-Hill, 1969. [65] JOHNSON J N, BARKER L M. Dislocation dynamics and steady plastic wave profiles in 6061-T6 aluminum [J]. Journal of Applied Physics, 1969, 40(11): 4321–4334. doi: 10.1063/1.1657194 [66] ALANKAR A, EISENLOHR P, RAABE D. A dislocation density-based crystal plasticity constitutive model for prismatic slip in α-titanium [J]. Acta Materialia, 2011, 59(18): 7003–7009. doi: 10.1016/j.actamat.2011.07.053 [67] ZHANG H M, DONG X H, DU D P, et al. A unified physically based crystal plasticity model for FCC metals over a wide range of temperatures and strain rates [J]. Materials Science and Engineering A, 2013, 564: 431–441. doi: 10.1016/j.msea.2012.12.001 [68] MONNET G, VINCENT L, DEVINCRE B. Dislocation-dynamics based crystal plasticity law for the low- and high-temperature deformation regimes of bcc crystal [J]. Acta Materialia, 2013, 61(16): 6178–6190. doi: 10.1016/j.actamat.2013.07.002 [69] NGUYEN T, LUSCHER D J, WILKERSON J W. A dislocation-based crystal plasticity framework for dynamic ductile failure of single crystals [J]. Journal of the Mechanics and Physics of Solids, 2017, 108: 1–29. doi: 10.1016/j.jmps.2017.07.020 [70] GRILLI N, JANSSENS K G F, NELLESSEN J, et al. Multiple slip dislocation patterning in a dislocation-based crystal plasticity finite element method [J]. International Journal of Plasticity, 2018, 100: 104–121. doi: 10.1016/j.ijplas.2017.09.015 [71] FROST H J, ASHBY M F. Motion of a dislocation acted on by a viscous drag through an array of discrete obstacles [J]. Journal of Applied Physics, 1971, 42(13): 5273–5279. doi: 10.1063/1.1659936 [72] LIM H, BATTAILE C C, CARROLL J D, et al. A physically based model of temperature and strain rate dependent yield in BCC metals: implementation into crystal plasticity [J]. Journal of the Mechanics and Physics of Solids, 2015, 74: 80–96. doi: 10.1016/j.jmps.2014.10.003 [73] KOCKS W. Thermodynamics and kinetics of slip [J]. Progress in Materials Science, 1975, 19: 1–281. doi: 10.1016/0079-6425(75)90005-5 [74] AUSTIN R A. Elastic precursor wave decay in shock-compressed aluminum over a wide range of temperature [J]. Journal of Applied Physics, 2018, 123(3): 035103. doi: 10.1063/1.5008280 [75] AUSTIN R A, MCDOWELL D L. A dislocation-based constitutive model for viscoplastic deformation of fcc metals at very high strain rates [J]. International Journal of Plasticity, 2011, 27(1): 1–24. doi: 10.1016/j.ijplas.2010.03.002 [76] AUSTIN R A, MCDOWELL D L. Parameterization of a rate-dependent model of shock-induced plasticity for copper, nickel, and aluminum [J]. International Journal of Plasticity, 2012, 32/33: 134–154. doi: 10.1016/j.ijplas.2011.11.002 [77] GAO C Y, ZHANG L C. Constitutive modelling of plasticity of fcc metals under extremely high strain rates [J]. International Journal of Plasticity, 2012, 32/33: 121–133. doi: 10.1016/j.ijplas.2011.12.001 [78] WANG Z Q, BEYERLEIN I J, LESAR R. Slip band formation and mobile dislocation density generation in high rate deformation of single fcc crystals [J]. Philosophical Magazine, 2008, 88(9): 1321–1343. doi: 10.1080/14786430802129833 [79] TSCHOPP M A, MCDOWELL D L. Influence of single crystal orientation on homogeneous dislocation nucleation under uniaxial loading [J]. Journal of the Mechanics and Physics of Solids, 2008, 56(5): 1806–1830. doi: 10.1016/j.jmps.2007.11.012 [80] SHEHADEH M A, ZBIB H M. On the homogeneous nucleation and propagation of dislocations under shock compression [J]. Philosophical Magazine, 2016, 96(26): 2752–2778. doi: 10.1080/14786435.2016.1213444 [81] MA A, ROTERS F, RAABE D. A dislocation density based constitutive model for crystal plasticity FEM including geometrically necessary dislocations [J]. Acta Materialia, 2006, 54(8): 2169–2179. doi: 10.1016/j.actamat.2006.01.005 [82] MA A, ROTERS F. A constitutive model for fcc single crystals based on dislocation densities and its application to uniaxial compression of aluminium single crystals [J]. Acta Materialia, 2004, 52(12): 3603–3612. doi: 10.1016/j.actamat.2004.04.012 [83] LLOYD J T, CLAYTON J D, AUSTIN R A, et al. Plane wave simulation of elastic-viscoplastic single crystals [J]. Journal of the Mechanics and Physics of Solids, 2014, 69: 14–32. doi: 10.1016/j.jmps.2014.04.009 [84] ALANKAR A, FIELD D P, ZBIB H M. Explicit incorporation of cross-slip in a dislocation density-based crystal plasticity model [J]. Philosophical Magazine, 2012, 92(24): 3084–3100. doi: 10.1080/14786435.2012.685964 [85] KESHAVARZ S, GHOSH S. Multi-scale crystal plasticity finite element model approach to modeling nickel-based superalloys [J]. Acta Materialia, 2013, 61(17): 6549–6561. doi: 10.1016/j.actamat.2013.07.038 [86] LIANG H, DUNNE F P E. GND accumulation in bi-crystal deformation: crystal plasticity analysis and comparison with experiments [J]. International Journal of Mechanical Sciences, 2009, 51(4): 326–333. doi: 10.1016/j.ijmecsci.2009.03.005 [87] GÜVENÇ O, BAMBACH M, HIRT G. Coupling of crystal plasticity finite element and phase field methods for the prediction of SRX kinetics after hot working [J]. Steel Research International, 2014, 85(6): 999–1009. doi: 10.1002/srin.201300191 [88] KONDO R, TADANO Y, SHIZAWA K. A phase-field model of twinning and detwinning coupled with dislocation-based crystal plasticity for HCP metals [J]. Computational Materials Science, 2014, 95: 672–683. doi: 10.1016/j.commatsci.2014.08.034 [89] CHEN L, CHEN J, LEBENSOHN R A, et al. An integrated fast Fourier transform-based phase-field and crystal plasticity approach to model recrystallization of three dimensional polycrystals [J]. Computer Methods in Applied Mechanics and Engineering, 2015, 285: 829–848. doi: 10.1016/j.cma.2014.12.007 [90] COTTURA M, APPOLAIRE B, FINEL A, et al. Coupling the phase field method for diffusive transformations with dislocation density-based crystal plasticity: application to Ni-based superalloys [J]. Journal of the Mechanics and Physics of Solids, 2016, 94: 473–489. doi: 10.1016/j.jmps.2016.05.016 [91] PARANJAPE H M, MANCHIRAJU S, ANDERSON P M. A phase field: finite element approach to model the interaction between phase transformations and plasticity in shape memory alloys [J]. International Journal of Plasticity, 2016, 80: 1–18. doi: 10.1016/j.ijplas.2015.12.007 [92] IDESMAN A V, LEVITAS V I, STEIN E. Elastoplastic materials with martensitic phase transition and twinning at finite strains: numerical solution with the finite element method [J]. Computer Methods in Applied Mechanics and Engineering, 1999, 173(1/2): 71–98. [93] HUANG M, BRINSON L C. A Multivariant model for single crystal shape memory alloy behavior [J]. Journal of the Mechanics and Physics of Solids, 1998, 46(8): 1379–1409. doi: 10.1016/S0022-5096(97)00080-X [94] BHATTACHARYYA A, WENG G J. An energy criterion for the stress-induced martensitic transformation in a ductile system [J]. Journal of the Mechanics and Physics of Solids, 1994, 42(11): 1699–1724. doi: 10.1016/0022-5096(94)90068-X [95] STRINGFELLOW R G, PARKS D M, OLSON G B. A constitutive model for transformation plasticity accompanying strain-induced martensitic transformations in metastable austenitic steels [J]. Acta Metallurgica et Materialia, 1992, 40(7): 1703–1716. doi: 10.1016/0956-7151(92)90114-T [96] LEBLOND J B, MOTTET G, DEVAUX J C. A theoretical and numerical approach to the plastic behaviour of steels during phase transformations: Ⅰ. derivation of general relations [J]. Journal of the Mechanics and Physics of Solids, 1986, 34(4): 395–409. doi: 10.1016/0022-5096(86)90009-8 [97] THAMBURAJA P, ANAND L. Polycrystalline shape-memory materials: effect of crystallographic texture [J]. Journal of the Mechanics and Physics of Solids, 2001, 49(4): 709–737. doi: 10.1016/S0022-5096(00)00061-2 [98] MA A X, HARTMAIER A. A study of deformation and phase transformation coupling for TRIP-assisted steels [J]. International Journal of Plasticity, 2015, 64: 40–55. doi: 10.1016/j.ijplas.2014.07.008 [99] SUN C Y, GUO N, FU M W, et al. Modeling of slip, twinning and transformation induced plastic deformation for TWIP steel based on crystal plasticity [J]. International Journal of Plasticity, 2016, 76: 186–212. doi: 10.1016/j.ijplas.2015.08.003 [100] LEE M G, KIM S J, HAN H N. Crystal plasticity finite element modeling of mechanically induced martensitic transformation (MIMT) in metastable austenite [J]. International Journal of Plasticity, 2010, 26(5): 688–710. doi: 10.1016/j.ijplas.2009.10.001 [101] TURTELTAUB S, SUIKER A S J. Transformation-induced plasticity in ferrous alloys [J]. Journal of the Mechanics and Physics of Solids, 2005, 53(8): 1747–1788. doi: 10.1016/j.jmps.2005.03.004 [102] TURTELTAUB S, SUIKER A S J. A multiscale thermomechanical model for cubic to tetragonal martensitic phase transformations [J]. International Journal of Solids and Structures, 2006, 43(14/15): 4509–4545. [103] SUIKER A S J, TURTELTAUB S. Computational modelling of plasticity induced by martensitic phase transformations [J]. International Journal for Numerical Methods in Engineering, 2005, 63(12): 1655–1693. doi: 10.1002/nme.1327 [104] MANCHIRAJU S, ANDERSON P M. Coupling between martensitic phase transformations and plasticity: a microstructure-based finite element model [J]. International Journal of Plasticity, 2010, 26(10): 1508–1526. doi: 10.1016/j.ijplas.2010.01.009 [105] FENG B, BRONKHORST C A, ADDESSIO F L, et al. Coupled elasticity, plastic slip, and twinning in single crystal titanium loaded by split-Hopkinson pressure bar [J]. Journal of the Mechanics and Physics of Solids, 2018, 119: 274–297. doi: 10.1016/j.jmps.2018.06.018 [106] GREEFF C W. Alpha-omega transition in Ti: equation of state and kinetics [C]//AIP Conference Proceedings. Atlanta, Georgia (USA). AIP, 2002: 225–228. [107] TJAHJANTO D D, TURTELTAUB S, SUIKER A S J. Crystallographically based model for transformation-induced plasticity in multiphase carbon steels [J]. Continuum Mechanics and Thermodynamics, 2008, 19(7): 399–422. doi: 10.1007/s00161-007-0061-x [108] THAMBURAJA P. A finite-deformation-based phenomenological theory for shape-memory alloys [J]. International Journal of Plasticity, 2010, 26(8): 1195–1219. doi: 10.1016/j.ijplas.2009.12.004 [109] THAMBURAJA P, PAN H, CHAU F S. Martensitic reorientation and shape-memory effect in initially textured polycrystalline Ti-Ni sheet [J]. Acta Materialia, 2005, 53(14): 3821–3831. doi: 10.1016/j.actamat.2005.03.054 [110] THAMBURAJA P. Constitutive equations for martensitic reorientation and detwinning in shape-memory alloys [J]. Journal of the Mechanics and Physics of Solids, 2005, 53(4): 825–856. doi: 10.1016/j.jmps.2004.11.004 [111] BARTON N R, BENSON D J, BECKER R. Crystal level continuum modelling of phase transformations: the α↔ε transformation in iron [J]. Modelling and Simulation in Materials Science and Engineering, 2005, 13(5): 707–731. doi: 10.1088/0965-0393/13/5/006 [112] AMOUZOU K E K, RICHETON T, ROTH A, et al. Micromechanical modeling of hardening mechanisms in commercially pure α-titanium in tensile condition [J]. International Journal of Plasticity, 2016, 80: 222–240. doi: 10.1016/j.ijplas.2015.09.008 [113] GURAO N P, KAPOOR R, SUWAS S. Deformation behaviour of commercially pure titanium at extreme strain rates [J]. Acta Materialia, 2011, 59(9): 3431–3446. doi: 10.1016/j.actamat.2011.02.018 [114] MEREDITH C S, LLOYD J T, SANO T. The quasi-static and dynamic response of fine-grained Mg alloy AMX602: an experimental and computational study [J]. Materials Science and Engineering A, 2016, 673: 73–82. doi: 10.1016/j.msea.2016.07.035 [115] ROHATGI A, VECCHIO K S. The variation of dislocation density as a function of the stacking fault energy in shock-deformed FCC materials [J]. Materials Science and Engineering A, 2002, 328(1/2): 256–266. [116] KALIDINDI S R. Incorporation of deformation twinning in crystal plasticity models [J]. Journal of the Mechanics and Physics of Solids, 1998, 46(2): 267–290. doi: 10.1016/S0022-5096(97)00051-3 [117] CLAYTON J D. Nonlinear elastic and inelastic models for shock compression of crystalline solids [M]. Cham: Springer International Publishing, 2019. [118] CLAYTON J. A continuum description of nonlinear elasticity, slip and twinning, with application to sapphire [J]. Proceedings of the Royal Society A: Mathematical, Physical and Engineering Sciences, 2009, 465(2101): 307–334. doi: 10.1098/rspa.2008.0281 [119] SALEM A A, KALIDINDI S R, DOHERTY R D, et al. Strain hardening due to deformation twinning in α-titanium: mechanisms [J]. Metallurgical and Materials Transactions A, 2006, 37(1): 259–268. doi: 10.1007/s11661-006-0171-2 [120] SALEM A A, KALIDINDI S R, SEMIATIN S L. Strain hardening due to deformation twinning in α-titanium: constitutive relations and crystal-plasticity modeling [J]. Acta Materialia, 2005, 53(12): 3495–3502. doi: 10.1016/j.actamat.2005.04.014 [121] KALIDINDI S R. A crystal plasticity framework for deformation twinning [M]//Continuum Scale Simulation of Engineering Materials. Weinheim, FRG: Wiley-VCH Verlag GmbH & Co. KGaA, 2005: 543–560. [122] ARDELJAN M, BEYERLEIN I J, MCWILLIAMS B A, et al. Strain rate and temperature sensitive multi-level crystal plasticity model for large plastic deformation behavior: application to AZ31 magnesium alloy [J]. International Journal of Plasticity, 2016, 83: 90–109. doi: 10.1016/j.ijplas.2016.04.005 [123] ARDELJAN M, MCCABE R J, BEYERLEIN I J, et al. Explicit incorporation of deformation twins into crystal plasticity finite element models [J]. Computer Methods in Applied Mechanics and Engineering, 2015, 295: 396–413. doi: 10.1016/j.cma.2015.07.003 [124] BEYERLEIN I J, TOMÉ C N. A dislocation-based constitutive law for pure Zr including temperature effects [J]. International Journal of Plasticity, 2008, 24(5): 867–895. doi: 10.1016/j.ijplas.2007.07.017 [125] MADEC R, DEVINCRE B, KUBIN L P. From dislocation junctions to forest hardening [J]. Physical Review Letters, 2002, 89(25): 255508. doi: 10.1103/PhysRevLett.89.255508 [126] SONG S G, GRAY G T III. Structural interpretation of the nucleation and growth of deformation twins in Zr and Ti: Ⅰ. application of the coincidence site lattice (CSL) theory to twinning problems in hcp structures [J]. Acta Metallurgica et Materialia, 1995, 43(6): 2325–2337. doi: 10.1016/0956-7151(94)00433-1 [127] SONG S G, GRAY G T III. Structural interpretation of the nucleation and growth of deformation twins in Zr and Ti: Ⅱ. TEM study of twin morphology and defect reactions during twinning [J]. Acta Metallurgica et Materialia, 1995, 43(6): 2339–2350. doi: 10.1016/0956-7151(94)00434-X [128] SONG S G, GRAY G T. Influence of temperature and strain rate on slip and twinning behavior of Zr [J]. Metallurgical and Materials Transactions A, 1995, 26(10): 2665–2675. doi: 10.1007/BF02669423 [129] 朱兆祥, 李永池, 王肖钧. 爆炸作用下钢板层裂的数值分析 [J]. 应用数学和力学, 1981, 2(4): 353–368.ZHU Z X, LI Y C, WANG X J. Numerical analysis of the spallation of steel target under the explosive loading [J]. Applied Mathematics and Mechanics, 1981, 2(4): 353–368. [130] RINEHART J S. Some quantitative data bearing on the scabbing of metals under explosive attack [J]. Journal of Applied Physics, 1951, 22(5): 555–560. doi: 10.1063/1.1700005 [131] ZHANG K S, ZHANG D, FENG R, et al. Microdamage in polycrystalline ceramics under dynamic compression and tension [J]. Journal of Applied Physics, 2005, 98(2): 023505. doi: 10.1063/1.1944908 [132] LLOYD J T, MATEJUNAS A J, BECKER R, et al. Dynamic tensile failure of rolled magnesium: simulations and experiments quantifying the role of texture and second-phase particles [J]. International Journal of Plasticity, 2019, 114: 174–195. doi: 10.1016/j.ijplas.2018.11.002 [133] LING C, BESSON J, FOREST S, et al. An elastoviscoplastic model for porous single crystals at finite strains and its assessment based on unit cell simulations [J]. International Journal of Plasticity, 2016, 84: 58–87. doi: 10.1016/j.ijplas.2016.05.001 [134] NGUYEN T, LUSCHER D J, WILKERSON J W. A dislocation-based crystal plasticity framework for dynamic ductile failure of single crystals [J]. Journal of the Mechanics and Physics of Solids, 2017, 108: 1–29. doi: 10.1016/j.jmps.2017.07.020 [135] NGUYEN T, LUSCHER D J, WILKERSON J W. The role of elastic and plastic anisotropy in intergranular spall failure [J]. Acta Materialia, 2019, 168: 1–12. doi: 10.1016/j.actamat.2019.01.033 [136] BAI Y L, DODD B. Adiabatic shear localization: occurrence, theories, and applications [M]. Oxford: Pergamon Press, 1992. [137] DODD B, BAI Y L. Adiabatic shear localization: frontiers and advances [M]. Elsevier, 2012. [138] ZENER C, HOLLOMON J H. Effect of strain rate upon plastic flow of steel [J]. Journal of Applied Physics, 1944, 15(1): 22–32. doi: 10.1063/1.1707363 [139] HINES J A, VECCHIO K S, AHZI S. A model for microstructure evolution in adiabatic shear bands [J]. Metallurgical and Materials Transactions A, 1998, 29(1): 191–203. doi: 10.1007/s11661-998-0172-4 [140] LEE W B, WANG H, CHAN C Y, et al. Finite element modelling of shear angle and cutting force variation induced by material anisotropy in ultra-precision diamond turning [J]. International Journal of Machine Tools and Manufacture, 2013, 75: 82–86. doi: 10.1016/j.ijmachtools.2013.09.007 [141] BRONKHORST C A, HANSEN B L, CERRETA E K, et al. Modeling the microstructural evolution of metallic polycrystalline materials under localization conditions [J]. Journal of the Mechanics and Physics of Solids, 2007, 55(11): 2351–2383. doi: 10.1016/j.jmps.2007.03.019 [142] WRIGHT T W. 绝热剪切带的数理分析 [M]. 李云凯, 孙川, 王云飞, 译. 北京: 北京理工大学出版社, 2013.WRIGHT T W. The physics and mathematics of adiabatic shear bands [M]. Translated by LI Y K, SUN C, WANG Y F. Beijing: Beijing Institute of Technology Press, 2003. [143] BARGMANN S, EKH M. Microscopic temperature field prediction during adiabatic loading using gradient extended crystal plasticity [J]. International Journal of Solids and Structures, 2013, 50(6): 899–906. doi: 10.1016/j.ijsolstr.2012.11.010 [144] CULVER R S. Thermal instability strain in dynamic plastic deformation [M]//Metallurgical Effects at High Strain Rates. Boston: Springer, 1973: 519–530. [145] BAI Y L. A criterion for thermo-plastic shear instability [M]//Shock Waves and High-Strain-Rate Phenomena in Metals. Boston: Springer, 1981: 277–284. [146] SCHOENFELD S E, WRIGHT T W. A failure criterion based on material instability [J]. International Journal of Solids and Structures, 2003, 40(12): 3021–3037. doi: 10.1016/S0020-7683(03)00059-3 [147] ZHANG Z, EAKINS D E, DUNNE F P E. On the formation of adiabatic shear bands in textured HCP polycrystals [J]. International Journal of Plasticity, 2016, 79: 196–216. doi: 10.1016/j.ijplas.2015.12.004 [148] RECHT R F. Catastrophic thermoplastic shear [J]. Journal of Applied Mechanics, 1964, 31(2): 189–193. doi: 10.1115/1.3629585 [149] DUSZEK-PERZYNA M K, PERZYNA P. Analysis of the influence of non-schmid and thermal effects on adiabatic shear band localization in elastic-plastic single crystals [M]//Finite Inelastic Deformations: Theory and Applications. Berlin, Heidelberg: Springer Berlin Heidelberg, 1992: 155–165. [150] DUSZEK-PERZYNA M K, PERZYNA P. Adiabatic shear band localization in elastic-plastic single crystals [J]. International Journal of Solids and Structures, 1993, 30(1): 61–89. doi: 10.1016/0020-7683(93)90132-Q [151] DUSZEK-PERZYNA M K, PERZYNA P. Adiabatic shear band localization of inelastic single crystals in symmetric double-slip process [J]. Archive of Applied Mechanics, 1996, 66(6): 369. doi: 10.1007/s004190050076 [152] RITTEL D, WANG Z G, MERZER M. Adiabatic shear failure and dynamic stored energy of cold work [J]. Physical Review Letters, 2006, 96(7): 075502. doi: 10.1103/PhysRevLett.96.075502 [153] BOUBAKER H B, MAREAU C, AYED Y, et al. Development of a hyperelastic constitutive model based on the crystal plasticity theory for the simulation of machining operations [J]. Procedia CIRP, 2019, 82: 20–25. doi: 10.1016/j.procir.2019.04.336 -







 下载:
下载:
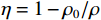
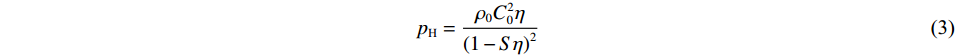
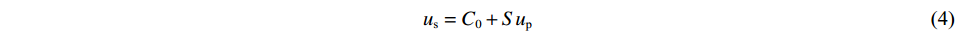
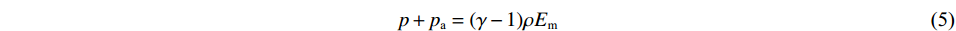
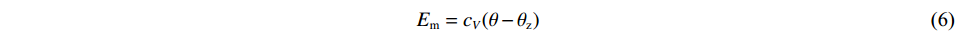










 下载:
下载: