具有梯度型刚度折叠收缩管的吸能性能

陈伟东; 门恒; 田晓耕

doi:10.11858/gywlxb.20190873

具有梯度型刚度折叠收缩管的吸能性能

doi: 10.11858/gywlxb.20190873

西安交通大学机械结构强度与振动国家重点实验室，陕西西安　710049

基金项目: 国家自然科学基金（11732007）

详细信息

作者简介:
陈伟东（1996－），男，硕士研究生，主要从事折叠结构的吸能特性研究. E-mail：chen_weidong04@163.com

通讯作者:
田晓耕（1967－），男，博士，教授，主要从事超轻结构吸能及优化、非经典热弹理论研究. E-mail：tiansu@mail.xjtu.edu.cn

中图分类号: O347; TB124
计量
- 文章访问数: 4793
- HTML全文浏览量: 1958
- PDF下载量: 35
出版历程
- 收稿日期: 2019-12-26
- 修回日期: 2020-01-20

Energy Absorption of Folded Shrink Tubes with Gradient Stiffness

State Key Laboratory for Strength and Vibration of Mechanical Structures, Xi’an Jiaotong University, Xi’an 710049, Shaanxi, China

摘要

摘要: 薄壁管是一种常见的吸能结构，在薄壁管中引入折纹可以诱导薄壁管发生变形，有效降低薄壁管屈曲的初始峰值力并提高其能量吸收。目前，大部分折纹管受到轴向压缩时，初始峰值力后的压溃力下降显著，降低了折纹管的吸能性能。为有效提高折纹管的吸能性能，并降低折纹管的初始峰值力，在方管中引入不同形式的折纹得到折叠收缩管，利用ABAQUS/Explicit模拟设计折叠收缩管的准静态压缩，得到其变形及力与位移曲线。结果显示，与传统方管和菱形折纹管相比，折叠收缩管的初始峰值力显著降低，且压溃力随着压缩距离的增加呈梯度上升趋势，大幅提升了薄壁管的能量吸收性能。另外，系统研究了折叠收缩管的几何参数对其性能的影响，获得了性能优异的折纹管。
- 折纹管 /
- 能量吸收 /
- 准静态压缩 /
- 有限元 /
- 梯度型刚度
Abstract: Thin-walled tube is a common energy-absorbing structure. The introduction of folds in thin-walled tube can induce the deformation of thin-walled tube, reduce the initial peak force of buckling of thin-walled tube and improve the energy absorption of thin-walled tube effectively. At present, when the folded tubes subjects to axial compression, the crushing force decreases significantly after the initial peak force, which lowers the energy absorption performance of folded tubes. In order to further reduce the initial peak force and increase the total energy absorbed of the folded tube, different forms of folded tube are introduced into the square tube to obtain a folded shrink tube. The relation between force and displacement and deformation of the designed folded tube under the quasi-static compression is obtained by using ABAQUS/Explicit. The results show that the collapse force of the folded shrink tube is in the form of a gradient during the compression process. Compared with traditional square tube and diamond tube, the folded shrink tube not only has lower initial peak force, but also can greatly improve the total energy absorption. The influence of geometric parameters on the performance of the folded tube was studied systematically. The best performance folded shrink tubes were obtained.
- folded tube /
- energy absorption /
- quasi-static compression /
- finite element /
- gradient stiffness

HTML全文

1. 引言

高静压(High Hydrostatic Pressure, HHP)技术是指在室温或温和条件下利用0.1~2 GPa的压力处理, 以达到杀菌或加工食物原料的目的。HHP处理使食品自身体积减小, 即在生物体高分子的立体结构中发生氢键结合、离子结合和疏水结合, 其结果不仅会影响生物细胞形态, 还能改变生物高分子立体结构, 引起蛋白质、酶等失活, 从而成为非热杀菌的手段之一^[1]; HHP对维生素、色素和风味物质等低分子化合物的共价键无明显影响, 故可用于改善食品组织结构或生成新型食品。HHP技术能够在不加热或不添加化学防腐剂的条件下, 保证食品安全, 并显著延长食品的冷藏期和货架期, 所得加工产品的质量是传统加工产品无法媲美的, 为生产高附加值的产品提供了很好的技术手段^[2]。

早在1899年, Hite将原奶在室温下用600 MPa高压处理1 h, 使其贮藏期延长了4 d^[3]。Bridgman因发现静水压下蛋白质的变性、凝固现象, 而获得了1946年的诺贝尔物理学奖。但直到1990年, HHP技术相关理论和装备的研究才有所突破与发展。日本首先实现了HHP技术在果汁、沙拉酱、果酱、海鲜、果冻等食品加工领域的商业化应用^[4]。2000年, 日本越后制果株式会社成功推出了采用HHP技术加工的方便米饭, 不仅方便、口感好, 而且充分地保留了米饭原有的营养成分, 成为目前日本最热销的超高压食品。加工海鲜类产品时, HHP技术不仅便于脱壳, 还能改善产品品质并延长货架期。目前, HHP加工的果蔬制品和肉制品在一些发达国家已经达到商业化生产的水平, 如鳄梨、草莓、苹果、猕猴桃等酱类制品^[5]。据不完全统计, 目前全球采用HHP技术加工的食品超过13万吨(数据来自NC Hyperbaric, 不包括亚洲)。

自1982年第一篇关于超高压处理淀粉的论文发表以来, 至今已有20多种淀粉被HHP处理过, 包括:A型的小麦、大麦、燕麦、黑麦、玉米、黏玉米、稻米、黏稻米、花生、苋菜红、高粱淀粉, B型的马铃薯、木薯淀粉等, C型的美人蕉、豌豆、木薯、小扁豆、绿豆、莲、巴巴苏仁、板栗淀粉等^[6-7]。

除了处理压力外, 另一个影响HHP淀粉结构的主要因素是水分含量。淀粉分子拥有众多羟基, 亲水性很强, 羟基之间通过氢键结合, 淀粉颗粒中的水分子也参与氢键的结合^[8]。淀粉颗粒具有相当高的渗透性, 水和水溶液能自由进入淀粉颗粒内部。在HHP处理下, 淀粉颗粒受压缩, 因此颗粒结晶结构的变化过程成为颗粒吸水膨胀和高压抑制膨胀两个相反作用力的博弈, 反映在淀粉颗粒结构上也有不同的表现。Vainionpaa等人^[9-10]研究发现, 在有限的水分范围内, 压力处理加速破坏淀粉颗粒中的有序结构, 有利于淀粉的融解, 或增强淀粉的可塑性^[11]。

对于淀粉颗粒中水分子的存在方式, 有两种不同观点:一种认为水分子参与结晶, 依据是淀粉在逐步加热、干燥、脱水过程中, 由于晶体结构中水分子的脱除, 颗粒由结晶结构转化为无定形结构^[8]; 另一种认为水分子并不参与结晶, 该观点由法国科学家Kainuma提出, 他把浸透玉米糖浆的淀粉糊精干燥至完全脱水状态, 发现淀粉仍然保持结晶结构^[12]。Perry等人^[13]应用小角中子散射方法, 研究了不同种类淀粉颗粒内部水分的分布, 实验结果显示, 无定形区内的水分含量远大于结晶区, 因此无定形区具有更开放的渗透性结构。本研究将从水分含量的角度出发, 研究高静压过程中不同类型淀粉的微观结构。

2. 实验材料与方法

2.1 实验材料处理

糯玉米淀粉(A型, 含微量直链淀粉)从国民淀粉上海分公司购得, 初始水分含量为12.77%, 蛋白质含量为0.48%, 灰分含量为0.51%;木薯淀粉(C型, 含17%直链淀粉)由广西武鸣安宁淀粉有限公司友情赞助, 水分含量为6.74%, 蛋白质含量为0.62%, 灰分含量为0.68%。用淀粉和蒸馏水配制成粉水比(淀粉质量和水质量之比)为1/10、3/10、5/10、7/10及1的溶液, 室温条件下搅拌均匀后, 装入聚乙烯袋中, 进行真空封口。将密封好的样品放入高静压处理釜中进行高压处理, 压力水平为600 MPa, 保压时间为30 min。高静压设备(HHP-750)由包头科发高压科技有限公司与中国农业大学国家果蔬工程中心联合研制, 传压介质为蒸馏水, 最大工作压力是600 MPa。让处理后的样品迅速在室温条件下进行冷冻干燥, 粉碎, 在4 ℃下保藏。

2.2 实验方法

2.2.1 光学显微镜观察

采用上海光学仪器厂生产的光学显微镜(59XA), 放大倍数为400(40×10)倍。按以下步骤操作:将样品配制成1%的淀粉乳, 取1滴放在载玻片上, 盖上盖玻片后置于光学显微镜下观察、拍照; 分别记录淀粉在正常光源及偏振光源下的形貌特征。

2.2.2 扫描电子显微镜(SEM)观察

采用日立公司的扫描电子显微镜(HITACHI S-3400N, Japan)。将双面胶带贴于扫描电子显微镜的载物台上, 用牙签粘取少量样品, 轻轻地在双面胶上涂抹均匀, 用吸耳球吹去多余的淀粉样品, 放入表面处理机中, 用离子溅射镀膜仪喷碳镀金2 h取出; 将载物台放入扫描电子显微镜中观察, 拍摄具有代表性的淀粉颗粒形貌。电子枪加速电压设为2 kV, 以减少对淀粉颗粒的破坏。

2.2.3 粒度分布分析

采用美国贝克曼库尔特有限公司的激光粒度分析仪(LS230)。将0.1 g淀粉溶于5 mL无水乙醇, 振荡10 min使淀粉颗粒分散均匀, 并用无水乙醇作分散剂, 按照仪器提示加入所需量的样品, 进行分散。在1 800 r/min转速下, 测定淀粉颗粒的微分粒度分布和颗粒分布的平均直径, 每件样品测3次。

2.2.4 X射线衍射分析

利用日本岛津公司的XRD-6000型X射线衍射仪进行淀粉晶体的X射线衍射分析。分析条件:特征射线CuK_α, 石墨单色器, 管压40 kV, 电流30 mA, 测量角度范围为2θ=4°~40°, 发射及防反射狭缝为1°, 接收狭缝宽0.3 mm, 扫描速度为1.5°/min, 步宽0.05°。为了避免不同样品中相对水分含量的差异影响相对结晶强度, 测量前应先将淀粉样品在室温下置于相对湿度100%的干燥皿中平衡48 h。

2.2.5 低场核磁共振分析

采用纽迈公司的核磁共振成像仪(PQ001), 磁场强度0.5 T, 线圈直径15 mm, 频率21.961 MHz, 温度31.99~32.00 ℃。实验前, 将淀粉样品烘干, 粉碎, 过200目筛后, 装入15 mm玻璃试管即可测试。

2.2.6 数据处理及分析

实验数据采用SPSS 16.0 for Windows统计分析软件和Microsoft Excel 2003数据处理系统进行统计处理, 计算标准偏差(±SE)或进行差异显著性分析(显著性水平取0.05), 并利用Origin 7.5数据分析和制图软件做实验数据图。各实验均重复进行3次。

3. 实验结果与讨论

3.1 颗粒形貌

3.1.1 糯玉米淀粉

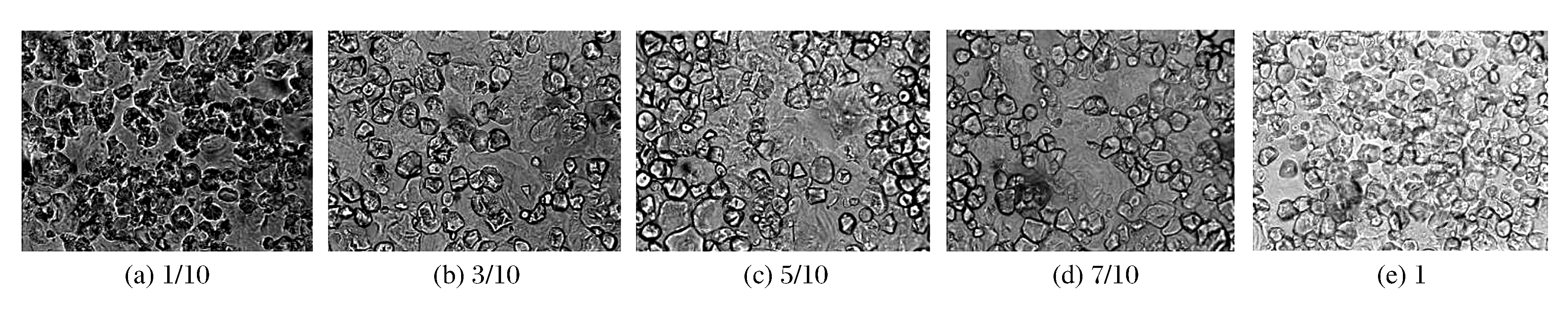
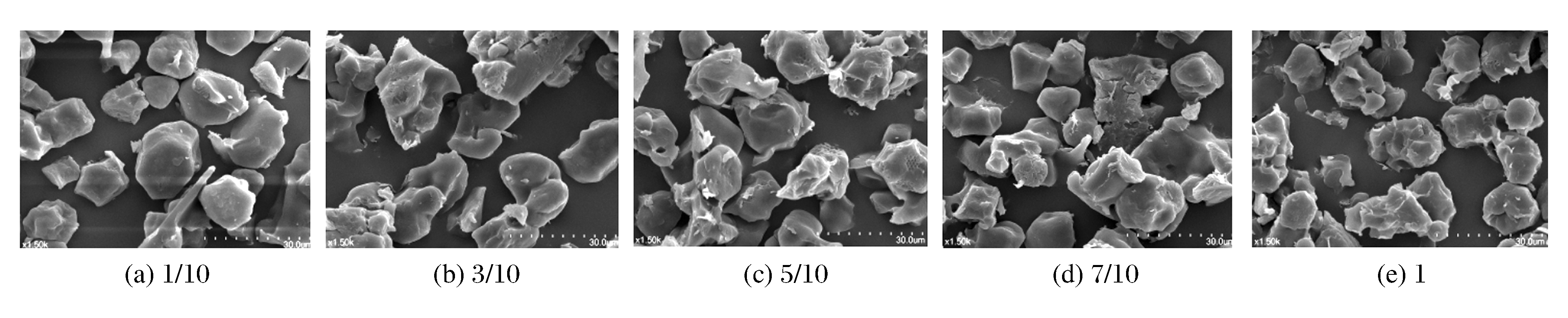
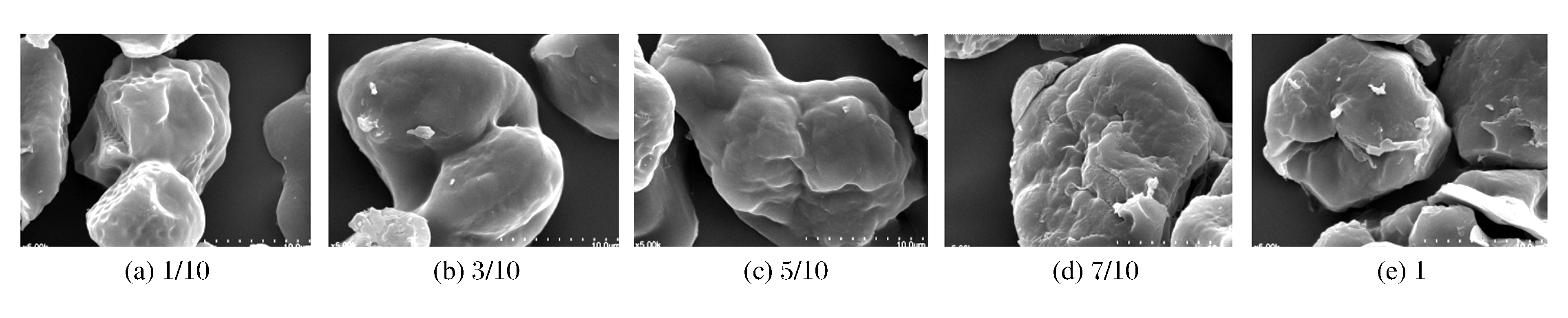
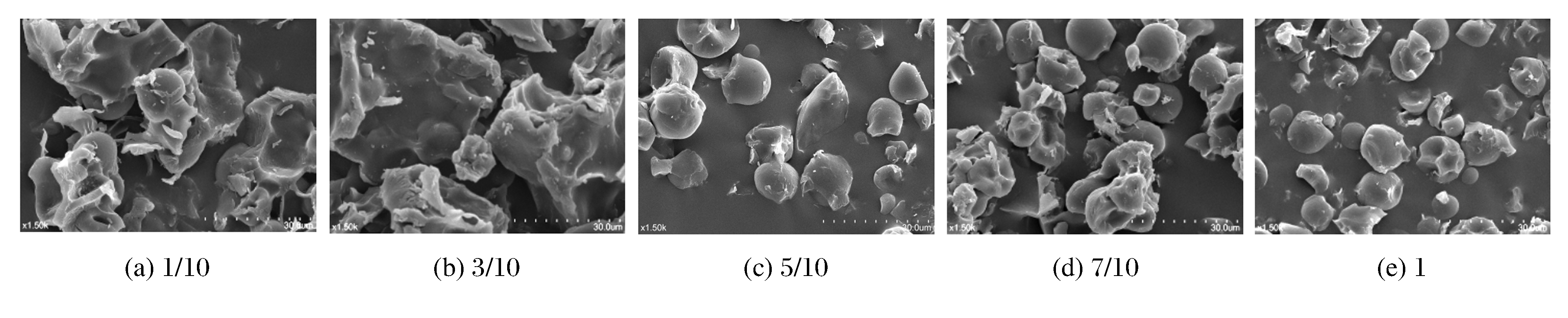
从图 1~图 4中可以看出, 当淀粉与水的比例不同时, 颗粒吸水膨胀的程度不同, 但颗粒之间都有不同程度的粘连。粉水比为1/10的样品水分含量高, 颗粒最光滑(见图 3(a)、图 4(a)), 原因是粉水比较低, 颗粒相互作用机会减少, 难以互相粘连变形。粉水比为7/10和1的样品颗粒水分含量少, 颗粒发生竞争性吸水, 表现出膨胀的不均匀性(见图 3(d)、图 3(e))。粉水比为3/10及5/10的样品颗粒已丧失自身形状, 发生变形(见图 1(b)、图 1(c)和图 2(b)、图 2(c)), 且出现很多碎片(见图 3(b)、图 3(c)), 淀粉颗粒充分膨胀, 粘连并聚合(见图 4(b)、图 4(c))。在600 MPa压力下, 糯玉米淀粉经历颗粒膨胀阶段^[14-15], 颗粒的结晶结构完全破坏; 但是从偏光显微照片发现, 存在偏光十字, 说明糯玉米淀粉在经历HHP吸水膨胀之后, 颗粒内部的支链淀粉分子立刻发生重排^[7], 表现出重结晶现象。

图 1 普通光学显微镜下不同粉水比的糯玉米淀粉(放大倍数：400，正常光源)

Figure 1. Light micrographs of waxy maize starch with various starch-water ratios treated under 600 MPa (Magnitude:400, bright light)

下载: 全尺寸图片幻灯片

图 2 普通光学显微镜下不同粉水比的糯玉米淀粉(放大倍数：400, 偏振光源)

Figure 2. Light micrographs of waxy maize starch with various starch-water ratios treated under 600 MPa (Magnitude:400, polarized light)

下载: 全尺寸图片幻灯片

图 3 扫描电子显微镜下不同粉水比的糯玉米淀粉(放大倍数：1 500)

Figure 3. Scanning electron micrographs of waxy maize starch with various starch-water ratios

下载: 全尺寸图片幻灯片

图 4 扫描电子显微镜下不同粉水比的糯玉米淀粉(放大倍数：5 000)

Figure 4. Scanning electron micrographs of waxy maize starch with various starch-water ratios treated under 600 MPa (Magnitude:5 000)

下载: 全尺寸图片幻灯片

高静压糊化的淀粉分子处于无序的高能态, 由于分子间势能的作用, 淀粉分子趋于有序重排, 结晶重新趋向平行排列, 形成具有结晶结构的白色凝沉物, 称为重结晶^[16]。Mile等人认为, 淀粉重结晶可分为两个阶段, 即短期重结晶和长期重结晶。短期重结晶主要是由于直链淀粉胶凝有序和结晶, 该过程可在糊化后较短时间内完成, 一般为几小时或十几小时。长期重结晶则主要由支链淀粉的外支链间有序堆积所引起, 是一个缓慢长期过程, 一般以天计^[16]。糯玉米淀粉几乎不含直链淀粉, 因此属于长期重结晶过程^[16]。

3.1.2 木薯淀粉

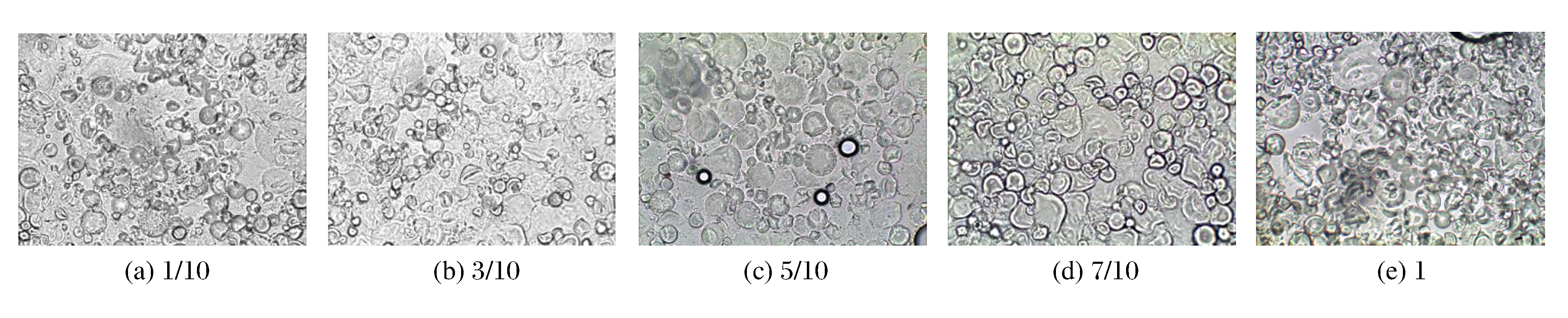
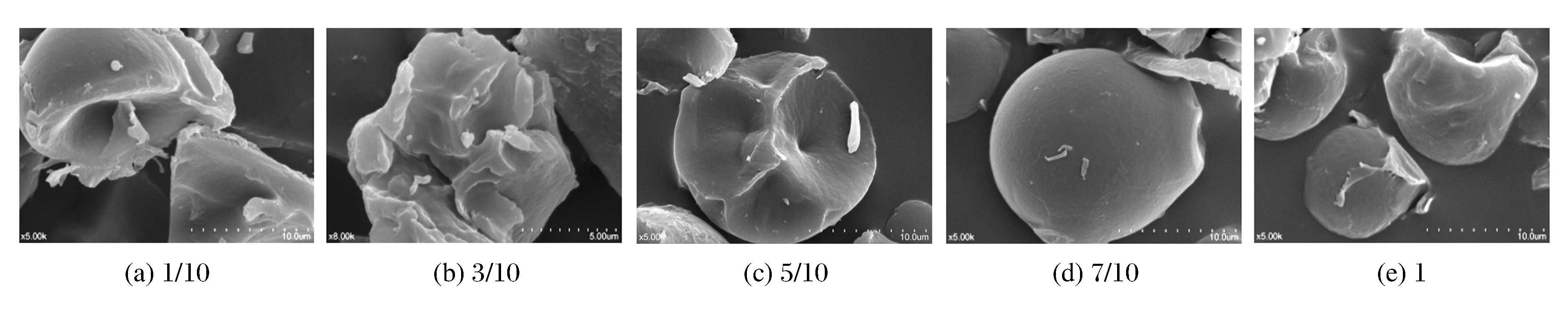
淀粉颗粒具有竞争占有水分的特点, 因此当水分含量发生变化时, 颗粒并不能全部在HHP的作用下发生吸水溶胀。如图 6所示, 木薯淀粉中粉水比为1/10、7/10及1的3组样品中, 偏光十字都未完全消失, 说明水分含量太高或太低都会影响淀粉的吸水膨胀及结晶结构变化。水分含量过高, 淀粉颗粒相互作用的机会减小, 颗粒膨胀程度较大(见图 7(a)), 但不能互相粘连(见图 8(a))。水分含量过低, 淀粉颗粒的膨胀受限, 表现为竞争性吸水, 颗粒膨胀不均匀, 大颗粒先吸水膨胀并失去结晶结构, 小颗粒不容易吸水仍保持完好的颗粒形态(见图 7(d)、图 7(e))。当粉水比为3/10或5/10时, 淀粉颗粒中的偏光十字几乎完全消失(见图 6(b)、图 6(c)), 而且颗粒整体膨胀适度, 存在一定程度的溶胀现象, 颗粒丧失自身的完整性(见图 7(b)、图 7(c)和图 8(b)、图 8(c))。

图 5 普通光学显微镜下不同粉水比的木薯淀粉(放大倍数：400，正常光源)

Figure 5. Light micrographs of tapioca starch with various starch-water ratios treated under 600 MPa (Magnitude:400, bright light)

下载: 全尺寸图片幻灯片

图 6 普通光学显微镜下不同粉水比的木薯淀粉(放大倍数：400, 偏振光源)

Figure 6. Light micrographs of tapioca starch with various starch-water ratios treated under 600 MPa (Magnitude:400, polarized light)

下载: 全尺寸图片幻灯片

图 7 扫描电子显微镜下不同粉水比的木薯淀粉(放大倍数：1 500)

Figure 7. Scanning electron micrographs of tapioca starch with various starch-water ratios treated under 600 MPa (Magnitude:1 500)

下载: 全尺寸图片幻灯片

图 8 扫描电子显微镜下不同粉水比的木薯淀粉(放大倍数：5 000)

Figure 8. Scanning electron micrographs of tapioca starch with various starch-water ratios treated under 600 MPa (Magnitude:5 000)

下载: 全尺寸图片幻灯片

3.2 粒度分布

在进行粒度分析时, 采用无水乙醇作为分散剂, 因为乙醇可以稳定淀粉的结晶结构^[17], 使高压处理后的淀粉颗粒较为稳定。而如果采用水作为分散剂, 对于结晶结构完全破坏或部分破坏的淀粉颗粒, 水作为塑化剂的作用更加明显, 颗粒在其作用下发生明显膨胀, 从而影响HHP处理后颗粒粒度分布的真实性。

随着粉水比的增大, 水分含量减少, 两种淀粉颗粒的平均粒径均逐渐减小。糯玉米淀粉颗粒的平均粒径从53.41±4.2 μm(对应粉水比1/10)下降到29.05±0.51 μm(对应粉水比1), 木薯淀粉颗粒的平均粒径从33.71±0.40 μm(对应粉水比1/10)下降到18.43±2.79 μm(对应粉水比1), 如表 1所示。颗粒粒径减小会降低微粒的沉降速率, 因此淀粉颗粒的粒径大小是考察体系稳定性的一个重要因素。粒径小的产品稳定性相对较高。综合考虑糊化和反应效率两个因素, 水分太高或太低都不利于结晶结构的破坏, 在粉水比为1/10、7/10及1的样品中, 结晶结构没有完全破坏, 而粉水比为3/10和5/10的样品能在HHP处理后发生适度膨胀, 且具有适当的颗粒粒度, 作为添加剂能够保证产品性能的稳定性, 不易发生沉淀或凝聚。

表 1 不同粉水比的糯玉米及木薯淀粉HHP处理后颗粒的粒径分布

Table 1. Parameters of particle size distribution for HHP modified waxy maize and tapioca starch with different starch-water ratios

Material	Starch-water ratio	Particle size distribution/(%)					Mean/(μm)	d_span	C_V/(%)	D_S/(%)
Material	Starch-water ratio	<10 μm	10~20 μm	20~40 μm	40~70 μm	>70 μm	Mean/(μm)	d_span	C_V/(%)	D_S/(%)
Waxy maize starch	1/10	8.71±0.70^ab	13.99±15.15^ab	26.60±1.27^b	21.95±0.64^ab	27.60±2.97^e	53.41±4.24^c	2.80±0.04^c	81.25±0.21^b	21.55±0.32^bc
	3/10	9.57±2.02^ab	14.83±4.20^a	28.05±7.00^ba	24.20±0.14^b	23.30±5.29^f	48.71±6.96^e	2.34±0.13^abc	75.15±0.92^ab	36.69±7.95^abc
	5/10	11.20±1.56^bcd	18.60±0.57^bc	31.75±1.06^c	24.00±2.26^ab	14.50±0.85^c	38.70±0.88^c	2.30±0.21^abc	74.30±5.94^ab	11.15±8.89^abc
	7/10	13.95±1.77^de	20.90±2.55^c	36.40±0.99^de	20.70±3.95^ab	8.08±0.65^b	32.85±0.80^ab	2.15±0.26^ab	78.15±9.55^ab	25.65±2.51^c
	1	14.95±0.92^e	21.95±1.91^c	38.10±0.85^e	21.45±2.76^ab	3.54±0.80^a	29.05±0.51^a	1.92±0.10^a	67.30±5.52^a	0.68±8.25^a
Tapioca starch	1/10	16.35±0.92^ab	19.20±0.00^ab	31.80±1.41^a	22.10±0.71^d	10.53±1.23^ab	33.71±0.40c	2.34±0.14^a	74.70±1.98^a	6.56±2.83^a
	3/10	16.85±1.20^ab	20.25±1.48^abc	31.25±0.07^a	20.80±1.70^cd	10.85±1.60^ab	33.46±1.48^b	2.46±0.10^a	77.55±3.04^a	10.63±4.33^a
	5/10	21.45±0.49^ab	26.20±1.84^bcd	30.70±2.40^a	16.85±1.34^bcd	4.61±3.32^a	26.55±2.93^ab	2.46±0.27^a	78.20±5.66^a	11.56±0.08^a
	7/10	25.50±0.85^b	32.20±0.71^de	25.90±0.42^a	12.00±0.28^abc	4.40±1.41^a	23.30±1.33^ab	2.77±0.04^a	88.30±4.95^a	25.97±7.06^a
	1	29.40±1.13^b	40.30±4.81^e	22.25±1.20^a	6.20±2.26^a	1.87±2.64^a	18.43±2.79^a	2.47±0.44^a	86.00±22.63	22.68±3.27^a
Note：(1) All values are mean values of triplicate determinations±standard deviations； (2) Values sharing the same uppercase letter within a line are not significantly different(P＜0.05); (3) Mean:De Brouckere diameter；d_span:Measurement of the width of size distribution, the narrower the distribution, the smaller the d_span is; C_V:Volumetric concentration; D_S:Swelling degree，D_S=(C_V-C_{V Native})/C_{V Native}.

下载: 导出CSV

| 显示表格

3.3 晶体结构

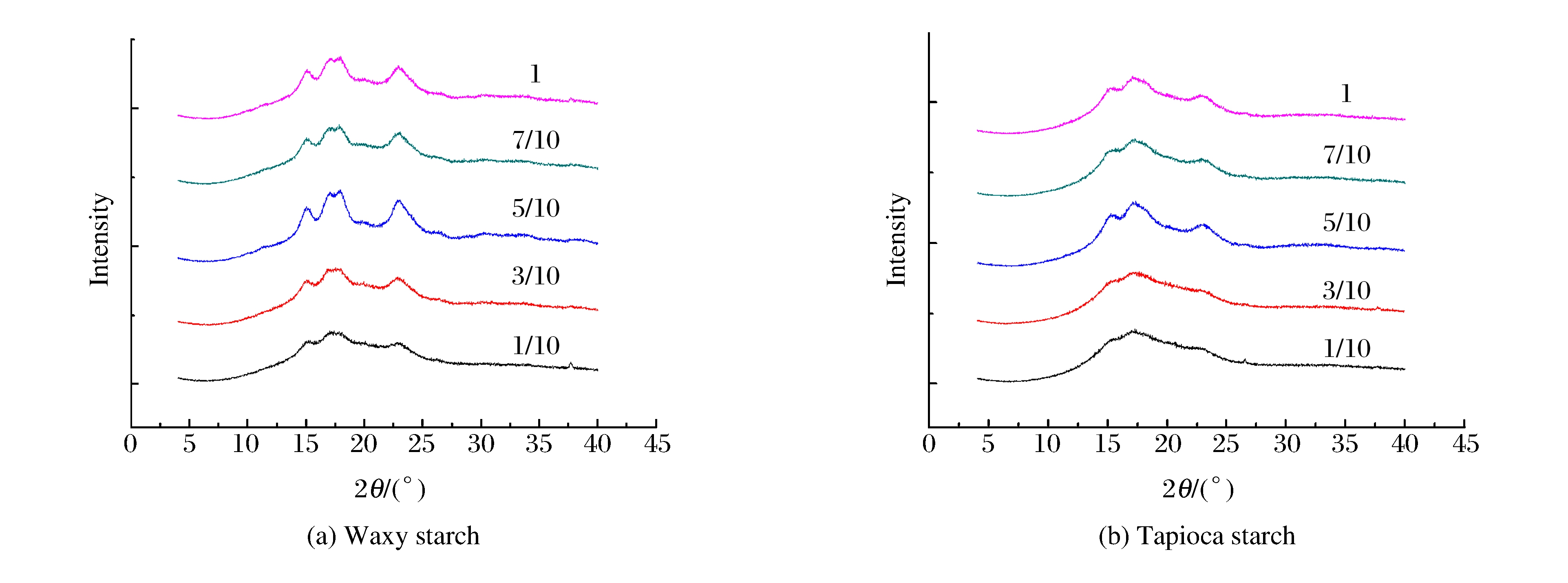
图 9(a)是不同粉水比的糯玉米淀粉样品在600 MPa高静压处理后的X射线衍射图谱。不同水分含量条件下, 糯玉米淀粉的晶型结构不变, 仍为A型, 但衍射峰强度明显不同。糯玉米淀粉经600 MPa高静压处理后, 结晶结构完全消失, 颗粒吸水膨胀, 崩解破裂后的淀粉颗粒迅速发生重结晶^[14-15]。水分含量对淀粉重结晶的影响非常显著(P < 0.05)。粉水比为1/10时, 样品的X射线衍射曲线仍为典型的非晶弥散峰(见图 9(a)), 相对结晶度为0(见表 2), 说明经600 MPa处理后, 该样品的重结晶程度很低, 因为过高的水分含量会抑制淀粉分子的交联缠绕和结晶重排^[18]。水分含量较高时, 虽然淀粉分子链迁移速率提高, 但由于淀粉浓度降低, 支链淀粉交联缠绕, 聚合为有序结构的机会减少。随着水分含量减少, 结晶度开始增加, X射线衍射强度增加。当粉水比为5/10时, 重结晶程度最高。当水分含量继续减少, 粉水比达到7/10甚至1时, X射线衍射强度减弱, 结晶度下降。这是由于水分含量较低时, 淀粉分子链迁移困难。因此, 水分含量过高和过低都会抑制淀粉分子的交联缠绕和结晶重排^[18]。

图 9 不同粉水比的糯玉米及木薯淀粉经600 MPa处理后的X射线衍射图谱

Figure 9. X-ray powder diffraction patterns of 600 MPa treated waxy maize and tapioca starch with different starch-water ratios

下载: 全尺寸图片幻灯片

表 2 不同粉水比的糯玉米和木薯淀粉在600 MPa HHP处理后的X射线衍射参数

Table 2. X-ray powder diffraction parameters for 600MPa HHP modified waxy maize and tapioca starch with different stach-water ratios

Material	Stach-water ratio	Half peak height				Peak area	Total area	Relative crystallinity/ (%)
Material	Stach-water ratio	15.0°	17.2°	17.8°	22.9°	Peak area	Total area	Relative crystallinity/ (%)
Waxy maize	1/10	2 293	2 996	3 232	2 307	0.33	20 106	0.00±0.00^a
	3/10	1 511	835	2 067	841	7 247	27 937	14.39±0.12^b
	5/10	1 735	2 182	2 042	2 351	340 735	990 797	34.39±0.08^d
	7/10	1 951	2 936	2 020	2 411	215 566	859 177	25.09±0.03^c
	1	2 039	2 429	2 017	2 406	274 046	1 045 979	26.20±0.04^d

Material	Starch-water ratio	Half peak height				Peak area	Total area	Relative crystallinity/ (%)
Material	Starch-water ratio	15.3°	17.2°		17.8°	Peak area	Total area	Relative crystallinity/ (%)
Tapioca starch	1/10	423.5	1 019		1 262	17.12	400 624	0.00±0.00^a
	3/10	847.5	1 817		775	115	877 436	0.01±0.15^a
	5/10	258	397		74	7 728	39 618	0.00±0.77^a
	7/10	1 992	2 373		1 837	270 048	580 312	40.54±0.08^b
	1	1 860	2 208		1 741	292 510	615 941	47.49±0.01^c
Note:The relative crystallinity values were triplicate determinations±standard deviations.

下载: 导出CSV

| 显示表格

木薯淀粉经600 MPa处理后, 发生晶体解体^[15]。由图 9(b)可见, 水分含量越高, 淀粉结晶度越低。当粉水比为1/10、3/10及5/10时, 相对结晶度都为0(见表 2), X射线衍射峰为弥散峰(见图 9(b)), 说明水分使微晶结构和微晶片层之间的无定型区域同时遭到破坏。而当淀粉颗粒中含水量较低(如粉水比为7/10或1)时, 结晶度比高压处理前的原淀粉结晶度还高(P < 0.05, 见表 2), 主要原因是当水分含量少时, 淀粉颗粒吸水膨胀受到两个因素限制, 一个是水分含量, 另一个是高静压力的作用。高静压力使一部分水进入淀粉颗粒内部, 被压入颗粒结晶区域, 从而导致结晶度增大。此外, 淀粉无定形区发生塑化作用, 导致颗粒中更多微晶结构的形成。

3.4 水分分布

当粉水比为3/10和5/10时, 糯玉米及木薯淀粉颗粒适度膨胀, 偏光十字完全消失, 性质稳定。此时, 糯玉米淀粉最容易发生重结晶, 木薯淀粉的结晶结构完全破坏。对该范围进行细分, 令粉水比分别为3/10、4/10和5/10, 分析经过HHP处理后, 各样品中水分的存在方式及数量, 并以此推测水分含量不同, 对糯玉米淀粉吸水膨胀后的重结晶过程, 及木薯淀粉结晶结构破坏过程的影响。

淀粉含有一定水分, 它以适当的数量, 定位、定向地存在于淀粉颗粒中。水分子的结合能力和流动性对淀粉的流变性质、稳定性、质构、外观以及对腐败的敏感性有很大影响。淀粉质原料和产品在加工、贮藏和运输等过程中, 内部的水分含量和分布状态经常会发生改变, 这也是导致原料稳定性下降、产品品质降低的主要原因之一。低场核磁共振广泛应用于生物体系中结合水的研究, 一方面因为它对被检测物无破坏性, 另一方面, 它可以测定能反映水分子流动性的氢核的纵向弛豫时间(原子核从激化状态回复到平衡排列状态的过程称为弛豫过程, 其所需时间为弛豫时间)t₁和横向弛豫时间t₂。当水和底物紧密结合时, 它高度固定, t₂较低; 而游离水流动性好, t₂较大。从而得知水分结合的方式及程度^[19]。

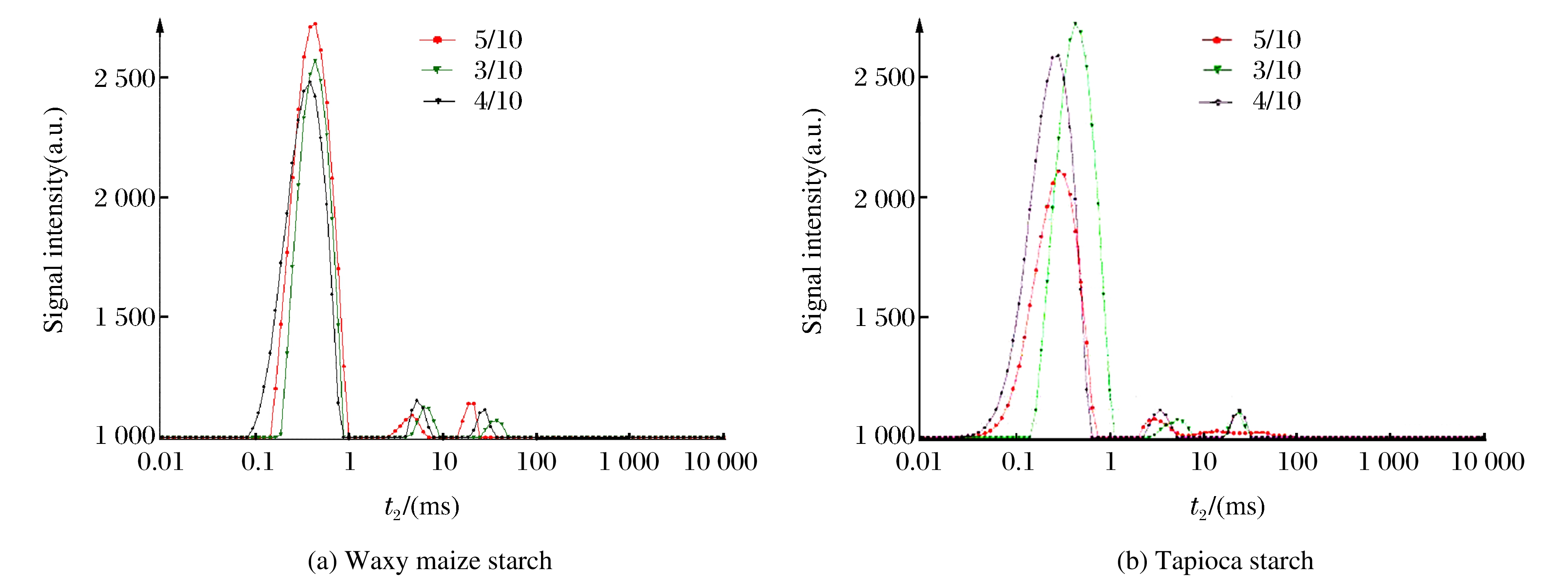
图 10是不同粉水比的糯玉米和木薯淀粉的低场核磁图谱, 横坐标表示横向弛豫时间t₂, 纵坐标表示信号强度; 出现的3个峰, 其对应的t₂从左向右分别记为t₂₁、t₂₂、t₂₃, 说明淀粉内部至少存在3种流动性不同的水。由图 10(a)可以看出, 糯玉米淀粉t₂的变化范围在0.1~100 ms之间。随着t₂的增加, 淀粉内部水分的结合力逐渐减弱, t₂₁∈[0.1, 1 ], 对应于淀粉中的活性较小的水, 流动性差, 为淀粉分子内部的结合水; t₂₂∈[1, 10], 大于t₂₁, 为不易流动的水; t₂₃∈[10, 100], 为易流动的水。t₂₁对应的信号强度远大于t₂₂和t₂₃, 因此HHP处理使淀粉中的水大都以结合态形式存在, 水分被牢牢地锁紧在淀粉颗粒中。粉水比为5/10的样品中, 结合水含量最高, 且各个信号峰值对应的t₂值最小, 说明HHP处理后, 5/10的糯玉米淀粉中水分结合得最牢固, 流动性低; 且水分参与重结晶, 形成晶体结构的一部分。

图 10 HHP处理不同粉水比的糯玉米和木薯淀粉的低场核磁共振图

Figure 10. Nuclear magnetic resonance patterns of HHP treated waxy maize and

下载: 全尺寸图片幻灯片

由图 10(b)可知, 木薯淀粉的t₂值范围为0.01~100 ms, 且各个峰所对应的t₂值比糯玉米淀粉的小, 说明HHP处理后木薯淀粉内的水分固定得更牢固。且对于粉水比5/10的样品, t₂₃峰几乎看不到, 可以认为5/10的木薯淀粉中几乎不含有自由水分。3/10的样品t₂值最大, 说明水分的流动性最大; 但代表结合水的峰面积最大, 说明结合水所占比例最多。

4. 结论与展望

水分对高静压处理淀粉的糊化及重结晶有重要影响。适度水分含量的淀粉颗粒较容易在HHP处理下发生结晶解体及溶胀现象。粉水比为3/10和5/10的样品具有适度的水分含量。在该水分含量下, 糯玉米淀粉发生结晶解体后吸水膨胀, 不同样品的重结晶程度4/10粉水比 > 3/10粉水比 > 5/10粉水比; 木薯淀粉颗粒结晶结构完全消失, 其结晶破坏程度为3/10粉水比 > 4/10粉水比 > 5/10粉水比。随着水分含量的增加, 糯玉米及木薯淀粉的粒度逐渐增大。干燥后淀粉中的水分主要以结合水的形式存在, 且水分参与重结晶。

目前, 高压导致不同类型淀粉水分的竞争性吸收机制仍然值得继续深入研究。

图 1 部分折叠收缩管结构

Figure 1. Partial structure of folded shrink tube part

下载: 全尺寸图片幻灯片

图 2 折纹管压缩示意图

Figure 2. Schematic diagram of folded tube compression

下载: 全尺寸图片幻灯片

图 3 折纹管材的应力-应变曲线

Figure 3. Material engineering stress–strain curve

下载: 全尺寸图片幻灯片

图 4 准静态压缩下方管和菱形管的变形模式

Figure 4. Deformation modes of square and folded tube under quasi-static compression

下载: 全尺寸图片幻灯片

图 5 准静态压缩下方管和菱形管的力-位移曲线

Figure 5. Force and displacement curves of square and folded tube under quasi-static compression

下载: 全尺寸图片幻灯片

图 6 折叠收缩管的变形过程

Figure 6. Deformation of folded shrink tube

下载: 全尺寸图片幻灯片

图 7 折叠收缩管的力-位移曲线

Figure 7. Force-displacement curves of the folded shrink tube

下载: 全尺寸图片幻灯片

图 8 两端结构不同的折叠收缩管的力-位移曲线

Figure 8. Force-displacement curves of the folded tubes with different structures at both ends

下载: 全尺寸图片幻灯片

图 9 连接结构不同的折叠收缩管力-位移曲线

Figure 9. Force-displacement curves of the folded tubes with different connection structures

下载: 全尺寸图片幻灯片

图 10 不同边长和壁厚时折叠收缩管的力-位移曲线

Figure 10. Force-displacement curves of folded shrink tubes with different edge length and wall thickness

下载: 全尺寸图片幻灯片

表 1 折纹管边长与壁厚的对应关系

Table 1. The corresponding relationship between the edge length and the wall thickness of the folded tube

b/mm	t/mm	b/t
40	1.500	26.667
50	1.200	41.667
60	1.000	60.000
70	0.857	81.667
80	0.750	106.667

下载: 导出CSV

表 2 折纹管准静态压缩模拟结果

Table 2. Quasi-static compression simulation results of folded tube

Thin walled tube	p_Imax/kN	p_Im/kN	W_I/J	p_IImax/kN	p_IIm/kN	W_II/J	p_max/kN	p_m/kN
C	35.59	11.53					35.59	11.53
S	23.88	18.11					25.32	18.11
C60-25.10.4-0	14.58	11.97	707.97	20.83	20.03	60.42	78.61	17.03
C60-30.0.3-0	15.35	13.26	512.72	31.36	24.63	616.35	31.36	18.40
C60-30.10.2-0	17.04	15.34	343.05	29.36	20.49	814.84	29.36	19.08
C60-30.10.3-0	14.61	12.99	404.03	32.27	22.78	709.73	32.27	18.58
C60-30.10.4-0	13.06	11.74	476.68	28.00	22.86	465.76	39.22	17.22
C60-30.20.3-0	14.11	12.96	323.85	35.69	21.68	776.22	35.69	18.75
C60-30.30.3-0	14.04	13.32	272.32	37.71	21.62	841.07	37.71	19.33
C60-35.10.4-0	12.43	11.69	337.39	25.64	21.76	603.20	28.72	18.22
S60-25.10.4-8	14.29	12.92	564.39	26.75	19.45	241.65	66.02	20.30
S60-30.0.3-8	15.29	13.60	563.69	27.28	23.58	464.36	30.00	18.09
S60-30.10.2-8	16.74	15.38	399.16	27.90	23.16	951.44	27.90	20.17
S60-30.10.3-8	14.56	13.30	446.63	27.77	23.34	709.25	27.77	18.65
S60-30.10.4-4	12.91	11.75	487.19	25.71	22.00	429.76	30.57	16.43
S60-30.10.4-8	13.02	11.99	506.97	24.96	21.91	390.70	30.78	16.41
S60-30.10.4-12	13.33	12.27	529.43	24.03	21.85	390.04	35.75	16.55
S60-30.10.4-16	13.54	12.44	547.42	24.45	22.26	462.71	38.48	16.34
S60-30.10.4-20	13.71	12.62	565.27	24.73	21.60	465.27	65.57	16.37
S60-30.20.3-8	14.08	13.19	357.51	30.54	22.70	861.02	30.54	18.95
S60-30.30.3-4	13.96	13.26	281.14	37.35	22.36	918.41	37.35	19.86
S60-30.30.3-8	14.01	13.42	294.32	28.17	22.19	941.43	30.39	19.46
S60-30.30.3-12	14.11	13.62	308.95	27.67	21.66	874.31	31.54	19.24
S60-30.30.3-16	14.48	13.83	331.41	26.98	21.25	746.88	33.44	19.55
S60-30.30.3-20	14.96	14.15	335.61	25.92	21.36	801.94	45.15	19.70
S60-35.10.4-8	12.47	11.83	345.92	27.42	22.45	760.73	27.42	17.89
H60-25.10.4-16	15.02	13.02	786.99	102.41	61.20	519.62	102.41	18.95
H60-30.0.3-16	15.89	13.74	556.07	37.15	29.12	830.22	37.15	20.10
H60-30.10.2-16	17.34	15.76	375.59	43.81	25.51	1149.31	43.81	22.14
H60-30.10.3-16	15.13	13.46	437.65	36.16	23.75	973.23	36.16	20.48
H60-30.10.4-8	13.45	11.98	495.54	39.01	27.82	767.19	39.01	18.32
H60-30.10.4-16	13.58	12.16	499.77	39.60	30.27	844.33	39.60	19.48
H60-30.10.4-24	13.69	12.41	517.13	44.85	32.16	804.22	60.97	20.89
H60-30.10.4-32	13.84	12.65	538.25	48.49	32.33	759.05	74.90	21.46
H60-30.10.4-40	14.09	12.85	557.04	35.21	28.13	420.96	78.53	21.89
H60-30.20.3-16	14.47	13.37	350.06	36.01	25.12	1075.92	36.01	20.68
H60-30.30.3-8	14.29	13.33	270.28	36.43	23.48	1097.22	36.43	20.37
H60-30.30.3-16	14.31	13.62	286.54	44.67	25.14	1195.94	44.67	21.59
H60-30.30.3-24	14.53	13.85	291.37	46.29	26.56	1271.32	46.29	22.68
H60-30.30.3-32	14.83	14.11	307.12	43.27	27.79	1233.83	43.27	23.61
H60-30.30.3-40	15.20	14.32	311.18	42.98	26.57	1221.30	42.98	22.59
H60-35.10.4-16	12.78	12.01	352.43	31.25	25.85	1013.33	31.25	19.94

下载: 导出CSV

表 3 折纹管吸能性能对比

Table 3. Comparison of energy absorption performance of folded tubes

	Relative to tube C/%				Relative to tube S/%
	$\delta$ _Imax	$\delta$ _Im	$\delta$ _max	$\delta$ _m	$\delta$ _Imax	$\delta$ _m
C60-35.10.4-0	−65.07	1.39	−19.30	58.02	−47.95	0.61
S60-30.30.3-8	−60.64	16.39	−14.61	68.78	−41.33	7.45
H60-30.30.3-32	−58.33	22.38	21.58	104.77	−37.90	30.37

下载: 导出CSV

表 4 连接结构参数改变

Table 4. Connection structure parameters change

Group No.	α₁/(°)	θ₁/(°)	x₁/mm
1	25	10	4
	30	10	4
	35	10	4
2	30	0	3
	30	10	3
	30	20	3
	30	30	3
3	30	10	2
	30	10	3
	30	10	4

下载: 导出CSV

参考文献(23)

[1]	SINGACE A A. Axial crushing analysis of tubes deforming in the multi-lobe mode [J]. International Journal of Mechanical Sciences, 1999, 41(7): 865–890. doi: 10.1016/S0020-7403(98)00052-6
[2]	WIERZBICKI T, ABRAMOWICZ W. On the crushing mechanics of thin-walled structures [J]. Journal of Applied Mechanics, 1983, 50(4a): 727–734. doi: 10.1115/1.3167137
[3]	SONG J, CHEN Y, LU G X. Light-weight thin-walled structures with patterned windows under axial crushing [J]. International Journal of Mechanical Sciences, 2013, 66: 239–248. doi: 10.1016/j.ijmecsci.2012.11.014
[4]	CHENG Q W, ALTENHOF W, LI L. Experimental investigations on the crush behaviour of AA6061-T6 aluminum square tubes with different types of through-hole discontinuities [J]. Thin-Walled Structures, 2006, 44(4): 441–454. doi: 10.1016/j.tws.2006.03.017
[5]	HAN H P, TAHERI F, PEG G N. Quasi-static and dynamic crushing behaviors of aluminum and steel tubes with a cutout [J]. Thin-Walled Structures, 2007, 45(3): 283–300. doi: 10.1016/j.tws.2007.02.010
[6]	DANESHI G H, HOSSEINIPOUR S J. Grooves effect on crashworthiness characteristics of thin-walled tubes under axial compression [J]. Materials & Design, 2002, 23(7): 611–617. doi: 10.1016/S0261-3069(02)00052-3
[7]	EYVAZIAN A, HABIBI M K, HAMOUDA A M, et al. Axial crushing behavior and energy absorption efficiency of corrugated tubes [J]. Materials & Design, 2014, 54: 1028–1038. doi: 10.1016/j.matdes.2013.09.031
[8]	ZHANG X W, SU H, YU T X. Energy absorption of an axially crushed square tube with a buckling initiator [J]. International Journal of Impact Engineering, 2009, 36(3): 402–417. doi: 10.1016/j.ijimpeng.2008.02.002
[9]	HANSSEN A G, LANGSETH M, HOPPERSTAD O S. Static and dynamic crushing of circular aluminium extrusions with aluminium foam filler [J]. International Journal of Impact Engineering, 2000, 24(5): 475–507. doi: 10.1016/S0734-743X(99)00170-0
[10]	KIM H S. New extruded multi-cell aluminum profile for maximum crash energy absorption and weight efficiency [J]. Thin-Walled Structures, 2002, 40(4): 311–327. doi: 10.1016/S0263-8231(01)00069-6
[11]	ZHANG X, HU H H. Crushing analysis of polygonal columns and angle elements [J]. International Journal of Impact Engineering, 2010, 37(4): 441–451. doi: 10.1016/j.ijimpeng.2009.06.009
[12]	TANG Z L, LIU S T, ZHANG Z H. Energy absorption properties of non-convex multi-corner thin-walled columns [J]. Thin-Walled Structures, 2012, 51: 112–120. doi: 10.1016/j.tws.2011.10.005
[13]	李笑, 李明. 折纸及其折痕设计研究综述 [J]. 力学学报, 2018, 50(3): 467–476. doi: 10.6052/0459-1879-18-031 LI X, LI M. A review of origami and its crease design [J]. Chinese Journal of Theoretical and Applied Mechanics, 2018, 50(3): 467–476. doi: 10.6052/0459-1879-18-031
[14]	SONG J, CHEN Y, LU G X. Axial crushing of thin-walled structures with origami patterns [J]. Thin-Walled Structures, 2012, 54: 65–71. doi: 10.1016/j.tws.2012.02.007
[15]	MA J Y, HOU D G, CHEN Y, et al. Quasi-static axial crushing of thin-walled tubes with a kite-shape rigid origami pattern: numerical simulation [J]. Thin-Walled Structures, 2016, 100: 38–47. doi: 10.1016/j.tws.2015.11.023
[16]	MA J Y, YOU Z. Energy absorption of thin-walled square tubes with a prefolded origami pattern—part I: geometry and numerical simulation [J]. Journal of Applied Mechanics, 2014, 81(1): 011003. doi: 10.1115/1.4024405
[17]	ZHOU C H, WANG B, LUO H Z, et al. Quasi-static axial compression of origami crash boxes [J]. International Journal of Applied Mechanics, 2017, 9(5): 1750066. doi: 10.1142/S1758825117500661
[18]	ZHOU C H, WANG B, MA J Y, et al. Dynamic axial crushing of origami crash boxes [J]. International Journal of Mechanical Sciences, 2016, 118: 1–12. doi: 10.1016/j.ijmecsci.2016.09.001
[19]	YANG K, XU S Q, SHEN J H, et al. Energy absorption of thin-walled tubes with pre-folded origami patterns: numerical simulation and experimental verification [J]. Thin-Walled Structures, 2016, 103: 33–44. doi: 10.1016/j.tws.2016.02.007
[20]	YUAN L, SHI H Y, MA J Y, et al. Quasi-static impact of origami crash boxes with various profiles [J]. Thin-Walled Structures, 2019, 141: 435–446. doi: 10.1016/j.tws.2019.04.028
[21]	WANG B, ZHOU C H. The imperfection-sensitivity of origami crash boxes [J]. International Journal of Mechanical Sciences, 2017, 121: 58–66. doi: 10.1016/j.ijmecsci.2016.11.027
[22]	ZHOU C H, ZHOU Y, WANG B. Crashworthiness design for trapezoid origami crash boxes [J]. Thin-Walled Structures, 2017, 117: 257–267. doi: 10.1016/j.tws.2017.03.022
[23]	XIE R K, HOU D G, MA J Y, et al. Geometrically graded origami tubes [C]//Proceedings of ASME 2016 International Design Engineering Technical Conferences and Computers and Information in Engineering Conference. Carolina: ASME, 2016.

施引文献

资源附件(0)

访问统计

点击查看大图

图(10) / 表(4)

计量

文章访问数: 4793
HTML全文浏览量: 1958
PDF下载量: 35

1. 引言
2. 实验材料与方法
2.1 实验材料处理
2.2 实验方法
3. 实验结果与讨论
3.1 颗粒形貌
3.2 粒度分布
3.3 晶体结构
3.4 水分分布
4. 结论与展望

具有梯度型刚度折叠收缩管的吸能性能

doi: 10.11858/gywlxb.20190873

作者简介: 陈伟东（1996－），男，硕士研究生，主要从事折叠结构的吸能特性研究. E-mail：chen_weidong04@163.com

通讯作者: 田晓耕（1967－），男，博士，教授，主要从事超轻结构吸能及优化、非经典热弹理论研究. E-mail：tiansu@mail.xjtu.edu.cn

计量

出版历程