Phenomenon of Local Densification in Negative Graded Metal Foam
-
摘要: 基于一维非线性的刚性-塑性硬化模型,研究了在恒速冲击作用下负梯度泡沫的冲击波控制方程和传播特性。采用LS-DYNA有限元软件对三维随机Voronoi技术生成的梯度泡沫金属模型进行数值模拟,验证了理论预测,并定义了冲击波模型下梯度泡沫材料的局部密实化应变与第二临界速度。通过对冲击速度、密度梯度、相对密度参数的影响研究发现:冲击波模型的理论解与有限元模型的数值解吻合较好,基于R-PH模型的冲击波理论能较好地预测负梯度泡沫金属的力学性能;局部密实化应变在不同冲击速度下存在3个增长阶段;密度梯度绝对值和相对密度越大,局部密实化应变越小,第二临界速度越大。最后讨论了负梯度泡沫中局部密实化现象对支撑端应力的影响。Abstract: Based on the one-dimensional nonlinear rigid-plastic hardening (R-PH) model, the control equations and mechanical response characteristics of the shock wave propagation of the negative graded foam under constant velocity impact are studied. The LS-DYNA finite element software is used to numerically simulate the graded metal foam model generated by the three-dimensional stochastic Voronoi technology to verify the theoretical prediction. The local densification strain and the second critical velocity of the graded foam material under the shock wave model are defined. By studying the effects of impact velocity, density gradient and relative density parameters, it is found that the theoretical solution of the shock wave model is in good agreement with the numerical solution of the finite element model. The shock wave theory based on the R-PH model can better predict the negative graded foam metal. The mechanical properties of the local densification strain have three growth stages at different impact velocities; the larger the absolute value of the density gradient and the relative density, the smaller the local densification strain and the larger the second critical velocity. Finally, the effect of local densification on the stress at the support end in the negative graded foam is explained.
-
传感器技术、通信技术、计算机技术构成了现代信息技术的3大支柱。压电式传感器以频响高、体积小、质量轻、频带宽等特点[1-3],成为高技术领域中制备小型化及多功能化器件的重要元件之一。薄膜型压电传感器的敏感单元为铁电聚合物薄膜,在电子、超声、水声、红外、导航、生物等多个领域应用广泛。早在19世纪40年代人们便开始了高分子聚合物压电性能研究[4-6],但直到70年代才将其制成压电薄膜传感器。压电聚合物通常为非导电性高分子材料,从原理上看没有可移动的电子电荷,但在某些特定的条件下(经延展拉伸、极化等),带负电荷的引力中心可以被改变,从而成为具有压电性的高分子压电薄膜。常见的高分子压电薄膜有聚氟乙烯(PVF)、聚偏二氟乙烯(PVDF)、聚氯乙烯(PVC)、尼龙11等。
动高压聚合物薄膜测量技术的核心内容之一是敏感单元压电性能研究[7-9]。PVDF传感器的敏感单元是PVDF压电薄膜。PVDF薄膜属于半结晶的高分子聚合物,晶区至少存在4种晶型结构(α相、β相、γ相和δ相),其压电性能直接与极性β相相关。在常温常压下,通过PVDF溶液挥发或熔融结晶得到的PVDF初始膜主要以非极性α相为主;对PVDF初始膜进行单轴拉伸或高电压极化处理,可产生具有更多压电β相的PVDF压电薄膜。
本研究首先探讨PVDF传感器的设计和制作工艺,包括初始膜制备、单轴拉伸取向诱导压电相、高压热极化试验、封装技术等;然后采取溶液等温结晶法制备初始PVDF薄膜,对初始膜进行单轴拉伸试验,分析不同拉伸倍率、拉伸速率和拉伸温度对PVDF薄膜中结晶相的影响;接着在高电场交流极化实验中,分析不同电场频率和幅值对PVDF薄膜位移电流的影响,获得薄膜本征位移电流曲线;最后对传感器的形状、尺寸、厚度进行设计,封装完成PVDF压电薄膜传感器。希望本研究结果有助于提高PVDF薄膜材料在动态冲击过程中的检测能力和可靠性,推进响应快、测量范围宽、灵敏度高的聚合物薄膜测量技术在动高压冲击实验中的应用。
1. PVDF敏感单元设计
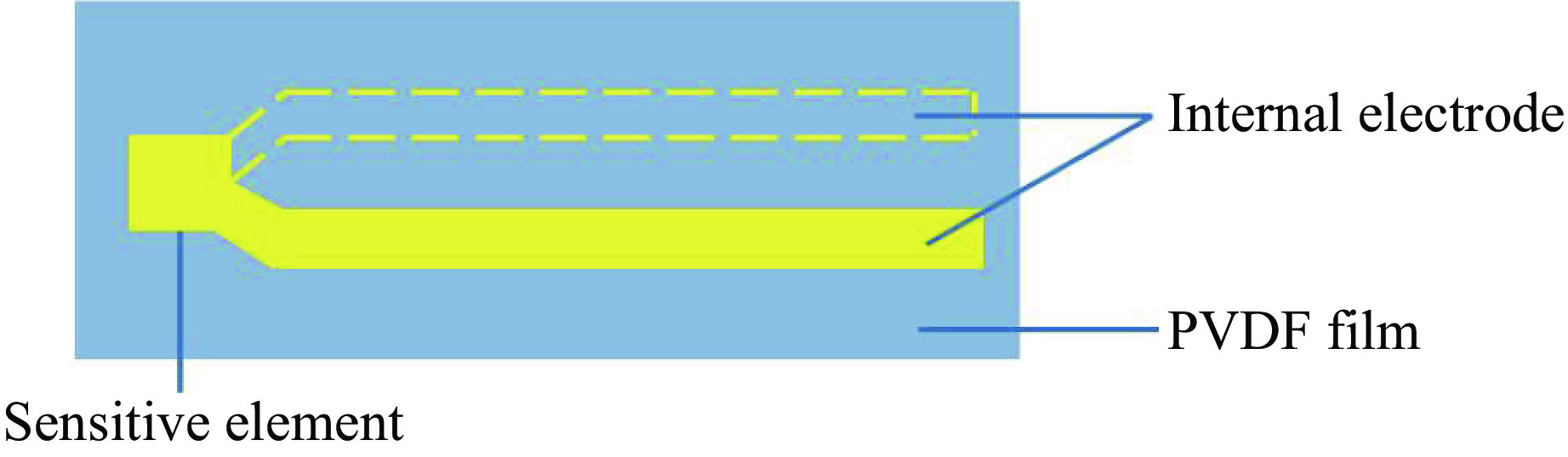
PVDF压力计上、下电极的重合部分为其敏感单元。当外力使PVDF压力计的敏感单元发生变形时,薄膜敏感单元表面就会产生与应力大小成正比的电荷量。本研究设计的敏感单元尺寸为3 mm × 3 mm,薄膜厚度选用
20μm 或30μm 。PVDF传感器总厚度小于0.1 mm,有利于提高响应时间。敏感单元电极结构如图1所示。1.1 电极制作和极化
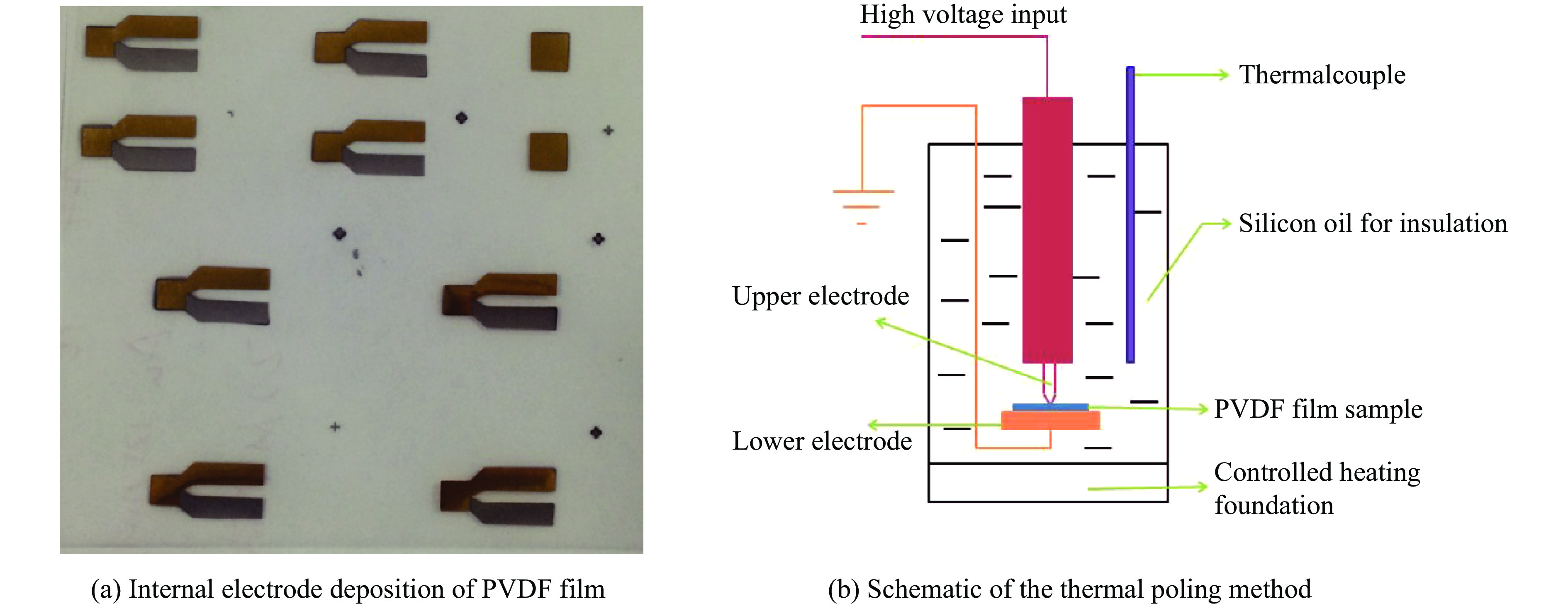
敏感单元使用双层电极结构,下电极Pt的厚度为50 nm,上电极Au的厚度为250 nm。敏感单元的尺寸直接影响PVDF薄膜的铁电性能测试,因此要精确控制敏感单元的尺寸。为了获得特定形状的内电极金属结构,使用掩膜法在PVDF薄膜衬底上的特定位置沉积金属电极结构。为了提高敏感单元面积精度,采用双面掩膜板结构,上、下掩膜板的对位精度控制在 ± 0.05 mm以内。PVDF薄膜的居里温度约为170 ℃,对热敏感,在镀电极过程中温度的上升会引起电极与PVDF薄膜间的热应力增大,影响电极和PVDF薄膜的黏结度,且对薄膜性能造成影响,因此在沉积金属电极时,需对沉积温度进行严格控制。上电极Au的导电性好,但与PVDF薄膜的黏合度较差,而金属Pt与PVDF薄膜的黏合度较好,但是活性较低,所以采用Au/Pt双层金属电极结构,能够发挥两种金属的各自优点,使电极结构的附着力强、导电性好。
在溅射金属薄膜电极过程中,有两个溅射参数需要重点关注,即溅射功率和工作压强。当溅射功率较大时,电离出的Ar+的能量较大,轰击靶材溅射出更多的粒子,粒子的迁移速率变大,易形成连续电极结构;但溅射功率过高时,溅射出的高能粒子将会产生反溅射作用,对薄膜衬底造成损害。当工作压强增大时,溅射粒子向薄膜运动的过程中受到室内气体散射的几率增大,薄膜上沉积的粒子能量减少,且平均粒径减小。过高的工作压强会降低沉积速率,不利于形成连续电极;而过低的工作压强会使沉积的电极表面粗糙度增大。本实验采用多次间歇溅射,溅射温度为室温。所得的PVDF薄膜衬底沉积金属薄膜内电极结构如图2(a)所示,可见,金属电极与薄膜无褶皱,电极边界清晰。
对于PVDF薄膜,一般采用拉伸工艺获得含量较多的压电β相,但此时薄膜中的偶极子排列无序,宏观上不显电性,需要经过人工极化处理。通过施加高电压可使薄膜中的偶极子沿外电场方向有序排列,当去除电场后,一部分偶极取向瞬间消失,另一部分偶极取向保留下来,称为剩余极化强度,它直接反映PVDF薄膜的压电性。PVDF是偶极电荷型压电铁电聚合物,极化以偶极子的转向优先,常采用热极化方法和电晕放电方法极化,此时偶极子取向由沉积在电介质中的空间电荷场诱导产生。电晕极化是在薄膜表面注入新的导电载流子,使薄膜的电导增强,击穿场强降低。热极化方法具有设备简单、操作方便、极化彻底等优点。因为空气的击穿场强较低,所以本研究采用热极化方法对PVDF薄膜进行高压热极化处理,如图2(b)所示。在样品盒内充满绝缘油,本研究选用硅油。硅油的介电常数高,将硅油作为绝缘介质时,可避免高电压的边缘飞弧,可施加极高的极化电压。热极化过程中伴随着分子链、离子、空间电荷的运动,导致薄膜表面弯曲变形,影响敏感面积的稳定极化,因此极化过程需要对样品施加一定的压强。
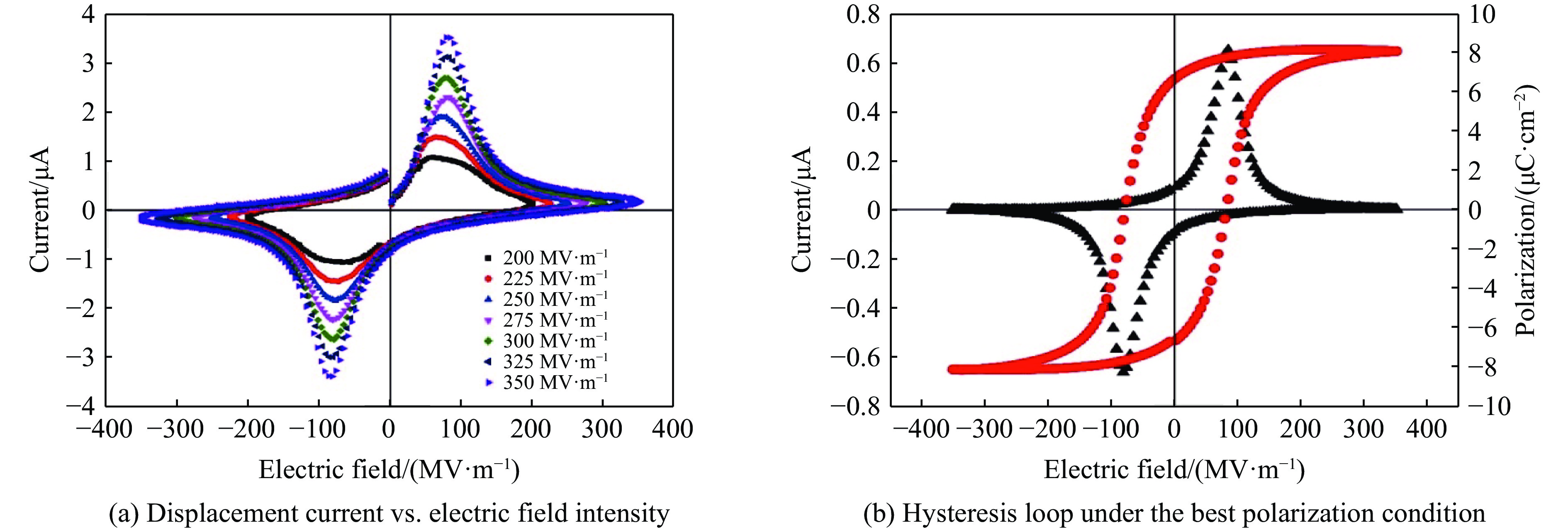
图3(a)显示了对
20μm 厚PVDF薄膜施加不同幅值极化电场时所得的位移电流曲线。可以看出,随着电场强度的增加,位移电流的电阻、电容效应越来越弱,说明350 MV/m的场强为20μm 厚PVDF薄膜的最佳热极化条件,此时的位移电流和电滞回线如图3(b)所示。1.2 敏感单元封装
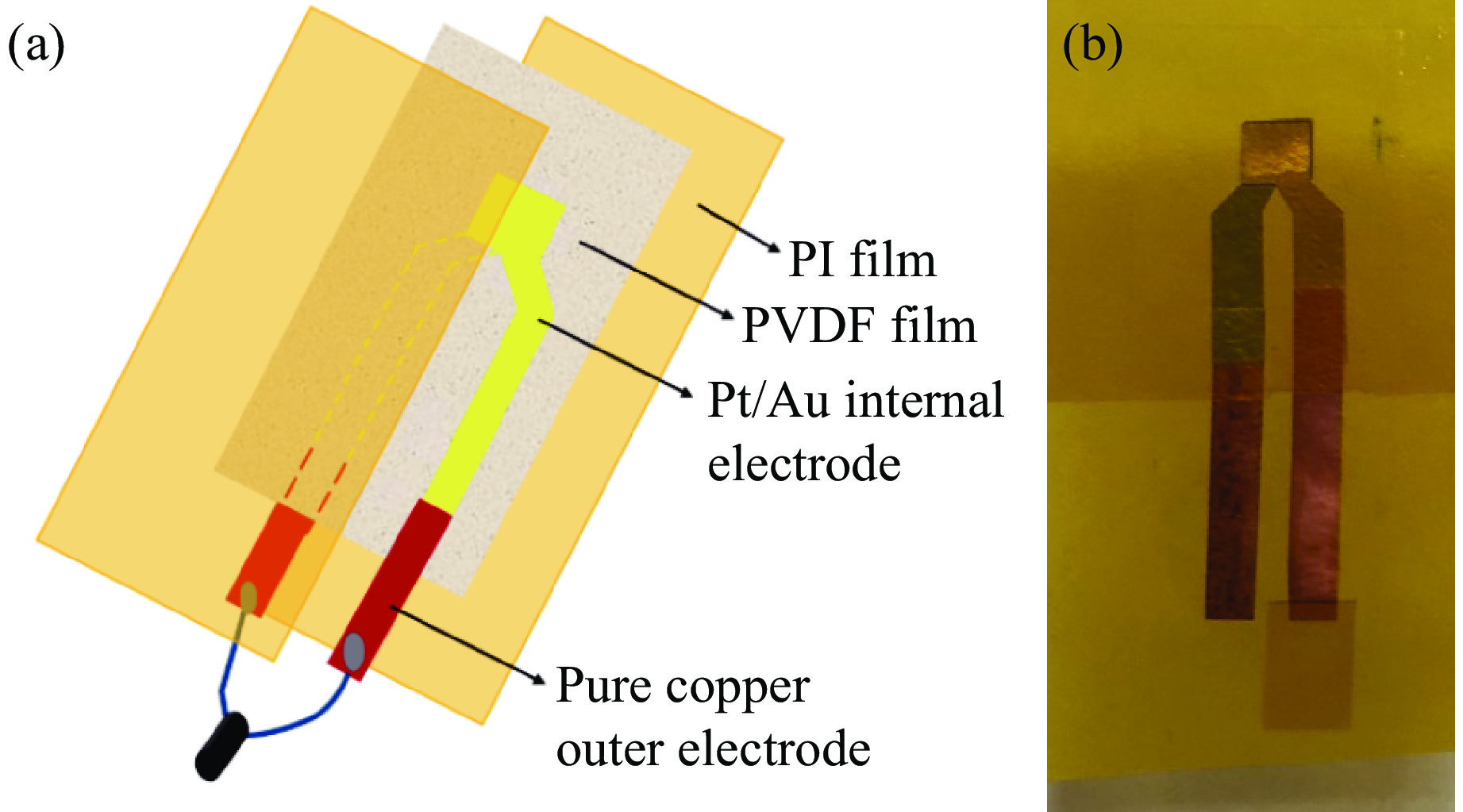
使用磁控溅射完成PVDF传感器内电极的制作后,内电极为Au/Pt双层金属电极结构,总厚度为300 nm。使用紫铜箔作为外电极,并用聚酰亚胺(PI)薄膜进行封装。因为PVDF本身为高阻元器件,外部保护膜的阻抗应大于传感器本身阻抗2个数量级以上,PI薄膜的电阻率为1 014~1 015
Ω ·m,PVDF薄膜体积电阻率为1 011Ω ·m,所以PI薄膜厚度应不小于PVDF薄膜厚度的1/10即可满足使用要求。本研究使用的PVDF薄膜的厚度分别为20μm 和30μm ,PI封装薄膜厚度为25μm 。图4为PVDF传感器所示3层结构封装示意图,上、下内电极的重叠部分为敏感单元,其尺寸为3 mm × 3 mm,内电极与外电极铜箔使用冷压技术连接,然后将低损耗同轴电缆与外电极铜箔焊接。2. 动态冲击设计和标定
2.1 测试系统组件选择
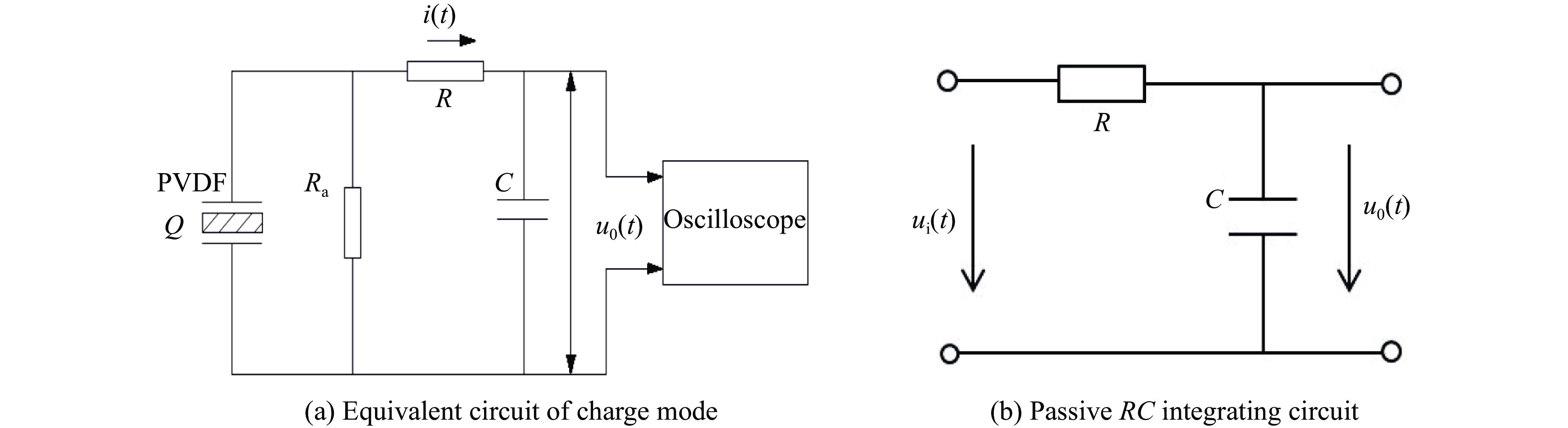
当外界压力使压电材料发生变形时,压电材料的表面会产生与应力大小成正比的电荷量,电介质内部的电偶极子排序改变上下表面的电势,与表面接触的两个电极上的电荷重新调整,以平衡PVDF表面电势,因此在电路中存在电荷流动。将PVDF压电薄膜等效为电容,测量所产生的电荷,可采用两种模式测量:电流模式和电荷模式。电荷法适合信号变化要求不高的数据采集模式,频响(上升时间)受电荷积分器响应特性的影响;电流法适合于高频响数据采集,尤其是压力高于20 GPa的数据采集,缺点是误差与测试电缆长度的关联性高。考虑到本研究在低压范围标定,电荷积分器满足频率响应,因此选取电荷模式。
电荷模式是将传感器并联一个匹配电容,将其产生的电荷Q(t)导入该电容,然后外接显示设备,输出与外界压力σ(t)直接对应的电压信号V(t),该方法也称直接测量法,如图5(a)所示,其中Ra传感器内阻。电荷测试部分采用无源RC积分电路,如图5(b)所示。在无源RC积分电路中,若时间常数τ = RC足够大,则外加电压时,电容上的电压只能慢慢上升。所以在测量时间远小于时间常数τ的时间范围内,电路达到稳态的时间长,电容C两端的电压很小,输入电压主要降落在电阻R上,此时充电电流ic和输出电压u0(t)为
ic=ui(t)/R (1) u0(t)=1C∫t0icdt=1C∫t0ui(t)Rdt=1RC∫t0ui(t)dt (2) 式中:ui(t)为输入电压。可以看出,输出电压u0(t)近似与输入电压ui(t)的时间积分值成比例。
∫t0[ui(t)/R]dt 为流过电阻R的电流积分,即电路中所产生的电荷Q(t),所以该种测量方式为电流模式,此时电路中的电荷为u0(t)⋅C=Q(t) (3) 测试元件中电容C的选择依据:RC积分电路中时间常数τ远远大于输入脉冲宽度(一般超过10倍)。计算时间常数τ ,若电容C的单位为F,R的单位为
Ω ,则时间常数τ的单位为s。当C =0.1μF 时τ=RC=50×0.1×10−6s=5000ns (4) 对于所测系统而言,频响(上升时间)为50~150 ns,所以选用C =
0.1μF 的电容,能够保证τ≫t (10倍以上)。本研究选用50
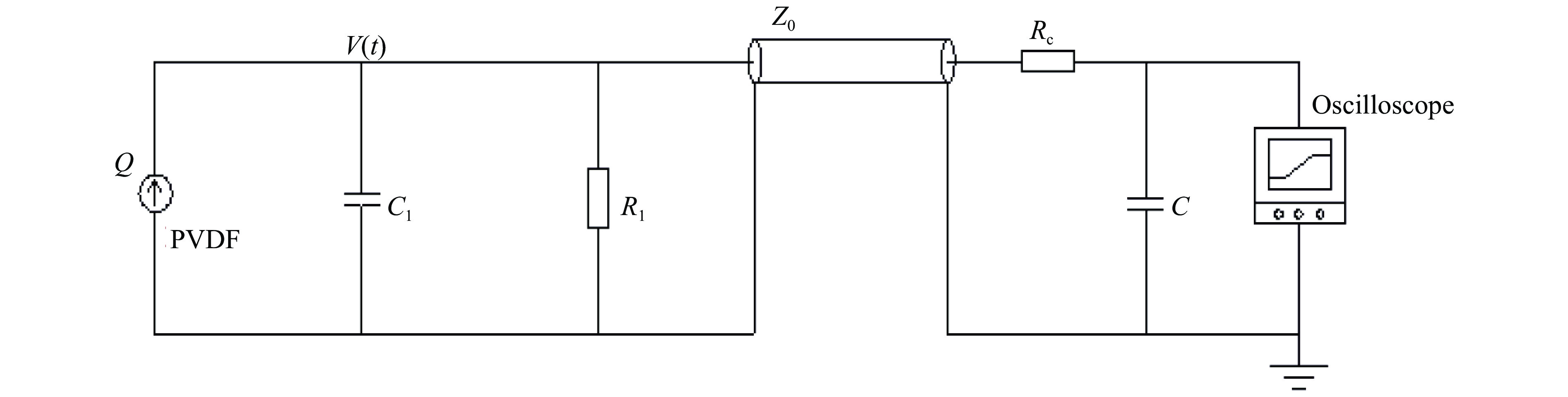
Ω 特征阻抗的同轴电缆,阻抗匹配的测试系统电路如图6所示,其中C1为电缆电容,R1为传感器泄漏电阻和电缆电阻,Z0为同轴电缆特征阻抗(50Ω ),Rc是与同轴电缆阻抗匹配的电阻阻值(50Ω ),C为0.1μF 。2.2 PVDF传感器动态冲击压缩实验标定方法
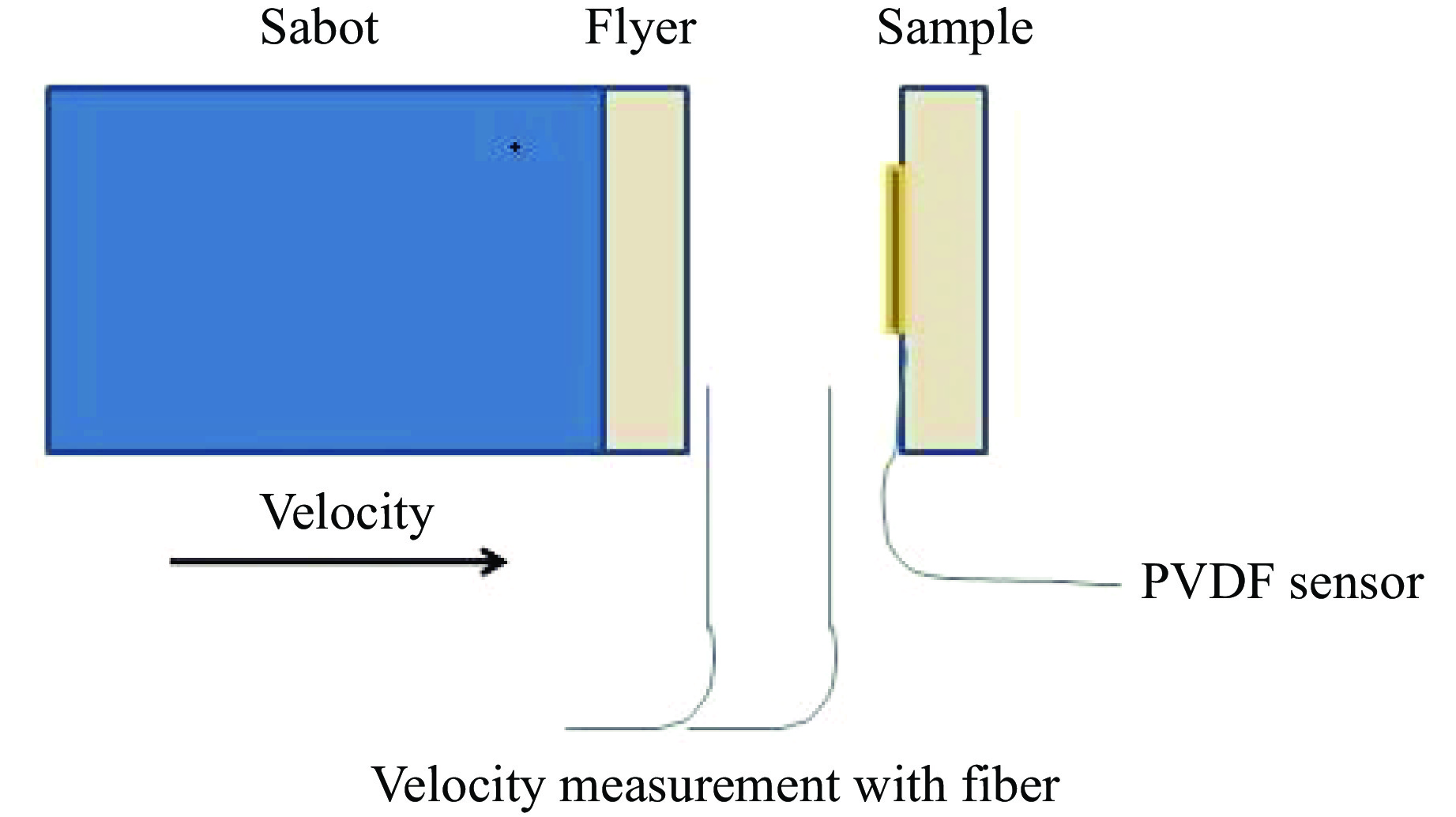
采用对称碰撞方法(飞片和靶为同种材料)标定PVDF传感器的动态压缩曲线。所选材料为PMMA、LY12铝和45钢,将PVDF传感器粘在样品和飞片的撞击面上,如图7所示。飞片击靶速度u0采用激光遮断法测量,利用飞片遮断测速环中不同路光纤的激光信号得到相应的时间差,测速环由3路光纤组成,每路光纤间距8.5 mm。
按照应力波理论中的阻抗匹配法,对称碰撞过程中靶中冲击波阵面后粒子速度up是飞片击靶速度u0的1/2,结合样品材料的冲击Hugoniot关系式,通过测量撞击速度u0可确定标定压力值
σ=ρ0Dup=ρ0(c0+λup)up=12ρ0(c0+12λu0)u0 (3) 式中:D为冲击波速度,km/s;ρ0为样品初始密度,g/cm3;c0为线性Hugoniot关系式中常系数,km/s;
λ 为线性Hugoniot关系式中常系数;u0为飞片撞击速度,km/s;up为样品中的粒子速度,km/s。式(5)给出了撞击压力σ与飞片击靶速度u0的对应关系。实验中所使用撞击材料的Hugoniot参数列于表1。表 1 Al和45钢的Hugoniot参数Table 1. Hugoniot parameters of Al and 45 steelMaterial ρ0/(g·cm–3) c0/(km·s–1) λ Al 2.785 5.328 1.338 45 steel 7.850 4.483 1.332 PVDF压电传感器的输出电荷由电荷积分器采集,然后由示波器输出电压得到聚合物薄膜敏感压力-电荷密度标定曲线。
2.3 30 μm厚PVDF传感器动态冲击压缩实验标定
对于敏感单元厚度为
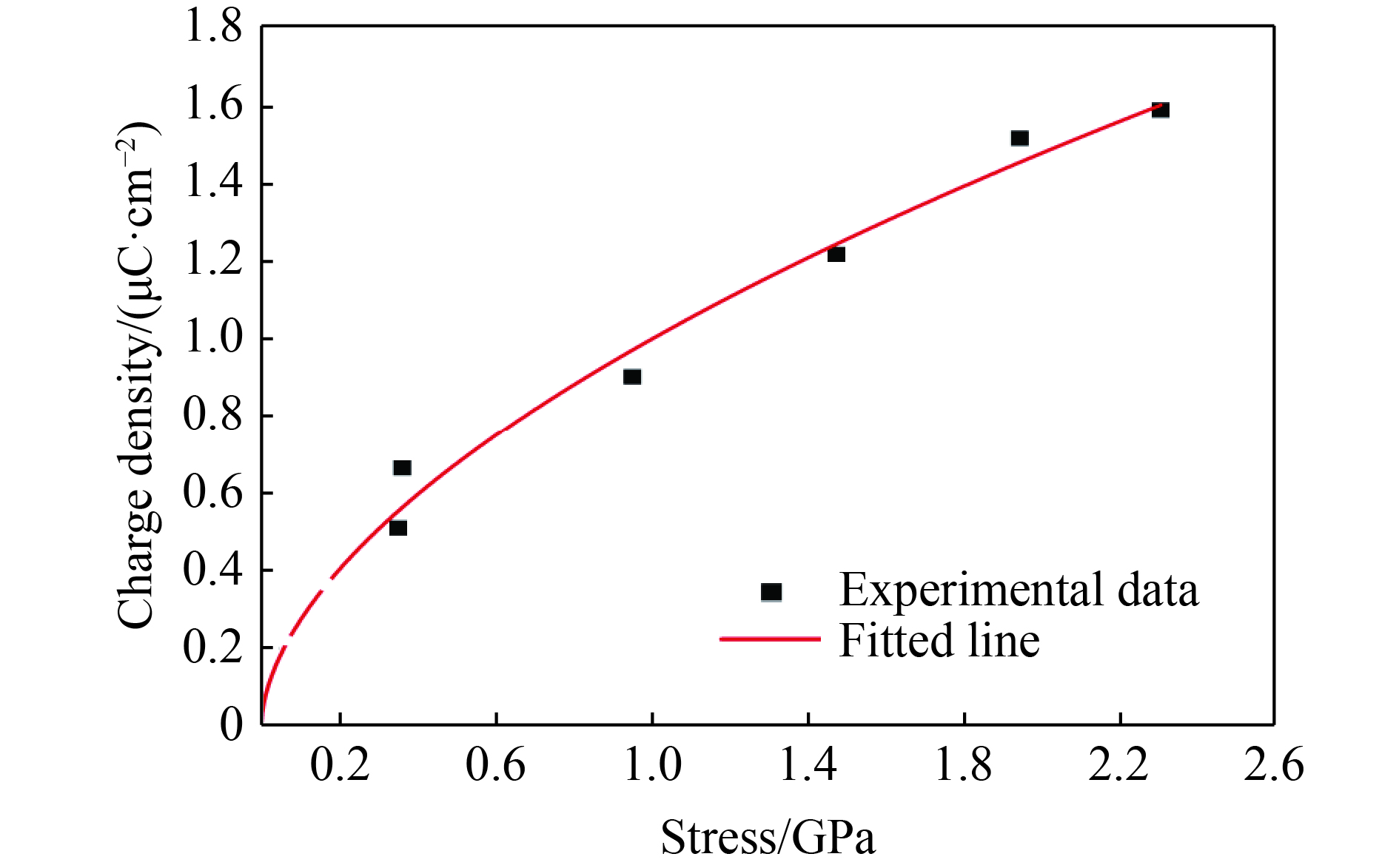
30μm 的PVDF传感器,在0.3~10.0 GPa压力区间由电荷法得到的实验结果如表2所示,其中:低压段采用气炮加载方式,高压段采用火炮加载方式,Umax为电压峰值,(Q/A)max为电荷密度峰值,d33为压电常数,Pr为剩余极化强度。表 2 电荷法实验结果Table 2. Experimental results by charge methodExp.No. Material of flyer u0/(m·s−1) p/GPa Umax/V (Q/A)max/(μC·cm−2) d33/(pC·N−1) Pr/(μC·cm−2) 0830-1 LY12 Al 48.434 0.361 2 0.598 67 0.665 2 12.0 8.279 7 0830-2 45 steel 83.384 1.473 0 1.097 90 1.219 9 10.8 7.528 9 0830-3 45 steel 109.557 1.942 8 1.367 27 1.519 2 10.5 7.878 1 0830-4 45 steel 129.536 2.303 8 1.433 01 1.592 2 15.5 7.987 1 0831-1 LY12 Al 126.221 0.950 6 0.813 58 0.904 0 15.2 10.301 7 1128-1 LY12 Al 47.137 0.351 5 0.459 01 0.510 0 15.1 7.806 1 1030 LY12 Al 628.531 5.395 0 1.636 01 1.817 8 15.6 6.934 0 1026 PMMA 1 004.704 2.426 1 1.120 07 1.244 5 15.3 7.832 2 1107 LY12 Al 970.275 8.069 3 1.883 43 2.092 7 11.4 7.216 3 1108 LY12 Al 1 250.441 10.725 5 2.357 62 2.619 6 12.8 8.287 2 PVDF传感器在一定压力范围内具有线性度,但是在较宽压力范围内不具备线性响应特性。本研究中,为了提高标定曲线的精度,选取幂函数、线性函数和多项式分别进行拟合。结果表明,幂函数的拟合精度最高,为此选择幂函数拟合标定曲线。采用幂函数,分别对表2中0.35~2.30 GPa和2.4~10.7 GPa压力区间的实验数据进行拟合。低压段拟合结果如图8所示,拟合曲线方程为
QA=(1.00166±0.03118)⋅σ(0.56511±0.04567) (4) 其相关指数为0.989,剩余标准差为6.611%。高压段采用
QA=aσb 函数进行拟合,如图9所示,拟合曲线方程为QA=(0.77960±0.09140)⋅σ(0.49843±0.05681) (5) 其相关指数为0.979,剩余标准差为10.015%。
3. 结 论
介绍了PVDF传感器研制过程的关键技术和工艺;在此基础上采用气炮加载装置进行了低压段和高压段一维平面应力标定方法研究,使用电荷测量模式,得到了敏感单元厚度为
30μm 的PVDF传感器在0.35~2.30 GPa和2.4~10.7 GPa压力范围的冲击压力与电荷密度关系。为了得到最佳的标定曲线,使用幂函数分别对低压段和高压段进行拟合,相关指数接近1,且剩余标准差最小。在标定实验过程中,当冲击压力大于10 GPa时,敏感单元厚度为30μm 的PVDF传感器的响应时间为37.2 ns,说明传感器具有较高的频率响应特性。 -
表 1 本构模型参数
Table 1. Constitutive model parameters
ρs/(kg·m–3) E/GPa ν σys/MPa Et/GPa 2 700 69 0.3 76 0.69 -
[1] ASHBY M F, EVANS A, FLECK N A, et al. Metal foams: a design guide [M]. UK: Heinemann Publishers, 2000. [2] GIBSON L J. Mechanical behavior of metallic foams [J]. Annual Review of Materials Science, 2000, 30: 191–227. doi: 10.1146/annurev.matsci.30.1.191 [3] ZHENG Z J, YU J L, LI J R. Dynamic crushing of 2D cellular structures: a finite element study [J]. International Journal of Impact Engineering, 2005, 32(1/2/3/4): 650–664. [4] LIU Y D, YU J L, ZHENG Z J, et al. A numerical study on the rate sensitivity of cellular metals [J]. International Journal of Solids and Structures, 2009, 46(22/23): 3988–3998. [5] ELNASRI I, PATTOFATTO S, ZHAO H, et al. Shock enhancement of cellular structures under impact loading: part I experiments [J]. Journal of the Mechanics and Physics of Solids, 2007, 55(12): 2652–2671. doi: 10.1016/j.jmps.2007.04.005 [6] BROTHERS A H, DUNAND D C. Mechanical properties of a density-graded replicated aluminum foam [J]. Materials Science and Engineering A, 2008, 489(1/2): 439–443. [7] HANGAI Y, TAKAHASHI K, YAMAGUCHI R, et al. Nondestructive observation of pore structure deformation behavior of functionally graded aluminum foam by X-ray computed tomography [J]. Materials Science and Engineering A, 2012, 556: 678–684. doi: 10.1016/j.msea.2012.07.047 [8] BRUCK H A. A one-dimensional model for designing functionally graded materials to manage stress waves [J]. International Journal of Solids and Structures, 2000, 37(44): 6383–6395. doi: 10.1016/S0020-7683(99)00236-X [9] KIERNAN S, CUI L, GILCHRIST M D. Propagation of a stress wave through a virtual functionally graded foam [J]. International Journal of Non-Linear Mechanics, 2009, 44(5): 456–468. doi: 10.1016/j.ijnonlinmec.2009.02.006 [10] CUI L, KIRENAN S, GILCHRIST M D. Designing the energy absorption capacity of functionally graded foam materials [J]. Materials Science and Engineering A, 2009, 507(1/2): 215–225. [11] 吴鹤翔, 刘颖. 梯度变化对密度梯度蜂窝材料力学性能的影响 [J]. 爆炸与冲击, 2013, 33(2): 163–168.WU H X, LIU Y. Influences of density gradient variation on mechanical performances of density-graded honeycomb materials [J]. Explosion and Shock Waves, 2013, 33(2): 163–168. [12] LIANG M Z, ZHANG G D, LU F Y, et al. Blast resistance and design of sandwich cylinder with graded foam cores based on the Voronoi algorithm [J]. Thin-Walled Structures, 2017, 112: 98–106. doi: 10.1016/j.tws.2016.12.016 [13] REID S R, PENG C. Dynamic uniaxial crushing of wood [J]. International Journal of Impact Engineering, 1997, 19(5/6): 531–570. [14] TAN P J, REID S R, HARRIGAN J J, et al. Dynamic compressive strength properties of aluminum foams. Part II–'shock' theory and comparison with experimental data and numerical modelsls [J]. Journal of the Mechanics and Physics of Solids, 2005, 53(10): 2206–2230. doi: 10.1016/j.jmps.2005.05.003 [15] WANG X K, ZHENG Z J, YU J L. Crashworthiness design of density-graded cellular metals [J]. Theoretical and Applied Mechanics Letters, 2013, 3(3): 031001. doi: 10.1063/2.1303101 [16] SHEN C J, YU T X, LU G. Double shock mode in graded cellular rod under impact [J]. International Journal of Solids and Structures, 2013, 50(1): 217–233. doi: 10.1016/j.ijsolstr.2012.09.021 [17] SHEN C J, LU G, YU T X. Investigation into the behavior of a graded cellular rod under impact [J]. International Journal of Impact Engineering, 2014, 74: 92–106. doi: 10.1016/j.ijimpeng.2014.02.015 [18] ZHENG Z J, WANG C F, YU J L, et al. Dynamic stress-strain states for metal foams using a 3D cellular model [J]. Journal of the Mechanics and Physics of Solids, 2014, 72: 93–114. doi: 10.1016/j.jmps.2014.07.013 [19] 蔡正宇, 丁圆圆, 王士龙, 等. 梯度多胞牺牲层的抗爆炸分析 [J]. 爆炸与冲击, 2017, 37(3): 396–404.CAI Z Y, DING Y Y, WANG S L, et al. Anti-blast analysis of graded cellular sacrificial cladding [J]. Explosion and Shock Waves, 2017, 37(3): 396–404. [20] 常白雪, 郑志军, 赵凯, 等. 梯度多胞材料耐撞性设计的简化模型和渐近解 [J]. 中国科学: 物理学 力学 天文学, 2018, 48(9): 233–241.CHANG B X, ZHENG Z J, ZHAO K, et al. A simplified model and its asymptotic solution for the crashworthiness design of graded cellular material [J]. Scientia Sinica: Physica Mechanica & Astronomica, 2018, 48(9): 233–241. [21] 常白雪, 郑志军, 赵凯, 等. 具有恒定冲击载荷的梯度泡沫金属材料设计 [J]. 爆炸与冲击, 2019, 39(4): 3–11.CHANG B X, ZHENG Z J, ZHAO K, et al. Design of gradient foam metal materials with a constant impact load [J]. Explosion and Shock Waves, 2019, 39(4): 3–11. [22] DING Y Y, WANG S L, ZHAO K, et al. Blast alleviation of cellular sacrificial cladding: a nonlinear plastic shock model [J]. International Journal of Applied Mechanics, 2016, 8(4): 1650057. doi: 10.1142/S1758825116500575 [23] AJDARI A, NAYEB-HASHEMI H, VAZIRI A. Dynamic crushing and energy absorption of regular, irregular and functionally graded cellular structures [J]. International Journal of Solids and Structures, 2011, 48(3/4): 506–516. [24] FAN J H, ZHANG J J, WANG Z H, et al. Dynamic crushing behavior of random and functionally graded metal hollow sphere foams [J]. Materials Science and Engineering A, 2013, 561: 352–361. doi: 10.1016/j.msea.2012.10.026 [25] ZHANG J J, WANG Z H, ZHAO L M. Dynamic response of functionally graded cellular materials based on the Voronoi model [J]. Composites Part B: Engineering, 2015, 85(1): 176–187. [26] 王礼立. 应力波基础 [M]. 北京: 国防工业出版社, 2005.WANG L L. Foundation of stress wave [M]. Beijing: National Defence Industry Press, 2005. [27] ZHU H X, HOBDELL J R, WINDLE A H. Effects of cell irregularity on the elastic properties of 2D Voronoi honeycombs [J]. Journal of the Mechanics and Physics of Solids, 2001, 49(4): 857–870. doi: 10.1016/S0022-5096(00)00046-6 [28] 张建军. 冲击载荷下梯度多孔金属力学行为研究 [D]. 太原: 太原理工大学, 2016.ZHANG J J. Investigations of mechanical behaviors of graded cellulartals subjected to impact loading [D]. Taiyuan: Taiyuan University of Technology, 2016. [29] YANG J, WANG S L, DING Y Y, et al. Crashworthiness of graded cellular materials: a design strategy based on a nonlinear plastic shock model [J]. Materials Science and Engineering A, 2017, 680(1): 411–420. [30] 王根伟, 王江龙. 负梯度闭孔泡沫金属的力学性能分析 [J]. 固体力学学报, 2017(1): 88–95.WANG G W, WANG J L. Mechanical properties of closed-cell metal foam with negative density gradient under impact load [J]. Chinese Journal of Solid Mechanics, 2017(1): 88–95. [31] LI Q M, MAGKIRIADIS I, HARRIGAN J J. Compressive strain at the onset of densification of cellular solids [J]. Journal of Cellular Plastics, 2006, 42(5): 371–392. doi: 10.1177/0021955X06063519 [32] WANG J L, LI X, WANG G W. Deformation modes of the graded closed-cell foam under impact loading [C]//2016 International Conference on Applied Mechanics, Electronics and Mechatronics Engineering. Beijing: DEStech Publications, 2016. [33] ZHENG Z J, LIU Y D, YU J L, et al. Dynamic crushing of cellular materials: continuum-based wave models for the transitional and shock modes [J]. International Journal of Impact Engineering, 2012, 42: 66–79. doi: 10.1016/j.ijimpeng.2011.09.009 -







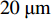
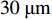

 下载:
下载:

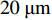
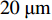

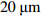
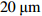


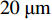
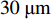
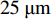


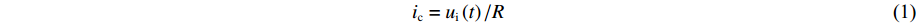
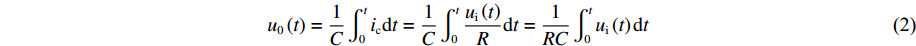
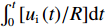
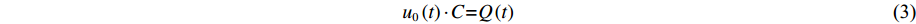


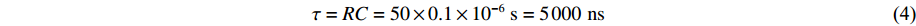

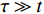



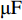


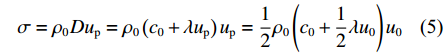
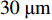

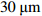
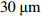
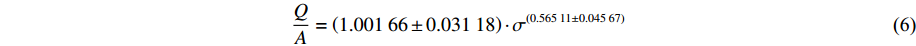


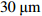
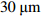
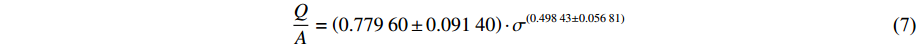
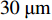
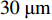

 下载:
下载: