A Macroscopic Dynamic Constitutive Model for Ceramic Materials
doi: 10.11858/gywlxb.20190863
-
Abstract: A macroscopic constitutive model is presented herein for ceramic materials subjected to dynamic loadings by closely following a previous study on concrete. The equation of state is described by a polynomial equation and the strength model takes into account various effects such as pressure hardening, Lode angle, strain rate, shear damage and tensile softening. In particular, the strength surface of ceramic materials is characterized by a new function which levels out at very high pressures and strain rate effect is taken into account by dynamic increase factor (DIF) which excludes inertial effect. The present model is verified against some available experimental data for ceramic materials in terms of pressure-volumetric response, quasi-static strength surface and strain rate effect. The model is further verified against the data for triaxial test by single element simulation approach and the test data for depth of penetration in AD99.5/RHA struck by tungsten alloy penetrators. Furthermore, comparisons are also made between numerical results of the present model and the JH-2 model. It is demonstrated that the present model can be employed to describe the mechanical behavior of ceramic materials under different loading conditions with reasonable confidence and is advantageous over the existing model.摘要: 基于已有混凝土材料的相关研究,建立了陶瓷材料在动态载荷作用下的宏观本构模型。模型中状态方程采用多项式描述,强度面模型中考虑了压力相关性、Lode角效应、应变率效应、剪切损伤及拉伸软化等的影响。采用一个新的函数来描述陶瓷材料的强度面,其在较高压力下趋于一个平台值;并采用动态增强因子(DIF)考察剥除惯性效应后陶瓷材料的真实应变率效应。通过将模型预测的压力-体应变响应、准静态强度面以及应变率效应与相关实验数据进行对比,验证了该模型。单个单元测试模拟得到的结果与三轴实验数据以及侵彻实验数据高度吻合,进一步验证了此模型。为显示模型的优越性,还与JH-2模型的预测结果进行了比较。结果表明:所提出的本构模型能够很好地预测陶瓷材料在不同加载条件下的力学行为,且优于现有的模型。
-
复合材料板具有较高的强度质量比、良好的耐腐蚀性和优异的可设计性,被广泛应用于航空航天和工业制造等领域[1]。在实际使用中复合材料板经常受到不同形式的冲击荷载,从而产生振动和屈曲问题,因此冲击载荷作用下复合材料板的动力稳定性问题备受关注。
近年来关于复合材料板的研究越来越多,尤其是冲击荷载作用下复合材料板的动力稳定性问题研究[2],对工程部件结构设计和使用具有重要的意义。Sun等[3]研究了在加热环境中应力波对功能梯度圆柱壳轴向冲击屈曲的影响;毛柳伟等[4]对弹性直杆在应力波作用下的动力分叉屈曲进行了分析与探讨,提出了求解应力波作用下直杆动力屈曲的数值方法;Lepik[5]讨论了在应力波影响下轴向压缩的弹塑性梁的屈曲;Zhang等[6]分析了不确定初始几何缺陷对薄板屈曲的影响;Abdelaziz等[7]利用双曲线剪切变形理论,分析了在各种边界条件下复合材料板的弯曲变形和屈曲;Kouchakzadeh等[8]采用线性和旋转弹簧的均匀分布来模拟边界条件,对矩形层压复合板的屈曲进行了分析;Czapski等[9]通过数值和实验方法,研究了残余应力对压缩至破坏期间薄壁层压板屈曲性能的影响。
在实际工程中,复合材料板多应用于振动环境,其在动力响应下的动态特性和振动分析是必不可少的,因而对该类材料的振动屈曲研究至关重要。Kuo[10]研究了两种非均匀分布纤维复合材料板的振动屈曲问题,Villarreal等[11]对典型正交异性板的本征频率和振动屈曲进行了理论分析,Eftekhari等[12]提出了通过组合应用有限元方法和微分正交方法求解矩形板的振动屈曲问题,Rehman等[13]探讨了壳体结构的缺陷和损坏对结构振动屈曲的影响,Sayyad等[14]将三角剪切变形理论应用于复合板的变形和振动屈曲研究。
关于复合材料板的振动屈曲问题已开展了较多的研究,但大多未考虑应力波效应对振动屈曲的影响,而动力屈曲一般与应力波相联系且具有局部发生的特点,研究含初始缺陷的复合材料板能更好地揭示实际工程中复合材料板在不同工况下发生动力屈曲的机理。基于此,本研究利用Kirchhoff薄板理论和Hamilton原理,考虑应力波效应,建立含初始几何缺陷的四边简支复合材料板的振动控制方程,得到板的屈曲临界荷载表达式,在此基础上通过数值计算讨论初始几何缺陷、振型函数初相位、铺层角度、屈曲模态阶数和铺层层数对复合材料板振动屈曲临界荷载的影响,为工程实际提供理论依据。
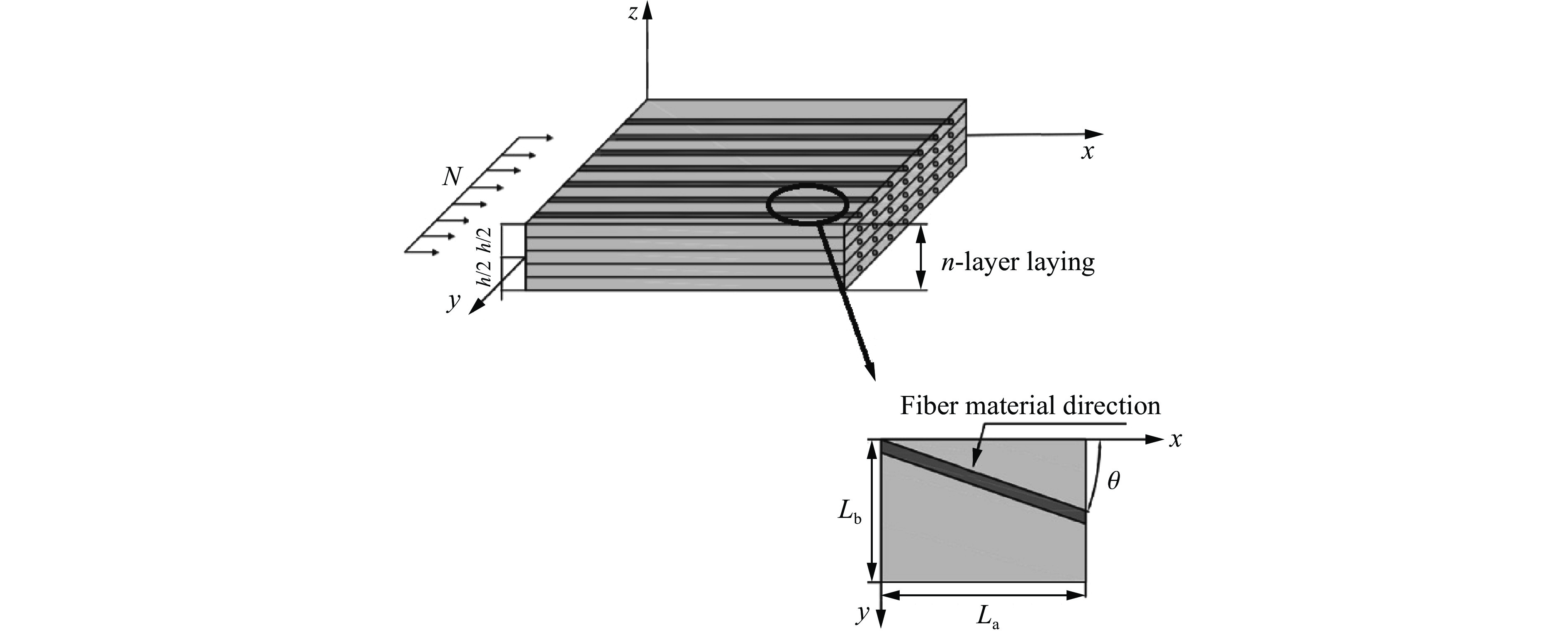
1. 复合材料板的控制方程
复合材料板在x = La处为固定边界条件,其余3边为简支边界条件,在
z = 0的中性面上受x方向的面内阶跃荷载N作用,如图1所示。板在z 方向上含初始几何缺陷w1,且x、y、z 方向的位移分别为u、v、w。复合材料板的长度、宽度和厚度分别为La、Lb、h,由n层单层板组成,θ 为单层板的铺层角度,即纤维材料铺设方向与x方向的夹角(见图1)。根据Kirchhoff薄板理论及经典弹性理论,复合材料板的位移与应变、弹性曲面的曲率和扭率的表达式为
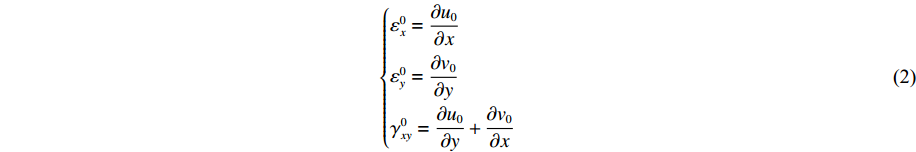
{w=w1+w0u=u0−z∂(w−w1)∂xv=v0−z∂(w−w1)∂y (1) {ε0x=∂u0∂xε0y=∂v0∂yγ0xy=∂u0∂y+∂v0∂x (2) {κx=−∂2(w−w1)∂x2κy=−∂2(w−w1)∂y2κxy=−2∂2(w−w1)∂x∂y (3) {εx=ε0x+zκxεy=ε0y+zκyγxy=γ0xy+zκxy (4) 式中:u0、v0、w0分别为复合材料板在x、y、
z 方向上的中面位移,ε0x 、ε0y 、γ0xy 分别为复合材料板中面应变分量,κx 、κy 、κxy 为中面的曲率和扭率,εx 、εy 、γxy 为复合材料板任意一点的应变。板的内力(Nx、Ny、Nxy)与内力矩(Mx、My、Mxy)之间的关系为
[NxNyNxyMxMyMxy]=[A11A12A16B11B12B16A12A22A26B12B22B26A16A26A66B16B26B66B11B12B16D11D12D16B12B22B26D12D22D26B16B26B66D16D26D66][ε0xε0yγ0xyκxκyκxy] (5) 式中:
Aij 、Bij 、Dij(i,j=1,2,6) 分别表示板的拉伸刚度、耦合刚度和弯曲刚度系数[15],表达式如下{Aij=n∑k=1¯Qkij(hk−hk−1)Bij=12n∑k=1¯Qkij(h2k−h2k−1)Dij=13n∑k=1¯Qkij(h3k−h3k−1) (6) 式中:
¯Qkij 为复合材料板第k 层的偏轴刚度系数¯Q=P−1Q(P−1)T (7) 式中:P为坐标转换矩阵,Q为刚度矩阵。
P=[cos2θsin2θ2sinθcosθsin2θcos2θ−2sinθcosθ−sinθcosθsinθcosθcos2θ−sin2θ] (8) Q=[Q11Q120Q12Q22000Q66] (9) 考虑材料为正交各向异性材料,设E1、E2、G12、μ12、μ21分别为板材料x和y方向的拉压弹性模量、剪切弹性模量、主泊松比和副泊松比,则有
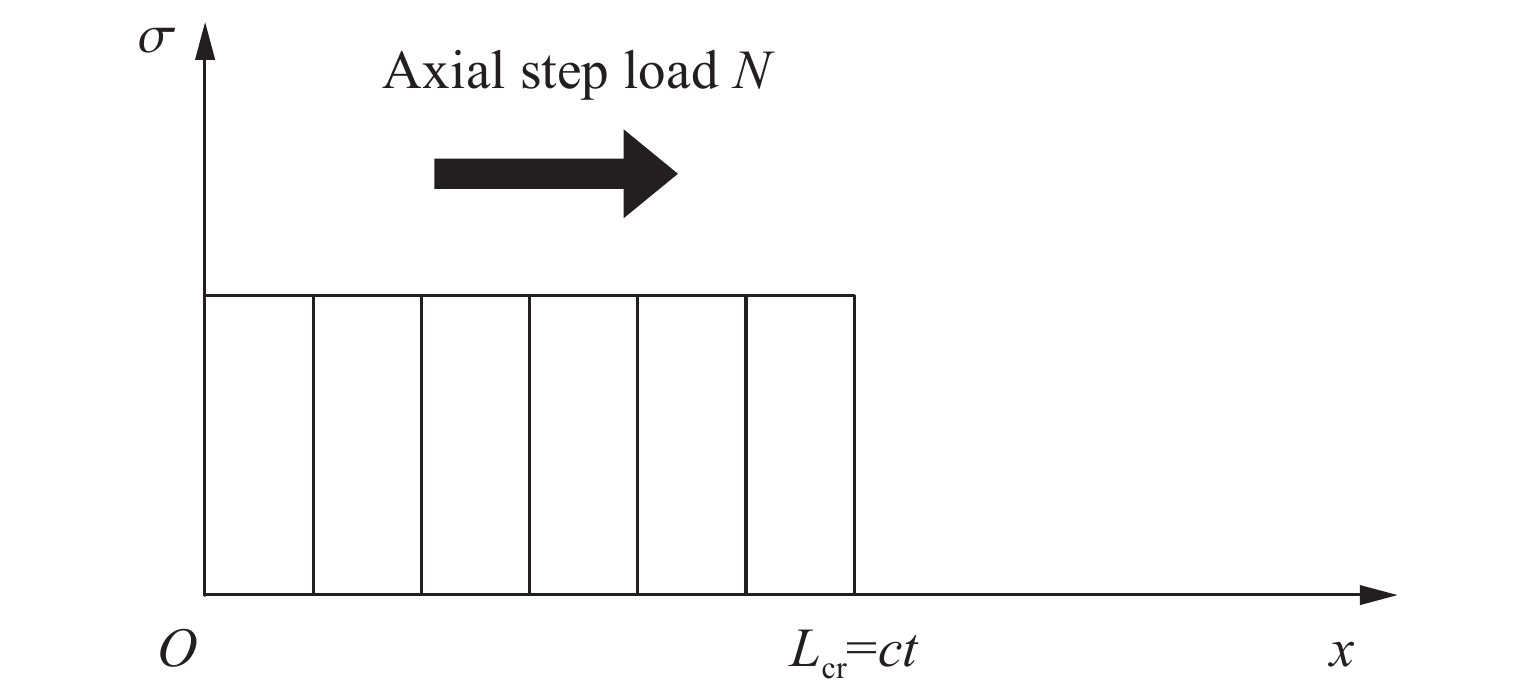
Q11=E11−μ12μ21,Q22=E21−μ12μ21,Q12=μ12E21−μ12μ21=μ21E11−μ12μ21,Q66=G12 (10) 复合材料板在左端受面内冲击荷载N作用(见图1)时,应力波沿x方向在板内传播,其应力变化如图2所示。
当应力波传播至波阵面位置Lcr(临界长度)时,板发生振动屈曲,板的内力Nt和应力波波速c分别表示为
Nt={N0⩽x⩽Lcr0x>Lcr,c=√A11ρh (11) 板发生振动屈曲时的变形能可以表示为
U=12∫Lcr0∫Lb0(Nxε0x+Nyε0y+Nxyγ0xy+Mxκx+Myκy+Mxyκxy)dxdy=12∫Lcr0∫Lb0[Nx∂u0∂x+Ny∂v0∂y+Nxy(∂u0∂x+∂v0∂y)+Mx(−∂2w0∂x2)+My(−∂2w0∂y2)+Mxy(−2∂2w0∂x∂y)]dxdy (12) 发生振动屈曲时的动能(考虑转动惯量)可以表示为
T=12∫h/2−h/2∫Lcr0∫Lb0ρ(k)[(∂u∂t)2+(∂v∂t)2+(∂w0∂t)2]dxdydz=12∫Lcr0∫Lb0[I0(∂u0∂t)2+I0(∂v0∂t)2+I0(∂w0∂t)2−2I1∂u0∂t∂2w0∂x∂t−2I1∂v0∂t∂2w0∂y∂t+I2(∂2w0∂x∂t)2+I2(∂2w0∂y∂t)2]dxdy (13) 式中:
(I0,I1,I2)=Nk∑k=1∫hkhk−1ρ(k)(1,z,z2)dz ,ρ(k) 为第k层材料的密度。板发生振动屈曲时的外力功可以表示为
W=12∫Lcr0∫Lb0Nt(∂w∂x)2dxdy (14) 考虑Hamilton变分原理,即
δ∫t2t1(T−U+W)dt=0 (15) 将式(12)~式(14)代入式(15)并进行变分计算,可得
∂Nx∂x+∂Nxy∂x−I0∂2u0∂t2+I1∂3w0∂x∂t2=0 (16) ∂Ny∂y+∂Nxy∂y−I0∂2v0∂t2+I1∂3w0∂y∂t2=0 (17) ∂2Mx∂x2+∂2My∂y2−2∂2Mxy∂x∂y−Nt(∂2w0∂x2+∂2w1∂x2)=I0∂2w0∂t2+I1(∂3u0∂x∂t2+∂3v0∂x∂t2)−I2(∂4w0∂x2∂t2+∂4w0∂y2∂t2) (18) 对于正交各向异性正规对称正交铺设的复合材料板,其刚度矩阵满足[16]
D16=D26=0,A16=A26=0,Bij=0 (19) 根据 Kirchhoff 薄板理论及经典弹性理论,薄板中面在变形过程中没有伸长变形,将板的本构关系代入式(16)~式(18)中,略去含
u0 和v0 的项,得到复合材料板在面向阶跃荷载激励下的控制方程D11∂4w0∂x4+(2D12+4D66)∂4w0∂x2∂y2+D22∂4w0∂y4+Nt(∂2w0∂x2+∂2w1∂x2)−I0∂2w0∂t2+I2(∂4w0∂x2∂t2+∂4w0∂y2∂t2)=0 (20) 设三边简支和应力波传播到Lcr(
w0(Lcr,y,t)=w′0(Lcr,y,t)=0 )时的振型函数[17]为w0(x,y,t)=Rij[siniπxLcr+ii+1sin(i+1)πxLcr]sinjπyLbsin(ωt+φ) (21) 由屈曲模态确定的缺陷分布形式是板结构最有可能发生的屈曲形式,能够很好地确定结构的缺陷敏感性[18]。对于复合材料板在制造过程中出现的初始几何缺陷,引入屈曲模态的
ε 倍变形作为初始几何缺陷[19],可以表示为w1(x,y)=εRij[siniπxLcr+ii+1sin(i+1)πxLcr]sinjπyLb (22) 式中:i、j为屈曲模态阶数,
i,j=1,2,3,⋯ ;Rij为板的第(i, j)阶模态幅值;ε 表示初始几何缺陷系数。根据式(21)和式(22),利用棣莫弗公式对控制方程式(20)中的各项进行求导并化简,得到
D11(πLcr)4[i4+(i+1)4]sin(ωt+φ)+(2D12+4D66)(πLcr)2[i2+(i+1)2](jπLb)2sin(ωt+φ)+2D22(jπLb)4sin(ωt+φ)−Nt(πLcr)2[i2+(i+1)2][sin(ωt+φ)+ε]+2I0ω2sin(ωt+φ)+I2ω2{(πLcr)2[i2+(i+1)2]+2(jπLb)2}sin(ωt+φ)=0 (23) 根据式(23)可以得到N的表达式
N={D11(πLcr)4[i4+(i+1)4]+(2D12+4D66)(πLcr)2[i2+(i+1)2](jπLb)2+2D22(jπLb)4+2I0ω2+I2ω2{(πLcr)2[i2+(i+1)2]+2(jπLb)2}}sin(ωt+φ)(πLcr)2[i2+(i+1)2][sin(ωt+φ)+ε] (24) 板发生屈曲时,临界条件为
ω=0 [20],代入式(24)可得振动屈曲临界荷载为Ncr=D11(πLcr)2[i4+(i+1)4]sinφ[i2+(i+1)2](sinφ+ε)+(2D12+4D66)(jπLb)2sinφ(sinφ+ε)+2D22(j2πL2b)2L2crsinφ[i2+(i+1)2](sinφ+ε) (25) 2. 算例分析
利用MATLAB数值分析应力波未反射时初始几何缺陷、初相位、铺层角度、屈曲模态阶数、铺设厚度以及铺层层数对复合材料板振动屈曲临界荷载的影响,使用的材料参数见表1[21]。
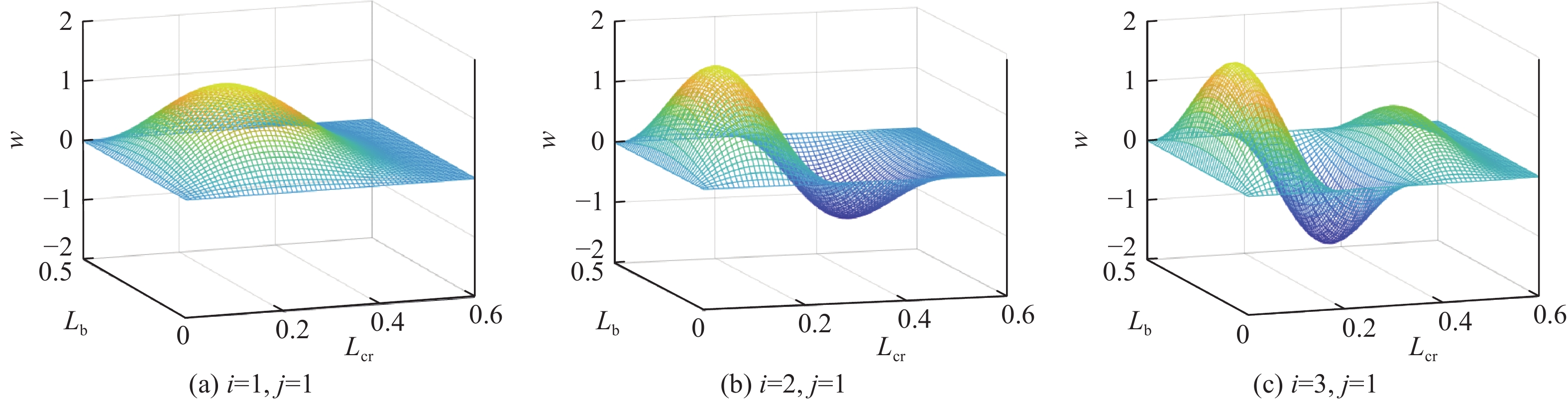
图3显示了复合材料板的模态阶数i为1、2、3,j为1时的屈曲模态。当板的x方向模态增大时,x方向的屈曲模态第一峰值增大且波数增加,而y方向的屈曲模态呈正对称分布。模态阶数的增加使板振动的屈曲模态变得更复杂。
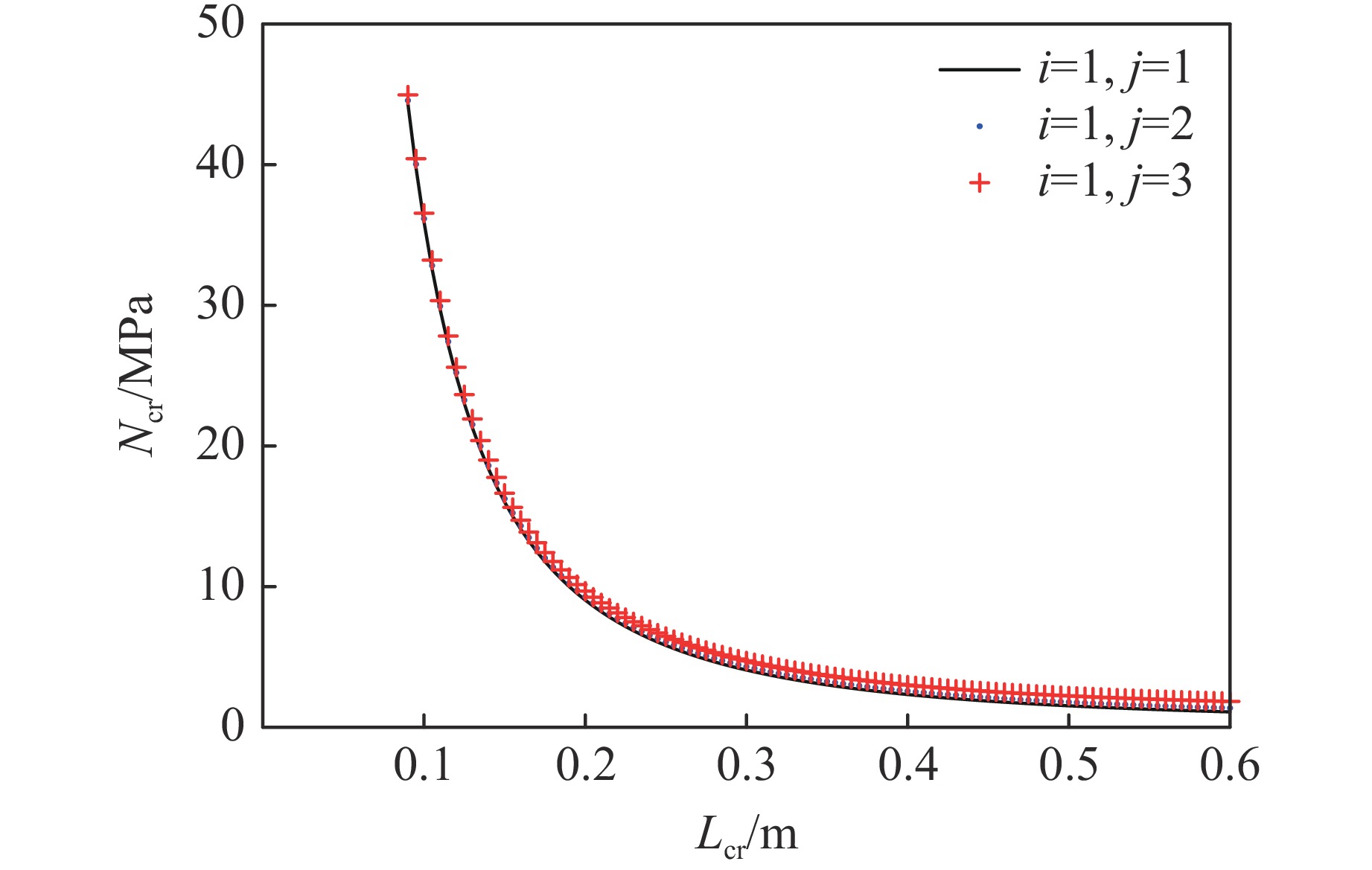
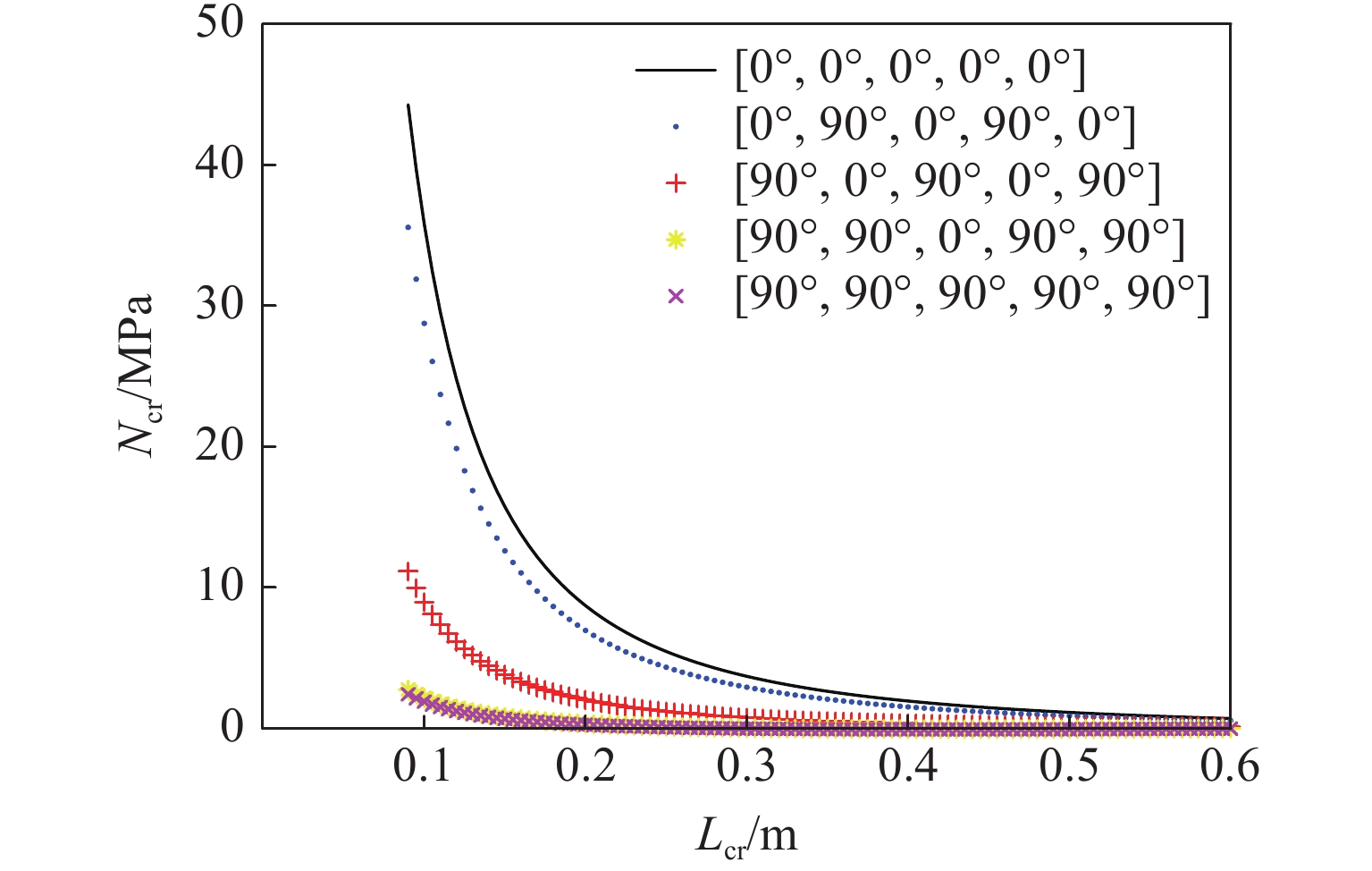
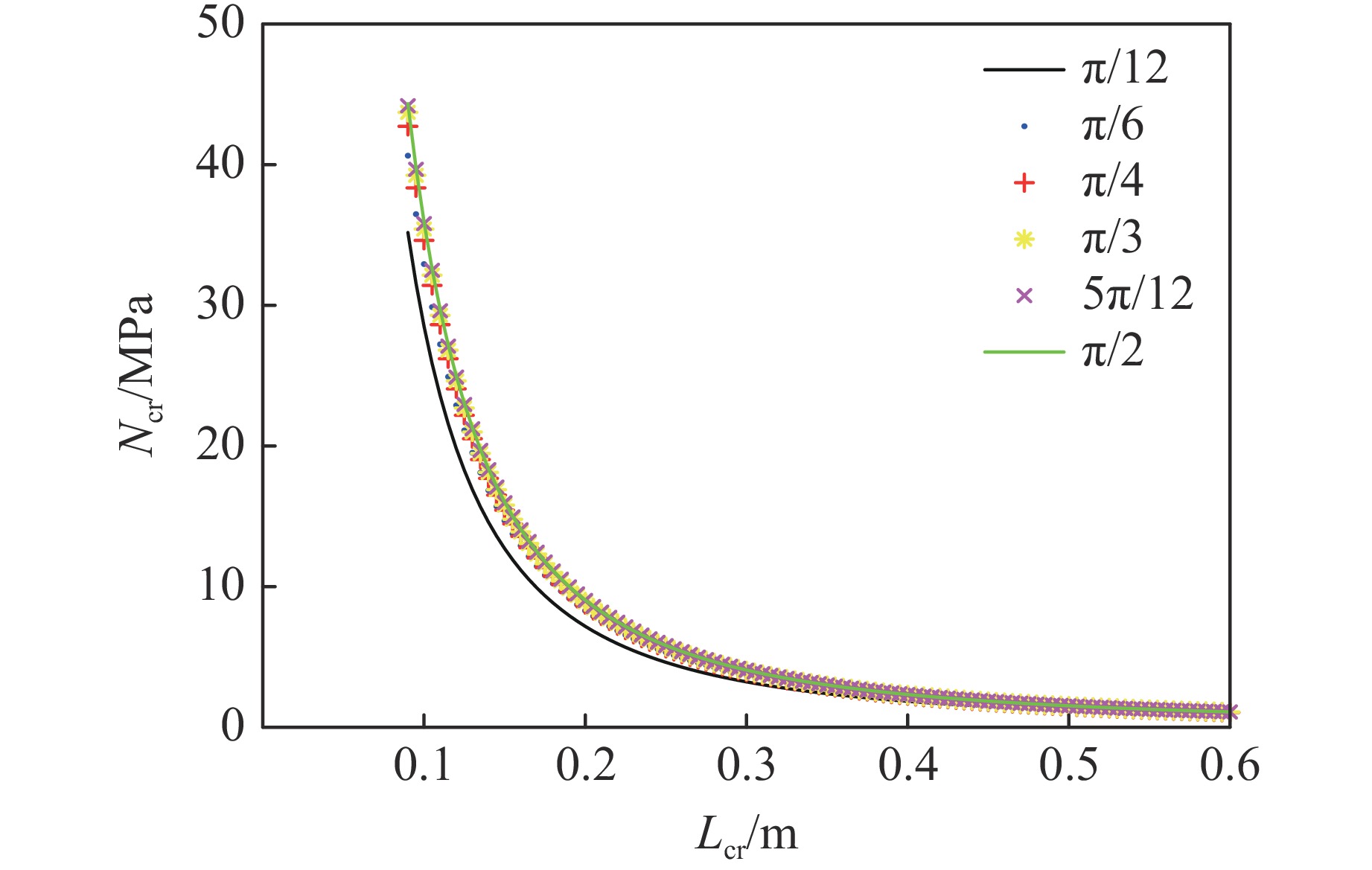
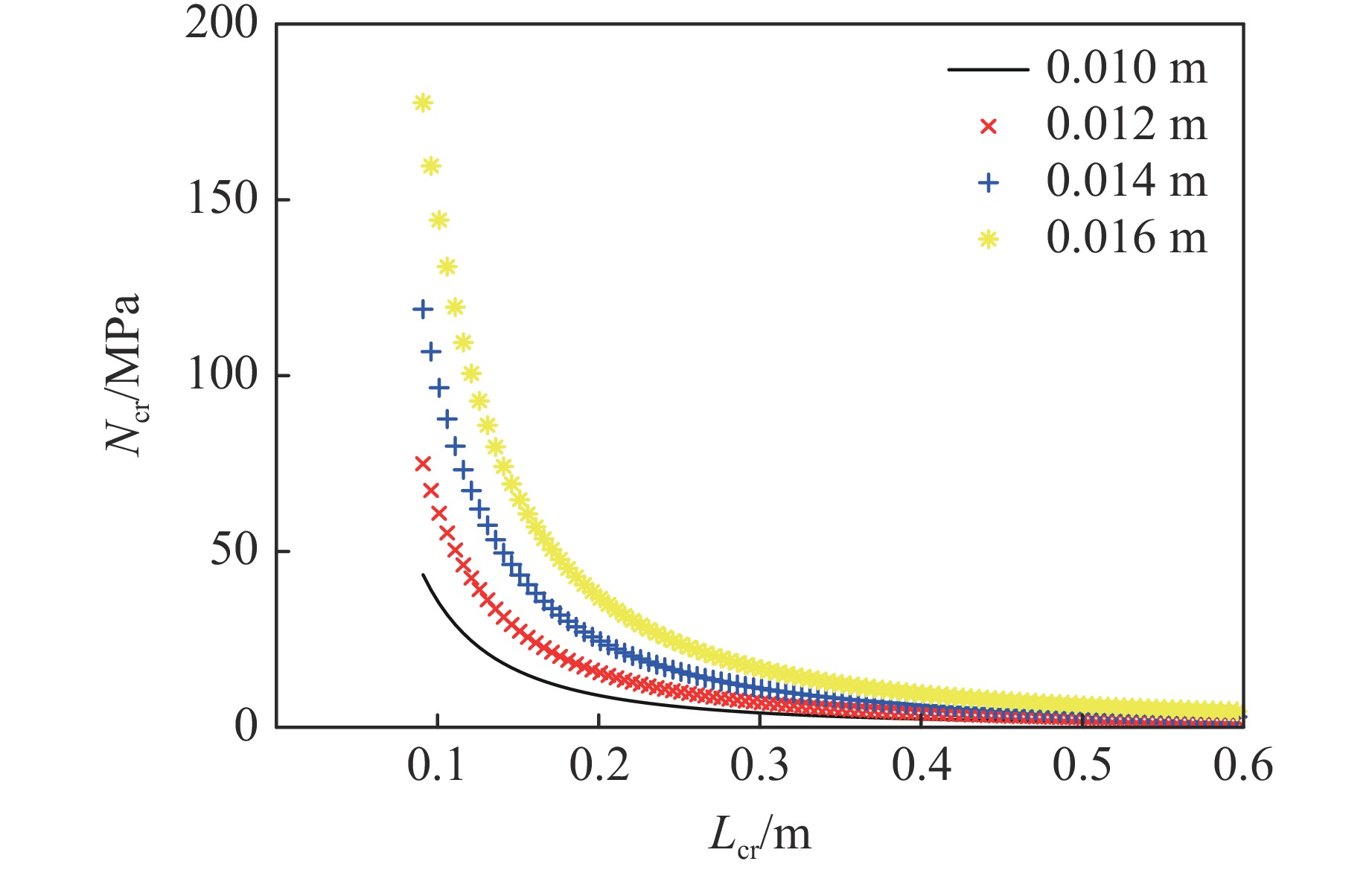
设置7组算例,分别以初始几何缺陷、初相位、铺层角度、x和y两个方向屈曲模态阶数、铺层层数及铺设厚度为变量进行算例分析,研究以上因素对板振动屈曲临界荷载的影响,算例参数见表2。
表 2 算例分析参数表Table 2. Example analysis parameter tableGroup Initial defect
coefficientOrder of mode Laying angle/(°) Initial phase Number of
layers laidThickness
of the plate/mx direction y direction A Variable i = 1 j = 1 [0, 0, 0, 0, 0] π/2 5 0.01 B 0.1 Variable j = 1 [0, 0, 0, 0, 0] π/2 5 0.01 C 0.1 i =1 Variable [0, 0, 0, 0, 0] π/2 5 0.01 D 0.1 i =1 j = 1 Variable π/2 5 0.01 E 0.1 i =1 j = 1 [0, 0, 0, 0, 0] Variable 5 0.01 F 0.1 i =1 j = 1 [0, 0, 0, 0, 0] π/2 Variable 0.01 G 0.1 i =1 j = 1 [0, 0, 0, 0, 0] π/2 5 Variable 将A组数据代入式(25)中,可以得到不同初始缺陷系数对复合材料板振动屈曲的影响,如图4所示。由Lcr-Ncr曲线可知:在应力波传播过程中,Ncr呈指数下降,分为敏感区和非敏感区。应力波在Lcr < 0.4 m区域传播时,Ncr的变化较陡峭,该区域为敏感区;应力波在Lcr > 0.4 m区域传播时,Ncr的变化趋于平缓,该区域为非敏感区,因此敏感分界点为0.4 m。当选取的初始缺陷系数增大时,临界荷载Ncr也随之增大。在敏感区,初始缺陷系数对临界荷载Ncr的影响较大,且随应力波传播呈减小趋势。此外,初始缺陷系数对非敏感区的影响较小。图4表明,初始几何缺陷系数越大,板越容易发生屈曲。
将B组数据代入式(25)中,可以得到不同x方向模态阶数对复合材料板振动屈曲的影响,如图5所示。由Lcr-Ncr曲线可知:当选取的x方向模态阶数增大时,临界荷载Ncr随之明显增大。在敏感区,x方向模态阶数对临界荷载Ncr的影响很大,并随应力波的传播不断减小,到达非敏感区之后影响较小并趋于稳定。图5表明,x方向模态阶数的增加会显著增大板的屈曲临界荷载。
将C组数据代入式(25)中,可以得到不同的y方向模态阶数对复合材料板振动屈曲的影响,如图6所示。由Lcr-Ncr曲线可知:当选取的y方向模态阶数增大时,临界荷载Ncr也随之变大。在应力波传播过程中,在敏感区y方向模态阶数对临界载荷基本没有影响,而在非敏感区有极小的影响。图6表明,y方向模态阶数的变化对板屈曲临界荷载基本没有影响。
将D组数据代入式(25)中,得到不同铺层角度对复合材料板振动屈曲的影响,如图7所示。由Lcr-Ncr曲线可知:在敏感区,不同的铺设角度对临界荷载Ncr的影响较大,且随应力波的传播不断减小,到达非敏感区后趋于稳定。图7表明,铺层角度小的单层板的层数越多,板的临界屈曲荷载越大,说明复合材料板的铺设角度直接影响板的屈曲临界荷载。
将E组数据代入式(25)中,得到不同初相位对复合材料板振动屈曲的影响,如图8所示。由Lcr-Ncr曲线可知:振型函数的初相位越大,对应的临界荷载越大。在敏感区,振型函数的初相位对临界荷载Ncr的影响较小,且随应力波的传播不断减小;到达非敏感区之后,影响趋于平缓。图8表明,振型函数的初相位越大,板的屈曲临界荷载越大。
将F组数据代入式(25)中,得到不同铺层层数对复合材料板振动屈曲的影响,如图9所示。由Lcr-Ncr曲线可知:当按照不同层数铺设时,敏感区的临界荷载Ncr的变化较大,且随应力波的传播不断减小;到达非敏感区之后变化较小并趋于平缓。图9表明,对于厚度固定且对称铺设的板,当铺设层数达到7时,其屈曲荷载随层数增加趋于稳定。
将G组数据代入式(25)中,得到不同铺设厚度对复合材料板振动屈曲的影响,如图10所示。由Lcr-Ncr曲线可知:板的铺设厚度越大,对应的临界荷载越大。在敏感区,不同的板厚对临界荷载Ncr的影响很大,且随应力波的传播不断减小,到达非敏感区后趋于稳定。图10表明,复合材料板的铺设厚度将直接决定板的屈曲临界荷载。
3. 结 论
基于Kirchhoff薄板理论和Hamilton变分原理,建立了具有初始几何缺陷的四边简支复合材料板的振动控制方程。采用伽辽金法,选取符合边界条件的振型函数求解控制方程,得到屈曲临界载荷表达式。数值计算结果表明:应力波在未发生反射前的传播过程中,复合材料板的振动屈曲临界载荷随着临界长度的增大、铺设厚度的减小、初始几何缺陷系数的增大、振型函数初相位的减小而减小;复合材料板的各层铺层角度与荷载作用方向的夹角越小,屈曲临界载荷越大,当对称铺设层数达7层时,临界荷载趋于稳定。研究结果可为工程中复合材料板的结构设计与应用提供一定的参考。
-
Figure 5. Comparison between the experimental data[25] and the predictions by the present model of strength varies with pressure at different strain rates of AlN
Figure 6. Comparison of stress-strain curves for BeO under triaxial compression between the present model and experimental data[22]
Figure 11. Comparison between the numerical results and the test data for the depth of penetration in AD99.7/RHA targets by flat-nosed tungsten alloy penetrators[35]
Table 1. Values of parameters for BeO in the present model
Equation of state parameters Constitutive model parameters K1/GPa K2/GPa K3/GPa ρc/(kg·m−3) Fm Wx Wy S 181.5 1 207.9 −2 991 3 030 3 3.8 2 1.25 Constitutive model parameters f′c/GPa ft/GPa B G/GPa λm λs l r 1.5 0.15 1.2 125 0.3 7.5 0.8 0.3 Table 2. Values of parameters for AlN in the present model
Equation of state parameters Constitutive model parameters ρc/(kg·m−3) K1/GPa K2/GPa K3/GPa K4/GPa K5/GPa K6/GPa Fm Wx Wy 3 229 181.5 1 207.9 −2 991 181.9 335.6 −283 3 3.8 2 Constitutive model parameters f′c/GPa ft/GPa B G/GPa S λm λs l r 3 0.3 1.7 127 1.25 0.3 7.5 0.8 0.3 Table 3. Values of various parameters for AlN ceramic (JH-2 model)
Equation of state parameters Constitutive model parameters ρc/(kg·m−3) K1/GPa K2/GPa K3/GPa a b C n m 3 229 201 260 0 1.36 1.0 0.013 0.75 0.65 Constitutive model parameters HEL/GPa pHEL/GPa σHEL/GPa μHEL T/GPa β d1 d2 9.0 5.0 6.0 0.024 2 0.32 1.0 0.02 1.85 Table 4. Values of various parameters for AD99.7 ceramic[35] (The present model)
Equation of state parameters Constitutive model parameters ρc/(kg·m−3) K1/GPa K1/GPa K3/GPa Fm Wx Wy S 3 809 181.5 1 207.9 −2 991 3 3.8 2 1.25 Constitutive model parameters f′c/GPa ft/GPa B G/GPa λm λs l r 3 0.3 1.4 135 0.3 7.5 0.8 0.3 Table 5. Values of various parameters for tungsten alloy[35] (JC model)
Constitutive model parameters ρc/(kg·m−3) G/GPa A1/GPa B1/GPa N1 C1 M1 ˙ε0/s−1 17 600 122 1.506 0.177 0.12 0.016 1.0 1.0 Constitutive model parameters cp/(J·kg−1·K−1) Tm/K Tr/K D1 D2 D3 D4 D5 134 1 723 300 2.0 0 0 0 0 Equation of state parameters Cs/(m·s−1) S1 S2 S3 γ0 A0 4 029 1.23 0 0 1.54 0.4 Table 6. Values of various parameters for RHA[35] (JC model)
Constitutive model parameters ρc/(kg·m−3) G/GPa A1/GPa B1/GPa N1 C1 M1 ˙ε0/s−1 7 800 77 0.792 0.51 0.26 0.014 1.03 1.0 Constitutive model parameters cp/(J·kg−1·K−1) Tm/K Tr/K D1 D2 D3 D4 D5 477 1 793 294 0.05 3.44 −2.12 0.002 0.61 Equation of state parameters Cs/(m·s−1) S1 S2 S3 γ0 A0 4 569 1.49 0 0 2.17 0.460 -
[1] FAHRENTHOLD E P. A continuum damage model for fracture of brittle solids under dynamic loading [J]. Journal of Applied Mechanics, 1991, 58(4): 904–909. doi: 10.1115/1.2897704 [2] RAJENDRAN A M. Modeling the impact behavior of AD85 ceramic under multiaxial loading [J]. International Journal of Impact Engineering, 1994, 15(6): 749–768. doi: 10.1016/0734-743X(94)90033-H [3] JOHNSON G R, HOLMQUIST T J. An improved computational constitutive model for brittle materials [J]. High Pressure Science and Technology, 2008, 309(1): 981–984. [4] SIMHA C H, BLESS S, BEDFORD A, et al. Computational modeling of the penetration response of a high-purity ceramic [J]. International Journal of Impact Engineering, 2002, 27(1): 65–86. doi: 10.1016/S0734-743X(01)00036-7 [5] RAVICHANDRAN G, SUBHASH G. A micromechanical model for high strain rate behavior of ceramics [J]. International Journal of Solids and Structures, 1995: 2627–2646. [6] ESPINOSA H D. On the dynamic shear resistance of ceramic composites and its dependence on applied multiaxial deformation [J]. International Journal of Solids and Structures, 1995, 32(21): 3105–3128. doi: 10.1016/0020-7683(94)00300-L [7] ESPINOSA H D, XU Y, BRAR N S. Micromechanics of failure waves in glass: Ⅱ, modeling [J]. Journal of the American Ceramic Society, 1997, 80(8): 2074–2085. [8] STEINBERG D J. Computer studies of the dynamic strength of ceramics [M]//Shock Waves. Berlin: Springer, 1992: 415–422. [9] XU H, WEN H M. A computational constitutive model for concrete subjected to dynamic loadings [J]. International Journal of Impact Engineering, 2016, 91: 116–125. doi: 10.1016/j.ijimpeng.2016.01.003 [10] XU H, WEN H M. Semi-empirical equations for the dynamic strength enhancement of concrete-like materials [J]. International Journal of Impact Engineering, 2013, 60: 76–81. doi: 10.1016/j.ijimpeng.2013.04.005 [11] ZHAO F Q, WEN H M. A comment on the maximum dynamic tensile strength of a concrete-like material [J]. International Journal of Impact Engineering, 2018, 115: 32–35. doi: 10.1016/j.ijimpeng.2018.01.009 [12] ZHAO F Q, WEN H M. Effect of free water content on the penetration of concrete [J]. International Journal of Impact Engineering, 2018, 121: 180–190. doi: 10.1016/j.ijimpeng.2018.06.007 [13] BRIDGMAN P W. Linear compressions to 30 000 kg/cm2, including relatively incompressible substances [J]. Proceedings of the American Academy of Arts and Sciences, 1949, 77(6): 189–234. doi: 10.2307/20023541 [14] HART H V, DRICKAMER H G. Effect of high pressure on the lattice parameters of Al2O3 [J]. Journal of Chemical Physics, 1965, 43(7): 2265–2266. doi: 10.1063/1.1697121 [15] SATO Y, AKIMOTO S. Hydrostatic compression of four corundum-type compounds: α-Al2O3, V2O3, Cr2O3, and α-Fe2O [J]. Journal of Applied Physics, 1979, 50(8): 5285–5291. doi: 10.1063/1.326625 [16] BASSETT W A, WEATHERS M S, WU T C, et al. Compressibility of SiC up to 68.4 GPa [J]. Journal of Applied Physics, 1993, 74(6): 3824–3826. doi: 10.1063/1.354476 [17] ROSENBERG Z, BRAR N S, BLESS S J. Dynamic high-pressure properties of AlN ceramic as determined by flyer plate impact [J]. Journal of Applied Physics, 1991, 70(1): 167–171. doi: 10.1063/1.350337 [18] XIA Q, XIA H, RUOFF A L. Pressure induced rocksalt phase of aluminum nitride: a metastable structure at ambient condition [J]. Journal of Applied Physics, 1993, 73(12): 8198–8200. doi: 10.1063/1.353435 [19] UENO M, ONODERA A, SHIMOMURA O, et al. X-ray observation of the structural phase transition of aluminum nitride under high pressure [J]. Physical Review B, 1992, 45(17): 10123. doi: 10.1103/PhysRevB.45.10123 [20] ROSENBERG Z, YAZIV D, YESHURUN Y, et al. Shear strength of shock-loaded alumina as determined with longitudinal and transverse manganin gauges [J]. Journal of Applied Physics, 1987, 62(3): 1120–1122. doi: 10.1063/1.339721 [21] BOURNE N K, MILLETT J, PICKUP I, et al. Delayed failure in shocked silicon carbide [J]. Journal of Applied Physics, 1997, 81(9): 6019–6023. doi: 10.1063/1.364450 [22] FENG R, RAISER G F, GUPTA Y M, et al. Material strength and inelastic deformation of silicon carbide under shock wave compression [J]. Journal of Applied Physics, 1998, 83(1): 79–86. doi: 10.1063/1.366704 [23] PICKUP I M, BARKER A K. Deviatoric strength of silicon carbide subject to shock [J]. AIP Conference Proceedings, 2000, 505(1): 573–576. [24] LEE M, BRANNON R M, BRONOWSKI D R. Uniaxial and triaxial compression tests of silicon carbide ceramics under quasi-static loading condition [R]. Albuquerque, New Mexico: Sandia National Laboratories, 2005. [25] CHEN W, RAVICHANDRAN G. Static and dynamic compressive behavior of aluminum nitride under moderate confinement [J]. Journal of the American Ceramic Society, 1996, 79(3): 579–584. [26] HEARD H C, CLINE C F. Mechanical behaviour of polycrystalline BeO, Al2O3 and AlN at high pressure [J]. Journal of Materials Science, 1980, 15(8): 1889–1897. doi: 10.1007/BF00550614 [27] WILKINS M L, CLINE C F, HONODEL C A. Fourth progress report of light armor program [R]. Livermore: Lawrence Radiation Laboratories, 1969. [28] HOLMQUIST T J, TEMPLETON D W, BISHNOI K D. Constitutive modeling of aluminum nitride for large strain, high-strain rate, and high-pressure applications [J]. International Journal of Impact Engineering, 2001, 25(3): 211–231. doi: 10.1016/S0734-743X(00)00046-4 [29] ZINSZNER J L, ERZAR B, FORQUIN P, et al. Dynamic fragmentation of an alumina ceramic subjected to shockless spalling: an experimental and numerical study [J]. Journal of the Mechanics and Physics of Solid, 2015, 85: 112–127. doi: 10.1016/j.jmps.2015.08.014 [30] GALVEZ F, RODRIGUEZ J, SANCHEZ V. Tensile strength measurements of ceramic materials at high rates of strain [J]. Le Journal de Physique IV, 1997, 7(C3): 151. [31] GALVEZ F, RODRIGUEZ J, SANCHEZ V. The spalling of long bars as a reliable method of measuring the dynamic tensile strength of ceramics [J]. International Journal of Impact Engineering, 2002, 27(2): 161–177. doi: 10.1016/S0734-743X(01)00039-2 [32] BOURNE N K. Shock-induced brittle failure of boron carbide [J]. Proceedings of the Royal Society of London. Series A: Mathematical, Physical and Engineering Sciences, 2002, 458: 1999–2006. doi: 10.1098/rspa.2002.0968 [33] VOGLER T J, REINHART W D, CHHABILDAS L C. Dynamic behavior of boron carbide [J]. Journal of Applied Physics, 2004, 95: 4173–4183. doi: 10.1063/1.1686902 [34] HAYUN S, PARIS V, DARIEL M P, et al. Static and dynamic mechanical properties of boron carbide processed by spark plasma sintering [J]. Journal of the European Ceramic Society, 2009, 29(16): 3395–3400. doi: 10.1016/j.jeurceramsoc.2009.07.007 [35] LUNDBERG P, WESTERLING L, LUNDBERG B. Influence of scale on the penetration of tungsten rods into steel-backed alumina targets [J]. International Journal of Impact Engineering, 1996, 18(4): 403–416. doi: 10.1016/0734-743X(95)00049-G -










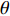

 下载:
下载:
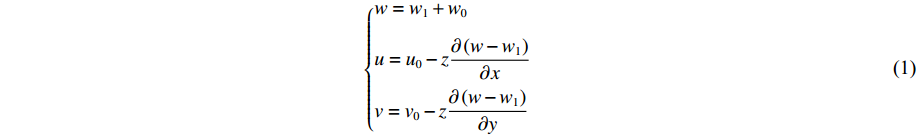

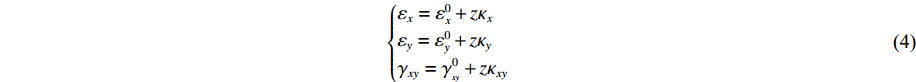

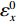
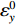
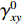
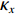
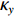
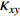
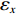
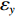
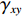
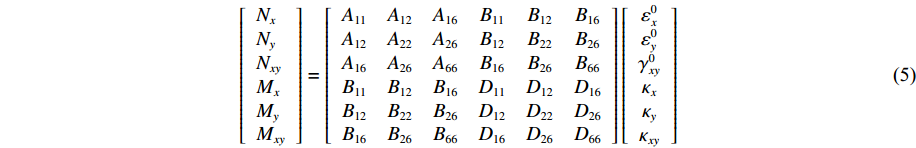
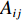

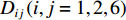
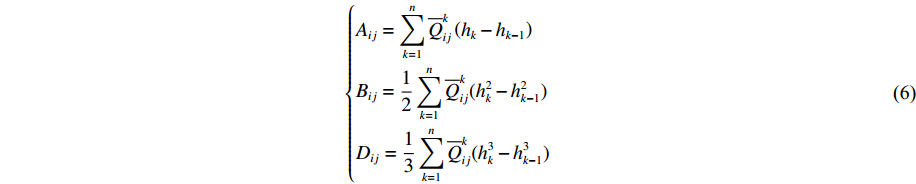
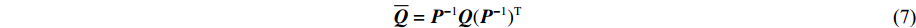
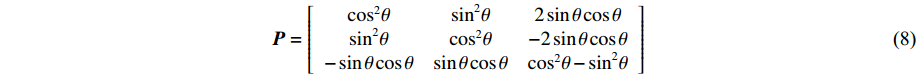
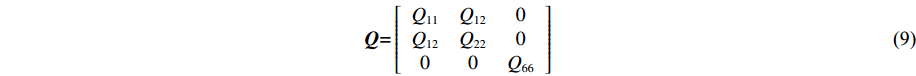
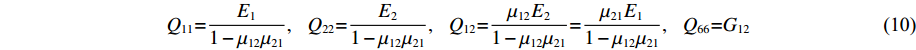

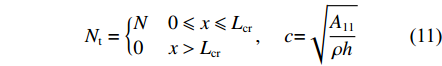
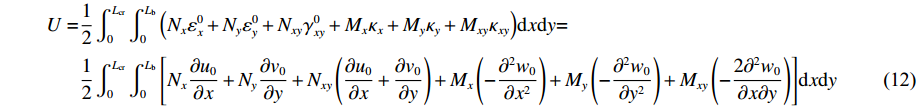
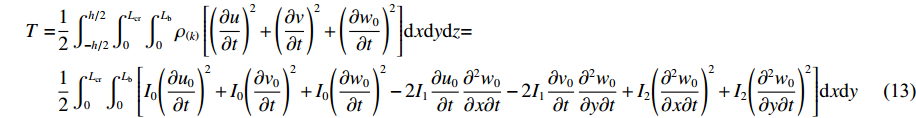
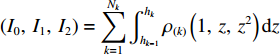
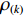
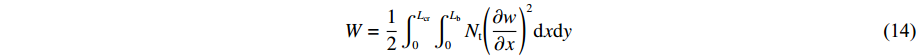
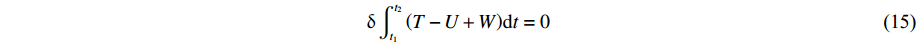
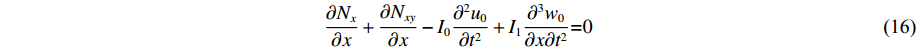
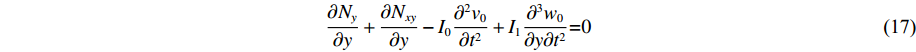
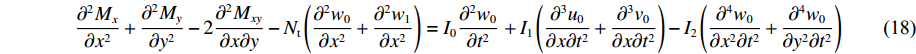
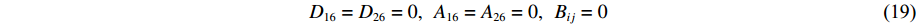
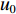
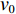
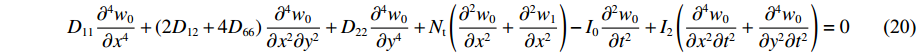
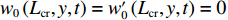
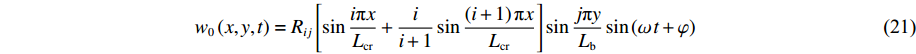
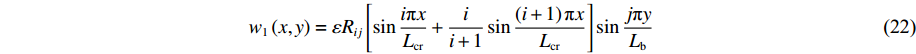
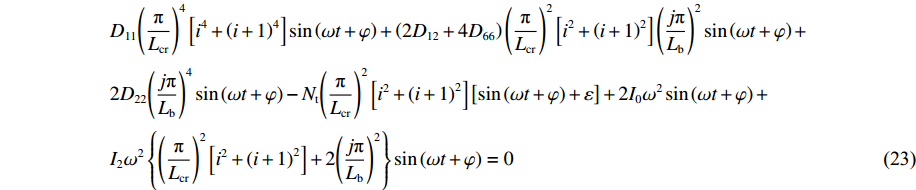
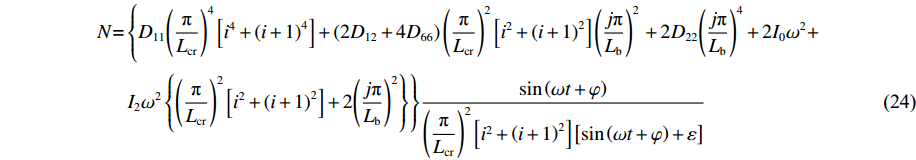

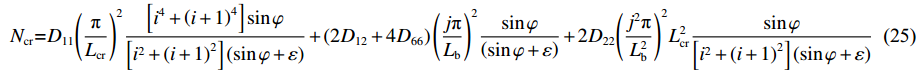









 下载:
下载: