Damage of 3D Random Aggregate Concrete under Ultrasonic Dynamic Load
-
摘要: 混凝土是一种由粗骨料与水泥砂浆组成的非均质复合材料。本研究利用APDL语言程序编写三维水泥混凝土骨料随机投放程序并导入ABAQUS中,同时赋予各相材料塑性损伤本构关系来研究混凝土动态加载下的破坏规律,运用超声波在混凝土破碎中的作用机理对混凝土动态损伤破坏过程进行模拟研究。结果表明:随着超声动态载荷的增大,粗骨料体积分数为40%的混凝土始终能够承受最大应力载荷;随着超声应力波幅值增大,混凝土在动载荷下的损伤值逐渐增大,且粗骨料体积分数为40%时,其抗损伤能力最优;当粗骨料最大粒径逐渐增大,或者当粗骨料最小粒径增大,混凝土级配不合理导致性能不稳定,更易损伤破坏。Abstract: Concrete is a heterogeneous that is composed of coarse aggregate and cement mortar. The dynamic damage process of concrete was numerically simulated by the action mechanism of ultrasonic in concrete crushing in this paper. The random placement procedure of 3D concrete aggregate was prepared by APDL and introduced into ABAQUS, and the plastic damage constitutive relationship of each phase material was applied to study concrete damage law for dynamic loading. The numerical simulation results show that with the increase of ultrasonic dynamic load, the concrete with 40% coarse aggregate can always withstand the maximum stress load. As the amplitude of ultrasonic stress wave increases, the damage value of concrete under dynamic load increases gradually, and the damage resistance is optimal when the volume fraction is 40%. When the maximum particle size of the coarse aggregate gradually increases, or the minimum particle size of the coarse aggregate increases, the concrete grading is unreasonable, resulting in unstable performance and more vulnerable to damage.
-
Key words:
- concrete /
- ultrasonic /
- random aggregate model /
- plastic damage
-
混凝土作为最常用的工程复合材料,已被广泛应用于城市道路与基础建设中。混凝土结构在常年累月的使用中难免会破损、老化,破坏周围的生态环境并威胁社会安全。针对废弃混凝土结构拆除问题,本研究提出了一种超声波破碎混凝土技术,该技术具有效率高、指向性好等特点,有望成为未来研究的热点。除此之外,为了更深入地掌握混凝土在超声波作用下的损伤破坏机理,其动态条件下的损伤力学性能亟待进一步探索。
已有的研究表明,超声波在混凝土中一般以应力波形式传播[1],具有强烈的瞬态行为。因此,细观的破坏过程很难通过试验观测,利用数值模拟手段不仅可以直观地展现细观破坏过程,而且可以为试验提供理论指导。目前,国内外对混凝土损伤性能的研究较多。Wittmann等[2]最早提出利用数值模拟方法分析混凝土细观层次的损伤力学性能。Bazant等[3]在此基础上创建了随机粒子模型,用于研究混凝土的损伤及细观裂纹扩展过程。Pederesn等[4]建立了骨料嵌入二维水泥砂浆基体的有限元模型,研究了加载速率对混凝土损伤性能的影响。Park等[5]模拟了高应变率条件下的混凝土破坏过程,分析了混凝土微观结构的损伤性能。李杰等[6]建立了混凝土单轴受压损伤机理模型,进一步研究了混凝土的单轴受压随机损伤本构关系。刘海峰等[7]以冲击载荷作用下的混凝土为对象,研究了二维层次混凝土的动态损伤力学性能。刘光廷等[8]利用蒙特卡洛方法建立了二维随机骨料模型,模拟了混凝土单边裂缝受拉的损伤断裂过程。然而,针对超声波作用下混凝土三维随机骨料损伤研究仍有待进一步完善。
本研究将混凝土视为由水泥砂浆与粗骨料组成的非均质复合材料,运用ANSYS软件经典界面自带的APDL语言程序编写混凝土三维随机骨料模型,并将创建的模型导入ABAQUS中,利用ABAQUS良好的非线性计算能力,研究超声波幅值、粗骨料体积分数及粒径对混凝土动态损伤力学性能的影响。
1. 骨料随机生成与投放算法
骨料随机生成与投放算法需要解决骨料生成和投放过程中的重叠判断这两个方面的问题。为使每一块骨料都有精确的位置描述,且避免求解多元高次方程,本算法中的骨料设定为凸性[9]。
1.1 骨料顶点生成方式
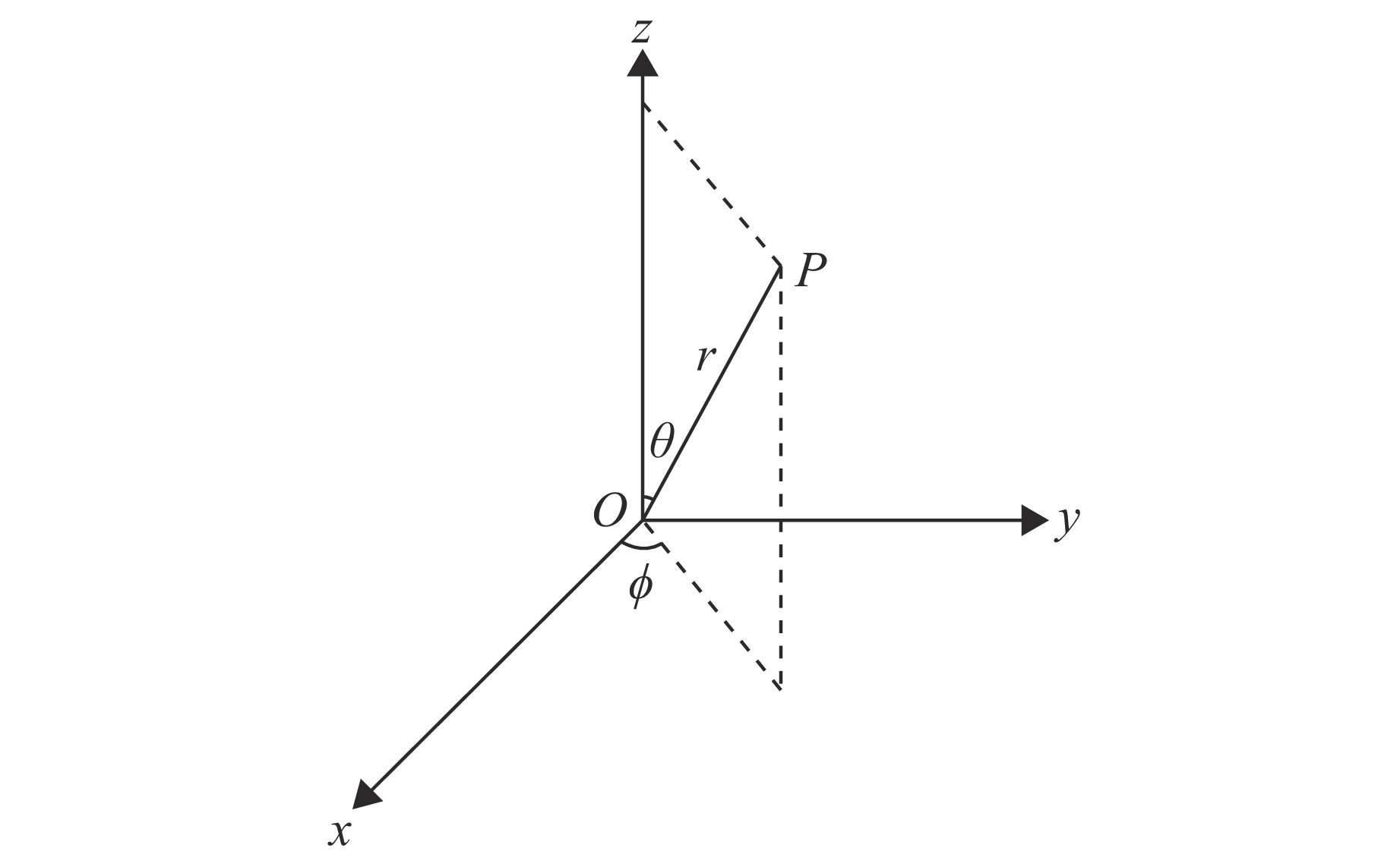
在空间生成随机点,以此反映骨料的形状特征。骨料顶点生成方法如图1所示。在三维球坐标中取一点P,点P的位置可以由参数r、θ、
1.2 骨料顶点间包络面的生成
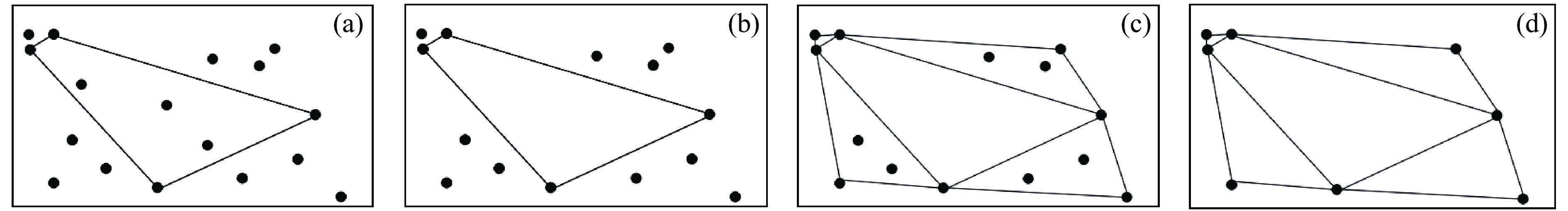
生成骨料顶点后,继续生成骨料顶点间的包络面即可完成骨料生成的全过程,采用文献[9]中所述的Quickqhull算法生成包络面。Quickqhull算法的基本原理是:(1)构造大致轮廓,如图2(a)所示,通过定位于凸包之上的4个端点,获取各顶点集合的一个包围四边形区域;(2)删除该四边形内部不在凸包之上的顶点,得到修正轮廓,如图2(b)所示;(3)对应包围四边形区域的4条边中的每一条边,找到外部距离最远的顶点,必为凸包上的点,这3点构成一个新的三角形,如图2(c)所示,删除新生成三角形中的内部点;(4)对于凸包上的每一条新边,重复上述过程,直至没有顶点位于边外为止,如图2(d)所示。三维凸包络面的原理与此类似。
1.3 骨料投放与模型生成
生成完整的骨料之后,便是投放骨料:(1)在APDL编程模块中,创建几何模型;(2)生成数量为
2. 有限元分析
2.1 仿真模型
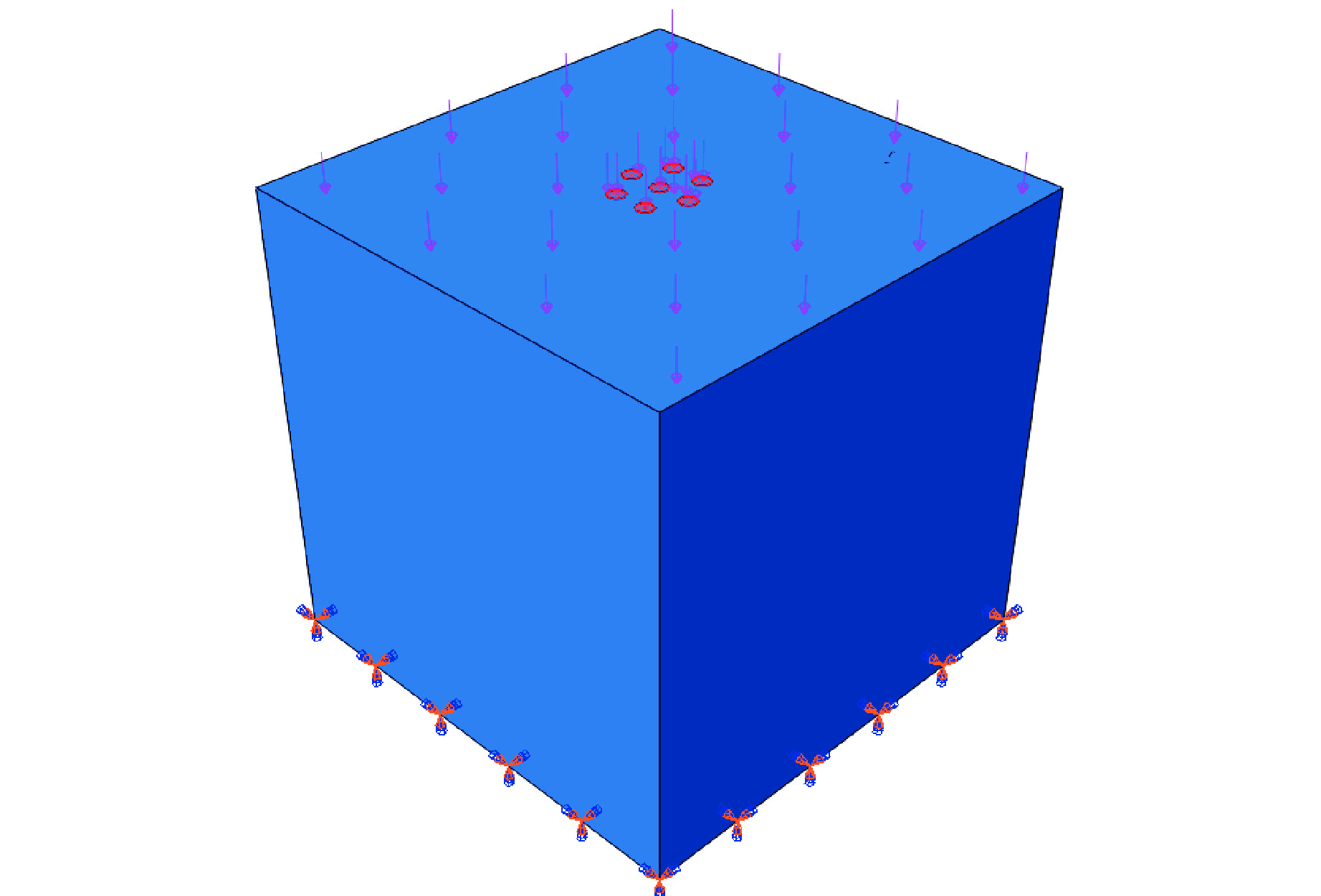
超声波作用下混凝土的损伤是一个典型的非线性过程,通常伴有明显的软化阶段,常规的隐式分析方法难以有效地解决此类复杂问题,故采用显示动力学计算方法求解。本研究在ABAQUS软件的Dynamic/Explicit模块下模拟了超声波加载下三维随机骨料混凝土的塑性损伤效应。采用3种不同尺寸的立方体有限元模型,边长分别为75、100、150 mm,有限元模型中粗骨料的粒径为5~10 mm。图4是边长为100 mm的有限元模型,粗骨料体积分数为40%,粒径为5~10 mm。为节省计算时间,保证计算精度,粗骨料与水泥砂浆的网格尺寸设置为2 mm,采用空间四面体结构的自由网格划分方式。
在三维混凝土立方模型底部施加固定约束,限制其3个坐标方向的移动与旋转自由度。在混凝土模型顶部面施加静态力载荷,侧面为自由边界条件;创建超声波频率大于20 kHz的应力波来模拟超声动态加载,加载区域为图4中的红色圆点阵区域,每个点区域面积为
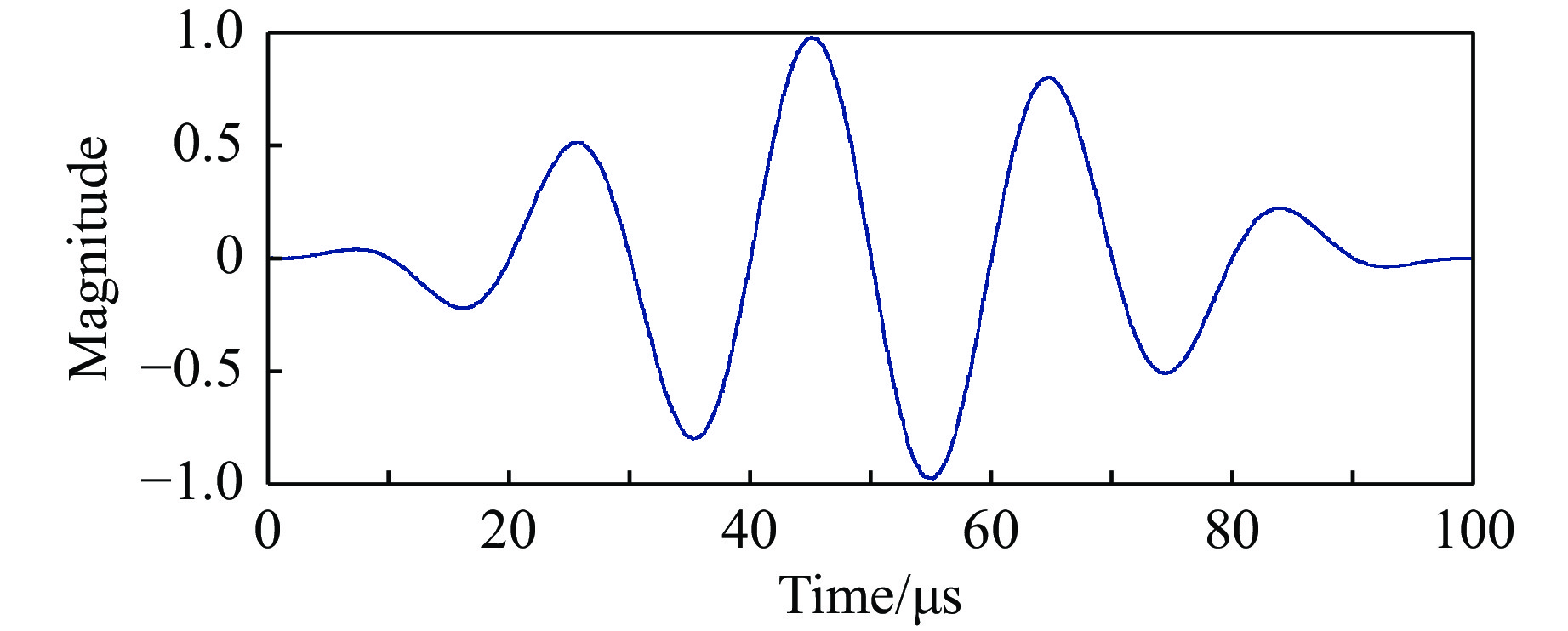
超声波加载破碎混凝土是复杂的非线性过程。为了更好地模拟该过程,在Dynamic/Explicit模块下建立两步操作:第一步加载静载荷完成模型预加载过程,施加的预压力为10 MPa;第二步加载超声动态载荷,应力波幅值范围为0~25 MPa。本仿真过程中选择能量较集中且频率为20 kHz的HANNING窗调制的正弦波,其表达式为
(1) 式中:F为应力波幅值函数;fc为超声波频率;当0 ≤ t ≤ 100时,
2.2 材料模型
2.2.1 混凝土塑性损伤本构模型
运用Matzenmiller等[13]提出的ABAQUS混凝土塑性损伤模型,在数值模拟中参考刘海峰等[7]的本构模型与参数,并将其转化运用于ABAQUS的材料属性中。
损伤时的有效应力
(2) 式中:
有效应力与弹性应变之间的关系为
(3) 式中:
(4) 以dc、dt分别表示混凝土受压和受拉时的刚度退化比率,则单轴受压力Dc和单轴受拉力Dt表示为
(5) (6) 由式(5)、式(6)及图6可知,混凝土在受到损伤后,初始的卸载刚度E0同时受到损伤分别退化为(1-dc)E0、(1-dt)E0。图6中σt、σc分别为拉应力和压应力,εt、εc分别为拉应变和压应变。
2.2.2 损伤因子的取值
在ABAQUS的塑性损伤模型中,需提供另外的损伤因子—非弹性应变曲线参考参数,综合能量等效原理可计算出损伤因子相关参数。应力作用在受损材料与无损材料上产生的弹性余能在形式上相同,因此把应力改为等效应力,把弹性模量改为受损伤时的等效弹性模量[14]。
无损伤弹性余能
(7) 等效有损伤弹性余能
(8) 又因为
(9) 式中:
(10) 单轴受压损伤因子计算公式为
(11) 单轴受拉损伤因子计算公式为
(12) 式中:αc、αt为混凝土受压、受拉的强度折减系数;
2.2.3 本构模型参数选取及验证
水泥砂浆和粗骨料模型的基本参数见表1,其中E为弹性模量,ν为泊松比,γ为容重,ψ为膨胀角,ε为流动势偏移量,Kc为不变应力之比,αf为双、单轴抗压强度之比。损伤因子及混凝土塑性损伤模型参数由2.3.2节的计算得出。由于骨料的强度及断裂能相对较高,故其应力-应变关系采用线弹性模型描述;而砂浆具有易被破坏的非线性特性,故使用混凝土塑性损伤模型表征[15]。
表 1 模型参数Table 1. Model parametersMaterial E/GPa ν γ/(N·m–3) ψ/(°) ε Kc αf Aggregate 23 0.167 2.6 Mortar 10.66 0.2 2.1 35 0.1 0.667 1.16 图7为超声波加载条件下混凝土的损伤破坏试验与仿真结果的对比。模拟试件的尺寸、粗骨料体积分数、粒径均与试验保持一致。由图7(a)可以看出,试件的宏观破坏主要集中在顶部,表现为部分或完全破坏,图7(b)中的破坏仿真呈现出与图7(a)相似的结果。与文献[7]中的试验对比可知,本研究建立的有限元模型及参数是有效的,可应用于超声波破碎混凝土的机理研究[14]。
3. 数值模拟结果分析
3.1 混凝土的损伤破坏模式
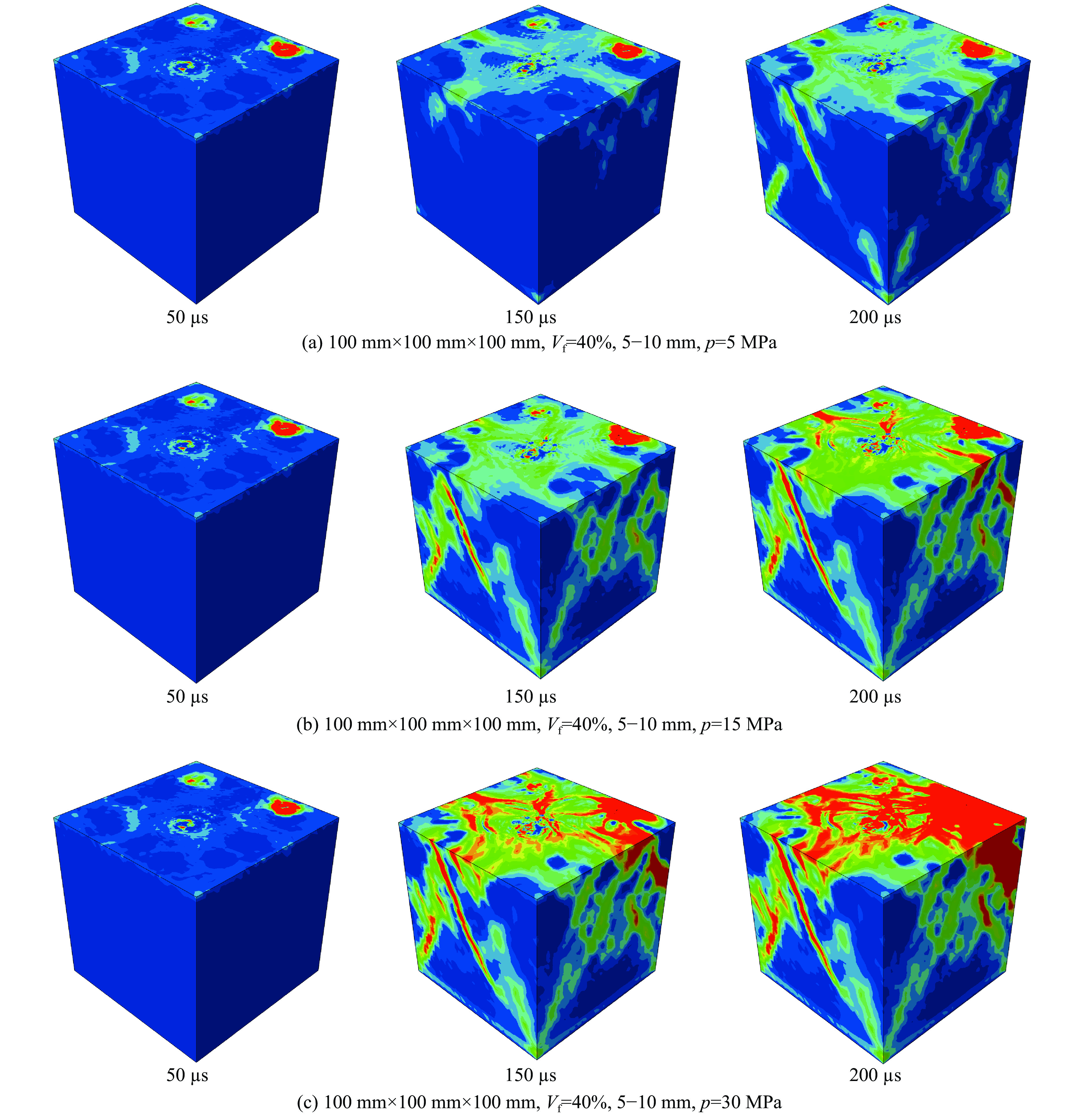
混凝土立方体模型的边长为100 mm,粗骨料体积分数为40%,粗骨料粒径为5~10 mm。超声波频率为20 kHz、加载时间相同、应力波幅值不同的损伤破坏云图如图8所示。由图8可知,当超声应力波幅值为5 MPa时,由于预压力的存在,混凝土只在表面产生部分损伤,整体结构没有明显变化,不会构成破坏。当超声应力波幅值为15 MPa时,损伤处形成细长条带状,并且连接成片,混凝土产生明显损伤,但超声波到达底部之前产生的损伤能量尚不足以造成混凝土的破坏,一部分超声波被反射回试件顶部,加强了顶部的应力/应变场,并促进了新的损伤带的产生和扩展。当超声应力波幅值达到30 MPa时,损伤条带区域不断增大,形成大片的整体区块状,此时产生的能量足以破坏混凝土,由图8(c)可以看出,损伤破坏是从超声波叠加的导入区开始,并且向下蔓延。由此可知,在加载时间相同的情况下,超声应力波幅值越大,最终产生的损伤越明显。
3.2 超声波幅值大小的影响
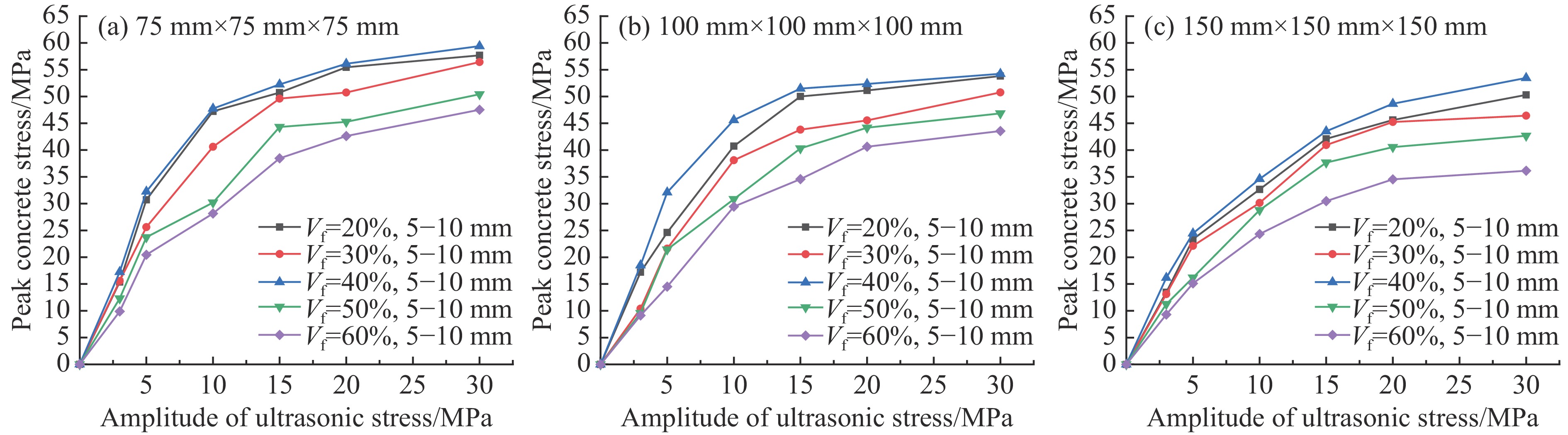
以粗骨料粒径同为5~10 mm,边长分别为75、100和150 mm的3种立方体混凝土试件为仿真模型,对其在不同粗骨料体积分数时的损伤破坏过程进行模拟研究,分析超声应力波幅值对其峰值应力的影响,结果如图9所示。由图9可知:随着应力波幅值的增大,混凝土峰值应力逐渐增大;在同尺寸模型中,粗骨料体积分数为40%的混凝土模型的峰值应力始终最大;且随着模型尺寸的增大,混凝土峰值应力有所减小,具有明显的尺寸效应。
3.3 粗骨料体积分数对损伤破坏的影响
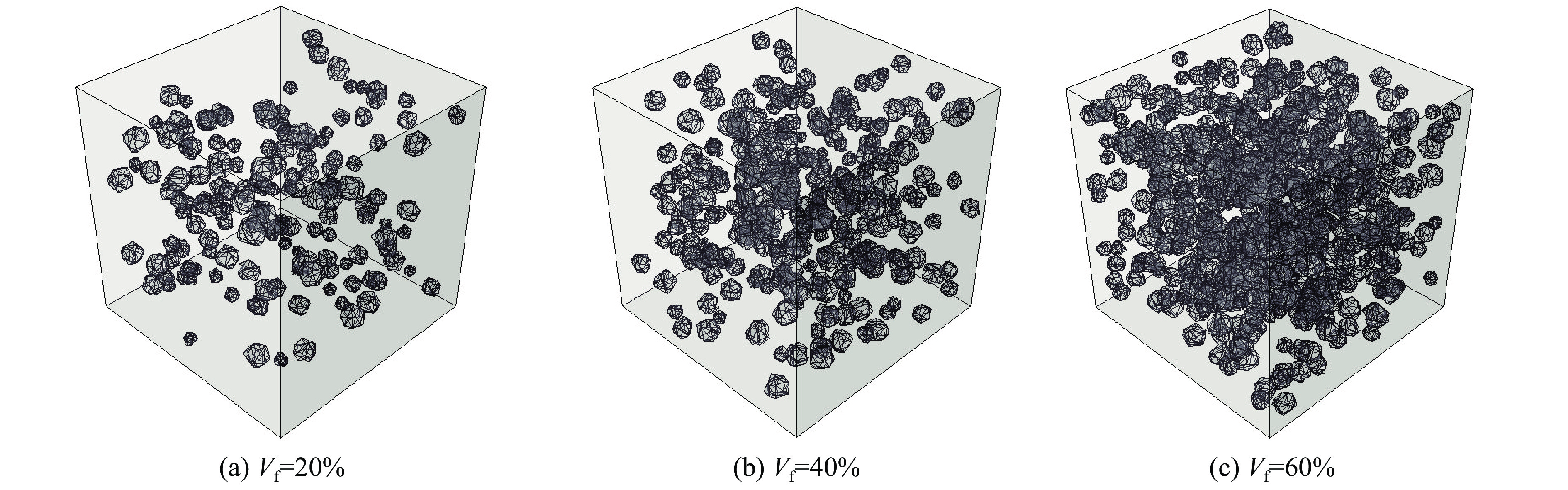
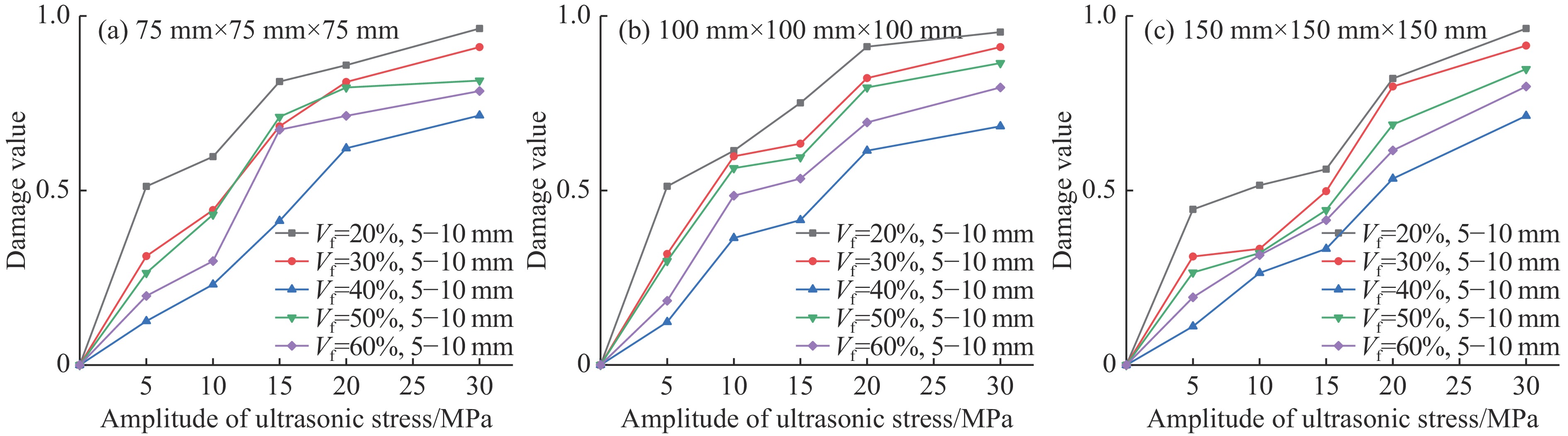
以粗骨料粒径为5~10 mm,粗骨料体积分数范围为20%~60%,边长分别为75、100和150 mm的立方体混凝土试件为仿真模型,分析在超声应力波幅值增大的条件下,粗骨料体积分数变化对混凝土损伤破坏特性的影响规律,结果如图10所示。由图10可知:随着超声应力波幅值的增大,混凝土在动载荷下的损伤值逐渐增大,且粗骨料体积分数为40%时,其抗损伤能力达到最优值。
3.4 粗骨料粒径的影响
3.4.1 粗骨料最大粒径的影响
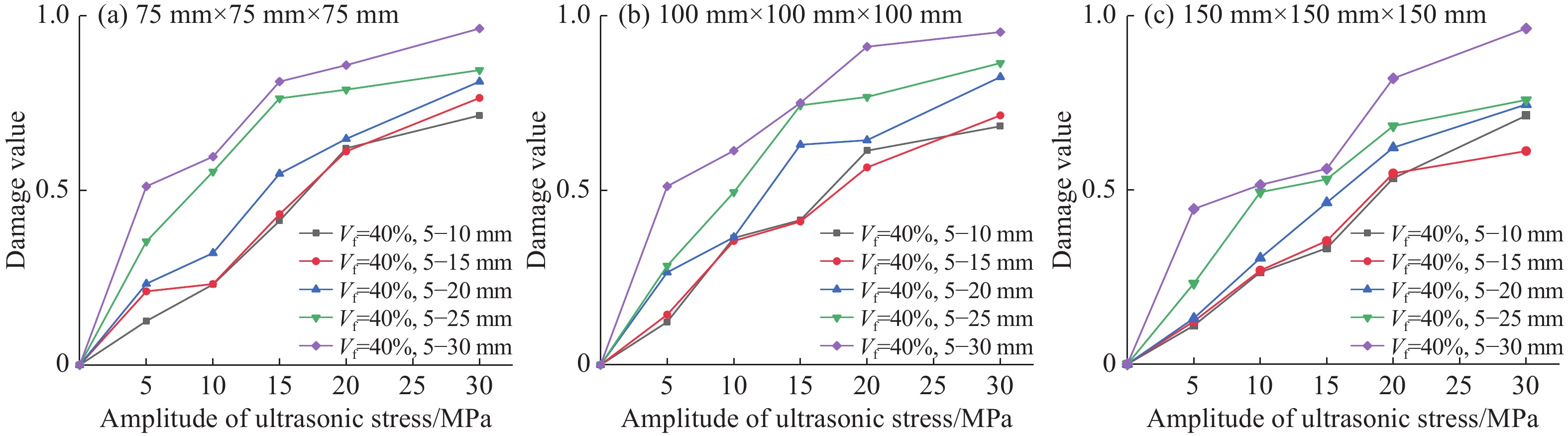
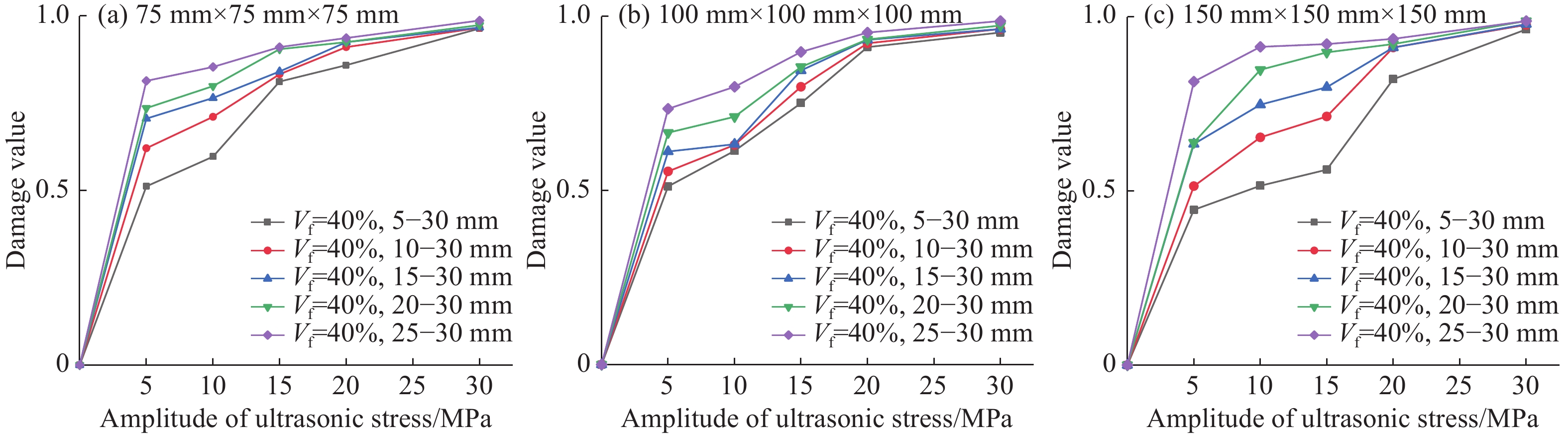
保持粗骨料最小粒径为5 mm不变,对粗骨料最大粒径在10~30 mm范围内分布的混凝土试件的超声波破坏过程进行模拟研究,分析粗骨料最大粒径对混凝土损伤破坏的影响,结果如图11所示。由图11可知,混凝土损伤值随着超声应力波幅值的增大而逐渐增大;当粗骨料粒径级配在5~30 mm范围内分布时,混凝土模型的损伤值最大;而骨料粒径级配在5~10 mm范围内分布时,混凝土模型的抗损伤能力最强。由此可知,粗骨料最大粒径能够影响混凝土的动态损伤行为,当骨料最大粒径逐渐增大时,混凝土内部级配出现不稳定现象的可能性增加,导致混凝土性能下降,抗损伤能力变弱。
3.4.2 粗骨料最小粒径的影响
保持粗骨料最大粒径30 mm不变,对粗骨料最小粒径在5~25 mm范围内分布的混凝土试件的动态损伤破坏进行模拟研究,分析粗骨料最小粒径对混凝土损伤破坏的影响,结果如图12所示。由图12可知,混凝土损伤值随着超声应力波幅值的增大而逐渐增大;当粗骨料粒径级配在5~30 mm范围内分布时,混凝土抗损伤能力最优,但通过与3.4.1节对比可知,混凝土损伤能力最优级配仍为5~10mm。由此可知,粗骨料的最小粒径能够影响混凝土的动态损伤破坏性能,若最小粒径过大,混凝土中的骨料粒径级配质量降低,导致混凝土内部强度下降,影响其抗损伤能力。
4. 结 论
利用ABAQUS的Explicit/Dynamic模块,通过编写三维随机骨料混凝土投放程序,对超声应力波幅值、粗骨料粒径、粗骨料体积分数不同的混凝土动态损伤过程进行了模拟研究,揭示了超声波、骨料粒径、骨料体积分数对超声动态载荷下混凝土损伤性能的影响规律,取得以下主要结论。
(1)对于超声波破碎,超声动态载荷加载比纯静载效果好。随着超声应力波幅值的增大,立方体混凝土试件的应力逐渐增大,损伤不断积累。模型尺寸直接影响混凝土的峰值应力,尺寸越大,峰值应力越小。粗骨料体积分数为40%时,混凝土的峰值应力达到最大值,且具有明显的尺寸效应。
(2)混凝土的损伤随着时间演化而逐渐积累,当粗骨料体积分数为40%时,其抗损伤能力最优,内部性能最稳定。
(3)混凝土粗骨料的最大粒径与最小粒径影响整体混凝土抗损伤性能。粗骨料最大粒径逐渐增大,混凝土越容易损伤破坏;粗骨料最小粒径增大,混凝土级配不合理,导致性能不稳定,更易损伤破坏。
-
表 1 模型参数
Table 1. Model parameters
Material E/GPa ν γ/(N·m–3) ψ/(°) ε Kc αf Aggregate 23 0.167 2.6 Mortar 10.66 0.2 2.1 35 0.1 0.667 1.16 -
[1] OLIVEIRA DIAS A R, AMANCIO F A, CARVALHO RAFAEL M F, et al. Study of propagation of ultrasonic pulses in concrete exposed at high temperatures [J]. Procedia Structural Integrity, 2018, 11(1): 84–90. [2] WITTMANN F H, ROELFSTRA P E, KAMP C L. Drying of concrete: an application of the 3L-approach [J]. Nuclear Engineering and Design, 1988, 105(2): 185–198. doi: 10.1016/0029-5493(88)90339-1 [3] BAZANT Z P, TABBARA M R, KAZEMI M T, et al. Random particle models for fracture of aggregate or fiber composites [J]. Journal of Engineering Mechanics, 1990, 116(8): 1686–1705. doi: 10.1061/(ASCE)0733-9399(1990)116:8(1686) [4] PEDERSEN R R, SIMONE A, SLUYS L J. Mesoscopic modeling and simulation of the dynamic tensile behaviour of concrete [J]. Cement Concrete Research, 2013, 50(1): 74–87. [5] PARK S W, XIA Q, ZHOU M. Dynamic behaviour of concrete at high strain rates and pressure: Ⅱ. numerical simulation [J]. International Journal of Impact Engineering, 2001, 25(9): 887–910. doi: 10.1016/S0734-743X(01)00021-5 [6] 李杰, 卢朝辉, 张其云. 混凝土随机损伤本构关系——单轴受压分析 [J]. 同济大学学报, 2003, 31(5): 505–509.LI J, LU Z H, ZHANG Q Y. Study on stochastic damage constitutive law for concrete material subjected to uniaxial compressive stress [J]. Journal of Tongji University, 2003, 31(5): 505–509. [7] 刘海峰, 韩莉. 二维骨料随机分布混凝土的动态力学性能数值模拟 [J]. 高压物理学报, 2016, 30(3): 191–199. doi: 10.11858/gywlxb.2016.03.003LIU H F, HAN L. Numerical simulation of dynamic mechanical behavior of concrete with two-dimensional random distribution of coarse aggregate [J]. Chinese Journal of High Pressure Physics, 2016, 30(3): 191–199. doi: 10.11858/gywlxb.2016.03.003 [8] 刘光廷, 王宗敏. 用随机骨料模型数值模拟混凝土材料的断裂 [J]. 清华大学学报(自然科学版), 1996, 36(1): 84–89. doi: 10.3321/j.issn:1000-0054.1996.01.007LIU G T, WANG Z M. Numerical simulation study of fracture of concrete materials using random aggregate model [J]. Journal of Tsinghua University (Science & Technology), 1996, 36(1): 84–89. doi: 10.3321/j.issn:1000-0054.1996.01.007 [9] 程书怀, 任志刚, 李培鹏, 等. 基于LS-DYNA的混凝土三维随机凹凸型骨料数值建模 [J]. 武汉理工大学学报, 2014, 36(12): 89–94, 121.CHENG S H, REN Z G, LI P P, et al. Numerical simulation of concrete aggregates with arbitrary shapes based on ANSYS/LS-DYNA [J]. Journal of Wuhan University of Technology, 2014, 36(12): 89–94, 121. [10] 李建波, 林皋, 陈建云. 随机凹凸型骨料在混凝土细观数值模型中配置算法研究 [J]. 大连理工大学学报, 2008, 48(6): 869–874. doi: 10.7511/dllgxb200806016LI J B, LIN G, CHEN J Y. Numerical generation and efficient distribution for random shape aggregates in mesoscopic concrete model [J]. Journal of Dalian University of Technology, 2008, 48(6): 869–874. doi: 10.7511/dllgxb200806016 [11] LIU H F, NING J G. Constitutive model for concrete subjected to impact loading [J]. Journal of Southeast University: English Edition, 2012, 28(1): 79–84. [12] 蒋橙炜, 陈启东, 顾泽堃. 超声破碎混凝土的力学模型与仿真分析 [J]. 机械制造与自动化, 2019, 48(2): 84–88.JIANG C W, CHEN Q D, GU Z K. Mechanical model and simulation analysis of ultrasonic crushed concrete [J]. Machine Building & Automation, 2019, 48(2): 84–88. [13] MATZENMILLER A, LUBLINER J, TAYLOR R L. A constitutive model for anisotropic damage in fiber-composites [J]. Mechanics of Materials, 1995, 20(2): 125–152. doi: 10.1016/0167-6636(94)00053-0 [14] 徐礼华, 李长宁, 李彪, 等. 循环受压状态下钢纤维混凝土一维弹塑性损伤本构模型研究 [J]. 土木工程学报, 2018, 51(11): 77–87.XU L H, LI C N, LI B, et al. Investigation on 1D elasto-plastic constitutive model of steel fiber reinforced concrete under uniaxial cyclic compression [J]. China Civil Engineering Journal, 2018, 51(11): 77–87. [15] 韩宇栋, 张君, 高原. 粗骨料体积含量对混凝土断裂参数的影响 [J]. 工程力学, 2013, 30(3): 191–197.HAN Y D, ZHANG J, GAO Y. Effect of coarse aggregate content on fracture parameters of concrete [J]. Engineering Mechanics, 2013, 30(3): 191–197. -







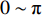
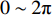

 下载:
下载:

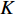
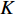


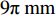
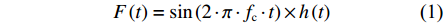


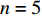


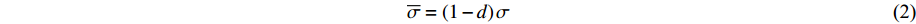

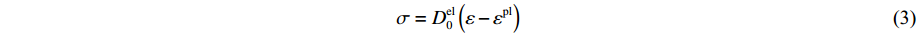
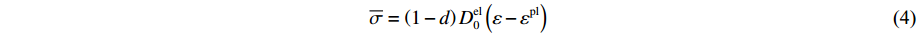
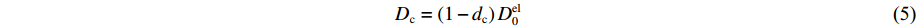
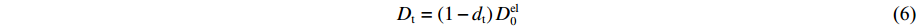

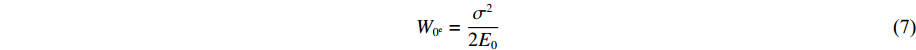
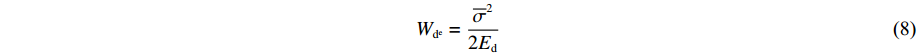
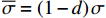
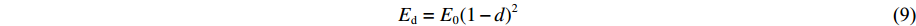
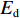
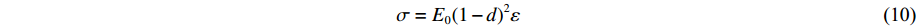
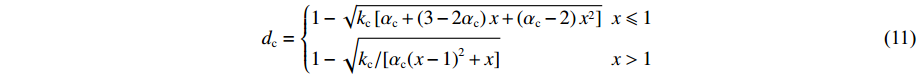
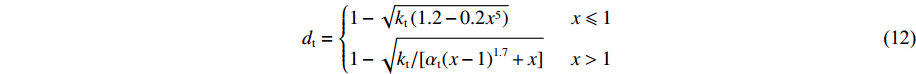
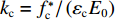








 下载:
下载: