钢纤维混凝土板在冲击与爆炸荷载下的K&amp;C模型

尹华伟; 蒋轲; 张料; 黄亮; 王陈凌

doi:10.11858/gywlxb.20190853

钢纤维混凝土板在冲击与爆炸荷载下的K&C模型

doi: 10.11858/gywlxb.20190853

尹华伟^{1, 2,},
蒋轲¹,
张料¹,
黄亮¹,
王陈凌¹

1.
湖南大学土木工程学院，湖南长沙　410082
2.
湖南湖大土木建筑工程检测有限公司，湖南长沙　410082

基金项目: 国家重点研发计划(2016YFC0701405)

详细信息

作者简介:
尹华伟（1972－），男，博士，副教授，主要从事爆炸与冲击动力学研究. E-mail: yhwzzy@163.com

中图分类号: O347.3; TU375
计量
- 文章访问数: 8053
- HTML全文浏览量: 3825
- PDF下载量: 60
出版历程
- 收稿日期: 2019-11-01
- 修回日期: 2019-11-25

K&C Model of Steel Fiber Reinforced Concrete Plate under Impact and Blast Load

1.
College of Civil Engineering, Hunan University, Changsha 410082, Hunan, China
2.
Civil Engineering Inspection and Test Limited Company of Hunan University, Changsha 410082, Hunan, China

摘要

摘要: 钢纤维混凝土(Steel fiber reinforced concrete，SFRC)具有优异的延性、韧性及能量吸收能力，被广泛应用于各类防护结构。K&C模型已成为研究普通混凝土构件动力响应的常用材料模型，但仍无法准确表征SFRC的动力特性。为了提高K&C模型在冲击及爆炸荷载作用下预测SFRC板动力响应的能力，对K&C模型进行了改进：基于大量三轴压缩实验数据，建立了新的失效强度面参数模型；采用反复试验法，建立了新的损伤演化模型，并校准了拉、压损伤参数；基于大量高应变率下SFRC的单轴压缩实验数据，建立了新的受压动力增强因子模型。通过LS-DYNA显式有限元动力分析软件模拟了SFRC板的动力响应，模拟结果验证了上述改进的有效性与可靠性。
- 钢纤维混凝土 /
- K&C模型 /
- 冲击荷载 /
- 爆炸荷载 /
- 动力响应
Abstract: Steel fiber reinforced concrete (SFRC) is widely used in protective structures due to its excellent ductility, toughness and energy absorption capacity. K&C model is a common constitutive model for studying the response of normal concrete components under impact and blast loads, but it cannot accurately characterize the dynamic response of SFRC. In order to improve prediction of K&C model for the dynamic response of SFRC plate under impact and blast load, this work improves K&C model: a new failure strength surface parameter model was established based on a large number of triaxial compression experimental data, a new damage evolution model was established by trial-and-error method, and the damage parameters of tensile and compressive were calibrated. A new compression dynamic increase factor (CDIF) model was established based on a large number of uniaxial compression experimental data of SFRC under high strain rate. The dynamic response of SFRC plate is simulated by explicit dynamic analysis software LS-DYNA. The effectiveness and reliability of the above improvements have been verified by simulation results.
- steel fiber reinforced concrete /
- K&C model /
- impact load /
- blast load /
- dynamic response

HTML全文

磁驱动固体套筒内爆是指电流通过金属套筒表面时，在洛仑兹力的作用下金属套筒径向向内箍缩内爆的物理过程。1973年，Turchi等^[1]首次提出磁驱动固体套筒内爆的概念。自20世纪90年代以来，磁驱动固体套筒实验被广泛应用于高压状态方程^[2]、材料本构^[3]、层裂损伤^[4]、磁瑞利-泰勒（Magneto-Rayleigh-Taylor，MRT）不稳定性发展^[5–6]、Richtmyer-Meshkov（RM）不稳定性发展^[7]等研究。

磁驱动固体套筒实验涉及热扩散、磁扩散、焦耳加热、弹塑性、断裂、层裂等物理过程，并伴有大变形、界面不稳定性等现象。磁驱动固体套筒理论有薄壳模型^[8–10]、不可压缩模型^[11–13]、电作用量-速度模型^[14–15]、全电路模型^[15]和磁流体力学模型^[16–17]等。这些理论模型已被用于脉冲功率装置、磁驱动固体套筒实验的模拟、设计和研究^[7–17]。阚明先等^[17]采用二维磁流体力学程序MDSC2模拟回流罩结构磁驱动固体套筒实验时发现，根据回流罩结构磁驱动固体套筒实验测量的电流或回路电流不能直接模拟磁驱动固体套筒，模拟的套筒速度总是比测量速度大，即回路电流并不完全从固体套筒表面流过。回路电流与固体套筒上通过的电流之间存在一个电流系数。由于MDSC2程序^[17]以外的理论计算或数值模拟都未提到电流系数，因此，本研究采用其他理论模型对磁驱动固体套筒实验进行模拟，分析回路电流与通过固体套筒的电流之间的关系，通过模拟分析不同回流罩结构固体套筒实验，进一步探讨磁驱动固体套筒实验中电流系数的影响因素和变化规律。

1. 负载结构

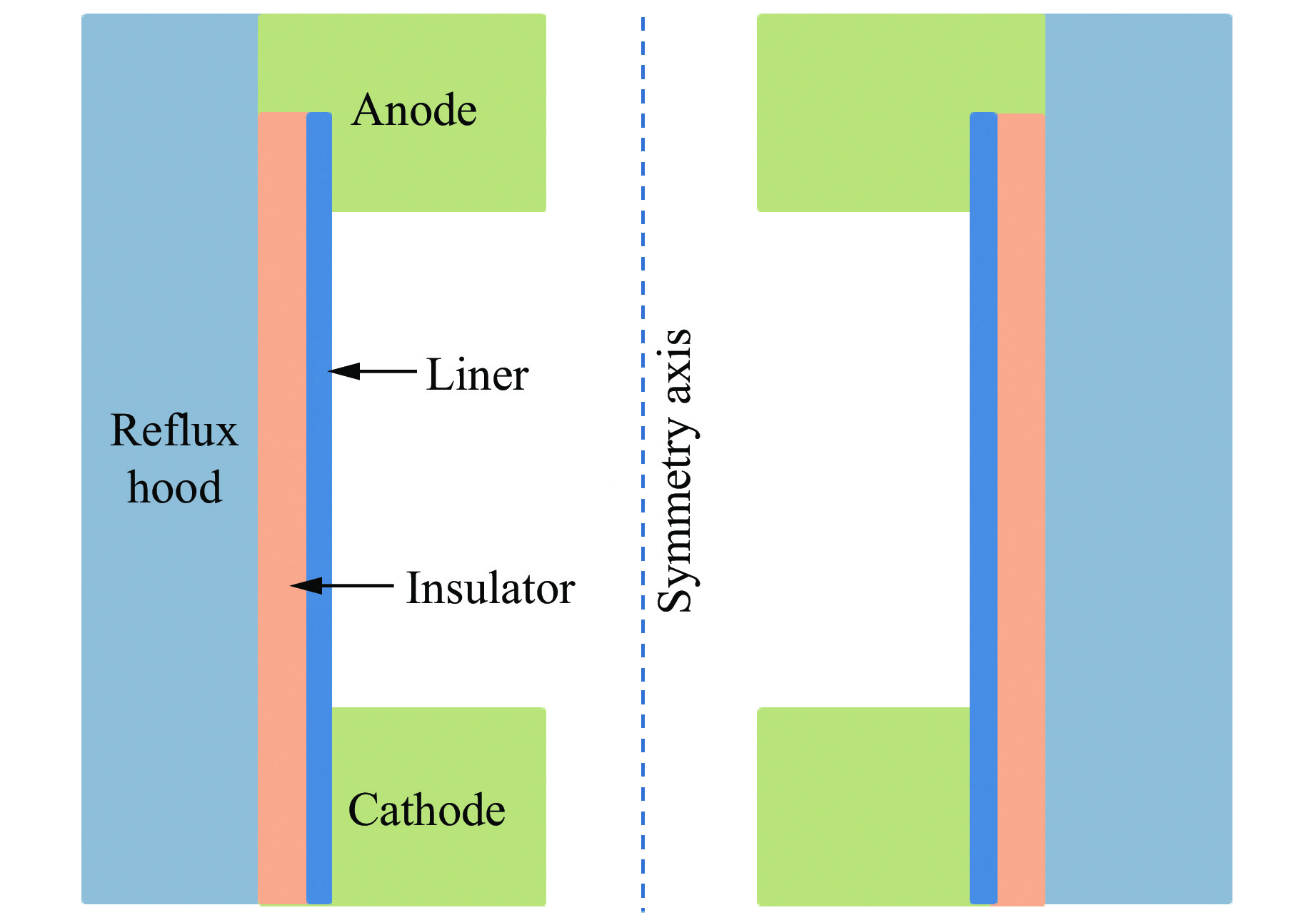
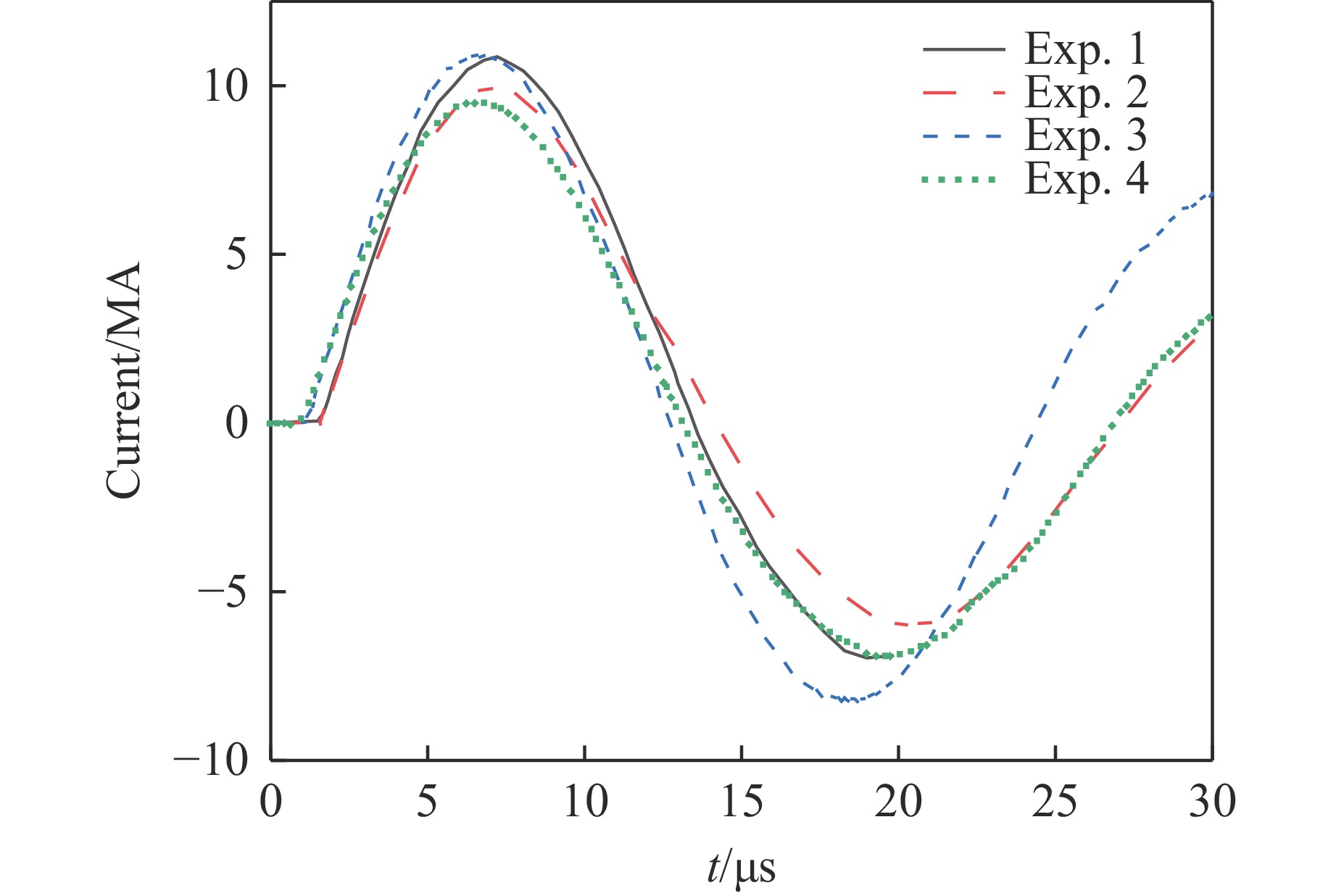
大电流脉冲装置上的固体套筒实验通常采用回流罩结构^{[15, 17–18]}。回流罩结构固体套筒实验的初始结构的rz剖面如图1所示，其中，虚线为对称轴。回流罩结构固体套筒实验装置从外到内依次为金属回流罩、绝缘材料和金属套筒，套筒两端为金属电极，上端为阳极，下端为阴极。回路电流从回流罩金属流入，绕过绝缘材料，经过套筒的外表面从阴极流出。电流加载后，电极外面的固体套筒被切割成与阴阳极之间的间隙等高的套筒，在洛仑兹力作用下沿径向向内箍缩。表1为FP-2装置^[19]中回流罩结构磁驱动固体套筒实验的套筒参数。图2显示了FP-2装置上不同实验测得的电流变化曲线，电流的上升时间约为5500 ns，电流峰值为9～11 MA。

图 1 回流罩固体套筒实验装置的初始结构剖面

Figure 1. Cross section of magnetically driven solid liner experiment setup with a reflux hood

下载: 全尺寸图片幻灯片

表 1 磁驱动固体套筒实验的套筒参数

Table 1. Liner parameters of the magnetically driven solid liner experiments

Exp. No.	Liner material	Liner’s inner radius/mm	Liner’s thickness/mm
1	Al	45	0.6
2	Al	30	0.6
3	Al	45	1.6
4	Al	30	1.9

下载: 导出CSV

| 显示表格

图 2 磁驱动固体套筒实验测得的电流

Figure 2. Measured currents for magnetically driven solid liner experiments

下载: 全尺寸图片幻灯片

2. 电流系数的不可压缩模型验证

在薄壳模型、不可压缩模型、电作用量-速度模型、全电路模型、磁流体力学模型等^[8–16]适用于磁驱动固体套筒的理论模型中，固体套筒边界的磁感应强度（B）为

B (t) = \frac{μ_{0} I_{\exp} (t)}{2 π r_{o}}

$B(t) = \frac{{{\mu _0}{I_{\exp }}(t)}}{{2\text{π} {r_{\mathrm{o}}}}}$

(1)

式中： ${\mu _0}$ 为真空磁导率，I_exp(t)为磁驱动实验测量电流，r_o为固体套筒的外半径。

二维磁驱动数值模拟程序MDSC2是由中国工程物理研究院流体物理研究所开发的二维磁流体力学程序^[20–21]。该程序已被广泛应用于磁驱动飞片发射、超薄飞片、磁驱动准等熵压缩、磁驱动样品等实验的模拟研究^[22–25]。最近，研究人员发现，采用MDSC2程序模拟FP-2装置上的磁驱动固体套筒实验时，基于实验测量的电流或回路电流并不能正确模拟套筒的动力学过程，模拟的套筒速度总是比实验测量值大。为正确模拟FP-2装置上的磁驱动固体套筒实验，需将边界磁感应强度公式^[17]修正为

B (t) = \frac{μ_{0} f_{c} I_{e x p} (t)}{2 π r_{o}}

$B(t)=\frac{{\mu }_{0}{f}_{{\mathrm{c}}}{I}_{\mathrm{exp}}(t)}{2\text{π} {r}_{{\mathrm{o}}}}$

(2)

式中：f_c为回流罩结构rz柱面套筒的电流系数，f_c<1。由于文献[17]之外的理论计算或数值模拟中均未提到电流系数f_c，因此，需要确定f_c是回流罩固体套筒实验固有的，还是MDSC2程序造成的。下面采用固体套筒的不可压缩模型理论确认电流系数是否存在。

在磁驱动固体套筒的不可压缩模型^[11–13]中，不考虑套筒的磁扩散，假设磁压只作用于套筒的外表面，且磁压做功全部转化为套筒动能，套筒不可压缩，只作径向运动。设 $\rho$ 为套筒密度，h为套筒高度，v_o为套筒外界面速度，r_i、v_i分别为套筒内半径和内界面速度，r、v为套筒内某点的径向位置（r_i≤r≤r_o）和速度，由不可压缩假设，有

r_{i} v_{i} = r_{o} v_{o}

${r}_{{\mathrm{i}}}{v}_{{\mathrm{i}}}={r}_{{\mathrm{o}}}{v}_{{\mathrm{o}}}$

(3)

r v = r_{o} v_{o}

$rv = {r_{\mathrm{o}}}{v_{\mathrm{o}}}$

(4)

则套筒总动能E_k为

E_{k} = \int_{r_{i}}^{r_{o}} ρ π r h v^{2} d r = π ρ h r_{o}^{2} v_{o}^{2} l n \frac{r_{o}}{r_{i}}

${E}_{{\mathrm{k}}}={\displaystyle {\int }_{{r}_{{\mathrm{i}}}}^{{r}_{{\mathrm{o}}}}}\rho \text{π} rh{v}^{2}{\rm d}r=\text{π}\rho h{r}_{{\mathrm{o}}}^{2}{v}_{{\mathrm{o}}}^{2}\mathrm{ln}\frac{{r}_{{\mathrm{o}}}}{{r}_{{\mathrm{i}}}}$

(5)

由于磁压只作用于套筒的外表面，且磁压做功全部转化为套筒动能，则

\frac{d E_{k}}{d t} = \frac{2 π}{μ_{0}} r_{o} h v_{o} B^{2}

$\frac{{\mathrm{d}}{E}_{{\mathrm{k}}}}{{\mathrm{d}}t}=\frac{2\text{π} }{{\mu }_{0}}{r}_{{\mathrm{o}}}h{v}_{{\mathrm{o}}}{B}^{2}$

(6)

将式(5)代入式(6)并积分，可得

\frac{d v_{o}}{d t} = - \frac{v_{o}^{2}}{r_{o}} - \frac{1}{l n (r_{o} / r_{i})} [\frac{B^{2}}{2 μ_{0} ρ r_{o}} + \frac{v_{o}^{2}}{2 r_{o}} (1 - \frac{r_{o}^{2}}{r_{i}^{2}})]

$\frac{{\mathrm{d}}{v}_{{\mathrm{o}}}}{{\mathrm{d}}t}=-\frac{{v}_{{\mathrm{o}}}^{2}}{{r}_{{\mathrm{o}}}}-\frac{1}{\mathrm{ln}({r}_{{\mathrm{o}}}/{r}_{{\mathrm{i}}})}\left[\frac{{B}^{2}}{2{\mu }_{0}\rho {r}_{{\mathrm{o}}}}+\frac{{v}_{{\mathrm{o}}}^{2}}{2{r}_{{\mathrm{o}}}}\left(1-\frac{{r}_{{\mathrm{o}}}^{2}}{{r}_{{\mathrm{i}}}^{2}}\right)\right]$

(7)

\frac{d v_{i}}{d t} = - \frac{v_{i}^{2}}{r_{i}} - \frac{1}{l n (r_{o} / r_{i})} [\frac{B^{2}}{2 μ_{0} ρ r_{i}} + \frac{v_{o}^{2}}{2 r_{i}} (1 - \frac{r_{i}^{2}}{r_{o}^{2}})]

$\frac{{\mathrm{d}}{v}_{{\mathrm{i}}}}{{\mathrm{d}}t}=-\frac{{v}_{{\mathrm{i}}}^{2}}{{r}_{{\mathrm{i}}}}-\frac{1}{\mathrm{ln}({r}_{{\mathrm{o}}}/{r}_{{\mathrm{i}}})}\left[\frac{{B}^{2}}{2{\mu }_{0}\rho {r}_{{\mathrm{i}}}}+\frac{{v}_{{\mathrm{o}}}^{2}}{2{r}_{{\mathrm{i}}}}\left(1-\frac{{r}_{{\mathrm{i}}}^{2}}{{r}_{{\mathrm{o}}}^{2}}\right)\right]$

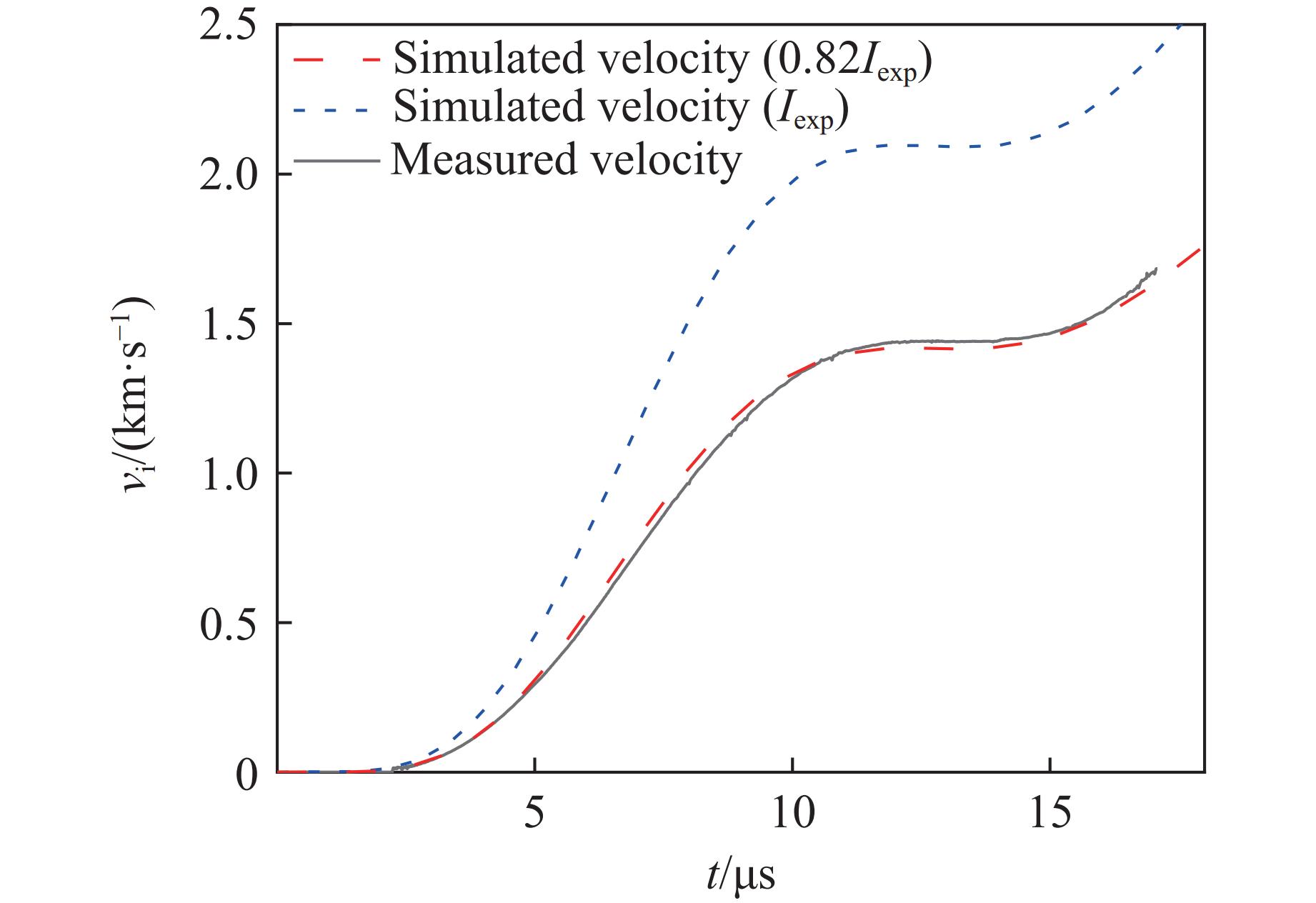
(8)

采用上述不可压缩模型，对固体套筒实验4进行不可压缩模型模拟验证。图3给出了采用不可压缩模型模拟得到的套筒内界面速度。显然，采用回路电流或测量电流直接模拟的套筒速度明显比实验测量速度大，后者是前者的0.82倍，即计算不可压缩模型的边界磁感应强度时不能用式(1)，而是用式(2)。不可压缩模型的模拟结果表明，对于回流罩固体套筒实验，回路电流或测量电流与固体套筒上通过的电流之间的电流系数不是MDSC2程序造成的，而是回流罩固体套筒实验固有的。

图 3 不可压缩模型模拟得到的套筒内界面速度

Figure 3. Liner interface velocity simulated by incompressible model

下载: 全尺寸图片幻灯片

3. 电流系数规律

从第2节的模拟可知，磁驱动固体套筒理论的边界磁感应强度公式中包含电流系数，它反映了有多少回路电流从套筒实际流过。在磁驱动实验中，实验测量的电流是流入回流罩之前的电流，即回路电流，而不是从套筒直接流过的电流。从套筒流过的电流很难被直接测量，因此，电流系数难以预知。回流罩的结构比较复杂，阴阳电极之间连有金属套筒、绝缘材料，金属套筒与绝缘材料之间是真空，回流罩结构的分流机制包括阴阳极间的并联电路分流、漏磁、真空击穿等。事实上，电流系数是通过数值模拟发现的，由磁流体力学程序模拟速度与磁驱动套筒实验测量速度的对比确定。当前的固体套筒实验的模拟都是后验的，无法直接正确预测，因此，研究电流系数的变化规律非常重要，是正确设计和预测固体套筒实验的基础。

由于磁流体力学模型^{[21, 26]}是包含固体弹塑性、热扩散、磁扩散等物理过程的可压缩模型，能够比不可压缩模型更加准确地描述磁驱动固体套筒实验，因此，下面将采用MDSC2程序对FP-2装置上开展的磁驱动固体套筒实验的电流系数变化规律进行研究。

图4给出了实验1～实验4的套筒内界面模拟速度。可以看出，应用式(2)的磁流体力学模型能正确描述磁驱动固体套筒实验。然而，不同的磁驱动固体套筒实验对应的电流系数是不同的。回流罩结构磁驱动固体套筒实验的电流系数和套筒的初始尺寸列于表2。

图 4 实验 1～实验4的套筒内界面速度

Figure 4. Interface velocities of the experimental liners for Exp. 1−Exp. 4

下载: 全尺寸图片幻灯片

表 2 磁驱动固体套筒实验的电流系数

Table 2. Current coefficients of the magnetically driven solid liner experiments

Exp. No.	Liner’s inner radius/mm	Liner’s thickness/mm	f_c
1	45	0.6	0.87
2	30	0.6	0.90
3	45	1.6	0.85
4	30	1.9	0.88

下载: 导出CSV

| 显示表格

由表2可知：电流系数是常数，不随时间的发展而变化，即电流系数与实验过程无关；对于不同的套筒，电流系数有所不同，说明电流系数与套筒的初始结构有关。由实验1和实验2可知，当套筒厚度相同时，若套筒内半径不同，则电流系数不同，且内半径越大，电流系数越小。对比实验1和实验3，或者实验2和实验4可知，当套筒内半径相同时，若套筒厚度不同，则电流系数不同，且套筒厚度越大，电流系数越小。

4. 结　论

采用不可压缩模型验证了回流罩结构磁驱动固体套筒实验中电流系数的存在，即回流罩结构磁驱动固体套筒实验的实验电流/回路电流并不完全从负载套筒的表面通过，实验电流/回路电流与套筒表面流过的电流之间存在一个电流系数。采用包含固体弹塑性、热扩散、磁扩散的磁流体力学模型，对回流罩结构磁驱动固体套筒实验的电流系数进行了确定和分析，结果显示，磁流体力学模型和有电流系数的边界磁感应强度公式能正确模拟回流罩结构磁驱动固体套筒实验。电流系数与套筒结构的关系为：

(1) 不同套筒对应的电流系数不同；

(2) 电流系数与实验过程无关，由套筒初始结构决定；

(3) 套筒厚度相同时，电流系数由套筒内半径决定，套筒内半径越大，电流系数越小；

(4) 套筒内半径相同时，电流系数由套筒厚度决定，套筒厚度越大，电流系数越小。

正确认识磁驱动固体套筒实验的电流系数变化规律，使磁驱动固体套筒实验的磁流体模拟从后验模拟发展成先验的准确设计和预测，有助于降低实验成本，加快柱面相关的实验研究。

图 1 钢纤维体积分数不同时的三轴压缩数据以及式（2）、式（5）的预测值

Figure 1. Triaxial compression data of different fiber contents as well as predictions from Eq.(2) and Eq.(5)

下载: 全尺寸图片幻灯片

图 2 改进的失效强度面

Figure 2. Modified failure strength surface

下载: 全尺寸图片幻灯片

图 3 损伤演化函数η(λ)的比较

Figure 3. Comparisons of damage evolution function η(λ)

下载: 全尺寸图片幻灯片

图 4 单轴压缩试验的应力-应变曲线与K&C模型的预测结果比较

Figure 4. Comparisons of experiment at stress-strain curve of UUC test and prediction of K&C models

下载: 全尺寸图片幻灯片

图 5 单轴拉伸试验的应力-应变曲线与K&C模型的预测结果比较

Figure 5. Comparisons of experiment at stress-strain curve of UUT test and prediction of K&C models

下载: 全尺寸图片幻灯片

图 6 不同模型得到的CDIF值与实验数据的比较

Figure 6. Comparisons of CDIF values obtained from different models and experiments

下载: 全尺寸图片幻灯片

图 7 新模型得到的CDIF值与实验数据的比较

Figure 7. Comparisons of CDIF values obtained from new models and experiments

下载: 全尺寸图片幻灯片

图 8 不同模型得到的TDIF比较

Figure 8. Comparisons of TDIF values obtained from different models

下载: 全尺寸图片幻灯片

图 9 SFRC靶板在平头弹丸冲击下的有限元模型

Figure 9. Finite element model of flat-ended projectile impacting SFRC plate

下载: 全尺寸图片幻灯片

图 10 不同撞击速度下SFRC板破坏模式的实验结果^[1]与数值模拟结果对比

Figure 10. Comparison of the damage patterns of SFRC plate from experimental results^[1] and numerical simulation under different impact velocities

下载: 全尺寸图片幻灯片

图 11 SFRC板在爆炸荷载作用下的有限元模型

Figure 11. Finite element model of SFRC plate under blast load

下载: 全尺寸图片幻灯片

图 12 不同纤维体积分数时SFRC板挠曲破坏模式的实验结果^[2]与数值预测对比

Figure 12. Comparisons of the damage patterns of SFRC plate from simulation predictions and experimental results^[2] under different fiber volume fractions

下载: 全尺寸图片幻灯片

表 1 改进的钢纤维混凝土K&C模型参数

Table 1. Parameters of the modified K&C model of SFRC

Strength surface
a₀/MPa		a₁	a₂/MPa⁻¹		a_0y/MPa		a_1y	a_2y/MPa⁻¹		a_1f	a_2f/MPa⁻¹
64.0		0.481	5.82×10⁻⁴		45.93		0.726	1.77×10⁻³		0.476	8.56×10⁻⁴

Damage								Others
b₁	b₂	b₃	α	α_c	α_d	λ_m	L_w/mm	f_c/MPa	f_t/MPa	ρ/(kg·m⁻³)	ν	ω
0.75	−1.50	1.15	3.00	0.381	1.90	9.5×10⁻⁵	24	175.3	13.8	2 600	0.19	0.5

下载: 导出CSV

表 2 平头弹丸的材料参数

Table 2. Materials parameters of the flat ended projectile

ρ/(g·cm⁻³)	G/GPa	A/MPa	B/MPa	N	C	M	T_m/K	T_R/K
7.83	210	792	510	0.26	0.014	1.03	1 793	294

ε/(μs⁻¹)	c_p/（J·kg⁻¹·K⁻¹）	SPALL	IT	D₁	D₂	D₃	D₄	D₅
1.0 × 10⁻⁴	4.77 × 10⁻⁵	3.0	0.0	4.00	0.00	0.00	0.00	0.00

下载: 导出CSV

表 3 实验数据与数值模拟结痂弹坑直径比较

Table 3. Comparisons of experimental data and numerical simulation of the scabbed crater diameter

Projectile velocity/(m·s⁻¹)	Scabbed crater diameter/mm			Model error/%
Projectile velocity/(m·s⁻¹)	Experiment	Original K&C	Modified K&C	Original K&C	Modified K&C
58.2	120.5	132	106	9.5	12.0
76.0	119.2	148	124	24.2	4.0
104.0	120.3	28	128	76.7	6.4

下载: 导出CSV

参考文献(29)

[1]	TAI Y S. Flat ended projectile penetrating ultra-high strength concrete plate target [J]. Theoretical and Applied Fracture Mechanics, 2009, 51(2): 117–128. doi: 10.1016/j.tafmec.2009.04.005
[2]	LUCCIONI B, ISLA F, CODINA R, et al. Effect of steel fibers on static and blast response of high strength concrete [J]. International Journal of Impact Engineering, 2017, 107(1): 23–27.
[3]	宋玉普, 赵国藩, 彭放, 等. 三向应力状态下钢纤维混凝土的强度特性及破坏准则 [J]. 土木工程学报, 1994, 27(3): 14–23. SONG Y P, ZHAO G F, PENG F, et al. Strength behavior and failure criteria of steel fiber concrete under triaxial stresses [J]. China Civil Engineering Journal, 1994, 27(3): 14–23.
[4]	王乾峰, 彭刚, 戚永乐. 围压条件下钢纤维混凝土动态压缩试验研究 [J]. 混凝土, 2009(3): 29–31. doi: 10.3969/j.issn.1002-3550.2009.03.009 WANG Q F, PENG G, QI Y L. Dynamical press test of SFRC under confined pressure [J]. Concrete, 2009(3): 29–31. doi: 10.3969/j.issn.1002-3550.2009.03.009
[5]	王志亮, 诸斌. 钢纤维混凝土三轴压缩下的强度和韧度特性 [J]. 建筑材料学报, 2012, 15(3): 301–305. doi: 10.3969/j.issn.1007-9629.2012.03.002 WANG Z L, ZHU B. Strength and toughness characteristic of steel fiber reinforced concrete in triaxial compression [J]. Journal of Building Materials, 2012, 15(3): 301–305. doi: 10.3969/j.issn.1007-9629.2012.03.002
[6]	CHI Y, XU L H, MEI G D, et al. A unified failure envelope for hybrid fibre reinforced concrete subjected to true triaxial compression [J]. Composite Structures, 2014, 109: 31–40. doi: 10.1016/j.compstruct.2013.10.054
[7]	SIRIJAROONCHAR K, EL-TAWIL S, PARRA-MONTESINOS G. Behavior of high performance fiber reinforced cement composites under multi-axial compressive loading [J]. Cement and Concrete Composites, 2010, 32(1): 62–72. doi: 10.1016/j.cemconcomp.2009.09.003
[8]	LU X, HSU C T T. Behavior of high strength concrete with and without steel fiber reinforcement in triaxial compression [J]. Cement and Concrete Research, 2006, 36(9): 1679–1685. doi: 10.1016/j.cemconres.2006.05.021
[9]	FARNAM Y, MOOSAVI M, SHEKARCHI M, et al. Behaviour of slurry infiltrated fibre concrete (SIFCON) under triaxial compression [J]. Cement and Concrete Research, 2010, 40(11): 1571–1581. doi: 10.1016/j.cemconres.2010.06.009
[10]	FANTILLI A P, VALLINI P, CHIAIA B. Ductility of fiber-reinforced self-consolidating concrete under multi-axial compression [J]. Cement and Concrete Composites, 2011, 33(4): 520–527. doi: 10.1016/j.cemconcomp.2011.02.007
[11]	REN G M, WU H, FANG Q, et al. Triaxial compressive behavior of UHPCC and applications in the projectile impact analyses [J]. Construction and Building Materials, 2016, 113(1): 1–14.
[12]	MALVAR L J, CRAWFORD J E, WESEVICH J W, et al. A plasticity concrete material model for DYNA3D [J]. International Journal of Impact Engineering, 1997, 19(9/10): 847–873.
[13]	CHI Y, XU L, ZHANG Y. Experimental study on hybrid fiber–reinforced concrete subjected to uniaxial compression [J]. Journal of Materials in Civil Engineering, 2014, 26(2): 211–218. doi: 10.1061/(ASCE)MT.1943-5533.0000764
[14]	MURUGESAN REDDIAR M K. Stress-strain model of unconfined and confined concrete and stress-block parameters [D]. Texas A & M University, 2009: 31–37.
[15]	BAZANT Z. P. Fracture mechanics of concrete structures [C]//Proceedings of the First International Conference on Fracture Mechanics of Concrete Structures. Amsterdam: Breckenridge, CO, 1992: 20−25.
[16]	董振英, 李庆斌, 王光纶, 等. 钢纤维混凝土轴拉应力应变特性的试验研究 [J]. 水利学报, 2002(5): 47–50. doi: 10.3321/j.issn:0559-9350.2002.05.010 DONG Z Y, LI Q B, WANG G L, et al. Experimental study on stress-strain characteristics of steel fiber reinforced concrete under uniaxial tension [J]. Journal of Hydraulic Engineering, 2002(5): 47–50. doi: 10.3321/j.issn:0559-9350.2002.05.010
[17]	Comité Euro-International du Béton, CEB-FIP Model Code 1990: Design Code [S]. London: Thomas Telford Limited, 1993.
[18]	WANG S S, ZHANG M H, QUEK S T. Effect of high strain rate loading on compressive behaviour of fibre-reinforced high-strength concrete [J]. Magazine of Concrete Research, 2011, 63(11): 813–827. doi: 10.1680/macr.2011.63.11.813
[19]	HAO Y, HAO H. Dynamic compressive behaviour of spiral steel fibre reinforced concrete in split Hopkinson pressure bar tests [J]. Construction and Building Materials, 2013, 48: 521–532. doi: 10.1016/j.conbuildmat.2013.07.022
[20]	SUN X W, ZHAO K, LI Y C, et al. A study of strain-rate effect and fiber reinforcement effect on dynamic behavior of steel fiber-reinforced concrete [J]. Construction and Building Materials, 2018, 158: 657–669. doi: 10.1016/j.conbuildmat.2017.09.093
[21]	WANG Y H, WANG Z D, LIANG X Y, et al. Experimental and numerical studies on dynamic compressive behavior of reactive powder concretes [J]. Acta Mechanica Solida Sinica, 2008, 21(5): 420–430. doi: 10.1007/s10338-008-0851-0
[22]	SU Y, LI J, WU C Q, et al. Effects of steel fibres on dynamic strength of UHPC [J]. Construction and Building Materials, 2016, 114(1): 708–718.
[23]	WANG Z L, LIU Y S, SHEN R F. Stress–strain relationship of steel fiber-reinforced concrete under dynamic compression [J]. Construction and Building Materials, 2008, 22(5): 811–819. doi: 10.1016/j.conbuildmat.2007.01.005
[24]	ACI Committee 446. Report on dynamic fracture of concrete [R]. Michigan: American Concrete Institute, 2004.
[25]	MALVAR L J, ROSS C A. Review of strain rate effects for concrete in tension [J]. Aci Materials Journal, 1998, 95(6): 735–739.
[26]	THOMAS R J, SORENSEN A D. Review of strain rate effects for UHPC in tension [J]. Construction and Building Materials, 2017, 153: 846–856. doi: 10.1016/j.conbuildmat.2017.07.168
[27]	PARK J K, KIM S W, KIM D J. Matrix-strength-dependent strain-rate sensitivity of strain-hardening fiber-reinforced cementitious composites under tensile impact [J]. Composite Structures, 2017, 162: 313–324. doi: 10.1016/j.compstruct.2016.12.022
[28]	PARK S H, KIM D J, KIM S W. Investigating the impact resistance of ultra-high-performance fiber-reinforced concrete using an improved strain energy impact test machine [J]. Construction and Building Materials, 2016, 125: 145–159. doi: 10.1016/j.conbuildmat.2016.08.027
[29]	赵春风, 王强, 王静峰, 等. 近场爆炸作用下核电厂安全壳穹顶钢筋混凝土板的抗爆性能 [J]. 高压物理学报, 2019, 33(2): 025101. doi: 10.11858/gywlxb.20180598 ZHAO C F, WANG Q, WANG J F, et al. Blast resistance of containment dome reinforced concrete slab in NPP under close-in explosion [J]. Chinese Journal of High Pressure Physics, 2019, 33(2): 025101. doi: 10.11858/gywlxb.20180598