Compressibility of FeNiP under High Pressure
-
摘要: 利用金刚石压腔技术和原位同步辐射X射线衍射技术，对FeNiP（
Pˉ62m ）的压缩性进行了实验研究。常温下，FeNiP在0～23.4 GPa压力范围内保持Pˉ62m 结构不变。用Birch-Murnaghan状态方程对单位晶胞体积随压力的变化关系（p-V关系）进行拟合，得到：体积模量K0=153(2) GPa，体积模量微商K′0 = 5.7(2)，零压下晶胞体积V0 = 101.6(1) Å3；或K0 = 167(1) GPa，K′0 = 4.0（固定值），V0 = 101.5(1) Å3。与Fe2P相比，FeNiP的体积模量更小，呈现出与Fe2P相反、与Ni2P相同的轴向压缩各向异性，据此探讨了Ni对(Fe,Ni)2P压缩性的影响。应用当前实验结果，估算了FeNiP、Fe2P、Fe3P、Fe2.15Ni0.85P和Fe3S在月球外核温压条件下的密度，通过与γ-Fe及月球外核密度的比较，得出Ni的加入会使“Fe-轻元素”体系的密度更接近月球外核密度，进一步阐释以多元合金体系（如Fe-Ni-S-P）为对象来研究行星核部物质组成更具合理性。Abstract: Compressibility of FeNiP (Pˉ62m ) has been studied up to 23.4 GPa by using diamond anvil cells (DAC) combined with in situ synchrotron X-ray diffraction (XRD) at room temperature. FeNiP remains the hexagonal structure at experimental pressure range. The pressure-volume (p-V) data has been fitted by the Birch-Murnaghan (B-M) equation of state, yielding K0 = 153(2) GPa,K′0 = 5.7(2), V0 = 101.6(1) Å3 or K0 = 167(1) GPa,K′0 = 4.0 (fixed), V0 = 101.5(1) Å3. FeNiP has smaller bulk modulus than Fe2P, and shows analogous axial compressibility to Ni2P. This might result from nickel’s doping effect on elastic properties of (Fe,Ni)2P. The densities of FeNiP, Fe2P, Fe3P, Fe2.15Ni0.85P and Fe3S have been estimated under the pressure-temperature conditions commensurate to the Moon’s outer core. The comparison shows that the doping of nickel could make (Fe,Ni)2P and (Fe,Ni)3P’s density approaching that of the Moon’s outer core.-
Key words:
- FeNiP /
- diamond anvil cell /
- X-ray diffraction /
- Moon /
- outer core
-
海床已成为一个全新的冲突领域，近海底爆炸可以对海底光缆、海底管道等设施造成严重的破坏，其破坏过程涉及近海底反射、多相流掺混、结构与流体的耦合作用，开展近海底爆炸冲击波时空演化规律研究对于水中兵器研制及海底设施抗爆抗冲击设计具有重要的意义。
目前，国内外对近海底水下爆炸研究已经取得了一定成果。基于Kelvin的冲击理论，Blake等[1]得出了判断自由面附近气泡射流方向和气泡运动方向的方法，该方法对近壁面也同样适用。张永坤[2]对沉底水雷在水下爆炸作用下的毁伤情况进行了研究，总结了水雷在不同情况下被毁伤的特点，为提高水下灭雷武器的作战能力奠定了理论基础。邵建军等[3]基于相似理论，研究了海底对爆炸的影响，发现海底底质变化显著影响水下爆炸的冲击波能和气泡能。杨莉等[4–7]在泥底、砂底及石底3种条件下进行了沉底爆炸试验，发现在3种底质条件下，气泡后期溃灭形态存在差异，最大峰值压力通常出现在装药靠近水底面一侧的斜上方，对于爆距较小的水底面测点，反射波追上入射波会形成马赫波。黄潇等[8]采用镜像法模拟海底边界的影响，发现自由场爆炸气泡比近海底爆炸气泡对潜艇施加的总纵弯矩更大。姚熊亮等[9]基于光滑粒子流体动力学（smooth particle hydrodynamics，SPH）方法模拟沉底水下爆炸，研究了水底底质厚度和炸药当量对冲击波压力的影响。邵宗战等[10]提出了沉底水雷海上爆炸威力的测量方法，给出了冲击波峰值压力的拟合方法及爆炸能量计算方法。前人在近海底水下爆炸冲击波载荷分析方面已取得了一些成果，然而，针对不同底质条件下近海底水下爆炸冲击波载荷时空分布规律的定量研究尚不充分。
本研究拟采用耦合欧拉-拉格朗日（coupled Eulerian-Lagrangian，CEL）方法建立近海底水下爆炸模型，探讨不同底质条件下近海底爆炸冲击波的时空分布规律，以期为近海底设施的抗爆抗冲击结构设计提供支撑。
1. 数值模型与数值验证
1.1 状态方程
本研究采用TNT炸药，爆轰产物通过JWL方程[11]描述
p=A(1−ωR1v)e−R1v+B(1−ωR2v)e−R2v+ωev (1) 式中：A、B、R1、R2及
ω 为与炸药状态有关的常数，v为爆轰产物的相对比容，e为炸药单位质量所蕴含的内能。TNT炸药的材料参数及JWL状态方程参数如表1[12]所示，其中：ρ为密度，D为爆速。ρ/(g·cm−3) D/(km·s−1) A/GPa B/GPa R1 R2 ω e/(kJ·g−1) 1.630 6.93 371.2 3.21 4.15 0.95 0.3 4.29 水采用Mie-Grüneisen状态方程描述，表达式[11]为
p=pH(1−Γ0η/2)+Γ0ρ0Em (2) 式中：pH为Hugoniot压力；ρ0为初始密度；Em为单位质量内能；Γ0为Grüneisen常数；η为名义体积压缩应变，
η=1−ρ0/ρ 。pH的表达式[11]为pH=ρ0C20η(1−Sη)2 (3) us=C0+Sup (4) 式中：us为冲击速度，up为粒子速度，S和C0为us-up曲线参数。水的状态方程参数列于表2[12]。
ρ/(g·cm−3) C0/(km·s−1) S Γ0 1.024 1.483 1.75 0.28 在数值模型中，设空气为理想气体，其状态方程[11]为
p+pa=(γ−1)ρEm (5) Em=cV(θ−θz) (6) 式中：γ为绝热指数，pa为外界压力，cV为比定容热容，
θ 为当前温度，θz 为绝对零度。空气的状态方程参数如表3[12]所示。ρ/(kg·m−3) γ pa/MPa cV/(J·g−1·K−1) 1.17 1.4 0.10 1.012 1.2 数值模型
为研究近海底水下爆炸冲击波的传播规律，建立了数值模型，欧拉域尺寸为15.0 m×6.5 m×15.0 m，模拟50 kg TNT在50 m水深处的近海底爆炸，TNT底部距海底0.05 m。将整个欧拉域的边界条件设置为流出无反射，海底底质采用模型1或模型2描述。模型1是一种较软的土壤模型，参考了Ambrosini等[13]和Luccioni等[14]的研究结果，具体参数见表4[13–14]，其中：E为杨氏模量，ν为泊松比，φ为内摩擦角，c为黏聚力。模型2为刚性固壁，通过在欧拉域中的海底底质区域放置拉格朗日刚体实现。
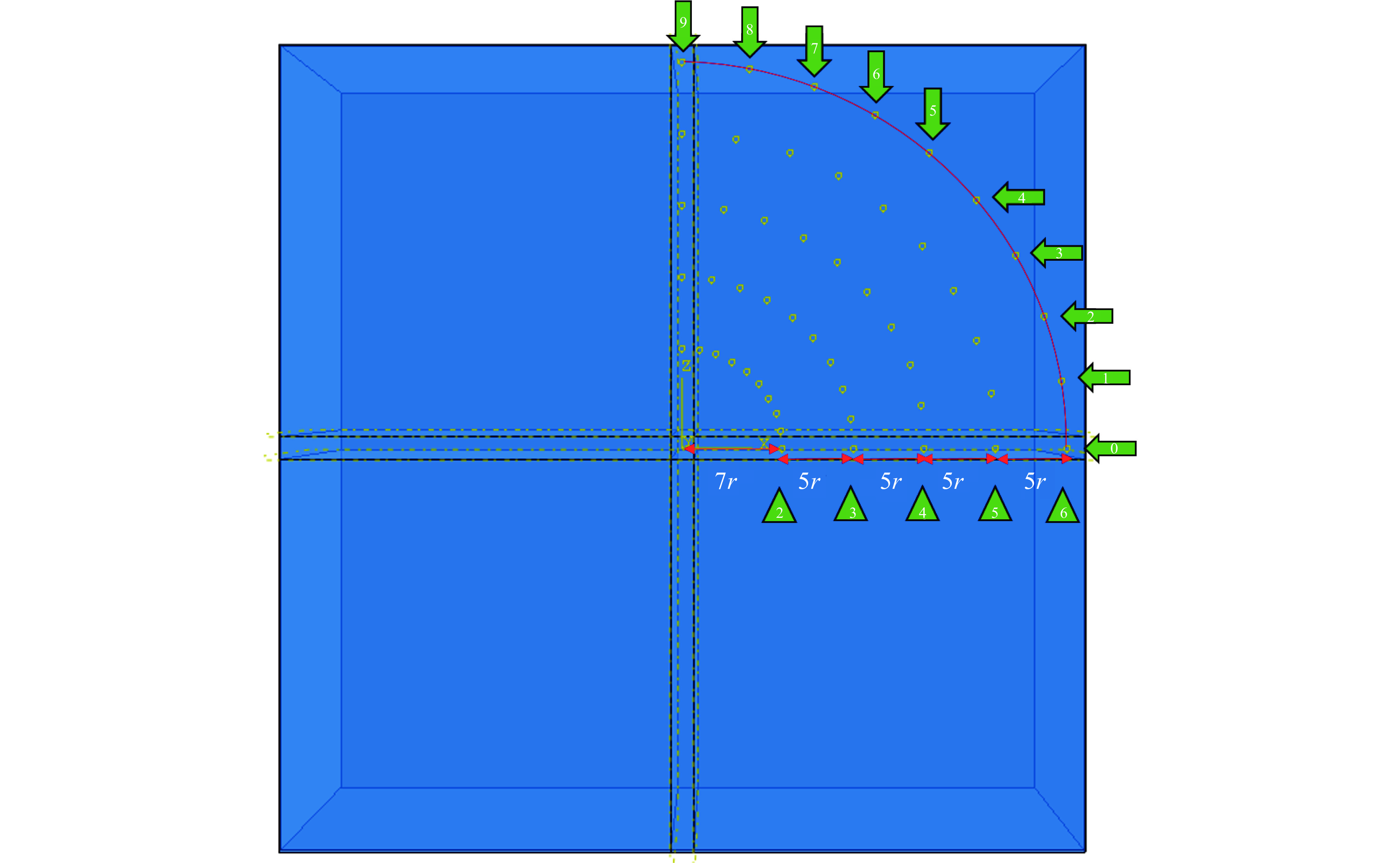
Seafloor sediment ρ/(kg·m−3) E/MPa ν φ/(°) c/MPa Model 1 1.4 50 0.3 24 0.1 为了更准确地获得同一位置的冲击波压力，在模型的不同位置设置测点，测点布局如图1所示。设装药半径为r，测点到爆心的距离为R，测点与水平方向的夹角为测点角度α。在距离爆心分别为7r、12r、17r、22r、27r，测点角度分别为0°、10°、20°、30°、40°、50°、60°、70°、80°、90°处布置测点，共计50个测点。为方便后续分析，用测点距爆心的距离（爆距）以及测点角度命名测点，爆距7r、12r、17r、22r、27r用数字2～6表示，测点角度0°～90°用数字0～9表示，例如：将爆距为7r、测点角度为0°的测点命名为2-0。
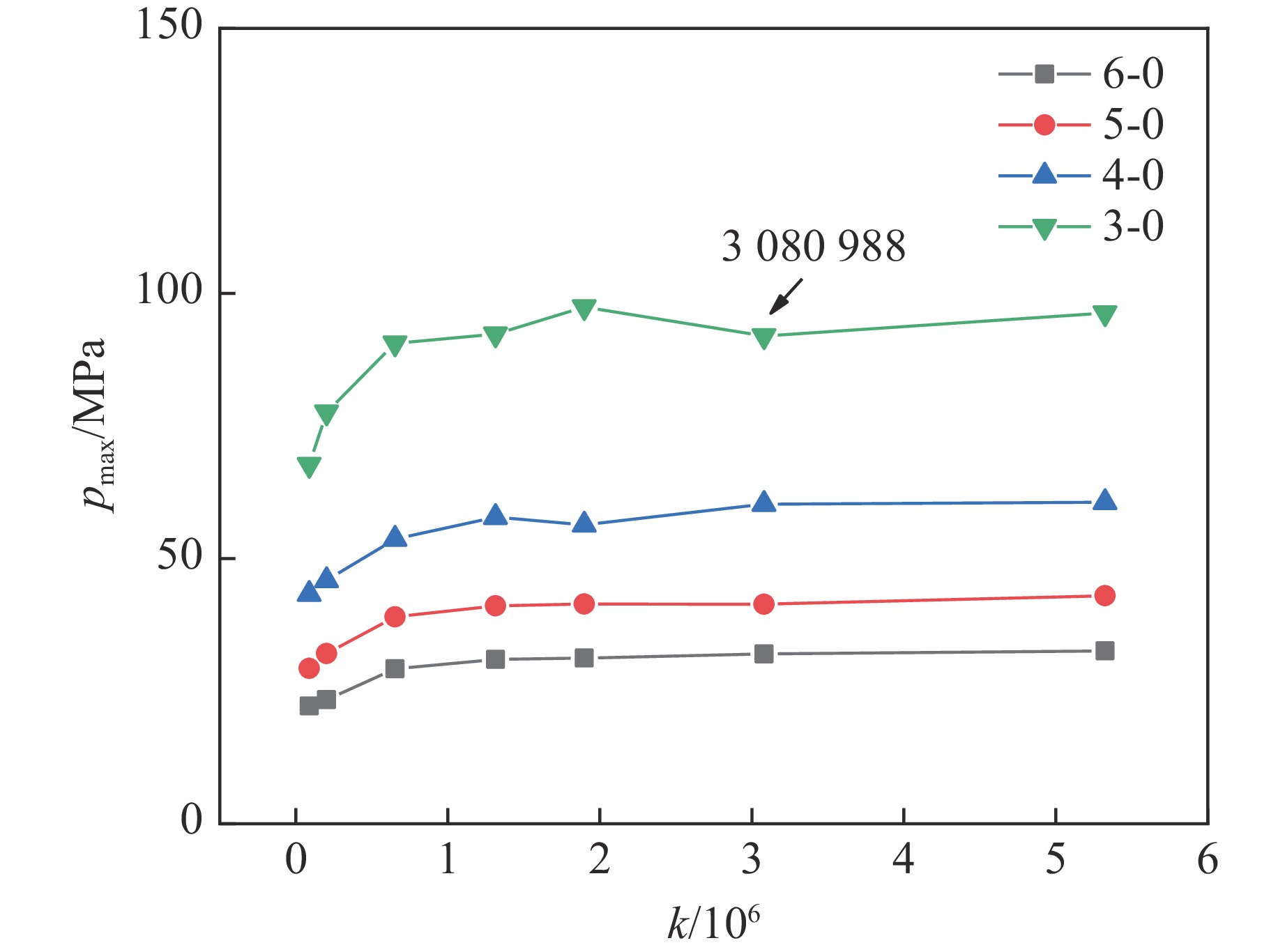
为验证结果的可靠性，对测点3-0、4-0、5-0、6-0测得的冲击波峰值压力进行收敛性分析，得到冲击波峰值压力pmax随网格数量k的变化曲线，如图2所示。当网格数量为3080988时，测点测得的冲击波峰值压力基本收敛。为此，选取网格数量为5324000来研究近海底水下爆炸冲击波的载荷特性。
图 2 不同网格数量下不同测点测得的压力峰值Figure 2. Peak pressures measured at different points and grid numbers1.3 数值验证
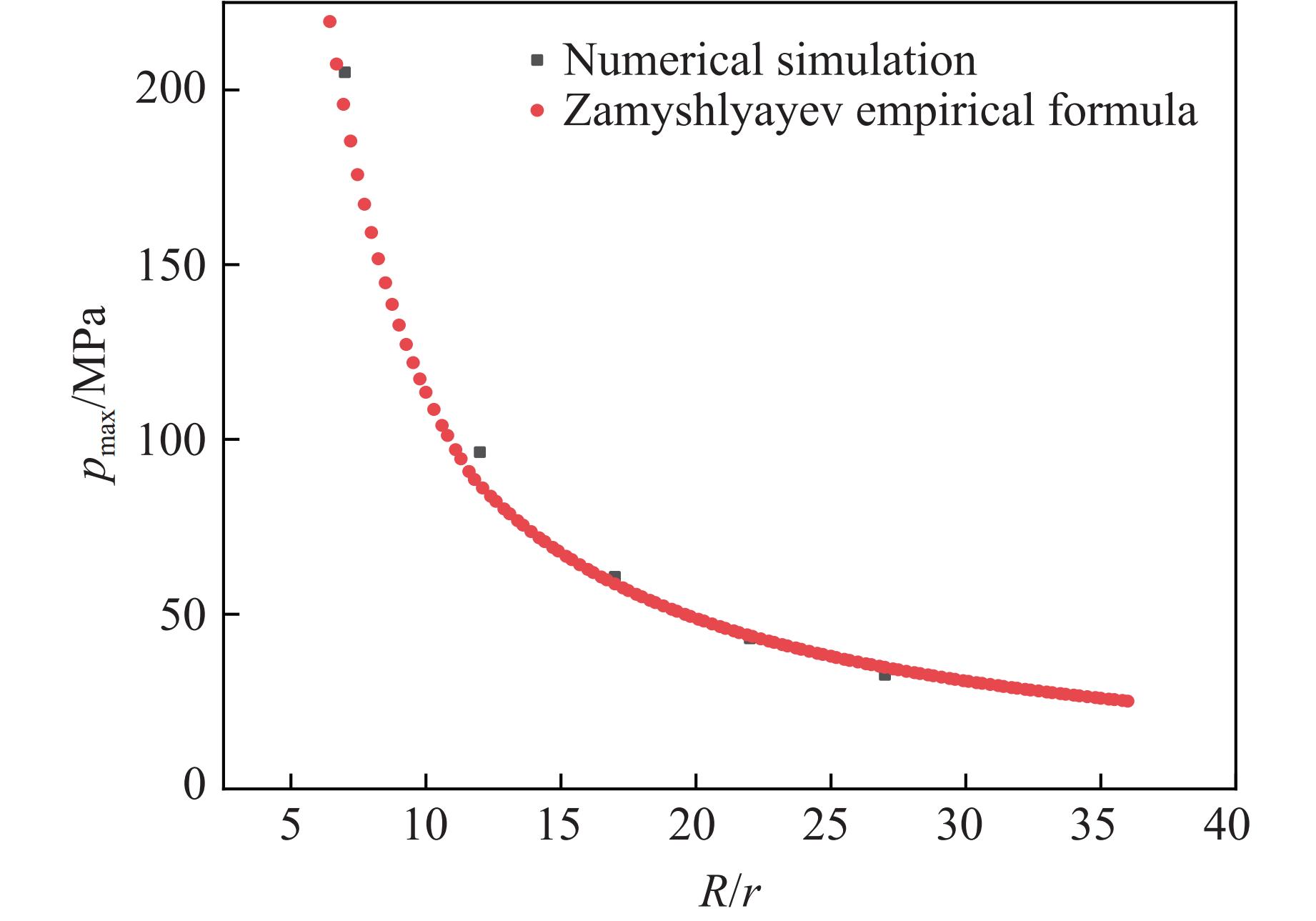
为验证模型的准确性，选取测点2-0、3-0、4-0、5-0、6-0在无海底底质时测得的峰值压力与Zamyshlyayev经验公式计算结果进行对比，如图3和表5所示。可以看出，数值模拟结果与经验公式拟合结果符合得较好，说明该模型能够用于近海底水下爆炸冲击波研究。
图 3 不同距离处冲击波峰值压力对比Figure 3. Comparison of peak pressure of shock waves at different distances表 5 数值模拟与经验公式结果对比Table 5. Comparison of numerical simulation and empirical formula resultsR/r Peak pressure/MPa Error/% Simulation Empirical formula 7 205.03 193.72 5.84 12 96.32 86.90 10.84 17 60.65 58.62 3.45 22 43.04 43.81 1.75 27 32.58 37.91 14.07 1.4 工况设置
为研究水深（H）及海底底质对近海底水下爆炸冲击波载荷特性的影响，仅改变海底底质及静水压力，其他条件保持不变，进行数值模拟，计算工况如表6所示，其中：工况1和工况2用于研究自由场与近海底冲击波压力的差异，工况2和工况3用于研究底质条件对近海底爆炸冲击波载荷特性的影响，工况2、工况4和工况5用于研究水深对近海底水下爆炸冲击波载荷特性的影响。
表 6 数值模拟工况设置Table 6. Settings of simulation casesCase H/m Seafloor sediment Explosive environment 1 50 Nothing Free field 2 50 Model 1 Near the seabed 3 50 Model 2 Near the seabed 4 100 Model 1 Near the seabed 5 150 Model 1 Near the seabed 2. 结果讨论与分析
2.1 自由场和近海底爆炸冲击波特性对比
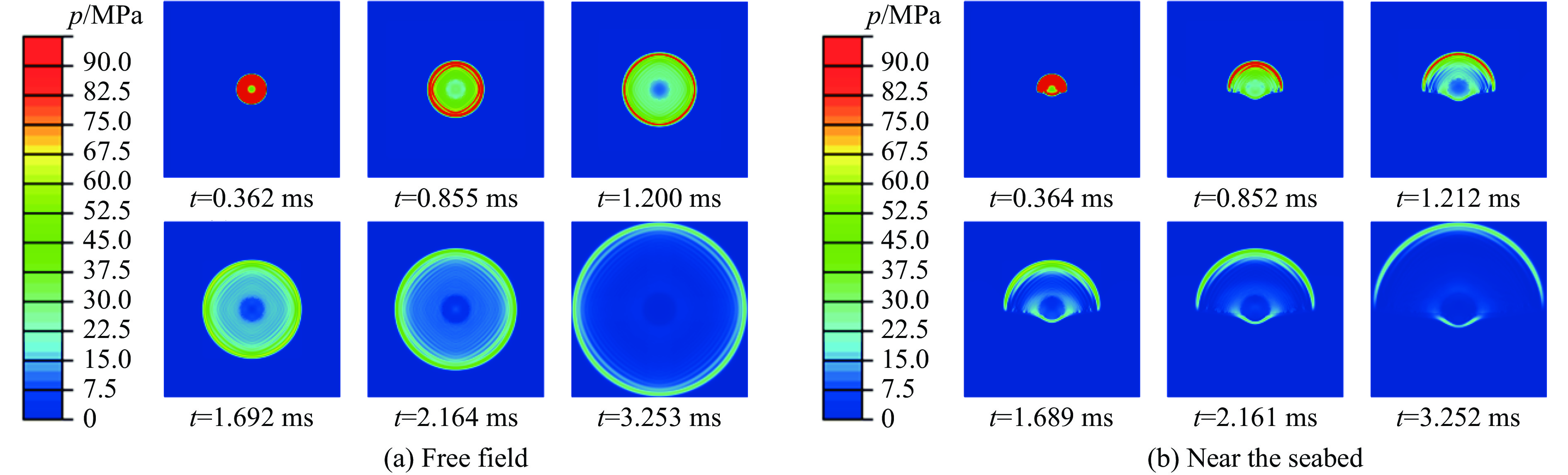
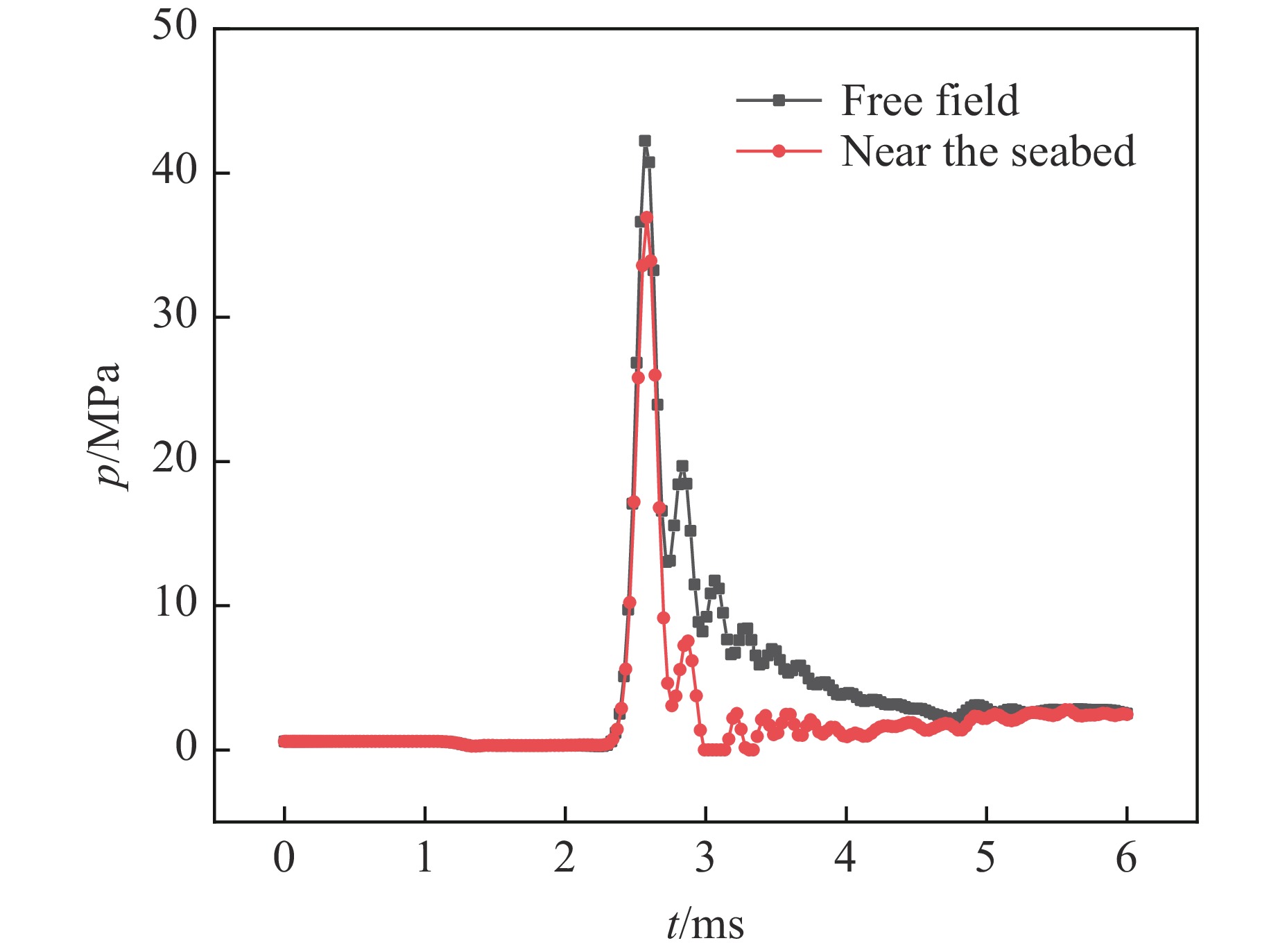
图4为工况1和工况2下不同时刻的冲击波压力云图，图5为测点5-3在工况1和工况2下测得的爆炸冲击波压力时程曲线。可以看出，自由场水下爆炸与近海底水下爆炸存在明显差别，受近海底条件的影响，近海底水下爆炸冲击波形状不是规则的圆形，冲击波波面上的压力峰值并非处处相等，而是越靠近海底区域，冲击波压力越小，测点5-3在近海底条件下测得的冲击波峰值压力及后续的脉动压力均小于自由场条件下测得的压力。由于水的冲击阻抗大于海底底质模型1的冲击阻抗，因此，水中冲击波在近海底的反射波为稀疏波，稀疏波追上冲击波波面将会导致冲击波压力降低；另外，海底底质模型1是一种较软且易变形的底质，冲击波作用在海底底质上，不仅会发生反射，还会发生透射，部分能量传递到海底底质中，使海底底质发生形变，冲击波能量也会发生损耗。因此，在近海底条件下，冲击波峰值压力和气泡脉动压力均小于自由场下的压力，理论与数值模拟结果相符。
图 4 自由场及近海底水下爆炸冲击波压力云图Figure 4. Pressure distribution of free-field and near-seabed underwater explosion shock wave
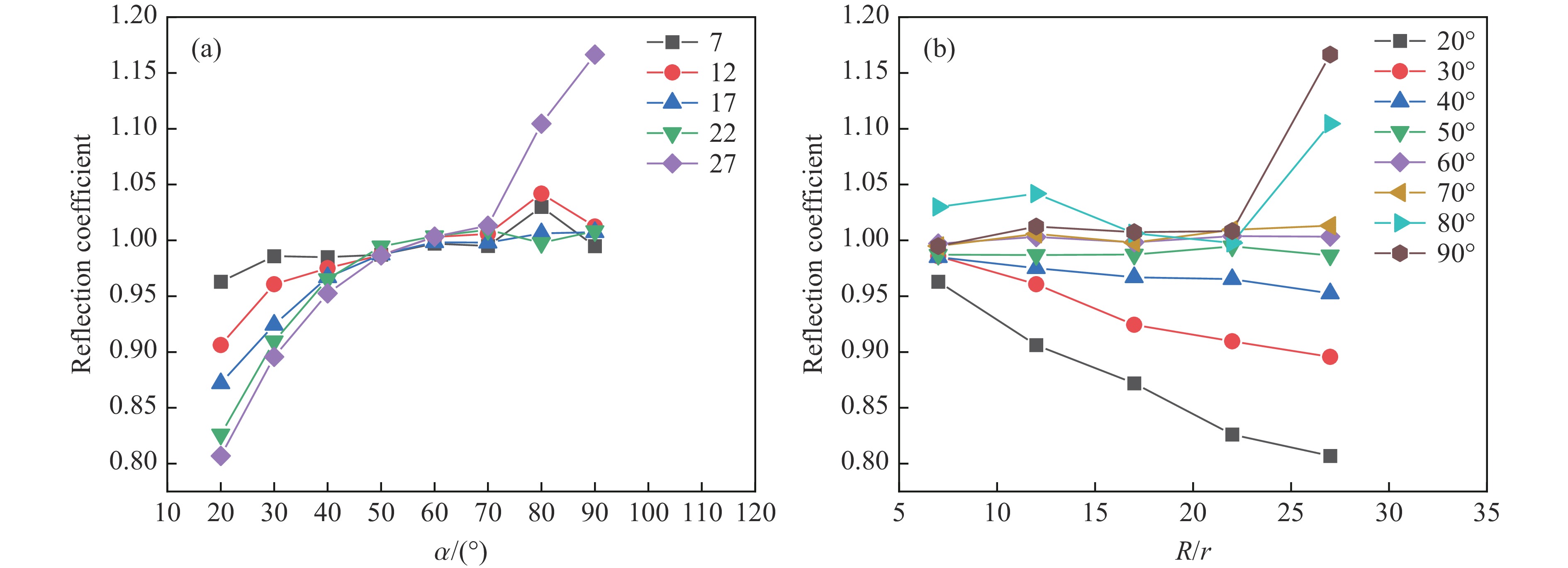
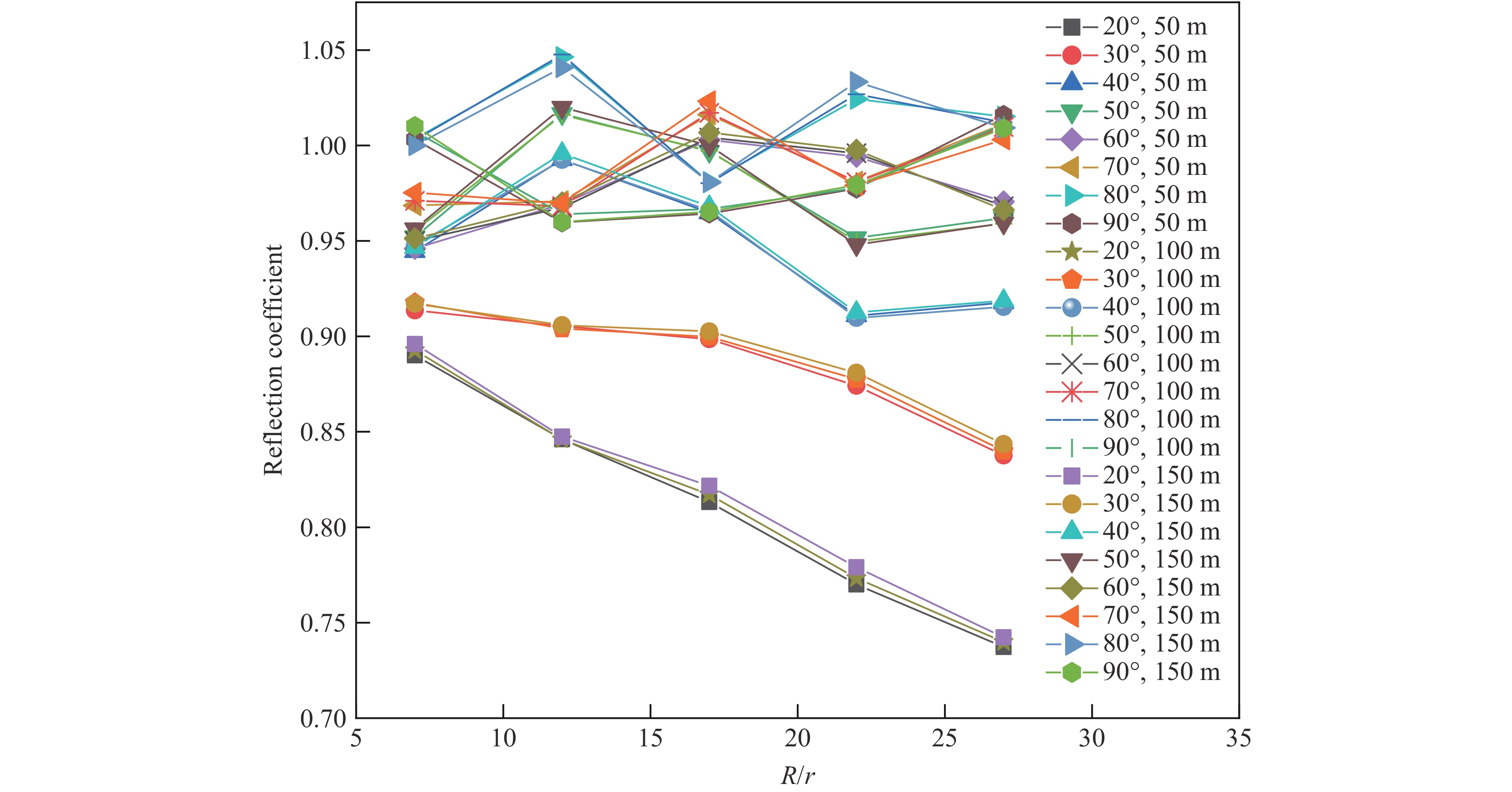
图 5 自由场及近海底工况下测点5-3的水下爆炸冲击波压力时程曲线Figure 5. Time history curves of shock wave pressure at measuring point 5-3 in free field and near seabed underwater explosion近海底水下爆炸时，靠近海底区域处的冲击波压力明显偏小，即不同测点角度（α）条件下的冲击波峰值压力存在差异。定义测点测得的冲击波峰值压力与自由场时的冲击波峰值压力之比为该测点的反射系数。计算所有测点的反射系数，结果如图6所示。当爆距比一定时，随着α的增大，反射系数也逐渐增大，即海底吸能现象和稀疏波的影响逐渐减小；α在0°～10°区间时，海底的吸能现象过于剧烈，故不对该角度范围进行深入研究；当α处于20°～30°区间时，测点的反射系数随爆距比增加呈减小趋势，即近海底反射的影响随爆距比的增大而增强；当α > 40°时，海底吸能现象不明显。
为了更细致地研究近海底反射规律，将自由场与近海底工况下测点2-2～测点2-9、测点3-2～测点3-9、测点4-2～测点4-9的冲击波峰值压力进行对比，结果如表7所示，可以看出：当α处于20°～30°时，近海底工况下，冲击波峰值压力是自由场工况下冲击波峰值压力的81%～91%；随着α的增大，测点距海底越来越远，近海底反射现象也越来越弱，近海底反射影响逐渐消失。
表 7 自由场与近海底测得的冲击波峰值压力对比Table 7. Comparison of peak pressures of free-field and near-seabed underwater explosion shock waveR/r α/(°) pmax/MPa Reflection
coefficientR/r α/(°) pmax/MPa Reflection
coefficientNear seabed Free field Near seabed Free field 7 20 179.29 201.44 0.89 12 60 92.73 95.67 0.97 7 30 186.21 203.80 0.91 12 70 95.00 97.86 0.97 7 40 193.43 204.80 0.94 12 80 98.33 93.98 1.05 7 50 194.98 204.80 0.95 12 90 92.42 96.30 0.96 7 60 192.78 203.80 0.95 17 20 47.78 58.76 0.81 7 70 195.10 201.43 0.97 17 30 53.24 59.25 0.90 7 80 202.46 201.74 1.00 17 40 56.52 58.58 0.96 7 90 196.41 195.79 1.00 17 50 58.40 58.58 1.00 12 20 82.82 97.87 0.85 17 60 59.44 59.27 1.00 12 30 86.62 95.67 0.91 17 70 61.07 60.10 1.02 12 40 93.96 94.66 0.99 17 80 60.00 61.17 0.98 12 50 96.23 94.65 1.02 17 90 57.77 59.91 0.96 2.2 海底底质对近海底爆炸冲击波载荷特性的影响
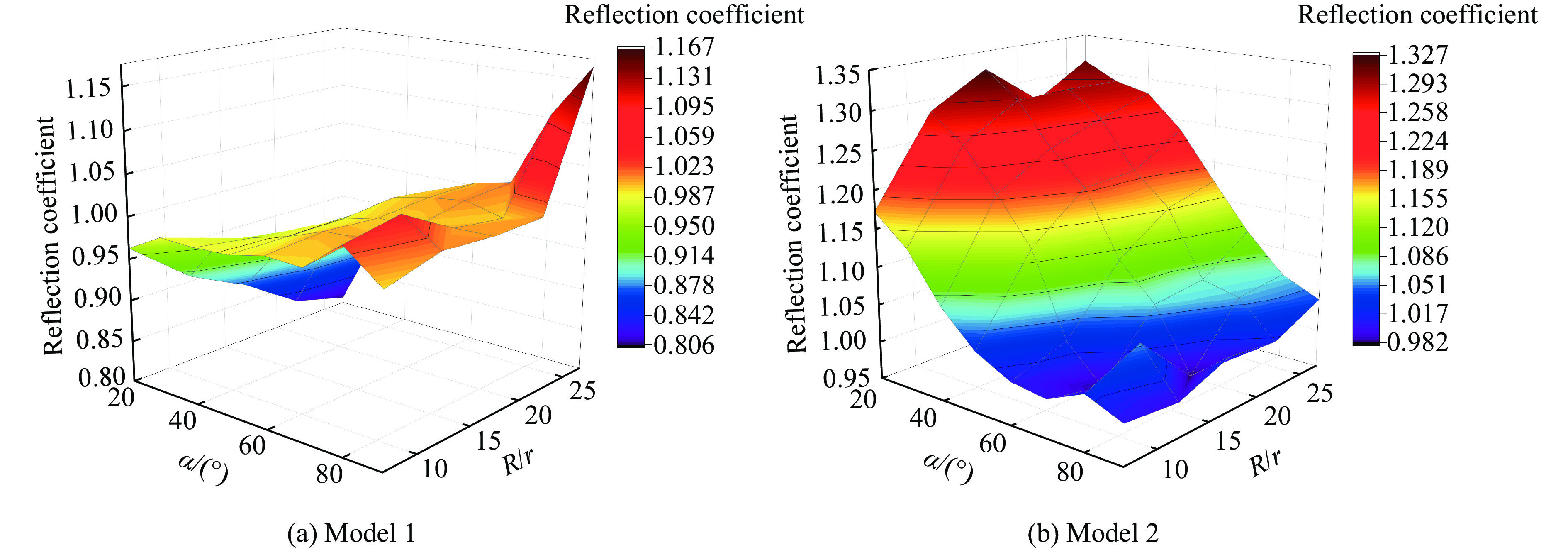
图7显示了工况2和工况3下相同测点测得的近海底冲击波反射系数对比。可以发现，2种底质条件下冲击波传播规律存在明显差异：当底质为模型1时，由于近海底反射波为稀疏波，稀疏波追上冲击波波面将导致冲击波峰值压力变小，且海底底质模型1较软，吸能作用较强，因此，绝大部分测点的反射系数小于1；当海底底质为模型2时，受刚固边界的影响，近海底反射波为压缩波，压缩波追上冲击波波面会导致冲击波峰值压力增大，且海底底质模型2的吸能作用较弱，绝大部分测点的反射系数大于1。无论底质是模型1还是模型2，反射系数异常均发生在爆距比为7～15、α在60°～90°范围内。
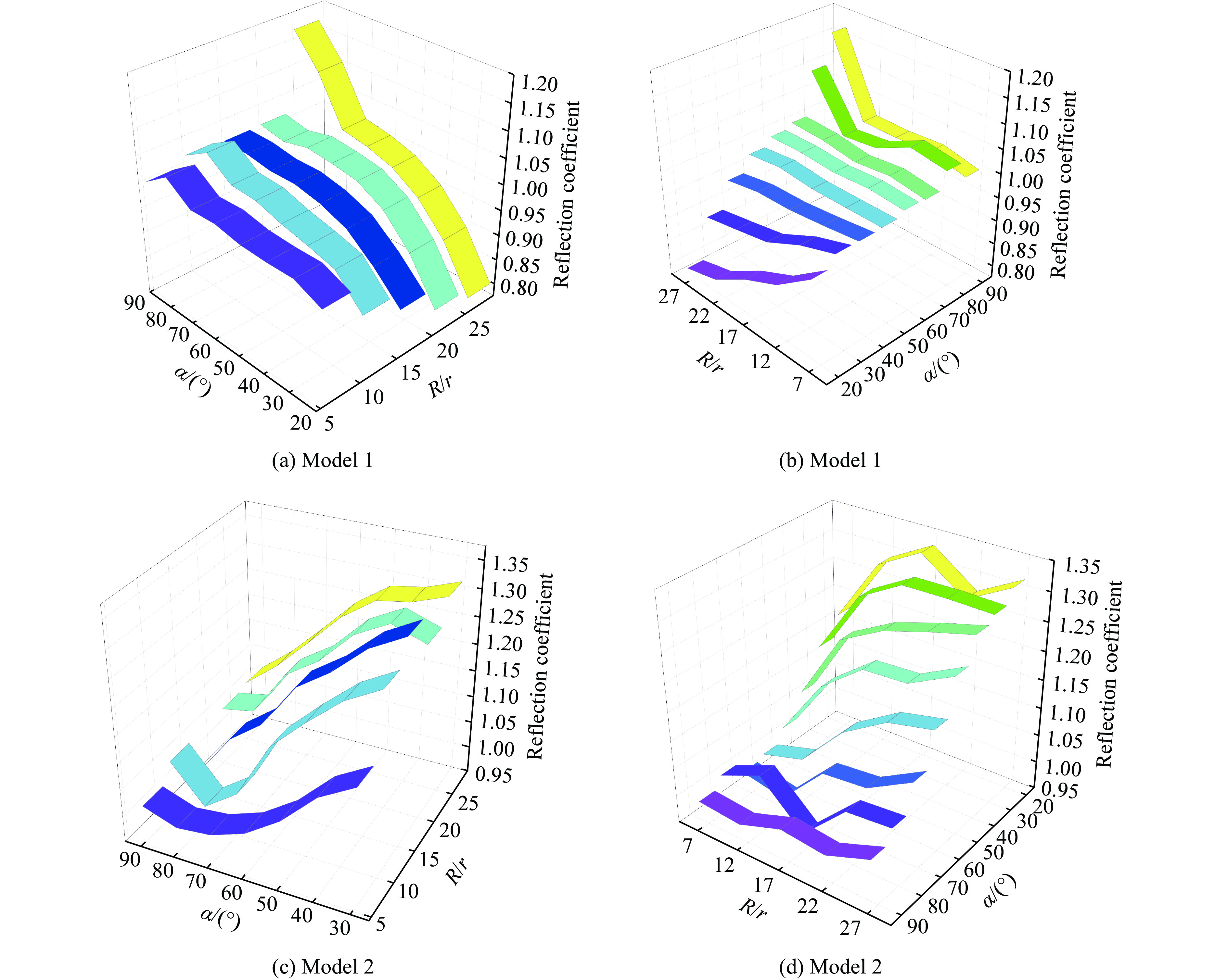
图 7 不同底质条件下测点反射系数的对比Figure 7. Comparison of reflection coefficient of test points under different substrate conditions为更清楚地研究不同底质条件对反射系数的影响，将不同底质条件下反射系数随测点角度和爆距比的变化绘制成图8。由图8(a)可知，当海底底质为模型1、爆距比保持不变时，反射系数随测点角度的增大呈增大趋势。由图8(c)可知，当海底底质为模型2、爆距比一定时，反射系数随测点角度的增大呈减小趋势。
图 8 不同底质条件下反射系数分布对比Figure 8. Comparison of reflection coefficient distribution under different substrate conditions当海底底质为模型1时，海底底质较软，海底反射稀疏波，对TNT爆炸冲击波的吸收较强，因此，近海底冲击波峰值压力减小，反射系数小于1是常态。图8(a)显示，当爆距比一定时，测点角度越大，反射系数就越大，因而可以推断，海底底质对不同测点角度处反射系数的影响随测点角度的增大而减弱。当海底底质为模型2时，海底底质较硬，海底反射压缩波，对冲击波的吸收很弱，因此，近海底冲击波峰值压力更大，反射系数大于1是常态。图8(c)显示，当爆距比一定时，测点角度越大，反射系数越小，同样可以得到海底底质对不同测点角度处反射系数的影响随测点角度增大而减弱的结论。
从图8(b)可以看出，当测点角度处于20°～40°区间时，不同测点测得的近海底冲击波反射系数随着爆距比的增大而减小；而图8(d)显示，当测点角度处于20°～60°区间时，不同测点测得的近海底冲击波反射系数随着爆距比的增大而增大。由于2种底质对近海底冲击波反射系数的影响截然相反，因此，综合图8(b)和图8(d)可以看出，测点角度在一定范围内时，海底底质对反射系数的影响随着测点爆距比的增大而增强。当测点角度超出该范围时，该现象减弱甚至消失。综合图8(a)～图8(d)可以发现，虽然海底底质发生了变化，不同海底底质对冲击波的影响效果不同，但是测点反射系数受显著影响的区域均集中在20°～50°范围。
为研究近海底水下爆炸反射波对冲击波的影响是否与起爆深度有关，将底质条件为模型1时不同水深处各测点的反射系数进行对比，结果如图9所示。从图9可以看出，随着水深的增加，同一测点处的反射系数基本一致，说明水深并不能显著影响反射系数，即水深的变化并不会对近海底反射现象造成显著影响，也不会对海底底质的吸能作用造成显著影响，近海底反射稀疏波对冲击波的削减作用并不会随着静水压力的变化而发生显著变化。
图 9 不同水深反射系数随测点角度及爆距比的变化关系Figure 9. Relationship between reflection coefficient and explosion distance ratio in different water depths3. 结 论
基于CEL方法建立了近海底水下爆炸数值模型，对近海底爆炸冲击波的时空分布规律进行了研究，探究了海底底质和水深对近海底爆炸冲击波载荷特性的影响规律，得到如下结论。
(1) 近海底水下爆炸冲击波载荷特性与自由场明显不同。当测点角度为20°～30°时，近海底反射系数为0.81～0.91；随着测点角度进一步增大，近海底反射的影响逐渐减弱；当测点角度达到80°～90°时，近海底反射的影响基本消失。
(2) 在一定的角度范围内，近海底反射的影响随着爆距比的增大而增强，超出该角度范围后，该现象基本消失。改变底质时，近海底反射的影响使冲击波峰值压力增强或减弱，并且影响区域基本一致。水深对近海底水下爆炸反射系数无显著影响。
(3) 海底底质材料属性不同时，其对爆炸冲击波的吸收作用也存在差异，近海底水下爆炸反射波的种类也不一致。当海底底质较硬时，海底底质对冲击波的吸收作用相对较弱，反射波为压缩波，致使测点角度在20°～50°范围内的冲击波峰值压力增大，当测点角度在20°～50°范围内且测点角度一定时，反射系数随爆距比的增大而增大；当海底底质较软时，海底底质对冲击波的吸收作用较强，近海底反射波为稀疏波，致使20°～50°角度范围内冲击波峰值压力减小，当测点角度在20°～50°范围内且测点角度一定时，反射系数随爆距比的增大而减小。海底底质对反射系数的影响区域主要集中在20°～50°测点范围内，超出该范围时，海底底质对反射系数的影响随着测点角度的增大而逐渐消失，海底底质对反射系数的影响随爆距比的增大而增强的现象也随测点角度的增大而逐渐消失。
-
图 1 FeNiP在Run 1（a）和Run 2（b）两次原位高压同步辐射XRD实验中部分压力条件下的衍射图谱（星号表示铼的衍射峰）
Figure 1. Representative synchrotron radiation XRD patterns of FeNiP at various pressures in Run 1 (a) and Run 2 (b)(The asterisks mark diffraction peaks of rhenium)
图 2 FeNiP的归一化轴长（a/a0和c/c0）及归一化轴长比值（（c/c0）/（a/a0））随压力的变化关系（Fe2P和Ni2P的p-（c/c0）/（a/a0）数据[23–24]也被列出用于比较）
Figure 2. Normalized axis length of FeNiP (a/a0 and c/c0) and normalized ratio of axis length ((c/c0)/(a/a0)) with the change of pressure (p-(c/c0)/(a/a0) data of Fe2P and Ni2P[23–24] are plotted for comparison.)
图 4 FeNiP的欧拉应变-标准化应力（εE-FE）关系
Figure 4. Eularian strain-normalized stress (εE-FE) plot of the p-V data based on the B-M equation of state
图 5 月球外核温压（4.8～5.0 GPa，1 800 K）条件下FeNiP、Fe2P、Fe3P、Fe2.15Ni0.85P、Fe3P和Fe3S的估算密度（γ-Fe和月球外核密度也绘出用以比较）
Figure 5. Calculated density of FeNiP, Fe2P, Fe3P, Fe2.15Ni0.85P, Fe3P, and Fe3S under the pressure-temperature conditions commensurate to the Moon’s outer core（4.8–5.0 GPa, 1 800 K）（Density of γ-Fe and the Moon’s outer core are plotted for comparison.）
表 1 FeNiP在不同压力下的晶胞参数
Table 1. Pressure dependence of unit-cell parameters of FeNiP
p/GPa a/Å c/Å V/Å3 p/GPa a/Å c/Å V/Å3 0.000 1 5.845（1） 3.433（1） 101.6（1） 7.9（1） 5.760（1） 3.379（1） 97.1（1） 1.0（1） 5.829（1） 3.426（1） 100.8（1） 9.1（1） 5.750（1） 3.372（1） 96.6（1） 2.8（1） 5.811（1） 3.413（1） 99.8（1） 10.5（1） 5.737（1） 3.362（1） 95.8（1） 3.3（1） 5.806（1） 3.410（1） 99.5（1） 11.6（1） 5.729（2） 3.353（2） 95.3（1） 3.8（1） 5.798（1） 3.406（1） 99.2（1） 13.5（1） 5.713（1） 3.346（1） 94.6（1） 4.8（1） 5.790（1） 3.400（1） 98.7（1） 18.1（1） 5.682（1） 3.320（1） 92.8（1） 5.7（1） 5.783（1） 3.392（1） 98.2（1） 20.7（1） 5.660（1） 3.306（1） 91.7（1） 6.6（1） 5.772（1） 3.385（1） 97.7（1） 23.4（1） 5.647（1） 3.292（1） 90.9（1） Note: Data of 1.0–9.1 GPa are from Run1, and data of 10.5–23.4 GPa are from Run 2; numbers in parentheses represent errors in the last digit. 
下载: 导出CSV
表 3 FeNiP、Fe2P、Fe3P、Fe2.15Ni0.85P和Fe3S的高温B-M状态方程参数
Table 3. High-temperature B-M equation of state parameters for FeNiP, Fe2P, Fe3P, Fe2.15Ni0.85P, and Fe3S
Material T0/K V0,T0/Å3 K0T0/GPa K′0T0 (∂KT∂T)p/(GPa⋅K−1) α0/（10–5 K–1） α1/（10–8 K–2） FeNiP 300 101.5 167 4.0 –3.75×10–2[54] 3.0[54] 2.8[54] Fe2P 300[23] 103.16[23] 175[23] 4.0[23] –3.75×10–2[54] 3.0[54] 2.8[54] Fe3P 300[20] 366.9[20] 161[20] 4.0[20] –3.75×10–2[54] 3.0[54] 2.8[54] Fe2.15Ni0.85P 300[21] 365.8[21] 185[21] 4.0[21] –3.75×10–2[54] 3.0[54] 2.8[54] Fe3S 300[54] 377.01[54] 150[54] 4.0[54] –3.75×10–2[54] 3.0[54] 2.8[54]
下载: 导出CSV
-
[1] BIRCH F. Density and composition of mantle and core [J]. Journal of Geophysical Research, 1964, 69: 4377–4388. doi: 10.1029/JZ069i020p04377 [2] DREIBUS G, WÄNKE H. Mars, a volatile-rich planet [J]. Meteoritics, 1985, 20: 367–381. [3] ANTONANGELI D, MORARD G, SCHMERR N C, et al. Toward a mineral physics reference model for the Moon’s core [J]. Proceedings of the National Academy of Sciences of the United States of America, 2015, 112(13): 3916–3919. doi: 10.1073/pnas.1417490112 [4] MCDONOUGH W F. Treatise on geochemistry: compositional model for the Earth’s core [M]. New York: Elsevier, 2003: 547–568. [5] FEI Y, PREWITT C T, MAO H K, et al. Structure and density of FeS at high pressure and high temperature and the internal structure of Mars [J]. Science, 1995, 268(5219): 1892–1894. doi: 10.1126/science.268.5219.1892 [6] STEENSTRA E S, LIN Y H, RAI N, et al. Carbon as the dominant light element in the lunar core [J]. American Mineralogist, 2017, 102(1): 92–97. doi: 10.2138/am-2017-5727 [7] SKÁLA R, CÍSAŘOVÁ I. Crystal structure of meteoritic schreibersites: determination of absolute structure [J]. Physics and Chemistry of Minerals, 2005, 31(10): 721–732. doi: 10.1007/s00269-004-0435-6 [8] BUSECK P R. Phosphide from metorites: barringerite, a new iron-nickel mineral [J]. Science, 1969, 165(3889): 169–171. doi: 10.1126/science.165.3889.169 [9] BRITVIN S N, RUDASHEVSKY N S, KRIVOVICHEV S V, et al. Allabogdanite, (Fe,Ni)2P, a new mineral from the Onello meteorite: the occurrence and crystal structure [J]. American Mineralogist, 2002, 87(8/9): 1245–1249. [10] PRATESI G. Icosahedral coordination of phosphorus in the crystal structure of melliniite, a new phosphide mineral from the Northwest Africa 1054 acapulcoite [J]. American Mineralogist, 2006, 91(2/3): 451–454. [11] REED S J B. Perryite in the kota-kota and south Oman enstatite chondrites [J]. Mineralogical Magazine and Journal of the Mineralogical Society, 1968, 36(282): 850–854. doi: 10.1180/minmag.1968.036.282.13 [12] MA C, BECKETT J R, ROSSMAN G R. Discovery of a new phosphide mineral, monipite (MoNiP), in an Allende Type B1 CAI [C]//72nd Meeting of the Meteoritical Society, 2009, 44(Suppl 7): A127. [13] 梅清风, 杨进辉. 地球早期演化的Hf-W同位素制约 [J]. 岩石学报, 2018, 34(1): 207–216.MEI Q F, YANG J H. Hf-W isotopic constraints on early evolution of the Earth [J]. Acta Petrologica Sinica, 2018, 34(1): 207–216. [14] WOOD B J, WALTER M J, WADE J. Accretion of the Earth and segregation of its core [J]. Nature, 2006, 441(7095): 825–833. doi: 10.1038/nature04763 [15] YIN Y, LI Z M, ZHAI S M. The phase diagram of the Fe-P binary system at 3 GPa and implications for phosphorus in the lunar core [J]. Geochimica et Cosmochimica Acta, 2019, 254: 54–66. doi: 10.1016/j.gca.2019.03.037 [16] STEWART A J, SCHMIDT M W. Sulfur and phosphorus in the Earth’s core: the Fe-P-S system at 23 GPa [J]. Geophysical Research Letters, 2007, 34(13): L13201. [17] SHA L K. Whitlockite solubility in silicate melts: some insights into lunar and planetary evolution [J]. Geochimica et Cosmochimica Acta, 2000, 64(18): 3217–3236. doi: 10.1016/S0016-7037(00)00420-8 [18] STEENSTRA E S, VAN WESTRENEN W. Lunar core composition [M]//Encyclopedia of Lunar Science. Cham: Springer International Publishing, 2016: 1–6. [19] GU T T, FEI Y W, WU X, et al. Phase stabilities and spin transitions of Fe3(S1– xP x) at high pressure and its implications in meteorites [J]. American Mineralogist, 2016, 101(1): 205–210. doi: 10.2138/am-2016-5466 [20] GU T T, FEI Y W, WU X, et al. High-pressure behavior of Fe3P and the role of phosphorus in planetary cores [J]. Earth and Planetary Science Letters, 2014, 390: 296–303. doi: 10.1016/j.jpgl.2014.01.019 [21] HE X J, GUO J Z, WU X, et al. Compressibility of natural schreibersite up to 50 GPa [J]. Physics and Chemistry of Minerals, 2019, 46(1): 91–99. doi: 10.1007/s00269-018-0990-x [22] NISAR J, AHUJA R. Structure behavior and equation of state (EOS) of Ni2P and (Fe1– xNi x)2P (allabogdanite) from first-principles calculations [J]. Earth and Planetary Science Letters, 2010, 295(3/4): 578–582. [23] DERA P, LAVINA B, BORKOWSKI L A, et al. High-pressure polymorphism of Fe2P and its implications for meteorites and Earth’s core [J]. Geophysical Research Letters, 2008, 35(10): L10301. [24] DERA P, LAVINA B, BORKOWSKI L A, et al. Structure and behavior of the barringerite Ni end-member, Ni2P, at deep Earth conditions and implications for natural Fe-Ni phosphides in planetary cores [J]. Journal of Geophysical Research, 2009, 114(B3): B03201. [25] WU X, MOOKHERJEE M, GU T T, et al. Elasticity and anisotropy of iron-nickel phosphides at high pressures [J]. Geophysical Research Letters, 2011, 38(20): L20301. [26] DUBROVINSKY L, DUBROVINSKAIA N, BYKOVA E, et al. The most incompressible metal osmium at static pressures above 750 gigapascals [J]. Nature, 2015, 525: 226–229. doi: 10.1038/nature14681 [27] HAMMERSLEY A P, SVENSSON S O, HANFLAND M, et al. Two-dimensional detector software: from real detector to idealised image or two-theta scan [J]. High Pressure Research, 1996, 14(4/5/6): 235–248. [28] MAO H K, XU J, BELL P M. Calibration of the ruby pressure gauge to 800 kbar under quasi-hydrostatic conditions [J]. Journal of Geophysical Research, 1986, 91(B5): 4673. doi: 10.1029/JB091iB05p04673 [29] SETO Y, NISHIO-HAMANE D, NAGAI T, et al. Development of a software suite on X-ray diffraction experiments [J]. The Review of High Pressure Science and Technology, 2010, 20(3): 269–276. doi: 10.4131/jshpreview.20.269 [30] TOBY B H. EXPGUI, a graphical user interface for GSAS [J]. Journal of Applied Crystallography, 2001, 34(2): 210–213. doi: 10.1107/S0021889801002242 [31] ANGEL R J, ALVARO M, GONZALEZ-PLATAS J. EosFit7c and a Fortran module (library) for equation of state calculations [J]. Zeitschrift für Kristallographie: Crystalline Materials, 2014, 229(5): 1165–1176. [32] FUJII H, HŌKABE T, FUJIWARA H, et al. Magnetic properties of single crystals of the system (Fe1-xNix)2P [J]. Journal of the Physical Society of Japan, 1978, 44(1): 96–100. doi: 10.1143/JPSJ.44.96 [33] MAEDA Y, TAKASHIMA Y. Mössbauer studies of FeNiP and related compounds [J]. Journal of Inorganic and Nuclear Chemistry, 1973, 35(6): 1963–1969. doi: 10.1016/0022-1902(73)80134-4 [34] BIRCH F. Finite elastic strain of cubic crystals [J]. Physical Review, 1947, 71(11): 809. doi: 10.1103/PhysRev.71.809 [35] ANGEL R J. Equations of state [J]. Reviews in Mineralogy and Geochemistry, 2000, 41(1): 35–59. doi: 10.2138/rmg.2000.41.2 [36] KLOTZ S, CHERVIN J C, MUNSCH P, et al. Hydrostatic limits of 11 pressure transmitting media [J]. Journal of Physics D: Applied Physics, 2009, 42(7): 075413. doi: 10.1088/0022-3727/42/7/075413 [37] DEWAELE A, LOUBEYRE P. Pressurizing conditions in helium-pressure-transmitting medium [J]. High Pressure Research, 2007, 27(4): 419–429. doi: 10.1080/08957950701659627 [38] RUEFF J P, RAYMOND S, YARESKO A, et al. Pressure-induced f-electron delocalization in the U-based strongly correlated compounds UPd3 and UPd2Al3: resonant inelastic X-ray scattering and first-principles calculations [J]. Physical Review B, 2007, 76(8): 085113. doi: 10.1103/PhysRevB.76.085113 [39] DEWAELE A, LOUBEYRE P, OCCELLI F, et al. Quasihydrostatic equation of state of iron above 2 Mbar [J]. Physical Review Letters, 2006, 97(21): 215504. doi: 10.1103/PhysRevLett.97.215504 [40] CHEN B, PENWELL D, KRUGER M. The compressibility of nanocrystalline nickel [J]. Solid State Communications, 2000, 115(4): 191–194. doi: 10.1016/S0038-1098(00)00160-5 [41] WILLIAMS J G, BOGGS D H, YODER C F, et al. Lunar rotational dissipation in solid body and molten core [J]. Journal of Geophysical Research: Planets, 2001, 106(E11): 27933–27968. doi: 10.1029/2000JE001396 [42] WILLIAMS J G, KONOPLIV A S, BOGGS D H, et al. Lunar interior properties from the GRAIL mission [J]. Journal of Geophysical Research: Planets, 2014, 119(7): 1546–1578. doi: 10.1002/2013JE004559 [43] LOGNONNÉ P, JOHNSON C L. Treatise in Geophysics: planetary seismology [M]. Oxford, UK: Elsevier, 2007: 69–122. [44] WIECZOREK M A. The constitution and structure of the lunar interior [J]. Reviews in Mineralogy and Geochemistry, 2006, 60(1): 221–364. doi: 10.2138/rmg.2006.60.3 [45] RAI N, VAN WESTRENEN W. Lunar core formation: new constraints from metal-silicate partitioning of siderophile elements [J]. Earth and Planetary Science Letters, 2014, 388: 343–352. doi: 10.1016/j.jpgl.2013.12.001 [46] STEENSTRA E S, RAI N, KNIBBE J S, et al. New geochemical models of core formation in the Moon from metal-silicate partitioning of 15 siderophile elements [J]. Earth and Planetary Science Letters, 2016, 441: 1–9. doi: 10.1016/j.jpgl.2016.02.028 [47] WEBER R C, LIN P Y, GARNERO E J, et al. Seismic detection of the lunar core [J]. Science, 2011, 331(6015): 309–312. doi: 10.1126/science.1199375 [48] MORARD G, BOUCHET J, RIVOLDINI A, et al. Liquid properties in the Fe-FeS system under moderate pressure: tool box to model small planetary cores [J]. American Mineralogist, 2018, 103: 1770–1779. [49] JING Z C, WANG Y B, KONO Y, et al. Sound velocity of Fe-S liquids at high pressure: implications for the Moon’s molten outer core [J]. Earth and Planetary Science Letters, 2014, 396: 78–87. doi: 10.1016/j.jpgl.2014.04.015 [50] CHI H, DASGUPTA R, DUNCAN M S, et al. Partitioning of carbon between Fe-rich alloy melt and silicate melt in a magma ocean: implications for the abundance and origin of volatiles in Earth, Mars, and the Moon [J]. Geochimica et Cosmochimica Acta, 2014, 139: 447–471. doi: 10.1016/j.gca.2014.04.046 [51] RIGHTER K, GO B M, PANDO K A, et al. Phase equilibria of a low S and C lunar core: implications for an early lunar dynamo and physical state of the current core [J]. Earth and Planetary Science Letters, 2017, 463: 323–332. doi: 10.1016/j.jpgl.2017.02.003 [52] RIGHTER K, DRAKE M J. Core formation in Earth’s Moon, Mars, and Vesta [J]. Icarus, 1996, 124(2): 513–529. doi: 10.1006/icar.1996.0227 [53] NEWSOM H E, DRAKE M J. Experimental investigation of the partitioning of phosphorus between metal and silicate phases: implications for the Earth, Moon, and Eucrite parent body [J]. Geochimica et Cosmochimica Acta, 1983, 47(1): 93–100. doi: 10.1016/0016-7037(83)90093-5 [54] CHANTEL J, JING Z C, XU M, et al. Pressure dependence of the liquidus and solidus temperatures in the Fe-P binary system determined by in situ ultrasonics: implications to the solidification of Fe-P liquids in planetary cores [J]. Journal of Geophysical Research: Planets, 2018, 123(5): 1113–1124. doi: 10.1029/2017JE005376 [55] MININ D A, SHATSKIY A F, LITASOV K D, et al. The Fe-Fe2P phase diagram at 6 GPa [J]. High Pressure Research, 2019, 39(1): 50–68. doi: 10.1080/08957959.2018.1562552 [56] CHEN B, GAO L, FUNAKOSHI K, et al. Thermal expansion of iron-rich alloys and implications for the Earth’s core [J]. Proceedings of the National Academy of Sciences of the United States of America, 2007, 104(22): 9162–9167. doi: 10.1073/pnas.0610474104 [57] TSUJINO N, NISHIHARA Y, NAKAJIMA Y, et al. Equation of state of γ-Fe: reference density for planetary cores [J]. Earth and Planetary Science Letters, 2013, 375: 244–253. doi: 10.1016/j.jpgl.2013.05.040 [58] FISCHER R A, CAMPBELL A J, CARACAS R, et al. Equations of state in the Fe-FeSi system at high pressures and temperatures [J]. Journal of Geophysical Research: Solid Earth, 2014, 119(4): 2810–2827. doi: 10.1002/2013JB010898 -
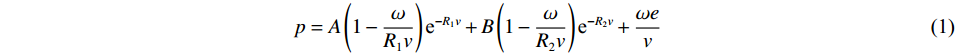
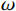
下载:
下载:
点击查看大图
计量
- 文章访问数: 12345
- HTML全文浏览量: 3608
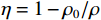
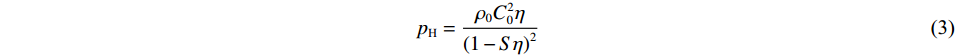
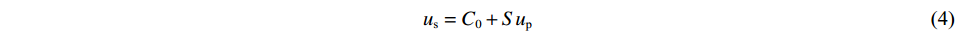
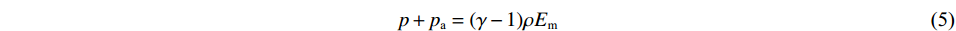
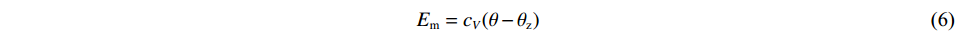
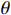
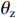
- PDF下载量: 64
