多晶体压剪试样静态加载有限元计算

赵伟业; 赵聃; 吕品; 金涛; 马胜国

doi:10.11858/gywlxb.20190836

多晶体压剪试样静态加载有限元计算

doi: 10.11858/gywlxb.20190836

赵伟业^{1, 2, 3,},
赵聃^{1, 2, 3,*, ,},
吕品^{1, 2, 3},
金涛^{1, 2, 3},
马胜国^{1, 2, 3}

1.
太原理工大学机械与运载工程学院应用力学研究所，山西太原　030024
2.
材料强度与结构冲击山西省重点实验室，山西太原　030024
3.
太原理工大学力学国家级实验教学示范中心，山西太原　030024

基金项目: 国家自然科学基金（11602158, 11802199, 11572214）；山西省自然科学青年基金（201601D021026）；山西省“1331工程”重点创新团队

详细信息

作者简介:
赵伟业（1992－），男，硕士研究生，主要从事多晶体有限变形数值计算研究.E-mail：939029385@qq.com

通讯作者:
赵　聃（1985－），男，博士，讲师，主要从事多晶体有限变形算法及多尺度本构理论研究.E-mail：zhaodan@tyut.edu.cn

中图分类号: O344.1
计量
- 文章访问数: 8227
- HTML全文浏览量: 3006
- PDF下载量: 30
出版历程
- 收稿日期: 2019-09-19
- 修回日期: 2019-10-25
- 刊出日期: 2020-02-25

Finite Element Calculation of Polycrystalline Shear-Compression Specimens with Static Loading

ZHAO Weiye^{1, 2, 3
,},
ZHAO Dan^{1, 2, 3,*
, ,},
LÜ Pin^{1, 2, 3},
JIN Tao^{1, 2, 3},
MA Shengguo^{1, 2, 3}

1.
Institute of Applied Mechanics, School of Mechanical and Vehicle Engineering, Taiyuan University of Technology, Taiyuan 030024, Shanxi, China
2.
Shanxi Key Laboratory of Material Strength & Structural Impact, Taiyuan 030024, Shanxi, China
3.
Taiyuan University of Technology Mechanics National Experimental Teaching Demonstration Center, Taiyuan 030024, Shanxi, China

摘要

摘要: 基于晶体塑性理论研究了晶体织构对数值计算结果的影响，建立了带有织构的多晶体压剪试样（SCS）模型。从材料和试样结构两方面研究了静态加载条件下微观晶粒在有限变形过程中对试样宏观力学性能的影响。由于模型几何结构的特殊性，重点对模型斜槽部分的应力、应变及变形特点进行了分析。考虑到试样在压缩过程中受摩擦的影响，数值分析了不同摩擦系数对变形过程的影响，在此基础上计算了相同摩擦系数下不同晶粒数目、不同单元数目以及单元类型对多晶体压剪模型力学性能的影响，并对试件关键部位不同取向晶粒的应力状态进行了分析。
- 多晶体 /
- 晶体织构 /
- 晶体塑性 /
- 压剪 /
- 摩擦系数
Abstract: The effect of crystal texture on the numerical results was studied based on the theory of crystal plasticity, and the polycrystalline compression shear sample (SCS) model with texture was established. The influence of micro-grain on the macroscopic mechanical properties in the process of finite deformation under static loading condition was studied in terms of material and sample structure. Because of the particularity of model geometry, the stress, strain and deformation characteristics of skewed slot were computed. Considering the effect of friction on the specimen during compression, the influence of friction coefficients on the deformation process was analyzed numerically. The influences of grain number, element number and element type on the mechanical properties of polycrystalline compression shear model under the same friction coefficient were calculated. The stress states of grain with different orientations in key parts of the specimen were also studied.
- polycrystalline /
- crystal texture /
- crystal plasticity /
- shear-compression /
- friction coefficient

HTML全文

冲击波在密实介质中的传播和衰减规律受到从事爆炸和防护等研究人员的高度重视。炸药的冲击波感度是其重要的安全性指标之一。隔板实验是早期建立的用于测定炸药冲击起爆性能的典型方法^[1]，通过升降法调整隔板厚度研究炸药的临界爆轰阈值。类似的改进的隔板实验(Modified Gap Test，MGT)^[2]则用于研究低压作用下炸药的反应阈值和爆轰阈值。可见，隔板实验中，经隔板衰减后的输出压力是一个重要的数据。有机玻璃作为一种常用的衰减材料，其衰减规律受到广泛关注。Keller^[3]、陈熙荣等^[4]、王作山等^[5]、王海福等^[6]对密实介质中冲击波的衰减进行了研究，应用不同的方法建立了密实介质中冲击波的衰减模型。韩秀凤等^[7]则对雷管输出冲击波在有机玻璃中的衰减进行了实验研究，由研究结果可知，冲击波在密实介质中传播时是按照指数规律衰减的，表达式为

$p={{p}_{0}}{{\text{e}}^{-\alpha x}}$

(1)

式中：p为冲击波进入密实介质传播距离x处的压力，GPa；p₀为冲击波进入密实介质时的初始压力，GPa；α为密实介质中冲击波压力衰减系数；x为冲击波在密实介质中传播的距离，mm。

由文献可知，由于研究者的实验方法及实验装置不同，其得出的衰减系数具有很大的差别。因此，衰减系数α并不是一成不变的。在一维强冲击波的作用下，α体现了隔板材料的属性，是一定值；而在其他情况，如散心爆轰波、雷管起爆输出冲击波等作用下，由于受到边侧稀疏等影响，其衰减系数将发生较大的变化。

本工作在研究低压冲击下炸药的反应阈值过程中，采用PVDF压电式压力传感器(简称PVDF计)测量了平面冲击波经过不同厚度有机玻璃隔板衰减后的输出压力，得到平面波透镜作用下有机玻璃隔板中冲击波的衰减系数，并与数值模拟结果及其他研究者的研究结果进行比较。

1. 实验研究

1.1 实验装置

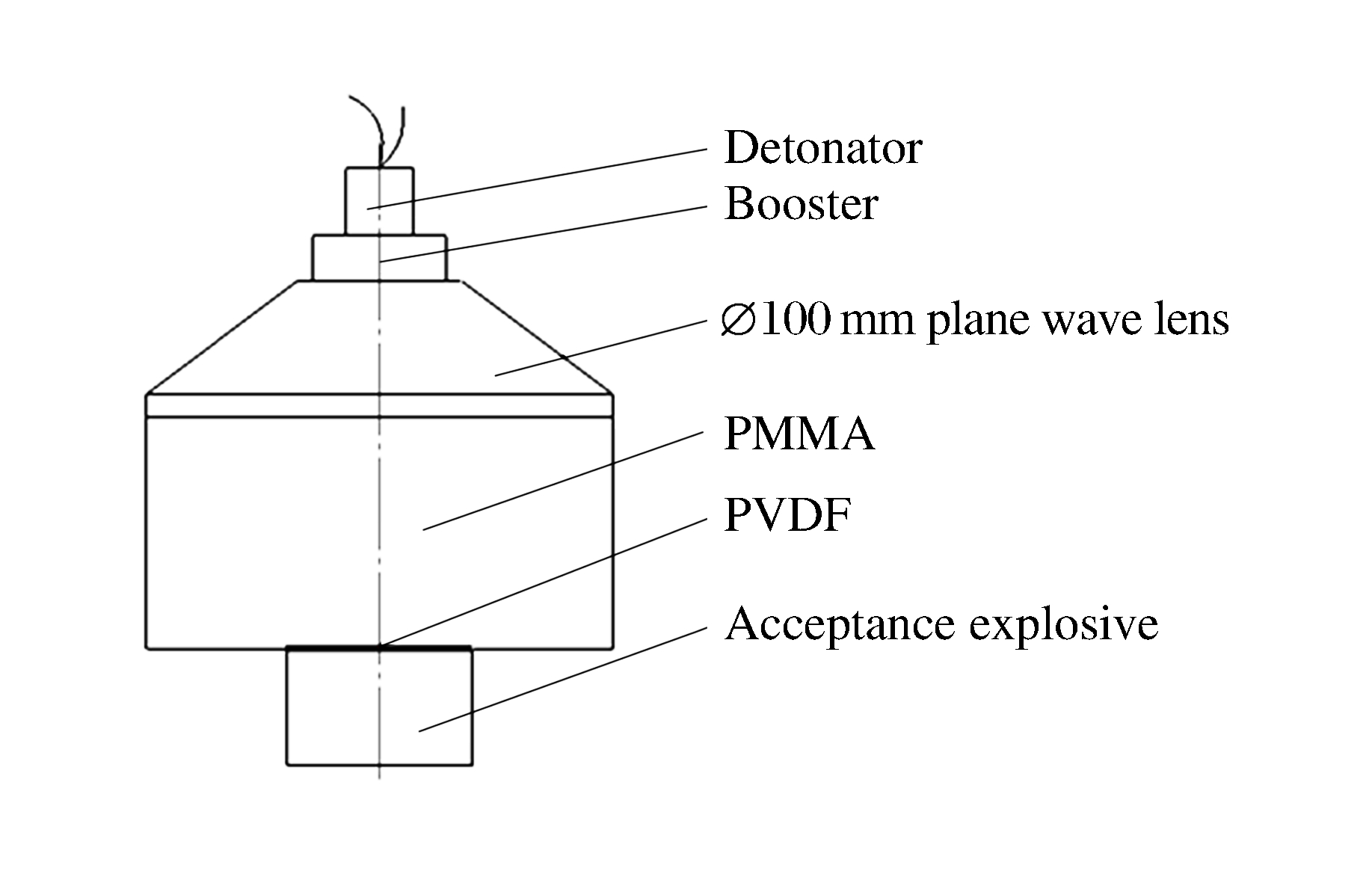
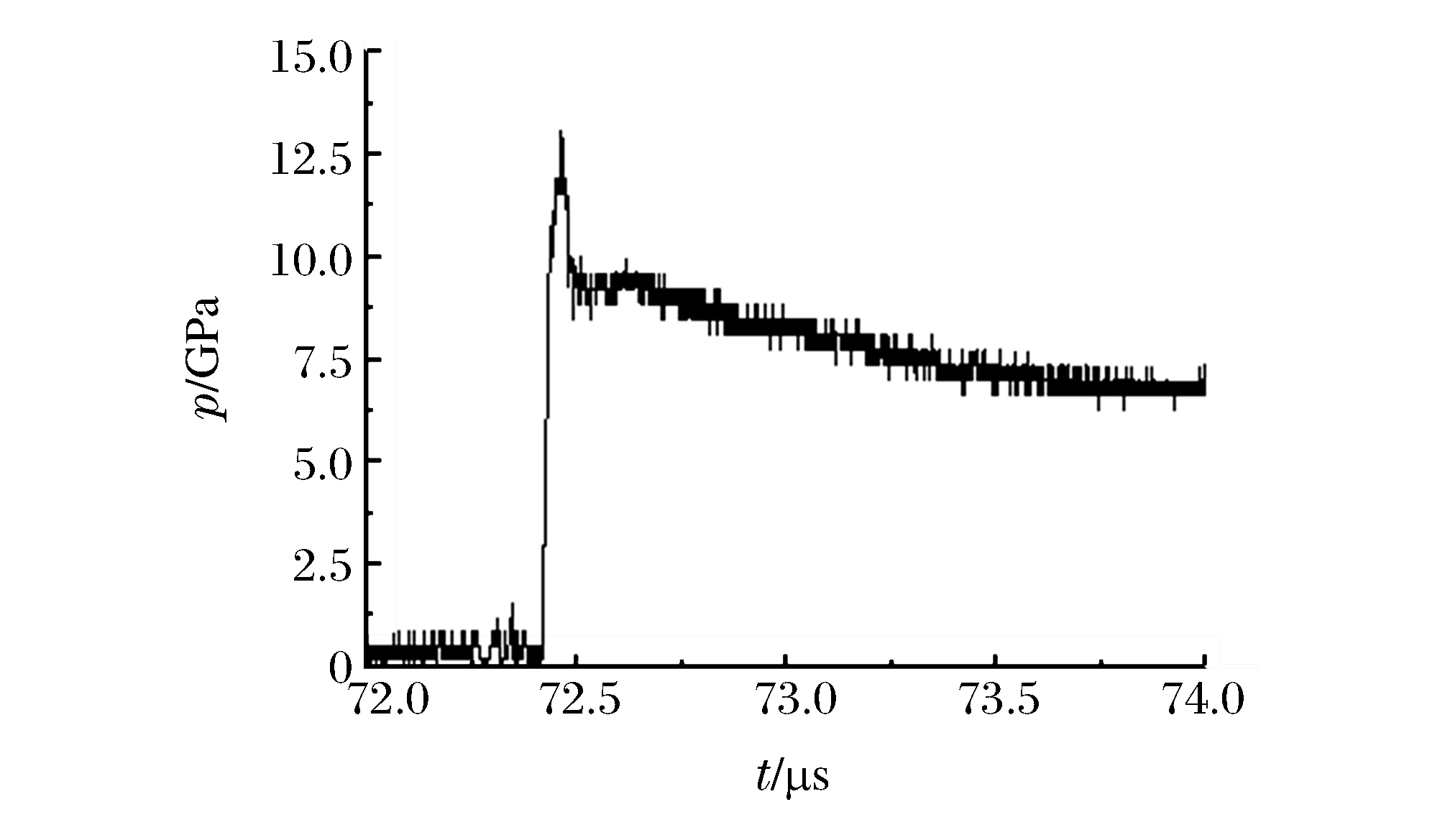
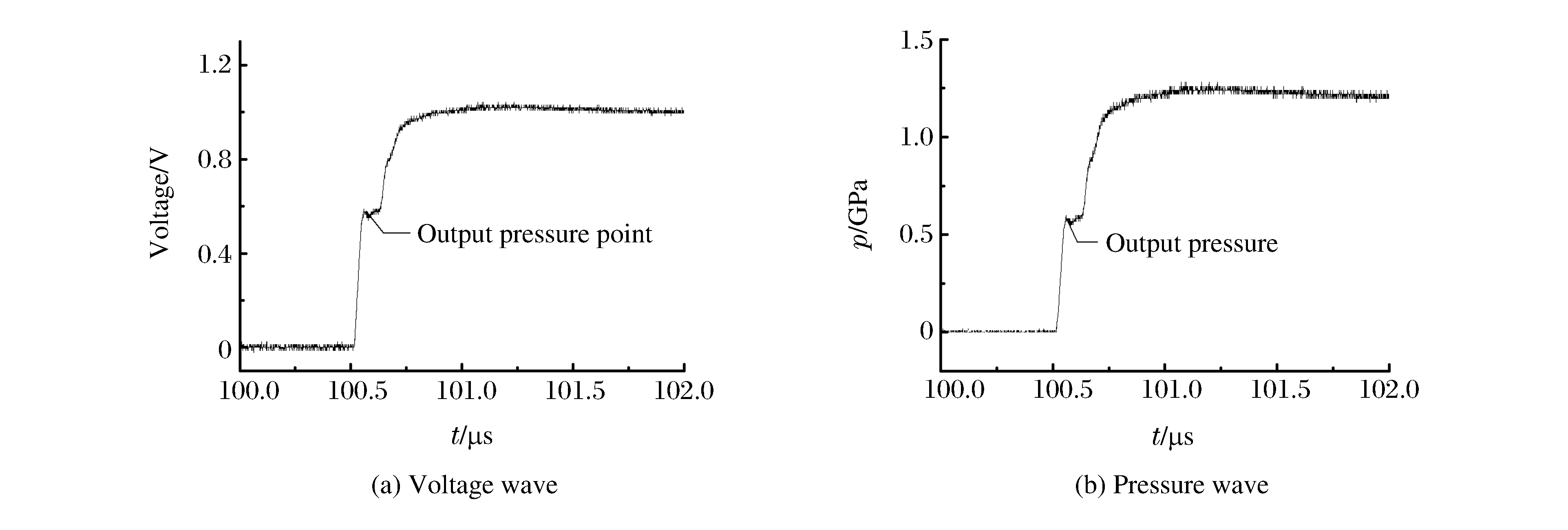
实验装置示意见图 1。平面波透镜爆炸后产生平面冲击波，在平面波透镜和有机玻璃界面处产生约10.15 GPa的压力(通过锰铜压力计测定，见图 2)，经不同厚度的Ø100 mm有机玻璃隔板衰减后，由PVDF计测量有机玻璃隔板与炸药间的压力-时间(p-t)历程。平面波透镜为RHT-901高爆速炸药和Ba(NO₃)₂/TNT(78/22, 质量比)的低爆速炸药组成，其中低爆速炸药的密度为2.45 g/cm³。实验用炸药柱为JO-9159，尺寸为Ø40 mm×25 mm，密度为1.842 g/cm³。PVDF计在低压下时间分辨率可达几十纳秒，压力测量精度高，可以得到准确的有机玻璃隔板中冲击波传播的衰减系数。但是，PVDF薄膜安装在被测物体内部或之间，需要涂抹硅胶排除其间的空气，因此，对安装精度的要求较高，胶层及薄膜也可能使测试结果偏小。

图 1 实验装置示意

Figure 1. Sketch of experimental facility

下载: 全尺寸图片幻灯片

图 2 界面压力测量结果

Figure 2. Interface pressure measured by manganin pressure gauge

下载: 全尺寸图片幻灯片

1.2 实验结果

实验测量了冲击波经厚度分别为50、70、80、90和100 mm的有机玻璃隔板衰减后的压力-时间历程，典型的实验波形和相应的压力波形见图 3。压力波形由实验波形结合PVDF计的标定方程得到，标定方程为^[8]

$p=\frac{1}{10}\left\{ 5.8{{\left( \frac{Q}{A} \right)}^{*}}+3.8{{\left[ {{\left( \frac{Q}{A} \right)}^{*}} \right]}^{1.6}}+0.55{{\left[ {{\left( \frac{Q}{A} \right)}^{*}} \right]}^{3.5}} \right\}$

(2)

图 3 隔板厚度为100 mm时的电压和压力波形

Figure 3. Voltage and pressure waves with 100 mm card gap

下载: 全尺寸图片幻灯片

式中:Q为电荷；A为PVDF计的敏感面积； ${{\left( \frac{Q}{A} \right)}^{\text{*}}}\text{=}0.018\left( \frac{Q}{A} \right)$ 为归一化电压幅值，它与积分器电容、准静态标定系数、标定时的温度以及积分器和记录器之间的衰减系数等密切相关^[9]。图 3中实验曲线上的第1个拐点代表隔板输出压力，第2个拐点代表炸药反应后的压力，这里只关注第1个拐点处的压力。不同隔板厚度对应的输出压力见表 1。

表 1 不同隔板厚度输出压力

Table 1. Output pressure with different card gap thicknesses

Card gap thickness/mm	Output pressure/GPa
50	2.24
70	1.30
80	1.09
90	0.82
100	0.65

下载: 导出CSV

| 显示表格

2. 数值模拟

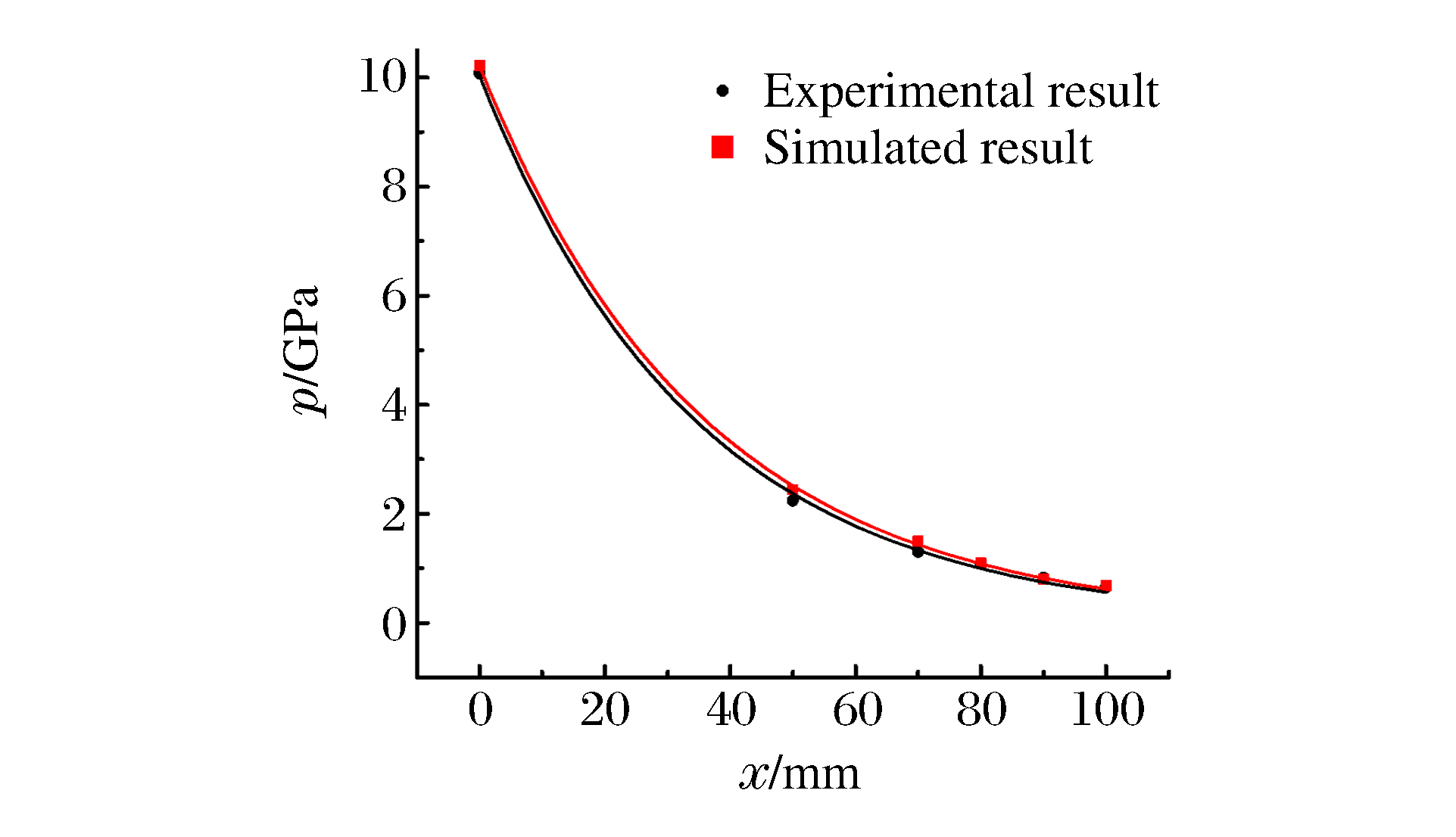
采用LS-DYNA软件对平面冲击波加载下有机玻璃的衰减情况进行了二维数值模拟，计算模型如图 4所示。加载炸药取低爆速炸药，采用MAT_HIGH_EXPLOSIVE_BURN材料模型和JWL状态方程，线性起爆产生平面冲击波，主要计算参数见表 2^[10]；有机玻璃采用MAT_ELASTIC_PLASTIC_ HYDRO材料模型和Grüneisen状态方程，主要计算参数见表 3，其中：D为炸药爆速，p_J为炸药爆压，a、b为材料的冲击雨贡纽参数。不同厚度有机玻璃对应的输出压力计算结果见图 5。

图 4 计算模型

Figure 4. Calculation model

下载: 全尺寸图片幻灯片

表 2 炸药的主要计算参数

Table 2. Main computational parameters of explosive

Material	Density/(g·cm^-3)	D/(km·s^-1)	p_J/GPa	A/GPa	B/GPa	R₁	R₂	ω
Ba(NO₃)₂/TNT(78/22)	2.6	4.476	11	1 265	1.55	5.8	2.0	0.6

下载: 导出CSV

| 显示表格

表 3 有机玻璃的主要计算参数

Table 3. Main computational parameters of PMMA

Material	Density/(g·cm^-3)	a/(km·s^-1)	b
PMMA	1.186	2.598	1.516

下载: 导出CSV

| 显示表格

图 5 实验结果与数值模拟结果比较

Figure 5. Comparison of experimental and simulated results

下载: 全尺寸图片幻灯片

炸药爆轰产物的JWL状态方程^[11]为

$p=A\left( 1-\frac{\omega }{{{R}_{1}}v} \right){{\text{e}}^{-{{R}_{1}}v}}\text{+}B\left( 1-\frac{\omega }{{{R}_{2}}v} \right){{\text{e}}^{-{{R}_{2}}v}}+\frac{\omega e}{v}$

(3)

式中:e为比内能；v为比容；A、B、R₁、R₂和ω为JWL状态方程参数。

由图 5可知，实验结果和数值模拟结果具有较好的一致性。由此说明实验结果是可靠的，数值模拟采用的计算参数是合理的。采用(1)式对实验结果进行指数拟合，可得平面波透镜作用下有机玻璃的衰减系数为0.028 89。

3. 讨论

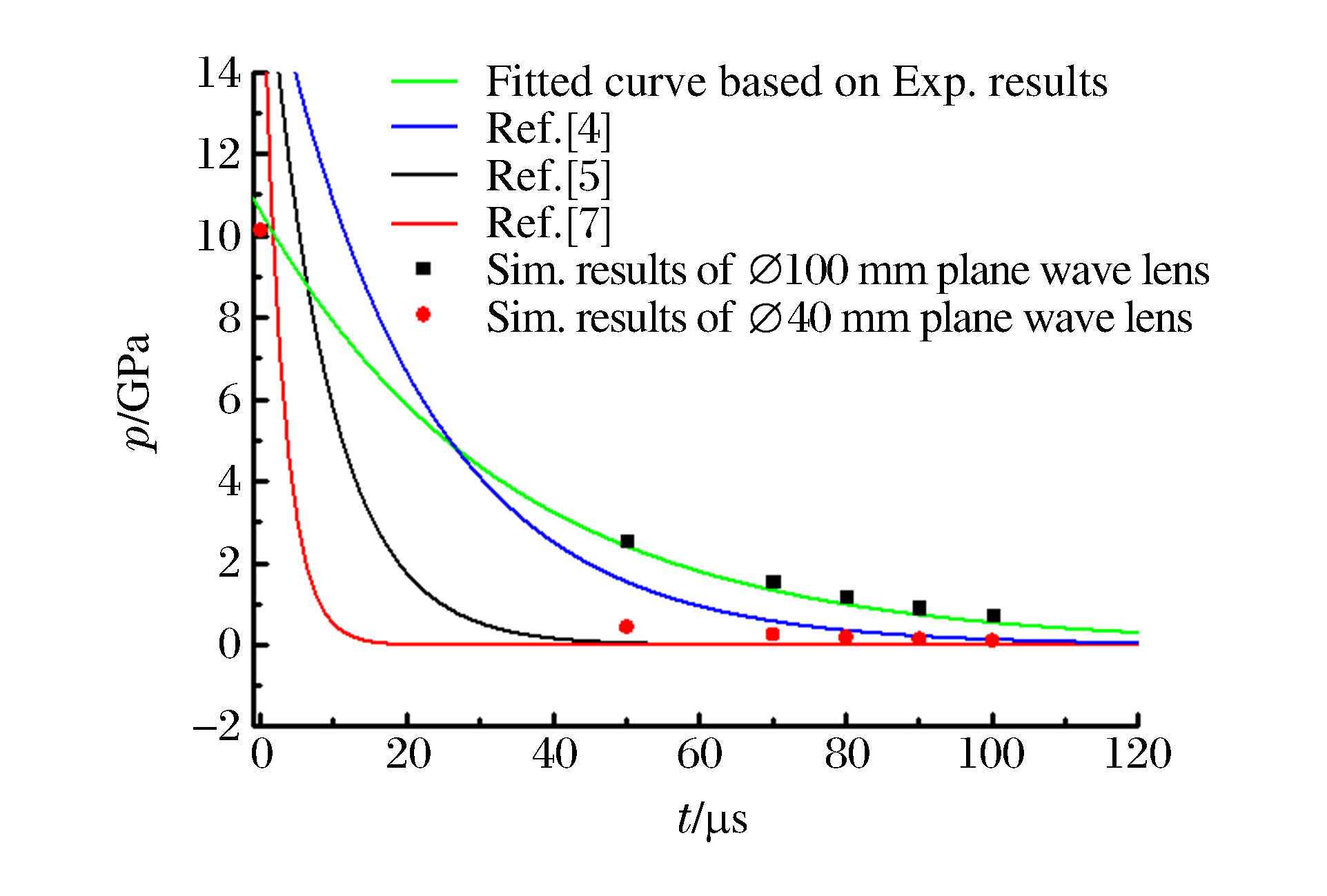
将本研究结果与其他研究者的研究结果进行比较，如图 6所示。由图 6曲线可以清晰地看出:本研究的冲击波衰减最慢; 文献[4]中采用Ø40 mm平面波透镜加载时，冲击波的衰减速率与本研究结果比较接近；而文献[7]中采用雷管起爆作为加载方式时，其衰减速率最快。可见，不同实验装置和实验条件下有机玻璃的衰减速率差别很大。实验装置的尺寸越大，加载冲击波的平面性越好，冲击波在有机玻璃中的衰减速率越慢，即衰减系数越小。这主要是由于冲击波在传播过程中受到边侧稀疏波的影响，实验装置尺寸大，则受到的影响小，实验结果能更真实地反映有机玻璃的衰减特性。可见，衰减系数体现了隔板材料的衰减特性，但同时也受到实验装置本身的影响，是特定条件下的参数。在采用有机玻璃或其他物质作为隔板材料时，应根据实际情况选取合适的衰减系数，才能比较准确地得到所需要的压力。本实验结果可为相关平面冲击波加载实验提供参考。

图 6 有机玻璃中冲击波衰减规律比较

Figure 6. Comparison of shock waveattenuation in PMMA

下载: 全尺寸图片幻灯片

4. 结论

(1) 采用Ø100 mm平面波透镜加载产生平面冲击波，用PVDF计测得距加载面不同距离处的冲击波压力，得到有机玻璃中冲击波的衰减系数为0.028 89。LS-DYNA模拟结果表明，计算结果与实验结果的一致性较好，说明实验结果可信度较高。

(2) 衰减系数受到实验装置尺寸大小及实验加载条件的影响，反映了材料在一定条件下的衰减特性。本实验结果受到侧向稀疏波的影响最小，比较真实地反映了有机玻璃的衰减特性。在实验设计时，应尽量减小稀疏波对加载冲击波的影响，以获得准确的实验压力条件。

图 1 多晶体随机织构和丝织构的极图

Figure 1. Polar graphs of polycrystalline with random texture and fiber texture

下载: 全尺寸图片幻灯片

图 2 具有不同初始织构的单向压缩应力-应变曲线

Figure 2. Unidirectional compression stress-strain curves with different initial textures

下载: 全尺寸图片幻灯片

图 3 多晶体压剪试样几何尺寸

Figure 3. Geometrical dimension of polycrystal shear-compression specimen

下载: 全尺寸图片幻灯片

图 4 多晶体压剪模型

Figure 4. Polycrystal shear-compression model

下载: 全尺寸图片幻灯片

图 5 不同摩擦系数情况下的应力云图

Figure 5. Stress nephogram with different friction coefficients

下载: 全尺寸图片幻灯片

图 6 不同摩擦系数情况下模型斜槽单元的平均切应力-平均切应变曲线

Figure 6. Average shear stress-average shear strain curve of the model’s chute element corresponding to different friction coefficients

下载: 全尺寸图片幻灯片

图 7 不同摩擦系数情况下模型斜槽单元的平均正应力-平均正应变曲线

Figure 7. Average normal stress-average normal strain curve of the model’s chute element corresponding to different friction coefficients

下载: 全尺寸图片幻灯片

图 8 摩擦系数不同时模型斜槽单元的平均Mises应力-平均等效应变曲线

Figure 8. Average Mises stress-average equivalent strain curve of the model’s chute element corresponding to different friction coefficients

下载: 全尺寸图片幻灯片

图 9 摩擦系数不同时模型顶面的力-位移曲线

Figure 9. Force-displacement curve of the top surface of the model corresponding to different friction coefficients

下载: 全尺寸图片幻灯片

图 10 模型斜槽部分一条棱上所选取的16个单元

Figure 10. 16 selected elements on an edge of the chute part of the model

下载: 全尺寸图片幻灯片

图 11 不同摩擦系数下特征晶粒在压缩方向的应变

Figure 11. Strain of characteristic grain in compression direction under different friction coefficients

下载: 全尺寸图片幻灯片

图 12 包含不同晶粒数目的压剪有限元模型

Figure 12. Finite element models of compression shear with different grain numbers

下载: 全尺寸图片幻灯片

图 13 不同晶粒数目对应模型的载荷-位移曲线

Figure 13. Force-displacement curves of models corresponding to different numbers of grains

下载: 全尺寸图片幻灯片

图 14 单元数目不同的模型

Figure 14. Models with different number of elements

下载: 全尺寸图片幻灯片

图 15 单元数目不同时模型的Mises变形云图

Figure 15. Mises deformation nephograms of models with different numbers of elements

下载: 全尺寸图片幻灯片

图 16 单元数目不同时模型的载荷-位移曲线

Figure 16. Force-displacement curves of models with different numbers of elements

下载: 全尺寸图片幻灯片

图 17 斜槽相同位置处晶粒的Mises应力-等效应变曲线

Figure 17. Mises stress-equivalent strain curve of grains at the same position in the chute

下载: 全尺寸图片幻灯片

图 18 两种单元类型对应的模型斜槽部分Mises变形云图

Figure 18. Mises deformation nephogram of the model with two element types

下载: 全尺寸图片幻灯片

图 19 两种单元类型对应的模型顶面力-位移曲线

Figure 19. Top surface force-displacement curve of model with two element types

下载: 全尺寸图片幻灯片

图 20 两种单元类型对应的模型斜槽部分单元的平均Mises应力-平均等效应变曲线

Figure 20. Average Mises stress-strain curves of the sloped part of the model with two element types

下载: 全尺寸图片幻灯片

表 1 6061铝合金材料参数^[23]

Table 1. Material parameters of 6061 aluminum alloy^[23]

C₁₁/GPa	C₁₂/GPa	C₄₄/GPa	m	q	${ { {\dot {\gamma } } }_{{0}}}$ /s^–1	h₀/MPa	τ₀/MPa	τ_s/MPa
108.200	61.300	28.500	20	1.1	1	96	23.5	54

下载: 导出CSV

表 2 不同摩擦系数对应模型的数值结果比较

Table 2. Numerical results of models corresponding to different friction coefficients

Friction coefficient	Maximum shear strain	Maximum normal strain	Maximum equivalent strain	Maximum force/N
0.025	0.740	−0.331	0.741	8 899.73
0.050	0.697	−0.263	0.625	9 566.97
0.100	0.651	−0.212	0.553	10 001.50
Relative maximum difference	13.7%	56.1%	34.0%	12.4%

下载: 导出CSV

表 3 特征晶粒欧拉角

Table 3. Euler angle of characteristic grains

Grain number	φ₁/(°)	$\psi$ /(°)	φ₂/(°)	Grain number	φ₁/(°)	$\psi$ /(°)	φ₂/(°)
1	69.24	167.34	8.61	9	334.93	38.19	29.33
2	44.05	92.39	45.56	10	30.45	127.11	356.19
3	356.63	154.92	76.61	11	59.93	105.73	313.11
4	305.74	23.07	333.37	12	88.45	166.05	33.32
5	45.14	101.42	64.90	13	74.86	154.71	302.25
6	282.88	86.40	282.11	14	76.35	51.48	307.37
7	350.37	146.78	286.51	15	79.65	77.10	351.57
8	49.77	3.76	329.80	16	357.28	56.00	72.22

下载: 导出CSV

表 4 单元数目不同时模型在不同压缩距离下的载荷及其最大相对偏差

Table 4. Loads of the model with different numbers of elements at different compression distance and their maximum relative differences

Number of elements	Load/N
Number of elements	1.0 mm	1.4 mm	1.8 mm	2.5 mm
8 026	−7 790.82	−8 180.42	−8 469.96	−8 726.07
14 604	−7 487.38	−7 824.37	−8 008.19	−8 157.61
23 835	−7 669.64	−8 038.55	−8 270.62	−8 436.06
Maximum relative difference	4.05%	4.55%	5.77%	6.97%

下载: 导出CSV

参考文献(24)

[1]	董湘怀, 仲町英治. 晶体塑性模型在板材成形计算机模拟中的应用 [J]. 中国机械工程, 1997, 8(4): 27–30, 118. DONG X H, NAKAMACHI E. Application of crystal plastic model in computer simulation of sheet metal forming [J]. China Mechanical Engineering, 1997, 8(4): 27–30, 118.
[2]	刘海军, 方刚, 曾攀. 基于晶体塑性理论的大变形数值模拟技术 [J]. 塑性工程学报, 2006, 13(2): 1–8, 28. doi: 10.3969/j.issn.1007-2012.2006.02.001 LIU H J, FANG G, ZENG P. Numerical simulation of large deformation based on the theory of crystal plasticity [J]. Journal of Plastic Engineering, 2006, 13(2): 1–8, 28. doi: 10.3969/j.issn.1007-2012.2006.02.001
[3]	皮华春, 韩静涛, 薛永栋. 金属塑性成形的晶体塑性学有限元模拟研究进展 [J]. 机械工程学报, 2006, 42(3): 15–21. doi: 10.3321/j.issn:0577-6686.2006.03.003 PI H C, HAN J T, XUE Y D. Progress in finite element simulation of metal plasticity forming with crystal plasticity [J]. Journal of Mechanical Engineering, 2006, 42(3): 15–21. doi: 10.3321/j.issn:0577-6686.2006.03.003
[4]	司良英. FCC金属冷加工织构演变的晶体塑性有限元模拟 [D]. 沈阳: 东北大学, 2009. SI L Y. Finite element simulation of crystal plasticity in FCC metal cold working texture evolution [D]. Shenyang: Northeastern University, 2009.
[5]	司良英, 邓关宇, 吕程. 基于Voronoi图的晶体塑性有限元多晶几何建模 [J]. 材料与冶金学报, 2009, 8(3): 193–197, 216. SI L Y, DENG G Y, LÜ C. Crystal plastic finite element polycrystalline geometry modeling based on Voronoi diagram [J]. Journal of Materials and Metallurgy, 2009, 8(3): 193–197, 216.
[6]	张丰果, 董湘怀. 微塑性成形模拟材料细观建模 [J]. 模具技术, 2011(3): 16–19. ZHANG F G, DONG X H. Microplastic forming simulates material microscopic modeling [J]. Mould Technology, 2011(3): 16–19.
[7]	郑文, 徐松林, 蔡超. 基于Hopkinson压杆的动态压剪复合加载实验研究 [J]. 力学学报, 2012, 44(1): 124–131. ZHENG W, XU S L, CAI C. Experimental study on dynamic compression shear composite loading based on Hopkinson compression bar [J]. Journal of Mechanics, 2012, 44(1): 124–131.
[8]	章超, 徐松林, 王道荣. 花岗岩动静态压剪复合加载实验研究 [J]. 固体力学学报, 2014, 35(2): 115–123. ZHANG C, XU S L, WANG D R. Experimental study on dynamic and static compressor-shear composite loading of granite [J]. Journal of Solid Mechanics, 2014, 35(2): 115–123.
[9]	李雪艳, 李志斌, 张舵. 闭孔泡沫铝准静态压剪性能研究 [J]. 高压物理学报, 2018, 32(3): 52–59. doi: 10.11858/gywlxb.20170655 LI X Y, LI Z B, ZHANG D. Study on quasi-static compressive shear properties of aluminum foam with closed-cell [J]. Journal of High Pressure Physics, 2018, 32(3): 52–59. doi: 10.11858/gywlxb.20170655
[10]	RITTEL D, LEE S, RAVICHANDRAN G. A Shear-compression specimen for large strain testing [J]. Experimental Mechanics, 2002, 42(1): 58–64. doi: 10.1007/BF02411052
[11]	RITTEL D, RAVICHANDRAN G, LEE S. Large strain constitutive behavior of OFHC copper over a wide range of strain rates using the shear compression specimen [J]. Mechanics of Materials, 2002, 34(10): 627–642. doi: 10.1016/S0167-6636(02)00164-3
[12]	DOROGOY A, RITTEL D. A numerical study of the applicability of the shear compression specimen to parabolic hardening materials [J]. Experimental Mechanics, 2006, 46(3): 355–366. doi: 10.1007/s11340-006-6414-8
[13]	DOROGOY A, RITTEL D. Numerical validation of the shear compression specimen. Part Ⅱ: dynamic large strain testing [J]. Experimental Mechanics, 2005, 45(2): 178–185. doi: 10.1007/BF02428191
[14]	DOROGOY A, RITTEL D. Numerical validation of the shear compression specimen. Part I: quasi-static large strain testing [J]. Experimental Mechanics, 2005, 45(2): 167–177. doi: 10.1007/BF02428190
[15]	DOROGOY A, RITTEL D, GODINGER A. Modification of the shear-compression specimen for large strain testing [J]. Experimental Mechanics, 2015, 55(9): 1627–1639. doi: 10.1007/s11340-015-0057-6
[16]	VURAL M, MOLINARI A, BHATTACHARYYA N. Analysis of slot orientation in shear-compression specimen (SCS) [J]. Experimental Mechanics, 2010, 51(3): 263–273.
[17]	ZHAO J, KNAUSS W G, RAVICHANDRAN G. A new shear-compression-specimen for determining quasistatic and dynamic polymer properties [J]. Experimental Mechanics, 2008, 49(3): 427–436.
[18]	PIERCE D, ASARO R J, NEEDLEMAN A. Material rate sensitivity and localized deformation in crystalline solids [J]. Acta Metall, 1983, 31(12): 1951–1976. doi: 10.1016/0001-6160(83)90014-7
[19]	ASARO R J, NEEDLEMAN A. Overview No. 42 texture development and strain hardening in rate dependent polycrystals [J]. Acta Metallurgica, 1985, 33(6): 923–953.
[20]	HUANG Y. A user-material subroutine incorporating single crystal plasticity in the ABAQUS finite element program: MECH 178 [R]. Harvard University, 1991.
[21]	王国军, 孙强. 4032合金热挤压棒材变形行为及形变织构 [J]. 黑龙江冶金, 2013, 33(2): 1–5, 8. WANG G J, SUN Q. Deformation behavior and deformation texture of hot extruded 4032 alloy bar [J]. Heilongjiang Metallurgy, 2013, 33(2): 1–5, 8.
[22]	HUANG S Y, ZHANG S R, LI D Y. Simulation of texture evolution during plastic deformation of FCC, BCC and HCP structured crystals with crystal plasticity based finite element method [J]. Transactions of Nonferrous Metals Society of China, 2011, 21(8): 1817–1825. doi: 10.1016/S1003-6326(11)60936-9
[23]	辛存, 赵聃, 闫晓鹏. 材料三维微结构表征及其晶体塑性有限元模拟 [J]. 计算力学学报, 2018, 36(2): 233–239. XIN C, ZHAO D, YAN X P. 3D modeling microstructure and crystal plasticity finite element simulation [J]. Journal of Computational Mechanics, 2018, 36(2): 233–239.
[24]	齐康, 闫昊, 陈祥瑶. 利用ABAQUS模拟不同模态下的金属切削过程 [J]. 机械工程与自动化, 2018(2): 93–94. QI K, YAN H, CHEN X Y. Metal cutting processes in different modes are simulated by ABAQUS [J]. Mechanical Engineering and Automation, 2018(2): 93–94.