Damage Evolution in Concrete Interfacial Transition Zone with Ultrasonic Dynamic Load
-
摘要: 混凝土是由粗骨料、水泥砂浆以及过渡区域组成的三相非均质复合材料。混凝土过渡区域(Interfacial transition zone, ITZ)是三相中最薄弱的环节,且难以观测,对混凝土的宏观力学性能有着重要影响。基于ABAQUS的Dynamic/Explicit模块,建立了能反映混凝土基质、骨料形状和 ITZ等真实细观结构的有限元模型,并应用该模型研究了过渡区域对混凝土损伤破坏的影响。研究结果表明:粗骨料的形状对混凝土损伤性能有一定影响,当骨料形状为凸多边形时,其抗损伤性能最弱;混凝土的抗损伤性能随着ITZ强度的降低而减弱,当ITZ的强度高于砂浆的60%时,抗损伤性能逐渐增强;随着ITZ厚度的增加,混凝土的抗损伤能力减弱。Abstract: Concrete is a three-phase material composed of coarse aggregate, cement mortar and interfacial transition zone (ITZ). The ITZ is the weakest of the three phases and difficult to observe, but it has a significant impact on the efficiency of concrete crushing. In order to study the impact of ITZ on the damage performance of concrete crushing, the finite element model that reflects real mesoscopic structure of concrete matrix, aggregate shape, and ITZ was established on the Dynamic/Explicit model in ABAQUS. The results showed that the shape of coarse aggregate has a certain influence on the damage performance of concrete, and when the shape is convex polygonal, its damage resistance is the weakest. The damage resistance ability of concrete decreases with the decrease of ITZ strength. When ITZ strength is higher than 60% of mortar, the damage resistance ability gradually increases. As the thickness of the ITZ area increases, the damage resistance ability decreases.
-
Key words:
- concrete /
- interfacial transition zone /
- damage performance /
- ultrasonic crushing
-
普通混凝土具有显著的脆性特征,钢纤维的引入极大地提高了混凝土的延性,并可减少结构部件的剥落飞射,避免了大量的人员伤亡,因此被广泛应用于各类防护结构。近几十年来,随着计算能力的高速发展,LS-DYNA显式有限元动力分析软件被广泛应用于工程结构的动态行为研究。
K&C模型常用于分析冲击荷载与爆炸荷载作用下混凝土结构的动力响应,研究人员只需输入混凝土的单轴抗压强度,其他参数可自动生成,然而该模型存在以下不足:(1)自动生成的强度面参数适用于普通混凝土,但不适用于三轴强度显著变化的钢纤维混凝土(Steel fiber reinforced concrete,SFRC);(2)K&C模型的损伤机制能够表征普通混凝土的力学行为,但对于韧性、延性、能量吸收能力显著增强的SFRC,不能准确表征其应变软化行为;(3)SFRC的应变率效应在很大程度上依赖于钢纤维的体积分数、抗拉强度、几何形状和混凝土的基体强度,因此普通混凝土的动力增强因子模型不适用于SFRC。
本研究将针对以上不足,对K&C模型进行改进,并运用LS-DYNA显式有限元动力分析软件模拟高速弹丸侵彻SFRC靶板[1]和爆炸荷载下SFRC板的动力响应[2],利用模拟结果论证改进后的K&C模型,以期能够准确描述SFRC的动力响应。
1. 改进K&C材料模型
1.1 改进失效强度面模型
K&C模型采用压子午线定义了3个独立的失效强度面
Δσm=a0+pa1+a2p,Δσy=a0y+pa1y+a2yp,Δσr=pa1f+a2fp (1) 式中:
Δσm 为最大强度面;Δσy 为初始屈服强度面;Δσr 为残余强度面;ai、aiy、aif(i = 0, 1, 2)为混凝土三轴压缩试验得到的一组参数;p为静水压力,p=(σ1+σ2+σ3)/3 ,其中σ1、σ2、σ3分别为第1、第2、第3主应力;失效强度面偏应力Δσ=√[(σ1−σ2)2+(σ2−σ3)2+(σ3−σ1)2]/2 。原K&C模型失效强度面参数为a0=0.296fc,a1=0.446,a2=0.081/fc (2) a0y=0.223fc,a1y=0.625,a2y=0.258/fc (3) a1f=0.442,a2f=0.118/fc (4) 式中:fc为单轴抗压强度。
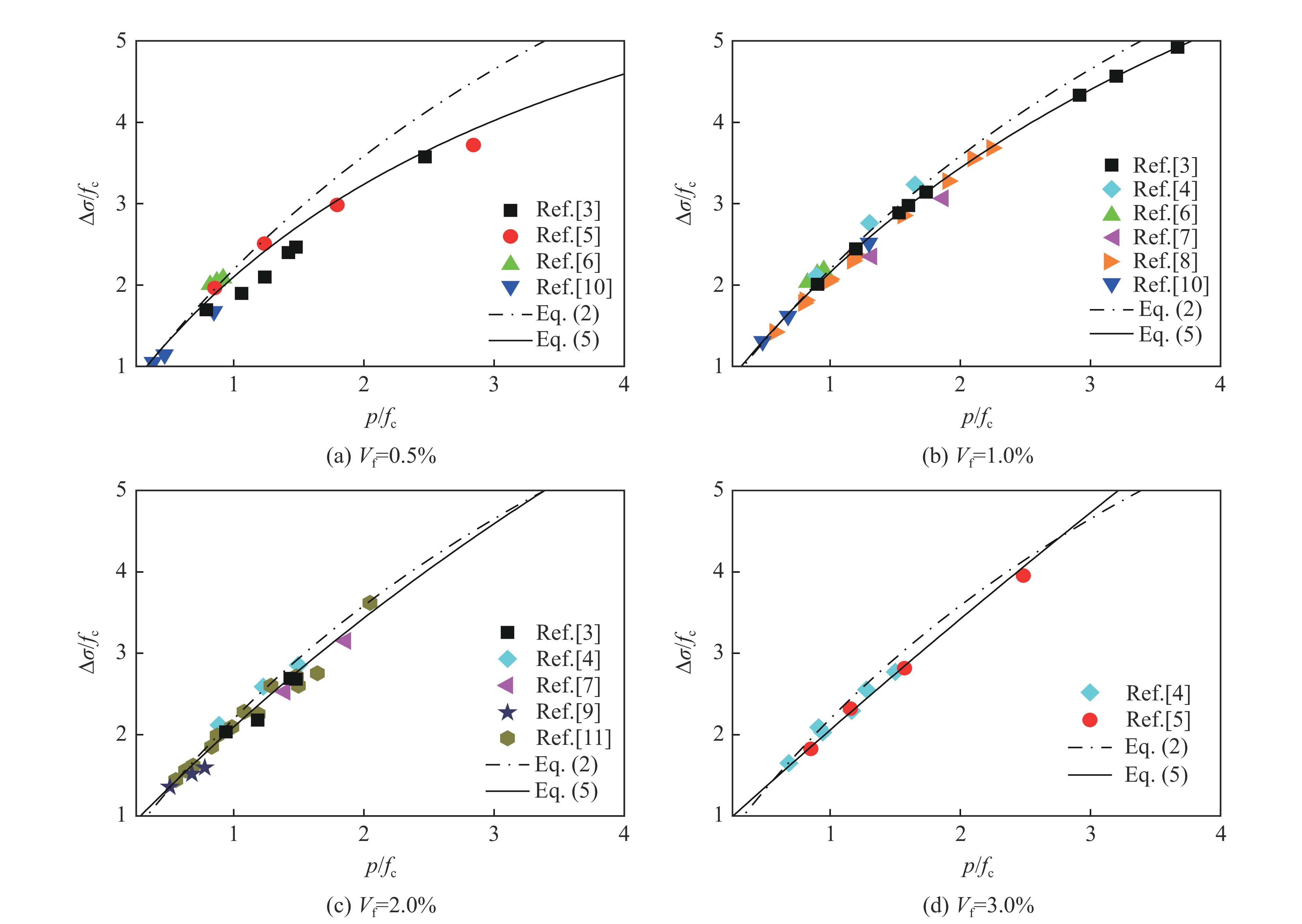
国内外学者研究发现,SFRC的三轴压缩强度与素混凝土有显著差异,且依赖于钢纤维体积分数[3-11]。为了明确钢纤维体积分数对SFRC三轴压缩强度的影响,本研究在原最大强度面参数模型的基础上,根据大量实验数据,采用最小二乘法建立了新的最大强度面参数模型
a0=(0.232+0.133Vf)fc,a1=0.378+0.103Vf,a2=(0.149−0.047Vf)/fc (5) 式中:Vf为钢纤维体积分数,0 < Vf < 3.0%。
图1给出了不同钢纤维体积分数SFRC的三轴压缩实验数据及根据式(2)、式(5)模型计算得到的预测值。由图1可知,式(5)与不同钢纤维体积分数的SFRC三轴压缩实验数据[3-11]的吻合程度优于式(2)。
为了确定初始屈服面,必须首先确定a0y、a1y、a2y。通常认为初始屈服面近似为三轴受压应力路径上
Δσ=0.45Δσm 处点的轨迹。最大破坏面上的点(p,Δσm) 与屈服面上对应的点(p′,Δσy) 的关系可表示为Δσy=0.45Δσm,p′=p−0.55Δσm/3 (6) 根据式(6)的假定及已提出的最大强度面模型,初始屈服面参数模型可表示为
a0y=(0.194+0.068Vf)fc,a1y=0.524+0.202Vf,a2y=(0.452−0.142Vf)/fc (7) 残余强度面参数的确定需要用到三轴压缩实验的残余强度数据,但相关的实验数据难以得到。根据Marlvar等[12]的建议,残余强度面参数可表示为
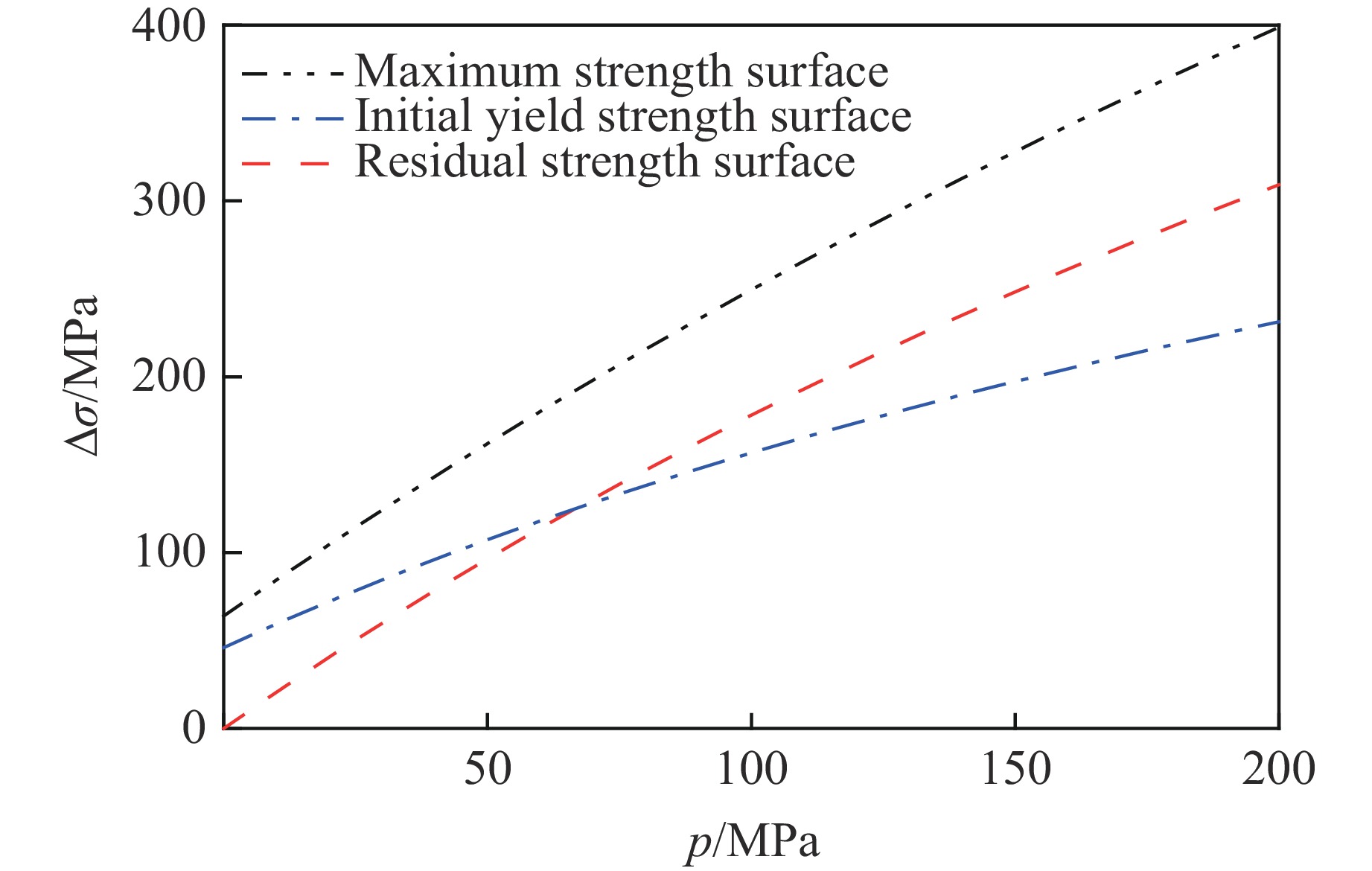
a1f=0.374+0.102Vf,a2f=(0.218−0.068Vf)/fc (8) 改进的失效强度面模型应按式(5)、式(7)、式(8)进行修正,当fc = 175.3 MPa,Vf = 1.0%时,SFRC的失效强度面模型如图2所示,该模型将应用于2.1节的讨论。
1.2 修正损伤演化函数
在K&C模型中,当偏应力超过初始屈服面,但未达到最大强度面时,当前多轴应力状态为两个强度面的线性插值;当偏应力超过最大强度面时,当前多轴应力状态取为最大强度面与残余强度面的线性插值,其关系式表示为
Δσ={η(Δσm−Δσy)+Δσyλ<λmη(Δσm−Δσr)+Δσrλ⩾ (9) 式中:λ为有效塑性应变,η为损伤比例因子,损伤演化函数η(λ)由输入的13组(η, λ)数据定义。演化全过程分为两个阶段:(1) λ < λm时为硬化段,
\lambda 从零开始逐渐积累到λm,η逐渐增大到1,材料的应变硬化发展到峰值,此时偏应力点位于最大强度面;(2)λ ≥ λm时,材料进入应变软化阶段,λm持续积累,η逐渐减小为零,此时偏应力点位于残余强度面。η与λ的关系描述了应力-应变全过程曲线,必须谨慎定义。原K&C模型的损伤演化函数η(λ)描述的损伤演化速度过快,不能正确表征SFRC的延性、韧性及断裂应变能。为了精准描述由塑性剪切应变和塑性体积应变引起的损伤演变过程,需要对损伤演化函数进行修正。
本研究使用分段函数[13-14]建立新的损伤演化模型,其关系式表示为
\eta (\lambda ) = \left\{ {\begin{aligned} & {\alpha \left(\frac{\lambda }{{{\lambda _{\rm{m}}}}}\right) + (3 - 2\alpha ){{\left(\frac{\lambda }{{{\lambda _{\rm{m}}}}}\right)}^2} + (\alpha - 2){{\left(\frac{\lambda }{{{\lambda _{\rm{m}}}}}\right)}^3}}\quad {\lambda < {\lambda _{\rm{m}}}}\\ & {\frac{{\lambda /{\lambda _{\rm{m}}}}}{{{\alpha _{\rm{c}}}{{(\lambda /{\lambda _{\rm{m}}} - 1)}^{{\alpha _{\rm{d}}}}} + \lambda /{\lambda _{\rm{m}}}}}}\quad\quad\quad\quad\quad\quad\quad\;{\lambda \geqslant {\lambda _{\rm{m}}}} \end{aligned}} \right. (10) 式中:α为控制应变硬化段的常量,αc、αd为控制应变软化段的常量,λm为有效塑性应变峰值。在LS-DYNA软件中建立单个单元模型,单元尺寸为2 mm,约束底面节点自由度,对顶面节点施加速度矢量。模拟SFRC的单轴压缩试验,通过反复试验法确定α、αc、αd、λm,直到模拟的应力-应变曲线与典型的SFRC应力-应变关系相符。基于大量试验研究,建议α = 3,αc = 0.381,αd = 1.90,λm = 9.5 × 10–5。改进的η(λ)将用于接下来的讨论,与原损伤演化函数的比较如图3所示。
虽然本节对η(λ)进行了修正,但是K&C模型中材料的软化行为由η(λ)与损伤参数b1、b2协同控制,因此要准确描述SFRC的软化段特性,还需对损伤参数进行校准,1.3节将介绍校准损伤参数b1、b2的简便方法,并进一步论证本研究所建立损伤演化函数η(λ)的有效性。
1.3 校准损伤参数
K&C模型中,有效塑性应变积累函数为
\lambda = \left\{ \begin{aligned} & \int_0^{{{\bar \varepsilon }^{\rm{p}}}} {\frac{{{\rm{d}}{{\bar \varepsilon }^{\rm{p}}}}}{{{\gamma _{\rm{f}}}{{(1 + p/{\gamma _{\rm{f}}}{f_{\rm{t}}})}^{{b_1}}}}}\;\;\;\;p \geqslant 0} \\ & \int_0^{{{\bar \varepsilon }^{\rm{p}}}} {\frac{{{\rm{d}}{{\bar \varepsilon }^{\rm{p}}}}}{{{\gamma _{\rm{f}}}{{(1 +p/{\gamma _{\rm{f}}}{f_{\rm{t}}})}^{{b_2}}}}}\;\;\;\;p < 0} \end{aligned} \right. (11) 式中:
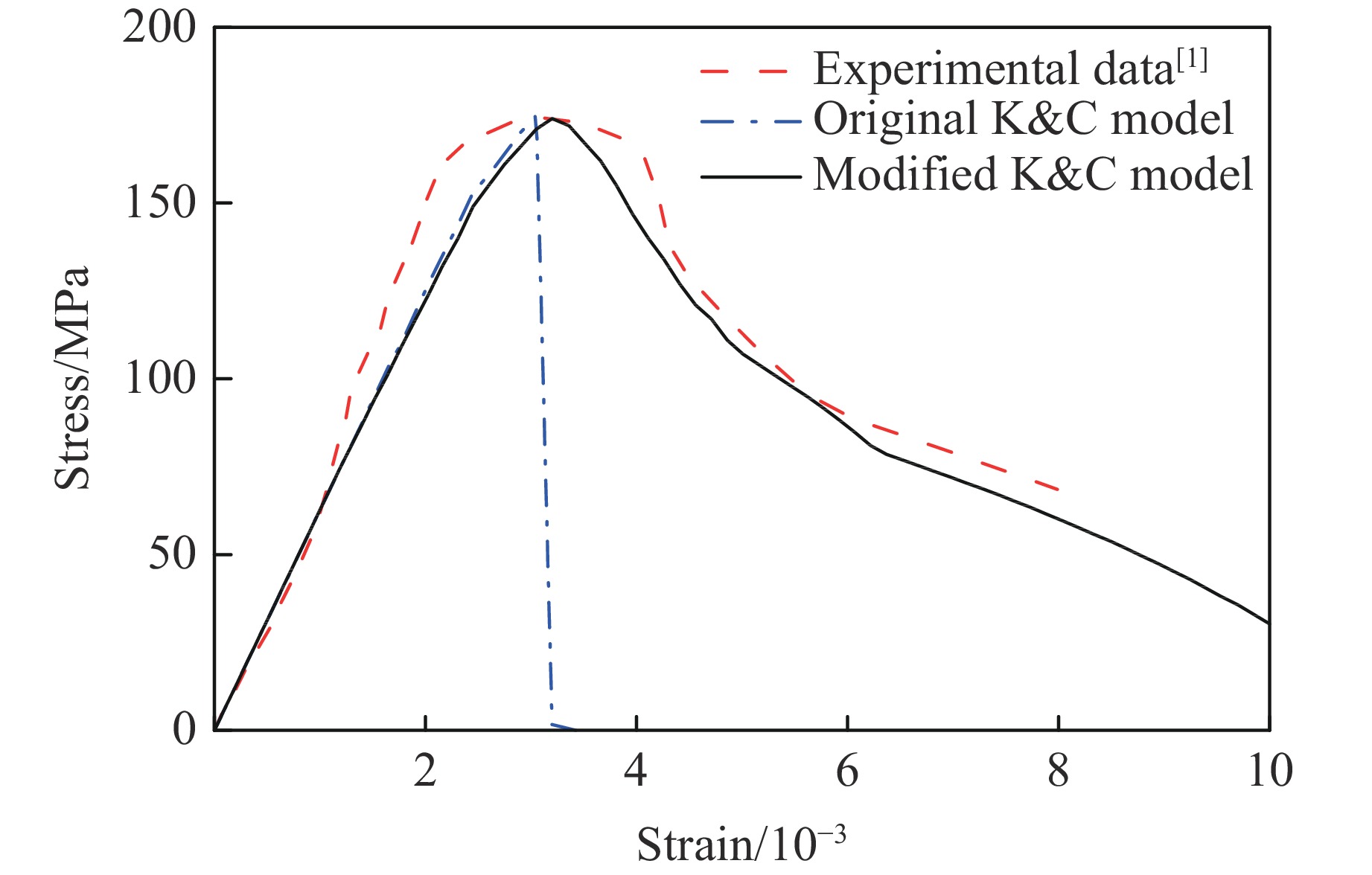
{\bar \varepsilon ^{\rm{p}}} 为有效塑性应变增量;γf为应变率增强因子;ft为单轴抗拉强度;b1、b2为损伤参数,分别控制单轴压缩与拉伸软化段的力学行为。Malvar等[12]建议损伤参数b1的取值应与单轴压缩试验(UUC)下的应力-应变曲线相匹配,建立单个单元模型,模拟fc = 175.3 MPa、Vf = 1.0%时SFRC的单轴压缩试验[1]。原K&C模型自动生成的损伤演化函数、损伤参数(b1 = 0)与修正后的损伤演化函数、损伤参数(b1 = 0.75)对应力-应变曲线的描述如图4所示。由图4可知,原K&C模型不能准确描述SFRC应变软化段的力学行为,而修正后的损伤模型较好地预测了SFRC的峰后特性,且与试验数据的吻合程度较高,进一步论证了修正后损伤演化函数η(λ)的有效性。
当应力点从最大强度面向残余强度破坏面移动时,材料进入软化阶段,参数b2控制了受拉应力-应变曲线的软化行为。Bazant等[15]认为,只有当SFRC的软化行为受到局部或特征长度控制时,网格细化后的应力-应变曲线才会客观,否则它们将依赖于网格尺寸。他们提出消除网格依赖性的方法是强迫单轴拉伸应力-应变曲线下方的面积为Gf/hw,其中,Gf为断裂应变能,hw为裂缝正面宽度或裂缝局部宽度,通常hw为最大骨料直径的1~6倍。Malvar等[12]归纳总结出b2取值的表达式为
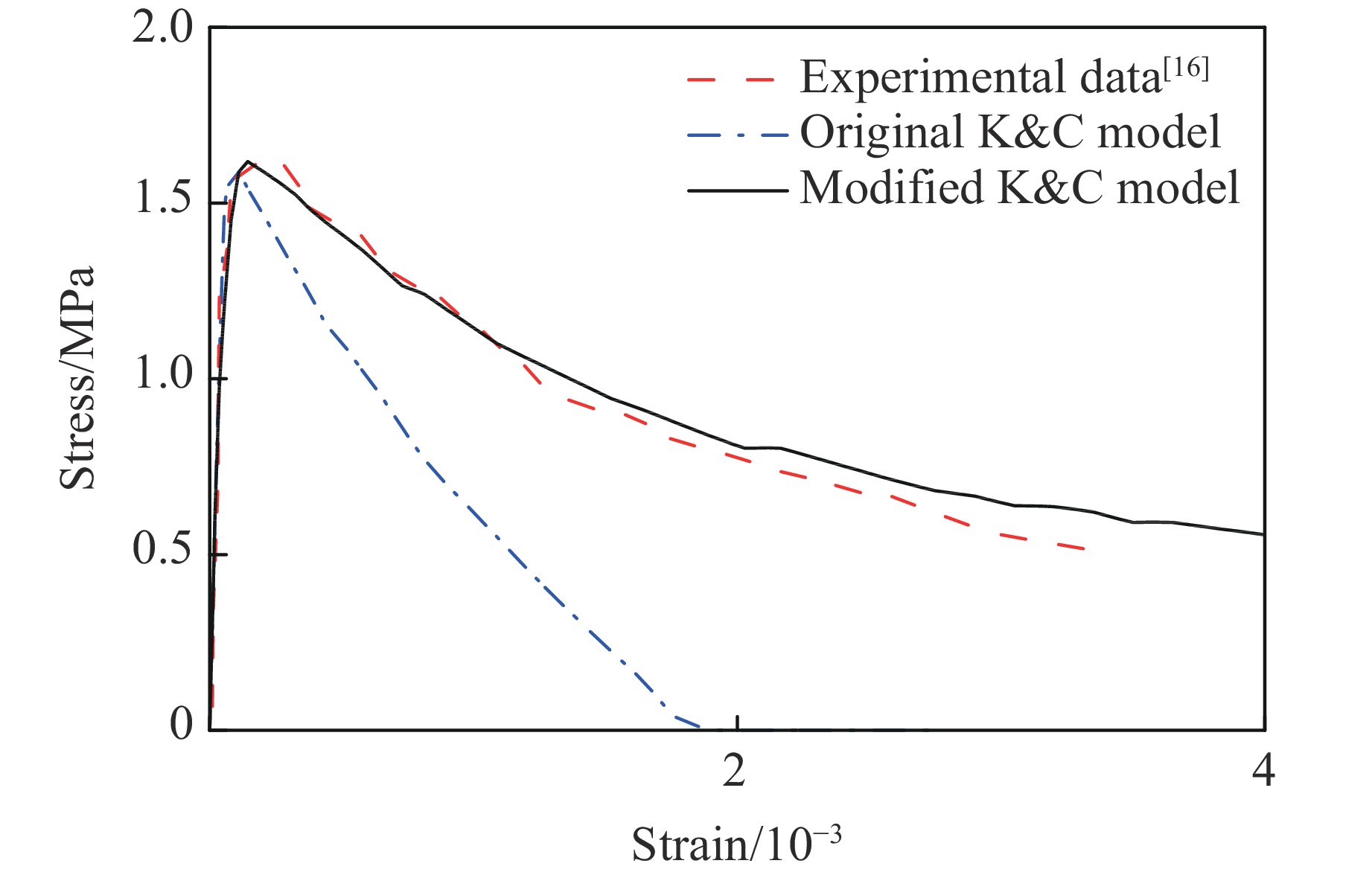
{{{b}}_2} = (0.09h_{\rm{w}}^2 - 0.98{h_{\rm{w}}} + 3.06)(1 - 0.004f_{\rm{c}}^2 + 0.097{f_{\rm{c}}} - 0.484) (12) 众所周知,当钢纤维掺入混凝土中时,SFRC的断裂能呈阶跃式增长,且随着钢纤维体积分数的增长而增长。因此式(12)对于普通混凝土是适宜的,却不能正确反映SFRC对能量的吸收能力。根据董振英等[16]的实验得到的SFRC的断裂应变能481 N/m,建立单个单元模型,以模拟单轴拉伸试验(UUT),采用Bazant建议的方法,强迫单轴拉伸应力-应变曲线下方的面积为Gf/hw,校准SFRC的拉伸损伤参数(b2 = 0.2),由式(12)自动生成的拉伸损伤参数b2 = 2.1。原模型与校准损伤参数及修正损伤演化函数后的K&C模型对单轴拉伸应力-应变曲线的描述如图5所示。由图5可知,修正后的K&C模型的应力-应变曲线软化段表现出更高的韧性、延性和断裂应变能,且与实验数据的吻合程度较高。
1.4 改进SFRC的受压动力增强因子模型
SFRC的骨料强度大于砂浆与交界面强度,在高应变率下,断裂机理发生改变,裂纹扩展极为迅速,裂纹的扩展可能直接通过骨料区域,而不是骨料与砂浆的交界面,混凝土的单轴受压强度增长可达100%以上,而单轴受拉强度增长可达600%以上。目前,已有几种应变率模型用于预测混凝土在动态压缩荷载下的强度增强效应。被广泛接受的模型由CEB-FIP[17]模式规范提出,定义动态强度和静态强度的比值与应变率的函数关系,即受压动力增强因子(CDIF)表示为
{\rm{CDIF}} = \frac{{{f_{{\rm{c,d}}}}}}{{{f_{{\rm{c,s}}}}}} = \left\{ {\begin{aligned} & {{{\left(\frac{{\dot \varepsilon }}{{{{\dot \varepsilon }_{\rm{s}}}}}\right)}^{1.026\delta }}}\quad\; {\dot \varepsilon < 30\;{{\rm{s}}^{ - 1}}} \\ & {{\gamma _{\rm{s}}}{{\left(\frac{{\dot \varepsilon }}{{{{\dot \varepsilon }_{\rm{s}}}}}\right)}^{{1}/{3}}}} \quad\;{\dot \varepsilon \geqslant 30\;{{\rm{s}}^{ - 1}}} \end{aligned}} \right. (13) 式中:fc,d为动态压缩强度;fc,s为静态压缩强度;
\dot \varepsilon 为当前应变率;{\dot \varepsilon _{\rm{s}}} 为基准静态应变率,{\dot \varepsilon _{\rm{s}}} =3 \times {10^{{\rm{ - }}5}}\; {{\rm{s}}^{ - 1}} ;lg γs = 6.156δ – 2,δ = fc,0/(5fc,0 + 9fc,s),fc,0 = 10 MPa。Wang等[18]开展了SFRC的霍普金森压杆试验研究,在CEB-FIP公式的基础上考虑了纤维效应,修正了过渡应变,提出了CDIF模型
{\rm{CDIF}} = \left\{ {\begin{aligned} & {{{\left(\frac{{\dot \varepsilon }}{{{{\dot \varepsilon }_{\rm{s}}}}}\right)}^{1.026\delta }}}\qquad\;\;\;\; {\dot \varepsilon < (30 + 23{{i}})\;{{\rm{s}}^{ - 1}}}\\ & {\eta {{\left(\frac{{\dot \varepsilon }}{{{{\dot \varepsilon }_{\rm{s}}}}}\right)}^{{{(1 + 0.05{{i}})}}/{3}}}}\quad {\dot \varepsilon \geqslant (30 + 23{{i}})\;{{\rm{s}}^{{\rm{ - }}1}}} \end{aligned}} \right. (14) 式中:i为考虑纤维效应的参数,i等于0或1,分别代表素混凝土及纤维增强混凝土;η = γs(1 – 0.339 2i),其他变量同CEB-FIP模型。
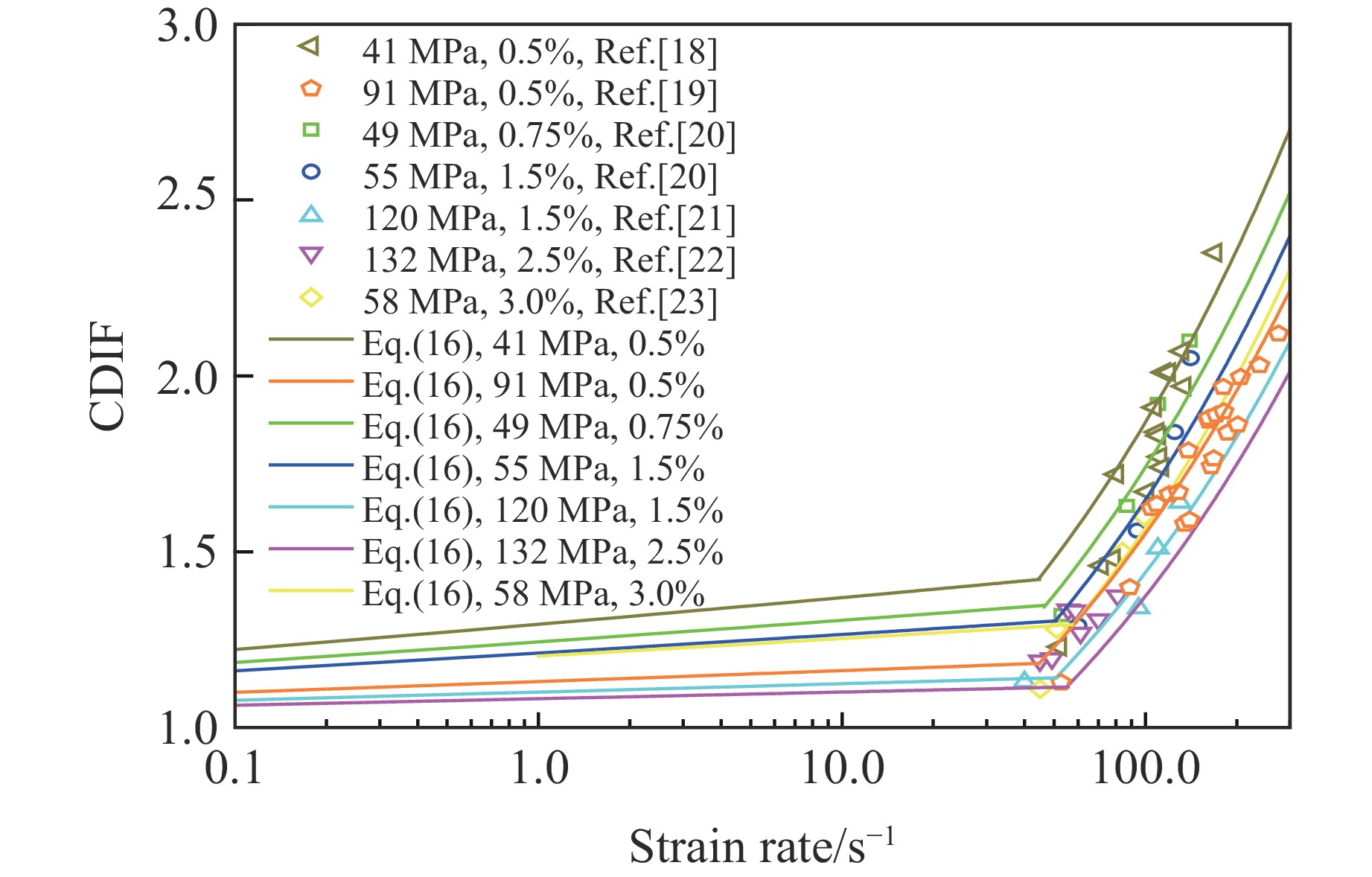
CEB-FIP模型能够较好地预测普通混凝土的DIF值,但对SFRC的DIF值估计过高,如图6所示。众多学者的研究数据[19-23]表明,过渡应变率之后,SFRC的应变率敏感性低于普通混凝土,原因在于:(1)根据ACI446[24]的研究结果,高应变率下混凝土强度的增长速率随着裂缝扩展速度的增大而增大,而SFRC的高密实性和钢纤维的阻裂作用使得裂缝扩展速率与素混凝土相比较慢;(2)钢纤维的应变率敏感性低于混凝土基体。因此需提出适用于SFRC的CDIF模型。
由于侧向惯性效应,在过渡应变率之后,DIF曲线的斜率急剧增大,表明混凝土的物理性能发生了变化。钢纤维的约束效应有效地减少了混凝土的横向膨胀,使得惯性效应更加显著,需要更高的过渡应变率触发SFRC的物理性能变化,因此有必要提出适用于SFRC的过渡应变率模型。
Wang等[18]提出的模型虽与众多学者的试验数据边界大致相符,但没有给出具体的钢纤维体积分数所对应的曲线,对过渡应变率模型的描述也不够精确。本研究在式(14)的基础上,引入了钢纤维体积分数Vf,并修正过渡应变率,通过最小二乘法回归分析大量实验数据,给出CDIF值,即
{\rm{CDIF}} = \left\{ {\begin{aligned} & {{{\left(\frac{{\dot \varepsilon }}{{{{\dot \varepsilon }_{\rm{s}}}}}\right)}^{1.026\delta }}}\qquad\quad\quad\quad {\dot \varepsilon < {\varepsilon _{\rm{t}}}\;{{\rm{s}}^{ - 1}}}\\ & {{\gamma _{{V_{\rm{f}}}}}{\gamma _{\rm{s}}}{{\left(\frac{{\dot \varepsilon }}{{{{\dot \varepsilon }_{\rm{s}}}}}\right)}^{{{(1 + 0.018{{\mathop V\nolimits} _{\rm{f}}})}}/{3}}}}\quad {\dot \varepsilon \geqslant {\varepsilon _{\rm{t}}}\;{{\rm{s}}^{ - 1}}} \end{aligned}} \right. (15) 式中:
{\varepsilon _{\rm{t}}} 为过渡应变率,{\varepsilon _{\rm{t}}} = 41.087 + 7.677 Vf – 0.746V_{\rm f}^2 ;{\gamma _{{V_{\rm{f}}}}} = 0.607exp( –Vf/4.972) + 0.294,{\gamma _{{V_{\rm{f}}}}} 为钢纤维体积分数因子;其他变量同CEB-FIP模型。如图7所示,式(15)得到的CDIF值与实验数据[19-23]吻合较好。1.5 SFRC受拉动力增强因子模型
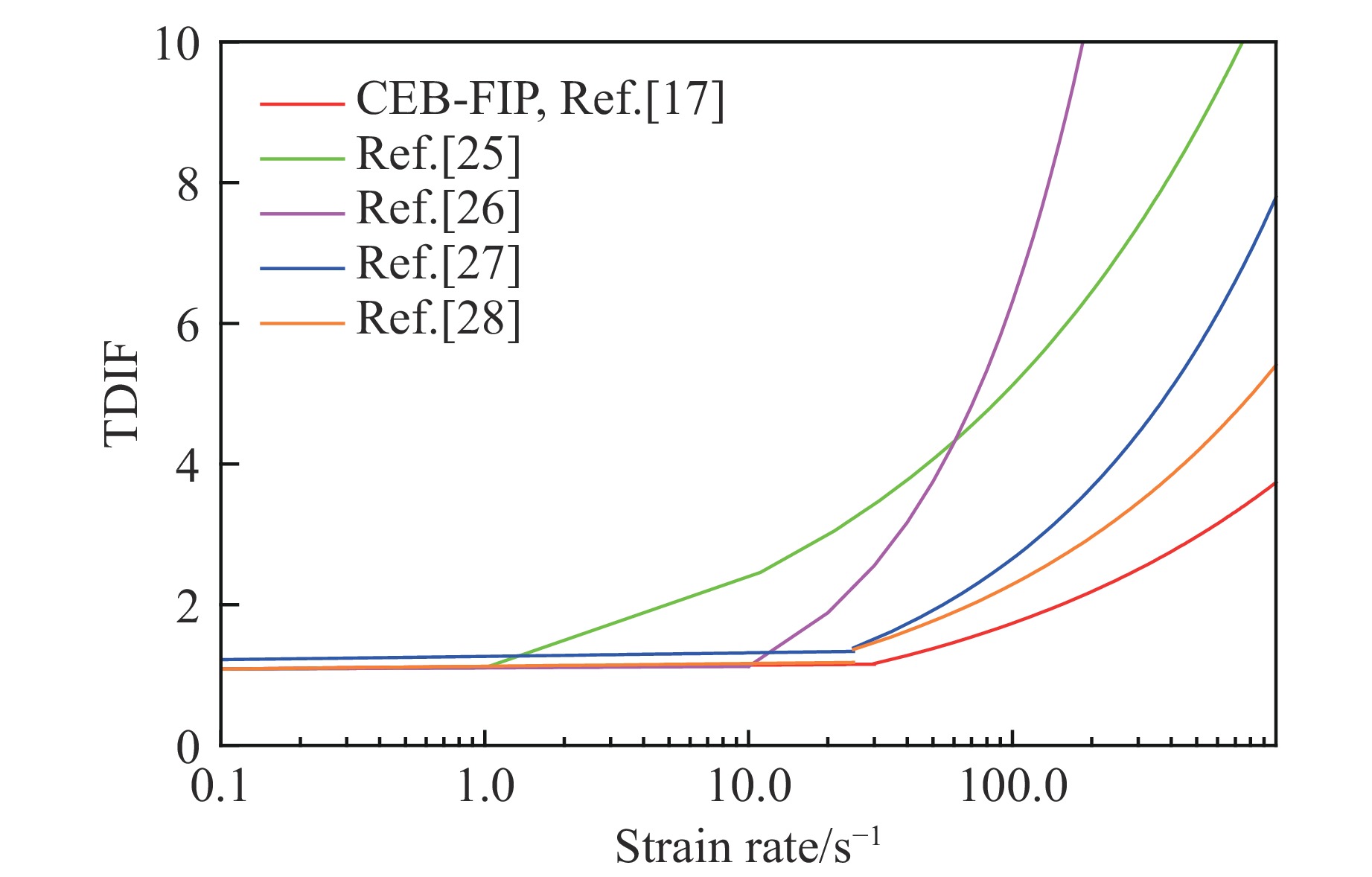
混凝土的动态抗拉强度表现出比抗压强度更高的应变率敏感性。目前研究人员在各自实验研究[17, 25-28]的基础上,提出了数种SFRC受拉动力增强因子模型(TDIF),如图8所示。由图8可以看出,TDIF模型存在较大差异,可能是因为混凝土的基体强度、纤维含量、形状、长径比、抗拉强度对TDIF值的影响较大。由于现有实验数据的分歧较大,尚不具备提出新的TDIF模型的条件,因此本研究中采用被广泛接受的CEB-FIP公式作为受拉增强因子模型
{\rm{TDIF}} = \frac{{{f_{{\rm{t,d}}}}}}{{{f_{{\rm{t,s}}}}}} = \left\{ {\begin{aligned} & {{{\left(\frac{{\dot \varepsilon }}{{{{\dot \varepsilon }_{\rm{s}}}}}\right)}^{1.016\delta }}}\quad\, {\dot \varepsilon < 30\;{{\rm{s}}^{ - 1}}}\\ & {\beta {{\left(\frac{{\dot \varepsilon }}{{{{\dot \varepsilon }_{\rm{s}}}}}\right)}^{{1}/{3}}}}\quad\;\; {\dot \varepsilon \geqslant 30\;{{\rm{s}}^{ - 1}}} \end{aligned}} \right. (16) 式中:ft,d为动态抗拉强度;ft,s为静态抗拉强度;
{\dot \varepsilon _{\rm{s}}} = 3 × 10–6 s–1;δ = fc,0/(10fc,0 + 6fc),fc,0 = 10 MPa;lg β = 7.11δ – 2.33。2. 数值模拟
2.1 SFRC板受冲击荷载
为了论证第1节中改进的失效强度面参数模型、损伤模型、受压动力增强因子模型的有效性与可靠性,对高速弹丸侵彻SFRC板[1]进行仿真分析。数值模拟所用SFRC板的钢纤维体积分数为1%,尺寸设定为310 mm × 310 mm × 50 mm,支撑于钢框架之上,自由跨度为240 mm。模拟所用的平头弹丸直径为25 mm,长度为75 mm,质量为297 g;弹丸材料为SKH-51工具钢,洛氏硬度HRC设定为60,侵彻过程中塑性变形较小。弹丸分别以58.2、76.0和104.0 m/s的速度对SFRC板进行模拟冲击实验。
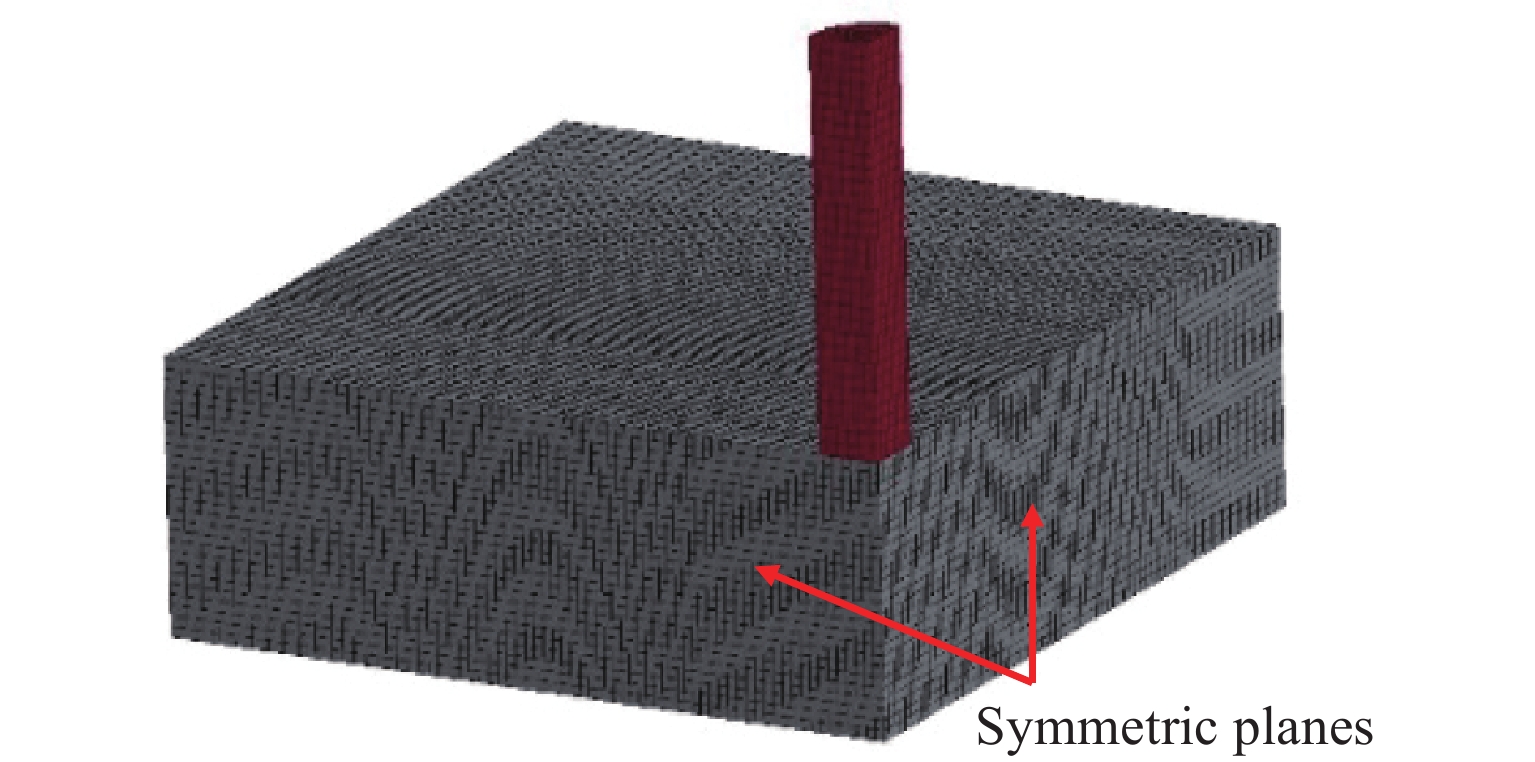
SFRC板的1/4计算模型如图9所示,对称面上施加对称约束。采用SOLID164单元对弹体和SFRC板进行离散,单元尺寸为2 mm,采用单点高斯积分拉格朗日算法。SFRC板采用改进的K&C材料模型,耦合*EOS_TABULATED_COMPACTION状态方程。最大强度面、初始屈服强度面、残余强度面参数值分别按式(5)、式(7)、式(8)确定,损伤演化函数按式(10)进行修正,损伤比例因子按1.3节的方法校准,DIF模型为式(15)和式(16)。SFRC板的主要材料参数列于表1。其中,Lw为裂缝局部宽度,ρ为密度,ν为泊松比,ω为膨胀系数。表1中未给出的参数均采用自动生成值。
表 1 改进的钢纤维混凝土K&C模型参数Table 1. Parameters of the modified K&C model of SFRCStrength surface a0/MPa a1 a2/MPa−1 a0y/MPa a1y a2y/MPa−1 a1f a2f/MPa−1 64.0 0.481 5.82×10−4 45.93 0.726 1.77×10−3 0.476 8.56×10−4 Damage Others b1 b2 b3 α αc αd λm Lw/mm fc/MPa ft/MPa ρ/(kg·m−3) ν ω 0.75 −1.50 1.15 3.00 0.381 1.90 9.5×10−5 24 175.3 13.8 2 600 0.19 0.5 弹丸采用*MAT_JOHNSON_COOK材料模型。该模型适用于应变率变化范围较大及材料软化引起的绝热温度升高问题,是弹丸冲击模拟研究中常用的模型,需耦合*EOS_GRÜNEISEN状态方程。弹丸的主要材料参数如表2所示。其中:G为剪切模量,A、B、N、C、M为输入的材料常数,Tm为熔化温度,TR为室内温度,ε为基准静态应变率,cp为定压比热容,SPALL为剥落处理方式,IT为塑性应变迭代选项,D1、D2、D3、D4、D5均为失效破坏参数。表2中未给出的参数均采用自动生成值。
表 2 平头弹丸的材料参数Table 2. Materials parameters of the flat ended projectileρ/(g·cm−3) G/GPa A/MPa B/MPa N C M Tm/K TR/K 7.83 210 792 510 0.26 0.014 1.03 1 793 294 ε/(μs−1) cp/(J·kg−1·K−1) SPALL IT D1 D2 D3 D4 D5 1.0 × 10−4 4.77 × 10−5 3.0 0.0 4.00 0.00 0.00 0.00 0.00 弹丸与靶板之间采用侵蚀接触算法*CONTACT_ERODING_SURFACE_TO_SURFACE,动摩擦因子与静摩擦因子均为0.15。采用关键字*INITIAL_VELOCITY_GENERATION产生弹丸初速度。沙漏控制方式采用LS-DYNA软件的标准黏性方式,计算时间步长比例因子为0.6。
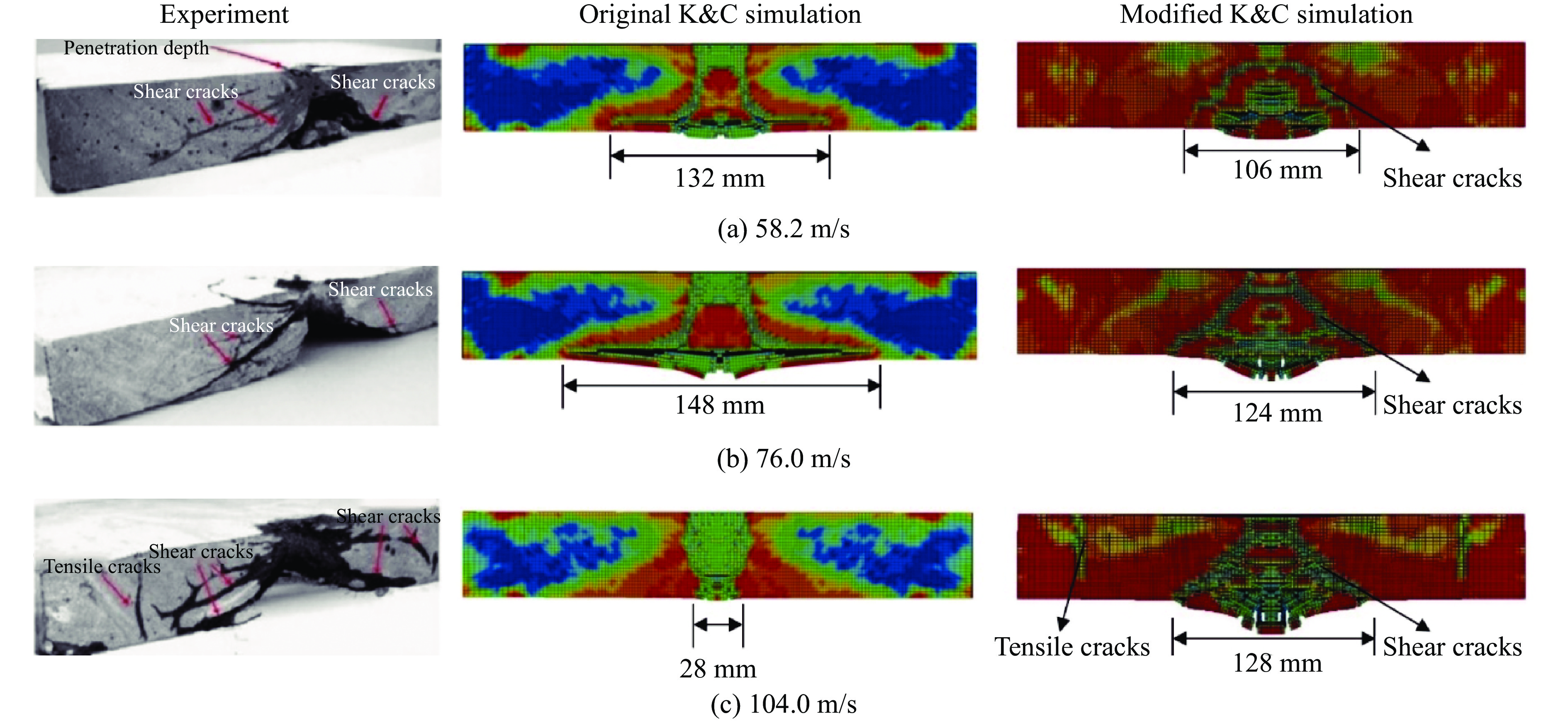
K&C材料模型未包含侵蚀失效准则。为了模拟SFRC板的破坏失效模式,还需引入侵蚀失效准则*MAT_ADD_EROSION,选取失效主应变
{\varepsilon _{\max}} 作为破坏判据,一旦单元主应变大于{\varepsilon _{\max}} ,该单元将被立即删除。侵蚀失效值依赖于网格尺寸,且因材料而异,并适用于特定研究环境。本研究在选择侵蚀失效值时,主要考虑避免单元的大量缺失和保持质量守恒。如图4中SFRC的峰值应变为0.003,考虑到在应变软化阶段,断裂应变为压缩应变的2倍,还需考虑应变率效应和纤维约束效应等其他影响(建议为10倍),因此失效主应变取为0.06。实验结果[1]及改进前后K&C模型预测的SFRC板动力响应的对比如图10所示。由图10可知,原K&C模型的预测结果与实验结果的破坏模式吻合较差,不能预测到实验中的破坏主裂缝,而改进的K&C模型的预测结果与实验结果的破坏模式吻合良好,准确描述了剪切裂缝和拉伸裂缝的发展。SFRC板在弹丸侵彻下的结痂直径与实验结果的比较如表3所示,原K&C模型预测的结痂直径与实验结果偏差较大,而改进的K&C模型精准地预测了弹丸速度为58.2、76.0和104.0 m/s时的结痂直径,与实验结果的相对偏差分别为12.0%、4.0%和6.4%。综上所述,原K&C模型的模拟结果表明,SFRC的脆性破坏特征显著,而改进的K&C模型则准确表征了SFRC的韧性、延性及能量吸收能力,说明改进的K&C模型能更准确地预测SFRC板受冲击荷载时的动力响应。
表 3 实验数据与数值模拟结痂弹坑直径比较Table 3. Comparisons of experimental data and numerical simulation of the scabbed crater diameterProjectile velocity/(m·s−1) Scabbed crater diameter/mm Model error/% Experiment Original K&C Modified K&C Original K&C Modified K&C 58.2 120.5 132 106 9.5 12.0 76.0 119.2 148 124 24.2 4.0 104.0 120.3 28 128 76.7 6.4 2.2 SFRC板受爆炸荷载作用
K&C模型常被用来分析爆炸荷载作用下混凝土结构的动力响应,赵春风等[29]采用K&C模型分析安全壳穹顶钢筋混凝土板的抗爆性能。为进一步验证改进的K&C模型在爆炸荷载作用下预测SFRC板动力响应的有效性和可靠性,对SFRC板在爆炸荷载作用下的动力响应[2]进行数值模拟。SFRC板的尺寸设为550 mm × 550 mm × 50 mm,支撑于钢框架之上,自由跨度为460 mm。引爆圆柱形炸药对钢纤维体积分数分别为0.5%、1.0%的SFRC板进行冲击加载,TNT炸药等效当量为0.488 kg,炸药质心距SFRC板表面0.272 5 m,比例距离为0.35 m·kg–1/3。
SFRC的1/4模型如图11所示,在对称面施加对称约束,空气域的非对称面采用关键字*BOUNDARY_NON_REFLECTING施加无反射边界条件,模拟无约束爆炸条件。数值计算模型由钢纤维混凝土板、TNT炸药和空气域组成。SFRC板采用拉格朗日算法,TNT炸药和空气域单元均采用单点多物质ALE算法。另需采用关键字*ALE_MULTI_MATERIAL_GROUP将TNT炸药与空气定义为ALE材料组,它代表一个独特的流体,可与模型中的拉格朗日SFRC结构产生相互作用。采用流固耦合机制*CONSTRAINED_LAGRANGE_IN_SOLID计算SFRC板与空气和炸药之间的相互作用。TNT材料模型采用*MAT_HIGH_EXPLOSIVE_BURN,并联立*EOS_JWL状态方程。空气域采用*MAT_NULL材料模型,并联立*EOS_LINEAR_POLYNOMIAL状态方程。钢纤维混凝土材料模型为改进的K&C模型,材料参数的校准方法及其他环境变量同2.1节。
当模拟爆炸冲击波到达SFRC板时,前表面材料处于三轴压缩应力状态,可导致弹坑状的材料局部破坏。另一部分冲击波透射到SFRC板后表面时,后表面材料处于多轴拉伸应力状态,可导致混凝土的剥落和碎片弹射。实验结果[2]与改进的K&C模型模拟结果的对比如图12所示。由图12可知:改进的K&C模型较好地预测了SFRC板背面混凝土的剥落现象;数值模拟与实验的破坏模式均为典型的对角线破坏,且吻合良好;随着钢纤维体积分数的增加,SFRC板损伤破坏的程度减轻。
3. 结 论
通过对原K&C混凝土模型进行改进,研究了钢纤维混凝土板在冲击与爆炸荷载作用下的动力响应,得出以下主要结论。
(1)根据大量SFRC三轴压缩实验数据,在原失效强度面模型的基础上,考虑了钢纤维体积分数对三轴强度的影响,采用最小二乘法建立了适用于SFRC的失效强度面模型。该模型能准确描述SFRC的失效强度面。
(2)通过LS-DYNA软件模拟单元轴向拉、压试验,采用反复试验法,建立了SFRC的损伤演化模型,介绍了校准拉、压损伤参数的简便方法,模拟结果表明,新的损伤机制能准确描述SFRC的应变软化段力学特征。
(3)根据大量高应变率下SFRC的单轴压缩实验数据,在CEB-FIP受压动力增强因子模型的基础上,考虑了钢纤维体积分数对DIF值及过渡应变率的影响,建立了SFRC的CDIF模型,该模型能准确表征SFRC受压时的应变率效应。
(4)通过LS-DYNA软件模拟了高速弹丸侵彻钢纤维混凝土靶板与爆炸荷载下钢纤维混凝土板的动力响应,模拟结果验证了改进的K&C模型,能够准确表征钢纤维混凝土板受冲击和爆炸荷载作用时的动力响应。
-
表 1 混凝土各相材料参数
Table 1. Concrete material parameters of each phase
Material Elastic model/GPa Poisson′s ratio μ Bulk density t/m3 Expansion angle/(°) Flow potential offset ε Ratio of double and uniaxial compressive strength αf Constant stress ratio Kc Aggregate 30.00 0.167 2.6 Mortar 10.66 0.167 2.1 30 0.1 1.16 0.666 7 ITZ 0.167 2.1 30 0.1 1.16 0.666 7 -
[1] 陈惠苏, 孙伟, STROEVEN P. 水泥基复合材料集料与浆体界面研究综述(二):界面微观结构的形成、劣化机理及其影响因素 [J]. 硅酸盐学报, 2004, 32(1): 70–79. doi: 10.3321/j.issn:0454-5648.2004.01.013CHEN H S, SUN W, STROEVEN P. Interfacial transition zone between aggregate and paste in cementitious composites (Ⅱ): mechanism of formation and degradation of interfacial transition zone microstructure, and its influence factors [J]. Journal of the Chinese Ceramic Society, 2004, 32(1): 70–79. doi: 10.3321/j.issn:0454-5648.2004.01.013 [2] 徐晶, 王先志. 纳米二氧化硅对混凝土界面过渡区的改性机制及其多尺度模型 [J]. 硅酸盐学报, 2018, 46(8): 1053–1058.XU J, WANG X Z. Effect of nano-silica modification on interfacial transition zone in concrete and its multiscale modelling [J]. Journal of the Chinese Ceramic Society, 2018, 46(8): 1053–1058. [3] YANG C C, CHO S W. Approximate migration coefficient of percolated interfacial transition zone by using the accelerated chloride migration test [J]. Cement and Concrete Research, 2005, 35: 344–350. doi: 10.1016/j.cemconres.2004.05.038 [4] AQUINO M J, LI Z, SHAH S P. Mechanical properties of the aggregate and cement interface [J]. Advanced Cement Based Materials, 1995, 2(6): 211–223. doi: 10.1016/1065-7355(95)90040-3 [5] LEE K M, PARK J H. A numerical model for elastic modulus of concrete considering interfacial transition zone [J]. Cement and Concrete Research, 2008, 38(3): 396–402. doi: 10.1016/j.cemconres.2007.09.019 [6] 王怀亮, 宋玉普. 多轴应力状态下混凝土的动态强度准则 [J]. 哈尔滨工业大学学报, 2014, 46(4): 93–97.WANG H L, SONG Y P. A dynamic strength criterion of concrete under multiaxial stress state [J]. Journal of Harbin Institute of Technology, 2014, 46(4): 93–97. [7] 杜修力, 金浏. 考虑过渡区界面影响的混凝土宏观力学性质研究 [J]. 工程力学, 2012, 29(12): 72–79. doi: 10.6052/j.issn.1000-4750.2011.04.0216DU X L, JIN L. Research on the influence of interfacial transition zone on the macro-mechanical properties of concrete [J]. Engineering Mechanics, 2012, 29(12): 72–79. doi: 10.6052/j.issn.1000-4750.2011.04.0216 [8] 王哲. 沿应变路径准静态加载时混凝土的极限状态现象 [J]. 北京交通大学学报, 2010, 34(1): 30–34. doi: 10.3969/j.issn.1673-0291.2010.01.007WANG Z. Phenomena of concrete limit state under quasi-static loading along strain paths [J]. Journal of Beijing Jiaotong University, 2010, 34(1): 30–34. doi: 10.3969/j.issn.1673-0291.2010.01.007 [9] GUINEA G V, EL-SAYED K, ROCCO C G, et al. The effect of the bond between the matrix and the aggregates on the cracking mechanism and fracture parameters of concrete [J]. Cement and Concrete Research, 2002, 32(12): 1961–1970. doi: 10.1016/S0008-8846(02)00902-X [10] 马巍, 任建伟, 胡俊, 等. 基于不同加载制度的轻骨料混凝土动态冲击性能 [J]. 硅酸盐通报, 2019, 38(4): 974–982.MA W, REN J W, HU J, et al. Dynamical shocking property of light-weighting aggregates concrete based on impact loading regimes [J]. Bulletin of the Chinese Ceramic Society, 2019, 38(4): 974–982. [11] 阮欣, 李越, 金泽人, 等. 混凝土二维细观骨料建模方法综述 [J]. 同济大学学报(自然科学版), 2018, 46(5): 604–612.RUAN X, LI Y, JIN Z R, et al. Review of two-dimensional meso-modeling methods of concrete aggregate [J]. Journal of Tongji University (Natural Science), 2018, 46(5): 604–612. [12] 刘建南, 张昌锁. 过渡区界面对混凝土劈裂性能影响的试验与数值模拟 [J]. 科学技术与工程, 2018, 18(18): 269–274. doi: 10.3969/j.issn.1671-1815.2018.18.044LIU J N, ZHANG C S. Experiment and numerical simulation on the influence of interfacial transition zone on concrete splitting performance [J]. Science Technology and Engineering, 2018, 18(18): 269–274. doi: 10.3969/j.issn.1671-1815.2018.18.044 [13] YANG C C. Effect of the interfacial transition zone on the transport and the elastic properties of mortar [J]. Magazine of Concrete Research, 2003, 55(4): 305–312. doi: 10.1680/macr.2003.55.4.305 [14] 过镇海, 李卫. 混凝土在不同应力-温度途径下的变形试验和本构关系 [J]. 土木工程学报, 1993, 26(5): 58–69. doi: 10.3321/j.issn:1000-131X.1993.05.001GUO Z H, LI W. Deformation testing and constitutive relationship of concrete under different stress-temperature paths [J]. China Civil Engineering Journal, 1993, 26(5): 58–69. doi: 10.3321/j.issn:1000-131X.1993.05.001 [15] 刘海峰, 韩莉. 二维骨料随机分布混凝土的动态力学性能数值模拟 [J]. 高压物理学报, 2016, 30(3): 191–199. doi: 10.11858/gywlxb.2016.03.003LIU H F, HAN L. Numerical simulation of dynamic mechanical behavior of concrete with two-dimensional random distribution of coarse aggregate [J]. Chinese Journal of High Pressure Physics, 2016, 30(3): 191–199. doi: 10.11858/gywlxb.2016.03.003 [16] 蒋橙炜, 陈启东, 顾泽堃. 超声破碎混凝土的力学模型与仿真分析 [J]. 机械制造与自动化, 2019, 48(2): 84–88.JIANG C W, CHEN Q D, GU Z K. Mechanical model and simulation analysis of ultrasonic crushed concrete [J]. Machine Building & Automation, 2019, 48(2): 84–88. [17] WEIBULL W. A statistical distributions function of wide applicability [J]. Journal of Applied Mechanics, 1951, 18: 293–297. -







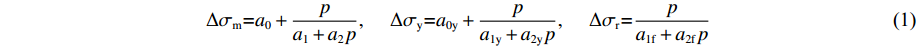


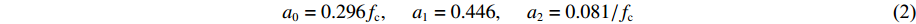
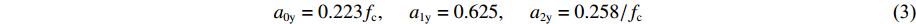
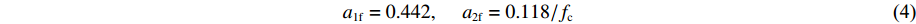
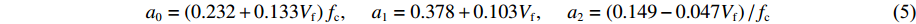

 下载:
下载:
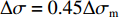
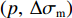
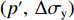
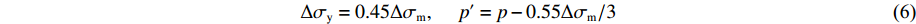
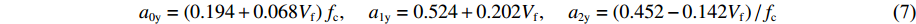
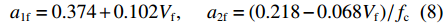

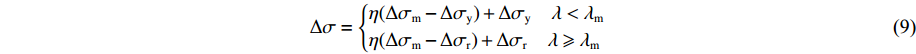
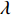
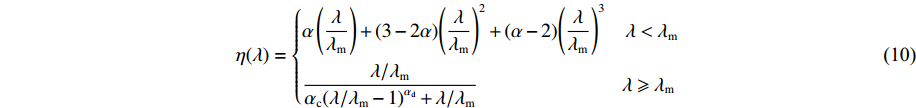

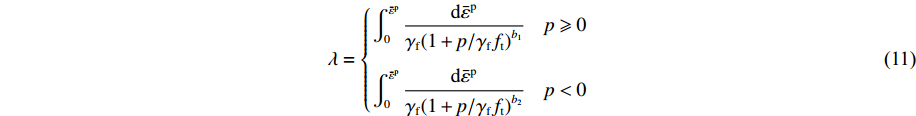



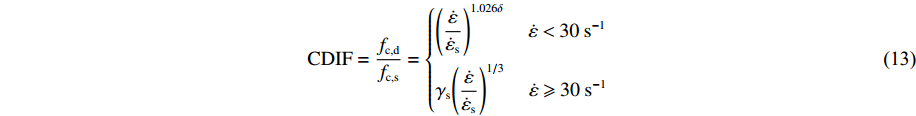
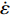
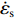
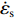
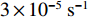
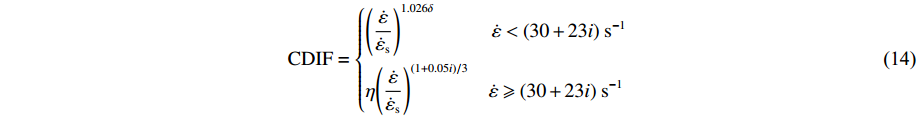

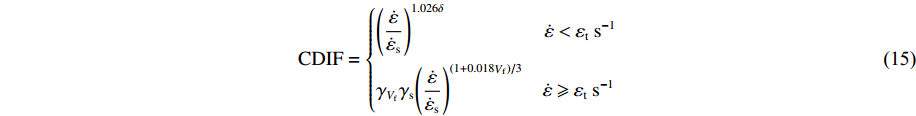
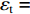
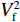
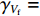
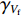


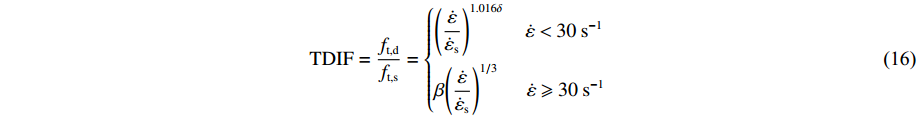
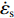

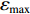
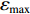




 下载:
下载: