Dynamic Characteristics of Phase Transition of Tin under Ramp Wave Loading
-
摘要: 利用磁驱动加载装置(CQ-4)和高精度速度测试装置(DPV),开展了斜波加载下锡的动态压缩实验。实验结果表明:锡在加载阶段经历了弹塑性转变和相变等物理过程,相变压力约为7.5 GPa。β–γ相变对应的特征速度随着锡厚度的增加,从676.3 m/s减小到636.8 m/s,对应的压力从7.62 GPa降低到7.11 GPa。结合Hayes多相状态方程和非平衡相变动力学模型,对锡的斜波压缩实验过程进行了模拟,数值计算结果可以较好地描述锡在加载阶段的弹塑性转变和相变等物理过程。讨论了体模量在不同热力学过程中的物理形式,计算结果显示,斜波压缩过程需考虑压力对体模量的修正。分析了相变弛豫时间、体模量等典型物理参数对速度波形的影响,结果表明,相变弛豫时间和各相初始自由能主要影响混合区部分速度波形,γ相的体模量参数只影响相变后的速度波形,β相的体模量参数会影响整体速度波形。Abstract: The dynamics of phase transition of tin under ramp wave loading was studied with experiment and simulation. The ramp wave compression experiment of tin was carried out with photonic Doppler velocimetry (PDV) and compact pulsed power generator CQ-4. The velocity wave profiles obtained experimentally show that tin undergoes physical processes such as elastoplastic transition and phase transition in the loading section, and the phase transition pressure is about 7.5 GPa. As the increase of thickness of tin, the characteristic velocity corresponding to the onset of phase transition decreased slightly from 676.3 m/s to 636.8 m/s, and the corresponding pressure was from 7.62 GPa to 7.11 GPa. The Hayes multi-phase equation of state and non-equilibrium phase transition kinetic model were employed to simulate the experimental process, and the numerical results can well describe the physical processes such as elastoplastic transformation and phase transformation in the loading section. The calculated results revealed that the correction of the bulk modulus with pressure needed to be considered under ramp wave compression. The influence of typical physical parameters, such as phase transition relaxation time and bulk modulus, on the velocity waveform was discussed. The results show that phase transition relaxation time and initial free energy mainly affect the velocity waveform in the mixing zone, the bulk modulus of the two phases affect the velocity waveform after phase transition and overall velocity waveform respectively.
-
Key words:
- phase transition /
- multi-phase equation of state /
- ramp wave loading /
- tin
-
金属锡有4个固体相和1个液态相,其结构对压力和温度非常敏感。针对锡的这些动力学特性,人们从实验[1-5]和理论[6-13]方面开展了深入研究。锡在常温常压下为I41/amd群的体立方正交结构(β相),温度低于287 K时转变为立方金刚石结构(α相),505 K时开始发生熔化[14]。利用静高压实验结合X射线衍射(XRD)装置观测到:常温下压力超过9.4 GPa时锡会发生β–γ相变[15-16],γ相为I4/mmm群的体立方正交结构,此相变为一级相变,会引起约2.6%的体积间断[2,17-21];当压力超过40 GPa[3-4]时γ相转变为bcc相,γ相和bcc相在40~56 GPa压力范围内可以共存,这一相变也是一级相变,对应的体积变化约为0.76%[14]。在冲击压缩实验中,锡的β–γ相变可以由Hugoniot数据[22]或速度波剖面[15-16]观测到,而γ–bcc相变因为体积间断太小无法识别。冲击加载下,锡在压力超过23 GPa时发生卸载熔化,在压力超过49 GPa时发生冲击熔化[16]。Vaboya等[20]、Barnett等[2]、Liu等[4] 和Cavaleri等[23]测量了锡的β相和γ相的等温压缩线。Rayne等[24]、Kamioka[25]、Hu等[26]和Song等[27]开展了锡的高压声速测量和计算。锡的冲击熔化性能也被广泛研究[9-10, 28]。Davis等[29]首次利用Z装置开展了斜波压缩下锡的动力学实验研究,观测到了锡的β–γ相变,并利用Hayes[30]多相状态方程对实验过程进行了数值模拟,计算结果和实验结果大致吻合,但在相变混合区存在较大差异。Anderson等[15]利用冲击实验开展了锡的相变和层裂研究,观测到了相变和层裂现象,给出了物理模型及参数,但未给出计算结果。
对于锡的多相状态方程,Anderson等[15]、Cox[31]、Buy等[32]分别构建了Grüneisen形式的物态方程,Khishchenko[33]建立了自由能形式的完全物态方程,但并未给出模型参数,张林等[34]基于德拜模型构建了锡的β相和γ相的自由能形式的完全物态方程,该模型计算的相图和等温压缩线可以和实验较好吻合。Song等[27]结合多相状态方程和多相Steinberg Guinan本构模型对锡的冲击实验进行了数值模拟,其中多相本构关系考虑了包氏效应(Bauschinger effect),可以更好地描述冲击卸载过程。
迄今为止,锡的相变动力学特性研究主要集中在静高压和冲击实验,斜波压缩实验技术在材料相变动力学研究方面具有一定的优势[35],但是将斜波加载技术用于锡的相变研究工作很少。另外,锡的多相状态方程研究主要集中在Grüneisen状态方程形式,自由能研究主要用于计算相图,利用基于自由能的多相状态方程对动力学实验过程开展数值模拟的工作未见公开报道。本研究基于磁驱动加载装置CQ-4[36]开展纯锡的斜波压缩动力学实验,探究锡的相变动力学特性;结合Hayes多相状态方程和非平衡相变速率模型,对实验动力学过程开展数值模拟,用以模拟锡的弹塑性转变和相变等物理过程。
1. 加载实验原理和负载区设计
1.1 磁驱动斜波加载实验原理
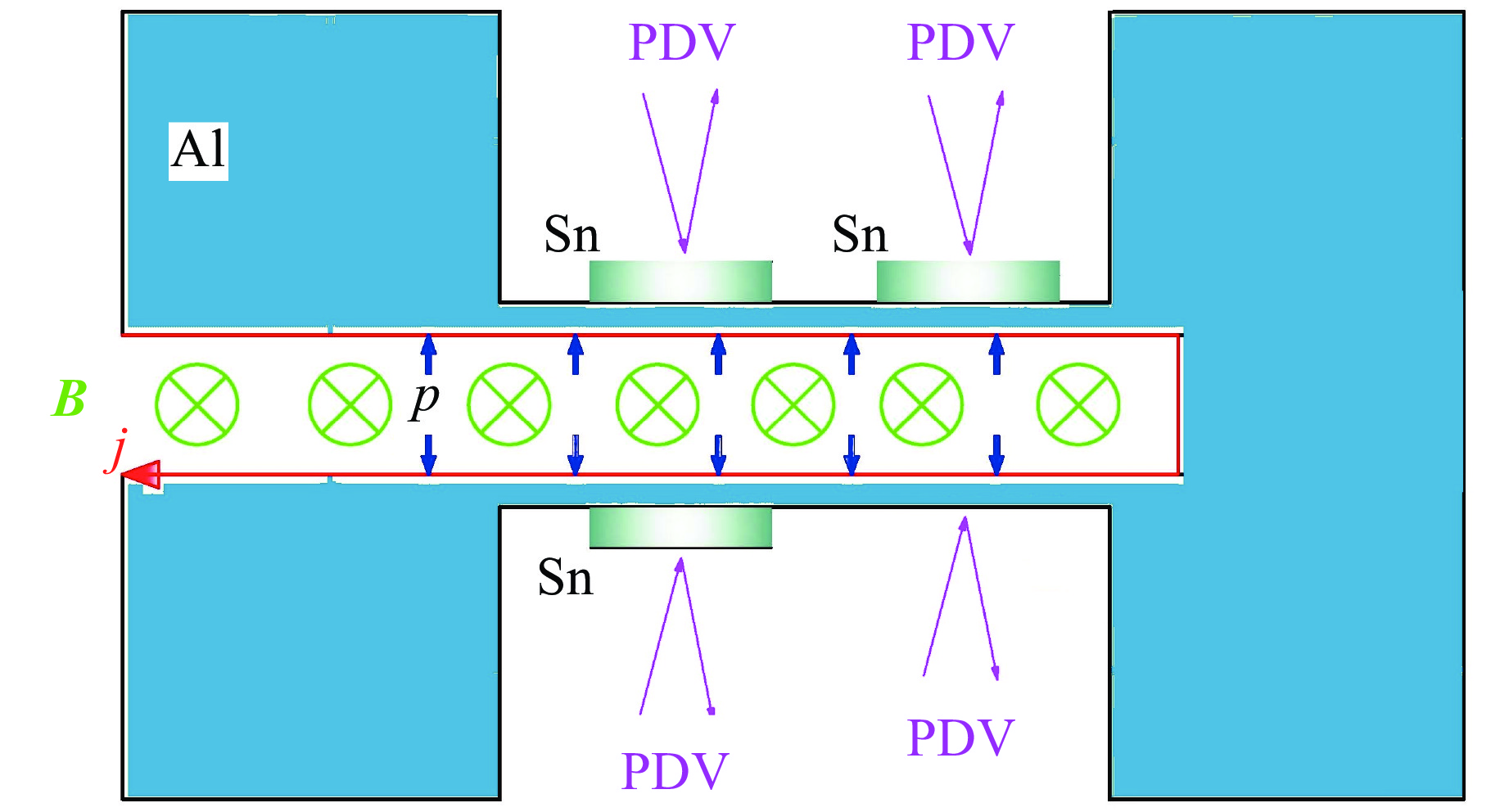
CQ-4装置是采用低电感电容器组储能、固体绝缘、平行板传输的紧凑型磁驱动加载装置,当工作电压为85 kV时,负载区可输出上升沿400~600 ns、峰值约4.0 MA的光滑脉冲电流[36]。磁驱动准等熵平面压缩装置的加载原理如图1所示。脉冲大电流流经由两个相近的平行导电平板构成的回路时,在两个电极板之间的间隙中感生脉冲强磁场。由于趋肤效应,脉冲电流沿电极板内表面流动,脉冲大电流和感生强磁场相互作用产生的洛伦兹力作用在电极板内表面,形成压缩应力脉冲(磁压力)并沿电极板厚度方向传播,作用在置于电极板上的样品。所产生的磁压力与放电电流之间的关系[37]为
p=12kμj2 (1) 式中:p为压力;μ为真空磁导率;j为流经极板内侧的线电流密度;k为电极构型系数,k值主要与极板构型、上下极板间隙、电极材料、放电波形等因素相关。
1.2 实验负载区设计
为保证在实验关注的时间、空间范围内对锡样品进行一维应变加载,且保证样品中不形成冲击波,需要对磁驱动斜波加载实验负载区构型进行优化设计,具体的设计方法见文献[37]。本实验的负载区布局如图1所示,单发实验设置4个速度测试点,分别测量3个不同厚度的锡样品自由面速度和1个铝极板自由面速度,4个测速点均设置在样品或极板中心位置。实验样品和极板的参数如表1所示。3个锡样品的直径均为8.0 mm,厚度分别为1.278、1.568和1.871 mm;极板宽度为8.0 mm,厚度分别为1.006、1.006、0.998和0.996 mm,铝极板自由面速度测试位置的极板厚度为0.996 mm。
表 1 实验条件Table 1. Experimental conditionExp. No. Position Material Size/(mm × mm) Plate_top left 1100Al 8.0 × 1.006 Sample_top left Sn ∅8.0 × 1.278 Plate_top right 1100Al 8.0 × 1.006 Shot 696 Sample_top right Sn ∅8.0 × 1.568 Plate_bottom left 1100Al 8.0 × 0.998 Sample_bottom left Sn ∅8.0 × 1.871 Plate_bottom right 1100Al 8.0 × 0.996 2. 实验结果分析
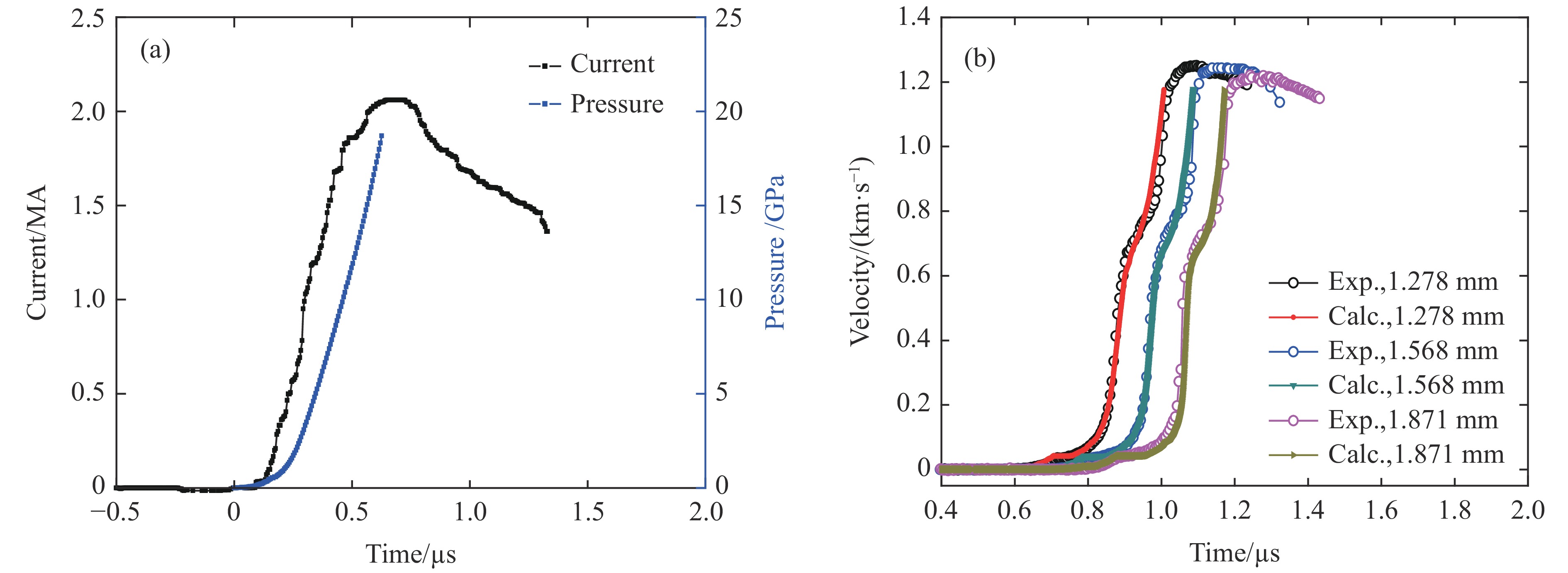
实验测得3种厚度的锡样品的自由面速度剖面如图2所示。由图2可知,3种厚度的锡样品的自由面速度剖面波形基本一致,只是幅值存在差异。随着加载压力的增加,锡的自由面速度平滑上升,依次经历弹塑性转变和相变过程。速度剖面上动力学过程对应的特征值如表2所示,锡的弹塑性拐点对应的特征速度uEP约为40 m/s,弹性极限为381.2 MPa,对应的屈服强度为197.0 MPa,3种厚度锡样品的特征值基本相等。β–γ相变对应的特征速度随着锡厚度的增加,从676.3 m/s减小到636.8 m/s,对应的压力从7.62 GPa降低到7.11 GPa,这是由相变应力波演化造成的,与现有的铁的冲击实验结果变化趋势相同。
表 2 速度波剖面上的特征值Table 2. Characteristic values of the velocity profilesThickness of Sn/mm uEP/(m·s–1) uPT/(m·s–1) pPT/GPa 1.278 40.0 676.3 7.62 1.568 39.6 660.0 7.41 1.871 40.2 636.8 7.11 3. 数值模拟与分析
为了准确地描述相变的动力学过程,除守恒方程外,还需要描述相变过程的相变动力学方程和多相状态方程。本研究采用率相关的非平衡相变动力学方程和Hayes多相状态方程。
3.1 相变动力学方程
非平衡相变动力学方程采用Hayes[30]模型,其物理背景为:相变速率与相变驱动力及可供初始相相变生长的空间成正比,具体形式为
∙ξ=H1rkBTG1−G2τ,H={1−ξG1−G2⩾D120−D21<G1−G2<D12ξG1−G2⩽−D21 (2) 式中:ξ为新相的质量分数,H为可供初始相相变生长的空间,G为Gibbs自由能,G1–G2为相变驱动力,r为单位体积中的原子数,kB为Boltzmann常数,T为当前温度,
τ 为相变弛豫时间,D12为初始相向新相转变的能障,D21为新相向初始相转变的能障。当相变弛豫时间τ = 0时,满足相变临界准则,相变瞬间完成,这就是平衡相变模型。3.2 锡的自由能
相变速率方程需要计算各相的Gibbs自由能,而Gibbs自由能也可以由Helmholtz自由能得到(G(v,T) = F(v,T) + pv)。锡在给定比容 v和温度 T 时的Helmholtz自由能[34]为
F(v,T)=ϕ0(v)+Fion(v,T)+Fel(v,T) (3) 式中:
ϕ0(v) 为固体的结合能,Fion(v,T) 为晶格的振动自由能,Fel(v,T) 为电子的自由能。固体的结合能只是比容的函数,与温度无关,具体表达式为
ϕ0(v)=Φ0(vR)+(BRB′R−pR)(v−vR)+BRvRB′R(B′R−1)[(vRv)B′R−1−1] (4) 式中:vR为参考比容,Φ0(vR)为结合能在参考比容的值,BR为绝对零度时固体在参考点处的体模量,
B′R 为BR对压强的导数,pR为对应的压强。在准谐振子近似下,晶格的振动自由能形式为
Fion(v,T)=NkB{98Θ(v)+3Tln[1−e−Θ(v)/T]−TD(Θ(v)T)} (5) 式中:N为固体单位质量的原子数,
Θ(V) 为德拜温度,D(z)为德拜积分。电子的自由能表达式为
Fel(v,T)=−12Γ(vR)(vvR)αT2 (6) 锡的Helmholtz自由能参数见表3。
3.3 多相状态方程和本构关系
Hayes[30]提出的多相状态方程广泛应用于材料动态压缩下相变过程的数值模拟研究,其压力和温度的具体计算形式为
{˙p=BS,ξ(˙εv−Δεv⋅˙ξ−αξ˙T)˙T=−TΔη⋅˙ξ+Asij˙γCijcp,ξ (7) 式中:ξ为某时刻各相质量分数,
BS,ξ 为该时刻的等熵体模量,Δεv 为相变引起的体应变间断,cp,ξ 为定压比热容,αξ 为体膨胀系数。对等熵体模量(
BS,ξ )进行细致分析。在冲击压缩相变实验中,材料的物理状态可以认为是从初始态跳跃到终态,在压力-比容平面上对应的路径是两条Rayleigh线,体波声速对应的是两条Rayleigh线的斜率,可以认为是常数,由热力学关系K0=ρ0C20 可知,各相的体模量也可设为常数。在斜波压缩相变实验中,材料的物理状态是连续的从初始态过渡到终态,在压力-比容平面上对应的路径是完整的(准)等熵线,体波声速、体模量等物理量都是连续变化的,并且是当前状态(压力、温度、比容等)的函数,不能再近似为常数。本研究只考虑压力对体模量的影响,斜波压缩过程中温度升高很小,可以忽略温度的影响,借鉴适用于等熵过程的Murnaghan状态方程,将等熵体模量写为BS=BS0+B′S0p (8) 式中:
BS 为等熵体模量,BS0 为初始等熵体模量,B′S0 为体模量对压力的一阶导数,p为压力。本研究中BS0 和BR相等,B′S0 和B′R 相等。本构关系采用Steinberg模型[38]
Y=Y0[1+κ(εp+εi)]n[1+Apη−1/3−C(T−300)] (9) 式中:Y0为初始屈服强度,
κ 和n为硬化系数,εp 为等效塑性应变,εi 为初始塑性应变,A和C分别为剪切模量对压力和温度的系数,η=V0/V。由于锡的β相和γ相强度较低,差异较小,对相变影响较小,本研究对两相采用相同的模型和参数,具体参数见表4。3.4 计算结果和分析
本研究以极板内表面压力历史为输入条件,加载压力由铝极板自由面速度历史结合反积分程序给出,图3(a)显示了加载电流和压力随时间的变化,电流上升沿约为550 ns,电流峰值约为2 MA,压力峰值约为18 GPa。3种厚度的锡的物理模型和对应的参数均相同,加载边界相同,图3(b)为计算和实验速度波形图。由图3(b)可知,计算和实验结果基本一致,计算结果能很好地再现锡样品在斜波压缩下经历的弹塑性转变和相变过程,且计算得到的弹塑性拐点和相变拐点数据与实验结果吻合,验证了物理模型及其参数的正确性。
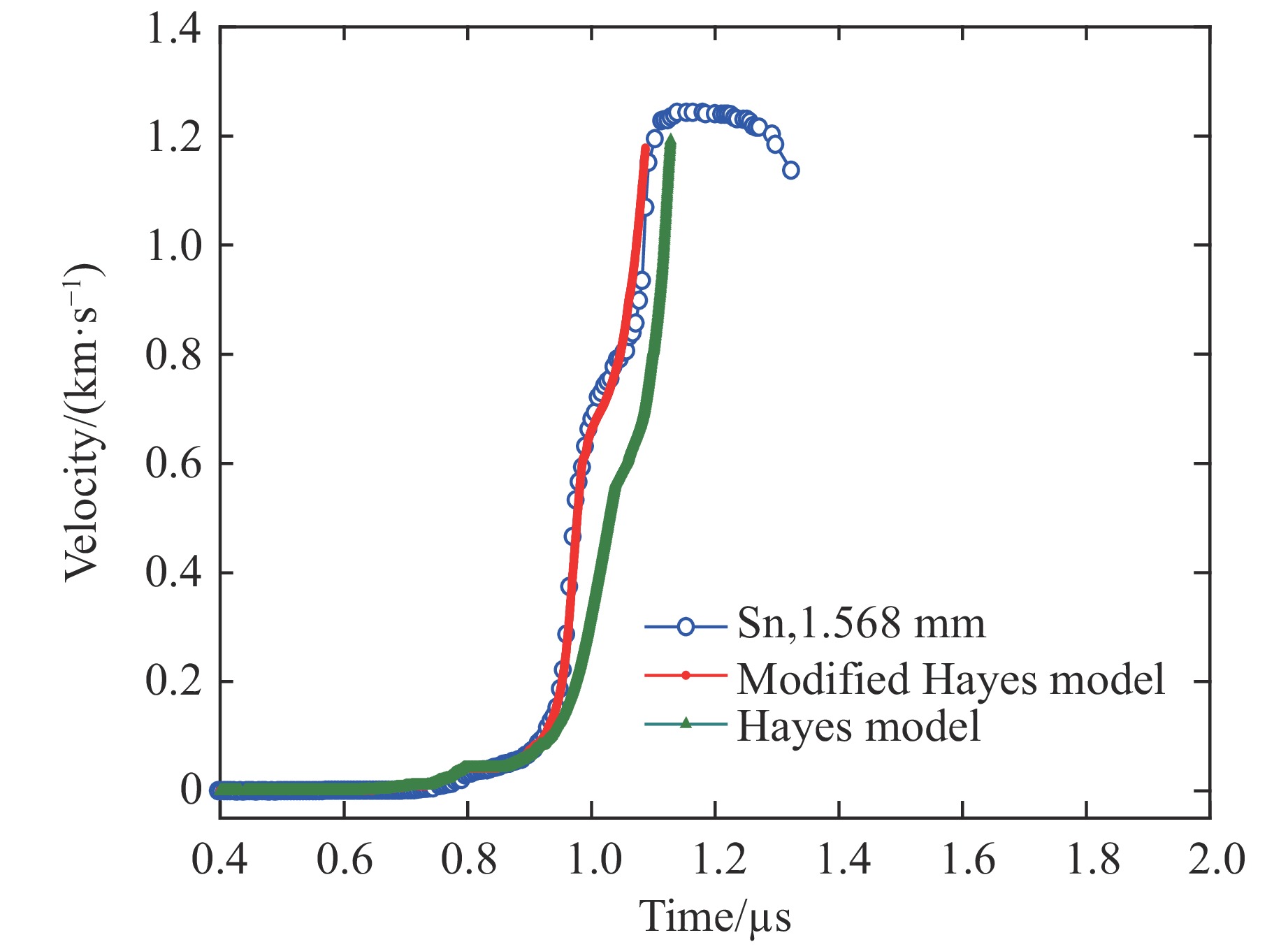
图4为考虑和未考虑式(8)对Hayes模型修正两种情况的计算结果和实验速度剖面,未考虑修正的Hayes模型
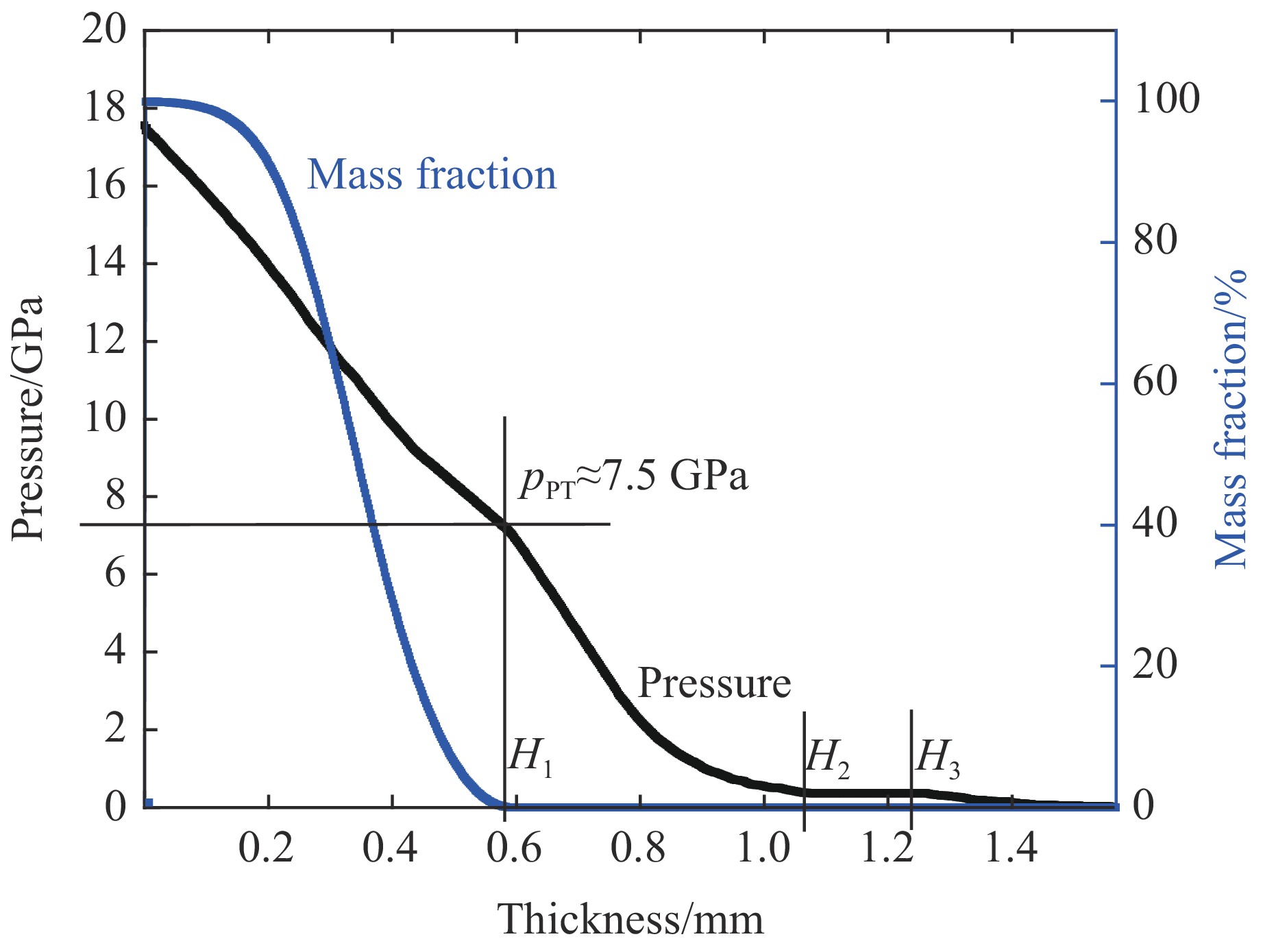
B′S0=0.0 ,其他参数不变。由图4可知:未修正模型得到的计算结果在发生弹塑性转变之后小于实验结果,相变起始点对应的特征速度小于实验值;修正后的Hayes模型得到的计算结果与实验结果吻合良好,两种情况最终得到的峰值速度基本相等。整体来看,未考虑修正模型得到的计算结果显示材料偏软,这是由于两种情况下的等效体模量不同。图5为0.7 μs时厚度为1.568 mm的锡样品中的压力和γ相的质量分数沿厚度方向的分布曲线,将厚度H = 0 mm处定义为加载面,H = 1.568 mm处定义为自由面。由图5 可知,压力在加载面处最高,约为18 GPa,随后沿锡的厚度方向降低,在自由面附近压力为零。由于压力作用及材料本身的动力学特性,根据锡所处热力学状态的不同,将压力沿样品厚度方向分布曲线分为4个区域:自由面至H3(H3 ≈ 1.26 mm)为压力较低的弹性段;H3至H2(H2 ≈ 1.07 mm)为压力基本相同的弹塑性转变段,H3处压力约为380 MPa;H2至H1(H1 ≈ 0.58mm)为压力逐渐上升的β相塑性段;H1至加载面,γ相质量分数从零递增到100%,这一区域为混合相区,H1处压力约为7.5 GPa。从H1处开始,向加载面方向压力上升的斜率明显减小,这是由相变引起的体应变间断Δεv 造成的,由于Δεv < 0,如果样品在某位置处发生相变,那么等效体应变中将会产生一个负的修正项Δεv⋅˙ξ ,该修正项会导致压力减小,可以近似地认为相变的同时产生一个稀疏波,该稀疏波的强度与材料相变引起的体应变间断和这一时刻的相变速率相关。3.5 模型参数对速度波形的影响
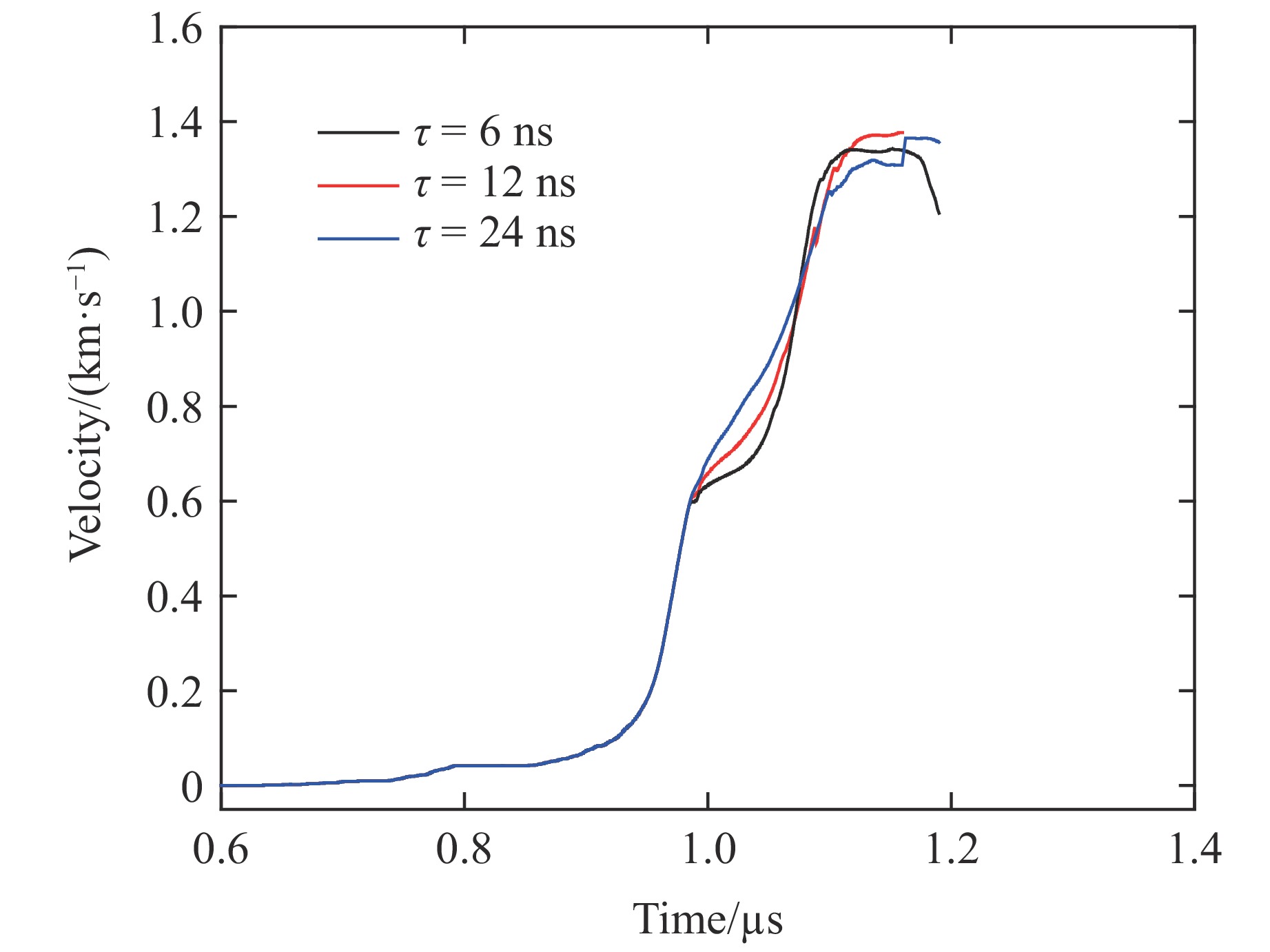
参数
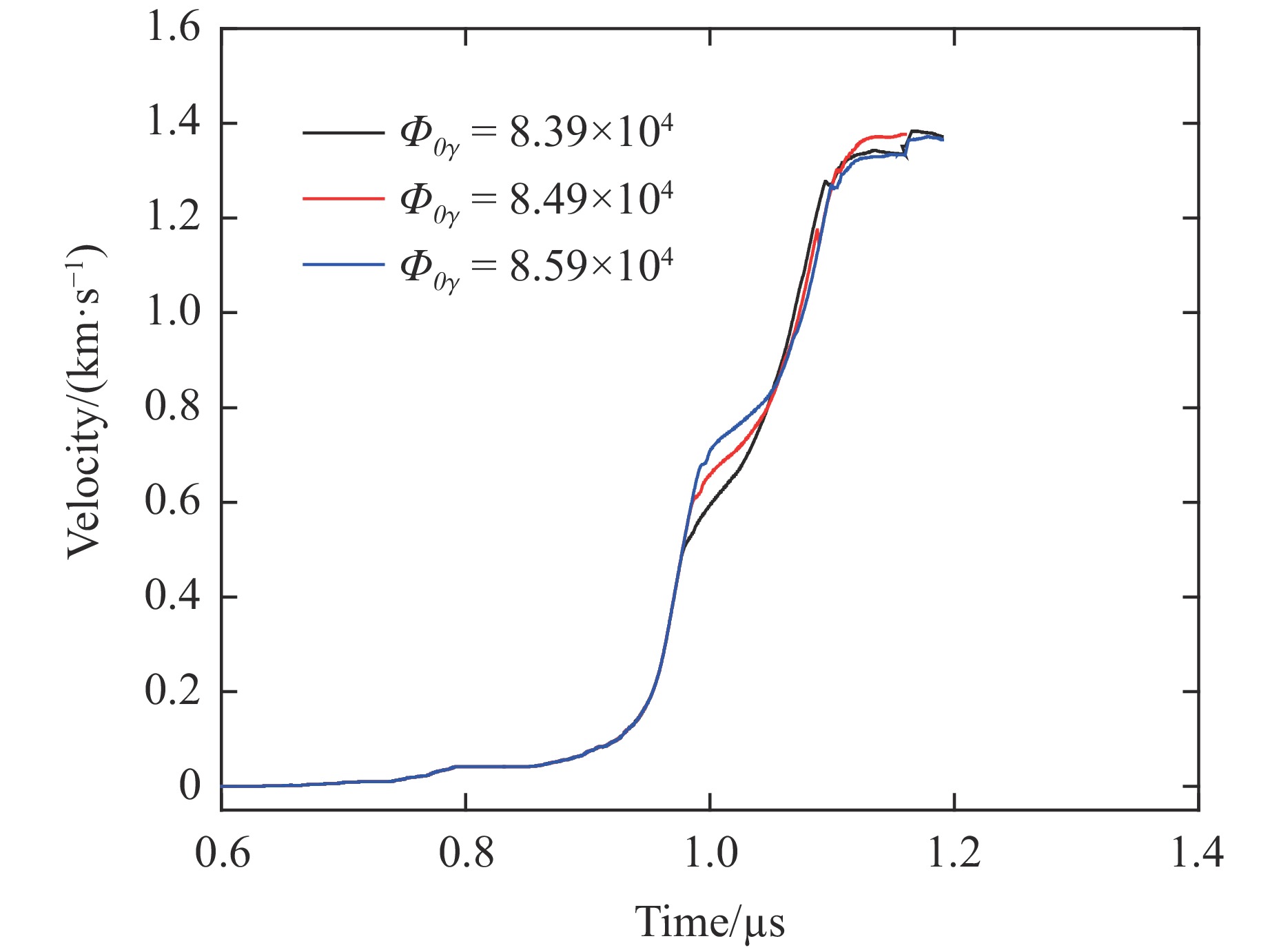
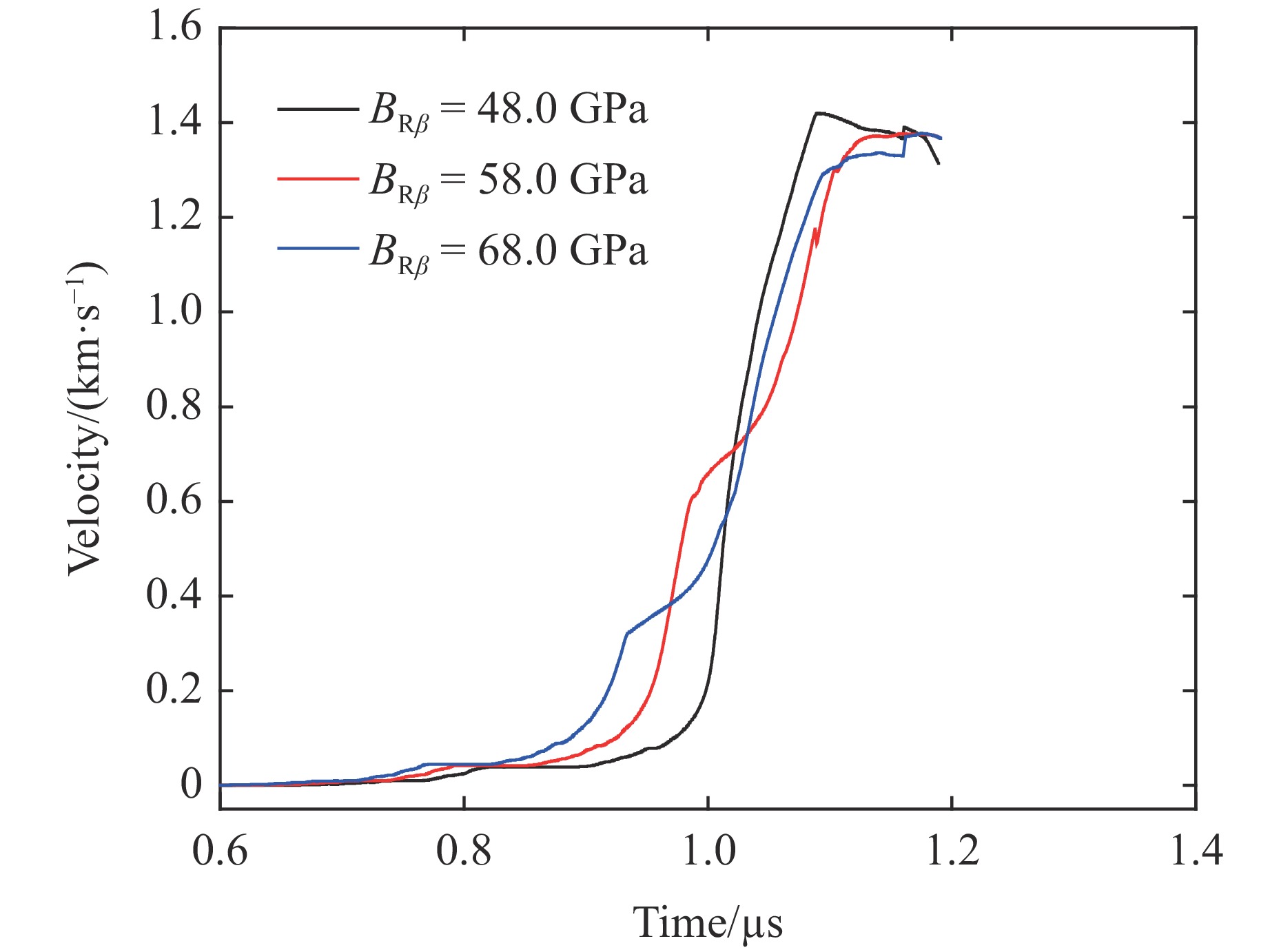
τ 为相变弛豫时间,其物理意义为相变建立平衡需要的时间。理论上τ 值越大,相变需要的时间越长,相变混合区跨越的压力区间越大,由β相向γ相的力学特性过渡越平稳。在速度波形上表现为,τ 值越大,相变混合区对应的速度区间斜率越平滑。不同相变弛豫时间对应的速度波形如图6所示,其他动力学参数不变,如表4所示,得到的计算结果与理论分析结果相同。参数Φ0为结合能在参考比容的值,也是Helmholtz自由能的初始值,理论分析时更关心各相间的相对值,因此一般将初始相的值设为零,由相变动力学方程式(2)可得,新相的Φ0值直接影响相变起始点。根据不同Φ0计算得到的结果如图7所示,其他动力学参数不变,得到的计算结果与理论预估趋势吻合,但是整体波形基本不变。参数BRβ为β相的初始体模量,由体波声速
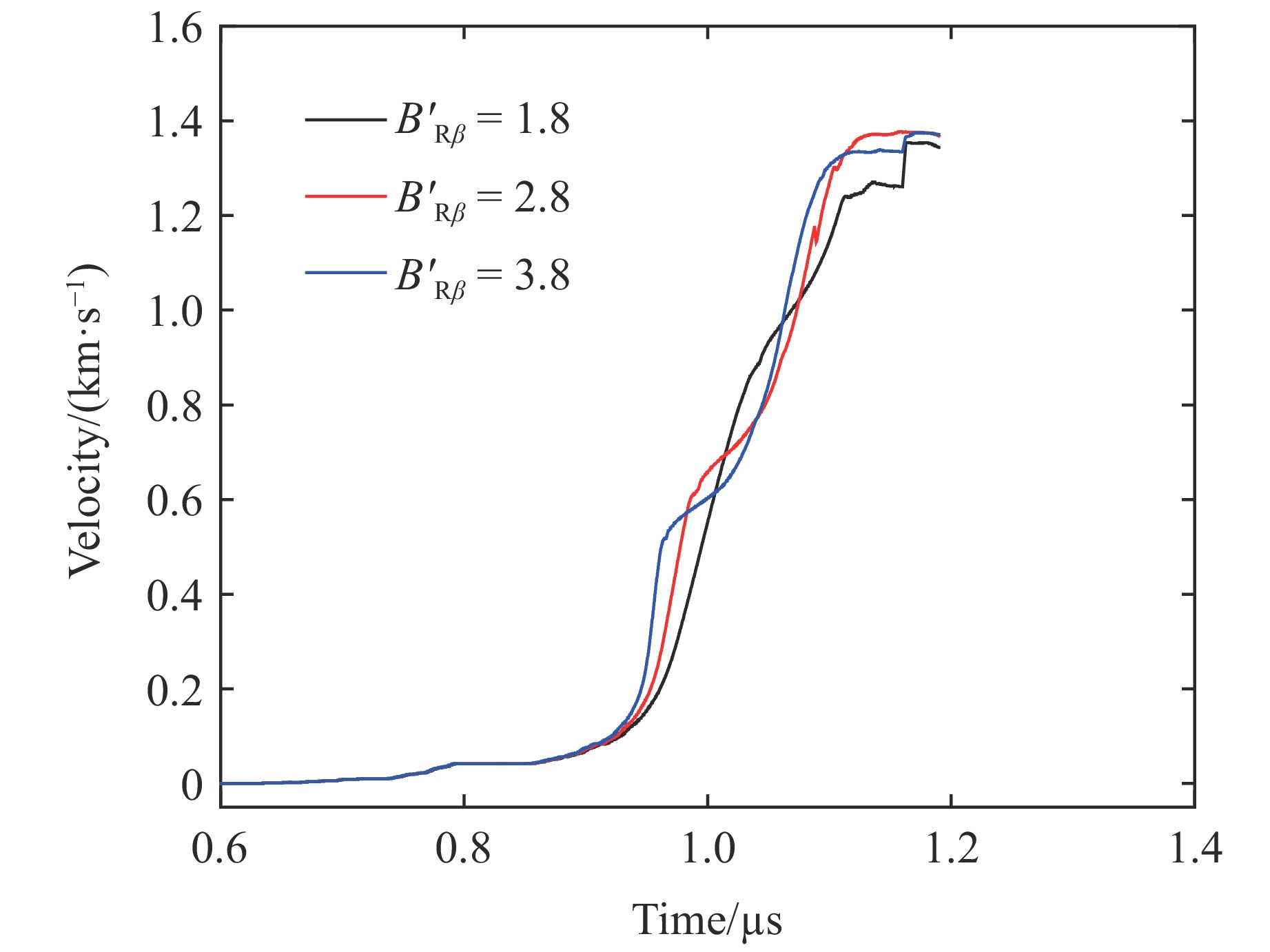
Cb=√B/ρ 可知,随着体模量的减小,声速减小,自由面速度起跳点推迟,弹塑性转变对应的速度平台被拉长。另外,在相同的比容和温度下,随着体模量的减小,压力减小,相变起始压力降低。不同BRβ值的速度波形见图8,其他动力学参数不变,计算得到的波形变化趋势和理论分析相同。参数B′Rβ 为β相的体模量对压力的一阶导数,它对体模量的影响虽然与压力有关,但在低压段表现不明显,随着压力的增加,影响越来越显著。图9为根据不同B′Rβ 数值计算得到的速度波形。由图9可知,在100 m/s内,相变速度几乎没有受到影响;随着加载压力的提高,B′Rβ 增大,初始相速度斜率明显提高,相变起始点对应的特征速度明显增加。BRγ和
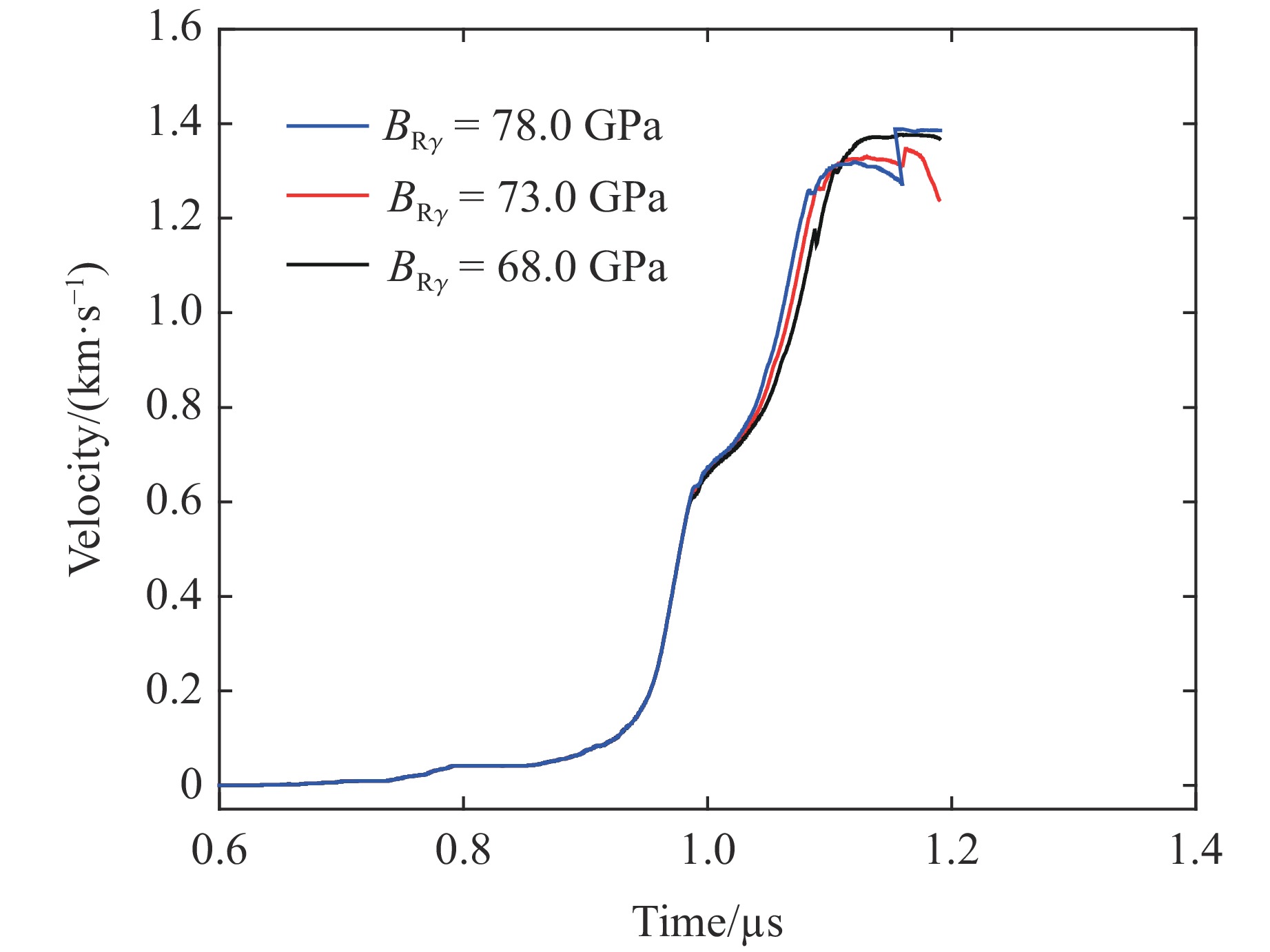
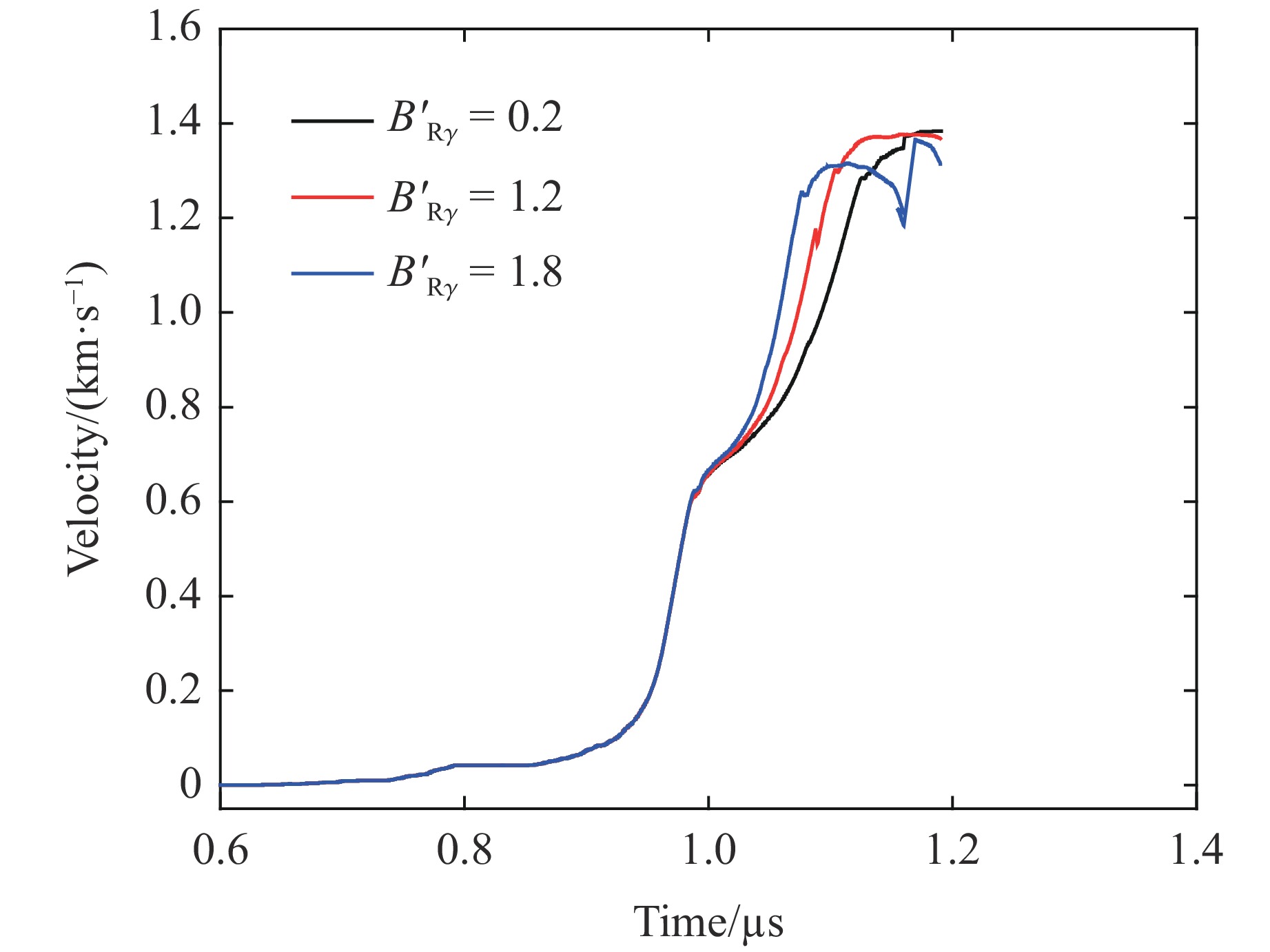
B′Rγ 分别为γ相的初始体模量和体模量对压力的一阶导数,与β相的参数相似,它们直接影响混合相和γ相对应的速度波形。不同BRγ和B′Rγ 值的速度波形如图10和图11所示。随着BRγ(或B′Rγ )的增加,相变前速度波形不变,相变开始后对应的速度斜率明显提高,与理论值吻合。4. 结 论
通过金属锡的斜波压缩实验,研究了锡的相变动力学特性,结合Hayes多相状态方程和非平衡相变动力学模型对锡的斜波压缩实验过程进行了模拟,得到以下结论。
(1)通过斜波压缩实验得到了不同厚度的锡样品后表面含有弹塑性转变和相变信息的自由面速度剖面;锡的β–γ相变对应的特征速度随着样品厚度的增加,从676.3 m/s到636.8 m/s略有减小,对应的压力从7.62 GPa降低到7.11 GPa。
(2)数值计算结果可以较好地描述锡在加载阶段的弹塑性转变和相变等物理过程。讨论了体模量在不同热力学过程中的物理形式,计算结果显示,斜波压缩过程需要考虑压力对体模量的修正。相变弛豫时间和结合能主要影响混合区部分速度波形,γ相的体模量参数只影响相变后的速度波形,而β相的体模量参数会影响整体速度波形。
感谢中国工程物理研究院流体物理研究所的吴刚、邓顺益、税荣杰、胥超和马骁在实验运行、维护和测速方面提供的帮助!
-
表 1 实验条件
Table 1. Experimental condition
Exp. No. Position Material Size/(mm × mm) Plate_top left 1100Al 8.0 × 1.006 Sample_top left Sn ∅8.0 × 1.278 Plate_top right 1100Al 8.0 × 1.006 Shot 696 Sample_top right Sn ∅8.0 × 1.568 Plate_bottom left 1100Al 8.0 × 0.998 Sample_bottom left Sn ∅8.0 × 1.871 Plate_bottom right 1100Al 8.0 × 0.996 表 2 速度波剖面上的特征值
Table 2. Characteristic values of the velocity profiles
Thickness of Sn/mm uEP/(m·s–1) uPT/(m·s–1) pPT/GPa 1.278 40.0 676.3 7.62 1.568 39.6 660.0 7.41 1.871 40.2 636.8 7.11 Phase vR/(cm3·g–1) TR/K pR/GPa Θ(vR)/K q1 BR/GPa B′R Φ0/(J·kg–1) Γ/(J·kg–1·K–2) α β 0.137 2 298.15 0 180.91 1.60 58.0 2.8 0 0.015 1.0 γ 0.119 8 298.15 8.664 187.77 1.38 78.1 0.2 85.3 × 103 0.015 1.0 -
[1] DUDLEY J D, HALL H T. Experimental fusion curves of indium and tin to 105 000 atmospheres [J]. Physical Review, 1960, 118(5): 1211–1216. doi: 10.1103/PhysRev.118.1211 [2] BARNETT J D, BEAN V E, HALL H T. X-ray diffraction studies on tin to 100 kilobars [J]. Journal of Applied Physics, 1966, 37(2): 875–877. doi: 10.1063/1.1708275 [3] DESGRENIERS S, VOHRA Y K, RUOFF A L. Tin at high pressure: an energy-dispersive X-ray-diffraction study to 120 GPa [J]. Physical Review B, 1989, 39(14): 10359–10361. doi: 10.1103/PhysRevB.39.10359 [4] LIU M, LIU L G. Compressions and phase transitions of tin to half a megabar [J]. High Temperatures-High Pressures, 1986, 18: 79–85. [5] SERVAS E M. Sound-velocity doppler laser interferometry measurements on tin [C]//Shock Compression of Condensed Matter-2001. Atlanta, GA(USA): American Institute of Physics, 2002, 620: 1200–1203. [6] CHEONG B H, CHANG K J. First-principles study of the structural properties of tin under pressure [J]. Physical Review B: Condensed Matter, 1991, 44(9): 4103–4108. doi: 10.1103/PhysRevB.44.4103 [7] CORKILL J L, GARCA A, COHEN M L. Erratum: Theoretical study of high-pressure phases of tin [J]. Physical Review B, 1991, 43(11): 9251–9254. doi: 10.1103/PhysRevB.43.9251 [8] CHRISTENSEN N E, METHFESSEL M. Density-functional calculations of the structural properties of tin under pressure [J]. Physical Review B, 1993, 48(9): 5797–5807. doi: 10.1103/PhysRevB.48.5797 [9] RAVELO R, BASKES M. Equilibrium and thermodynamic properties of grey, white, and liquid tin [J]. Physical Review Letters, 1997, 79(13): 2482–2485. doi: 10.1103/PhysRevLett.79.2482 [10] BERNARD S, MAILLET J B. First-principles calculation of the melting curve and hugoniot of tin [J]. Physical Review B, 2002, 66(1): 012103. doi: 10.1103/PhysRevB.66.012103 [11] AGUADO A. First-principles study of elastic properties and pressure-induced phase transitions of Sn: LDA versus GGA results [J]. Physical Review B: Condensed Matter and Materials Physics, 2003, 67(21): 212104. doi: 10.1103/PhysRevB.67.212104 [12] YU C, LIU J, LU H, et al. Ab initio calculation of the properties and pressure induced transition of Sn [J]. Solid State Communications, 2006, 140(11/12): 538–543. [13] CUI S X, CAI L C, FENG W X, et al. First-principles study of phase transition of tin and lead under high pressure [J]. Physica Status Solidi, 2008, 245(1): 53–57. doi: 10.1002/pssb.200743240 [14] TONKOV E Y, PONYATOVSKY E G. Phase transformations of elements under high pressure [M].Boca Raton, FL: CRC Press, 2005. [15] ANDERSON W W, CVERNA F, HIXSON R S, et al. Phase transition and spall behavior in β-tin [C]//AIP Conference Proceedings. American Institute of Physics, 2000: 443–446. [16] MABIRE C, HEREIL P L. Shock induced polymorphic transition and melting of tin [C]//Shock Compression of Condensed Matter-1999. New York: American Institute of Physics, 2000, 505(1): 93–96. [17] STAGER R A, BALCHAN A S, DRICKMER H G. High-pressure phase transition in metallic tin [J]. Journal of Chemical Physics, 1962, 37(5): 1154–1154. [18] STROMBERG H D, STEPHENS D R. Effects of pressure on the electrical resistance of certain metals [J]. Journal of Physics and Chemistry of Solids, 1964, 25(9): 1015–1022. doi: 10.1016/0022-3697(64)90039-3 [19] MARTIN J E, SMITH P L. Tin and indium antimonide at very high pressures [J]. British Journal of Applied Physics, 1965, 16(4): 495–500. doi: 10.1088/0508-3443/16/4/313 [20] VABOYA S N, KENNEDY G C. Compressibility of 18 metals to 45 kbar [J]. Journal of Physics and Chemistry of Solids, 1970, 31(10): 2329–2345. doi: 10.1016/0022-3697(70)90247-7 [21] OHTANI A, MIZUKAMI S, KATAYAMA M, et al. Multi-anvil apparatus for high pressure X-ray diffraction [J]. Japanese Journal of Applied Physics, 1977, 16(10): 1843–1848. doi: 10.1143/JJAP.16.1843 [22] MARSH S P. LASL shock hugoniot data [M]. Berkeley: University of California Press, 1980: 141. [23] CAVALERI M E, PLYMATE T G, STOUT J H. A pressure volume temperature equation of state for Sn (β) by energy dispersive X-ray diffraction in a heated diamond anvil cell [J]. Journal of Physics and Chemistry of Solids, 1988, 49(8): 945–956. doi: 10.1016/0022-3697(88)90012-1 [24] RAYNE J A, CHANDRASEKHAR B S. Elastic constants of β tin from 4.2 K to 300 K [J]. Physical Review, 1960, 120(6): 1658–1663. [25] KAMIOKA H. Temperature variations of elastic moduli up to eutectic temperature in tin-bismuth alloys [J]. Japanese Journal of Applied Physics, 1983, 22(12): 1805–1809. [26] HU J B, ZHOU X M, DAI C D, et al. Shock-induced bct-bcc transition and melting of tin identified by sound velocity measurements [J]. Journal of Applied Physics, 2008, 104(8): 083520. doi: 10.1063/1.3003325 [27] SONG H F, LIU H F, ZHANG G C, et al. Numerical simulation of wave propagation and phase transition of tin under shock-wave loading [J]. Chinese Physics Letters, 2009, 26(6): 066401. doi: 10.1088/0256-307X/26/6/066401 [28] KIEFER B, DUFFY T, UCHIDA T, et al. Melting of tin at high pressures [R]. APS User Activity Report, 2002. [29] DAVIS J, HAYES D B. Isentropic compression experiments on dynamic solidification in tin [J]. Journal of Membrane Science, 2004, 476(1): 20–29. [30] HAYES D B. Wave propagation in a condensed medium with N transforming phases: application to solid-I-solid-Ⅱ-liquid bismuth [J]. Journal of Applied Physics, 1975, 46(8): 3438–3443. doi: 10.1063/1.322065 [31] COX G A. A Multi-phase equation of state and strength model for tin [C]//Shock Compression of Condensed Matter-2005. Baltimore, Maryland (USA) : American Institute of Physics, 2006, 845(1): 208–211. [32] BUY F, VOLTZ C, LLORCA F. Thermodynamically based equation of state for shock wave studies: application to the design of experiments on tin [C]//Shock Compression of Condensed Matter-2005. Baltimore, Maryland (USA): American Institute of Physics, 2006, 845(1): 41–44. [33] KHISHCHENKO K V. Equation of state and phase diagram of tin at high pressures [J]. Journal of Physics: Conference Series, 2008, 121(2): 022025. doi: 10.1088/1742-6596/121/2/022025 [34] 张林, 李英华, 李雪梅, 等. 锡的β和γ两相物态方程 [C]//第六届全国爆炸力学实验技术学术会议, 2010: 301–307. [35] 种涛, 王桂吉, 谭福利, 等. 磁驱动准等熵压缩下铁的相变 [J]. 中国科学:物理学 力学 天文学, 2014, 44(6): 630–636.CHONG T, WANG G J, TAN F L, et al. Phase transition of iron under magnetically driven quasi-isentropic compression [J]. Scientia Sinica: Physica, Mechanica & Astronomica, 2014, 44(6): 630–636. [36] WANG G J, LUO B Q, ZHANG X P, et al. A 4 MA, 500 ns pulsed power generator CQ-4 for characterization of material behaviors under ramp wave loading [J]. Review of Scientific Instruments, 2013, 84(1): 015117. doi: 10.1063/1.4788935 [37] HALL C A, ASAY J R, KNUGSON M D, et al. Experimental configuration for isentropic compression of solids using pulsed magnetic loading [J]. Review of Scientific Instruments, 2001, 72(9): 3587–3595. doi: 10.1063/1.1394178 [38] STEINBERG D J. Equation of state and strength properties of selected materials [R]. Livermore, California: Lawrence Livermore National Laboratory, 1996. -








 下载:
下载:
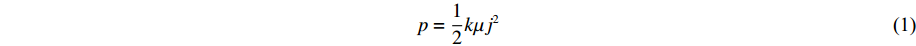

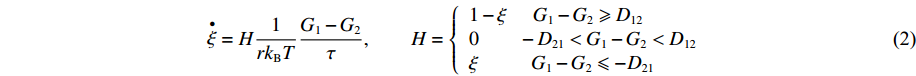
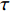
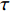
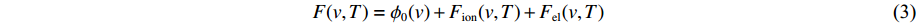

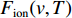
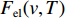

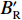
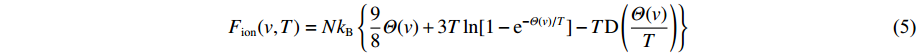

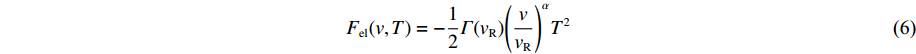
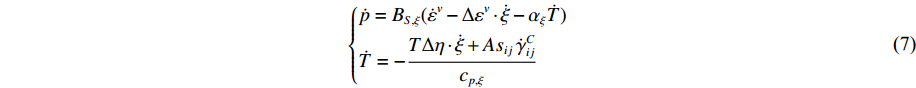
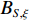

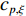
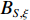
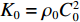
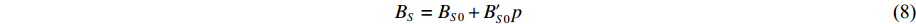
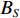

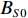
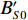
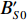
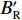
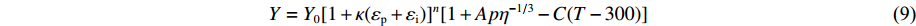
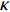
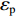
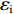

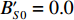
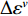
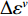
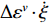


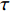
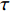





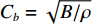
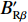
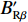
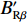


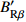
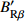
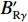
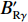
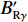


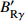
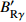

 下载:
下载: