Numerical Simulation on Interlaminar Fracture Toughness of 3D Printed Mortar Laminated Composites
-
摘要: 通过有限元数值模拟研究了3D打印浆砌层合结构复合材料的层间断裂韧性。首先建立了基于内聚力原理和位移控制加载法的I型和II型断裂韧性有限元模型,模拟复合材料层间张开和错开的过程,随后通过有限元数值模拟与模型试验对比分析,验证了有限元数值方法的可靠性,最后分析了复合材料初始裂纹长度、断裂韧性、起始界面刚度、界面强度、黏结层厚度以及净距等参数对3D打印浆砌层合结构复合材料层间力学性能的影响。研究结果表明:对I型模型,减小初始裂纹长度、增大断裂韧性和增大黏结层厚度均能提高层间承载能力,起始界面刚度和界面强度的改变对拉伸力峰值无明显变化;对II型模型,减小初始裂纹长度、增强界面强度、增大断裂韧性和减小黏结层厚度均能提高层间承载能力,起始界面刚度的改变对荷载-位移曲线无明显影响。Abstract: In this paper, the interlaminar fracture toughness of 3D printed mortar laminated composite was investigated by finite element numerical simulation. Firstly, finite element models of the model-I and model-II fracture toughness were established based on cohesive principle and displacement control loading method, and used to simulate the interlaminar opening and staggering process of composites. Then the reliability of the finite element numerical method was verified by compared with the experiment results. Finally, the effects of initial crack length, fracture toughness, initial interface stiffness, interface strength, bonding layer thickness and clear distance on the mechanical properties of 3D printed mortar laminated composite were analyzed. The results show that, for the model-I, reducing the initial crack length, increasing the fracture toughness and increasing the bonding layer thickness can improve interface bearing capacity; and the change of initial interface stiffness and interface strength has no effect on the peak value of tensile force. For the model-II, reducing the initial crack length, enhancing the interface strength, increasing the fracture toughness value and reducing the bonding layer thickness can improve the interface bearing capacity; and the change of the initial interface stiffness has no significant effect on the load-displacement curve.
-
高性能轻质复合材料越来越多地应用于工程材料领域,尤其是贝壳珍珠层的应用最广泛。贝壳珍珠层的最大优点是高强度和高抗破裂韧性,这些优越性能得益于其独特的多层次微结构。珍珠层由体积分数为95%的文石碳酸钙和5%的有机质组成,两者交叉堆叠成有序的层状结构[1],即浆砌层合结构,其中有机质的桥连作用以及诱导裂纹偏转作用强化了材料的韧性[2-3]。根据贝壳这种浆砌层合结构,可制备具有硬材料基体和黏结层的复合材料。其多层次微结构如图1[4]所示。
鉴于贝壳珍珠层这种浆砌层合结构的诸多优点,随之出现了很多浆砌结构材料制造技术,其中3D打印技术作为新兴的制造技术,被越来越多地用在工业生产和人们的生活中,如航空航天中的机翼、生物材料中的牙齿等,同时也使仿生浆砌层合结构复合材料的制备更方便。马骁勇等[5]研究发现,三维打印贝壳仿生结构断裂发生在类橡胶软材料内部,因此浆砌层合结构内部层间的分层与脱黏对整体结构力学性能有着重要影响。而浆砌层合结构的软硬交替界面的力学性能可通过分析复合材料的层间断裂韧性来研究,为此本工作着眼于3D打印复合材料层间断裂韧性。
近些年来,针对复合材料层间断裂韧性的有限元计算模型发展迅速。Xu等[6]的内聚力模型发展较完善,且具有自身的理论原理,广泛应用于复合材料断裂与分层破坏研究中。其中,许多学者将内聚力模型引入标准复合材料层间扩展试验模型中,如Hosseini等[7]基于内聚力模型研究了编织玻璃环氧基复合材料的断裂韧性,Hua等[8]借助内聚力模型研究了玻璃钢铝层合板的非对称双悬臂梁,宗要武[9]基于内聚力模型开展了钢纤维水泥基材料的界面性能分析。众多学者还将内聚力模型引入冲击载荷下复合材料分层损伤的有限元模拟中,较好地反映了复合材料结构分层损伤破坏的全过程。如Alfaro等[10]基于内聚力模型研究了三维层合板的断裂和分层,Liu等[11]基于内聚力模型研究了层合板动态双悬臂梁,赵丽滨等[12]总结了纤维增强复合材料层合板分层行为研究进展以及内聚力方法。在上述模型基础上,本研究在内聚力模型中考虑了3D打印浆砌层合结构的裂纹萌生和扩展行为,并分析了模型参数的影响。
基于此,针对3D打印浆砌层合结构复合材料的I型和II型断裂韧性进行有限元模拟,通过有限元数值模拟与模型试验的对比分析,验证有限元模拟的正确性,随后考察初始裂纹长度、断裂韧性、起始界面刚度、界面强度、黏结层厚度以及净距对3D打印浆砌层合结构复合材料层间力学性能的影响。
1. 层间断裂韧性的有限元模拟
1.1 内聚力本构模型及损伤判据
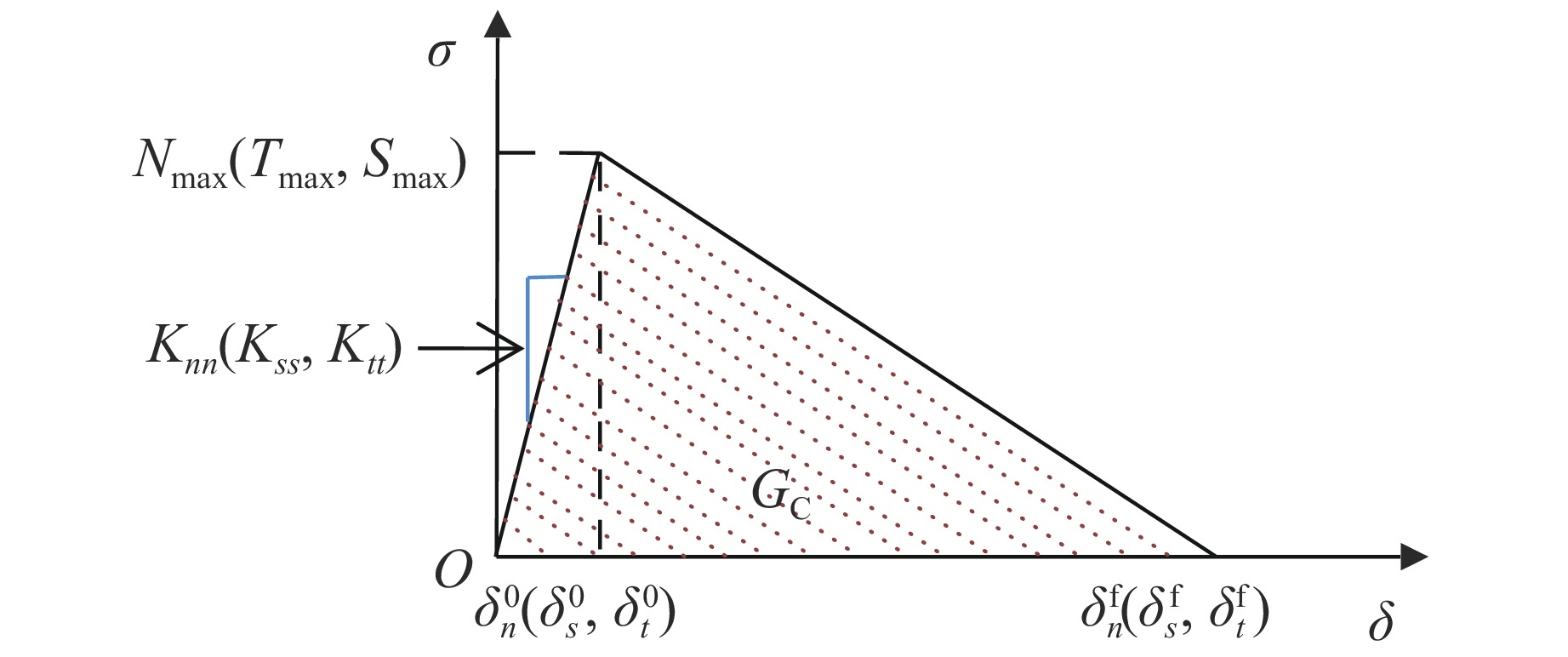
采用双线性拉伸分离准则来模拟黏结层接触面的黏性力学行为,其本构关系如图2所示。
用内聚力单元来模拟材料的损伤破坏过程,分为两个阶段:损伤起始和损伤演化[13]。
针对损伤起始,采用二次应力准则
(⟨σn⟩Nmax)2+(σsSmax)2+(σtTmax)2=1 (1) 式中:n是单元法向,对应于I型断裂;s、t是单元的两个切线方向,对应于II型和III型断裂;
σn 、σs 、σt 分别为法向和两个切向的应力,Nmax 、Smax 、Tmax 分别为法向和两个切向的峰值强度。针对损伤演化,采用基于能量的损伤演化BK准则
GC=GIC+(GIIC−GIC)(GshearGT)η (2) Gshear=GII+GIII (3) GT=GI+Gshear (4) 式中:
GIC 、GIIC 分别为法向和II型切向的断裂韧性值,GI 、GII 、GIII 分别为法向和两个切向的能量释放率,η为材料常数。损伤起始后会有应力的变化,需要引入刚度弱化系数D
D=δfm(δmaxm−δ0m)δmaxm(δfm−δ0m) (5) δ0m=√⟨δn⟩2+δ2s+δ2t (6) δfm=2GC√⟨σn⟩2+σ2s+σ2t (7) 式中:
δ0m 为破坏起始时单元节点的张开量,δfm 为破坏演化时节点的张开量,δmaxm 为在荷载历程中节点的最大张开量。1.2 I型和II型断裂韧性有限元数值模型
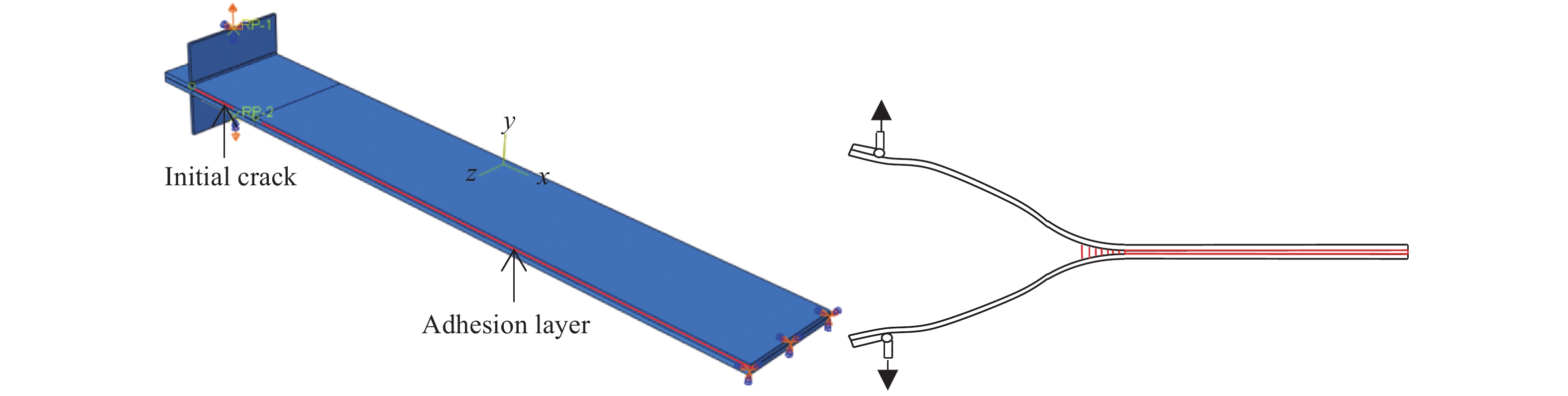
采用ABAQUS进行I型材料断裂韧性的有限元数值模拟,如图3所示。数值模拟选用三维建模,长240 mm,宽37 mm,初始裂纹长32 mm。采用动态模拟准静态,进行复合材料裂纹张开过程模拟,边界条件:保持试样右端固定,在距试样左端14 mm处绑定一个铁片,施加竖向位移荷载,在铁片控制点处实现材料的张开。黏结层采用内聚力单元和扫略网格描述,且布置局部边界种子,尺寸为0.5 mm。
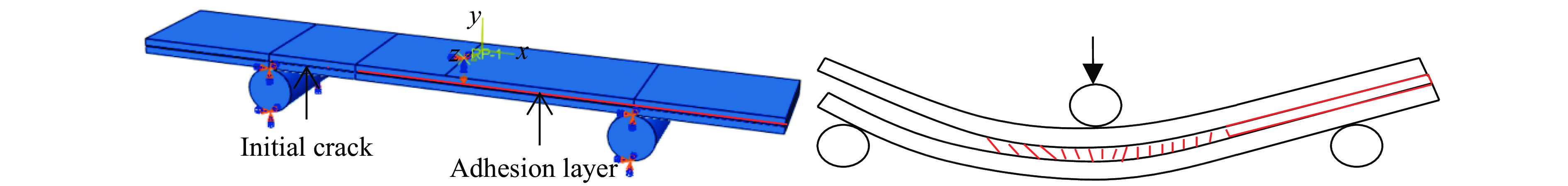
采用ABAQUS进行II型材料断裂韧性的有限元数值模拟,如图4所示。数值模拟采用三维模型,长140 mm,宽25 mm,净距80 mm,初始裂纹长20 mm。采用动态模拟准静态,进行复合材料裂纹错开过程模拟,试样上表面中心线与参考点绑定,其边界条件:保持两个支座固定,通过对参考点施加竖向位移荷载实现材料的错开。黏结层采用内聚力单元和扫略网格描述,且布置局部边界种子,尺寸为0.5 mm。考虑到支座处和初始裂纹处上下表面的接触,采用了通用接触。
通过对实验数据结果的分析,确定了内聚力模型层间断裂韧性和节点张开量。由图2的本构关系可知,层间断裂韧性为图形与坐标轴围成的面积,进而确定界面强度,初始斜率为起始界面刚度。具体参数如表1所示,其中T为黏结层厚度,Knn和Kss为I型和II型模型的刚度参数。
表 1 内聚力参数Table 1. Cohesive parametersT/mm Nmax/ MPa Smax/ MPa GI/(N∙mm−1) GII/(N∙mm−1) Knn/(N∙mm−3) Kss/(N∙mm−3) 0.16 0.35 2.0 0.40 0.73 15 625 31 250 0.20 0.50 2.5 0.55 0.65 12 500 25 000 1.3 数值模拟与实验结果对比验证
为了验证有限元计算结果的正确性,进行了I型和II型断裂韧性的模型试验。试样由Objet 260 Connex 3光固化三维打印机制备。通过SolidWorks软件设计模型并导入三维打印机程序中,然后利用双喷头3D打印机将模型打印成最终试样。试样尺寸与有限元模型尺寸一致,试样上下两层硬材料采用不透明灰色树脂材料VeroGray,通过准静态实验,得到其弹性模量和泊松比分别为1 250 MPa和0.3;中间黏结层采用类橡胶高分子材料TangoBlack,通过准静态实验,得到其弹性模量和泊松比分别为0.4 MPa和0.33。采用ASTM D5528-01[14]标准进行I型断裂韧性试验,如图5(a)所示,通过试样拉伸实现裂纹张开,得到荷载-位移曲线;采用NASA/TM-2010-216838[15]标准进行II型断裂韧性试验,如图5(b)所示,通过试样弯曲实现层间裂纹错开,得到荷载-位移曲线。
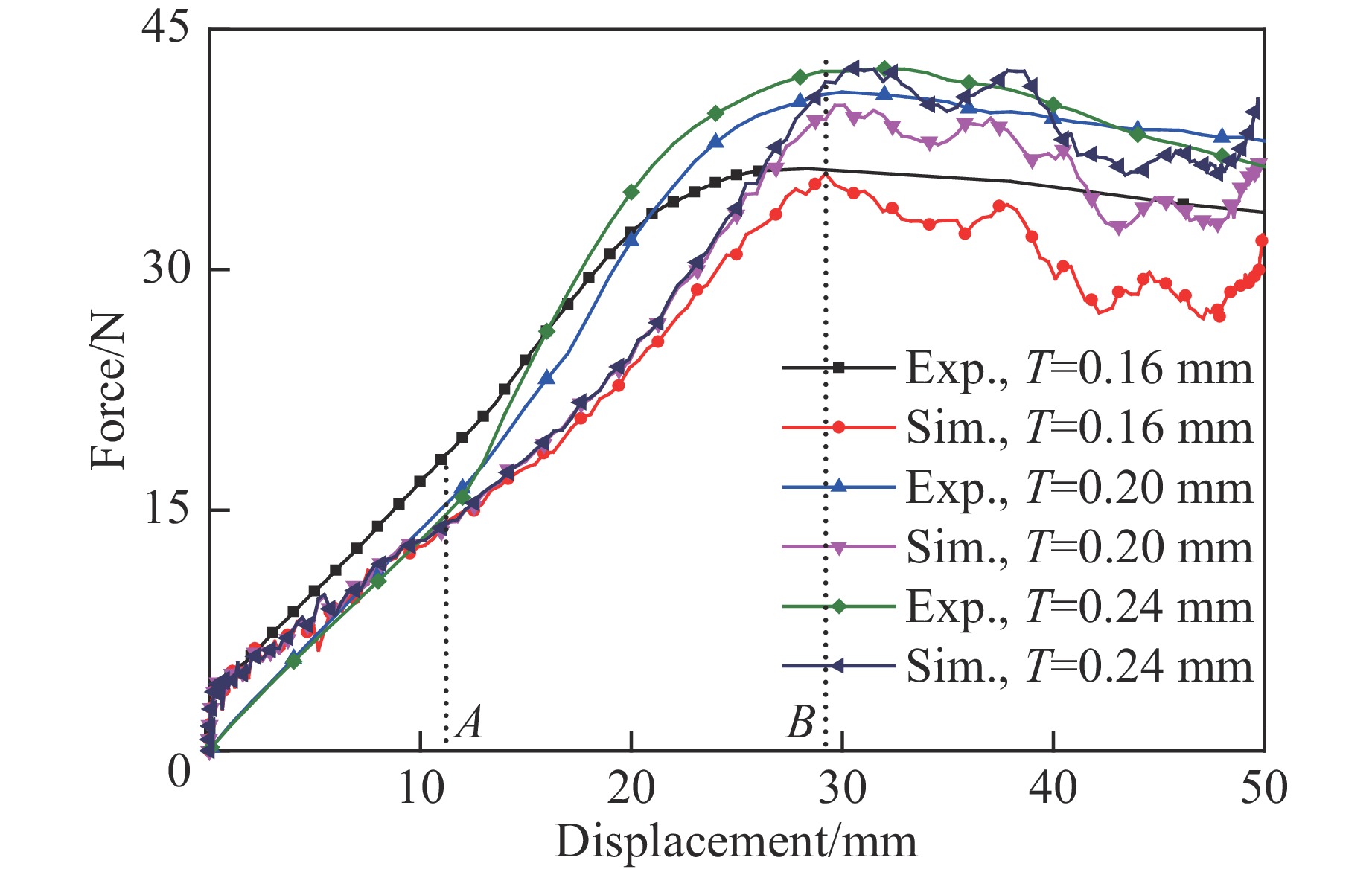
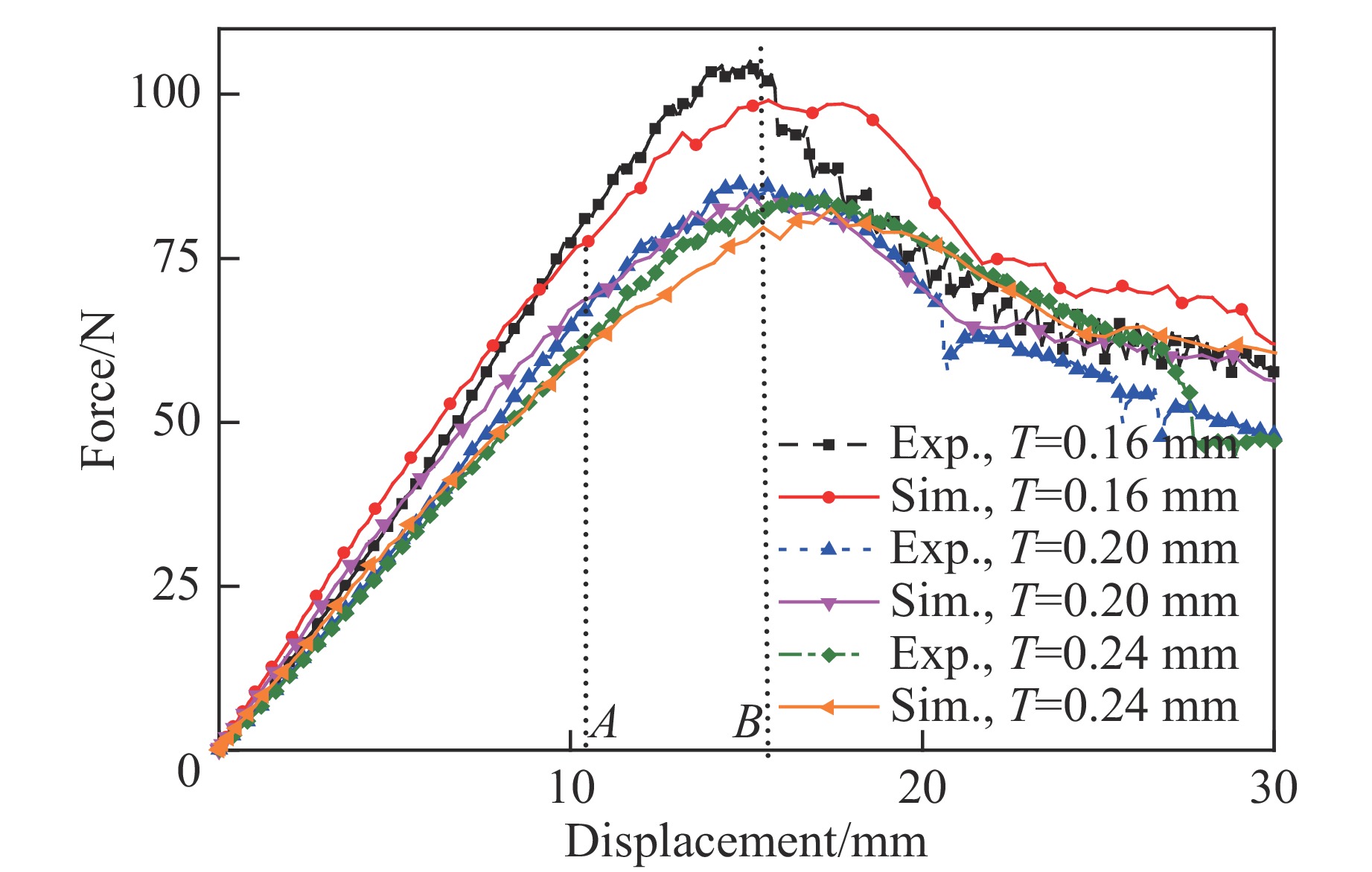
DCB和ENF试验与模拟结果的对比分别如图6和图7所示,其中A点和B点分别代表黏结层局部损伤起始点和损伤演化点[16-17]。由图6和图7可知,不同黏结层厚度的数值模拟结果与试验测量所得荷载-位移曲线具有相同的变化趋势。损伤起始点和损伤演化点的数值模拟结果与试验实测值吻合较好,从而验证了数值建模过程的可行性及模拟结果的可靠性。因此,本工作采用该建模方法进行后续数值模拟研究。
对于I型试样,以黏结层厚度为0.20 mm的工况为例进行分析,如图6所示。由图6可知,荷载-位移曲线的数值模拟结果与实测值具有相同的变化趋势,且能较好地预测局部损伤起始点和损伤演化点,但因存在损伤致使试样实际变形大于数值模拟结果,所以数值模拟所得拉伸力比实测值略小。
对于II型试样,以黏结层厚度为0.16 mm的工况为例进行分析,如图7所示。可见,荷载-位移曲线的数值模拟结果与试验模型具有相同的变化趋势,且能较好地预测损伤起始点。损伤演化点的荷载变小,这是由于试验过程中试样产生微弱错动,一定程度上延缓了裂纹的损伤,增大了试样的弯曲力。
2. 数值模拟结果分析
2.1 I型数值模拟结果分析
针对中间黏结层厚度为0.20 mm的I型模拟结果进行初始裂纹长度、断裂韧性、起始界面刚度与界面强度的研究分析,并讨论黏结层厚度的影响。
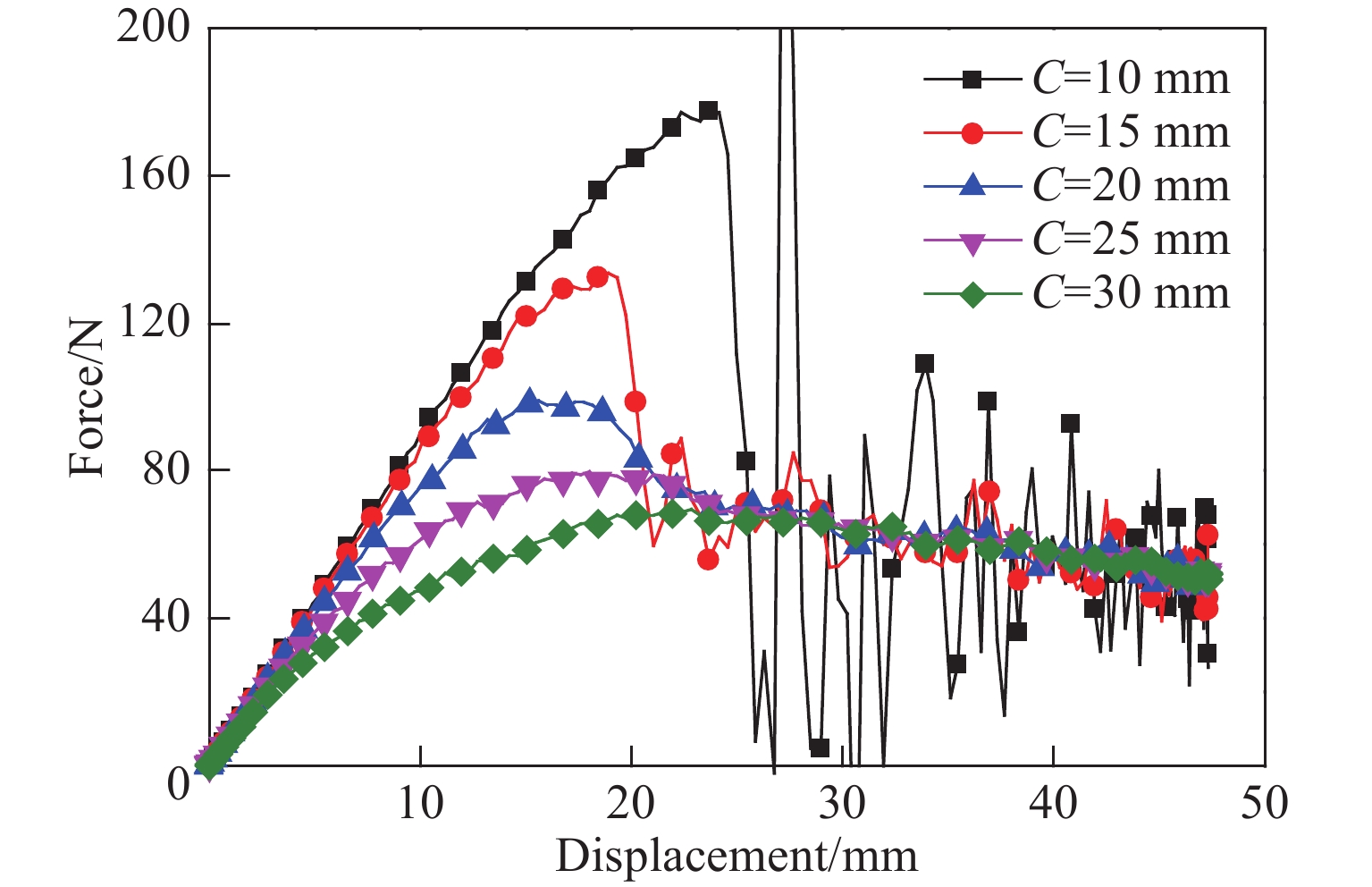
2.1.1 初始裂纹长度的影响
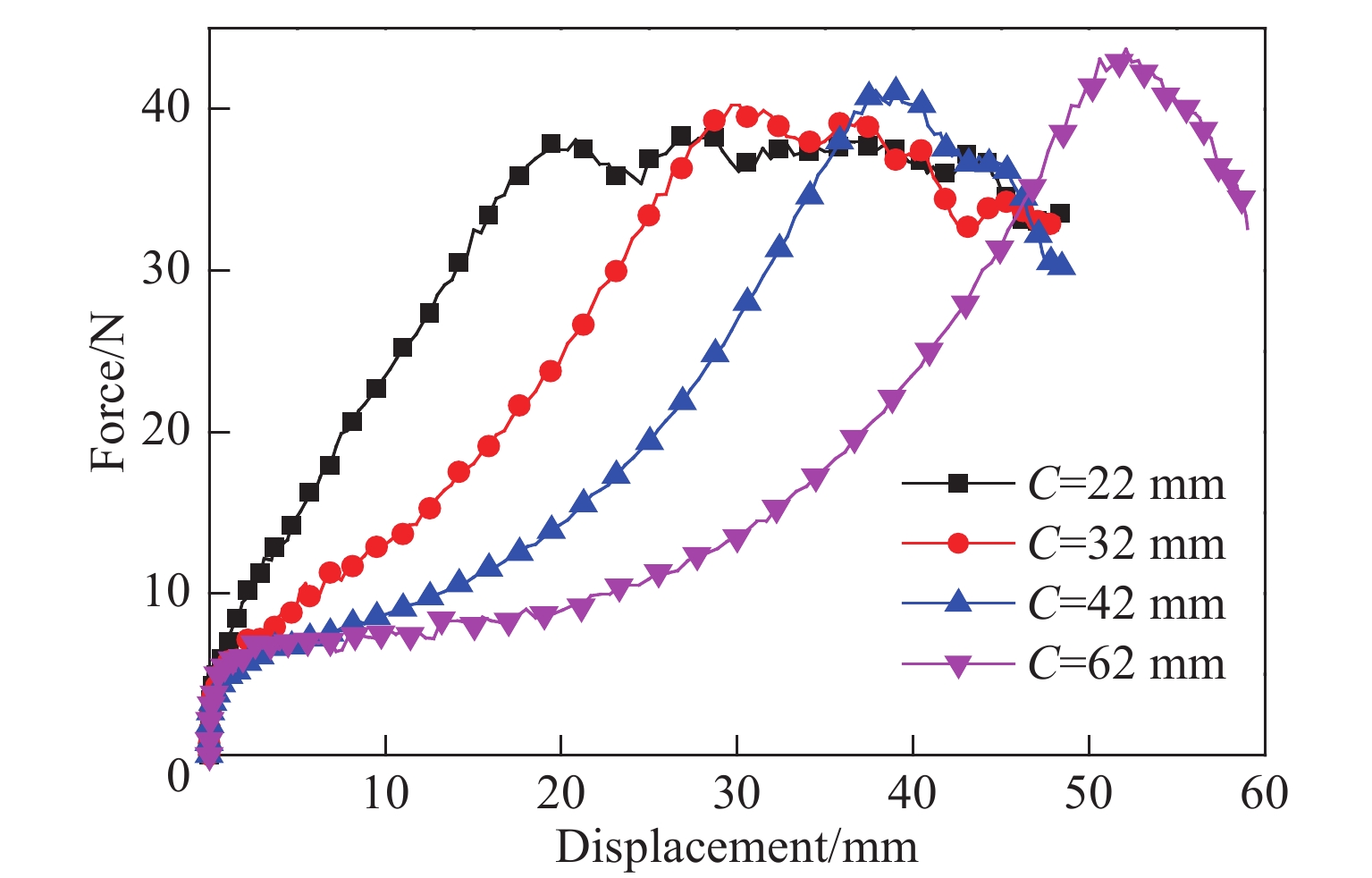
不同初始裂纹长度C的荷载-位移曲线如图8所示。由图8可知,I型试样荷载峰值所对应的位移随初始裂纹长度的增大而增大,拉伸力峰值略有增大。由修正梁理论[14]公式推断可知:当断裂韧性不变时,失效裂纹长度增大,在拉伸力峰值增幅不大的情况下,荷载峰值所对应的位移会增大。
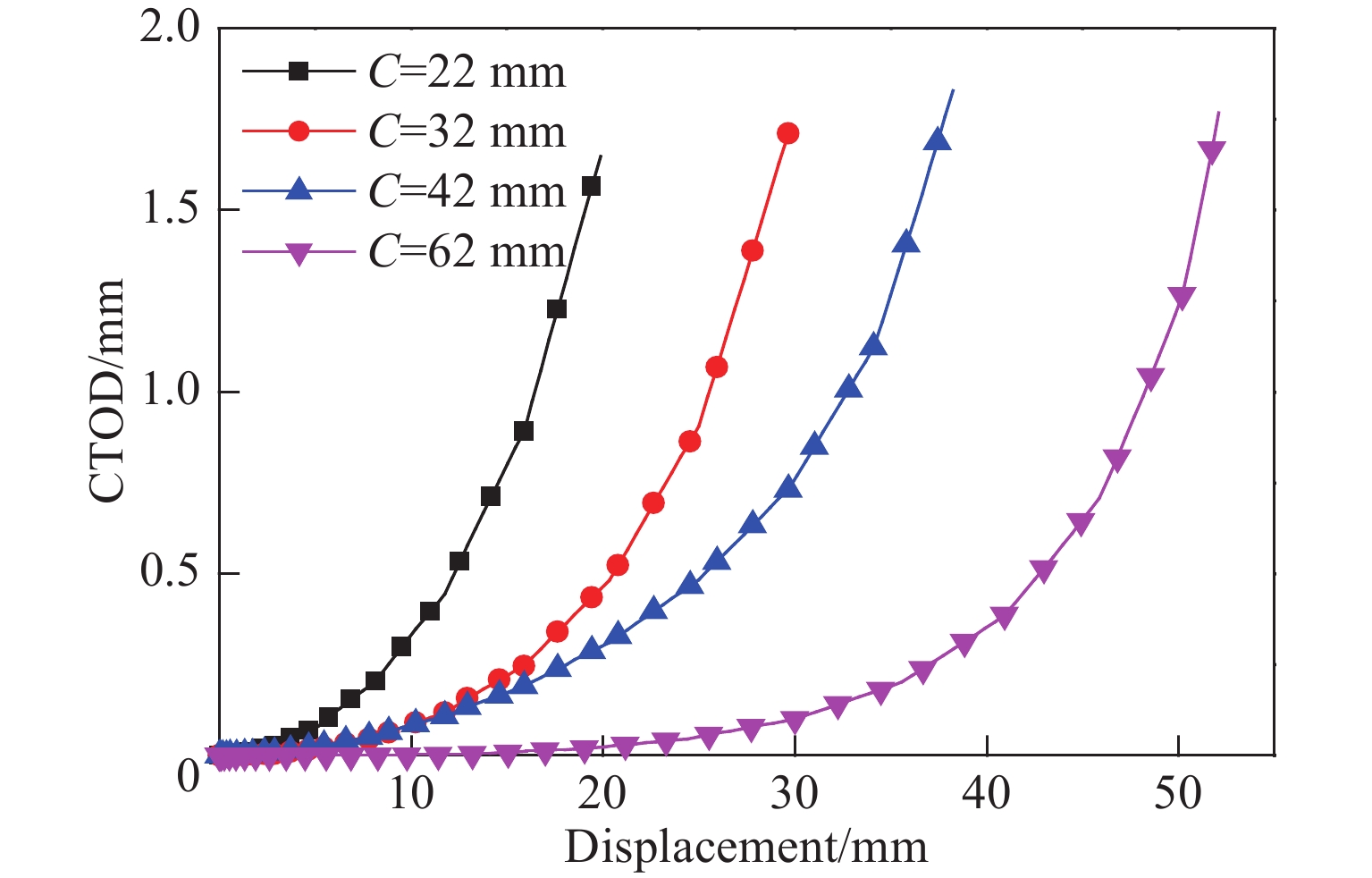
此外,在局部损伤起始点之前,荷载增长速率随初始裂纹长度的增大而减小。如图9所示,在损伤演化点之前,达到损伤演化点的裂纹尖端开口位移(CTOD)相同,裂纹长度增大182%,即由22 mm增大到62 mm;拉伸位移增大160%,即由20 mm增大到52 mm,表明试样的裂纹拉伸张开呈非线性,这是由试样弯曲变形和尖端黏结层阻碍共同导致。在损伤演化后,载荷下降速度随初始裂纹长度的增大而加快,表明初始裂纹长度越小,材料的韧性越强,材料不会瞬时失去承载力。
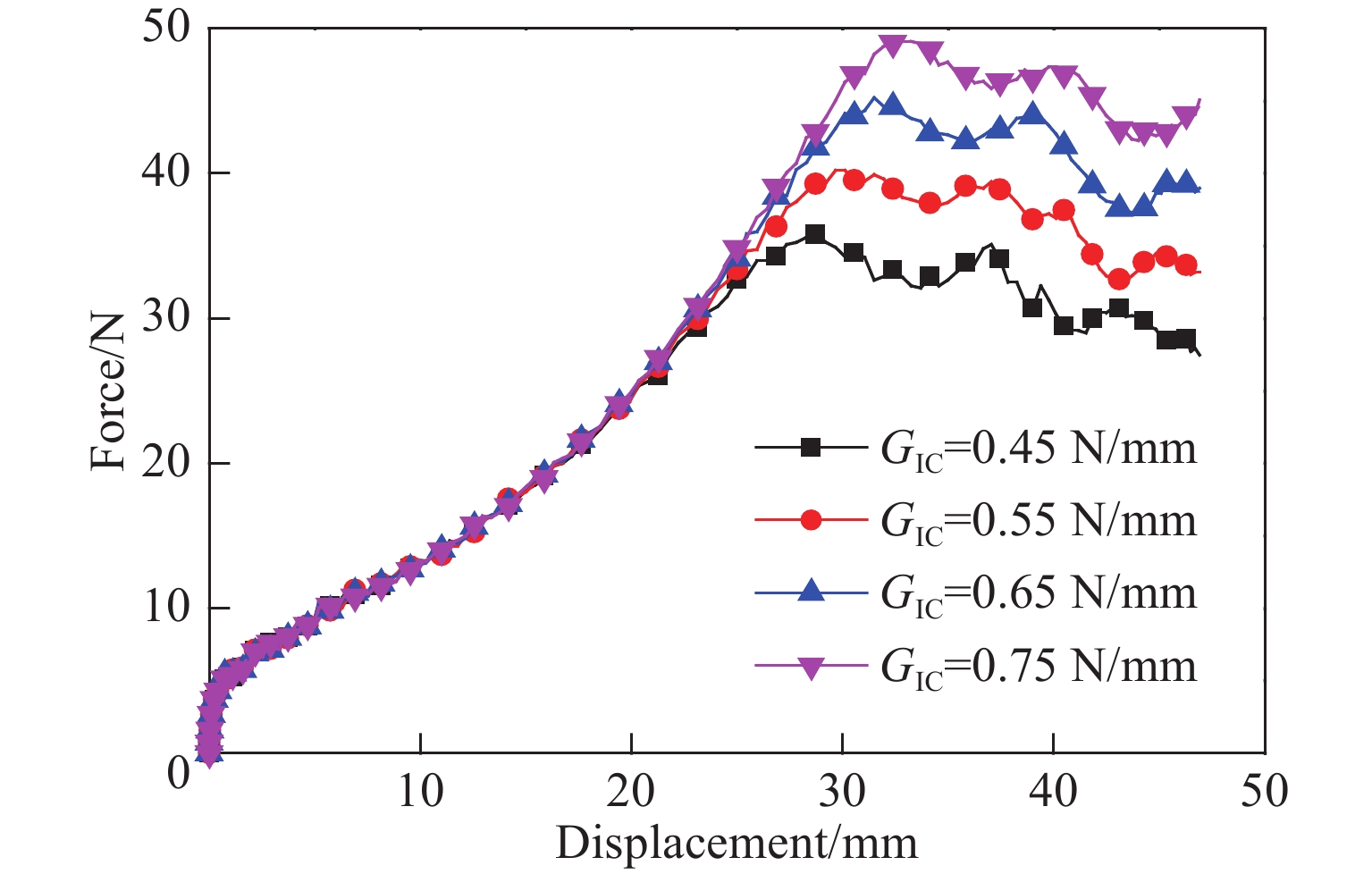
2.1.2 断裂韧性的影响
不同断裂韧性的荷载-位移曲线如图10所示。由图10可知,拉伸力峰值随断裂韧性的增强而增大。这是由于断裂韧性增强,层间抵抗破坏能力增大,因此需要更大的拉伸力峰值使材料破坏。保持相同的断裂韧性增加值0.1 N/mm,则拉伸力峰值的增加幅度为4 N,说明断裂韧性对I型试样层间破坏具有显著影响。
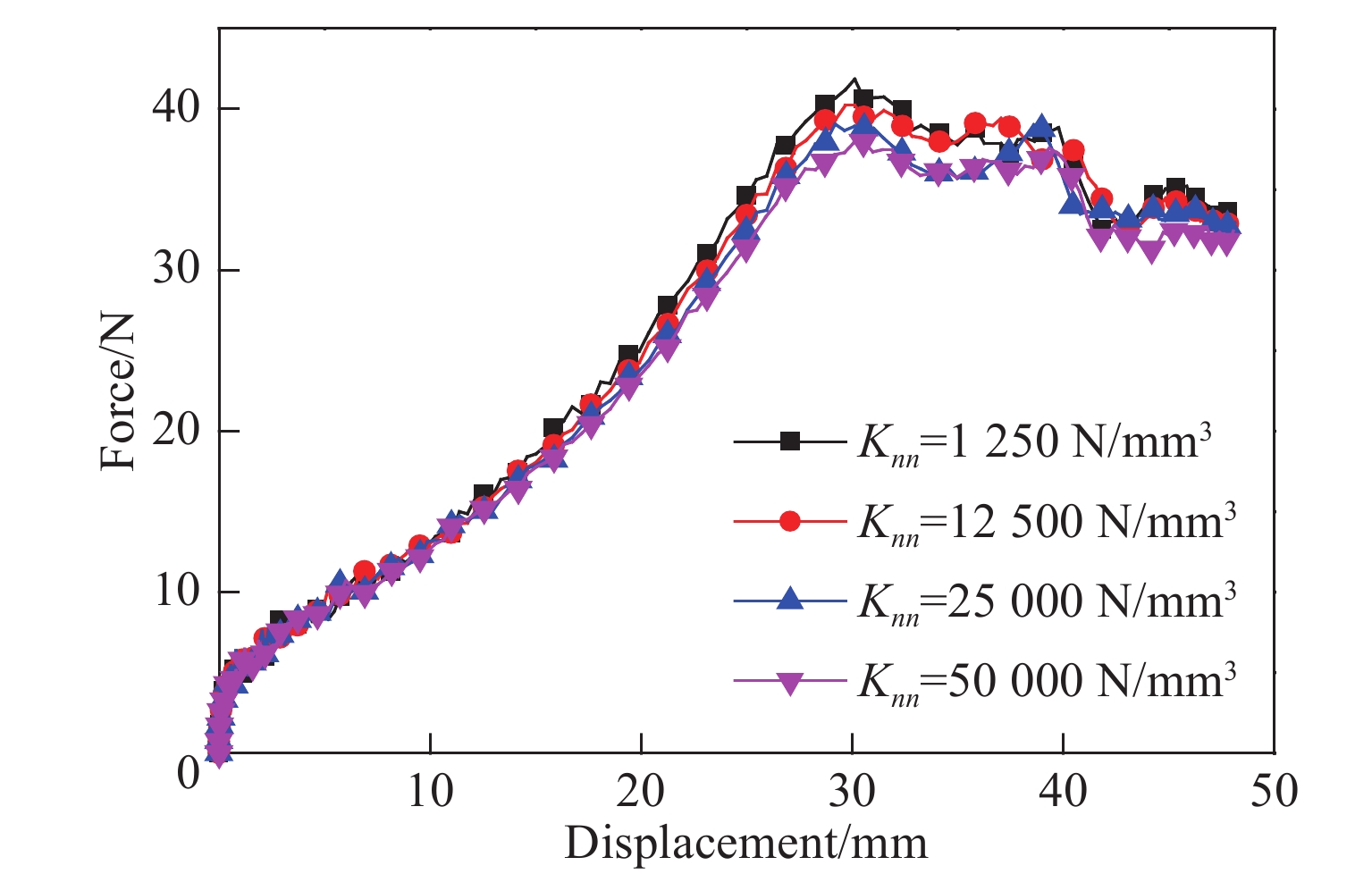
2.1.3 起始界面刚度的影响
不同起始界面刚度的荷载-位移曲线如图11所示,Knn = 12 500 N/mm3为模拟试样黏结层厚度为0.20 mm时的刚度参数值。由图11可知,拉伸力峰值随起始界面刚度的增大而略有下降。这是由于起始界面刚度的改变仅影响破坏起始时的单元节点张开量,不会影响整体材料的破坏能。因此刚度参数值在一定范围内变化时,对荷载-位移曲线的影响并不显著。
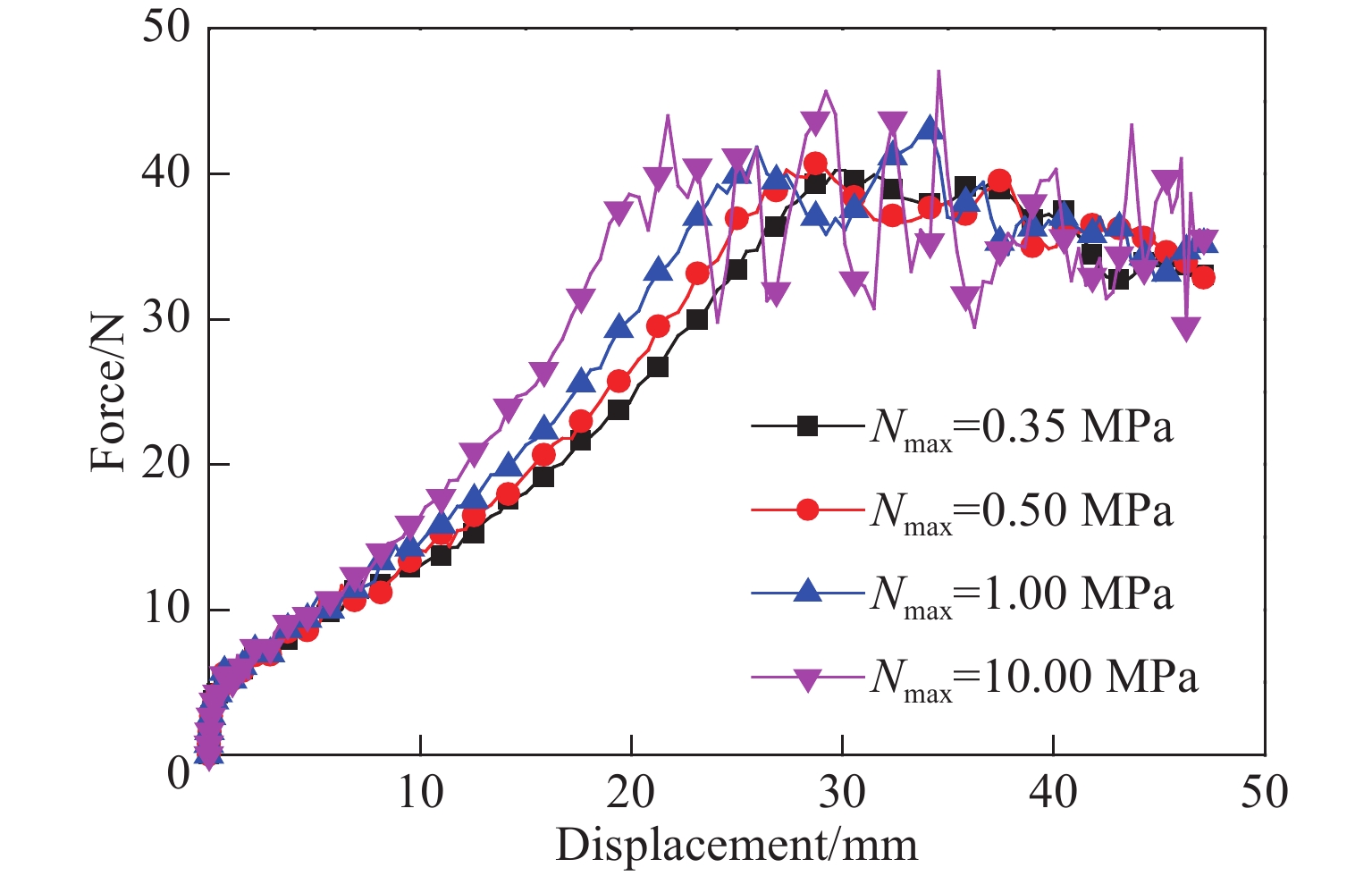
2.1.4 界面强度的影响
不同界面强度的荷载-位移曲线如图12所示。由图12可知,拉伸力峰值随界面强度的增加无明显变化,而荷载峰值所对应的位移减小。这是由于界面强度越大,损伤演化时的节点错开量越小,导致试样破坏时张开位移变小,荷载峰值所对应的位移减小。
2.1.5 黏结层厚度的影响
不同黏结层厚度的荷载-位移曲线如图6所示。由图6可知,拉伸力峰值随着黏结层厚度的增加而增加,且达到拉伸力峰值对应的位移增大。保持相同的黏结层厚度增加值0.04 mm,则拉伸力峰值增量减小,由4.72 N降为1.51 N。当黏结层厚度增加到一定程度后,拉伸力峰值将不会产生明显的增长。
2.2 II型数值模拟结果分析
针对中间黏结层厚度为0.16 mm的II型模拟结果进行初始裂纹长度、断裂韧性、起始界面刚度、界面强度与净距的研究分析,并讨论黏结层厚度的影响。
2.2.1 初始裂纹长度的影响
不同初始裂纹长度C的荷载-位移曲线如图13所示。由图13可知,II型试样的弯曲力峰值随初始裂纹长度的增大而减小。初始裂纹长度越小,起始刚度越大,达到弯曲力峰值后,黏结层产生破坏并引起弯曲力下降,且下降幅度较大。这是由于黏结层剪切力增大,层间损伤抵抗力增强,导致弯曲力峰值增大。当试样处于损伤演化后期,瞬时黏性层破坏长度较大,导致荷载骤然下降,曲线出现剧烈抖动。同样表明初始裂纹长度越小,材料的韧性越强,越不容易破坏,但材料破坏后承载力降幅很大。
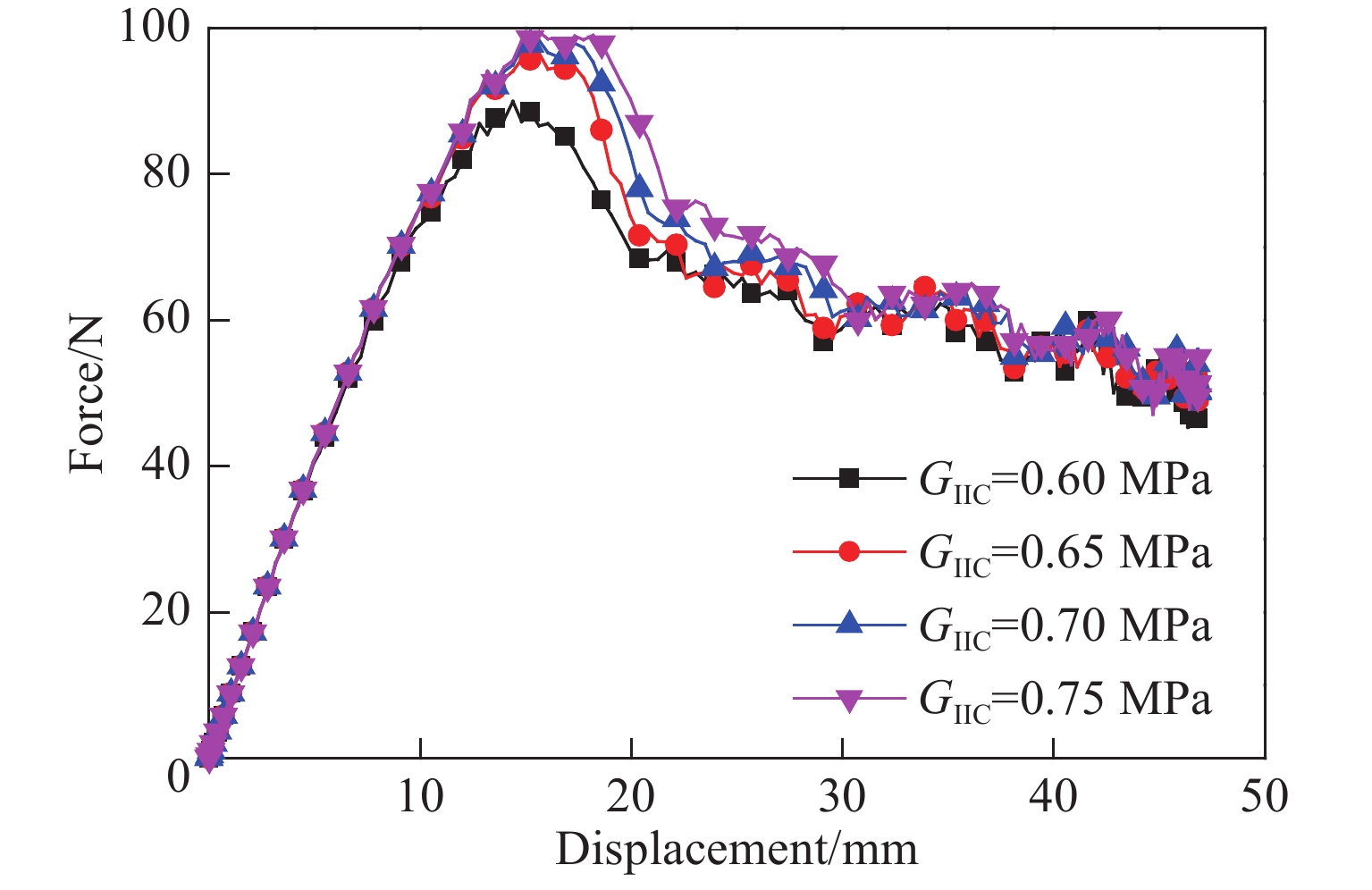
2.2.2 断裂韧性的影响
不同断裂韧性的荷载-位移曲线如图14所示。由图14可知,弯曲力峰值随断裂韧性的增加而增大。这是由于黏结层抵抗破坏的能力随断裂韧性的增强而增强,试样越不容易发生破坏。
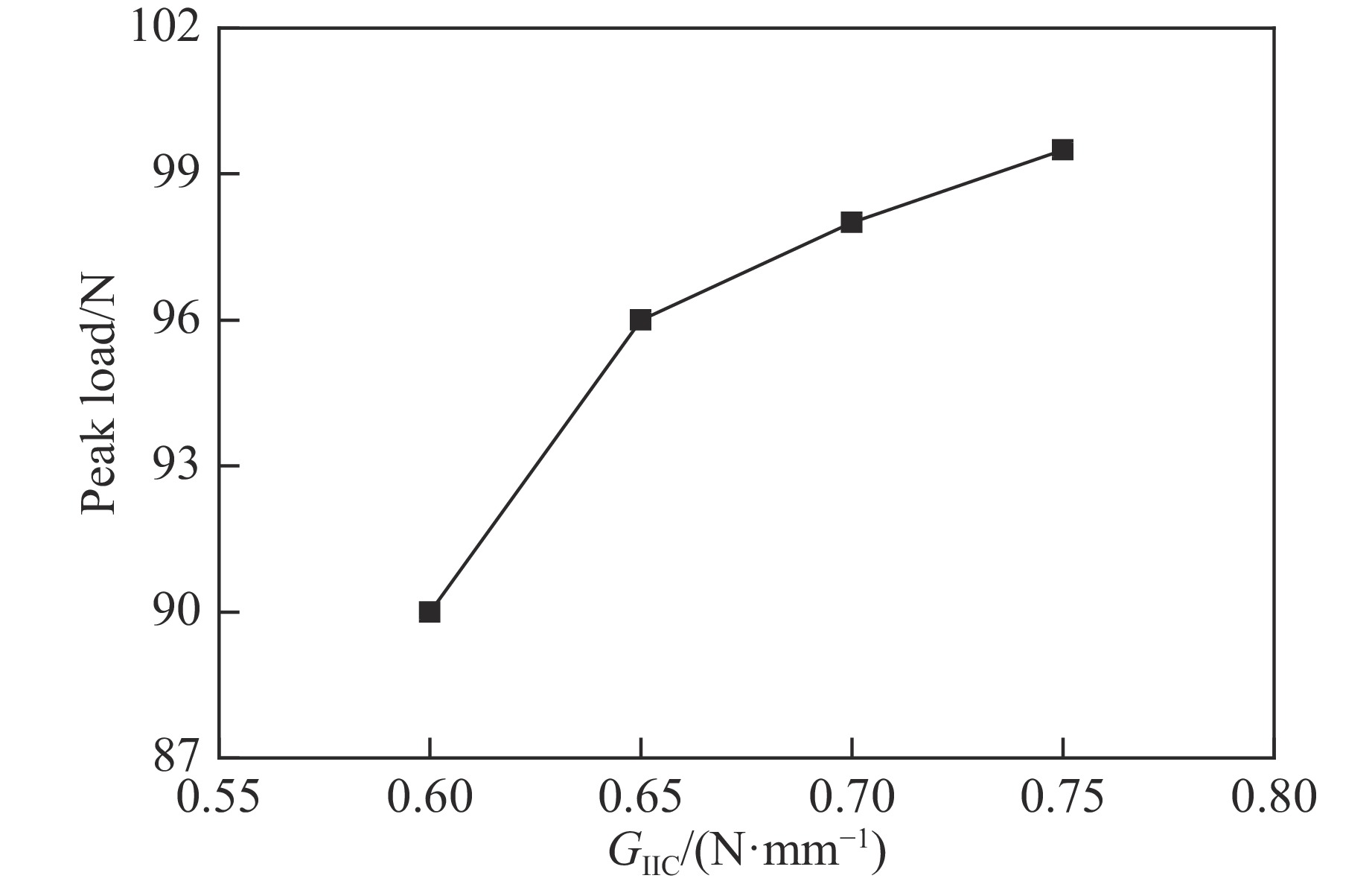
此外,弯曲力峰值与断裂韧性之间的关系曲线如图15所示。由图15可知,保持相同的断裂韧性增加值0.05 N/mm,弯曲力峰值增长率变化依次为120、40、30,表明断裂韧性的增强对弯曲力峰值的影响越来越小。
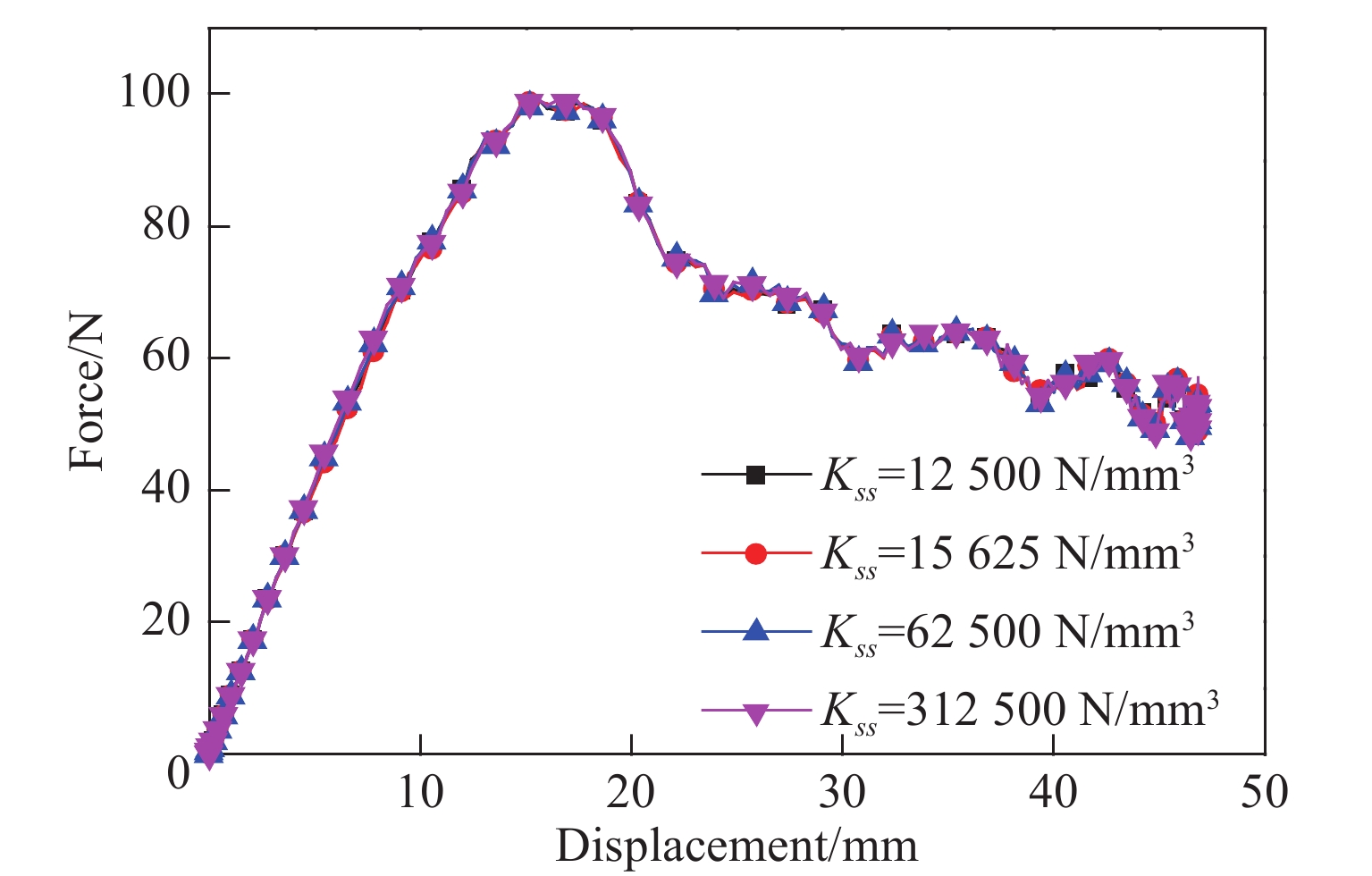
2.2.3 起始界面刚度的影响
不同起始界面刚度的荷载-位移曲线如图16所示。由图16可知,荷载-位移曲线随起始界面刚度的增大无显著变化。这是由于起始界面刚度仅影响试样破坏起始时的单元节点错开量,不会影响整体试样的破坏能。
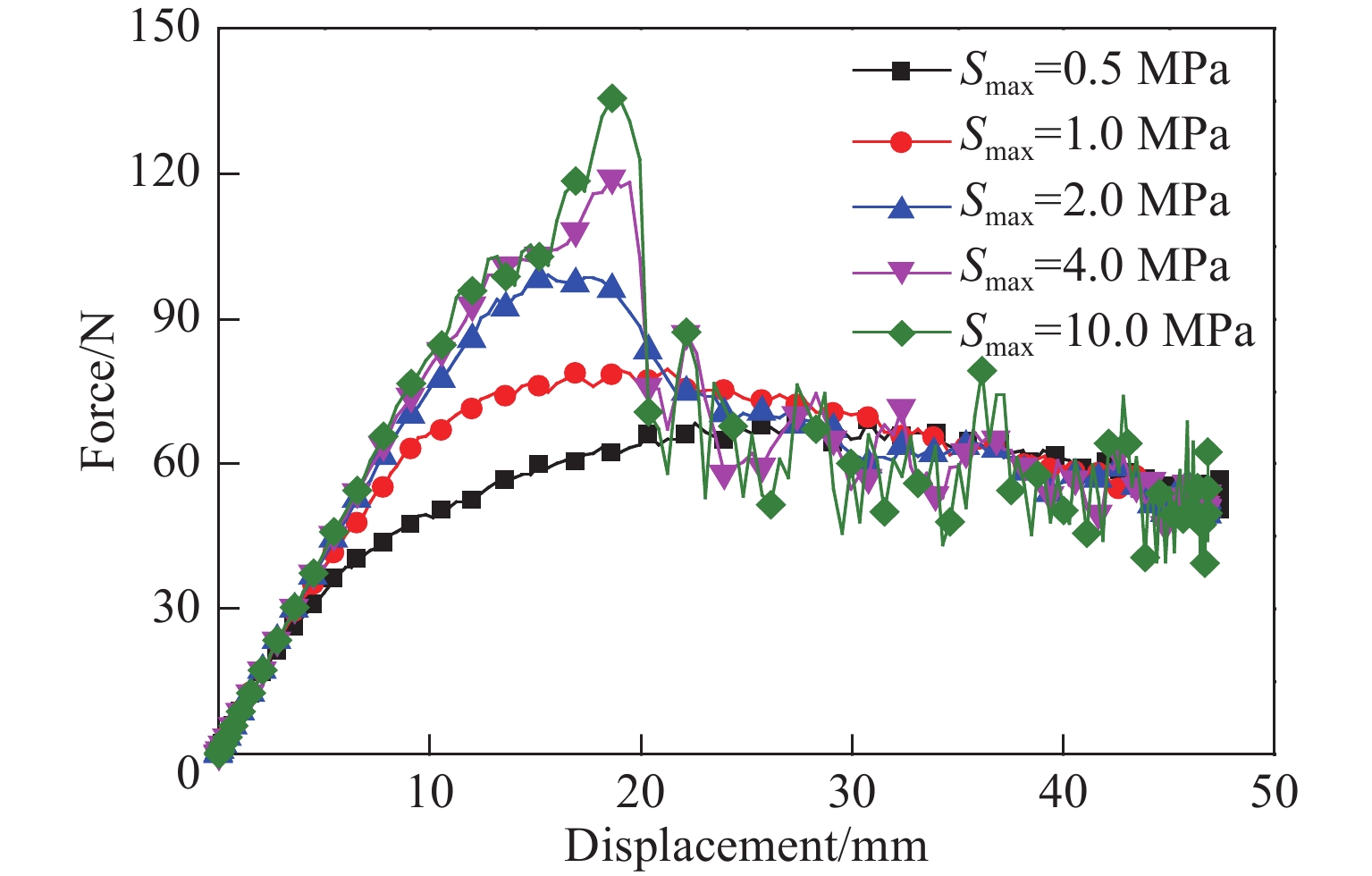
2.2.4 界面强度的影响
不同界面强度的荷载-位移曲线如图17所示。由图17可知,弯曲力峰值随界面强度的增加而增大。这是由于保持断裂韧性不变,破坏演化阶段的节点错开量随起始界面刚度的增大而减小,达到单元破坏所需的应力增大,因此所需的外荷载增大。
图17中Smax = 2.0 MPa为模拟II型试样黏结层的应力参数值。可见,当Smax大于2.0 MPa时,节点错开量减小,中间黏结层在达到损伤演化点前发生破坏,导致曲线在弯曲力峰值点前出现抖动;当Smax小于2.0 MPa时,节点位移错开量增加,中间黏结层能够抵挡损伤破坏,曲线平滑。
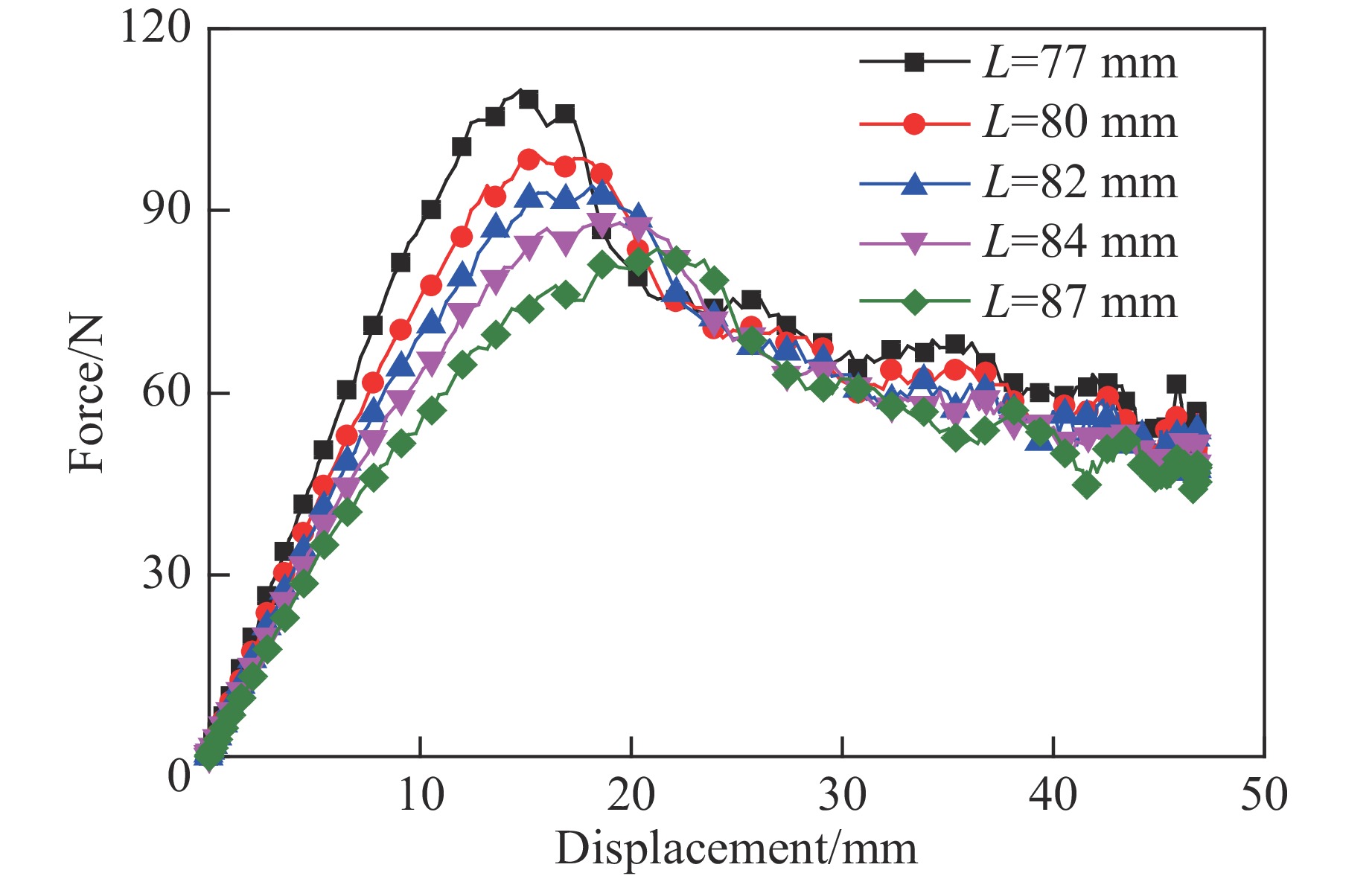
2.2.5 净距的影响
不同净距L下的荷载-位移曲线如图18所示。由图18可知,弯曲力峰值随净距的增大而减小,荷载峰值对应的位移增大。
净距的改变影响了初始裂纹长度和试样跨距两部分。弯曲力峰值随初始裂纹长度的增大而减小(见图13)。随着跨距增大,试样整体抗弯刚度降低,位移加载相同距离时所对应的弯曲力减小,因此试样破坏时所对应的位移增大。因此初始裂纹长度和试样跨距共同导致了弯曲力峰值的减小以及荷载峰值对应位移的增大。
2.2.6 黏结层厚度的影响
不同黏结层厚度条件下的荷载-位移曲线如图7所示。由图7可知,弯曲力峰值随黏结层厚度的增大而减小,弯曲力峰值对应的位移在15.5 mm左右。保持相同的黏结层厚度增加值0.04 mm,则弯曲力峰值减小量降低,由17.93 N降为2.74 N。因此当黏结层厚度增大到一定程度后,弯曲力峰值同样不会产生明显的增长。
3. 结 论
通过有限元模拟对3D打印浆砌层合结构复合材料的I型和II型断裂韧性进行了分析,研究了初始裂纹长度、断裂韧性、起始界面刚度、界面强度、黏结层厚度以及净距等参数对3D打印浆砌层合结构复合材料层间力学性能的影响,得到以下结论。
(1) 对于3D打印浆砌层合结构复合材料I型模型,减小初始裂纹长度、增加断裂韧性值以及增大黏结层厚度都能够增强材料韧性,使层间具有较高的承载能力。其中损伤演化后荷载下降速度随初始裂纹长度的减小而平稳缓慢下降,起始界面刚度和界面强度的改变对荷载峰值无显著变化,界面强度的增大会引起载荷峰值对应位移的减小。
(2) 对于3D打印浆砌层合结构复合材料II型模型,减小初始裂纹长度、增加断裂韧性、增强界面强度、减小黏结层厚度和减小净距都能够增强材料韧性,使层间具有较高的承载能力。其中II型断裂韧性的增大对荷载峰值的影响越来越小;净距的减小会引起载荷峰值对应位移的减小,引起材料瞬间破坏;起始界面刚度对试样层间性能无显著影响。
-
表 1 内聚力参数
Table 1. Cohesive parameters
T/mm Nmax/ MPa Smax/ MPa GI/(N∙mm−1) GII/(N∙mm−1) Knn/(N∙mm−3) Kss/(N∙mm−3) 0.16 0.35 2.0 0.40 0.73 15 625 31 250 0.20 0.50 2.5 0.55 0.65 12 500 25 000 -
[1] SCHÄFFER T E, IONESCUZANETTI C, PROKSCH R, et al. Does abalone nacre form by heteroepitaxial nucleation or by growth through mineral bridges [J]. Chemistry of Materials, 1998, 10(8): 946–946. [2] SHAO Y, ZHAO H P, FENG X Q, et al. Discontinuous crack-bridging model for fracture toughness analysis of nacre [J]. Journal of the Mechanics and Physics of Solids, 2012, 60(8): 1400–1419. doi: 10.1016/j.jmps.2012.04.011 [3] 万欣娣, 任凤章, 刘平, 等. 贝壳珍珠层的研究现状 [J]. 材料导报, 2006, 20(10): 21–24. doi: 10.3321/j.issn:1005-023X.2006.10.006WAN X D, REN F Z, LIU P, et al. Research status of shell nacre [J]. Materials Reports, 2006, 20(10): 21–24. doi: 10.3321/j.issn:1005-023X.2006.10.006 [4] BERTOLDI K, BIGONI D, DRUGAN W J. Nacre: an orthotropic and bimodular elastic material [J]. Composites Science and Technology, 2008, 68(6): 1363–1375. doi: 10.1016/j.compscitech.2007.11.016 [5] 马骁勇, 梁海弋, 王联凤. 三维打印贝壳仿生结构的力学性能 [J]. 科学通报, 2016, 61(7): 728–734.MA X Y, LIANG H Y, WANG L F. Mechanical properties of three-dimensional printed shell biomimetic structures [J]. Science Bulletin, 2016, 61(7): 728–734. [6] XU X P, NEEDLEMAN A. Void nucleation by inclusion debonding in a crystal matrix [J]. Modelling and Simulation in Materials Science and Engineering, 1993, 1(2): 111–132. doi: 10.1088/0965-0393/1/2/001 [7] HOSSEINI M R, TAHERI-BEHROOZ F, SALAMAT-TALAB M. Mode I interlaminar fracture toughness of woven glass/epoxy composites with mat layers at delamination interface [J]. Polymer Testing, 2019, 78: 105943. doi: 10.1016/j.polymertesting.2019.105943 [8] HUA X G, LI H G, LU Y, et al. Interlaminar fracture toughness of glare laminates based on asymmetric double cantilever beam (ADCB) [J]. Composites Part B: Engineering, 2019, 163: 175–184. doi: 10.1016/j.compositesb.2018.11.040 [9] 宗要武. 基于内聚力模型的钢纤维水泥基材料界面性能分析 [D]. 重庆: 重庆大学, 2018: 23–27.ZONG Y W. Analysis of interfacial bonding properties of cement-based materials with steel fibers based on cohesive zone model [D]. Chongqing: Chongqing University, 2018: 23–27. [10] ALFARO M V C, SUIKER A S J, RENÉ D B, et al. Analysis of fracture and delamination in laminates using 3D numerical modelling [J]. Engineering Fracture Mechanics, 2009, 76(6): 761–780. doi: 10.1016/j.engfracmech.2008.09.002 [11] LIU Y, DER M F P, SLUYS L J. Cohesive zone and interfacial thick level set modeling of the dynamic double cantilever beam test of composite laminate [J]. Theoretical and Applied Fracture Mechanics, 2018, 96: 617–630. doi: 10.1016/j.tafmec.2018.07.004 [12] 赵丽滨, 龚愉, 张建宇. 纤维增强复合材料层合板分层扩展行为研究进展 [J]. 航空学报, 2019, 40(1): 509–522.ZHAO L B, GONG Y, ZHANG J Y. A survey on delamination growth behavior in fiber reinforced composite laminates [J]. Acta Aeronauticaet Astronautica Sinica, 2019, 40(1): 509–522. [13] 寇剑锋, 徐绯, 郭家平, 等. 黏聚力模型破坏准则及其参数选取 [J]. 机械强度, 2011, 33(5): 714–718.KOU J F, XU F, GUO J P, et al. Failure criterion of cohesion model and its parameter selection [J]. Mechanical Strength, 2011, 33(5): 714–718. [14] American Society for Testing and Materials. Standard test method for mode Ⅰ interlaminar fracture toughness of unidirectional fiber-reinforced polymer matrix composites: ASTM D5528-01 [S]. West Conshohocken, PA: ASTM, 2007. [15] O’BRIEN T K, JOHNSTON W M, TOLAND G J. Mode II interlaminar fracture toughness and fatigue characterization of a graphite epoxy composite material: NASA/TM-2010-216838 [R]. Hampton, VA: NASA, 2010. [16] ARRESE A, BOYANO A I, DE G J, et al. A novel procedure to determine the cohesive law in DCB tests [J]. Composites Science and Technology, 2017, 152: 76–84. doi: 10.1016/j.compscitech.2017.09.012 [17] ARRESE A, INSAUSTI N, MUJIKA F, et al. A novel experimental procedure to determine the cohesive law in ENF tests [J]. Composites Science and Technology, 2019, 170: 42–50. doi: 10.1016/j.compscitech.2018.11.031 -








 下载:
下载:

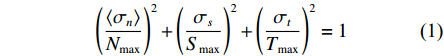
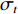
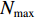

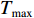
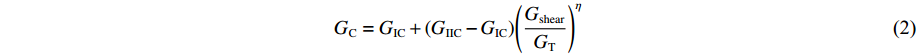
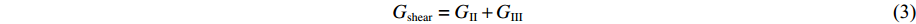
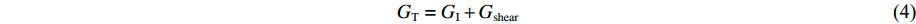
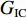
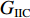
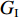

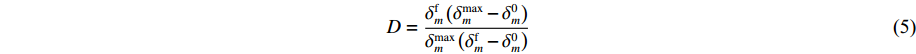
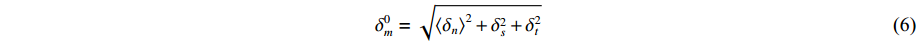
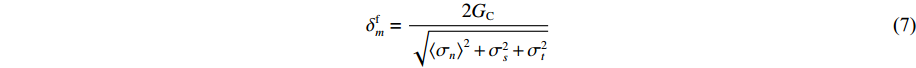
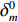
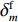


















 下载:
下载: