Numerical Analysis of Sensitivity of Tin Rayleigh-Taylor Instability to Model Parameters
-
摘要: 利用自研的爆轰与冲击动力学欧拉计算程序和Steinberg-Guinan（SG）本构模型，数值模拟分析了样品初始参数（初始振幅、初始波长、样品初始厚度）和SG本构模型初始参数对爆轰驱动锡Rayleigh-Taylor（RT）不稳定性增长的影响。结果表明金属锡样品的初始参数对其RT不稳定性增长有很大的影响。RT不稳定性增长随着初始振幅的减小而减小，且存在一个截止初始振幅；存在一个最不稳定的模态（波长），当初始波长大于该波长时，RT不稳定性增长随着初始波长的减小而增大，反之，RT不稳定性增长随着初始波长的减小而减小；样品厚度的增大可以抑制RT不稳定性增长，而且存在一个样品截止厚度。金属锡的RT不稳定性增长对其SG本构模型应变硬化系数和应变硬化指数的变化不敏感，而对压力硬化系数和热软化系数比较敏感。从采用扰动增长法预估材料强度的角度来说，修正压力硬化系数以获得锡合理的材料强度是合理的途径。
-
关键词:
- Rayleigh-Taylor不稳定性 /
- 材料强度 /
- 本构模型
Abstract: The sensitivity of Rayleigh-Taylor instability of tin driven by explosion to the initial parameters of sample (initial amplitude, wavelength and thickness of sample) and the initial parameters of SG constitutive model are numerical investigated by using an in-house Eulerian detonation and shock wave code. It concludes that the initial parameters of sample have a significant effect on the RT instability of Sn. The Sn RT instability grows more slowly with the initial amplitude decreasing, and a cutoff initial amplitude exists. A most unstable mode (wavelength) exists, when the initial wavelength is larger than this value, the RT instability grows faster as the initial wavelength diminishes; on the contrary, the RT instability grows slower as the initial wavelength decreases. The larger thickness of sample can restrain the growth of RT instability greatly, and a cutoff thickness of sample also exists. The Sn RT instability growth is not sensitive to the strain hardening coefficient and exponent, and it is greatly sensitive to the pressure hardening coefficient and thermal softening coefficient. But it should be a practical path to estimate the material strength of Sn through modifying the pressure hardening coefficient of SG model.-
Key words:
- Rayleigh-Taylor instability /
- material strength /
- constitutive model
-
铝-聚四氟乙烯（Al/PTFE）是一种新型氟聚物基活性材料，具有高能和钝感双重特性，在冲击作用下会发生剧烈的放热反应，是典型的冲击引发型含能材料[1-3]。活性材料可以依靠自身的动能和化学能对目标进行双重毁伤，这种特性被广泛应用于防空、反导破障等军事领域，成为一类重要的国防工业升级用和民用新型含能材料[4]，具有广阔的发展前景。
国内外学者针对Al/PTFE活性材料的力学和反应性能开展了大量相关工作，并对颗粒尺寸与材料力学性能的作用规律进行了系统性研究。Joshi等[5]公布的铝-聚四氟乙烯模压烧结专利，被广泛应用于Al/PTFE及相似含能材料的制备。阳世清等[6]采用冷压烧结法制备了Al/PTFE活性材料，测试其理化性能、热分解性能和力学性能，并进一步研究压制成型和烧结工艺参数的作用规律。赵鹏铎等[7]和徐松林等[8]研究了Al含量及应变率对Al/PTFE力学性能和反应特性的影响。Herbold等[9]研究了Al/PTFE在不同填充物下的工程应变及性能变化。葛超等[10-11]从微观角度，采用有限元方法系统研究了Al颗粒粒径及分布特征对Al/PTFE材料力学性能的影响。Osborne等[12]研究了Al颗粒粒径对Al/PTFE热分解过程的影响。吴家祥等[13]对经过特殊热处理的Al/PTFE分别进行了准静态压缩和落锤撞击试验，结果表明在较低应变率（10–2～102 s–1）范围内，随着Al粒径增大，Al/PTFE活性材料发生活化由易变难。Al/PTFE作为一种复合材料，其组分含量、粒径尺寸等原材料差异对材料力学性能及反应特性有显著影响。
在冲击引发及能量释放方面：王海福等[14]针对活性破片毁伤威力评估问题，提出了一种动态测量活性破片能量输出特性的方法；Mock等[15]研究了Al/PTFE杆的冲击引发临界条件及准则，并深入研究了能量释放与撞击速度的关系以及对Al颗粒增强特征尺寸的影响。葛超等[16]通过16 mm口径气枪发射Al/PTFE圆柱破片碰撞靶板的试验和冲击压缩的理论分析，研究材料在冲击条件下的反应阈值，试验结果表明：碰撞应力和应变率均对Al/PTFE的冲击反应有影响，并且共同决定了材料的反应情况，只有在应变率和应力均高于相应阈值时，材料在撞击条件下才会发生反应。
为探究成型压强及铝粉粒径对Al/PTFE活性材料冲击反应特性的影响，采用分离式霍普金森压杆（SHPB）装置撞击Al/PTFE试件，并通过高速摄影记录冲击过程，用试件恰好发生反应时撞击杆的撞击速度表示试件反应阈值，通过分析试验曲线及高速摄影观察结果，探究不同成型压强下Al/PTFE反应难易程度以及铝粉粒径对其反应阈值的作用规律，为研究Al/PTFE活性材料的反应机理以及材料制备、生产、运输和储存过程中的安全性提供参考。
1. 试验方法
1.1 试件制备
采用模压烧结法制备试验用试件，其中铝粉的平均粒径分别为10、30和200
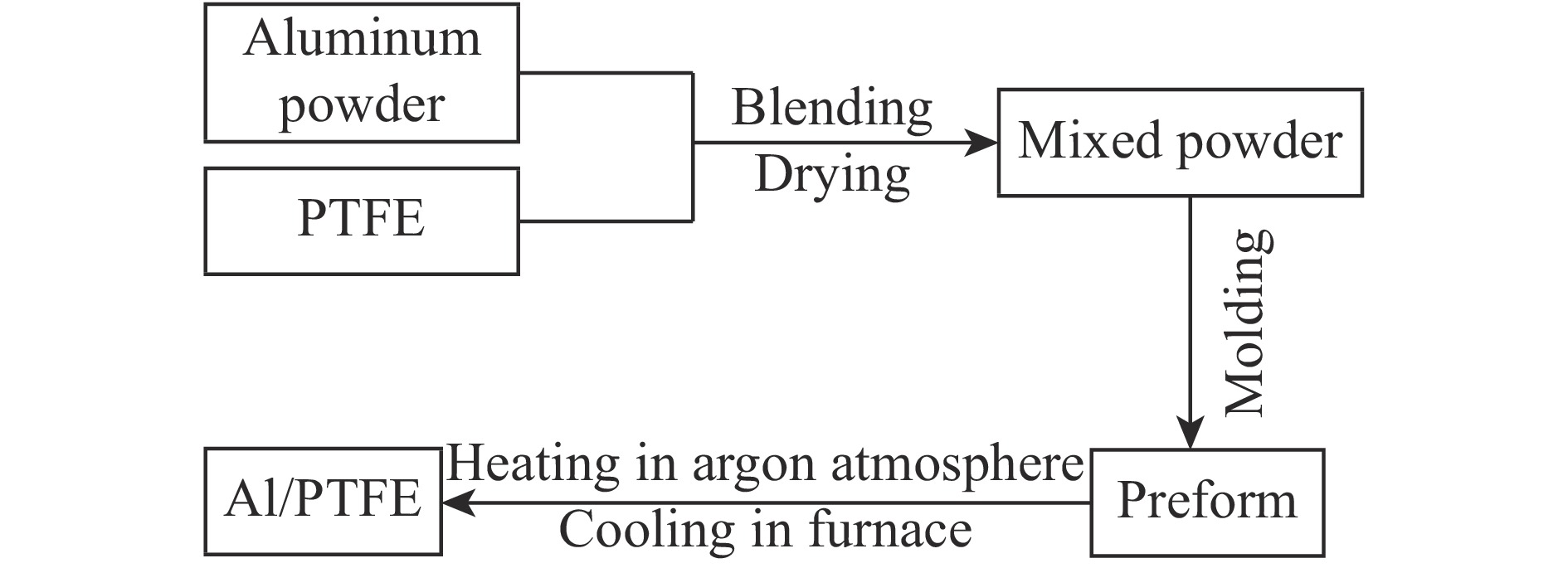
${\text{μ}}{\rm{m}}$ ，密度2.72 g/cm3；PTFE粉体的平均粒径为15${\text{μ}}{\rm{m}}$ ，密度2.17 g/cm3。基于Al和PTFE的主反应方程式4Al+3C2F4=4AlF3+6C，设置其质量分数分别为26.5%和73.5%，按此配比称取材料粉体并加入适量无水乙醇混合，使用V型混粉机混粉10 h。混粉均匀后将糊状原材料放在恒温鼓风干燥箱中烘干，干燥温度55 ℃。干燥后，称取适量粉体于压制模具中，使用万能材料试验机压制成型，通过控制试验机压力调节成型压强，将压制成型的试件保压200 s后取下、脱模，静置24 h后置于氩气气氛的箱式气氛炉中无压烧结，经过一定处理后降至室温并取出。设计试件尺寸为$\varnothing$ 10 mm×5 mm，磨平称量后进行试验。试件制备流程如图1所示。表1为不同铝粉粒径的Al/PTFE试件在不同成型压强下的孔隙率，可以看出：随着成型压强的升高，孔隙率降低，但试件材料整体孔隙率较小。
表 1 不同成型压强下Al/PTFE试件的孔隙率Table 1. Porosities of Al/PTFE reactive materials prepared under different molding pressuresMolding pressure/MPa Porosities of Al/PTFE/% 10 ${\text{μ}}{\rm{m}}$ Al particle 30 ${\text{μ}}{\rm{m}}$ Al particle 200 ${\text{μ}}{\rm{m}}$ Al particle 30 4.9 4.8 3.8 50 4.0 3.6 3.2 80 3.1 2.9 2.5 100 2.5 2.4 1.4 120 2.3 1.9 1.2 1.2 试验过程
试验采用的分离式霍普金森压杆（SHPB）试验装置主要由加载驱动系统、弹性压杆系统、信号测试和数据处理系统组成。加载驱动系统依靠压缩空气提供动力源，弹性压杆系统由撞击杆、入射杆、透射杆和吸收杆组成，杆件直径均为14.5 mm。撞击杆长度300 mm，由不同气室压力驱动以产生不同幅值的压缩波，速度由入射杆前激光测速装置测得。试验用钢质入射杆和透射杆长度均为1200 mm，通过分别贴在入射杆和透射杆相应位置的电阻应变片采集信号，并连接超动态信号测试分析系统。应变片接入电桥，根据应变片电阻变化得到输出电压波形，根据应变与输出电压的关系得到试验微应变数据并进行处理。
试验过程中通过高速摄影观察并记录材料冲击过程：若材料发生反应，则调节气室压力以降低撞击杆速度；若未观察到材料发生反应，则提高撞击杆速度。通过反复试验，最终获得材料的冲击反应速度阈值。
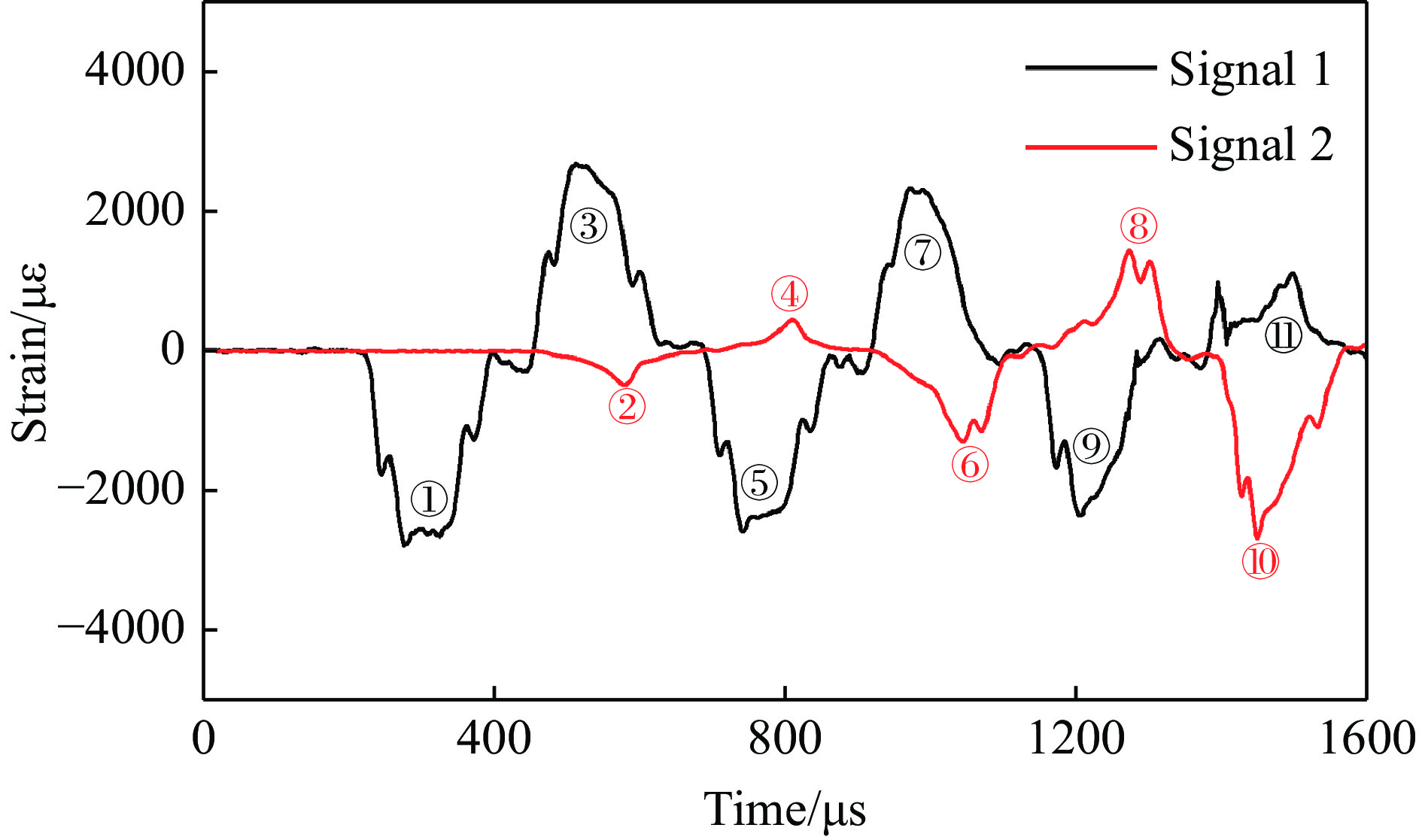
图2显示由SHPB装置中应变片采集的数据曲线，其中信号1和信号2分别为入射杆和透射杆上采集的信号。试验发现：应力波在入射杆中往复传播，对试件进行多次加载，每次应力脉冲的时间间隔约为475
${\text{μ}}{\rm{s}}$ ，单次脉冲长度约为150${\text{μ}}{\rm{s}}$ 。2. 结果与分析
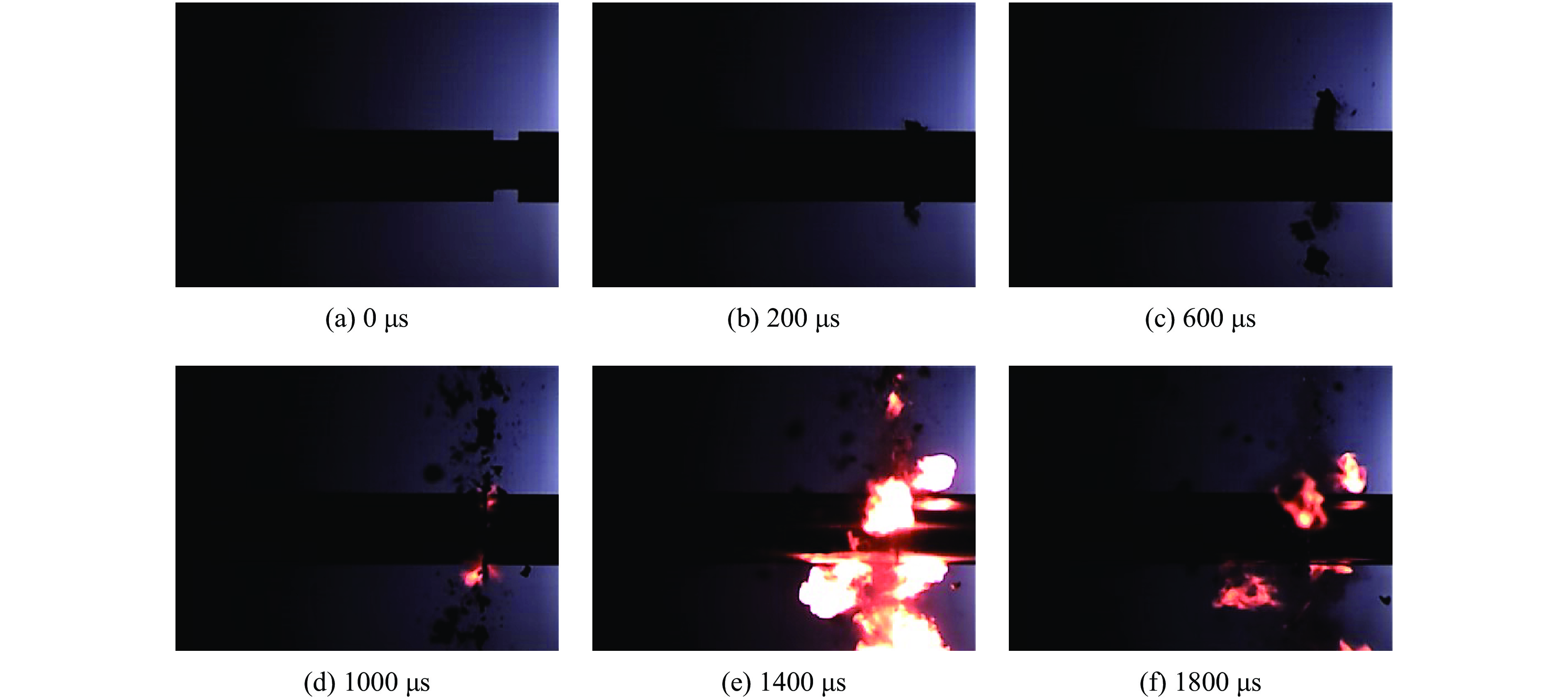
实验过程中通过高速摄影装置记录活性材料的冲击反应图像，其中高速摄影装置的拍摄帧率为20 000 幅/秒。如图3所示，试件（铝粉粒径10
${\text{μ}}{\rm{m}}$ 、成型压强100 MPa、撞击速度27.69 m/s）在受到冲击压缩后横截面积骤增，并在第一个压缩波抵达试件端面后约150${\text{μ}}{\rm{s}}$ 发生破碎和飞散；试件在受到冲击后1000${\text{μ}}{\rm{s}}$ 左右发生剧烈反应，发出明亮的火光并伴随响亮的爆鸣声，如图3（d）所示。不同铝粉粒径的Al/PTFE活性材料的反应特性试验结果统计如图4所示，激光测速仪记录撞击速度，点火延迟时间定义为从试件受到冲击时刻至记录火光发生时刻的时间长度。
图 4 不同成型压强试件反应阈值及点火延迟时间Figure 4. Thresholds and delay time of impact-ignition of Al/PTFE with different molding pressures2.1 成型压强对冲击反应速度阈值的影响
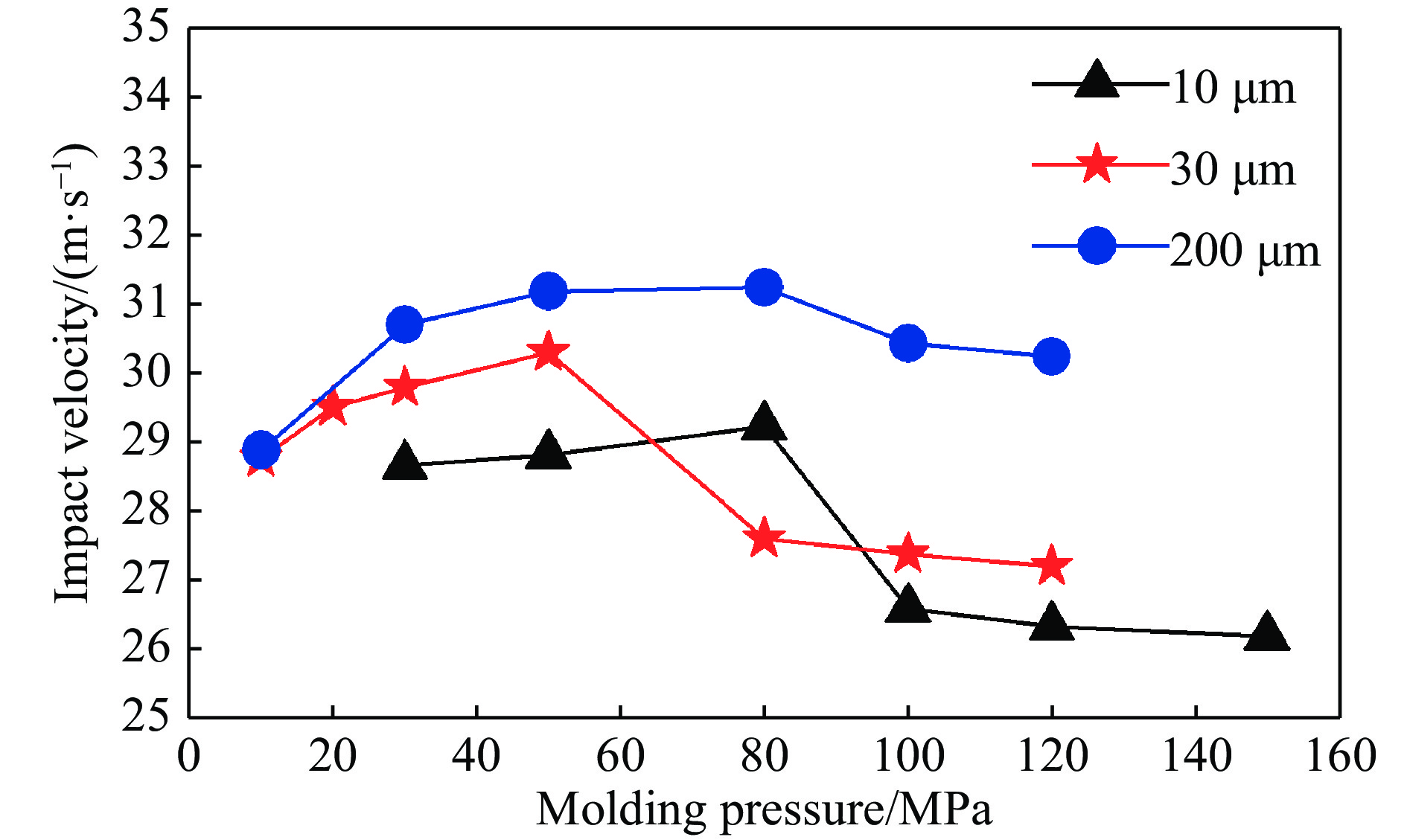
图5为不同铝粉粒径的Al/PTFE活性材料的冲击反应速度阈值随成型压强的变化规律，其中活性材料的冲击反应速度阈值定义为材料可以发生冲击引发反应的临界速度。
图 5 不同成型压强的Al/PTFE试件冲击反应速度阈值Figure 5. Thresholds of impact-ignition of Al/PTFE with different molding pressure从图5中可以看出，不同铝粉粒径活性材料的冲击反应速度阈值均随成型压强的增加呈先上升后下降的趋势。当成型压强小于50 MPa时，冲击反应速度阈值随成型压强的增加而增大；当成型压强达到80 MPa时，铝粉粒径30
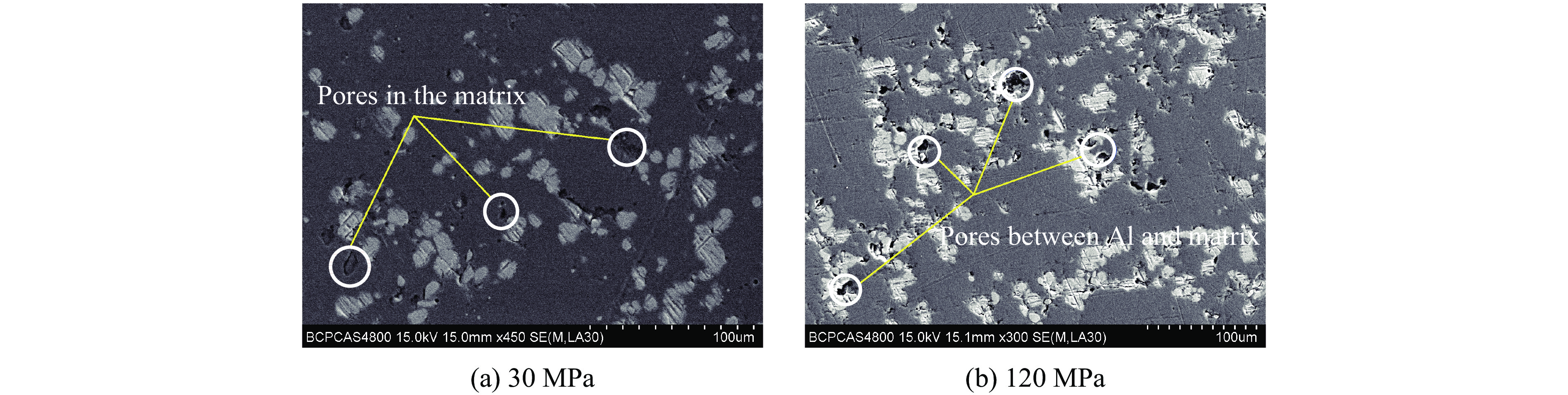
${\text{μ}}{\rm{m}}$ 活性材料的冲击反应速度阈值率先发生大幅下降，约为9%；当成型压强达100 MPa时，铝粉粒径10${\text{μ}}{\rm{m}}$ 活性材料的冲击反应速度阈值率大幅下降约9%，铝粉粒径30${\text{μ}}{\rm{m}}$ 和200${\text{μ}}{\rm{m}}$ 活性材料的冲击反应速度阈值也呈下降趋势，但下降幅度较小；成型压强为100 MPa以上时，活性材料的冲击反应速度阈值随成型压强的增加而减小。通常认为，非均质含能材料点火是多种“热点”机理共同作用的结果，其中起主导作用的热点机理取决于含能材料自身物化性质、材料微观结构（颗粒尺寸和粒径分布、孔隙或缺陷等）、加载条件以及热力学过程等因素[17-18]。为了探究Al/PTFE活性材料的点火特性随成型压强变化的原因，通过扫描电子显微镜（SEM）观察得到不同成型压强下Al/PTFE试件（铝粉粒径10
${\text{μ}}{\rm{m}}$ ）的微观结构，如图6所示。
图 6 不同成型压强制备的Al/PTFE试件的SEM图像Figure 6. SEM images of Al/PTFE reactive materials prepared under different molding pressures从图6（a）中可以看出，当成型压强较低（30 MPa）时，试件基体中存在大量的大体积空隙；随着成型压强升高，试件中的空隙数量减少，体积变小，与表1中试件孔隙率随成型压强变化规律对应；成型压强较高（120 MPa）的试件中存在大量介于Al颗粒和PTFE基体之间的空隙，如图6（b）所示。结合试件制作过程中的实际情况发现：该现象是由于高成型压强下所制试件内部的残余应力在烧结过程中释放，试件发生回弹变形所致，与烧结前后试件尺寸变化相对应。
结合扫描电镜结果与“热点”理论分析，试件受到冲击作用时，内部空隙迅速发生变形，材料内部摩擦产生大量的热，变形能和摩擦产生的热量在局部堆积，使局部温度迅速升高；另一方面，基体中各过程产生的热量向四周扩散，为局部高温“热点”的形成提供能量。上述过程最终导致Al颗粒和PTFE基体界面形成“热点”从而引发整个试件。随着试件成型压强升高，试件中的空隙数量减少，特别是介于Al颗粒和PTFE基体之间空隙明显减少，“热点”形成机会减小，试件的冲击反应速度阈值增大；成型压强高的试件由于烧结过程中的残余应力释放等因素，内部产生大量介于Al颗粒和PTFE基体之间的空隙，“热点”形成机会增大，试件冲击反应速度阈值减小。
2.2 试件点火延迟时间
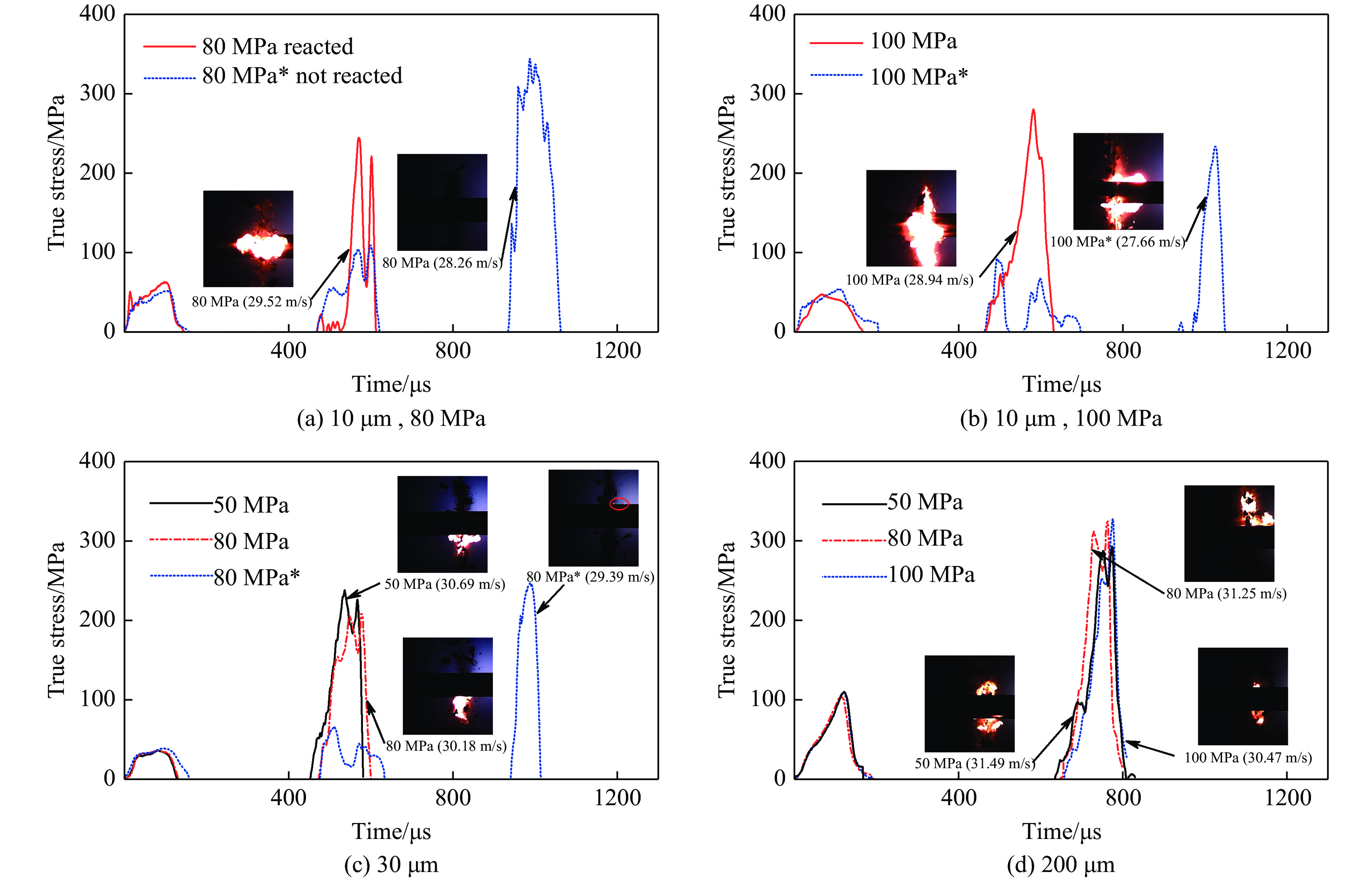
由图4可以看出，活性材料在SHPB装置加载下的点火延迟时间受撞击速度和材料的成型压强影响显著。当铝粉粒径分别为10
${\text{μ}}{\rm{m}}$ 和30${\text{μ}}{\rm{m}}$ 时，材料的点火延迟时间主要集中在600～650${\text{μ}}{\rm{s}}$ 和1000～1100${\text{μ}}{\rm{s}}$ 之间，且只有较高成型压强的试件在较低撞击速度下才能够于1000～1100${\text{μ}}{\rm{s}}$ 发生反应；而铝粉粒径为200${\text{μ}}{\rm{m}}$ 的活性材料试件点火延迟时间均在600${\text{μ}}{\rm{s}}$ 附近。为了进一步探究活性材料在冲击载荷下的反应机理，将试验中所采集到SHPB杆上的应变-时间信息进行数据处理，得到了活性材料在多次脉冲载荷下的应力-时间曲线，如图7所示。
图 7 不同颗粒尺寸条件下Al/PTFE试件的真实应力-时间曲线Figure 7. True stress-time curves of Al/PTFE with different particle sizes由图7可知，活性材料的点火延迟时间与第2、3次应力脉冲时间存在对应关系。随着应力波在杆中的往复弹射，多次脉冲载荷在试件中的应力幅值依次增大。对于铝粉粒径为10
${\text{μ}}{\rm{m}}$ 的试件，如图7（a）、图7（b）所示，当成型压强低于100 MPa时，若撞击速度达到材料的冲击反应速度阈值，试件仅在第2次应力脉冲为600～650${\text{μ}}{\rm{s}}$ 、应力幅值为200 MPa左右时发生反应，尽管第3次应力脉冲（1000～1100${\text{μ}}{\rm{s}}$ ）应力幅值高于300 MPa；当成型压强高于100 MPa时，材料率先在第3次脉冲应力幅值200 MPa左右发生化学反应，且随着撞击速度提高，试件上加载的应力脉冲幅值也随之提高，当第2次应力脉冲幅值达到200 MPa左右时，试件在第2次脉冲处可以发生反应，这也是试件的冲击反应速度阈值在成型压强100 MPa时发生骤降的原因。对于铝粉粒径为30${\text{μ}}{\rm{m}}$ 的试件，如图7（c）所示，成型压强对材料冲击点火特性的影响规律与铝粉粒径为10${\text{μ}}{\rm{m}}$ 的试件类似，只是致使活性材料点火特性发生转变的成型压强由100 MPa降至80 MPa。然而，铝粉粒径为200${\text{μ}}{\rm{m}}$ 的试件呈现的点火延迟时间与前两组有所不同，材料的点火反应只发生在第2次应力脉冲处，且引发材料反应的最低应力幅值均高于前者，约为300 MPa，如图7（d）所示。2.3 铝粉粒径对冲击反应速度阈值的影响
从前文中可以看出，Al/PTFE活性材料点火特性受铝粉颗粒粒径的影响。不同铝粉粒径活性材料的冲击反应速度阈值均随成型压强的增加呈先上升后下降趋势。图8为不同成型压强下各铝粉粒径试件的冲击反应速度阈值。由图8可知，当成型压强小于50 MPa时，活性材料的冲击反应速度阈值随铝粉粒径的增加而增加，该现象与吴家祥等[19]在Al/PTFE活性材料撞击试验中观察到的现象相似。
图 8 不同铝粉粒径的Al/PTFE试件的冲击反应速度阈值Figure 8. Thresholds of impact-ignition of Al/PTFE with different particle sizes of Al铝粉粒径为200
${\text{μ}}{\rm{m}}$ 的Al/PTFE试件在成型压强相同条件下的冲击反应速度阈值比铝粉粒径为10${\text{μ}}{\rm{m}}$ 的Al/PTFE试件高（约7%），其中成型压强为100 MPa及以上Al/PTFE试件的冲击反应速度阈值的相对偏差约为15%；铝粉粒径为10和30${\text{μ}}{\rm{m}}$ 的Al/PTFE试件具有相似的点火特性变化趋势，成型压强高的试件因可以在较低的撞击速度下于第3次应力脉冲处发生反应，其冲击反应速度阈值骤降（约9%）；铝粉粒径为200${\text{μ}}{\rm{m}}$ 的Al/PTFE试件未发现在第3次应力脉冲反应，因此其冲击反应速度阈值降低约3%，明显小于铝粉粒径为10和30${\text{μ}}{\rm{m}}$ 的Al/PTFE试件的骤减幅度。对此现象进行分析，原因如下。（1）金属颗粒增强复合材料在受压时，金属颗粒之间相互挤压，产生力链[20]（Force Chain），力链中金属颗粒受压变形可承载应力；力链经历生成、破坏和再生3个过程，材料中力链承载能力与力链数目、力链强弱和力链再生能力等有关。10
${\text{μ}}{\rm{m}}$ 粒径的铝颗粒力链再生能力强，可以承载更高的应力，但在高应变率冲击下200${\text{μ}}{\rm{m}}$ 粒径铝颗粒产生的单条力链承载能力远高于粒径小的铝颗粒力链。（2）金属颗粒表面可吸附多条聚合物大分子链，材料受到冲击时，应力通过金属颗粒和聚合物基体之间的分子链分散，致使材料的韧性增大；粒径大的铝颗粒的比表面积小，其所能吸附的分子链数目小，进而导致材料的韧性降低。铝粉粒径为200
${\text{μ}}{\rm{m}}$ 的Al/PTFE试件韧性低，在冲击过程中易发生碎裂，无法在第3次应力脉冲处发生反应。（3）大粒径铝颗粒的比表面积小，因此反应物Al与氧化剂PTFE的接触面积小；烧结过程中，基体在高温条件下发生流动、变形，填充大颗粒与基体之间的部分空隙。Al与氧化剂PTFE的接触面积降低使得材料在冲击过程中局部“热点”产生的难度更高。
3. 结 论
采用分离式霍普金森压杆（SHPB）装置撞击Al/PTFE试件，并通过高速摄影记录冲击过程，用撞击杆撞击速度表示试件反应阈值。通过分析试验曲线及高速摄影观察结果，总结不同成型压强下Al/PTFE的反应难易程度以及不同铝粉粒径对其反应阈值的影响，得到的结论如下：
（1）不同铝粉粒径的Al/PTFE活性材料的冲击反应速度阈值随成型压强的增加呈先上升后下降趋势，结合扫描电镜图像和试件压制过程分析，该现象与高成型压强制得的试件在烧结过程中残余应力释放有关；
（2）试件的点火延迟时间与第2次应力脉冲（600～700
${\text{μ}}{\rm{s}}$ ）或第3次应力脉冲（1000～1100${\text{μ}}{\rm{s}}$ ）时间对应；（3）随着铝粉粒径的增大，在成型压强相同的条件下，试件的冲击反应速度阈值升高；铝粉粒径为200
${\text{μ}}{\rm{m}}$ 的Al/PTFE试件未在第3次应力脉冲发生反应，因此其冲击反应速度阈值未发生骤降。 -
图 2 Lindquist等爆轰驱动铝实验的扰动振幅比较
Figure 2. Comparison of perturbation amplitudes of Lindquist et al.’s experiments driven by explosion
图 5 不同初始振幅时的扰动振幅增长曲线
Figure 5. Perturbation amplitude growth for different initial amplitude
图 6 不同初始波长时的扰动振幅增长曲线
Figure 6. Perturbation amplitude growth for different initial wavelength
图 7 不同样品初始厚度时的扰动振幅增长曲线
Figure 7. Perturbation amplitude growth for different initial thickness of sample
图 8 应变硬化系数不同时的扰动振幅增长曲线
Figure 8. Perturbation amplitude growth for different strain hardening coefficient
图 9 应变硬化指数不同时的扰动振幅增长曲线
Figure 9. Perturbation amplitude growth for different strain hardening exponent
图 10 压力硬化系数不同时的扰动振幅增长曲线
Figure 10. Perturbation amplitude growth for different pressure hardening coefficient
图 11 热软化系数不同时的扰动振幅增长曲线
Figure 11. Perturbation amplitude growth for different thermal softening coefficient
表 1 JO-9159炸药JWL状态方程参数
Table 1. EOS parameters of JO-9159 explosive
ρ0/(g·cm–3) pCJ/GPa DCJ/(km·s–1) α/GPa σ/GPa R1 R2 ω 1.86 36 8.862 934.8 12.7 4.6 1.1 0.37 
下载: 导出CSV
表 2 锡的Mie-Grüneisen状态方程参数
Table 2. Mie-Grüneisen EOS parameters of Sn
ρ0/(g·cm–3) c/(km·s–1) γ0 $\alpha $ S1 S2 S3 7.287 2.61 2.18 0.47 1.51 0 0
下载: 导出CSV
表 3 锡的SG本构模型参数
Table 3. SG constitutive model parameters of Sn
Y0/GPa Ymax/GPa G0/GPa β n A/GPa–1 B/K–1 0.16 0.22 17.9 2 000.0 0.06 0.086 6 2.12×10–3
下载: 导出CSV
-
[1] TAYLOR G I. The instability of liquid surfaces when accelerated in a direction perpendicular to their planes. I [J]. Proceedings of the Royal Society of London Series A, 1950, 201(1065): 192–196. [2] RICHTMYER R D. Taylor instability in shock acceleration of compressible fluids [J]. Communications on Pure and Applied Mathematics, 1960, 13(2): 297–319. doi: 10.1002/cpa.3160130207 [3] CAMPBELL E M, HUNT J T, BLISS E S, et al. Nova experimental facility [J]. Review of Scientific Instruments, 1986, 57(8): 2101–2106. doi: 10.1063/1.1138755 [4] DIMONTE G, TERRONES G, CHERNE F J, et al. Use of the Richtmyer-Meshkov instability to infer yield stress at high-energy densities [J]. Physical Review Letters, 2011, 107(26): 264502. doi: 10.1103/PhysRevLett.107.264502 [5] PARK H S, LORENZ K T, CAVALLO R M, et al. Viscous Rayleigh-Taylor instability experiments at high pressure and strain rate [J]. Physical Review Letters, 2010, 104(13): 135504. doi: 10.1103/PhysRevLett.104.135504 [6] PARK H S, REMINGTON B A, BECKER R C, et al. Strong stabilization of the Rayleigh-Taylor instability by material strength at megabar pressures [J]. Physics of Plasmas, 2010, 17(5): 056314. doi: 10.1063/1.3363170 [7] NUCKOLLS J, WOOD L, THIESSEN A, et al. Laser compression of matter to super-high densities: thermonuclear (CTR) applications [J]. Nature, 1972, 239(5368): 139–142. doi: 10.1038/239139a0 [8] MCCRORY R L, MONTIERTH L, MORSE R L, et al. Nonlinear evolution of ablation-driven Rayleigh-Taylor instability [J]. Physical Review Letters, 1981, 46(5): 336–339. doi: 10.1103/PhysRevLett.46.336 [9] LINDL J D, MEAD W C. Two-dimensional simulation of fluid instability in laser-fusion pellets [J]. Physical Review Letters, 1975, 34(20): 1273–1276. doi: 10.1103/PhysRevLett.34.1273 [10] KIFONIDIS K, PLEWA T, SCHECK L, et al. Non-spherical core collapse supernovae-II. the late-time evolution of globally anisotropic neutrino-driven explosions and their implications for SN 1987 A [J]. Astronomy & Astrophysics, 2006, 453(2): 661–678. [11] MAC LOW M M, ZAHNLE K. Explosion of comet Shoemaker-Levy 9 on entry into the Jovian atmosphere [J]. The Astrophysical Journal, 1994, 434: L33–L36. doi: 10.1086/187565 [12] SHUVALOV V V, ARTEMIEVA N A. Numerical modeling of Tunguska-like impacts [J]. Planetary and Space Science, 2002, 50(2): 181–192. doi: 10.1016/S0032-0633(01)00079-4 [13] KAUS B J P, PODLADCHIKOV Y Y. Forward and reverse modeling of the three-dimensional viscous Rayleigh-Taylor instability [J]. Geophysical Research Letters, 2001, 28(6): 1095–1098. doi: 10.1029/2000GL011789 [14] MOLNAR P, HOUSEMAN G A, CONRAD C P. Rayleigh-Taylor instability and convective thinning of mechanically thickened lithosphere: effects of non-linear viscosity decreasing exponentially with depth and of horizontal shortening of the layer [J]. Geophysical Journal International, 1998, 133(3): 568–584. doi: 10.1046/j.1365-246X.1998.00510.x [15] MILES J W. Taylor instability of a flat plate, General atomic division of general dynamics: GAMD-7335 [R]. 1966. [16] WHITE G N. A one degree of freedom model for the Tayloy instability of an ideally plastic metal plate: LA-5225-MS [R]. Los Alamos, NM: Los Alamos National Laboratory, 1973. [17] ROBINSON A C, SWEGLE J W. Acceleration instability in elastic-plastic solids. II. analytical techniques [J]. Journal of Applied Physics, 1989, 66(7): 2859–2872. doi: 10.1063/1.344191 [18] PIRIZ A R, CELA J J L, CORTAZAR O D, et al. Rayleigh-Taylor instability in elastic solids [J]. Physical Review E, 2005, 72(5): 056313. doi: 10.1103/PhysRevE.72.056313 [19] PIRIZ A R, LÓPEZ CELA J J, TAHIR N A. Rayleigh-Taylor instability in elastic-plastic solids [J]. Journal of Applied Physics, 2009, 105(11): 116101. doi: 10.1063/1.3139267 [20] PIRIZ A R, CELA J J L, TAHIR N A. Linear analysis of incompressible Rayleigh-Taylor instability in solids [J]. Physical Review E, 2009, 80(4): 046305. doi: 10.1103/PhysRevE.80.046305 [21] BAI X B, WANG T, ZHU Y X, et al. Expansion of linear analysis of Rayleigh-Taylor interface instability of metal materials [J]. World Journal of Mechanics, 2018, 8(4): 94–106. doi: 10.4236/wjm.2018.84008 [22] BARNES J F, BLEWETT P J, MCQUEEN R G, et al. Taylor instability in solids [J]. Journal of Applied Physics, 1974, 45(2): 727–732. doi: 10.1063/1.1663310 [23] LORENZ K T, EDWARDS M J, GLENDINNING S G, et al. Accessing ultrahigh-pressure, quasi-isentropic states of matter [J]. Physics of Plasmas, 2005, 12(5): 056309. doi: 10.1063/1.1873812 [24] BARNES J F, JANNEY D H, LONDON R K, et al. Further experimentation on Taylor instability in solids [J]. Journal of Applied Physics, 1980, 51(9): 4678–4679. doi: 10.1063/1.328339 [25] LINDQUIST M J, CAVALLO R M, LORENZ K T, et al. Aluminum Rayleigh Taylor strength measurements and calculations [R]. Livermore, CA: Lawrence Livermore National Laboratory, 2007. [26] DE FRAHAN M T H, BELOF J L, CAVALLO R M, et al. Experimental and numerical investigations of beryllium strength models using the Rayleigh-Taylor instability [J]. Journal of Applied Physics, 2015, 117(22): 225901. doi: 10.1063/1.4922336 [27] WANG T, BAI J S, CAO R Y, et al. Numerical investigations of perturbation growth in aluminum flyer driven by explosion [J]. Chinese Journal of High Pressure Physics, 2018, 32(3): 032301. [28] OLSON R T, CERRETA E K, MORRIS C, et al. The effect of microstructure on Rayleigh-Taylor instability growth in solids [J]. Journal of Physics: Conference Series, 2014, 500(11): 112048. doi: 10.1088/1742-6596/500/11/112048 [29] 何长江, 周海兵, 杭义洪. 爆轰驱动金属铝界面不稳定性的数值分析 [J]. 中国科学G辑, 2009, 39(9): 1170–1173.HE C J, ZHOU H B, HANG Y H. Numerical study on the instability of metal Al driven by detonation [J]. Science in China (Series G), 2009, 39(9): 1170–1173. [30] 郝鹏程, 冯其京, 胡晓棉. 内爆加载金属界面不稳定性的数值分析 [J]. 爆炸与冲击, 2016, 36(6): 739–744. doi: 10.11883/1001-1455(2016)06-0739-06HAO P C, FENG Q J, HU X M. A numerical study of the instability of the metal shell in the implosion [J]. Explosion and Shock Waves, 2016, 36(6): 739–744. doi: 10.11883/1001-1455(2016)06-0739-06 [31] 刘军, 冯其京, 周海兵. 柱面内爆驱动金属界面不稳定性的数值模拟研究 [J]. 物理学报, 2014, 63(15): 155201. doi: 10.7498/aps.63.155201LIU J, FENG Q J, ZHOU H B. Simulation study of interface instability in metals driven by cylindrical implosion [J]. Acta Physica Sinica, 2014, 63(15): 155201. doi: 10.7498/aps.63.155201 [32] SLUTZ S A, HERRMANN M C, VESEY R A, et al. Pulsed-power-driven cylindrical liner implosions of laser preheated fuel magnetized with an axial field [J]. Physics of Plasmas, 2010, 17(5): 056303. doi: 10.1063/1.3333505 [33] MCBRIDE R D, SLUTZ S A, JENNINGS C A, et al. Penetrating radiography of imploding and stagnating beryllium liners on the Z accelerator [J]. Physical Review Letters, 2012, 109(13): 135004. doi: 10.1103/PhysRevLett.109.135004 [34] PARK H S, ARSENLIS A, BARTON N R. Stabilization of the Rayleigh-Taylor instability by material strength at high pressure and high strain rates [C]//16th International Workshop on the Physics of Compressible Turbulent Mixing. Marseilles, France, 2018. [35] REMINGTON B A, PARK H S, PRISBREY S T, et al. Progress towards materials science above 1 000 GPa (10 Mbar) on the NIF laser: LLNL-CONF-411555 [R]. Livermore, CA: Lawrence Livermore National Laboratory, 2009. [36] JENSEN B J, CHERNE F J, PRIME M B, et al. Jet formation in cerium metal to examine material strength [J]. Journal of Applied Physics, 2015, 118(19): 195903. doi: 10.1063/1.4935879 [37] BELOF J L, CAVALLO R M, OLSON R T, et al. Rayleigh-Taylor strength experiments of the pressure-induced α→ε→α′ phase transition in iron [C]//AIP Conference Proceedings. American Institute of Physics, 2012, 1426(1): 1521–1524. [38] LINDQUIST M J, CAVALLO R M, LORENZ K T, et al. Aluminum Rayleigh Taylor strength measurements and calculations [C]//LEGRAND M, VANDENBOOMGAERDE M. 10th International Workshop on Physics of Compressible Turbulent Mixing. Paris, France, 2006. -
下载:
下载:
点击查看大图
计量
- 文章访问数: 8475
- HTML全文浏览量: 3233
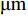
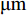
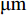
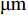
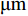
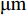
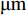
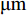
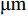
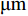
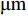
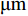
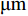
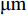
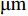
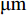
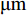
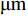
- PDF下载量: 28
