Effects of Carbon on (Mg,Fe)SiO3 Bridgmanite under the Lower Mantle Pressure-Temperature Conditions
-
摘要: 利用激光加温金刚石对顶砧技术模拟下地幔温压条件（36～88 GPa, 1 850～2 800 K），探索了碳与含铁的(Mg,Fe)SiO3布里奇曼石的相互作用过程。同步辐射X射线衍射实验表明，(Mg,Fe)SiO3布里奇曼石与碳在大于42 GPa、2 000 K的温压条件下发生了氧化还原反应，即(Mg,Fe)SiO3布里奇曼石中的二价铁（Fe2+）被单质碳还原成金属铁（Fe0）；而在较低的温压条件下，布里奇曼石中的Fe2+可以稳定存在。该结果表明，在下地幔深部的温压条件下，CCO缓冲的氧逸度值比IW缓冲更低，热力学计算结果也证实了这一结果。实验结果为地幔深部氧化还原条件的不均一性和局部极端还原状态的出现提供了解释。Abstract: In this study we investigated the interaction of carbon with iron in the (Mg,Fe)SiO3 bridgmanite under the conditions corresponding to the Earth’s lower mantle (36–88 GPa, 1 850–2 800 K) using a laser-heated diamond anvil cell. Synchrotron X-ray diffraction measurements of the run products showed that Fe2+ in bridgmanite can be reduced to metallic Fe by carbon under the pressure and temperature conditions higher than 42 GPa and 2 000 K. The coexisting metallic Fe and Fe-depleted bridgmanite in the run products suggests that the CCO buffer produces lower oxygen fugacity than the of Fe-FeO (IW) buffer, which is further confirmed by the thermodynamic calculation. The experimental results in this study could provide a potential explanation for the presence of redox heterogeneities and highly reducing regions in the deep mantle.
-
Key words:
- bridgmanite /
- deep carbon /
- lower mantle /
- redox reaction
-
高熵合金（High entropy alloy，HEA）于2004年首先由Yeh等[1]提出并命名，一般可以定义为由5种或5种以上元素组元按等原子比或近似等原子比合金化形成的新材料，每种元素占总原子数的5%～35%之间。随着人们对高熵合金的不断研究，其组成元素的数量由开始的5种及5种以上放宽到4种及以上金属元素。高熵合金具有高强度[1]、高硬度[2]、耐腐蚀[3]和耐摩擦[4]等优异的力学、化学和热学性能，在航空航天、船舶制造等工业材料上有较好的应用前景。传统的合金会由于金属种类的增加而导致其材料脆化[5]，但高熵合金和以往的传统金属不同，虽然是多种金属元素的杂乱混合，却在物理、化学、力学等方面具有传统合金无法比拟的优异性能。
高熵合金在各方面的优异性能激发了广大学者的研究兴趣，从理论到实验再到数值模拟的研究层出不穷。数值模拟计算是对传统理论和实验方法的有力补充，分子模拟技术在原子尺度揭示和预测材料的各项性能具有重要意义。Ko等[6]研究了碳含量对CoCrFeMnNi高熵合金微观组织的影响，发现铸态的CoCrFeMnNi和CoCrFeMnNiC0.1合金均具有树枝状微结构，晶粒尺寸随着碳含量的增加而减小。Xie等[7]采用分子动力学（MD）模拟方法研究在硅基上的AlCoCrCuFeNi高熵合金薄膜的生长，研究了不同原子含量对生长的薄膜及其原子结构的影响，从模拟结果可以观察到HEA的不同原子结构由原子数量、原子尺寸差异决定。Afkhama等[8]通过分子动力学模拟了AlxCrCoFeCuNi高熵合金在不同温度下的拉伸行为，研究了该合金在室温、高温和各种应变速率下的变形行为和机理，其拉伸试验结果表明：随着温度的升高，屈服应力显著下降；该合金在所有测试条件下都表现出超塑性行为；更重要的是，铝含量的增加会导致屈服应力和弹性模量显著降低。Choi等[9]基于Monte Carlo、分子动力学和分子静力学等原子模拟方法研究了单个元素对等原子CoCrFeMnNi高熵合金固溶硬化的影响，预测了在0 K时密排六方（HCP）结构比面心立方（FCC）结构更稳定。Zhang等[10]使用熔化和快速淬火的方法构建AlCrFeCuNi原子模型，通过原子模拟方法从微观角度解释了AlCrFeCuNi系高熵合金在拉伸载荷作用下的力学性质及原子结构变化，结果表明AlCrFeCuNi系高熵合金不仅有很高的强度，还具有良好的可塑性。
目前已经研制出的AlCrFeCuNi系高熵合金在力学性能方面尤为突出，表现出高强度、高硬度、耐磨和抗高温等一系列独特性能；然而在制备过程和实际应用中，过高或过低的外界温度[11]、合金中某一元素含量的变化都可能导致其原子晶格结构和力学性能发生改变。本研究采用分子动力学方法，模拟轴向拉伸载荷作用下AlCrFeCuNi高熵合金的力学性能，并分析在拉伸载荷作用过程中的微观原子晶格结构变化，同时着重研究温度和Al含量对AlCrFeCuNi高熵合金力学性能的影响。
1. 模型与势函数
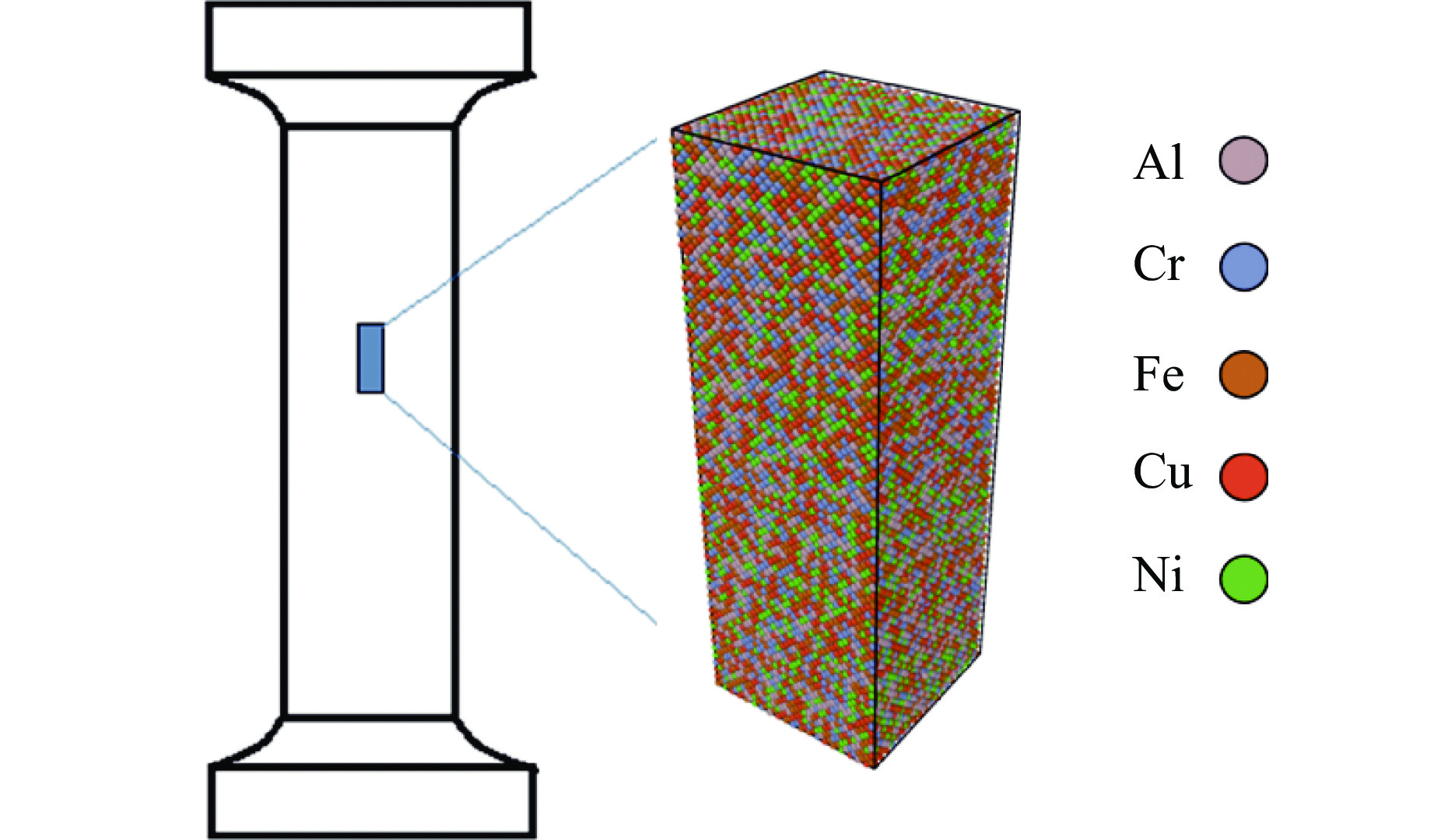
目前常用的高熵合金实验制备方法一般为真空电弧熔铸法：在电弧炉内加入配比好的物料，然后经高温熔化浇铸到高温的铸型中，再经冷却、退火，最后切割成制品[12]。因此在本研究中，采用分子动力学方法模拟高熵合金的实验制备过程，进而建立模型，具体做法如下[13]：（1）AlCrFeCuNi高熵合金中5种原子按所要求的比例随机分布在FCC晶体结构上，选取Al、Cr、Fe、Cu和Ni 5种单晶中晶格常数最大的一个作为AlCrFeCuNi高熵合金晶格常数，进行能量最小化和弛豫，采用共轭梯度算法进行几何结构优化，且使原子结构达到平衡；（2）模拟熔化阶段，体系中所有原子都以0.04 K/fs的速率加热到1 500 K并在该温度下进行一段时间的弛豫，以达到平衡；（3）模拟淬火阶段，同样以0.04 K/fs的速率降低体系温度，直到300 K，并进行一段时间的弛豫以达到平衡。通过高温熔化再到冷却退火，用分子动力学方法模拟实验制备过程，从而建立AlCrFeCuNi高熵合金的分子动力学模型，如图1所示。
分子动力学方法使用的势函数是经验势，而经验势是否能准确描述原子间的相互作用决定了模拟结果的正确性。目前金属元素的势函数中，使用较为成熟的原子势是嵌入原子势（Embedded atom method, EAM），在AlCrFeCuNi高熵合金分子动力学模型的构建过程及之后的模拟计算中，采用嵌入原子势[14]描述Cr-Fe-Ni和Al-Cu之间的相互作用，形式如下
Ei=Fα∑j≠iρi(ri,j)+12∑j≠iϕα,β(ri,j) (1) 式中：Ei是原子i的总能量；F表示嵌入能函数，是原子的电子密度
ρ 的函数；Φ 表示对势相互作用；α和β表示原子i和原子j的元素类型；rij表示原子i和原子j之间的距离。对于还没有描述的剩余原子间相互作用的势函数，包括Cr-Cu、Fe-Cu、Ni-Cu、Cr-Al、Fe-Al、Ni-Al之间的势函数，在本研究中，采用Morse对势函数描述[10]。在已知A、B两类原子自身相互作用的内聚能D、逆长度标度因子
α 和平衡距离r0的情况下，采用Lorentz-Berthelot （L-B）[15]混合律估算A类原子与B类原子相互作用时的Morse参数。L-B混合律如下{DA−B=√DADBαA−B=(αA+αB)/2r0A−B=√σAσB+ln2/αA−BσA,B=r0A,B−ln2/αA,B (2) 式中：DA-B表示A、B两类原子间的混合内聚能；
α A-B表示混合后的晶格常数，r0A-B表示原子A与原子B之间的平衡距离。表1列出了使用L-B混合律计算出的Morse势参数。Atom pair D/eV α/Å–1 r0/Å Cr-Cu 0.389 04 1.465 4 2.628 9 Fe-Cu 0.378 32 1.373 6 2.645 4 Ni-Cu 0.379 72 1.389 3 2.618 2 Cr-Al 0.345 41 1.368 5 2.819 9 Fe-Al 0.335 89 1.276 7 2.837 6 Ni-Al 0.337 13 1.292 4 2.808 3 2. 数值模拟
采用分子动力学方法，使用LAMMPS软件实现分子动力学模拟计算，计算流程大概分为4步：系统初始化；创建原子晶胞，建立模型；能量最小化和弛豫；模拟加载过程。模拟过程中采用周期性边界条件，用EAM和Morse对势描述模型中各类原子间的相互作用。创建的模型大小为20a×20a×60a（a为晶格常数），整个模型共有9.6×104个原子。选取合适的时间步长既可以节省计算时间，又可以保证模拟的准确度，为了充分利用CPU工作效率，整个模拟过程的时间步长选取1 fs。在模拟加载前，对整个体系进行能量最小化和系综弛豫，使整个模拟体系达到平衡状态。采用共轭梯度算法（Conjugate gradient methods, CG）进行能量最小化可以更精确地找到势能最低点，选择在等温等压系综（NPT）下进行充分弛豫。采用Verlet积分法求解分子动力学积分方程，使用Nose-Hoover热浴法控制体系温度。
加载过程中，除施加变形的z方向外，其他两个方向压力始终控制为零，通过Nose-Hoover热浴法进行温度控制，在NPT系综下，通过给模拟盒子施加一定的变形速率来实现模型加载过程。本研究中，应变率取为0.001 ps–1（109 s–1），每隔一定的时间步施加应变，体系按设置条件弛豫放松，重新定位每个原子的位置，如此循环实现模型的准静态加载。
3. 结果与分析
3.1 拉伸力学性能
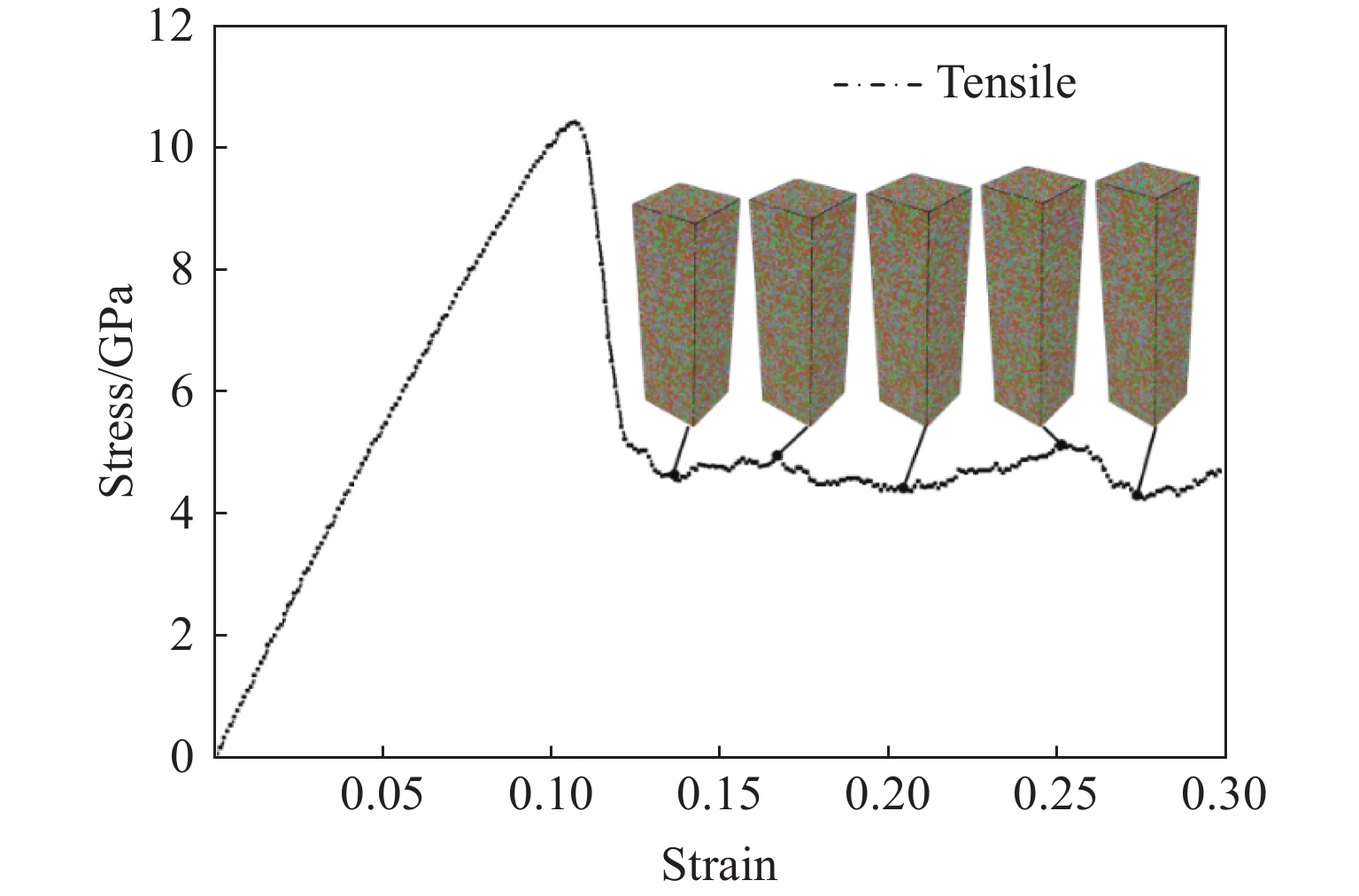
AlCrFeCuNi1.4高熵合金在常温（300 K）、拉伸载荷作用下的应力-应变曲线如图2所示。从图中可以明显地观察到整个体系在拉伸载荷作用下经历了3个阶段的变形，分别为弹性变形阶段、屈服阶段和塑性变形阶段。在拉伸开始时，应力-应变呈直线关系，服从胡克定律，应力随应变线性变化，对应图2曲线中的线性部分，而变形表现为弹性变形，该阶段中若停止加载则变形随之消失，与单晶金属拉伸过程中的弹性变形一致。通过拟合应力-应变曲线中0～0.05应变段的斜率，可以得到该拉伸方向的杨氏模量E，为108.77 GPa，与其他学者的实验和模拟结果118 GPa[8]非常接近。Afkhama等[8]研究得到AlCrCoFeCuNi高熵合金的屈服强度约为3.5 GPa，明显低于本研究的AlCrFeCuNi1.4高熵合金屈服强度10.3 GPa，可以证明Co元素的加入会明显降低该系高熵合金的强度。弹性阶段结束后，应力-应变曲线呈现非线性变化，曲线出现了断崖式的下降，对应出现应力峰值，材料开始进入屈服阶段。继续加载，曲线出现屈服平台，该阶段应力不增加或只小幅度上下波动，模型继续伸长变形，属于不均匀塑性变形阶段。
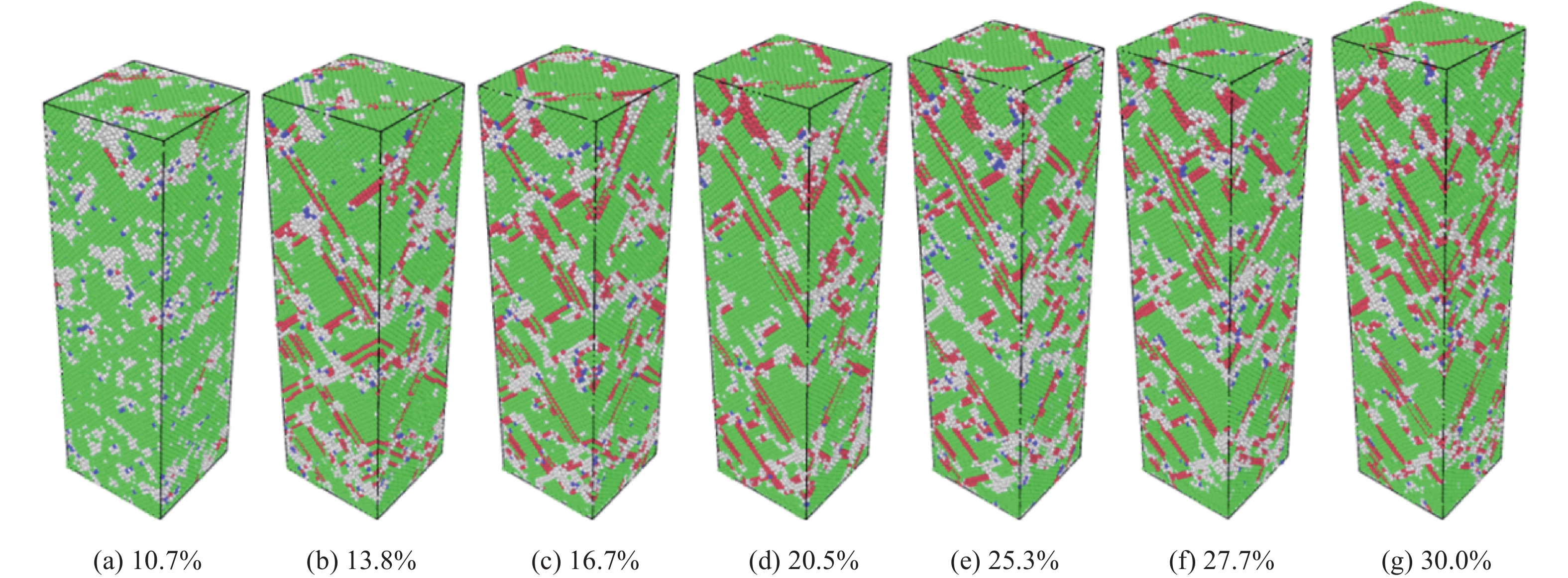
为了研究在拉伸载荷作用下AlCrFeCuNi高熵合金的微观结构变化，截取了在常温加载到不同拉伸应变时的原子结构图，如图3所示。通过共同近邻分析（Common neighbor analysis, CNA）能够观察到不同拉伸应变下的微观原子结构，图中以不同颜色区分不同的原子结构：绿色代表FCC原子，红色代表HCP原子，蓝色代表BCC原子，而白色代表不满足任何基本原子结构的无序原子。
图3（a）为10.7%应变下的原子状态，对应弹性阶段结束开始进入屈服阶段，此时AlCrFeCuNi高熵合金的原子晶体结构大部分为FCC结构，有少量BCC和HCP结构原子，同时出现无规则排列的无序原子。图3（b）对应于13.8%拉伸应变下的原子结构图，可以明显看到层状的HCP结构原子，且以45°和135°方向排列。在材料学中，一般认为FCC密排面原子错排层大于两层是孪晶结构，小于或等于两层的认为是堆垛层错，孪晶和层错的形成会加强材料的塑性性能。从图3（a）到图3（b），孪晶和层错的形成导致模型出现应力松弛，对应应力-应变曲线在该阶段出现应力骤降，且下降速率与体系孪晶和层错的形成速度有关。图3（c）～图3（g）对应应力-应变曲线出现屈服平台、材料出现不均匀塑性变形阶段。该阶段内，孪晶和层错不断产生、生长、消失，如此循环导致了屈服平台的出现，应力小幅度上下波动，AlCrFeCuNi高熵合金产生不均匀的塑性变形。
3.2 温度对力学性能的影响
在加载过程中，通过改变模拟温度得到AlCrFeCuNi高熵合金在不同温度下的应力-应变曲线和拉伸力学性能。从图4可以看出，体系温度从100 K上升到1 000 K的过程中，模型都经历了同样的弹性—屈服—不均匀塑性变形过程，应力-应变曲线的总体趋势一致。随着温度的不断升高，弹性模量、屈服应力和对应的屈服应变都呈减小趋势，且温度越高，屈服阶段应力从峰值处下降到屈服平台的速率越慢。与AlCrCoFeCuNi高熵合金的应力-应变曲线[8]不同的是，该曲线在屈服阶段有明显的下降过程，从原子尺度可以解释为孪晶和层错的出现比较突然，当达到上屈服点时会出现明显的应力下降，说明Co元素对合金在拉伸过程中出现的孪晶和层错有较大影响。从能量角度来讲，温度越高，原子热运动增加，导致结构不稳定，强度降低。
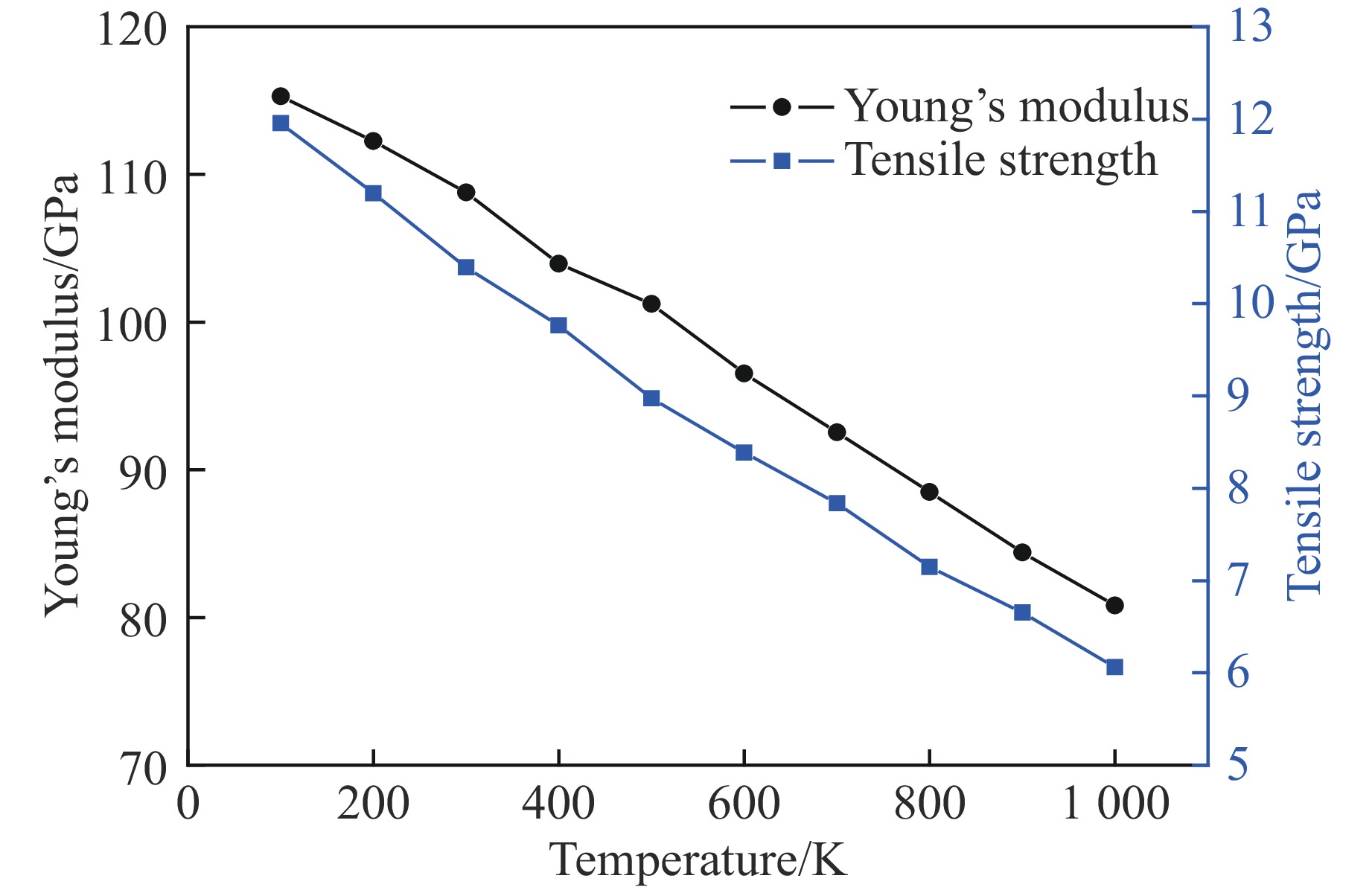
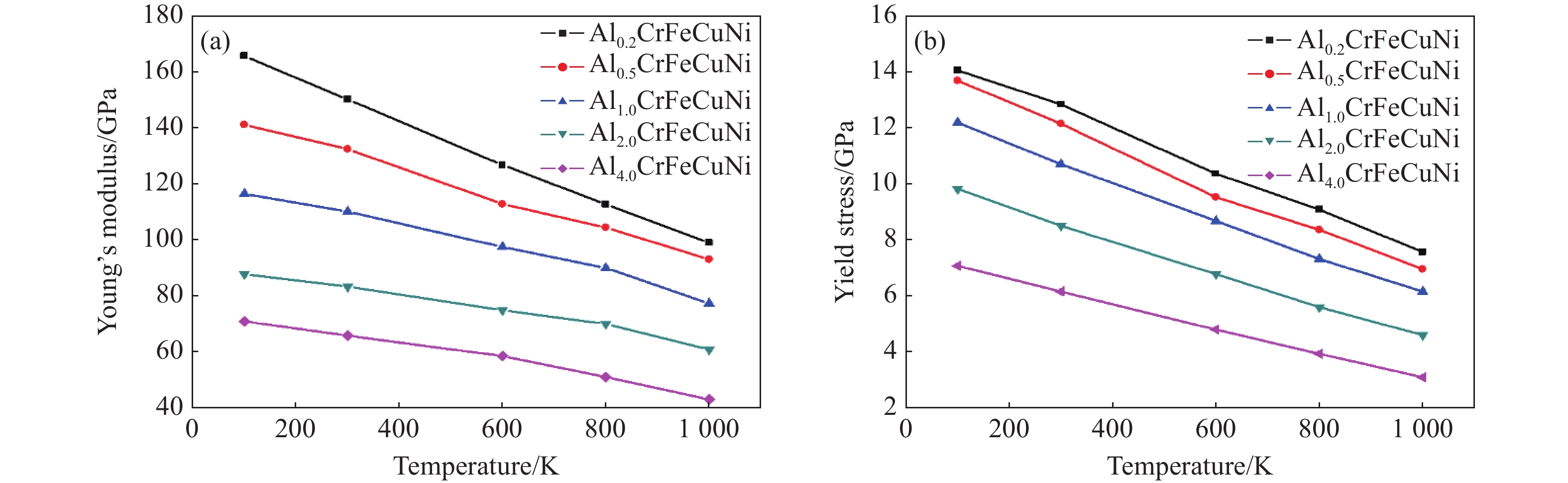
图 4 不同温度下的拉伸应力-应变曲线Figure 4. Stress-strain relations under uniaxial tensile loading at different temperatures为了量化结果，表2给出了不同温度下AlCrFeCuNi1.4高熵合金的杨氏模量、屈服应力和对应的屈服应变。由表2可知：随着温度的增加，AlCrFeCuNi1.4高熵合金的杨氏模量从100 K的115.273 GPa下降到1 000 K的80.808 GPa，减小了29.899%；此外屈服应力也从11.954 GPa下降到6.060 GPa，减小了49.306%。这表明体系温度从100 K升高到1 000 K对材料的拉伸力学性能影响较大。为了更明显地观察材料的拉伸力学性能随温度的变化趋势，选取不同温度下的杨氏模量和拉伸强度，如图5所示。可见AlCrFeCuNi1.4高熵合金的杨氏模量和拉伸强度随温度的升高近似呈线性降低。
表 2 不同温度下的拉伸力学性能Table 2. Mechanical properties under uniaxial tensile loading at different temperaturesTemperature/K Young’s modulus/
GPaYield stress/
GPaYield strain Temperature/K Young’s modulus/
GPaYield stress/
GPaYield strain 100 115.273 11.954 0.115 600 96.507 8.383 0.097 200 112.251 11.197 0.111 700 92.532 7.838 0.092 300 108.768 10.390 0.107 800 88.503 7.147 0.088 400 103.950 9.765 0.103 900 84.382 6.652 0.087 500 101.228 8.975 0.099 1 000 80.808 6.060 0.086 
图 5 不同温度下杨氏模量和拉伸强度的变化趋势Figure 5. Young’s modulus and tensile strength at different temperatures3.3 Al含量对力学性能的影响
采用同样的方法构造AlxCrFeCuNi高熵合金分子动力学模型，其中各元素的原子比为x∶1∶1∶1∶1，x分别取为0.2、0.5、1.0、2.0、4.0，AlxCrFeCuNi高熵合金中Al原子的摩尔分数
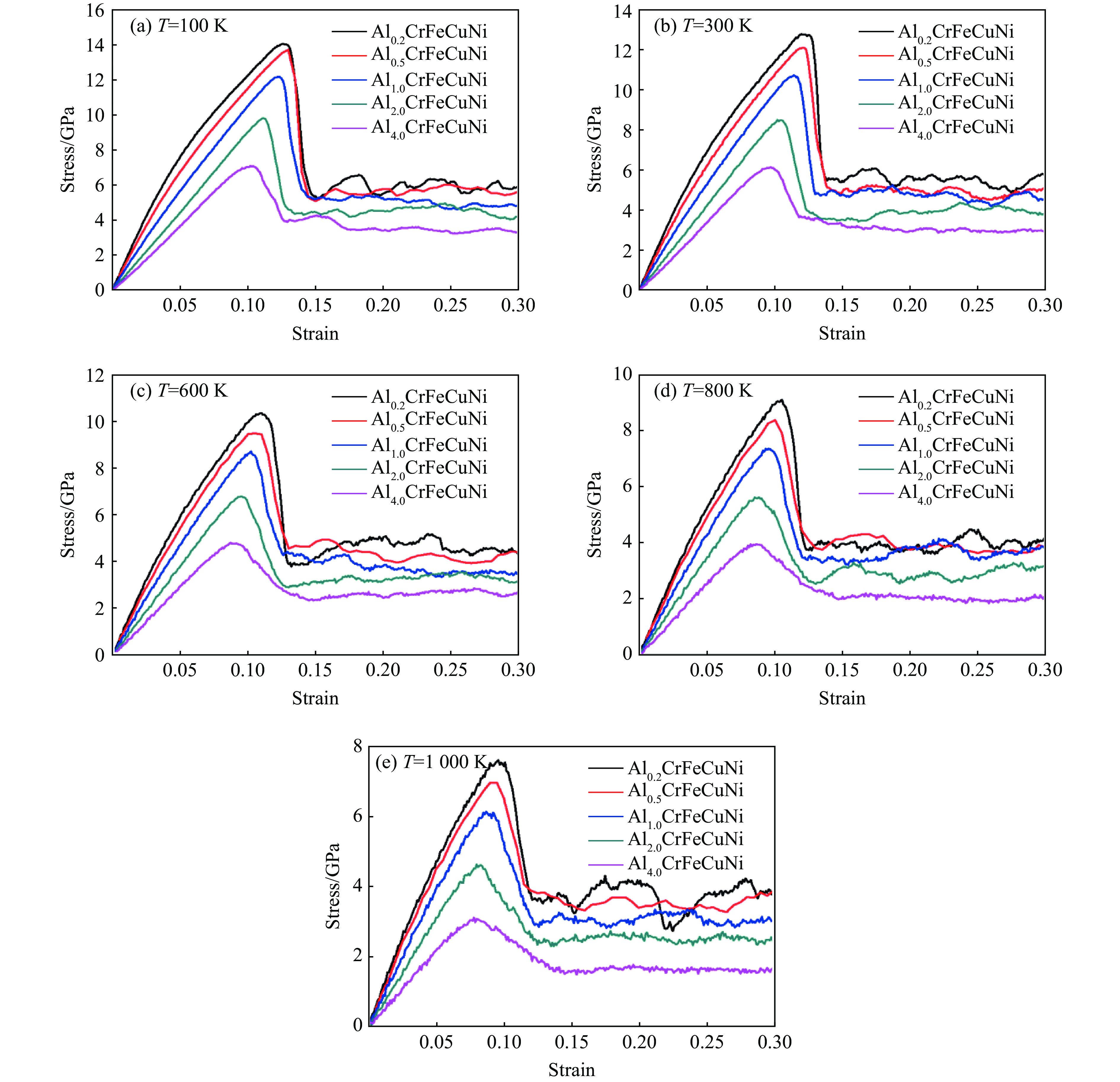
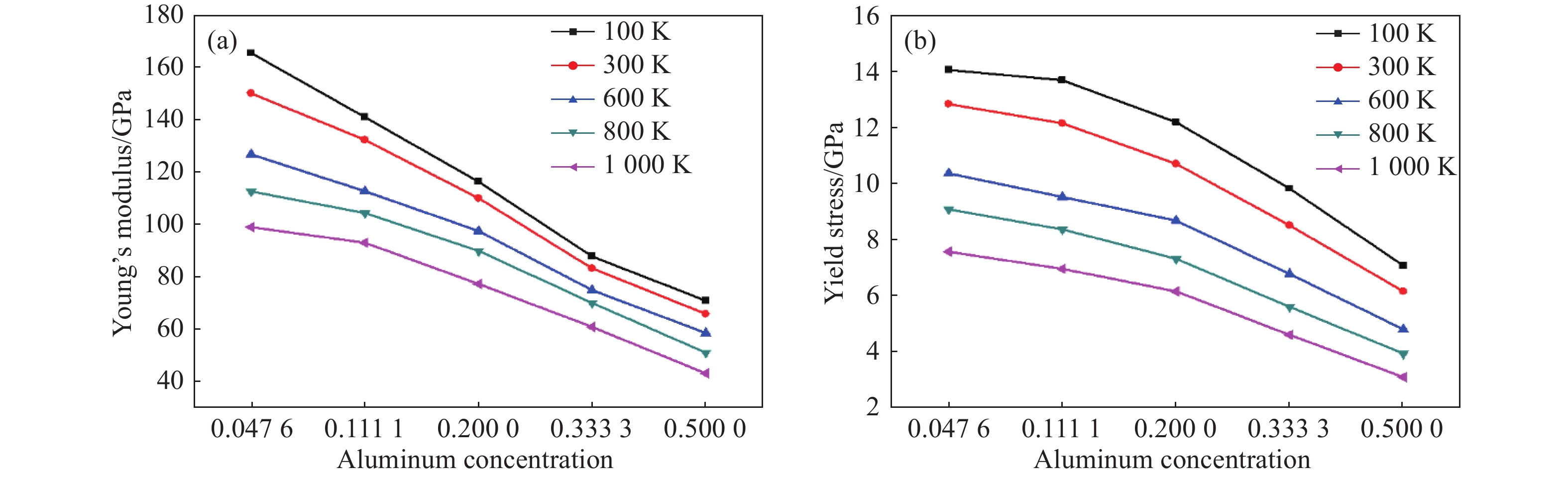
ηAl 和各元素的原子数n如表3所示。表 3 AlxCrFeCuNi高熵合金中Al百分含量和各元素原子个数Table 3. The number of different kinds of atoms of AlxCrFeCuNix ηAl/% n(Al) n(Cr) n(Fe) n(Cu) n(Ni) 0.2 4.76 4 515 23 151 22 920 22 980 22 434 0.5 11.11 10 929 21 528 21 423 21 165 20 955 1.0 20.00 19 428 19 338 19 419 18 870 18 945 2.0 33.33 32 238 16 071 16 284 15 666 15 741 4.0 50.00 47 988 12 000 12 336 12 078 11 598 由于高熵合金在性能上的鸡尾酒效应，不同Al含量的AlxCrFeCuNi高熵合金的力学性能差异较大。在不同温度下，对不同Al含量的AlxCrFeCuNi高熵合金进行分子动力学拉伸模拟，图6给出了在温度T=100, 300, 600, 800, 1 000 K，Al含量x=0.2, 0.4, 1.0, 2.0, 4.0条件下的应力-应变曲线。从图6中可知：在每个温度下，AlxCrFeCuNi高熵合金都经历了弹性变形阶段、屈服阶段和塑性变形阶段，杨氏模量和屈服应力随着Al含量的增加而降低；不论在高温还是低温下，每条曲线从弹性阶段结束到塑性阶段开始都经历了一个明显的下降过程，且下降速率和下降的高度随着材料中Al含量的增加而降低；同时，随着Al含量的增加，峰值应力对应的应变也在降低，表明拉伸载荷作用下材料会在更小的应变处开始屈服，更早地进入塑性阶段。Al含量的增加会导致合金从FCC相向BCC相转变，这一转变增加了合金的自由体积含量，活化剪切带，使其更容易产生变形，进而导致合金的相关力学性能降低。
图 6 不同温度下AlxCrFeCuNi高熵合金的拉伸应力-应变曲线Figure 6. Stress-strain relations of AlxCrFeCuNi under uniaxial tension loading at different temperatures随着温度的提高，AlxCrFeCuNi高熵合金的杨氏模量和屈服应力显著下降。表4详细给出了在不同温度下5种Al含量的AlxCrFeCuNi高熵合金在拉伸载荷作用下的杨氏模量和屈服应力，为了更为直观地研究其变化规律，依据表中数据作图7和图8。
表 4 AlxCrFeCuNi高熵合金在不同温度下的杨氏模量和屈服应力Table 4. Young’s modulus and yield stress of AlxCrFeCuNi at different temperatures and different Al concentrationsT/K Young’s modulus/GPa Yield stress/GPa x=0.2 x=0.5 x=1.0 x=2.0 x=4.0 x=0.2 x=0.5 x=1.0 x=2.0 x=4.0 100 165.61 141.06 116.39 87.83 70.94 14.05 13.68 12.19 9.82 7.08 300 150.16 132.42 110.13 83.28 65.87 12.83 12.15 10.70 8.51 6.16 600 126.82 112.77 97.55 74.98 58.55 10.36 9.52 8.68 6.78 4.81 800 112.70 104.47 89.96 70.05 51.08 9.08 8.37 7.32 5.61 3.94 1 000 99.06 93.09 77.37 60.94 43.13 7.57 6.96 6.16 4.61 3.10 
图 7 不同温度下的杨氏模量(a)和屈服应力(b)Figure 7. Young’s modulus (a) and yield stress (b) at different temperatures
图 8 不同Al含量下的杨氏模量(a)和屈服应力(b)Figure 8. Young’s modulus (a) and yield stress (b) at different Al concentrations从图7中可以得到，随着温度的升高，AlxCrFeCuNi高熵合金的拉伸杨氏模量和屈服应力都近似呈线性下降趋势，与前述研究结果以及Afkhama等[8]研究的AlCrCoFeCuNi高熵合金基本吻合。这证明AlCrFeCuNi高熵合金具有强烈的温度效应，其力学性能会随着温度的升高而产生近似线性下降的变化；Al含量越少，随着温度的升高，杨氏模量和屈服应力的下降幅度越大。
从图8可以看出，AlCrFeCuNi高熵合金的杨氏模量和屈服应力都随着Al含量的增加而降低，且温度越低，下降幅度越大。
4. 结 论
通过模拟高熵合金的实验制备过程构造了AlCrFeCuNi高熵合金的分子动力学模型，使用EAM嵌入势和Morse势描述原子间相互作用，采用分子动力学模拟方法，通过改变模拟盒子大小实现对模型的拉伸加载，研究了AlCrFeCuNi高熵合金的力学性能，得到以下结论。
（1）AlCrFeCuNi1.4高熵合金在拉伸载荷作用下均经历3个变形过程，分别为弹性变形阶段、屈服阶段和不均匀塑性变形阶段。AlCrFeCuNi1.4高熵合金在拉伸过程中表现出超高塑性，从原子结构的变化来看，孪晶和层错的产生和生长是其高塑性的主要原因之一。
（2）分析了温度对AlCrFeCuNi1.4高熵合金拉伸力学性能和变形行为的影响。随着模拟温度的不断升高，AlCrFeCuNi1.4高熵合金的弹性模量、抗拉强度等力学性能均显著减小。这是由于体系温度升高会加剧原子的热运动，更容易造成结构失稳。
（3）在不同温度下研究不同Al含量的AlxCrFeCuNi高熵合金在拉伸载荷作用下的力学性能，发现杨氏模量和屈服应力随着Al含量的增加而降低。AlxCrFeCuNi高熵合金具有很强的温度效应，其杨氏模量和屈服应力随着温度的升高而降低，且下降趋势接近线性。温度越低，Al含量越小，杨氏模量和屈服应力的下降幅度越大。
-
图 2 样品Sa104-1在88 GPa的高压原位（a）和卸载至常温常压（b）的XRD谱 (Bdg、CS、Stv分别代表布里奇曼石、CaCl2型结构的SiO2、斯石英，hcp和bcc分别代表六方密堆积结构和体心立方结构的金属铁。高压下的XRD谱中没有发现相应压力下hcp-Fe [24]的衍射峰。)
Figure 2. XRD patterns of Sa104-1 at 88 GPa (a) and ambient conditions (b), respectively (Bdg=bridgmanite, CS=silica with the CaCl2–type structure, Stv=stishovite, hcp=iron with the hexagonal close-packed structure, bcc=iron with the body-centered cubic structure. Diffraction peaks of the hcp-Fe [24] were not observed in the high-pressure XRD pattern.)
图 3 Sa104-3样品在 36 GPa高压原位（a）和卸载至常温常压（b）的XRD谱（Bdg代表布里奇曼石，Stv代表斯石英，hcp和bcc分别指代六方密堆积结构和体心立方结构的金属铁。高压下或复原至常温常压后所采集的XRD谱中均没有观测到hcp-Fe或bcc-Fe [24]的衍射峰。）
Figure 3. XRD patterns of Sa104-3 at 36 GPa (a) and ambient conditions (b), respectively (Bdg=bridgmanite, CS=silica with the CaCl2–type structure, Stv=stishovite, hcp=iron with the hexagonal close-packed structure, bcc=iron with the body-centered cubic structure. Diffraction peaks of either hcp-Fe or bcc-Fe [24] were not observed in the XRD patterns.)
图 4 在两种不同温压条件下合成的布里奇曼石在卸压过程中的p-V关系：（a）在88 GPa、2 400 K温压条件下合成的Sa104-1样品；（b）在36 GPa、1 850 K温压条件下合成的Sa104-3样品
Figure 4. p-V relations on decompression of two bridgmanite samples synthesized under two different p-T conditions, respectively: (a) sample Sa104-1 synthesized under 88 GPa and 2 400 K; (b) sample Sa104-3 synthesized under 36 GPa and 1 850 K
图 5 在常温常压下(Mg,Fe)SiO3布里奇曼石的晶胞体积与含铁量的关系
Figure 5. Unit-cell volumes of (Mg,Fe)SiO3 bridgmanite as a function of iron content under ambient conditions
表 1 含碳的Mg0.85Fe0.15SiOx凝胶样品合成条件和实验产物
Table 1. Experimental condition and run products of Mg0.85Fe0.15SiOx gel (carbon bearing)
Sample p/GPa T/K Pressure medium Pressure marker Run products In situ Ambient Sa104-1 88(1) 2 400(100) Ne Ne Bdg, CS Bdg, Stv, bcc-Fe Sa82-1 43(1) 2 800(200) SiO2 Au Bdg, Stv Bdg, Stv, bcc-Fe Sa104-2 42(1) 2 000(100) SiO2 Au Bdg, Stv Bdg, Stv, bcc-Fe Sa104-3 35(1) 1 850(100) Ne Ne Bdg, Stv Bdg, Stv Notes: (1) Pressures were determined by the equations of state of Ne or Au[21] after T quench, respectively;
(2) Run products were identified by power XRD under high pressure and ambient conditions, respectively;
(3) Bdg=bridgmanite, CS= silica with CaCl2-type structure, Stv=stishovite, bcc-Fe=metallic iron with body-centered cubic structure.
下载: 导出CSV
表 2 不同条件下合成的布里奇曼石的晶胞参数在卸压中随压力的变化
Table 2. Pressure-dependent unit-cell lattice parameters of bridgmanite synthesized under different pressure-temperature (p-T) conditions
Sample p/GPa a/Å b/Å c/Å V/Å3 Sa104-1 88(1) 4.395(1) 4.624(1) 6.386(2) 129.79(4) 87(1) 4.404(1) 4.624(1) 6.391(1) 130.13(3) 82(1) 4.417(1) 4.640(1) 6.410(1) 131.37(3) 80(1) 4.420(1) 4.646(1) 6.422(2) 131.88(5) 71(1) 4.446(1) 4.673(1) 6.470(3) 134.38(5) Room pressure 4.778(2) 4.929(2) 6.899(3) 162.46(7) Sa82-1 43(1) 4.557(1) 4.742(1) 6.591(1) 142.42(3) Room pressure 4.790(1) 4.922(1) 6.898(4) 162.62(7) Sa104-2 42(1) 4.530(1) 4.707(1) 6.641(1) 141.59(3) Room pressure 4.779(1) 4.932(1) 6.898(1) 162.56(3) Sa104-3 36(1) 4.597(1) 4.773(1) 6.643(1) 145.73(3) 33(1) 4.601(1) 4.793(1) 6.653(1) 146.70(4) 20(1) 4.677(1) 4.836(1) 6.758(2) 152.83(4) 17(1) 4.747(1) 4.828(1) 6.721(2) 153.72(5) 14(1) 4.701(1) 4.868(1) 6.779(1) 155.15(3) Room pressure 4.789(1) 4.938(1) 6.908(1) 163.36(3) Note: (1) Pressures were determined by the equations of state of Ne or Au[21] after T quench;
(2) The data were collected along the decompression path.
下载: 导出CSV
表 3 热状态方程参数
Table 3. Parameters for thermal equation of state
Material V0/(cm3·mol–1) K0/GPa K′0 γ0 q θ /K Ref. FeSiO3(Bdg) 25.400 272 4.1 1.44 1.4 765 [47] FeO(B1) 12.256 146.9 4.0 1.42 1.3 380 [42] SiO2(Stv) 14.017 302 5.24 1.71 1.0 1 109 [48] SiO2(CS) 14.017 341 3.2 2.14 1.0 1 109 [48] Note: (1) V0–volume at ambient conditions, K0–bulk modulus, K′0–pressure derivative of K0, γ0–Grüneisen parameter at ambient conditions, q–logarithmic volume derivative of the Grüneisen parameter, θ–Debye temperature;
(2) K0 and K′0 are parameters for Birch-Murnaghan equation of state, and γ0, q0 and θ are parameters used for Mie-Grüneisen relation.
下载: 导出CSV
-
[1] FROST D J, MCCAMMON C A. The redox state of Earth’s mantle [J]. Annual Review of Earth and Planetary Sciences, 2008, 36(1): 389–420. doi: 10.1146/annurev.earth.36.031207.124322 [2] STAGNO V, FROST D J. Carbon speciation in the asthenosphere: experimental measurements of the redox conditions at which carbonate-bearing melts coexist with graphite or diamond in peridotite assemblages [J]. Earth and Planetary Science Letters, 2010, 300(1/2): 72–84. [3] LITASOV K D, SHATSKIY A. Carbon-bearing magmas in the Earth’s deep interior [M]//Magmas Under Pressure. Amsterdam: Elsevier, 2018: 43–82. [4] DASGUPTA R, HIRSCHMANN M M. Melting in the Earth’s deep upper mantle caused by carbon dioxide [J]. Nature, 2015, 440(7084): 659–662. [5] ROHRBACH A, SCHMIDT M W. Redox freezing and melting in the Earth’s deep mantle resulting from carbon-iron redox coupling [J]. Nature, 2011, 472(7342): 209–212. doi: 10.1038/nature09899 [6] DASGUPTA R, HIRSCHMANN M M. The deep carbon cycle and melting in Earth’s interior [J]. Earth & Planetary Science Letters, 2010, 298(1/2): 1–13. [7] SAAL A E, HAURI E H, LANGMUIR C H, et al. Vapour undersaturation in primitive mid-ocean-ridge basalt and the volatile content of Earth’s upper mantle [J]. Nature, 2002, 419(6906): 451–455. doi: 10.1038/nature01073 [8] HIRSCHMANN M M, DASGUPTA R. The H/C ratios of Earth’s near-surface and deep reservoirs, and consequences for deep Earth volatile cycles [J]. Chemical Geology, 2009, 262(1/2): 4–16. [9] DASGUPTA R. Ingassing, storage, and outgassing of terrestrial carbon through geologic time [J]. Reviews in Mineralogy and Geochemistry, 2013, 75(1): 183–229. doi: 10.2138/rmg.2013.75.7 [10] HAZEN R M, DOWNS R T, JONES A P, et al. Carbon mineralogy and crystal chemistry [J]. Reviews in Mineralogy and Geochemistry, 2013, 75(1): 7–46. doi: 10.2138/rmg.2013.75.2 [11] LEUNG I S. Silicon carbide cluster entrapped in a diamond from Fuxian, China [J]. American Mineralogist, 1990, 75(9/10): 1110–1119. [12] SCHRAUDER M, NAVON O. Solid carbon dioxide in a natural diamond [J]. Nature, 1993, 365(6441): 42–44. doi: 10.1038/365042a0 [13] KAMINSKY F. Mineralogy of the lower mantle: a review of ‘super-deep’ mineral inclusions in diamond [J]. Earth-Science Review, 2012, 110(1/2/3/4): 127–147. doi: 10.1016/j.earscirev.2011.10.005 [14] SMITH E M, SHIREY S B, NESTOLA F, et al. Large gem diamonds from metallic liquid in Earth’s deep mantle [J]. Science, 2016, 354(6318): 1403. doi: 10.1126/science.aal1303 [15] STAGNO V, TANGE Y, MIYAJIMA N, et al. The stability of magnesite in the transition zone and the lower mantle as function of oxygen fugacity [J]. Geophysical Research Letters, 2011, 38(19): 570–583. [16] MAEDA F, OHTANI E, KAMADA S, et al. Diamond formation in the deep lower mantle: a high-pressure reaction of MgCO3 and SiO2 [J]. Scientific Reports, 2017, 7: 40602. doi: 10.1038/srep40602 [17] LI X, ZHANG Z, LIN J F, et al. New high pressure phase of CaCO3 at the topmost lower mantle: Implication for the deep mantle carbon transportation [J]. Geophysical Research Letters, 2018, 45: 1355–1360. doi: 10.1002/2017GL076536 [18] MARTIROSYAN N S, LITASOV K D, LOBANOV S S, et al. The Mg-carbonate-Fe interaction: implication for the fate of subducted carbonates and formation of diamond in the lower mantle [J]. Geoscience Frontiers, 2019, 10(4): 1449–1458. doi: 10.1016/j.gsf.2018.10.003 [19] DORFMAN S M, BADRO J, NABIEI F, et al. Carbonate stability in the reduced lower mantle [J]. Earth and Planetary Science Letters, 2018, 489: 84–91. doi: 10.1016/j.jpgl.2018.02.035 [20] HAMILTON D L. The preparation of silicate compositions by a gelling method [J]. Mineralogical Magazine, 1968, 36(282): 832–838. doi: 10.1180/minmag.1968.036.282.11 [21] YINGWEI F, ANGELE R, MARK F, et al. Toward an internally consistent pressure scale [J]. Proceedings of the National Academy of Sciences of the United States of America, 2007, 104(22): 9182–9186. doi: 10.1073/pnas.0609013104 [22] CAMPBELL A J, DANIELSON L, RIGHTER K, et al. High pressure effects on the iron-iron oxide and nickel-nickel oxide oxygen fugacity buffers [J]. Earth and Planetary Science Letters, 2009, 286(3/4): 556–564. [23] MENG Y, HRUBIAK R, ROD E, et al. New developments in laser-heated diamond anvil cell with in situ synchrotron X-ray diffraction at High Pressure Collaborative Access Team [J]. Review of Scientific Instruments, 2015, 86(7): 072201. doi: 10.1063/1.4926895 [24] PRAKAPENKA V B, KUBO A, KUZNETSOV A, et al. Advanced flat top laser heating system for high pressure research at GSECARS: application to the melting behavior of germanium [J]. High Pressure Research, 2008, 28(3): 225–235. doi: 10.1080/08957950802050718 [25] HOLLAND T J B, REDFERN S A T. Unit cell refinement from powder diffraction data: the use of regression diagnostics [J]. Mineralogical Magazine, 1997, 61(404): 65–77. doi: 10.1180/minmag.1997.061.404.07 [26] BOFFA-BALLARAN T, KURNOSOV A, GLAZYRIN K, et al. Effect of chemistry on the compressibility of silicate perovskite in the lower mantle [J]. Earth and Planetary Science Letters, 2012, 333/334: 181–190. doi: 10.1016/j.jpgl.2012.03.029 [27] DORFMAN S M, MENG Y, PRAKAPENKA V B, et al. Effects of Fe-enrichment on the equation of state and stability of (Mg,Fe)SiO3 perovskite [J]. Earth and Planetary Science Letters, 2013, 361(1): 249–257. [28] KUDOH Y, PREWITT C T, FINGER L W, et al. Effect of iron on the crystal structure of (Mg,Fe)SiO3 perovskite [J]. Geophysical Research Letters, 1990, 17(10): 1481–1484. doi: 10.1029/GL017i010p01481 [29] FEI Y, WANG Y, FINGER L W. Maximum solubility of FeO in (Mg, Fe)SiO3-perovskite as a function of temperature at 26 GPa: implication for FeO content in the lower mantle [J]. Journal of Geophysical Research Solid Earth, 1996, 101(B5): 11525–11530. doi: 10.1029/96JB00408 [30] LUNDIN S, CATALLI K, J. SANTILLÁN, et al. Effect of Fe on the equation of state of mantle silicate perovskite over 1 Mbar [J]. Physics of the Earth and Planetary Interiors, 2008, 168(1): 97–102. [31] ITO E, YAMADA H. Stability relations of silicate spinels, ilmenites, and perovskites [M]//High Pressure Research in Geophysics. Tokyo: Center for Publication, 1982: 405-419. [32] MAO H K, HEMLEY R J, FEI Y, et al. Effect of pressure, temperature, and composition on lattice parameters and density of (Fe,Mg)SiO3-perovskites to 30 GPa [J]. Journal of Geophysical Research: Solid Earth, 1991, 96(B5). [33] WANG Y, WEIDENER D J, LIEBERMANN R C, et al. P-V-T equation of state of (Mg, Fe)SiO3 perovskite: constraints on composition of the lower mantle [J]. Physics of the Earth and Planetary Interiors, 1996, 83(1): 13–40. [34] FIQUET G, ANDRAULT D, DEWAELE A, et al. P-V-T, equation of state of MgSiO3, perovskite [J]. Physics of the Earth & Planetary Interiors, 1998, 105(1/2): 21–31. [35] TANGE Y, TAKAHASHI E, NISHIHARA Y, et al. Phase relations in the system MgO-FeO-SiO2 to 50 GPa and 2 000 ℃: an application of experimental techniques using multianvil apparatus with sintered diamond anvils [J]. Journal of Geophysical Research Solid Earth, 2009, 114(B2): 1–14. [36] ANDRAULT D, BOLFAN-CASANOVA N, GUIGNOT N. Equation of state of lower mantle (Al,Fe)-MgSiO3 perovskite [J]. Earth and Planetary Science Letters, 2001, 193(3/4): 501–508. [37] FROST D J, LIEBSKE C, LANGENHORST F, et al. Experimental evidence for the existence of iron-rich metal in the Earth’s lower mantle [J]. Nature, 2004, 428(6981): 409–412. doi: 10.1038/nature02413 [38] MCCAMMON C A. The crystal chemistry of ferric iron in Fe0.05Mg0.95SiO3 perovskite as determined by Mössbauer spectroscopy in the temperature range 80–293 K [J]. Physics & Chemistry of Minerals, 1998, 25(4): 292–300. [39] MCCAMMON C A, LAUTERBACH S, SEIFERT F, et al. Iron oxidation state in lower mantle mineral assemblages: I. empirical relations derived from high-pressure experiments [J]. Earth and Planetary Science Letters, 2004, 222(2): 435–449. doi: 10.1016/j.jpgl.2004.03.018 [40] IOTA V, YOO C S, CYNN H. Quartzlike carbon dioxide: an optically nonlinear extended solid at high pressures and temperatures [J]. Science, 1999, 283(5407): 1510–1513. doi: 10.1126/science.283.5407.1510 [41] TSCHAUNER O, MAO H K, HEMLEY R J. New Transformations of CO2 at high pressures and temperatures [J]. Physical Review Letters, 2001, 87(7): 075701. doi: 10.1103/PhysRevLett.87.075701 [42] LITASOV K D, GONCHAROV A F, HEMLEY R J. Crossover from melting to dissociation of CO2 under pressure: implications for the lower mantle [J]. Earth & Planetary Science Letters, 2011, 309(3/4): 318–323. [43] BOATES B, TEWELDEBERHAN A M, BONEV S A. Stability of dense liquid carbon dioxide [J]. Proceedings of the National Academy of Sciences, 2012, 109(37): 14808–14812. doi: 10.1073/pnas.1120243109 [44] TEWELDEBERHAN A M, BOATES B, BONEV S A. CO2 in the mantle: melting and solid–solid phase boundaries [J]. Earth and Planetary Science Letters, 2013, 373: 228–232. doi: 10.1016/j.jpgl.2013.05.008 [45] DZIUBEK K F, MARTIN E, DEMETRIO S, et al. Crystalline polymeric carbon dioxide stable at megabar pressures [J]. Nature Communications, 2018, 9(1): 3148. doi: 10.1038/s41467-018-05593-8 [46] HOLLAND T J B, POWELL R. An improved and extended internally consistent thermodynamic dataset for phases of petrological interest, involving a new equation of state for solids [J]. Journal of Metamorphic Geology, 2011, 29: 333–383. doi: 10.1111/j.1525-1314.2010.00923.x [47] XU W, LITHGOW-BERTELLONI C, STIXRUDE L, et al. The effect of bulk composition and temperature on mantle seismic structure [J]. Earth and Planetary Science Letters, 2008, 275(1/2): 70–79. [48] FISCHER R A, CAMPBELL A J, CHIDESTER B A, et al. Equations of state and phase boundary for stishovite and CaCl2-type SiO2 [J]. American Mineralogist, 2018, 103(5): 792–802. doi: 10.2138/am-2018-6267 [49] NAKAJIMA Y, FROST D J, RUBIE D C. Ferrous iron partitioning between magnesium silicate perovskite and ferropericlase and the composition of perovskite in the Earth’s lower mantle [J]. Journal of Geophysical Research Solid Earth, 2012, 117: B08201. [50] FROST D J, WOOD B J. Experimental measurements of the fugacity of CO2 and graphite/diamond stability from 35 to 77 kbar at 925 to 1 650 ℃ [J]. Geochimica et Cosmochimica Acta, 1997, 61(8): 1565–1574. doi: 10.1016/S0016-7037(97)00035-5 [51] WILDING M C, HARTE B, HARRIS J W. Evidence for a deep origin for Sao Luiz diamonds [C]//Fifth International Kimberlite Conference, 1991. [52] KLEIN-BENDAVID O, WIRTH R, NAVON O. Micrometer-scale cavities in fibrous and cloudy diamonds: a glance into diamond dissolution events [J]. Earth and Planetary Science Letters, 2007, 264(1/2): 89–103. doi: 10.1016/j.jpgl.2007.09.004 [53] VAN DER HILST R D, WIDIYANTORO S, ENGDAHL E R. Evidence for deep mantle circulation from global tomography [J]. Nature, 1997, 386(6625): 578–584. doi: 10.1038/386578a0 [54] STAGNO V, OJWANG D O, MCCAMMON C A, et al. The oxidation state of the mantle and the extraction of carbon from Earth’s interior [J]. Nature, 2013, 493(7430): 84–88. doi: 10.1038/nature11679 [55] HIROSE K, TAKAFUJI N, SATA N, et al. Phase transition and density of subducted MORB crust in the lower mantle [J]. Earth and Planetary Science Letters, 2005, 237(1/2): 239–251. [56] HIROSE K, FEI Y, MA Y, et al. The fate of subducted basaltic crust in the Earth’s lower mantle [J]. Nature, 1999, 397(397): 53–56. -
下载:
下载:
点击查看大图
计量
- 文章访问数: 11245
- HTML全文浏览量: 3051
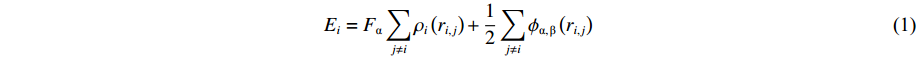
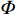
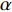
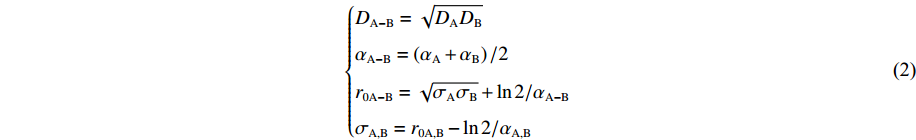
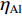
- PDF下载量: 65
