p-α and p-λ Model for Describing Shock Compressive Behavior of W-Cu Powder Mixture
-
摘要: 研究了3种p-
α 模型和p-λ 模型在预测非均质W-Cu混合粉末冲击压实响应的适用性。利用Mie-Grüneisen方法和Barry等压混合法,基于单质W、Cu粉末的Hugoniot关系预测了同孔隙度的W-Cu混合粉末的Hugoniot线,在高压段与实验结果符合较好,但在低压段与实验偏差较大。分别应用3种p-α 模型和p-λ 模型对实验结果进行拟合,发现除p-α PL模型外,其他模型均较好地描述W-Cu混合粉末的冲击压缩响应,受经验参数选择的影响,所有模型的压溃强度和压缩路径各不相同,预测功能较差。-
关键词:
- W-Cu混合粉末 /
- 冲击压缩 /
- p-α模型 /
- Hugoniot测量
Abstract: This study investigated the applicability of three p-α models and p-λ model for predicting shock compaction response of heterogeneous W-Cu powder mixture. Mie-Grüneisen method and Barry isobaric mixing method were employed to predict the Hugoniot of W-Cu powder mixture with the same porosity based on the Hugoniot relationships. At high pressure section, the results were in good agreement with the experimental results, but it deviated greatly at the low pressure section. The p-α models and p-λ model were applied to fit the experimental results, and it was found that all the other models were able to describe the shock compression response of W-Cu powder mixture except p-α PL model. The crush strength and compression path of all models are different due to selection of empirical parameters, and they are with poor prediction function.-
Key words:
- W-Cu powder mixture /
- shock compressive /
- p-α model /
- Hugoniot measurement
-
多孔材料和粉末材料的冲击压缩一直是重点研究方向。早期研究主要通过多孔或粉末材料的冲击压缩,获得在固体材料中无法获得的热力学状态,以研究完全物态方程,此类研究关注高压区域,忽略粉末材料的自身强度[1-2]。粉末的冲击压实利用冲击波作用于非晶或纳米晶粉末,在瞬态高温高压下得到近乎致密的块体,且仍保持原始晶粒尺寸,是制备亚稳态材料的一种非常有潜力和前景的方法。为了避免高压导致的高温和裂纹问题,往往在1~10 GPa的低压范围内实现压实,因此,粉末在低压区的致密化行为对于粉末冲击压实研究非常重要。
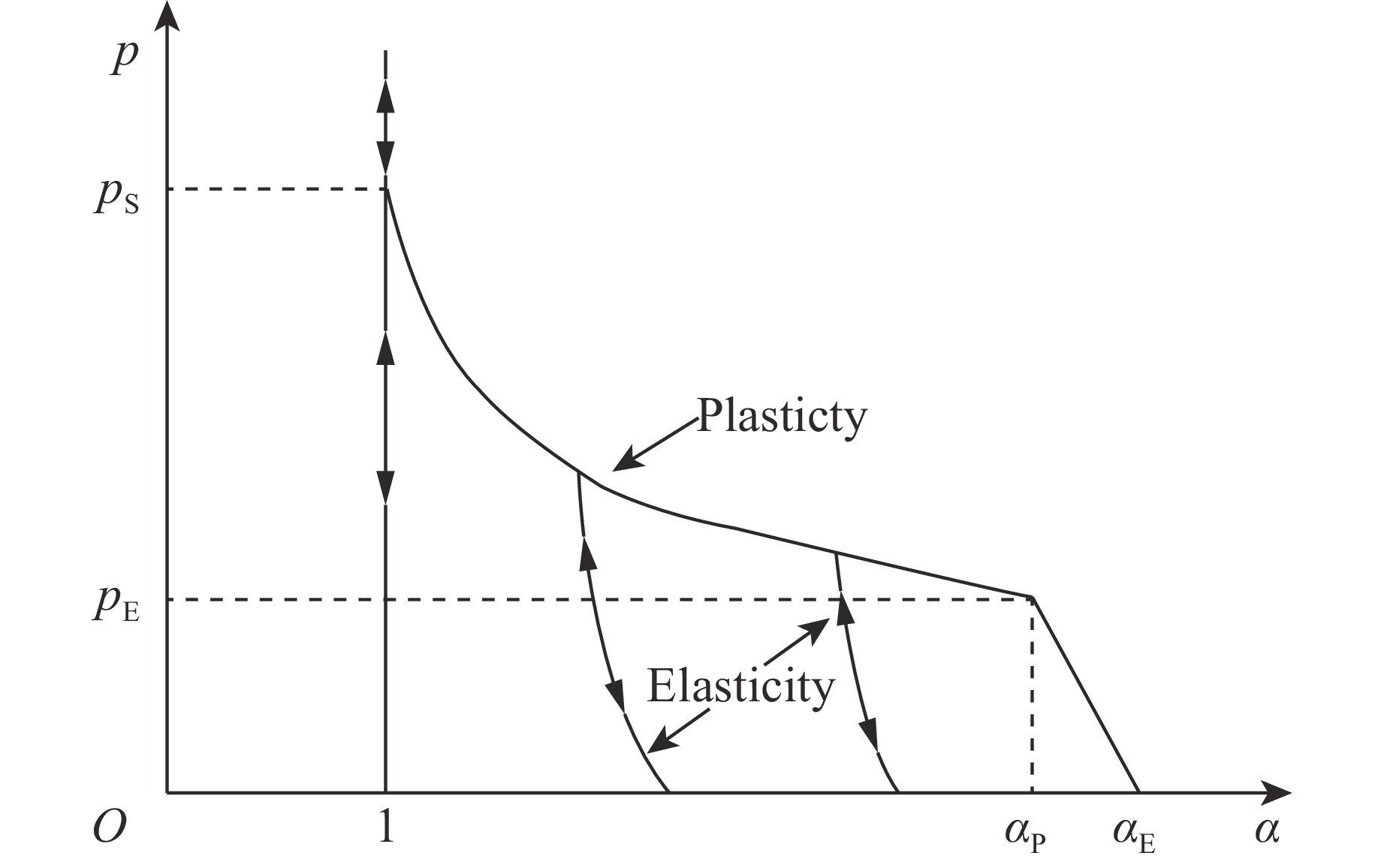
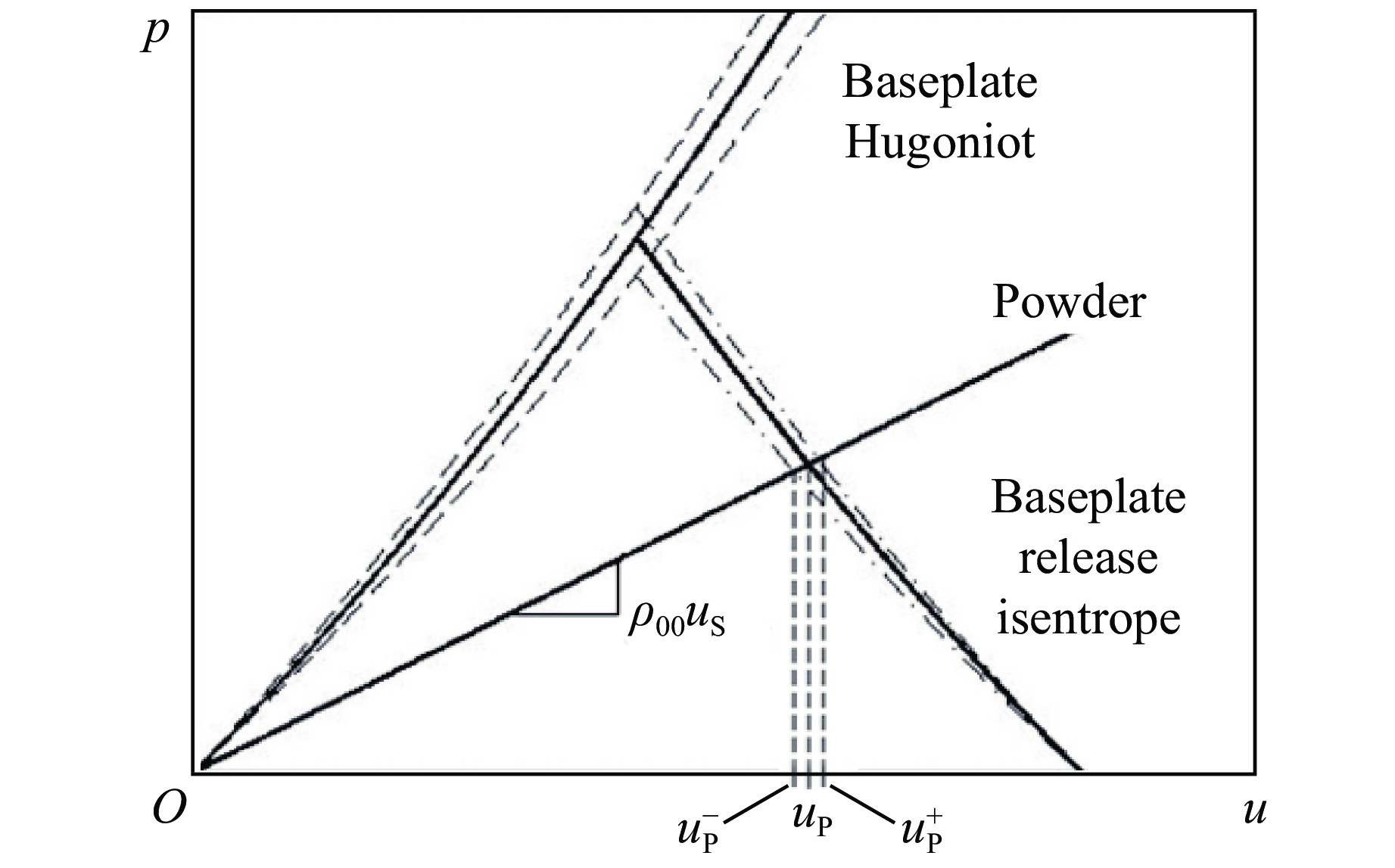
粉末在低压区的冲击压实是一个复杂的过程,受到多种因素的影响,包括粉末材料的弹塑性、初始颗粒的大小和形状等[3]。为了在较宽的压力范围内更好地描述粉末的压实过程,一般将这一过程分解为弹性段和塑性段,如图1所示,其中:
αE 、pE为弹性段结束时的孔隙度和压力,由于在整个压缩过程中,弹性段仅占很小部分,一般都忽略不计,多数模型取αE=α0 ;当压力超过pE后,进入塑性段,随着颗粒形变和孔隙坍缩,孔隙率逐渐降低,压力达到pS时,粉末完全压实,pS也被称为压溃强度。目前已经有较多模型描述粉末压实响应,但存在两个假设前提:(1)忽略了剪切强度;(2)在同等压力、温度条件下,粉末和固体材料的比内能是相等的。假设(1)使得模型只能描述由于孔隙闭合导致的不可逆压实;假设(2)忽略了孔隙和颗粒的表面能,更适合描述颗粒较大的粉末体系。在众多描述颗粒或粉末材料动态压实行为的模型中,以p-
α 模型[4-7]和p-λ 模型[8]应用最为广泛,是典型的描述型模型,这类模型结合了粉末材料本征特性,通过实验数据拟合获得模型的关键参数。尽管模型的具体形式不同,但都包含一个或者多个经验拟合参数。通常情况下这些参数与可测量的物理参数没有直接关系,只是为了使模型更好地与实验数据匹配,这种灵活性使模型可以很好地描述某种特定粉末系统的冲击压实过程。对于由不同性质粉末组成的混合物,粉末冲击压实过程变得更加复杂。目前并没有有效的方法建立混合粉末冲击压缩方程,一般可通过0 K混合法则[9-10]或质量分数加权平均法则[11-12]先得到混合固体Hugoniot线,再经等容Mie-Grüneisen方法计算得到粉体的冲击压缩方程,但这种方法的计算过程繁琐,需要专门程序计算,而且其有效性缺乏实验验证。
本研究测量了W-Cu混合粉末的Hugoniot数据,系统分析了测量误差;然后分别应用p-
α 和p-λ 等模型对测量数据进行拟合;利用Mie-Grüneisen方法[13]得到同孔隙度的W、Cu单质粉末的Hugoniot线,按照Barry等压混合法则[14]预测同孔隙度的W-Cu混合粉末的Hugoniot线,并与测量得到的数据进行对比,探讨p-α 和p-λ 等模型在描述混合粉末冲击压缩行为时的适用性和经单质粉末Hugoniot线预测混合粉末Hugoniot线的可行性。1. 实验测试
1.1 W-Cu混合粉末Hugoniot测量实验装置
实验使用的W和Cu单质粉末均为市购,纯度均为99.9%,粉末粒度均为
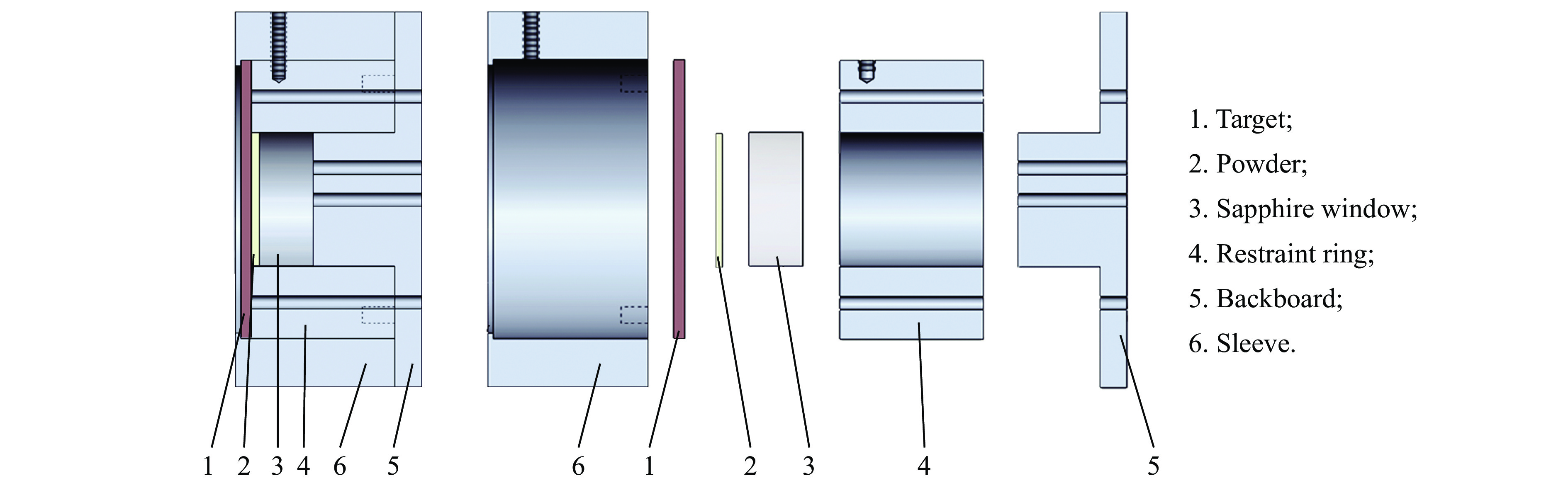
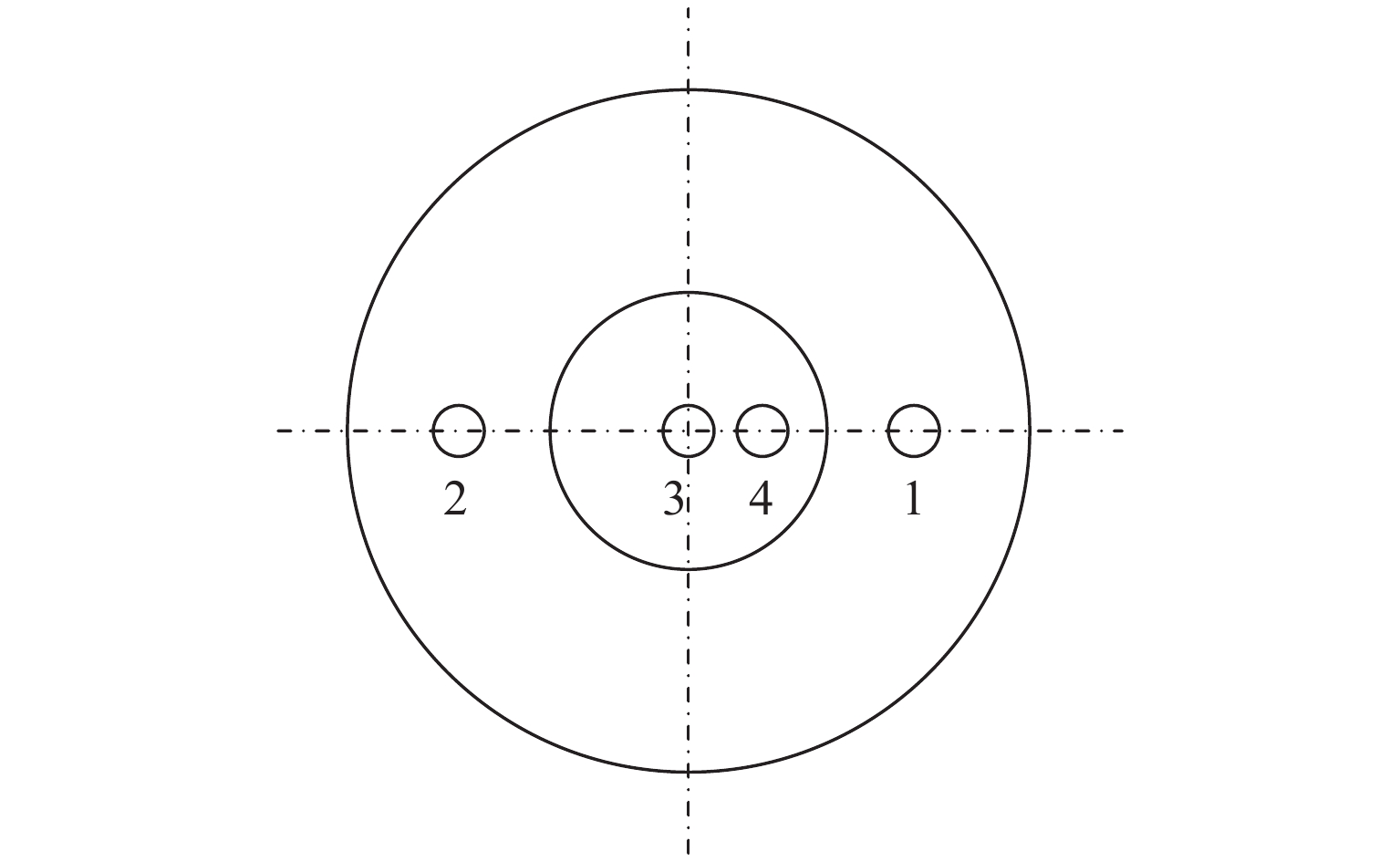
1μm 。W、Cu粉按照质量比76∶24混合,经V型混料机混合均匀得到W-Cu混合粉末。图2为实验测量所用装置的结构示意图,将无氧铜基板和约束环依次安装于中空的304不锈钢套筒后,形成一个容器,再依次装入W-Cu混合粉末和蓝宝石窗口,并通过背板确保粉末后表面与窗口材料紧密接触。实验使用二级轻气炮进行加载,飞片使用与基板同材质的无氧铜,应用光纤位移干涉仪(Displacement interferometer system for any reflector,DISAR)[15],通过基板背面和窗口背面设置的4个光纤探头,分别记录冲击波抵达粉末样品上下表面的时间,探头布置的空间位置如图3所示。2. 参数测量及误差分析
2.1 粉末初始密度
粉末初始密度
ρ00 主要通过调整粉体厚度来控制,粉末厚度由组装件整体厚度减去基板中心、蓝宝石窗口及背板厚度计算得到。厚度测量精度为10–4 mm,粉末质量称量精度为10–3 g。每组取多次测量的平均值,并在初始密度的不确定度分析中加入了每个位置测量的方差。粉末中存在局部的不均匀性,但无法确定不均匀部位的位置和范围,因此初始孔隙密度ρ00 的测量误差取最大值,即直接取粉体厚度测量的最大值和最小值来确定。初始密度的测量误差会在阻抗匹配计算中传递,对Hugoniot参数(uP, p和V)的测量误差影响较大。2.2 飞片速度
飞片速度vI由炮口的电磁线圈测量得到,该系统的测量误差为0.5%。由于飞片在炮膛中会发生偏转,形成的倾角可由图3中1、2号探针确定后扣除。
2.3 冲击波速度
冲击波速度uS根据冲击波分别到达图3中1、2号探针和3、4号探针记录的时间和粉末厚度计算得出,即
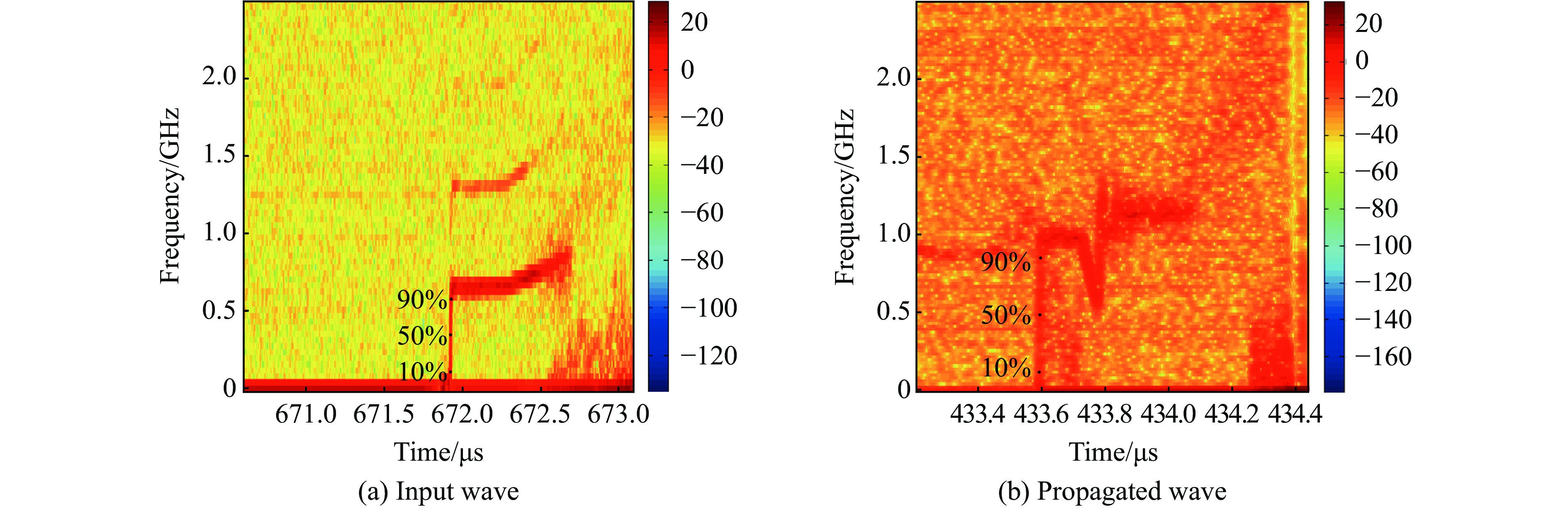
uS=∆xi/∆ti ,其中∆xi 和∆ti 分别是粉末厚度和冲击波传播时间。粉末厚度的标准差为δ(∆xi)=(δx2B+δx2W)1/2 ,其中xB表示基板位移,xW表示粉末厚度[16]。∆ti 即冲击波分别抵达1、2号探针和3、4号探针的时间差,其标准差为δ(∆ti) 。图4所示为粉体上下表面处探针的DISAR信号。由于飞片飞行过程中会发生偏转,1、2号探针信号存在时间差,也会导致冲击波速度测量误差,这一误差也体现在δ(∆ti) 中。在两个标准差范围内一个实验的平均冲击速度总误差为 图 4 典型的入射波(a)和传播波(b)的DISAR轮廓曲线(显示了结构化波形,以及50%的均衡到达时间和10%、90%的不确定到达时间,用于计算冲击波速度)Figure 4. Typical extracted profile for input (a) and propagated waves (b) that illustrating structured waveforms (Locations of 50% equilibrium time of arrival and 10% and 90% uncertainty arrival times used for calculation of shock velocity are also marked.)
图 4 典型的入射波(a)和传播波(b)的DISAR轮廓曲线(显示了结构化波形,以及50%的均衡到达时间和10%、90%的不确定到达时间,用于计算冲击波速度)Figure 4. Typical extracted profile for input (a) and propagated waves (b) that illustrating structured waveforms (Locations of 50% equilibrium time of arrival and 10% and 90% uncertainty arrival times used for calculation of shock velocity are also marked.)δuSuS=[∑i2[δ(∆xi)∆xi]2+∑i2(δ(∆ti)∆ti)2]12 (1) 2.4 粒子速度
粉末的粒子速度uP使用阻抗匹配法计算,计算原理和误差分布如图5所示。根据文献[17],粒子速度的总误差是系统误差和实验误差之和,即
δuP=δuEP+δuSP 。粉末粒子速度的系统误差是δuP=[(δuSP,I−B)2+(δuSP)2]1/2 (2) 式中:
uSP,I−B 表示靶板Hugoniot误差,uSP 表示靶板等熵线上的误差。实验误差为δuEP={[δuP(uS)]2+[δuP(vI)]2+[δuP(ρ00)]2+[δuP(ρ0I)]2[δuP(ρ0B)]2}1/2 (3) 式中:
ρ0I 和ρ0B 分别是飞片和基板的初始密度。2.5 压 力
压力的初始值p0和粉末初始速度u0等于零,则粉末中的压力p可直接由下式计算
p=ρ0(uS−u0)uP (4) 压力的误差
δp/p 也包括系统误差和实验误差。由于p是ρ00 、uS、uP的函数,因此,压力p的系统误差为δσσ=δρ00ρ00+δuSuS+δuPuP (5) 实验误差为[17]
(δσσ)E={(1+uSuP∂uP∂uS)2(∂uSuS)2+(1+ρ00uP∂uP∂ρ00)2(∂ρ00ρ00)2+[∂uP(v1)uP]2+(∂uP(ρ0I)uP)[∂uP(ρ0I)uP]2+(∂uP(ρ0B)uP)[∂uP(ρ0B)uP]2}12 (6) 2.6 冲击压缩比容
粉末中的冲击压缩比容V由
V=V0[1−(uP−u0)/(uS−u0)] 计算得到,其中V=1/ρ 。V的系统误差为(δV/V)S=(η−1)|δuSP|/uP ,其中η 是粉末压缩度,η=ρ/ρ00 。实验误差是(δVV)E={(η−1)2(1−uSuP∂uP∂uS)2(∂uSuS)2+[1+(η−1)ρ00uP∂uP∂ρ00]2(∂ρ00ρ00)2+(η−1)2F2} (7) 式中:
F2=[∂uP(V1)uP]2+[∂uP(ρ0I)uP]2+[∂uP(ρ0B)uP]2。 2.7 测量结果分析
实验测量的所有Hugoniot参数及其误差范围如表1所示。可以看到uS的误差较大,主要是粉体厚度测量误差较大所致,也导致粉末初始密度和压缩密度误差均接近10%。压力超过4 GPa后,粉体已接近致密。
表 1 实验相关参数计算结果及误差范围Table 1. Calculated results and errors of the related experimental parametersNo. ρ00/(g·cm–3) vI/ (km·s–1) uS/(km·s–1) uP/(km·s–1) p/GPa ρ/(g·cm–3) 1 10.696±0.910 0.622±0.003 1.254±0.041 0.457±0.019 6.126±0.434 16.826±1.122 2 10.484±0.731 0.270±0.001 0.913±0.055 0.213±0.013 2.038±0.223 13.668±0.536 3 10.707±1.229 0.784±0.004 1.549±0.089 0.546±0.028 9.056±0.913 16.639±1.324 4 10.237±1.370 0.508±0.003 1.107±0.030 0.388±0.016 4.392±0.211 15.752±1.370 3. 模型拟合
3.1 p-
α 模型p-
α 模型被广泛用于描述均质和非均质粉末的弹塑性压实响应[18]。其中α=V/VS 是一个描述粉末膨胀程度的参数,V是粉末的比容,VS是相应固体材料在相同温度T和压力p下的比容。p-α 模型具有不同的表达形式,本研究仅关注其中最常见的3种。3.1.1 二次改进模型(Modified quadratic,MQ)
p-
α 模型常用于描述动态冲击加载下塑性多孔材料的冲击特性。当应力范围较大时,要分解为弹性和塑性两部分。此模型中忽略了剪切强度的存在,并且假设在等温等压下,多孔材料和固体材料的内能相等。在低压力段,弹性压实响应决定压实行为和从部分压实状态下的释放过程。通常初始的弹性状态αE 很小,在计算中经常会忽略,或设为αE=α0 直到达到阈值压力。塑性变形中的
α 是对Butcher和Karnes方法[6]的二次改进。一般公式如下α=1+(αE−1)(pS−ppS−pE)N (8) 式中:N是经验拟合参数,可以视实验具体情况改变。
3.1.2 静态球面公式(Static spherical,SS)
Carroll和Holt[7]通过将多孔材料中孔洞的坍塌用一个由相同密度的空心球的压实相平衡来补充p-
α 模型。假设应力张量为球形,只考虑压力p。静态球形公式可对压实响应进行预测,因此压实响应由材料性质和孔洞的几何形状决定。假设固体材料不可压缩,将孔洞坍塌分为3个区域。在最初的弹塑性区,观察到在压力达到临界值前孔隙度基本没有发生变化。这使压实过程可以得到简化:在压实过程的前两个区域,压力小于pE时孔隙率的变化可以忽略不计。pE的大小使用下式计算pE=23Ylnα0α0−1 (9) 式中:Y是固体材料的屈服强度,
α0 是初始孔隙度。压力大于pE时为第3个阶段,材料塑性变形,压实由孔洞坍塌造成,此时的孔洞坍塌关系可以用下式简化α=(1−e−3p/2Y)−1 (10) 静态球面的p-
α 模型只考虑了与速率无关的屈服特性,因此可能不适用于高速率敏感粉体材料的压实响应,而且此模型忽略了颗粒形态可能造成的影响。3.1.3 幂律公式(Power law,PL)
Brown等[19]的p-
α 模型与前两种不同,他们用同一种机制描述弹塑性压实,直接描述了脆性微粒系统的动态压实,并没有将弹塑性机制分开研究。幂律公式的表达式如下α=(PS/p)1n (11) 式中:PS是压碎强度,n是经验参数。
3.2 p-
λ 模型p-
λ 模型明确地将单个成分的物质属性包含在一个非均匀混合物中[20]。与p-α 模型类似,该模型使用单内部状态变量λ描述压实过程并忽略粉末混合物的剪切强度,只考察了一般的p-V响应。该模型分为3个不同的区域:一端为不平衡的弹性响应,另一端为单个分量的压力平衡状态,两者之间为过渡区。假设压力较小时变形是弹性的。使用两种不同的附加模型定义混合物的体积弹性模量K和弹性响应:等压和等应变。等压响应是混合模量的下限,等应变响应是上限。在选择合适的混合模量后,通过应变的定义确定了非平衡弹性区混合物的p-V响应;在原方程的基础上,提出了一个简单的质量分数加性方程。在过渡区,假定材料以非平衡弹性状态和压力平衡状态的某种组合形式存在。材料在给定压力值下的相对量通过变量k来分配,k定义为完全压实状态下的物质质量分数。λ取值0~1。当材料完全处于不平衡的弹性状态下λ = 0;当材料处在压力平衡状态时,λ = 1。λ的具体函数关系为λ=1−e−(p/Y)n (12) 式中:p是组件的局部压力差异;Y是固体的屈服强度,当混合物屈服时,会同时在混合物中引发屈服和压实;n无法从单个组件属性中进行定义。
3.3 拟合结果
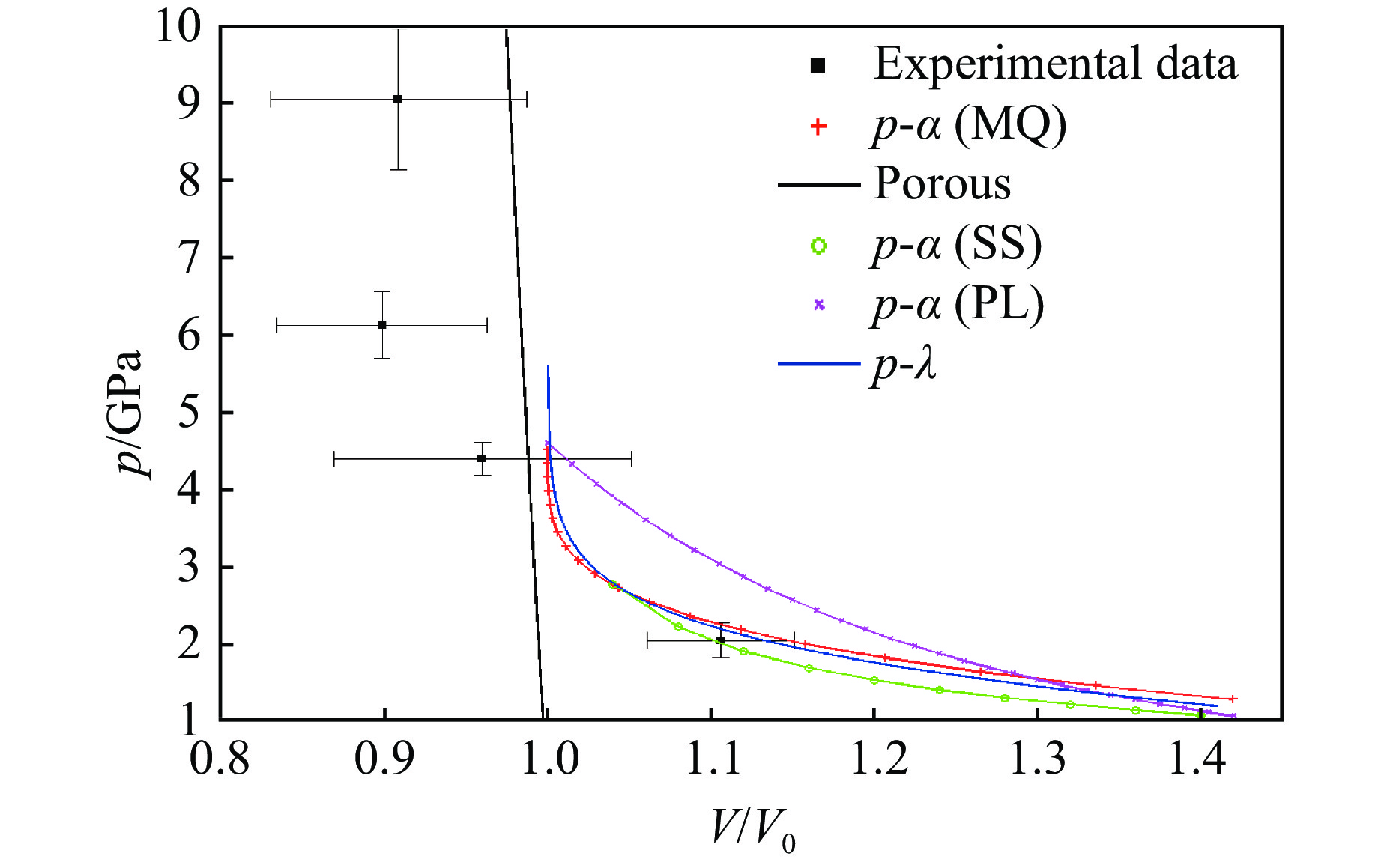
为了检验测量数据与模型拟合的精度,必须给出混合粉末的Hugoniot线,当压力超过粉体的致密压力后,测量数据点应该落在计算的粉末Hugoniot线上。本研究粉末的Hugoniot线使用等容Mie-Grüneisen方法计算得到。首先,基于W、Cu固体Hugoniot数据计算得到相同孔隙度的W、Cu粉体Hugoniot线,再经由Barry提出的两相混合Hugoniot原理计算得到W-Cu混合粉末的Hugoniot线。该原理主要存在两个基本假设:
(1)各组分压力相等;
(2)
V(p)=∑ni=1miVi(p) ,EC(V)=∑ni=1miECi(V) ,∑ni=1mi=1 ,EC是材料比内能。对于p-
α 模型,孔隙度α 可由N和n的值经拟合得到,pE是从弹性压缩转为塑性压缩的临界值,αE 是相应的孔隙度,pS由实验数据和Mie-Grüneisen粉末状态方程的交点确定。p-λ 模型中的曲线拟合与上述方法类似。混合物的屈服强度Y采用体积分数平均法计算。压力计算基于飞片和靶板材料的状态方程(EOS)。所用参数在表2中给出,其中C0和S是线性冲击状态方程uS=C0+SuP参数 , γ0是Grüneisen常数。符合最佳拟合结果的数据在表3中给出。表 2 组分和混合物的相关冲击和材料特性Table 2. Relevant shock and material properties of constituents and mixturesMaterial ρ0/(g·cm–3) C0/(km·s–1) S γ0 V0/(cm3·g–1) Y/GPa W 19.35 4.064 1.204 1.78 0.052 0 1.700 Cu 8.93 3.910 1.510 1.97 0.112 1 0.065 W-Cu 15.117 4.027 1.277 1.83 0.066 4 1.030 表 3 模型拟合参数Table 3. Model fitting parametersModel Parameters pS/GPa pE/GPa αE N p-α(MQ) 4.60 0.057 7 1.359 1.952 p-α(PL) pS/GPa n 4.60 7.80 p-α(SS) Y/GPa 1.273 4 p-λ n 1.51 图6为多个模型的拟合结果。可以看到粉末在4~5 GPa压力下达到致密,压力继续升高后,数据点落到计算得到的粉体Hugoniot线上。除p-
α PL模型外,其他模型均较好地描述了W-Cu混合粉末的冲击压缩响应。但在孔隙度接近零时,预测结果均与计算的粉末Hugoniot线有一定偏差,这是由于Mie-Grüneisen方法忽略了材料强度和实验初始孔隙度测量误差所致。p-α MQ和p-α PL模型中都存在压溃强度pS,经拟合得到pS= 4.6 GPa;参数N影响曲线的曲率,N取值大则曲率增加,p-α PL模型受N取值影响较大,拟合效果较差。p-α SS模型仅受粉末的屈服强度Y的影响,完全忽略了粉末的微观尺度效应(颗粒形状和大小),但得到了较好的拟合结果,预测的压溃强度pS为4~5 GPa,与p-α MQ和p-α PL模型一致。p-λ 模型也可以较好地描述低压段的冲击压实响应,但孔隙度无限接近于零时,在屈服强度Y一定的前提下,参数n的取值决定了曲线的曲率,当n减小时,曲率减小,pS急剧增大,而Y的取值影响pE的值。由于低于4 GPa的数据点仅有一个,所以很难确定其余模型哪个更适合描述W-Cu混合粉末的冲击压缩响应。拟合过程中发现,所有模型通过参数调整都可以获得较好的拟合效果,是典型的描述型模型,预测性较差。如p-α MQ和p-α PL模型中的参数pS无法通过实验测量,p-α SS和p-λ 模型中的Y受混合颗粒特性(如颗粒的尺寸、颗粒级配和颗粒形状等)的影响显著,无法准确预测。此外,除p-α PL模型外,其余模型均能很好地描述粉末压缩中由弹性转塑性的行为,而p-α PL模型经参数调整后也可以描述这一转变,但其曲线曲率变小,如图6所示,与实验数据差别较大。4. 结 论
测量了W-Cu混合粉末的Hugoniot数据,系统分析了测量误差,发现粉末致密度测量精度对结果影响较大。利用Mie-Grüneisen方法和Barry等压混合法,可以利用单质W、Cu粉末的Hugoniot关系很好地预测同孔隙度W-Cu混合粉末的Hugoniot线,但由于忽略了粉体强度和粉体初始密度测量误差较大的原因,预测结果在低压段与实验偏差较大。分别应用3种p-
α 模型和p-λ 模型对实验结果进行拟合,发现除p-α PL模型外,其他模型均能较好地描述W-Cu混合粉末的冲击压缩响应,表明这些原本用于描述单质粉末的冲击压实响应的模型对于更为复杂的混合粉末也是适用的,但所有模型的拟合精度均受经验参数选择的影响,预测能力较差。 -
图 4 典型的入射波(a)和传播波(b)的DISAR轮廓曲线(显示了结构化波形,以及50%的均衡到达时间和10%、90%的不确定到达时间,用于计算冲击波速度)
Figure 4. Typical extracted profile for input (a) and propagated waves (b) that illustrating structured waveforms (Locations of 50% equilibrium time of arrival and 10% and 90% uncertainty arrival times used for calculation of shock velocity are also marked.)
表 1 实验相关参数计算结果及误差范围
Table 1. Calculated results and errors of the related experimental parameters
No. ρ00/(g·cm–3) vI/ (km·s–1) uS/(km·s–1) uP/(km·s–1) p/GPa ρ/(g·cm–3) 1 10.696±0.910 0.622±0.003 1.254±0.041 0.457±0.019 6.126±0.434 16.826±1.122 2 10.484±0.731 0.270±0.001 0.913±0.055 0.213±0.013 2.038±0.223 13.668±0.536 3 10.707±1.229 0.784±0.004 1.549±0.089 0.546±0.028 9.056±0.913 16.639±1.324 4 10.237±1.370 0.508±0.003 1.107±0.030 0.388±0.016 4.392±0.211 15.752±1.370 表 2 组分和混合物的相关冲击和材料特性
Table 2. Relevant shock and material properties of constituents and mixtures
Material ρ0/(g·cm–3) C0/(km·s–1) S γ0 V0/(cm3·g–1) Y/GPa W 19.35 4.064 1.204 1.78 0.052 0 1.700 Cu 8.93 3.910 1.510 1.97 0.112 1 0.065 W-Cu 15.117 4.027 1.277 1.83 0.066 4 1.030 表 3 模型拟合参数
Table 3. Model fitting parameters
Model Parameters pS/GPa pE/GPa αE N p-α(MQ) 4.60 0.057 7 1.359 1.952 p-α(PL) pS/GPa n 4.60 7.80 p-α(SS) Y/GPa 1.273 4 p-λ n 1.51 -
[1] ALTSHULER L V, KRUPNIKOV K K, LEDENEV B N, et al. Dynamic compressibility and equation of state for iron under high pressure [J]. Soviet Physics-JETP, 1958: 34. [2] MCQUEEN R G, MARSH S P, TAYLOR J W, et al. The equation of state of solids from shock wave studies [M]. Los Alamos, New Mexico: University of California, 1970: 293–417. [3] FREDENBURG D A. Shock compaction and impact response of thermit powder mixtures [D]. Georgia: Georgia Institute of Technology, 2010. [4] HERRMANN W. Constitutive equation for the dynamic compaction of ductile porous materials [J]. Journal of Applied Physics, 1969, 40(6): 2490–2499. doi: 10.1063/1.1658021 [5] DAI C D, EAKINS D E, THADHANI N N. Dynamic densification behavior of nanoiron powders under shock compression [J]. Journal of Applied Physics, 2008, 103(9): 093503. doi: 10.1063/1.2908209 [6] BUTCHER B M, KARNES C H. Dynamic compaction of porous iron [J]. Journal of Applied Physics, 1969, 40(7): 2967–2976. doi: 10.1063/1.1658109 [7] CARROLL M M, HOLT A C. Static and dynamic pore‐collapse relations for ductile porous materials [J]. Journal of Applied Physics, 1972, 43(4): 1626–1636. doi: 10.1063/1.1661372 [8] GRADY D, KERLEY E G I, KUHNS L D, et al. Computational modeling and wave propagation in media with inelastic deforming microstructure [J]. Journal de Physique, IV: Proceedings of International Conference, 2000, 10(9): 15–20. [9] MEYERS M A. Shock waves: equations of state [M]. John Wiley & Sons, Inc., 2007. [10] MCQUEEN R G, MARSH S P. Equation of state for nineteen metallic elements from shock‐wave measurements to two megabars [J]. Journal of Applied Physics, 1960, 31(7): 1253–1269. doi: 10.1063/1.1735815 [11] ALEKSEEV Y F, AL’TSHULER L V, KRUPNIKOVA V P. Shock compression of two-component paraffin-tungsten mixtures [J]. Journal of Applied Mechanics Technical Physics, 1971, 12(4): 624–627. [12] BATSANOV S S. Effects of explosions on materials [M]. New York: Springer, 1994. [13] MEYERS M A. Dynamic Behavior of Materials [M]. San Diego: University of California, 1994. [14] KRUEGER B R, MUTZ A H, VREELAND T. Correlation of shock initiated and thermally initiated chemical reactions in a 1∶1 atomic ratio nickel‐silicon mixture [J]. Journal of Applied Physics, 1991, 70(10): 5362–5368. doi: 10.1063/1.350217 [15] WENG J, TAN H, WANG X, et al. Optical-fiber interferometer for velocity measurements with picosecond resolution [J]. Applied Physics Letters, 2006, 89(11): 111101. doi: 10.1063/1.2335948 [16] FREDENBURG D A, KOLLER D D, RIGG P A, et al. High-fidelity Hugoniot analysis of porous materials [J]. Review of Scientific Instruments, 2013, 84(1): 013903. doi: 10.1063/1.4774394 [17] MITCHELL A C, NELLIS W J. Shock compression of aluminum, copper, and tantalum [J]. Journal of Applied Physics, 1981, 52(5): 3363–3374. doi: 10.1063/1.329160 [18] FREDENBURG D A, THADHANI N N. On the applicability of the P- α and P-λ models to describe the dynamic compaction response of highly heterogeneous powder mixtures [J]. Journal of Applied Physics, 2013, 113(4): 043507. doi: 10.1063/1.4788700[19] BROWN J L, VOGLER T J, GRADY D E, et al. Dynamic compaction of sand [C]//Shock Compression of Condensed Matter-2007, 2007: 1363–1366. [20] NEEL C H. Shock compression of a heterogeneous, porous polymer composite [J]. Dissertations & Theses-Gradworks, 2010. -













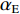
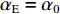

 下载:
下载:
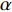
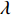
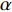
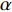
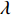
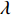
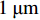



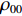
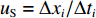

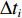
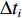
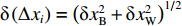


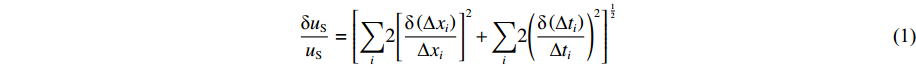
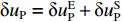

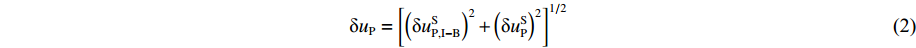

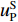
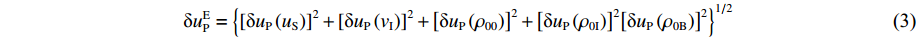


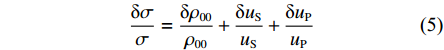

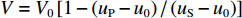
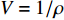

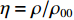
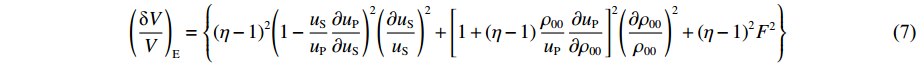
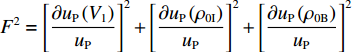

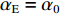
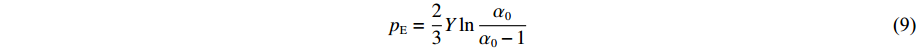

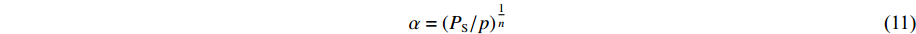

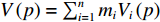

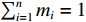
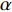
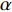
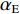
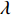
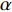
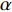

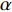
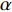
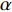
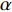
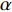
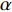
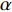
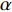
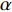
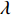
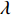

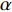
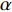
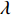

 下载:
下载: