Progress of Aluminum Projectile Impacting on Plate with Hypervelocity
-
摘要: 以空间碎片防护为背景,回顾了超高速铝弹丸正撞击单层和双层铝合金防护结构的研究进展,讨论了目前针对超高速撞击的弹丸发射技术和数值模拟方法(如Euler方法、无网格方法等)的优缺点。数值模拟不仅建立在离散方法上,还需要提供准确的材料本构模型和状态方程。介绍了常用材料模型(包括Johnson-Cook、Steinberg-Guinan模型)和状态方程(包括Tillotson、ANEOS、SESAME、GRAY三相状态方程)。基于实验和数值模拟,目前对7 km/s以下的超高速撞击物理过程已经认识得比较清楚。对单层板,重点讨论了板的穿孔特征和孔径模型;对双层板,除了前板的穿孔外,还讨论了碎片云的分布特征、材料相变、碎片云的相态分布、弹丸形状的影响、碎片云的散布模型以及碎片云对后板造成的破坏特征。最后介绍了工程防护中较为重要的防护结构的弹道极限方程。单层板和双层板的弹道极限方程研究近年来取得了较大进展。本文回顾了国内外常用的弹道极限方程以及近年来新提出的理论模型和基于人工神经网络的模型等。Abstract: This paper focuses on the protection of debris clouds in space and the progress of the studies of Al projectile impacting on single shield and Whipple shields are discussed. The advantages and drawbacks of the widely used experimental method for launching hypervelocity projectile and numerical methods for hypervelocity impact such as Euler methods and meshfree methods are introduced. The numerical simulation is usually based on the constitutive laws and the equation of states. In this paper we have reviewed the constitutive law including Johnson-Cook and Steinberg-Gruinan, while for the equation of states we include the Tillotson, ANEOS, SESAME, GRAY. The mechanics and physics for the hypervelocity impact below 7 km/s are now well understood based on the progresses made by the experiments and numerical simulations. Then, for the single shield we mainly focus on the perforation by the projectile and the models predicting the hole size. For the Whipple shield we have discussed the characteristics of the debris clouds evolution, the phase states of the materials, the models predicting the evolution of the debris clouds and damage features of the second wall of the Whipple structure induced by the debris clouds. Finally we discussed the ballistic limit equations which are very important to the protection in the engineering. Great progresses have been achieved for the ballistic equations for the single and double shields structures based on the experiments and numerical simulations. We have discussed the commonly used ones and the models which are newly developed recently including theoretical models and the models from artificial intelligence.
-
Key words:
- debris clouds /
- hypervelocity impact /
- ballistic limit equation
-
复合材料是一种性能优良、可设计性强的新型材料,具有比强度、比模量高等优点,被广泛应用于航空航天、汽车制造、风力发电叶片以及压力容器等领域[1-3]。其中玻璃纤维增强环氧树脂基壳体的应用率较高,也是近年来的研究重点[4-5]。用于管道和压力容器等的复合材料壳体因承受较大的力学载荷,壳体内部会发生基体开裂、纤维与基体脱粘、层合板分层和纤维基体同时断裂等损伤,损伤部位产生应力集中,当应力大于材料的许用应力后,会对整个系统的安全构成潜在的威胁[6-8]。因此,通过研究应力、应变等重要参数从而理解复合材料结构壳体的力学响应行为,对提高含有断裂缺陷的复合材料的安全性能具有重要意义[9-11]。
目前,科研人员对复合材料壳体的损伤已进行了大量的有限元模拟研究,通过建立渐进损伤有限元模型预测了复合材料壳体的损伤失效过程。Leh等[12]建立了连续和渐进损伤两种有限元模型,对纤维缠绕压力容器的爆破压力进行预测,与试验结果相比,渐进损伤模型能够更好地模拟复合材料储氢容器的爆破过程。Wang等[13]将材料递减规则和基于表面行为单元结合建立渐进损伤模型,预测了铝内胆碳纤维缠绕复合材料储氢气瓶的极限承载能力和复杂的失效行为。Liu等[14]对3种不同尺寸的碳纤维缠绕压力容器进行失效分析,采用Hashin失效准则并基于能量损伤演变规律,得到与实验结果相符的失效性质参数和爆破强度。杨斌等[15]为了分析混杂复合材料层合板在冲击载荷下的损伤演变过程,利用有限元分析软件Abaqus建立了预测复合材料层合板在低速冲击作用下损伤的三维有限元模型。
还有很多学者采用应变片或光纤埋入复合材料结构以实时监测的实验方法,研究了复合材料层合板和壳体在外压作用下的应变响应过程。Okabe等[16]采用光纤布拉格光栅传感器识别碳纤维增强塑料层合板的裂纹位置,将光纤光栅传感器嵌入层合板交叉层中,并在拉伸载荷作用下测量反射光谱,从测量的光谱判断裂纹位置,证明了小直径光纤光栅传感器也可用于裂纹位置的识别。肖飚等[17]在玻璃纤维缠绕金属内胆复合材料压力容器的制备过程中,将应变传感器埋在金属内胆与玻璃纤维/环氧树脂复合材料层之间,得到了具有原位监测功能的纤维缠绕压力容器。Kanerva等[18]原位测试了碳纤维增强复合材料的层间变化,结果表明埋入应变片可以精准监测复合材料的层间状态。应变参数可以有效反映复合材料层合板及壳体的受力状态,而将有限元模拟和应力和应变响应结合考虑复合材料壳体的损伤行为从而判断整个压力容器健康情况的相关研究还很少。开展含有断裂缺陷的复合材料壳体的力学行为分析,能够为复合材料壳体检测及容器的安全检测提供一定的数据基础。
本研究将在玻璃纤维缠绕环氧树脂基压力容器的圆柱体上截取试样,通过拉伸、双悬臂梁(DCB)和三点端部开口弯曲(3ENF)试验对复合材料层合板进行基本力学性能评估,编写进用于判断材料失效的VUMAT用户子程序中,并采用有限元软件Abaqus分析不同深度断裂缺陷对复合材料压力容器应力、应变的影响。
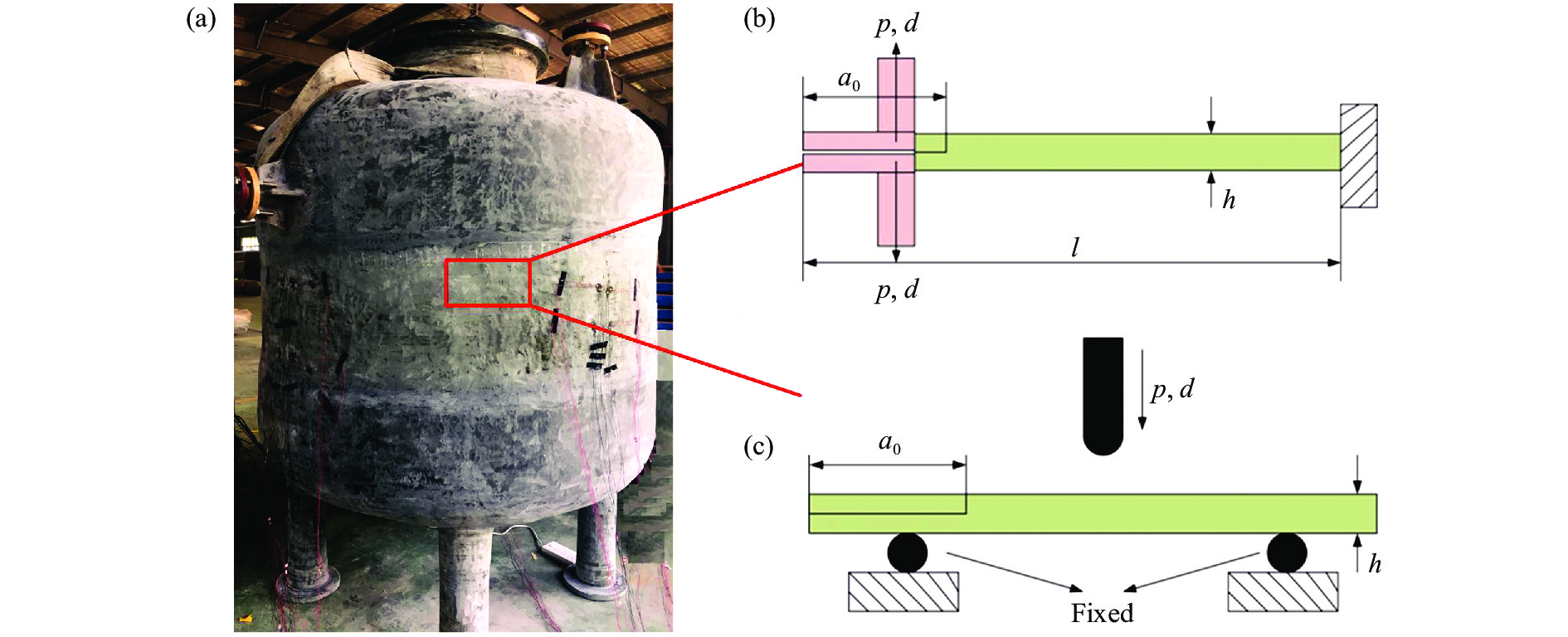
1. 试样制备
将圆柱形模具固定在旋转操作箱上,使旋转操作箱不停转动,将含有环氧树脂预浸料的玻璃纤维缠绕在模具表面,缠绕到一定厚度后脱模,形成完整的压力容器,如图1(a)所示。该容器的内径、高度和厚度分别为1600、1620和18 mm,容积为3 m3,设计压力为0.375 MPa。玻璃纤维部分包括玻璃纤维缠绕线层(Y)、布层(C)和毡层(M),3种纤维层的铺层顺序为M/Y/C/Y/C/Y/C/M/Y/C/Y/C/Y/C/M。按照ASTM D3039/D3039M-08标准,采用水切割方法在压力容器筒体段的0°、90°和45°方向截取试样,试样尺寸分别为220 mm × 25 mm × 18 mm、220 mm × 25 mm × 18 mm和160 mm × 25 mm × 18 mm,每种试样各取5份。
根据裂纹产生原因不同,复合材料可以划分为3种分层模式:Ⅰ型断裂又称张开型裂纹,由与层合板面垂直的力产生,分层扩展方向与外载荷方向垂直,Ⅱ型和Ⅲ型分别称滑移型断裂和撕开型断裂,与面内剪应力有关,分别由平行和垂直于裂纹扩展方向的面内力产生。考虑到复合材料压力容器是由内压而产生的力学行为特点,主要考虑Ⅰ型和Ⅱ型两种基本断裂模式,测量这两种类型分层的断裂韧性,从而确定该材料的层间性能。根据ISO 15024标准、ASTM D5528-13标准和航空工业HB7402-1996标准,采用DCB试验方法测量I型复合材料的层间断裂韧性,如图1(b)所示。试样采用单向层板,厚度(h)为15 mm,长度(l)为220 mm,预制分层长度(a0)为25 mm,试样宽度(b)为25 mm。根据ASTM D7905标准、日本JSAK7086标准和中国航空工业HB7403-1996标准,采用3ENF试验方法测定Ⅱ型复合材料的分层断裂韧性,如图1(c)所示。试样采用单向层板,厚度(h)为15 mm,长度(l)为100 mm,预制分层长度(a0)为25 mm,试样宽度(b)为10 mm。
2. 基本力学性能测试
力学性能测试在INSTRON-8032电液伺服动态万能材料疲劳试验机上进行,试验机的最大载荷为100 kN,测试速度为2 mm/min。通过拉伸试件测试获得试样的刚度参数:横向拉伸模量Ex、纵向拉伸模量Ey、泊松比vxy和剪切模量Gxy。由于所取试样的纤维方向与其主方向的夹角θ为12°,通过偏轴公式式(1)~式(4)得到最终的刚度参数:纤维主方向拉伸模量E1、与纤维垂直方向拉伸模量E2、泊松比v12和剪切模量G12。
1Ex=1E1cos4θ+(1G12−2v12E1)sin2θcos2θ+1E2sin4θ (1) 1Ey=1E1sin4θ+(1G12−2v12E1)sin2θcos2θ+1E2cos4θ (2) −vxyEx=−v12E1(sin4θ+cos4θ)+(1E1+1E2−1G12)sin2θcos2θ (3) 1Gxy=1G12(sin4θ+cos4θ)+4(1+2v21E1+1E2−12G12)sin2θcos2θ (4) 根据修正梁理论,DCB试验中Ⅰ型分层试样的层间应变能释放率
RⅠ\!\!c=3Fpcd2b(a+Δ) (5) 其中
F=1−310(da)2−23(dl1a2) (6) 式中:pc为试样端部施加的载荷,d为端部位移即分层开口位移,a为分层长度,F为考虑大位移和加载块影响时的修正因子,Δ为分层长度的修正量。对于Ⅱ型分层,应变能释放率
RⅡ\!c=9a20pcd2b(38l30+3a30) (7) 式中:l0为弯曲加载跨距的一半,d为端部位移即弯曲试样中心挠度。试样采用预制分层的单向层板,通过加载平台施加载荷。
3. 有限元模拟方法
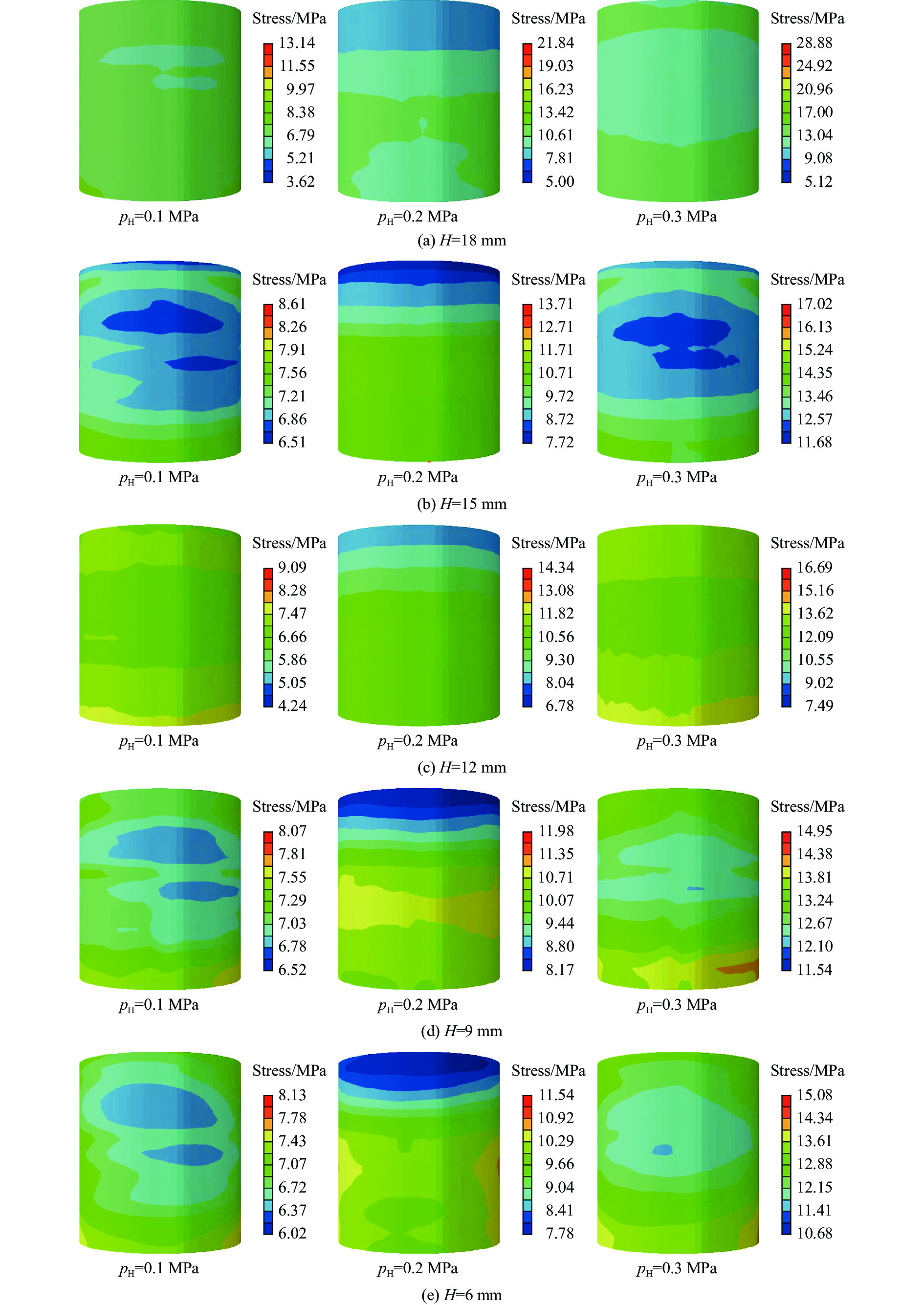
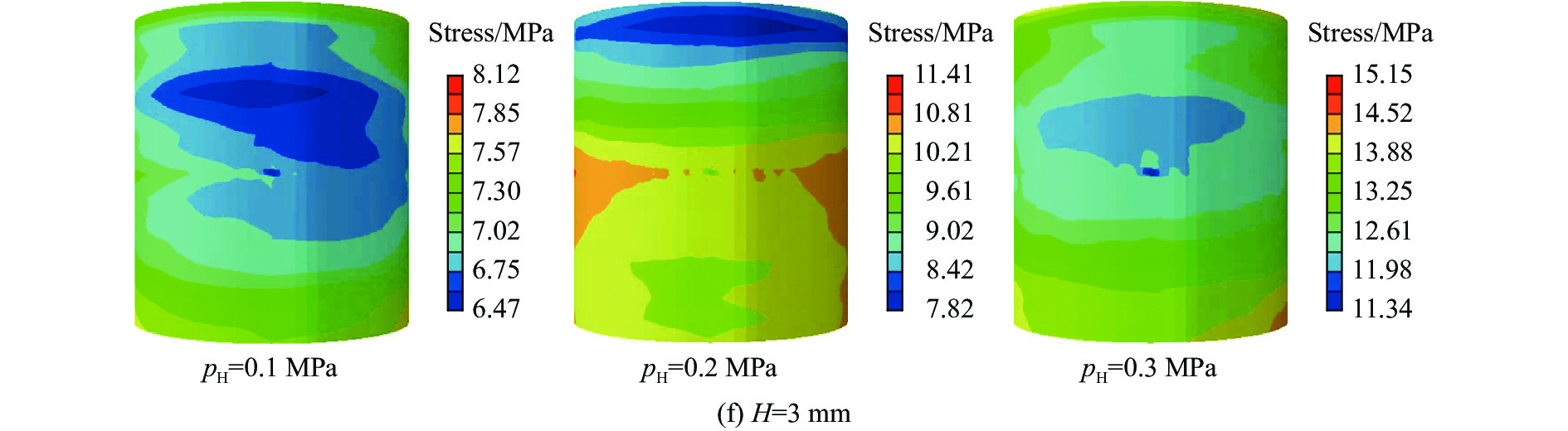
如图2所示,利用ABAQUS建立含有不同深度断裂缺陷的复合材料壳体的有限元分析模型,预测其在内压载荷作用下的应力和应变响应。同时,编写用于定义材料参数及判断材料失效的VUMAT用户子程序,采用Hashin 3D失效准则,并考虑材料的刚度递减规律,参数的选取参考文献[19]。复合材料壳体的厚度为18 mm,根据纤维的缠绕工艺特点,将复合材料壳体分为6层。断裂缺陷的长度、宽度和厚度分别为50、2和3 mm。第1层断裂缺陷深度为18 mm,第2层为15 mm,第3层为12 mm,第4层为9 mm,第5层为6 mm,第6层为3 mm。内部压力分别设定为0.1、0.2和0.3 MPa。
4. 结果与讨论
玻璃纤维增强环氧树脂基复合材料的刚度和强度参数列于表1和表2中,表中数据为5个试样的平均值和方差。表中:E1、E2和E3分别为纤维方向、垂直纤维方向和试样厚度方向的拉伸模量,G12、G13和G23为3个面内的剪切模量,v12、v13和v23为3个面内的泊松比,XT和XC分别为纤维方向的拉伸和压缩强度,YT和YC分别为垂直纤维方向的拉伸和压缩强度,ZT和ZC分别为试样厚度方向的拉伸和压缩强度,S12、S13和S23表示3个面内的剪切强度,其中厚度方向的相关参数取自文献[20-21]。可以看到,复合材料主向的拉伸强度为(222.7 ± 18)MPa,弹性模量为39.39 GPa,横向的拉伸强度为(136 ± 22)MPa,弹性模量为18.1 GPa,层间强度Ⅰ型和Ⅱ型断裂韧性分别为(4.67 ± 0.24)kJ/m2和(4.98 ± 0.26)kJ/m2。
图3为不同深度断裂损伤的应力分布结果,H为断裂深度,pH为内压。如图3(a)所示,当断裂损伤深度为18 mm,内压载荷为0.1 MPa时,应力范围为3.621~13.140 MPa;内压载荷为0.2 MPa时,应力范围为5.002~21.840 MPa;内压载荷为0.3 MPa时,应力范围为5.116~28.880 MPa。最大应力分布在筒体的底端,主要是由于此处不仅受到内压载荷的作用,还受到位移的限制,并承受着筒体的整体重力。含有不同断裂深度壳体的应力结果均有此特点。如图3(b)所示,当断裂损伤深度为15 mm,内压载荷为0.1、0.2和0.3 MPa时,筒体底端的应力高达8.614、13.710和17.020 MPa,而断裂位置处的应力相对较小,主要是由于断裂缺陷位置与其相邻的壳体无接触,内部受到的载荷并没有传递到最外层壳体上,导致最外层壳体断裂缺陷位置处的应力较小。如图3(c)所示,当断裂损伤深度为12 mm,内压载荷为0.1、0.2和0.3 MPa时,缺陷位置处的应力分别为6.664、11.190和12.860 MPa,均小于最大应力。如图3(d)和图3(e)所示,当断裂损伤的深度分别为9和6 mm时,应力的分布较为相似,依然具有以上特点,不同的是随着深度减小,最大应力基本保持不变,内压载荷为0.1 MPa时,最大应力分别为8.066和8.130 MPa。这主要是因为断裂缺陷距离内压载荷面越来越远,对整个壳体应力分布的影响也越来越小,直到断裂缺陷出现在最外层壳体上,如图3(f)所示,当内压分别为0.1、0.2和0.3 MPa时,最大应力分别为8.122、11.410和15.150 MPa,断裂缺陷对整个壳体的应力分布影响最小。
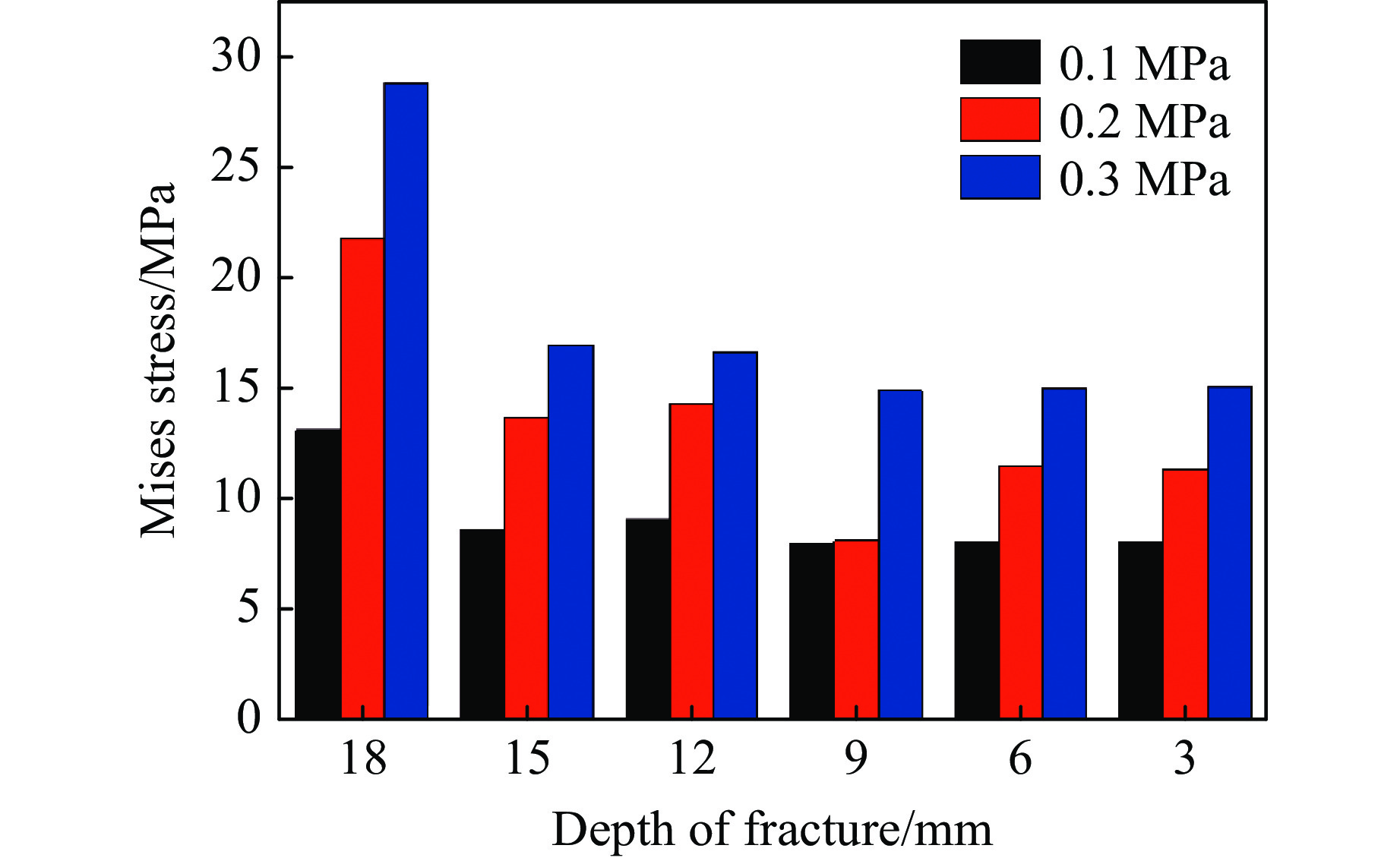
由图3可以看出,随着内压载荷增大,最大应力也不断增大,且最大应力出现在壳体底端附近,缺陷位置处的应力相对较小。统计上述不同内压载荷下的最大应力值,如图4所示。可以明显看出,随着内压增加,应力也不断增大。当断裂缺陷发生在第1层时,复合材料压力容器的应力最大。内压为0.1 MPa时,最大Mises应力高达13.14 MPa;内压为0.2 MPa时,最大Mises应力高达21.84 MPa;内压为0.3 MPa时,最大Mises应力高达28.8 MPa。当断裂缺陷逐渐靠近外壁,即与内压作用面较远时,最大应力趋于不变,保持平稳状态,断裂缺陷在第1层产生的最大应力是在第6层时的2倍。
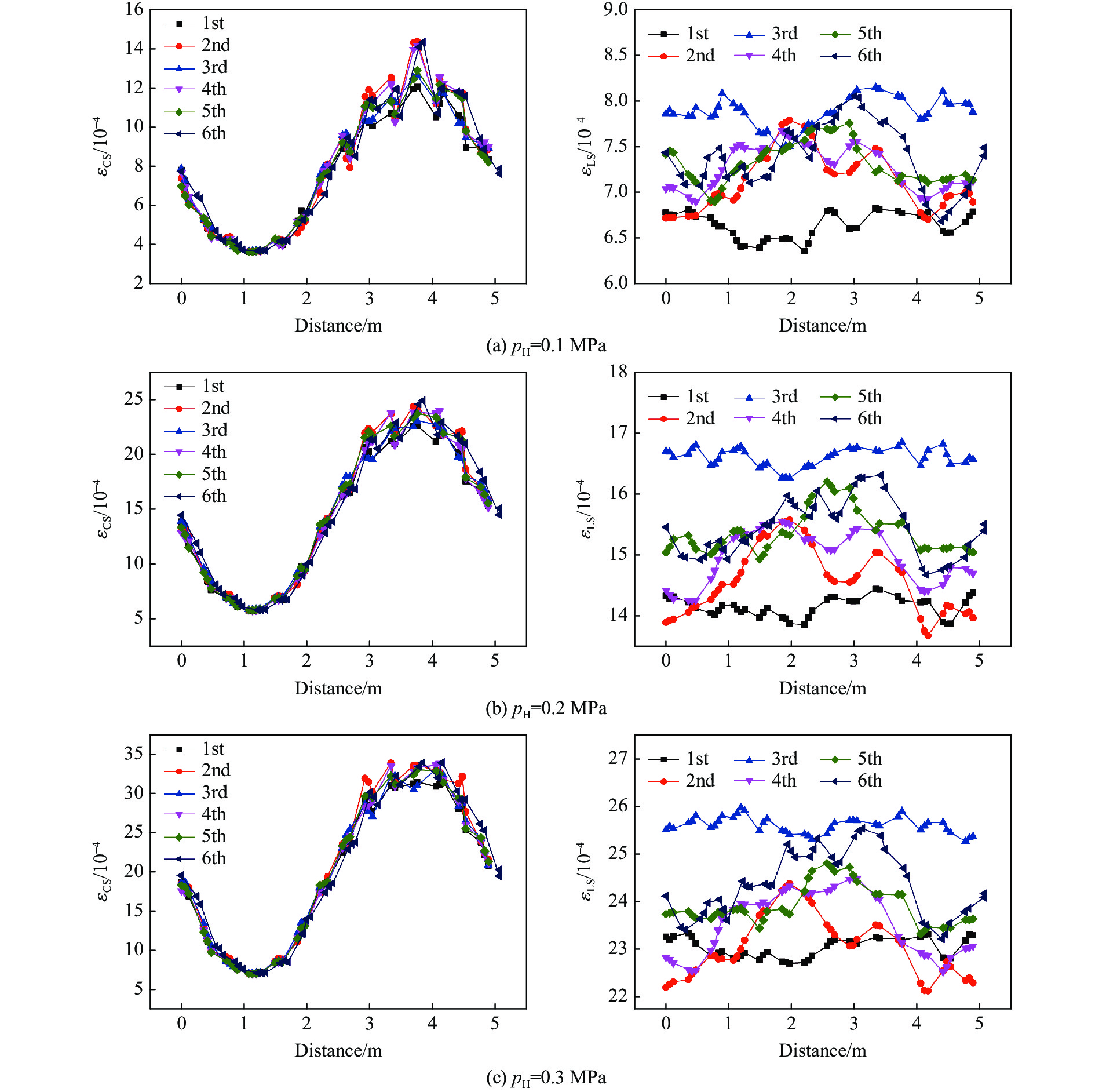
为了探究壳体外壁应变的变化情况,选择穿过断裂缺陷中心位置处的圆周路径,得到含有不同深度断裂缺陷的压力容器壳体的应变曲线,如图5所示。在图5中,
ε CS为周向应变,ε LS为纵向应变,横坐标零处为缺陷中心位置,横轴为与缺陷中心的周向距离。如图5(a)所示,当内压为0.1 MPa时,周向应变在3.0 × 10−4~1.50 × 10−3之间波动,曲线的形状与反正弦曲线相似,因此可进一步拟合,得到周向应变与距断裂缺陷距离的关系函数,而纵向应变在6.3 × 10−4~8.3 × 10−4之间波动。随着距离增加,纵向应变比较稳定,尤其是当断裂缺陷位于第1层和第6层时,几乎稳定在6.5 × 10−4~8.0 × 10−4范围内,这是由研究路径决定的,因研究路径位于壳体的最外层,当断裂缺陷位于第1层时,缺陷对研究路径上的应变影响较小,而缺陷位于第6层时,则是内压对最外层的影响小而导致应变分布较为均匀。如图5(b)和图5(c)所示,当内压为0.2和0.3 MPa时,周向和纵向应变的范围增大,且最大值也相应增加。当内压为0.2 MPa时,周向应变在5.0 × 10−4~2.50 × 10−3之间波动,纵向应变在1.35 × 10−3~1.70 × 10−3之间波动;当内压为0.3 MPa时,周向应变在5.0 × 10−4~3.50 × 10−3之间波动,纵向应变在2.20 × 10−3~2.60 × 10−3之间波动。综上所述,当内压为0.3 MPa时,周向应变的变化范围最大,可根据应变值确定断裂缺陷的位置和深度。因此,对该内压下的周向应变与距离曲线进行拟合,拟合采用waveform的sine公式,得到不同深度断裂缺陷的周向应变(
ε CS)与缺陷周向位置(x)的关系式(即式(8)~式(13)),可以较准确地确定不同深度断裂缺陷的周向位置和周向应变的关系。在实际工程实践中,采用应变检测方法确定壳体的应变分布,再通过关系式即可确定缺陷的周向位置和深度,为复合材料压力容器和管道检测提供一定的数据基础。18mm(1st):εCS=1989.3+1272.4sin(πx−2414.02546.6) (8) 15mm(2nd):εCS=2086.3+1390.2sin(πx−2421.22546.5) (9) 12mm(3rd):εCS=2026.8+1304.3sin(πx−2548.02543.0) (10) 9mm(4th):εCS=2027.8+1345.4sin(πx−2421.32552.7) (11) 6mm(5th):εCS=2035.4+1340.3sin(πx−2509.82564.6) (12) 3mm(6th):εCS=2045.2+1333.6sin(πx−2509.82564.6) (13) 5. 结 论
对玻璃纤维增强环氧树脂基复合材料层合板进行了基本力学性能试验,并利用Abaqus有限元软件建立了含有不同深度断裂缺陷的复合材料壳体的三维有限元模型,将所得的基本力学性能参数用于有限元模拟中,通过对模拟结果与实验结果进行对比分析,得到以下结论:
(1)复合材料主向的拉伸强度和弹性模量均大于横向,层间强度Ⅰ型和Ⅱ型断裂韧性相差不大;
(2)应力和应变参数可以反映出复合材料壳体断裂缺陷的位置,通过有限元模拟,能够很方便地得到含有断裂缺陷壳体的应力及应变分布情况,复合材料壳体的最大应力在筒体端部位置,缺陷位置处的应力相对较小;
(3)随着内压增加,复合材料壳体的Mises应力也不断增加,当断裂缺陷在第1层时,Mises应力最大,应变随着与缺陷位置距离的增加,呈现规律性变化,通过拟合公式,可以初步判断断裂损伤的周向位置和深度,为复合材料壳体的损伤检测提供一定的数据基础。
-
图 7 (a)(b) Mo和Pb弹丸高速撞击碎片云形态对比[169, 171];(c) Pb弹丸数值模拟密度云图[136];(d) MPM数值模拟结果[110];(e) 铝弹丸的数值模拟相态分布[153](红色为气态,绿色为液态,淡蓝色为固态)
Figure 7. (a)(b) Comparison of the debris clouds of Mo and Pb projectiles[169, 171]; (c) density clouds from the simulation of Pb projectile[136]; (d) results from MPM simulation; (e) phase clouds from the simulation of Al projectile[153]( red for gases, green for liquids and cyan for solids)
表 1 实验加载方式和典型克/亚克级发射参数
Table 1. Experimental methods and typical parameters for projectile with mass in gram or sub-gram
Methods Year Material Velocity/(km·s–1) Shape Mass/g Comments and sources Three-stage light gas gun 1993 Al 9.52 Flyer plate 0.78 Sandia National Laboratories[48] 2017 Al 10.1 Flyer plate 0.22 Institute of Fluid Physics[49] Magnetically driven device 2011 Al 45 Flyer plate 0.79 Z accelerator, Sandia National Laboratories[50] 2014 Al 8.7 Flyer plate 0.12 CQ-4, Institute of Fluid Physics[51] 2014 Al 11.5 Flyer plate 0.15 PTS, Institute of Fluid Physics[52] Electric gun 2019 Mylar 10 Flyer plate 0.30 Institute of Fluid Physics ISCL (Inhibited Shaped Charge Launcher) 1995 Al 11.16 Cylinder 1.02 Southwest Research Institute[53] 表 2 铝平面对称碰撞时相变对应的速度和压力
Table 2. The velocity of the Al projectile and the pressure from impacting
Source/Phase
changeIncipient melting
due to releaseComplete melting
due to releaseIncipient vaporization
due to releaseComplete vaporization
due to releaseHopkins et al.[169] 2.7 km/s, 65 GPa 3.38 km/s, 89 GPa Anderson et al.[170] 2.85 km/s, 71 GPa 3.45 km/s, 94 GPa 5.2 km/s, 174 GPa Bjork[171] 6.2 km/s, 225 GPa 2700 GPa Shockey et al.[172] 2.6–3.6 km/s 3.3–4.6 km/s 5.5–7.5 km/s 12.5–16.5 km/s Pierazzo et al.[173] 73 GPa 106 GPa 315 GPa Source/Phase
changeIncipient melting
due to shockComplete melting
due to shockTang[153] 125 GPa 160 GPa 表 3 弹丸质量相同、形状不同所得到碎片云的参数
Table 3. The parameters of debris cloud generated by hypervelocity impact of projectiles with the same mass and different shapes
Shape of projectile Dimensions Mass/g Axial length/mm Radical length/mm Sphere ∅5.02 mm 0.180 89 44.5 40.5 Cylinder ∅5.02 mm×4.6 mm 0.182 61 46.5 44.0 Disk ∅5.02 mm×1.0 mm 0.181 06 45.5 32.2 -
[1] 张庆明, 黄风雷. 超高速碰撞动力学引论[M]. 北京: 科学出版社, 2000: 1.ZHANG Q M, HUANG F L. Introduction to dynamics of hypervelocity impact [M]. Beijing: Science Press, 2000: 1. [2] 曲广吉, 韩增尧. 空间碎片超高速撞击动力学建模与数值仿真技术 [J]. 中国空间科学技术, 2002(5): 26–30. doi: 10.3321/j.issn:1000-758X.2002.05.005QU G J, HAN Z Y. Dynamical modeling and numerical simulation of hypervelocity space debris impact [J]. Chinese Space Science and Technology, 2002(5): 26–30. doi: 10.3321/j.issn:1000-758X.2002.05.005 [3] WHIPPLE F L. Meteorites and space travel [J]. Astronomical Journal, 1947, 1161: 131–147. [4] WICKLEIN M, RYAN S, WHITE D M, et al. Hypervelocity impact on CFRP: testing, material modelling, and numerical simulation [J]. International Journal of Impact Engineering, 2008, 35(12): 1861–1869. doi: 10.1016/j.ijimpeng.2008.07.015 [5] HUANG J, MA Z, REN L, et al. A new engineering model of debris cloud produced by hypervelocity impact [J]. International Journal of Impact Engineering, 2013, 56: 32–39. doi: 10.1016/j.ijimpeng.2012.07.003 [6] CHRISTIANSEN E L. Design and performance equations for advanced meteoroid and debris shields [J]. International Journal of Impact Engineering, 1993, 14(1): 145–156. [7] 龚自正, 杨继云, 张文兵, 等. 航天器空间碎片超高速撞击防护的若干问题 [J]. 航天器环境工程, 2007, 24(3): 125–130. doi: 10.3969/j.issn.1673-1379.2007.03.001GONG Z Z, YANG J Y, ZHANG W B, et al. Spacecraft protection from the hypervelocity impact of space meteoroid and orbital debris [J]. Spacecraft Environment Engineering, 2007, 24(3): 125–130. doi: 10.3969/j.issn.1673-1379.2007.03.001 [8] SCHONBERG W P. Characterizing secondary debris impact ejecta [J]. International Journal of Impact Engineering, 2001, 26(1): 713–724. [9] FAHRENTHOLD E P, HORBAN B A. An improved hybrid particle-element method for hypervelocity impact simulation [J]. International Journal of Impact Engineering, 2001, 26(1): 169–178. [10] CORVVONATO E, DESTEFANIS R, FARAUD M. Integral model for the description of the debris cloud structure and impact [J]. International Journal of Impact Engineering, 2001, 21: 115–128. [11] COHEN L J. A debris cloud cratering model [J]. International Journal of Impact Engineering, 1995, 17(1/2/3): 229–240. [12] MAIDEN C J, MCMILLAN A R. An investigation of the protection afforded a spacecraft by a thin shield [J]. AIAA Journal, 1964: 1992–1998. [13] PIEKUTOWSKI A J. Fragmentation-initiation threshold for spheres impacting at hypervelocity [J]. International Journal of Impact Engineering, 2003, 29: 563–574. doi: 10.1016/j.ijimpeng.2003.10.005 [14] PIEKUTOWSKI A J. Characteristics of debris clouds produced by hypervelocity impact of aluminum spheres with thin aluminum plates [J]. International Journal of Impact Engineering, 1993, 14(1): 573–86. [15] BASHUROV V V, BEBENIN G V, BELOV G V, et al. Experimental modeling and numerical simulation of high- and hypervelocity space debris impact to spacecraft shield protection [J]. International Journal of Impact Engineering, 1997, 20(1): 69–78. [16] COUR-PALAIS B G. The shape effect of non-spherical projectiles in hypervelocity impacts [J]. International Journal of Impact Engineering, 2001, 26: 129–143. doi: 10.1016/S0734-743X(01)00075-6 [17] IYER K A, POORMON K L, DEACON R M, et al. Hypervelocity impact response of Ti-6Al-4V and commercially pure titanium [J]. Procedia Engineering, 2013, 58: 127–137. doi: 10.1016/j.proeng.2013.05.016 [18] FRIICHTENICHT J F, SLATTERY J C. Ionization associated with hypervelocity impact: D-2091 [R]. USA: NASA, 1963. [19] CRAWFORD D A, SCHULTZ P H. Laboratory observation of impact-generated magnetic fields [J]. Nature, 1988, 336(6194): 50–52. doi: 10.1038/336050a0 [20] CRAWFORD D A, SCHULTZ P H. Laboratory investigation of impact-generated plasma [J]. Journal of Geophysical Research: Planets, 1991, 96: 18807–18817. doi: 10.1029/91JE02012 [21] CRAWFORD D A, SCHULTZ P H. The production and evolution of impact-generated magnetic fields [J]. International Journal of Impact Engineering, 1993, 14: 205–216. doi: 10.1016/0734-743X(93)90021-X [22] GRUN D E, KISSEL J. The ion-composition of the plasma produced by impacts of fast dust particles [J]. Planetary and Space Science, 1977, 25(2): 135–147. doi: 10.1016/0032-0633(77)90017-4 [23] DIETZEL H, EICHORN G, FECHTIG H, et al. The HEOS2 and HELIOS micrometeoroid experiments [J]. Journal of Physics E: Scientific Instruments, 1973, 6(3): 209–217. doi: 10.1088/0022-3735/6/3/008 [24] GRÜN E, FECHTIG H, HANNER M S, et al. The Galileo dust detector [J]. Space Science Reviews, 1992, 60: 317–340. [25] RATCLIFF P R, MC DONNELL J A M, FIRTH J G, et al. The cosmic dust analyser [J]. Journal of the British Interplanetary Society, 1992, 45: 355–358. [26] CRAWFORD D A, SCHULTZ P H. Electromagnetic properties of impact-generated plasma, vapor and debris [J]. International Journal of Impact Engineering, 1999, 23: 169–180. doi: 10.1016/S0734-743X(99)00070-6 [27] RATCLIFF P R, REBER M, COLE M J, et al. Velocity thresholds for impact plasma production [J]. Advances in Space Research, 1997, 20(8): 1471–1476. doi: 10.1016/S0273-1177(97)00419-5 [28] RATCLIFF P R, ALLAHDADI F. Characteristics of the plasma from a 94 km/s micro-particle impact [J]. Advances in Space Research, 1996, 17(12): 87–91. doi: 10.1016/0273-1177(95)00763-5 [29] GAULT D E, HEITOWOT E D. The partition of energy for hypervelocity impact craters formed in rocks [C]//Proceedings of the 6th Hypervelocity Impact Symposium, 1963, 2: 419. [30] 柳森, 谢爱民, 黄洁, 等. 超高速碰撞碎片云的激光阴影照相技术 [J]. 实验流体力学, 2005, 19(2): 35–39. doi: 10.3969/j.issn.1672-9897.2005.02.007LIU S, XIE A M, HUANG J, et al. Laser shadowgraph for the visualization of hypervelocity impact debris cloud [J]. Journal of Experimnets in Fluid Mechanics, 2005, 19(2): 35–39. doi: 10.3969/j.issn.1672-9897.2005.02.007 [31] 柳森, 李毅, 黄洁, 等. 用于验证数值仿真的Whipple屏超高速撞击试验结果 [J]. 宇航学报, 2005, 26(4): 505–508. doi: 10.3321/j.issn:1000-1328.2005.04.024LIU S, LI Y, HUANG J, et al. Hypervelocity impact test results of Whipple shield for the validation of numerical simulation [J]. Journal of Astronautics, 2005, 26(4): 505–508. doi: 10.3321/j.issn:1000-1328.2005.04.024 [32] 马兆侠, 黄洁, 石安华, 等. 铝球超高速撞击铝板反溅碎片云团辐射特性研究 [J]. 实验流体力学, 2014, 28(2): 90–94.MA Z X, HUANG J, SHI A H, et al. Study on radiation characteristics of ricochet debris cloud form aluminum plate subjected to hypervelocity impacts by aluminum projectile [J]. Journal of Experimnets in Fluid Mechanics, 2014, 28(2): 90–94. [33] 兰胜威, 柳森, 覃金贵, 等. 不同密度弹丸对水冰的超高速撞击成坑实验 [J]. 宇航学报, 2018, 39(9): 1054–1059.LAN S W, LIU S, QIN J G, et al. Hypervelocity impact cratering in water ice by projectiles with different densities [J]. Journal of Astronautics, 2018, 39(9): 1054–1059. [34] 庞宝君, 林敏, 张凯, 等. 丝网防护屏碎片云特性数值模拟研究 [J]. 高压物理学报, 2013, 27(3): 391–397. doi: 10.11858/gywlxb.2013.03.012PANG B J, LIN M, ZHANG K, et al. Numerical simulation of debris cloud characteristics of the mesh shields [J]. Chinese Journal of High Pressure Physics, 2013, 27(3): 391–397. doi: 10.11858/gywlxb.2013.03.012 [35] 龚自正, 杨继运, 代福, 等. CAST空间碎片超高速撞击试验研究进展 [J]. 航天器环境工程, 2009, 26(4): 301–306. doi: 10.3969/j.issn.1673-1379.2009.04.001GONG Z Z, YANG J Y, DAI F, et al. M/OD hypervelocity impact tests carried out in CAST [J]. Spacecraft Environment Engineering, 2009, 26(4): 301–306. doi: 10.3969/j.issn.1673-1379.2009.04.001 [36] 冉宪文, 张若棋, 徐志宏, 等. 超高速碰撞条件下铝靶熔化临界速度的理论估算及Grüneisen参数的影响[C]//第四届全国空间碎片专题研讨会. 南京, 2007. [37] 裴晓阳, 唐蜜, 钟敏, 等. 超高速撞击下碎片云相分布的数值模拟研究[C]//第十四届全国物理力学学术会议缩编文集, 2016: 228. [38] 李宝宝. 超高速碰撞下相变效应的数值模拟研究[D]. 长沙: 国防科学技术大学, 2010.LI B B. The numerical simulation study on effect of phase transition in hypervelocity impacting [D]. Changsha: University of Defense Technology, 2010. [39] TANG E L, WANG H L, XIA J, et al. Experimental study on plasma discharge induced by high-velocity impact solar array associated with projectile incidence angles [J]. International Journal of Applied Electromagnetics and Mechanics, 2016, 51(2): 107–117. doi: 10.3233/JAE-150119 [40] 唐恩凌, 张庆明, 张健. 超高速碰撞LY12铝靶产生等离子体的特征参量测量 [J]. 弹箭与制导学报, 2008, 28(4): 110–112. doi: 10.3969/j.issn.1673-9728.2008.04.034TANG E L, ZHANG Q M, ZHANG J. Characteristic parameter measurement of plasma generated during hypervelocity impact on LY12 Aluminum target [J]. Jounral of Projectiles, Rochets, Missiles and Guidance, 2008, 28(4): 110–112. doi: 10.3969/j.issn.1673-9728.2008.04.034 [41] 马月芬, 张庆明, 吴碧, 等. 超高速碰撞产生等离子体的电磁场测量方法 [J]. 北京理工大学学报, 2011, 31(9): 1118–1121.MA Y F, ZHANG Q M, WU B, et al. Measurement method of electromagnetic fields of plasma produced by hypervelocity impact [J]. Transactions of Beijing Institute of Technology, 2011, 31(9): 1118–1121. [42] 马月芬, 张庆明, 李一磊, 等. 超高速碰撞产生的电磁场对通信电路的干扰 [J]. 北京理工大学学报, 2011, 31(7): 859–862.MA Y F, ZHANG Q M, LI Y L, et al. Interference on communication circuits due to electromagnetic fields generated by hypervelocity impact [J]. Transactions of Beijing Institute of Technology, 2011, 31(7): 859–862. [43] SONG W D, LV Y T, LI J Q, et al. Influence of impact conditions on plasma generation during hypervelocity impact by aluminum projectile [J]. Physics of Plasmas, 2016, 23: 073506. doi: 10.1063/1.4956440 [44] 栗建桥, 宋卫东, 宁建国. 超高速撞击产生的等离子体特性研究 [J]. 高压物理学报, 2011, 27(4): 542–548. doi: 10.11858/gywlxb.2013.04.012LI J Q, SONG W D, NING J G. A study on characteristics of plasma generated by hypervelocity impact [J]. Chinese Journal of High Pressure Physics, 2011, 27(4): 542–548. doi: 10.11858/gywlxb.2013.04.012 [45] 宁建国, 栗建桥, 宋卫东. 超高速碰撞产生等离子体的毁伤特性研究 [J]. 力学学报, 2014, 46(6): 853–861.NING J G, LI J Q, SONG W D. Investigation of plasma damage properties generated by hypervelocity impact [J]. Chinese Journal of Theoretical and Applied Mechanics, 2014, 46(6): 853–861. [46] SONG W, LÜ Y, WANG C, et al. Investigation on plasma generated during hypervelocity impact at different impact velocities and angles [J]. Physics of Plasmas, 2015, 22(12): 123519. doi: 10.1063/1.4938516 [47] 李怡勇, 沈怀荣, 李智. 超高速撞击动力学及航天器防护研究进展 [J]. 力学与实践, 2009, 31(2): 11–16.LI Y Y, SHEN H R, LI Z. Advances in hypervelocity impact dynamics and spacecraft protection research [J]. Mechanics in Engineering, 2009, 31(2): 11–16. [48] BOSLOUGH M B, ANG J A, CHHABLLDAS L C, et al. Hypervelocity testing of advanced shielding concepts for spacecraft against impacts to 10 km/s [J]. International Journal of Impact Engineering, 1993, 14: 95–106. doi: 10.1016/0734-743X(93)90012-V [49] 王翔, 王青松, 彭建祥, 等. 三级炮超高速发射技术在空间碎片防护研究中的初步应用 [J]. 高能量密度物理, 2017(4): 115–122.WANG X, WANG Q S, PENG J X, et al. The application of three-stage gun in the study of space debris [J]. High Energy Density Physics, 2017(4): 115–122. [50] LEMKE R W, KNUDSON M D, DAVIS J P. Magnetically driven hyper-velocity launch capability at the Sandia Z accelerator [J]. International Journal of Impact Engineering, 2011, 38(6): 480–485. doi: 10.1016/j.ijimpeng.2010.10.019 [51] ZHANG X, WANG G, ZHAO J, et al. High velocity flyer plates launched by magnetic pressure on pulsed power generator CQ-4 and applied in shock Hugoniot experiments [J]. Review of Scientific Instruments, 2014, 85(5): 055110. doi: 10.1063/1.4875705 [52] 王贵林, 郭帅, 沈兆武, 等. 基于聚龙一号装置的超高速飞片发射实验研究进展 [J]. 物理学报, 2014, 63(19): 196201. doi: 10.7498/aps.63.196201WANG G L, GUO S, SHEN Z W. Recent advances in hyper-velocity flyer launch experiments on PTS [J]. Acta Physica Sinica, 2014, 63(19): 196201. doi: 10.7498/aps.63.196201 [53] WALKER J D, GROSCH D J, MULLIN S A. A hypervelocity fragment launcher based on an inhibited shaped charge [J]. International Journal of Impact Engineering, 1993(14): 763–774. [54] 杨继运. 二级轻气炮模拟空间碎片超高速碰撞试验技术 [J]. 航天器环境工程, 2006, 23(1): 16–22. doi: 10.3969/j.issn.1673-1379.2006.01.003YANG J Y. Simulation of space debris hypervelocity impact using two stage light gas gun [J]. Spacecraft Environment Engineering, 2006, 23(1): 16–22. doi: 10.3969/j.issn.1673-1379.2006.01.003 [55] SEILER F, LGRA O. Hypervelocity lauchers [M]. Springer, 2016: 23–52. [56] CANNING T N, SEIFF A, JAMES C S. Ballistic range technology [M]. North Atlantic Treaty Origanization, 1970: 9–54. [57] EXOW B L, WICKERT M, THOMA K, et al. The extra-large light-gas gun of the Fraunhofer EMI: applications for impact cratering research [J]. Meteoritics & Planetary Science, 2013, 48(1): 3–7. [58] 王金贵. 气体炮原理与技术 [M]. 北京: 国防工业出版社, 2001: 198–202.WANG J G. Principle and technology of gas gun [M]. Beijing: National Defense Industry Press, 2001: 198–202. [59] CHHABILDAS L C, KMETYK, L N, REINHART W D, et al. Enhanced hypervelocity launcher-capabilities to 16 km/s [J]. International Journal of Impact Engineering, 1995, 17: 183–194. doi: 10.1016/0734-743X(95)99845-I [60] OSHER J E, HENRY G B, CHAU H, et al. Operating characteristics and modelling of the LLNL 100-kV electric gun [J]. IEEE Transactions on Plasma Science, 1989, 17(3): 392–402. doi: 10.1109/27.32247 [61] 张文兵, 龚自正, 杨继运, 等. 用于模拟空间碎片超高速撞击的激光驱动飞片系统 [J]. 空间碎片研究, 2007, 7(1): 26–30.ZHANG W B, GONG Z Z, YANG J Y, et al. The laser-driven flyer system for space debris hypervelocity impact simulations [J]. Space Debris Research, 2007, 7(1): 26–30. [62] PIEKUTOWSKI A J. Effects of scale on debris cloud properties [J]. International Journal of Impact Engineering, 1997, 20: 639–50. doi: 10.1016/S0734-743X(97)87451-9 [63] PIEKUTOWSKI A J, POORMON K L. Effects of scale on the performance of Whipple shields for impact velocities ranging from 7 to 10 km/s [J]. Procedia Engineering, 2013, 58: 642–652. doi: 10.1016/j.proeng.2013.05.074 [64] 贾祖朋, 张树道, 蔚喜军. 多介质流体动力学计算方法[M]. 北京: 科学出版社, 2014: 1–25.JIA Z P, ZHANG S D, WEI X J. Numerical methods for dynamics of multi-material [M]. Beijing: Science Press, 2014: 1–25. [65] PEERY J S, CARROLL D E. Multi-material ALE methods in unstructured grids [J]. Computer Methods in Applied Mechanics and Engineering, 2000, 187(3/4): 591–619. [66] WINGATE C A, STELLINGWERF R F, DAVIDSON R F, et al. Models of high velocity impact phenomena [J]. International Journal of Impact Engineering, 1993, 14: 819–830. doi: 10.1016/0734-743X(93)90075-I [67] FREY J D, JANICOT F, GARAUD X, et al. The validation of hydrocodes for orbital debris impact simulation [J]. International Journal of Impact Engineering, 1993, 14: 255–265. doi: 10.1016/0734-743X(93)90025-3 [68] BURKETT M W, CLANCY S P, MAUDLIN P J, et al. Coupled plasticity and damage modeling and their applications in a three-dimensional Eulerian hydrocode [J]. International Journal of Impact Engineering, 2006, 33: 126–132. doi: 10.1016/j.ijimpeng.2006.09.068 [69] HORNER J K. A comparison of ballistic limit with adaptive-mesh Eulerian hydrocode predictions of one- and two-plate aluminum shielding protection against millimeter-sized Fe-Ni space debris [J]. International Journal of Impact Engineering, 2008, 35(12): 1602–1605. doi: 10.1016/j.ijimpeng.2008.07.039 [70] TRUCANO T G, MC GLAUN J M. Hypervelocity impact calculations using CTH: case studies [J]. International Journal of Impact Engineering, 1990, 10: 601–613. doi: 10.1016/0734-743X(90)90092-A [71] GRIMALDI A, SOLLO A, GUIDA M, et al. Parametric study of a SPH high velocity impact analysis–a birdstrike windshield application [J]. Composite Structures, 2013, 96: 616–630. doi: 10.1016/j.compstruct.2012.09.037 [72] MICHEL Y, CHEVALIER J M, DURIN C, et al. Hypervelocity impacts on thin brittle targets: experimental data and SPH simulations [J]. International Journal of Impact Engineering, 2006, 33: 441–451. doi: 10.1016/j.ijimpeng.2006.09.081 [73] SHAW A, REID S R. Heuristic acceleration correction algorithm for use in SPH computations in impact mechanics [J]. Computer Methods in Applied Mechanics and Engineering, 2009, 198(49/50/51/52): 3962–3974. [74] LIU X, OSHER S, CHAN T. Weighted essentially non-oscillatory schemes [J]. Journal of Computational Physics, 1994, 115(1): 200–212. doi: 10.1006/jcph.1994.1187 [75] COCKBURN B. Discontinuous Galerkin methods [J]. Zeitschrift fur Angewandte Mathematik und Mechanik, 2003, 83(11): 731–754. doi: 10.1002/(ISSN)1521-4001 [76] BEISSEL S R, GERLACH C A, JOHNSON G R. Hypervelocity impact computations with finite elements and meshfree particles [J]. International Journal of Impact Engineering, 2006, 33: 80–90. doi: 10.1016/j.ijimpeng.2006.09.047 [77] KOKH S, LAGOUTIÈRE F. An anti-diffusive numerical scheme for the simulation of interfaces between compressible fluids by means of a five-equation model [J]. Journal of Computational Physics, 2010, 229(8): 2773–2809. doi: 10.1016/j.jcp.2009.12.003 [78] SHUKLA R K. Nonlinear preconditioning for efficient and accurate interface capturing in simulation of multicomponent [J]. Journal of Computational Physics, 2014, 276: 508–540. doi: 10.1016/j.jcp.2014.07.034 [79] XIAO F, LI S, CHEN C. Revisit to the THINC scheme: a simple algebraic VOF algorithm [J]. Journal of Computational Physics, 2011, 230(19): 7086–7092. doi: 10.1016/j.jcp.2011.06.012 [80] SAMBASIVAN S K, UDAYKUMAR H S. A sharp interface method for high-speed multi-material flows: strong shocks and arbitrary material pairs [J]. International Journal of Computational Fluid Dynamics, 2011, 25(3): 139–162. doi: 10.1080/10618562.2011.558011 [81] KIPP M E, GRADY D E. High-pressure shock compression of solids II dynamic fracture and fragmentation [M]. Springer, 1996: 238. [82] MCGLAUN J M, THOMPSON S L, ELRICK M G. CTH: A three-dimensional shock wave physics code [J]. International Journal of Impact Engineering, 1990, 10: 351–360. doi: 10.1016/0734-743X(90)90071-3 [83] 王言金, 刘军. Whipple防护结构超高速碰撞的欧拉数值模拟: GF-A 23030504 [R]. 北京: 北京应用物理与计算数学研究所, 2014.WANG Y J, LIU J. Eulerian numerical study of hypervelocity impacts on Whipple shields: GF-A 23030504 [R]. Beijing: Institute of Applied Physics and Computational Mathematics, 2014. [84] 梁仙红, 李征, 何长江, 等. 多介质流体力学两步欧拉方法的模型封闭性方法 [J]. 计算物理, 2010, 27(5): 658–664. doi: 10.3969/j.issn.1001-246X.2010.05.004LIANG X H, LI Z, HE C J, et al. Closing relations in two-step Eulerian method for multifluid dynamics [J]. Chinese Journal of Computational Physics, 2010, 27(5): 658–664. doi: 10.3969/j.issn.1001-246X.2010.05.004 [85] THOMPSON S L, MCGLAUN J L. CSQIII-an Eulerian finite difference program for two-dimensional material response: user’s manual: Sandia Report SAND87-2763 [R]. Albuquerque: Sandia National Laboratories, 1988. [86] HERTEL E S, MCINTOSH R L, PATTERSON B C. A comparison of phase change phenomena in CTH experimental data [J]. International Journal of Impact Engineering, 1995, 17: 399–408. doi: 10.1016/0734-743X(95)99865-O [87] POVARNITSYN M E, KHISHCHENKO K V, LEVASHOV P R. Simulation of melting and vaporization of metals at hypervelocity impact [J]. Journal of Physics: Conference Series, 2008(98): 042025. [88] CHEN J, MICHAEL H, CHI S. Meshfree methods: progress made after 20 years [J]. Journal of Engineering Mechanics, 2017, 143(4): 04017001. doi: 10.1061/(ASCE)EM.1943-7889.0001176 [89] GINGOLD R A, MONAGHAN J J. Smoothed particle hydrodynamics: theory and application to non-spherical stars [J]. Monthly Notices Royal Astronomy Society, 1977, 181(3): 375–389. doi: 10.1093/mnras/181.3.375 [90] GUAN P C, CHI S W, CHEN J S. Semi-Lagrangian reproducing kernel particle method for fragment-impact problems [J]. International Journal of Impact Engineering, 2011(38): 1033–1047. [91] SULSKY D, CHEN Z, SCHREYER. A particle method for history-dependent materials [J]. Computer Methods in Applied Mechanics and Engineering, 1994, 118: 179–196. doi: 10.1016/0045-7825(94)90112-0 [92] LI B, HABBAL F, ORTIZ M. Optimal transportation meshfree approximation schemes for fluid and plastic flows [J]. International Journal for Numerical Methods in Engineering, 2010(83): 1541–1579. [93] LUCY L B. A numerical approach to the testing of the fission hypothesis [J]. The Astronomical Journal, 1977, 82: 1013–1024. doi: 10.1086/112164 [94] MONAGHAN J J. An introduction to SPH [J]. Computer Physics Communications, 1988, 48(1): 89–96. doi: 10.1016/0010-4655(88)90026-4 [95] LIBERSKY L D, PETSCHEK A G. Smooth particle hydrodynamics with strength of materials [M]//Advances in the Free-Lagrange Method Including Contributions on Adaptive Gridding and the Smooth Particle Hydrodynamics Method. Heidelberg: Springer Berlin Heidelberg, 1991: 248–257. [96] LIU M B, LIU G R. Smoothed particle hydrodynamics (SPH): an overview and recent developments [J]. Archives of Computational Methods in Engineering, 2010, 17(1): 25–76. doi: 10.1007/s11831-010-9040-7 [97] 乐莉, 闫军, 钟秋海. 超高速撞击仿真算法分析 [J]. 系统仿真学报, 2014, 16(9): 1941–1943.YUE L, YAN J, ZHONG Q H. Simulations of debris impacts using three different algorithms [J]. Journal of System Simulation, 2014, 16(9): 1941–1943. [98] HIERMAIER S, KONKE D, STILP A J, et al. Computational simulation of the hypervelocity impact of Al-spheres on thin plates of different materials [J]. International Journal of Impact Engineering, 1997, 20(1): 363–374. [99] 王林, 胡秀章, 李永池, 等. 基于LS-DYNA的超高速撞击SPH数值模拟研究 [J]. 防护工程, 2010, 32(2): 32–38.WANG L, HU X Z, LI Y C, et al. Numerical simulation of hypervelocity impact by smoothed particle hydrodynamics using LS-DYNA [J]. Protective Engineering, 2010, 32(2): 32–38. [100] 徐英, 时家明, 林志丹. 撞击物形状和速度对高速撞击结果的影响 [J]. 弹箭与制导学报, 2010, 30(2): 106–110. doi: 10.3969/j.issn.1673-9728.2010.02.032XU Y, SHI J M, LIN Z D. On the shape and velocity of impact bodies in hypervelocity impact [J]. Journal of Projectiles, Rockets, Missiles and Guidance, 2010, 30(2): 106–110. doi: 10.3969/j.issn.1673-9728.2010.02.032 [101] LIBERSKY L D, PETSCHEK A G, CARNEY T C, et al. High strain Lagrangian hydrodynamics: a threedimensional SPH code for dynamic material response [J]. Journal of Computational Physics, 1993, 109(1): 67–75. doi: 10.1006/jcph.1993.1199 [102] ZHOU C E, LIU G R, LOU K Y. Three-dimensional penetration simulation using smoothed particle hydrodynamics [J]. International Journal of Computational Methods, 2007, 4(4): 671–691. doi: 10.1142/S0219876207000972 [103] MEDINA D F, CHEN J K. Three-dimensional simulations of impact induced damage in composite structures using the parallelized SPH method [J]. Composites: Part A, 2000, 31(8): 853–860. doi: 10.1016/S1359-835X(00)00031-2 [104] HIERMAIER S, KÖNKE D, STILP A J, et al. Computaional simulation of the hypervelocity impact of Al-spheres on thin plates of different materials [J]. International Journal of Impact Engineeringn, 1997, 20(1): 363–374. [105] GROENENBOOM P H L. Numerical simulation of 2D and 3D hypervelocity impact using the SPH option in PAM-SHOCK [J]. International Journal of Impact Engineering, 1997, 20: 309–323. doi: 10.1016/S0734-743X(97)87503-3 [106] FARAUD M, DESTEFANIS R, PALMIERI D, et al. SPH simulations of debris impacts using two different computer codes [J]. International Journal of Impact Engineering, 1999, 23: 249–260. doi: 10.1016/S0734-743X(99)00077-9 [107] HARLOW F H. The particle-in-cell computing method for fluid dynamics [J]. Methods for Computational Physics, 1964, 3: 319–343. [108] BRACKBILL J U, RUPPEL H M. FLIP: a method for adaptively zoned, particle-in-cell calculations of fluid flows in two dimensions [J]. Journal of Computational Physics, 1986, 65: 314–343. doi: 10.1016/0021-9991(86)90211-1 [109] 廉艳平, 张帆, 刘岩, 等. 物质点的理论和应用 [J]. 力学进展, 2013, 43(2): 237–264.LIAN Y P, ZHANG F, LIU Y, et al. Material point method and its applications [J]. Advaced in Mechanics, 2013, 43(2): 237–264. [110] 黄鹏. 金属及岩土冲击动力学问题的物质点法研究[D]. 北京: 清华大学, 2010: 69–70.HUANG P. Material point method for metal and soil impact dynamics problems [D]. Beijing: Tsinghua University, 2010: 69–70. [111] LIU P, LIU Y, ZHANG X, et al. Investigation on high-velocity impact of micron particles using material point method [J]. International Journal of Impact Engineering, 2015, 75: 241–254. doi: 10.1016/j.ijimpeng.2014.09.001 [112] ZHANG C, KALIA R K, NAKANO A, et al. Hypervelocity impact induced deformation modes in α-alumina [J]. Applied Physics Letters, 2007, 91: 071906. doi: 10.1063/1.2753092 [113] SAMELA J, KAI N. Atomistic simulation of the transition from atomistic to macroscopic cratering [J]. Physical Review Letters, 2008, 101(2): 027601. doi: 10.1103/PhysRevLett.101.027601 [114] ANDERS C, BRINGA E M, URBASSEK H M. Crater production by energetic nanoparticle impact on Au nanofoams [J]. Applied Physics Letters, 2016, 108(11): 113108. doi: 10.1063/1.4944420 [115] 巨圆圆, 张庆明, 龚良飞, 等. 球形弹丸超高速撞击铝靶的分子动力学模拟 [J]. 航天器环境工程, 2018, 35(2): 153–157. doi: 10.3969/j.issn.1673-1379.2018.02.009JU Y Y, ZHANG Q M, GONG L F, et al. Molecular dynamics simulation for hypervelocity impact of spherical projectile to aluminum target [J]. Spacecraft Environment Engineering, 2018, 35(2): 153–157. doi: 10.3969/j.issn.1673-1379.2018.02.009 [116] JARAMILLO-BOTERO A, AN Q, THEOFANIS P L, et al. Large-scale molecular simulations of hypervelocity impact of materials [J]. Procedia Engineering, 2013, 58: 167–176. doi: 10.1016/j.proeng.2013.05.020 [117] 李毅, 柳森. 航天器铝合金面板的超高速撞击数值模拟 [J]. 载人航天, 2004(6): 52–55. [118] 冯春, 李世海, 刘晓宇. 一种基于颗粒接触的二维无网格方法及其在高速冲击模拟中的应用 [J]. 爆炸与冲击, 2014, 34(3): 292–299. doi: 10.11883/1001-1455(2014)03-0292-08FENG C, LI S H, LIU X Y. A 2D particle contact-based meshfree method and its application to hypervelocity impact simulation [J]. Explosion and Shock Waves, 2014, 34(3): 292–299. doi: 10.11883/1001-1455(2014)03-0292-08 [119] JOHNSON G R, STRYK R A. Conversion of 3D distorted elements into meshless particles during dynamic deformation [J]. International Journal of Impact Engineering, 2003, 28(9): 947–966. doi: 10.1016/S0734-743X(03)00012-5 [120] JOHNSON G R, BEISSEL S R, GERLACH C A. Another approach to a hybrid particle-finite element algorithm for high-velocity impact [J]. International Journal of Impact Engineering, 2011, 38(5): 397–405. doi: 10.1016/j.ijimpeng.2011.01.002 [121] JOHNSON G R, BEISSEL S R, STRYK R A. A generalized particle algorithm for high velocity impact computations [J]. Computational Mechanics, 2000, 25(2/3): 245–256. [122] JOHNSON G R, COOK W H. Fracture characteristics of three metals subjected to various strains, strain rates, temperatures and pressures [J]. Engineering Fracture Mechanics, 1985, 21(1): 31–48. doi: 10.1016/0013-7944(85)90052-9 [123] JOHNSON G R, COOK W H. A constitutive model and data for metals subjected to large strains, high strain rates and high temperatures [C]//Seventh International Symposium on Ballistics. The Hague, Netherlands, 1983. [124] STEINBERG D J, COCHRAN S G, GUINAN M W. A constitutive model for metals applicable at high-strain rate [J]. Journal of Applied Physics, 1980, 51(3): 1498–1504. doi: 10.1063/1.327799 [125] SELYUTINA N S, PETROV Y V. Structural and temporal features of high-rate deformation of metals [J]. Doklady Physics, 2017, 62(2): 102–105. doi: 10.1134/S1028335817020136 [126] SCHÄFER F K. An engineering fragmentation model for the impact of spherical projectiles on thin metallic plates [J]. International Journal of Impact Engineering, 2006, 33: 745–762. doi: 10.1016/j.ijimpeng.2006.09.067 [127] STEINBERG D J, LUND C M. A constitutive model for strain rates from 10-4 to 106 s-1 [J]. Journal of Applied Physics, 1989, 65(4): 1528–1533. doi: 10.1063/1.342968 [128] 张伟, 庞宝君, 贾斌, 等. 弹丸超高速撞击防护屏碎片云数值模拟 [J]. 高压物理学报, 2004, 18(1): 47–52. doi: 10.3969/j.issn.1000-5773.2004.01.009ZHANG W, PANG B J, JIA B, et al. Numerical simulation of debris cloud produced by hypervelocity impact of projectile on bumper [J]. Chinese Journal of High Pressure Physics, 2004, 18(1): 47–52. doi: 10.3969/j.issn.1000-5773.2004.01.009 [129] MCQUEEN R G, MARSH S P, FRITZ J N. Ultrabasic rocks and the composition of the upper mantle [J]. Journal of Geophysical Research: Planets, 1967, 72: 4999. doi: 10.1029/JZ072i020p04999 [130] BJORK R L, OLSHAKER A E. The role of melting and vaporization in hypervelocity impact: RM-3490-PR [R]. USA: USA Air Force, 1965. [131] TANG E L, ZHANG Q M, ZHANG J. Preliminary study on diagnostic techniques for transient plasma generated by hypervelocity impact [J]. Plasma Science and Technology, 2008, 10(6): 735–738. doi: 10.1088/1009-0630/10/6/16 [132] KRAUS R G, STEWART S T, SWIFT D C, et al. Shock vaporization of silica and the thermodynamics of planetary impact events [J]. Journal of Geophysical Research, 2012, 117(E9): 371–387. [133] HORNUNG K, MICHEL K W. Equation-of-state data of solids from shock vaporization [J]. Journal of Chemical Physics, 1971, 56(5): 2072–2078. [134] HORNUNG K, MALAMA Y G, THOMA K. Modeling of the very high velocity impact process with respect to in-situ ionization measurements [J]. Advances in Space Research, 1996, 17(12): 77–86. doi: 10.1016/0273-1177(95)00762-4 [135] HORNUNG K. Impact vaporization and ionization of cosmic dust particles [J]. Astrophysics and Space Science, 2000, 274: 355–363. doi: 10.1023/A:1026553502542 [136] POVARNITSYN M E, KHISHCHENKO K V, LEVASHOV P R. Hypervelocity impact modeling with different equations of state [J]. International Journal of Impact Engineering, 2006, 33: 625–633. doi: 10.1016/j.ijimpeng.2006.09.078 [137] BRUNDAGE A L. Implementation of Tillotson equation of state for hypervelocity impact of metals, geologic materials, and liquids [J]. Procedia Engineering, 2013, 58: 461–470. doi: 10.1016/j.proeng.2013.05.053 [138] BERGH M, GARCIA V. A computational models for assessing high-velocity debris impact in space applications [J]. Shock Waves, 2017, 27(4): 675–684. doi: 10.1007/s00193-017-0709-9 [139] TILLOTSON J H. Metallic equations of state for hypervelocity impact: General Atomic Report GA-3216 [R]. San Diego, CA: General Atomic, 1962. [140] SCHONBERG W P. Characterizing material states in orbital debris impacts [J]. Proceedings of SPIE -The International Society for Optical Engineering, 1995, 2483: 31–39. [141] THOMPSON S L, LAUSON H S. Improvements in the Chart D radiation-hydrodynamic CODE III: revised analytic equations of state: SC-RR-71-0714 [R]. Albuquerque, New Mexico: Sandia National Laboratories, 1972. [142] MELOSH H J. A hydrocode equation of state for SiO2 [J]. Meteoritics & Planetary Science, 2007, 42(12): 2079–2098. [143] LITTLEFIELD D L. ANEOS extensions for modeling hypervelocity impact [J]. International Journal of Impact Engineering, 1997, 20: 533–544. doi: 10.1016/S0734-743X(97)87442-8 [144] COLLINS G S, MELOSH H J. Improvments to ANEOS for multiple phase transitions [C]//45th Lunar and Planetary Science Conference, 2014: 2664. [145] JOHNSON J D. The SESAME databse [C]// 12th Symposium on Thermophysical Properties Boulder. Colorado, 1994: LA-UR-9401451. [146] LYON P, JOHNSON J D. SESAME: the Los Alamos national laboratory equation of state database: LA-UR-92-3407 [R]. USA: Los Alamos national laboratory, 1992. [147] 唐蜜, 刘仓理, 李平, 等. 超高速撞击产生碎片云相分布数值模拟 [J]. 强激光与粒子束, 2012, 24(9): 2203–2206.TANG M, LIU C L, LI P. Numerical simulation of phase distribution of debris cloud generated by hypervelocity impact [J]. High Power Laser and Particle Beams, 2012, 24(9): 2203–2206. [148] CHHABILDAS L C, REINHART W D, THORNHILL T F, et al. Debris generation and propagation phenomenology from hypervelocity impacts on aluminum from 6 to 11 km/s [J]. International Journal of Impact Engineering, 2003, 29: 185–202. doi: 10.1016/j.ijimpeng.2003.09.016 [149] ROYCE E B. A three-phase equation of state for metals: UCRL-51121 [R]. USA: Lawrence Livermore Lab, 1971. [150] GROVER R. Liquid metal equation of state based on scaling [J]. Journal of Chemical Physics, 1971, 55(7): 3435–3441. doi: 10.1063/1.1676596 [151] YOUNG D A, ALDER B J. Critical point of metals from the van der Waals model [J]. Physics Review A, 1971, 3(1): 364–371. doi: 10.1103/PhysRevA.3.364 [152] 于继东, 李平, 王文强, 等. 金属铝固液气完全物态方程研究 [J]. 物理学报, 2014, 63(11): 116401. doi: 10.7498/aps.63.116401YU J D, LI P, WANG W Q, et al. A solid-liquid-gas three phase complete equation of state of aluminum [J]. Acta Physica Sinica, 2014, 63(11): 116401. doi: 10.7498/aps.63.116401 [153] 唐蜜. 基于欧拉方法的超高速撞击程序研制及碎片云相分布数值模拟 [D]. 绵阳: 中国工程物理研究院, 2015: 85–98.TANG M. Development of hypervelocity impact codes based on Euler method and numerical study of the phase distribution in debris cloud [D]. Mianyang: China Academy of Engineering Physics, 2015: 85–98. [154] PIEKUTOWSKI A J, POORMON K L. Holes formed in thin aluminum sheets by spheres with impact velocities from 2 to 10 km/s [J]. Procedia Engineering, 2015, 103: 482–489. doi: 10.1016/j.proeng.2015.04.063 [155] PIEKUTOWSKI A J. Formation and description of debris clouds produced by hypervelocity impact: NASA-CR-201000 [R]. USA: NASA, 1995. [156] MESPOULET J, HÉREIL P L, ABDULHAMID H, et al. Experimental study of hypervelocity impacts on space shields above 8 km/s [J]. Procedia Engineering, 2017, 204: 508–515. doi: 10.1016/j.proeng.2017.09.748 [157] HILL S A. Determination of an empirical model for the prediction of penetration hole diameter in thin plates from hypervelocity impact [J]. International Journal of Impact Engineering, 2004, 30(3): 303–321. doi: 10.1016/S0734-743X(03)00079-4 [158] DE CHANT L J. A high velocity plate penetration hole diameter relationship based on late time stagnation point flow concepts [J]. Applied Mathematics and Computation, 2005, 170(1): 410–424. doi: 10.1016/j.amc.2004.12.047 [159] HOSSEINI M, ABBAS H. Growth of hole in thin plates under hypervelocity impact of spherical projectiles [J]. Thin-Walled Structures, 2006, 44(9): 1006–1016. doi: 10.1016/j.tws.2006.08.024 [160] ABBAS H, ALSAYED S H, ALMUSALLAM T H, et al. Characterization of hole-dameter in thin metallic plates perforate by spherical projectiles using genetic algorithms [J]. Archive of Applied Mechanics, 2011, 81(7): 907–924. doi: 10.1007/s00419-010-0459-y [161] ROSENBERG Z, KOSITSKI R. The hole diameter in metallic plates impacted by hypervelocity projectiles [J]. International Journal of Impact Engineering, 2017, 102: 147–155. doi: 10.1016/j.ijimpeng.2016.12.015 [162] SHINAR G I, BARNEA N, RAVID M, et al. An analytical model for the cratering of metallic targets by hypervelocity long rods [C]// 15th International Symposium on Ballistics. Jerusalem, 1995: 59-66. [163] JOLLY W H, SCHONBERG W P. Analytical prediction of hole diameter in thin plates due to hypervelocity impact of spherical projectiles [J]. Shock and Vibration, 1997, 4(5/6): 379–390. [164] 迟润强, 庞宝君, 何茂坚, 等. 球形弹丸超高速正撞击薄板破碎状态实验研究 [J]. 爆炸与冲击, 2009, 29(3): 231–236. doi: 10.3321/j.issn:1001-1455.2009.03.002CHI R Q, PANG B J, HE M J, et al. Experimental investigation for deformation and fragmentation of spheres penetrating sheets at hypervelocity [J]. Explosion and Shock Waves, 2009, 29(3): 231–236. doi: 10.3321/j.issn:1001-1455.2009.03.002 [165] 汪庆桃, 吴克刚, 陈志阳. 圆柱形长杆超高速正碰撞薄板结构破碎效应 [J]. 振动与冲击, 2017, 36(5): 54–60.WANG Q T, WU K G, CHEN Z Y. Fragmentation effect of a long cylindrical rod with a hypervelocity normally impacting a thin plate structure [J]. Journal of Vibration and Shock, 2017, 36(5): 54–60. [166] PIEKUTOWSKI A J. Formation and description of debris cloud produced by hypervelocity impact: NASA-CR-201000 [R]. USA: NASA, 1995. [167] SCHMIDT R M, HOUSEN K R, BJORKMAN M D, et al. Advanced all-metal orbital debris shield performance at 7 to 17 km/s [J]. International Journal of Impact Engineering, 1995, 17: 719–730. doi: 10.1016/0734-743X(95)99894-W [168] POORMON K L, PIEKUTOWSKI A J. Comparisions of cadmium and aluminum debris clouds [J]. International Journal of Impact Engineering, 1995, 17: 639–648. doi: 10.1016/0734-743X(95)99887-W [169] HOPKINS A K, LEE T W, SWIFT H F. Materials phase transformation effects upon performance of spaced bumper systems [J]. Journal of Spacecraft and Rockets, 1970, 9(5): 342–345. [170] ANDERSON C E JR, TRUCANO T G, MULLIN S A. Debris cloud dynamics [J]. International Journal of Impact Engineering, 1990, 9(1): 89–113. doi: 10.1016/0734-743X(90)90024-P [171] BJORK R L. The physics of hypervelocity lethality [J]. International Journal of Impact Engineering, 1987, 5: 129–154. doi: 10.1016/0734-743X(87)90034-0 [172] SHOCKEY D A, CURRAN D R, OSHER J E, et al. Disintegration behavior of metal rods subjected to hypervelocity impact [J]. International Journal of Impact Engineering, 1987, 5: 585–593. doi: 10.1016/0734-743X(87)90073-X [173] PIERAZZO E, VICKERY A M, MELOSH H J. A reevaluation of impact melt production [J]. Icarus, 1997, 127(2): 408–423. doi: 10.1006/icar.1997.5713 [174] QUINTANA S N, CRAWFORD D A, SCHULTZ P H. Analysis of impact melt and vapor production in CTH for planetary applications [J]. Procedia Engineering, 2015, 103: 499–506. doi: 10.1016/j.proeng.2015.04.065 [175] POVARNITSYN M E, KHISHCHENKO K V, LEVASHOV P R. Simulation of shock-induced fragmentation and vaporization in metals [J]. International Journal of Impact Engineering, 2008, 35(12): 1723–1727. doi: 10.1016/j.ijimpeng.2008.07.011 [176] 宋卫东, 吕旸涛, 栗建桥. 超高速碰撞产生等离子体的电磁特性研究[C]// 第十四届全国物理力学学术会议缩编文集, 2016. [177] 龙仁荣, 张庆明. 超高速弹丸碰撞薄板产生碎片云的运动模型分析 [J]. 北京理工大学学报, 2009, 29(3): 193–196.LONG R R, ZHANG Q M. Dynamic model for debris clouds produced from impacts of hypervelocity projectiles with thin sheets [J]. Transactions of Beijing Institute of Technology, 2009, 29(3): 193–196. [178] SWIFT H F. Impact dynamics [M]. New York: John Wiley & Sons, 1982. [179] PIEKUTOWSKI A J. A simple dynamic model for the formation of debris clouds [J]. International Journal of Impact Engineering, 1990, 10(1): 453–471. [180] BLESS S J. Bumper debris cloud structure estimated by shock calculations [J]. Journal de Physique III, 1991, 1(3): 903–908. [181] 郑建东, 龚自正, 席爽, 等. 超高速撞击碎片云模型研究综述[C]//第六届全国空间碎片学术交流会, 2011: 671–682.ZHENG J D, GONG Z Z, XI S, et al. Review of debris cloud models produced by hypervelocity impact of space debris [C]// 6th Symposium on debris in space, 2011: 671–682. [182] NEBOLSINE P E, GELB A, LEGNER H H, et al. Simple model for the debris velocity and distribution due to a catastrophic impact [C]// AIAA Space Programs and Technologies Conference and Exhibit. USA: AIAA, 1994 [183] SCHONBERG W P, WILLIAMSEN J E. Empirical hole size and crack length models for dual-wall systems under hypervelocity projectile impact [J]. International Journal of Impact Engineering, 1997, 20(6): 711–722. [184] 迟润强. 弹丸超高速撞击薄板碎片云建模研究[D]. 哈尔滨: 哈尔滨工业大学, 2010: 100–120.CHI R Q. Research and modeling of debris cloud produced by hypervelocity impact of projectile with thin plate [D]. Harbin: Harbin Institute of Technology, 2010: 100–120. [185] 管公顺, 朱耀, 迟润强, 等. 铝双层板结构撞击损伤的板间距效应实验研究 [J]. 材料科学与工艺, 2008, 16(5): 692–695. doi: 10.3969/j.issn.1005-0299.2008.05.025GUAN G S, ZHU Y, CHI R Q, et al. Experimental investigation of space effect on damage of aluminum dual-wall structure by hypervelocity impact [J]. Materials Science & Technology, 2008, 16(5): 692–695. doi: 10.3969/j.issn.1005-0299.2008.05.025 [186] PIEKUTOWSKI A J, POORMON K L, CHRISTIANSEN E L, et al. Performance of Whipple shields at impact velocities above 9 km/s [J]. International Journal of Impact Engineering, 2011, 38(6): 95–503. [187] COUR-PALAIS B G. Hypervelocity impact in metals, glass and composites [J]. International Journal of Impact Engineering, 1987, 5: 221–237. doi: 10.1016/0734-743X(87)90040-6 [188] PIEKUTOWSKI A J. Debris clouds generated by hypervelocity impact of cylindrical projectiles with thin aluminum plates [J]. International Journal of Impact Engineering, 1987, 5: 509–518. doi: 10.1016/0734-743X(87)90066-2 [189] VERMA P N, DHOTE K D. Characterising primary fragment in debris cloud formed by hypervelocity impact of spherical stainless steel projectile on thin steel plate [J]. International Journal of Impact Engineering, 2018, 120: 118–125. doi: 10.1016/j.ijimpeng.2018.05.003 [190] SCHONBERG W P. Concise history of ballistic limit equations for multi-wall spacecraft shielding [J]. REACH-Reviews in Human Space Exploration, 2016(1): 46–54. [191] 袁俊刚, 曲广吉, 闫军. 国外空间碎片防护结构弹道极限方程分析 [J]. 空间碎片, 2007, 7(7): 21–25.YUAN J G, QU G J, YAN J. Analysis for development of ballistic limit equations of space debris shield configurations from other countries [J]. Space Debris Research, 2007, 7(7): 21–25. [192] 闫军, 曲广吉, 郑世贵. 空间碎片超高速撞击弹道极限方程的研究评述 [J]. 航天器工程, 2005, 14(2): 42–46.YAN J, QU G J, ZHENG S G. Comments on the ballistic limit equations of space debris with hypervelocity [J]. Spacecraft Engineering, 2005, 14(2): 42–46. [193] HAYASHIDA K B, ROBINSON J H. Double-plate penetration equations: NASA/TM-2000-209907 [R]. USA: NASA Marshall Space Flight Cener, 2000. [194] LI W. The relationship between Brinell hardness and strength of material [J]. Heavy Cast Forg, 1994, 65(3): 48–51. [195] ZHANG X, JIA G, HUANG H. An approach for constituting double/multi wall BLE by single wall BLE of spacecraft shield [J]. International Journal of Impact Engineering, 2014, 69: 114–121. doi: 10.1016/j.ijimpeng.2014.02.009 [196] FISH R H, SUMMERS J L. The effect of material properties on threshold penetration [C]// Proceedings of the Seventh Hypervelocity Impact Symposium. Tampa, 1965. [197] FROST V C. Meteoroid damage assessment: NASA SP-8042 [R]. USA: NASA, 1970. [198] CORONADO A R, GIBBINS M N, WRIGHT M A, et al. Space station integrated wall design and penetration damage control: NAS8-36426 [R]. USA: NASA, 1987. [199] HOLSAPPLE K A, SCHMIDT R M. On the scaling of crater dimensions 2 impact process [J]. Journal of Geophysical Research, 1982, 87: 1849–1870. doi: 10.1029/JB087iB03p01849 [200] COUR-PALAIS B G. Hypervelocity impact investigations and meteoroid shielding experience related to Apollo and Skylab: NAS-S-82-05009 [R]. USA: NASA, 1984. [201] HAYASHIDA K B, ROBINSON J H. Single wall penetration equations: NASA TM-103565 [R]. USA: NASA, 1991. [202] CHRISTIANSEN E L. Shield sizing and response equations: NASA-SN3-91-42 [R]. USA: NASA, 1991. [203] LEE M, CHO Y J. Characterization of the ballistic limit curve for hypervelocity impact of sphere onto single plate [J]. Journal of Mechanical Science and Technology, 2011, 25(9): 2457–2463. doi: 10.1007/s12206-011-0716-1 [204] 贾斌, 盖芳芳, 马志涛, 等. 5A06铝合金单层板超高速撞击弹道极限分析 [J]. 材料科学与工艺, 2007, 15(5): 636–639. doi: 10.3969/j.issn.1005-0299.2007.05.011JIA B, GAI F F, MA Z T. Ballistic limit analysis of aluminum 5A06 single wall plate subjected to hypervelocity impact [J]. Materials Science & Technology, 2007, 15(5): 636–639. doi: 10.3969/j.issn.1005-0299.2007.05.011 [205] 徐小刚, 贾光辉, 黄海. 单层板超高速撞击弹道极限方程综合建模 [J]. 弹箭与制导学报, 2007, 15(5): 636–639.XU X G, JIA G H, HUANG H. Integrated modeling of ballistic limit equations of single plate under hypervelocity impact [J]. Journal of Projectiles, Rockets, Missiles and Guidance, Materials Science & Technology, 2007, 15(5): 636–639. [206] 张晓天, 谌颖, 贾光辉. 航天器单层板结构弹道极限的支持向量机预测模拟 [J]. 宇航学报, 2014, 35(3): 298–305. doi: 10.3873/j.issn.1000-1328.2014.03.008ZHANG X T, CHEN Y, JIA G H. Support vector machine model for spacecraft single wall ballistic limit prediction [J]. Journal of Astronautics, 2014, 35(3): 298–305. doi: 10.3873/j.issn.1000-1328.2014.03.008 [207] COUR-PALAIS B G. Meteoroid protection by multi-wall structures [C]//AIAA Hypervelocity Impact Conference. Cincinnati, 1969: 69–372. [208] REIMERDES H G, NLKE D, SCHÄFER F. Modified Cour-Palais/Christiansen damage equations for double-wall structures [J]. International Journal of Impact Engineering, 2006, 33: 645–654. doi: 10.1016/j.ijimpeng.2006.09.036 [209] CHRISTIANSEN E L, KERR J H. Ballistic limit equations for spacecraft shielding [J]. International Journal of Impact Engineering, 2001, 26(1): 93–104. [210] 贾光辉, 张平, 李轩, 等. 双层板弹道极限方程的速度区间修正方法 [J]. 空间碎片研究与应用, 2012, 12(4): 25–30.JIA G H, ZHANG P, LI X, et al. Whipple ballistic limit equations optimization method via correcting the velocity region [J]. Space Debris Research and Application, 2012, 12(4): 25–30. [211] RYAN S, CHRISTIANSEN E L. A ballistic limit analysis programme for shielding against micrometeroids and orbital debris [J]. Acta Astronautica, 2011, 69(5/6): 245–257. [212] RYAN S, THALER S. Artificial neural networks for characterizing Whipple shield performance [J]. International Journal of Impact Engineering, 2013, 56: 61–70. doi: 10.1016/j.ijimpeng.2012.10.011 [213] MILLER J E, BJORKMAN M D, CHRISTIANSEN E L, et al. Analytic ballistic performance model of Whipple shields [J]. Procedia Engineering, 2015, 103: 389–397. doi: 10.1016/j.proeng.2015.04.037 [214] 袁俊刚, 曲广吉, 韩增尧, 等. 空间碎片防护结构弹道极限方程综合建模研究 [J]. 空间碎片研究, 2008, 8(2): 14–19.YUAN J G, QU G J, HAN Z Y, et al. Modeling ballistic limit of M/OD Whipple shield [J]. Space Debris Research, 2008, 8(2): 14–19. [215] 郑建东, 龚自正, 童靖宇, 等. 新型高精度Whipple防护结构弹道极限方程的精度对比 [J]. 空间碎片研究与应用, 2012, 12(1): 28–32.ZHENG J D, GONG Z Z, TONG J Y. Accuracy comparison of a new type with high accuracy Whipple shield ballistic limit equations [J]. Space Debris Research and Application, 2012, 12(1): 28–32. [216] 贾光辉, 欧阳智江, 蒋辉, 等. Whipple防护结构弹道极限方程的多指标修正 [J]. 宇航学报, 2013, 34(12): 1651–1656. doi: 10.3873/j.issn.1000-1328.2013.12.016JIA G H, OU Y Z Z, JIANG H, et al. Multiple indicator correction for Whipple shield ballistic limit equation [J]. Journal of Astronautics, 2013, 34(12): 1651–1656. doi: 10.3873/j.issn.1000-1328.2013.12.016 -








 下载:
下载:
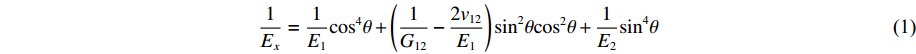
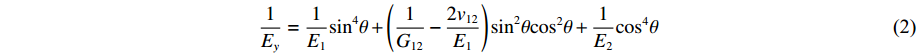
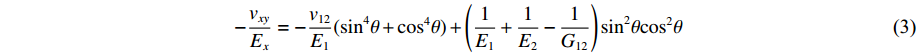
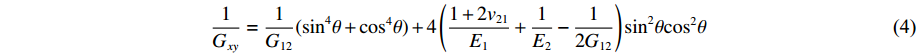
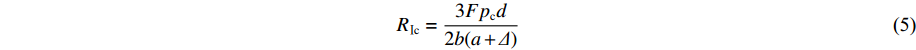

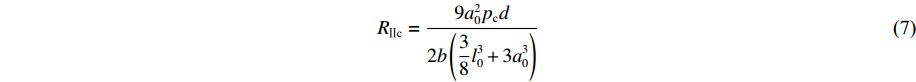




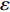

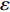
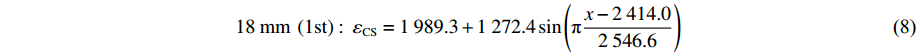
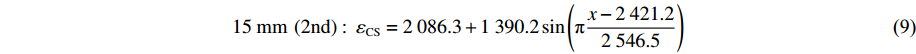
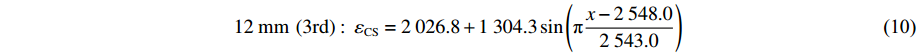
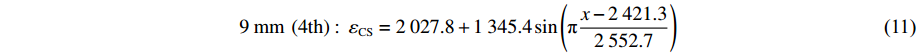
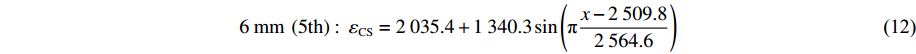
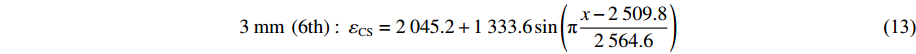

 下载:
下载: