Review on Evaluation of Temperature-Pressure Equation of State of Porous Materials
-
摘要: 多孔材料是一种重要的结构和功能材料,在过滤、催化、屏蔽和冲击防护等重要工程领域具有广泛的应用。多孔材料的物理力学行为极其复杂,虽然经过多年研究,但是仍未完全理解其在极端条件下的响应行为。以冲击加载下多孔材料的压力和温度变化特点为例,对目前常用的几种具有代表性的以密实材料Hugoniot为参照线的多孔材料物态方程模型进行深入分析,并对多种模型的优劣进行比较,在此基础上提出了一种分段处理多孔材料冲击波数据的方法。以多孔Cu为例,展示了该方法的有效性。这种方法将为发展更为精细严格的多孔材料状态方程理论提供有益的参考。Abstract: As an important structural and functional material, porous material has been widely used in the engineering fields such as filtration, catalysis, shielding and impact protection. Because of its complex physical-mechanical behavior, even with years of research, its response under extreme conditions still has not been fully understood. Taking the characteristics of pressure and temperature change of porous materials under shock loading as an example, this paper makes an in-depth analysis of several typical models of equation of state for porous materials by choosing Hugoniot of the dense material as the reference line and compares them. On the basis of this analysis, an approach of piecewise processing the shock data of porous materials is proposed and its effectiveness is demonstrated for porous copper. This approach may be helpful in developing an accurate and rigorous theory of the equation of state of porous materials.
-
Key words:
- porous materials /
- Debye temperature /
- equation of state /
- shock Hugoniot
-
仿贝壳珍珠层复合材料作为新型复合材料,有着优异的力学性能,不仅具有很高的强度,而且具有很好的韧性,近年来引起了学术界的不断关注[1-6]。很多发达国家非常重视贝壳结构材料和仿生材料研究,如美国等国家设置了专门的经费来研究贝壳生物材料的仿生设计和性能,用于装甲防弹衣和防爆装置。贝壳珍珠层复合材料的优异力学性能与其微观结构密切联系,为此研究人员对珍珠质的微观结构特征(体积分数、片剂长宽比、重叠长度等)进行了深入分析,试图将其与模型的力学性能联系起来[7-12]。Dutta等[13]研究了珍珠层中裂纹的萌生规律,评估了重叠长度对裂纹尖端驱动力的影响。Kotha等[14]的研究显示,低纵横比的文石片可以制造出具有高韧性的复合材料。Barthelat等[15]发现,珍珠层没有实现稳定状态的裂纹扩展,并将其归因于片层拔出增韧机制。其他学者也发现珍珠层内部和外部韧化机制阻止了裂纹的扩展[16-21]。
Barthelat等[15]通过观察发现,在每层贝壳珍珠层中,平板的排列与Voronoi图相似,从一个红色鲍鱼标本的光学图像中可以看到每个贝壳层压板都有矿物片的随机分布,并与其他珍珠层成键。基于这些光学图像,他们生成了一个由两层贝壳的平板结构组成的几何模型,用于有限元分析。自1907年Shamos和Hoey提出分治算法的最初定义和描述之后,Voronoi图便成为众多学科的中心主题之一。Voronoi图所具有的自然描述性和操纵能力,使其获得了广泛应用[22-24]。尽管Voronoi图对科学和工程中的各种应用具有重大的潜在影响,但是在很多领域包括仿生结构领域,Voronoi结构对材料力学性能的影响还未得到透彻的理解,为此本工作将探讨Voronoi结构的随机性对仿贝壳珍珠层结构力学性能的影响。
为了研究仿贝壳珍珠层Voronoi随机模型结构的动态力学响应,首先建立一种铝/乙烯基复合材料结构的三维Voronoi模型,然后对模型在弹丸冲击载荷下的动态力学性能进行有限元模拟分析,最后讨论黏结层厚度和Voronoi模型分块尺寸对模型抗冲击力学性能的影响。
1. Voronoi随机模型生成
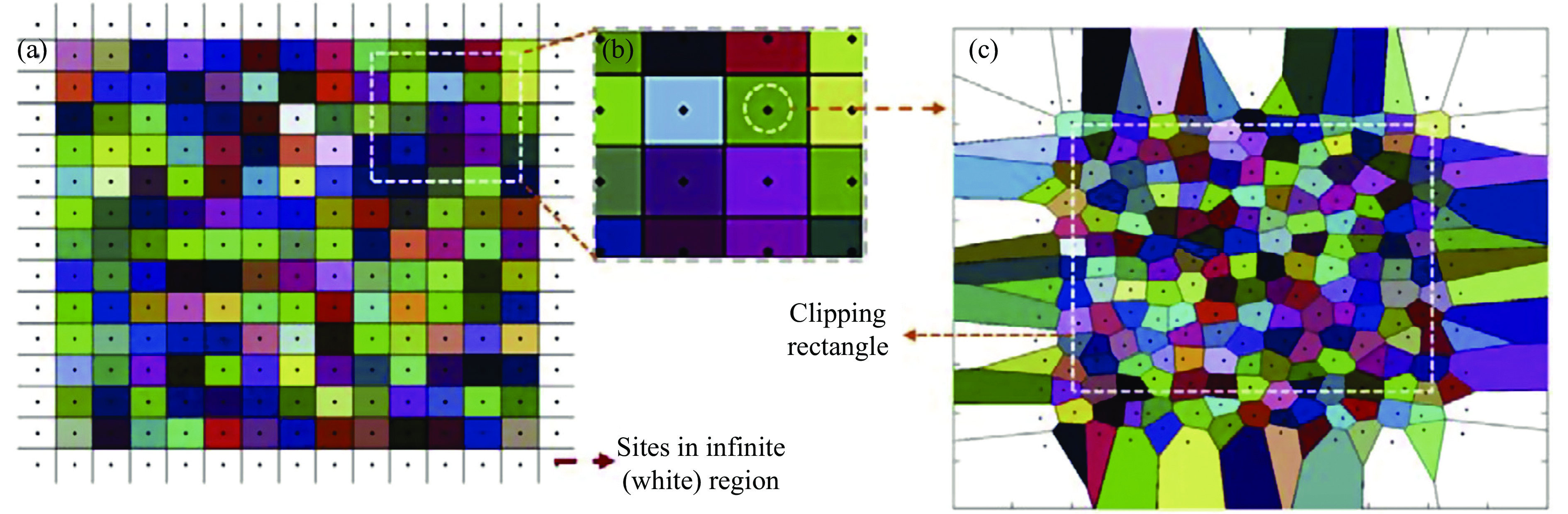
利用文献[25]给出的随机Voronoi技术生成Voronoi随机模型。图1描述了仿贝壳珍珠层随机Voronoi结构的生成技术。图1(a)显示了由网格组成的Voronoi初始构型,每个网格内都包含1个站点;站点可以在圆内随机移动,如图1(b)所示,站点位置
(x,y) 由极坐标控制方程决定 图 1 (a) Voronoi图的初始网格构型;(b)每个站点都在一个圆圈区域内随机移动;(c)新的Voronoi图是从新的站点系统中生成,通过矩形裁剪,形成有限区域的随机Voronoi图[25]Figure 1. (a) Initial grid formation of Voronoi diagram; (b) each site moves randomly within a circle region; (c) a new Voronoi diagram is generated from the new site system, and by a rectangle cut, a finite Voronoi diagram is generated[25]
图 1 (a) Voronoi图的初始网格构型;(b)每个站点都在一个圆圈区域内随机移动;(c)新的Voronoi图是从新的站点系统中生成,通过矩形裁剪,形成有限区域的随机Voronoi图[25]Figure 1. (a) Initial grid formation of Voronoi diagram; (b) each site moves randomly within a circle region; (c) a new Voronoi diagram is generated from the new site system, and by a rectangle cut, a finite Voronoi diagram is generated[25]x=x0+rcosθ,y=y0+rsinθ (1) 式中:
(x0,y0) 为网格内站点的参考位置;r 为(0,R) 之间的随机值,R 为圆的半径;θ 为(0,2π) 之间的随机值。通过站点的随机移动,最终形成新的随机Voronoi图,如图1(c)所示。新生成的Voronoi图由一个无限大的区域组成,采用矩形切割使新生成的Voronoi图限定在有限区域内,丢弃有限区域外的站点和多边形网格,最终形成仿贝壳珍珠层随机Voronoi模型。2. Voronoi模型有限元分析
2.1 几何建模
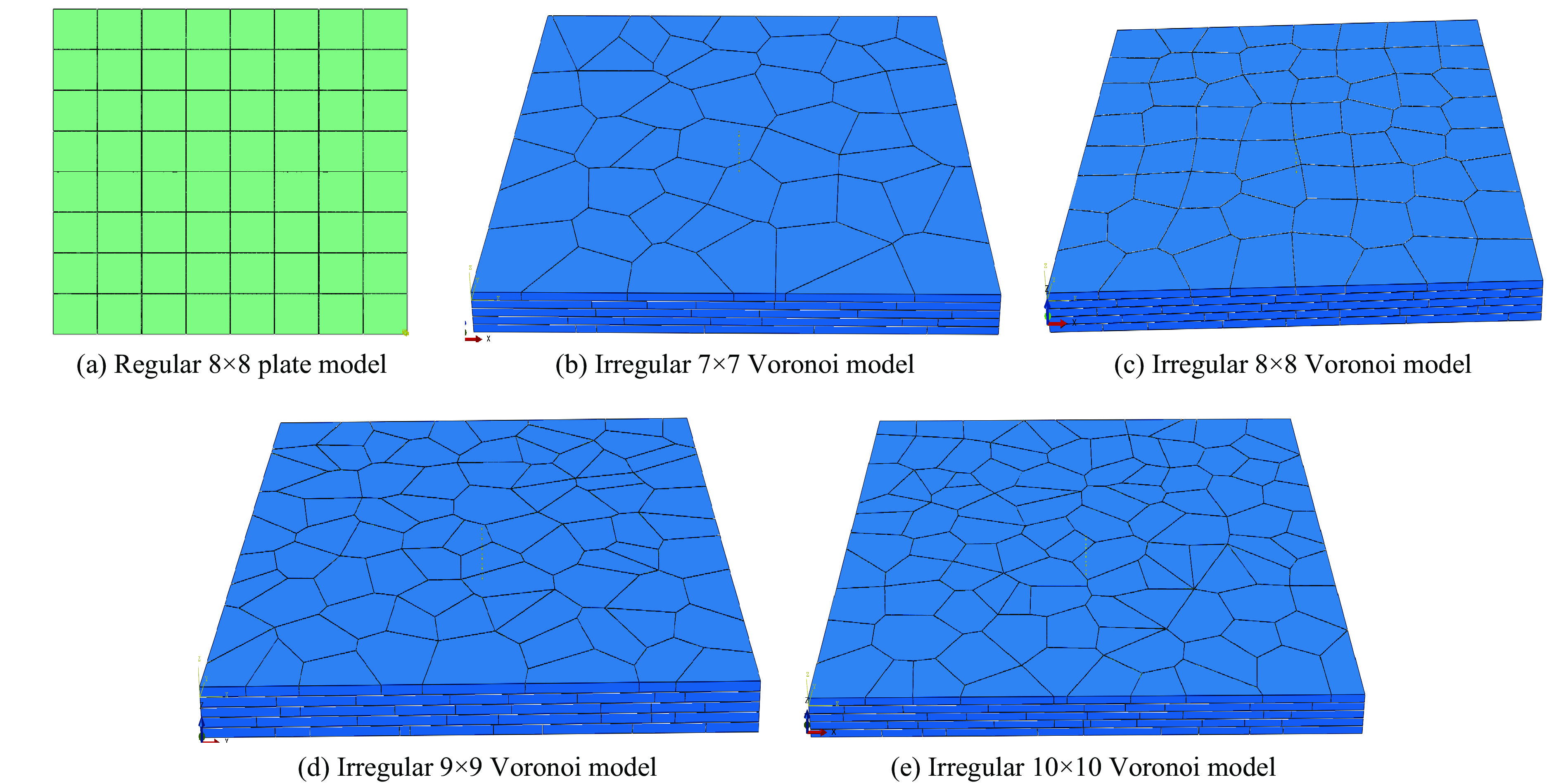
图2给出了规则片板单元模型以及4种不同分块尺寸的不规则Voronoi片板模型,5种模型的总体几何尺寸相同,均为240 mm × 240 mm × 15 mm,模型总层数均为5层。图2(a)为规则片板模型,每个规则片板的几何尺寸为30 mm × 30 mm × 3 mm,每层由8 × 8共64个片板组成,图2(b)~图2(e)分别给出了7 × 7、8 × 8、9 × 9和10 × 10分块的Voronoi不规则模型,每种模型均包括5层不同的随机单层结构,每层厚度为3 mm。
为了模拟仿贝壳珍珠层片层之间受冲击破坏时的脱黏现象,采用了内聚力Cohesive模型。通过合理的参数选择,内聚力Cohesive模型能够部分描述贝壳珍珠层内部层与层之间的变形和失效现象[2]。在片板之间以及板层之间插入Cohesive黏结层,考虑3种黏结层厚度0.1、0.2和0.3 mm,讨论黏结层厚度对模型冲击损伤的影响。
内聚力Cohesive模型的牵引分离定律涉及黏性牵引应力矢量
T={tn,ts,tt} ,其中下标n、s和t分别表示一个法向和两个切向分量。这些变量之间满足双线性二次黏聚律[25](tnt0n)2+(tst0s)2+(ttt0t)2=1 (2) 式中:
t0n 、t0s 、t0t 分别表示变形垂直于界面以及在第一、第二剪切方向上的最大应力。Cohesive黏结层刚度退化速率满足
(GnG0n)2+(GsG0s)2+(GtG0t)2=1 (3) 式中:
Gn 、Gs 、Gt 分别为正向和两个切向的断裂能,G0n 、G0s 、G0t 分别为正向和两个切向引起破坏所需的最大断裂能。2.2 材料参数
仿贝壳珍珠层三维Voronoi结构模型包括两种材料模型,其中片层采用铝AA5083-H116,片层之间Cohesive黏性层使用乙烯树脂材料。表1和表2列出了两种材料参数[19, 25],其中
ρ 为密度,ν 为泊松比,E为弹性模量,Es和Et为两个切向弹性模量。表 1 铝片的材料参数Table 1. Parameters of aluminum plateMaterial ρ/(kg·m−3) ν E/GPa Aluminum 2750 0.3 72 表 2 Cohesive模型的材料参数Table 2. Parameters of Cohesive modelt0n/MPa t0s/MPa t0t/MPa G0n/(kJ·m−2) G0s/(kJ·m−2) G0t/(kJ·m−2) ρ/(kg·m−3) Es/GPa Et/GPa 80 80 80 1 1 1 1 850 4 1.5 在大应变情况下,铝合金的本构关系可采用Johnson-Cook模型描述
σ=(A+Bεn)(1+Cln˙ε˙ε0)[1−(T−TrT−Tm)m] (4) 式中:
σ 为等效应力,A 为材料在常温准静态下的屈服强度,B 为应变强化系数,n 为强化指数,ε 为等效塑性应变,˙ε 为塑性应变率,˙ε0 为初始应变率,C 为应变率敏感系数,m 为温度软化系数,Tm 为材料的熔点,Tr 为参考温度(取常温),T 为当前温度。表3列出了Johnson-Cook模型参数[25]。2.3 加载条件与边界条件
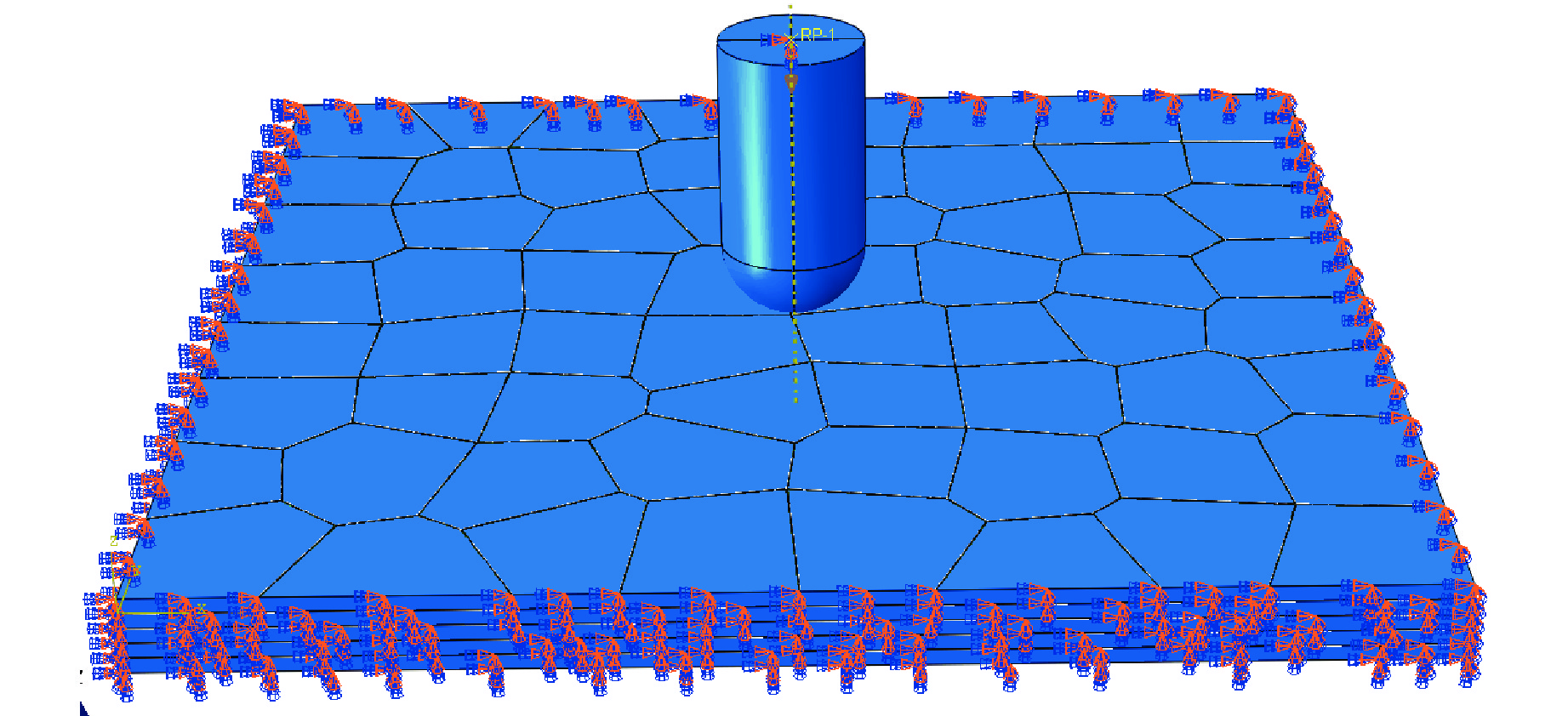
图3显示了模型的边界条件和加载条件。弹丸以18 m/s的初速度冲击Voronoi模型,弹丸速度属于中低速范围。弹丸模型上半部分是一个半径15 mm、长45 mm的圆柱体,下半部分是一个半径为15 mm的半球体,总长为60 mm,刚体属性。冲击载荷下仿贝壳珍珠层三维Voronoi模型的边界条件为4个侧边均完全固定,弹丸与复合结构模型的接触为通用接触,弹丸作用在复合结构模型中心。
黏结层网格类型采用COH3D8,铝片网格类型采用C3D8R,黏结层网格大小为1 mm,铝片网格大小为2 mm。在该网格密度下,模型的网格单元总数达到167230。节点总数为567001时,最大应力值保持稳定,网格的收敛性较好。
3. 模拟结果分析
3.1 有限元分析
在珍珠层结构中,片板滑动机制被认为是激活内在和外在韧化机制的关键因素,可以阻止裂纹扩展。该机制分别引起内聚力和残余塑性应变,从而闭合裂纹。由于弹丸冲击载荷方向垂直于Voronoi板模型,冲击载荷破坏的主要形式是黏性层剥离,因此片板滑动引起的增韧机制在这种特定的冲击加载问题中不占主导地位。在冲击载荷下,损伤和变形耗散的能量比摩擦接触要多得多,Voronoi模型对珍珠层结构负载分配和能量吸收机制的影响是所要考虑的主要因素。本研究首先分析冲击载荷下模型的动态响应,在此基础上考察不规则Voronoi片板等几何因素对动态力学特性和能量分配的影响。
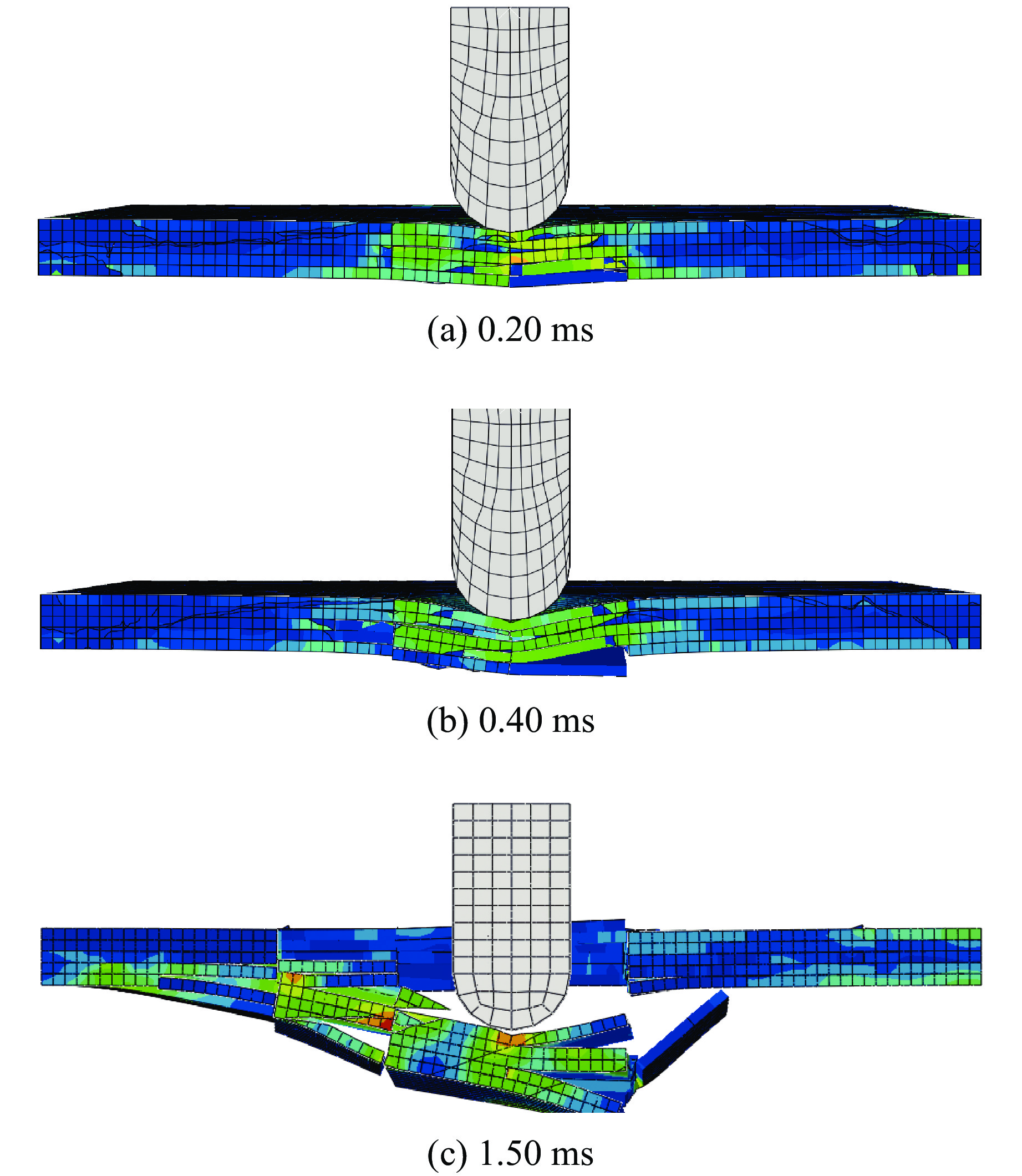
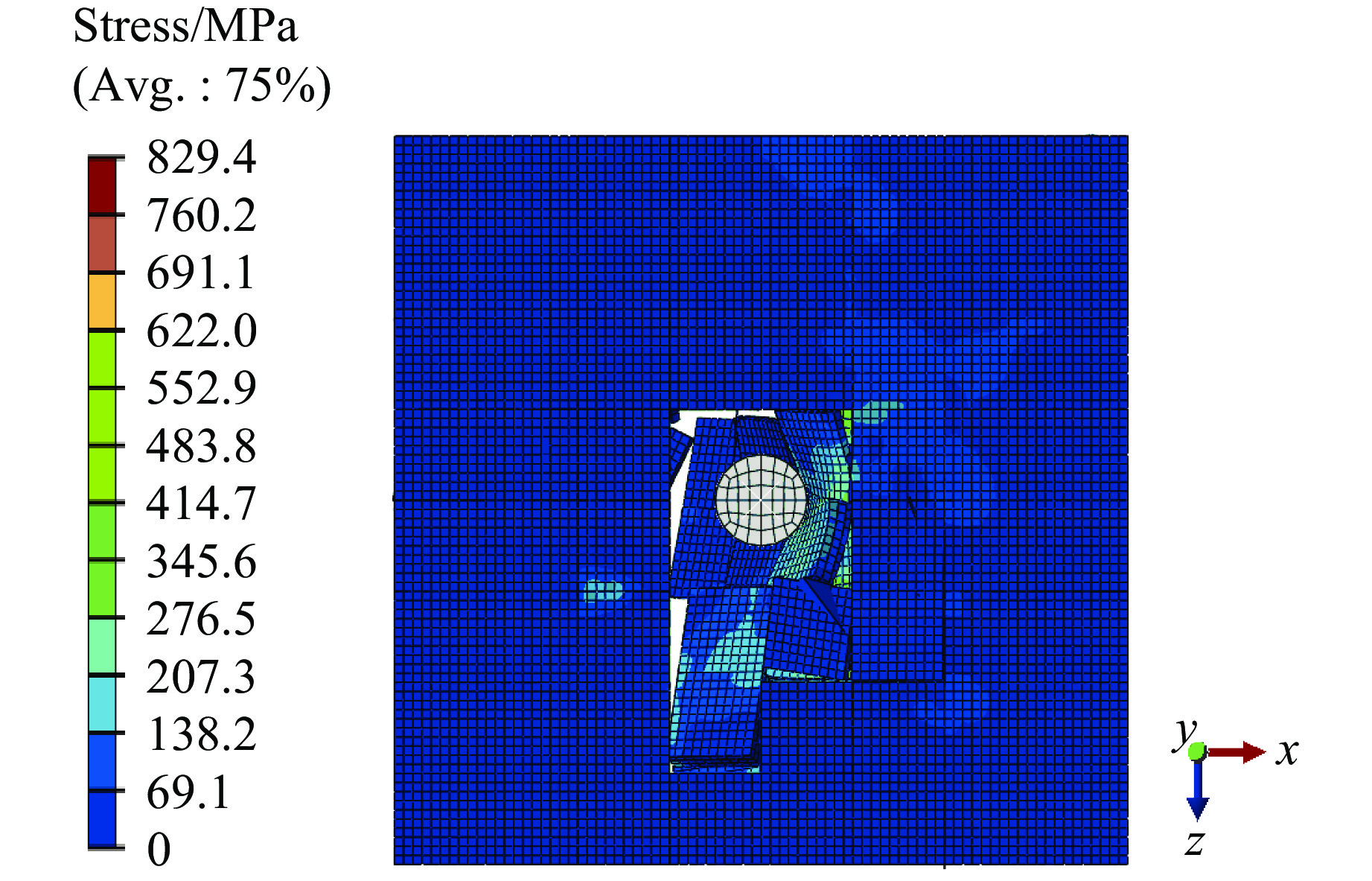
图4和图5分别为不同时刻规则片板模型与Voronoi片板模型受弹丸冲击时的应力云图剖视图。通过对比可以发现:在规则模型中,应力主要集中在弹丸冲击点及附近区域,远离冲击点区域的应力很小;在Voronoi模型中,应力分布区域更大,受力更加均匀。规则模型受冲击后很快就被冲破;而Voronoi模型的冲击模拟结果显示,其最大应力载荷小于规则模型,最终弹丸并未完全贯穿模型。
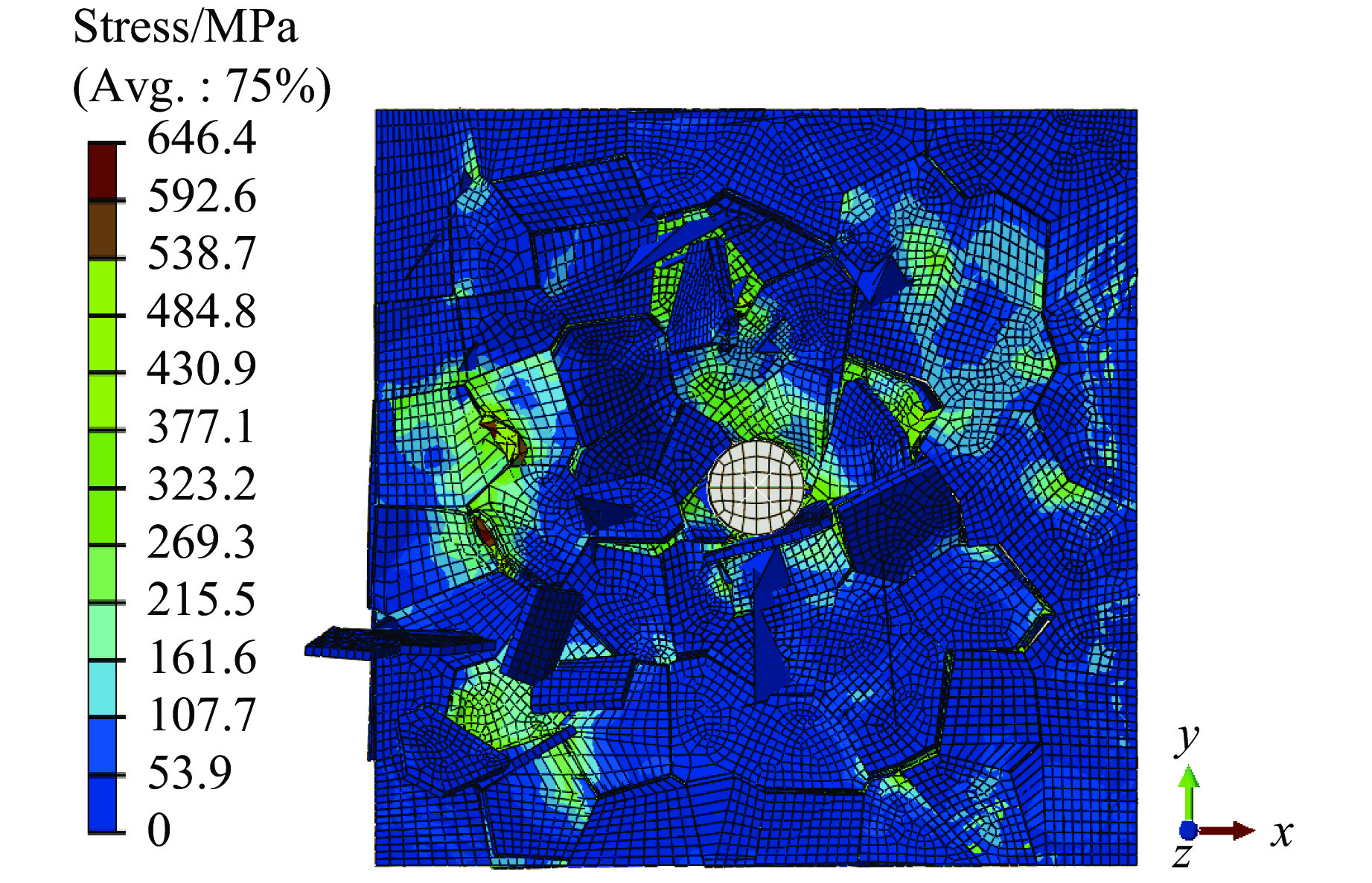
图6和图7分别为规则片板模型和Voronoi片板模型受弹丸冲击3.00 ms时应力云图的俯视图。从受弹丸冲击破坏情况来看:规则模型中脱黏现象主要集中在弹丸冲击点附近区域;而Voronoi模型的冲击影响区域更大,基本遍布整个模型。对于Voronoi模型,冲击区域发生变形时,其余片板受挤压后也发生了脱黏现象,吸收更多的冲击能量,从而有利于冲击能量的扩散与吸收,使模型的更多部分承担冲击负载,即增加承载区域,减小应力集中,更好地发挥能量共享机制。因此Voronoi片板模型抵抗冲击荷载的能力明显优于规则片板模型。
3.2 黏结层厚度和分块尺寸对冲击吸能的影响
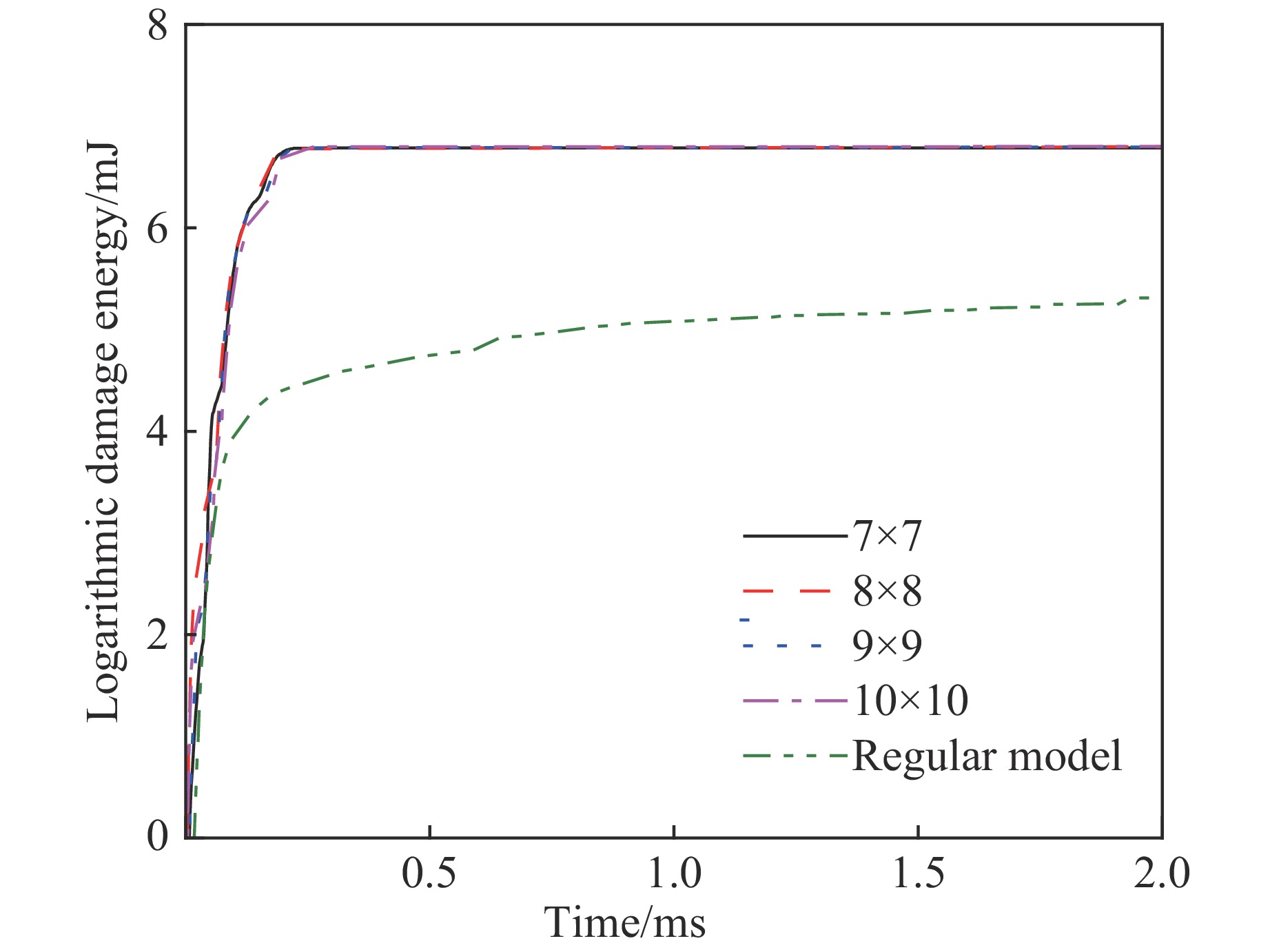
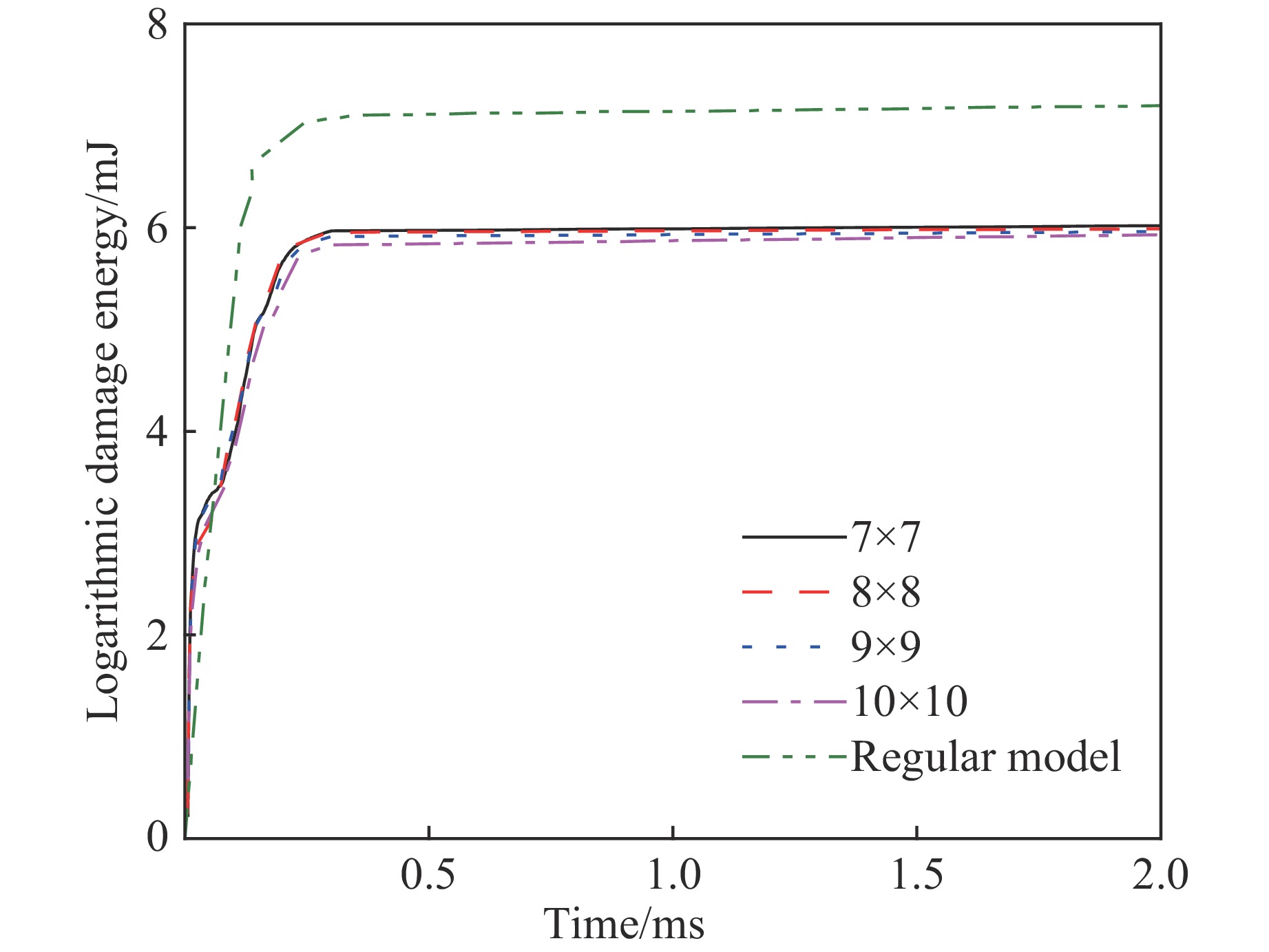
图8和图9分别给出了规则模型和不同分块Voronoi模型的损伤耗散能和塑性耗散能对比。在模型总尺寸相同的情况下,Voronoi模型的损伤耗散能远远高于规则片板模型,而塑性耗散能则小于规则片板模型,说明在冲击载荷作用下Voronoi模型抵抗冲击的能力优于规则片板模型。不同分块Voronoi模型的损伤耗散能和塑性耗散能差别不大,分块尺寸对Voronoi模型抗冲击性能的影响很小。
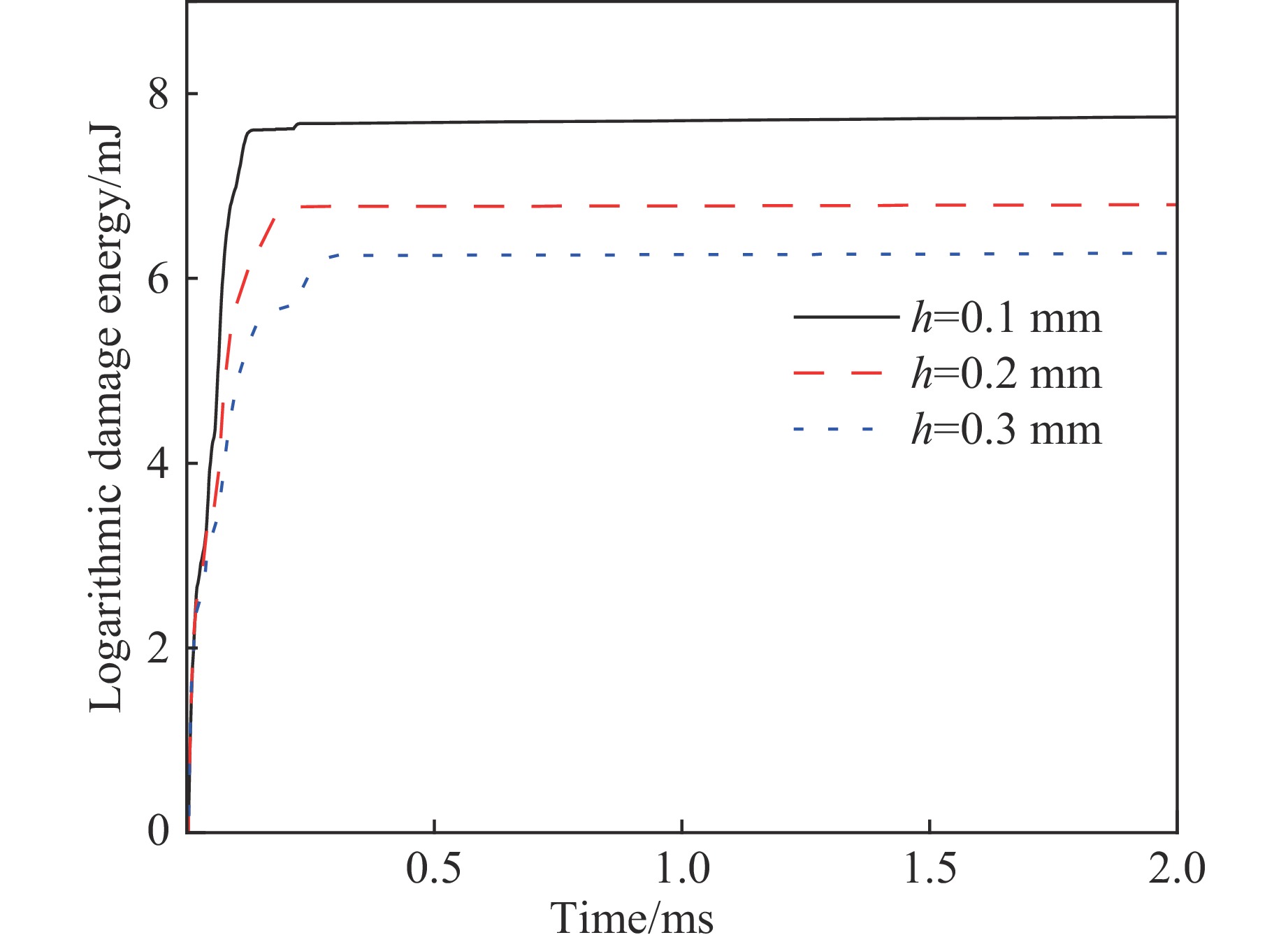
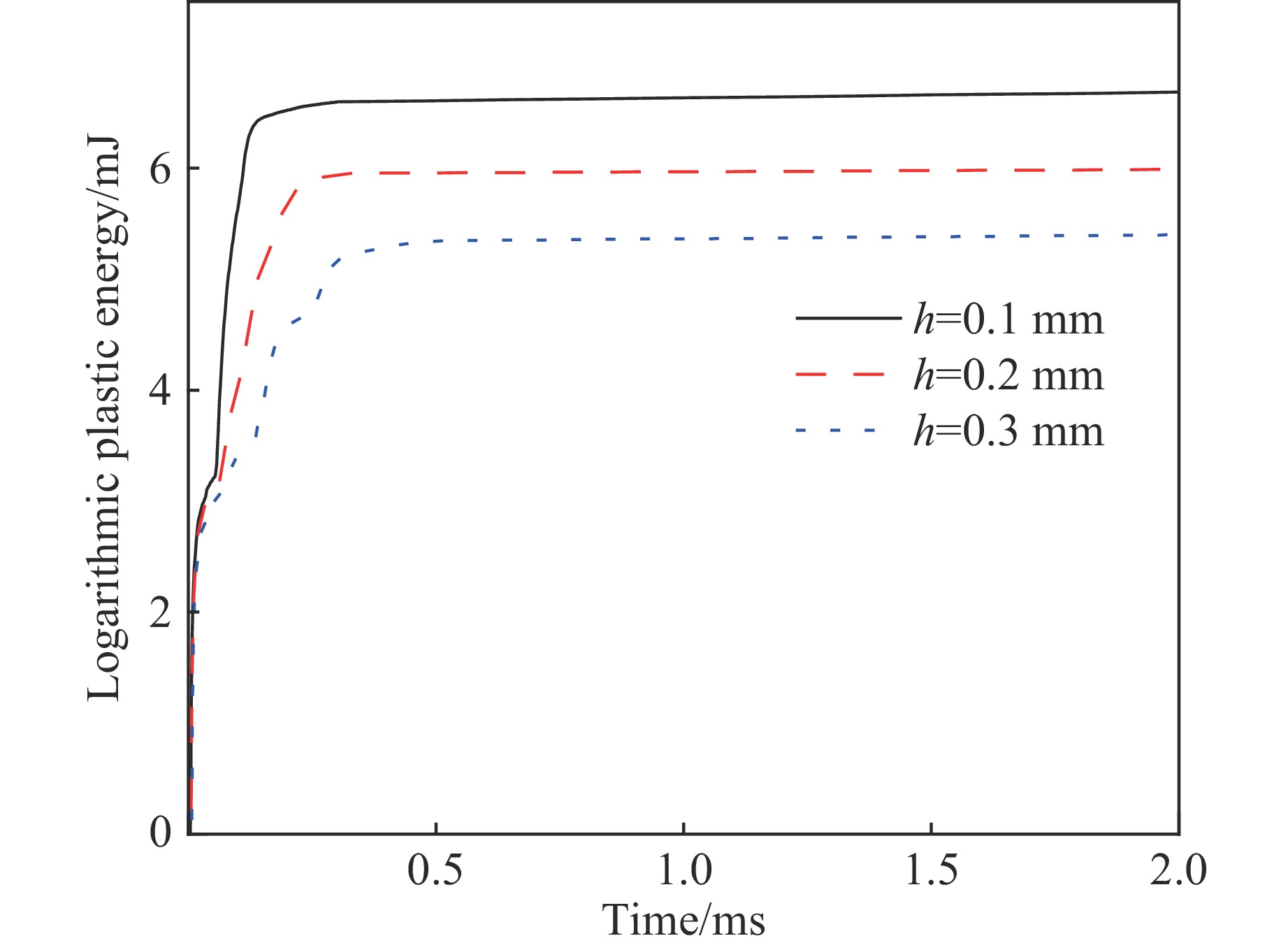
图10和图11分别给出了不同黏结层厚度(h)的Voronoi模型的损伤耗散能和塑性耗散能。可以看出,黏结层对损伤耗散能和塑性耗散能的影响很明显。黏结层越薄,模型整体吸能越大,越薄的黏结层使模型具有更高的抗弯刚度,抗冲击性能越强。由此可见,Voronoi模型的不规则性是仿贝壳珍珠层复合结构模型抗冲击性能的影响因素,对贝壳结构韧性的提升发挥着重要作用。
4. 结 论
通过有限元数值模拟研究了仿贝壳珍珠层Voronoi模型在弹丸冲击载荷下的动态力学响应,得到如下主要结论。
(1)从冲击破坏受损情况来看,不规则Voronoi模型的冲击影响区域比规则模型更大,基本遍布整个模型。对于Voronoi模型,当冲击区域发生变形时,其余片板受挤压后发生脱黏现象,从而吸收更多的冲击能量,有利于冲击能量的扩散与吸收,让模型的更多部分承担冲击负载,即增加承载区域,并且减小应力集中,更好地发挥共享机制。规则模型的脱黏现象主要集中在弹丸冲击点及其附近区域。
(2)在模型总尺寸相同的情况下,Voronoi片板模型的损伤耗散能远远高于规则片板模型,而塑性耗散能则小于规则模型。在冲击载荷作用下,不规则Voronoi片板模型抵抗冲击的能力优于规则片板模型。
(3)分块尺寸对Voronoi模型抗冲击性能的影响很小,而黏结层对损伤耗散能和塑性耗散能的影响很明显,黏结层越薄,模型整体吸能越大,抗冲击性能越强。
鉴于目前制备具有Voronoi结构的金属/高分子材料复合实验模型具有一定困难,因此未对模拟结果进行实验验证。随着3D打印技术的进一步发展,可采用金属/高分子材料混合3D打印技术制备仿贝壳珍珠层Voronoi实验模型,届时即可对铝/乙烯基复合三维Voronoi模型的数值模拟工作进行验证,进而开展更深入的研究。
-
表 1 不同孔隙度Cu的D-u数据拟合参数
Table 1. The parameters for the D-u data of Cu with high porosity
ρ00/(g·cm–3) m c0/(km·s–1) λ λ' 7.900 1.13 1.920 46 2.414 25 –0.146 04 7.315 1.22 1.168 50 2.684 83 –0.199 24 6.326 1.41 0.421 78 2.694 98 –0.183 45 5.742 1.56 0.247 99 2.476 93 –0.117 16 4.508 1.98 0.090 92 2.105 15 –0.080 52 表 2 低孔隙度Cu冲击固相温度与液相温度函数参数
Table 2. The parameters for the shock temperature in the solid and liquid phases of Cu with low porosity
ρ00/(g·cm–3) TH=a+bpH+cp2H+dp3H TL=a+bpL+cp2L+dp3L a b c d a b c d 7.900 722.153 20 –17.753 26 0.735 67 –0.002 68 5911.275 89 –84.108 46 0.597 35 –8.591 44×10–4 7.315 868.336 33 –26.095 54 1.312 99 –0.006 91 3603.769 33 –55.517 72 0.537 68 –9.226 82×10–4 6.326 1195.581 88 –61.546 11 4.200 98 –0.044 56 –1936.853 58 54.373 03 –0.072 41 2.025 16×10–4 5.742 1342.781 34 –71.452 26 6.891 94 –0.107 30 –519.446 31 45.078 97 –0.040 80 1.391 05×10–4 4.508 –150.530 72 52.113 33 –0.158 49 5.409 68×10–4 表 3 高孔隙度Cu的D-u数据处理参数
Table 3. The fitted parameters to the D-u experimental data of porous Cu with different porosities
ρ00/(g·cm–3) m c0/(km·s–1) λ λ′ 3.5720 2.5 0.04 1.645 0.01 2.9767 3 0.041 1.456 8 0.026 5 2.073 32 1.182 42 10–9 2.5514 3.5 0.068 1.344 8 0.035 1 2.2325 4 0.112 1.3 0.023 2.966 17 0.866 16 0.015 61 1.6388 5.45 0.225 1.141 31 0.038 64 1.2403 7.2 0.346 83 0.993 52 0.052 32 -
[1] 刘培生. 多孔材料引论[M]. 北京: 清华大学出版社, 2004. [2] GIBSON L J, ASHBY M F. Cellular solids: structure and properties [M]. Cambridge: Cambridge University Press, 1999. [3] 赵信峰, 方炎. 金属铜纳米孔洞阵列膜制备方法研究 [J]. 物理学报, 2016, 55(7): 3785–3789.ZHAO X F, FANG Y. Metal nano-hole array copied from AAO template [J]. Acta PhysicaSinica, 2016, 55(7): 3785–3789. [4] 张华伟, 李言祥. 金属熔体中气泡形核的理论分析 [J]. 物理学报, 2007, 56(8): 4864–4871. doi: 10.3321/j.issn:1000-3290.2007.08.082ZHANG H W, LI Y X. Study on bubble nucleation in liquid metal [J]. Acta PhysicaSinica, 2007, 56(8): 4864–4871. doi: 10.3321/j.issn:1000-3290.2007.08.082 [5] ROSA M E. An introduction to solid foams [J]. Philosophical Magazine Letters, 2008, 88(9-10): 637–645. doi: 10.1080/09500830802302014 [6] 谭华. 实验冲击波物理导引[M]. 北京: 国防工业出版社, 2007: 56. [7] 经福谦. 实验物态方程导引[M]. 北京: 科学出版社, 1986: 134. [8] 李西军, 龚自正, 刘福生. 铁高压熔化线的测量——熔化机理的影响 [J]. 高压物理学报, 2001, 15(3): 221–225. doi: 10.3969/j.issn.1000-5773.2001.03.009LI X J, GONG Z Z, LIU F S. A problem in measurements of high pressure melting curve of iron: influenceof melting mechanism on the melting temperature [J]. Chinese Journal of High Pressure Physics, 2001, 15(3): 221–225. doi: 10.3969/j.issn.1000-5773.2001.03.009 [9] LI X J, GONG Z Z, JING F Q, et al. Sound velocities in porous iron shocked to 177 GPa and the implications for shock melting [J]. Chinese Physics Letters, 2001, 18(12): 1632. doi: 10.1088/0256-307X/18/12/327 [10] WU Q, JING F Q. Thermodynamic equation of state and application to Hugoniot predictions for porous materials [J]. Journal of Applied Physics, 1996, 80(8): 4343. doi: 10.1063/1.363391 [11] BOSHOFF M L, VILJOEN H J. Comparative study of analytical methods for Hugoniot curves of porous materials [J]. Journal of Applied Physics, 1999, 86(3): 1245. doi: 10.1063/1.370878 [12] GENG H Y, WU Q, TAN H, et al. Extension of the Wu–Jing equation of state for highly porous materials: Calculations to validate and compare the thermoelectron model [J]. Journal of Applied Physics, 2002, 92(10): 5917–5923. doi: 10.1063/1.1516618 [13] TRUNIN R F, SIRNAKOV G V, SUTULOV Y N, et al. Compressibility of porous metals in shock waves [J]. Soviet Physics–JETP, 1989, 69(3): 580–588. [14] TRUNIN R F, MEDVEDEV A B, FUNTIKOV A I, et al. Shock compression of porous iron, copper, and tungsten, and their equation of state in the terapascal pressure range [J]. Soviet Physics–JETP, 1989, 68(2): 356–361. [15] 耿华运, 吴强, 谭华. 热力学物态方程参数的统计力学表示 [J]. 物理学报, 2001, 50(7): 1334. doi: 10.3321/j.issn:1000-3290.2001.07.027 [16] 耿华运, 谭华, 吴强. 比容可分性及其在自由电子气体模型中的应用 [J]. 高压物理学报, 2001, 15(3): 199–204. doi: 10.3969/j.issn.1000-5773.2001.03.006GENG H Y, TAN H, WU Q. Aeparability of specific wolume and its application to free-GAS model of thermoelectrons [J]. Chinese Journal of High Pressure Physics, 2001, 15(3): 199–204. doi: 10.3969/j.issn.1000-5773.2001.03.006 [17] GENG H Y, WU Q, TAN H, et al. Bulk sound velocity of porous materials at high pressures [J]. Chinese Physics, 2002, 11(11): 1188. doi: 10.1088/1009-1963/11/11/317 [18] 陈俊祥, 于继东, 耿华运, 等. 多孔材料的温度和压强计算 [J]. 物理学报, 2017, 66(5): 056401. doi: 10.7498/aps.66.056401CHEN J X, YU J D, GENG H Y, et al. Temperature and pressure calculation of porous materials [J]. Acta Physica Sinica, 2017, 66(5): 056401. doi: 10.7498/aps.66.056401 [19] CAO X X, CHEN J X, YU Y, et al. An equation of state for abnormal expansion of shocked porous materials [J]. Journal of Applied Physics, 2018, 124(21): 215903. doi: 10.1063/1.5047233 [20] 陈俊祥. 《实验物态方程导引》附录(内部资料)[Z]. 2019. [21] 于渌, 郝柏琳, 陈晓松. 相变和临界现象[M]. 北京: 科学出版社, 2005: 27. [22] 汤文辉, 张若棋. 物态方程理论及计算概论 [M]. 北京: 高等教育出版社, 1999. -







 下载:
下载:
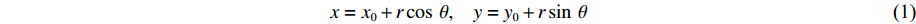
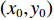
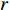
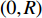
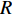
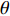
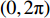

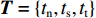
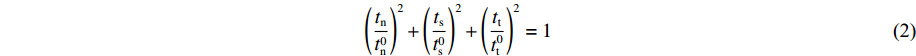
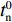
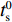
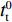
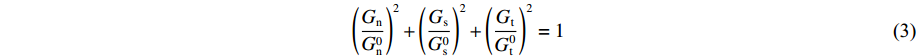
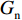
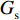
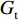
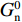
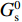
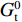
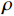

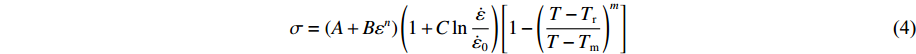
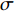
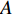
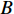

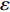
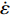
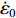

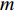
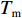












 下载:
下载: