Fragmentation Process of Quartz Glass Spheres Impacting Rigid Wall
-
摘要: 利用高速枪对石英玻璃球撞击刚性靶板进行了实验研究,分析了不同速度下球体的破碎过程和失效模式。当冲击速度低于临界破坏速度时,石英玻璃球以略低于原速从靶板回弹;当超过临界破坏速度时,球体呈现“压缩破碎区-表面剥落区-剪切破坏区”的破坏结构;进一步提高碰撞速度,剪切破坏区的扩展导致球体碎裂为若干“月牙状”的碎块;更高撞击速度下,石英玻璃球发生坍塌式破碎,在远离撞击端处产生层裂现象。利用离散元软件对球体的撞击破坏过程进行了模拟研究,球体在高速碰撞下的破碎可以分为弹性压缩、整体破碎和二次撞击3个阶段。球体碎裂前Hertz接触理论可以较好描述其撞击力,而破碎后的撞击力由于碎裂卸载远小于理论值,且偏差随冲击速度逐渐增加。Abstract: The high-speed gun is used to study the impact of quartz glass ball on rigid target plate. The crushing process and failure mode of the ball at different speeds are analyzed. When the impact velocity is lower than the critical failure velocity, the quartz glass ball rebounds from the target plate, and the rebounding speed is slightly below the original speed; when the critical speed is exceeded, the sphere exhibits a “compressed fracture zone–surface spalling zone–shear failure zone” failure structure; further increasing the collision velocity, the expansion of the shear failure zone causes the sphere to be fragmented into several “crescent” fragments. At higher impact speeds, the quartz glass ball collapses and spalls at a distance away from the impact end. Furthermore, the discrete element software is utilized to simulate the impact damage process of the sphere. The crushing of the sphere under high-speed collision can be divided into three stages: elastic compression, integral crushing, secondary impact. Before the ball breaks, the Hertz contact theory can describe its impact force well, but the crushing force is much smaller than the theoretical value due to the fracture unloading, and the deviation gradually increases with the increasing impact speed.
-
Key words:
- quartz glass /
- fragmentation /
- spallation /
- discrete element method
-
球体的冲击破碎是颗粒物质力学的基本问题。球体结构材料广泛存在于日常生活和工程应用中,例如煤粒破碎[1-2]、落石灾害[3]、化工产品[4]等。研究球体材料在冲击载荷下的破碎,对于提高颗粒物质的加工技术、节约能源、促进经济发展、有效监控及预防自然灾害等具有重要价值。
早期国内外研究人员对单颗粒的冲击破碎开展了大量的实验研究,研究的重点是颗粒的临界破碎速度、颗粒的破碎模式、碎片形貌以及碎块的统计特征等。Andrews等[5-6]对钠钙玻璃球和陶瓷球进行了研究,在临界速度下,球体从刚性靶板回弹;超过临界速度后,钠钙玻璃球碎裂为大量尺寸不均的细小碎片,而陶瓷球则碎裂为若干大小均匀形似“月牙状”的碎块,并将钠钙玻璃球体破碎归因于球体压缩端的剪切应力,而将陶瓷球体碎裂归因于球体中部的冲击拉伸应力。Salman等[7-9]对不同类型材料和大小的脆性球体进行试验,结果表明大部分脆性材料的破坏模式是相似的,即:在中低速下,球体的局部裂纹扩展导致局部破碎,在撞击端形成压缩平台;在高速下,球体碎裂为大量难以识别的粉状碎片。Chau[10]、Wu[11]等根据冲击动能的转换分析了脆性球体破碎后碎块的统计特征。
在数值计算方面,易洪昇等[12]使用ABAQUS软件模拟了冲击过程中的球体内部应力情况,由于颗粒破碎涉及大变形,采用有限元方法会出现网格畸变问题,因此不适合模拟大变形和损伤现象。离散元方法(Discrete element method, DEM)能够满足岩体工程中破裂和裂纹发展等问题的研究,适合于从本质上研究固体介质的力学特性[13]。Shen等[14]利用离散元软件分析了球形岩石在不同应变率情况下碎块特征、碎块数量、损伤率等的变化情况。Carmona等[15]采用离散元方法研究了球体碎裂过程中裂纹的扩展过程,发现碎裂的大块碎片质量符合两参数的Weibull分布。
以石英玻璃球作为典型的脆性球体,开展了较广泛冲击速度下石英玻璃球撞击刚性壁的破坏现象研究,利用离散元数值软件PFC3D分析了石英玻璃球在不同撞击速度下的撞击碎裂过程。
1. 实验装置及试件
实验采用高速气枪作为石英玻璃球的加载发射装置,撞击区域使用碳化钨材料作为近似刚性靶板,碳化钨的弹性模量为720 GPa,密度为15.6 g/cm3,泊松比为0.21。碳化钨周围附有厚壁钢块,如图1所示。采用Kirana超高速摄像机记录球体的破碎过程,拍摄幅频为2×105帧每秒,高速相机采用断路方式触发,触发线设置在气枪的出口位置。在球体撞击区域的外围设计了一个有机玻璃防护装置用于收集碎片。
高速气枪出口距离靶板250 mm,通过圆柱形长杆调节高速气枪和靶板的垂直度,从而实现玻璃球的正撞破坏。通过水平放置和位于球体上方放置的高速相机,实验观察石英玻璃球的飞行姿态,结果表明玻璃球体未发生明显偏转,撞击靶板位置为初始预设位置。根据超高速相机拍摄可获得玻璃球体的飞行时间,从而确定玻璃球体飞行的平均速度,近似等效为球体撞击速度。
实验试件采用昊天玻璃制品有限公司生产的超纯石英玻璃球,其SiO2的质量分数大于99.99%。球体直径为(10.00±0.02)mm,质量为(1.150±0.015)g,具有良好的透光性,且表面无明显缺陷。
2. 碎裂过程及结果分析
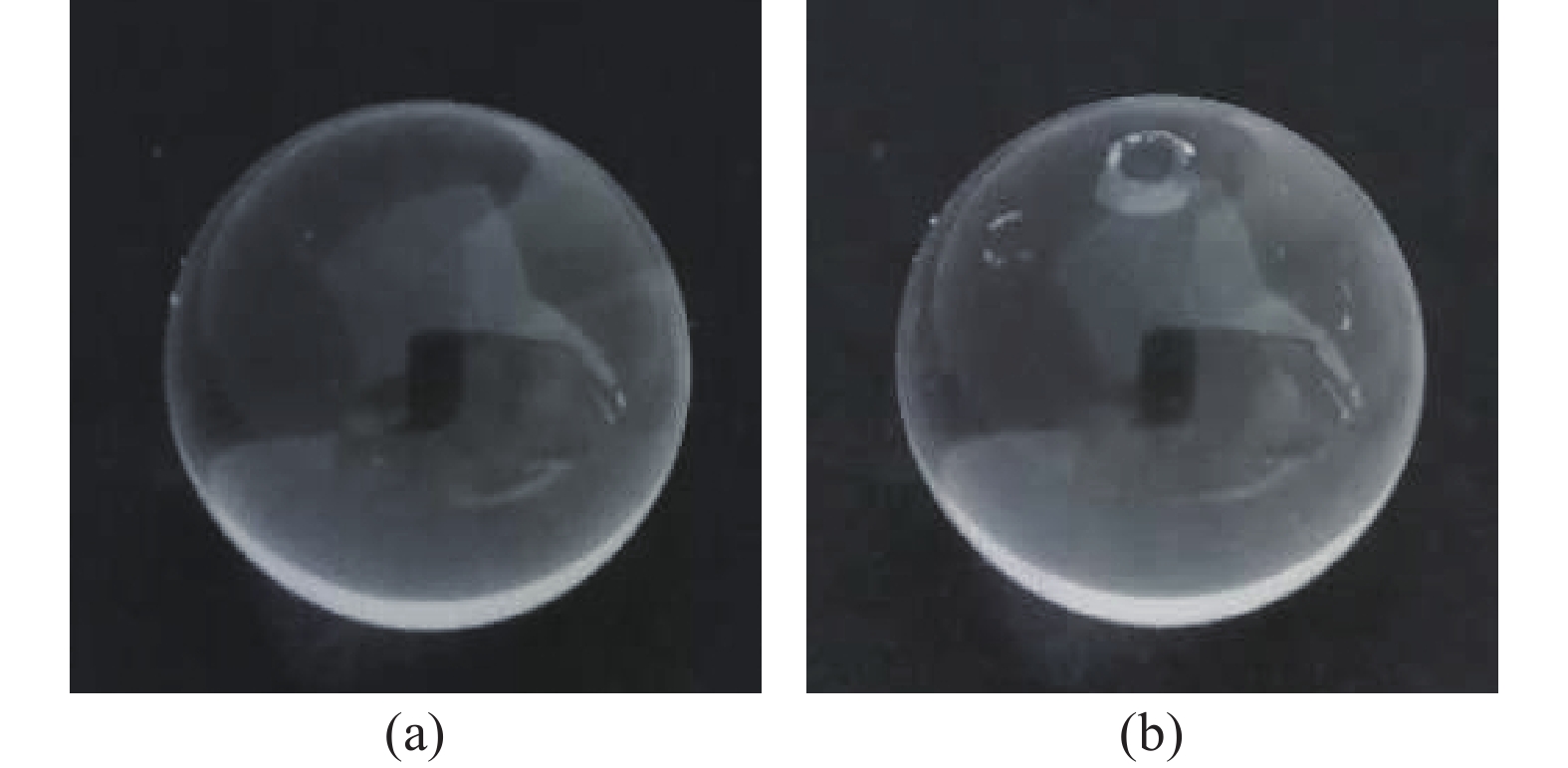
为了研究玻璃球的临界冲击破碎速度,在低速下开展了大量的实验。实验结果表明:当石英玻璃球以11.87 m/s撞击刚性靶板时,碰撞为近似弹性碰撞,球体以略低于原速(回弹速度10.25 m/s)从刚性壁回弹,除却撞击点应力集中破坏外,球体内部没有产生宏观可见裂纹。石英玻璃球撞击前和回弹后如图2所示。
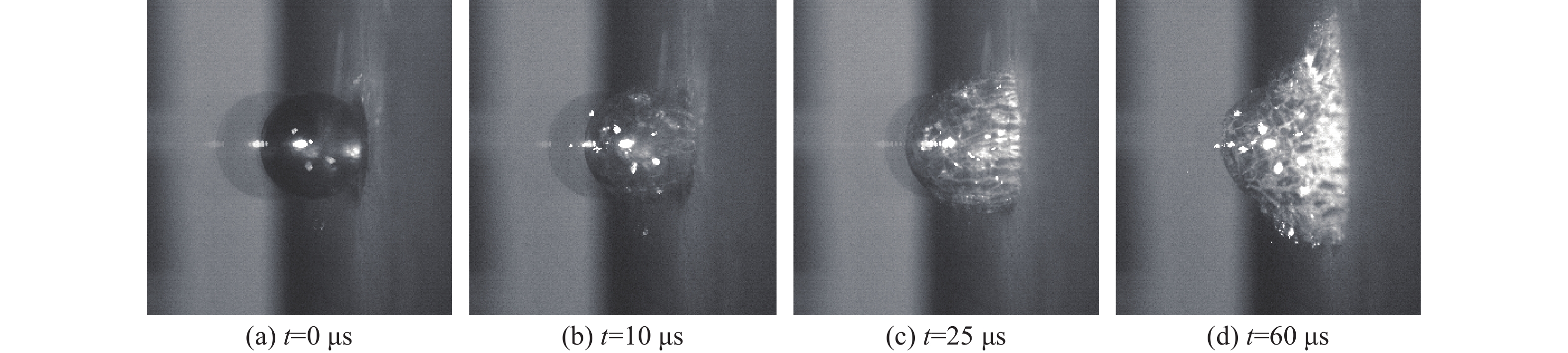
图3是冲击速度为78 m/s的冲击破碎过程。从图3可以看出,球体的破碎可以分为3个阶段。在初始阶段,撞击端首先发生破坏,形成经过子午面的宏观裂纹,同时形成细条状碎片,这些碎片以撞击点为中心向四周扩散,而在远离撞击端部分还保持相对完整;随着撞击的持续进行,在撞击端的碎片继续向四周运动,在远离撞击端的部分产生“龟甲状”的裂纹,并最终碎裂为若干“圆锥状”的碎块。
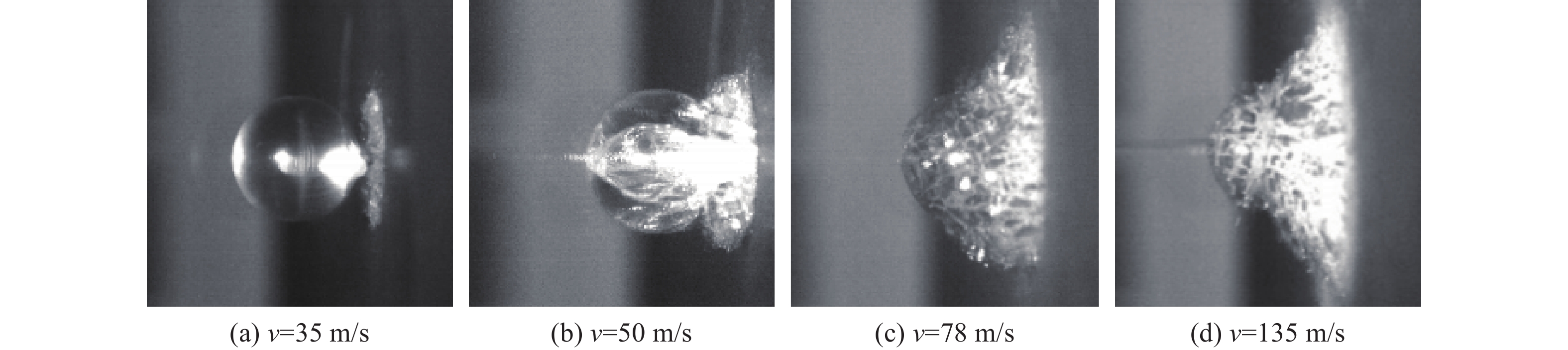
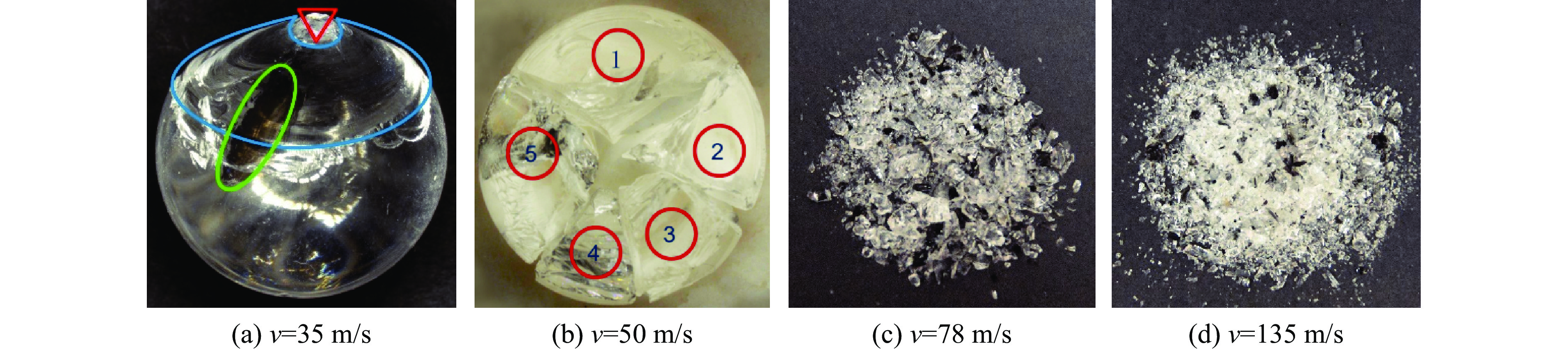
当冲击速度为35 m/s时,球体从刚性壁回弹,在撞击端产生了一些细小的碎片,如图4(a)所示。图5(a)表明,实验回收后的碎片存在3个破坏区域,分别为压缩破碎区、表面剥落区以及剪切破坏区。其中压缩破碎区是一个圆锥状的区域,这块区域的石英玻璃已不具备透光性,说明这一区域的石英玻璃发生了剧烈的压缩破碎,但是这些碎块还有部分镶嵌在球体上并没有从球体上脱落。在压缩破碎区的周围是一个环形的表面剥落区,受到表面波作用一些片状的碎片从球体上剥落,产生层片状的碎片。在球体内部,石英玻璃的折光性发生了改变,说明在剪切力的作用下石英玻璃内部产生剪切裂纹带,剪切裂纹带由压缩破碎区开始起裂,沿着子午面由撞击端向试件内部扩展。
当冲击速度为50 m/s时,从球体的透光性可以看出,球体完全碎裂为若干碎块,如图4(b)所示。在撞击端同样会出现类似于35 m/s时的压缩破碎现象,并且涉及的范围更大。由于冲击动能的增加,剪切破碎区完全贯穿了球体的子午面,最终导致石英玻璃球碎裂成若干类似于“月牙状”的碎块,如图5(b)所示。
当冲击速度为78 m/s时,与上述几种破碎情况完全不同,石英玻璃球不再从刚性壁回弹,而是发生了“坍塌式”的破碎,如图4(c)所示。在撞击端,产生大量细小的碎块,在球体的另一端表面有许多类似于“经线形”和“纬线形”的宏观裂纹,最终产生大量类似于“龟甲状”的碎片,内部也产生大量细小碎片,如图5(c)所示。
当冲击速度为135 m/s时,发生“蘑菇云”似的破碎现象,如图4(d)所示。产生的碎片更加细小,在远离撞击端的地方发生明显的“层裂”现象。球体在高速撞击过程中碎裂为极细小的碎片,透明球体不易观察裂纹发展,将石英玻璃球表面涂黑,有利于观测球体表面裂纹扩展。由图5(d)可知,随着速度的增加,球体内部破碎更为剧烈,产生大量粉末状细小碎片。
综上所述,动态冲击下石英玻璃球的破坏形式主要有4种特征:(1)局部破碎,在球体上形成3个破碎区域;(2)中心劈裂,沿着冲击轴线碎裂为类似于“月牙状”的碎片;(3)坍塌破坏,石英玻璃球整体破碎,碎裂为若干碎块;(4)层裂破坏,在远离撞击端的地方产生“蘑菇云”似的“层裂”破坏。
3. 离散元数值计算
3.1 离散元模型的建立
选择目前离散元中使用广泛的平行黏结模型(Linear parallel bond mode)。平行黏结模型本质上是用一层线性的黏结体将相互重叠的颗粒绑定起来,这些黏结的颗粒能很好地模拟岩石、玻璃等脆性材料的压缩破坏过程[16]。
为了使模型具有较高的密实度,将模型中的孔隙率设定为0.2,最大颗粒与最小颗粒的粒径比为3∶2。颗粒间的摩擦系数对材料的宏观参数影响不大[17],故取经验值0.577。
根据文献[18-19]的标定方法,通过单轴压缩标定了模型的弹性模量、泊松比和抗压强度,通过单轴拉伸标定抗拉强度,由三点弯标定弯曲强度,带缺口的三点弯标定断裂韧性和颗粒半径。最终得到的主要微观参数如表1所示。标定的宏观参数如表2所示,得到石英玻璃的宏观参数与制造商提供的材料参数基本一致,因此可以用于模拟石英玻璃的冲击破碎。
表 1 石英玻璃离散元模型的主要微观参数Table 1. Main microscopic parameters of discrete element mode of quartz glassEffective modulus of linear contact/GPa Normal to shear stiffness ratio of linear contact Porosity Minimum radius of particles/mm Size ratio of maximum and minimum particles Tensile strength of contact/MPa Shear strength of contact/MPa 55 2.9 0.2 0.1 1.5 300 600 表 2 石英玻璃在常态下的物理参数Table 2. Physical properties of quartz glass under ambient conditionMethod Equivalent density/(kg·m–3) Elastic modulus/GPa Poisson’s ratio Compressive strength/MPa Tensile strength/MPa Bending strength/MPa Fracture toughness/(N·m–3/2) Manufacture provide 2.203 77.8 0.170 860 50 67.0 0.78 DEM numerical simulation 2.203 78.0 0.172 798 50 67.4 0.85 根据上述标定的离散元微观参数建立与实验同尺寸的几何模型。采用刚性墙面模拟实验中的刚性壁,在模拟初始时刻,给所有颗粒施加垂直撞击刚性壁的速度,使其匀速撞击刚性壁。
3.2 石英玻璃球冲击刚性壁破碎过程
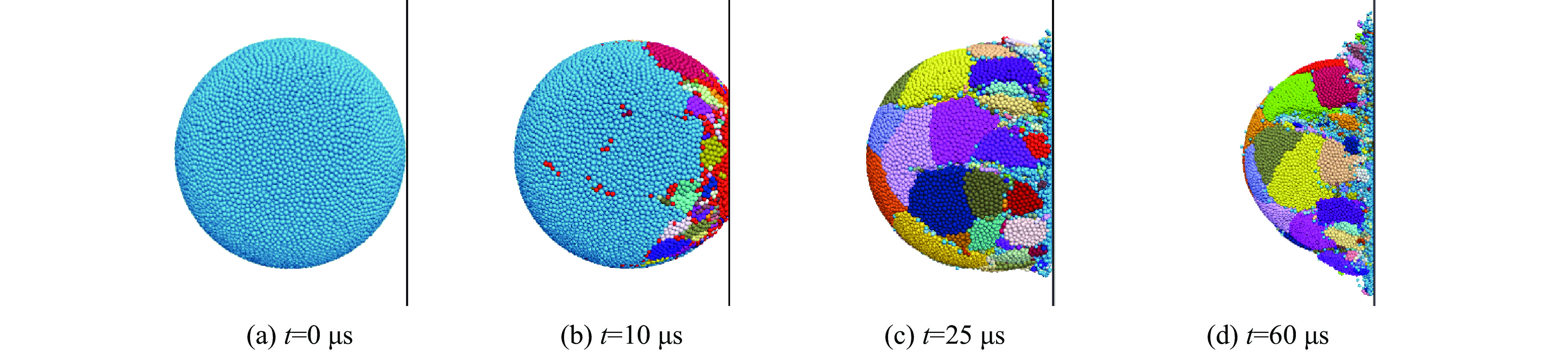
图6是球体以78 m/s撞击刚性壁的过程。模拟中不同颜色代表的是碰撞过程中产生的不同碎块。对比图3和图6可知,模拟与实验吻合较好,模拟中玻璃球的破坏过程存在明显的时序性。在初始时刻,在撞击端发生局部的破碎,产生细小的碎片,同时裂纹向远离撞击端的地方传播;随着撞击的进行,撞击端产生的碎片向外运动,远离撞击端的地方碎裂为较大的锥形碎块,当撞击端处的细小碎片运动到更远处时,这些较大的锥形碎块以一定的速度继续撞击刚性壁。
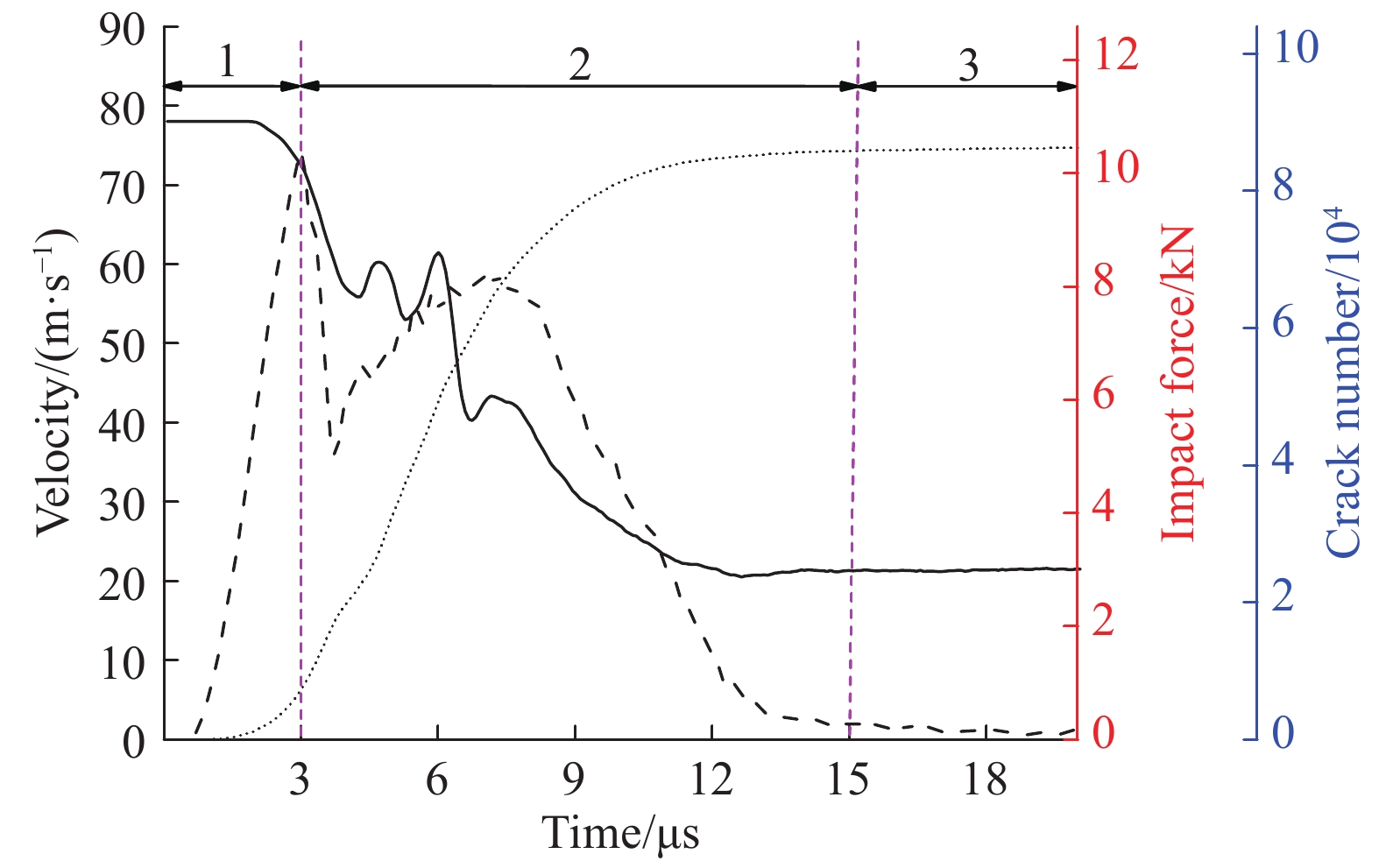
球体以78 m/s撞击刚性壁的过程中,球体速度(左端粒子平均速度)、球体内部裂纹数量(粒子之间的力键)以及球体撞击力的时程曲线如图7所示。石英玻璃球撞击破碎过程大致可分为3个阶段:第1阶段,可近似为弹性压缩过程,球体所受到的冲击载荷也近似线性增加,大约经历
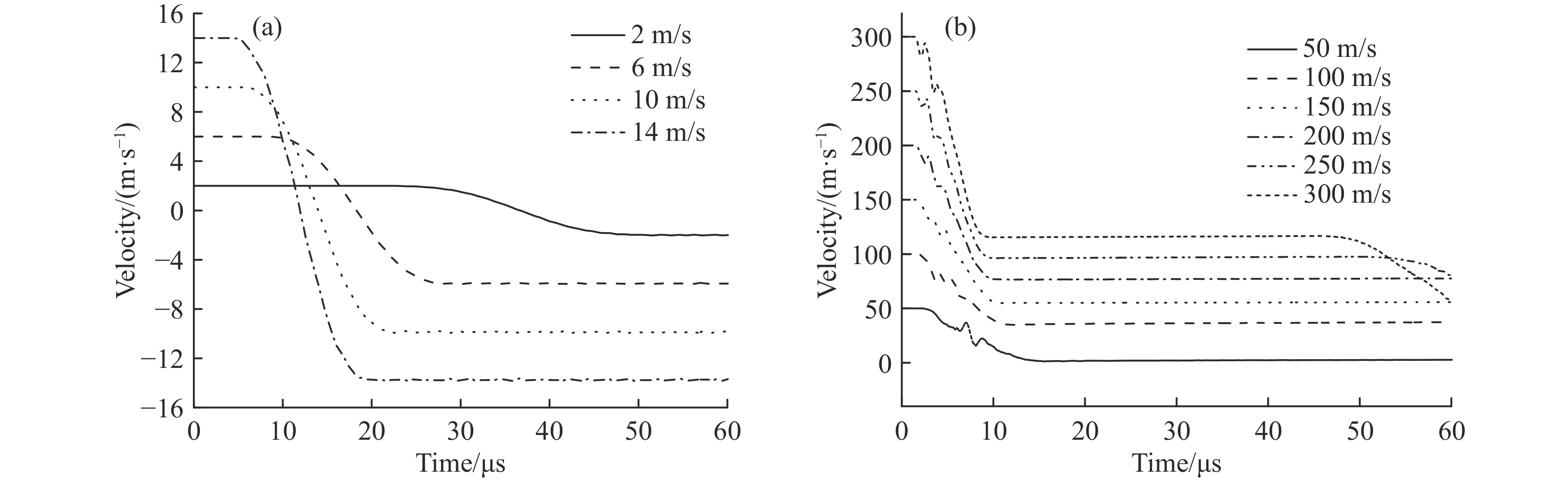
3μs 后撞击力达到最大值,在弹性波还未传播至左端时,球体速度保持不变,之后速度下降,在球体撞击端裂纹发展并产生少量碎片,球体整体裂纹发展较缓慢;第2阶段,为球体破碎阶段,撞击力在碎裂过程中发生明显振荡,同时球体速度也存在显著振荡,这是由于该阶段大量密集裂纹的生成,使弹性卸载波在球体中传播并来回反射,最终球体撞击端(右端粒子)几乎完全破坏,球体裂纹数整体稳定,撞击力也卸载为零附近;第3阶段,为球体二次撞击阶段,远离撞击端(左端粒子)在碎裂过程中还保留残余速度,在球体整体破碎后仍以较低的速度再次撞击刚性壁,但对石英玻璃球整体的撞击力和裂纹增长影响甚微。图8为在不同速度下石英玻璃球速度随时间变化的曲线。图8(a)显示了撞击速度低于临界破碎速度时球体速度的变化过程。可以看出,球体撞击靶板后匀速下降,最终几乎以原速从靶板回弹。图8(b)显示了撞击速度高于临界破碎速度时球体速度的变化过程。可以看出,球体左端粒子在撞击刚性壁后平均速度急剧下降并保留部分残余速度,撞击速度越大,速度下降越快,残余速度也越大。球体左端粒子以残余速度自由飞行,石英玻璃球撞击端(右端粒子)完全破碎,左端粒子在向右运动过程中没有任何阻力,将球体匀速运动部分对时间积分,发现球体的运动距离均约为球体直径的1/2。
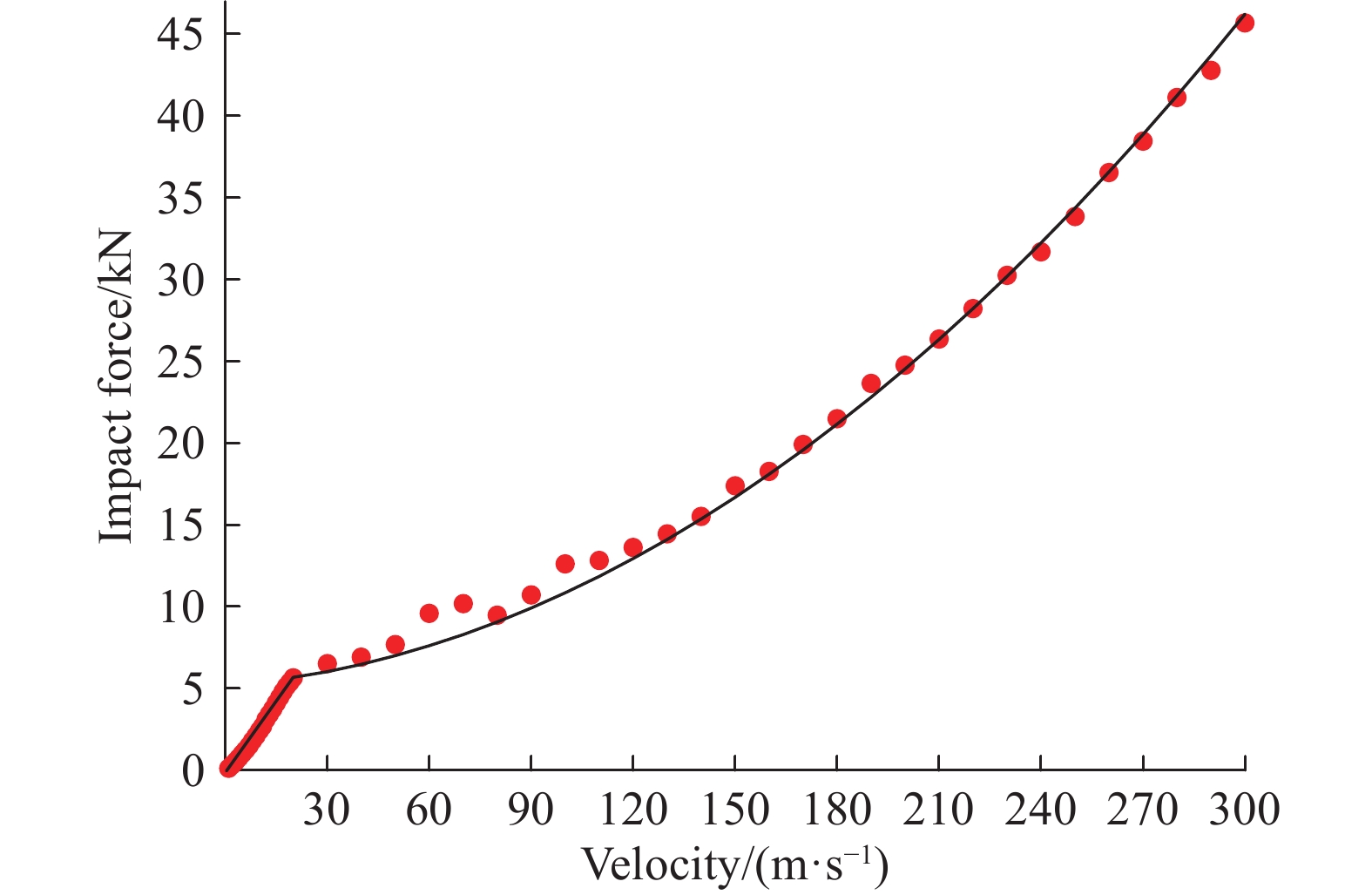
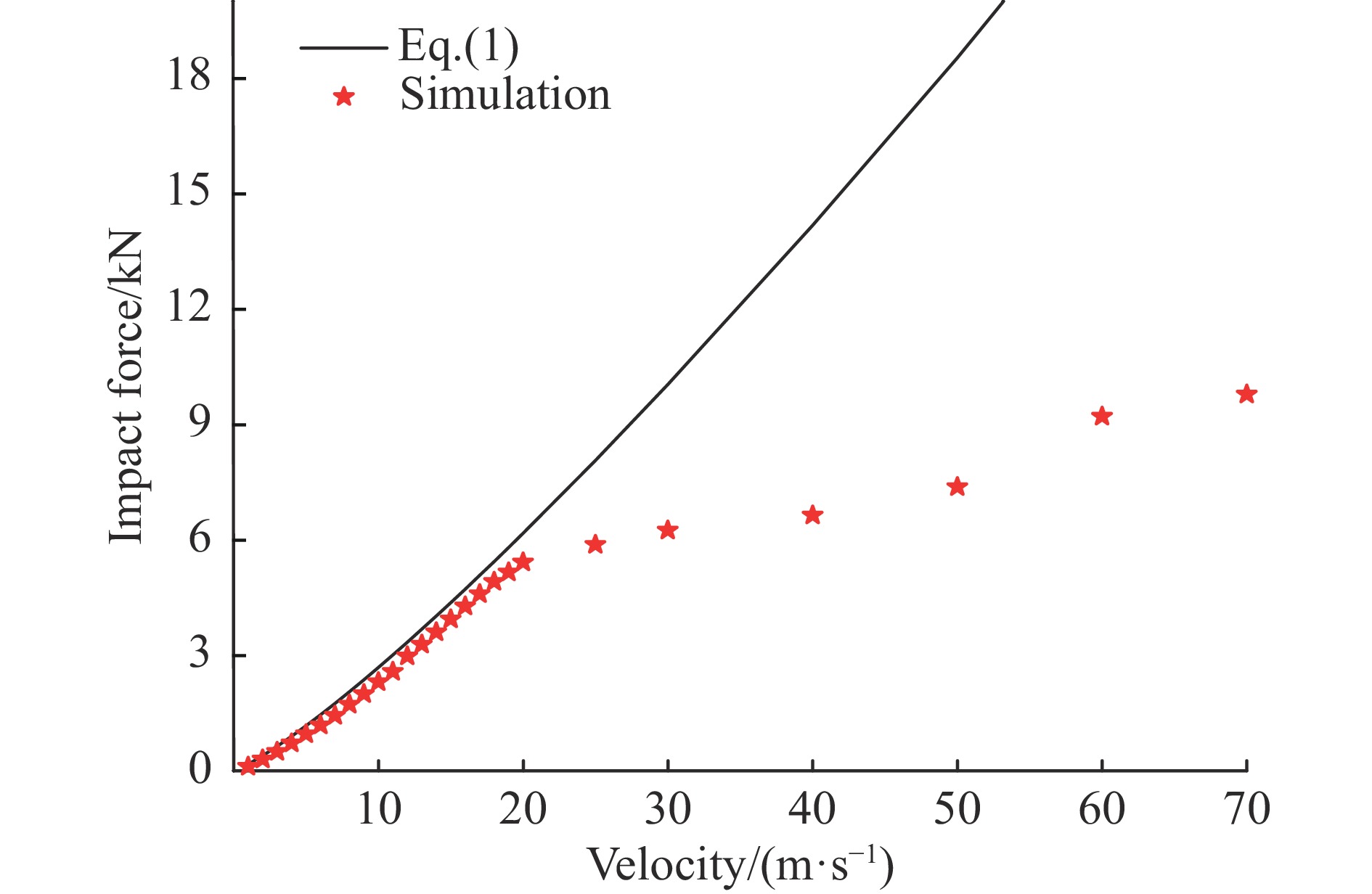
图9给出了0~300 m/s撞击速度下石英玻璃球撞击力随撞击速度的变化规律。从图9可以看出,撞击速度为20 m/s左右时为撞击力增长规律的临界转折点,此前球体未产生明显破碎,此后球体破碎使得撞击力上涨趋势大幅衰减。
在临界转折速度前,石英玻璃球撞击刚性靶板过程可近似认为是弹性碰撞,通过Hertz接触理论可得弹性碰撞接触力[20]
Pm=(53πρ)35(34KI)−25V65R2 (1) 式中:
KI=1−μ12E1+1−μ22E2 ,其中E1、μ1 和E2、μ2 分别为球体材料和刚性靶板的弹性模量和泊松比;ρ 为球体材料的密度;R为球体的半径。图10给出了临界转折速度附近石英玻璃球撞击力随撞击速度的变化规律。从图10可以看出:当速度低于临界转折速度时,球体不破碎,内部几乎不产生裂纹,球体整体近似弹性碰撞,Hertz接触理论可以较好地预测石英玻璃球的撞击力;而速度高于临界转折速度时,球体发生破碎,不再具有球体整体性,破碎过程将卸载撞击力,并消耗部分能量,飞散的碎片也将带走大量动能;随着冲击速度的增加,石英玻璃球的极限撞击将等效于流体撞击。
4. 结 论
冲击速度在临界破坏速度下为弹性碰撞,球体以略低于原速从刚性壁回弹,除却撞击点应力集中破坏外,球体内部没有产生宏观可见裂纹。低速碰撞时,球体呈现“压缩破碎区-表面剥落区-剪切破坏区”的破坏结构,剪切裂纹沿着子午面由撞击端向试件内部扩展,并还不足以形成碎片;中速碰撞时,由于剪切破坏区充分扩展,致使球体碎裂为若干“月牙状”的碎块;高速碰撞时,石英玻璃球发生坍塌式破碎,表面产生大量类似于“龟甲状”的碎片,内部也产生大量细小碎片;当速度更高时,在远离撞击端有明显的层裂现象产生,球体破碎产生大量粉末状碎片。
离散元软件PFC3D再现了石英玻璃球撞击刚性壁的过程,球体在高速碰撞下破碎可以分为弹性压缩、整体破碎和二次撞击3个阶段。石英玻璃球撞击力随撞击速度呈两段式增长,在球体碎裂前,撞击过程近似为弹性碰撞过程,Hertz接触理论可以较好地描述其撞击力,在球体碎裂后,破碎过程将卸载撞击力,并消耗部分能量,飞散的碎片也将带走大量动能,撞击力远低于Hertz接触理论值,并且随着撞击速度增大,偏差越大。
-
表 1 石英玻璃离散元模型的主要微观参数
Table 1. Main microscopic parameters of discrete element mode of quartz glass
Effective modulus of linear contact/GPa Normal to shear stiffness ratio of linear contact Porosity Minimum radius of particles/mm Size ratio of maximum and minimum particles Tensile strength of contact/MPa Shear strength of contact/MPa 55 2.9 0.2 0.1 1.5 300 600 表 2 石英玻璃在常态下的物理参数
Table 2. Physical properties of quartz glass under ambient condition
Method Equivalent density/(kg·m–3) Elastic modulus/GPa Poisson’s ratio Compressive strength/MPa Tensile strength/MPa Bending strength/MPa Fracture toughness/(N·m–3/2) Manufacture provide 2.203 77.8 0.170 860 50 67.0 0.78 DEM numerical simulation 2.203 78.0 0.172 798 50 67.4 0.85 -
[1] 刘瑜, 杜长龙, 付林, 等. 煤块冲击破碎速度研究 [J]. 振动与冲击, 2011, 30(3): 18–21. doi: 10.3969/j.issn.1000-3835.2011.03.005LIU Y, DU C L, FU L, et al. Impact crushing velocity of lump coal [J]. Journal of Vibration and Shock, 2011, 30(3): 18–21. doi: 10.3969/j.issn.1000-3835.2011.03.005 [2] 李艳焕, 邵良杉, 徐振亮. 煤粒冲击粉碎临界速度的数值实验分析 [J]. 振动与冲击, 2017, 36(5): 227–230.LI Y H, SHAO L B, XU Z L. Numerical analysis for critical velocity of coal impacting and comminution [J]. Journal of Vibration and Shock, 2017, 36(5): 227–230. [3] 沈位刚, 赵涛, 唐川, 等. 落石冲击破碎特征的加载率相关性研究 [J]. 工程科学与技术, 2018(1): 43–50.SHEN W G, ZHAO T, TANG C, et al. Loading rate dependency of impact induced rock fragmentation during rockfall [J]. Advanced Engineering Sciences, 2018(1): 43–50. [4] 房丽娜, 马正先, 李慧, 等. 粉碎设备及技术的发展历程与研究进展 [J]. 有色矿冶, 2005(Suppl 1): 178–180.FANG L N, MA Z X, LI H, et al. Development history and research progress of crushing equipment and technology [J]. Non-Ferrous Mining and Metallurgy, 2005(Suppl 1): 178–180. [5] ANDREWS E W, KIM K S. Threshold conditions for dynamic fragmentation of glass particles [J]. Mechanics of Materials, 1999, 31(11): 689–703. doi: 10.1016/S0167-6636(99)00024-1 [6] ANDREWS E W, KIM K S. Threshold conditions for dynamic fragmentation of ceramic particles [J]. Mechanics of Materials, 1998, 29(11): 161–180. [7] SALMAN A D, GORHAM D A. The fracture of glass spheres [J]. Powder Technology, 2000, 107(1): 179–185. [8] SALMAN A D, GORHAM D A, VERBA A. A study of solid particle failure under normal and oblique impact [J]. Wear, 1995, 186(95): 92–98. [9] SALMAN A D, REYNOLDS G K, FU J S, et al. Descriptive classification of the impact failure modes of spherical particles [J]. Powder Technology, 2004, 143(26): 19–30. [10] CHAU K T, WEI X X, WONG R H C, et al. Fragmentation of brittle spheres under static and dynamic compressions: experiments and analyses [J]. Mechanics of Materials, 2000, 32(9): 543–554. doi: 10.1016/S0167-6636(00)00026-0 [11] WU S Z, CHAU K T, YU T X. Crushing and fragmentation of brittle spheres under double impact test [J]. Powder Technology, 2004, 143/144: 41–55. doi: 10.1016/j.powtec.2004.04.028 [12] 易洪昇, 徐松林, 单俊芳, 等. 不同加载速度下脆性颗粒的破坏特性 [J]. 爆炸与冲击, 2017, 37(5): 913–922. doi: 10.11883/1001-1455(2017)05-0913-10YI H S, XU S L, SHAN J F, et al. Fracture characteristics of brittle particles at different loading velocities [J]. Explosion and Shock Waves, 2017, 37(5): 913–922. doi: 10.11883/1001-1455(2017)05-0913-10 [13] POTYONDY D O. A bonded-particle model for rock [J]. International Journal of Rock Mechanics & Mining Sciences, 2004, 41(8): 1329–1364. [14] SHEN W G, ZHAO T, CROSTA G B, et al. Analysis of impact-induced rock fragmentation using a discrete element approach [J]. International Journal of Rock Mechanics & Mining Sciences, 2017, 98: 33–38. [15] CARMONA H A, WITTEL F K, KUN F, et al. Fragmentation processes in impact of spheres [J]. Physical Review E, 2008, 77(5): 051302. [16] XIA M, ZHAO C B. Simulation of rock deformation and mechanical characteristics using clump parallel-bond models [J]. Journal of Central South University, 2014, 21(7): 2885–2893. doi: 10.1007/s11771-014-2254-3 [17] YANG B, JIAO Y, LEI S. A study on the effects of microparameters on macroproperties for specimens created by bonded particles [J]. Engineering Computations, 2006, 23(6): 607–631. doi: 10.1108/02644400610680333 [18] PARK J W, SONG J J. Numerical simulation of a direct shear test on a rock joint using a bonded-particle model [J]. International Journal of Rock Mechanics & Mining Sciences, 2009, 46(8): 1315–1328. [19] 熊迅, 李天密, 马棋棋, 等. 石英玻璃圆环高速膨胀碎裂过程的离散元模拟 [J]. 力学学报, 2018, 50(3): 178–188.XIONG X, LI T M, MA Q Q, et al. Discrete element simulation of the high velocity expansion and fragmentation of quartz glass rings [J]. Chinese Journal Theoretical and Applied Mechanics, 2018, 50(3): 178–188. [20] KNIGHT C G, SWAIN M V, CHAUDHRI M M. Impact of small steel spheres on glass surfaces [J]. Journal of Materials Science, 1977, 12(8): 1573–1586. doi: 10.1007/BF00542808 -








 下载:
下载:





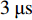



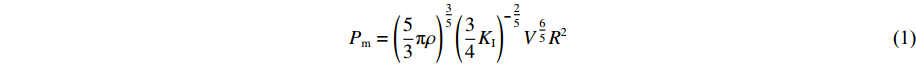
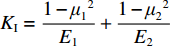
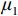
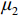
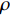


 下载:
下载: