Phase Retrieval and Reconstruction of Coherent Diffraction Imaging
-
摘要: 相干衍射成像是一种对材料体密度敏感的超高分辨成像技术。相较于传统表面敏感的超高分辨成像技术,相干衍射成像利用了硬X射线的强穿透能力,可以深入材料体内部进行成像,且成像分辨能力可以根据成像布局进行调整,最高达到原子级空间分辨能力。这种灵活的空间分辨调整依赖于相干衍射成像独特的相位复原技术,即通过对图像成像强度的过采样,利用含约束的迭代算法同时获得光场的强度及相位,进而对样品进行重建;同时结合图像定向及组合技术,相干衍射成像可以实现对样品的三维重建。本文主要从成像原理、复原算法和重建方法介绍相干衍射成像技术,并结合实验进展及模拟研究展示该技术在多种重建情形下具备的诊断能力,以期较为全面地给出相干衍射成像技术的发展趋势。Abstract: The coherent diffraction imaging (CDI) is an ultra-high resolution imaging technique that is sensitive to the density of the material. Compared to the surface-sensitive imaging methods with ultra-high resolution, the CDI is able to probe the interior of the sample by taking advantages of hard X-rays. According to the imaging layout, the space resolution of CDI is variable and can reach up to an atomic scale. This feature depends on the iterative phase retrieval method that almost becomes the signature of CDI. Based on oversampling a sample in a detected image, the phase and intensity of X-ray beam can be retrieved simultaneously by iterative calculations with constraints, and then are used to reconstruct the sample. Meanwhile, the three-dimensional reconstruction could be realized by combining image orientating and merging techniques. Here we present the imaging theory, phase retrieval and reconstruction methods of the CDI technique, and its diagnostic ability in a variety of reconstruction situations by experimental and simulation examples, to hopefully provide a systematic introduction of its development.
-
在X射线成像领域,公认的成像分辨能力极限与X射线波长同量级。考虑两种最普遍的成像情形:一种是成像点与样品确定点空间位置对应的成像,可归为空域成像,特点是成像图像通常直接反映样品形貌;另一种是成像点与样品空间频率确定处对应的成像,可归为频域成像,直接观察频域图像并不能获得样品的形貌信息。在空域成像中,被探测光子来自于样品点(无论是透射、散射,还是荧光过程),通过直接测量光子位置,可推断样品在该点的空间信息。但根据不确定性原理,光子位置测量的不确定性难以超过光子波长尺度,因此对应的样品点空间定位也不超过光子波长。在频域成像中,以原子级分辨的X射线衍射(X-Ray Diffraction,XRD)技术为例,通过布拉格公式可以明确首个非零级布拉格峰的位置,即
$$ 2d\sin \theta = n\lambda $$ (1) 式中:d为晶格常数,
$\theta $ 为衍射角,n为衍射级次,$\lambda$ 为波长。显然$\sin \theta \leqslant 1$ 的要求决定了当至少存在第一个布拉格峰时,能够获得的晶格常数(即分辨率d)无法小于$\lambda$ /2。尽管X射线成像的潜在分辨能力很高,但是至今为止鲜有能够对一般样品(晶体或非晶体)达到X射线波长级分辨能力的成像技术,尤其在硬X射线波段,主要的限制因素包括光源尺寸、聚焦能力及X射线探测器的像素尺寸等。对于无透镜的空域成像,例如同轴相位衬度成像(PCI)、同轴全息成像等,探测器的像素尺寸在
$10 \;{\text{μ}}{\rm{m}}$ 以上,若放大比较小,则成像空间分辨能力有限,而当提升放大比时(达1000),有限光源尺寸又会被同等放大,导致空间分辨下降;对于采用光学元件进行聚焦成像的传统布局空域成像,例如透射X射线显微(TXM)成像,元件对X射线束的聚焦能力最高可达10 nm水平[1],提升至波长级仍面临极大困难;对于一些频域成像,例如傅里叶变换全息术(FTH),样品分辨受参考光束等效光源尺寸的限制,仅靠图像处理技术难以达到X射线波长水平。与上述成像技术相比,相干X射线衍射成像(Coherent Diffracion Imaging, CDI)技术应用于非晶体样品时更容易达到更高的空间分辨能力。作为一种频域成像技术,CDI不同于FTH依赖一个已知的参考光束进行成像,而是直接利用经过样品并传播足够距离的相干光进行成像,整个成像系统做到了最大限度的简化,样品与探测阵列间不需要任何光学元件,且原则上也不需要在样品前聚焦光束。相对应的,CDI的图像处理也不像FTH那样简单进行一次傅里叶变换即可得到初步的样品实空间像。进行CDI图像处理时,通常需要多步迭代过程以实现所谓的相位复原。从发展过程来看,CDI是一种基于图像处理技术而发展起来的成像技术。
1. CDI的成像原理
目前主流CDI实验单次成像的样品尺寸在几十微米及以下,通过探测相干光与样品相互作用后的弹性散射光进行成像。
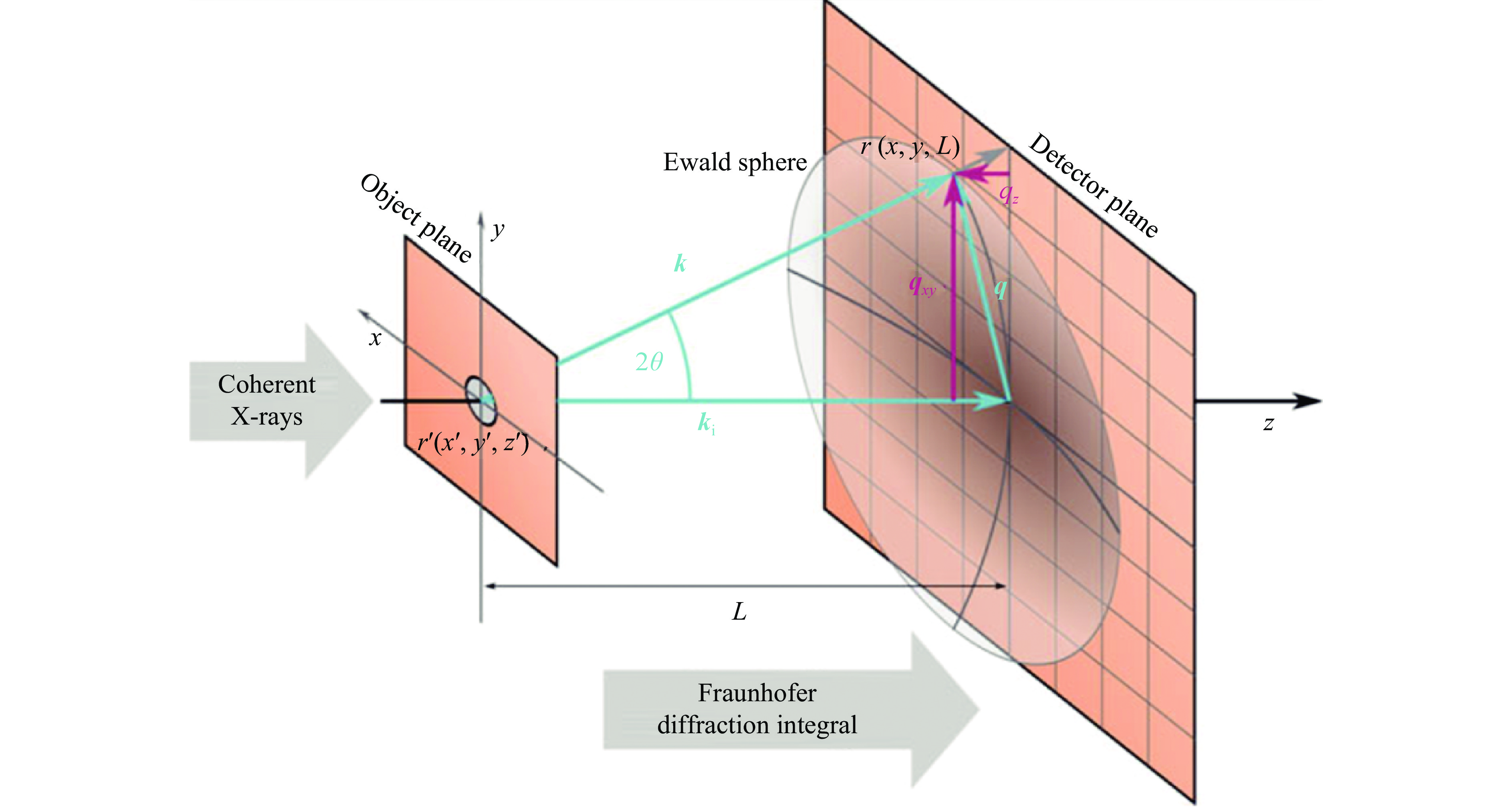
在光散射的描述体系中,基本的散射过程如图1所示,设样品点位置为r'(x', y', z'),探测器平面像素位置为r(x, y, L),光场为
$\varphi $ 。探测器像元上的散射光可描述为[2]$$ {\varphi_{ r}} = \int {{\varphi_0}({ r}'){r_{\rm{e}}}f({ r}')\rho ({ r}')P(2\theta )\exp {({{\rm i}{{k}}z'}})\exp {({{\rm i}{{k}}\left| {{ r}- { r}'} \right|}})} {\rm{d}}{ r}' $$ (2) 式中:re为电子经典半径,表明X射线与物质的散射相互作用十分微弱;f(r')为样品立体元的散射形状因子,当立体元为构成样品的原子时,f(r')即原子的形状因子;
$\rho \left( {{ r}'} \right)$ 为样品立体元的密度,$P\left( {2\theta } \right)$ 为散射的偏振因子;等号右侧最后两项描述了单色光的传播,其中第1项采用了平面波近似,当样品厚度远远小于样品到光源的距离时,该近似足够准确。整个积分式描绘了光的相干叠加过程,在通常的CDI实验布局下,探测器中心位于透射光路上,相比于L,探测器的尺寸足够小,可以应用旁轴近似,此时有$${\text{z}}' + \left| {{ r} - { r}'} \right| = z' + \sqrt {{{(x - x')}^2} + {{(y - y')}^2} + {{(L - z')}^2}} \approx L + \frac{{{x^2} + {y^2}}}{{2L}} + \frac{{x{'^2} + y{'^2}}}{{2L}} - \frac{{xx' + yy'}}{L} + \frac{{z'({x^2} + {y^2})}}{{2{L^2}}}$$ (3) 带入(2)式可得
$${\phi _r} = {r_{\rm{e}}}\exp \left({{\rm i}{{k}}L}\right)\exp\left({{\rm i}{{k}}\frac{{{x^2} + {y^2}}}{{2L}}}\right)\int {\phi _0}(r')f(r')P(2\theta )\rho (r')\exp \left({\rm i}{k}\frac{{x'^2} +{y'^2}}{{2L}}\right) \exp {({ - {\rm i}{{{q}}_x}x'}})\exp {({ - {\rm i}{{{q}}_y}y'}})\exp {({ - {\rm i}{{{q}}_z}z'}}){\rm{d}}r' $$ (4) 式中:k为入射波矢;q(qx, qy, qz)为散射光与入射光之间的动量转移,其各分量可近似表示为
$$ {{{q}}_x} = \frac{x}{L}{{k}},\quad {{{q}}_y} = \frac{y}{L}{{k}},\quad {{{q}}_z} = - \frac{{{x^2} + {y^2}}}{{2{L^2}}}{{k}} $$ (5) (4)式的积分项中包含一个单纯与样品坐标相关的相位修正项,表现了样品有限尺寸对散射光干涉结果的影响,即表征了近场菲涅尔区衍射成像的特点[3]。若假设样品足够小,则散射角
${2\theta }$ 近似与样品坐标无关,且q不再需要进行(3)式的近似展开,从(2)式可直接获得[4]$$ {\varphi _{{ r}}} = {r_{\rm{e}}}P(2\theta ){\phi _0}\int {{\rho _{\rm{e}}}({ r}')} \exp {({ - {\rm i}{{q}} \cdot { r}'}}){\rm{d}}{ r}' $$ (6) 式中:
${\rho _{\rm{e}}}$ 为样品的电子密度。最终探测器像元接受的光强为$$ {I_{ r}} = {\left| {{\varphi _{ r}}} \right|^2}\Delta \varOmega $$ (7) 从(6)式可以看出,探测器面阵上散射光场的分布对应样品电子密度三维傅里叶变换的一个球面,即Ewald球面。当对样品旋转成像时,探测的Ewald球面相交,直至足够描绘出整个傅里叶变换的三维结构,由此可进行样品密度的三维表征。
2. CDI的相位复原
根据(6)式,若已知样品密度的傅里叶变换结果,就能够对样品密度进行重建。然而,面阵探测器只能测量变换结果的幅值,缺少变换结果的相位,导致重建无法进行,即CDI中所谓的相位问题。相位问题的实质就是对样品密度进行频率采样时,无法获得频谱的相位信息,使得采样数不足,无法提供足够的样品信息。为了解决相位问题,Sayre[5]指出,可以通过测量每两个衍射(频域)信号之间的强度信息,即将频域采样率扩大一倍,来恢复原频域采样点处的相位信息。这是因为样品密度的自关联函数分布恰好是样品密度分布的两倍大,能够确定自关联函数的信息就足以确定样品的密度分布,该要求的最低限度就是采样率扩大一倍。考虑对(7)式两边进行傅里叶逆变换,即
$${\rm{FT}}^{ - 1}\left[\frac{{{I_{ r}}}}{{\Delta \varOmega }}\right] = {\rm{FT}}^{ - 1}[{\left| {{\varphi _{ r}}} \right|^2}] = {\rm{FT}}^{ - 1}[{\varphi _{ r}}\varphi _{ r}^*] = {\rm{FT}}^{ - 1}[{\varphi _{ r}}] * {\rm{FT}}^{ - 1}[\varphi _{ r}^*] = {\rho _{\rm{e}}}({ r}') * {\rho _{\rm{e}}}( - { r}')$$ (8) (8)式结果就是密度的自关联函数。Sayre提出的采样方式就是单纯依据成像数据对相位进行复原,同时需要在成像时对样品过采样,这与目前CDI技术实际采用的方式极为相似。
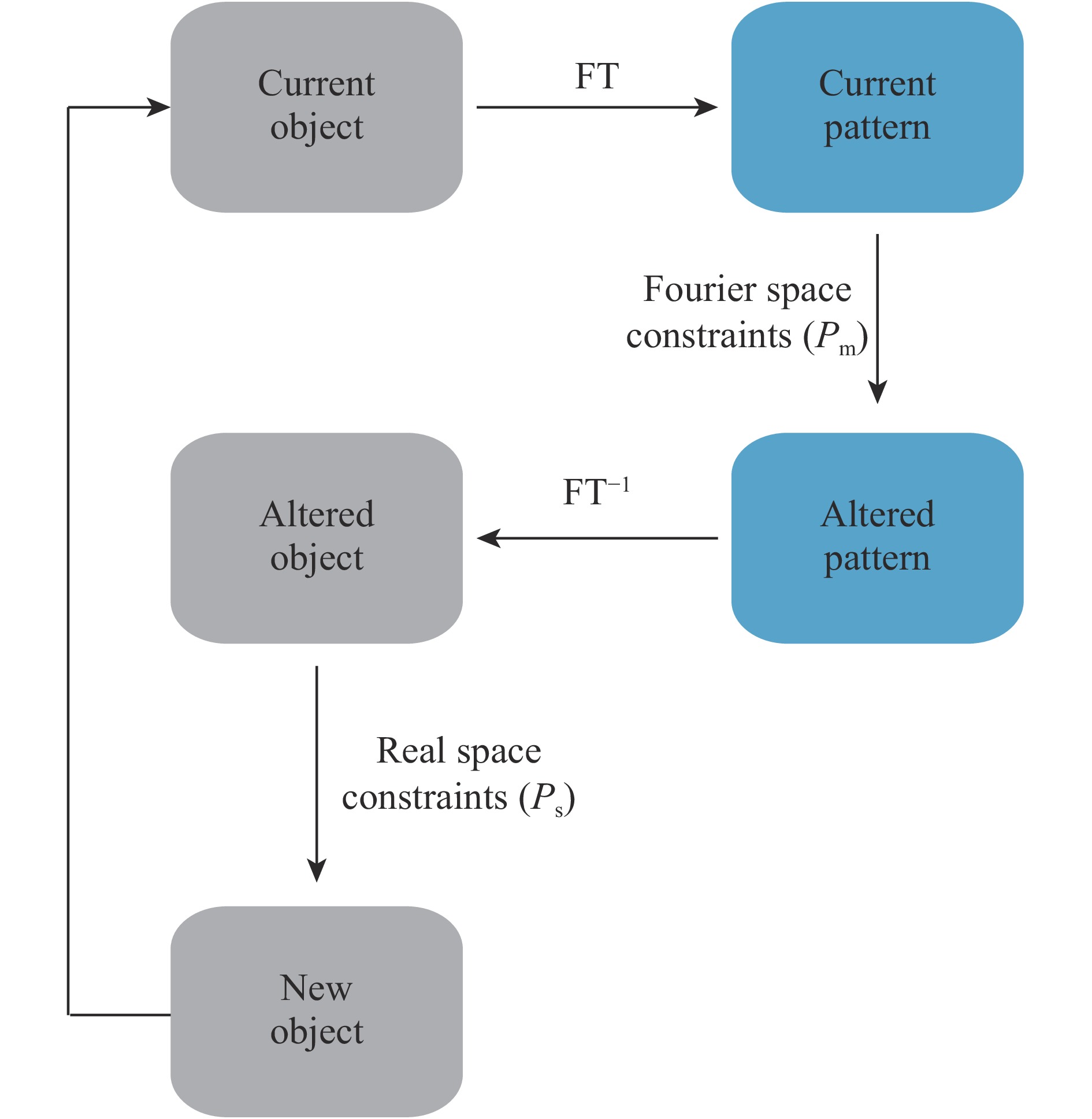
在Sayre给出了相位复原原理后,Gerchberg等[6]和Fienup[7]提出了具体的相位复原迭代算法。该迭代算法的原理是同时利用测量的成像(频域)强度数据和样品密度(空域)的已知信息,如样品的大小等,利用频域与空域的变换关系,反复在频域与空域进行变换,且在每一步变换中施加强度约束(等于测量值)并保留相位,通过反复迭代实现相位复原,具体流程见图2。在Gerchberg等的研究基础上,Fienup给出了分属两大类的多种算法:一类是梯度搜索算法,包括误差递减ER算法、最速下降SD算法等;另一类是输入输出算法,包括基本型输入输出IO算法、混合输入输出HIO算法等。至今,Fienup给出的部分算法依然是CDI相位复原中的主流算法,其他应用广泛的算法还包括Difference Map算法[8]、GHIO(Guided Hybrid Input-Output)算法[9]、RAAR (Relaxed Averaged Alternating Reflectors)算法[10]以及用于动态调整约束的Shrink-Wrap算法[11]。
影响相位复原的一个重要因素是过采样率
$\alpha $ 。过采样率定义为实际采样率与待恢复相位采样率的比值,显然过采样率超过1时,空域的范围超过真实样品的大小,超出部分可认定为零值,零值区域即为空域的约束。从Sayre的方法出发,至少要求在每个维度上过采样率达到2才能实现最基本的相位复原。1998年,Miao等[12]给出了另一种解释。根据(6)式,散射光是对实数密度的傅里叶变换,因此具有中心对称性。若探测面阵像素总数为N,则独立的频域强度数据为N/2,待恢复的频域相位数据亦为N/2,空域已知的零值区为$\left( {1 - 1/\alpha } \right)N$ ,未知样品区为$N/\alpha $ 。要唯一求解未知数据,则至少要求已知数据数目等于未知数据数目,等价于要求$\alpha $ 至少为2,此时对过采样率的要求变为总值不小于2,而对每个维度的过采样率没有要求。根据这个思路,当被变换的电子密度不再是实数时,频域图像不具有对称性,频域的已知与未知数据个数等量变化,最终对$\alpha $ 的要求依然是不小于2。当然,$\alpha $ 为2只是最低要求,实际进行迭代计算时很难达到收敛,相关研究指出$\alpha $ 为3~7时可以得到较好的相位复原结果[13]。从上述相位复原方法可以看出,频域与空域的变换关系(即(6)式)发挥着核心作用。需要明确指出,(6)式成立的基本条件之一是入射光为相干光,严格地讲即从样品上任意两点出发的散射光在成像面阵上的每个点都是相干叠加的,这样采集到的图像才是样品密度的频域信号。当光源相干性不足时,仅能获得部分样品的频域信息,也就无法重建完整的样品密度分布[14]。正是因为对光源相干性提出了极高的要求,CDI实验仅在高相干性的同步辐射源及近似全相干的X射线自由电子激光(XFEL)源上获得了突破性的研究成果。
3. CDI的研究进展
3.1 CDI的实验实现
1999年,在相位复原方法基本成熟之后,最早的CDI实验由Miao等[15]完成。该实验采用了近平面波照射的小角散射布局,形成平面波CDI方法,成为一系列CDI方法中最直接和典型的形式,可被看做基本型CDI。
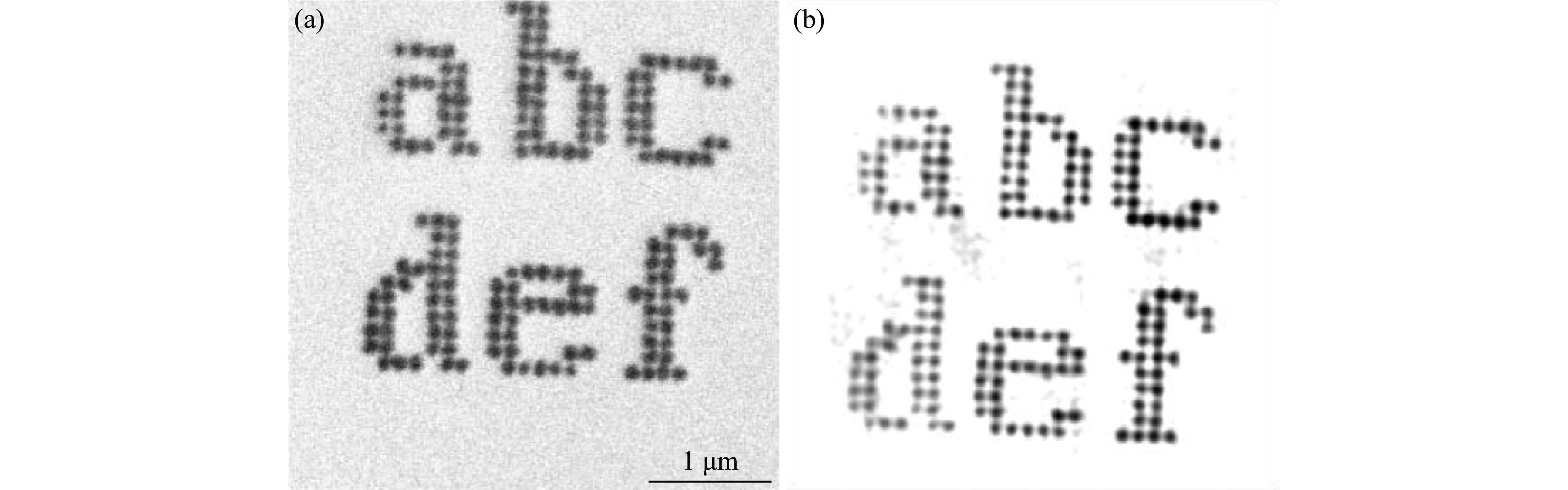
实验采用的样品是一系列百纳米尺寸金颗粒拼成的英文字母,如图3(a)所示。这些金颗粒附着在100 nm厚的氮化硅薄膜上,形成近二维样品,由于金颗粒的散射远远大于衬底薄膜,对收集到的成像图像进行处理可直接得到金颗粒的空间分布。实验利用的光源为美国NSLS同步辐射装置X1A线站提供的1.7 nm波长的X射线源,X射线束经过单色化处理以提升时间相干性,通过直径为
$10\;{\text{μ}}{\rm{m}}$ 的小孔以提升空间相干性,最终实现近似平面波照射到样品上。实验布局与图1类似,由于透射光强远大于散射光强,为了保护探测器,使其能够高灵敏地测量散射光,在探测器中心透射光范围内加入直径为
$220\;{\text{μ}}{\rm{m}}$ 的金属丝阻挡透射光。当然,金属丝也会阻挡中心区域的散射光,缺失的散射信号会导致所谓的“低频缺失”问题,当低频缺失范围超过样品频域图像的中心散斑大小时,样品重建质量下降甚至失败[16]。为了克服这个难题,实验中利用低分辨的样品光学显微图像,以光学图像为假想样品,利用(6)式和(7)式补足中心缺失的低频信号。这种方法的巧妙之处在于,虽然光学显微图像的空间分辨低,但是恰好能够提供样品大尺度的分布信息,即低频信息。补足的衍射图像用于相位重建,根据实验提供的参数,实际过采样率约为9.5,完全满足过采样条件。经过1000次迭代,重建算法很好地给出了金颗粒的空间分布,结果如图3(b)所示。
Miao等的实验可看做对非晶样品的二维重建,实验所达到的空间分辨率不是很高(约为75 nm)。随后的实验研究则针对样品的三维重建以及更高的空间分辨率,并且研究样品也拓展到晶态物质,尤其是纳米晶颗粒。
3.2 三维重建的CDI实验
3.2.1 基于图像拼接的三维重建
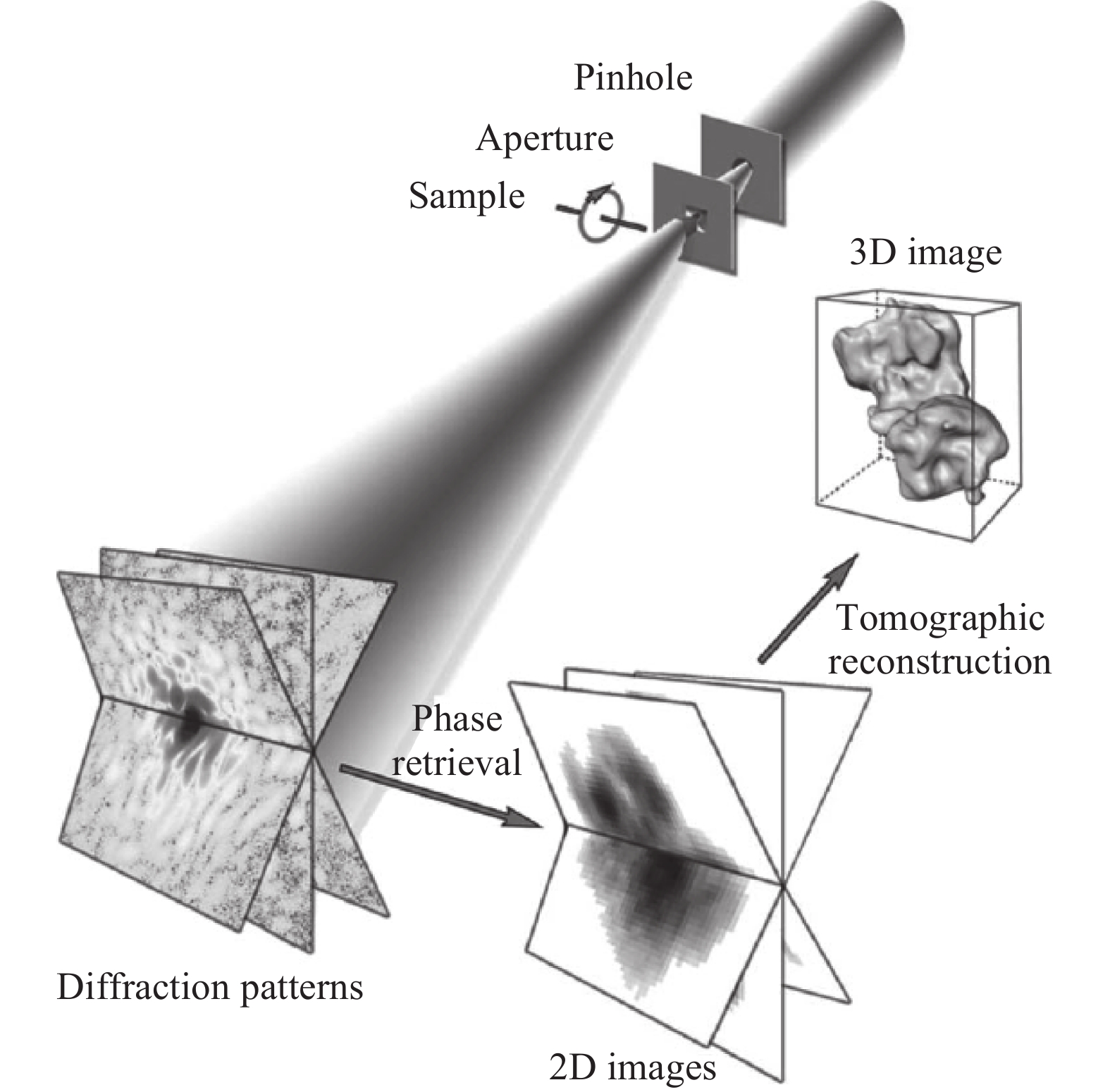
与二维重建相比,利用CDI技术的三维重建不是对单一图像的处理,而是涉及大量的频域图像。一种方案是对这些频域图像进行正确分类并融合拼接,以构成频域空间的三维数据集,然后进行迭代重建。在这种方法中,利用三维数据集进行相位恢复或样品重建的步骤并不比二维重建复杂,难点在于获取三维数据集。这涉及对采集的每张二维频域图像在三维频域空间的定位、临近位置图像的拼接与融合。对于可操控的样品,其空间取向在成像前已经确定,图像定位十分简单。但对于很小的生物大分子和纳晶样品:一则样品在单次成像后会由于辐照损伤而被破坏,很难重复成像;二则这类样品采用交叉束测量方式,实验中很难操控样品的空间取向,也就难以事前对图像进行定位。因此,需要利用成像图像本身的信号特征(如衍射峰等)确定图像取向,完成三维拼接。
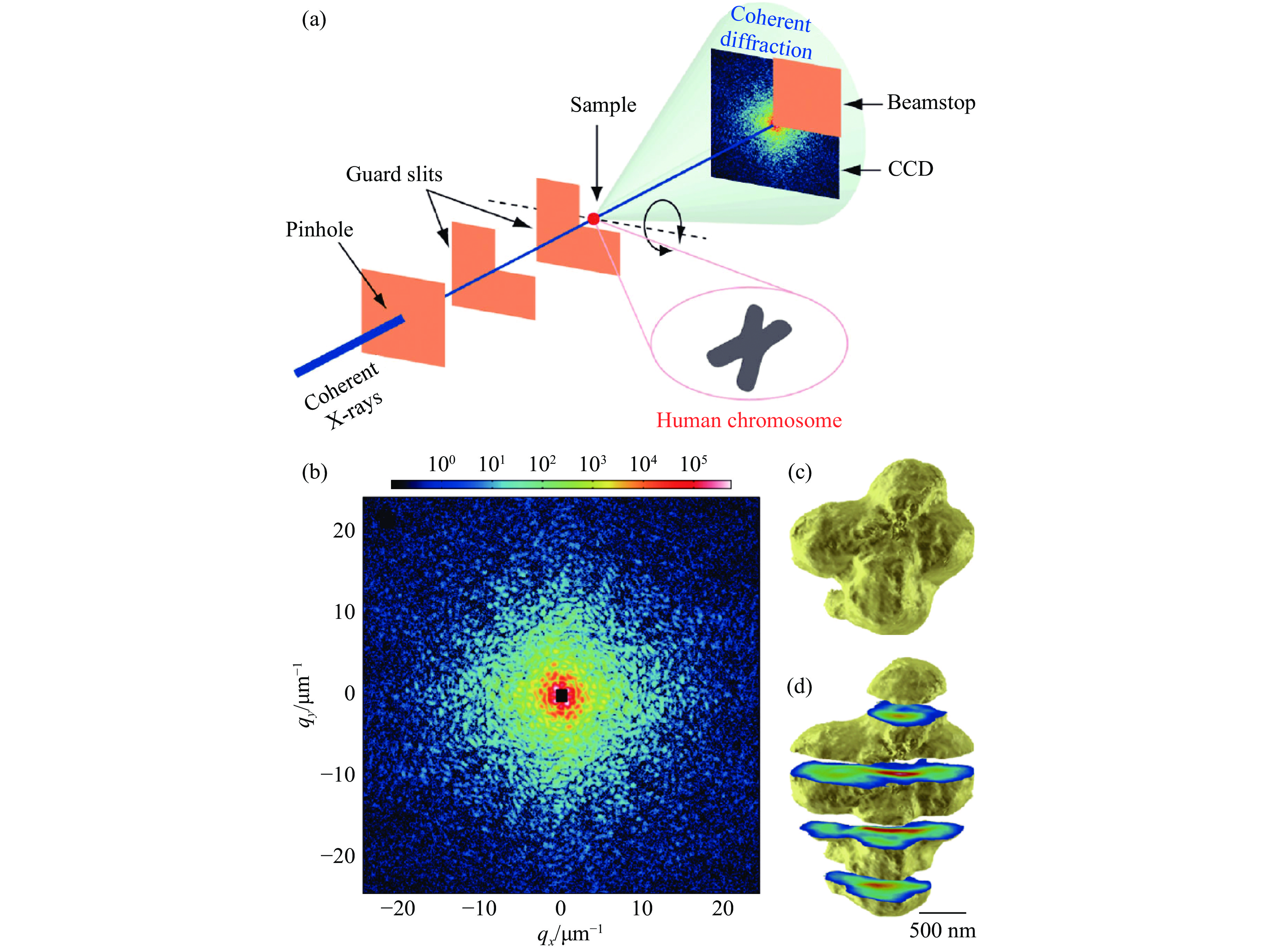
图4展示了可操控方向样品的三维图像重建实验[17]和实验结果。实验样品为人肿瘤细胞的染色体,通过化学方法将其固定在100 nm厚的氮化硅薄膜上,以旋转薄膜的方式调整染色体方向。实验光源为日本SPring-8同步辐射BL29XUL线站提供的5 keV的X射线光源。进行样品三维重建时,选取了38个方向获取的衍射图像,通过插值法得到三维衍射图像,并利用HIO算法恢复相位。由于三维重建使用的衍射图像数较少,其空间分辨从二维投影重建时的约38 nm下降为约120 nm。
图5展示了不可操控取向生物样本的三维重建实验[18]。实验样品为直径约450 nm的病毒,成像光源是美国LCLS装置提供的XFEL脉冲。实验首先将样品制备为气溶胶并以窄束形式喷出,与XFEL脉冲序列交叉,当交叉点上同时存在病毒颗粒和XFEL脉冲时,就能在探测器上获得散射图像。当然为了进行单颗粒成像并保证成像质量,当病毒颗粒与XFEL脉冲“擦过”或单个XFEL脉冲与多个病毒颗粒作用时,应当认为获得的散射图像无效。因此一个完整的图像处理过程应当至少包括“命中检定→无效数据剔除→图像定向→拼接融合→三维重建”几个步骤。
实验图像定向采用了Duane等[19]提出的EMC算法,这是目前主流的CDI图像定向算法。经过定向的二维图像可拼接成三维图像。为了提高信噪比,也可以先把取向相近的图像融合成一幅图像,再进行三维拼接。实验最终确定了198幅图像,拼接结果如图5(a)所示。通过HIO算法进行三维重建,重建结果如图5(b)所示,重建样品的分辨率约为125 nm。在进行此实验之前,仅有对该种病毒表面衣壳的三维成像,其内部结构尚不清楚,利用CDI技术获得的重建结果不仅给出了病毒的形貌特征,同时也给出了病毒内部的层次结构,实现了真正的三维成像。
3.2.2 基于二维重建结果的样品三维重建
进行样品三维重建的另一种方案是对单个频域图像进行二维重建,获得三维样品的二维密度投影后,再利用多角度的密度投影重建样品三维密度。这种方案与计算机断层成像(CT)技术的重建方式相近,更适用于样品空间取向已知且成像数据较少的情形。
图6展示了利用这种方案的三维样品重建实验[20]。实验样品为GaN量子点粒子,光源为SPring-8同步辐射BL29XUL线站提供的5 keV X射线。通过旋转样品的方法, 在–70°~70°之间获得27幅样品衍射图样。利用GHIO算法,对二维衍射图像进行投影重建,获取不同角度的面密度分布,然后利用等倾断层扫描方法获取了GaN量子点的三维微观结构,达到的空间分辨率约为17 nm。
3.3 高分辨的二维重建CDI
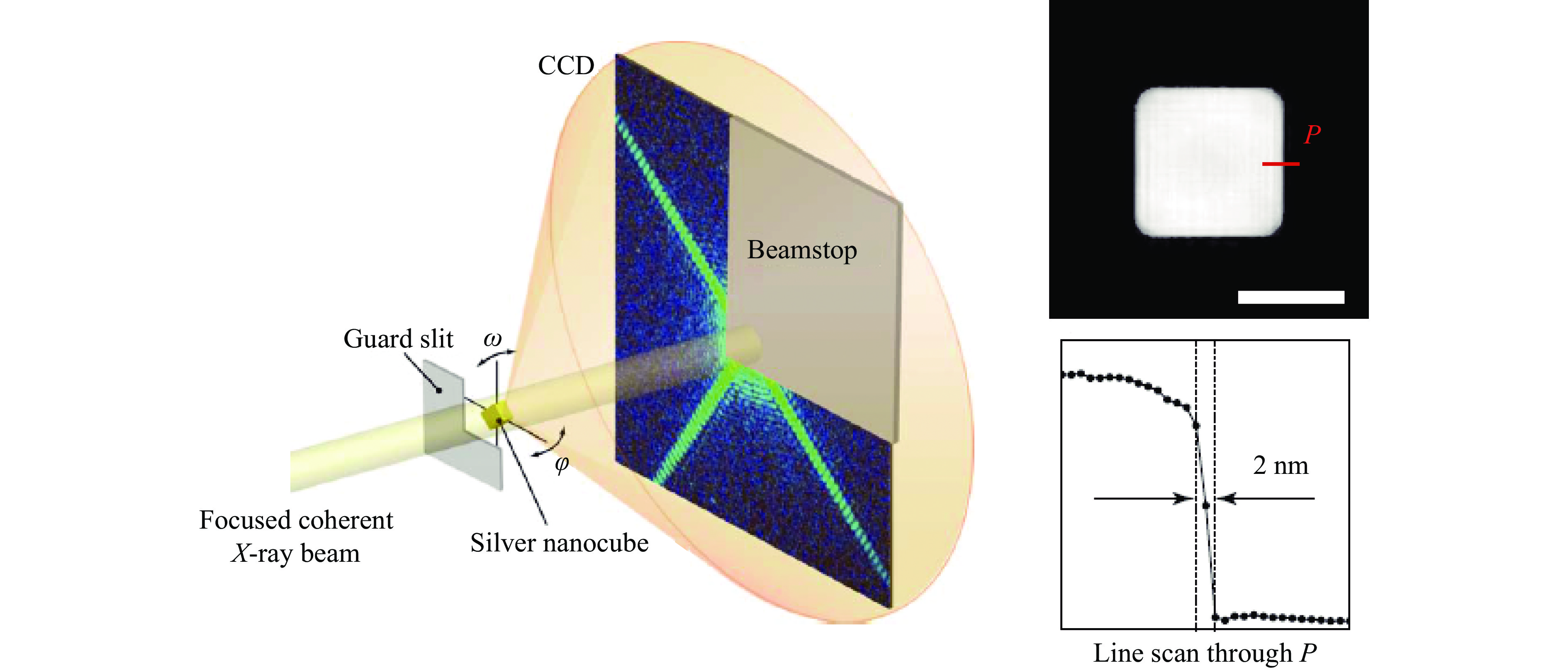
与三维重建相比,二维重建更容易达到超高空间分辨率。图7展示了达到2 nm空间分辨的CDI实验[21]。实验也是在SPring-8同步辐射装置BL29XUL线站上完成的,光子能量为11.8 keV,样品为150 nm厚的银立方体颗粒。相位恢复使用HIO算法和Shrink-Wrap算法,重建的银颗粒在边界处的空间分辨率达到2 nm。相比于其他超高分辨的实验技术,2 nm的分辨水平并不突出,但其他技术通常只对样品表面敏感,穿透样品的能力不强,而CDI技术可用于检测更厚的样品,且可以达到很高的空间分辨能力,该实验提供了很好的例证。
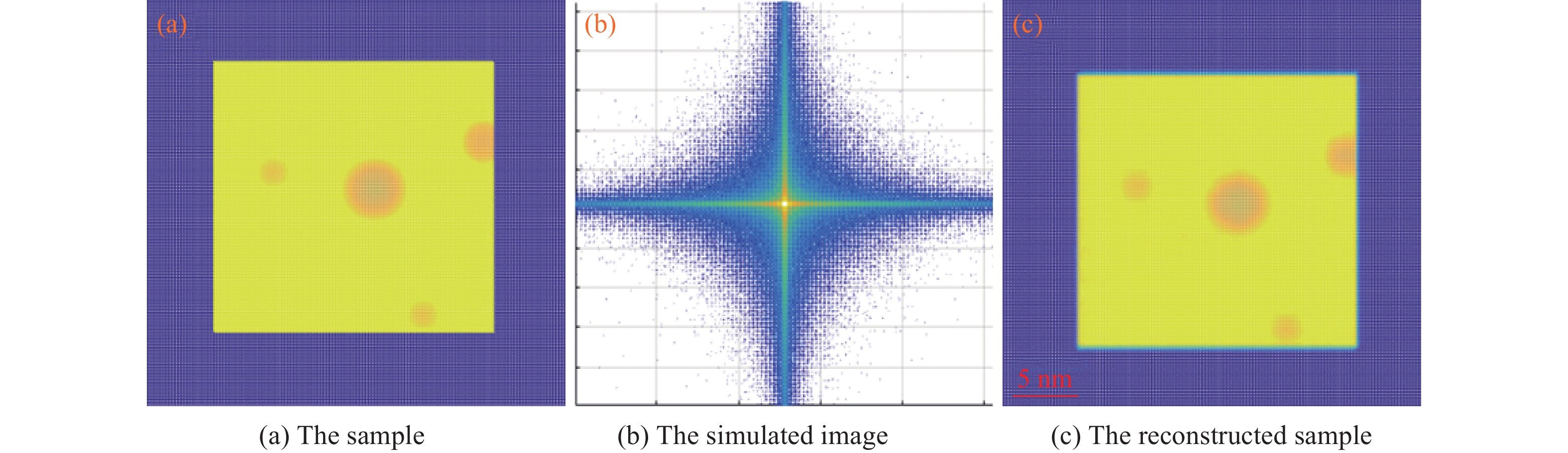
为了验证二维CDI重建的超高分辨能力,我们进行了模拟实验,如图8所示。实验样品是边长为50 nm的Cu立方纳晶,其中包含位置随机的球形孔洞,用于成像的光子能量为8.435 keV。模拟过程中加入了对入射光束的阻挡和随机噪声等因素,得到的成像图像仅在中心和两个轴线上有可观强度,相位恢复使用了HIO和Shrink-Wrap算法。从图8中可以看出,分布在纳晶内部的孔洞得到很好的复原,图像的空间分辨可达0.5 nm。
高空间分辨、三维成像是CDI技术最突出的优势,同时也是一种很好的无损检测方法。当使用同步辐射光源时,较低的光子通量使得样品损伤很小;而当使用超强的XFEL光源时,可利用单发测量实现破坏前成像。因此,CDI技术在材料科学、生命科学等领域均有极高的应用价值。
4. CDI技术的扩展
前面介绍的实验均为基本的平面波型CDI实验,探测器放置在透射光束方向,目的是获取样品的密度分布。除此之外,还有多种扩展型的CDI实验方案,旨在扩大CDI实验的诊断能力或通过重建相位获取更丰富的样品内部信息。
4.1 扫描型CDI
单次CDI成像中,(5)式决定了在固定探测条件下的频域采样间隔,根据(6)式该间隔又决定了可成像的样品尺寸。扫描型CDI[22–25]的目的是扩大成像视场范围,使得更大尺寸样品的CDI成像成为可能。该方法是利用一个稳定光源对大块样品进行扫描式成像,每次只对其中一个小区域成像,且使相邻的成像区域发生交叠。由于相邻图像都有来自相同样品区的贡献,将临近图像一起纳入迭代算法可以使相位复原计算的收敛速度加快。图9展示了该方法对一个最外圈宽度为70 nm的菲涅尔波带片的重建结果[22],图9(a)为强度图像,图9(b)为相位图像,从中能够清楚地分辨出波带片的最外圈,同时在放大图中也可以表征出波带片的不均匀性。
虽然扫描型CDI可以对大尺寸样品进行成像,但是其相位重建方法是基于一个不变的光源,这个条件有时很难满足,从而在很大程度上限制了该方法的应用范围。
4.2 布拉格型CDI
布拉格型CDI[26–31]主要是针对晶体样品进行成像的扩展技术。晶体样品存在固有的周期性,这个周期性也会在频域空间体现出来。当在频域空间平移整数个周期时,(6)式的频域图像将不发生任何变化,但图像对应的频域中心已经移至某个布拉格点处,即成像在某个特定散射角附近进行。显然,只有晶体样品可以移至某个布拉格格点附近进行CDI成像。
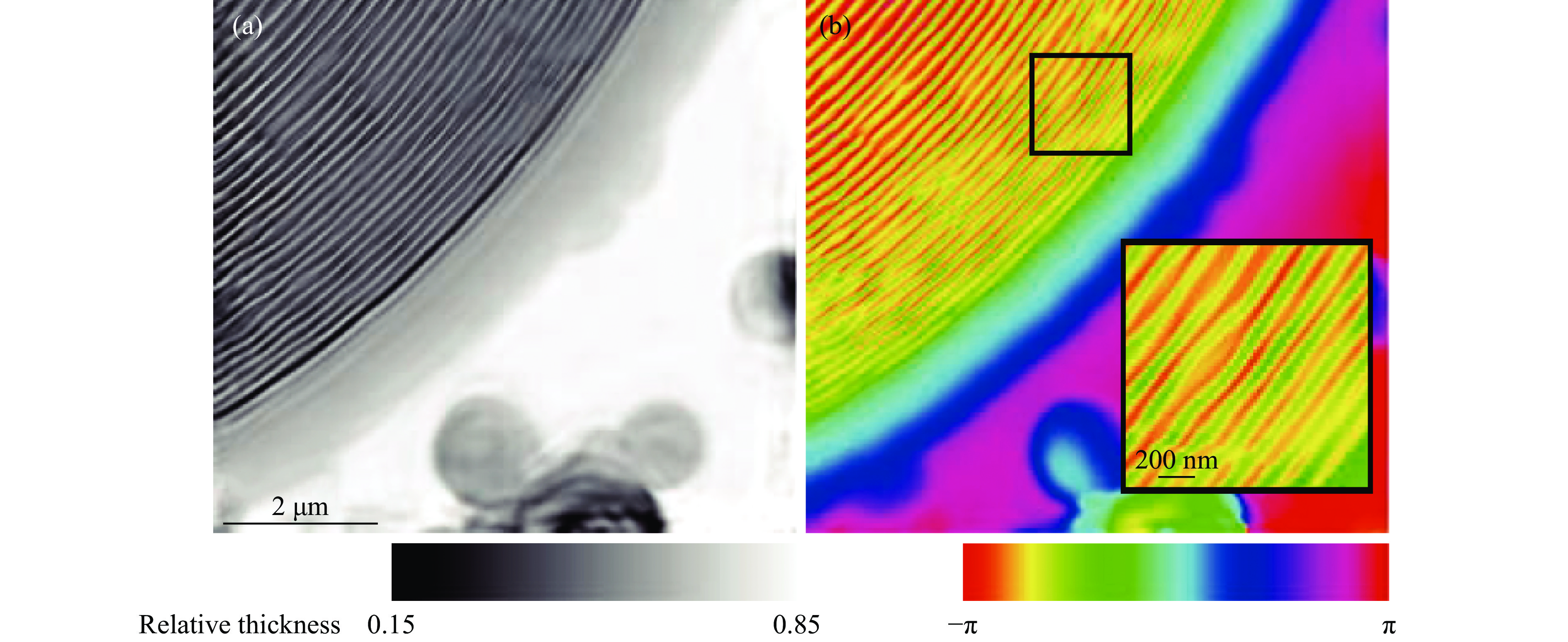
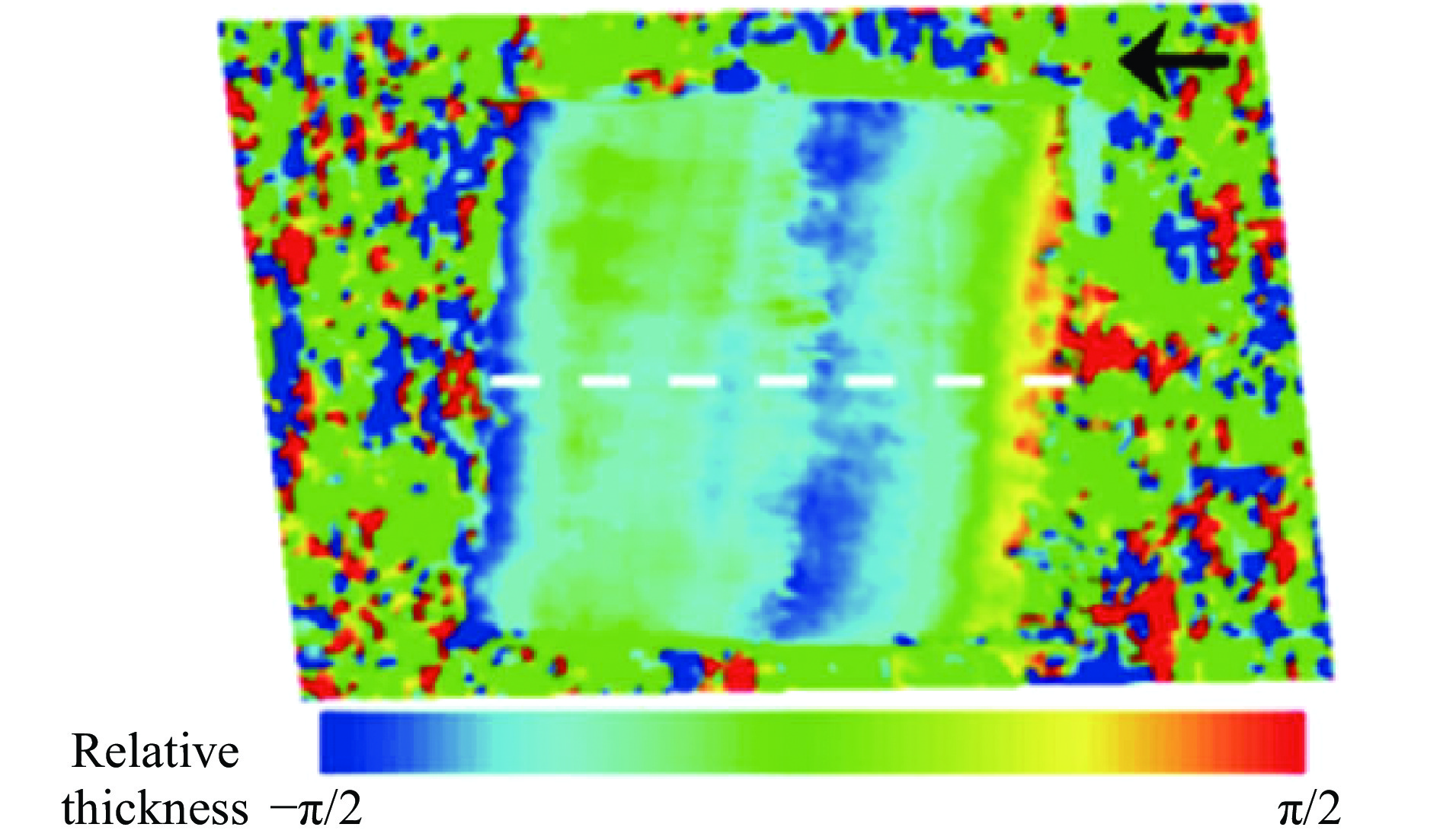
对于晶体样品,原子位置坐标可以用一个周期性的晶格坐标u(r)加上一个原子相对晶格的偏移坐标u'(r)表示。根据(6)式,前者构成了布拉格格点,后者在完美晶格的傅里叶变换上叠加了一个纯粹的相位exp(–iqu')。显然,利用CDI的相位重建技术获取相位信息后,该相位可以直接表征晶体内部的应变分布情况,如图10所示[31]。
5. 总结及展望
本文从成像原理、相位复原方法和研究进展及技术拓展等方面介绍了相干衍射成像技术。微观CDI的成像公式表明:CDI成像是满足远场条件下的散射光成像,其图像是样品三维密度傅里叶变换的一个剖面。利用这种傅里叶变换关系,在对样品图像进行过采样的条件下,可以通过迭代算法对成像光场的强度及相位进行复原,以此给出样品的密度分布信息。若能够在三维空间中拼接出完整的傅里叶变换图像,即可实现对诊断样品的三维重建。
CDI技术的突出优势有:成像系统极为简化,成像分辨灵活可调且可达到原子级空间分辨,具有三维成像能力,能够做到近似无损检测,具有丰富的样品诊断能力。通过几个典型CDI实验展示了该技术应用于不同诊断情形下灵活多样的重建能力,且通过对CDI的实验设置及相位复原方法进行扩展,可以实现更为丰富的样品诊断能力。
未来CDI可以向更大的诊断样品和更高的空间分辨能力两个方向发展。更高的空间分辨要求更精密的成像设备和更优质的光源,更大的诊断样品则对光源亮度及相干性提出了更高要求。当介观样品达到或超出探测光束尺寸时,用于探测的相干光场变得非均匀,且透射光场由于衍射效应与散射光场高度重叠,为相干衍射成像的重建带来了新的困难,也为相应的实验布局设置、相位复原及样品重建方法等研究提供了新的机遇。
可以预期,成像光源的进步将极大促进CDI成像能力的提升。随着基于衍射极限技术的新型同步辐射光源的建设,光源相干性及亮度均会显著提高,从而极大提升CDI技术的结构诊断能力。伴随近乎横向全相干自由电子激光XFEL装置的发展,介观样品的CDI成像是一种可被寄望于动力学过程动态诊断的重要XFEL实验技术。
-
-
[1] CHAO W, HARTENECK B D, LIDDLE J A, et al. Soft X-ray microscopy at a spatial resolution better than 15 nm [J]. Nature, 2005, 435(7046): 1210. doi: 10.1038/nature03719 [2] BARBER J L, BARNES C W, SANDBERG R L, et al. Diffractive imaging at large fresnel number: challenge of dynamic mesoscale imaging with hard X-rays [J]. Physical Review B, 2014, 89(18): 184105. doi: 10.1103/PhysRevB.89.184105 [3] XIAO X H, SHEN Q. Wave propagation and phase retrieval in fresnel diffraction by a distorted-object approach [J]. Physical Review B, 2005, 72(3): 033103. doi: 10.1103/PhysRevB.72.033103 [4] MIAO J W, AMONETTE J E, NISHINO Y, et al. Direct determination of the absolute electron density of nanostructured and disordered materials at sub-10-nm resolution [J]. Physical Review B, 200, 68(1): 012201. [5] SAYER D. Some implications of a theorem due to shannon [J]. Acta Crystallographica, 1952, 5: 843. [6] GERCHBERG R W, SAXTON W O. A practical algorithm for the determination of phase from image and diffraction plane pictures [J]. Optik, 1972, 35: 237. [7] FIENUP J R. Phase retrieval algorithm: a comparison [J]. Applied Optics, 1982, 21: 2758. doi: 10.1364/AO.21.002758 [8] ELSER V. Phase retrieval by iterated projections [J]. Journal of the Optical Society of America, 2003, 20(1): 40. doi: 10.1364/JOSAA.20.000040 [9] CHEN C C, MIAO J, WANG C W, et al. Application of optimization technique to noncrystalline X-ray diffraction microscopy: guided hybrid input-output method [J]. Physical Review B, 2007, 76(6): 064113. doi: 10.1103/PhysRevB.76.064113 [10] LUKE D R. Relaxed averaged alternating reflections for diffraction imaging [J]. Inverse Problems, 2005, 21: 37. doi: 10.1088/0266-5611/21/1/004 [11] MARCHESINI S, HE H, CHAPMAN H N, et al. X-ray image reconstruction from a diffraction pattern alone [J]. Physical Review B, 2003, 68: 140101. doi: 10.1103/PhysRevB.68.140101 [12] MIAO J, SYAER D, CHAPMAN H N. Phase retrieval from the magnitude of the fourier transforms of nonperiodic objects [J]. Josa A, 1998, 15: 1662. doi: 10.1364/JOSAA.15.001662 [13] 周光照, 佟亚军, 陈灿, 等. 相干X射线衍射成像的数字模拟研究 [J]. 物理学报, 2011, 60(2): 028701.ZHOU G Z, TONG Y J, CHEN C, et al. Digital simulation for coherent X-ray diffractive imaging [J]. Acta Physica Sinica, 2011, 60(2): 028701. [14] VARTANYANTS I A, ROBINSON I K. Partial coherence effects on the imaging of small crystals using coherent X-ray diffraction [J]. Journal of Physics: Condensed Matter, 2001, 13(47): 10593. doi: 10.1088/0953-8984/13/47/305 [15] MIAO J W, CHARALAMBOUS P, KIRZ J, et al. Extending the methodology of X-ray crystallography to allow imaging of micrometer-sized non-crystalline specimens [J]. Nature, 1999, 400: 342. doi: 10.1038/22498 [16] MIAO J W, NISHINO Y, KOHNURA Y, et al. Quantitative image reconstruction of GaN quantum dots from oversampled diffraction intensities alone [J]. Physical Review Letters, 2005, 95(8): 085503. doi: 10.1103/PhysRevLett.95.085503 [17] NISHINO Y, TAKAHASHI Y, IMAMOTO N, et al. Three-dimensional visualization of a human chromosome using coherent X-ray diffraction [J]. Physical Review Letters, 2009, 102(1): 018101. doi: 10.1103/PhysRevLett.102.018101 [18] EKEBERG T, SVENDA M, ABERGEL C, et al. Three-dimensional reconstruction of the giant mimivirus particle with an X-ray free-electron laser [J]. Physical Review Letters, 2015, 114(9): 098102. doi: 10.1103/PhysRevLett.114.098102 [19] DUANE N T, ELSER V. Reconstruction algorithm for single-particle diffraction imaging experiments [J]. Physical Review E, 2009, 80(2): 026705. doi: 10.1103/PhysRevE.80.026705 [20] MIAO J W, CHEN C C, SONG C, et al. Three-dimensional GaN-Ga2O3 core shell structure revealed by X-ray diffraction microscopy [J]. Physical Review Letters, 2006, 97(21): 215503. doi: 10.1103/PhysRevLett.97.215503 [21] TAKAHASHI Y, NISHINO Y, TSUTSUMI R, et al. High-resolution projection image reconstruction of thick objects by hard X-ray diffraction microscopy [J]. Physical Review B, 2010, 82(21): 214102. doi: 10.1103/PhysRevB.82.214102 [22] THIBAULT P, DIEROLF M, MENZEL A, et al. High-resolution scanning X-ray diffraction microscopy [J]. Science, 2008, 321(5887): 379. doi: 10.1126/science.1158573 [23] RODENBURG J M, HURST A C, CULLIS A G, et al. Hard-X-ray lensless imaging of extended objects [J]. Physical Review Letters, 2007, 98(3): 034801. doi: 10.1103/PhysRevLett.98.034801 [24] KLAUS G, PIERRE T, SEBASTIAN K, et al. Quantitative biological imaging by ptychographic X-ray diffraction microscopy [J]. Proceedings of the National Academy of Sciences of the United States of America, 2010, 107(2): 529. doi: 10.1073/pnas.0905846107 [25] DIEROLF M, MENZEL A, THIBAULT P, et al. Ptychographic X-ray computed tomography at the nanoscale [J]. Nature, 2010, 467(7314): 436. doi: 10.1038/nature09419 [26] ROBINSON I K, VARTANYANTS I A, WILLIAMS G J, et al. Reconstruction of the shapes of gold nanocrystals using coherent X-ray diffraction [J]. Physical Review Letters, 2001, 87(19): 195505. doi: 10.1103/PhysRevLett.87.195505 [27] WILLIAMS G J, PFEIFER M A, VARTANYANTS I A, et al. Three-dimensional imaging of microstructure in Au nanocrystals [J]. Physical Review Letters, 2003, 90(17): 175501. doi: 10.1103/PhysRevLett.90.175501 [28] PFEIFER M A, WILLIAMS G J, VARTANYANTS I A, et al. Three-dimensional mapping of a deformation field inside a nanocrystal [J]. Nature, 2006, 442(7098): 63. doi: 10.1038/nature04867 [29] NEWTON M C, LEAKE S J, HARDER R, et al. Three-dimensional imaging of strain in a single ZnO nanorod [J]. Nature Materials, 2010, 9(2): 279. [30] HARDER R, ROBINSON I. Coherent X-ray diffraction imaging of strain at the nanoscale [J]. Nature Materials, 2009, 8(4): 291. doi: 10.1038/nmat2400 [31] GANG X, OUSSAMA M, MANFRED R, et al. Coherent X-ray diffraction imaging and characterization of strain in silicon-on-insulator nanostructures [J]. Advanced Materials, 2014, 26(46): 7747. doi: 10.1002/adma.v26.46 -







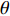
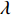
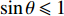
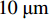

 下载:
下载:
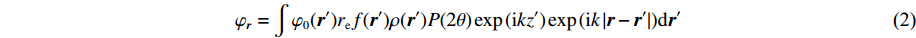
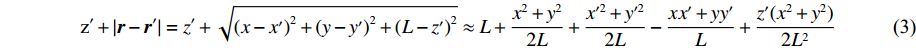
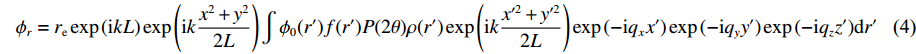

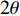
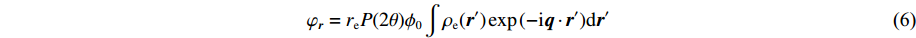
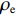
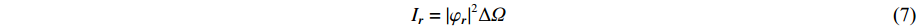
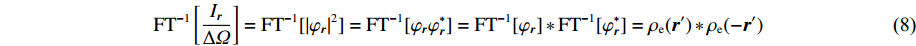

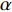
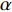
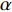
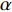
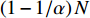
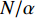
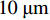










 下载:
下载: