动载下准脆性材料的泛形裂纹研究

明德涵; 欧卓成; 杨筱; 段卓平; 黄风雷

doi:10.11858/gywlxb.20190754

动载下准脆性材料的泛形裂纹研究

doi: 10.11858/gywlxb.20190754

北京理工大学爆炸科学与技术国家重点实验室，北京　100081

基金项目: 国家自然科学基金（11772056）

详细信息

作者简介:
明德涵（1994－），男，硕士研究生，主要从事材料与结构冲击动力学研究. E-mail: bitmdh@163.com

通讯作者:
欧卓成（1961－），男，博士，教授，主要从事材料与结构冲击动力学研究. E-mail: zcou@bit.edu.cn

中图分类号: O346.1
计量
- 文章访问数: 8684
- HTML全文浏览量: 3084
- PDF下载量: 31
出版历程
- 收稿日期: 2019-04-03
- 修回日期: 2019-04-24

Ubiquitiform Crack of Quasi-Brittle Materials under Dynamic Loading

State Key Laboratory of Explosion Science and Technology, Beijing Institute of Technology, Beijing 100081, China

摘要

摘要: 为建立动态拉伸载荷作用下准脆性材料裂纹扩展路径的泛形表征，提出了一种非均匀准脆性材料动态裂纹扩展的泛形模型，计算得到的泛形裂面复杂度与已有实验数据吻合较好。结果表明：动态拉伸载荷作用下的裂纹扩展路径是泛形的，其复杂度随加载应变率的增大而减小，并与材料动态拉伸承载能力的空间随机分布无关，且随Weibull分布形状参数m的增加而减小。研究结果为分析动态拉伸载荷作用下准脆性材料的裂纹扩展机理即泛形表征提供了依据。
- 准脆性材料 /
- 泛形裂纹 /
- 应变率 /
- 复杂度
Abstract: To investigate the ubiquitiformal characteristic of the crack extension path in a heterogeneous quasi-brittle material under the dynamic tensile loadings, a ubiquitiformal model is developed in this paper, and the calculated numerical results for the ubiquitiform complexity are in agreement with the previous experiments. It is found that such a crack extension path is indeed of a ubiquitiform, and its complexity decreases with the increase of the loading strain-rate. Moreover, it is also found that the complexity is independent of the randomness of the spatial distribution of the dynamic tensile load-carrying capacity of the material under consideration, and the complexity decreases with increasing shape parameter m of the Weibull distribution. Thus, this work can be taken as a basis for analyzing further the mechanism as well as the ubiquitiformal characteristic of the crack profile in a quasi-brittle material under the dynamic tensile loadings.
- quasi-brittle materials /
- ubiquitiform crack /
- strain-rate /
- complexity

HTML全文

研究金属靶板在弹丸冲击作用下的响应和破坏对军用武器和防护结构的设计和评估有重要的意义。单层金属靶板在刚性平头弹丸正撞击下的破坏模式可分为：带有整体变形的简单剪切破坏和局部化的绝热剪切冲塞破坏，从能量吸收的角度而言前者优于后者。在工程实践中，常用双层板结构代替单层板。对于单层金属板，很多学者做了相关的理论、实验和数值模拟研究。Wen和Jones^[1-2]对刚性平头弹丸低速正撞击下固支的软钢圆板和铝合金圆板的响应和破坏进行了系统的实验研究，并根据实验结果和理论分析提出了刚性平头弹丸正撞下金属靶板低速穿透的Wen-Jones模型。BØrvik等^[3]通过实验、理论分析和数值模拟研究了不同厚度固支Weldox460E圆板在平头弹撞击下的变形和穿透，通过初始速度和残余速度求出不同厚度靶板的弹道极限值。Chen等^[4]利用刚塑性分析方法研究了平头弹撞击金属圆板的问题，考虑了结构的整体响应和局部剪切破坏。这些研究能够较好地描述和预测平头弹撞击下单层金属靶板的破坏模式和抗弹性能。

相比于单层板冲击失效响应方面的大量实验、数值和理论的研究文献，在公开发表的文章中仅有少量文章研究了多层板的抗弹性能。理论方面只提出了简单模型，大部分都是实验和数值模拟研究，且得出的结论也大相径庭。Radin等^[5]做了很多平头弹穿透单层和多层2024-0铝板的实验，发现单层板的弹道极限均高于等厚度的多层板，同时用理论分析模型计算了弹丸的弹道极限，分析结果和实验一致。张伟等^[6]通过实验研究发现厚度较小时，同样厚度的单层钢板的弹道极限要高于等厚接触式双层板的弹道极限。Teng等^[7]通过ABAQUS/Explicit研究了金属单层板和等厚双层板的抗弹性能，数值模拟结果表明：双层板弹道极限高于单层板7%~25%。对平头弹而言，Dey等^[8]实验得到的等厚接触式双层板的弹道极限约高于单层板弹道极限47.2%，与实验结果相比，数值模拟结果(数值模拟结果约高22.9%)大大低估了双层板的抗弹性能。

目前对接触式双层板在平头弹撞击下的抗弹性能和破坏模式尚未有统一的结论，仍需要进行大量的研究工作。我们对等厚接触式双层金属板在平头弹撞击下的穿透破坏进行了理论研究，基于先前单层金属板的穿透理论和实验观察提出一个等厚接触式双层金属板穿透的新模型，并与相关实验数据和其他理论模型进行比较和讨论。

1. 等厚接触式双层板穿透的理论模型

图 1给出了单层板和等厚接触式双层板在平头弹撞击下的示意图。单层板厚度为H，双层板总厚为H，上、下板等厚度，各为H/2，板的半径为R，平头弹弹径为d(d=2a)。由于靶板间的相互作用，双层板的变形和破坏相较单层板要复杂很多。

图 1 平头弹加载固支金属圆板示意图

Figure 1. Schematic of fully-clamped circular metal plates loaded by flat-ended projectile

下载: 全尺寸图片幻灯片

1.1 第一层板的能量吸收

令F为平头弹作用在双层板上时所受的总作用力，F₁为第一层板所受的作用力，F₂为第二层板所受的作用力，则F=F₁+F₂；第一层板的总体变形W_o1，第二层板的总体变形为W_o2。根据文献[1]，有

对第一层板：

${F_1} = {K_{1{\rm{m}}}}{W_{{\rm{o}}1}} + {F_{1{\rm{c}}}}$

(1)

对第二层板：

${F_2} = {K_{2{\rm{m}}}}{W_{{\rm{o}}2}} + {F_{2{\rm{c}}}}$

(2)

式中:K_1m和K_2m分别为第一层和第二层板的薄膜刚度，且有K_1m=K_2m=K_m=2πN₀/ln(R/a)，N₀为靶板单位长度的薄膜力，且有N₀=σ_yH/2，σ_y为靶板材料的屈服应力；F_1c和F_2c为两层板的静态极限载荷，且有 ${F_{1{\rm{c}}}} = {F_{2{\rm{c}}}} = {F_{\rm{c}}} = \left( {4/\sqrt 3 } \right){\rm{ \mathsf{ π} }}{M_0}\left[ {1 + \left( {1 + \sqrt 3 /2} \right)/\ln \left( {R/a} \right)} \right]$ ，M₀=σ_y(H/2)²/4为靶板单位长度的极限塑性弯矩。第一层板穿透破坏之前，第二层板紧贴第一层板，该过程第一层板和第二块板的整体变形基本相同，可以表示为

${W_{{\rm{o}}1}} \approx {W_{{\rm{o}}2}} = {W_{\rm{o}}}$

(3)

结合F=F₁+F₂和方程(1)、方程(2)，有

$F = 2{K_{\rm{m}}}{W_{\rm{o}}} + 2{F_{\rm{c}}}$

(4)

对于固支金属圆板，等效应变ε_e^[9]可以表达为

$\varepsilon _{\rm{e}}^2 = \frac{4}{3}\left( {\varepsilon _r^2 + {\varepsilon _r}{\varepsilon _\theta } + \varepsilon _\theta ^2} \right) + \frac{1}{3}\gamma _{rz}^2$

(5)

式中:ε_r、ε_θ、γ_rz分别为径向应变、周向应变和横向剪应变，对于厚度为H/2的靶板，其值可表示为

${\varepsilon _r} = \frac{{W_0^2}}{{2{a^2}{{\ln }^2}\left( {a/R} \right)}} + \frac{{{W_0}\left( {H/2} \right)}}{{2{a^2}\ln \left( {a/R} \right)}}$

(6)

${\varepsilon _\theta } = - \frac{{{W_0}\left( {H/2} \right)}}{{2{a^2}\ln \left( {a/R} \right)}}$

(7)

第一层板横向剪应变^[1]可以表示为

${\gamma _{rz}} = \mathit{\Delta }/e = {\left( {F/{F_{\rm{u}}}} \right)^{1/n}}{\gamma _{\rm{c}}}$

(8)

式中: γ_c为临界剪应变；Δ为弹丸压入深度，Δ_c为临界压入深度；e为剪切带半宽度；F_u为发生剪切冲塞破坏的临界剪切力，F_u=τ_uA_s，τ_u=σ_u[0.41H/(2d)+0.42]，A_s=πdH/2，σ_u为极限拉伸应力。令(5)式中的等效应变ε_e等于拉伸破坏应变ε_f(ε_e=ε_f)，就可以从方程(5)~方程(8)求得第一层板穿透破坏时的最大整体变形W_o1f

$\begin{array}{l} \varepsilon _{\rm{f}}^2 = \frac{{16}}{3}{\left( {\frac{H}{{2d}}} \right)^4}\left[ {\frac{{{{\left( {2{W_{{\rm{o1f}}}}/H} \right)}^4}}}{{{{\ln }^4}\left( {a/R} \right)}} + \frac{{{{\left( {2{W_{{\rm{o1f}}}}/H} \right)}^3}}}{{{{\ln }^3}\left( {a/R} \right)}} + \frac{{{{\left( {2{W_{{\rm{o1f}}}}/H} \right)}^2}}}{{{{\ln }^2}\left( {a/R} \right)}}} \right] + \\ \;\;\;\;\;\;\;\;\frac{1}{3}{\left\{ {\frac{2}{{\lambda \left[ {0.41H/\left( {2d} \right) + 0.42} \right]}}\left\{ {\frac{{2{W_{{\rm{o1f}}}}/d}}{{\ln \left( {R/a} \right)}} + \frac{1}{{\sqrt 3 }}\left[ {1 + \frac{{\sqrt 3 /2}}{{\ln \left( {R/a} \right)}}} \right]\frac{H}{{2d}}} \right\}} \right\}^{2/n}}\gamma _{\rm{c}}^2 \end{array}$

(9)

将由(9)式求得的不同总厚度H对应的第一层板的最大整体变形W_o1f代入方程(10)、方程(11)即可求得第一层板的整体变形耗能(E_bm1)

${E_{{\rm{bm1}}}} = \int_0^{{W_{{\rm{o1f}}}}} {{F_1}{\rm{d}}S} = \int_0^{{W_{{\rm{o1f}}}}} {\left( {{K_{\rm{m}}}{W_{01}} + {F_{\rm{c}}}} \right){\rm{d}}{W_{{\rm{o1}}}}} + \frac{{{K_{\rm{m}}}}}{2}W_{{\rm{o1f}}}^2 + {F_{\rm{c}}}{W_{{\rm{o1f}}}}$

(10)

和局部剪切耗能(E_s1)

${E_{{\rm{s1}}}} = \int_0^{{\Delta _{\rm{c}}}} {{F_{\rm{s}}}{\rm{d}}\Delta } = \frac{{{F_{\rm{u}}}{\Delta _{\rm{c}}}}}{{n + 1}}{\left( {\frac{{{K_{\rm{m}}}{W_{{\rm{o1f}}}} + {F_{\rm{c}}}}}{{{F_{\rm{u}}}}}} \right)^{\left( {n + 1} \right)/n}}$

(11)

第一层板穿透破坏吸收的总能量E_p1为整体变形耗能E_bm1和局部剪切耗能E_s1之和，即E_p1=E_bm1+E_s1。

1.2 第二层板的能量吸收

在贯穿第一层板后，平头弹前端附着第一层板的塞块撞击第二层板。图 2给出了第二层板组合弹丸(平头弹+塞块)作用下破坏示意图。第一层板的塞块在平头弹和第二层板作用下边缘厚度变薄，剖面近似于四分之一圆，中间部分近似成平面。在组合弹丸(平头弹+塞块)作用下，第二层板的整体变形增大，因薄膜拉伸造成局部厚度变薄。

图 2 第二层板破坏示意图

Figure 2. Schematic of second plate failure

下载: 全尺寸图片幻灯片

如图 2所示，根据实验和数值模拟结果第一层板塞块的厚度约为初始厚度的0.9倍，即H₁≈0.45H。第二层板的初始厚度为H₀=H/2，发生破坏时破坏处的厚度为H₂，由塑性变形体积不变可以有 ${\rm{ \mathsf{ π} }}r_0^2{H_0} = {\rm{ \mathsf{ π} }}r_2^2{H_2}$ ，即 ${r_2}/{r_0} = \sqrt {{H_0}/{H_2}}$ ，根据工程应变和真实应变的关系可得第二层圆板在破坏处的径向真实应变，即 ${\varepsilon _{r1}} = \ln \sqrt {{H_0}/{H_2}} = \left( { - 1/2} \right)\ln \left( {2{H_2}/H} \right)$ 。

根据文献[]，将第二层板破坏处的径向应变近似表示为 ${\varepsilon _{r2}} = \frac{{W_{{\rm{o}}2{\rm{f}}}^2}}{{2r_1^2{{\ln }^2}\left( {{r_1}/R} \right)}} + \frac{{{W_{{\rm{o}}2{\rm{f}}}}H}}{{4r_1^2\ln \left( {{r_1}/R} \right)}}$ ，其中r₁为破坏处距离弹丸中心的距离，根据本模型可得r₁=a－H₁+H₁sin(π/4)，由ε_r1=ε_r2可求得第二层板破坏时的最大整体变形W_o2f与总厚度H及第二层板破坏时最终厚度H₂之间的函数关系

$- \frac{1}{2}\ln \left( {\frac{{2{H_2}}}{H}} \right) = \frac{{W_{{\rm{o2f}}}^2}}{{2r_1^2{{\ln }^2}\left( {{r_1}/R} \right)}} + \frac{{{W_{{\rm{o2f}}}}H}}{{4r_1^2\ln \left( {{r_1}/R} \right)}}$

(12)

而厚度为H/2的单层板在平头弹撞击下的最大整体变形W_of^[8]可以表示为

$\begin{array}{l} \varepsilon _{\rm{f}}^2 = \frac{{16}}{3}{\left( {\frac{H}{{2d}}} \right)^4}\left[ {\frac{{2{{\left( {{W_{{\rm{0f}}}}/H} \right)}^4}}}{{{{\ln }^4}\left( {a/R} \right)}} + \frac{{{{\left( {2{W_{{\rm{0f}}}}/H} \right)}^3}}}{{{{\ln }^3}\left( {a/R} \right)}} + \frac{{{{\left( {2{W_{{\rm{0f}}}}/H} \right)}^2}}}{{{{\ln }^2}\left( {a/R} \right)}}} \right] + \\ \;\;\;\;\;\;\;\frac{1}{3}{\left\{ {\frac{1}{{\lambda \left[ {0.41H/\left( {2d} \right) + 0.42} \right]}}\left\{ {\frac{{2{W_{{\rm{0f}}}}/d}}{{\ln \left( {R/a} \right)}} + \frac{1}{{\sqrt 3 }}\left[ {1 + \frac{{1 + \sqrt 3 /2}}{{\ln \left( {R/a} \right)}}} \right]\frac{H}{{2d}}} \right\}} \right\}^{2/n}}\gamma _{\rm{c}}^2 \end{array}$

(13)

对于不同厚度的双层板，靶板的厚度越小，第一层板的塞块厚度H₁越小，r₁=a－H₁+H₁sin(π/4)的值与平头弹半径a越接近。当靶板厚度趋于零时，有r₁|_H→0=a，此时第二层板与平头弹穿透厚为H/2单层板的速度场和整体变形场相同^[1]，有ε_r2=ε_r，即(W_o2f=W_of)|_H→0，文字表述为当靶板厚度趋于零时第二层板的整体变形和平头弹穿透厚度为H/2的单层靶板的整体变形相同。由于公式较为复杂且包含隐式形式，求解较为复杂，用Matlab软件求解隐式方程组(12)式、(13)式，得到第二层板的最终厚度H₂以及(12)式、(13)式对应的函数关系，(12)式、(13)式的函数图像在H=0处相交。将求得的H₂代入(12)式即可求得双层靶第二层靶板的最大整体变形W_o2f与总厚度H间的关系，进而可求得第二层板的总体变形耗能E_bm2

${E_{{\rm{bm2}}}} = \frac{{{K_{\rm{m}}}}}{2}W_{{\rm{o2f}}}^2 + {F_{\rm{c}}}{W_{{\rm{o2f}}}}$

(14)

和局部拉伸耗能E_t2的近似值

${E_{{\rm{t2}}}} = EV \approx {\sigma _{\rm{y}}}{\varepsilon _{{r_1}}}{\rm{ \mathit{ π} }}r_1^2{H_2}$

(15)

第二层板穿透的能量消耗E_p2=E_bm2+E_t2，则可以得到平头弹穿透双层板所消耗的总能量E_p为

${E_{\rm{p}}} = {E_{{\rm{p1}}}} + {E_{{\rm{p2}}}} = {E_{{\rm{bm1}}}} + {E_{{\rm{s1}}}} + {E_{{\rm{bm2}}}} + {E_{{\rm{t2}}}}$

(16)

1.3 应变率效应

以上得到的是准静态条件下的穿透能量，动态冲击下需要考虑材料的应变率效应。材料的应变率效应可以用Cowper-Symonds经验公式来描述, 即

${\sigma _{\rm{d}}} = {\sigma _{\rm{y}}}\left[ {1 + {{\left( {{{\dot \varepsilon }_{\rm{m}}}/D} \right)}^{1/q}}} \right]$

(17)

式中: σ_d为材料的动态屈服应力，D和q为描述材料应变率的敏感性常数， ${\dot \varepsilon _{\rm{m}}}$ 为靶板的平均应变率。对双层靶而言，第一、二层板的平均应变率^[1]可分别写为

${{\dot \varepsilon }_{{\rm{m1}}}} = \frac{{2{W_{{\rm{o1f}}}}{v_{{\rm{bl}}}}}}{{3\sqrt 2 Ra{{\ln }^2}\left( {a/R} \right)}}$

(18)

${{\dot \varepsilon }_{{\rm{m2}}}} = \frac{{2{W_{{\rm{o2f}}}}{v_{{\rm{bl}}}}}}{{3\sqrt 2 R{r_1}{{\ln }^2}\left( {{r_1}/R} \right)}}$

(19)

式中: v_bl为弹道极限。将能量公式中的静态屈服应力σ_y用动态屈服应力σ_d代替，可得到动态情况下平头弹穿透靶板所消耗的能量 $E_{\rm{p}}^{\rm{d}}$ 。令 $E_{\rm{p}}^{\rm{d}} = Mv_{{\rm{bl}}}^2/2$ ，可以得到平头弹撞击下双层靶的弹道极限, 即

${v_{{\rm{bl}}}} = \sqrt {\frac{{2E_{\rm{p}}^{\rm{d}}}}{M}}$

(20)

式中: M为平头弹质量。

2. 结果与讨论

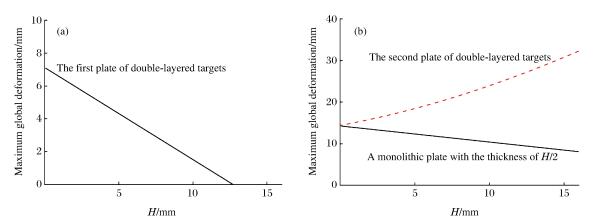
将本研究的理论模型结果与文献中的相关实验结果进行比较和讨论。针对Dey等^[8]做的平头弹撞击等厚接触式双层Weldox700E钢板的实验，模型中的相关参数值见表 1，根据本研究模型可以求得不同厚度的第一层板和第二层板的最大整体变形(见图 3)，并得到第二层板破坏处的最终厚度为H₂≈0.41H。从图 3(a)可以看出，双层靶中的第一层板的总体变形随着总厚度的增加而减少，而第二层板正好相反，其总体变形随着厚度的增加而增加。图 3(b)给出了单层板在平头弹作用下总体变形随厚度的变化情况, 即其总体变形随着厚度的增加而逐步减少。

表 1 Weldox700E钢板相关参数值^{[3, 9, 11]}

Table 1. Parameters for Weldox700E steel plates^{[3, 9, 11]}

σ_y/MPa	σ_u/MPa	ρ_t/(kg·m^-3)	n	γ_c	D/s^－1
859	877	7 850	0.12	1.4	4.6×10⁷

d/mm	M/g	R/mm	a/mm	q	ε_f
20	197	250	10	7.33	1.05

下载: 导出CSV

| 显示表格

图 3 双层Weldox700E钢板的最大整体变形

Figure 3. Maximum global deformation of double-layered plates of Weldox700E

下载: 全尺寸图片幻灯片

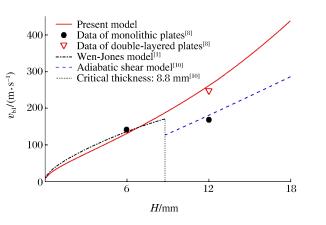
图 4给出了本研究双层板理论模型求得的等厚接触式双层Weldox700E钢板的弹道极限与Dey等的实验结果及单层板的Wen-Jones模型^[1]和绝热剪切模型^[10]的对比。从图 4可以看出, 本研究模型能较好地预测等厚接触式双层板的弹道极限值。且对于单层板，当发生局部化的绝热剪切破坏时，等厚接触式双层板的弹道极限要明显大于单层板的弹道极限；当发生带有整体变形的简单剪切破坏时，等厚接触式双层板和单层板的弹道极限几乎相同。

图 4 本研究理论模型与Weldox700E板实验数据和单层板模型的比较

Figure 4. Comparison of present model with experimental data for Weldoc700E plates and theoretical models for monolithic plates

下载: 全尺寸图片幻灯片

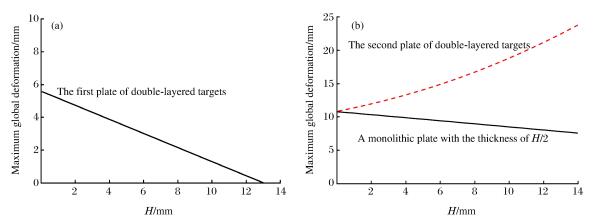
针对张伟等^[6]的平头弹撞击等厚接触式双层Q235钢板的实验，模型中的相关参数值见表 2，用同样的方法可以求得不同厚度的第一层板和第二层板的最大整体变形(见图 5)，根据本研究理论可得第二层板破坏处的最终厚度为H₂≈0.315H。接触式双层Q235钢板中第一层板和第二层板的总体变形规律与接触式双层Weldox700E钢板类似，见图 5。

表 2 Q235钢板相关参数值^{[6, 9, 12]}

Table 2. Parameters for Q235 steel plates^{[6, 9, 12]}

σ_y/MPa	σ_u/MPa	ρ_t/(kg·m^-3)	n	γ_c	D/s^-1
229	335	7 800	0.08	0.8	1 400

d/mm	M/g	R/mm	a/mm	q	ε_f
12.7	34.5	85	6.35	1.5	1.05

下载: 导出CSV

| 显示表格

图 5 双层Q235钢板的最大整体变形

Figure 5. Maximum global deformation of double-layered plates of Q235

下载: 全尺寸图片幻灯片

图 6给出了用本研究能量模型求得的等厚接触式双层Q235钢板的弹道极限与张伟等的实验结果及单层板的Wen-Jones模型^[1]和绝热剪切模型^[10]的对比。从图 4可以发现, 本研究模型与张伟等的等厚接触式双层板的实验弹道极限值吻合得很好。且对于单层板，当发生带有整体变形的简单剪切破坏时，等厚接触式双层板和单层板的弹道极限几乎相同；当发生局部化的绝热剪切破坏时，等厚接触式双层板的弹道极限要明显大于单层板的弹道极限。

图 6 本研究理论模型与Q235钢板实验数据和单层板模型的比较

Figure 6. Comparison of present model with experimental data for Q235 plates and theoretical models for monolithic plates

下载: 全尺寸图片幻灯片

3. 结论

通过分析等厚接触式双层板的破坏模式，基于Wen-Jones模型和应变失效准则得到了接触式双层板穿透的理论模型。结果表明:理论预测与有限的实验数据结果吻合得很好；当总厚度大于单层板绝热剪切冲塞临界厚度值时，双层板的弹道极限明显高于单层板的弹道极限；小于该值时，双层板的弹道极限与单层板的弹道极限差别不大。

图 1 由Weibull分布表征的强度分布直方图

Figure 1. Histogram of the strength distribution characterized by Weibull distribution

下载: 全尺寸图片幻灯片

图 2 动态拉伸承载能力分布

Figure 2. Typical distribution of the dynamic tensile load-carrying capacity

下载: 全尺寸图片幻灯片

图 3 裂纹主向和偏转角

Figure 3. Crack primary direction and the deflection angle

下载: 全尺寸图片幻灯片

图 4 不同局部应变率下的裂纹轮廓线

Figure 4. Profile of the cracks under different local strain-rate

下载: 全尺寸图片幻灯片

图 5 计盒维数法计算泛形复杂度

Figure 5. Box-counting dimension method is used to compute the ubiquitiformal complexity

下载: 全尺寸图片幻灯片

图 6 局部应变率为10³ s^–1的泛形裂纹轮廓线

Figure 6. Profile of ubiquitiform cracks under the local strain rate of 10³ s^–1

下载: 全尺寸图片幻灯片

表 1 复杂度的数值计算结果

Table 1. Numerical results of the complexity C

Strain rate/s⁻¹ C
S1 S2 S3 S4 S5 Avg.

10^–6 1.381 1.382 1.379 1.381 1.383 1.381
10 1.215 1.213 1.210 1.212 1.208 1.212
10² 1.182 1.182 1.180 1.178 1.179 1.180
10³ 1.127 1.132 1.134 1.131 1.142 1.133

下载: 导出CSV

表 2 不同形状参数下的泛形复杂度

Table 2. Ubiquitiform complexity under different shape parameters

m C m C

5 1.142 8 1.086
6 1.126 9 1.057
7 1.108 10 1.033

下载: 导出CSV

参考文献(27)

[1]	MANDELBROT B B, PASSOJA D E, PAULLAY A J. Fractal character of fracture surfaces of metals [J]. Nature, 1984, 308(5961): 721–722. doi: 10.1038/308721a0
[2]	王金安, 谢和平. 岩石断裂表面分形测量的尺度效应 [J]. 岩石力学与工程学报, 2000, 19(1): 11–17. doi: 10.3321/j.issn:1000-6915.2000.01.003 WANG J A, XIE H P. Scale effect on fractal measurement of rock fracture surfaces [J]. Chinese Journal of Rock Mechanics and Engineering, 2000, 19(1): 11–17. doi: 10.3321/j.issn:1000-6915.2000.01.003
[3]	裴建良, 苏立, 刘建锋, 等. 层状大理岩间接拉伸试验及断口形貌和断裂机理分析 [J]. 四川大学学报(工程科学版), 2014, 46(4): 39–45. PEI J L, SU L, LIU J F, et al. Indirect tensile test of layered marble and analysis of fracture morphology and mechanism [J]. Journal of Sichuan University (Engineering Science Edition), 2014, 46(4): 39–45.
[4]	SAGAR R V, PRASAD B K R. Fracture analysis of concrete using singular fractal functions with lattice beam network and confirmation with acoustic emission study [J]. Theoretical and Applied Fracture Mechanics, 2011, 55(3): 192–205. doi: 10.1016/j.tafmec.2011.07.003
[5]	党发宁, 方建银, 丁卫华. 基于CT的混凝土试样静动力单轴拉伸破坏裂纹分形特征比较研究 [J]. 岩石力学与工程学报, 2015, 34(Suppl 1): 2922–2928. DANG F N, FANG J Y, DING W H. Fractal comparison research of fracture of concrete samples under static and dynamic uniaxial tensile using CT [J]. Chinese Journal of Rock Mechanics and Engineering, 2015, 34(Suppl 1): 2922–2928.
[6]	LIANG H, PAN F S, CHEN Y M, et al. Influence of the strain rates on tensile properties and fracture interfaces for Mg-Al alloys containing Y [J]. Advanced Materials Research, 2011, 284/286: 1671–1677. doi: 10.4028/www.scientific.net/AMR.284-286.1671
[7]	OU Z C, LI G Y, DUAN Z P, et al. Ubiquitiform in applied mechanics [J]. Journal of Theoretical and Applied Mechanics, 2014, 52: 37–46.
[8]	CARPINTERI A. Fractal nature of material microstructure and size effects on apparent mechanical properties [J]. Mechanics of Materials, 1994, 18(2): 89–101. doi: 10.1016/0167-6636(94)00008-5
[9]	CARPINTERI A, PUZZI S. Self-similarity in concrete fracture: size-scale effects and transition between different collapse mechanisms [J]. International Journal of Fracture, 2008, 154(1/2): 167–175.
[10]	BORODICH F M. Some fractal models of fracture [J]. Journal of the Mechanics and Physics of Solids, 1997, 45(2): 239–259. doi: 10.1016/S0022-5096(96)00080-4
[11]	LI G Y, OU Z C, XIE R, et al. A ubiquitiformal one-dimensional steady-state conduction model for a cellular material rod [J]. International Journal of Thermophysics, 2016, 37(4): 1–13.
[12]	OU Z C, YANG M, LI G Y, et al. Ubiquitiformal fracture energy [J]. Journal of Theoretical and Applied Mechanics, 2017, 55(3): 1101–1108.
[13]	LI J Y, OU Z C, TONG Y, et al. A statistical model for ubiquitiformal crack extension in quasi-brittle materials [J]. Acta Mechanica, 2017, 228(7): 1–8.
[14]	WEIBULL W. A statistical distribution of wide applicability [J]. Journal of Applied Mechanics, 1951, 18(2): 293–297.
[15]	OU Z C, DUAN Z P, HUANG F L. Analytical approach to the strain rate effect on the dynamic tensile strength of brittle materials [J]. International Journal of Impact Engineering, 2010, 37(8): 942–945. doi: 10.1016/j.ijimpeng.2010.02.003
[16]	WANG Y D, DAN W J, XU Y F, et al. Fractal and morphological characteristics of single marble particle crushing in uniaxial compression tests [J]. Advances in Materials Science and Engineering, 2015, 2015(1): 1–10.
[17]	ISA K, ABU A R K, SOUSA R L. Computational modelling of fracture propagation in rocks using a coupled elastic-plasticity-damage model [J]. Mathematical Problems in Engineering, 2016, 2016: 3231092.
[18]	GRANGE S, FORQUIN P, MENCACCI S, et al. On the dynamic fragmentation of two limestones using edge-on impact tests [J]. International Journal of Impact Engineering, 2008, 35(9): 977–991. doi: 10.1016/j.ijimpeng.2007.07.006
[19]	ZHU W C, TANG C A. Numerical simulation on shear fracture process of concrete using mesoscopic mechanical model [J]. Construction and Building Materials, 2002, 16(8): 453–463. doi: 10.1016/S0950-0618(02)00096-X
[20]	PETROV Y, KAZARINOV N, BRATOV V. Dynamic crack propagation: quasistatic and impact loading [J]. Procedia Structural Integrity, 2016, 2: 389–394. doi: 10.1016/j.prostr.2016.06.050
[21]	LIU L G, OU Z C, DUAN Z P, et al. Strain-rate effects on deflection/penetration of crack terminating perpendicular to bimaterial interface under dynamic loadings [J]. International Journal of Fracture, 2011, 167(2): 135–145. doi: 10.1007/s10704-010-9533-2
[22]	PEI C W, YAO Y, CHEN D G, et al. Experimental study of the tensile bond strength in concrete aggregate-paste interfacial transition zone [J]. Applied Mechanics and Materials, 2012, 193/194: 1384–1388. doi: 10.4028/www.scientific.net/AMM.193-194.1384
[23]	WONG T F, WONG R H C, CHAU K T, et al. Microcrack statistics, Weibull distribution and micromechanical modeling of compressive failure in rock [J]. Mechanics of Materials, 2006, 38(7): 664–681. doi: 10.1016/j.mechmat.2005.12.002
[24]	SMIRNOV I, KONSTANTINOV A, BRAGOV A, et al. The structural temporal approach to dynamic and quasi-static strength of rocks and concrete [J]. Procedia Structural Integrity, 2017, 6: 34–39. doi: 10.1016/j.prostr.2017.11.006
[25]	谢和平, 高峰, 周宏伟, 等. 岩石断裂和破碎的分形研究 [J]. 防灾减灾工程学报, 2003, 23(4): 1–9. XIE H P, GAO F, ZHOU H W, et al. Fractal fracture and fragmentation in rocks [J]. Journal of Disaster Prevention and Mitigation Engineering, 2003, 23(4): 1–9.
[26]	SAKELLARIOU M. On the fractal character of rock surfaces [J]. International Journal of Rock Mechanics and Mining Science & Geomechanics Abstracts, 1991, 28(6): 527–533.
[27]	YAN D, LIN G. Dynamic properties of concrete in direct tension [J]. Cement and Concrete Research, 2006, 36(7): 1371–1378. doi: 10.1016/j.cemconres.2006.03.003

施引文献

资源附件(0)

访问统计

点击查看大图

图(6) / 表(2)

计量

文章访问数: 8684
HTML全文浏览量: 3084
PDF下载量: 31

1. 等厚接触式双层板穿透的理论模型
1.1 第一层板的能量吸收
1.2 第二层板的能量吸收
1.3 应变率效应
2. 结果与讨论
3. 结论

Strain rate/s⁻¹	C
Strain rate/s⁻¹	S1	S2	S3	S4	S5	Avg.
10^–6	1.381	1.382	1.379	1.381	1.383	1.381
10	1.215	1.213	1.210	1.212	1.208	1.212
10²	1.182	1.182	1.180	1.178	1.179	1.180
10³	1.127	1.132	1.134	1.131	1.142	1.133

m	C	m	C
5	1.142	8	1.086
6	1.126	9	1.057
7	1.108	10	1.033

动载下准脆性材料的泛形裂纹研究

doi: 10.11858/gywlxb.20190754

作者简介: 明德涵（1994－），男，硕士研究生，主要从事材料与结构冲击动力学研究. E-mail: bitmdh@163.com

通讯作者: 欧卓成（1961－），男，博士，教授，主要从事材料与结构冲击动力学研究. E-mail: zcou@bit.edu.cn

计量

出版历程