New Developments of Time-Dependent Density Functional Theory and Its Applications
-
摘要: 密度泛函理论在材料计算研究领域得到了广泛的应用,然而它无法处理含时问题和材料的激发态性质。Runge-Gross定理奠定了含时密度泛函理论的基础,为研究这两类问题提供了有效的手段。经过三十多年的发展,含时密度泛函已被应用到量子化学、材料计算等多个领域,人们也更加了解其优势和不足。目前,含时密度泛函理论和方法仍在迅速发展。本文简要回顾含时密度泛函方法的发展历史,介绍近年来含时密度泛函在理论和应用方面的一些重要进展,总结当前在含时密度泛函领域存在的重要难题以及面临的挑战,展望其发展方向和趋势。Abstract: Nowadays density functional theory which was introduced in the mid-1960s has wide applications in material simulations. However, it is not able to deal with time-dependent problems and excited properties of materials. Time-dependent density functional theory (TDDFT) based on Runge-Gross theorem, provides a viable way to deal with these two problems. After thirty years’ development, TDDFT has been widely applied to many fields, such as quantum chemistry and material simulation, and our understanding of its advantages and weaknesses also grows. To date, TDDFT theory and method still develop rapidly. Here a brief historical review of TDDFT is first introduced. Then it is followed by a discussion of recent important developments on theory and applications of TDDFT. Finally we summarize some important problems and challenges that TDDFT are facing and attempt to offer some thoughts about where TDDFT will be progressing.
-
海床已成为一个全新的冲突领域,近海底爆炸可以对海底光缆、海底管道等设施造成严重的破坏,其破坏过程涉及近海底反射、多相流掺混、结构与流体的耦合作用,开展近海底爆炸冲击波时空演化规律研究对于水中兵器研制及海底设施抗爆抗冲击设计具有重要的意义。
目前,国内外对近海底水下爆炸研究已经取得了一定成果。基于Kelvin的冲击理论,Blake等[1]得出了判断自由面附近气泡射流方向和气泡运动方向的方法,该方法对近壁面也同样适用。张永坤[2]对沉底水雷在水下爆炸作用下的毁伤情况进行了研究,总结了水雷在不同情况下被毁伤的特点,为提高水下灭雷武器的作战能力奠定了理论基础。邵建军等[3]基于相似理论,研究了海底对爆炸的影响,发现海底底质变化显著影响水下爆炸的冲击波能和气泡能。杨莉等[4–7]在泥底、砂底及石底3种条件下进行了沉底爆炸试验,发现在3种底质条件下,气泡后期溃灭形态存在差异,最大峰值压力通常出现在装药靠近水底面一侧的斜上方,对于爆距较小的水底面测点,反射波追上入射波会形成马赫波。黄潇等[8]采用镜像法模拟海底边界的影响,发现自由场爆炸气泡比近海底爆炸气泡对潜艇施加的总纵弯矩更大。姚熊亮等[9]基于光滑粒子流体动力学(smooth particle hydrodynamics,SPH)方法模拟沉底水下爆炸,研究了水底底质厚度和炸药当量对冲击波压力的影响。邵宗战等[10]提出了沉底水雷海上爆炸威力的测量方法,给出了冲击波峰值压力的拟合方法及爆炸能量计算方法。前人在近海底水下爆炸冲击波载荷分析方面已取得了一些成果,然而,针对不同底质条件下近海底水下爆炸冲击波载荷时空分布规律的定量研究尚不充分。
本研究拟采用耦合欧拉-拉格朗日(coupled Eulerian-Lagrangian,CEL)方法建立近海底水下爆炸模型,探讨不同底质条件下近海底爆炸冲击波的时空分布规律,以期为近海底设施的抗爆抗冲击结构设计提供支撑。
1. 数值模型与数值验证
1.1 状态方程
本研究采用TNT炸药,爆轰产物通过JWL方程[11]描述
p=A(1−ωR1v)e−R1v+B(1−ωR2v)e−R2v+ωev (1) 式中:A、B、R1、R2及
ω 为与炸药状态有关的常数,v为爆轰产物的相对比容,e为炸药单位质量所蕴含的内能。TNT炸药的材料参数及JWL状态方程参数如表1[12]所示,其中:ρ为密度,D为爆速。水采用Mie-Grüneisen状态方程描述,表达式[11]为
p=pH(1−Γ0η/2)+Γ0ρ0Em (2) 式中:pH为Hugoniot压力;ρ0为初始密度;Em为单位质量内能;Γ0为Grüneisen常数;η为名义体积压缩应变,
η=1−ρ0/ρ 。pH的表达式[11]为pH=ρ0C20η(1−Sη)2 (3) us=C0+Sup (4) 式中:us为冲击速度,up为粒子速度,S和C0为us-up曲线参数。水的状态方程参数列于表2[12]。
在数值模型中,设空气为理想气体,其状态方程[11]为
p+pa=(γ−1)ρEm (5) Em=cV(θ−θz) (6) 式中:γ为绝热指数,pa为外界压力,cV为比定容热容,
θ 为当前温度,θz 为绝对零度。空气的状态方程参数如表3[12]所示。1.2 数值模型
为研究近海底水下爆炸冲击波的传播规律,建立了数值模型,欧拉域尺寸为15.0 m×6.5 m×15.0 m,模拟50 kg TNT在50 m水深处的近海底爆炸,TNT底部距海底0.05 m。将整个欧拉域的边界条件设置为流出无反射,海底底质采用模型1或模型2描述。模型1是一种较软的土壤模型,参考了Ambrosini等[13]和Luccioni等[14]的研究结果,具体参数见表4[13–14],其中:E为杨氏模量,ν为泊松比,φ为内摩擦角,c为黏聚力。模型2为刚性固壁,通过在欧拉域中的海底底质区域放置拉格朗日刚体实现。
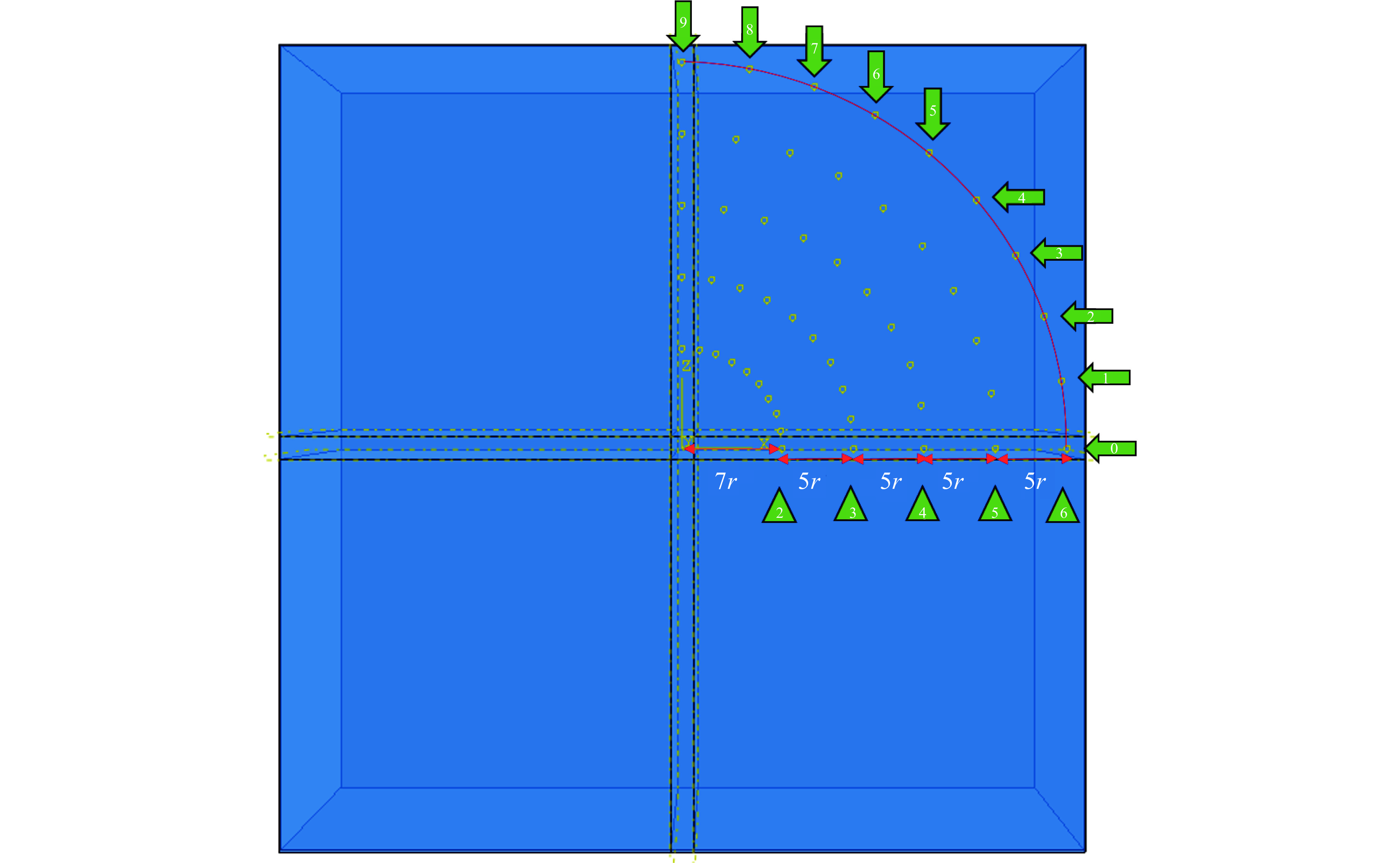
为了更准确地获得同一位置的冲击波压力,在模型的不同位置设置测点,测点布局如图1所示。设装药半径为r,测点到爆心的距离为R,测点与水平方向的夹角为测点角度α。在距离爆心分别为7r、12r、17r、22r、27r,测点角度分别为0°、10°、20°、30°、40°、50°、60°、70°、80°、90°处布置测点,共计50个测点。为方便后续分析,用测点距爆心的距离(爆距)以及测点角度命名测点,爆距7r、12r、17r、22r、27r用数字2~6表示,测点角度0°~90°用数字0~9表示,例如:将爆距为7r、测点角度为0°的测点命名为2-0。
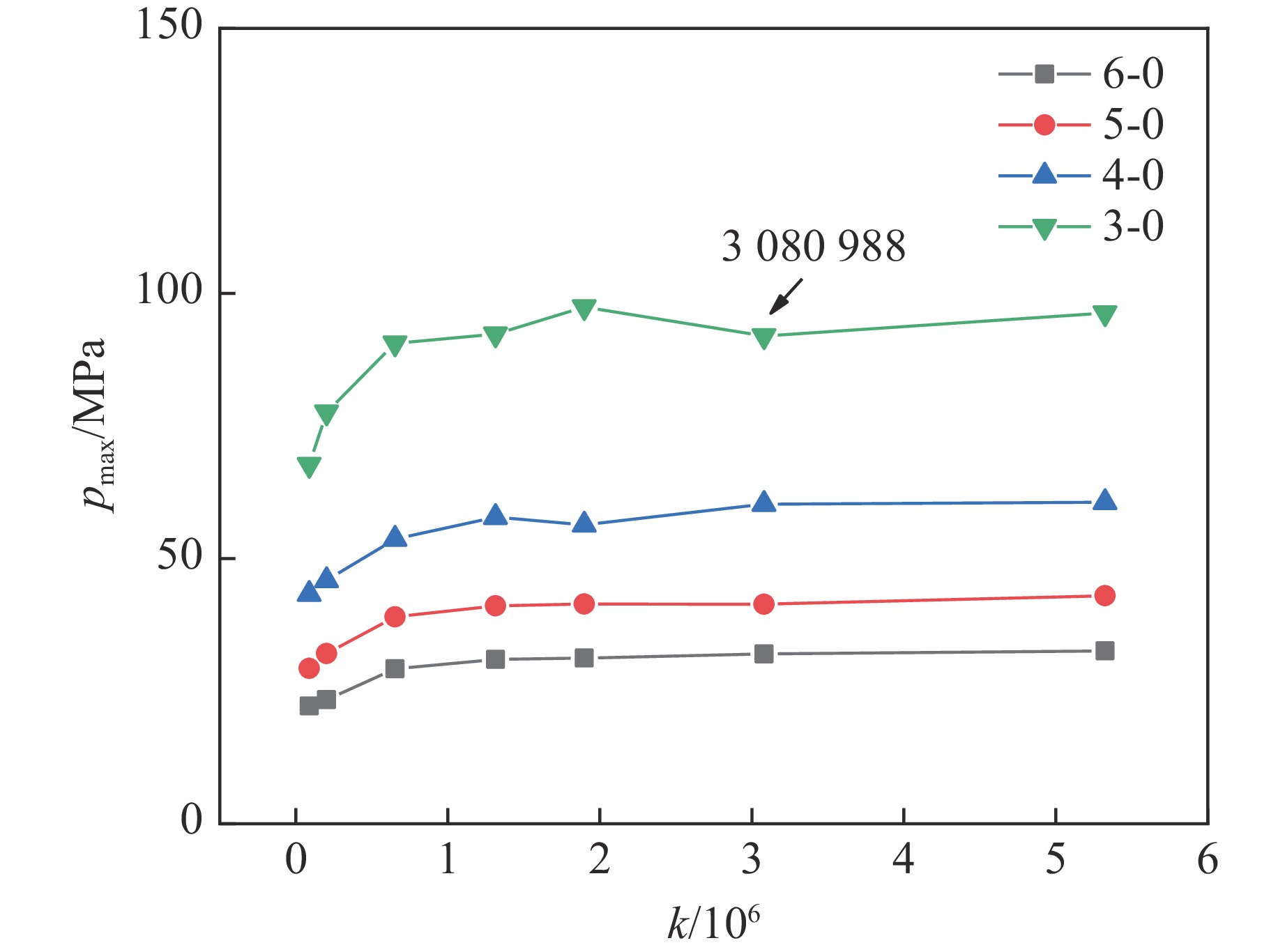
为验证结果的可靠性,对测点3-0、4-0、5-0、6-0测得的冲击波峰值压力进行收敛性分析,得到冲击波峰值压力pmax随网格数量k的变化曲线,如图2所示。当网格数量为3080988时,测点测得的冲击波峰值压力基本收敛。为此,选取网格数量为5324000来研究近海底水下爆炸冲击波的载荷特性。
1.3 数值验证
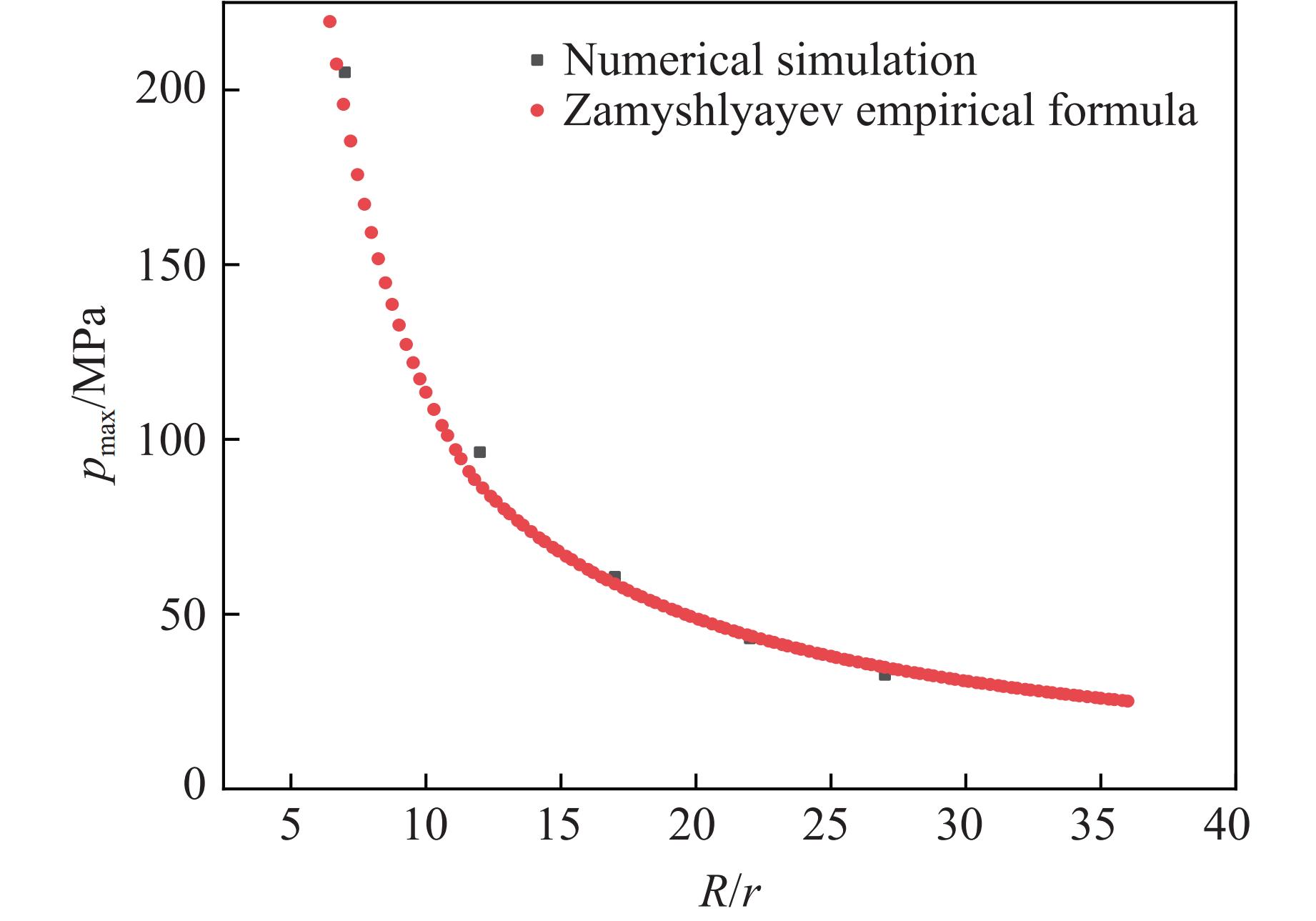
为验证模型的准确性,选取测点2-0、3-0、4-0、5-0、6-0在无海底底质时测得的峰值压力与Zamyshlyayev经验公式计算结果进行对比,如图3和表5所示。可以看出,数值模拟结果与经验公式拟合结果符合得较好,说明该模型能够用于近海底水下爆炸冲击波研究。
表 5 数值模拟与经验公式结果对比Table 5. Comparison of numerical simulation and empirical formula resultsR/r Peak pressure/MPa Error/% Simulation Empirical formula 7 205.03 193.72 5.84 12 96.32 86.90 10.84 17 60.65 58.62 3.45 22 43.04 43.81 1.75 27 32.58 37.91 14.07 1.4 工况设置
为研究水深(H)及海底底质对近海底水下爆炸冲击波载荷特性的影响,仅改变海底底质及静水压力,其他条件保持不变,进行数值模拟,计算工况如表6所示,其中:工况1和工况2用于研究自由场与近海底冲击波压力的差异,工况2和工况3用于研究底质条件对近海底爆炸冲击波载荷特性的影响,工况2、工况4和工况5用于研究水深对近海底水下爆炸冲击波载荷特性的影响。
表 6 数值模拟工况设置Table 6. Settings of simulation casesCase H/m Seafloor sediment Explosive environment 1 50 Nothing Free field 2 50 Model 1 Near the seabed 3 50 Model 2 Near the seabed 4 100 Model 1 Near the seabed 5 150 Model 1 Near the seabed 2. 结果讨论与分析
2.1 自由场和近海底爆炸冲击波特性对比
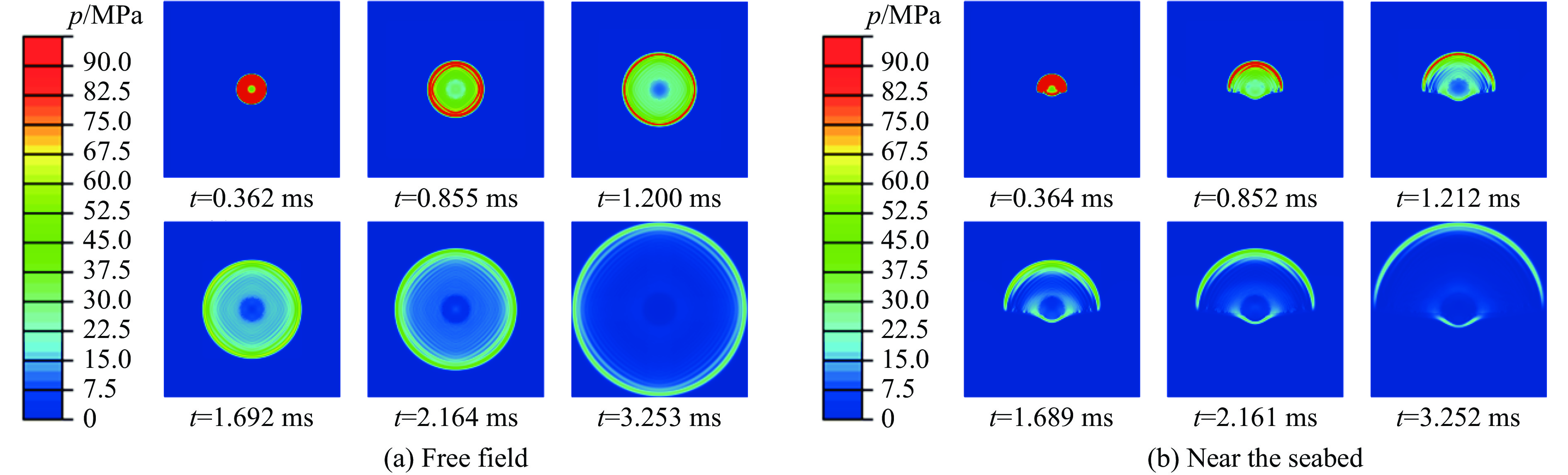
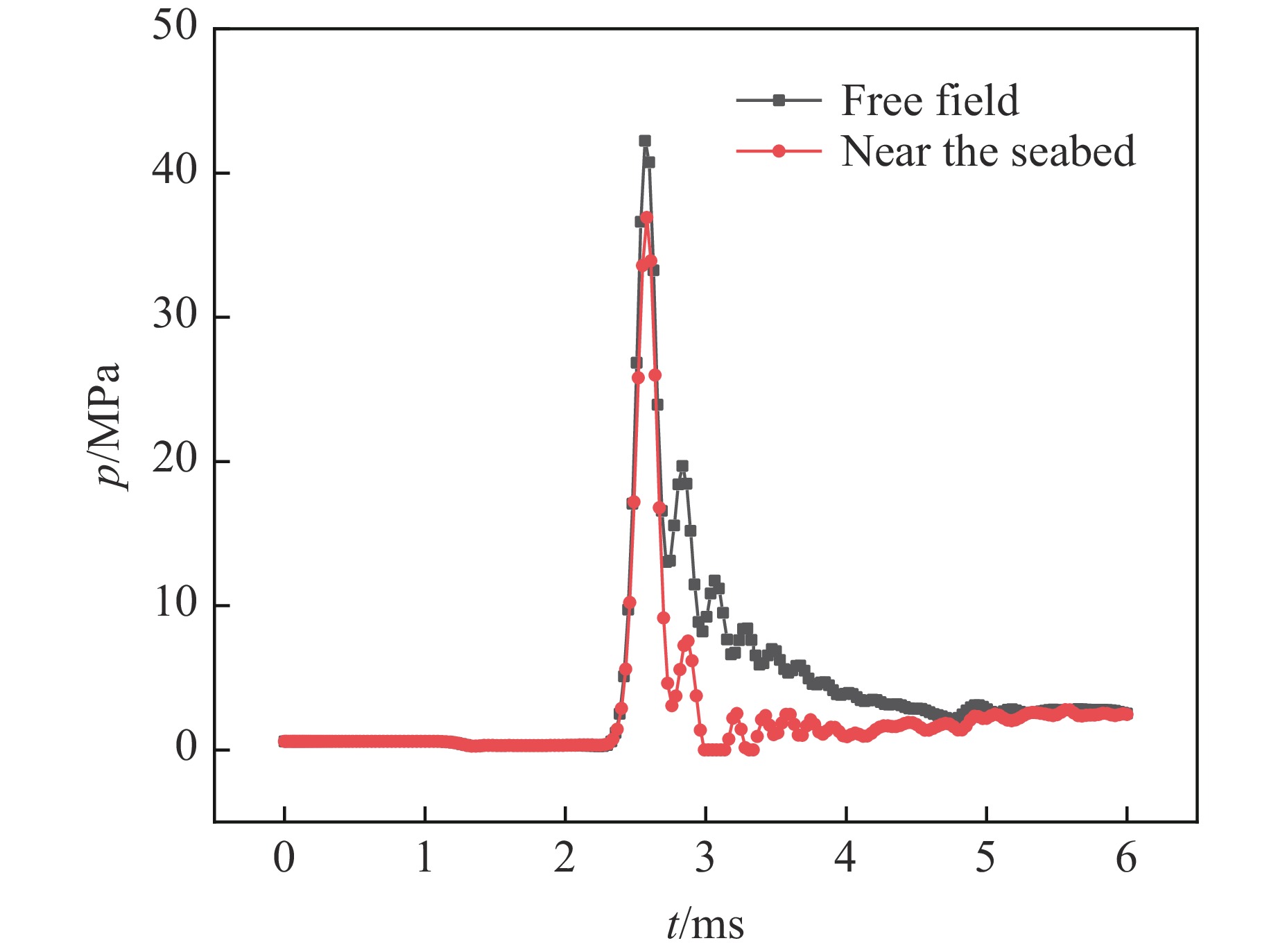
图4为工况1和工况2下不同时刻的冲击波压力云图,图5为测点5-3在工况1和工况2下测得的爆炸冲击波压力时程曲线。可以看出,自由场水下爆炸与近海底水下爆炸存在明显差别,受近海底条件的影响,近海底水下爆炸冲击波形状不是规则的圆形,冲击波波面上的压力峰值并非处处相等,而是越靠近海底区域,冲击波压力越小,测点5-3在近海底条件下测得的冲击波峰值压力及后续的脉动压力均小于自由场条件下测得的压力。由于水的冲击阻抗大于海底底质模型1的冲击阻抗,因此,水中冲击波在近海底的反射波为稀疏波,稀疏波追上冲击波波面将会导致冲击波压力降低;另外,海底底质模型1是一种较软且易变形的底质,冲击波作用在海底底质上,不仅会发生反射,还会发生透射,部分能量传递到海底底质中,使海底底质发生形变,冲击波能量也会发生损耗。因此,在近海底条件下,冲击波峰值压力和气泡脉动压力均小于自由场下的压力,理论与数值模拟结果相符。
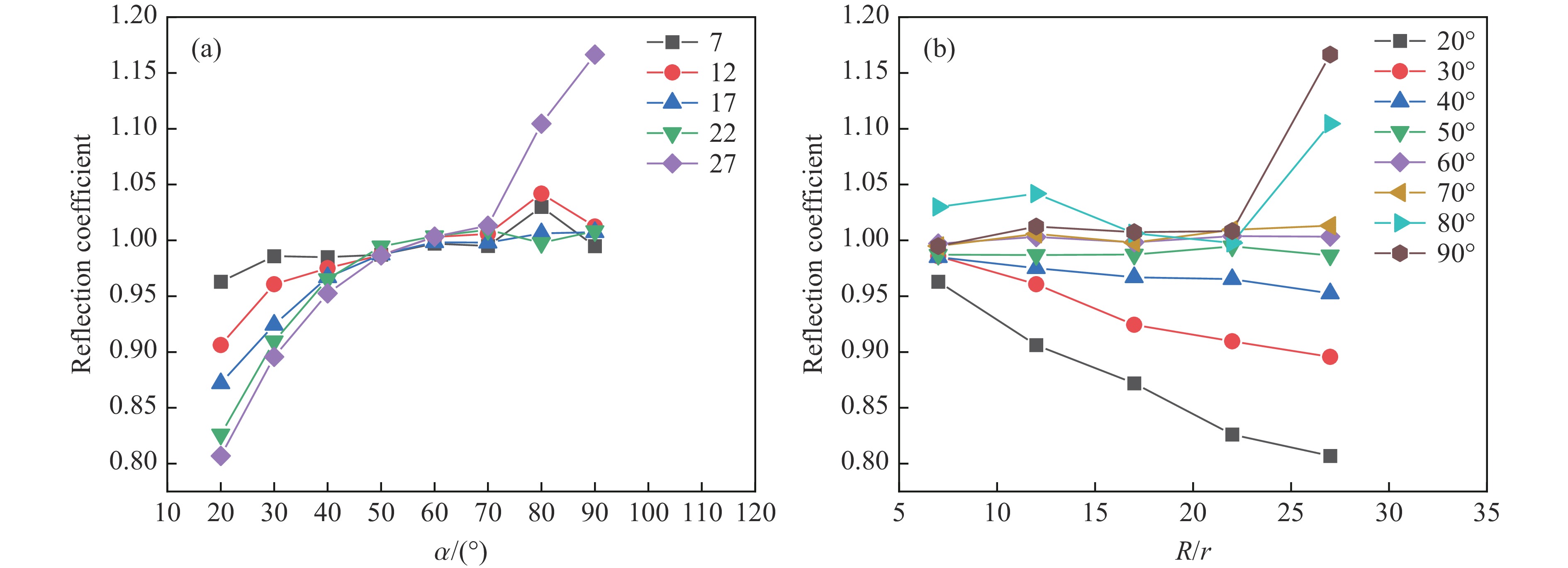
近海底水下爆炸时,靠近海底区域处的冲击波压力明显偏小,即不同测点角度(α)条件下的冲击波峰值压力存在差异。定义测点测得的冲击波峰值压力与自由场时的冲击波峰值压力之比为该测点的反射系数。计算所有测点的反射系数,结果如图6所示。当爆距比一定时,随着α的增大,反射系数也逐渐增大,即海底吸能现象和稀疏波的影响逐渐减小;α在0°~10°区间时,海底的吸能现象过于剧烈,故不对该角度范围进行深入研究;当α处于20°~30°区间时,测点的反射系数随爆距比增加呈减小趋势,即近海底反射的影响随爆距比的增大而增强;当α > 40°时,海底吸能现象不明显。
为了更细致地研究近海底反射规律,将自由场与近海底工况下测点2-2~测点2-9、测点3-2~测点3-9、测点4-2~测点4-9的冲击波峰值压力进行对比,结果如表7所示,可以看出:当α处于20°~30°时,近海底工况下,冲击波峰值压力是自由场工况下冲击波峰值压力的81%~91%;随着α的增大,测点距海底越来越远,近海底反射现象也越来越弱,近海底反射影响逐渐消失。
表 7 自由场与近海底测得的冲击波峰值压力对比Table 7. Comparison of peak pressures of free-field and near-seabed underwater explosion shock waveR/r α/(°) pmax/MPa Reflection
coefficientR/r α/(°) pmax/MPa Reflection
coefficientNear seabed Free field Near seabed Free field 7 20 179.29 201.44 0.89 12 60 92.73 95.67 0.97 7 30 186.21 203.80 0.91 12 70 95.00 97.86 0.97 7 40 193.43 204.80 0.94 12 80 98.33 93.98 1.05 7 50 194.98 204.80 0.95 12 90 92.42 96.30 0.96 7 60 192.78 203.80 0.95 17 20 47.78 58.76 0.81 7 70 195.10 201.43 0.97 17 30 53.24 59.25 0.90 7 80 202.46 201.74 1.00 17 40 56.52 58.58 0.96 7 90 196.41 195.79 1.00 17 50 58.40 58.58 1.00 12 20 82.82 97.87 0.85 17 60 59.44 59.27 1.00 12 30 86.62 95.67 0.91 17 70 61.07 60.10 1.02 12 40 93.96 94.66 0.99 17 80 60.00 61.17 0.98 12 50 96.23 94.65 1.02 17 90 57.77 59.91 0.96 2.2 海底底质对近海底爆炸冲击波载荷特性的影响
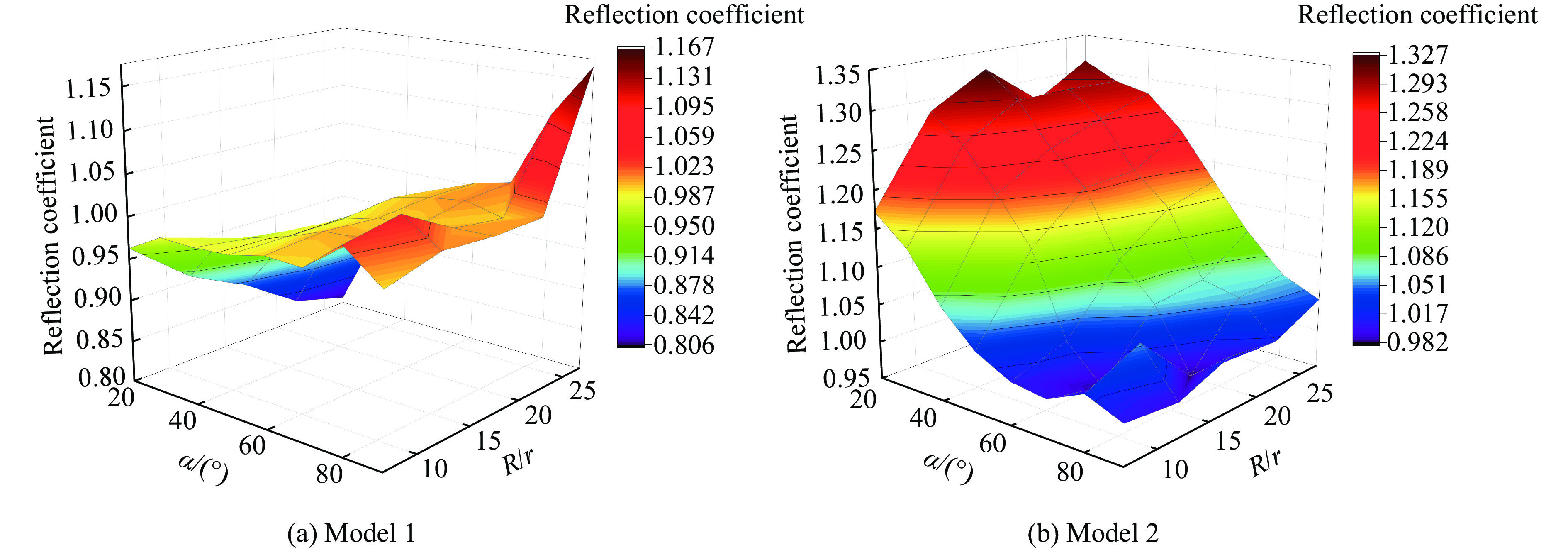
图7显示了工况2和工况3下相同测点测得的近海底冲击波反射系数对比。可以发现,2种底质条件下冲击波传播规律存在明显差异:当底质为模型1时,由于近海底反射波为稀疏波,稀疏波追上冲击波波面将导致冲击波峰值压力变小,且海底底质模型1较软,吸能作用较强,因此,绝大部分测点的反射系数小于1;当海底底质为模型2时,受刚固边界的影响,近海底反射波为压缩波,压缩波追上冲击波波面会导致冲击波峰值压力增大,且海底底质模型2的吸能作用较弱,绝大部分测点的反射系数大于1。无论底质是模型1还是模型2,反射系数异常均发生在爆距比为7~15、α在60°~90°范围内。
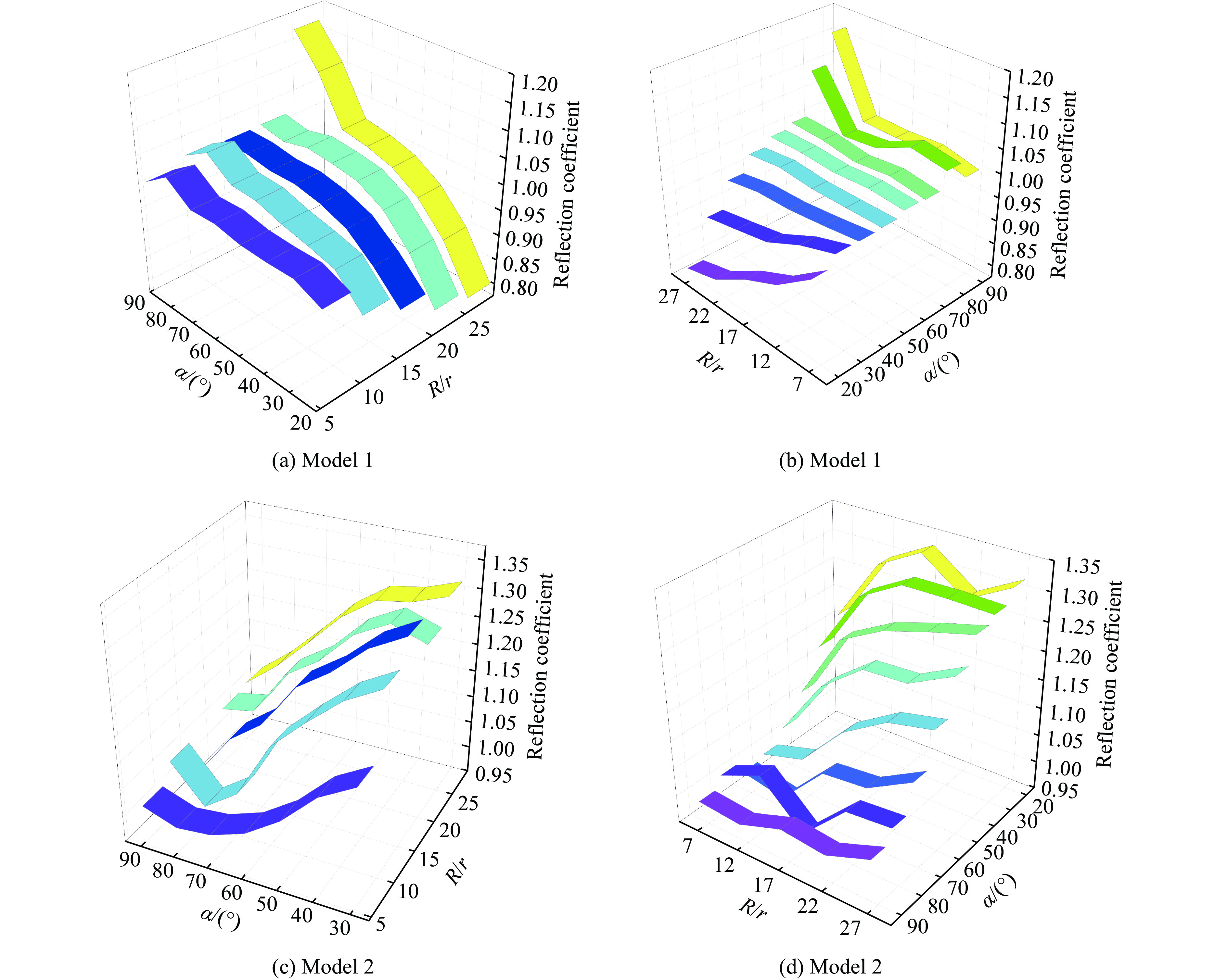
为更清楚地研究不同底质条件对反射系数的影响,将不同底质条件下反射系数随测点角度和爆距比的变化绘制成图8。由图8(a)可知,当海底底质为模型1、爆距比保持不变时,反射系数随测点角度的增大呈增大趋势。由图8(c)可知,当海底底质为模型2、爆距比一定时,反射系数随测点角度的增大呈减小趋势。
当海底底质为模型1时,海底底质较软,海底反射稀疏波,对TNT爆炸冲击波的吸收较强,因此,近海底冲击波峰值压力减小,反射系数小于1是常态。图8(a)显示,当爆距比一定时,测点角度越大,反射系数就越大,因而可以推断,海底底质对不同测点角度处反射系数的影响随测点角度的增大而减弱。当海底底质为模型2时,海底底质较硬,海底反射压缩波,对冲击波的吸收很弱,因此,近海底冲击波峰值压力更大,反射系数大于1是常态。图8(c)显示,当爆距比一定时,测点角度越大,反射系数越小,同样可以得到海底底质对不同测点角度处反射系数的影响随测点角度增大而减弱的结论。
从图8(b)可以看出,当测点角度处于20°~40°区间时,不同测点测得的近海底冲击波反射系数随着爆距比的增大而减小;而图8(d)显示,当测点角度处于20°~60°区间时,不同测点测得的近海底冲击波反射系数随着爆距比的增大而增大。由于2种底质对近海底冲击波反射系数的影响截然相反,因此,综合图8(b)和图8(d)可以看出,测点角度在一定范围内时,海底底质对反射系数的影响随着测点爆距比的增大而增强。当测点角度超出该范围时,该现象减弱甚至消失。综合图8(a)~图8(d)可以发现,虽然海底底质发生了变化,不同海底底质对冲击波的影响效果不同,但是测点反射系数受显著影响的区域均集中在20°~50°范围。
为研究近海底水下爆炸反射波对冲击波的影响是否与起爆深度有关,将底质条件为模型1时不同水深处各测点的反射系数进行对比,结果如图9所示。从图9可以看出,随着水深的增加,同一测点处的反射系数基本一致,说明水深并不能显著影响反射系数,即水深的变化并不会对近海底反射现象造成显著影响,也不会对海底底质的吸能作用造成显著影响,近海底反射稀疏波对冲击波的削减作用并不会随着静水压力的变化而发生显著变化。
3. 结 论
基于CEL方法建立了近海底水下爆炸数值模型,对近海底爆炸冲击波的时空分布规律进行了研究,探究了海底底质和水深对近海底爆炸冲击波载荷特性的影响规律,得到如下结论。
(1) 近海底水下爆炸冲击波载荷特性与自由场明显不同。当测点角度为20°~30°时,近海底反射系数为0.81~0.91;随着测点角度进一步增大,近海底反射的影响逐渐减弱;当测点角度达到80°~90°时,近海底反射的影响基本消失。
(2) 在一定的角度范围内,近海底反射的影响随着爆距比的增大而增强,超出该角度范围后,该现象基本消失。改变底质时,近海底反射的影响使冲击波峰值压力增强或减弱,并且影响区域基本一致。水深对近海底水下爆炸反射系数无显著影响。
(3) 海底底质材料属性不同时,其对爆炸冲击波的吸收作用也存在差异,近海底水下爆炸反射波的种类也不一致。当海底底质较硬时,海底底质对冲击波的吸收作用相对较弱,反射波为压缩波,致使测点角度在20°~50°范围内的冲击波峰值压力增大,当测点角度在20°~50°范围内且测点角度一定时,反射系数随爆距比的增大而增大;当海底底质较软时,海底底质对冲击波的吸收作用较强,近海底反射波为稀疏波,致使20°~50°角度范围内冲击波峰值压力减小,当测点角度在20°~50°范围内且测点角度一定时,反射系数随爆距比的增大而减小。海底底质对反射系数的影响区域主要集中在20°~50°测点范围内,超出该范围时,海底底质对反射系数的影响随着测点角度的增大而逐渐消失,海底底质对反射系数的影响随爆距比的增大而增强的现象也随测点角度的增大而逐渐消失。
-
表 1 一些气相Ar-TCNE体系的激发能E(单位eV)和振动强度f的计算和实验对比[57]
Table 1. Excitation energies E (eV) and oscillator strengths f of several gas phase Ar-TCNE systems: theory and experiment[57]
Ar B3LYP BNL (γ=0.5) BNL γ∗ Exp.[27] E f E γ∗ E f E f Benzene 2.1 0.03 4.4 0.33 3.8 0.03 3.59 0.02 Toluene 1.8 0.04 4.0 0.32 3.4 0.03 3.36 0.03 O-xylene 1.5 0 3.7 0.31 3.0 0.01 3.15 0.05 Naphthalene 0.9 0 3.3 0.32 2.7 0 2.60 0.01 -
[1] DIRAC P A M. Quantum mechanics of many-electron systems [J]. Proceedings of the Royal Society of London Series A, 1929, 123(792): 714–733. doi: 10.1098/rspa.1929.0094 [2] HOHENBERG P, KOHN W. Inhomogeneous electron gas [J]. Physical Review, 1964, 136(3B): B864–B871. doi: 10.1103/PhysRev.136.B864 [3] KOHN W, SHAM L J. Self-consistent equations including exchange and correlation effects [J]. Physical Review, 1965, 140(4A): A1133–A1138. doi: 10.1103/PhysRev.140.A1133 [4] BLOCH F. Bremsvermögen von Atomen mit mehreren Elektronen [J]. Zeitschrift für Physik A Hadrons and Nuclei, 1933, 81(5): 363–376. [5] PEUCKERT V. A new approximation method for electron systems [J]. Journal of Physics C: Solid State Physics, 1978, 11(24): 4945–4956. doi: 10.1088/0022-3719/11/24/023 [6] ZANGWILL A, SOVEN P. Density-functional approach to local-field effects in finite systems: photoabsorption in the rare gases [J]. Physical Review A, 1980, 21(5): 1561. doi: 10.1103/PhysRevA.21.1561 [7] DEB B M, GHOSH S K. Schrödinger fluid dynamics of many-electron systems in a time-dependent density-functional framework [J]. The Journal of Chemical Physics, 1982, 77(1): 342–348. doi: 10.1063/1.443611 [8] GHOSH S K, DEB B M. Dynamic polarizability of many-electron systems within a time-dependent density-functional theory [J]. Chemical Physics, 1982, 71(2): 295–306. doi: 10.1016/0301-0104(82)87030-4 [9] GHOSH S K, DEB B M. A density-functional calculation of dynamic dipole polarizabilities of noble gas atoms [J]. Theoretica Chimica Acta, 1983, 62(3): 209–217. doi: 10.1007/BF00548835 [10] GHOSH S K, DEB B M. A simple density-functional calculation of frequency-dependent multipole polarizabilities of noble gas atoms [J]. Journal of Molecular Structure: THEOCHEM, 1983, 103: 163–176. doi: 10.1016/0166-1280(83)85017-9 [11] BARTOLOTTI L J. Time-dependent extension of the Hohenberg-Kohn-Levy energy-density functional [J]. Physical Review A, 1981, 24(4): 1661–1667. doi: 10.1103/PhysRevA.24.1661 [12] BARTOLOTTI L J. Time-dependent Kohn-Sham density-functional theory [J]. Physical Review A, 1982, 26(4): 2243–2244. doi: 10.1103/PhysRevA.26.2243 [13] BARTOLOTTI L J. Variation-perturbation theory within a time-dependent Kohn-Sham formalism: an application to the determination of multipole polarizabilities, spectral sums, and dispersion coefficients [J]. The Journal of Chemical Physics, 1984, 80(11): 5687–5695. doi: 10.1063/1.446637 [14] BARTOLOTTI L J. Velocity form of the Kohn-Sham frequency-dependent polarizability equations [J]. Physical Review A, 1987, 36(9): 4492–4493. doi: 10.1103/PhysRevA.36.4492 [15] RUNGE E, GROSS E K U. Density-functional theory for time-dependent systems [J]. Physical Review Letters, 1984, 52(12): 997–1000. doi: 10.1103/PhysRevLett.52.997 [16] LEVY M. Universal variational functionals of electron densities, first-order density matrices, and natural spin-orbitals and solution of the v-representability problem [J]. Proceedings of the National Academy of Sciences, 1979, 76(12): 6062–6065. doi: 10.1073/pnas.76.12.6062 [17] LIEB E H. Density functionals for Coulomb systems [J]. International Journal of Quantum Chemistry, 1983, 24: 243–277. doi: 10.1002/(ISSN)1097-461X [18] VAN LEEUWEN R. Mapping from densities to potentials in time-dependent density-functional theory [J]. Physical Review Letters, 1999, 82(19): 3863–3866. doi: 10.1103/PhysRevLett.82.3863 [19] RUGGENTHALER M, GIESBERTZ K J H, PENZ M, et al. Density-potential mappings in quantum dynamics [J]. Physical Review A, 2012, 85(5): 052504. doi: 10.1103/PhysRevA.85.052504 [20] RUGGENTHALER M, VAN LEEUWEN R. Global fixed-point proof of time-dependent density-functional theory [J]. EPL (Europhysics Letters), 2011, 95(1): 13001. doi: 10.1209/0295-5075/95/13001 [21] ULLRICH C A. Time-dependent density-functional theory: concepts and applications [M]. OUP Oxford, 2011. [22] GIULIANI G, VIGNALE G. Quantum theory of the electron liquid [M]. Cambridge: Cambridge University Press, 2005. [23] ULLRICH C A, YANG Z. A brief compendium of time-dependent density functional theory [J]. Brazilian Journal of Physics, 2014, 44(1): 154–188. doi: 10.1007/s13538-013-0141-2 [24] GROSS E K U, KOHN W. Local density-functional theory of frequency-dependent linear response [J]. Physical Review Letters, 1985, 55(26): 2850–2852. doi: 10.1103/PhysRevLett.55.2850 [25] CASIDA M E. Time-dependent density functional response theory for molecules [M]//Recent Advances in Computational Chemistry. World Scientific, 1995: 155–192. [26] HIRATA S, HEAD-GORDON M. Time-dependent density functional theory within the Tamm-Dancoff approximation [J]. Chemical Physics Letters, 1999, 314(3/4): 291–299. [27] CASTRO A, MARQUES M A L, RUBIO A. Propagators for the time-dependent Kohn-Sham equations [J]. The Journal of Chemical Physics, 2004, 121(8): 3425–3433. doi: 10.1063/1.1774980 [28] BURNUS T, MARQUES M A L, GROSS E K U. Time-dependent electron localization function [J]. Physical Review A, 2005, 71(1): 010501. doi: 10.1103/PhysRevA.71.010501 [29] POHL A, REINHARD P G, SURAUD E. Towards single-particle spectroscopy of small metal clusters [J]. Physical Review Letters, 2000, 84(22): 5090–5093. doi: 10.1103/PhysRevLett.84.5090 [30] VÉNIARD V, TAIEB R, MAQUET A. Photoionization of atoms using time-dependent density functional theory [J]. Laser Physics, 2003, 13(4): 465–474. [31] DE GIOVANNINI U, VARSANO D, MARQUES M A L, et al. Ab initio angle-and energy-resolved photoelectron spectroscopy with time-dependent density-functional theory [J]. Physical Review A, 2012, 85(6): 062515. doi: 10.1103/PhysRevA.85.062515 [32] ROHRINGER N, PETER S, BURGDÖRFER J. Calculating state-to-state transition probabilities within time-dependent density-functional theory [J]. Physical Review A, 2006, 74(4): 042512. doi: 10.1103/PhysRevA.74.042512 [33] LI Y, ULLRICH C A. Time-dependent transition density matrix [J]. Chemical Physics, 2011, 391(1): 157–163. doi: 10.1016/j.chemphys.2011.02.001 [34] D’AGOSTA R, VIGNALE G. Non-V-representability of currents in time-dependent many-particle systems [J]. Physical Review B, 2005, 71(24): 245103. doi: 10.1103/PhysRevB.71.245103 [35] VIGNALE G. Mapping from current densities to vector potentials in time-dependent current density functional theory [J]. Physical Review B, 2004, 70(20): 201102. doi: 10.1103/PhysRevB.70.201102 [36] VIGNALE G, KOHN W. Current-dependent exchange-correlation potential for dynamical linear response theory [J]. Physical Review Letters, 1996, 77(10): 2037–2040. doi: 10.1103/PhysRevLett.77.2037 [37] VIGNALE G, ULLRICH C A, CONTI S. Time-dependent density functional theory beyond the adiabatic local density approximation [J]. Physical Review Letters, 1997, 79(24): 4878–4881. doi: 10.1103/PhysRevLett.79.4878 [38] MAITRA N T, SOUZA I, BURKE K. Current-density functional theory of the response of solids [J]. Physical Review B, 2003, 68(4): 045109. doi: 10.1103/PhysRevB.68.045109 [39] YABANA K, BERTSCH G F. Time-dependent local-density approximation in real time [J]. Physical Review B, 1996, 54(7): 4484–4487. [40] BERTSCH G F, IWATA J I, RUBIO A, et al. Real-space, real-time method for the dielectric function [J]. Physical Review B, 2000, 62(12): 7998–8002. [41] YABANA K, SUGIYAMA T, SHINOHARA Y, et al. Time-dependent density functional theory for strong electromagnetic fields in crystalline solids [J]. Physical Review B, 2012, 85(4): 045134. [42] MARQUES M A L, CASTRO A, BERTSCH G F, et al. Octopus: a first-principles tool for excited electron–ion dynamics [J]. Computer Physics Communications, 2003, 151(1): 60–78. [43] CASTRO A, APPEL H, OLIVEIRA M, et al. Octopus: a tool for the application of time-dependent density functional theory [J]. Physica Status Solidi (B), 2006, 243(11): 2465–2488. [44] ANDRADE X, STRUBBE D, DE GIOVANNINI U, et al. Real-space grids and the Octopus code as tools for the development of new simulation approaches for electronic systems [J]. Physical Chemistry Chemical Physics, 2015, 17(47): 31371–31396. [45] DREUW A, HEAD-GORDON M. Failure of time-dependent density functional theory for long-range charge-transfer excited states: the zincbacteriochlorin-bacteriochlorin and bacteriochlorophyll-spheroidene complexes [J]. Journal of the American Chemical Society, 2004, 126(12): 4007–4016. doi: 10.1021/ja039556n [46] HIERINGER W, GÖRLING A. Failure of time-dependent density functional methods for excitations in spatially separated systems [J]. Chemical Physics Letters, 2006, 419(4/5/6): 557–562. [47] AUTSCHBACH J. Charge-transfer excitations and time-dependent density functional theory: problems and some proposed solutions [J]. ChemPhysChem, 2009, 10(11): 1757–1760. doi: 10.1002/cphc.v10:11 [48] CASIDA M E, GUTIERREZ F, GUAN J, et al. Charge-transfer correction for improved time-dependent local density approximation excited-state potential energy curves: analysis within the two-level model with illustration for H2 and LiH [J]. The Journal of Chemical Physics, 2000, 113(17): 7062–7071. doi: 10.1063/1.1313558 [49] HU C, SUGINO O, MIYAMOTO Y. Modified linear response for time-dependent density-functional theory: application to Rydberg and charge-transfer excitations [J]. Physical Review A, 2006, 74(3): 032508. doi: 10.1103/PhysRevA.74.032508 [50] HU C, SUGIN O. Average excitation energies from time-dependent density functional response theory [J]. Journal of Chemical Physics, 2007, 126: 074112. doi: 10.1063/1.2436887 [51] TAWADA Y, TSUNEDA T, YANAGISAWA S, et al. A long-range-corrected time-dependent density functional theory [J]. The Journal of Chemical Physics, 2004, 120(18): 8425–8433. doi: 10.1063/1.1688752 [52] TOKURA S, TSUNEDA T, HIRAO K. Long-range-corrected time-dependent density functional study on electronic spectra of five-membered ring compounds and free-base porphyrin [J]. Journal of Theoretical and Computational Chemistry, 2006, 5(4): 925–944. doi: 10.1142/S0219633606002684 [53] VYDROV O A, SCUSERIA G E. Assessment of a long-range corrected hybrid functional [J]. The Journal of Chemical Physics, 2006, 125(23): 234109. doi: 10.1063/1.2409292 [54] LIVSHITS E, BAER R. A well-tempered density functional theory of electrons in molecules [J]. Physical Chemistry Chemical Physics, 2007, 9(23): 2932–2941. doi: 10.1039/b617919c [55] PEACH M J G, TELLGREN E I, SAŁEK P, et al. Structural and electronic properties of polyacetylene and polyyne from hybrid and coulomb-attenuated density functionals [J]. The Journal of Physical Chemistry A, 2007, 111(46): 11930–11935. doi: 10.1021/jp0754839 [56] LIVSHITS E, BAER R. A density functional theory for symmetric radical cations from bonding to dissociation [J]. The Journal of Physical Chemistry A, 2008, 112(50): 12789–12791. doi: 10.1021/jp803606n [57] STEIN T, KRONIK L, BAER R. Reliable prediction of charge transfer excitations in molecular complexes using time-dependent density functional theory [J]. Journal of the American Chemical Society, 2009, 131(8): 2818–2820. doi: 10.1021/ja8087482 [58] MAITRA N T, ZHANG F, CAVE R J, et al. Double excitations within time-dependent density functional theory linear response [J]. The Journal of Chemical Physics, 2004, 120(13): 5932–5937. doi: 10.1063/1.1651060 [59] GRITSENKO O V, BAERENDS E J. Double excitation effect in non-adiabatic time-dependent density functional theory with an analytic construction of the exchange–correlation kernel in the common energy denominator approximation [J]. Physical Chemistry Chemical Physics, 2009, 11(22): 4640–4646. doi: 10.1039/b903123e [60] ROMANIELLO P, SANGALLI D, BERGER J A, et al. Double excitations in finite systems [J]. The Journal of Chemical Physics, 2009, 130(4): 044108. doi: 10.1063/1.3065669 [61] SANGALLI D, ROMANIELLO P, ONIDA G, et al. Double excitations in correlated systems: a many–body approach [J]. The Journal of Chemical Physics, 2011, 134(3): 034115. doi: 10.1063/1.3518705 [62] SÄKKINEN N, MANNINEN M, VAN LEEUWEN R. The Kadanoff–Baym approach to double excitations in finite systems [J]. New Journal of Physics, 2012, 14(1): 013032. doi: 10.1088/1367-2630/14/1/013032 [63] HUIX-ROTLLANT M, IPATOV A, RUBIO A, et al. Assessment of dressed time-dependent density-functional theory for the low-lying valence states of 28 organic chromophores [J]. Chemical Physics, 2011, 391(1): 120–129. doi: 10.1016/j.chemphys.2011.03.019 [64] FURCHE F, AHLRICHS R. Absolute configuration of D2-symmetric fullerene C84 [J]. Journal of the American Chemical Society, 2002, 124(15): 3804–3805. doi: 10.1021/ja012207d [65] LIU J, LIANG W Z. Molecular-orbital-free algorithm for the excited-state force in time-dependent density functional theory [J]. The Journal of Chemical Physics, 2011, 134(4): 044114. doi: 10.1063/1.3548063 [66] LEVINE B G, KO C, QUENNEVILLE J, et al. Conical intersections and double excitations in time-dependent density functional theory [J]. Molecular Physics, 2006, 104(5/6/7): 1039–1051. [67] TAPAVICZA E, TAVERNELLI I, ROTHLISBERGER U, et al. Mixed time-dependent density-functional theory/classical trajectory surface hopping study of oxirane photochemistry [J]. The Journal of Chemical Physics, 2008, 129(12): 124108. doi: 10.1063/1.2978380 [68] KADUK B, VAN VOORHIS T. Communication: conical intersections using constrained density functional theory–configuration interaction [J]. Journal of Chemical Physics, 2010, 133: 061102. doi: 10.1063/1.3470106 [69] MAITRA N T. On correlated electron-nuclear dynamics using time-dependent density functional theory [J]. The Journal of Chemical Physics, 2006, 125(1): 014110. doi: 10.1063/1.2210471 [70] GONZE X, GHOSEZ P, GODBY R W. Density-polarization functional theory of the response of a periodic insulating solid to an electric field [J]. Physical Review Letters, 1995, 74(20): 4035. doi: 10.1103/PhysRevLett.74.4035 [71] GHOSEZ P, GONZE X, GODBY R W. Long-wavelength behavior of the exchange-correlation kernel in the Kohn-Sham theory of periodic systems [J]. Physical Review B, 1997, 56(20): 12811–12817. doi: 10.1103/PhysRevB.56.12811 [72] ONIDA G, REINING L, RUBIO A. Electronic excitations: density-functional versus many-body Green’s-function approaches [J]. Reviews of Modern Physics, 2002, 74(2): 601–659. doi: 10.1103/RevModPhys.74.601 [73] BOTTI S, SOTTILE F, VAST N, et al. Long-range contribution to the exchange-correlation kernel of time-dependent density functional theory [J]. Physical Review B, 2004, 69(15): 155112. doi: 10.1103/PhysRevB.69.155112 [74] SHARMA S, DEWHURST J K, SANNA A, et al. Bootstrap approximation for the exchange-correlation kernel of time-dependent density-functional theory [J]. Physical Review Letters, 2011, 107(18): 186401. doi: 10.1103/PhysRevLett.107.186401 [75] SHARMA S, DEWHURST J K, SANNA A, et al. Enhanced excitonic effects in the energy loss spectra of LiF and Ar at large momentum transfer [J]. New Journal of Physics, 2012, 14(5): 053052. doi: 10.1088/1367-2630/14/5/053052 [76] TANCOGNE-DEJEAN N, MÜCKE O D, KÄRTNER F X, et al. Impact of the electronic band structure in high-harmonic generation spectra of solids [J]. Physical Review Letters, 2017, 118(8): 087403. doi: 10.1103/PhysRevLett.118.087403 [77] BACZEWSKI A D, SHULENBURGER L, DESJARLAIS M P, et al. X-ray thomson scattering in warm dense matter without the chihara decomposition [J]. Physical Review Letters, 2016, 116(11): 115004. doi: 10.1103/PhysRevLett.116.115004 [78] MO C, FU Z, KANG W, et al. First-principles estimation of electronic temperature from X-ray thomson scattering spectrum of isochorically heated warm dense matter [J]. Physical Review Letters, 2018, 120(20): 205002. doi: 10.1103/PhysRevLett.120.205002 [79] SPERLING P, GAMBOA E J, LEE H J, et al. Free-electron X-ray laser measurements of collisional-damped plasmons in isochorically heated warm dense matter [J]. Physical Review Letters, 2015, 115(11): 115001. doi: 10.1103/PhysRevLett.115.115001 [80] RAMAKRISHNAN R, HARTMANN M, TAPAVICZA E, et al. Electronic spectra from TDDFT and machine learning in chemical space [J]. The Journal of Chemical Physics, 2015, 143(8): 084111. doi: 10.1063/1.4928757 [81] FUKS J I, NIELSEN S E B, RUGGENTHALER M, et al. Time-dependent density functional theory beyond Kohn–Sham Slater determinants [J]. Physical Chemistry Chemical Physics, 2016, 18(31): 20976–20985. doi: 10.1039/C6CP00722H [82] NIELSEN S E B, RUGGENTHALER M, VAN LEEUWEN R. Many-body quantum dynamics from the density [J]. EPL (Europhysics Letters), 2013, 101(3): 33001. doi: 10.1209/0295-5075/101/33001 [83] ELLIOTT P, MAITRA N T. Propagation of initially excited states in time-dependent density-functional theory [J]. Physical Review A, 2012, 85(5): 052510. doi: 10.1103/PhysRevA.85.052510 [84] CASTRO A, MARQUES M A L, ALONSO J A, et al. Excited states dynamics in time-dependent density functional theory [J]. The European Physical Journal D, 2004, 28(2): 211–218. doi: 10.1140/epjd/e2003-00306-3 [85] ALONSO J L, ANDRADE X, ECHENIQUE P, et al. Efficient formalism for large-scale ab initio molecular dynamics based on time-dependent density functional theory [J]. Physical Review Letters, 2008, 101(9): 096403. doi: 10.1103/PhysRevLett.101.096403 [86] MENG S, KAXIRAS E. Real-time, local basis-set implementation of time-dependent density functional theory for excited state dynamics simulations [J]. The Journal of Chemical Physics, 2008, 129(5): 054110. doi: 10.1063/1.2960628 [87] MENG S, KAXIRAS E. Mechanisms for ultrafast nonradiative relaxation in electronically excited eumelanin constituents [J]. Biophysical Journal, 2008, 95(9): 4396–4402. doi: 10.1529/biophysj.108.135756 [88] TULLY J C. Molecular dynamics with electronic transitions [J]. The Journal of Chemical Physics, 1990, 93(2): 1061–1071. doi: 10.1063/1.459170 [89] TAPAVICZA E, TAVERNELLI I, ROTHLISBERGER U. Trajectory surface hopping within linear response time-dependent density-functional theory [J]. Physical Review Letters, 2007, 98(2): 023001. doi: 10.1103/PhysRevLett.98.023001 [90] TAVERNELLI I, CURCHOD B F E, ROTHLISBERGER U. Mixed quantum-classical dynamics with time-dependent external fields: a time-dependent density-functional-theory approach [J]. Physical Review A, 2010, 81(5): 052508. doi: 10.1103/PhysRevA.81.052508 [91] KRISHTAL A, CERESOLI D, PAVANELLO M. Subsystem real-time time dependent density functional theory [J]. The Journal of Chemical Physics, 2015, 142(15): 154116. doi: 10.1063/1.4918276 [92] PAVANELLO M. On the subsystem formulation of linear-response time-dependent DFT [J]. The Journal of Chemical Physics, 2013, 138(20): 204118. doi: 10.1063/1.4807059 [93] NEUGEBAUER J. Couplings between electronic transitions in a subsystem formulation of time-dependent density functional theory [J]. The Journal of Chemical Physics, 2007, 126(13): 134116. doi: 10.1063/1.2713754 -







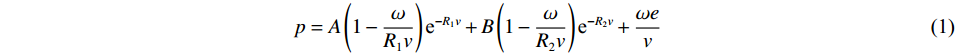
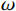
 下载:
下载:
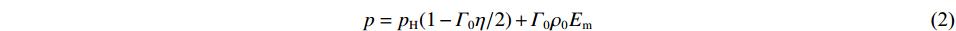
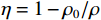
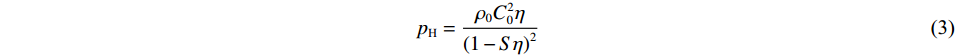
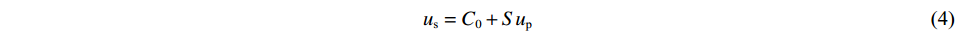
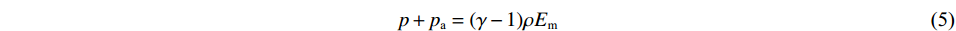
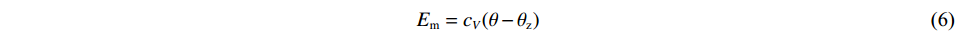
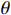
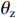










 下载:
下载: