Scattering Characteristics of Sub-Millimeter Metal Particle Group Driven by Explosion
-
摘要: 采用试验与数值模拟相结合的方法,研究了爆炸驱动下亚毫米级WC颗粒群的飞散特性及其影响因素。首先对重金属嵌层碳纤维复合材料(CFRP)壳体开展静爆试验研究,测得距爆心一定距离处颗粒速度;然后基于离散元方法(DEM),依据实体情况对WC颗粒层的颗粒进行无序建模与数值模拟,分析了颗粒无序排列时不同颗粒、装填比及长径比对颗粒速度的影响规律。结果表明:在相同装填比下,颗粒粒径越大,单个颗粒所获得的速度越小;端部附近内外层颗粒速度相同,相对轴向位置X/L=0.62附近速度差最大;长径比在0.5~1.5范围内时,随着长径比的增加,颗粒的速度及速度差增大,起爆端相对于非起爆端颗粒速度增加较小。Abstract: Under the implosion of the carbon fiber composite (CFRP) shell embedded in dense inert metal particle, the damage elements dominated by dense inert metal particles will be formed. Thus, accurately acquiring and predicting the scattering performance of heavy metal particle cluster driven by internal-burst is of great significance for the design and evaluation of damage capability of low collateral damage munitions. In this paper, both the experimental study and numerical simulation are adopted to investigate the scattering characteristics and influencing factors of the sub-millimeter WC particle group under explosion. Based on the discrete element method (DEM), the disordered model and numerical simulation of particles in WC particle layer are carried out according to the entity condition, the effects of different particles, loading ratio and length-to-diameter ratio on particle velocity are analyzed. The results show that the larger size of a particle can result in a lower velocity under the condition of the same loading ratio. The outer layer particle velocity at the end is the same, but the velocity difference near the relative axial position X/L=0.62 is the largest. When the ratio of length to diameter is in the range of 0.5–1.5, both the particle velocity and velocity difference increase with the ratio of length to diameter, and the incremental velocity of the particles at the detonating end is smaller than that at the non-initiating end.
-
Key words:
- discrete element method /
- sub-millimeter metal particle /
- explosive drive
-
当前很多学者通过实验和数值仿真研究了聚脲衰减冲击波的特性[1-3]。曾必强等[4]通过数值模拟方法研究了不同初始速度的随进弹受前级装药爆炸冲击波影响产生的后抛、侧偏、翻转和力学响应规律,发现随进弹的后抛加速作用主要由前级装药爆炸产生的爆炸冲击波作用到弹体表面而产生,随进弹的侧偏现象则是作用在弹体的冲击波与冲击波后方的爆轰产物共同作用的结果。姜夕博等[5]、徐森等[6]研究了冲击波在有机玻璃中的衰减特性。侯海周等[7]的实验结果表明,药柱冲击波经酚醛隔板传播时,其峰值压力随着隔板厚度的增加呈指数衰减。然而,单层材料的隔爆能力十分有限,需要通过增加隔爆层厚度提升衰减冲击波能力,但是却占用了战斗部空间,增加了战斗部质量。近年来,许多学者对多层组合介质中冲击波的衰减特性开展了大量研究[8-10]。董永香等[11]通过分析不同介质层中和层界面的爆炸波形和幅值得出,在脆性材料中放置泡沫材料可以有效减小爆炸波应力峰值,降低自由表面的拉伸应力波幅值,软夹层在多层介质中能够起到明显的削波和增加加载时间的作用,中间夹层改变了爆炸波能量的分配。陈闯等[12]通过实验与数值模拟相结合的方法,将45钢、铝、有机玻璃进行不同组合,设计了顺序波阻抗梯度、逆序波阻抗梯度以及硬软硬3种多层隔爆结构,结果表明:逆序波阻抗梯度隔爆结构的输出冲击波压力最小,隔爆效果最佳。
为了在有限的战斗部空间内尽可能提高隔爆效果并减轻隔爆体质量,本研究在后级随进弹头部壳体表面加装隔爆体,采用ANSYS/LS-DYNA有限元软件进行数值模拟,研究不同结构的隔爆特性,以期使后级随进弹能顺利随进并侵彻后续目标靶。
1. 高压下冲击波在分层材料中的传播
高压下固体材料通常被认为是无黏性的可压缩流体,一般用介质Hugoniot关系曲线处理冲击波在两种不同介质上的反射和透射问题。定义介质的冲击阻抗为介质初始密度(固有)ρ0和冲击波速度DS的乘积,可近似用初始密度ρ0和声速C0的乘积表示。处理波从介质A传入介质B的最好方法是阻抗匹配法。碰撞界面的右行/左行冲击波满足连续方程和动量守恒。由界面连续条件可知:两介质界面上的粒子速度和压力相同。由一维冲击波理论可知,冲击波在两种界面处的反射波和透射波类型取决于两种介质的阻抗大小。当介质A的阻抗大于介质B时,反射波为稀疏波,反之反射波为冲击波[13-14]。
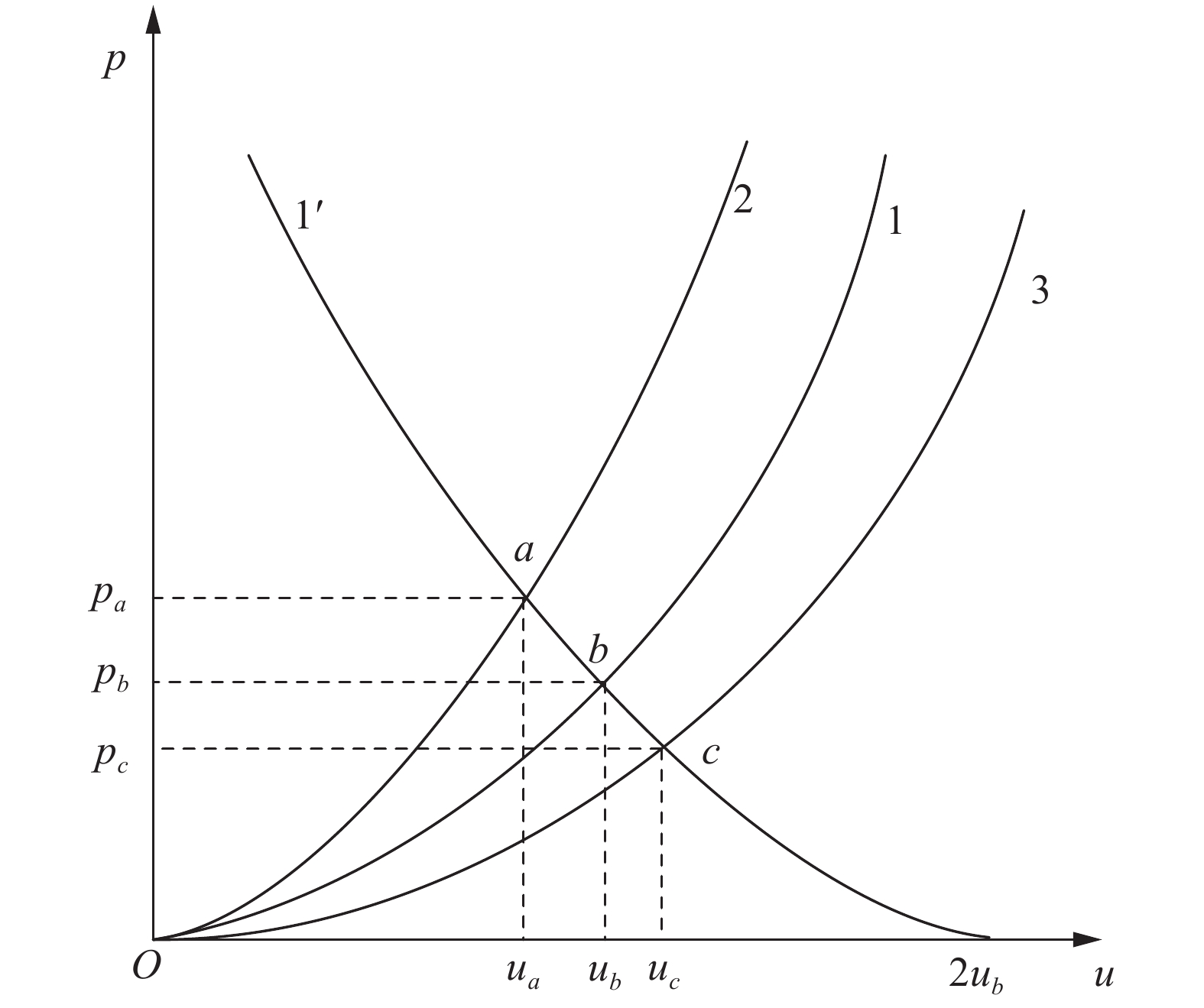
当冲击波传播至两层介质分界面处,可以利用介质的Hugoniot关系计算透射波和反射波,如图1所示,曲线1~曲线3分别表示不同介质的Hugoniot关系曲线,介质的阻抗越大,由原点出发与Hugoniot关系曲线上某点连线的斜率越大。图1反映了压力幅值为Pb的冲击波从介质A传入介质B的情形,分界面处的状态既在反射波Hugoniot曲线1′(曲线1′和曲线1呈镜像对称关系)上,也在介质B的右行透射波Hugoniot曲线上,即a点状态。同理,冲击波从介质A传入介质C时,状态从b点变为c点。
当固体处在高压条件下时,宋博等[15]提出用p=Au2分析冲击波在多层介质中的传播,其中A可用来表示材料的“相对软硬”程度,A值越大,材料越“硬”,反之,材料越“软”。假设冲击波初始状态为b(pb, ub),则曲线1′可以表示为
p=A1(u−2ub)2u<2ub (1) 则对于c点
p=A1(u−2ub)2=A2u2u<2ub (2) b点状态为pb=A1ub2,联立(2)式,消去u,求得透射系数T2为
T2=ppb=4(1+A1A2)2 (3) 由(3)式可以得出冲击波传播n层介质后的透射系数
T′n=22(1+A1A2)2×22(1+A2A3)2×⋯×22(1+An−1An)2=n−1∏i=122(1+AiAi+1)2=t2n (4) 其中
tn=n−1∏i=121+AiAi+1=2n−1n−1∏i=1(1+AiAi+1) (5) 由此可知对于相同层数(n)的相同介质来说,如果有某种顺序的排列结构,使得
Sn=n−1∏i=1(1+AiAi+1) (6) 存在最大值,那么这种结构衰减冲击波的性能最好,即这种结构能最大限度地削弱透射波强度。
2. 多层介质组合衰减冲击波特性分析及数值模拟
第1节的分析仅考虑了不同介质的排列次序对衰减冲击波的影响,而未考虑每层介质材料本身对波的衰减及组合介质不同结构对衰减冲击波的影响。下面通过数值模拟方法首先确定最佳组合介质,以期最大限度地削弱透射波,同时兼顾质量和体积。
2.1 模型建立
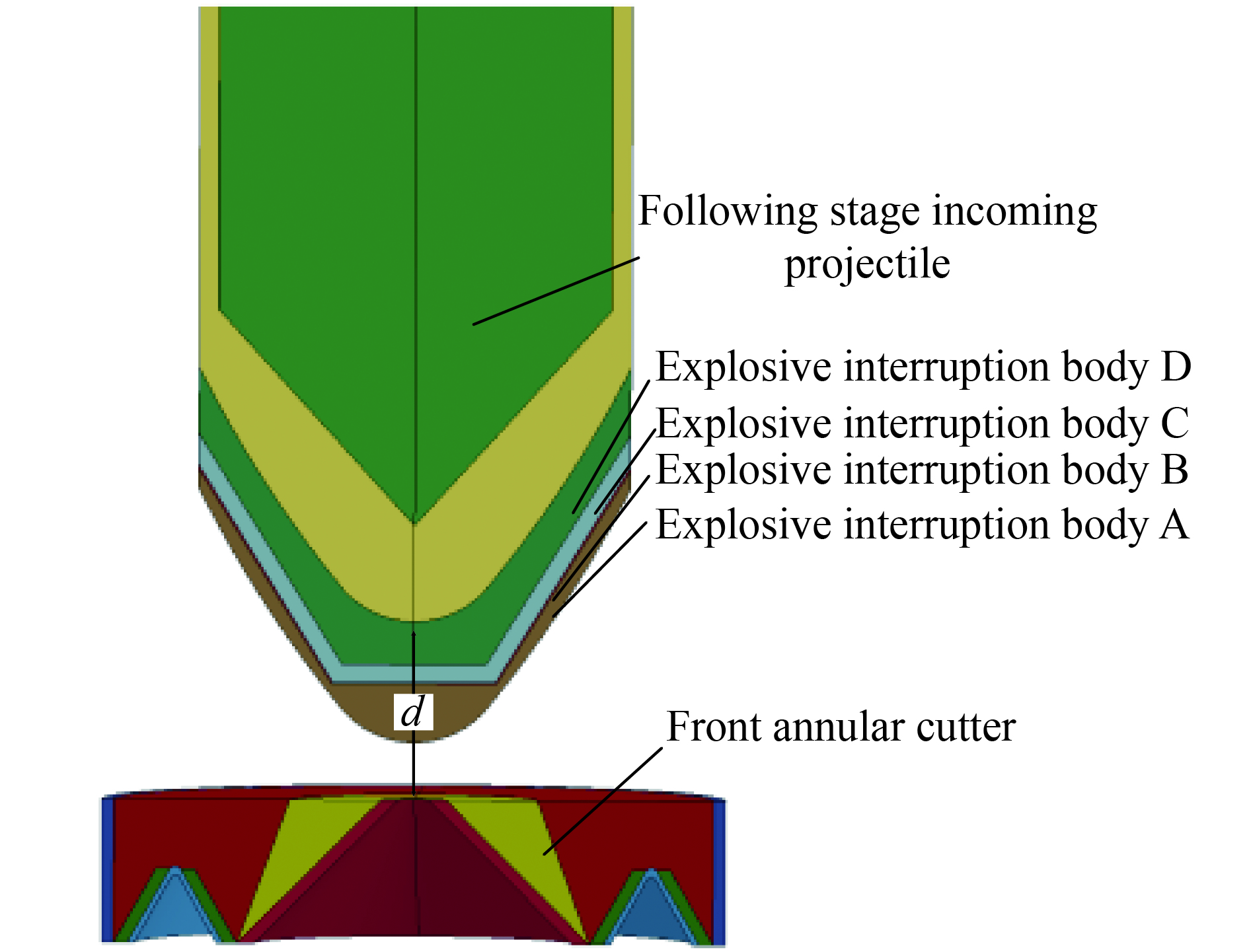
为了便于建立计算模型,作以下假设:(1)空气、炸药、药型罩和装药壳体均为连续介质;(2)整个爆炸过程为绝热过程。串联战斗部及隔爆结构如图2所示。为了保证后级随进弹能顺利侵彻,隔爆体A选择金属介质,考虑到计算模型和爆炸载荷具有对称特性,为提高计算效率,建立1/4模型,施加对称边界条件。计算模型由炸药、空气、药型罩、壳体、后级随进弹和隔爆体组成,其中炸药、空气和药型罩定义为Euler网格,壳体、后级随进弹和隔爆体定义为Lagrange网格,隔爆体之间设置面面自动接触。选用多物质ALE(Arbitrary Lagrange-Euler)方法模拟分析前级爆轰场对后级随进弹的影响。起爆方式采用顶端环形起爆。
2.2 材料模型及状态方程
炸药选用B炸药,采用MAT_HIGH-EXPLOSIVE-BURN模型和JWL状态方程[16-17]描述
p=A(1−ωR1ν)e−R1v+B(1−ωR2ν)e−R2v+ωEν (7) 式中:p为爆轰压力;E为炸药比内能;v为相对比容;A、B、R1、R2、ω为参数,取值列于表1。表1中:ρ为密度,D为B炸药爆速,pCJ为CJ面压力,V0为初始相对比容。
表 1 B炸药材料参数Table 1. Material parameters of composition Bρ/(g·cm-3) D/(m·s-1) pCJ/GPa A/GPa B/GPa R1 R2 ω E0/GPa V0 1.713 7 500 28.6 524.2 7.678 4.2 1.1 0.34 8.499 1.0 药型罩材料选用紫铜,采用MAT_Steinberg材料模型和Grüneisen状态方程[18]描述
p=ρ0C2μ[1+(1−γ02)μ−a2μ2][1−(S1−1)μ−S2μ2μ+1−S3μ3(μ+1)2]2+(γ0+aμ)E (8) 式中:E为单位体积内能;ρ0为材料初始密度;C为us与up的交点,其中us为冲击波速度,up为粒子速度;S1、S2和S3是us-up曲线的斜率;γ0为Grüneisen系数;a为对γ0的修正;μ=(1/V)-1,V为当前空气的相对体积。
壳体选用30CrMnSiNi2A钢,用MAT_Johnson-Cook材料模型和Grüneisen状态方程描述;铝选用MAT_Johnson-Cook材料模型和Grüneisen状态方程描述,参数见表2,其中AJC、BJC、n、m为Johnson-Cook材料模型参数,Tm为材料熔化温度,T0为常压下的熔化温度,a为对Grüneisen系数γ0的修正。空气采用MAT_Null材料模型和Linear-Polynomial状态方程描述。
表 2 30CrMnSiNi2A钢和金属铝计算参数Table 2. Material performance parameters of 30CrMnSiNi2A steel and aluminumMaterial AJC/MPa BJC/MPa n C m Tm/K T0/K S1 γ0 a Steel 1 280 420 0.30 0.030 1.00 1 793 294 1.490 2.17 0.46 Al 265 426 0.34 0.015 1.00 775 294 1.345 2.13 0.10 聚脲选用MAT_PIECEWISE_LINEAR_PLASTICITY材料模型[19]描述。该模型常用来模拟弹塑性材料,屈服准则由断裂应变定义,应力-应变曲线由屈服强度、剪切模量定义或者通过8组塑性应变和应力的数组定义,应变率的影响采用Cowper-Symbols模型,弹性体材料各参数为[20-21]: ρ=1 020 kg/m3,杨氏模量E=230 MPa,泊松比ν=0.4,初始屈服强度σ0=14 MPa。
3. 数值模拟结果与分析
用B1、B2、B3和B4分别表示隔爆体A/B、B/C、C/D、D/后级弹壳体的界面,计算中两个界面处均采用面面自动接触条件。表3列出了5种工况。
表 3 不同工况及简便书写方式Table 3. Different conditions and indicationsCondition Material A B C D Ⅰ Steel Polyurea Aluminum foam Aluminum Ⅱ Aluminum Polyurea Aluminum foam Aluminum Ⅲ Aluminum Aluminum foam Aluminum foam Aluminum Ⅳ Aluminum Aluminum foam Aluminum foam Aluminum foam Ⅴ Aluminum Polyurea Polyurea Polyurea 3.1 爆轰波在介质中的衰减
工程实践表明:具有软夹层的硬软硬多层介质具有较明显的抗冲击和抗爆炸能力,其中硬层抗击近距离的爆炸波作用,发挥其刚度与强度效应,而软层由多孔泡沫材料组成,波阻抗低,强度和刚度低,吸波能力强。如果外加爆炸波的持续时间足够长,软夹层不再发挥作用,软夹层下面的硬层将受到较大的冲击作用。
这里假定几种材料的Hugoniot曲线分别为p=A1u(硬质钢),p=A2u(铝),p=A3u(泡沫铝),p=A4u (聚脲),并且有A1>A2>A3>A4。
3.2 硬软硬组合介质的隔爆能力分析
(1)工况I
对“硬质钢-聚脲-泡沫铝-铝”结构,有
Sn1=(1+A1A4)(1+A3A4)(1+A2A4) (9) 装药起爆后,冲击波首先撞击复合隔爆结构中的硬质钢,该碰撞过程为非弹性碰撞。在该冲击速度下钢板发生弹塑性变形,历时很短,通常钢板发生局部的弹塑性变形,而其他区域受碰撞挤压作用影响较小。当冲击波传播至B1界面时,发生反射和透射,聚脲作为一种弹性涂覆体,其冲击阻抗小于硬质钢,界面B1处反射稀疏波,然而聚脲层的厚度仅为1 mm,衰减冲击波的能力有限。冲击波从介质B传入介质C时,在界面B2处反射冲击波,接下来讨论中间泡沫铝层的塑性变形和卸载冲击波强度的变化规律。泡沫铝在冲击载荷作用下产生递增硬化的变形即破坏波(压实波),其非线性应力-应变曲线可简化成3个阶段:弹性段、屈服塑性段、压实段,其力学性能除了与泡沫的泡孔结构有关外,还与基体材料性质、应变率和密度等有关,而且对静水压有很强的依赖性。应力波在泡沫铝中经历以下两个步骤:一是泡沫铝压实过程中的冲击波传播;二是泡沫铝压实后,应力波在包括外层硬质钢、聚脲涂覆层、压实泡沫铝以及后端铝介质的多次反射和透射。冲击波从介质C传入介质D时,在界面B3处反射冲击波,经过前面3层介质的衰减作用后,铝介质的冲击压力较低,铝介质作为一种软金属材料,在冲击载荷作用下易发生变形和破坏,因而能最大限度地吸收能量,最大程度地减小后级装药的压力峰值。
为了检验工况I衰减冲击波的能力,查看随进弹壳体最大应力集中的区域以及装药压力峰值的区域和时刻。t=264 μs时,装药内部靠近轴线区域压力(见图3(a))达到最大值,为143.6 MPa;t=189 μs时,外侧壳体应力集中区域的应力峰值如图3(b)所示,可见该区域未发生塑性变形;应力峰值附近某单元应力-时间变化关系如图3(c)所示。
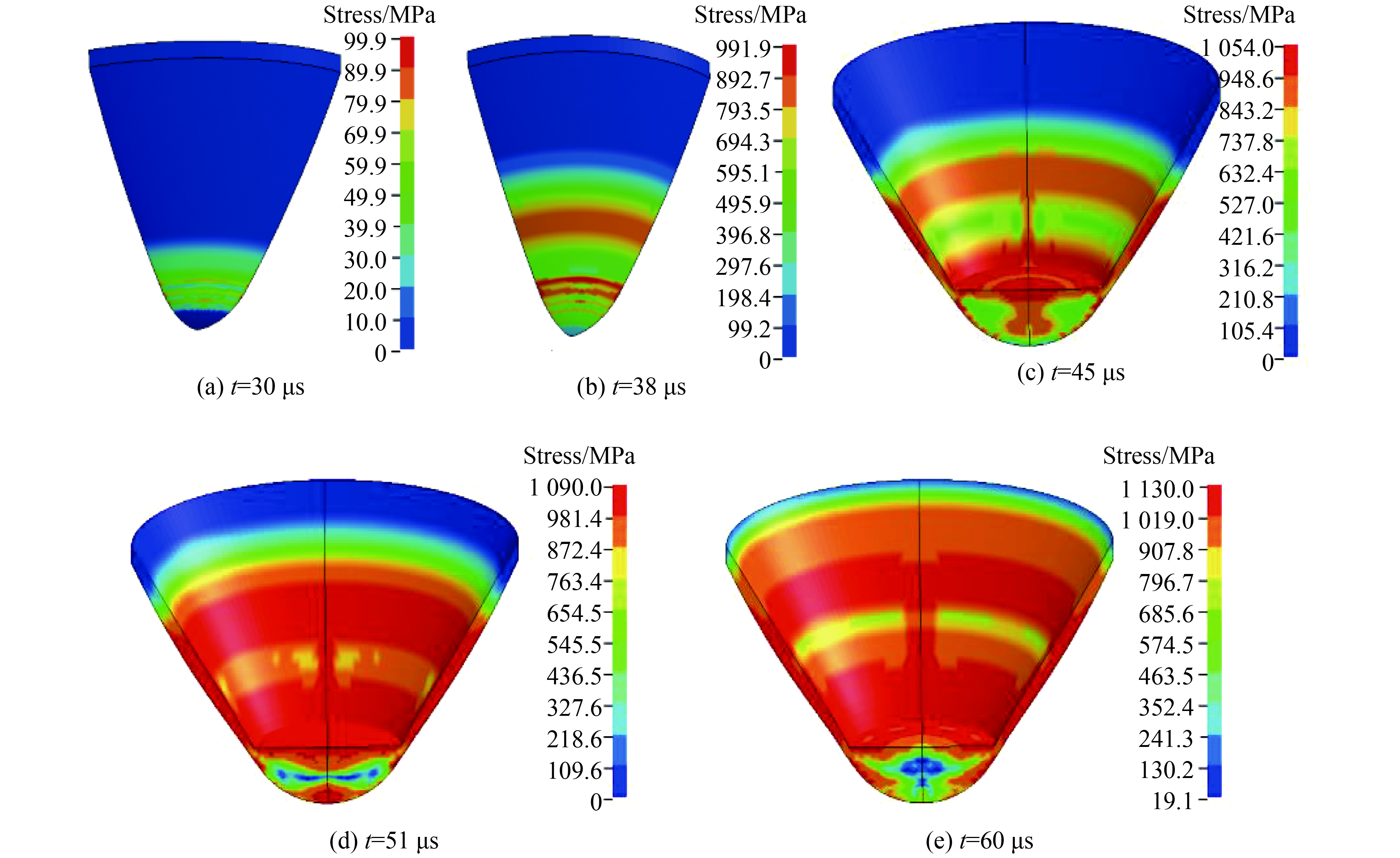
由图4可知:t=30 μs时,外层钢介质头部尖端靠里区域首先发生弹性变形;随着冲击波在介质中继续传播,t=38 μs时,钢介质弹性变形区域向后级随进弹方向移动;t=45 μs时,应力集中区域为钢介质最薄处;t=51 μs时,钢介质最薄处应力峰值继续增加;t=60 μs时,应力峰值达到最大值,为1 130 MPa。前级装药采用中空结构,装药起爆后爆轰波向后运动并汇聚的过程中,首先作用到钢介质尖端靠里区域,考虑到节约质量成本,钢介质头部尖端区域可适当减薄。
(2)工况II
与工况I相比,为了控制质量,外侧介质A由密度较大的硬质钢变为密度较小的铝。透射系数影响因子为
Sn2=(1+A2A4)(1+A3A4)(1+A2A4) (10) 比较Sn1和Sn2的大小,得到Sn1>Sn2,可知工况I所示结构中衰减冲击波压力的性能优于工况II所示结构。
装药起爆后,冲击波首先撞击复合隔爆结构中的金属铝,该碰撞过程为非弹性碰撞。金属铝的屈服强度小,在高速冲击载荷下铝板发生破坏,部分区域发生弹塑性变形。冲击波在聚脲层、泡沫铝和后端铝介质传播衰减分析方法与工况I类似。
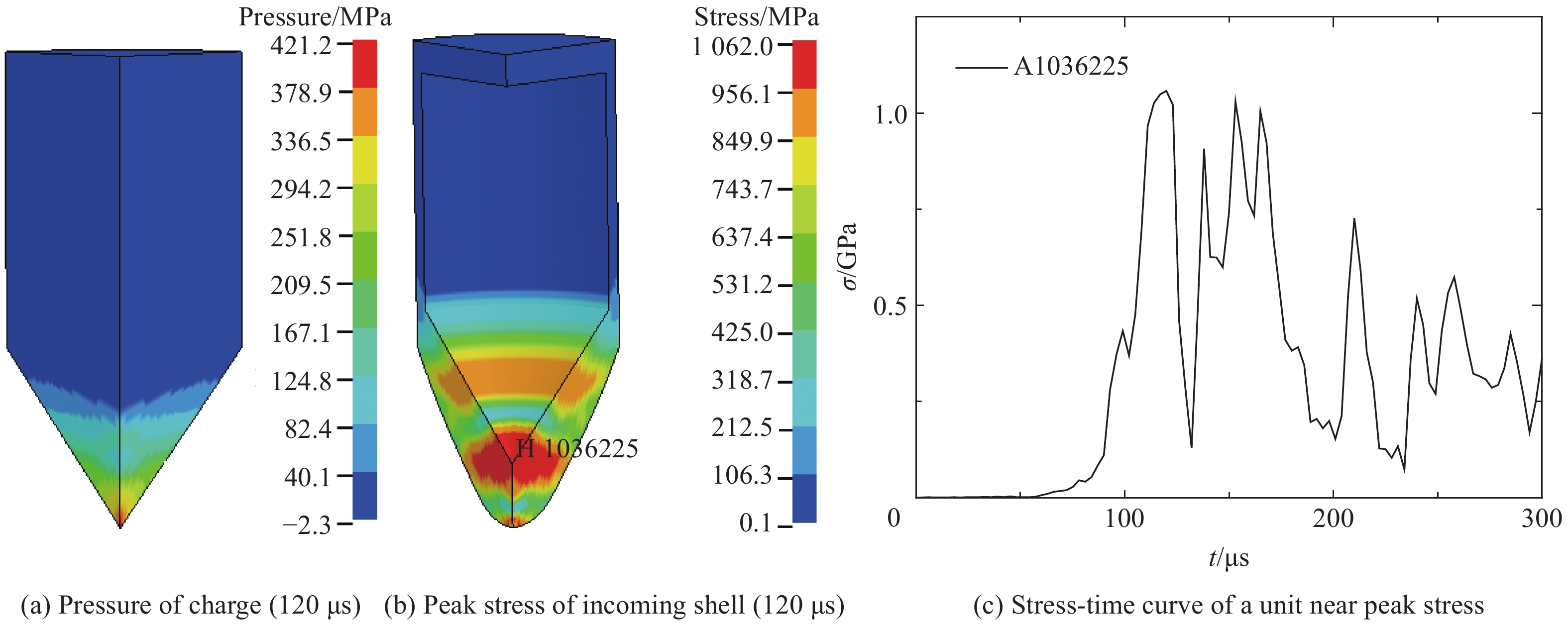
由图5可知:t=120 μs时,装药尖端压力达到最大值,为421.2 MPa;相同时刻,外侧壳体应力出现最大值1 062.0 MPa,见图5(b);应力峰值附近某单元的应力-时间曲线如图5(c)所示,后级随进弹壳体未进入塑性变形段。硬软硬结构存在不利的一面:随着作用时间的增加,对于多层介质而言,在波动效应后期结构振动效应增强。
(3)工况III
工况III所示隔爆结构为铝-泡沫铝-铝硬软硬结构,与工况II相比,将介质B换为泡沫铝。含有低阻抗软夹层的多层介质能够减小应力幅值,增加波形脉宽(即增加作用时间),从而减小由于应力波峰超过材料强度而引起局部破坏的可能性。
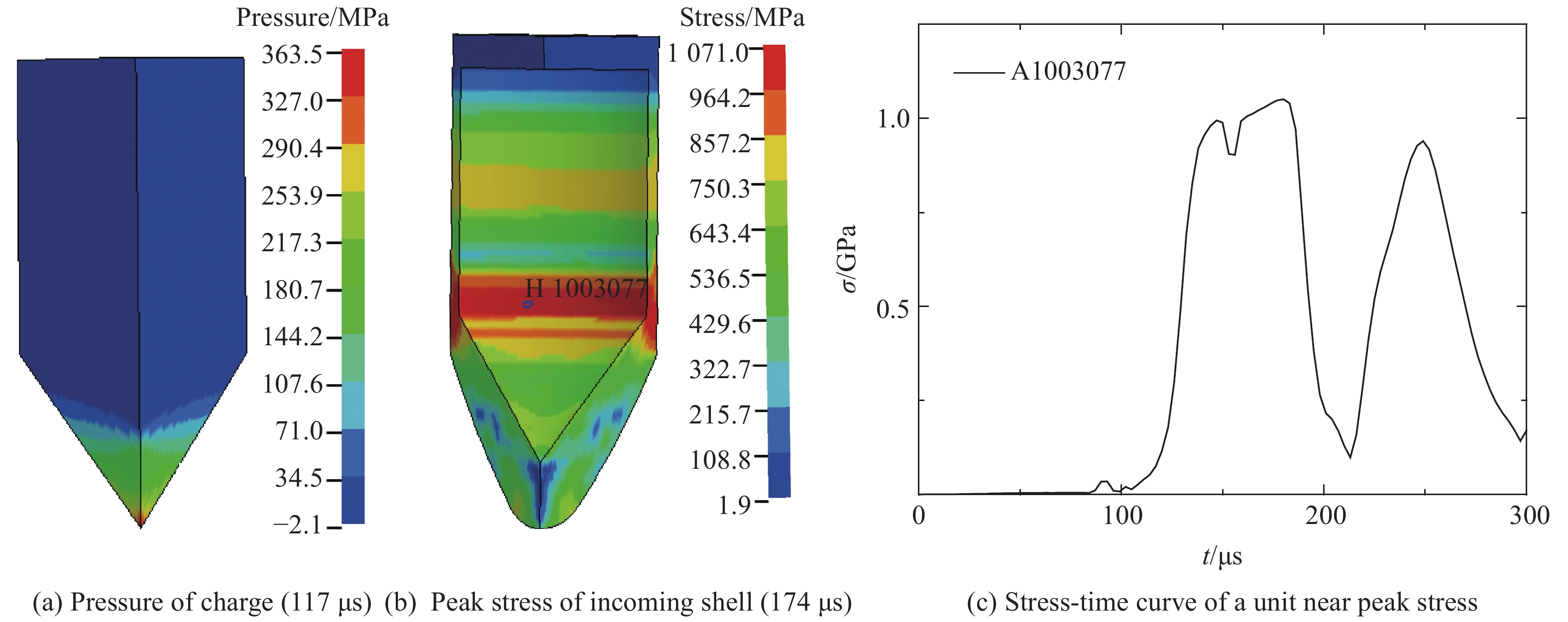
由图6可知:t=117 μs时,装药尖端压力达到最大值363.5 MPa,与中间有聚脲层时对应的压力峰值时刻基本一致;t=174 μs时,外侧壳体应力峰值为1 062.0 MPa,峰值区域如图6(b)所示;应力峰值附近单元的应力-时间曲线如图6(c)所示,后级随进弹壳体未进入塑性变形段。
3.3 硬软两层组合介质的隔爆能力分析
(1)工况IV
隔爆介质为两层,外层为金属铝,里层为泡沫铝,透射系数影响因子为
Sn4=1+A2A3 (11) 对比工况IV(见图7)和工况III:将厚端铝介质改为泡沫铝后,增加了泡沫铝的厚度,在爆炸冲击波作用下,泡沫铝内衬层能够吸收更多的能量,随进弹装药压力峰值比硬软硬结构中装药应力峰值出现得晚些;冲击波继续传播过程中,泡沫铝衰减冲击波的能力不再增加,最终泡沫铝被压实,密度增加,弹性模量也增加,紧挨软夹层的金属层受到较大的冲击作用,继续衰减冲击波。
(2)工况V
隔爆介质分层排列顺序为金属铝和弹性涂覆体聚脲,其透射系数影响因子为
Sn5=1+A2A4 (12) 计算可得Sn5>Sn4。
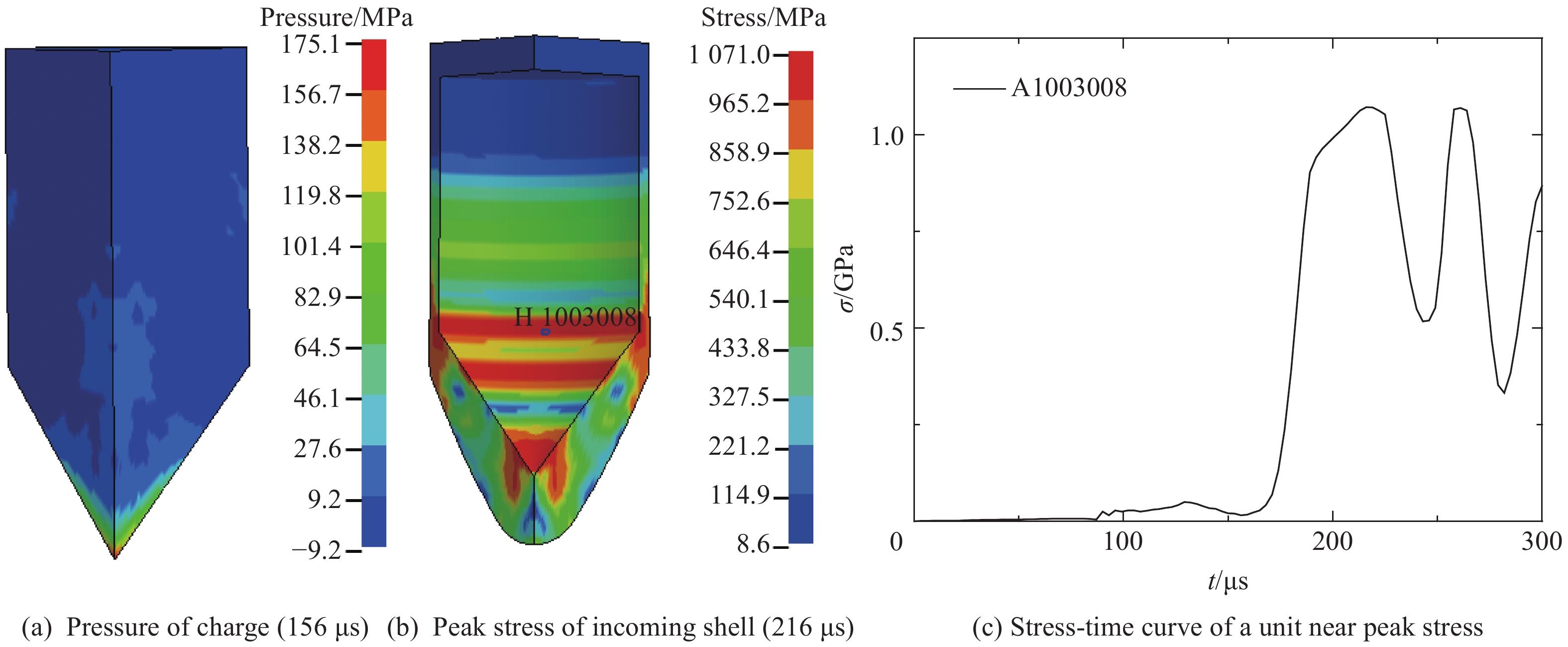
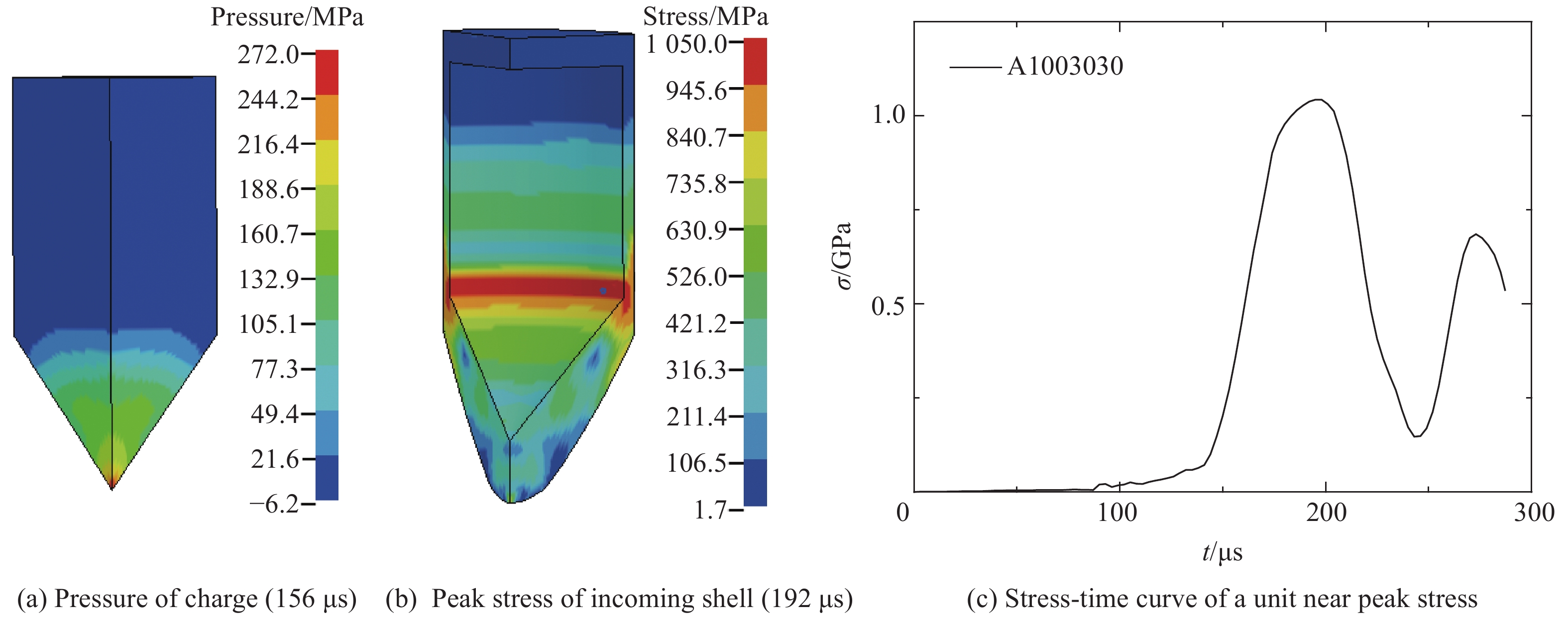
考虑将隔爆结构简化为金属铝-聚脲结构(即工况V),对比工况IV(即将泡沫铝改为弹性介质聚脲),则弹性体厚度明显增加。工况V中装药压力及壳体应力见图8:t=156 μs时,装药尖端压力达到最大值272.0 MPa,与工况IV中出现压力峰值时刻一致;t=192 μs时,外侧壳体应力峰值为1 050.0 MPa;应力峰值附近单元的应力-时间曲线显示,后级随进弹壳体未进入塑性变形段。聚脲作为一种喷涂材料,受制作工艺限制,喷涂有效厚度有限,该工况的聚脲层厚度为4 cm,远大于聚脲层的最大有效厚度。
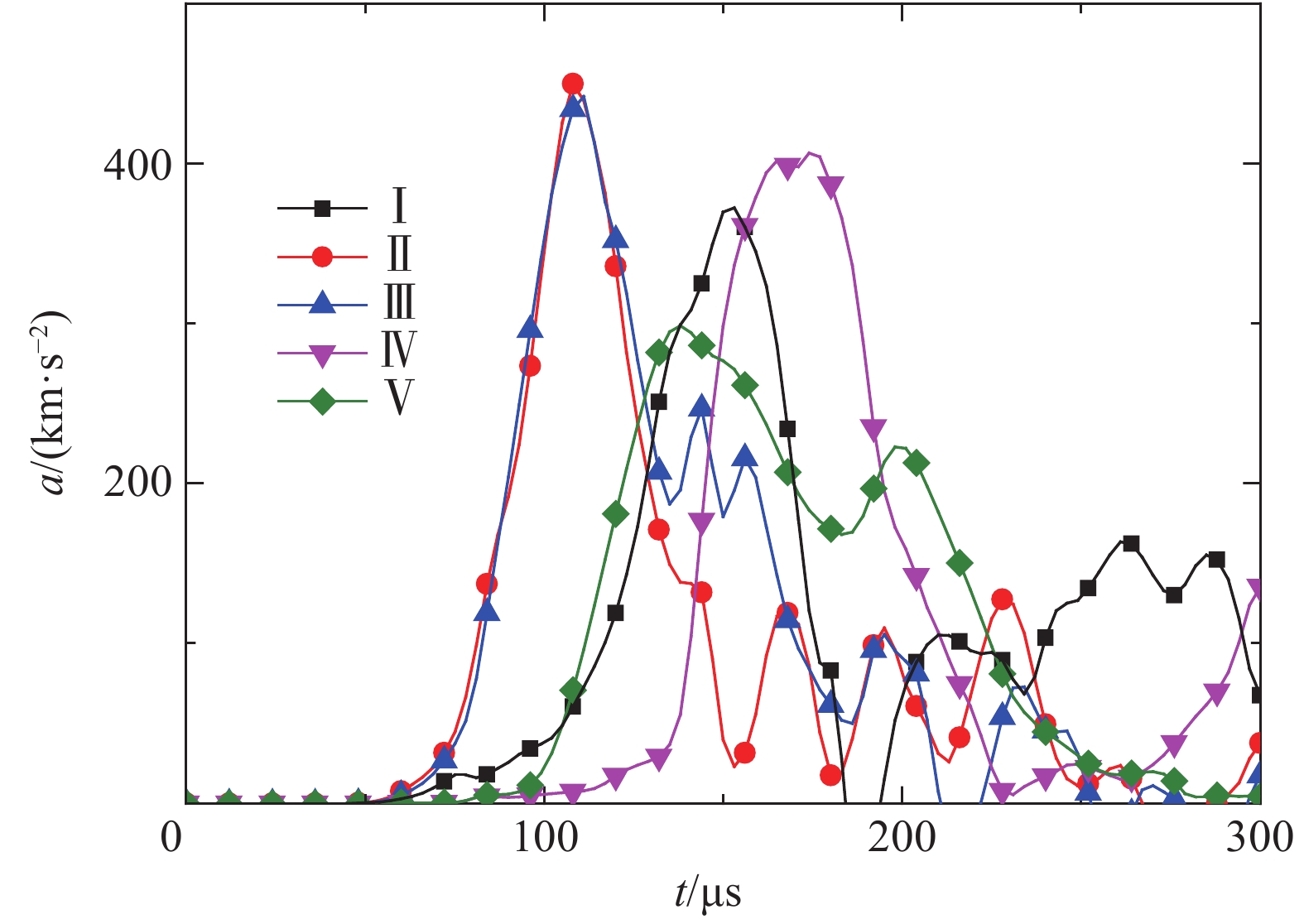
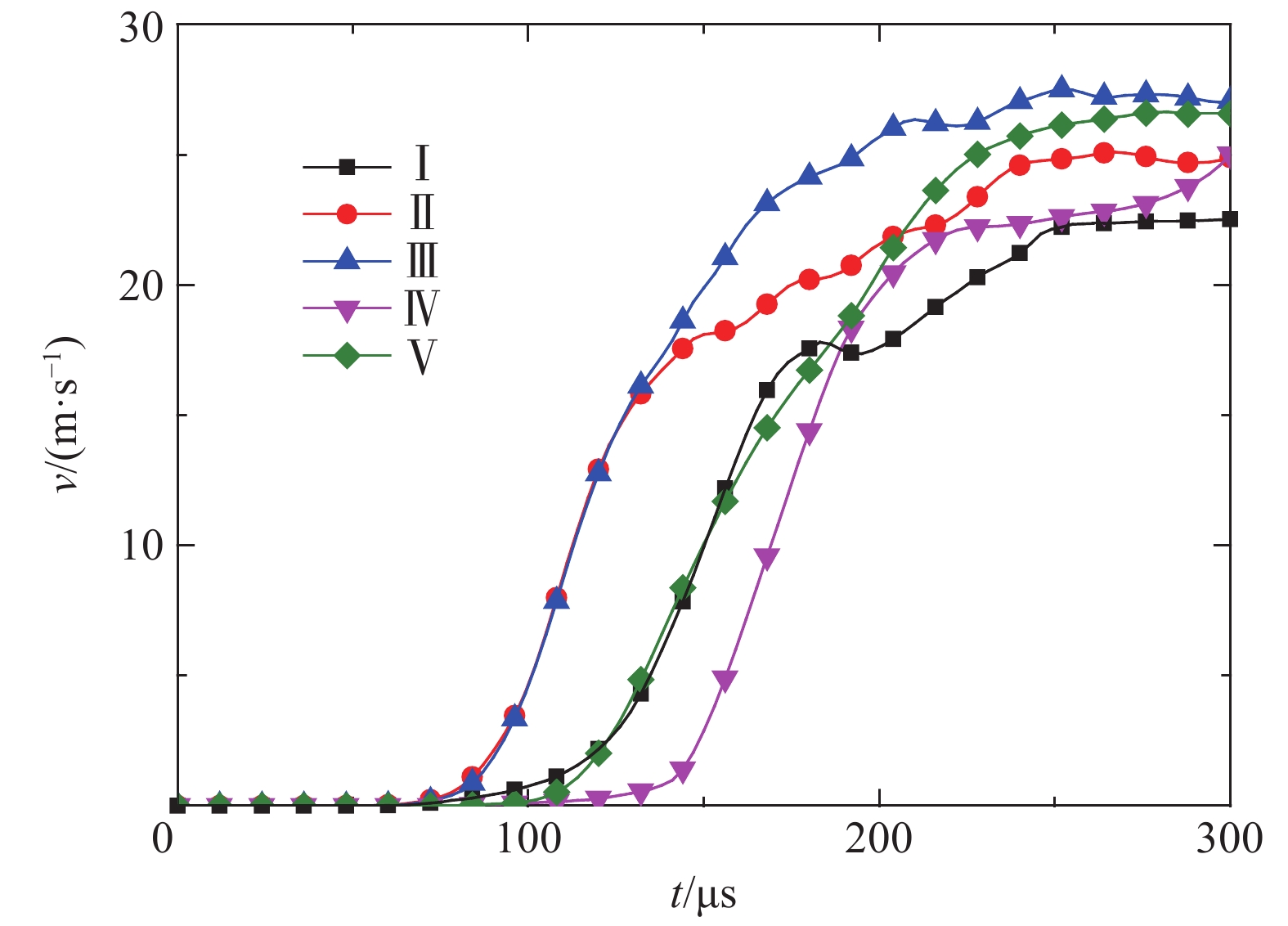
由图9可知:采用工况II和工况III所示隔爆结构时,后级随进弹出现加速度峰值的时间最早,且峰值最大;工况I所示隔爆结构中后级弹的加速度峰值最小,工况IV和工况I出现加速度峰值的时间基本一致;工况V所示结构延缓了冲击波作用到后级随进弹的时间,出现加速度峰值的时间最晚。由图10可知,采用上述5种隔爆结构时,后级随进弹速度增加到一定值时不再增加。
3.4 总结分析
采用工况I的隔爆结构时,后端装药的压力峰值出现得最晚,且压力峰值最小,仅为143.6 MPa;受质量成本的限制,工况II中介质A由密度较大的硬质钢换成密度较小的金属铝,金属铝的屈服强度较低,爆炸冲击载荷下容易发生破坏,部分区域发生弹塑性变形,装药压力峰值区域为装药尖端区域,远早于A介质为硬质钢的情形,且峰值压力为421.2 MPa,远大于工况I中对应的峰值压力,但后级壳体应力峰值与工况I的应力峰值相近,均未进入塑性变形区;工况III与工况II相比,介质B由弹性涂覆体聚脲改为泡沫铝,即隔爆结构转变为“铝-泡沫铝-铝”硬软硬结构,由于介质B的厚度仅为1 mm,对冲击波反射和透射的影响不大,因此硬软硬结构出现装药压力峰值的时刻与工况II基本一致,装药峰值压力小于工况II的情形;工况IV和工况V都降低了隔爆层数,分别为铝-泡沫铝结构和铝-聚脲结构,对比透射系数影响因子,前者小于后者,因此得出铝-聚脲结构隔爆效果优于铝-泡沫铝结构,但工况V中聚脲层的厚度过大,无法实现实际应用。接下来将改进隔爆结构,使之既能充分提升隔爆性能,节约体积和质量成本,又能实现实际应用。
4. 改进结构的数值模拟结果与讨论
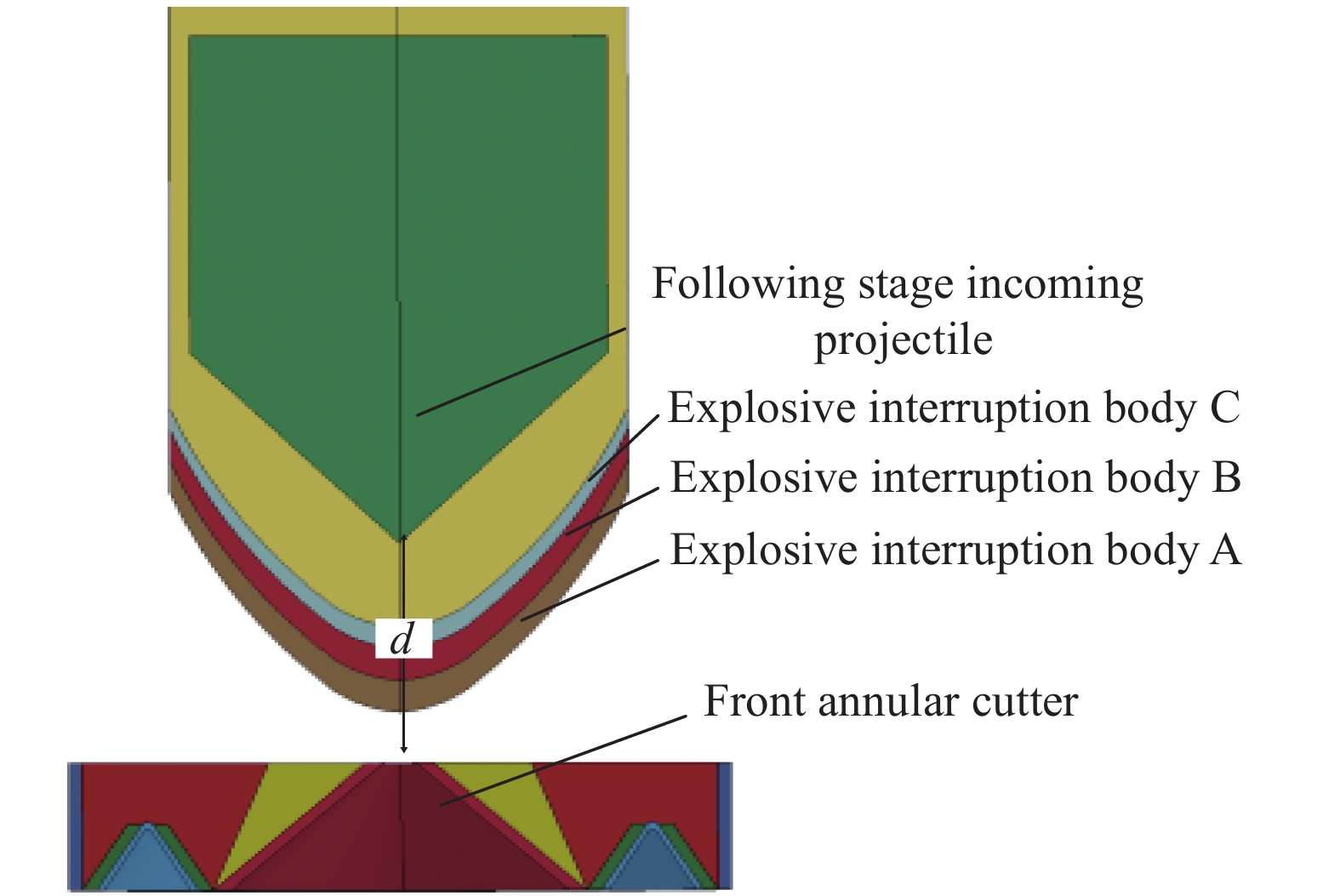
由第3节分析可知,装药起爆后,冲击波首先作用到最外层隔爆体头部靠里区域。为了控制总质量,适当减薄金属层尖端区域的厚度,改进后隔爆结构如图11所示,保持后级弹尖端与前级聚能装药结构后端的距离不变,即图中d。表4列出了4种改进结构。
表 4 改进结构Table 4. Improved structuresCondition Material A B C Ⅰ′ Steel Aluminum Polyurea Ⅱ′ Steel Aluminum Aluminum foam Ⅲ′ Aluminum Aluminum Polyurea Ⅳ′ Aluminum Aluminum Polyurea(2 cm) 4.1 逆序波阻抗结构的隔爆能力分析
(1)工况I′
工况I′下透射系数影响因子为
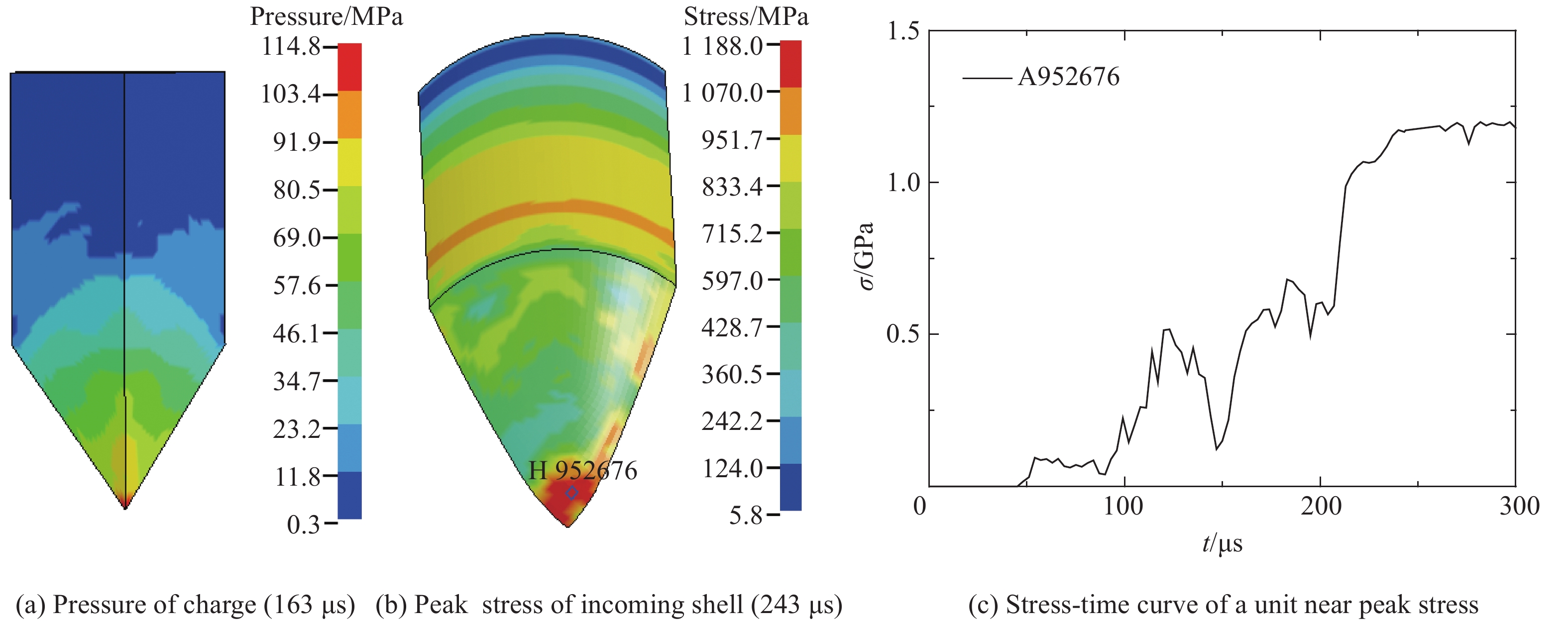
S′n1=(1+A1A2)(1+A2A4) (13) 由图12可知:t=163 μs时,装药尖端压力达到最大值114.8 MPa;t=243 μs时,外侧壳体应力峰值为1 188.0 MPa;应力峰值附近单元的应力-时间曲线显示,后级随进弹壳体尖端区域进入塑性变形区。
(2)工况II′
工况II′下,透射系数影响因子为
S′n2=(1+A1A2)(1+A2A3) (14) 比较可得,工况I′对应的透射系数大于工况II′,说明工况I′所示隔爆结构的衰减冲击波能力大于工况II′。由图13可知:t=246 μs时,装药尖端压力达到最大值125.7 MPa;t=297 μs时,外侧壳体应力峰值为1 209.0 MPa;应力峰值附近单元的应力-时间曲线显示,后级随进弹壳体尖端区域进入塑性变形区。
4.2 改进结构硬软两层组合介质的隔爆能力分析
(1)工况III′
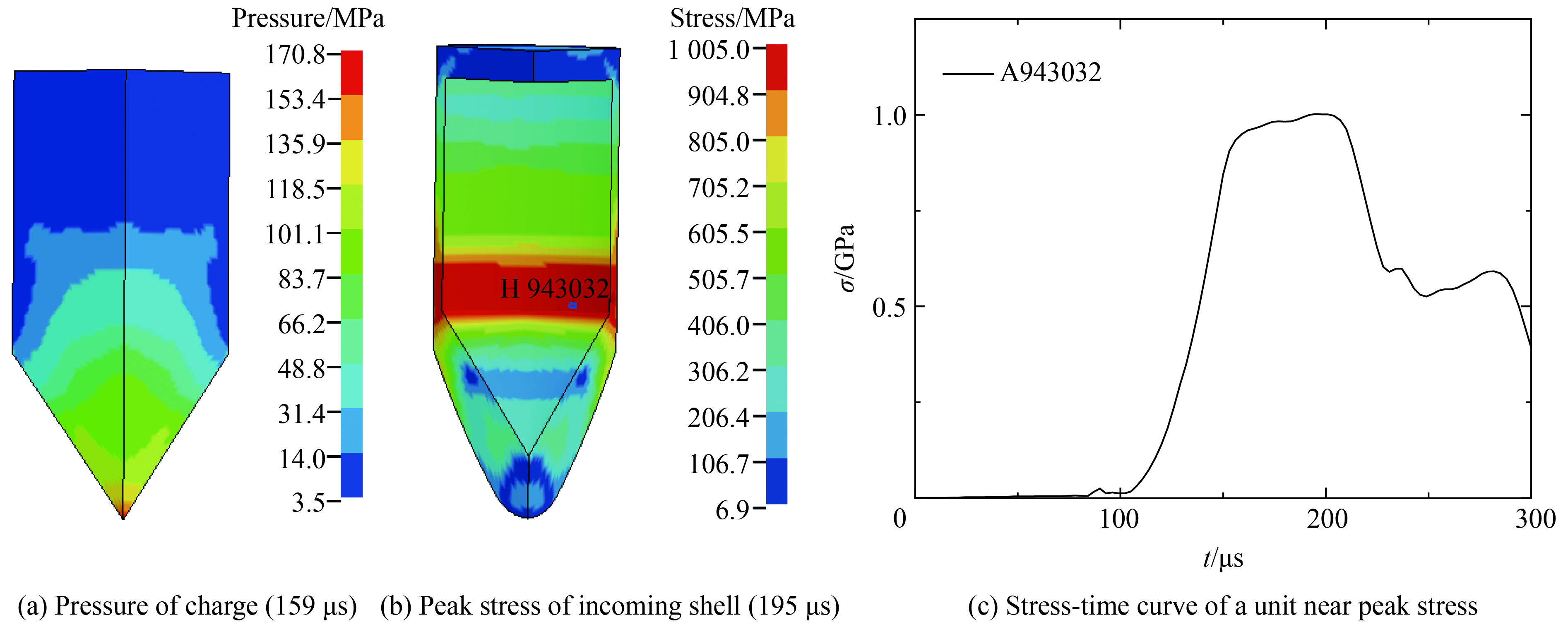
将最外层密度较大的硬质钢改为密度较小的金属铝,采用两层隔爆层,即金属铝-聚脲结构。由图14可知:t=159 μs时,装药内部尖端区域压力达到最大值170.8 MPa;t=195 μs时,外侧壳体应力峰值为1 005.0 MPa;应力峰值附近单元的应力-时间曲线显示,后级随进弹壳体未进入塑性变形区。
(2)工况IV′
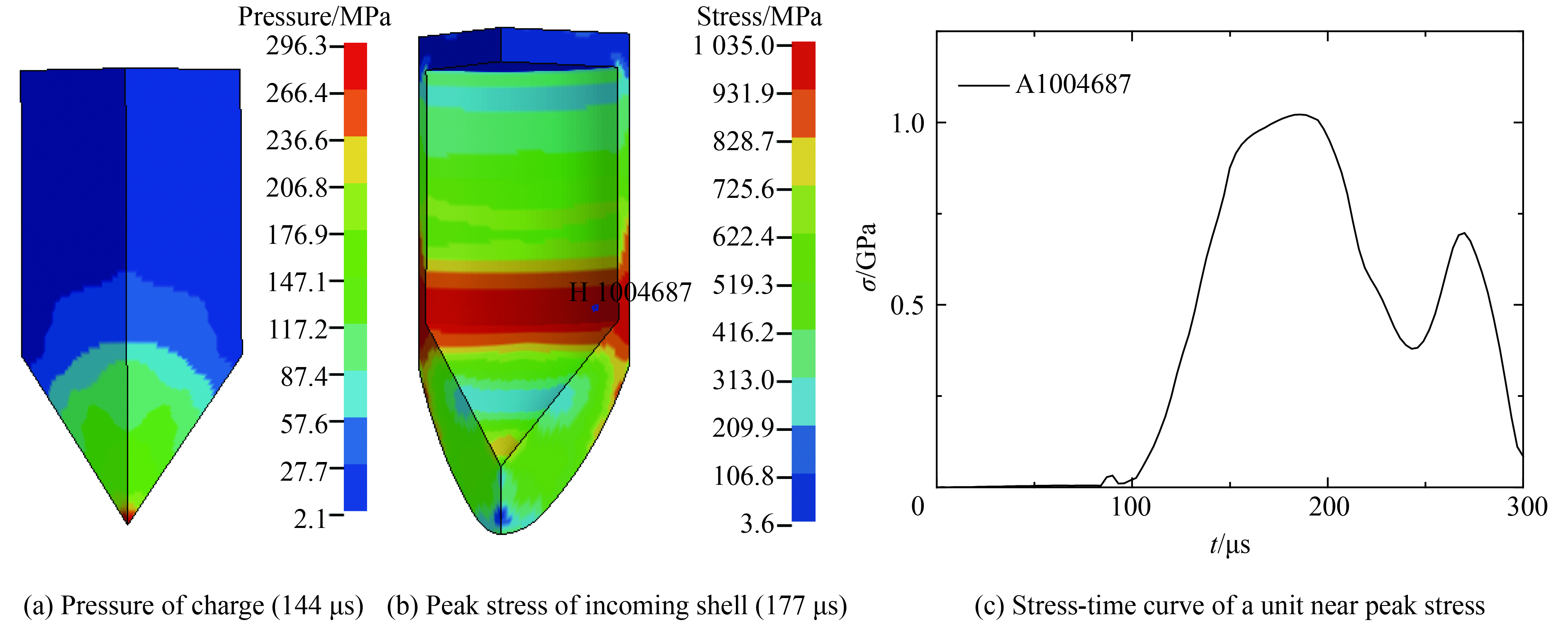
考虑到聚脲喷涂的厚度有限,将3 cm聚脲层减薄至2 cm,研究其隔爆性能。由图15可知:t=144 μs时,装药内部尖端区域压力达到最大值296.3 MPa;t=177 μs时,外侧壳体应力峰值为1 035.0 MPa;应力峰值附近单元的应力-时间曲线显示,后级随进弹壳体未进入塑性变形区。
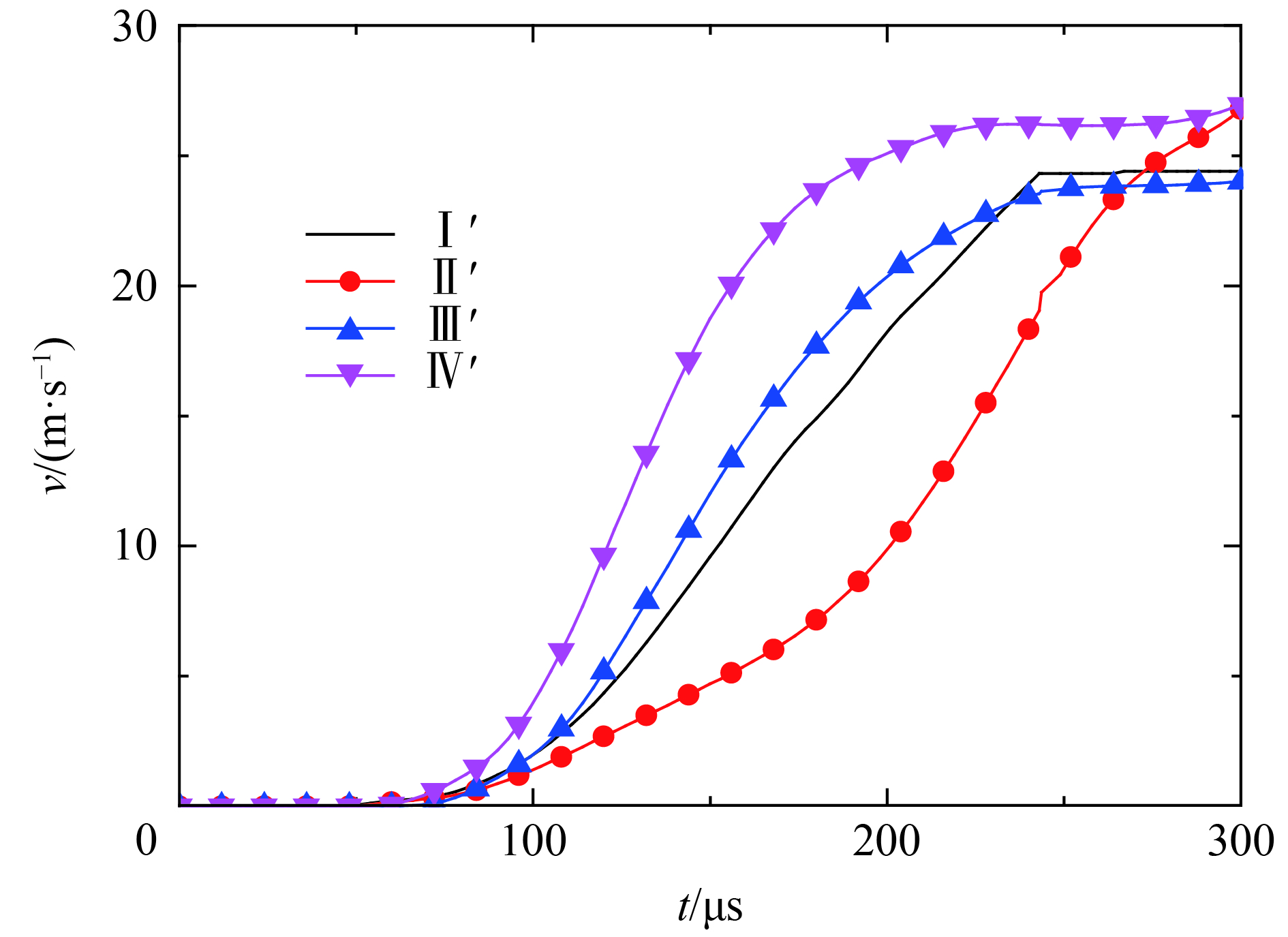
由图16和图17可知:采用工况IV′所示隔爆结构时,后级随进弹出现加速度峰值的时间最早,且峰值最大;工况I′所示隔爆结构中,后级随进弹的加速度峰值最小;工况IV′和工况III′出现加速度峰值的时间基本一致;工况II′所示结构延缓了冲击波作用到后级随进弹的时间,出现加速度峰值的时间最晚。由图17可知,采用以上所述4种隔爆结构时,后级随进弹速度增加到一定值时将不再增加。
4.3 改进结构的隔爆能力总结
总结改进后的工况:采用工况I′对应的隔爆结构时,后端装药压力峰值最小,仅为114.8 MPa;受质量成本的限制,工况II′中将介质C换成泡沫铝,延缓了冲击波作用到达后级随进弹的时间。但是在工况I′和工况II′对应的隔爆结构中,由于外层硬质钢挤压变形,使得后级随进战斗部壳体头部发生变形,进入塑性变形区;工况IV′与工况III′相比,介质C对应弹性涂覆体聚脲的厚度减薄,装药压力较工况III′所示结构有所提高,但增幅不大,后端壳体应力峰值的变化不大。
5. 结 论
采用数值模拟方法研究不同介质组合衰减冲击波的能力,通过对比后级弹壳体应力峰值及装药压力峰值,考察不同介质组合的隔爆能力,得出以下结论:(1)前级中空结构装药起爆后,爆炸冲击波首先向后级随进弹头靠里区域汇聚,而不是向弹头尖端区域汇聚;(2)在“硬软硬”隔爆结构中,将外层介质A由密度较大的硬质钢改为金属铝后,后级弹壳体应力峰值的变化很小,故确定隔爆结构中外层金属介质为铝,从而大大节约质量成本;(3)“硬软”两层组合介质隔爆结构中,铝-聚脲的隔爆能力优于铝-泡沫铝结构,最终确定“软”隔爆介质为聚脲,但聚脲的喷涂厚度有限,需要进一步减薄聚脲层的厚度;(4)改进结构中,减薄了外层金属介质层尖端的厚度,若保持隔爆层厚度不变,通过增加金属铝的厚度降低聚脲层的厚度,最终确定聚脲层的厚度为2 cm,从而既节约质量和体积成本,又具有优良的隔爆性能,能够充分削弱作用在后级随进弹上的冲击波强度,使后级随进弹的加速度和速度较小。
-
表 1 装药参数
Table 1. Charge parameters
ρ/(g·cm−3) R/mm L/mm CFRP thickness/mm Thickness of particle layer/mm Inner case Outer case 1.69 20 50 2 3 3 表 2 试验结果
Table 2. Experimental results
No. Distance Measured velocity/(m·s–1) Fixed velocity/(m·s–1) 1 37.5d 705 757 2 40.0d 685 743 3 62.5d 635 697 表 3 钨颗粒参数设置
Table 3. Parameters of tungsten particle
ρ/(g·cm–3) G/GPa A/GPa B/GPa N C M TM/K TR/K ˙ε0/s–1 cp/(J·g-1·K-1) 17.6 250 1.37 1.51×10–2 0.12 0.016 1.0 1 498 294 1.0 1.35×10–3 PC Spall IT D1 D2 D3 D4 D5 c0/(m·s–1) S1 γ0 –1.75 3.0 0.0 2.0 1.77 –3.4 0 0 3 800 1.44 1.58 表 4 空气的状态方程参数
Table 4. Equation-of-state parameters of air
ρ/(g·cm–3) C0 C1 C2 C3 C4 C5 C6 1.293×10–3 0 0 0 0 0.4 0.4 0 表 5 炸药的JWL状态方程参数
Table 5. JWL equation-of-state parameters of explosive
ρ/(g·cm–3) AE/GPa BE/GPa R1 R2 ω pCJ/GPa DCJ/(m·s–1) e0/GPa 1.69 669.9 12.901 4.3 1.2 0.3 36 8 600 10 表 6 炸药爆炸驱动不同颗粒的初速
Table 6. Maximum driving speed of different particles
Granularity/mm ρ/(g·cm–3) vmax/(m·s–1) Average velocity/(m·s–1) 0.2 17.6 1 249 1 060 0.5 17.6 984 823 0.7 17.6 806 686 0.5 19.3 574 476 -
[1] 刘意. 高密度惰性金属炸药爆轰与粒子流形成过程研究[D]. 北京: 北京理工大学, 2015: 7–37.LIU Y. Detonation of dense inert metal explosive and the formation of particles flow [D]. Beijing: Beijing Institute of Technology, 2015: 7–37. [2] FROST D L, ORNTHANALAI C, ZAREI Z, et al. Particle momentum effects from the detonation of heterogeneous explosives [J]. Journal of Applied Physics, 2007, 101(11): 113529. doi: 10.1063/1.2743912 [3] GARDNER D R. Near-field dispersal modeling for liquid fuel-air explosives: SAND-90-0686 [R]. USA: New Mexico, Sandia National Laboratories, 1990. [4] GLASS M W. Far-field dispersal modeling for liquid fuel-air explosive: SAND90-0687 [R]. USA: New Mexico, Sandia National Laboratories, 1990. [5] BORISOV A A. Research on the explosive dispersion of FAE [R]. Bulawayo, Naweed Zaman, 1996. [6] ZHANG F, FROST D L, THIBAULT P A, et al. Explosive dispersal of solid particles [J]. Shock Waves, 2001, 10(6): 431–443. doi: 10.1007/PL00004050 [7] 申超. 重金属粉末嵌层CFRP壳体内爆下低附带毁伤特性表征[D]. 北京: 北京理工大学, 2015: 9–22.SHEN C. The low collateral damage characterization of CFRP shell structure with heavy mental powder embedded as a layer under implosion [D]. Beijing: Beijing Institute of Technology, 2015: 9–22. [8] 白春华, 陈亚红, 李建平, 等. 爆炸抛撒金属颗粒群的装药方式 [J]. 爆炸与冲击, 2010, 30(6): 652–657. doi: 10.11883/1001-1455(2010)06-0652-06BAI C H, CHEN Y H, LI J P, et al. Charge forms for explosion dispersal of metal particles [J]. Explosion and Shock Waves, 2010, 30(6): 652–657. doi: 10.11883/1001-1455(2010)06-0652-06 [9] CUNDALL P A. A computer model for simulating progress, large scale movements in blocky rock systems [C]//Proceedings of the Symposium of the International Society for Rock Mechanics. Nancy, France, 1971. [10] CUNDALL P A, STRACK O D L. Discussion: a discrete numerical model for granular assemblies [J]. Géotechnique, 1980, 30(3): 331–336. doi: 10.1680/geot.1980.30.3.331 [11] LIU T, FLECK N A, WADLEY H N G, et al. The impact of sand slugs against beams and plates: coupled discrete particle/finite element simulations [J]. Journal of the Mechanics and Physics of Solids, 2013(61): 1798–1821. [12] OWEN P J, CLEARY P W. Prediction of screw conveyor performance using the discrete element method (DEM) [J]. Powder Technology, 2009, 193(3): 274–288. doi: 10.1016/j.powtec.2009.03.012 [13] SAWAMOTO Y, TSUBOTA H, KASAI Y, et al. Analytical studies on local damage to reinforced concrete structures under impact loading by discrete element method [J]. Nuclear Engineering and Design, 1998, 179(2): 157–177. doi: 10.1016/S0029-5493(97)00268-9 [14] CLEARY P W. Industrial particle flow modelling using discrete element method [J]. Engineering Computations, 2009, 26(6): 698–743. doi: 10.1108/02644400910975487 [15] 薛琨, 许俊彪, 白春华. 爆炸驱动颗粒射流形成与演化的试验研究 [J]. 振动与冲击, 2014, 33(7): 126–132.XUE K, XU J B, BAI C H. Tests for formation and evolement of particle jets driven by and explosion [J]. Journal of Vibration and Shock, 2014, 33(7): 126–132. [16] 宋玉江, 周涛, 沈飞, 等. 双层预制破片爆炸驱动早期行为研究 [J]. 火炸药学报, 2018, 41(3): 308–313.SONG Y J, ZHOU T, SHEN F, et al. Research on the behavior of initial stage about explosively-driven double-layered premade fragments [J]. Chinese Journal of Explosives & Propellants, 2018, 41(3): 308–313. [17] 黄长强, 朱鹤松. 球形破片对靶板极限穿透速度公式的建立 [J]. 弹箭与制导学报, 1993(2): 58–61.HUANG C Q, ZHU H S. Establishment of the formula for the ultimate penetration speed of spherical fragments on the target plate [J]. Journal of Projectiles and Guides, 1993(2): 58–61. [18] 熊冉, 高欣宝, 许兴春, 等. 破片侵彻金属薄板后的剩余速度研究 [J]. 爆破, 2013, 30(4): 41–44. doi: 10.3963/j.issn.1001-487X.2013.04.009XIONG R, GAO X B, XU X C, et al. Research on residual velocity of fragment after penetrating metallic sheet [J]. Blasting, 2013, 30(4): 41–44. doi: 10.3963/j.issn.1001-487X.2013.04.009 [19] 倪妍. CFRP壳体战斗部低附带毁伤特征表征[D]. 北京: 北京理工大学, 2014: 30–56.NI Y. Characterization of low incidental damage of CFRP shell warhead [D]. Beijing: Beijing Institute of Technology, 2014: 30–56. [20] BABU V, KULKARNI K, KANKANALAPALLI S, et al. Sensitivity of particle size in discrete element method to particle gas method (DEM_PGM) coupling in underbody blast simulations [C]//Ravi Thyagarajan 14th International LS-DYNA Conference. USA: Sanjay, 2016: 191–212. [21] 楼建锋, 王政, 洪滔, 等. 钨合金杆侵彻半无限厚铝合金靶的数值研究 [J]. 高压物理学报, 2009, 23(1): 65–71. doi: 10.11858/gywlxb.2017.03.001LOU J F, WANG Z, HONG T, et al. Numerical study on penetration of seni-infinite aluminum-alloy targets by tungsten-alloy rod [J]. Chinese Journal of High Pressure Physics, 2009, 23(1): 65–71. doi: 10.11858/gywlxb.2017.03.001 [22] 张世林. 轴向预制破片战斗部破片飞散特性影响因素分析[D]. 太原: 中北大学, 2012: 35–46.ZHANG S L. Analyze on the factors of dispersion characteristic of axial prefabricated fragments [D]. Taiyuan: North University of China, 2012: 35–46. [23] 谭多望, 温殿英, 张忠斌, 等. 球形破片长距离飞行时速度衰减规律研究 [J]. 高压物理学报, 2002, 16(4): 271–275. doi: 10.3969/j.issn.1000-5773.2002.04.006TAN D W, WEN D Y, ZHANG Z B, et al. Long-distance flight performances of spherical fragments [J]. Chinese Journal of High Pressure Physics, 2002, 16(4): 271–275. doi: 10.3969/j.issn.1000-5773.2002.04.006 [24] 印立魁, 蒋建伟. 多层球形预制破片战斗部破片初速场的计算模型 [J]. 含能材料, 2014, 22(3): 300–305. doi: 10.3969/j.issn.1006-9941.2014.03.006YIN L K, JIANG J W. Calculation model of initial velocity on multilayered spherical fragments warhead [J]. Chinese Journal of Energetic Materials, 2014, 22(3): 300–305. doi: 10.3969/j.issn.1006-9941.2014.03.006 -








 下载:
下载:
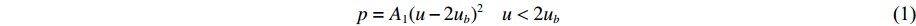

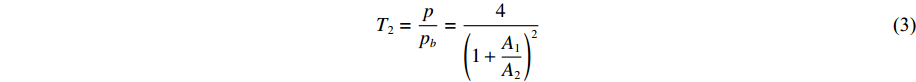
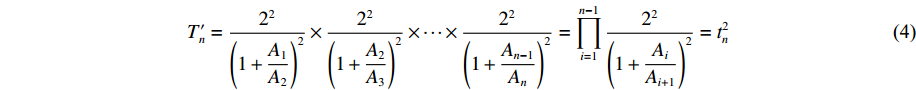
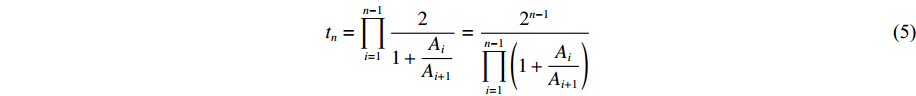
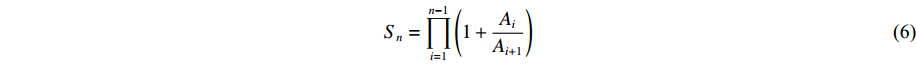






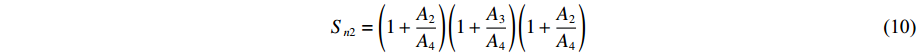


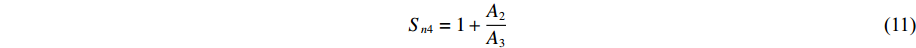

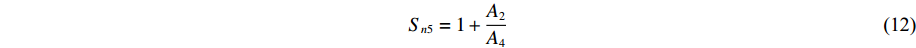




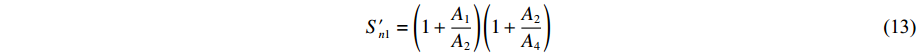

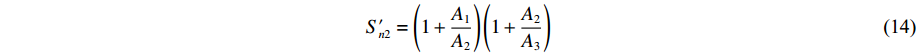






 下载:
下载: