Melting of MB2 Alloy under Shock Compression
-
摘要: 采用反向碰撞实验技术,结合具有高时空分辨率的全光纤激光干涉测速技术,对镁铝合金开展了极端动态压缩条件下的动力学行为实验研究,获得了镁铝合金在30~73 GPa压力范围内的Hugoniot和声速实验数据。深入的数据分析表明,所获得的Hugoniot数据与早期的Hugoniot数据一致,但是纵波声速却呈现出明显的向体波声速转变的趋势,对应冲击加载下镁铝合金的固-液熔化相变,相变压力区间为40~57 GPa。Abstract: Reverse impact experiments were performed at a shock pressure range from 30 GPa to 73 GPa to investigate the dynamic response of magnesium aluminum alloy. A displacement interferometer system for any reflector was employed to measure the impact velocity and sample/window interface particle velocities. The Hugoniot data obtained in this study had a good agreement with the data published before, however, the sound velocities extracted from our experiments showed a transition from longitudinal to bulk sound velocity. This discontinuity is attributed to melting transition under shock compression, and the transition pressure range is estimated from 40 GPa to 57 GPa.
-
Key words:
- shock compression /
- melting /
- sound velocity /
- magnesium aluminum alloy /
- phase transition
-
镁铝合金(MB2)作为一类特殊的合金材料,具有低密度、高强度、易机械加工、耐腐蚀等特点,广泛应用于车辆工程、航空航天等领域,其动态加载下的力学和物理特性对相关结构设计等具有重要意义。国内外对MB2合金的早期研究主要集中于力学特性方面,通过开展低压动态响应特性实验研究,获得了材料的弹塑性响应[1]、动态损伤[2]及层裂行为[3]的初步认识,近年来Millett等[4]通过一维冲击加载研究了MB2合金在早期变形和位错条件下随加载应力、加载脉宽而变化的弹塑性和剪切强度行为。另外,对动态加载下MB2合金的物态方程及相变研究已开展了一系列工作,主要集中于冲击Hugoniot数据测量[5],然而与动态加载下物理、力学特性紧密相关的相变研究尚处于起步阶段。声速作为应力扰动在材料中传播的定量表征参量,是获知材料动态响应特性(如相变、屈服强度、剪切模量)的主要途径之一[6–7],然而相关的研究工作却鲜见报道。
本研究拟采用反向碰撞实验技术[8–9],结合具有高时空分辨率的全光纤激光干涉测速技术DPS(Doppler Pin System)[10],对MB2合金开展30~73 GPa压力范围内的冲击Hugoniot及声速测量实验,并与早期实验数据进行对比验证,分析MB2合金的冲击熔化行为。
1. 实 验
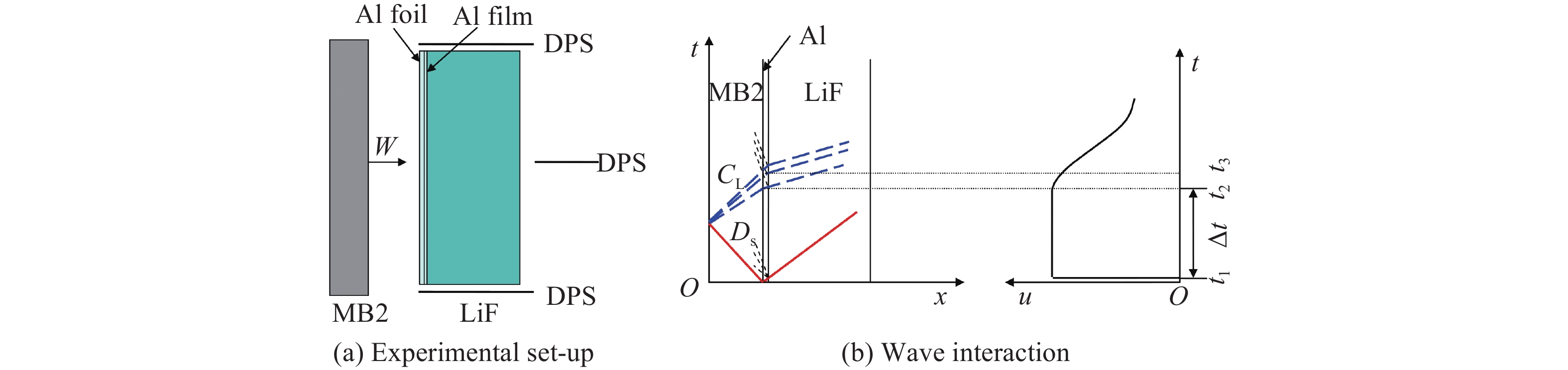
本研究涉及低压力区的声速测量,为此采用反向碰撞实验设计,即将样品材料制作成飞片,撞击LiF透明窗口,其原理如图1所示。在拉氏坐标下,飞片以速度W直接撞击窗口(t=t1),在飞片和窗口中分别产生左行冲击波和右行冲击波,引起飞片/窗口界面粒子速度的突跃。当飞片中的左行冲击波到达后界面时,将反射中心稀疏波,该稀疏波的波速就是材料在冲击压缩下的声速。如果样品材料发生冲击熔化,则中心稀疏波将以单一塑性波的形式在样品内传播,并在飞片/窗口界面处(t=t2)发生卸载,引起粒子速度的下降。如果样品材料没有发生冲击熔化,则中心稀疏波包括传播速度较快的弹性波和传播速度相对较慢的塑性波。当传播速度较快的弹性卸载波到达飞片/窗口界面时(t=t2),界面粒子速度下降,在速度剖面上形成第1个拐点;当塑性卸载波到达飞片/窗口界面时(t=t3),界面粒子速度再次突变,在速度剖面上形成第2个拐点;当后续稀疏波陆续到达飞片/窗口界面时,会导致界面粒子速度的连续下降;如果在卸载过程中样品材料发生相变,则也会在速度剖面上形成拐点。
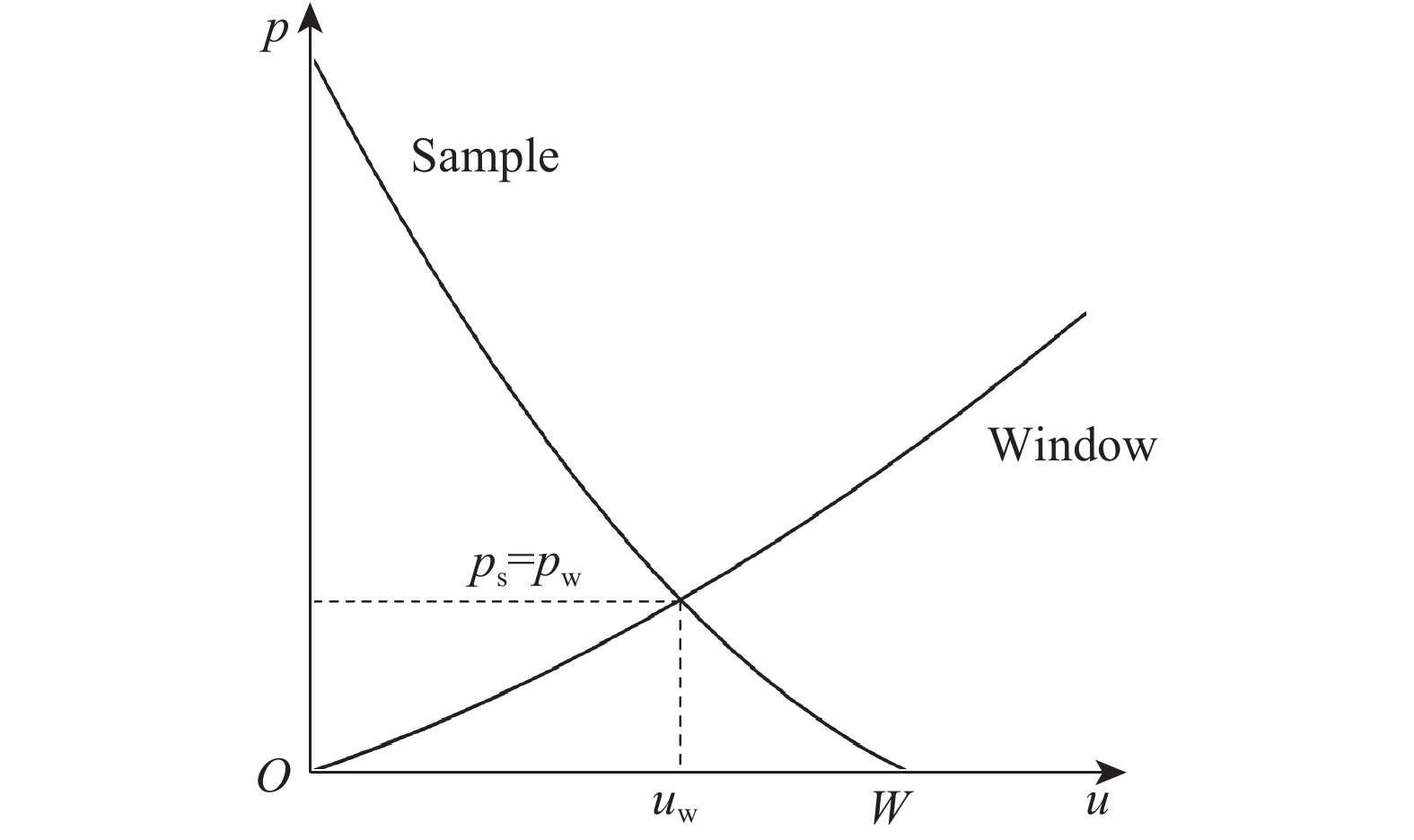
根据图2所示的界面连续性条件(其中p为冲击压力,u为粒子速度),结合Rankine-Hugoniot关系[11],可以得到样品内的冲击波速度Ds为
Ds=ρ0wDwuwρ0s(W−uw)(1) 式中:D、
ρ 、W分别为冲击波速度、密度和飞片速度,下标s和w分别对应样品和窗口。如果窗口材料的D-u曲线满足线性关系Dw=C0w+λwuw(2) 式中:C0w和
λw 为窗口材料的Hugoniot参数,则(1)式可表示为Ds=ρ0w(C0w+λwuw)uwρ0s(W−uw) (3) 因此,只需要测得飞片(样品)击靶速度W和窗口的波后粒子速度uw,就可以获得样品的粒子速度us(us=W–uw)和对应的冲击波速度Ds。随后,由波系作用(见图1(b))的几何关系可知,样品材料Hugoniot状态的拉格朗日纵波声速CL为
CL=DshsDst12−hs (4) 相应的欧拉纵波声速Cl为
Cl=DshsDst12−hsDs−usDs (5) 式中:hs为样品厚度,下标1和2对应波剖面上不同的时间点。
可以看到,反向碰撞法以波剖面测量为基础,波系作用简单,通过波剖面的粒子速度及时间信息得到高压声速,实验数据具有较高的精度,但是由于可供选择的窗口材料种类较少,目前使用的LiF窗口的阻抗较低,导致实验压力范围有限。
2. 实验结果与分析
实验在中国工程物理研究院流体物理研究所
∅ 30 mm二级轻气炮上进行。将MB2合金飞片安装在弹丸上,将弹丸发射至稳定的弹道速度W,并撞击LiF单晶窗口,通过DPS测量飞片击靶速度以及飞片/窗口界面粒子速度,获得待测材料的冲击波速度和声速。为了提高测试界面对DPS入射光的反射效率,避免靶室残留气体对测试的干扰,窗口击靶面上镀1μm 厚的铝膜并贴8μm 厚铝箔。LiF窗口折射率修正采用Rigg等[12]的公式uw=0.7895×u0.9918 (6) 式中:uw代表修正后的窗口界面粒子速度,u代表实测界面粒子速度,二者单位均为km/s。其中LiF密度为2.638 g/cm3,冲击波速度D和粒子速度u的关系为D=5.150+1.352u(单位km/s)[5]。
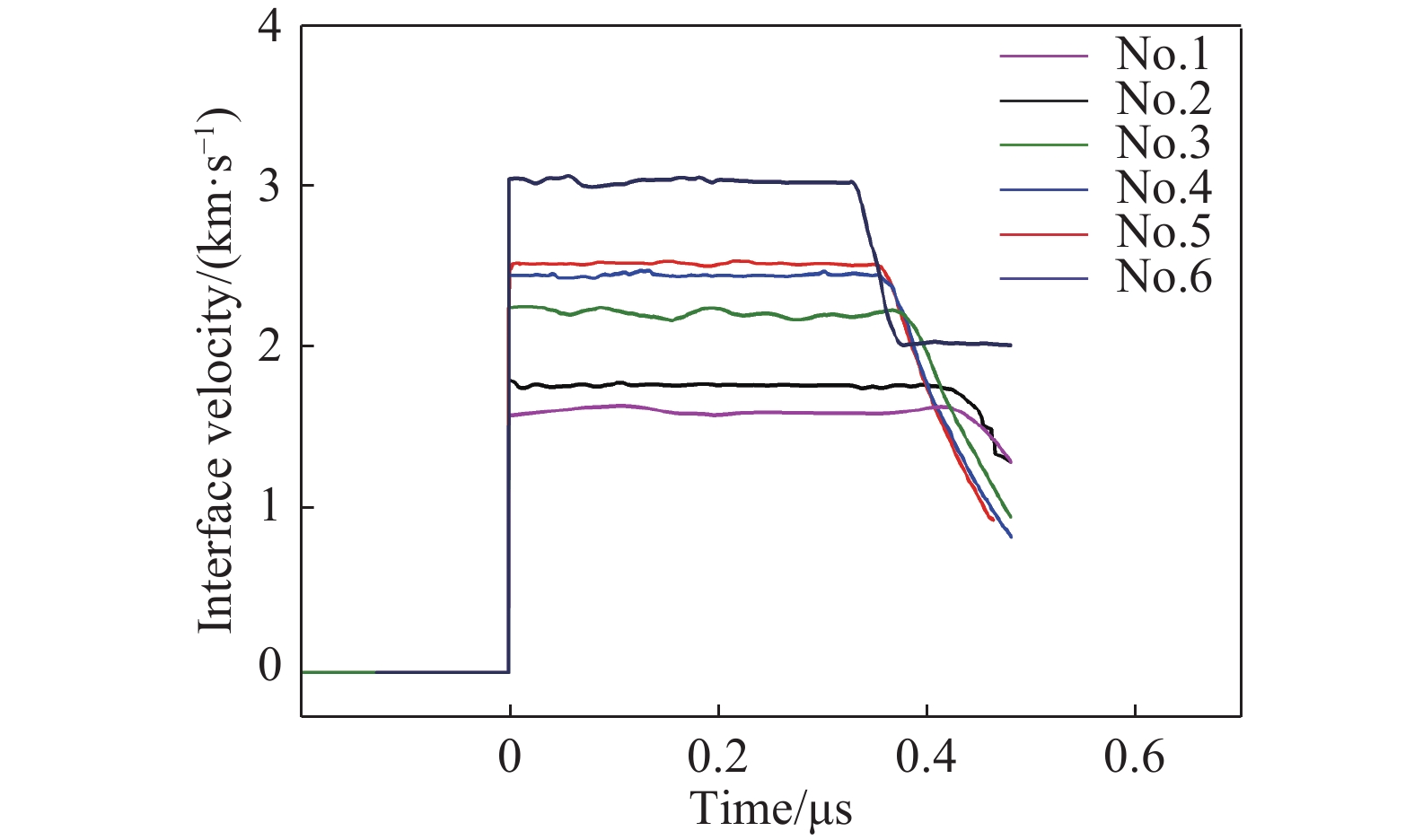
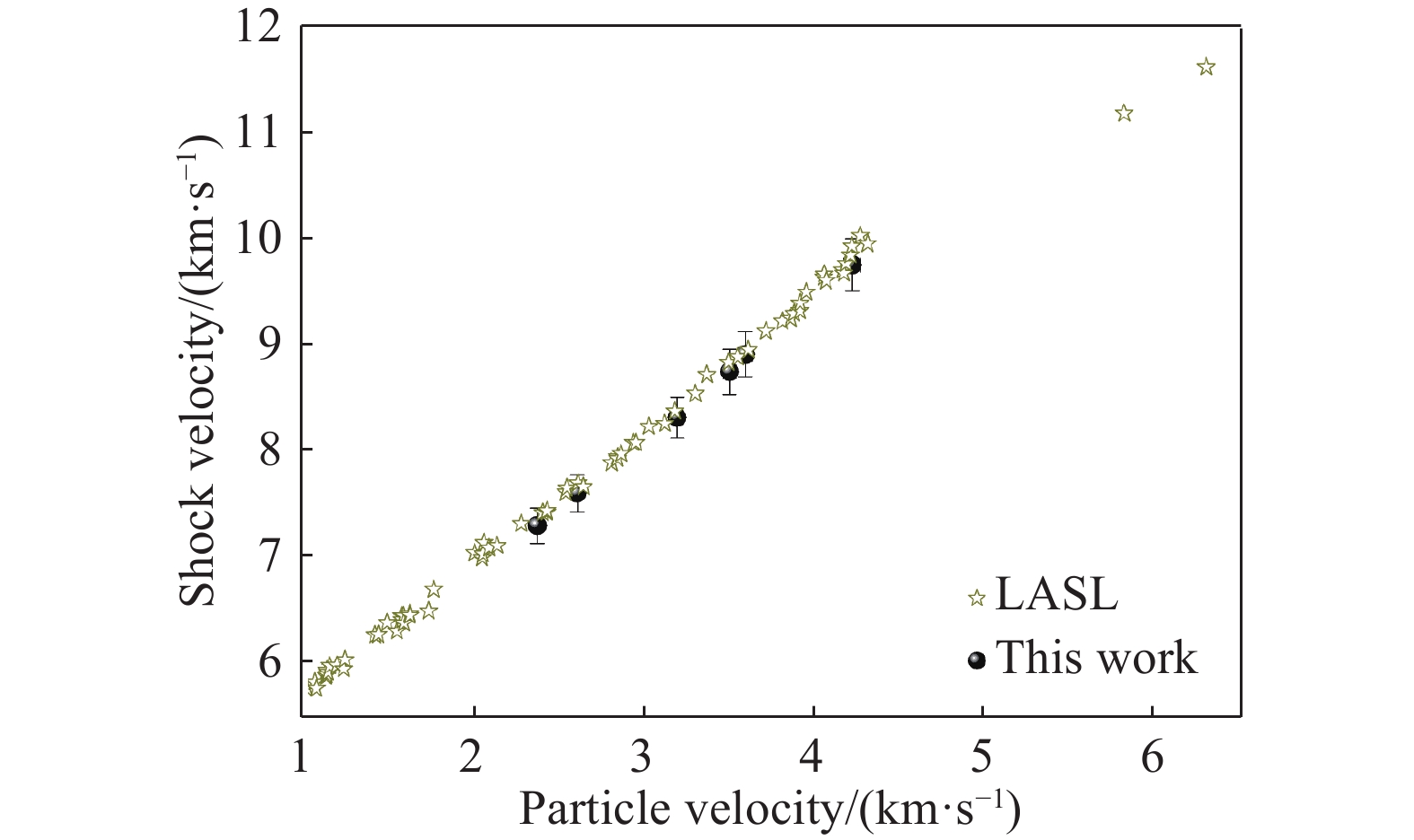
图3给出了6发实验测得的MB2/LiF界面粒子速度剖面(平台高度随加载压力的增大而升高)。通过界面粒子速度剖面,由(1)式~(6)式可得MB2样品在30~73 GPa冲击压力范围内的冲击波速度-粒子速度和声速-压力数据,实验结果列于表1,其中密度采用排水法测量,实测值为(1.775±0.004) g/cm3。冲击波速度-粒子速度数据如图4所示,图中还显示了美国洛斯阿拉莫斯国家实验室(LASL)发表的实验数据[5]。从图4中可以看出,本研究获得的实验数据与已有实验数据具有较好的一致性。
表 1 MB2样品冲击实验参数及结果Table 1. Shock experiment parameters and results of MB2Exp.No. hs/mm W/(km·s–1) uw/(km·s–1) us/(km·s–1) Ds/(km·s–1) p/GPa Cl/(km·s–1) 1 1.980±0.004 3.949±0.020 1.589±0.016 2.360±0.026 7.303±0.166 30.6±0.4 7.983±0.297 2 1.997±0.004 4.358±0.020 1.763±0.018 2.595±0.027 7.607±0.175 35.0±0.5 9.101±0.394 3 1.978±0.004 5.379±0.027 2.195±0.022 3.184±0.030 8.317±0.191 47.0±0.7 9.167±0.402 4 1.981±0.004 5.928±0.030 2.435±0.024 3.493±0.039 8.746±0.214 54.2±0.9 8.912±0.380 5 1.981±0.004 6.100±0.030 2.514±0.025 3.586±0.039 8.907±0.213 56.7±0.9 8.601±0.348 6 1.987±0.004 7.220±0.036 3.003±0.030 4.217±0.050 9.747±0.245 73.0±1.2 9.723±0.463 从图3可以看到:6发实验测得的界面粒子速度剖面质量良好,粒子速度剖面对应的冲击波、卸载波到达样品/窗口界面的特征信号清晰;加载压力为30.6和35.0 GPa时卸载剖面的弹-塑性特征明显,表明MB2合金在该冲击压力下尚未完全熔化;随着加载压力的升高,卸载剖面的弹-塑性特征逐渐消失,当加载压力达到73.0 GPa时,弹-塑性卸载特征完全消失,表明MB2已完全进入熔化相区,与图5所示的不同加载压力下声速转变特征一致。
如图5所示,当加载压力由30.6 GPa增加到35.0 GPa时,纵波声速逐渐增大,由7.983 km/s增大至9.101 km/s;但是,当加载压力增大至47.0 GPa时,纵波声速逐渐向体波声速偏转,跃变为9.167 km/s,预示着随着加载压力的升高,材料内部的剪切效应减小,冲击熔化发生;直至压力达到56.7 GPa时,纵波声速转变为体波声速,由此进一步确认了冲击加载下MB2合金发生熔化。Urtiew等[13]通过理论预测MB2合金在57 GPa附近开始熔化,该结果与本研究根据声速判定的冲击熔化区域基本一致,从而进一步证实了MB2合金在该压力范围内发生冲击熔化,只是Urtiew等预估的理论压力略高。图5中的实线是根据以下公式[11]计算得到的体波声速曲线
C2b=12V2(γV)HpH−V2dpHdV[1−12(γV)H(V0−VH)] (7) 式中:Cb为体波声速;
ρ 、γ 分别为密度和Grüneisen系数,ργ=ρ0γ0 ,ρ0 为材料初始密度,γ0 =1.43[14]。图5中的虚线是基于吴-经方程[15]计算得到的体波声速曲线。可以看出,理论计算的体波声速曲线较冲击加载实验值偏低约10%。这主要是由于合金材料自身成键及结合能的影响,难以采用传统的混合法则或物理模型准确计算物态方程的基本参数,如Grüneisen系数等,由此导致理论预测结果与实验结果出现差异(通常理论值偏低)。根据不确定度传递关系[16],当全部直接测量量(输入量)Yi彼此独立不相关时,由其确定的间接测量量z的合成不确定度
Δz 由下式确定Δz2=N∑i=1(∂f∂yi)2Δy2i (8) 式中:yi为输入量Yi的直接测量值,z为被测量的测量值,f为z和yi的函数关系,N为输入量的总个数,
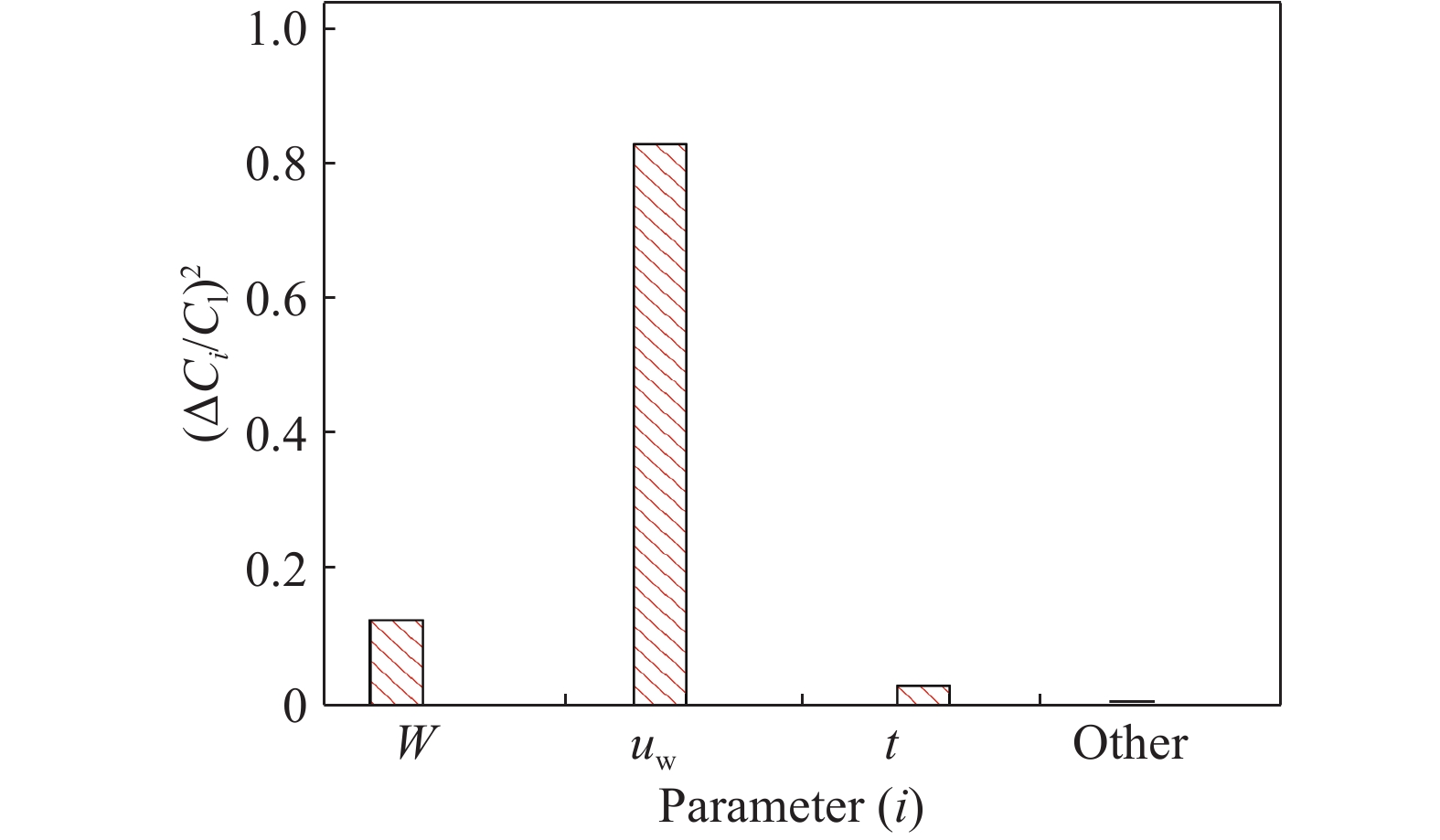
Δyi 为直接测量值的不确定度。因而,当密度为ρ0s 、厚度为hs 的飞片撞击窗口时,样品/窗口界面处粒子速度跳跃,实验测得飞片速度W 、界面粒子速度uw 、时间间隔(剖面平台)t12 ,相应的测量不确定度为Δρ0s 、Δhs 、ΔW 、Δuw 、Δt12 ,而窗口Hugoniot参数(ρ0w 、C0w 、λw )的不确定度(Δρ0w 、ΔC0w 、Δλw )已知。由于各测量量是独立测量的,(C0w ,λw )相互作用项较小,可忽略不计,据此可根据不确定度传递律确定声速测量的不确定度。如图6所示,就该例反碰撞实验(No.2)而言,影响声速测量不确定度的因素很多,包括样品初始密度、厚度、飞片速度、界面粒子速度、追赶时间、窗口材料冲击Hugoniot参数等。样品内部冲击压缩状态(如粒子速度、冲击波速度、冲击压力等)均通过飞片速度、界面粒子速度及窗口Hugoniot参数计算获得,影响声速测量不确定度的主要因素在于飞片速度、界面粒子速度及稀疏波追赶时间(平台时间),所占比例(即对(8)式中各平方项求和,下同)约为声速测量不确定度的99%,其余如初始密度、厚度等参量在当前诊断水平下对声速测量不确定度的贡献较小,约占总体的1%。在现有诊断条件下,飞片速度采用DPS直接测量,测量扩展不确定度不大于0.5%;界面粒子速度剖面的测量不确定度主要受平台区速度及稀疏波追赶时间的影响,影响因素包含干涉信号数据转换精度、窗口折射率修正、起跳及卸载时刻的判断等,综合而言,平台区速度测量的扩展不确定度不大于1%,追赶时间测量的扩展不确定度约6 ns。总体而言,传递至声速的测量扩展不确定度不超过5%。
3. 结 论
采用反向碰撞实验技术,结合具有高时空分辨率的DPS,获得了MB2合金在30~73 GPa压力范围内的冲击Hugoniot及声速数据。随着加载压力的升高,MB2合金纵波声速呈现出明显的向体波声速转变的趋势,预示着材料内部的剪切效应逐渐减小,冲击熔化发生,其相变压力区间为40~57 GPa。该实验结果与不同加载压力下卸载波剖面对应的弹-塑性转变特征完全一致,由此进一步确认了冲击加载下MB2合金熔化相变的发生。
感谢中国工程物理研究院流体物理研究所黄金、康强、叶素华、方茂林、向曜民、陈志云等在实验过程中给予帮助。
-
表 1 MB2样品冲击实验参数及结果
Table 1. Shock experiment parameters and results of MB2
Exp.No. hs/mm W/(km·s–1) uw/(km·s–1) us/(km·s–1) Ds/(km·s–1) p/GPa Cl/(km·s–1) 1 1.980±0.004 3.949±0.020 1.589±0.016 2.360±0.026 7.303±0.166 30.6±0.4 7.983±0.297 2 1.997±0.004 4.358±0.020 1.763±0.018 2.595±0.027 7.607±0.175 35.0±0.5 9.101±0.394 3 1.978±0.004 5.379±0.027 2.195±0.022 3.184±0.030 8.317±0.191 47.0±0.7 9.167±0.402 4 1.981±0.004 5.928±0.030 2.435±0.024 3.493±0.039 8.746±0.214 54.2±0.9 8.912±0.380 5 1.981±0.004 6.100±0.030 2.514±0.025 3.586±0.039 8.907±0.213 56.7±0.9 8.601±0.348 6 1.987±0.004 7.220±0.036 3.003±0.030 4.217±0.050 9.747±0.245 73.0±1.2 9.723±0.463 -
[1] GAO C Y, ZHANG L C, GUO W G, et al. Dynamic plasticity of AZ31 magnesium alloy: experimental investigation and constitutive modeling [J]. Materials Science and Engineering A, 2014, 613: 379–389. doi: 10.1016/j.msea.2014.06.112 [2] 胡昌明, 李英雷, 胡时胜, 等. 高温-高应变率下MB2合金的动态力学性能及变形机理 [J]. 兵器材料科学与工程, 2009, 32(5): 8–11 doi: 10.3969/j.issn.1004-244X.2009.05.003HU C M, LI Y L, HU S S, et al. Dynamic mechanical properties and deformation mechanical of MB2 alloy under high temperature and high strain rates [J]. Ordnance Material Science and Engineering, 2009, 32(5): 8–11 doi: 10.3969/j.issn.1004-244X.2009.05.003 [3] SCHMIDT R M, DAVIES F W, LEMPRIERE B M, et al. Temperature dependent spall threshold of four metal alloys [J]. Journal of Physics and Chemistry of Solids, 1978, 39(4): 375–385. doi: 10.1016/0022-3697(78)90079-3 [4] MILLETT J C F, STIRK S M, BOURNE N K, et al. On the behaviour of the magnesium alloy, AZ61 to one-dimensional shock loading [J]. Acta Materialia, 2010, 58(17): 5675–5682. doi: 10.1016/j.actamat.2010.06.042 [5] MARSH S P. LASL shock Hugoniot data [M]. Berkeley: University of California Press, 1980: 208. [6] YU Y Y, TAN H, HU J B, et al. Shear modulus of shock-compressed LY12 aluminium up to melting point [J]. Chinese Physics B, 2008, 17(1): 264–269. doi: 10.1088/1674-1056/17/1/046 [7] 胡建波, 谭华, 俞宇颖, 等. 铝的动态屈服强度测量及再加载弹性前驱波的形成机理分析 [J]. 物理学报, 2008, 57(1): 405–410 doi: 10.3321/j.issn:1000-3290.2008.01.063HU J B, TAN H, YU Y Y, et al. Measurements of dynamic yield strength of aluminum alloy and mechanism analysis of elastic precursor during reloading [J]. Acta Physica Sinica, 2008, 57(1): 405–410 doi: 10.3321/j.issn:1000-3290.2008.01.063 [8] 谭叶, 俞宇颖, 戴诚达, 等. 反向碰撞法测量Bi的低压Hugoniot数据 [J]. 物理学报, 2011, 60(10): 106401 doi: 10.7498/aps.60.106401TAN Y, YU Y Y, DAI C D, et al. Measurement of low-pressure Hugoniot data for bismuth with reverse-impact geometry [J]. Acta Physica Sinica, 2011, 60(10): 106401 doi: 10.7498/aps.60.106401 [9] 俞宇颖, 谭叶, 戴诚达, 等. 钒的高压声速测量 [J]. 物理学报, 2014, 63(2): 026202YU Y Y, TAN Y, DAI C D, et al. Sound velocities of vanadium under shock compression [J]. Acta Physica Sinica, 2014, 63(2): 026202 [10] WENG J D, TAN H, WANG X, et al. Optical-fiber interferometer for velocity measurements with picosecond resolution [J]. Applied Physics Letters, 2006, 89(11): 111101. doi: 10.1063/1.2335948 [11] 谭华. 实验冲击波物理导 [M]. 北京: 国防工业出版社, 2006: 5.TAN H. Introduction to experimental shock-wave physics [M]. Beijing: National Defense Industry Press, 2006: 5. [12] RIGG P A, KNUDSON M D, SCHARFF R J, et al. Determining the refractive index of shocked [J]. Journal of Applied Physics, 2014, 116(3): 033515. doi: 10.1063/1.4890714 [13] URTIEW P A, GROVER R. The melting temperature of magnesium under shock loading [J]. Journal of Applied Physics, 1977, 48(3): 1122–1126. doi: 10.1063/1.323789 [14] 徐锡申, 张万箱. 实用物态方程理论导引 [M]. 北京: 科学出版社, 1986: 527.XU X S, ZHANG W X. Introduction to equation of state theory [M]. Beijing: National Defense Industry Press, 1986: 527. [15] WU Q, JING F Q. Thermodynamic equation of state and application to Hugoniot predictions for porous materials [J]. Journal of Applied Physics, 1996, 80(8): 4343–4349. doi: 10.1063/1.363391 [16] 国家质量技术监督局计量司. 测量不确定度评定与表示指南 [M]. 北京: 中国计量出版社, 2000. -








 下载:
下载:

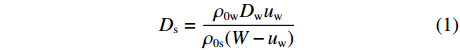
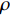
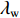
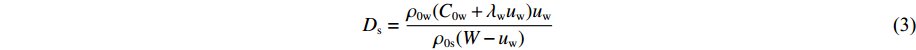
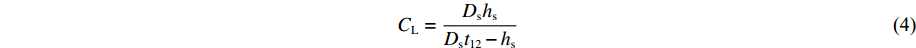

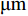
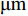
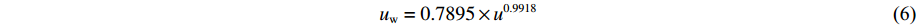



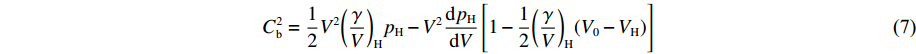
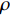
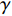
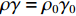
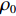
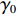

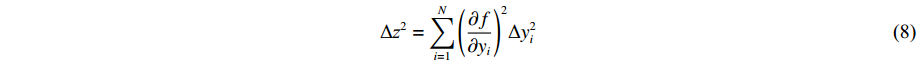
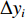
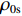
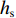
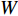
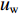
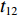
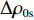
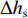
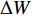

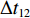
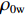
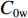
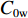
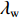
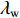
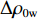
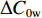
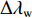


 下载:
下载: