Numerical Simulation on the Performance of Shaped Charge with Explosively Welded Aluminum Copper Liner
-
摘要: 为提高射流侵彻性能,根据聚能射流装置的射流形成特点,设计了爆炸复合铝铜金属体作为药型罩的聚能射流装置。此装置依据已有的锥角为42°的聚能装药紫铜药型罩改进而来。利用LS-DYNA软件中的MMALE多物质算法,对此装置的射流形成、侵彻金属靶体全过程进行数值模拟。在保持装药量不变的情况下,计算了当铝铜药型罩锥角分别为36°、38°、40°和42°时的射流形成及侵彻过程。结果表明:射流头部速度随着铝铜药型罩锥角的减小而增大;且锥角为38°时射流穿深最大。相比单纯金属铜药型罩情况,射流头部速度提高了13.2%,侵彻深度提高了14.5%。Abstract: According to the character of jet formation in shaped charge device, a new type of charge assembly, with metallic liner of aluminum-copper bond fabricated by explosively welding technique, has been proposed in order to acquire the improvement on penetration capability from such charge. The device is modified from the available conical shaped charge with single copper liner material and 42° conical apex angle. Multi-material arbitrary Lagrangian-Eulerian (MMALE) method in LS-DYNA software package is employed as the numerical simulation tool to fulfill the calculations for the whole processes involving jet formation and ensuing penetration into target. Charges with apex angles varying from 36°, 38°, 40°, and 42° respectively have been calculated for comparison. The results show that the head velocity of the jet increases with the decreasing value of apex angle. Furthermore, 38° apex angle charge reaches maximum penetration depth. Compared to shaped charge with single copper liner, such design of the charge presents 13.2% improvement in jet head velocity and 14.5% rising in penetration depth.
-
Key words:
- shaped charge /
- penetration /
- welded aluminum copper liner /
- LS-DYNA
-
聚能射流装置(中空炸药体内加薄壁金属件药型罩)引爆后,由爆轰产物压垮药型罩所形成的金属射流具有强大的穿透和毁伤能力[1]。此原理在军事上用于研发反装甲武器的破甲弹,在民用方面可应用于石油天然气开采中油气井套管打孔用射孔弹[2-5]。因此,如何提高聚能射流的侵彻性能成为一个重要的研究课题。
聚能射流装置主要由炸药和药型罩两部分构成,其中:炸药装药在性能提高和使用方面一直没有较大进展,而改进药型罩则成为可能提高射流性能的趋势。Dil"Din等[6]曾用机械法压制铝铜复合罩,进行射流侵彻性能实验,但复合罩的性能测试结果却只有纯铜罩的60%。Larocca等[7]的实验结果表明,与普通机械轧制罩相比,爆炸复合工艺制成的双层药型罩形成的射流稳定性大幅提高。臧涛成等[8]采用爆炸复合铝铜药型罩制成射孔弹,实验发现射流头部速度较纯铜药型罩提高了10%,但射流侵彻性能相差不大。郑宇等[9]通过AUTODYN软件对双层铝铜药型罩聚能射流装置进行了仿真研究,发现当铝铜罩的厚度比为1时侵彻性能最好,对靶体的平均侵深相较于纯铜药型罩提高了23.8%。乔金超等[10]通过LS-DYNA对大锥角线型成型装药不同金属双层药型罩射流的形成进行仿真计算,发现在装药结构不变的情况下,双层罩外层材料采用铝时侵彻性能较纯铜罩提高了16.6%。然而,目前通过数值模拟进行双层药型罩设计时,将单层药型罩变为双层药型罩后,药型罩微元压垮速度的变化均未考虑。
为此,本研究在一种传统纯铜药型罩聚能射流装置设计的基础上,提出了使用爆炸复合铝铜板作为药型罩材料,根据压垮速度情况,对药型罩锥角进行重新设计。通过LS-DYNA多物质ALE(MMALE)算法[11],对铝铜厚度比为1且药型罩具有不同锥角情况,进行射流形成、侵彻过程的数值模拟。
1. 计算模型和方法
1.1 计算模型
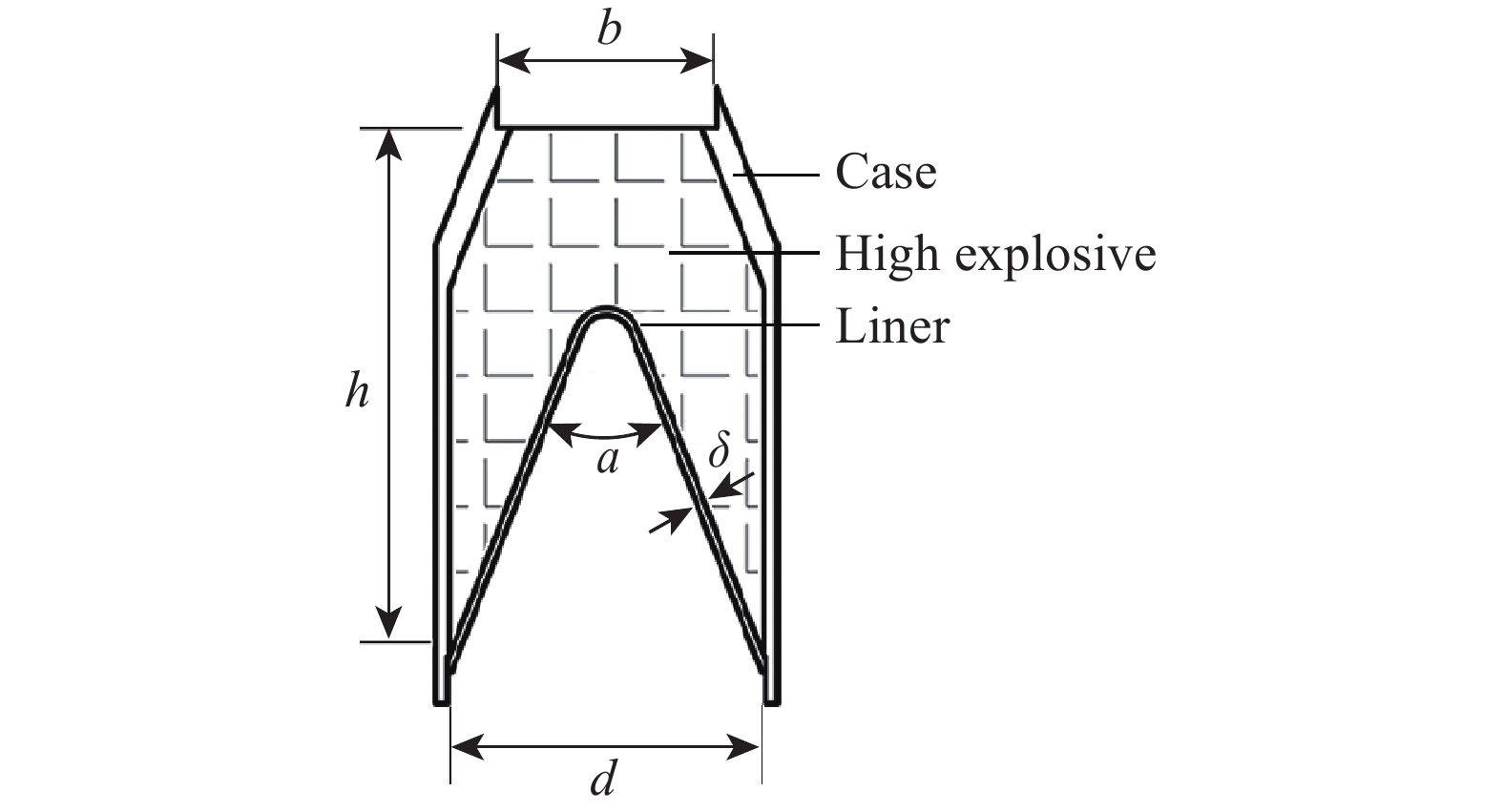
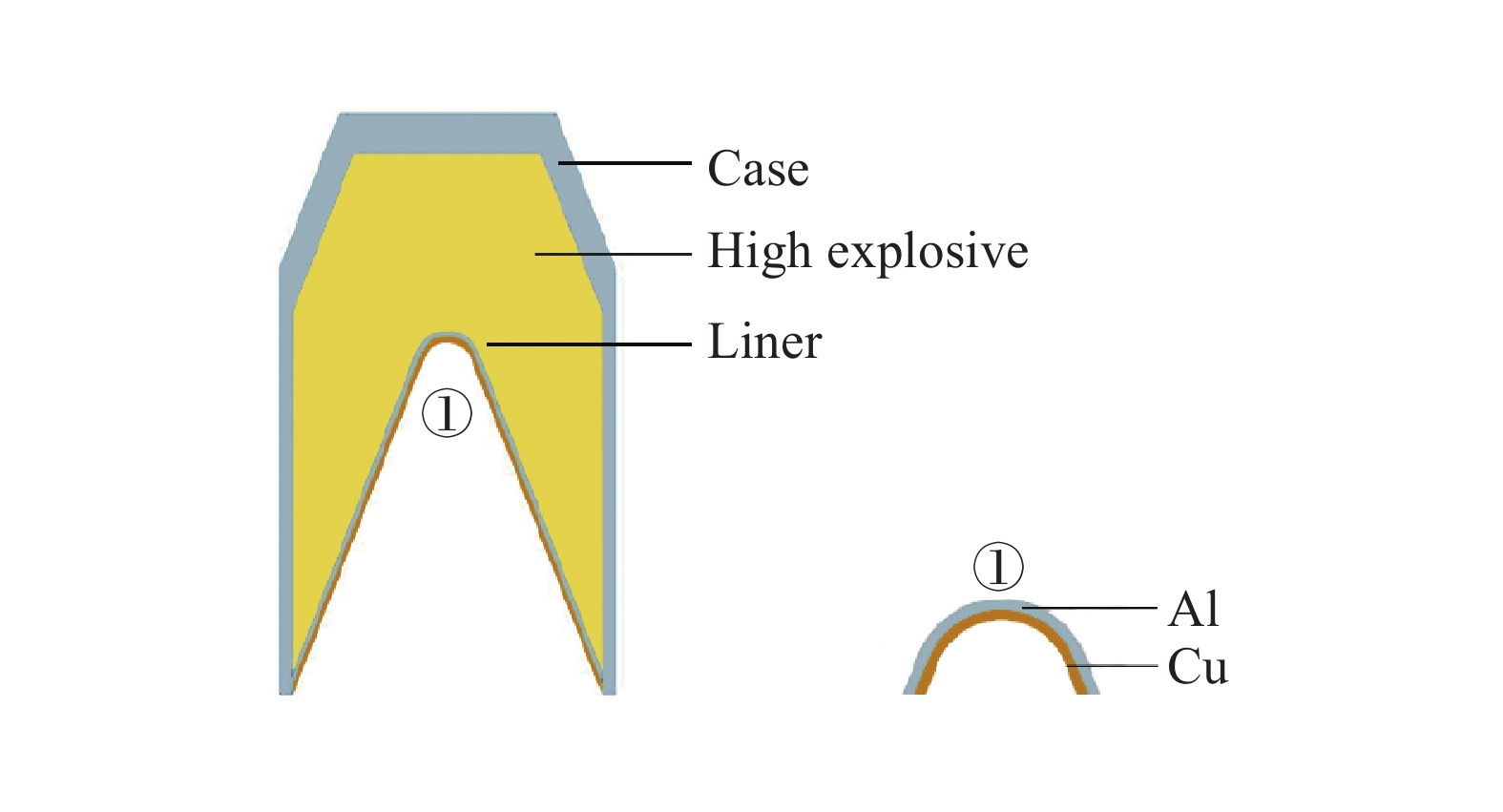
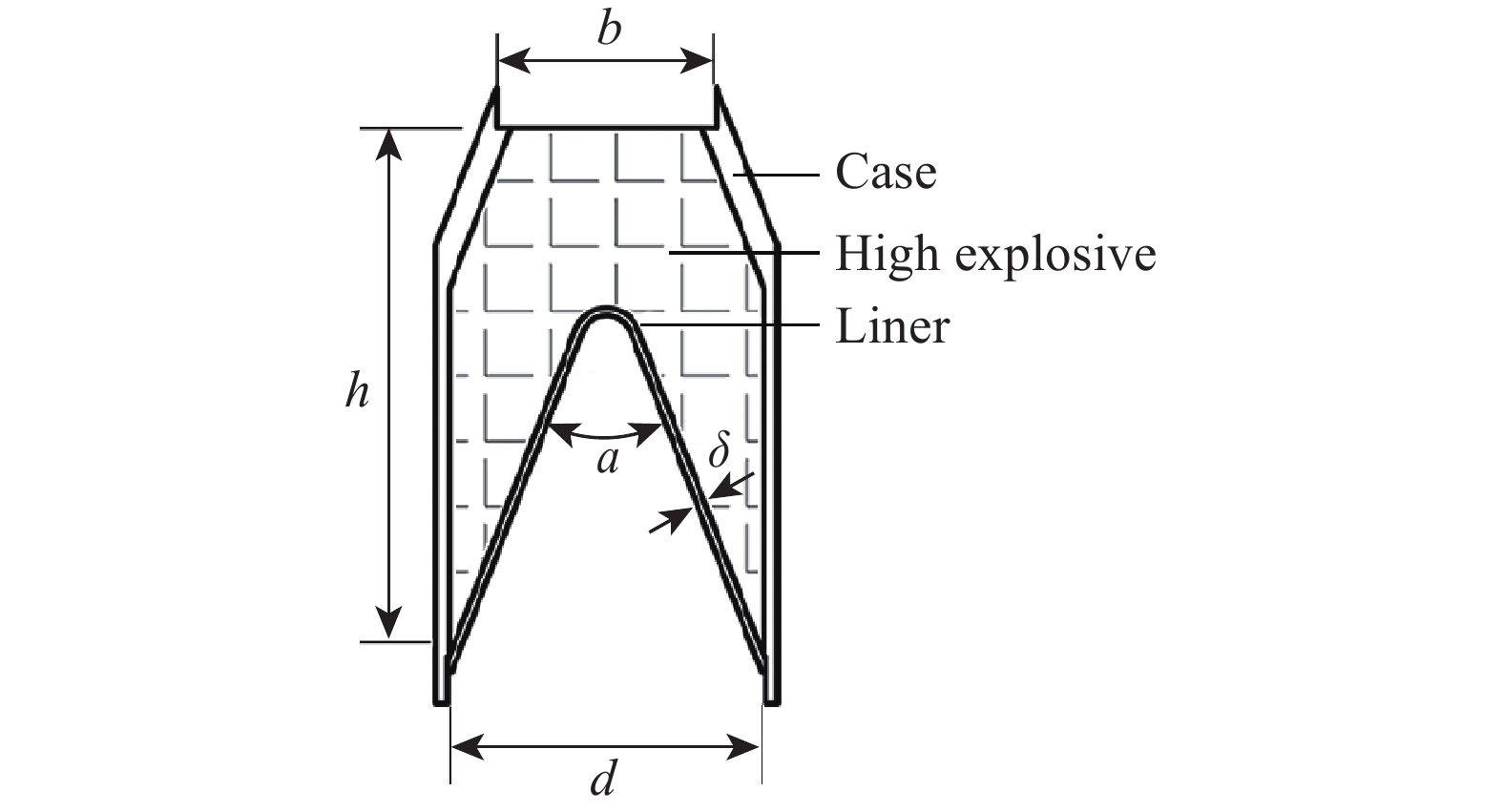
图1为传统聚能射流装置模型,由铝制壳体、Octol(HMX和TNT炸药的质量比为78∶22)炸药和紫铜药型罩3部分组成,具体几何参数如表1所示[12]。图2是铝铜复合体药型罩聚能射流装置模型。复合铝铜药型罩结构分为两层,靠近炸药一侧为铝层,另一侧为铜层,图中①为顶部区域标记。铝铜厚度比定为1,除药型罩变为双层外,其他几何参数均与经典装置一致。与经典聚能射流装置相比,复合药型罩由于其整体密度小,同等药量下的压垮速度增高,因此通过减小锥角从而使射流微元更多地向轴向汇聚。目前,将铝铜药型罩锥角依次设定为36°、38°、40°、42°共4种情况,比较复合药型罩与经典42°锥角纯铜药型罩的射流性能差异。
表 1 模型几何参数Table 1. Geometrical parameters in shaped charge configurationδ/cm h/cm d/cm α/(°) b/cm 0.21 15.24 8.38 42 5.86 Note: δ, h, d, α, b are liner thickness, height of charge, diameter, apex angle, and top diameter, respectively. 1.2 计算设定
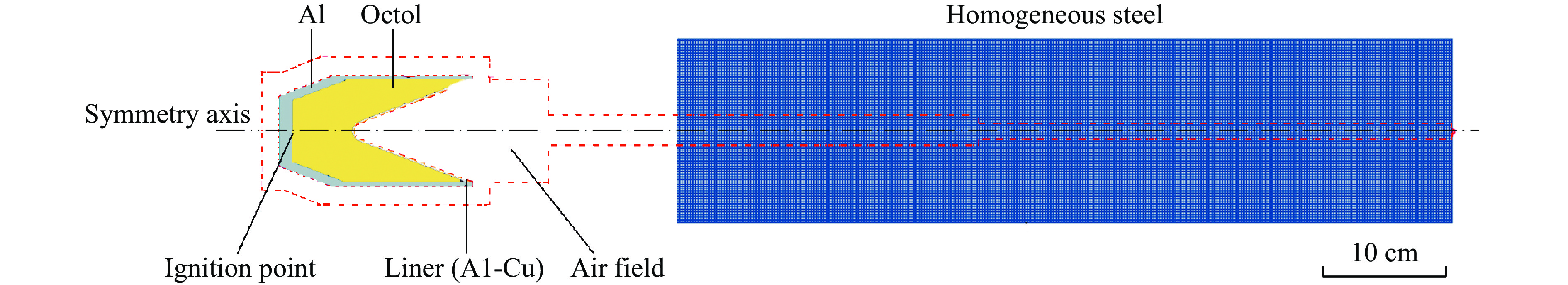
图3给出了聚能射流装置及靶体的完整分布模型截面图,其中靶体横截面直径为15.24 cm,高度为62.87 cm,射流装置与靶体的间距为16.76 cm。由于装置结构和加载载荷具有几何对称性,在实际计算过程中,取轴线上下半部截面其一计算即可。在边界施加固定约束边界条件。
外壳、炸药和药型罩以及空气域采用尺寸为0.05 cm的网格划分,其中对于局部不规则几何部分,网格尺寸自适应调整。靶体的网格尺寸取0.1 cm,均采用非渐变网格以提高计算的稳定性。图3中红色虚线所示为空气域,主要考虑物质流动需要几何空间而增加。靶体物质采用拉格朗日算法,聚能射流装置及空气域部分采用多物质ALE算法,金属射流和靶体之间为流固耦合接触。起爆点设在装药对称轴顶点,爆轰传播计算采用Programmed Burn模式。
1.3 状态方程及材料模型
炸药爆轰产物采用Jones-Wilkins-Lee(JWL)状态方程[13]描述。Octol炸药的CJ爆轰性能参数[14]和JWL状态方程参数[14]列于表2。射流装置中的金属材料采用Grüneisen状态方程[15]描述,相关的物性参数[16]列于表3。金属材料强度模型采用Steinberg模型,相关参数列于表4[17]。考虑到侵彻过程中的变形特征,对靶体材料采用运动硬化弹塑性模型[18]模拟,关联参数列在表5[19]。计算中,当靶体单元中即时等效应变超过材料本身等效失效应变时,删除此单元,空间由空气填充处理。
ρ0/(g·cm–3) D/(km·s–1) pCJ/GPa E0/(J·m–3) ω 1.821 8.48 34.2 9.6 0.38 A/GPa B/GPa C/GPa R1 R2 748.6 13.38 1.167 4.50 1.20 Note: ω, A, B, C, R1 and R2 are JWL EOS parameters; ρ0, D, pCJ and E0 are density, detonation velocity, CJ presure, and explosive energy per volume, respectively. 表 3 铝和铜的Grüneisen状态方程参数[16]Table 3. Parameters in Grüneisen equation of state of aluminum and copper[16]Material ρ0/(g·cm–3) C0/(km·s–1) S Γ Troom/K cv/(J·kg–1·K–1) Aluminum 2.78 5.39 1.339 1.97 300 884 Copper 8.93 3.94 1.489 2.02 300 383 Note: S is constant; C0, Γ, Troom, cv are sound velocity, Grüneisen coefficient, room temperature, and specific heat capacity at constant volume, respectively. 表 4 铝和铜材料的Steinberg强度模型参数[17]Table 4. Parameters in Steinberg strength model of aluminum and copper[17]Material ρ0/(g·cm–3) G0/GPa Y0/GPa β n (−G′r/G0)×103/K–1 Aluminum 2.78 27.6 0.29 125 0.10 0.62 Copper 8.93 47.7 0.12 36 0.45 0.38 Note: β and n are constants; G0, Y0 and are shear modulus, yield strength, and G′r shear modulus per time derivative,respectively. 表 5 钢靶弹塑性随动硬化模型参数[19]Table 5. Target material parameters in elastic-plastic-kinematic strength model[19]Material ρ0/(g·cm–3) Ep/GPa μ Y/GPa Ce Pe/s–1 ɛeff Steel 7.83 2.07 0.3 0.011 1 6 500 4 0.7 Note: Pe, Ce and ɛeff are constants; Ep, μ and Y are platic modulus, Poisson’s ratio and yield strength, respectively. 2. 数值模拟结果和分析
2.1 纯铜药型罩的射流形成及侵彻
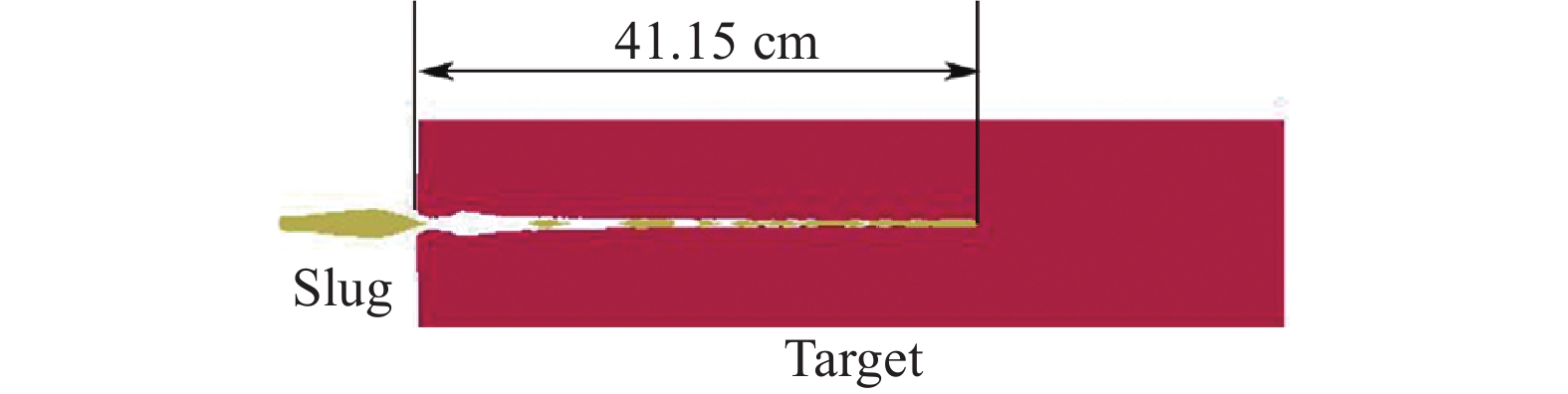
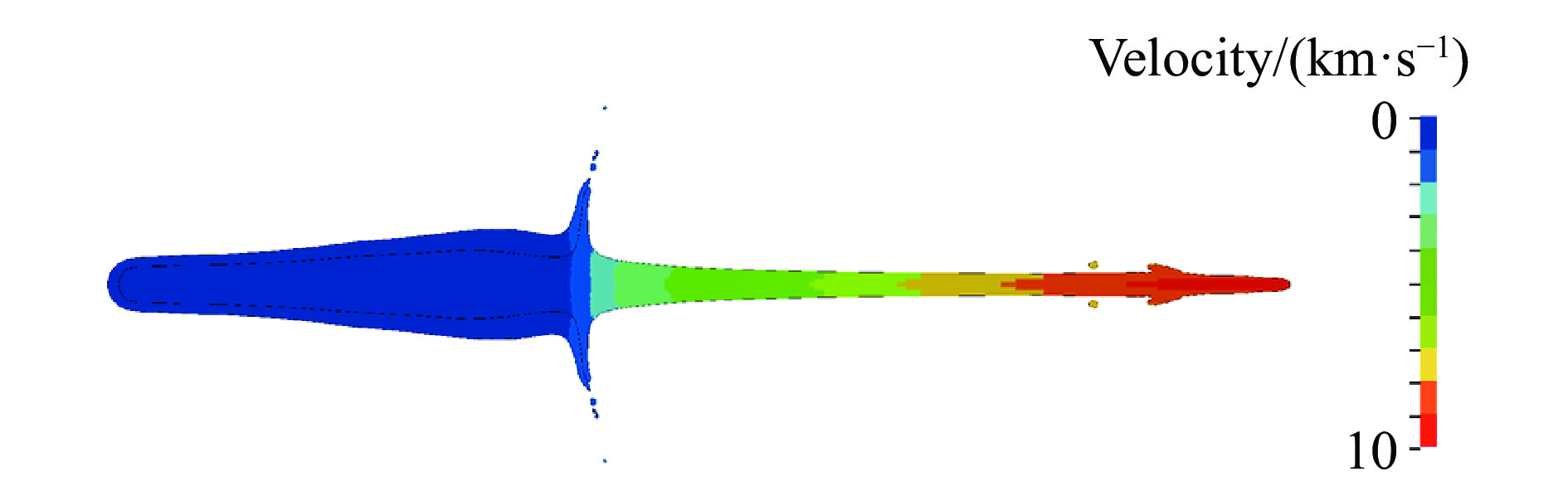
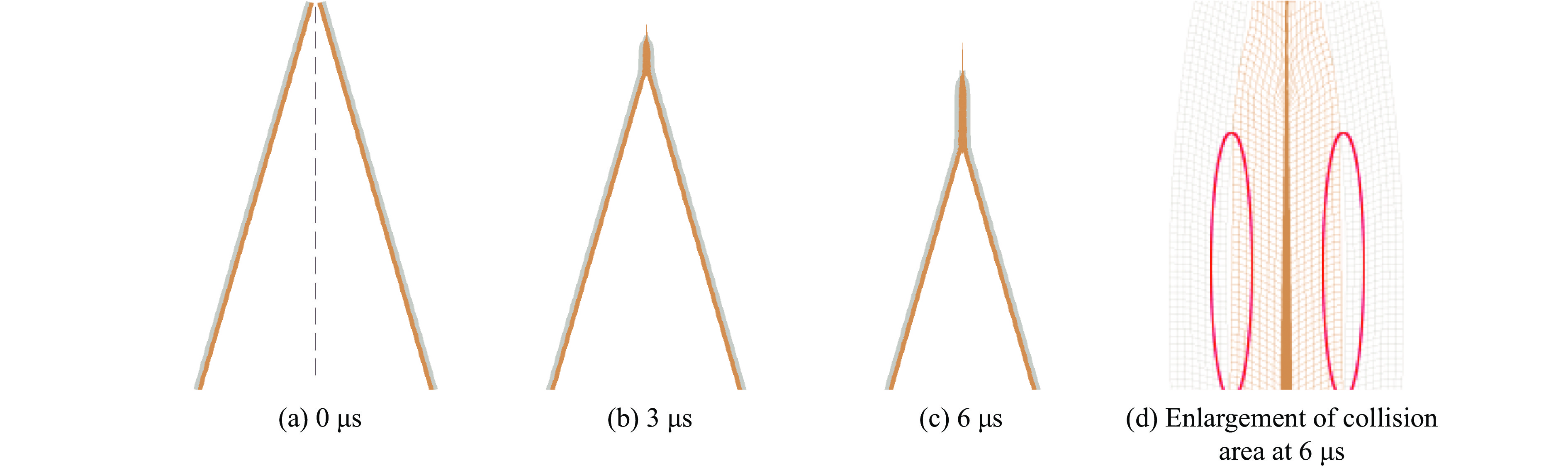
在进行改进型聚能射流装置计算前,开展了42°锥角纯铜药型罩聚能射流装置的射流形成和侵彻的计算,结果见表6。图4给出了射流的形成过程,图5给出了侵彻过程的变化。装药起爆后7 μs时,药型罩被压垮,开始形成射流;30 μs后射流头部速度开始趋于稳定,此时头部速度可达到8.23 km/s,与文献[12]中给出的实验值8.30 km/s基本吻合。同时计算获得的射流对靶体的总侵彻深度为41.15 cm,而文献[12]中的实验侵彻深度在38.56~40.23 cm范围内变化,计算值稍大于实验值。
表 6 纯铜药型罩射流侵彻计算和实验结果对比Table 6. Comparison on computational and experimental results of jet and penetration by charge with single copper linerJet head velocity/(km·s–1) Penetration depth/cm Experiment This calculation Experiment This calculation 8.30 8.23 38.58–40.23 41.15 2.2 不同锥角铝铜药型罩射流头部侵彻速度
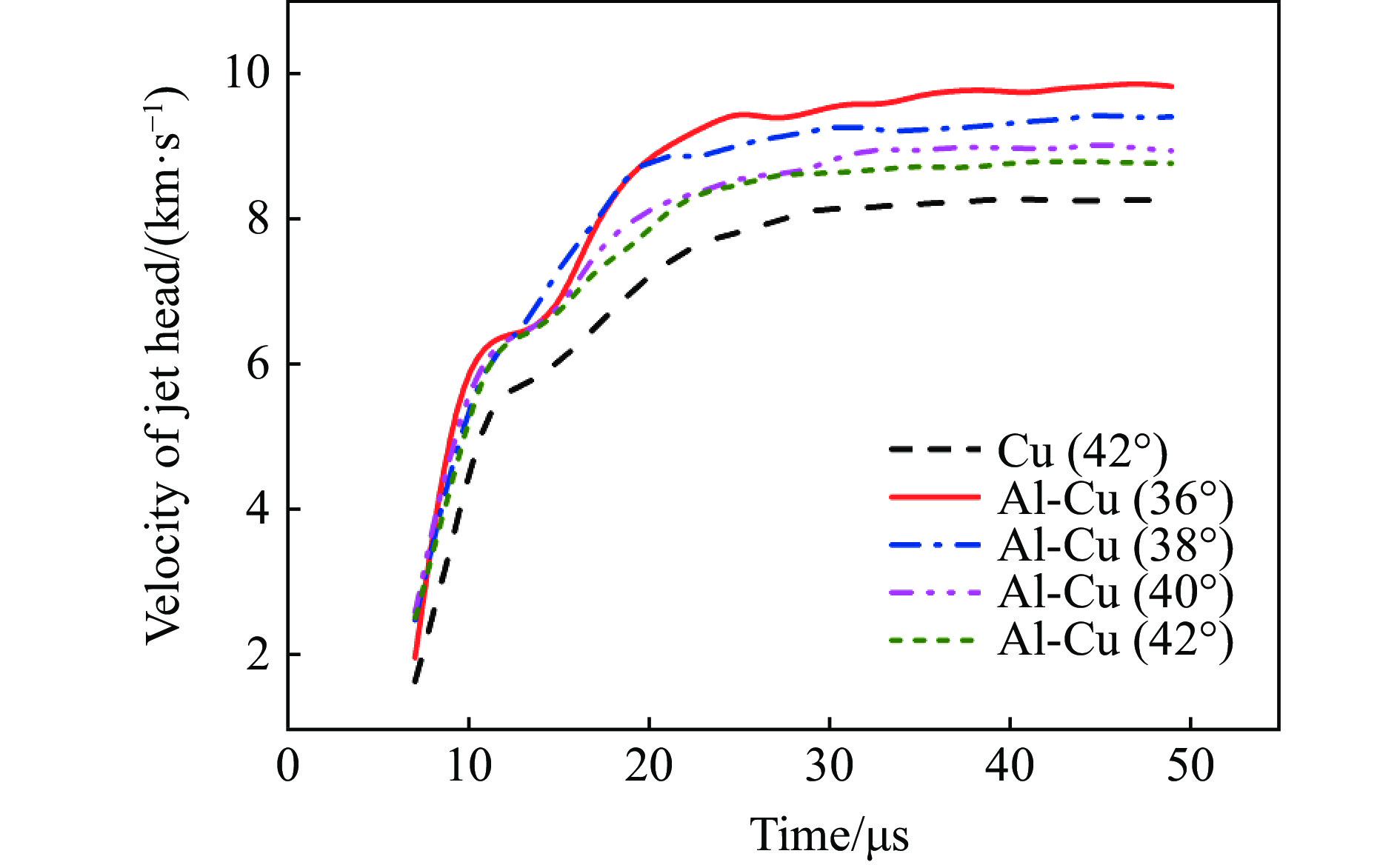
对于铝铜药型罩,在锥角分别为36°、38°、40°、42°的条件下,开展射流形成过程计算,得到不同锥角下射流头部速度。图6给出了相应射流头部侵彻速度-时间变化曲线,同时给出42°纯铜药型罩射流头部的侵彻速度变化。可以看出,4种不同锥角的铝铜药型罩均比传统42°纯铜药型罩头部速度高,且头部速度随着药型罩锥角的减小而增大。其中,36°铝铜药型罩射流头部侵彻速度最高,达到9.74 km/s,相比纯铜药型罩提高了18.3%;而38°铝铜药型罩射流头部侵彻速度为9.31 km/s,相比纯铜药型罩提高了13.2%。
2.3 不同锥角铝铜药型罩射流侵彻深度
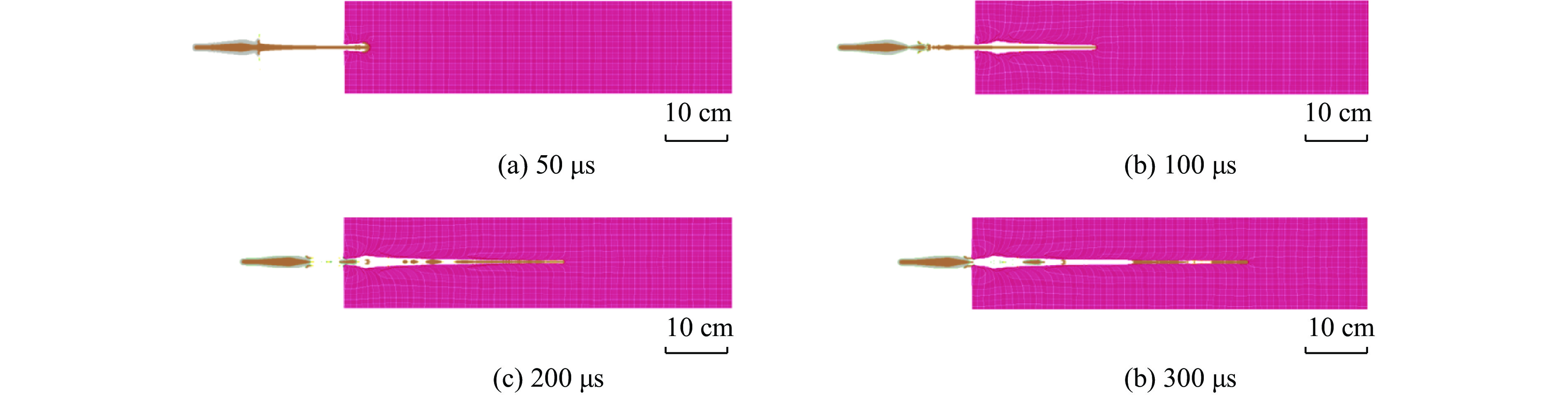
不同锥角铝铜药型罩射流对靶体侵彻过程的计算表明:在保持锥角42°不变时,直接将纯铜药型罩换为铝铜复合罩后,其射流侵彻深度反而下降;而当改变成小锥角时,侵彻深度将会提高,结果在表7中给出。这说明药型罩材质的改变导致压垮速度和压垮角改变,原有纯铜药型罩的锥角并不能使铝铜药型罩射流达到更好的侵彻效果。虽然在2.2节中得到36°药型罩射流具有最大头部速度,但是在侵彻深度方面的表现并不是最好;而锥角为38°时的铝铜药型罩具有最好的射流侵彻性能,侵彻深度可达47.10 cm,相比纯铜药型罩提高了14.5%。图7给出了计算得到的38°锥角铝铜药型罩射流侵彻靶体的过程。
表 7 不同锥角铝铜药型罩与纯铜药型罩射流计算结果对比Table 7. Computational results on penetration by aluminum-copper liner with various apex angelsMaterial α/(°) Penetration depth/cm Cu 42° 41.15 Al-Cu 42° 40.01 40° 44.23 38° 47.01 36° 44.02 图8是不同锥角药型罩射流侵彻靶体时的侵彻深度-时间变化曲线。图8中同时给出了42°锥角纯铜药型罩的侵彻深度变化趋势。通过2.2节分析可知,36°锥角铝铜药型罩射流头部的侵彻速度最高,但随着时间推移,侵彻深度增加速度逐渐放缓,相比38°锥角的侵彻情况,最终的侵彻深度有所降低,说明侵彻过程不是单纯由射流头部速度决定,同时也与射流中后部速度以及射流尺寸有关。整体比较可发现,铝铜药型罩在锥角为38°时的最终侵彻深度最大。通过数值模拟研究,得到最佳的锥角顶角设计值,为今后的实验设计提供有益的技术参数。
2.4 射流速度分布及动能
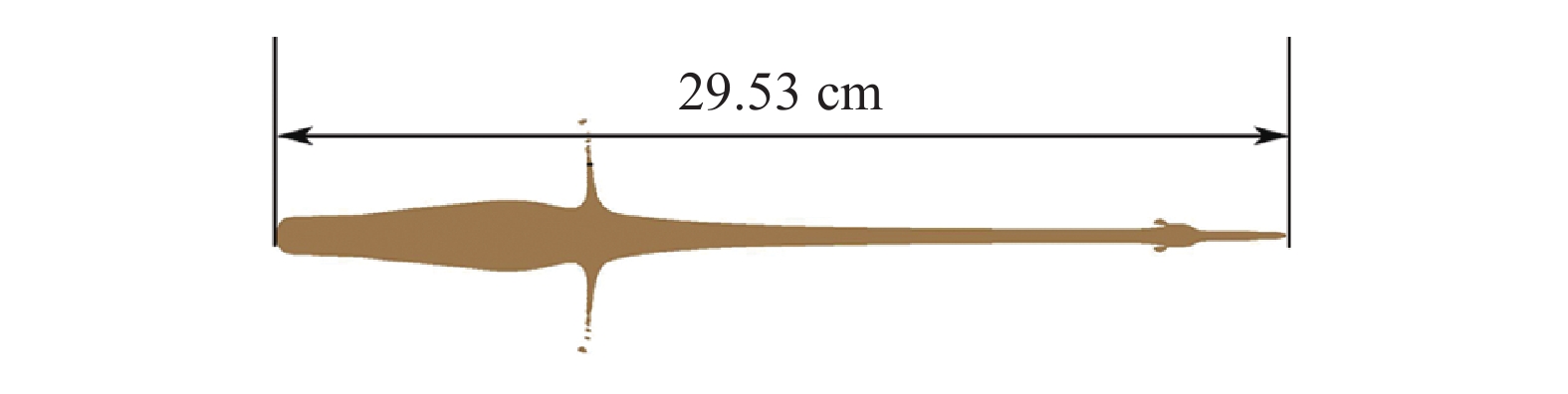
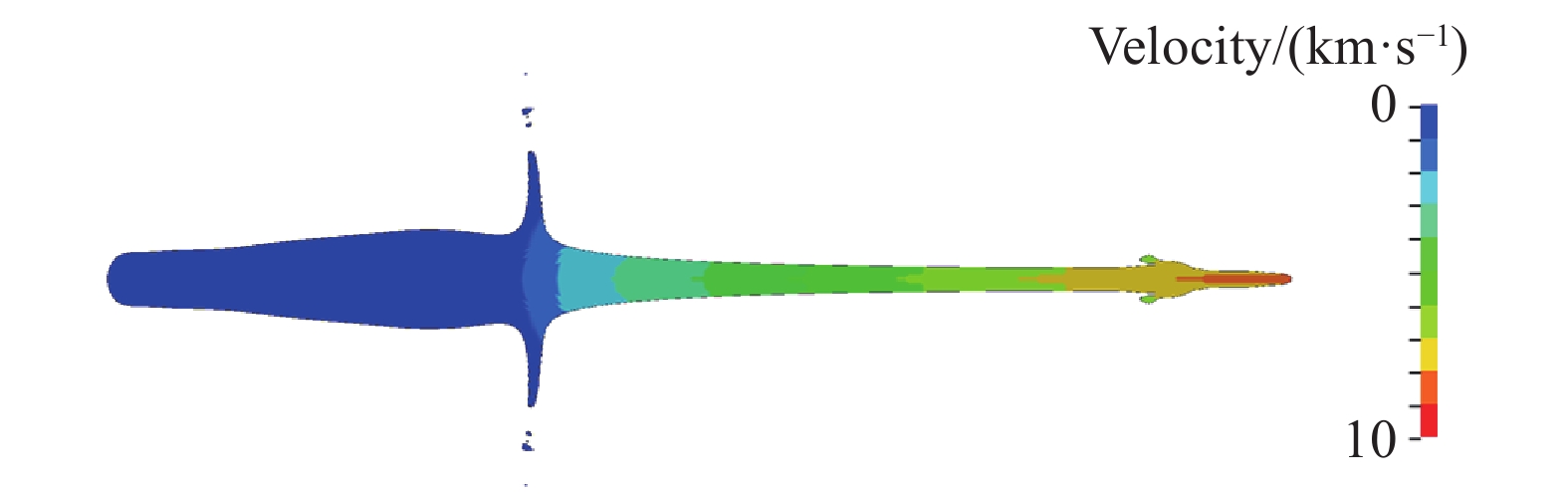
考虑射流在侵彻靶体前的速度和动能分布,将铝铜复合药型罩与单纯铜药型罩的射流情况进行对比。取38°锥角铝铜复合药型罩聚能装置作为代表进行数据归纳。图9给出了计算单纯铜药型罩时的射流分布图像。图10则是计算得到的38°锥角铝铜药型罩产生的射流形貌。通过形貌对比可以明显看到,侵靶前在射流的前半部分,相比于纯铜药型罩情况,38°锥角铝铜药型罩具有较高的射流速度。
同时,通过分析射流有效侵彻段动能获得侵靶穿深大小的推测依据。根据动能将整个射流分成若干射流段,然后进行动能累加。射流后部杵体的速度一般在1.0 km/s以下且体积大,基本不会对最终穿深产生影响。在若干n段中的射流分割中,设射流段半径为射流分段半径
r ,长度为l ,射流密度为铜材料密度ρCu,射流段的平均速度为va,射流分段动能为Evi,整体射流的有效侵彻动能为Ev,则有va=vmin {E_{vi}} = \frac{{{\text{π}}{r^2}l{\rho _{\rm{Cu}}}v_{\rm{a}}^2}}{2} (1) {E_v} = \sum\limits_{i = 1}^n {{E_{vi}}} 上述两种射流情况的分段数据如表8和表9所示。可以看出,锥角38°铝铜药型罩射流的有效侵彻动能较纯铜药型罩射流高9%左右。
表 8 锥角38°铝铜药型罩射流各分段速度分布及总动能Table 8. Jet velocity values at different locations along its elongation and total jet kinetic energy from the charge with aluminum copper welded liner at 38° apex anglePortion i Velocity/(km·s–1) va/(km·s–1) r/cm l/cm Evi/kJ Ev/kJ 1 2.0–3.0 2.5 0.54 0.45 11.50 454.71 2 3.0–7.0 5.0 0.28 6.09 167.43 454.71 3 7.0–8.0 7.5 0.23 2.29 95.58 454.71 4 8.0–9.6 8.8 0.17 5.74 180.19 454.71 表 9 单层铜药型罩射流各分段速度分布及总动能Table 9. Jet velocity values at different locations along jet elongation and total jet kinetic energy from the charge with single copper linerPortion i Velocity/(km·s–1) va/(km·s–1) r/cm l/cm Evi/kJ Ev/kJ 1 2.0–3.0 2.5 0.58 1.21 35.68 414.13 2 3.0–7.0 5.0 0.28 8.83 242.76 414.13 3 7.0–8.0 7.5 0.21 3.40 118.30 414.13 4 7.0–8.3 7.7 0.11 1.75 17.38 414.13 2.5 计算方法分析讨论
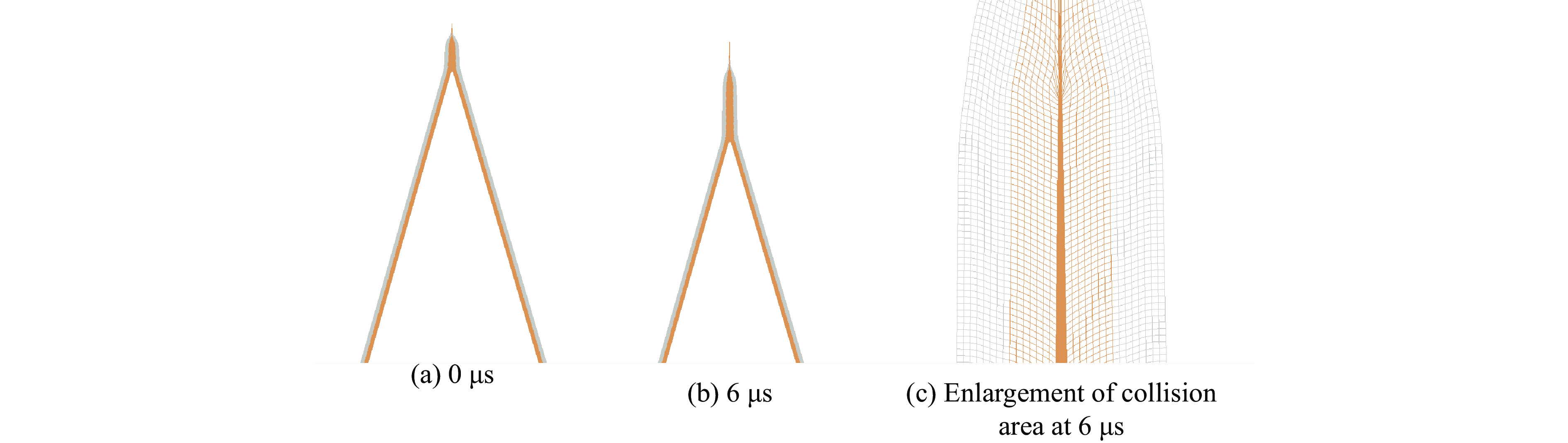
分析铝铜复合药型罩情况发现,铝铜之间的结合强度不同会导致射流结果发生不同程度的变化。理论上采用Lagrange模式计算能较好地解决此问题。Lagrange方法在计算剧烈大变形、高速流体运动时很容易产生网格畸变,导致后期计算结果不准确甚至计算出错,但初期变形阶段仍可以实施Lagrange计算。为此,针对铝铜有结合和无结合两种方式的平板对称斜碰进行计算,以显示两者差异,计算初始图如图11(a)所示。取平板整体运动速度为3.5 m/s,接近聚能射流药型罩碰撞速度,两板沿中垂线对称放置成42°夹角。图11和图12分别为两种复合板斜碰撞的计算形貌。从计算结果可以看出:铝铜板没有结合强度时,碰撞后分离现象明显,铝铜间缝隙扩大,如图11(d)所示;而具有良好结合强度的板材在碰撞后仍能够保持原有的结合,如图12(c)所示。可以推断,无结合强度的铝铜药型罩会对射流的稳定性造成很大影响。
另一方面,具有良好结合强度的铝铜复合药型罩聚能装置的计算中, 爆炸复合界面的结合强度是通过多物质欧拉法计算中混合网格处理实现的。在多物质欧拉法计算中,混合网格中的应力是通过各组分的物质量占有率,将各物质相应的应力取出,进行加权平均得到网格统一应力[18]。若多物质处于压缩状态,无论物质界面是否有结合强度都可以用这种处理方法。但对处于拉伸应力状态下的多物质网格,若界面没有结合强度,则上述处理方法明显同实际情况不符,因为没有结合强度的界面网格不能承受拉伸应力,因此在程序中若不进行特殊处理,则默认为具有某种结合强度处理。而在已有的双层金属射流和爆炸成形弹丸的计算中,关于此论点并没有具体指出。当然,对于此问题,准确实施界面强度的计算还有待于今后的进一步改进。
3. 结 论
在经典聚能射流装置的基础上,提出改进型双层铝铜药型罩聚能射流装置以及改进思路,采用LS-DYNA软件进行改进型装置射流与侵彻能力的模拟计算。首先对同等药量加速单层铜板和双层铝铜板情况进行计算,给出了铝铜药型罩提高药型罩压垮速度的依据,进而通过数值方法研究几种不同锥角情形下铝铜药型罩射流和侵彻行为,得到以下结论:
(1) 在装药量不变的情况下,铝铜药型罩射流头部速度随着药型罩锥角的减小而增大;
(2) 当保持铝铜厚度比为1时,38°锥角铝铜药型罩的射流和侵彻性能最好,当锥角大于或小于38°时,侵彻性能均会下降。
-
表 1 模型几何参数
Table 1. Geometrical parameters in shaped charge configuration
δ/cm h/cm d/cm α/(°) b/cm 0.21 15.24 8.38 42 5.86 Note: δ, h, d, α, b are liner thickness, height of charge, diameter, apex angle, and top diameter, respectively. ρ0/(g·cm–3) D/(km·s–1) pCJ/GPa E0/(J·m–3) ω 1.821 8.48 34.2 9.6 0.38 A/GPa B/GPa C/GPa R1 R2 748.6 13.38 1.167 4.50 1.20 Note: ω, A, B, C, R1 and R2 are JWL EOS parameters; ρ0, D, pCJ and E0 are density, detonation velocity, CJ presure, and explosive energy per volume, respectively. 表 3 铝和铜的Grüneisen状态方程参数[16]
Table 3. Parameters in Grüneisen equation of state of aluminum and copper[16]
Material ρ0/(g·cm–3) C0/(km·s–1) S Γ Troom/K cv/(J·kg–1·K–1) Aluminum 2.78 5.39 1.339 1.97 300 884 Copper 8.93 3.94 1.489 2.02 300 383 Note: S is constant; C0, Γ, Troom, cv are sound velocity, Grüneisen coefficient, room temperature, and specific heat capacity at constant volume, respectively. 表 4 铝和铜材料的Steinberg强度模型参数[17]
Table 4. Parameters in Steinberg strength model of aluminum and copper[17]
Material ρ0/(g·cm–3) G0/GPa Y0/GPa β n ({-G'_r/G_0})×103/K–1 Aluminum 2.78 27.6 0.29 125 0.10 0.62 Copper 8.93 47.7 0.12 36 0.45 0.38 Note: β and n are constants; G0, Y0 and are shear modulus, yield strength, and {G'_r} shear modulus per time derivative,respectively. 表 5 钢靶弹塑性随动硬化模型参数[19]
Table 5. Target material parameters in elastic-plastic-kinematic strength model[19]
Material ρ0/(g·cm–3) Ep/GPa μ Y/GPa Ce Pe/s–1 ɛeff Steel 7.83 2.07 0.3 0.011 1 6 500 4 0.7 Note: Pe, Ce and ɛeff are constants; Ep, μ and Y are platic modulus, Poisson’s ratio and yield strength, respectively. 表 6 纯铜药型罩射流侵彻计算和实验结果对比
Table 6. Comparison on computational and experimental results of jet and penetration by charge with single copper liner
Jet head velocity/(km·s–1) Penetration depth/cm Experiment This calculation Experiment This calculation 8.30 8.23 38.58–40.23 41.15 表 7 不同锥角铝铜药型罩与纯铜药型罩射流计算结果对比
Table 7. Computational results on penetration by aluminum-copper liner with various apex angels
Material α/(°) Penetration depth/cm Cu 42° 41.15 Al-Cu 42° 40.01 40° 44.23 38° 47.01 36° 44.02 表 8 锥角38°铝铜药型罩射流各分段速度分布及总动能
Table 8. Jet velocity values at different locations along its elongation and total jet kinetic energy from the charge with aluminum copper welded liner at 38° apex angle
Portion i Velocity/(km·s–1) va/(km·s–1) r/cm l/cm Evi/kJ Ev/kJ 1 2.0–3.0 2.5 0.54 0.45 11.50 454.71 2 3.0–7.0 5.0 0.28 6.09 167.43 454.71 3 7.0–8.0 7.5 0.23 2.29 95.58 454.71 4 8.0–9.6 8.8 0.17 5.74 180.19 454.71 表 9 单层铜药型罩射流各分段速度分布及总动能
Table 9. Jet velocity values at different locations along jet elongation and total jet kinetic energy from the charge with single copper liner
Portion i Velocity/(km·s–1) va/(km·s–1) r/cm l/cm Evi/kJ Ev/kJ 1 2.0–3.0 2.5 0.58 1.21 35.68 414.13 2 3.0–7.0 5.0 0.28 8.83 242.76 414.13 3 7.0–8.0 7.5 0.21 3.40 118.30 414.13 4 7.0–8.3 7.7 0.11 1.75 17.38 414.13 -
[1] 谭多望, 孙承纬. 成型装药研究新进展 [J]. 爆炸与冲击, 2008, 28(1): 50–51. doi: 10.3321/j.issn:1001-1455.2008.01.009TAN D W, SUN C W. Progress in studies on shaped charge [J]. Explosion and Shock Waves, 2008, 28(1): 50–51. doi: 10.3321/j.issn:1001-1455.2008.01.009 [2] 戴兰宏. 工程科学前沿的拓荒者——郑哲敏 [J]. 力学进展, 2013, 43(3): 265–294. doi: 10.6052/1000-0992-13-033DAI L H. A pioneer in the frontier of engineering science—Zhe-Min Zheng [J]. Advances in Mechanics, 2013, 43(3): 265–294. doi: 10.6052/1000-0992-13-033 [3] 龚柏林, 李明, 初哲, 等. 贫铀合金药型罩聚能破甲性能实验研究 [J]. 高压物理学报, 2018, 32(3): 035102.GONG B L, LI M, CHU Z, et al. Penetration performance of depleted uranium alloys liner [J]. Chinese Journal of High Pressure Physics, 2018, 32(3): 035102. [4] 龚柏林, 初哲, 王长利, 等. 基于贫铀合金药型罩的聚能弹破甲后效实验研究 [J]. 高压物理学报, 2018, 32(6): 065104.GONG B L, CHU Z, WANG C L, et al. Experimental research on armor penetration aftereffect produced by depleted uranium alloys liner shaped charge [J]. Chinese Journal of High Pressure Physics, 2018, 32(6): 065104. [5] 潘文强, 付代轩, 赖康华, 等. 含能射孔弹双层药型罩穿孔性能研究 [J]. 爆破器材, 2017, 46(2): 31. doi: 10.3969/j.issn.1001-8352.2017.02.007PAN W Q, FU D X, LAI K H, et al. Study on penetration performance of Bi-layer liner in energetic penetrating charge [J]. Explosive Materials, 2017, 46(2): 31. doi: 10.3969/j.issn.1001-8352.2017.02.007 [6] DIL’’DIN Y M, KOLMAKOV A I, LADOV L V, et al. Effect of the width of the diffusion zone in multilayer lining of shaped charges on the shaping effect [J]. Combustion, Explosion and Shock Waves, 1980, 16(6): 660–663. [7] LAROCCA E W, STRIKE R. Method of making a bimetallic shaped charge liner: 4807795 [P]. 1989-02-28. [8] 臧涛成, 胡焕性, 邵琦. 破甲弹复合罩性能研究 [J]. 火炸药学报, 1998(4): 44–47.ZANG T C, HU H X, SHAO Q. The performance study of shaped charge liner [J]. Chinese Journal of Explosives & Propellants, 1998(4): 44–47. [9] 郑宇, 王晓鸣, 李文彬, 等. 双层药型罩侵彻半无限靶板的数值仿真研究 [J]. 南京理工大学学报(自然科学版), 2008, 32(3): 313–317.ZHENG Y, WANG X M, LI W B, et al. Numerical simulation on double-layered shaped charge liner penetration into semi-infinite target [J]. Journal of Nanjing University of Science and Technology, 2008, 32(3): 313–317. [10] 乔金超, 吴越, 贾磊朋. 双层线型药型罩侵彻靶体的数值分析 [J]. 兵工自动化, 2017, 36(9): 27–30.QIAO J C, WU Y, JIA L P. Numerical analysis of double-layer composite linear liner penetrate target [J]. Ordnance Industry Automation, 2017, 36(9): 27–30. [11] LS-DYNA Manual R.9448 [CP]. Livermore Software Technology Corporation, 2018. [12] DIPERSIO R, SIMON J, MERENDINO A B. Penetration of shaped-charge jets into metallic targets [R]. Maryland: Ballistics Research Laboratory, 1965. [13] LEE E L, HORNIG H C, KURY J W. Adiabatic expansion of high explosive detonation products: UCRL-50422 [R]. Livermore: Lawrence Livermore National Laboratory Report, 1968. [14] DOBRATZ B M, CRAWFORD P C. LLNL explosive handbook [M]. California: U.S. Government Printing Office, 1987: 8–22. [15] MEYERS M A. Dynamic behavior of materials [M]. John Wiley & Sons, 1994: 124. [16] KATAYAMA M. Numerical and experimental study on the shaped charge for space debris assessment [J]. Acta Astronautica, 2001, 48(5): 363–372. [17] STEINBERG D J, COCHRAN S G, GUINAN M W. A constitutive model for metals applicable at high-strain rate [J]. Journal of Applied Physics, 1980, 51(3): 1498–1504. doi: 10.1063/1.327799 [18] KRIEG R D, KEY S W. Implementation of a time dependable plasticity theory into structural computer programs, constitutive of equations in viscoplasticity: computational and engineering aspects [M]. New York: American Society of Mechanical Engineers, 1976: 125–137. [19] 时党勇, 李裕春, 张胜民. 基于ANSYS/LS-DYNA 8.1进行显示动力分析 [M]. 2版. 北京: 清华大学出版社, 2005: 313–326.SHI D Y, LI Y C, ZHANG S M. Explicit dynamic analysis based on ANSYS/LS-DYNA 8.1 [M]. 2nd ed. Beijing: Tsinghua University Press, 2005: 313–326. -








 下载:
下载:









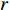

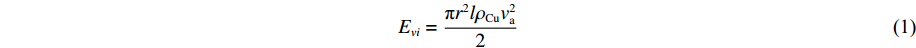



 下载:
下载: