Overview of the Study of Dynamical Plastic Deformation Based on Dislocation Dynamics Method
-
摘要: 金属材料的动态塑性变形行为是一个多尺度的瞬变动力学过程,是物理学、力学以及材料科学等学科的交汇点,相关研究对工程应用具有重要的指导意义。动态载荷作用下,微观层面单个缺陷行为与介观层面缺陷群的集体演化行为交织在一起,导致金属材料呈现复杂的宏观力学现象。已有研究表明,金属材料的动态塑性变形与准静态变形存在显著差异,并且受到诸多内部及外部因素的影响。近几十年来,人们发展了位错动力学方法研究金属材料的动态塑性变形。但是由于动态变形问题的复杂性,对动态塑性变形的认识仍然存在不足。本文从计算方法和变形理论两个方面对该领域国内外发展历史及重要进展进行了回顾,以期为动态塑性变形研究提供有益的参考。Abstract: Study of the dynamic plastic deformation of crystalline metals is a typical multi-scale problem, and is an assembly point of multi-scale science. Under dynamic loading, behaviors of defects at micro-scale and collective behaviors of an assembly of defects at meso-scale contribute to the complex constitutive behaviors at macroscale together. It is found experimentally that constitutive behavior of metals under dynamic loading quite differs from that under moderate loading conditions, and are influenced by an amount of external and internal factors, which makes it hard to recognize the fundamental origin of the dynamical plastic deformation. Dislocation dynamics method is developed to unravel the dynamical plastic deformation. Despite of several tens of years of studies, physical principle of dynamical plastic deformation is still poorly understood. In this article, we reviewed the study of dynamical plastic deformation based on dislocation dynamics method from the viewpoint of computational method and deformation theory.
-
Key words:
- dynamical plastic deformation /
- dislocation dynamics /
- dynamic loading
-
材料动态塑性变形研究在爆破工程、航天航空工程以及相关行业中有广泛的应用背景。例如爆炸冲击波与材料和结构的作用、爆炸加工与粉末爆炸压实、空间碎片与航天器等结构的碰撞、运输工具的碰撞以及装甲等结构防护等研究中,都需要对材料的动态响应有较为充分的了解[1]。
材料的变形行为,就其本质而言是材料内部非均匀微细观结构被激活并随时空演化的动力学过程,是典型的宏观、介观和微观耦合的多尺度现象,涉及力学、材料学和物理学等学科,具有重要的学术价值。白以龙院士在2005年Applied Mechanics Reviews的一篇综述中,对固体材料的力学响应问题进行了归纳:“‘There is another sort of problems, i.e. strength and plasticity theory, for which even essential physical formulation is still not available for engineering applications,’ Tsien wrote about 40 years ago in his well-known book Physical Mechanics. …… Unfortunately, this problem still remains a compelling challenge until now”[2]。在该综述发表十余年后的今天,白以龙院士的评述仍然适用,材料强度行为的物理本质仍然是力学学科亟待解决的重要问题之一。
在早期的动态变形研究中,人们主要通过测量宏观应力-应变曲线及速度波剖面获取宏观强度进而推测材料的变形过程,依此建立的宏观唯象模型难以反映底层的物理机制,预测能力有限。近年来,人们意识到如果只停留在宏观层面,而不深入到微介观分析中去,就很难理解导致材料动态变形的根本原因,因此关注的焦点逐渐从连续介质力学上的理解向介观尺度乃至原子尺度转变。时至今日,人们普遍认为晶体材料的塑性变形由位错、孪晶以及位移型相变主导。位错作为最基本的塑性剪切单元,了解位错的行为及影响对认识塑性变形的底层机理尤为重要。位错概念的提出要追溯至20世纪初,Volte等[3]、Love[4]首次在连续介质力学中引入了位错的概念。Orowan等[5]于1934年给出了位错的物理图像,并将晶体材料的塑性变形机制与位错联系起来。著名的Orowan方程描述了塑性变形与位错运动以及位错生成的关系。位错理论提出以后,人们从不同空间尺度发展了多种计算方法,对位错的行为及影响都有了充分的认识。尽管如此,已有研究大多针对准静态加载条件下的变形,对更高应变率下动态塑性变形问题的认识还存在很大局限。
在一定加载条件下,微观尺度上的位错形核、增殖和反应等行为与介观尺度上的位错集体演化行为交织在一起,最终导致材料宏观动态变形行为的出现。对于高应变率下的塑性变形行为,位错和位错分布的时空尺度关联及耦合效应导致相关研究十分复杂。而介观尺度上所体现的、与宏观和微观层次不同的物理现象及异质性正是该复杂行为的主要内容。从介观尺度出发研究材料的动态变形行为,一个核心问题是如何描述该尺度下材料的微结构演化规律,并与宏观、微观都联系起来。就已有研究来看,分子动力学方法可以较有效地研究动载下位错的行为及其对局域变形的影响,但受限于计算规模,分子动力学难以处理较大空间尺度以及较大变形下的塑性变形行为。离散位错动力学通过追踪位错线的演化,可以实现对更大尺度变形的研究,但现有的计算资源仍然不足以支撑采用离散位错动力学方法研究宏观尺度的塑性变形行为,特别是大应变问题。连续位错动力学通过和有限元耦合可以直接与宏观力学响应耦合,因此也成为目前研究动态变形的主要有效手段。连续位错动力学方法也有自身的局限性,例如如何全面地考虑微介观变形机理。从长远看,建立能够将多种计算方法耦合在一起的多尺度方法势在必行,但这不仅需要从不同研究尺度上进行努力,还需要进一步探究尺度之间的衔接问题等。
1. 基于位错动力学方法的动态塑性变形研究
材料动态塑性变形与准静态塑性变形存在显著差异。在研究准静态加载条件下材料的塑性变形时,人们假设材料在变形过程中始终处于平衡状态,忽略掉位错等微结构的惯性效应以及应力波传播等非平衡因素对变形的影响,从而材料的响应只与外加载荷幅值等因素相关。与准静态加载条件下材料变形行为不同,动载下载荷通常在很短的时间内发生显著变化,例如核爆中心压力可以在几微秒内上升到103~104 GPa量级,此时就必须计及位错等微结构以及“物质微元的惯性效应”[6]对塑性变形的影响。具有惯性的物质受到随时间变化的外载荷作用时,其运动和响应过程总是伴随着位错加速、减速以及应力波的传播、反射与相互作用等过程。这些因素交织在一起,导致材料呈现复杂的宏观力学行为。近几十年来,人们针对典型动态塑性变形问题,从计算方法和位错理论两个方面出发对位错的行为及影响等进行了大量的研究。
1.1 位错动力学计算方法研究进展
人们主要通过原子模拟和介观模拟两种手段研究位错的行为及影响。原子模拟包括分子动力学模拟与蒙特卡罗计算,介观模拟包括离散位错动力学、连续位错动力学、动力蒙特卡罗方法以及相场方法等[7]。由于动态变形的特殊性,在实际研究中更多地采用分子动力学、离散位错动力学和连续位错动力学这3种方法。
从根源上讲,材料的宏观特性源于其底层原子之间的相互作用。这些底层的相互作用使得原子形成特定的晶体结构,位错正是由于原子错位而形成的典型线缺陷。原子模拟方法是研究位错自身演化行为最有效的手段,通过分子动力学模拟可以明确位错运动以及演化机制。由于分子动力学计算规模较大,因此该方法不适用于描述较多位错的集体演化行为。
离散位错动力学方法将位错线离散为若干直线段,通过追踪每条位错线的运动及演化,最终达到描述材料塑性变形的目的。离散位错动力学方法的研究大致经历了由二维到三维、由准静态到动态的发展过程。就离散位错动力学的发展历史来看,长久以来,人们都采用该方法研究准静态加载条件下的塑性变形行为,而对于较高应变率下的动态变形行为直至近年来才有人涉及。在准静态假设下,位错源点位置的改变对域内场点的影响可以近似认为是及时的,因为在经历足够长的时间之后,源点的影响始终能够通过应力波传递至场点。在处理动态变形问题时,情况则完全不同。由于变形速率十分迅速,在经历一次加载之后,位错源点位置改变对场点应力分布的影响或许还没有传递至场点。此时,就需要考虑动态应力场。Markenscoff和Clifton[8]早在1981年就对二维动态应力场进行了推导。Lazar[9]推导得到了三维动态应力场。时至2015年,Gurrutxaga-Lerma等[10]才首次在程序中将其实现,并用于解决动态变形问题。目前主流的离散位错动力学程序主要包括Cai等[11]开发的Paradis程序、加州大学洛杉矶分校的Ghoniem等[12]开发的程序、华盛顿州立大学Zbib等[13]开发的micr3d程序、Needleman等[14]开发的程序以及法国Kubin等[15–17]开发的MobiDic程序。
由于离散位错动力学的计算需要追踪每条位错线的运动与演化,计算消耗资源巨大,计算规模一般有限,仅适用于小变形问题。在发展离散位错动力学的同时,人们也发展了基于位错密度的连续位错动力学方法。连续位错将位错密度作为内变量,通过描述位错密度的演化实现对塑性变形的描述,通过与有限元耦合,从而可用于计算更大尺度及更大变形下的变形行为。与离散位错动力学不同的是,连续位错动力学需要为各种类型的位错提供演化控制方程,包括可动位错、不可动位错、几何必须位错以及位错输运等。连续位错动力学由Gilman、Kock[18]等首先提出,在他们提出的模型中,位错密度作为内变量描述变形过程。若更加细致地考虑连续位错动力学,则还需要考虑位错输运。这在研究准静态加载条件下材料的变形行为时得到采用,但尚未见动态变形研究考虑该因素[19]。连续位错动力学的研究一般伴随着晶体塑性有限元方法,通过描述位错密度的演化为单元提供塑性变形。连续位错方法本身并不需要区分塑性变形过程是动态或者准静态。由于动态变形机制与准静态存在较大的差异,要准确描述动态变形行为,关键在于如何给出合适的位错密度控制方程,包括其随应力、压力、应变、温度以及应变率的变化等。在研究冲击加载问题时,还需要对有限元部分作流体弹塑性处理,即对变形进行球量和偏量的拆分。
已有的计算方法都有其特定的局限性,要更加准确地描述真实的动态变形过程,就需要建立一个能够准确反映各个尺度下位错的行为及影响的多尺度模型。Lawrence Livermore实验室所建立的多尺度强度模型可以从一定程度上实现对各个尺度下位错行为及影响的描述[20]。多尺度强度模型适用于斜波加载下体心立方(BCC)金属的动态变形问题,对于其他加载条件下的变形问题是否适用还未得到验证。此外,多尺度强度模型对位错亚结构演化的考虑相对简单,对介观层面位错演化行为的描述也不够准确。尽管如此,多尺度模型仍建立了一套完整的架构,为后续类似模型的发展提供了参考。
1.2 动载下位错理论研究进展
从微介观层面看,材料的动态变形是材料内部非均匀微细观微结构被外加载荷激活并随时空演化的动力学过程,是典型的宏观、介观和微观耦合的多尺度现象。位错作为最基本的剪切变形单元,了解其在动载条件下的行为和影响对于深入认识材料的动态变形行为至关重要。
从现有研究看,动态塑性变形过程中位错行为主要受如下两大类因素影响:外部加载因素,包括应变率、应力、温度以及加卸载路径等;内部结构因素,包括晶格结构、多晶材料的晶界、织构以及非均匀缺陷等。在这两类因素的作用下,位错的加速、减速、成核、增殖、塞积及湮灭等行为与冲击波的传播效应耦合在一起,导致材料呈现复杂的力学响应。人们发展了多种数值模拟手段,以期厘清动态塑性变形过程中位错的行为及其对宏观塑性变形行为的影响。
1.2.1 动态载荷作用下的位错理论
动态载荷作用下材料的塑性变形行为与位错行为之间的关系可以由Orowan方程描述
˙γij=∑βρβMVβDbβinβj+˙ρβˉlbβinβj (1) 式中:上标
β 表示滑移系,ρM 为运动位错密度,b为伯格斯矢量,n为滑移面法向矢量,VD 为位错速度,ˉl 为位错运动的平均距离,˙ρ 为位错生成速率。与广泛使用的、仅考虑位错滑移对塑性变形贡献的Orowan方程不同,位错生成对动态载荷作用下材料的塑性变形也有显著贡献。基于这一物理图像,动态载荷作用下位错行为的研究大致可以分为两类:位错速度演化行为研究和位错密度演化行为研究。通常情况下,人们认为位错运动主导较低应变率下的塑性变形。位错运动速度随外加应力增大而增大,从而更加充分地协调塑性变形。实验和模拟都表明,根据外加应力和位错运动速度大致可以将主导位错运动机制分为3类:热激活位错运动、声子拖曳机制、相对论效应[21]。在Peierls力以下,外加应力不足以使位错摆脱束缚启动滑移,位错需要在热能的帮助下发生运动,此时热激活机制主导位错运动。特别地,对于BCC金属,热激活机制一般通过Double-kink运动与成核的方式实现。在Peierls力以上,外加应力足以克服势垒,位错不再需要热能的帮助即可启动滑移,运动位错所需克服的主要阻碍为声子黏性,此时声子拖曳机制主导位错运动。原子模拟表明,对于BCC金属,螺位错运动过程中所需克服的阻碍还包括位错钉扎以及退钉扎过程贡献的阻碍[22]。随着外加应力继续增大,位错速度不会无限制增大,当位错速度逼近横波声速时,位错运动受到相对论效应的控制,此时位错速度主要受声速控制。大量原子模拟以及实验结果都验证了这一物理图像。而超声速位错是否存在,目前尚存在疑问。
在较高的应变率下,特别是在冲击载荷作用下,位错运动速度的提升已经来不及协调多余的塑性变形,此时就需要位错生成机制参与塑性变形。Armstrong等[23]指出,较高应变率下材料的动态变形主要由热激活位错生成机制主导。在早期的研究中,人们针对冲击载荷作用下位错生成提出了不同的理论模型。例如:Smith、Hornbogen等提出的一个位错界面模型,Meyers、Zaretsky提出的均匀成核模型等,Weertman针对强冲击和弱冲击加载提出了两种不同机制。由于位错理论的匮乏,早期的位错模型都存在这样或那样的问题,特别是一些模型认为超声速位错存在于强动载荷下的动态变形过程中[24]。
Austin和McDowell[25]于2011年对冲击波作用下位错密度的演化行为进行了较为全面的归纳和总结。在他们提出的亚结构演化模型中,位错密度演化包括位错的生成和位错的湮灭两类。其中,位错密度一般通过增殖或者成核两种机制增加,通过湮灭以及热力学涨落两种方式消失。冲击波作用下,位错密度演化的物理图像如下:冲击波诱导材料产生极高的剪应力,剪应力驱动位错运动与演化;晶体中已有的位错通过F-R或者B-H源发生增殖;当外加载荷足够强,例如逼近材料的理想强度时,没有缺陷的晶体中也会通过成核生成新的位错,成核又可以分为均匀成核和非均匀成核两种;运动的位错在与晶格黏性以及阻碍等作用的过程中其速度会降低,从而成为不可动位错;具有相反符号的两个位错接近时会发生湮灭;热力学涨落也会导致部分位错恢复成完美晶格。
1.2.2 不同外部加载因素作用下位错的行为及影响
在不同外加载荷条件下,材料往往呈现不同的宏观力学行为及微结构响应特征。由于现有研究手段难以对微结构演化行为进行动态原位诊断,人们无从得知强动载荷作用下微结构的演化规律。现有研究中,主要通过原子模拟获得微结构动态演化的规律,通过介观动力学方法将原子模拟获得的机理性认识应用于解释宏观力学行为。本节简要介绍从位错动力学出发对典型外加载荷因素如何影响动态变形及位错演化行为进行的研究。
(1)应变率
材料动态变形最显著的特征即为率敏感性。早在1988年,Follansbee等[26]就从实验中发现材料的强度随着应变率的增大而增大,这种增长趋势在应变率超过104 s–1时更加显著。材料的这一率相关特性又被称为率敏感性。从位错运动的层面上看,材料的率效应实则是位错惯性效应的体现。对于材料强度的率效应,人们从不同方面给出了解释。早期研究中提出了一系列理论模型解释较低应变率下材料强度的率相关关系,包括Z-A模型、MTS模型等。然而这些模型都不能对较高应变率下材料的强度进行解释。人们普遍认为,主导位错运动机制的转变导致了率敏感性的出现。
以Kanel为代表的俄罗斯科学家认为主导位错运动机制由热激活转变为声子拖曳机制导致了强度率敏感性的出现[27]。Follansbee等[26]认为在较高的应变率下热激活机制仍然主导位错运动,并且不会出现由热激活到声子拖曳机制的转变,率敏感性的出现是由于结果演化的率敏感性。Rusinek通过他们的MRK模型指出,热激活机制与声子拖曳机制在较高的应变率下共存[28]。基于实验结果,Z-A模型指出位错拖曳机制并不是导致率敏感性出现的原因。Armstrong等指出,随着应变率的升高,主导位错运动机制由热激活位错运动转变为热激活位错生成导致材料率敏感性的出现。Fan等[29]指出,率敏感性是由于激活体积随应力变化以及应变率诱发的非Arrhenius行为等因素导致的。
(2)环境温度
由上述内容可知,不同的应变率范围内,主导材料发生塑性变形的机制存在显著差异。这一差异不仅使材料表现出复杂的率相关效应,还表现出复杂的温度相关特性。在研究材料的准静态变形行为时,人们一般认为热激活机制主导位错运动导致材料强度呈现随温度升高而降低的热软化趋势。然而,较高应变率下材料的强度却呈现出相反的趋势。
近年来,Kanel等[30–36]在一系列加温冲击加载实验中发现材料强度随环境温度升高而增大的反常温度效应。在强度相对较高的BCC金属冲击加载实验中,他们发现了更为反常的现象。在对多晶钽进行的冲击实验中,Kanel等并没有发现异于低应变率情形的实验现象;但在金属钒和金属铁的冲击加载实验中却发现,钒和铁的动态屈服强度随温度的变化趋势并不呈现简单的单调关系[35]。
对于不同晶格结构材料表现出来的不同温度效应,Kanel等也尝试给出了定性的解释。他们认为声子拖曳机制主导位错运动导致材料发生热硬化。对于面心立方(FCC)金属中的反常温度效应,Krasnikov等[37]认为声子拖曳机制主导位错运动导致该现象的出现,并且通过一个基于位错密度的介观模型再现了该实验现象。近年来,Gurrutxaga-Lerma[38]通过动态离散位错动力学模拟发现,冲击波作用下,弹性前驱波的演化主要受波阵面上成核位错所辐射应力场屏蔽效应的影响,该辐射应力场与位错运动速度呈正相关。对于FCC金属铝,波阵面上位错运动速度趋近于横波声速,而横波声速受剪切模量控制并且随温度升高而减小,因此位错辐射应力场也呈现同样的趋势,弹性前驱随温度升高而增大。而对于BCC金属,由于其内部位错运动阻抗及黏性都相对较大,位错速度难以逼近横波声速,因此这一效应不再适用,特别是对于弱冲击加载问题。针对BCC铁动态强度随温度演化的不敏感性,Gurrutxaga-Lerma等通过同样的方法指出,是由Peierls力主导位错运动、并且与声子拖曳相互作用所导致的。
(3)应力
典型的与应力相关的动态变形特征有Swegle和Grady[39]根据实验现象总结得到的应变率-应力四次关系,即冲击加载下冲击上升前沿的应变率与应力的四次幂成正比
˙ε∝p4 (2) 从早期的一些实验结果看,该关系在很多材料中都近似成立,包括金属和非金属材料。Crowhurst等[40]通过实验验证了四次律在1010 s–1应变率下仍然成立,但其物理内涵目前还不明确。揭示Swegle-Grady四次律的物理内涵也是美国MaRie计划的重要研究内容之一。
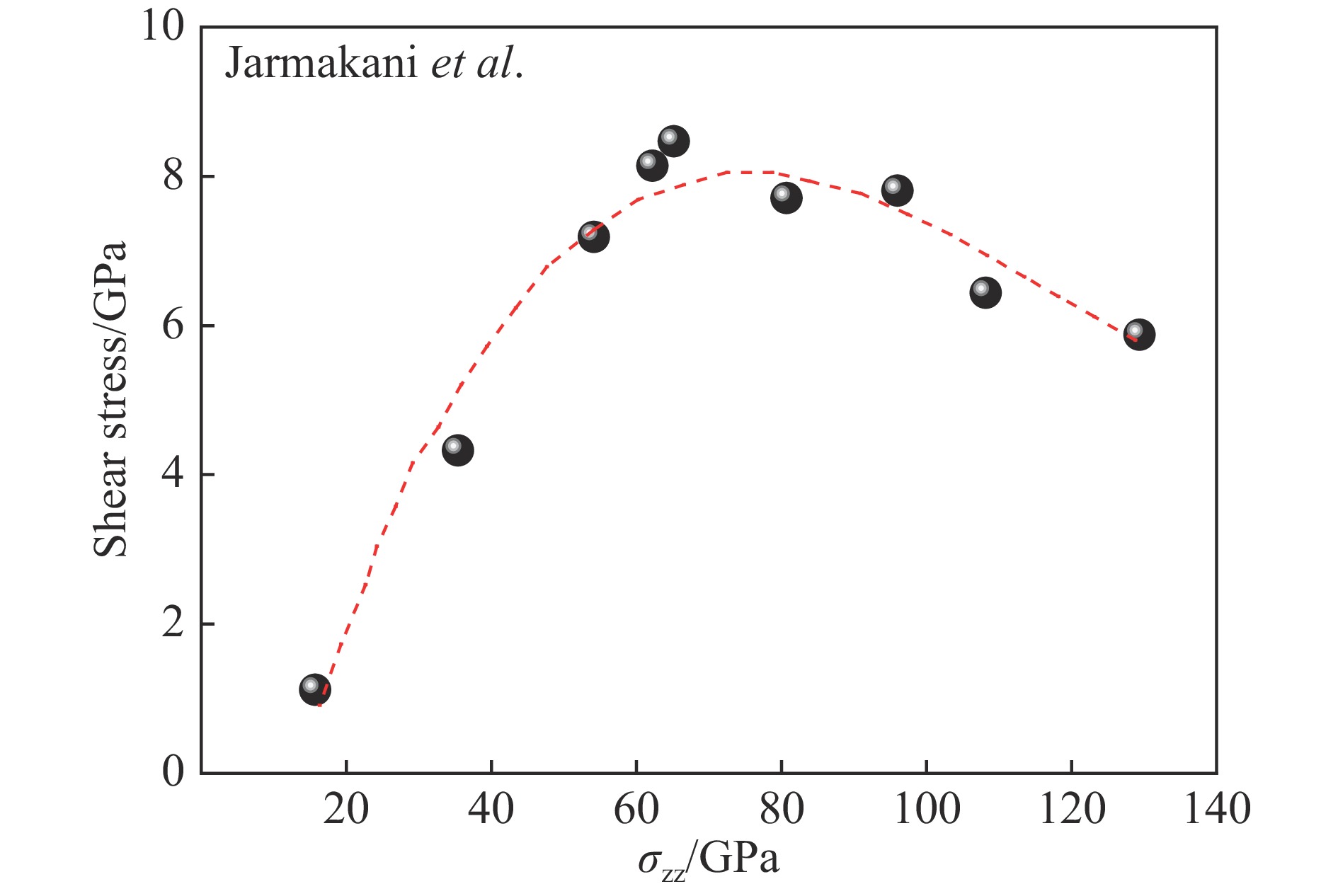
加载应力的变化除了影响应变率以外,还会导致变形机制发生变化。Cao等[41]采用不同加载压力对冲击加载下铜的微结构演化行为进行了研究,实验发现:当采用5 GPa的加载压力时,材料中会出现大量的位错网络;当采用30 GPa的加载压力时,材料中会出现大量的滑移带和堆垛层错;当采用50 GPa的加载压力时,材料内部会出现更多、更复杂的微结构,如孪晶、位错网络、变形带以及滑移带等。Jarmakani等[42]通过分子动力学方法模拟了不同冲击压力下金属Ni中塑性变形机制的转变,得到了各种机制之间的转变阈值压力,并将较高压力下剪切强度随应力衰减的行为归因于位错成核,如图1所示。
(4)卸载与再加载
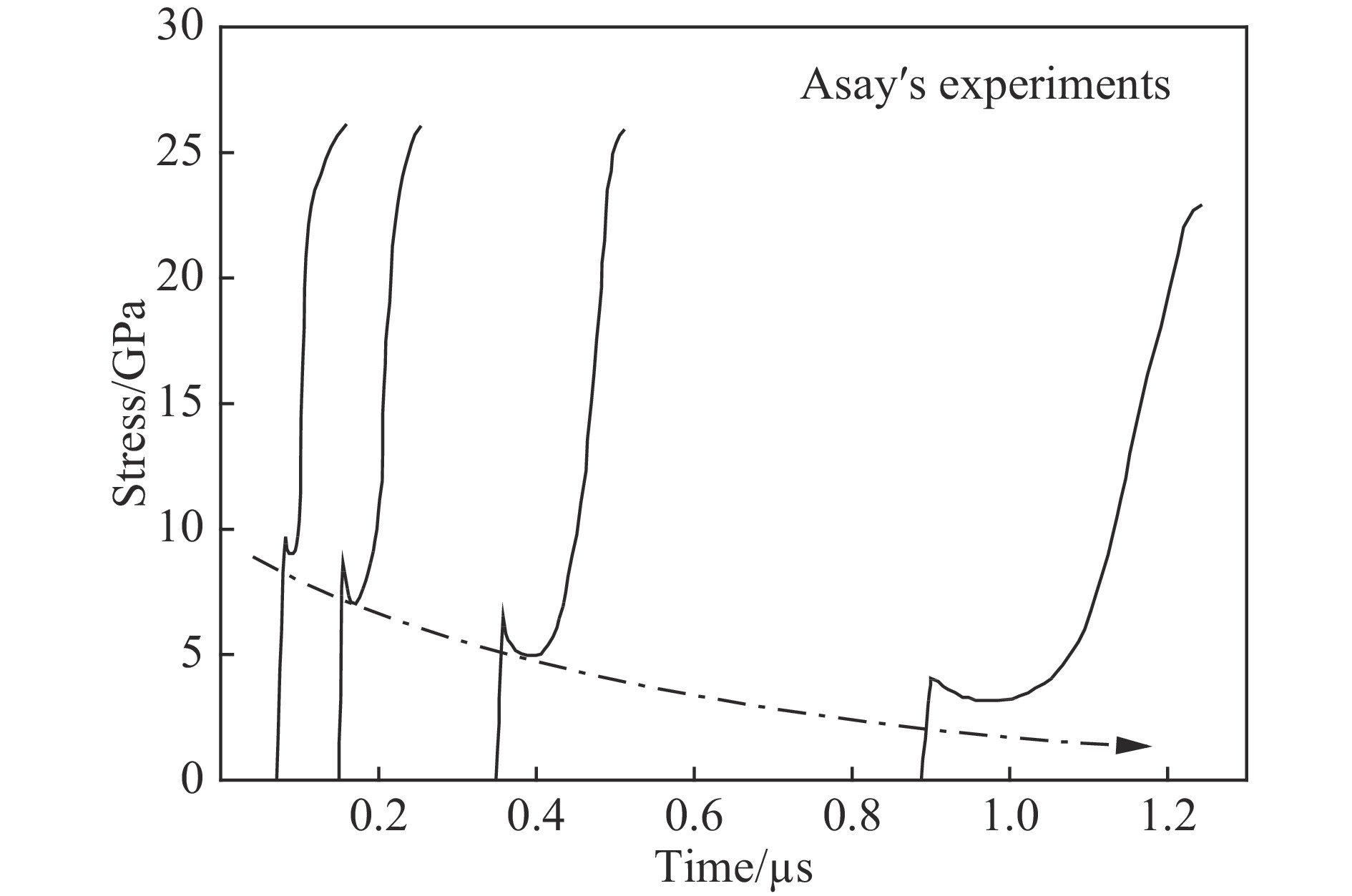
以上主要介绍了应变率、压力、温度等外部因素对加载段位错行为的影响,加载段反映了事先未经历变形的材料的特性。冲击波作用下,材料在经历加载段的弹塑性变形之后达到Hugoniot态,位错等微结构停止演化,材料内部处于亚稳态。随着后界面反射的稀疏波或压缩波到达后,处于亚稳态的位错将重新启动演化,材料将呈现出更加复杂的特性。根据反射波的不同,材料内部将分别发生卸载或者再加载过程,后界面反射的稀疏波将对材料进行卸载,压缩波则对材料进行再加载。
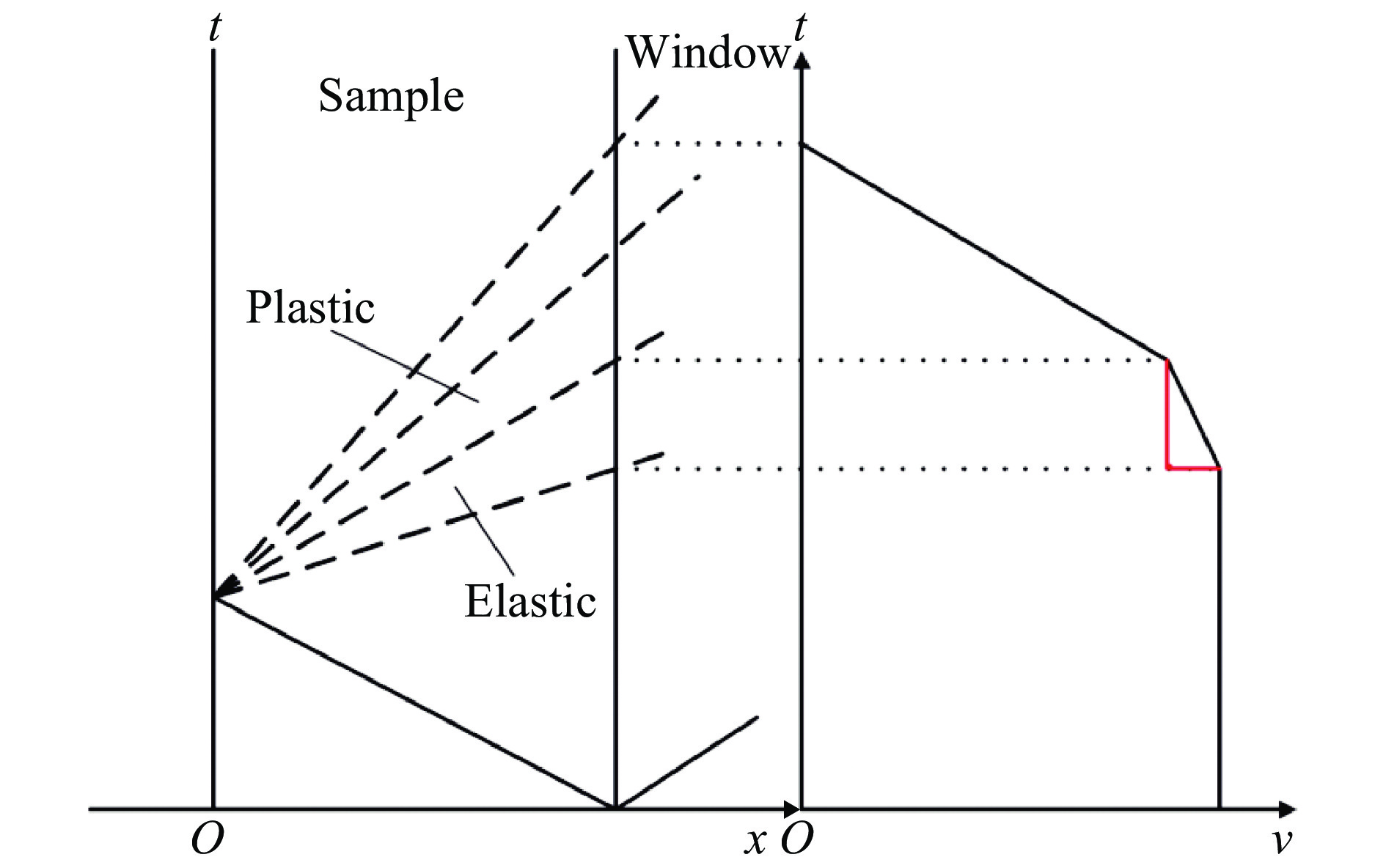
对于理想弹塑性材料,完整的加-卸载波剖面如图2所示,加载段和卸载段的弹性段与塑性段出现明显的分离现象,弹、塑性波之间有明显的平台。实验中却发现,金属铝的卸载段与再加载段波形并不呈现出弹、塑性波完全分离的状态[43–44],Gray、Asay和Johnson等将该现象称为“准弹性现象”[45]。
对于材料卸载与再加载时出现的准弹性现象,人们从不同的角度出发给出了一些解释。Cochran、Guinan[46]和Gray等都将准弹性卸载现象归结为Bauschinger效应。Johnson等则从位错层面区分了Bauschinger效应与准弹性卸载现象,指出这两个概念是具有相同物理机制的两种不同宏观表现形式。Johnson等[47]对于准弹性卸载现象的解释如下:外加载荷激发位错运动,可动位错遇到障碍时发生塞积,塞积的位错会在滑移面上施加一个与加载正向应力相反的应力,因此当反向加载时,材料屈服所需的应力变小;卸载时,剪应力减小,内应力导致位错反向移动,从而在弹性变形中伴随着少量的塑性变形。Dwivedi、Asay等[48]建立了二维介观模型,详细讨论了晶粒间杂质的存在、晶界导致硬化现象、微孔存在等对于卸载与再加载波剖面的影响,并将准弹性现象归因于材料内部结构分布的异质性。中国工程物理研究院的潘昊[49]和姚松林等[50]采用一个基于位错密度的本构模型再现了准弹性卸载现象,并分别将该现象归因于剪切声速的变化和可动位错向不可动位错转化导致的临界分切应力渐进增长。
(5)斜波加载
在不同的加载路径作用下,材料热力学状态的不同也导致位错运动行为呈现显著不同。长久以来,人们大多采用冲击加载手段研究高应变率下材料的塑性变形行为。然而,冲击载荷作用下变形沿着绝热路径进行,绝热变形过程往往伴随着温升,这样一来变形就同时受到多种因素的共同影响,相关问题的研究也就更加复杂。相较于冲击加载,斜波加载下材料的变形路径近似为准等熵路径,可以在较小的温升下达到较高的压力。基于这些原因,斜波加载手段正逐渐成为人们研究材料高压力学性能的主要手段之一。
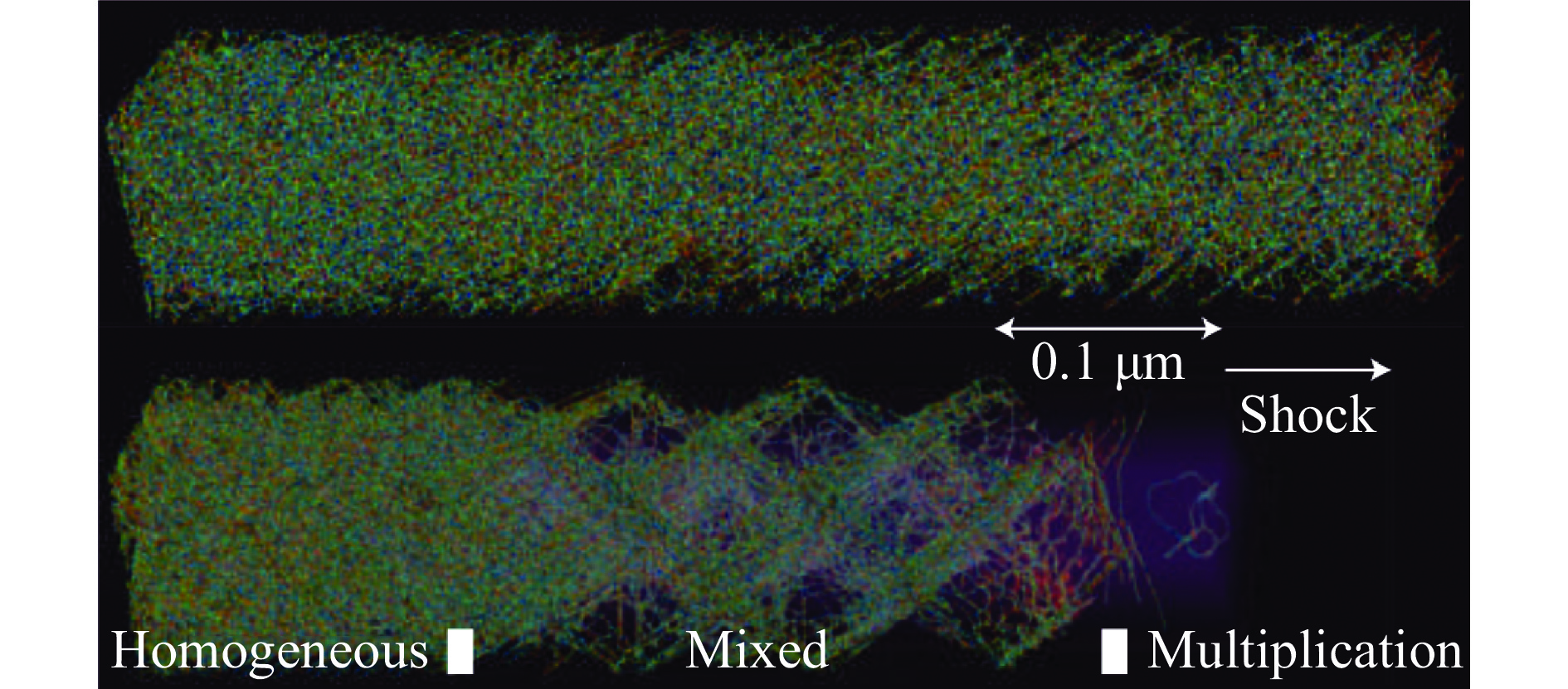
斜波加载下材料的变形行为十分复杂,不仅体现在变形过程中宽广的应变率变化范围,还体现在变形路径存在由准等熵到冲击的转变过程,这将使材料的黏性效应和惯性效应更加显著。在不同的变形路径下,底层的位错运动机制也存在显著差异[51]。Bringa等[52]基于分子动力学模拟结果指出,不同加载方式将导致FCC金属内部位错亚结构演化存在显著差异,如图3所示。具体来讲,冲击加载下位错主要以成核的形式生成,而斜波加载下位错亚结构演化则会出现由增殖向成核的转变。
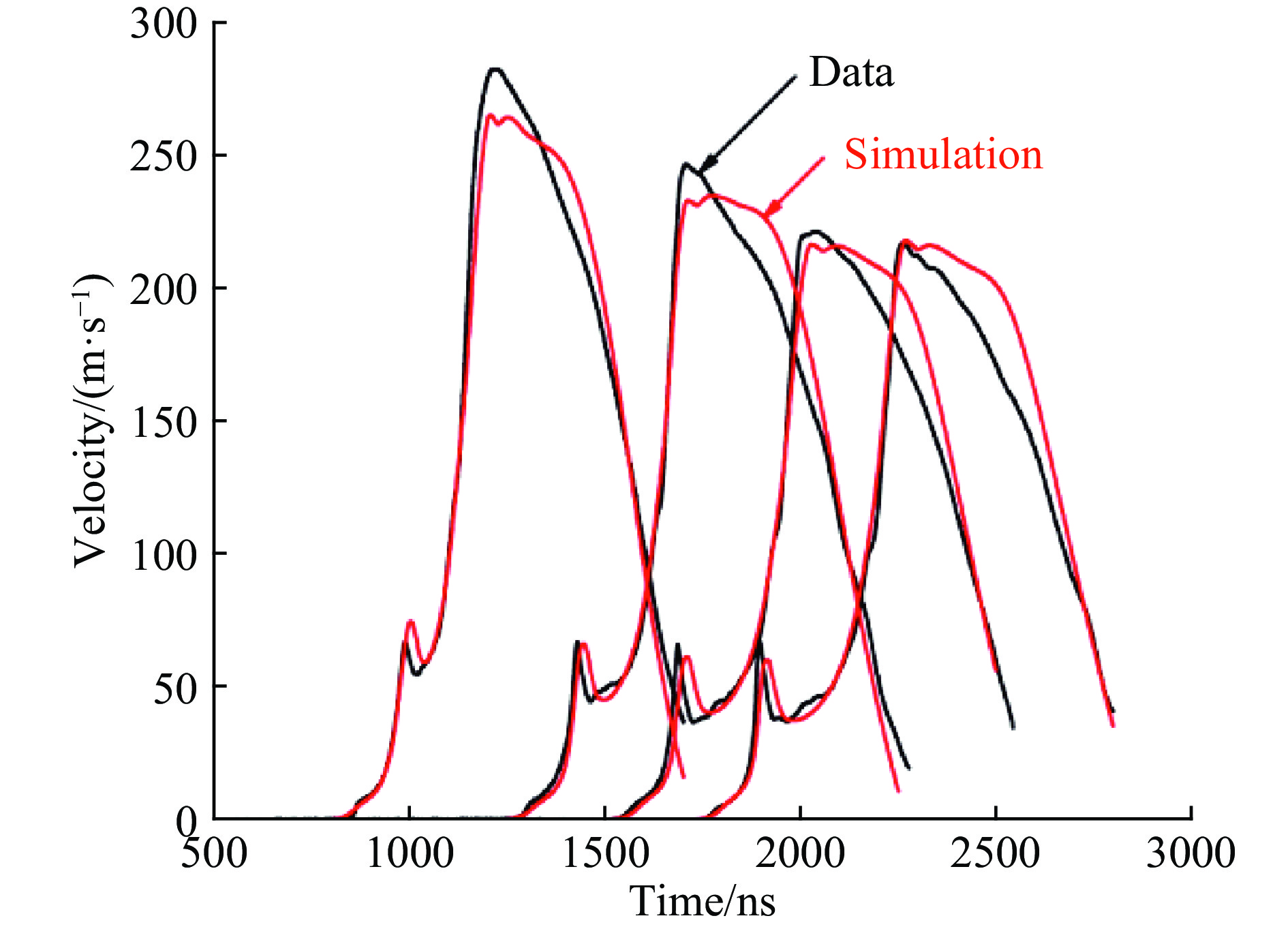
Ding等[51]针对斜波加载问题提出了一种基于位错的本构模型,可以再现准等熵极限的回跳现象以及塑性前沿的演化,如图4所示。然而,该模型无法准确描述准等熵极限不随传播距离衰减的现象。从位错动力学角度讲,当外加应力超过临界分切应力(位错启动阈值)时,位错启动滑移,塑性变形开始,塑性耗散导致剪应力减小直至达到临界分切应力的水平。在较高的应变率下,外加载荷导致应力迅速上升,有限的塑性变形来不及耗散掉足够的能量使应力始终保持在临界分切应力的水平,因此存在一个弛豫过程,准等熵极限的回跳现象即表征了这样一个过程。该模型之所以无法准确描述准等熵极限的演化行为,其根源在于该模型没有充分考虑位错亚结构演化,并且对位错动力学的描述不准确。因此,该模型无法弄清何种机制主导了斜波加载下的塑性变形,预测性也就不强。尽管如此,该模型首次从位错运动行为出发对斜波加载问题进行研究,其意义仍然十分重大。
除Ding等以外,Lawrence Livermore实验室提出的多尺度强度模型[20]也从底层位错运动机制出发研究斜波加载下金属材料的动力学响应。多尺度模型更加侧重于材料的多尺度行为,其建立对于材料多尺度行为的研究具有较强的指导意义。但是该模型涉及的尺度太多,计算量相对较大,应用起来也相对复杂。此外,该模型对位错亚结构演化的描述也相对简单,如位错成核与俘获过程等,因此对真实的斜波加载下材料变形行为的描述仍然存在不足。
总的来说,人们对斜波加载下位错的行为及影响的研究仍然较为匮乏。这一方面是由于斜波加载存在较大范围的应变率变化,率效应在材料的力学响应过程中扮演着更加重要的角色;另一方面,由于变形路径存在由力学响应到热力学响应的转变,使得准确描述变形路径的转变以及不同变形路径下位错的演化行为更加困难。
(6)冲击波传播
一般地,材料的强度为其内禀属性,不随材料尺寸变化。然而通过测量雨贡纽极限得到的强度却会随样品厚度的增大而衰减,Asay[53]及Kanel[33–34]等均从实验中发现了该现象,如图5所示。Duvall[54]通过理论推导,得到了雨贡纽极限随距离衰减的表达式
dσHELdh=−43G˙γcl (3) 式中:h为传播距离,G为剪切模量,
˙γ 为塑性应变率,cl 为纵波声速。(2)式表明,冲击波传播过程中,塑性变形引起的能量耗散导致材料的雨贡纽极限随传播距离衰减。在理想弹塑性假设下,我们认为变形是一个稳态过程,从而无需考虑位错的加速、减速、增殖与湮灭等过程的时间效应。在这一物理图像下,弹性前驱波到达后,应力迅速上升至屈服应力状态,在塑性冲击波到达前,应力始终保持在屈服状态,因此理想弹塑性假设下宏观雨贡纽极限不随时间或空间发生变化。然而,在真实的动态变形过程中,较高的加载应变率导致应力在极短的时间内(<ns)迅速上升,在该时间尺度内,位错来不及进行大量增殖,较少的位错滑移不足以将应力迅速松弛至屈服应力状态,因此剪应力随冲击波传播发生持续衰减,直至屈服应力。2015年,帝国理工学院的Gurrutxaga-Lerma等[10]通过其所建立的动态离散位错动力学方法对该问题进行了深入研究,并从位错运动层面给出了该现象的物理图像:冲击波作用下,冲击波阵面上较高的应力驱动位错形成,运动方向与冲击波传播方向一致的位错所发射的动态应力场将对材料内部应力起屏蔽作用,正是运动位错发射应力场与冲击波的相互作用导致弹性前驱衰减现象的出现。
弹性前驱的衰减现象表明真实的动态塑性变形过程呈现时间上非稳态和空间上非均匀特性。随着应力波在材料中传播,局域的塑性变形导致应力在传播过程中发生持续衰减,因此应力分布呈现时间上非稳态和空间上非均匀的特性。而应力非均匀分布又将导致不同区域内位错微结构演化呈现差异,回收表征实验也验证了这一观点。在动态变形研究过程中,越来越多的实验结果表明,材料的动态变形过程是一个空间上非均匀的过程,因此人们对动态变形的认识逐渐从匀质性向异质性转变。
1.2.3 材料结构因素作用下位错的行为及影响
除外加载荷因素外,材料结构因素也会对位错运动行为造成显著影响,从而导致材料呈现不同的塑性变形行为。典型的材料结构因素包括晶体结构、晶界、织构以及孔洞等。在以往的研究中,人们往往只关注晶体结构的差异对位错运动行为的影响,对材料分布作平均化描述并对变形过程作稳态假设,而忽略了材料内部可能存在的结构及缺陷分布对变形的影响。随着精密物理实验的发展,人们逐渐认识到真实的动态变形过程的非均匀特性,因此也逐渐开始关注非均匀微结构等对位错运动行为的影响。
(1)晶体结构
就位错的运动行为来看,不同晶格结构材料中位错运动行为也存在显著差异。在FCC金属中,位错线主要通过{111}面进行扩展,极少发生位错线跨滑移面的交滑移行为;而在BCC金属中,螺位错更容易发生交滑移现象,位错运动的三维特性更强。
在动态变形研究中,Zerilli和Armstrong[55]基于实验观察并分析了不同晶格结构的热激活位错运动,首次区分晶格结构提出了基于位错的本构模型。其研究表明,BCC金属比FCC金属具有更高的应变率敏感性和温度敏感性;此外,FCC金属激活面积与应变有关,而BCC金属则与应变无关(激活面积为位错在克服势垒启动运动时所扫过的面积)。他们就此指出,BCC金属中位错主要通过热激活机制克服Peierls势垒。Peierls势垒在晶格中周期分布,不受塑性变形影响,因此BCC金属激活面积与应变无关。而FCC金属中位错所需克服的势垒主要为林位错,林位错随应变增大而减小,因此FCC金属的激活面积随应变增加而减小。Z-A模型是第一个具有物理理论基础,在热激活位错运动理论框架下提出而非通过实验曲线拟合的本构模型。
(2)多晶效应
在实际应用中,我们所接触的材料大多为多晶材料。多晶材料可以视为若干单晶体的集合,其最显著的特征为存在晶界以及织构。晶粒取向的分布以及晶界的存在导致多晶材料比单晶材料具有更加复杂的力学响应特性。著名的Hall-Petch效应反映了晶粒的尺寸分布对材料强度的影响。在准静态条件下的变形行为研究中,人们引入了应变梯度理论,提出一种表征变形非均匀性的几何必须位错,从而在不显含晶粒尺寸的情况下即可模拟出Hall-Petch效应[56]。按照应变梯度理论,由于晶界处晶格失配较为严重,因此几何必须位错主要集中在晶界处。Kazmi和Murr[57]在1979年的透射电镜(TEM)实验中证实了这一观点。目前,人们提出了两种物理模型来描述晶界:一种把晶界视为对位错部分透明的,另一种则把晶界完全视为对位错运动的阻碍。在不可穿越的晶界模型中,Evers等[56]引入了晶界位错来描述晶界处的晶格失配。
除上述效应外,人们还发现多晶效应显著影响冲击波前特征。例如,Bringa等[58]采用分子动力学方法模拟了冲击波在纳米晶中的传播,结果表明冲击波阵面宽度受到压力、晶粒尺寸及时间的影响。此外,从模拟结果还得知,晶界对波阵面宽度的影响小于晶粒之间取向差造成的影响。冲击波阵面演化是冲击波效应、材料结构效应与位错动力学行为的强耦合共同作用的结果,如何解读这一特征目前仍然存在困难。
(3)空洞与氦泡等非均匀缺陷对位错行为的影响
真实材料中往往还存在空洞或氦泡等缺陷,对材料的局域塑性变形及宏观变形行为具有显著影响[59]。由于空洞与氦泡的尺寸较小,约为纳米至百纳米量级,因此相关研究主要通过原子模拟进行。人们采用分子动力学方法对空洞与氦泡的行为及其对局域塑性变形的影响进行了研究。例如:Davila等[60]指出冲击诱导延性金属中的空洞塌缩通过发射剪切位错环实现,Lubarda等[61]指出位错发射所对应的临界剪应力与空洞的尺寸有关。除此之外,王海燕[62]、邵建立[63]等对氦泡局域应力分布导致的位错演化的非均匀性进行了研究。尽管如此,受限于计算资源,人们还无法直接通过原子模拟对较大尺寸样品的宏观力学行为进行研究,因此对材料的一些宏观力学行为暂时还不能给出准确解释。例如,现有理论无法将空洞与氦泡等非均匀缺陷对局域变形的影响与材料的宏观屈服强度、拉伸强度以及相应强度依赖性联系起来[59, 64]。
2. 存在的重要难题和挑战
纵观近半个世纪的发展,基于位错动力学方法对材料动态变形的研究中仍然存在如下难题与挑战。
(1)超声速位错
超声速位错是否存在对于变形机理的认识具有十分重要的意义。迄今为止,该问题仍然没有定论。Weertman[65]基于相对论理论通过数学推导将位错速度分为3个区域,分别为次声速区域、跨声速区域和超声速区域。在这一物理图像下,人们接受了超声速位错的存在。但关于超声速位错是否存在,目前还存在争议。Gumbsch和Gao[66]通过分子动力学模拟获得了超声速位错,但其速度并非由静止状态加速得到,而是在极高的加载应力下直接产生的。Nosenko等[67]在一种等离子晶体中也发现了超声速“位错”,但此“位错”与真实晶体材料中的位错显然还存在不同。Gurrutxaga-Lerma等[38]在揭示FCC金属热硬化现象时也假设了超声速位错不存在,进而才得出剪切模量随温度减小导致FCC金属动态屈服强度发生热硬化的结论。显然,如果超声速位错存在于真实的动态变形过程中,其将颠覆现有的动态变形理论。
(2)率效应与温度效应
关于强度的率敏感性,现有理论众说纷纭,人们公认位错演化主导机制随应变率增大发生改变导致材料呈现率敏感性,但没有任何一个理论得到公认。该问题的复杂性根源于率效应往往伴随着压力效应,而在实验研究中很难实现这两种因素的解耦。尽管Volger等[68]通过数值手段采用Steinberg-Guinan (SG)模型得出,零压下率敏感性的转变阈值约为107 s–1;但是这种线性的处理方式是否适用于动态变形的复杂非线性问题,仍然有待商榷。此外,SG模型本身适用的应变率范围也有限。
另一个显著的率相关特性为Swegle四次律[39]。四次律体现了应力与应变率的强耦合特性,正是这种强耦合特性使人们难以认清导致其出现的原因。美国的MaRie计划也将其列为重要的研究内容之一。尽管可以从计算上再现这一现象,但目前还没有研究从位错动力学行为出发对该现象给出解释。
除率效应以外,超高应变率下材料复杂的温度效应也有待进一步研究。例如,BCC金属和HCP金属更容易出现孪晶,孪晶对动态强度的影响目前并没有研究。另一个复杂的问题是,铁磁性材料的动态强度与铁磁相变等也存在关联。实验表明,铁磁性材料Ni和Fe的动态强度在Curie点附近会发生显著的衰减[69–70]。
(3)复杂变形路径对动态变形的影响
动态变形的复杂性不仅体现在影响变形的因素多,还体现在变形的路径依赖性。典型的准弹性现象就是动态变形路径依赖性的体现。经历压缩状态后,材料在再加载或者卸载状态下呈现不同的强度特征。就现有研究来看,人们还没能从位错的角度将两种准弹性现象进行统一。此外,动态变形的路径依赖性还体现在对加载路径的依赖上。例如冲击加载和斜波加载下,材料就处于不同的热力学路径,不同热力学路径下材料变形存在本质的区别;特别是绝热路径下温升对位错演化的影响,都有待进一步研究予以确认。
(4)多晶效应
大量的实验研究表明,材料结构的非均匀特性对其塑性变形具有显著影响。尽管针对准静态变形过程中的相关特征进行了大量研究,但动态塑性变形过程中的相关研究相对较少。Meyers等[24]仅从冲击前沿上升时间出发研究了冲击波作用下的晶界效应,对于晶界局域变形特征则没有细致地研究。就准静态变形研究来看,晶界的存在会导致位错塞积或者穿透;而动态变形过程中晶界如何影响位错的演化还需要进一步研究。
(5)多尺度衔接
就现有研究来看,分子动力学方法是研究位错行为及其对局域变形影响最直接的工具;基于分子动力学研究结果,位错动力学在一定程度上可以研究位错的集体演化行为。但总的来看,这两种计算方法规模较大,仍然难以直接与宏观变形相耦合,因此采用这两种计算方法无法准确揭示一些典型宏观实验现象的底层机理。多尺度模型的提出为解决这一问题提供了思路,如何建立一个充分考虑各个尺度变形机制,并且可以有效解决动态塑性变形问题的多尺度模型是动态塑性变形研究的长远目标。
3. 发展趋势和展望
动态载荷作用下材料的动态塑性变形行为非常复杂。特别是在强动载荷加载下,材料往往处于高压、高应变率、高温以及大变形等极端条件下;此外,不同材料的响应过程有着各自不同的微结构演化特征。这些因素在变形过程中相互耦合,导致相关研究十分困难。材料的塑性变形本质上是材料内部的位错等微结构被激活并随时空演化的动力学过程,是典型的宏观、介观和微观耦合的多尺度现象。要解决动态变形所遇到的问题,需要明确各个尺度下位错等微结构的演化行为及其对变形的影响。人们分别从不同尺度出发对位错的行为及影响进行了研究;但由于动态变形问题的复杂性,对强动载荷下的位错行为及影响的认识仍然不够充分,尚有大量的问题亟待解决。要从根源上解决这些问题,需要从实验、理论和模拟三方面共同努力。精密物理实验(例如自由电子激光实验技术)可以确认缺陷形成机制和主导因素,并追踪其动态演化,实现微结构演化的定量测量;介观尺度下动态变形理论可以确认缺陷的集体演化规律和控制因素,从理论上将微观变形机制与宏观变形行为耦合起来;介观模拟通过描述缺陷的集体演化规律及其控制因素,可以实现对宏观塑性行为的预测,从而将理论与实验联系起来。只有实验、理论和模拟三方面协同研究才有望最终解决动态塑性变形问题。
感谢清华大学航天航空学院柳占立老师、崔一南老师在位错理论方面给予的指导。
-
-
[1] MEYERS M A. 材料的动力学行为 [M]. 张庆明等, 译. 北京: 国防工业出版社, 2006: 1–4. [2] BAI Y L, WANG H Y, XIA M F, et al. Statistical mesomechanics of solid, linking coupled multiple space and time scales [J]. Applied Mechanics Reviews, 2005, 58(6): 372–388. doi: 10.1115/1.2048654 [3] HIRTH J P, LOTHE J. Theory of dislocations [M]. New York: John Wiley and Sons, 1982: 3. [4] LOVE A E H. A treatise on the mathematical theory of elasticity [M]. Cambridge: Cambridge University Press, 1927. [5] OROWAN E. Die mechanischen festigkeitseigenschaften und die realstruktur der kristalle [J]. Zeitschrift für Kristallographie, 1934, 89(605): 634. [6] 王礼立. 应力波基础[M]. 第2版. 北京:国防工业出版社, 2010: 1–4. [7] BULATOV V V, CAI W. Computer simulations of dislocations [M]. Oxford: Oxford University Press, 2006. [8] MARKENSCOFF X, CLIFTON R J. The nonuniformly moving edge dislocation [J]. Journal of the Mechanics, Physics and Solids, 1981, 29(3): 253–262. doi: 10.1016/0022-5096(81)90029-6 [9] LAZAR M. On the elastic fields produced by non-uniformly moving dislocations: a revisit [J]. Philosophical Magazine, 2011, 91(25): 3327–3342. doi: 10.1080/14786435.2011.579584 [10] GURRUTXAGA-LERMA B, BALINT D S, DINI D. Attenuation of the dynamic yield point of shocked aluminum using elastodynamic simulations of dislocation dynamics [J]. Physical Review Letters, 2015, 114: 174301. doi: 10.1103/PhysRevLett.114.174301 [11] CAI W. Atomistic and mesoscale modeling of dislocation mobility [D]. Cambridge: Massachusetts Institute of Technology, 2001. [12] GHONIEM N M, TONG S H, SUN L Z. Parametric dislocation dynamics: a thermodynamics-based approach to investigations of mesoscopic plastic deformation [J]. Physical Review B: Condensed Matter, 2000, 61(2): 913–927. doi: 10.1103/PhysRevB.61.913 [13] ZBIB H M, RUBIA T D D L. A multiscale model of plasticity [J]. International Journal of Plasticity, 2002, 18(9): 1133–1163. doi: 10.1016/S0749-6419(01)00044-4 [14] GIESSEN E, NEEDLEMAN A. Discrete dislocation plasticity: a simple planar model [J]. Modelling and Simulation in Materials Science and Engineering, 1995, 3(5): 689–735. doi: 10.1088/0965-0393/3/5/008 [15] KUBIN L P, CANOVA G, CONDAT M, et al. Dislocation microstructures and plastic flow: a 3D simulation [J]. Solid State Phenomena, 1992(23/24): 455–472. [16] DEVINCRE B, MADEC R, MONNET G, et al. Modeling crystal plasticity with dislocations dynamics simulations: the ‘microMegas’ code [M]// THOMAS O, PONCHET A, FOREST S. Mechanics of Nano-Objects. Paris: Presses des Mines, 2011: 81–89. [17] MADEC R, KUBIN L P. Dislocation strengthening in FCC metals and in BCC metals at high temperatures [J]. Acta Materilia, 2017, 126: 166–173. doi: 10.1016/j.actamat.2016.12.040 [18] KOCK U F. Laws for work-hardening and low-temperature creep [J]. Journal of Engineering Materials and Technology, 1976, 98(1): 76–85. doi: 10.1115/1.3443340 [19] ZAISER M, NIKITAS N, HOCHRAINER T, et al. Modelling size effects using 3D density-based dislocation dynamics [J]. Philosophical Magazine, 2007, 87: 11–21. doi: 10.1080/14786430600863047 [20] BARTON N R, BERNIER J V, BECKER R, et al. A multiscale strength model for extreme loading conditions [J]. Journal of Applied Physics, 2011, 109(7): 073501. doi: 10.1063/1.3553718 [21] JHONSTON W G, GILMAN J J. Dislocation velocities, dislocation densities, and plastic flow in lithium fluoride crystals [J]. Journal of Applied Physics, 1959, 30(2): 129. doi: 10.1063/1.1735121 [22] MARIAN J, CAI W, BULATOV V V. Dynamic transition from smooth to rough to twinning in dislocation motion [J]. Nature Materials, 2004, 3: 158–163. doi: 10.1038/nmat1072 [23] ARMSTRONG R W, ARNOLD W, ZERILLI F J. Dislocation mechanics of copper and iron in high rate of deformation tests [J]. Journal of Applied Physics, 2009, 105(2): 023511. doi: 10.1063/1.3067764 [24] MEYERS M A, JARMAKANI H, BRINGA E M, et al. Dislocations in shock compression and release [M]// HIRTH J P, KUBIN L. Dislocations in Solids. The Netherlands: North-Holland, 2009: 91-197. [25] AUSTIN R A, MCDOWELL D L. A dislocation-based constitutive model for viscoplastic deformation of fcc metals at very high strain rates [J]. International Journal of Plasticity, 2011, 27(1): 1–24. doi: 10.1016/j.ijplas.2010.03.002 [26] FOLLANSBEE P S, KOCKS U F. A constitutive description of the deformation of copper based on the use of the mechanical threshold stress as an internal state variable [J]. Acta Metall, 1988, 36(1): 81–83. doi: 10.1016/0001-6160(88)90030-2 [27] KANEL G I. Unusual behavior of usual materials in shock waves [J]. Journal of Physics: Conference Series, 2014, 500: 012001. doi: 10.1088/1742-6596/500/1/012001 [28] GAO C Y, ZHANG L C. Constitutive modeling of plasticity of fcc metals under extremely high strain rates [J]. International Journal of Plasticity, 2012, 32/33: 121–133. doi: 10.1016/j.ijplas.2011.12.001 [29] FAN Y, OSETSKY Y N, YIP S, et al. Onset mechanism of strain-rate-induced flow stress upturn [J]. Physical Revivew Letters, 2012, 109(13): 135503. doi: 10.1103/PhysRevLett.109.135503 [30] KANEL G I, RAZORENOV S V, BAUMUNG K, et al. Dynamic yield and tensile strength of aluminum single crystals at temperatures up to the melting point [J]. Journal of Applied Physics, 2001, 90(1): 136. doi: 10.1063/1.1374478 [31] ZARETSKY E B, KANEL G I. Effect of temperature, strain, and strain rate on the flow stress of aluminum under shock-wave compression [J]. Journal of Applied Physics, 2012, 112(7): 073504. doi: 10.1063/1.4755792 [32] ZARETSKY E B, KANEL G I. Response of copper to shock-wave loading at temperatures up to melting point [J]. Journal of Applied Physics, 2013, 114(8): 083511. doi: 10.1063/1.4819328 [33] KANEL G I, GARKUSHIN G V, SAVINYKH A S, et al. Shock response of magnesium single crystals at normal and elevated temperatures [J]. Journal of Applied Physics, 2014, 116(14): 143504. doi: 10.1063/1.4897555 [34] ZARETSKY E B, KANEL G I. Plastic flow in shock-loaded silver at strain rates from 104 s–1 to 107 s–1 and temperatures from 296 K to 1233 K [J]. Journal of Applied Physics, 2011, 110(7): 073502. doi: 10.1063/1.3642989 [35] ZARETSKY E B, KANEL G I. Tantalum and vanadium response to shock-wave loading at normal and elevated temperatures. non-monotonous decay of the elastic wave in vanadium [J]. Journal of Applied Physics, 2014, 115(24): 243502. doi: 10.1063/1.4885047 [36] RAZORENOV S V, ZARETSKY E B, SAVINYKH A S. The spall strength and Hugoniot elastic limit of mono crystalline and polycrystalline copper near melting temperature [J]. Journal of Physics: Conference Series, 2014, 500: 112053. doi: 10.1088/1742-6596/500/11/112053 [37] KRASNIKOV V S, MAYER A E, YALOVETS A P. Dislocation based high rate plasticity model and its application to plate-impact and ultra short electron irradiation simulations [J]. International Journal of Plasticity, 2011, 27(8): 1294–1308. doi: 10.1016/j.ijplas.2011.02.008 [38] BEÑAT GURRUTXAGA-LERMA, BALINT D S, DINI D, et al. The effect of temperature on the elastic precursor decay in shock loaded FCC aluminum and BCC iron [J]. International Journal of Plasticity, 2017, 96: 135–155. doi: 10.1016/j.ijplas.2017.05.001 [39] SWEGLE J W, GRADY D E. Shock viscosity and the prediction of shock wave rise times [J]. Journal of Applied Physics, 1995, 58(2): 692–701. [40] CROWHURST J C, ARMSTRONG M R, KNIGHT K B, et al. Invariance of the dissipative action at ultrahigh strain rates above the strong shock threshold [J]. Physical Review Letters, 2011, 107(14): 144302. doi: 10.1103/PhysRevLett.107.144302 [41] CAO B Y, LASSILA D H, SCHNEIDER M S, et al. Effect of shock compression method on the defect substructure in monocrystalline copper [J]. Materials Science and Engineering A, 2005, 409(1/2): 270–281. [42] JARMAKANI H N, BRINGA E M, ERHART P, et al. Molecular dynamics simulations of shock compression of nickel: from monocrystals to nanocrystals [J]. Acta Materilia, 2008, 56(19): 5584–5604. doi: 10.1016/j.actamat.2008.07.052 [43] LIPKIN J, ASAY J R. Reshock and release of shock-compressed 6061-T6 aluminum [J]. Journal of Applied Physics, 1977, 48(1): 182–189. doi: 10.1063/1.323306 [44] ASAY J R, LIPKIN J. Self-consistent technique for estimating the dynamic yield strength of a shock-loaded material [J]. Journal of Applied Physics, 1978, 49(7): 4242–4247. doi: 10.1063/1.325340 [45] 俞宇颖. 强冲击载荷作用下LY12铝合金的准弹性卸载特性及层裂研究 [D]. 绵阳: 中国工程物理研究院, 2006: 73–80.YU Y Y. Study on the quasi-elastic release behavior and spallation of LY12 aluminum alloy under strong loading [D]. Mianyang: China Academy of Engineering Physics, 2006: 73–80. [46] COCHRAN S G, GUINAN M W. Bauschinger effect in uranium: ECRL-17105 [R]. 1976. [47] JOHNSON J N, HIXSON R S, GRAY G T, et al. Quasielastic release in shock-compressed solids [J]. Journal of Applied Physics, 1992, 72(2): 429–441. doi: 10.1063/1.351871 [48] DWIVEDI S K, ASAY J R, GUPTA Y M. Two-dimensional mesoscale simulations of quasielastic reloading and unloading in shock compressed aluminum [J]. Journal of Applied Physics, 2006, 100(8): 083502. doi: 10.1063/1.2357640 [49] 潘昊. 基于晶体塑性理论研究织构对材料动态性能的影响 [D]. 绵阳: 中国工程物理研究院, 2017.PAN H. Study on texture effect to dynamic behavior of material based on crystal plasticity theory [D]. Mianyang: China Academy of Engineering Physics, 2017. [50] YAO S L, PEI X Y, YU J D, et al. A dislocation-based explanation of quasi-elastic release in shock loaded aluminum [J]. Journal of Applied Physics, 2017, 121(3): 035101. doi: 10.1063/1.4974055 [51] DING J L, ASAY J R, AO T. Modeling of the elastic precursor behavior and dynamic inelasticity of tantalum under ramp wave loading to 17 GPa [J]. Journal of Applied Physics, 2010, 107(8): 083508. doi: 10.1063/1.3373388 [52] BRINGA E M, ROSOLANKOVA K, RUDD R E, et al. Shock deformation of FCC metals on subnanosecond timescales [J]. Nature Materials, 2006, 5(10): 805–809. doi: 10.1038/nmat1735 [53] ASAY J R, FOWLES G R, GUPTA Y M. Determination of material relaxation properties from measurements on decaying elastic shock fronts [J]. Journal of Applied Physics, 1972, 43(2): 744–746. doi: 10.1063/1.1661195 [54] DUVALL G E. Stress waves in anelastic solids [M]. Berlin: Springer-Verlag, 1964:20. [55] ZERILLI F J, ARMSTRONG R W. Dislocation-mechanics-based constitutive relations for material dynamics calculation [J]. Journal of Applied Physics, 1987, 61(5): 1816–1825. doi: 10.1063/1.338024 [56] EVERS L P, BREKELMANS W A M, GEERS M G D. Scale dependent crystal plasticity framework with dislocation density and grain boundary effects [J]. International Journal of Solids and Structures, 2004, 41(18/19): 5209–5230. [57] KAZMI B, MURR L E. Anomalous residual shock hardening in nickel and stainless steel at a short pulse duration [J]. Scripta Metallurgica, 1979, 13(11): 993–997. doi: 10.1016/0036-9748(79)90191-1 [58] BRINGA E M, CARO A, VICTORIA M, et al. The atomistic modeling of wave propagation in nanocrystals [J]. Journal of the Minerals, Metals and Materials Society, 2005, 57(9): 67–70. doi: 10.1007/s11837-005-0119-9 [59] GLAM B, STRAUSS M, ELIEZER S, et al. Shock compression and spall formation in aluminum containing helium bubbles at room temperature and near the melting temperature: experiments and simulations [J]. International Journal of Impact Engineering, 2004, 65(4): 1–12. [60] DAVILA L P, ERHART P, BRINGA E M, et al. Atomistic modeling of shock-induced void collapse in copper [J]. Applied Physical Letters, 2005, 86(16): 161902. doi: 10.1063/1.1906307 [61] LUBARDA V A, SCHNEIDER M S, KALANTAR D H, et al. Void growth by dislocation emission [J]. Acta Materilia, 2004, 52(6): 1397–1408. doi: 10.1016/j.actamat.2003.11.022 [62] 王海燕. 氦泡对延性金属材料静态和动态力学性质影响的研究 [D]. 成都: 四川大学, 2008. [63] SHAO J L, WANG P, HE A M. Compression-induced stacking fault tetrahedral around He bubble in Al [J]. Journal of Applied Physics, 2011, 116: 163516. [64] REISMAN D B, WOLFER W G, ELSHOLZ A, et al. Isentropic compression of irradiated stainless steel on the Z accelerator [J]. Journal of Applied Physics, 2003, 93(11): 8952–8957. doi: 10.1063/1.1571969 [65] WEERTMAN J. Moving dislocations in the shock front [M]// MEYERS M A, MURR L E. Shock Waves and High Strain Rate Phenomena in Metals, 1981: 469. [66] GUMBSCH P, GAO H. Dislocations faster than the speed of sound [J]. Science, 1999, 283(5404): 965–968. doi: 10.1126/science.283.5404.965 [67] NOSENKO V, ZHDANOV S, MORFILL G. Supersonic dislocations observed in a plasma crystal [J]. Physical Review Letters, 2007, 99(2): 025002. doi: 10.1103/PhysRevLett.99.025002 [68] VOLGER T J. On measuring the strength of metals at ultrahigh strain rates [J]. Journal of Applied Physics, 2009, 106(5): 053530. doi: 10.1063/1.3204777 [69] ZARETSKY E B. Impact response of nickel in the 150–1150 K temperature range [J]. Journal of Applied Physics, 2009, 105: 093508. doi: 10.1063/1.3122523 [70] ZARETSKY E B, KANEL G I. Yield stress, polymorphic transformation, and spall fracture of shock-loaded iron in various structural states and at various temperatures [J]. Journal of Applied Physics, 2015, 117(19): 195901. doi: 10.1063/1.4921356 -







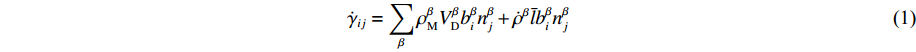

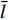
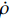


 下载:
下载:




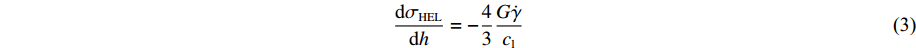

 下载:
下载: