Advances in the Study of Dynamic Response of Crystalline Materials by Crystal Plasticity Finite Element Modeling
-
摘要: 作为连续尺度上描述各向异性非均质材料弹塑性变形的重要模拟工具,晶体塑性有限元能够有效预测材料的宏观力学性能,在工程设计方面起着重要的作用。在实际工程应用中,许多晶体材料在高应力、高变形率、高温等极端条件下服役,此时各向异性非均匀的微介观结构演化是理解材料动态响应的关键,这给晶体塑性有限元带来了巨大的机遇和挑战。首先简要综述了晶体塑性有限元的原理和方法,然后着重介绍其在材料动态响应中的应用,最后展望其在材料动态响应模拟方面的发展方向。Abstract: As an important simulation tool for describing the elastoplastic deformation of anisotropic heterogeneous materials on continuum scales, crystal plasticity finite element (CPFE) modeling can effectively predict macroscopic mechanical properties of materials, thus plays a critical role in engineering design. In the practical engineering applications, many crystalline materials work at extreme conditions such as high stress, high deformation rate, and high temperature. The anisotropic heterogeneous microstructure evolutions under such conditions are the key factors to understanding the dynamic response of materials, and it brings great opportunities and challenges for CPFE. In this paper, we firstly review the theory and model of CPFE, and then introduce the applications of this method in study of dynamic response of crystalline materials, and discuss the challenges and open questions of CPFE in modeling material dynamic response at last.
-
炸药ZND模型认为爆轰波阵面有一定厚度,由极薄的前导冲击波和一定厚度的后继化学反应区组成,化学反应在反应区内进行并完成,冲击波和化学反应区以同一速度沿爆炸物向前传播。由于化学电离和热电离,爆轰波阵面处和化学反应区中会生成带电离子,这为爆电耦合效应(Explosion-electricity coupling,EEC)提供了一个基本条件。在炸药爆炸的过程中,给炸药的反应区中注入一定的外置电能,当大电流从导电的反应区流过时,将有一部分能量沉积到炸药的反应区中,与炸药本身的爆炸能量进行耦合,这部分能量可能通过焦耳加热,也可能通过更复杂的耦合形式来补充爆轰波能量,提升炸药输出威力。
1979年,Toton[1]研究了均匀的电场和磁场对凝聚炸药定常爆轰的影响,选取TNT作为凝聚炸药的代表,计算了焦耳加热对爆速和爆压的预期增益,理论上可以实现爆压最大增加12%和爆速增加6%。1999年,Lee等[2]研究了通过电能输入来提高炸药爆轰性能的可能性,向引爆的炸药提供5 kJ的电能,实验结果表明,炸药爆速平均提高2.7%~3.2%,局部提高8.2%~10.4%。2013年,Piehler等[3]将储存的电能从160 kJ(5.5 kV)电容器组转移到爆炸反应爆轰前沿后面的导电区,实验装置的爆炸部分由两块铜板(50.00 cm × 2.54 cm × 1.27 cm)组成,铜板之间由不同厚度的PrimaSheet-1000炸药层隔开,初步研究结果表明,在向反应区输入电能时,0.1 cm厚炸药的爆速提高了约4.2%,0.2 cm厚炸药的爆速提高了约2.6%。
上述研究均表明,外置电能的注入能够提升炸药的爆轰参数,证实了爆电耦合效应的可行性,但上述研究均以炸药爆速单一参量作为表征手段,且并未对炸药爆电耦合过程中的电学性能及反应过程进行解释。为此,本研究以平板塑性炸药为研究对象,设计了平板炸药的装药装置,使用自主组建的高能量脉冲功率电源系统,通过匹配炸药爆炸与脉冲电源放电时间使得爆电耦合能量最大化,新增以光子多普勒测速技术(Photonic Doppler velocimetry,PDV)测量的爆压作为爆电耦合增益的主要表征参量来进行爆电耦合研究。
1. 爆电耦合效应验证实验
1.1 样品与试验装置
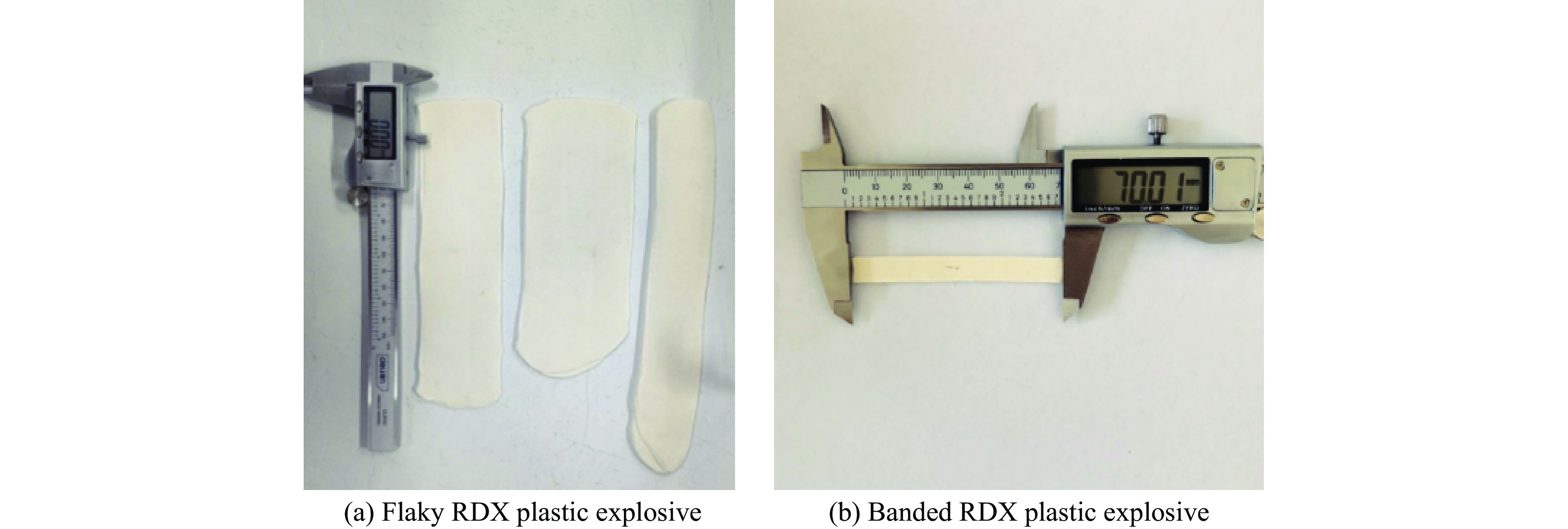
RDX塑性炸药为自制炸药,主要成分是质量分数为85%的黑索金和10%的天然橡胶。天然橡胶的添加使该塑性炸药成型晾干之后能够像橡皮一样具有很好的延展性,可以直接用刀片裁成试验需要的形状,在试验过程中受到挤压也不会断裂,非常适合爆电耦合效应研究。制作的塑性炸药如图1(a)所示,厚度为3 mm,用刀片裁切为如图1(b)所示的炸药条,尺寸为70 mm × 8 mm × 3 mm。
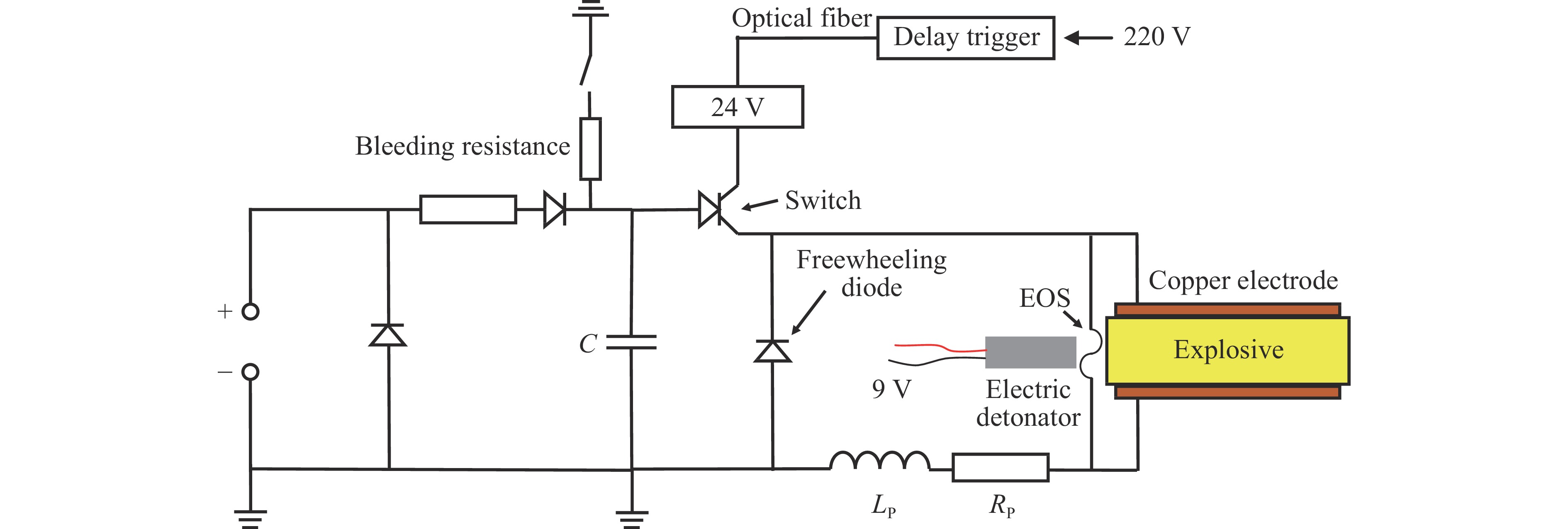
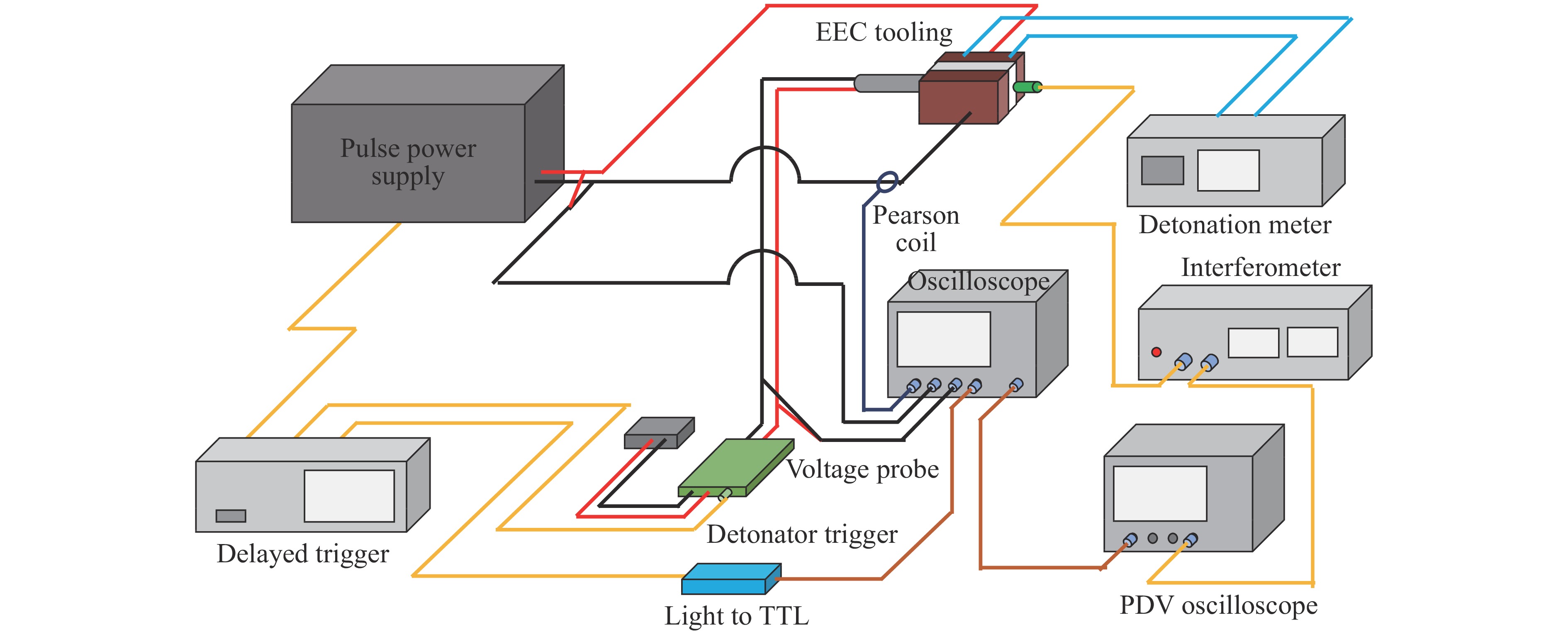
图2为爆电耦合试验示意图,其中LP为回路电感,RP为回路电阻。脉冲功率电源搭配两个2.5 mF的电容,充电电压为3 kV,能够提供22.5 kJ的能量,炸药爆炸开关(Explosive opening switch, EOS)为一设计好的导电铜箔,固定在铜电极两端,用于确保回路电流能够上升到峰值。当回路电流到达峰值时,电雷管炸断EOS开关并起爆炸药,当原本的电流回路被切断时,电流必然会寻找另一条电阻低的通路,此时炸药爆轰反应区中由于高导电率等离子体的存在,电流可以从狭小的反应区中流过,通过焦耳加热及其他耦合方式使电能耦合在反应区内,增大反应区粒子速度。泄放电阻和续流二极管用于保护电路,半导体开关用延迟触发装置通过光纤触发。
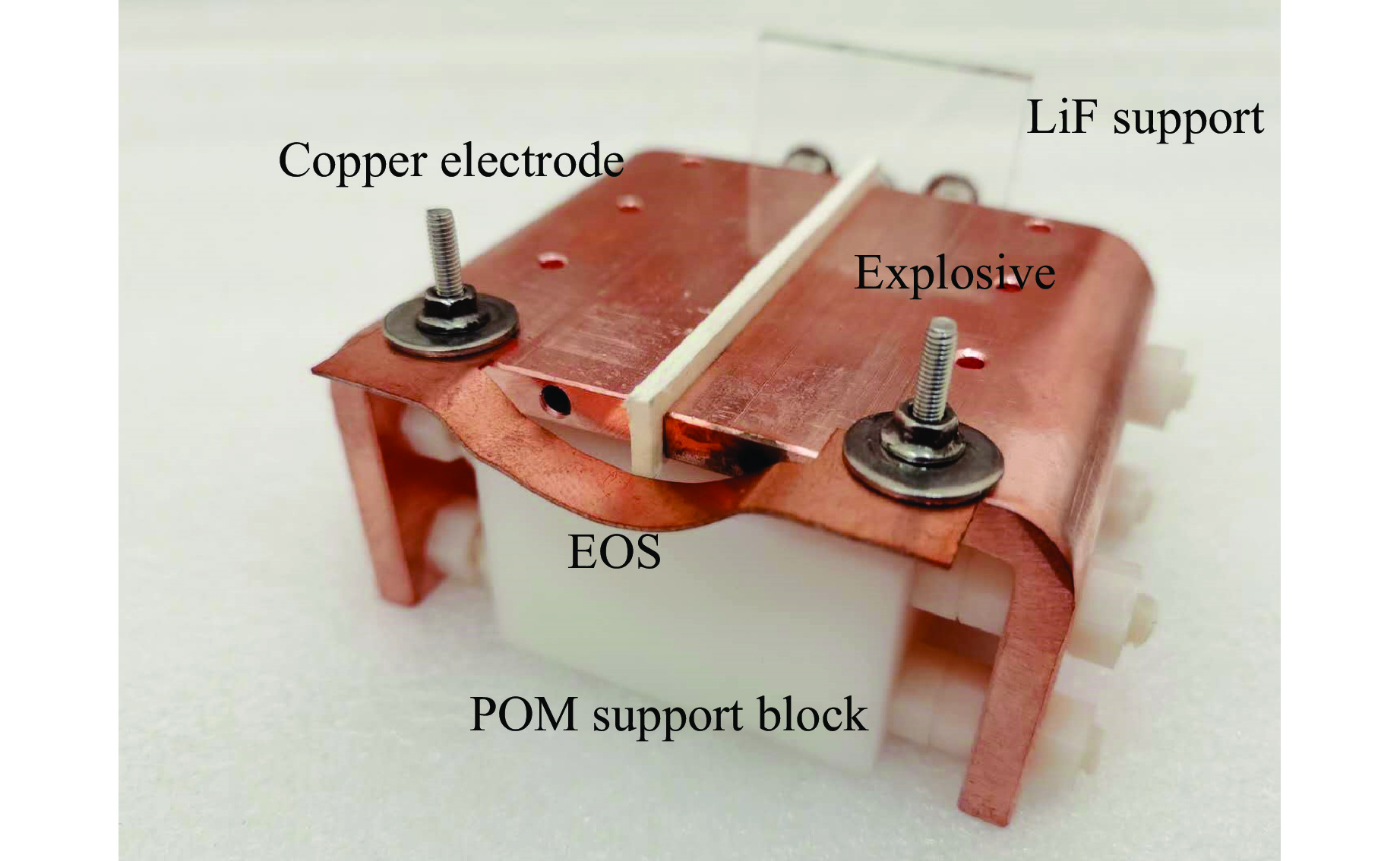
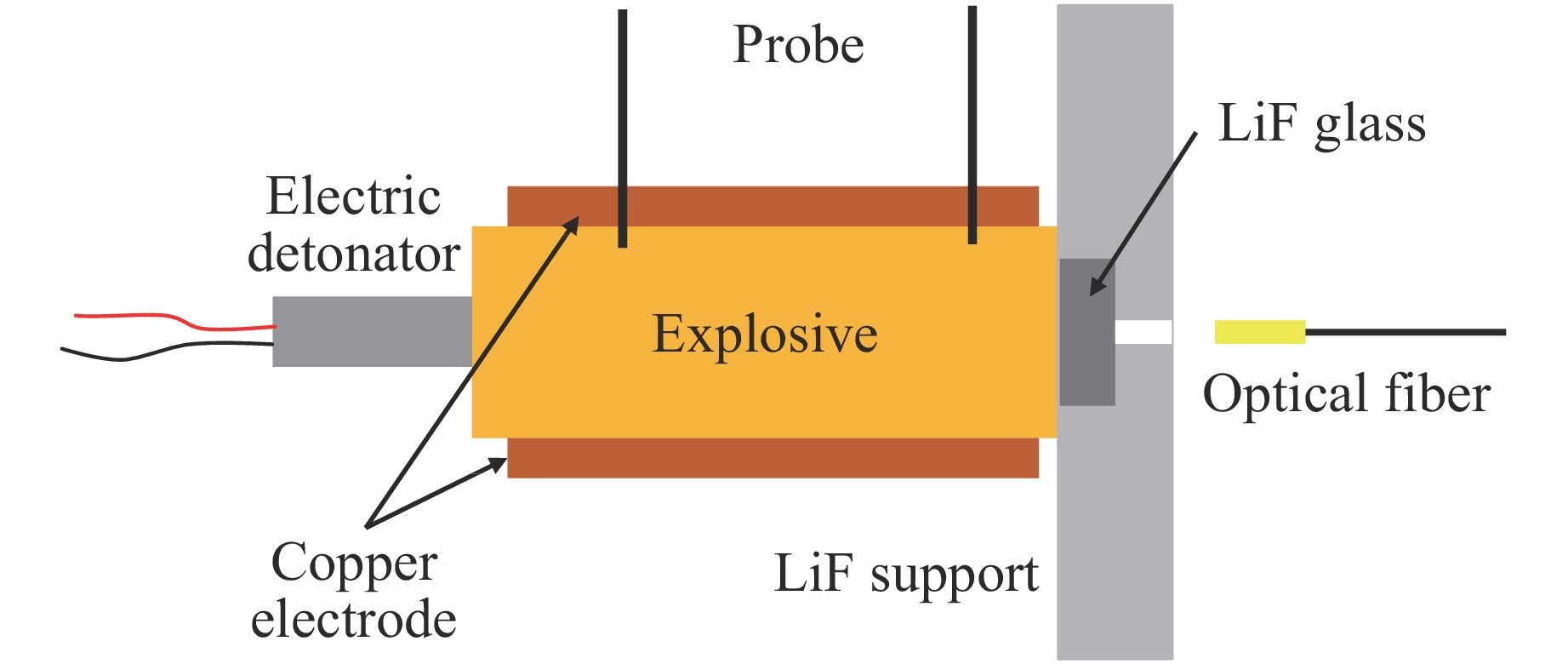
试验样品安装在如图3所示的工装中。工装整体由左右两块铜极与支撑块组成,炸药以长条片状夹在两铜极间,EOS开关用螺栓固定在两铜极的输入端,保证雷管在起爆炸药的同时可以炸断EOS开关。铜极侧面设计了4个
∅ 6 mm的通孔和一个M10的螺纹孔,使用M6的尼龙螺纹杆穿过通孔来连接支撑块和两铜极,螺纹孔用于连接回路电极。输出端设计两个M3的内螺纹孔,用于固定LiF支撑工装。1.2 实验过程
1.2.1 脉冲功率装置的放电规律
时间匹配是试验的关键点,要求在回路电流达到峰值时,雷管起爆炸药,切断EOS开关,从而将能量注入反应区中。试验时,因为续流二极管的存在,电流不会在回路中振荡,而是缓慢下降直至降为零。所以,需要确定回路电流达到电流峰值的时刻,才能在该时刻断开EOS开关、起爆炸药。同时,当炸药在回路电流达到峰值时刻起爆能保证有更多能量耦合到爆炸产物中,使爆电耦合效应达到最大化。为了减小时间匹配上的难度并保护某些回路元器件,在回路中增加了22 μH的电感。虽然电感的加入会降低回路中的峰值电流,但是可以显著延长回路放电时间,增大回路周期。
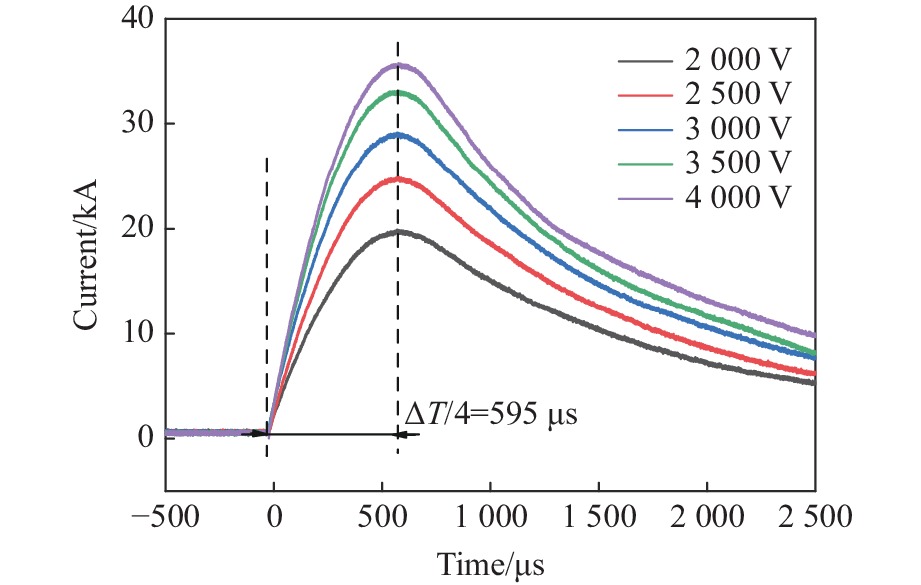
将脉冲功率装置各元器件按照电路图连接,电缆线长度等条件保持与后续试验一致,测试端为不带雷管的实验工装,使用Rogowski线圈准确记录回路中的电流信号。充电电源电压以2000 V为起点,以500 V为梯度进行回路短路电流测试,试验所测的短路放电电流曲线如图4所示,ΔT为放电周期。在回路负载确定的情况下(试验时加入EOS开关,约等于短路放电),回路1/4放电周期基本固定在595 μs左右。因此,在综合考虑雷管的作用时间和回路脉冲峰值时间之后,同步触发器的通道1设置为雷管触发回路,延迟时间为0 μs,通道2设置为回路电容放电半导体开关,延迟时间为1000
μs ,通道3设置为PDV采集信号,延迟时间为1600μs ,这样可以保证在雷管输出时回路中的电流已经达到或者接近电流峰值,且PDV光纤也能准确地采集到粒子速度曲线。1.2.2 炸药爆电耦合的爆速与爆压增益
试验以爆速和爆压作为主要表征参量来研究爆电耦合对炸药输出性能的影响,将不加电和加电两者测量的数据进行对比。其中爆速采用爆速仪测量,爆压则通过PDV测量炸药输出端界面粒子速度进而计算求得。
爆速仪测速装置原理:用直流电源起爆电雷管,电雷管起爆测试炸药,炸药开始爆轰,处在高温高压状态的炸药波阵面处的产物瞬间被电离成负离子和正离子;当炸药波阵面到达第1根探针时,探针导通,爆速仪记录下探针导通的时刻,计时开始;波阵面到达第2根探针时,探针同样输出一个电信号给爆速仪,此时计时结束;通过记录爆轰波经过两个波阵面的时间间隔Δt,根据放置探针的长度L,智能爆速仪可自行求解出所测炸药爆速D,并将其输出到显示屏上。
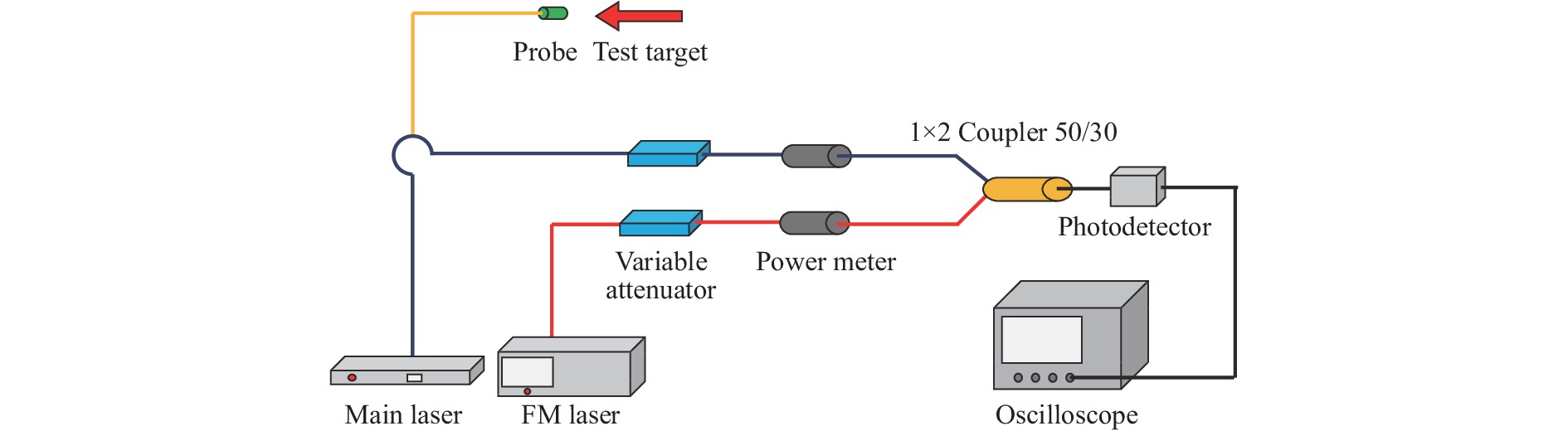
PDV测速技术的原理是基于物体运动的光学多普勒效应。将激光探头表面的反射光作为参考光,飞片表面的反射光作为信号光,参考光和信号光发生干涉后,利用探测器检测参考光和信号光的差拍干涉信号,并实现光信号到电信号的转换,电信号再经激光放大器放大,通过示波器采集,最后通过快速傅里叶变换处理,就能获得界面粒子的速度历程[4]。PDV是全光纤系统,通过参考光和反射光的频差获得速度信息,速度测量上限只受数字示波器的频带宽度限制,具有信噪比高、体积小和系统稳定等优点。图5为PDV作用原理示意图。
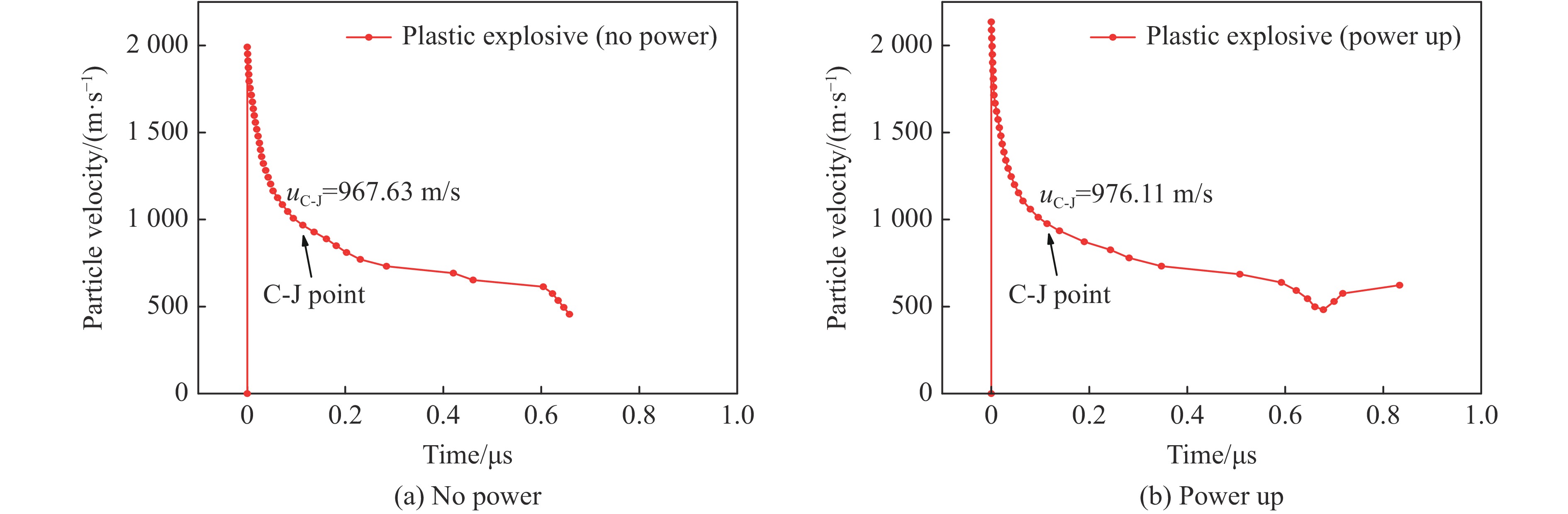
基于PDV测速技术,将界面粒子速度随时间的变化与ZND爆轰模型中的压力分布假设相对应,将速度曲线中出现的速率变化折点看作爆轰波结构中的Chapman-Jouguet(C-J)点,因此只要测出炸药爆轰产物界面粒子速度曲线,就可以得出爆轰反应结束时间和爆轰反应区宽度,进而求出C-J点和von Neumann(VN)峰压力[5-6]。相比于其他方法,该方法的物理过程比较明确,速度分辨率和时间分辨率均较高。
实验中,为了在某一加载压力下保持对样品较长的观测时间,通常在样品后端面粘贴透明窗口材料,形成加窗激光干涉测速系统。本试验使用的是LiF单晶窗口,在冲击波作用下,受窗口材料折射率变化的影响,加窗激光干涉测速系统的实测界面粒子速度不再等于真实的粒子速度,需要对窗口速度进行修正,真实的界面粒子速度与实测界面粒子速度之间的修正关系为[7]
us=uw1.2678 (1) 式中:us为真实粒子速度,uw为实测粒子速度。
界面粒子速度法的主要试验装置包括:爆电耦合装置、LiF玻璃、三维光学调控平台、激光干涉测速仪以及示波器。将表面镀了1
μm 厚铝膜的LiF玻璃嵌入支撑板的凹槽中,镀铝膜的一侧紧贴炸药,用螺丝与铜极紧固,光纤固定在三维可调光学平台上,调整光纤光斑使其正对支撑块上面的输出孔。图6为界面粒子速度测量示意图。在进行爆电耦合试验时,由于爆速和爆压两者的测量并不会互相影响,所以可以同时测量两个参量。图7为试验过程的完整示意图。同步触发器先触发雷管起爆装置,1000
μs 延迟后再触发脉冲回路开关,此时回路电流开始上升,1600μs 延迟左右回路电流达到峰值,雷管点火起爆炸药并炸断EOS开关,随着炸药爆轰的传播,依次触发爆速仪的探针1和探针2,爆速仪测出爆速,随后爆轰波从末端输出,PDV采集到紧贴在炸药输出端的Al膜运动速度,等效为输出端界面粒子速度。2. 结果与讨论
2.1 爆速增益与电学特性
塑性炸药的初始爆速v0为6364 m/s,比黑索金炸药的爆速8498 m/s低很多。其原因主要有以下两点:(1) 天然橡胶和添加剂的加入降低了黑索金炸药的质量比;(2) 该塑性炸药的密度仅为1.30 g/cm3,密度过小注定其爆速不会太高。为此,需要在相同的条件下进行加电与不加电实验,才能够最准确地表征爆电耦合效应的影响。
爆速仪测量数据如表1所示。由表1可知,在爆电耦合条件下,塑性炸药爆速vEEC增加了227 m/s,达到6591 m/s,增幅3.57%。由此可见,在爆电耦合条件下,爆速有一定的增长。从导电角度分析,大部分电能是从导电性较好的反应区流过,一小部分从爆轰产物区流过,此外还有极小部分通过击穿空气放电,这部分能量没有提升炸药的性能。假设爆轰产物区和击穿空气放电的能量占比较小,大部分电能沉积到炸药的冲击波阵面和化学反应区,则可以将整个反应时间内的累积电能作为沉积总电能进行计算。
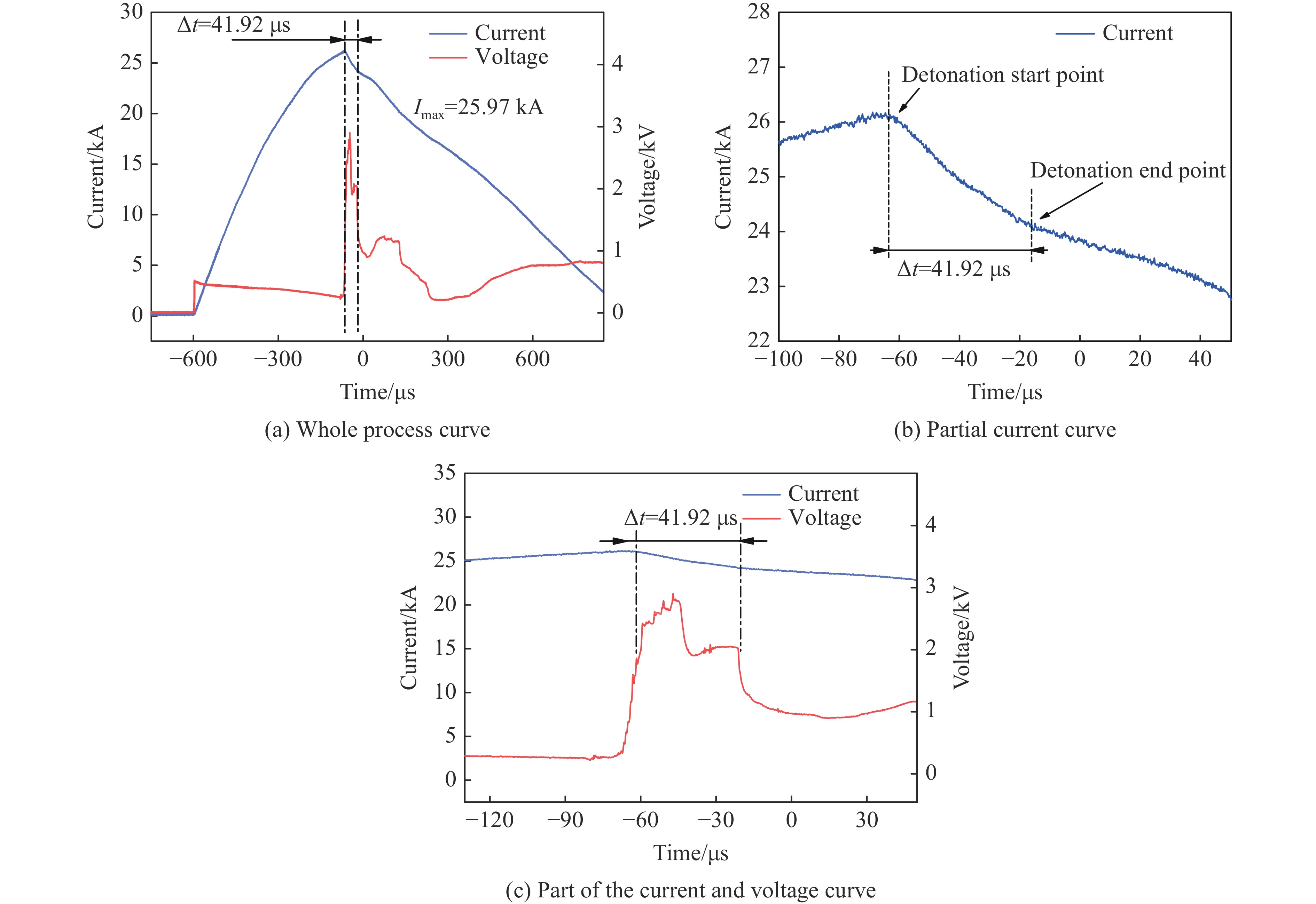
表 1 爆电耦合下塑性炸药的爆速增益Table 1. Detonation velocity gain of the plastic explosive under EECρ0/(g·cm−3) Thickness/mm v0/(m·s−1) vEEC/(m·s−1) Increment/(m·s−1) Percentage/% 1.30 3.00 6364 6591 227 3.57 使用Rogowski线圈和电压探头测量炸药两端的电流和电压参数,得到爆电耦合过程中回路电流和电压曲线,如图8所示。从爆电耦合电流和电压曲线可以看出:回路开关在−600.00 μs打开,电流开始上升。−79.61 μs时,电压曲线出现抖动,电压开始逐渐上升,这是因为雷管开始炸断EOS开关,铜箔电阻不断增大。−64.61 μs时,EOS开关被完全炸断,电压曲线出现一个小峰值,电雷管引爆塑性炸药开始绝热压缩并发生化学反应,电流从导电性能良好的EOS开关转移到爆轰反应区,回路电流出现抖动并逐渐下降,此时为爆轰开始点,之后电阻继续增大,电压曲线上升。−44.81 μs时,爆轰波成长至稳定,电压曲线出现峰值并下降。−22.69 μs时,电流曲线出现另一个抖动,电压曲线陡然下降,此为反应结束点,整个过程的持续时间为41.92 μs,之后剩下的电流通过击穿空气释放电能。根据之前设置的延迟时间计算可得,雷管点火时间为1535.39 μs,电流上升了535.39 μs,峰值电流为25.97 kA。
研究爆电耦合效应时,把爆轰波视为一个带有化学反应和注入了额外电能的冲击波处理,通过3个守恒方程推导出额外电能注入时爆速的计算公式
D2C-J=2(k2−1)(Q″+Ee) (2) 式中:DC-J为炸药爆速,单位m/s;k为多方指数,由于不加电和加电的试验条件一致,故可以通过不加电爆速试验求出多方指数,代入爆电耦合爆速计算公式中;
Q″ 为炸药的爆热,单位J/g,塑性炸药为自制炸药,根据经验公式可计算出该塑性炸药的爆热为5020.96 J/g;Ee为单位质量炸药所获得的电能,单位J/g,将电流和电压曲线的乘积对反应时间进行积分,所得能量除以炸药质量可得Ee,即Ee=1m∫tbtaUIdt (3) 式中:ta为整个反应的开始时间,单位
μs ;tb为整个反应的结束时间,单位μs ;U为对应时刻的电压,单位V;I为对应时刻的电流,单位A;m为炸药质量,单位g。经过计算,塑性炸药经过爆电耦合效应后,预测爆速为6577.62 m/s,与实测爆速6591 m/s比较,相对误差仅为0.2%。反应过程中,沉积在反应区的能量为711.32 J,能量利用率仅为3.16%,说明爆电耦合效应研究仍有极大的进步空间。
2.2 爆压增益
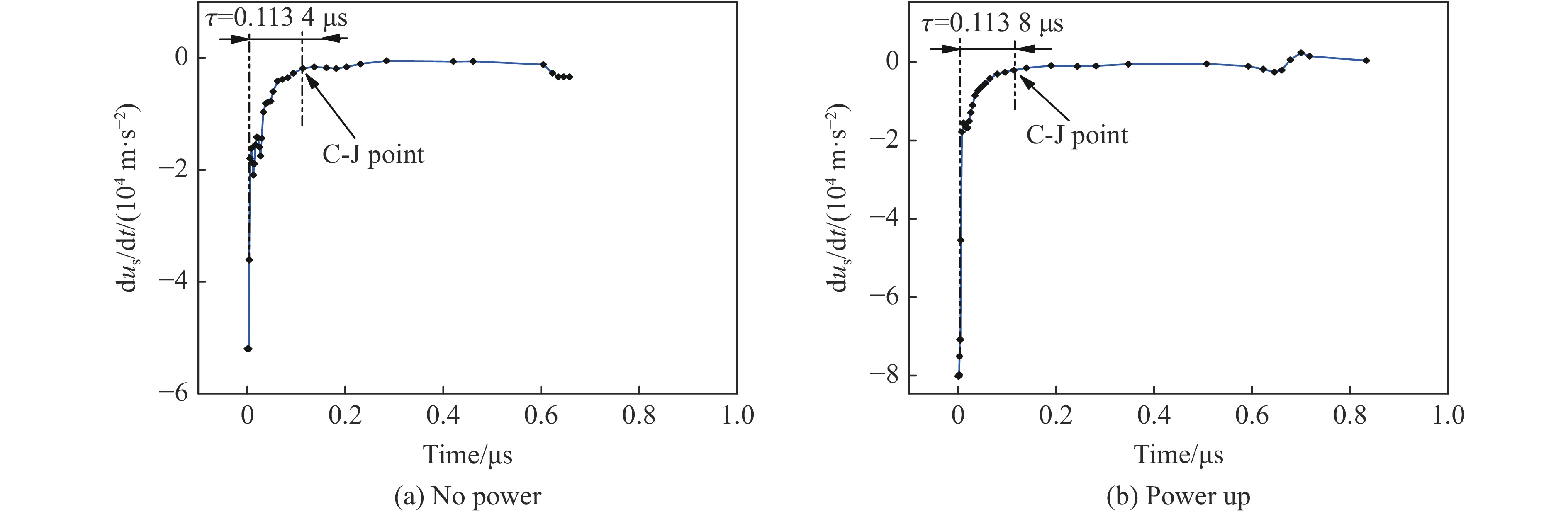
PDV示波器的带宽为33 GHz,采样率为80 GSa/s,利用PDV测量样品-LiF窗口界面粒子速度,得到原始数据,经过傅里叶变换之后,得到未修正粒子速度原始图。对粒子速度原始图进行描点操作,随后用式(1)进行修正,得到修正后的界面粒子速度-时间曲线,如图9所示。由图9可知,粒子速度-时间曲线上存在一个明显的拐点(C-J点),这个拐点将曲线分成两部分,对应ZND模型中的爆轰反应区和Taylor膨胀区。为了确定该点的具体位置,对光滑后的界面粒子速度进行一阶求导,如图10所示。dus/dt曲线在初始阶段上升较快,对应炸药的快反应阶段,炸药化学反应的能量释放主要发生在这一阶段。随后dus/dt曲线缓慢下降,该过程对应炸药的慢反应阶段。炸药化学反应结束后,即C-J点之后,爆轰产物发生膨胀,受稀疏波的影响,界面粒子速度缓慢下降,对应的界面粒子速度一阶导数为接近于零的定值,通过读取dus/dt曲线上的拐点可以确定C-J点。
由平板装药的界面粒子速度-时间曲线(图9)可得:先导冲击波过后,粒子速度出现突跃,随后化学反应开始,界面粒子速度经历两个阶段:第1阶段,粒子速度下降较快,时间在100 ns以内,为快速化学反应阶段;第2阶段,粒子速度变化较平稳,持续时间较长,对应小颗粒固相碳凝聚过程。反应前期粒子速度均下降较快,这是因为炸药太薄,稀疏波很快反射回来,导致冲击波下降速度变快。
当试验确定了塑性炸药的VN峰值粒子速度uVN和C-J点的粒子速度uC-J之后,则可以利用冲击波阻抗匹配关系计算炸药反应区内的压力[8-9]
p=12us[ρm0(C0+λus)+ρ0DC-J] (4) 式中:p为炸药界面与窗口处的压力,单位GPa;us为界面粒子速度,单位km/s;
ρ m0为LiF窗口的初始密度,单位g/cm3;C0和λ 为LiF窗口的冲击绝热线常数;ρ 0为炸药的初始密度,单位g/cm3;DC-J的单位为km/s;ρ m0为LiF窗口材料的初始密度,为2.641 g/cm3,C0 =(5.176 ± 0.023)km/s,λ = 1.353 ± 0.010。设a为炸药反应区宽度(单位mm),
τ 为化学反应持续时间(单位μs ),则a=∫τ0(DC-J−us)dt (5) 由图9可以得到VN峰值点时刻与C-J点时刻,以此求出炸药反应区持续时间,之后根据式(4)计算各炸药的VN峰值压力pVN与C-J点压力pC-J,再根据式(5)确定炸药化学反应区宽度[10-11],得到塑性炸药平板装药在爆电耦合作用下的爆压增益
δpVN 和δpC-J ,如表2所示。表 2 塑性炸药爆电耦合效应爆压增益情况Table 2. Detonation pressure gain of the plastic explosive under EECLoading conditions uVN/(m·s−1) uC-J/(m·s−1) pVN/GPa pC-J/GPa τ/μs a/mm δpVN/% δpC-J/% No power 1990.70 967.63 28.98 12.32 0.1134 0.58 10.28 2.19 Power up 2135.57 976.11 31.96 12.59 0.1138 0.61 由表2可知,爆电耦合效应对塑性炸药的压力参数有一定的提升作用,VN峰值点压力提升了10.28%,C-J点压力提升了2.19%,VN峰值点的压力增益大于C-J点压力增益。根据分析,电能沉积的主要区域为后续的化学反应区,但是沉积在化学反应区的电能并不能导致塑性炸药前导冲击波处VN峰值压力的提升,因此VN峰值处压力的提升是由于外部电场的影响,并且外电场对炸药爆压的影响大于注入反应区中电能的影响。研究表明,随着外电场的增强,炸药的引发键键长变短,解离能增加[12-13]。另外,外电场的加入,也在一定程度上增加了爆速,这也解释了为什么用沉积能量计算出来的炸药爆速小于实际爆速。
3. 结 论
针对电能与炸药爆炸的化学能结合问题,设计、加工、组装了一系列完整的爆电耦合效应加载及测试装置,使用爆速仪测量塑性炸药爆速,用PDV测速系统测量塑性炸药表面粒子速度,从典型的爆速和爆压参数对塑性炸药进行爆电耦合效应评估,得出以下结论。
(1) 试验结果表明,爆电耦合作用使塑性炸药的爆速提高了3.57%,VN峰值压力提高了10.28%,C-J压力提高了2.19%。
(2) 通过电流和电压曲线的变化规律,定性地分析了爆电耦合效应的全过程,并将沉积的电能代入推导的爆速计算公式,对爆电耦合效应产生的爆速增益进行了预测。经验证,该计算公式的计算误差仅为0.2%,对于爆电耦合效应计算具有一定的参考价值。
(3) 分析了塑性炸药爆电耦合效应的可能作用机理,解释了炸药爆速增长和爆压增长的主要原因,其中爆速增长的主要影响因素为反应区中注入的电能。VN峰值压力的增长主要是外电场的影响,在炸药爆炸之前,两个高压电极之间的外部电场缩短了关键化学键的结合长度,从而增大了炸药输出能量。
感谢南京理工大学钱华研究员和李宛桐同学提供了RDX塑性炸药,感谢西安近代化学研究所宋浦研究员和南京理工大学韩志伟副研究员对研究工作的指导。
-
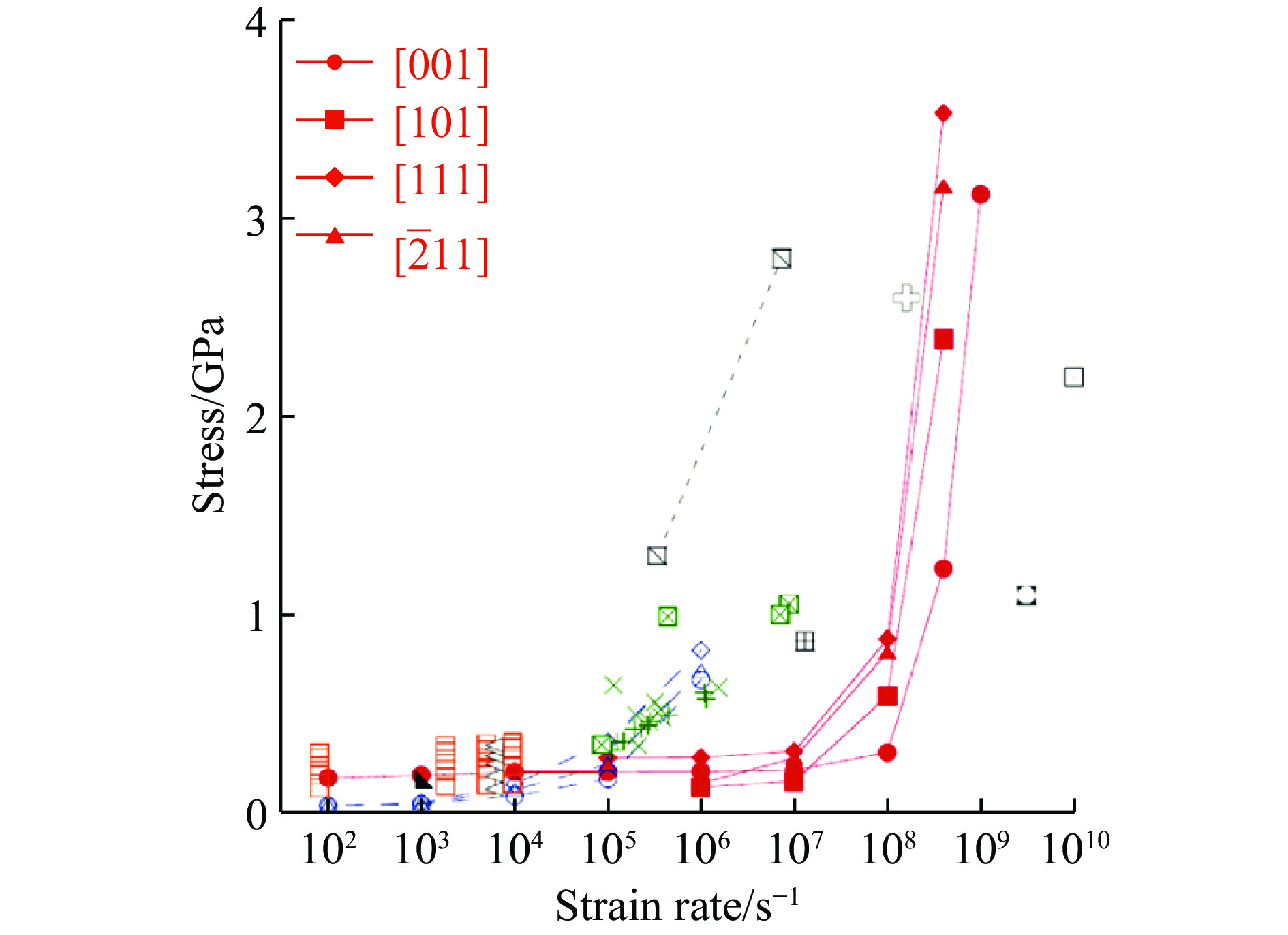
图 4 RDX在[111]方向受到1.25 GPa加载时粒子速度剖面[100](实线是实验结果[101],虚线为模拟结果)
Figure 4. Particle velocity profile of
α -RDX single crystal loaded up to 1.25 GPa along the [111] crystallographic direction (Solid lines correspond to the experimental results [101] while dashed lines correspond to the model predictions [100].)图 5 利用Lagrangean框架建立的唯象CPFE模拟等径角板牙的高应变率变形:(a)几何构型,其中V为此区域外加的速度场;(b)变形率分布[102]
Figure 5. High strain rate deformation of equal-diameter dies simulated by the phenomenological CPFE within the Lagrangean framework. (a) shows the geometry configuration where V is the imposed velocity in the specified domains, and (b) is the deformation rate distribution [102]
图 6 利用Winey-Gupta模型模拟LiF受[100]方向冲击时的纵波历史(实线为实验测量值,虚线为模拟值;曲线上方数字表示试样的厚度,单位为毫米[105])
Figure 6. Measured and simulated longitudinal stress histories for LiF single crystals shocked along the [100] orientation by Winey-Gupta model [105] (The solid lines are the experimental data and the dashed lines are the simulations. The numbers above the curves indicate the sample thickness in mm. Time is relative to the moment of impact.)
图 7 利用Austin- McDowell模型模拟6061-T6的Hugoniot塑性变形的临界剪切应力(数据点为实验结果;左上角给出了数据点的来源,尺寸参数表示晶粒尺寸,具体信息见文献[106])
Figure 7. The critical shear stress of Hugoniot plastic deformation of 6061-T6 simulated by the Austin-McDowell model (The symbols show the experimental results. The legend gives the sources of the experimental data, and the measurements show the grain sizes, please see Ref.[106] for the detail information.)
-
[1] TAYLOR G I. Plastic strain in metals [J]. Journal of the Institute of Metals, 1938, 62: 307–324. [2] BISHOP J F W, HILL R. A theory of the plastic distortion of a polycrystalline aggregate under combined stresses [J]. The London, Edinburgh, and Dublin Philosophical Magazine and Journal of Science, 1951, 42(327): 414–427. doi: 10.1080/14786445108561065 [3] BISHOP J F W, HILL R. A theoretical derivation of the plastic properties of a polycrystalline face-centered metal [J]. Philosophical Magazine, 1951, 42(334): 1298–1307. [4] KRONER E. On the plastic deformation of polycrystals [J]. Acta Metallurgica, 1961, 9(2): 155–161. doi: 10.1016/0001-6160(61)90060-8 [5] CURTIN W A, MILLER R E. Atomistic/continuum coupling in computational materials science [J]. Modelling and Simulation in Materials Science and Engineering, 2003, 11(3): R33. doi: 10.1088/0965-0393/11/3/201 [6] ARSENLIS A, PARKS D M, BECKER R, et al. On the evolution of crystallographic dislocation density in non-homogeneously deforming crystals [J]. Journal of the Mechanics and Physics of Solids, 2004, 52(6): 1213–1246. doi: 10.1016/j.jmps.2003.12.007 [7] VITEK V, MROVEC M, BASSANI J L. Influence of non-glide stresses on plastic flow: from atomistic to continuum modeling [J]. Materials Science and Engineering: A, 2004, 365(1/2): 31–37. [8] ZIENKIEWICZ O C, CHEUNG Y K. The finite element method in structural and continuum mechanics [M]. London: McGraw-Hill, 1967. [9] ZIENKIEWICZ O C, TAYLOR R L. The finite element method for solid and structural mechanics [M]. Elsevier Ltd, 2005. [10] ZHAO Z, MAO W, ROTERS F, et al. A texture optimization study for minimum earing in aluminium by use of a texture component crystal plasticity finite element method [J]. Acta Materialia, 2004, 52(4): 1003–1012. doi: 10.1016/j.actamat.2003.03.001 [11] RICE J R. Inelastic constitutive relations for solids: an internal-variable theory and its application to metal plasticity [J]. Journal of the Mechanics and Physics of Solids, 1971, 19(6): 433–455. doi: 10.1016/0022-5096(71)90010-X [12] ASARO R J, RICE J R. Strain localization in ductile single crystals [J]. Journal of the Mechanics and Physics of Solids, 1977, 25(5): 309–338. doi: 10.1016/0022-5096(77)90001-1 [13] ARSENLIS A, PARKS D M. Crystallographic aspects of geometrically-necessary and statistically-stored dislocation density [J]. Acta Materialia, 1999, 47(5): 1597–1611. doi: 10.1016/S1359-6454(99)00020-8 [14] ARSENLIS A, PARKS D M. Modeling the evolution of crystallographic dislocation density in crystal plasticity [J]. Journal of the Mechanics and Physics of Solids, 2002, 50(9): 1979–2009. doi: 10.1016/S0022-5096(01)00134-X [15] EVERS L P, PARKS D M, BREKELMANS W A M, et al. Crystal plasticity model with enhanced hardening by geometrically necessary dislocation accumulation [J]. Journal of the Mechanics and Physics of Solids, 2002, 50(11): 2403–2424. doi: 10.1016/S0022-5096(02)00032-7 [16] EVERS L P, BREKELMANS W A M, GEERS M G D. Scale dependent crystal plasticity framework with dislocation density and grain boundary effects [J]. International Journal of Solids and Structures, 2004, 41(18/19): 5209–5230. [17] CHEONG K S, BUSSO E P. Discrete dislocation density modelling of single phase FCC polycrystal aggregates [J]. Acta Materialia, 2004, 52(19): 5665–5675. doi: 10.1016/j.actamat.2004.08.044 [18] MA A, ROTERS F. A constitutive model for fcc single crystals based on dislocation densities and its application to uniaxial compression of aluminium single crystals [J]. Acta Materialia, 2004, 52(12): 3603–3612. doi: 10.1016/j.actamat.2004.04.012 [19] MA A, ROTERS F, RAABE D. A dislocation density based constitutive model for crystal plasticity FEM including geometrically necessary dislocations [J]. Acta Materialia, 2006, 54(8): 2169–2179. doi: 10.1016/j.actamat.2006.01.005 [20] MA A, ROTERS F, RAABE D. On the consideration of interactions between dislocations and grain boundaries in crystal plasticity finite element modeling-theory, experiments, and simulations [J]. Acta Materialia, 2006, 54(8): 2181–2194. doi: 10.1016/j.actamat.2006.01.004 [21] EVERS L P, BREKELMANS W A M, GEERS M G D. Non-local crystal plasticity model with intrinsic SSD and GND effects [J]. Journal of the Mechanics and Physics of Solids, 2004, 52(10): 2379–2401. doi: 10.1016/j.jmps.2004.03.007 [22] THAMBURAJA P, ANAND L. Polycrystalline shape-memory materials: effect of crystallographic texture [J]. Journal of the Mechanics and Physics of Solids, 2001, 49(4): 709–737. doi: 10.1016/S0022-5096(00)00061-2 [23] LAN Y J, XIAO N M, LI D Z, et al. Mesoscale simulation of deformed austenite decomposition into ferrite by coupling a cellular automaton method with a crystal plasticity finite element model [J]. Acta Materialia, 2005, 53(4): 991–1003. doi: 10.1016/j.actamat.2004.10.045 [24] KALIDINDI S R. Incorporation of deformation twinning in crystal plasticity models [J]. Journal of the Mechanics and Physics of Solids, 1998, 46(2): 267–290. doi: 10.1016/S0022-5096(97)00051-3 [25] STAROSELSKY A, ANAND L. Inelastic deformation of polycrystalline face centered cubic materials by slip and twinning [J]. Journal of the Mechanics and Physics of Solids, 1998, 46(4): 671–696. doi: 10.1016/S0022-5096(97)00071-9 [26] MARKETZ W T, FISCHER F D, KAUFFMANN F, et al. On the role of twinning during room temperature deformation of γ-TiAl based alloys [J]. Materials Science & Engineering A, 2002, 329: 177–183. [27] SALEM A A, KALIDINDI S R, SEMIATIN S L. Strain hardening due to deformation twinning in α-titanium: constitutive relations and crystal-plasticity modeling [J]. Acta Materialia, 2005, 53(12): 3495–3502. doi: 10.1016/j.actamat.2005.04.014 [28] WEI Y J, ANAND L. Grain-boundary sliding and separation in polycrystalline metals: application to nanocrystalline fcc metals [J]. Journal of the Mechanics and Physics of Solids, 2004, 52(11): 2587–2616. doi: 10.1016/j.jmps.2004.04.006 [29] WEI Y, SU C, ANAND L. A computational study of the mechanical behavior of nanocrystalline fcc metals [J]. Acta Materialia, 2006, 54(12): 3177–3190. doi: 10.1016/j.actamat.2006.03.007 [30] RAABE D, KLOSE P, ENGL B, et al. Concepts for integrating plastic anisotropy into metal forming simulations [J]. Advanced Engineering Materials, 2002, 4(4): 169–180. doi: 10.1002/1527-2648(200204)4:4<>1.0.CO;2-G [31] SACHTLEBER M, ZHAO Z, RAABE D. Experimental investigation of plastic grain interaction [J]. Materials Science and Engineering A, 2002, 336(1/2): 81–87. [32] RAABE D, SACHTLEBER M, WEILAND H, et al. Grain-scale micromechanics of polycrystal surfaces during plastic straining [J]. Acta Materialia, 2003, 51(6): 1539–1560. doi: 10.1016/S1359-6454(02)00557-8 [33] ROTERS F, EISENLOHR P, HANTCHERLI L, et al. Overview of constitutive laws, kinematics, homogenization and multiscale methods in crystal plasticity finite-element modeling: theory, experiments, applications [J]. Acta Materialia, 2010, 58(4): 1152–1211. doi: 10.1016/j.actamat.2009.10.058 [34] SALVADO F C, TEIXEIRA-DIAS F, WALLEY S M, et al. A review on the strain rate dependency of the dynamic viscoplastic response of FCC metals [J]. Progress in Materials Science, 2017, 88: 186–231. doi: 10.1016/j.pmatsci.2017.04.004 [35] MOLINARI A, RAVICHANDRAN G. Constitutive modeling of high-strain-rate deformation in metals based on the evolution of an effective microstructural length [J]. Mechanics of Materials, 2005, 37(7): 737–752. doi: 10.1016/j.mechmat.2004.07.005 [36] JOHNSON G R. A constitutive model and data for metals subjected to large strains, high strain rates, and high temperatures [C]. Proceedings of the 7th International Symposium on Ballistics, 1983: 541-547. [37] KHAN A S, HUANG S. Experimental and theoretical study of mechanical behavior of 1100 aluminum in the strain rate range 10-5–104 s-1 [J]. International Journal of Plasticity, 1992, 8(4): 397–424. doi: 10.1016/0749-6419(92)90057-J [38] LIN Y C, CHEN X M. A critical review of experimental results and constitutive descriptions for metals and alloys in hot working [J]. Materials & Design, 2011, 32(4): 1733–1759. [39] KOCKS U F. Laws for work-hardening and low-temperature creep [J]. Journal of Engineering Materials and Technology, 1976, 98(1): 76–85. doi: 10.1115/1.3443340 [40] LIN Y C, CHEN M S, ZHONG J. Constitutive modeling for elevated temperature flow behavior of 42CrMo steel [J]. Computational Materials Science, 2008, 42(3): 470–477. doi: 10.1016/j.commatsci.2007.08.011 [41] BODNER S R, PARTOM Y. Constitutive equations for elastic-viscoplastic strain-hardening materials [J]. Journal of Applied Mechanics, 1975, 42(2): 385–389. doi: 10.1115/1.3423586 [42] STEINBERG D J, COCHRAN S G, GUINAN M W. A constitutive model for metals applicable at high-strain rate [J]. Journal of Applied Physics, 1980, 51(3): 1498–1504. doi: 10.1063/1.327799 [43] ZERILLI F J, ARMSTRONG R W. Dislocation-mechanics-based constitutive relations for material dynamics calculations [J]. Journal of Applied Physics, 1987, 61(5): 1816–1825. doi: 10.1063/1.338024 [44] MEYER H W JR. A modified Zerilli-Armstrong constitutive model describing the strength and localizing behavior of Ti-6A1-4V: ARL-CR-0578 [R]. Aberdeen, MD: Dynamic Science Inc., 2006. [45] MECKING H, KOCKS U F. Kinetics of flow and strain-hardening [J]. Acta Metallurgica, 1981, 29(11): 1865–1875. doi: 10.1016/0001-6160(81)90112-7 [46] NEMAT-NASSER S, LI Y. Flow stress of fcc polycrystals with application to OFHC Cu [J]. Acta Materialia, 1998, 46(2): 565–577. doi: 10.1016/S1359-6454(97)00230-9 [47] TAYLOR G I. The mechanism of plastic deformation of crystals. Part I. theoretical [J]. Proceedings of the Royal Society of London A, 1934, 145(855): 362–387. doi: 10.1098/rspa.1934.0106 [48] Taylor G I. The mechanism of plastic deformation of crystals. Part II. comparison with observations [J]. Proceedings of the Royal Society of London A, 1934, 145(855): 388–404. doi: 10.1098/rspa.1934.0107 [49] OROWAN E. Zur Kristall Plastizität IIII [J]. Zeitschrift für Physik, 1934, 89: 605–659. doi: 10.1007/BF01341478 [50] POLANYI M. Über eine Art Gitterstörung, die einen Kristall plastisch machen könnte [J]. Zeitschrift für Physik, 1934, 89(9/10): 660–664. [51] PEIRCE D, ASARO R J, NEEDLEMAN A. An analysis of nonuniform and localized deformation in ductile single crystals [J]. Acta Metallurgica, 1982, 30(6): 1087–1119. doi: 10.1016/0001-6160(82)90005-0 [52] HARREN S V, DEVE H E, ASARO R J. Shear band formation in plane strain compression [J]. Acta Metallurgica, 1988, 36(9): 2435–2480. doi: 10.1016/0001-6160(88)90193-9 [53] HARREN S V, ASARO R J. Nonuniform deformations in polycrystals and aspects of the validity of the Taylor model [J]. Journal of the Mechanics and Physics of Solids, 1989, 37(2): 191–232. doi: 10.1016/0022-5096(89)90010-0 [54] BECKER R. Analysis of texture evolution in channel die compression-I. effects of grain interaction [J]. Acta Metallurgica et Materialia, 1991, 39(6): 1211–1230. doi: 10.1016/0956-7151(91)90209-J [55] BECKER R, BUTLER J F, HU H, et al. Analysis of an aluminum single crystal with unstable initial orientation (001)[110] in channel die compression [J]. Metallurgical Transactions A, 1991, 22(1): 45–58. doi: 10.1007/BF03350948 [56] ZHAO Z, RAMESH M, RAABE D, et al. Investigation of three-dimensional aspects of grain-scale plastic surface deformation of an aluminum oligocrystal [J]. International Journal of Plasticity, 2008, 24(12): 2278–2297. doi: 10.1016/j.ijplas.2008.01.002 [57] MIKA D P, DAWSON P R. Effects of grain interaction on deformation in polycrystals [J]. Materials Science and Engineering: A, 1998, 257(1): 62–76. doi: 10.1016/S0921-5093(98)00824-7 [58] SARMA G B, DAWSON P R. Texture predictions using a polycrystal plasticity model incorporating neighbor interactions [J]. International Journal of Plasticity, 1996, 12(8): 1023–1054. doi: 10.1016/S0749-6419(96)00040-X [59] SARMA G B, RADHAKRISHNAN B, ZACHARIA T. Finite element simulations of cold deformation at the mesoscale [J]. Computational Materials Science, 1998, 12(2): 105–123. doi: 10.1016/S0927-0256(98)00036-6 [60] BEAUDOIN A J JR, MECKING H, KOCKS U F. Development of localized orientation gradients in fcc polycrystals [J]. Philosophical Magazine A, 1996, 73(6): 1503–1517. doi: 10.1080/01418619608242998 [61] BACHU V, KALIDINDI S R. On the accuracy of the predictions of texture evolution by the finite element technique for fcc polycrystals [J]. Materials Science and Engineering A, 1998, 257(1): 108–117. doi: 10.1016/S0921-5093(98)00828-4 [62] ZHAO Z, KUCHNICKI S, RADOVITZKY R, et al. Influence of in-grain mesh resolution on the prediction of deformation textures in fcc polycrystals by crystal plasticity FEM [J]. Acta Materialia, 2007, 55(7): 2361–2373. doi: 10.1016/j.actamat.2006.11.035 [63] ZHAO Z, ROTERS F, MAO W, et al. Introduction of a texture component crystal plasticity finite element method for anisotropy simulations [J]. Advanced Engineering Materials, 2001, 3(12): 984–990. doi: 10.1002/1527-2648(200112)3:12<984::AID-ADEM984>3.0.CO;2-L [64] RAABE D, ROTERS F. Using texture components in crystal plasticity finite element simulations [J]. International Journal of Plasticity, 2004, 20(3): 339–361. doi: 10.1016/S0749-6419(03)00092-5 [65] MELCHIOR M A, DELANNAY L. A texture discretization technique adapted to polycrystalline aggregates with non-uniform grain size [J]. Computational Materials Science, 2006, 37(4): 557–564. doi: 10.1016/j.commatsci.2005.12.002 [66] TÓTH L S, VAN HOUTTE P. Discretization techniques for orientation distribution functions [J]. Texture, Stress, and Microstructure, 1992, 19(4): 229–244. doi: 10.1155/TSM.19.229 [67] EISENLOHR P, ROTERS F. Selecting a set of discrete orientations for accurate texture reconstruction [J]. Computational Materials Science, 2008, 42(4): 670–678. doi: 10.1016/j.commatsci.2007.09.015 [68] FLECK N A, MULLER G M, ASHBY M F, et al. Strain gradient plasticity: theory and experiment [J]. Acta Metallurgica et Materialia, 1994, 42(2): 475–487. doi: 10.1016/0956-7151(94)90502-9 [69] FLECK N A, HUTCHINSON J W. Strain gradient plasticity [J]. Advances in Applied Mechanics, 1997, 33: 296–361. [70] NIX W D, GAO H. Indentation size effects in crystalline materials: a law for strain gradient plasticity [J]. Journal of the Mechanics and Physics of Solids, 1998, 46(3): 411–425. doi: 10.1016/S0022-5096(97)00086-0 [71] GAO H, HUANG Y. Geometrically necessary dislocation and size-dependent plasticity [J]. Scripta Materialia, 2003, 48(2): 113–118. doi: 10.1016/S1359-6462(02)00329-9 [72] BIELER T R, EISENLOHR P, ROTERS F, et al. The role of heterogeneous deformation on damage nucleation at grain boundaries in single phase metals [J]. International Journal of Plasticity, 2009, 25(9): 1655–1683. doi: 10.1016/j.ijplas.2008.09.002 [73] STAROSELSKY A, ANAND L. A constitutive model for hcp materials deforming by slip and twinning: application to magnesium alloy AZ31B [J]. International Journal of Plasticity, 2003, 19(10): 1843–1864. doi: 10.1016/S0749-6419(03)00039-1 [74] SUIKER A S J, TURTELTAUB S. Computational modelling of plasticity induced by martensitic phase transformations [J]. International Journal for Numerical Methods in Engineering, 2005, 63(12): 1655–1693. doi: 10.1002/(ISSN)1097-0207 [75] HUTCHINSON J W. Bounds and self-consistent estimates for creep of polycrystalline materials [J]. Proceedings of the Royal Society of London A, 1976, 348(1652): 101–127. doi: 10.1098/rspa.1976.0027 [76] PEIRCE D, ASARO R J, NEEDLEMAN A. Material rate dependence and localized deformation in crystalline solids [J]. Acta Metallurgica, 1983, 31(12): 1951–1976. doi: 10.1016/0001-6160(83)90014-7 [77] VOCE E. The relationship between stress and strain for homogeneous deformation [J]. Journal of the Institute of Metals, 1948, 74: 537–562. [78] BRONKHORST C A, KALIDINDI S R, ANAND L. Polycrystalline plasticity and the evolution of crystallographic texture in FCC metals [J]. Philosophical Transactions of the Royal Society A, 1992, 341(1662): 443–477. [79] HALL E O. The deformation and ageing of mild steel: III. discussion of results [J]. Proceedings of the Physical Society: Section B, 1951, 64(9): 747. doi: 10.1088/0370-1301/64/9/303 [80] PETCH N J. The cleavage strength of polycrystals [J]. Journal of the Iron and Steel Institute, 1953, 174: 25–28. [81] NYE J F. Some geometrical relations in dislocated crystals [J]. Acta Metallurgica, 1953, 1(2): 153–162. doi: 10.1016/0001-6160(53)90054-6 [82] GREENWOOD G W, JOHNSON R H. The deformation of metals under small stresses during phase transformations [J]. Proceedings of the Royal Society of London A, 1965, 283(1394): 403–422. doi: 10.1098/rspa.1965.0029 [83] PATEL J R, COHEN M. Criterion for the action of applied stress in the martensitic transformation [J]. Acta Metallurgica, 1953, 1(5): 531–538. doi: 10.1016/0001-6160(53)90083-2 [84] TURTELTAUB S, SUIKER A S J. Transformation-induced plasticity in ferrous alloys [J]. Journal of the Mechanics and Physics of Solids, 2005, 53(8): 1747–1788. doi: 10.1016/j.jmps.2005.03.004 [85] TURTELTAUB S, SUIKER A S J. A multiscale thermomechanical model for cubic to tetragonal martensitic phase transformations [J]. International Journal of Solids and Structures, 2006, 43(14/15): 4509–4545. [86] DOQUET V. Twinning and multiaxial cyclic plasticity of a low stacking-fault-energy fcc alloy [J]. Acta Metallurgica et Materialia, 1993, 41(8): 2451–2459. doi: 10.1016/0956-7151(93)90325-M [87] SCHLÖGL S M, FISCHER F D. The role of slip and twinning in the deformation behaviour of polysynthetically twinned crystals of TiAl: a micromechanical model [J]. Philosophical Magazine A, 1997, 75(3): 621–636. doi: 10.1080/01418619708207193 [88] MECKING H, HARTIG C, KOCKS U F. Deformation modes in γ-TiAl as derived from the single crystal yield surface [J]. Acta Materialia, 1996, 44(4): 1309–1321. doi: 10.1016/1359-6454(95)00308-8 [89] VASUDEVAN A K, DOHERTY R D. Grain boundary ductile fracture in precipitation hardened aluminum alloys [J]. Acta Metallurgica, 1987, 35(6): 1193–1219. doi: 10.1016/0001-6160(87)90001-0 [90] SURESH S, VASUDEVAN A K, TOSTEN M, et al. Microscopic and macroscopic aspects of fracture in lithium-containing aluminum alloys [J]. Acta Metallurgica, 1987, 35(1): 25–46. doi: 10.1016/0001-6160(87)90210-0 [91] NGUYEN T, LUSCHER D J, WILKERSON J W. A dislocation-based crystal plasticity framework for dynamic ductile failure of single crystals [J]. Journal of the Mechanics and Physics of Solids, 2017, 108: 1–29. [92] ZIKRY M A, NEMAT-NASSER S. High strain-rate localization and failure of crystalline materials [J]. Mechanics of Materials, 1990, 10(3): 215–237. doi: 10.1016/0167-6636(90)90044-G [93] SCHOENFELD S E. Dynamic behaviour of polycrystalline tantalum [J]. International Journal of Plasticity, 1998, 14(9): 871–890. doi: 10.1016/S0749-6419(98)00034-5 [94] KNEZEVIC M, BEYERLEIN I J, LOVATO M L, et al. A strain-rate and temperature dependent constitutive model for BCC metals incorporating non-Schmid effects: application to tantalum-tungsten alloys [J]. International Journal of Plasticity, 2014, 62: 93–104. doi: 10.1016/j.ijplas.2014.07.007 [95] LIM H, BATTAILE C C, CARROLL J D, et al. A physically based model of temperature and strain rate dependent yield in BCC metals: implementation into crystal plasticity [J]. Journal of the Mechanics and Physics of Solids, 2015, 74: 80–96. doi: 10.1016/j.jmps.2014.10.003 [96] LIM H, HALE L M, ZIMMERMAN J A, et al. A multi-scale model of dislocation plasticity in α-Fe: incorporating temperature, strain rate and non-Schmid effects [J]. International Journal of Plasticity, 2015, 73: 100–118. doi: 10.1016/j.ijplas.2014.12.005 [97] BECKER R. Effects of crystal plasticity on materials loaded at high pressures and strain rates [J]. International Journal of Plasticity, 2004, 20(11): 1983–2006. doi: 10.1016/j.ijplas.2003.09.002 [98] SIMMONS G, WANG H. A handbook of single crystal elastic constants and calculated aggregate properties [M]. Cambridge, Mass: MIT Press, 1971. [99] LUSCHER D J, BRONKHORST C A, ALLEMAN C N, et al. A model for finite-deformation nonlinear thermomechanical response of single crystal copper under shock conditions [J]. Journal of the Mechanics and Physics of Solids, 2013, 61(9): 1877–1894. doi: 10.1016/j.jmps.2013.05.002 [100] DE S, ZAMIRI A R. A fully anisotropic single crystal model for high strain rate loading conditions with an application to α-RDX [J]. Journal of the Mechanics and Physics of Solids, 2014, 64: 287–301. doi: 10.1016/j.jmps.2013.10.012 [101] CAWKWELL M J, RAMOS K J, HOOKS D E, et al. Homogeneous dislocation nucleation in cyclotrimethylene trinitramine under shock loading [J]. Journal of Applied Physics, 2010, 107(6): 063512. doi: 10.1063/1.3305630 [102] CAZACU O, IONESCU I R. Dynamic crystal plasticity: an Eulerian approach [J]. Journal of the Mechanics and Physics of Solids, 2010, 58(6): 844–859. doi: 10.1016/j.jmps.2010.04.001 [103] CLAYTON J D. Analysis of shock compression of strong single crystals with logarithmic thermoelastic-plastic theory [J]. International Journal of Engineering Science, 2014, 79: 1–20. doi: 10.1016/j.ijengsci.2014.02.016 [104] WINEY J M, GUPTA Y M. Nonlinear anisotropic description for shocked single crystals: thermoelastic response and pure mode wave propagation [J]. Journal of Applied Physics, 2004, 96(4): 1993–1999. doi: 10.1063/1.1767294 [105] WINEY J M, GUPTA Y M. Nonlinear anisotropic description for the thermomechanical response of shocked single crystals: inelastic deformation [J]. Journal of Applied Physics, 2006, 99(2): 023510. doi: 10.1063/1.2161414 [106] AUSTIN R A, MCDOWELL D L. A dislocation-based constitutive model for viscoplastic deformation of fcc metals at very high strain rates [J]. International Journal of Plasticity, 2011, 27(1): 1–24. doi: 10.1016/j.ijplas.2010.03.002 [107] CLIFTON R J. Analysis of the laser velocity interferometer [J]. Journal of Applied Physics, 1970, 41(13): 5335–5337. doi: 10.1063/1.1658673 [108] LLOYD J T, CLAYTON J D, AUSTIN R A, et al. Plane wave simulation of elastic-viscoplastic single crystals [J]. Journal of the Mechanics and Physics of Solids, 2014, 69: 14–32. doi: 10.1016/j.jmps.2014.04.009 [109] LUSCHER D J, MAYEUR J R, MOURAD H M, et al. Coupling continuum dislocation transport with crystal plasticity for application to shock loading conditions [J]. International Journal of Plasticity, 2016, 76: 111–129. doi: 10.1016/j.ijplas.2015.07.007 [110] LUSCHER D J, ADDESSIO F L, CAWKWELL M J, et al. A dislocation density-based continuum model of the anisotropic shock response of single crystal α-cyclotrimethylene trinitramine [J]. Journal of the Mechanics and Physics of Solids, 2017, 98: 63–86. doi: 10.1016/j.jmps.2016.09.005 [111] HANSEN B L, BEYERLEIN I J, BRONKHORST C A, et al. A dislocation-based multi-rate single crystal plasticity model [J]. International Journal of Plasticity, 2013, 44: 129–146. doi: 10.1016/j.ijplas.2012.12.006 [112] SHAHBA A, GHOSH S. Crystal plasticity FE modeling of Ti alloys for a range of strain-rates. Part I: a unified constitutive model and flow rule [J]. International Journal of Plasticity, 2016, 87: 48–68. doi: 10.1016/j.ijplas.2016.09.002 [113] LLOYD J T, CLAYTON J D, BECKER R, et al. Simulation of shock wave propagation in single crystal and polycrystalline aluminum [J]. International Journal of Plasticity, 2014, 60: 118–144. doi: 10.1016/j.ijplas.2014.04.012 [114] BARTON N R, BENSON D J, BECKER R. Crystal level continuum modelling of phase transformations: the α↔ϵ transformation in iron [J]. Modelling and Simulation in Materials Science and Engineering, 2005, 13(5): 707. doi: 10.1088/0965-0393/13/5/006 [115] ADDESSIO F L, LUSCHER D J, CAWKWELL M J, et al. A single-crystal model for the high-strain rate deformation of cyclotrimethylene trinitramine including phase transformations and plastic slip [J]. Journal of Applied Physics, 2017, 121(18): 185902. doi: 10.1063/1.4983009 [116] KNEZEVIC M, ZECEVIC M, BEYERLEIN I J, et al. Strain rate and temperature effects on the selection of primary and secondary slip and twinning systems in HCP Zr [J]. Acta Materialia, 2015, 88: 55–73. doi: 10.1016/j.actamat.2015.01.037 [117] ARDELJAN M, BEYERLEIN I J, MCWILLIAMS B A, et al. Strain rate and temperature sensitive multi-level crystal plasticity model for large plastic deformation behavior: application to AZ31 magnesium alloy [J]. International Journal of Plasticity, 2016, 83: 90–109. doi: 10.1016/j.ijplas.2016.04.005 [118] MEREDITH C S, LLOYD J T, SANO T. The quasi-static and dynamic response of fine-grained Mg alloy AMX602: an experimental and computational study [J]. Materials Science and Engineering A, 2016, 673: 73–82. doi: 10.1016/j.msea.2016.07.035 [119] GARCÍA-GRAJALES J A, FERNÁNDEZ A, LEARY D, et al. A new strain rate dependent continuum framework for Mg alloys [J]. Computational Materials Science, 2016, 115: 41–50. doi: 10.1016/j.commatsci.2015.12.046 [120] 潘昊, 王升涛, 吴子辉, 等. 孪晶对Be材料冲击加-卸载动力学影响的数值模拟研究 [J]. 物理学报, 2018, 67(16): 164601. doi: 10.7498/aps.67.20180451PAN H, WANG S T, WU Z H, et al. Effect of twining on dynamic behaviors of beryllium materials under impact loading and unloading [J]. Acta Physica Sinica, 2018, 67(16): 164601. doi: 10.7498/aps.67.20180451 [121] CLAYTON J D. Dynamic plasticity and fracture in high density polycrystals: constitutive modeling and numerical simulation [J]. Journal of the Mechanics and Physics of Solids, 2005, 53(2): 261–301. doi: 10.1016/j.jmps.2004.06.009 [122] VOGLER T J, CLAYTON J D. Heterogeneous deformation and spall of an extruded tungsten alloy: plate impact experiments and crystal plasticity modeling [J]. Journal of the Mechanics and Physics of Solids, 2008, 56(2): 297–335. doi: 10.1016/j.jmps.2007.06.013 [123] DEMIR E, RAABE D, ZAAFARANI N, et al. Investigation of the indentation size effect through the measurement of the geometrically necessary dislocations beneath small indents of different depths using EBSD tomography [J]. Acta Materialia, 2009, 57(2): 559–569. doi: 10.1016/j.actamat.2008.09.039 [124] DMITRIEVA O, DONDL P W, MÜLLER S, et al. Lamination microstructure in shear deformed copper single crystals [J]. Acta Materialia, 2009, 57(12): 3439–3449. doi: 10.1016/j.actamat.2009.03.035 [125] HUANG H, VAN SWYGENHOVEN H. Atomistic simulations of mechanics of nanostructures [J]. MRS bulletin, 2009, 34(3): 160–166. doi: 10.1557/mrs2009.46 -








 下载:
下载:







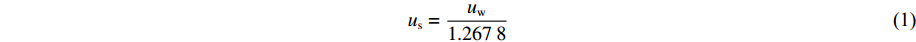
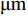





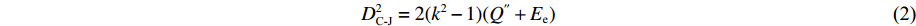
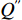
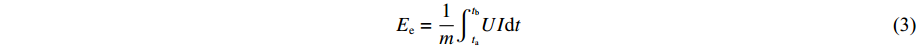




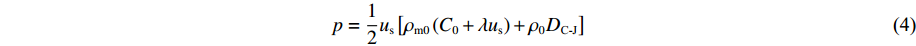
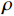
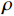
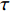

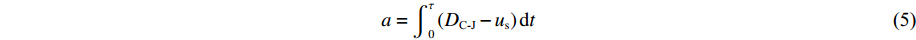
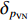
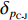









 下载:
下载: