Pressure-Induced Phase Transformations of IrSb from First-Principles Calculations
-
摘要: 基于第一性原理并结合粒子群优化算法的卡里普索（CALYPSO）晶体结构预测方法，研究在0～100 GPa压力下，过渡金属铱和类金属锑组成的化合物IrSb的相变行为和物理性质。研究发现：在常压下，具有立方结构
α -IrSb相的空间群为P63/mmc，与实验结果一致；在压力为16.4 GPa时，发现了一种新型立方结构β -IrSb相，其空间群为C2/c；在76.5～100 GPa压力范围内，其稳定结构为空间群是P-1的γ -IrSb相。声子色散关系计算结果表明：α -IrSb相、β -IrSb相和γ -IrSb相在各自的布里渊区没有出现虚频，具有动力学稳定性。计算得出3个相的形成焓均小于零，说明3个相均具有热力学稳定性。能带结构计算结果表明：3个相的晶体结构在费米面附近导带和价带均发生交叠，3个相均呈现金属性。计算并讨论了各相的电荷转移情况，研究发现：Ir原子是受主，Sb原子是施主，电荷从Sb原子向Ir原子转移。Abstract: Based on first-principle calculations and the structure prediction method CALYPSO of particle swarm optimization algorithms, phase transition behaviors and physical properties of IrSb in the pressure range of 0–100 GPa have been systematically studied. At ambient pressure, the space group ofα -IrSb phase with cubic structure is P63/mmc, in consistency with experimental results. A new cubic structure,β -IrSb phase, is found at 16.4 GPa with the space group of C2/c. When the pressure is above 76.5 GPa, the space group becomes P-1. The phonon dispersion shows thatα -,β - andγ -IrSb phases have no virtual frequency in the whole Brillouin zone, thus the three phases are dynamically stable. Calculated results show that the formation enthalpy of three phases are less than zero, indicating that all the three phases have the thermodynamic stability. Band structure calculations show that all the three phases have the overlapping of conduction bands and valence bands near Fermi surface, thus are metallic phases. The charge transfer of each phase is calculated and discussed, in which Ir atoms are the acceptor and Sb atoms are the donor.-
Key words:
- high pressure /
- first-principle calculations /
- crystal structure prediction /
- IrSb
-
薄壁结构的抗冲击特性在航天航空、机械工程和交通运输等不同领域的设计中极具重要性[1-3]。随着工程领域对材料轻量化的要求，多孔结构因具有质量轻、高比能、高容积效率等优势[4-5]，常作为缓冲、吸能元件，被广泛用作飞机、导弹及汽车等行业的结构件。
王璠等[6]通过对纤维增强复合材料圆柱壳的吸能特性进行试验，研究了铺层方式和引发方式对该结构吸能效果的影响和压溃过程的破坏机理。罗国强等[7]采用离散元方法模拟了多种孔洞排布方式的PMMA多孔材料在冲击加载过程中早期孔洞塌缩破坏、应力分布与粒子速度等冲击响应行为，得到了孔洞破坏形式、应力集中区域等结果。Bich等[8]提出了一种有缺陷偏心加筋功能梯度圆柱薄壳在轴压作用下的非线性静力和动力屈曲分析方法。Zhang等[9]从理论、实验与数值模拟3个方面论证了多胞金属管的吸能效果高于单胞金属管。
随着多孔材料在工程领域应用的逐渐深入，将多孔材料壳体作为吸能结构成为国内外学者关注的焦点[10-12]，诸多学者对轻质多孔结构的力学特性开展了研究[13-15]。因此，研究多孔圆柱壳结构在冲击载荷下的吸能以及破坏特性具有重要的工程背景和一定的理论价值。
本研究以正、负泊松比两种多孔构型的层合圆柱壳模型为基础，以层合方式和孔单元数目为变量设计模型。在轴向压缩实验的基础上，使用有限元模拟软件ABAQUS对设计的模型进行冲击模拟分析。通过分析数值模拟结果，得到参数变化对层合多孔圆柱壳吸能特性的影响。
1. 模型介绍
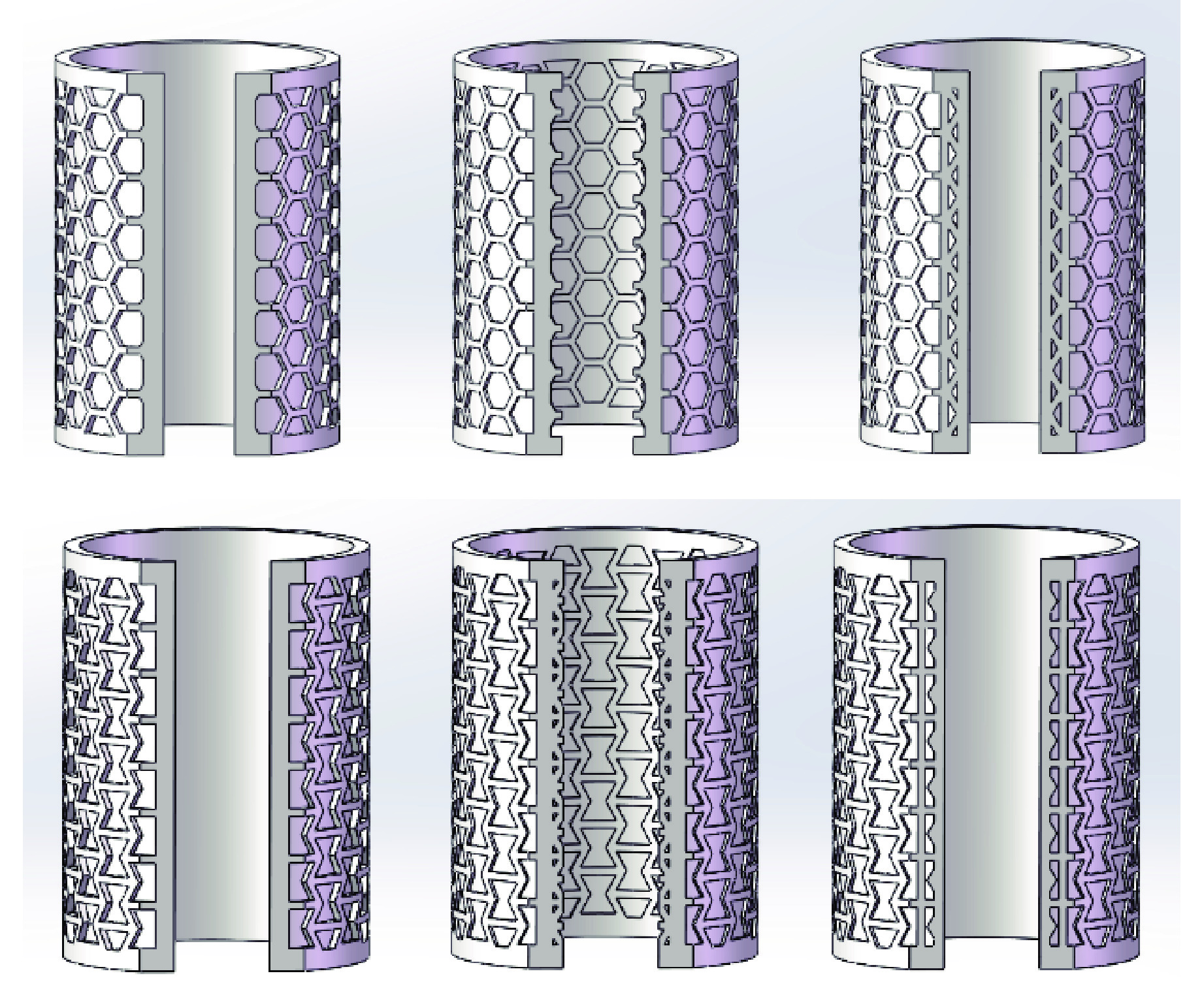
研究层合多孔圆柱壳的层合方式以及孔单元数目对其冲击吸能特性的影响，在保证质量不变的前提下，设计不同层合方式和孔数的六边与内凹多孔圆柱壳模型。图1为3种不同层合方式的圆柱壳模型，分别为外多孔层、内实心层的2层层合圆柱壳，内外为多孔层、中间为实心层的3层圆柱壳，以及多孔层与实心层相间排列的4层圆柱壳（最外为多孔层）。图2为改变孔单元数目的圆柱壳模型，轴向孔数分别为8、12、16，其对应的周向孔数为24、36、48，表1给出了各个模型的几何尺寸。
表 1 层合多孔圆柱壳的几何尺寸Table 1. Geometric dimensions of laminated cellular cylindrical shellNumber of axial cell Number of circumferential cell Thickness of each layer/mm Height/mm Diameter/mm 8 24 2/2 106 78.4 12 36 2/2 106 78.4 16 48 2/2 106 78.4 8 24 1/2/1 106 78.4 8 24 1/1/1/1 106 78.4 2. 实验与数值模拟验证
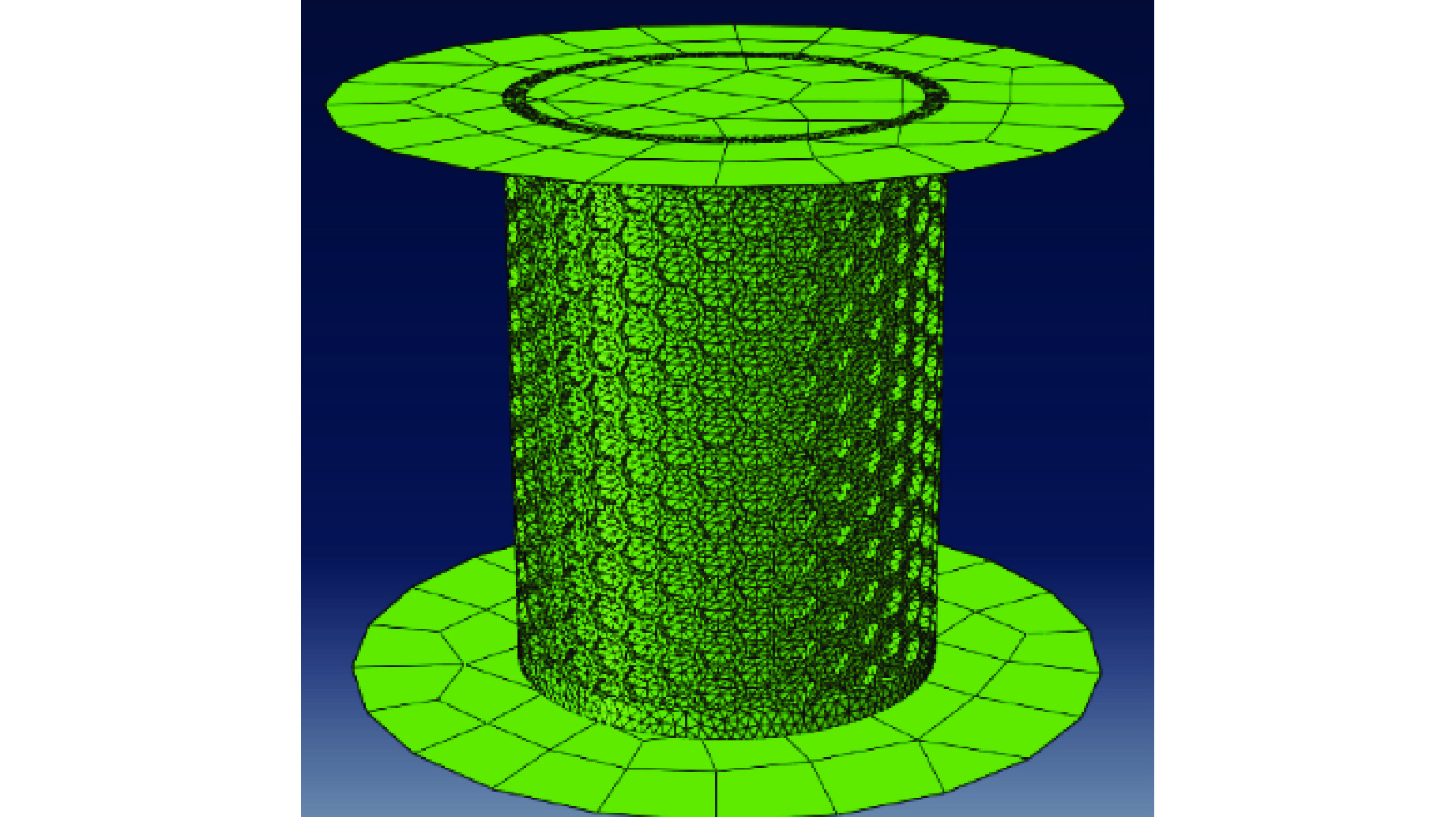
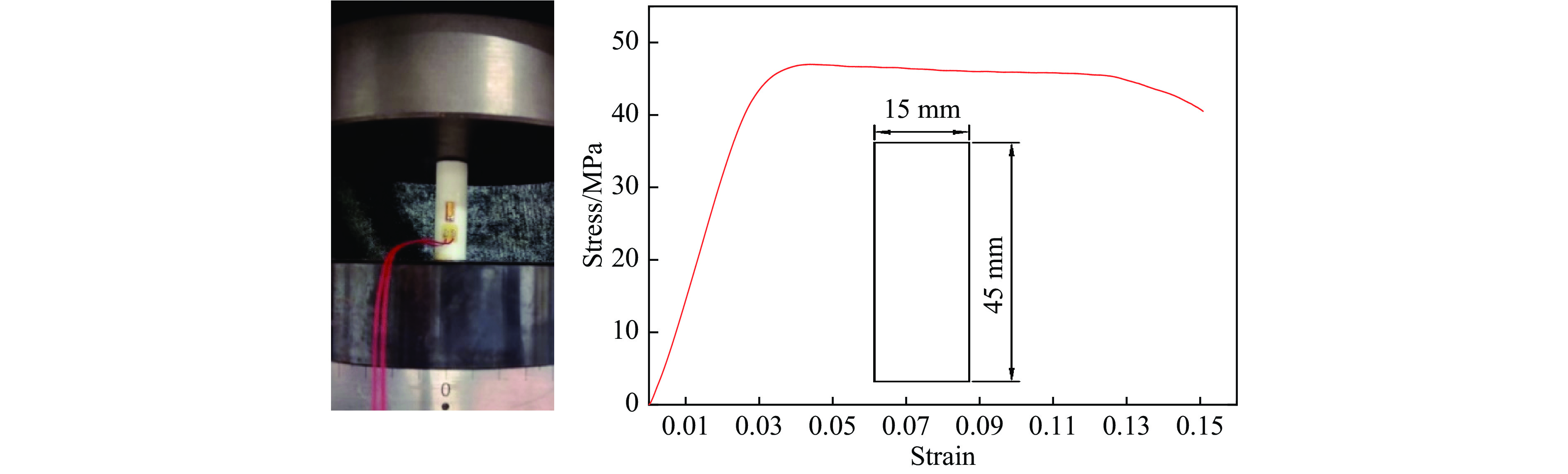
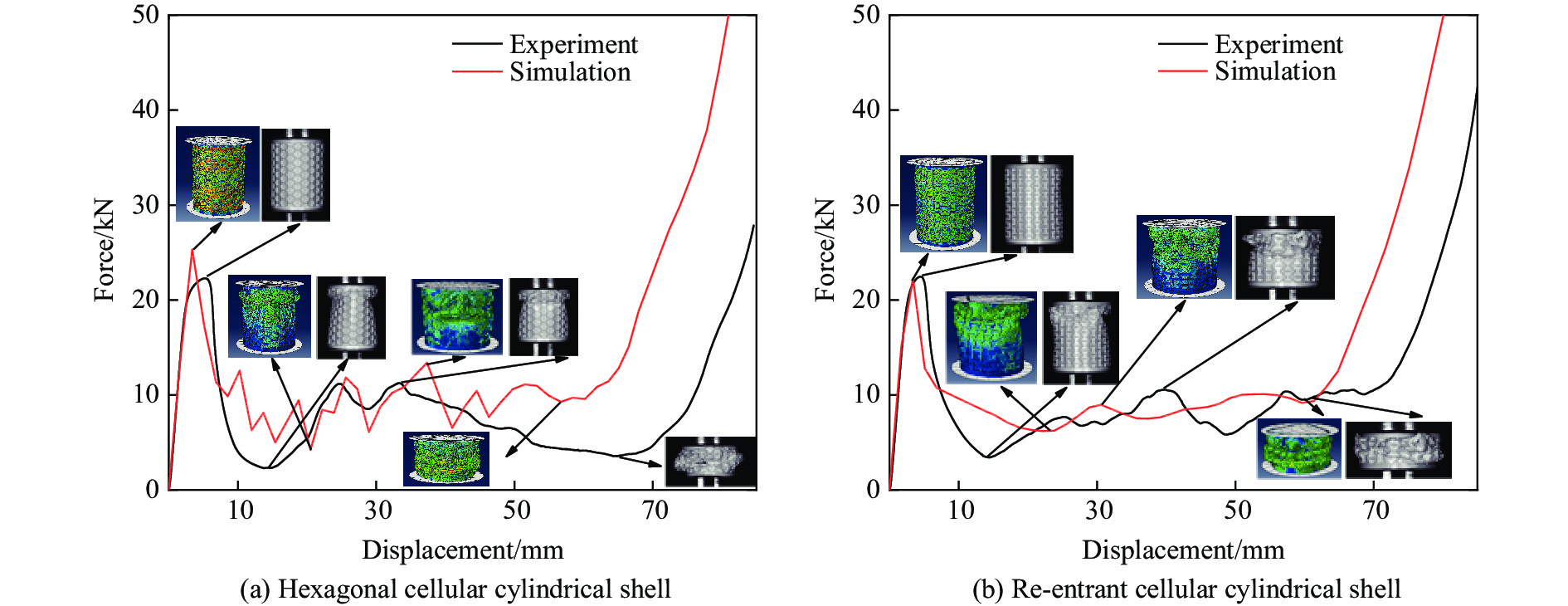
如图3所示，采用聚乳酸（PLA）材料制备实验所需的2层六边多孔圆柱壳与内凹多孔圆柱壳，使用万能材料试验机进行压缩实验，所用PLA材料的密度为1.25 g/cm3。实验采用位移加载的方式压缩85 mm，压缩速度为1 mm/min。使用有限元模拟软件ABAQUS完成准静态压缩数值模拟分析，同样施加85 mm位移荷载。有限元模型如图4所示，使用刚体板模拟上下压头，中间圆柱壳模型使用四面体单元，圆柱壳网格尺寸设置为2 mm。对下刚板完全固定，上刚板轴向压缩，考虑刚性板与圆柱壳的接触摩擦，摩擦因数取0.3。数值模拟分析使用的材料参数由压缩实验测定，如图5所示，圆柱体试样尺寸为
∅ 15 mm×45 mm。图5同时给出了PLA的应力-应变曲线，得到PLA的弹性模量E=1.57 GPa，泊松比μ =0.35。
图 5 PLA材料的压缩实验与应力-应变曲线Figure 5. Compression experiment and stress-strain curve of PLA material图6给出了实验与数值模拟的力-位移曲线对比。可以看到，实验与数值模拟结果在模型被完全压实之前吻合较好，峰值荷载的大小和出现的位置一致。其中六边圆柱壳的压缩力波动较大，而负泊松比内凹圆柱壳的压缩力波动平缓。在数值模拟中未考虑构件断裂，其误差影响主要出现在大应变阶段，这也是数值模拟的密实化压缩位移均小于实验值的原因，随着构件断裂，结构进一步被压缩密实。图7为实验与数值模型在相同应变时的变形模式对比。可以看到，数值模拟结果能够较好地模拟实验的变形模式。
图 6 实验与数值模拟得到的力-位移曲线对比Figure 6. Comparisons of compressive force-displacement curves between experiment and simulation
图 7 实验与数值模拟得到的变形模式对比Figure 7. Comparisons of deformation mode between experiment and simulation综合图6和图7可以看出，结构的变形可以划分为5个阶段：第1阶段，结构受压缩荷载一直达到初始峰值荷载，此阶段可以认为是弹性变形阶段，结构未发生明显形变；第2阶段，结构发生屈服，承受的压缩力快速下降，塑性变形明显，出现第1个褶皱；结构出现褶皱之后，结构承载力在波动中开始上升，达到第2个较低的峰值荷载，为第3阶段；第4阶段，结构承载力继续下降，出现第2个褶皱，与第1个褶皱十字相叠；第5阶段，结构基本压实，承载力持续上升。
3. 冲击仿真模拟
3.1 有限元网格及算法验证
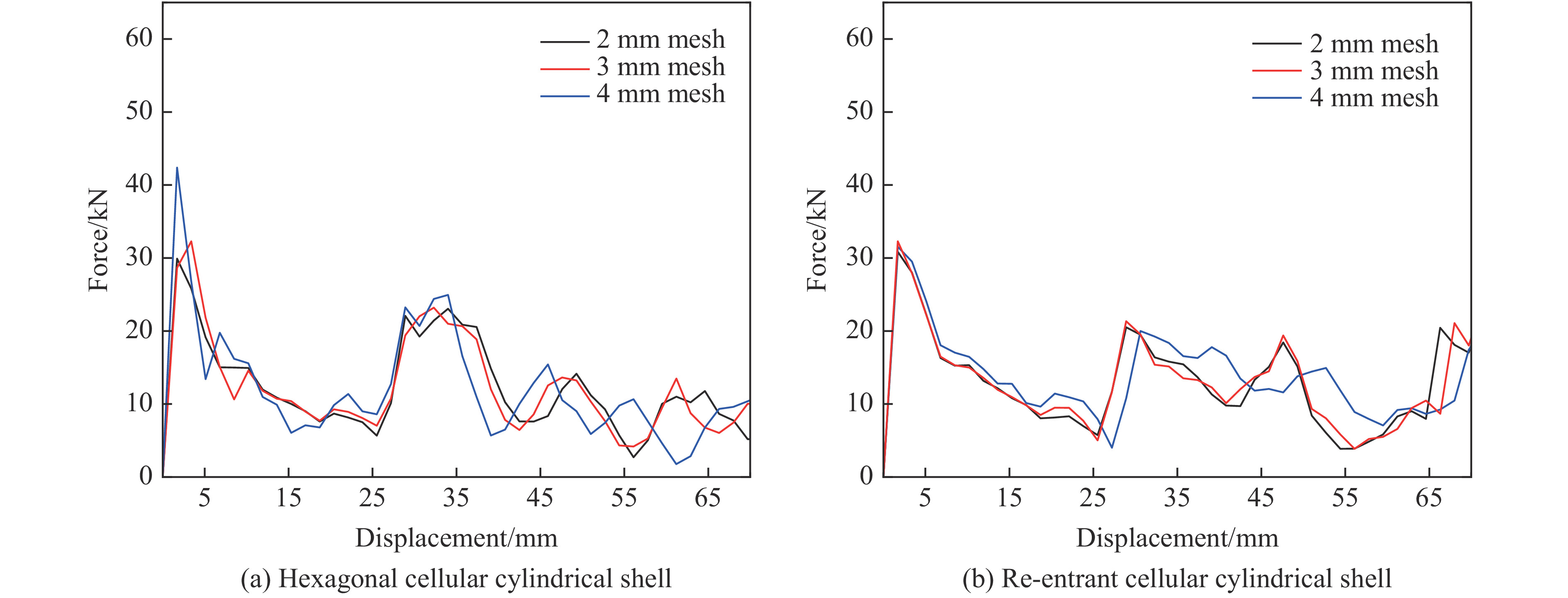
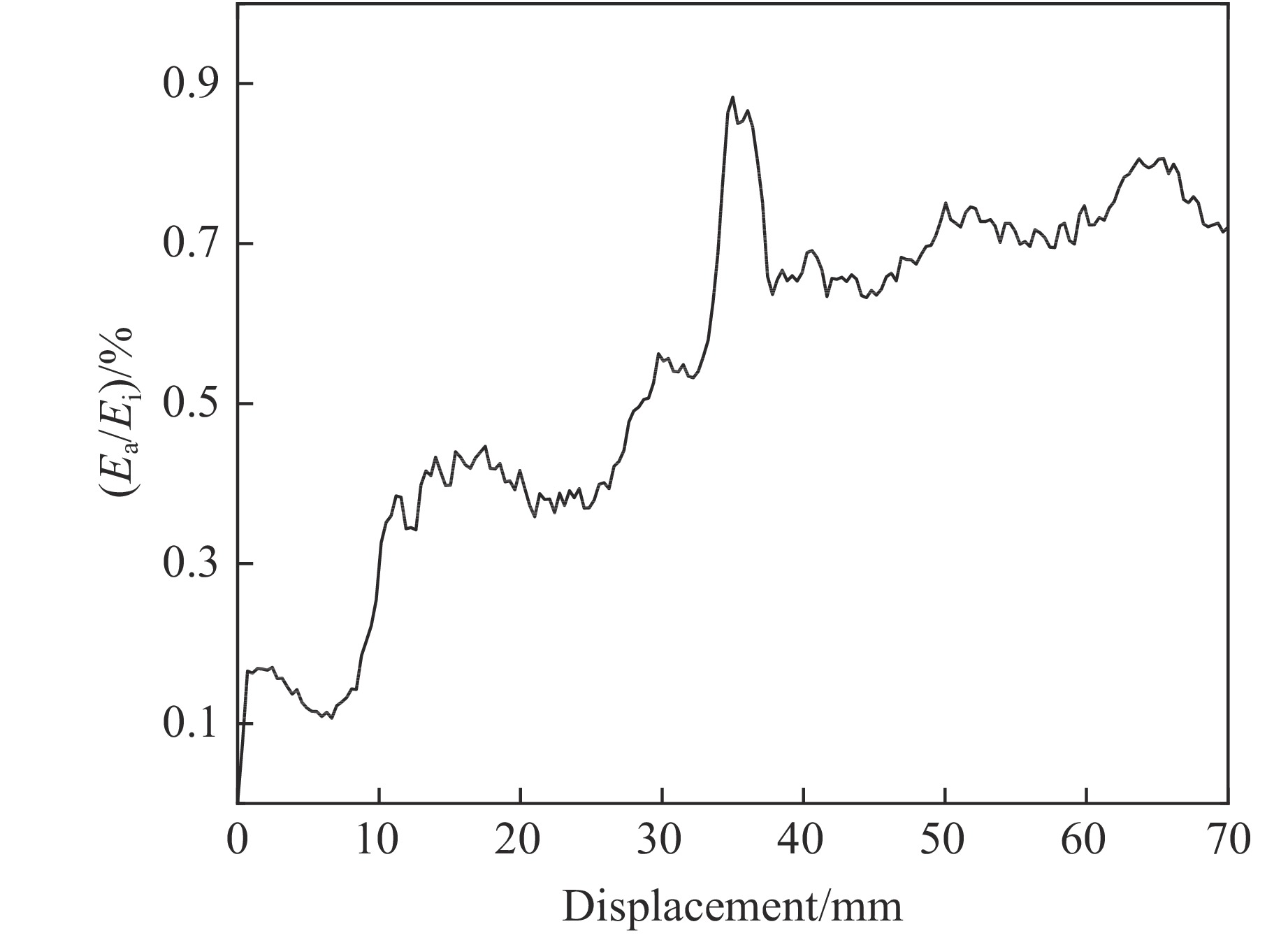
使用经过实验测试的PLA材料参数，对六边与内凹圆柱壳有限元模型进行网格敏感性验证，分别使用尺寸为2、3、4 mm的四面体单元划分网格，图8为不同尺寸网格的六边圆柱壳有限元模型。图9为两种圆柱壳在50 m/s冲击速度下的力-位移曲线。可以看到，采用2和3 mm网格计算的力-位移曲线基本接近，4 mm网格则有一些差别，考虑计算精度与效率之后选择了精度更高的2 mm网格进行计算。沙漏现象是存在于有限元分析中的一种零能变形模式，产生零应变和应力。伪应变能为控制沙漏变形所耗散的主要能量，图10为伪应变能（Ea）与内能（Ei）之比，其比值越小，说明伪应变能较小，即沙漏现象不明显。由图10可知，伪应变能与内能之比小于1%，说明数值模拟中没有出现明显的沙漏现象，可知有限元模拟分析中网格划分较合理。
图 8 3种尺寸网格的有限元仿真模型Figure 8. Finite element simulation models of three kinds of meshes3.2 冲击速度对变形模式的影响
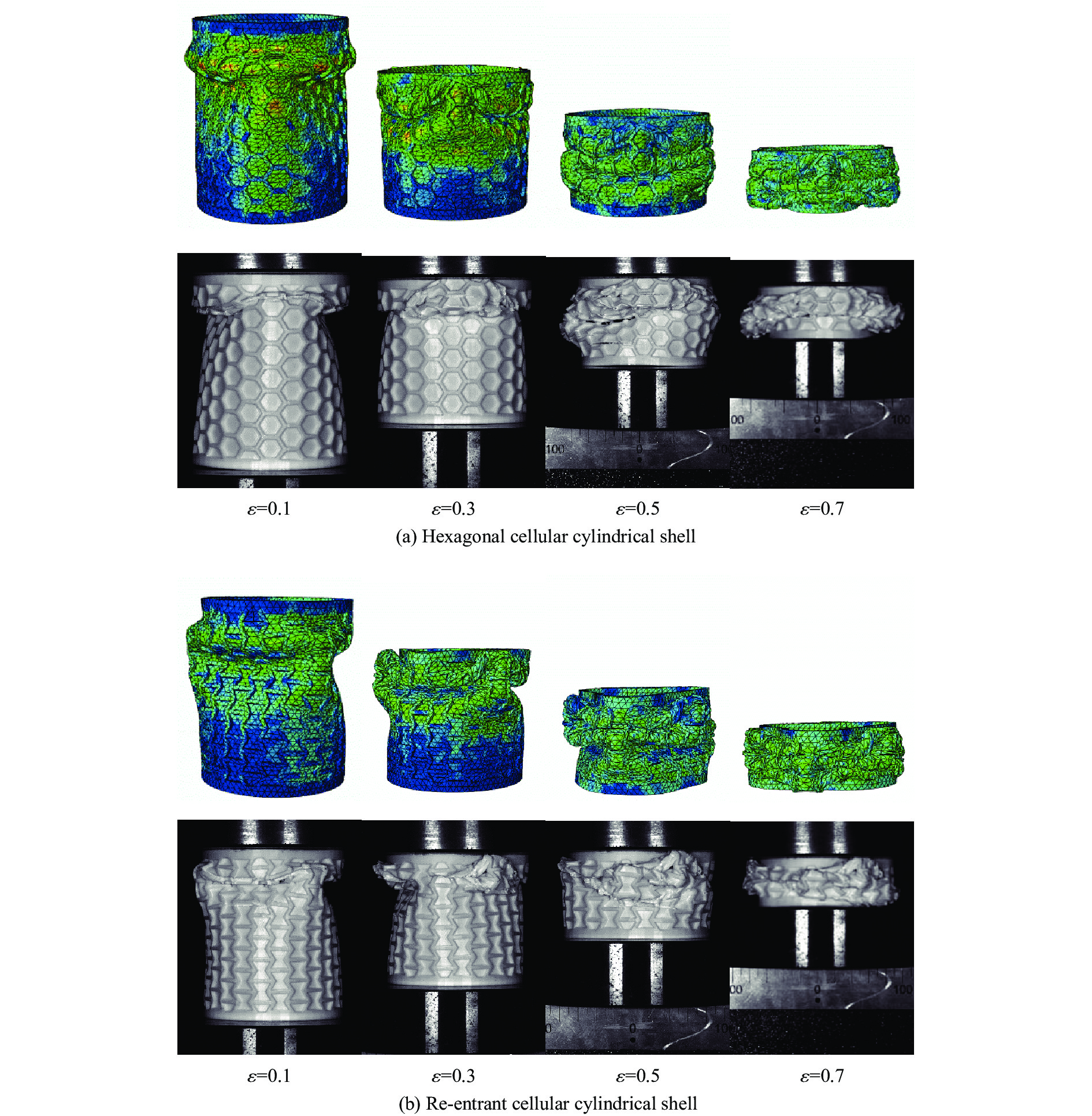
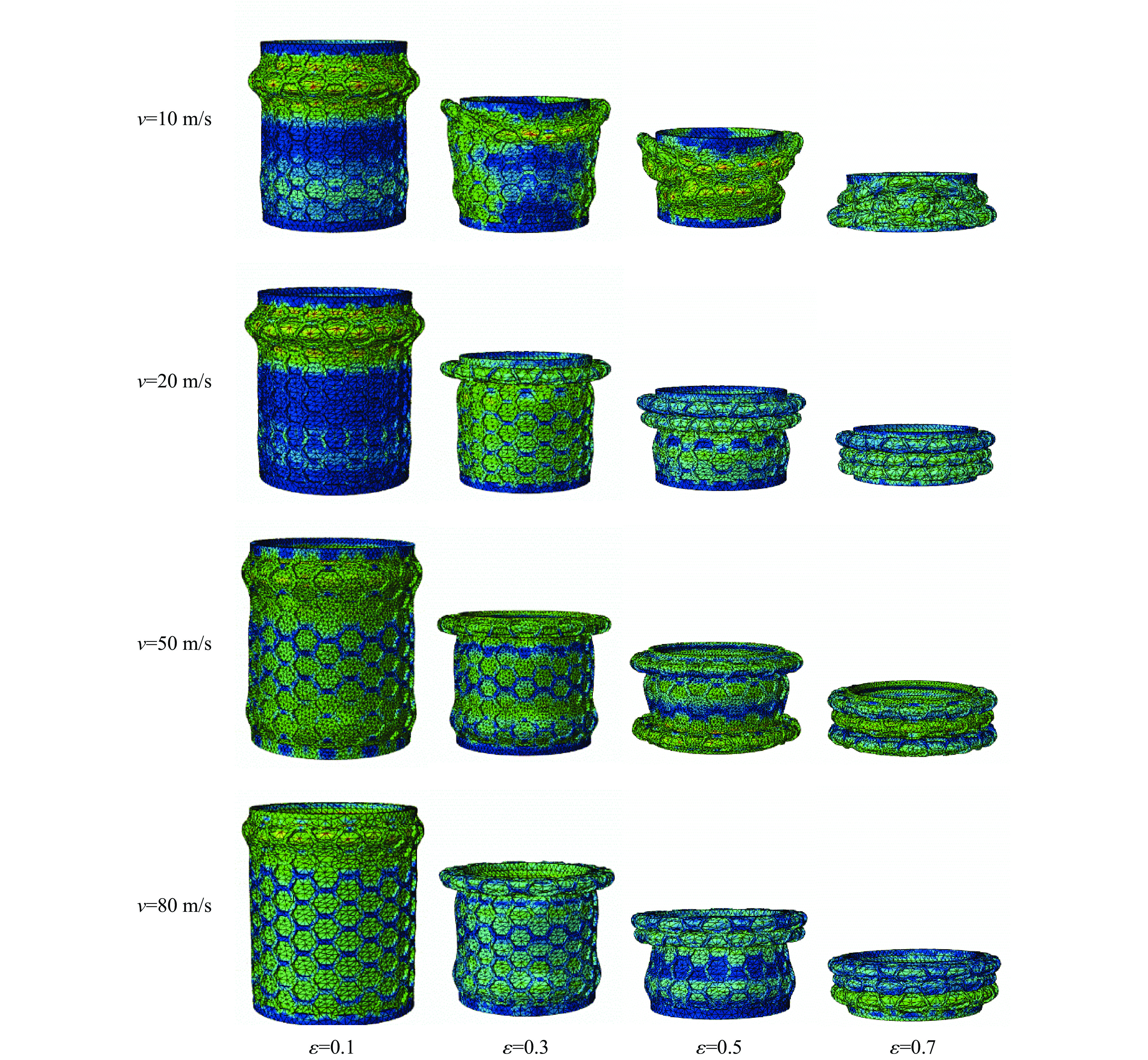
数值模拟中采用2 mm网格，图11为六边多孔圆柱壳在不同冲击速度下的变形模式。从图11中可以看到，随着应变的增加，模型逐渐出现褶皱。
ε =0.1时，不同速度的计算结果均出现中心对称的圆形褶皱，继续加载时，冲击速度为10 m/s的模型出现了不规则的变形模式，20、50、80 m/s冲击速度的模型则继续出现圆形褶皱。同样的现象可以在图12的内凹圆柱壳变形模式中观察到，冲击速度为10 m/s的内凹圆柱壳模型在第1个圆形褶皱之后也出现了不规则变形，速度提升之后则只出现圆形褶皱。研究[16]表明，圆柱壳在轴向冲击载荷下的变形模式受冲击速度的影响，存在变形模式变化的临界速度。本研究中，当冲击速度超过某一临界速度时，层合多孔圆柱壳的变形模式由圆形褶皱混合不规则变形转变为仅有圆形褶皱。
图 11 六边多孔圆柱壳在不同冲击速度下的变形模式Figure 11. Deformation mode of hexagonal cellular cylindrical shell at different impact velocities3.3 层合方式的影响
由图11和图12中的变形模式可以看到，中高速冲击得到的变形模式较规则和稳定，选择冲击速度v=50 m/s分析层合方式与孔单元数目对层合多孔圆柱壳冲击吸能特性的影响。使用总吸能（Et）、峰值压缩力（Fp）、平均压缩力（Fa） 3种评价指标分析层合多孔圆柱壳的冲击吸能特性。
总吸能Et为结构受荷载变形吸收的能量，可由力-位移曲线中力对位移的积分得到
Et=∫l0F(x)dx (1) 式中：F为冲击压缩力，
l 为冲击压缩的距离。峰值压缩力Fp为结构在冲击压缩密实之前的压缩力峰值，通常出现在冲击荷载的初始阶段。平均压缩力Fa可以由总吸能得到
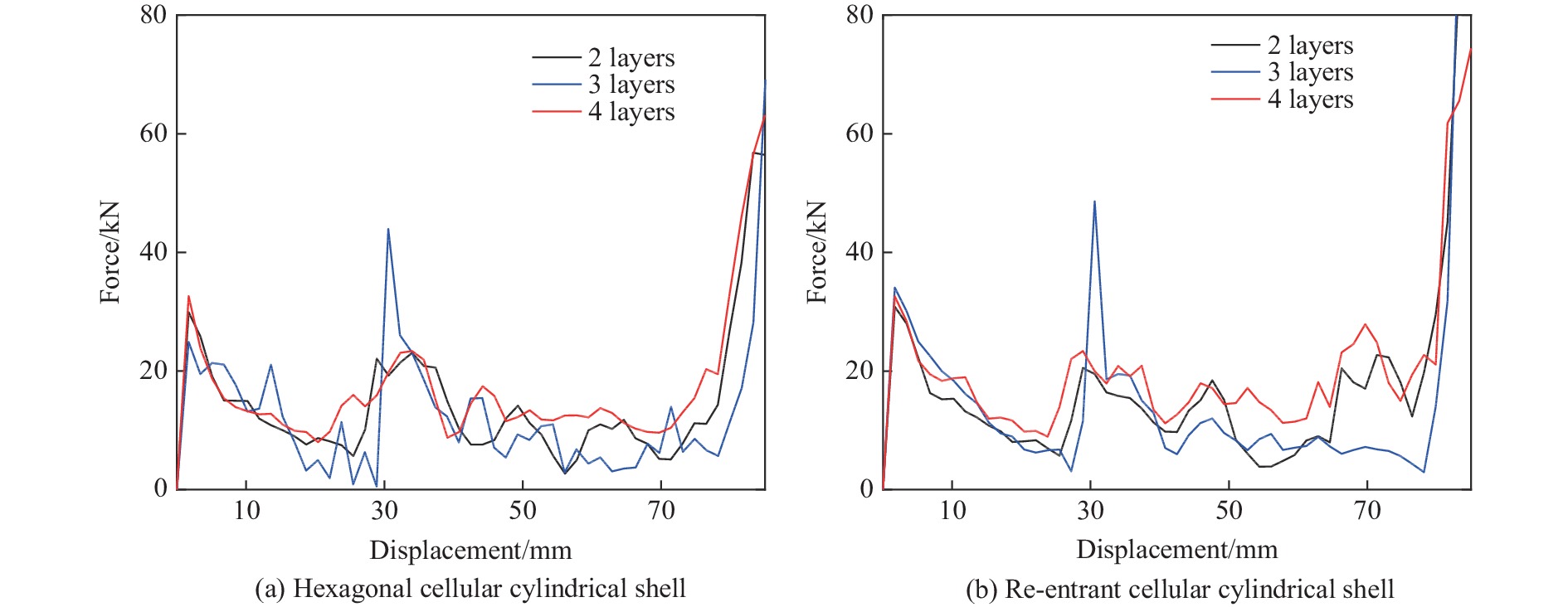
Fa=Et/l (2) 图13为不同层合方式多孔圆柱壳模型在冲击荷载作用下的力-位移曲线。从图13可以看出，不同层合方式圆柱壳模型的力-位移曲线总体比较相似，压缩力有两个较明显的峰值。对于六边多孔圆柱壳，4层圆柱壳初始峰值压缩力大于2层，3层压缩力最小，内凹多孔圆柱壳初始峰值压缩力均较接近。从六边与内凹圆柱壳的力-位移曲线可以看出，3层圆柱壳的第2个峰值压缩力远大于2、4层圆柱壳，甚至超过了初始峰值压缩力。在第2个峰值之后，4层圆柱壳的压缩力高于2、3层圆柱壳。
图 13 不同层合方式多孔圆柱壳的力-位移曲线Figure 13. Compressive force-displacement curves of cellular cylindrical shell with different laminated ways计算位移达到80 mm时的峰值压缩力和平均压缩力，结果如表2所示。4层圆柱壳的总吸能与平均压缩力皆大于其他两种结构，3层圆柱壳的总吸能与平均压缩力最小，峰值压缩力却最大，分析是由内外多孔层导致的。对比正、负泊松比多孔圆柱壳的吸能特性，2、3、4层内凹圆柱壳的总吸能较六边圆柱壳分别提高17.4%、22.5%、18.3%，峰值压缩力相差不大，平均压缩力更高。
表 2 不同层合方式下的冲击吸能指标Table 2. Impact energy absorption parameters of different laminated wayCellular units Layer number Et/J Fp/kN Fa/kN Hexagon 2 1 206.0 29.9 12.2 3 1 032.1 43.9 11.0 4 1 414.3 32.6 14.5 Re-entrant 2 1 416.7 30.8 13.7 3 1 264.6 48.6 11.8 4 1 673.5 32.6 17.0 3.4 孔单元数目的影响
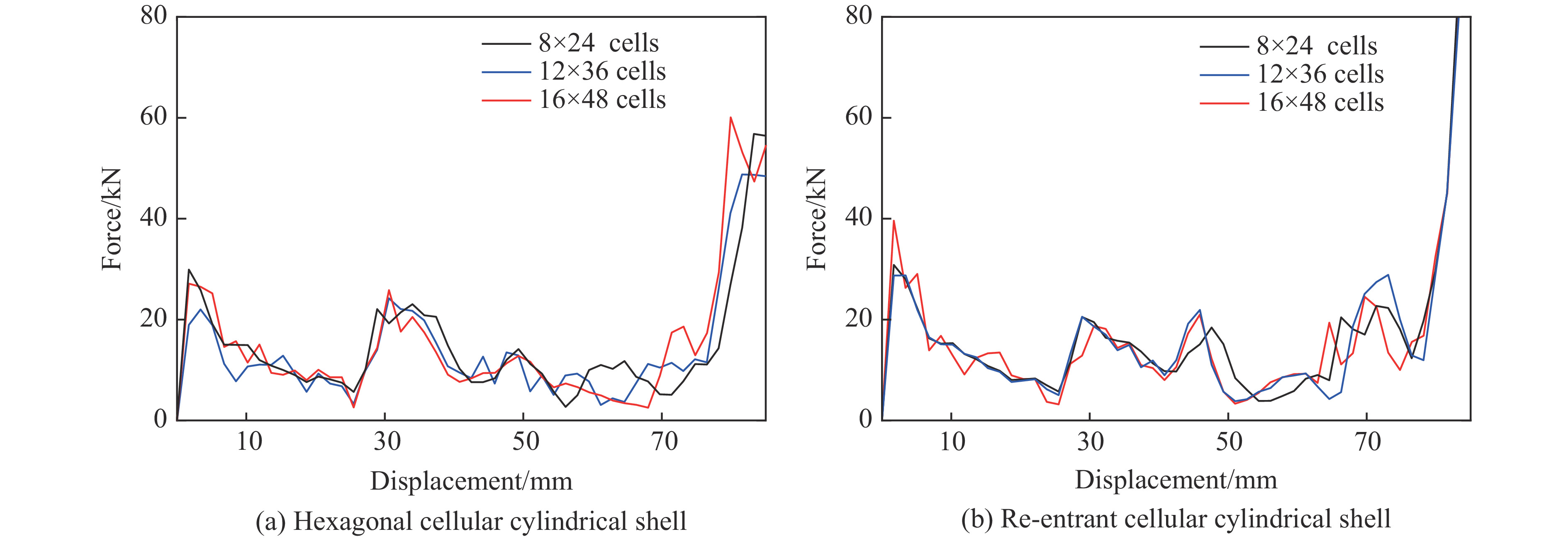
图14为不同孔单元数目圆柱壳模型在冲击载荷作用下的力-位移曲线。可以看到，相同质量下多孔圆柱壳的孔单元数目对力-位移曲线的影响较小，3种孔数的曲线都较接近。六边圆柱壳有2个明显的峰值压缩力，内凹圆柱壳较平缓，在初始峰值压缩力之后有3个较小的峰值压缩力。
图 14 不同孔数多孔圆柱壳的力-位移曲线Figure 14. Compressive force-displacement curves of cellular cylindrical shell with different cells number同样计算总吸能Et、峰值压缩力Fp和平均压缩力Fa 3种吸能指标，如表3所示。可以看到，孔数最多的六边圆柱壳吸能效果更好，内凹圆柱壳则是孔数最少的模型吸能效果更好。孔数对平均压缩力的影响较小。16×48孔圆柱壳的峰值压缩力较高，12×36孔圆柱壳的峰值压缩力相对较小。内凹圆柱壳的总吸能同样大于六边圆柱壳，其在力-位移曲线上也表现出更多的峰值。
表 3 不同孔数多孔圆柱壳的冲击吸能指标Table 3. Impact energy absorption parameters of of cellular cylindrical shell with different cells numberCellular units Cell number Et/J Fp/kN Fa/kN Hexagon 8×24 1 206.0 29.9 12.2 12×36 1 169.7 25.8 11.2 16×48 1 266.4 29.4 11.9 Re-entrant 8×24 1 416.7 30.8 13.7 12×36 1 383.4 28.9 13.4 16×48 1 394.0 39.6 13.3 4. 结 论
研究了不同层合方式、不同孔单元数目的层合多孔圆柱壳在冲击荷载下的吸能特性。在轴向压缩实验的基础上，利用 ABAQUS 有限元软件对多孔圆柱壳模型在准静态、不同冲击速度下的压缩行为进行了数值模拟。基于结构的变形模式，分析了不同层合方式与孔数对其承载能力、吸能特性的影响，得出以下结论。
(1) 随着冲击速度的提升，多孔圆柱壳从轴对称的三角变形模式逐渐变成中心对称的圆形变形模式，其中速度为10 m/s的模型同时具有三角变形模式和圆形变形模式。
(2) 4层圆柱壳有更好的吸能效果与更高的平均压缩力，3层圆柱壳的峰值压缩力远大于其他两种层合圆柱壳。
(3) 孔单元数目对吸能效果的影响与多孔构型有关，孔单元数目最多的六边圆柱壳的吸能效果最好，而在内凹圆柱壳中则相反。
(4) 同样参数下，具有负泊松比特性的内凹圆柱壳的吸能效果较六边圆柱壳更好，平均压缩力也更大。
-
图 1 IrSb的热力学焓差曲线（a）以及
α -IrSb相、β -IrSb相和γ -IrSb相体积随压强变化（b）Figure 1. Calculated enthalpy difference as a function of pressure relative to IrSb （a） and calculated volume versus pressure of
α -IrSb，β -IrSb andγ -IrSb （b）
图 2
α -IrSb相、β -IrSb相和γ -IrSb相的晶体结构Figure 2. Crystal structures of
α -IrSb，β -IrSb andγ -IrSb
图 3
α -IrSb、β -IrSb和γ -IrSb相的声子色散关系与投影态密度Figure 3. Phonon-dispersion curves and the projected density of states of
α -IrSb，β -IrSb, andγ -IrSb
图 4
α -IrSb相、β -IrSb相和γ -IrSb相的能带结构和电子态密度Figure 4. Band structure and partial density of states of
α -IrSb，β -IrSb andγ -IrSb表 1
α -IrSb相、β -IrSb相和γ -IrSb的晶格参数和原子位置Table 1. Lattice parameters and atomic coordinates of
α -IrSb,β -IrSb andγ -IrSbPhase Pressue/GPa Space group Lattice parameters Wyckoff positions α-IrSb 0 P63/mmc a=4.082 Å，b=4.082 Å，c=5.634 Å
α=90.0°，β=90.0°，γ=120.0°Ir1：2a (0, 0, 0) Sb1：2c (0.333, 0.000, 0.500) β-IrSb 16.4 C2/c a=11.207 Å，b=5.326 Å，c=5.061 Å
α=90.0°，β=110.7°，γ=90.0°Ir1：8f (0.400, 0.673, 1.361) Sb1：8f (0.000, 0.500, 0.152) γ-IrSb 76.5 P-1 a=4.812 Å，b=5.034 Å，c=5.033 Å
α=98.8°，β=99.6°，γ=92.1°Ir1：2i (0.217, 0.525, 0.204) Ir3：2i (0.676, 0.877, 0.183) Sb1：2i (0.200, 0.032, 0.297) Sb3：2i (0.722, 0.393, 0.289) 
下载: 导出CSV
表 2
α -IrSb相、β -IrSb相和γ -IrSb相的Bader电荷转移Table 2. Calculated Bader charges of
α -IrSb,β -IrSb andγ -IrSbPhase Pressue/GPa Space group Atom Number Charge value/e δ/e α–IrSb 0 P63/mmc Ir 2 9.60 –0.60 Sb 2 4.40 0.60 β–IrSb 16.4 C2/c Ir 8 9.66 –0.66 Sb 8 4.34 0.66 γ–IrSb 76.5 P-1 Ir 2 9.64 –0.64 2 9.75 –0.75 Sb 2 4.30 0.70 2 4.31 0.69
下载: 导出CSV
-
[1] HEMLEY R J. Effects of high pressure on molecules [J]. Annual Review of Physical Chemistry, 2000, 51(1): 763–800. doi: 10.1146/annurev.physchem.51.1.763 [2] SCHTTION V, BINI R. Molecules under extreme conditions: chemical reactions at high pressure [J]. Physical Chemistry Chemical Physics, 2003, 5(10): 1951–1965. doi: 10.1039/b301381b [3] GROCCHALA W, HOFFMANN R, FENG J, et al. The chemical imagination at work in very tight places [J]. Cheminform, 2007, 46(20): 3620–3642. [4] CERENIUS Y, DUBROVINSKY L. Compressibility measurements on iridium [J]. Journal of Alloys and Compounds, 2000, 306: 26–29. doi: 10.1016/S0925-8388(00)00767-2 [5] WIBERG E, WIBERGN, HOLLEMAN A F. Inorganic chemistry [M]. Academic Press, 2001. [6] KUZMIN R N. X-ray diffraction study of the structure of IrSb [J]. Soviet Physics, Crystallography, 1958, 3(3): 366–368. [7] ZHURAVLEV N N, ZHDANOV G S. X-ray diffraction determination of the structure of CoSb3, RhSb3, and IrSb3 [J]. Soviet Physics, Crystallography, 1956, 1(5): 404. [8] HULLIGER F. Semiconducting compounds with skutterudite structure [J]. Helvetica Physica Acta, 1961, 34: 782–786. [9] GLEN A, SLACK, VENETA G, TSOUKALA. Some properties of semiconducting IrSb3 [J]. Journal of Applied Physics, 1994, 76: 1665. doi: 10.1063/1.357750 [10] 杨明宇, 杨倩, 张勃, 等. 5d过渡金属原子掺杂六方氮化铝单层的磁性及自旋轨道耦合效应:可能存在的二维长程磁有序 [J]. 物理学报, 2017, 66(6): 063102.YANG M Y, YANG Q, ZHANG B, et al. Electronic structures, magnetic properties and spin-orbital coupling effects of aluminum nitride monolayers doped by 5d transition metal atoms: possible two-dimensional long-range magnetic orders [J]. Acta Physica Sinica, 2017, 66(6): 063102. [11] WANG Y, LV J, ZHU L, et al. Crystal structure prediction via particle swarm optimization [J]. Physics, 2010, 82(9): 7174–7182. [12] KRESSE G, JOUBERT D. From ultrasoft pseudopotentials to the projector augmented-wave method [J]. Physical Review B, 1999, 59(3): 1758–1775. doi: 10.1103/PhysRevB.59.1758 [13] KRESSE G, HAFNERR J. Ab initio molecular-dynamics simulation of the liquid-metal; amorphous-semiconductor transition in germanium [J]. Physical Review B, 1994, 49(20): 14251–14269. doi: 10.1103/PhysRevB.49.14251 [14] KRESSE G, HAFNERR J. Ab initio molecular dynamics for liquid metals [J]. Physical Review B, 1993, 47(1): 558–561. doi: 10.1103/PhysRevB.47.558 [15] PHILLIPS J C. Energy-band interpolation scheme based on a pseudopotential [J]. Physical Review, 1958, 112(3): 685–695. doi: 10.1103/PhysRev.112.685 [16] 陈舜麒.计算材料科学 [M]. 北京: 化学工业出版社, 2005: 100–105.CHEN S L. Computational materials science [M]. Beijing: Chemical Industry Press, 2005: 100–105. [17] JOHN P P, KIERON B, MATTHIAS E. Generalized gradient approximation made simple [J]. Physical Review Letters, 1996, 77(18): 3865–3868. doi: 10.1103/PhysRevLett.77.3865 [18] MONKHORST H J, PACNK J D. Special points for Brillouin-zone integrations [J]. Physical Review B, 1976, 16(4): 1746–1747. [19] THIRUMALAI D, HALL R W, BERNE B. A path integral Monte Carlo study of Liquid neon and the quantum effective pair potential [J]. The Journal of Chemical Physics, 1984, 81(6): 2523–2527. doi: 10.1063/1.447985 [20] BORN M, HUANG K, LAX M. Dynamical theory of crystal lattices [J]. American Journal of Physics, 1956, 23(7): 474–483. [21] WANG Y C, LV J, MA Y M, et al. Superconductivity of MgB2 under ultrahigh pressure: a first-principles study [J]. Physical Review B, 2009, 80(9): 092505. doi: 10.1103/PhysRevB.80.092505 [22] XU L F, ZHAO Z S, WANG L M, et al. Prediction of a three-dimensional conductive superhard material: diamond-like BC2 [J]. The Journal of Physical Chemistry C, 2010, 114(51): 22688–22690. doi: 10.1021/jp106926g [23] BADER R F. Atoms in molecules [J]. Accounts of Chemical Research, 1985, 18(1): 9–15. doi: 10.1021/ar00109a003 [24] OGANOV A R, CHEN J, GATTI C, et al. Ionic high-pressure form of elemental boron [J]. Nature, 2009, 460(7252): 863–868. -
下载:
下载:
点击查看大图
计量
- 文章访问数: 8218
- HTML全文浏览量: 3301
- PDF下载量: 54
