First-Principles Investigations on Metallic Silicon Allotropes
-
摘要: 从理论上提出了一种新型金属性硅的同素异形体hP12-Si。 hP12-Si结构可以看作是由六元环形成的一种隧道型结构，与之前报道的Si24结构近似。弹性常数和声子谱的计算结果验证了该结构在常压下的稳定性。通过结构遗传性和热力学稳定性分析表明，可以效仿Si24的制备方法，通过预先合成出高压前驱物LiSi12再除去其中的Li原子来获得hP12-Si。在这种结构中, 有一半的硅原子为5配位，其他硅原子为4配位。电子结构计算表明，该结构具有金属导电性，导电性主要是由于5配位原子的存在导致价电子具有离域性。Abstract: A new metallic metastable silicon allotrope hP12-Si has been theoretically proposed using the particle swarm optimization method. The hP12-Si structure can be seen as a combination of a tunnel-type structure formed from six-membered sp3 silicon rings, which is similar to the previously reported Si24 structure. Its stability was verified by calculating its elastic constants and phonon spectrum. The analysis of structural heritability and thermodynamic stability shows that hP12-Si might be obtained by removing Li atoms from the pre-synthetic LiSi12 precursor, which is analogous with the recent preparation of Si24. There are 50% five coordinated silicon atoms, whereas the others are four coordinated in the hP12-Si structure. Electronic band structure calculation indicated that this structure could perform the metallic properties, which might be resulted from the delocalization of valence electrons caused by the existence of five coordinated atoms.
-
Key words:
- metallic silicon /
- first-principles /
- high density silicon /
- structural transition
-
硅作为现代科学技术的核心材料，已经被广泛应用于集成电路[1–2]和太阳能电池[3–4]领域。但是，常见的Si-I相带隙不易调节并且是间接带隙不利于光吸收，因此不得不发展其他半导体材料代替硅，例如采用昂贵且高毒性的砷化镓代替硅生产高效的太阳能电池[5]。诚然，其他元素也可以合成满足实际应用的新材料，但是拥有丰富储量、无毒性而且成本较低的硅依然是最值得开发利用的材料，因此一直受到极大关注。众所周知，除了常压下最稳定的Si-I相，在不同压力条件下硅还拥有极为丰富的同素异形体[6]，硅的同素异形体中只有高压相具有金属性，但是这些高压相均不能被截留；在常压下，硅的体结构中只有bc8（Si-III）相具有半金属性，其他相均具有半导体的性质。在硅的新结构理论预测方面，一般关注的只是具有半导体性质的硅的新结构，例如间接带隙的硅结构有M相、Z相[7]、bct-Si[8]、M4相[9]、T12相[10]、st12相[11]、C2/m-16相、C2/m-20相、Amm2相、I-4相[12]和Si10[13]，而直接带隙的硅结构有P213相[14]、oF16-Si、tP16-Si、mC12-Si和tI16-Si[15]。具有金属性硅的新结构目前只有Wang等[16]理论预测的隧道型结构硅的同素异形体m-Si20，但是其能量太高，实验合成具有一定难度。
此外，硅还容易与金属反应得到多种多样的金属硅化物，将金属原子从硅化物中去除可以得到新型的硅亚稳相。例如，2014年Wen等[17]采用高温高压方法，以碱金属钠和硅为原料合成了Na4Si24，然后除去其中的钠原子，制备了一种带隙约为1.3 eV的隧道型准直接带隙硅同素异形体Si24；2018年Sung等[18]理论预测了超导P6/m-Si6结构，设计的合成路径也是通过除去对应理论预测的P6/m-NaSi6结构中的钠原子制备新型的超导硅结构P6/m-Si6。新的制备方法增加了获得新型硅材料的技术手段，使发掘新型硅材料的可能性大幅增加。
目前，在硅体结构的实验和理论研究方面，拥有金属性并且常压下稳定的硅同素异形体鲜有报道。本研究将提出一种新型金属性硅的同素异形体hP12-Si，该相的制备可以效仿Si24的合成方法，先合成对应的金属硅化物LiSi12，再除去其中的金属Li原子。该结构具有金属导电性，并且可以在常压下以亚稳相形式存在。
1. 计算方法
采用基于粒子群算法的CALYPSO软件[19–20]，在0 GPa压力下，对单胞原子数小于16的硅晶体结构进行系统搜索；采用基于密度泛函理论（Density Functional Theory，DFT）的CASTEP软件包[21]进行结构优化以及后续性质的理论计算。赝势采用超软赝势（Ultrasoft Pseudopotentials）[22–23]；交换关联泛函选用局域密度近似（Local-Density Approximation，LDA）的CA-PZ形式[24–25]；平面波截止能为400 eV；k点的选取采用Monkhorst-Pack方法[26]，最大分割间隔为0.04×2π Å–1。结构优化过程的收敛条件为：总能量变化、最大离子位移、内应力和离子的Hellmann-Feynman力分别小于5.0×10–6 eV/atom、5.0×10–4 Å、0.02 GPa和0.01 eV/Å。选取原胞计算晶体结构的弹性常数、体弹性模量和剪切模量，在–0.005～0.005的应变范围内平均取6个应变点；在单胞基础上的2×2×2超胞上进行布居数计算。本研究的结构表现为金属性，所以采用基于CASTEP软件[21]的有限位移方法计算声子谱[27]。所有计算参数都经过了仔细的收敛性测试和准确性测试。
2. 结果与讨论
2.1 晶体结构
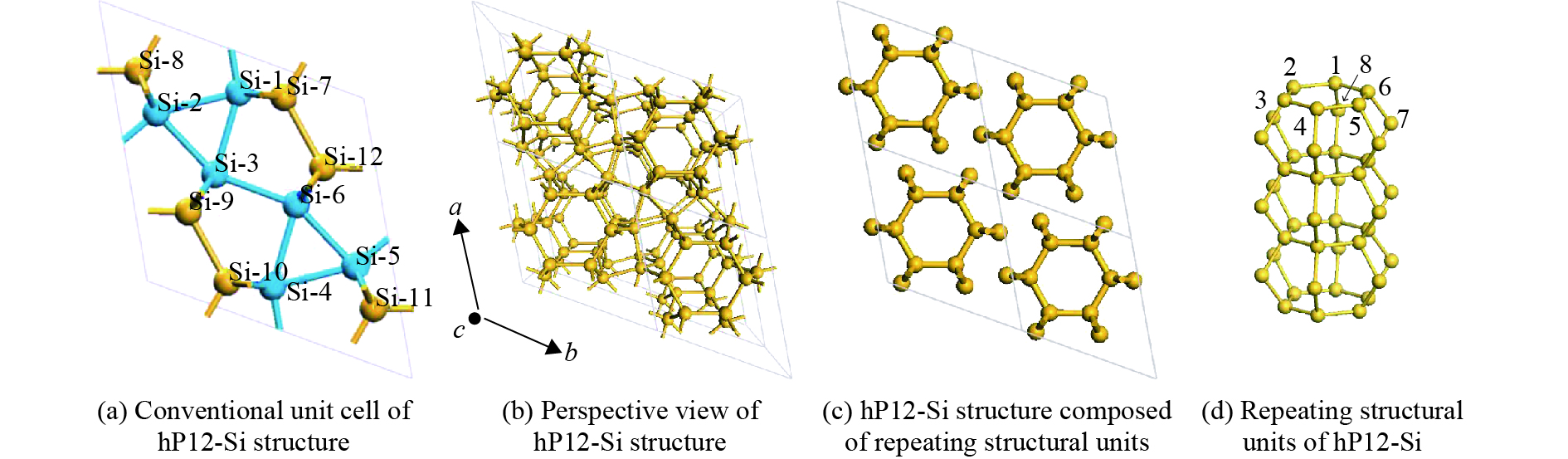
通过晶体结构预测软件[3]，找到一种同近期实验合成Si24结构[17]类似的隧道型结构硅的同素异形体hP12-Si，如图1（a）所示。在图1（b）中，沿c轴方向hP12-Si明显由六元环、五元环和三元环构成的隧道型结构组合而成，其中六元环和三元环是平面结构，它们之间通过五元环连接构成hP12-Si。如图1（c）所示，断开其中三元环的化学键，可以解析出组成hP12-Si结构的重复单元，这些重复单元像“齿轮”一样互相咬合，并且保持最优距离构成了hP12-Si。图1（d）显示了hP12-Si重复结构单元的立体图，像一串“灯笼”垒在一起构成了类似Si24中的笼型结构，所以“灯笼”内可以填入合适的间隙原子。
图1（a）为hP12-Si的常规单胞结构，属于六方晶系，每个单胞含有12个原子（Si-1～Si-12），在常压下的晶格参数和原子位置见表1。其中Si-1～Si-6（蓝色）是图1（c）中“齿轮”结构最外面一圈的硅原子，与内部组成六元环的Si-7～Si-12硅原子不同，最外一圈的硅原子起着连接最近邻“齿轮”结构的作用，在平面内形成了三元环。hP12-Si结构里有一半的原子是5配位，与Wang等[16]提到的m-Si20结构很像，推测该结构可能也具有金属性。如表2中的数据所示，hP12-Si结构中存在4种键长，其中构成重复单元的键长2.321 Å和2.327 Å都略小于Si-I的键长2.328 Å，起连接作用的其他两种键长都比Si-I大。虽然hP12-Si的结构是开放式框架结构，但是其密度（2.512 g/cm3）却大于Si-I（2.402 g/cm3），主要是由于hP12-Si结构中重复单元的键长相对较短，并且重复单元之间互相咬合、紧密排列导致的。另外，hP12-Si结构可能像硅的其他高密度相一样，也是压力驱动型结构[28]。
表 1 常压下hP12-Si单胞的晶体结构数据Table 1. Crystallographic data for hP12-Si conventional cellSpace group a/Å c/Å ρ/(g·cm–3) Atomic positions P6/m (175) 8.202 3.823 2.512 Si:6k (0.499 33, 0.846 42, 0.5) Si:6j (0.679 02, 0.892 27, 0.0) 表 2 hP12-Si单胞中化学键的布居数和键长Table 2. Populations and length of silicon bond in hP12-Si conventional cellSilicon bond Populations Length of silicon bond/Å Si-7—Si-11, Si-7—Si-12, Si-8—Si-10 0.76 2.321 Si-8—Si-12, Si-9—Si-10, Si-9—Si-11 Si-1—Si-7, Si-2—Si-8, Si-3—Si-9 0.77 2.327 Si-4—Si-10, Si-5—Si-11, Si-6—Si-12 Si-1—Si-2, Si-1—Si-3, Si-2—Si-3 0.33 2.462 Si-4—Si-5, Si-4—Si-6, Si-5—Si-6 Si-1—Si-4, Si-2—Si-5, Si-3—Si-6 0.41 2.514 2.2 机械和动力学稳定性
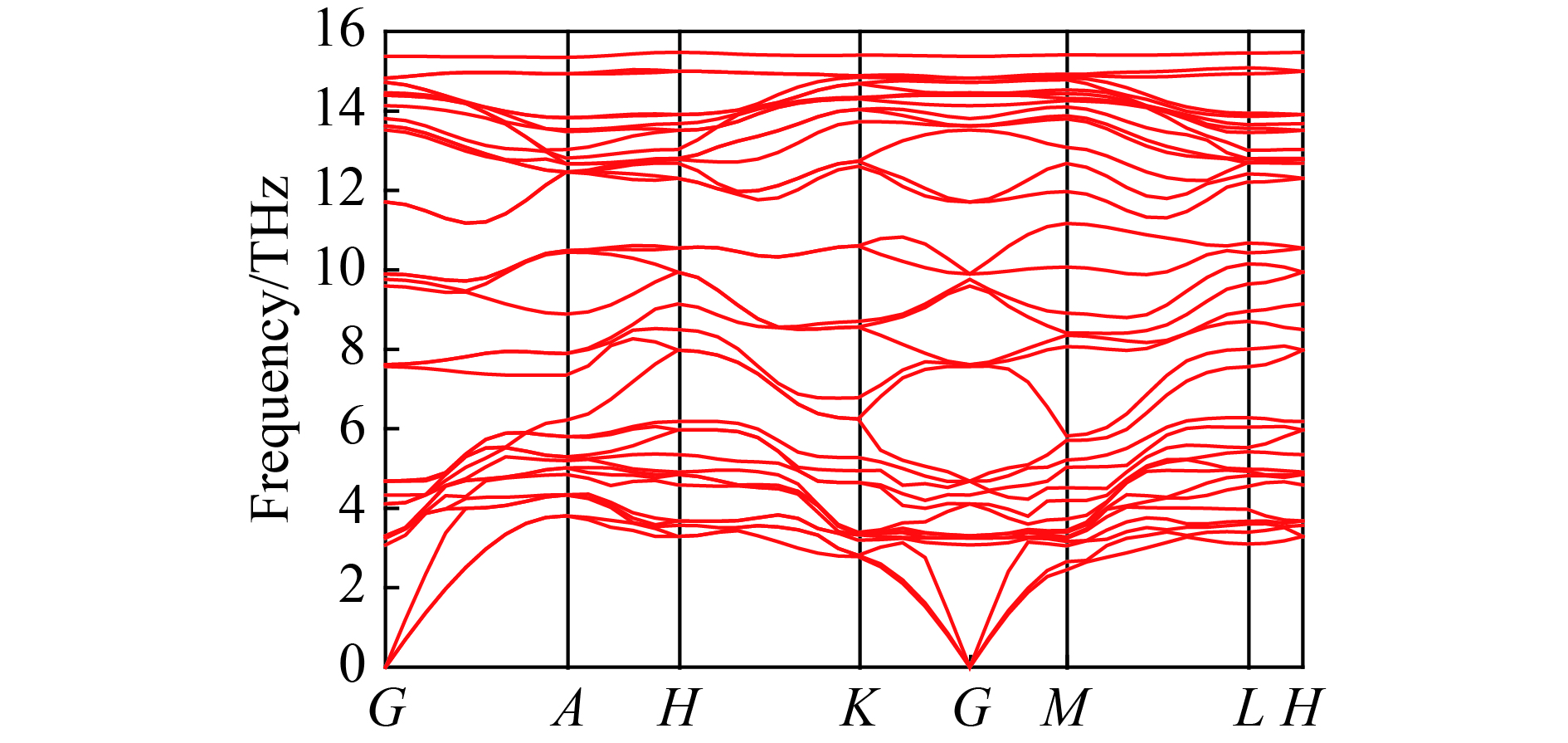
为了证明hP12-Si结构的机械稳定性和动力学稳定性，分别计算其弹性常数和声子谱。对比hP12-Si、Si24和Si-I结构在线性弹性应变范围内的弹性常数，如表3所示。由于hP12-Si结构属于六方晶系，其机械稳定性判据为：C11>|C12|，2(C13)2<C33(C11+C12)，C44>0，C66>0[29–31]，显然hP12-Si结构的弹性常数满足六方晶系的机械稳定性判据，因此是机械稳定的。hP12-Si结构的体弹性模量和剪切模量分别为94 GPa和51 GPa，大小处于Si24和Si-I结构的弹性模量之间，说明其机械强度也应该处于Si24和Si-I结构之间。hP12-Si结构的动力学稳定性是通过声子谱判定，图2为hP12-Si结构的声子谱，在整个布里渊区里没有出现虚频，因此是动力学稳定的。上述稳定性研究的结果表明：常压下，实验合成的hP12-Si可以稳定存在。
表 3 常压下hP12-Si、Si24和Si-I结构的密度、弹性常数、体弹性模量和剪切模量Table 3. Calculated density, elastic constants , bulk moduli, and shear moduli of hP12-Si, Si24 and Si-I at ambient pressureStructure ρ/(g·cm–3) Elastic constants/GPa B/GPa G/GPa C11 C22 C33 C44 C55 C66 C12 C13 C23 hP12-Si 2.512 166 166 148 51 51 56 66 66 94 94 51 Si24 2.236 164 204 147 37 42 51 40 46 85 85 50 Si-I 2.402 161 161 161 76 76 76 62 62 95 95 64 2.3 热力学稳定性
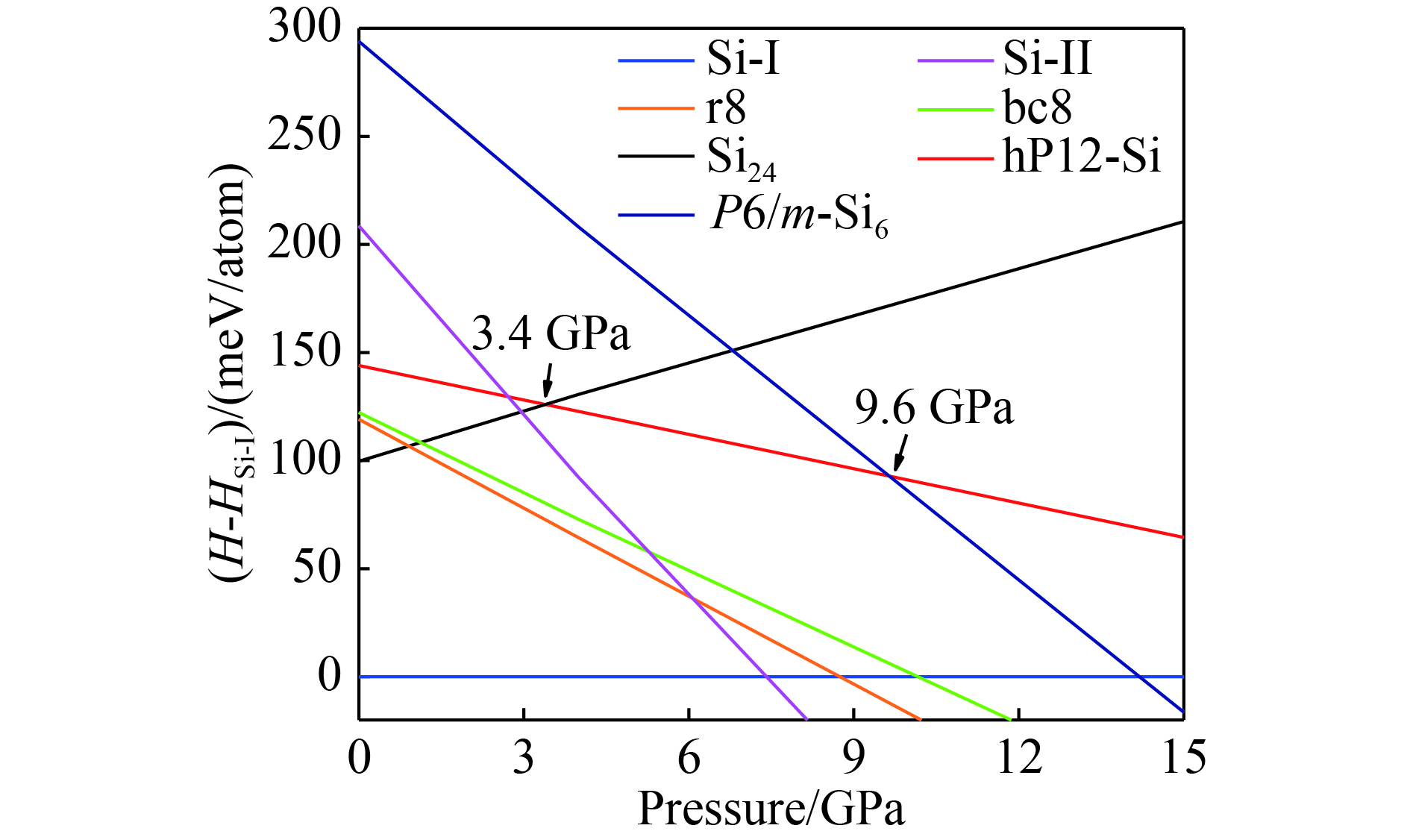
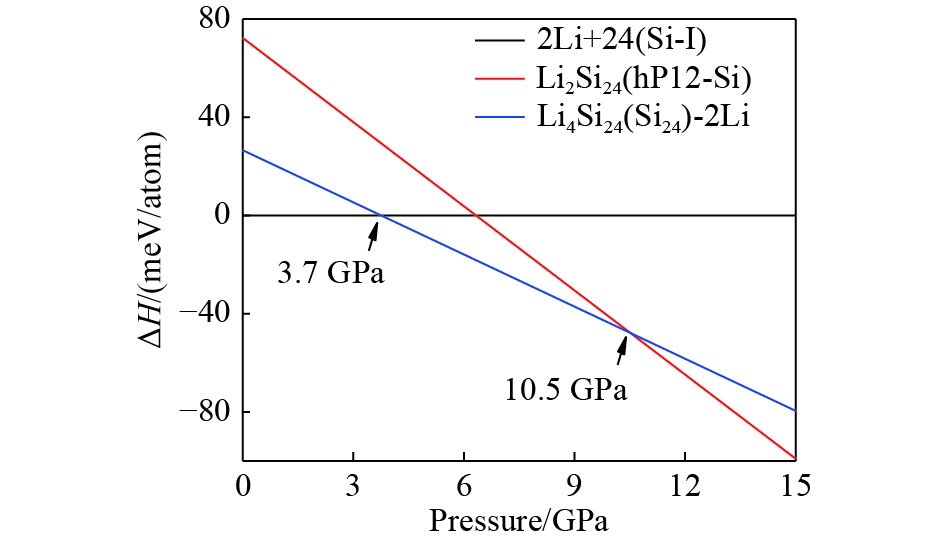
热力学稳定性是证明hP12-Si结构存在和能否被合成的先决条件。图3为hP12-Si和前人已报道的硅亚稳相（Si-II、bc8、r8、P6/m-Si6和Si24）与Si-I对比的焓压曲线。在所研究的压力范围内，随着压力升高，硅的最稳定相由Si-I变成了Si-II相，说明如果仅仅考虑热力学因素，任何压力条件下这些硅的亚稳相都不可能稳定存在。然而在不同的实验条件下，这些能量相对较高的硅的亚稳相（bc8、r8和Si24）已经被成功制备，正如焓压曲线分析的结果一样，动力学因素在低压力区硅的相变过程中的作用至关重要。在常压下，hP12-Si的能量高于稳定相Si-I而低于高压相Si-II，说明它也是亚稳的；随着压力升高，hP12-Si相对于Si-I结构的焓差越来越小，说明相对于Si-I结构而言hP12-Si是压力驱动型结构；随着压力继续升高至3.4～9.6 GPa时，hP12-Si结构的能量同时低于Si24和P6/m-Si6，说明在该压力范围内hP12-Si比已知的Si24和P6/m-Si6结构更稳定。
图 3 硅同素异形体相对于Si-I相的焓压曲线Figure 3. Enthalpies of various Si allotropes relative to Si-I as a function of pressure此外，通过结构遗传性[13]分析，可以在一定程度上判断单质相变过程的难易。从结构遗传的角度看，拥有相似隧道型结构的hP12-Si和Si24结构之间的相变能垒可能比其他低压区的三维网状硅结构（Si-I、bc8、r8和Si-II）之间的相变能垒小。所以，推测hP12-Si可以通过Si24结构直接高压相变制备，或者采用类似合成Si24结构的实验方法制备。
2.4 合成路径
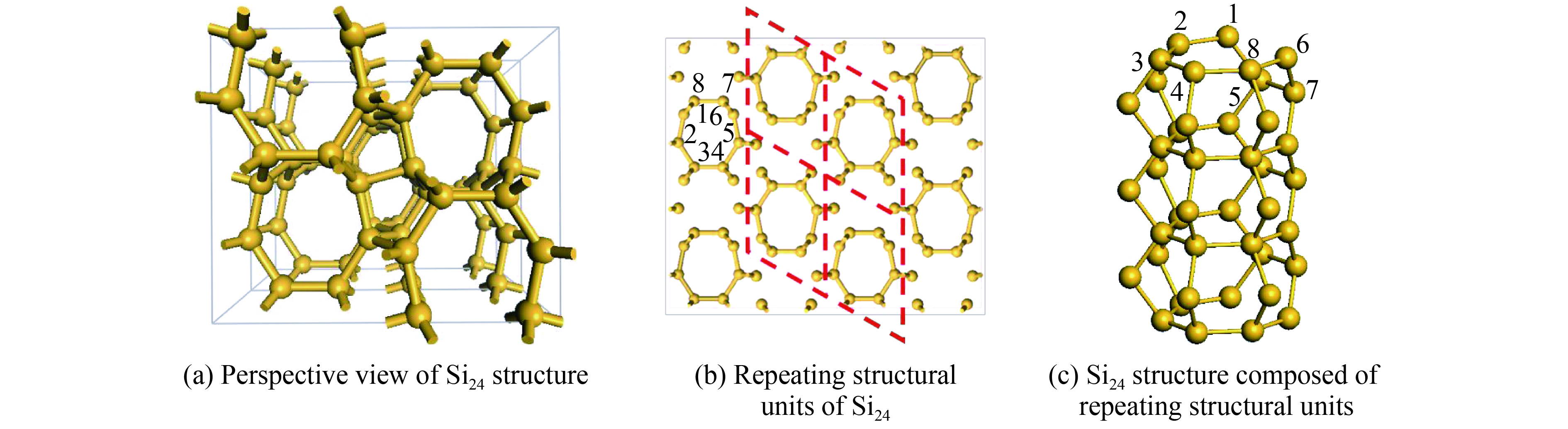
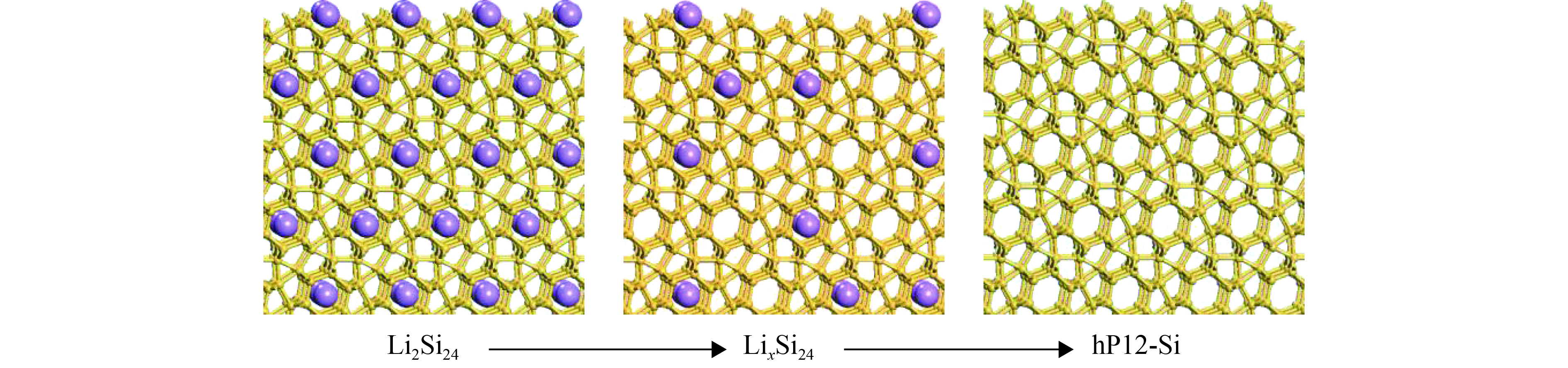
为了得到相对合理的合成hP12-Si的实验方法，通过类似结构分析方法解析了已经实验合成的Si24的晶体结构，图4为Si24晶体的结构模型。如图4（a）所示，Si24常规单胞晶体是由八元环和五元环组合成的隧道型结构，该结构与hP12-Si结构类似。如图4（b）所示，解析Si24晶体结构的重复单元并且断开结构之间的连接，发现Si24的重复单元（红色线框内）之间的“咬合”方式与hP12-Si的分解结构（图1（c））类似，所以本质区别即为两者重复单元之间的异同。
图4（c）和图1（d）分别为Si24和hP12-Si重复单元组成的部分结构，两者的区别是“灯笼”口部分原子的成键不同。在图4（c）中，Si24重复单元中标号7和8位置处的原子断键，然后标号1和6处的原子成键，由原来的八元环（图4（c））变成了六元环（图1（d））导致“灯笼”口收紧，标号7和8处两个原子恰好变成了hP12-Si的组成单元。分析对比hP12-Si和Si24晶体结构的特点，发现两者结构之间差别非常小，只需作微小的改变。虽然两者结构之间存在很强的遗传性，但是从Si24直接相变到hP12-Si需要满足理想的、统一的断键和成键过程，这个过程在一个完整的三维体结构里发生并不容易。可以借鉴由Na4Si24合成Si24结构的过程[1]，以及由P6/m-NaSi6合成P6/m-Si6的实验方法[2]。如果将Na换成Li，由于Li的原子半径更小，同时施加更高的压力使得“灯笼”口变小（图1（d）），很有可能先合成对应的金属硅化物Li2Si24（即LiSi12），然后采用类似的方法[1–2]除去“灯笼”里的Li原子从而得到hP12-Si。如图5所示，通过计算拥有hP12-Si结构框架的金属硅化物Li2Si24、拥有Si24框架的金属硅化物Li4Si24与单质Li和Si-I组成的原料之间的焓压曲线，发现在压力超过约10.5 GPa后拥有hP12-Si结构框架的金属硅化物Li2Si24（即LiSi12）成为相对最稳定的结构。图6为Li2Si24脱Li生成hP12-Si的过程，图中紫色为Li原子、黄色为硅原子, 仿照合成Si24的方法除去结构内的Li原子后可以得到hP12-Si。目前，理论预测和结构遗传性分析结果均能很好地支持这种设计方案，但是与合成理论预测的P6/m-Si6相同，这种合成hP12-Si的方法还有待进一步的实验检验。
图 5 Li2Si24和Li4Si24原料的焓压曲线Figure 5. Enthalpies of Li2Si24 and Li4Si24 phase relative to reactants (Li and Si-I) as a function of pressure
图 6 Li2Si24脱Li过程Figure 6. Schematic of the compositional change from Li2Si24 (left) to hP12-Si (right)2.5 电子性质
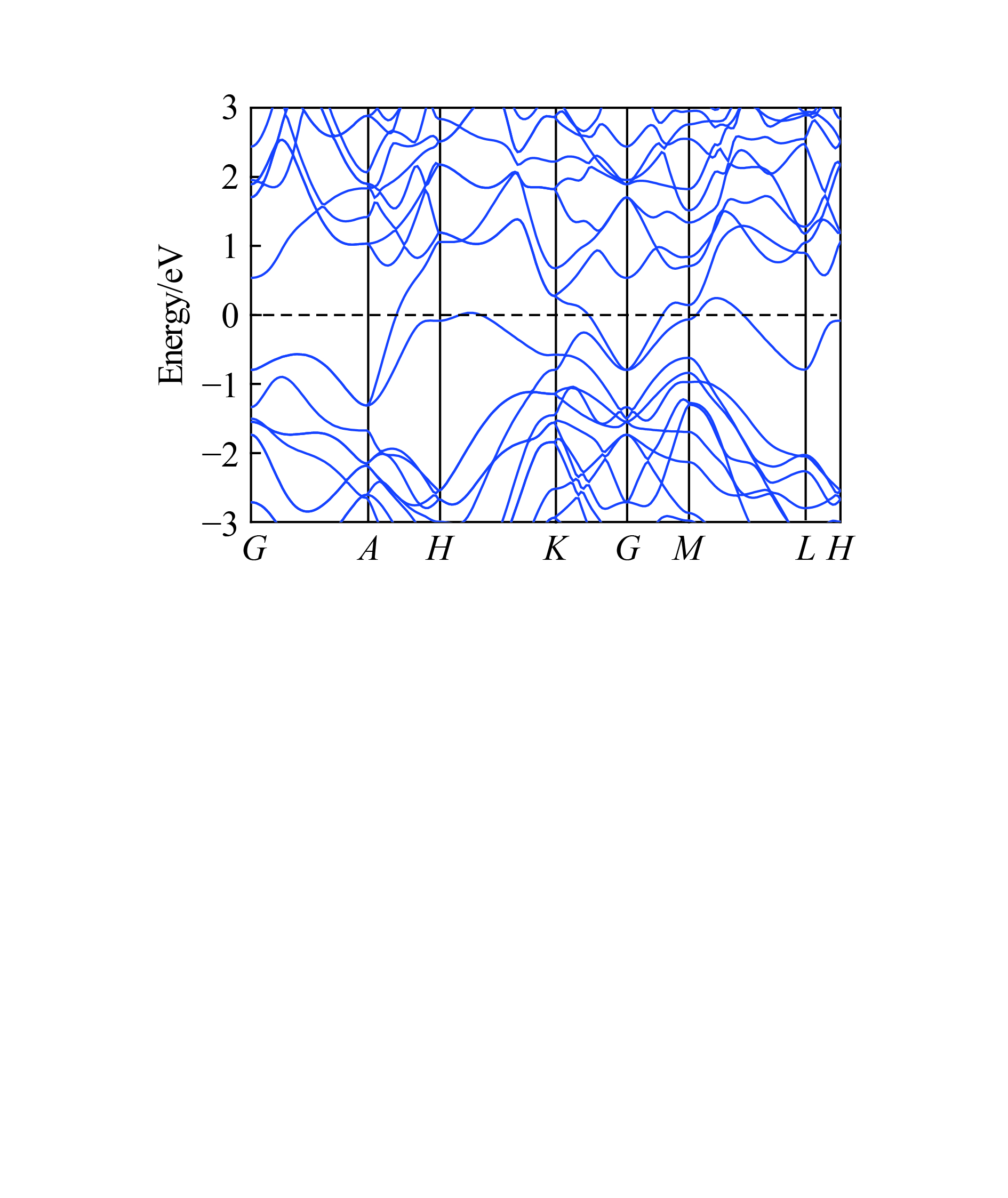
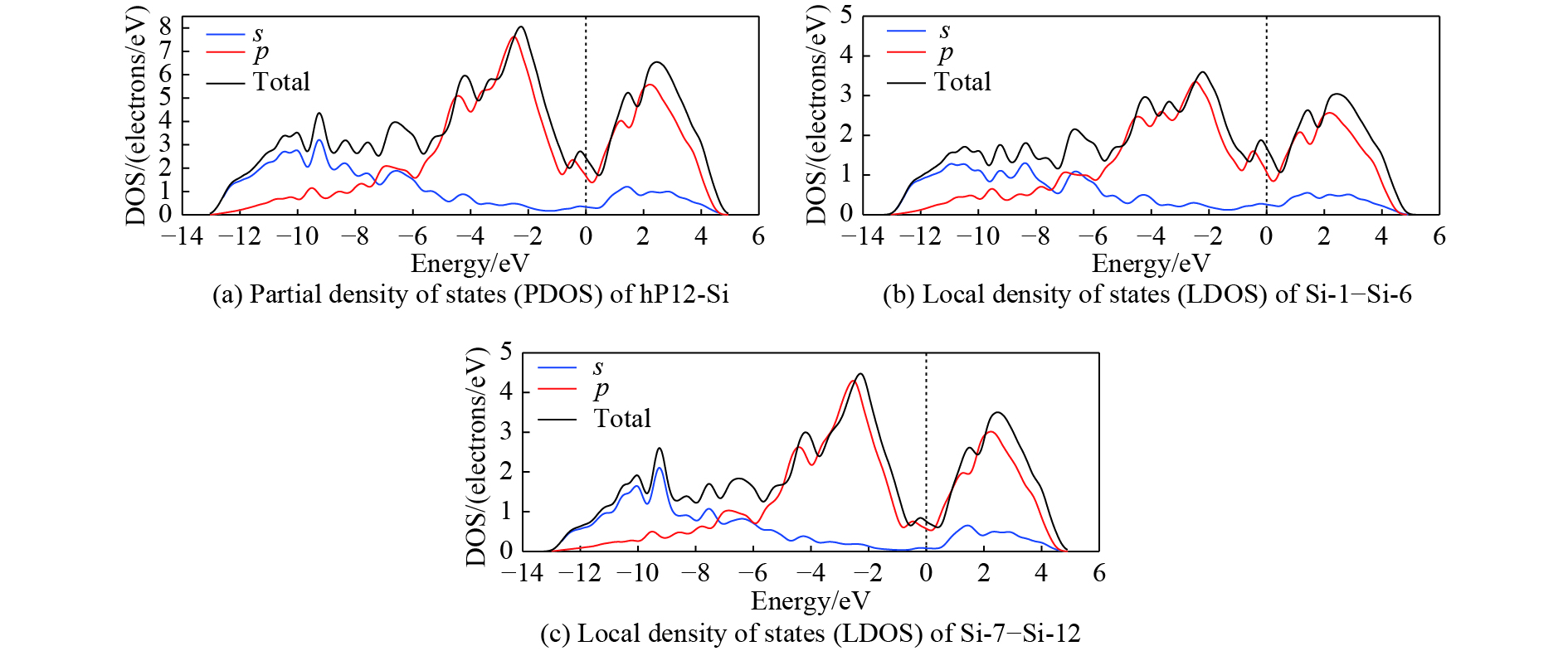
硅原子含有4个价电子，常压下结构中的硅原子通常以sp3杂化方式成键，因此价电子被局域在共价键上，没有或者只有很少的自由电子。尽管硅的金属性在其高压相中十分普遍，但是实验获得的常压下亚稳相中并没有发现具有良好金属性的硅同素异形体。如图7所示，在hP12-Si的能带结构中有两条能带穿过费米能级，表明hP12-Si具有金属导电性。如图8（a）所示，从电子分波态密度图中可知，hP12-Si的导电性主要来自硅的p轨道电子。由于在费米能级附近的电子处于成键态，说明价电子未能将价带填满（能带图中也能看出），因此容易与其他物质结合，形成满电子化合物。从图8（a）中还可以看出费米能级处存在一个小峰，通过分析hP12-Si的局域电子态密度（图8（b）和图8（c）），可以发现每个硅原子对费米能级处的小峰均有贡献，但是主要贡献来自于具有5配位的Si-1～Si-6原子。
实验发现，硅的金属性同素异形体只出现在高压相中，主要由于高压导致了硅原子配位数的改变，使其价电子不能完全局域在共价键上，因而出现了自由电子。随着外界压力升高，硅原子的配位数不断增加，共价键也随之减弱，从4配位的Si-I相中的三维共价键网络，直到12配位的Si-X相中变成完全的金属键[32]。例如，Si24结构中全部都是4配位的硅原子，电子都局域在共价键上，没有自由电子，所以无法导电。而hP12-Si的导电性源于其结构中具有5配位的硅原子，导致部分电子离域而出现自由电子。同时，由于出现5配位原子，硅原子之间也表现出一定的离子性，如表4中的电子布居数所示。Si-1～Si-6原子周围有5个最近邻原子，比4配位的硅原子需要更多的电子参与成键，因此分别从相邻的硅原子获得部分电荷。
表 4 常压下hP12-Si结构的原子位置、配位数和布居数Table 4. Wyckoff positions, coordination numbers, and populations of silicon atoms in hP12-SiAtomic number Wyckoff positions Coordination numbers Populations Si-1–Si-6 6k (0.499 33, 0.846 42, 0.5) 5 –0.02 Si-7–Si-12 6j (0.679 02, 0.892 27, 0.0) 4 0.02 3. 结 论
通过理论计算的方法提出了一种新型金属性硅的同素异形体hP12-Si。研究表明该结构具有机械稳定性和动力学稳定性，说明它可以在常压下亚稳存在。由于存在5配位的硅原子，导致hP12-S结构中出现了部分离域电子，从而表现出一定的金属性，该结构与Si24和P6/m-Si6的结构相似，都拥有隧道型结构。经结构遗传性和热力学稳定性分析发现：可以效仿Si24的制备方法，先合成LiSi12再除去其中的Li原子来获得hP12-Si。亚稳金属性硅同素异形体的发现，可能将硅的应用拓展到更广的领域。
-
图 3 硅同素异形体相对于Si-I相的焓压曲线
Figure 3. Enthalpies of various Si allotropes relative to Si-I as a function of pressure
图 5 Li2Si24和Li4Si24原料的焓压曲线
Figure 5. Enthalpies of Li2Si24 and Li4Si24 phase relative to reactants (Li and Si-I) as a function of pressure
图 6 Li2Si24脱Li过程
Figure 6. Schematic of the compositional change from Li2Si24 (left) to hP12-Si (right)
表 1 常压下hP12-Si单胞的晶体结构数据
Table 1. Crystallographic data for hP12-Si conventional cell
Space group a/Å c/Å ρ/(g·cm–3) Atomic positions P6/m (175) 8.202 3.823 2.512 Si:6k (0.499 33, 0.846 42, 0.5) Si:6j (0.679 02, 0.892 27, 0.0) 
下载: 导出CSV
表 2 hP12-Si单胞中化学键的布居数和键长
Table 2. Populations and length of silicon bond in hP12-Si conventional cell
Silicon bond Populations Length of silicon bond/Å Si-7—Si-11, Si-7—Si-12, Si-8—Si-10 0.76 2.321 Si-8—Si-12, Si-9—Si-10, Si-9—Si-11 Si-1—Si-7, Si-2—Si-8, Si-3—Si-9 0.77 2.327 Si-4—Si-10, Si-5—Si-11, Si-6—Si-12 Si-1—Si-2, Si-1—Si-3, Si-2—Si-3 0.33 2.462 Si-4—Si-5, Si-4—Si-6, Si-5—Si-6 Si-1—Si-4, Si-2—Si-5, Si-3—Si-6 0.41 2.514
下载: 导出CSV
表 3 常压下hP12-Si、Si24和Si-I结构的密度、弹性常数、体弹性模量和剪切模量
Table 3. Calculated density, elastic constants , bulk moduli, and shear moduli of hP12-Si, Si24 and Si-I at ambient pressure
Structure ρ/(g·cm–3) Elastic constants/GPa B/GPa G/GPa C11 C22 C33 C44 C55 C66 C12 C13 C23 hP12-Si 2.512 166 166 148 51 51 56 66 66 94 94 51 Si24 2.236 164 204 147 37 42 51 40 46 85 85 50 Si-I 2.402 161 161 161 76 76 76 62 62 95 95 64
下载: 导出CSV
表 4 常压下hP12-Si结构的原子位置、配位数和布居数
Table 4. Wyckoff positions, coordination numbers, and populations of silicon atoms in hP12-Si
Atomic number Wyckoff positions Coordination numbers Populations Si-1–Si-6 6k (0.499 33, 0.846 42, 0.5) 5 –0.02 Si-7–Si-12 6j (0.679 02, 0.892 27, 0.0) 4 0.02
下载: 导出CSV
-
[1] KILBY J S. Miniaturized electronic circuits [J]. Google Patents, 1964. [2] NOYCE R N. Semiconductor device-and-lead structure: US 2981877 [P]. 1961–04–25. [3] CARLSON D E, WRONSKI C R. Amorphous silicon solar cell [J]. Applied Physics Letters, 1976, 28(11): 671–673. doi: 10.1063/1.88617 [4] GREEN M A. Solar cells: operating principles, technology, and system applications [J]. Prentice-Hall, 1982. [5] GREEN M A, EMERY K, HISHIKAWA Y, et al. Solar cell efficiency tables (Version 45) [J]. Progress in Photovoltaics: Research and Applications, 2015, 23(1): 1–9. doi: 10.1002/pip.v23.1 [6] MUJICA A, RUBIO A, MUNOZ A, et al. High-pressure phases of group-IV, III-V, and H-VI compounds [J]. Reviews of Modern Physics, 2003, 75(3): 863–912. doi: 10.1103/RevModPhys.75.863 [7] BAUTISTA-HERNANDEZ A, RANGEL T, ROMERO A H, et al. Structural and vibrational stability of M and Z phases of silicon and germanium from first principles [J]. Journal of Applied Physics, 2013, 113(19): 193504. doi: 10.1063/1.4804668 [8] FUJIMOTO Y, KORETSUNE T, SAITO S, et al. A new crystalline phase of four-fold coordinated silicon and germanium [J]. New Journal of Physics, 2008, 10(8): 083001. [9] WU F, JUN D, KAN E, et al. Density functional predictions of new silicon allotropes: electronic properties and potential applications to Li-battery anode materials [J]. Solid State Communications, 2011, 151(18): 1228–1230. doi: 10.1016/j.ssc.2011.06.001 [10] ZHAO Z, TIAN F, DONG X, et al. Tetragonal allotrope of group 14 elements [J]. Journal of the American Chemical Society, 2012, 134(30): 12362–12365. doi: 10.1021/ja304380p [11] MALONE B D, SAU J D, COHEN M L. Ab initio survey of the electronic structure of tetrahedrally bonded phases of silicon [J]. Physical Review B, 2008, 78(3): 35210. doi: 10.1103/PhysRevB.78.035210 [12] FAN Q, CHAI C, WEI Q, et al. Novel silicon allotropes: stability, mechanical, and electronic properties [J]. Journal of Applied Physics, 2015, 118(18): 185704. doi: 10.1063/1.4935549 [13] LUO K, ZHAO Z, MA M, et al. Si10: a sp3 silicon allotrope with spirally connected Si5 tetrahedrons [J]. Chemistry of Materials, 2016, 28(18): 6441–6445. doi: 10.1021/acs.chemmater.6b02484 [14] XIANG H J, HUANG B, KAN E, et al. Towards direct-gap silicon phases by the inverse band structure design approach [J]. Physical Review Letters, 2013, 110(11): 13–16. [15] WANG Q, XU B, SUN J, et al. Direct band gap silicon allotropes [J]. Journal of the American Chemical Society, 2014, 136(28): 9826–9829. doi: 10.1021/ja5035792 [16] WANG Q, LUO K, MA M, et al. A new metastable metallic silicon allotrope [J]. Chinese Science Bulletin (Chinese Version), 2015, 60(27): 2616. doi: 10.1360/N972015-00200 [17] WEN Z, LU G, MAO S, et al. Silicon nanotube anode for lithium-ion batteries [J]. Electrochemistry Communications, 2013, 29: 67–70. doi: 10.1016/j.elecom.2013.01.015 [18] SUNG H J, HANG W H, LEE I H, et al. Superconducting open-framework allotrope of silicon at ambient pressure [J]. Physical Review Letters, 2018, 120(15): 157001. doi: 10.1103/PhysRevLett.120.157001 [19] BAI J, ZENG X C, TANAKA H, et al. Metallic single-walled silicon nanotubes [J]. Proceedings of the National Academy of Sciences of the United States of America, 2004, 101(9): 2664–2668. doi: 10.1073/pnas.0308467101 [20] HEVER A, BERNSTEIN J, HOD O. Structural stability and electronic properties of sp3 type silicon nanotubes [J]. The Journal of chemical physics, 2012, 137(21): 214702. doi: 10.1063/1.4767389 [21] LIN C, POVINELLI M L. Optical absorption enhancement in silicon nanowire arrays with a large lattice constant for photovoltaic applications [J]. Optics Express, 2009, 17(22): 19371–19381. doi: 10.1364/OE.17.019371 [22] CLARK S J, SEGALL M D, PICKARD C J, et al. First principles methods using CASTEP [J]. Zeitschrift für Kristallographie–Crystalline Materials, 2005, 220(5/6): 567–570. [23] WANG Y, LV J, ZHU L, et al. CALYPSO: A method for crystal structure prediction [J]. Computer Physics Communications, 2012, 183(10): 2063–2070. doi: 10.1016/j.cpc.2012.05.008 [24] WANG Y, LV J, ZHU L, et al. Crystal structure prediction via particle-swarm optimization [J]. Physical Review B, 2010, 82(9): 094116. [25] LAASONEN K, CAR R, LEE C, et al. Implementation of ultrasoft pseudopotentials in ab initio molecular dynamics [J]. Physical Review B, 1991, 43(8): 6796–6799. doi: 10.1103/PhysRevB.43.6796 [26] VANDERBILT D. Soft self-consistent pseudopotentials in a generalized eigenvalue formalism [J]. Physical Review B, 1990, 41(11): 7892–7895. doi: 10.1103/PhysRevB.41.7892 [27] PERDEW J P, ZUNGER A. Self-interaction correction to density-functional approximations for many-electron systems [J]. Physical Review B, 1981, 23(10): 5048–5079. doi: 10.1103/PhysRevB.23.5048 [28] PURVEE B, SADHNA S. Pressure induced structural phase transitions–a review [J]. Central European Journal of Chemistry, 2012, 10(5): 1391–1422. [29] CEPERLEY D M, ALDER B J. Ground state of the electron gas by a stochastic method [J]. Physical Review Letters, 1980, 45(7): 566–569. doi: 10.1103/PhysRevLett.45.566 [30] MONKHORST H J, PACK J D. Special points for Brillouin-zone integrations [J]. Physical Review B, 1976, 13(12): 5188. doi: 10.1103/PhysRevB.13.5188 [31] KRESSE G, FURTHMÜLLER J, HAFNER J. Ab initio force constant approach to phonon dispersion relations of diamond and graphite [J]. Europhysics Letters, 1995, 32(9): 729. doi: 10.1209/0295-5075/32/9/005 [32] TSE J S, KLUG D D, PATCHKOVSKII S, et al. Chemical bonding, electron-phonon coupling, and structural transformations in high-pressure phases of Si [J]. The Journal of Physical Chemistry B, 2006, 110(8): 3721–3726. doi: 10.1021/jp0554341 -
下载:
下载:
点击查看大图
计量
- 文章访问数: 11515
- HTML全文浏览量: 4426
- PDF下载量: 40
