Free Vibrations of Pre/Post-Buckled Graphene-Reinforced Epoxy Resin Matrix Nanocomposite Beams
-
摘要: 采用微分求积法分析了石墨烯增强纳米复合材料梁屈曲前后的自由振动问题。考虑石墨烯纳米片在基体中随机排列和定向排列的情况,采用Halpin-Tsai微观力学模型估算两种模式下石墨烯纳米复合材料的弹性模量,并通过Hamilton原理建立基于一阶剪切变形理论下梁的动力学控制方程。利用微分求积法计算得到石墨烯纳米复合材料梁的临界屈曲载荷及屈曲前后的固有频率。数值计算结果表明:按合理排列模式掺杂较多的薄石墨烯纳米片,会大幅度提高梁的临界屈曲载荷以及屈曲前的固有频率;但屈曲发生后,同样的做法却会使结构的刚度降低。Abstract: Free vibration of pre/post-buckled graphene-reinforced nanocomposite beams was analyzed by the differential quadrature method. Considering the random distribution and directional arrangement of graphene nanoplatelets in the matrix, Young’s moduli of graphene nanocomposites in two modes were estimated by Halpin-Tsai micromechanical model. The first-order shear deformation theory was used to establish the governing equations of beams by Hamilton principle. The critical buckling loads of the graphene-reinforced nanocomposite beam and the natural frequencies in the pre/post-buckling regimes were calculated by the differential quadrature method. Numerical results show that dispersing more graphene platelets with less single layers and arranging them in a reasonable mode will greatly increase the critical buckling loads of the beams and the natural frequencies in pre-buckling regime. However, the same approach reduces the stiffnesses of the beams in the post-buckling regime.
-
Key words:
- graphene /
- distribution mode /
- differential quadrature method /
- buckling /
- natural frequency
-
It is very important for mining and civil construction to predict the morphology distribution of cracks induced by blasting. Hence, many researchers have paid their attention to dynamic fracture behavior of rocks due to drilling and blasting operations[1-3]. A number of experiments and numerical simulations have been conducted to investigate the blasting-induced fractures in the near borehole zone as well as in the far field[4-5]. In order to gain high fidelity in simulating the complex responses of rocks subjected to blasting loading, a realistic constitutive model is required. In the last 20 years, various macro-scale material models have been proposed, from relatively simple ones to more sophisticated, and their capabilities in describing actual nonlinear behavior of material under different loading conditions have been evaluated[6].
During blasting operation, chemical reactions of explosive in borehole occur rapidly, and instantaneously a shock/stress wave applies to borehole wall. Initially, a crushed zone around the borehole is developed by the shock/stress wave. Then, a radial shock/stress wave propagates away from borehole, and its magnitude decreases. Once the radial shock/stress drops below the local dynamic compressive strength, no shear damage occurs. At the same time, a tensile tangential stress with enough strength can be developed behind the radial compressive stress wave, which results in an extension of the existing flaws or a creation of new radial cracks. If there is a nearby free boundary, the incident compressive stress wave changes to a tensile stress wave upon reflection, and reflects back into the rock. In this case, if the dynamic tensile strength of rock is exceeded, spall cracks appear close to the free boundary.
The purpose of this paper is to conduct a numerical study on borehole blasting-induced fractures in rocks. First, a dynamic constitutive model for rocks based on the previous work of concrete[7] is briefly described, and the values of various parameters in the model for granite are estimated. The model is then employed to simulate the borehole blasting-induced fractures in granitic rocks. Comparisons between the numerical results and the experimental observations are made, and a discussion is given.
1. Dynamic Constitutive Model for Rocks
A number of models for concrete-like materials, such as TCK model[8], HJC model[9], RHT model[10], K&C model[11], have been developed. The sophisticated numerical models are increasingly used as they are capable of describing the material behavior under high strain rate loading. However, these models have been found to have some serious flaws, and cannot predict the experimentally observed crack patterns or exhibit improper behavior under certain loading conditions[7, 12-15].
In the following, a dynamic constitutive model for rocks is briefly described according to equation of state (EOS) and strength model, based on the previous work on concrete[7].
1.1 EOS
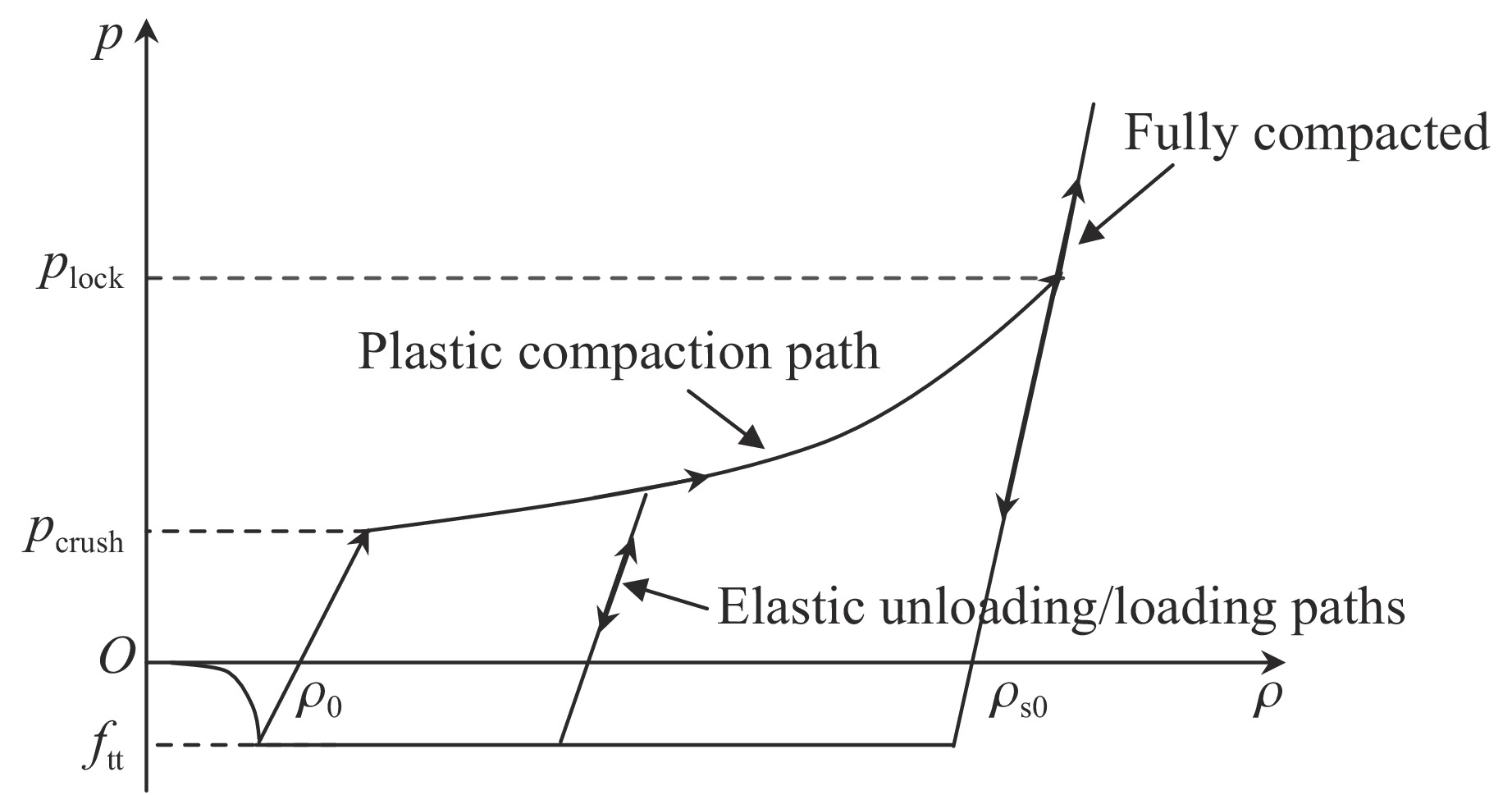
A typical form of EOS is the so-called p-
α relation, which is proved to be capable of representing brittle material’s response behavior at high pressures, and it allows for a reasonably detailed description of the compaction behavior at low pressure ranges as well, as shown schematically in Fig.1.pcrush corresponds to the pore collapse pressure beyond which plastic compaction occurs, andplock is the pressure when porosityα reaches 1,ftt is the tensile strength,ρ0 is the initial density,ρs0 refers to the density of the initial solid. Figure 1. Schematic diagram of EOS[7]
Figure 1. Schematic diagram of EOS[7]The EOS for compression (p≥0) is given by
p=K1ˉμ+K2ˉμ2+K3ˉμ3 (1) where p denotes pressure, K1, K2, K3 are constants, and
ˉμ is defined byˉμ=ραρ0α0−1=αα0(1+μ)−1 (2) where
ρ is the current density,μ=ρ/ρ0−1 specifies the volumetric strain,α0 =ρ s0/ρ 0 andα=ρs/ρ represent the initial porosity and the current porosity, respectively.ρs refers to the density of fully compacted solid. Physically,α is a function of the hydro-static pressure p, and is expressed asα=1+(α0−1)(plock−pplock−pcrush)n (3) where n is the compaction exponent.
When material withstands hydro-static tension, the EOS for tension (p<0) is given by
p=K1ˉμ (4) α=α0(1+p/K1)/(1+μ) (5) 1.2 Strength Model
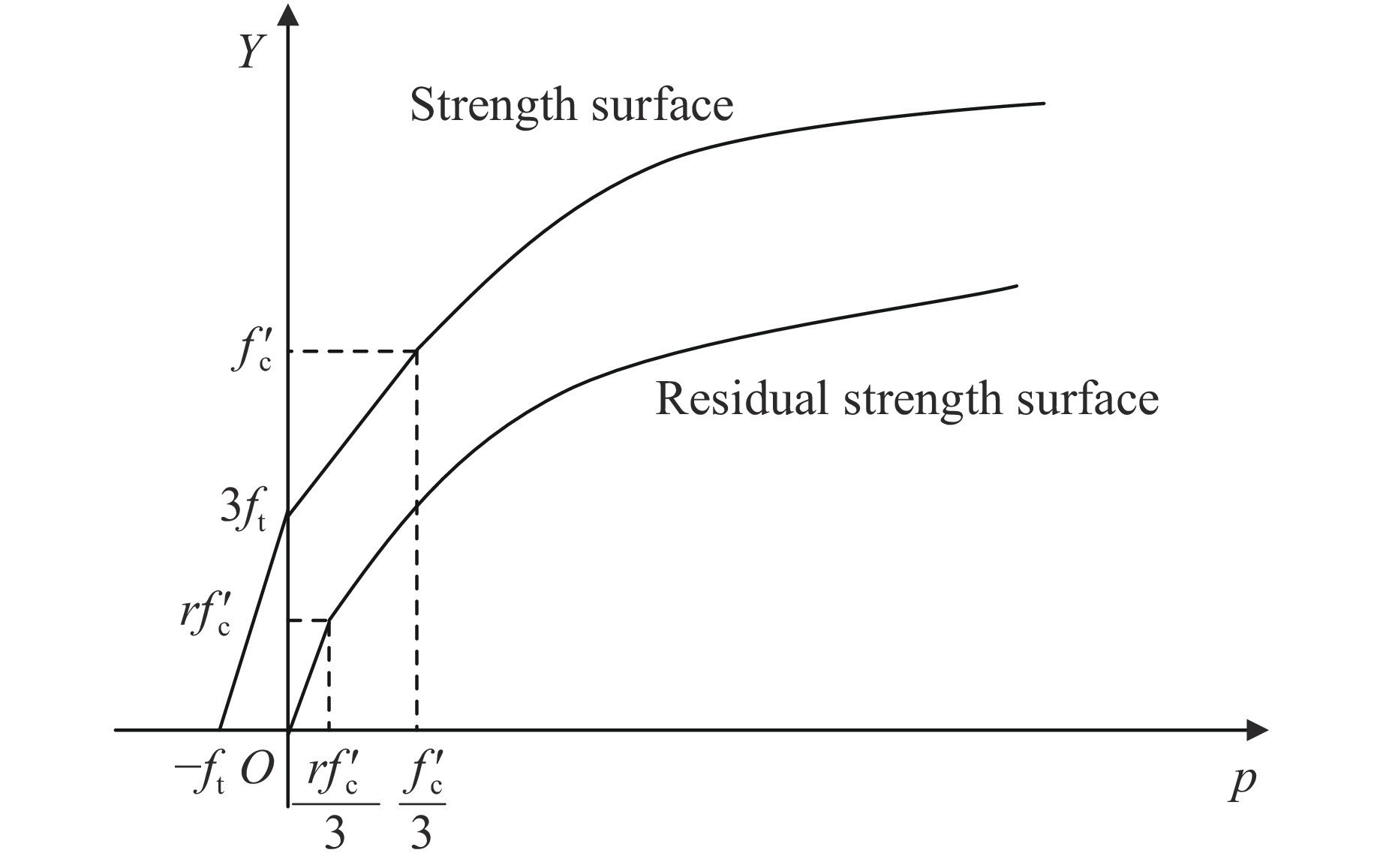
The strength model takes into account various effects, such as pressure hardening, damage softening, third stress invariant (Lode angle) and strain rate. The strength surface Y, shown schematically in Fig.2, can be written as[7]
Y={3(p+ftt)R(θ,e) p<0[3ftt + 3p(fcc−3ftt)/fcc]R(θ,e) 0⩽p⩽fcc/3{fcc+Bfc′[p/fc′−fcc/(3fc′)]N}R(θ,e) p>fcc/3 (6) where p is the hydro-static pressure, parameters B and N are constants, R(θ,e) is a function of the Lode angle θ and the tensile-to-compressive meridian ratio e,
fc′ is the static uni-axial compressive strength, the compressive strengthfcc and the tensile strengthftt are defined byfcc=fc′Dm_tηc (7) ftt=ftDtηt (8) where
ft is the static uni-axial tensile strength.Dm_t is the compression dynamic increase factor due to strain rate effect only, and can be expressed as[7, 16]Dm_t=(Dt−1)ft/fc′+1 (9) where
Dt is the tension dynamic increase factor determined byDt={tanh[(lg˙ε˙ε0−Wx)S][FmWy−1]+1}Wy (10) where
Fm ,Wx ,Wy andS are experimental constants,˙ε is the strain rate, and˙ε0 is the reference strain rate, usually taken˙ε0=1.0 s−1 .ηc is the damage function for compression, which can be expressed asηc={l+(1−l)η(λ)λ⩽λmr+(1−r)η(λ)λ>λm (11) where l and r are constants[7],
λm is the value of shear damage (λ ) when strength reaches its maximum value under compression.η(λ) is defined asη(λ)=aλ(λ−1)exp(−bλ) (12) in which a and b can be determined by setting
η(λ) = 1 and∂η∂λ=0 whenλ =λ m.ηt is the damage function for tension which can be written asηt=[1+(c1εtεfrac)3]exp(−c2εtεfrac)−εtεfrac(1+c31)exp(−c2) (13) where c1 and c2 are constants[7],
εt denotes the tensile strain andεfrac is the fracture strain.The residual strength (
rfc′ ) surface for rocks, shown schematically in Fig.2, can be obtained from Eq.(6) by settingftt=0 andfcc=rfc′ , vizY={3pR(θ,e) 0<p⩽rfc′/3{rfc′+Bfc′[p/fc′−rfc′/(3fc′)]N}R(θ,e) p>rfc′/3 (14) 2. Numerical Simulations
Granite is selected for investigating the dynamic fractures which result from borehole blast loading.
2.1 Evaluation of Various Parameters in the Model
Table 1 lists the values of the various parameters used in the dynamic constitutive model for granite. As to how to determine the values of the various parameters in the model, more details are presented in [7, 15-17].
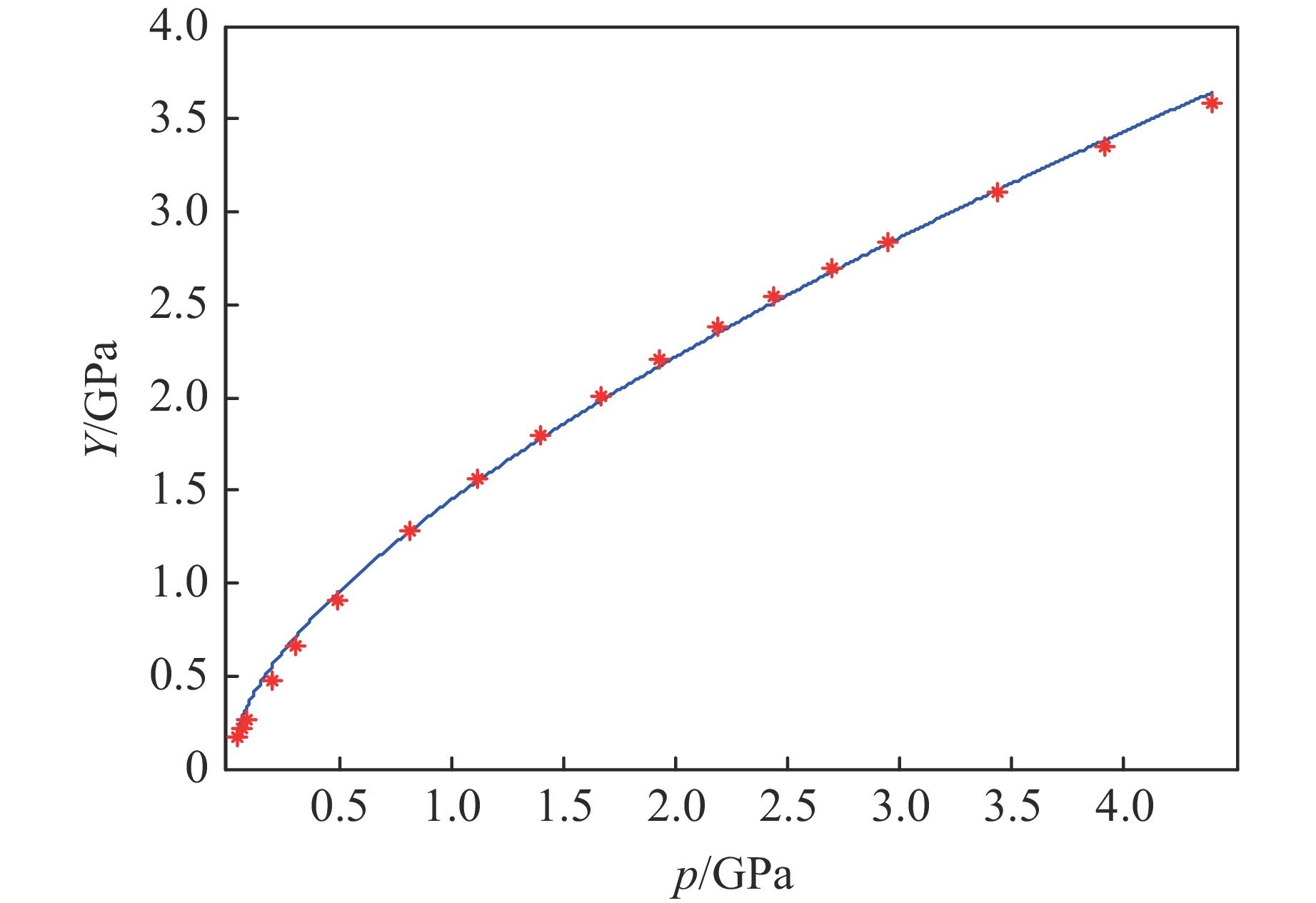
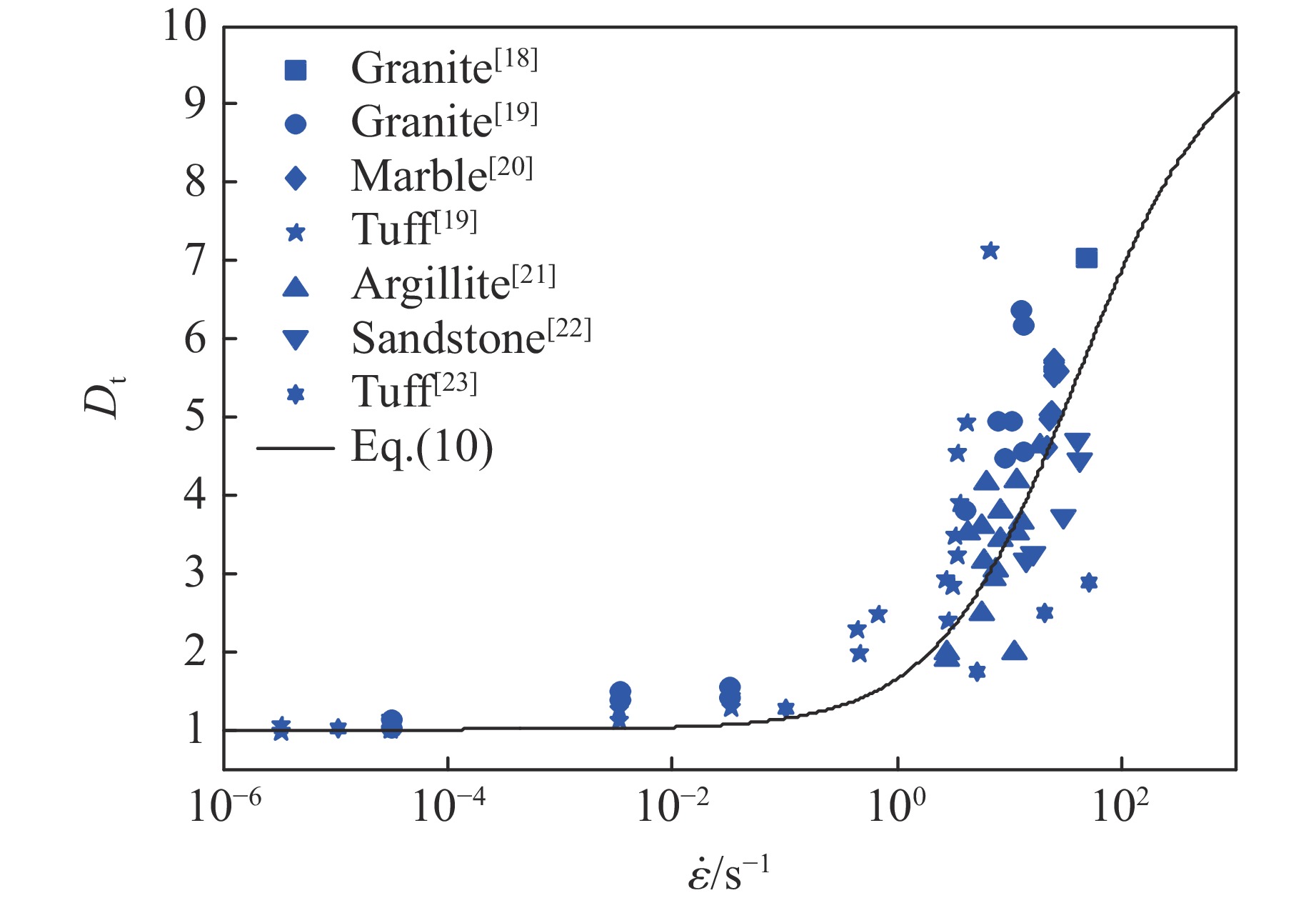
p-α relation ρ0/(kg∙m−3) pcrush/MPa plock/GPa n K1/GPa K2/TPa K3/TPa 2660 50.5 3 3 25.7 −3 150 Strength surface Strain rate effect fc′/MPa ft/MPa B N G/GPa Fm Wx 161.5 7.3 2.59 0.66 21.9 10 1.6 Strain rate effect Shear damage Wy S ˙ε0/s−1 λs λm l r 5.5 0.8 1.0 4.6 0.3 0.45 0.3 Lode effect Tensile damage e1 e2 e3 c1 c2 εfrac 0.65 0.01 5 3 6.93 0.007 Fig.3 shows the comparison of the strength surface between Eq.(6) (with B=2.59, N=0.66) and the triaxial test data for granite[17]. It can be seen from Fig.3 that a good agreement is obtained. Similarly, Fig.4 shows the tensile strengths/dynamic increase factor obtained by Eq.(10) and the test results of various rocks at different strain rates[18-23]. It is clear from Fig.4 that a good agreement is achieved.
 Figure 3. Comparison of the strength surface between Eq.(6) (with B=2.59, N=0.66) and the triaxial test data for granite[17]
Figure 3. Comparison of the strength surface between Eq.(6) (with B=2.59, N=0.66) and the triaxial test data for granite[17]2.2 Numerical Results
In the following, numerical simulations are carried out for the response of the granite targets subjected to borehole blasting loading. The dynamic fracture behavior of two kinds of granite samples are studied, namely, cylindrical sample as reported in the literature and square sample as examined in our own laboratory.
2.2.1 Cylindrical Rock Sample
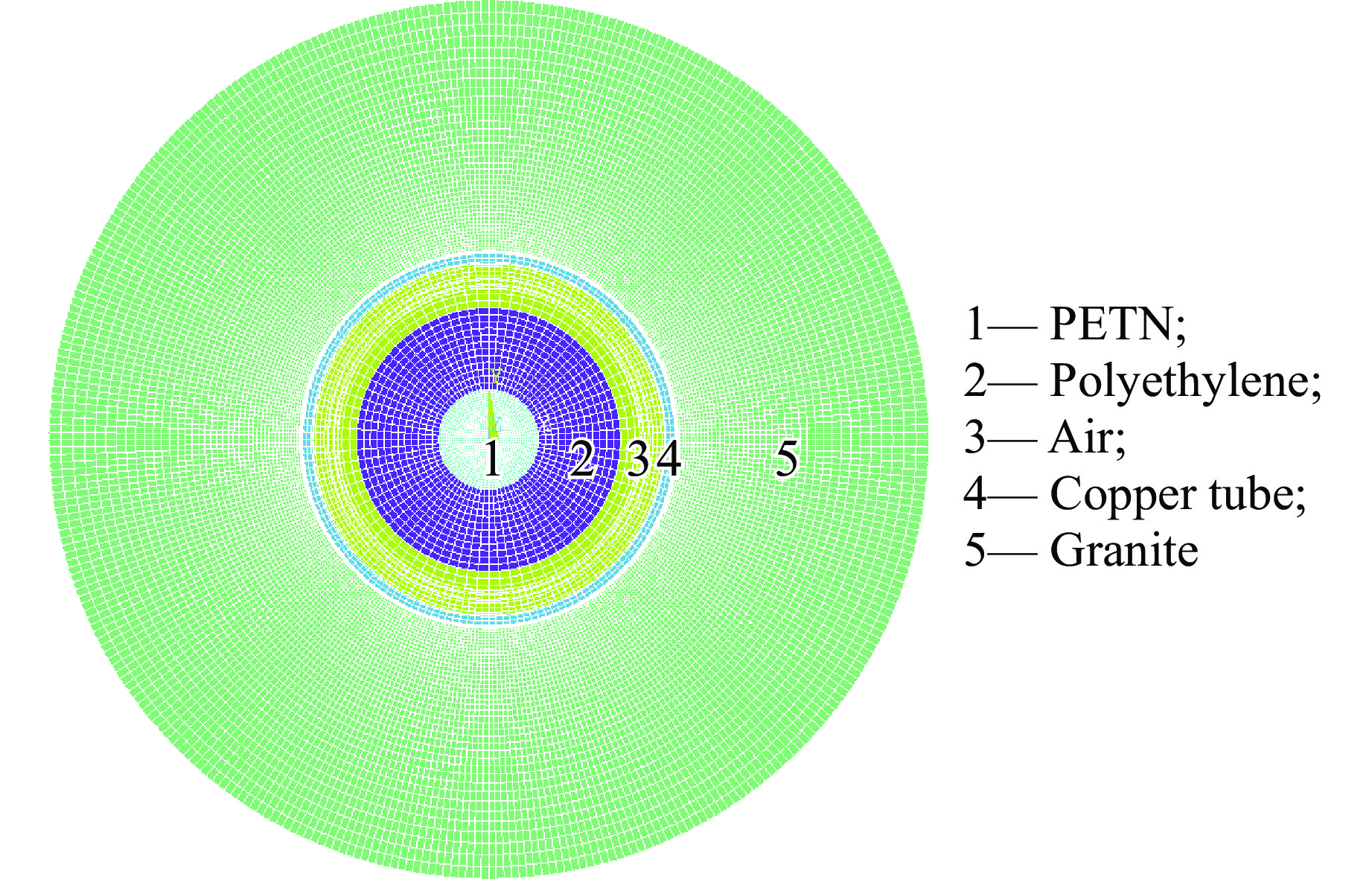
In consideration of the sizes of the cylindrical granite samples prepared for laboratory-scale blasting experiments by Dehghan Banadaki and Mohanty[17] (with a diameter of 144 mm, a height of 150 mm and a borehole diameter of 6.45 mm), a circular plane strain model with an outer diameter of 144 mm is made in our simulation, as shown in Fig.5, being a scaled close-up view of the borehole region. Multi-material Euler solver is used for modeling PETN explosive, polyethylene and air. Lagrangian descriptions are used for modeling the copper tube and granite.
The material model and the properties of PETN explosive, polyethylene, air and copper tube used in the simulation are given in Ref.[17]. The values of various parameters in the constitutive model for granite are listed in Table 1.
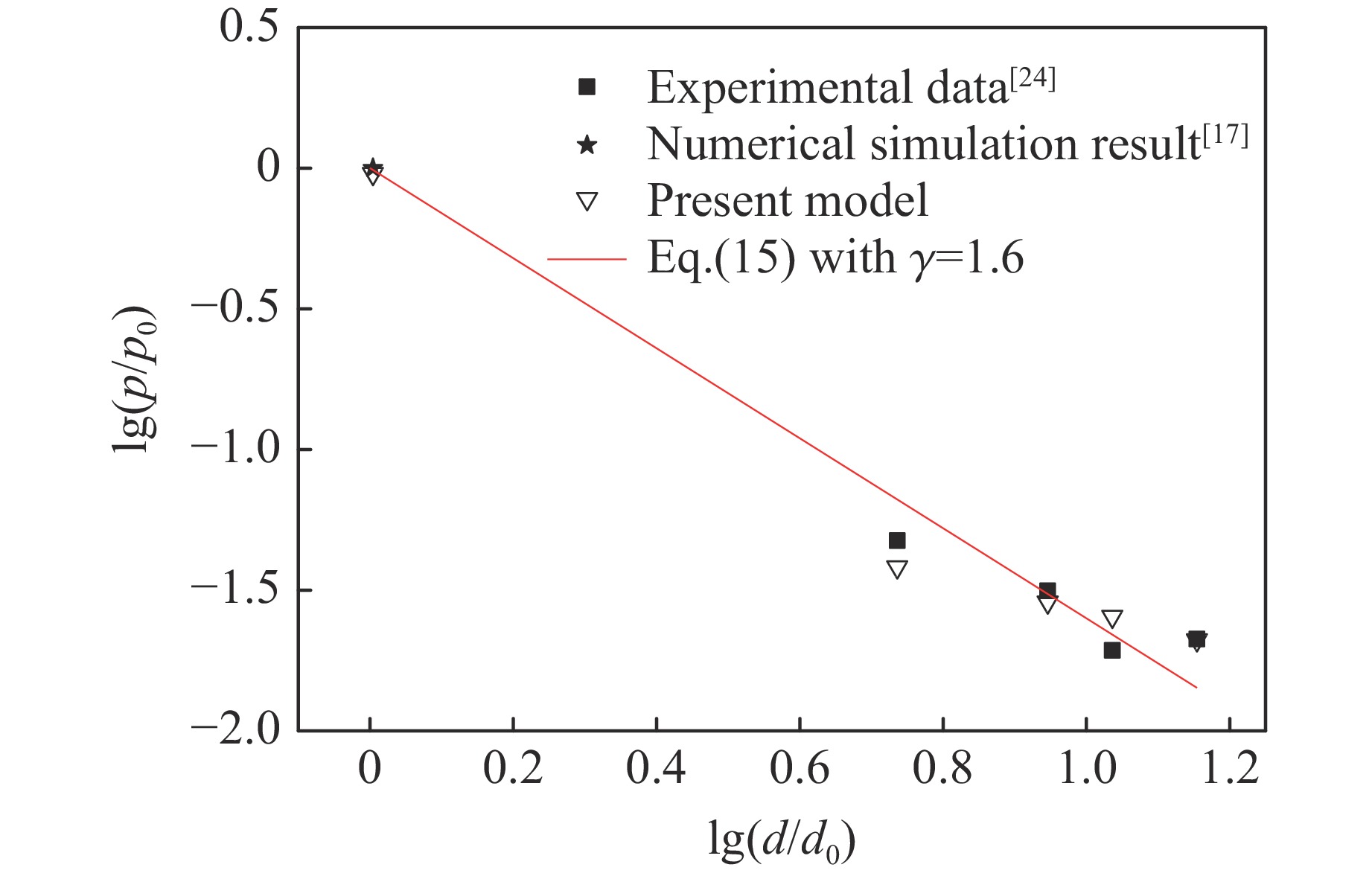
Fig.6 shows the comparison of the peak pressures between our simulation results of the present model, the numerical results[17], and the experimental results by Dehghan Banadaki and Mohanty[24]. It can be seen from Fig.6 that a good agreement is obtained.
In order to characterize the damping behavior of stress in granite, the peak pressure p in granite is expressed in an exponential form as
pp0=(dd0)−γ (15) where p0 is the peak pressure on the borehole wall, d0 is the initial radius of the borehole, d is the distance from the center point of the borehole,
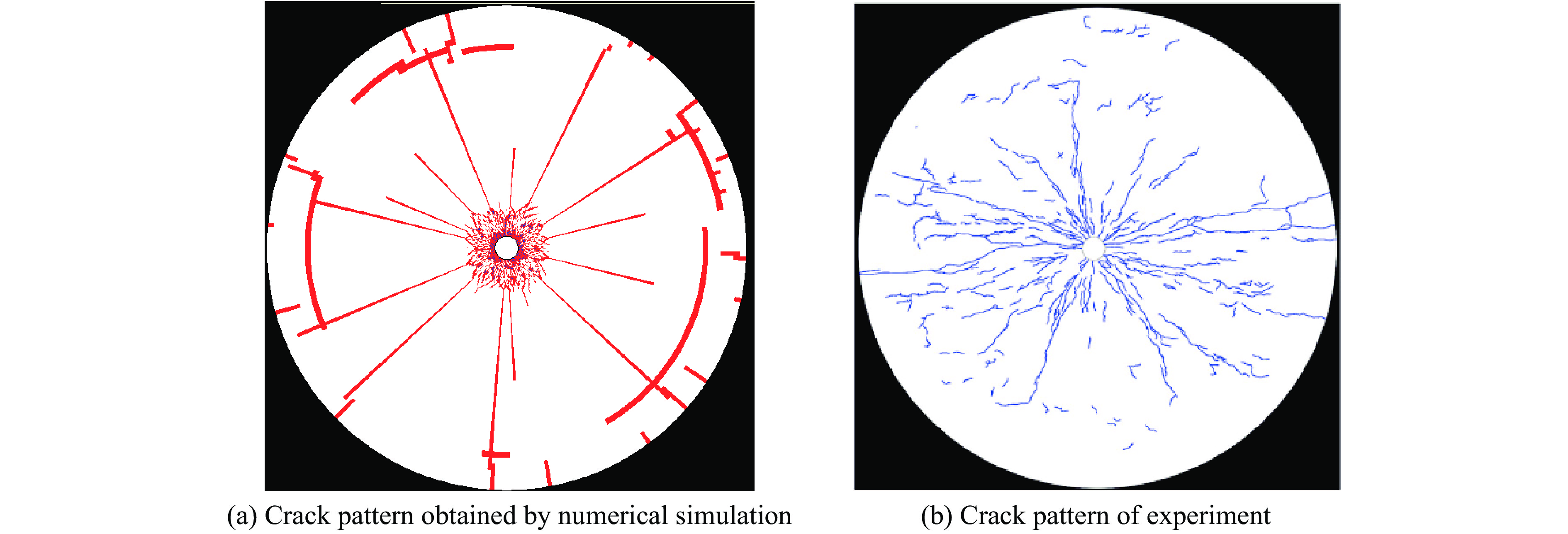
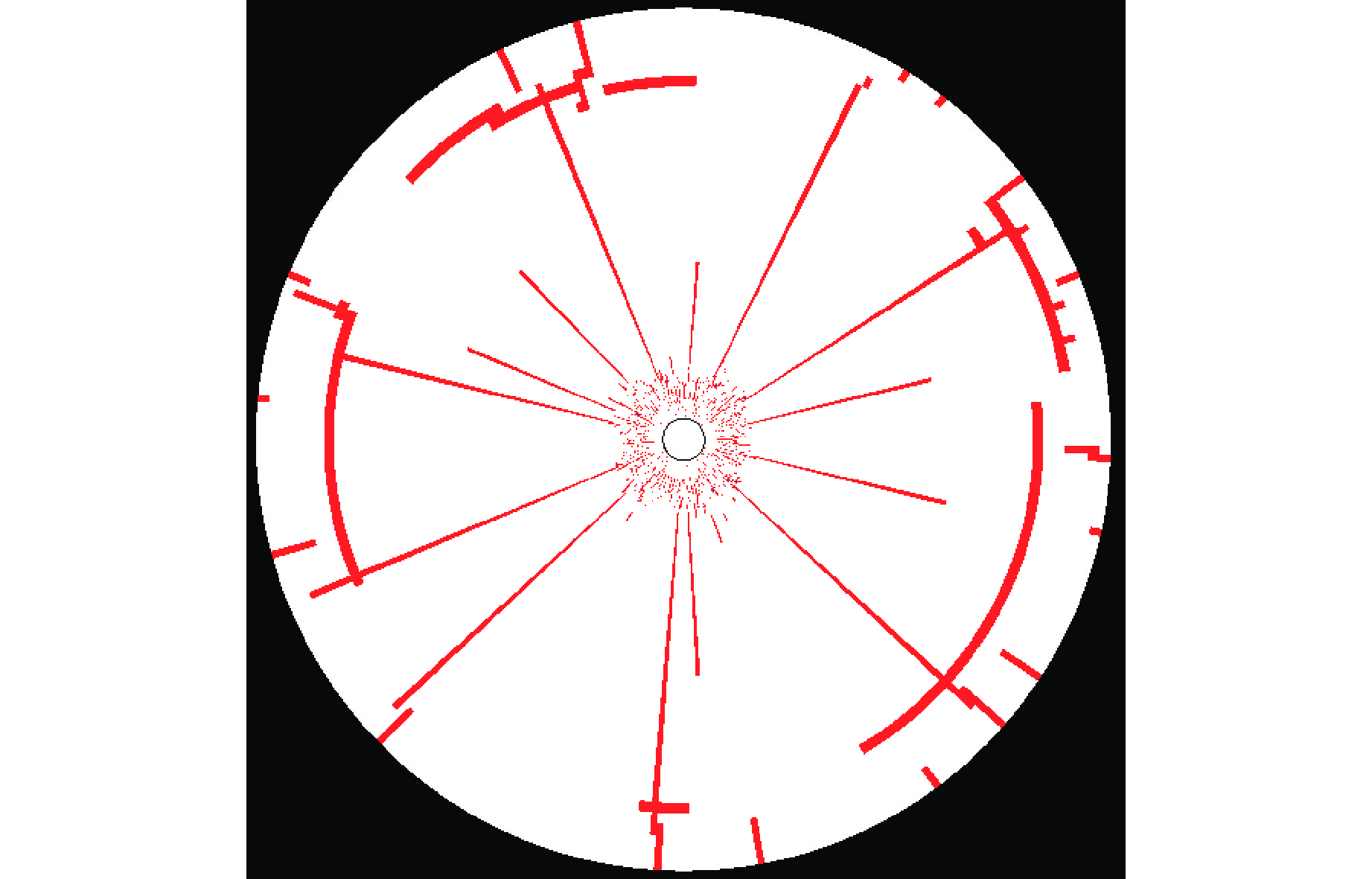
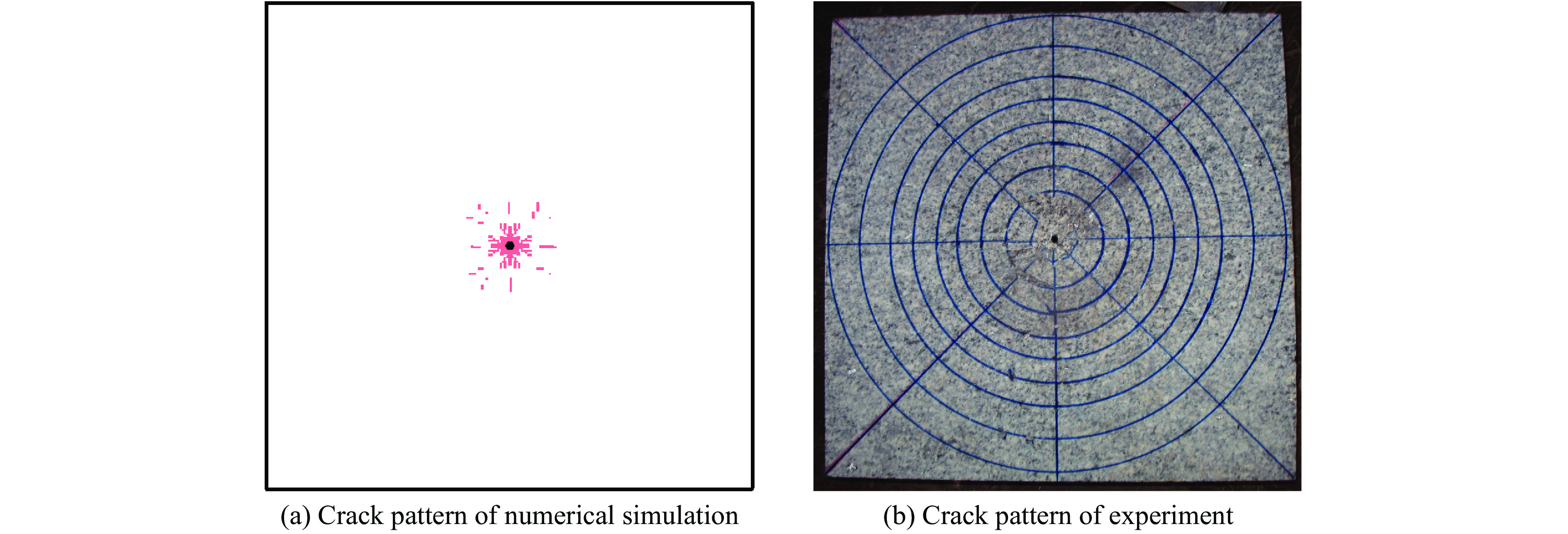
γ is an index. It is evident from Fig.6 that Eq.(15) withγ =1.6 correlates well with the experimental results.Fig.7 shows the comparison between the crack patterns predicted numerically based on the present model and the one observed experimentally in the cylindrical granite sample[17]. It is clear that a relatively good agreement on the crack pattern is obtained. It is also clear that the stress waves produce three distinct crack regions in the cylindrical granite sample: densely populated smaller cracks around the borehole, a few large radial cracks propagating towards the outer boundary, and circumferential cracks close to the sample boundary which are due to the reflected tension stress.
 Figure 7. Comparison of the crack patterns between the numerical prediction and the experiment of the cylindrical granite sample[17]
Figure 7. Comparison of the crack patterns between the numerical prediction and the experiment of the cylindrical granite sample[17]In order to make an assessment of the contributions of both the compression/shear stress and the tensile stress to the crack patterns, Fig.8 shows the numerically predicted crack pattern which results from the tension stress only. Fom Fig.8 and Fig.7(a), it is apparent that there are virtually few changes in crack patterns, both having the large radial and circumferential cracks caused by the same tensile stress, however, the smaller cracks around the borehole in Fig.8 are much less than those in Fig.7(a). In another word, crack patterns are mainly caused by tensile stress, and smaller cracks around borehole are created largely by compression/shear stress.
2.2.2 Square Granite Sample
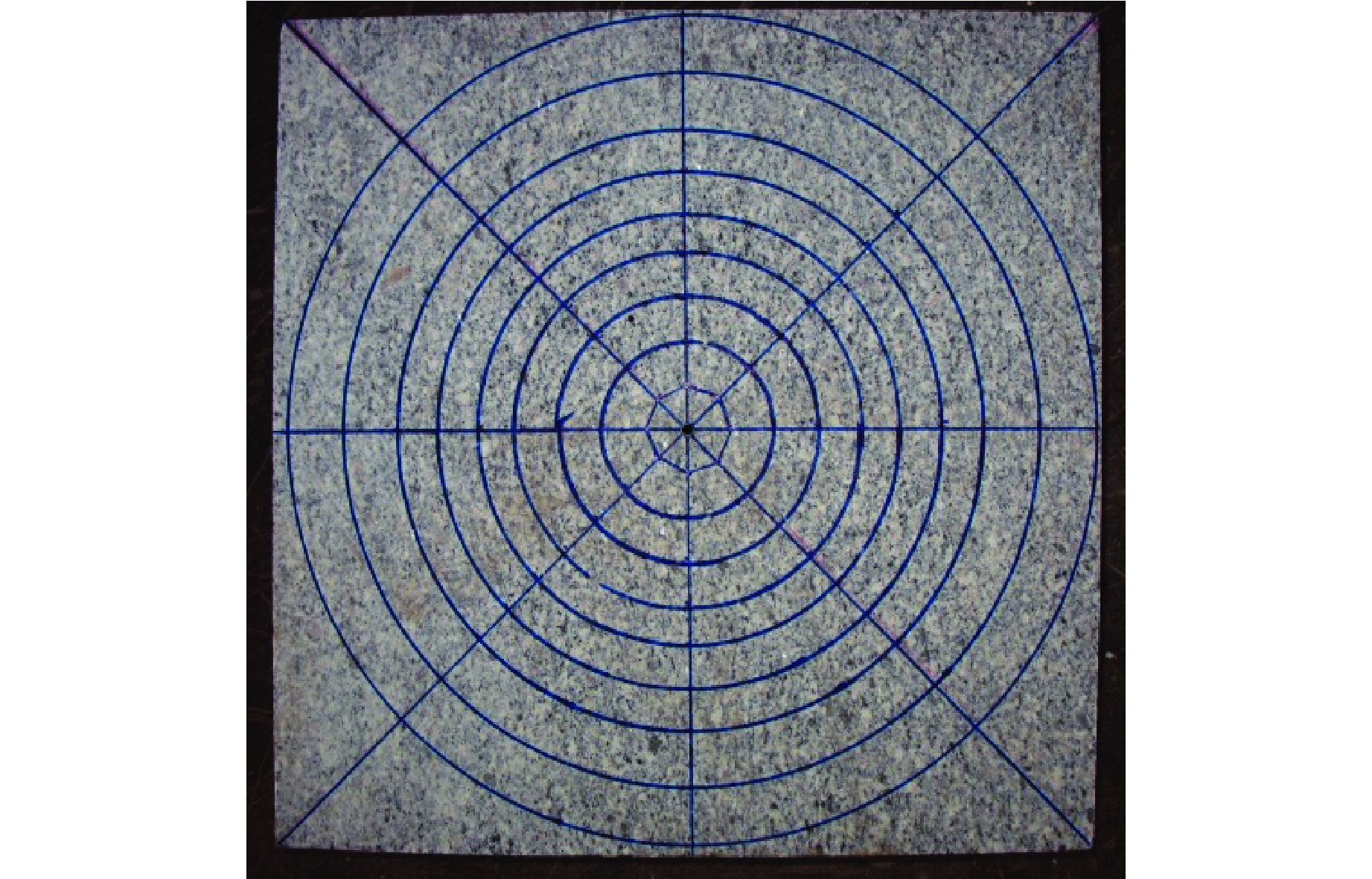
The laboratory-scale single-hole blasting tests are also carried out in order to validate further the accuracy and the reliability of the present model. Two square granite samples with a side length of 400 mm and a height of 100 mm are employed in the experiments. The borehole diameter is 4 mm and a series of concentric rings is drawn on the top surface of the samples (see Fig.9), so that the damage regions induced by detonation can be assessed visually.
A cylindrical RDX explosive enclosed by an aluminum sheath (see Fig.10) is tightly installed in the borehole of the No.1 sample, while an unwrapped RDX explosive is inserted into the borehole of the No.2 sample. The density of the RDX is 1700 kg/m3, and the material model and properties of the RDX explosive and the aluminum sheath used in the experiments are given in ref.[25]. The values of the various parameters in the constitutive model for granite are listed in Table 1.
Fig.11 and Fig.12 show the comparisons of the crack patterns between the numerical predictions from the present model and the ones observed experimentally in the square granite samples. It can be seen from Fig.11 and Fig.12 that good agreements are obtained. It should be mentioned here that No.1 sample receives less damage due to less RDX explosive used in the test, and that No.2 sample is broken up into four major pieces due to more RDX explosive employed in the experiment. Severe damages and small cracks are induced in the vicinity of the boreholes of both samples, as can be seen clearly from Fig.11(b) and Fig.12(b).
3. Conclusions
A numerical study on the borehole blasting-induced fractures in rocks is conducted in this paper, using a dynamic constitutive model developed previously for concrete. Two kinds of granite rocks are simulated numerically, one in the cylindrical form and the other in the square form. The numerical results are compared with the corresponding experiments. Main conclusions can be drawn as follows.
(1) The crack patterns predicted numerically from the present model are found to be in good agreement with the experimental observations, both in cylindrical and square granite samples subjected to borehole blasting loading.
(2) The peak pressures predicted numerically based on the present model are found to be in good agreement with the test data.
(3) Crack pattern observed experimentally in the rock sample is mainly caused by the tensile stress, while the smaller cracks in the vicinity of the borehole are created largely by compression/shear stress.
(4) The consistency between the numerical results and the experimental observations demonstrates the accuracy and reliability of the present model. Thus the model can be used in the numerical simulations of the response and the failure of rocks under blasting loading.
-
表 1 定向排列状态下片状增强纳米复合材料增强因子
Table 1. Enhancement factors of flake reinforced nanocomposites
Material parameter ζ G12 3LG/4TG G13,G23 LG/TG 表 2 环氧树脂及石墨烯的材料参数
Table 2. Material parameters of epoxy resins and GPLs
表 3 不同约束下石墨烯纳米复合材料梁的一阶屈曲荷载
Table 3. The first-order critical buckling load of graphene-reinforced nanocomposite beams with different boundary conditions
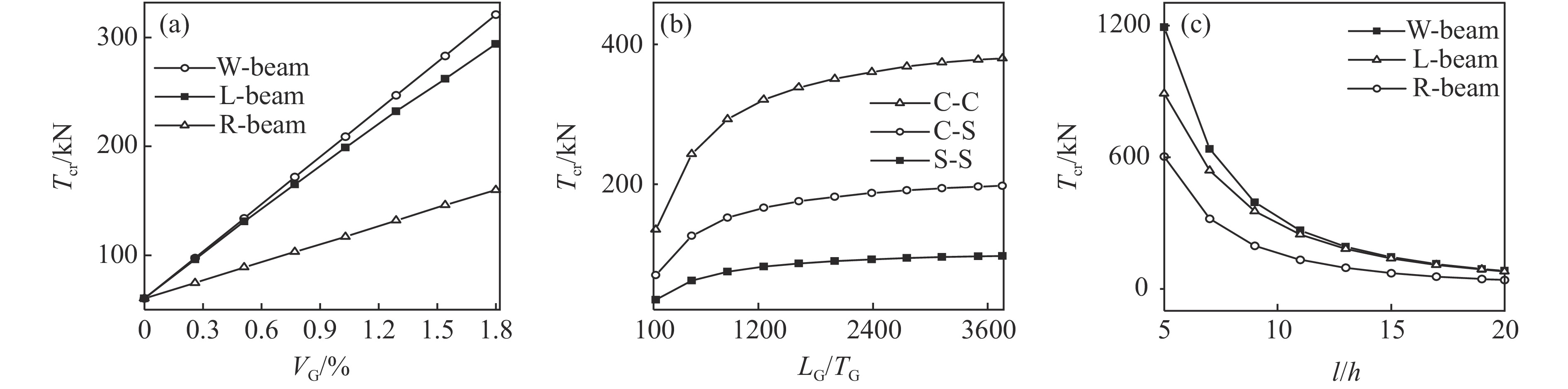
Boundary condition R-beam L-beam W-beam Euler beam This work Euler beam This work Euler beam This work S-S 40.93 40.76 82.16 79.87 82.16 81.72 C-C 163.71 160.36 328.65 293.84 328.65 320.72 C-S 83.53 82.98 167.68 158.00 167.68 166.23 -
[1] SASHA S, DIKIN D A, DOMMETT G H B, et al. Graphene-based composite materials [J]. Nature, 1990, 442(2): 282–283. [2] RAHMAN R, HAQUE A. Molecular modeling of crosslinked graphene-epoxy nanocomposites for characterization of elastic constants and interfacial properties [J]. Composites Part B: Engineering, 2013, 54(9): 353–364. [3] JI X Y, CAO Y P, FENG X Q. Micromechanics prediction of the effective elastic moduli of graphene sheet-reinforced polymer nanocomposites [J]. Modelling & Simulation in Materialsence & Engineering, 2010, 18(4): 1–16. [4] KING J A, KLIMEK D R, MISKIOGLU I, et al. Mechanical properties of graphene nanoplatelet/epoxy composites [J]. Journal of Applied Polymer Science, 2013, 128(6): 4217–4223. doi: 10.1002/app.v128.6 [5] ZHAO X, ZHANG Q, CHEN D, et al. Enhanced mechanical properties of graphene-based poly (vinyl alcohol) composites [J]. Macromolecules, 2010, 43(5): 2357–2363. doi: 10.1021/ma902862u [6] RAFIEE M, RAFIEE J, YU Z Z, et al. Buckling resistant graphene nanocomposites [J]. Applied Physics Letters, 2009, 95(22): 10–18. [7] NGUYEN T K, VO T P, THAI H T. Static and free vibration of axially loaded functionally graded beams based on the first-order shear deformation theory [J]. Composites Part B: Engineering, 2013, 55(55): 147–157. [8] PARASHAR A, MERTINY P. Representative volume element to estimate buckling behavior of graphene/polymer nanocomposite [J]. Nanoscale Research Letters, 2012, 7(1): 515–518. doi: 10.1186/1556-276X-7-515 [9] FENG C, KITIPORNCHAI S, YANG J. Nonlinear bending of polymer nanocomposite beams reinforced with non-uniformly distributed graphene platelets (GPLs) [J]. Composites Part B: Engineering, 2017, 110(1): 132–140. [10] YANG J, WU H, KITIPORNCHAI S. Buckling and postbuckling of functionally graded multilayer graphene platelet-reinforced composite beams [J]. Composite Structures, 2017, 161(1): 111–118. [11] LIAN G, TUAN C C, LI L, et al. Vertically aligned and interconnected graphene networks for high thermal conductivity of epoxy composites with ultralow loading [J]. Chemistry of Materials, 2016, 28(17): 6096–6104. doi: 10.1021/acs.chemmater.6b01595 [12] WU S, LADANI R B, JIN Z, et al. Aligning multilayer graphene flakes with an external electric field to improve multifunctional properties of epoxy nanocomposites [J]. Carbon, 2015, 94(1): 607–618. [13] ZHANG Y F, HAN D, ZHAO Y H, et al. High-performance thermal interface materials consisting of vertically aligned graphene film and polymer [J]. Carbon, 2016, 109(1): 552–557. [14] 韩依廷. 定向排列石墨烯/聚合物复合材料研究进展 [J]. 石化技术, 2017, 24(12): 207–208. doi: 10.3969/j.issn.1006-0235.2017.12.167HAN Y T. Research progress of directional arrangement of graphene/polymer composites [J]. Petrochemical Industry Technology, 2017, 24(12): 207–208. doi: 10.3969/j.issn.1006-0235.2017.12.167 [15] AFFDL J C H, KARDOS J L. The Halpin-Tsai equations: a review [J]. Polymer Engineering & Science, 1976, 16(5): 344–352. [16] WANG F, LIAO X, XIONG X. Characteristics of the differential quadrature method and its improvement [J]. Mathematical Problems in Engineering, 2015(6): 1–9. [17] CHOUDHURY A. Preparation and characterization of nanocomposites of poly-p-phenylene benzobisthiazole with graphene nanosheets [J]. Rsc Advances, 2014, 4(17): 8865–8870. [18] VAN ES M. Polymer-clay nanocomposites: the importance of particle dimensions [D]. Delft, Netherlands: Delft University of Technology, 2001: 18–37. [19] BERT C W. Differential quadrature and its application in engineering, by Chang Shu, Springer, London, 2000 [J]. International Journal of Robust & Nonlinear Control, 2010, 11(14): 1398–1399. [20] LIU X, METCALF T H, ROBINSON J T, et al. Shear modulus of monolayer graphene prepared by chemical vapor deposition [J]. Nano Letters, 2012, 12(2): 1013–1017. doi: 10.1021/nl204196v [21] YASMIN A, DANIEL I M. Mechanical and thermal properties of graphite platelet/epoxy composites [J]. Polymer, 2004, 45(24): 8211–8219. doi: 10.1016/j.polymer.2004.09.054 [22] RAFIEE M A, JAVAD R, ZHOU W, et al. Enhanced mechanical properties of nanocomposites at low graphene content [J]. Acs Nano, 2009, 3(12): 3884–3890. doi: 10.1021/nn9010472 [23] XIAO L, METCALF T H, ROBINSON J T, et al. Internal friction and shear modulus of graphene films [J]. Solid State Phenomena, 2012, 184(1): 319–324. [24] LIANG J, YI H, LONG Z, et al. Molecular-level dispersion of graphene into poly (vinyl alcohol) and effective reinforcement of their nanocomposites [J]. Advanced Functional Materials, 2010, 19(14): 2297–2302. -









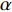
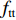
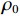
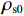
 DownLoad:
DownLoad:
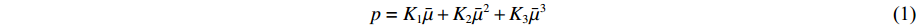
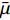


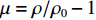
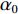
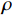
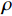
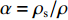
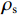
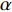
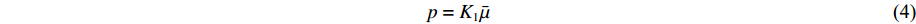
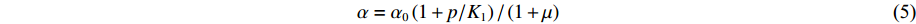

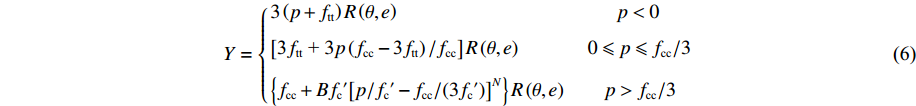
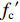
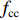


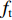
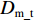
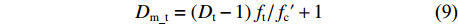
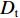
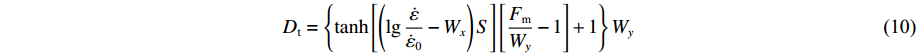
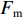
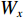
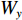

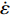
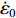
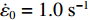
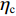
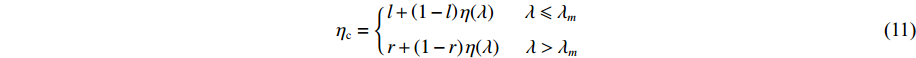
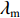
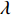
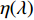
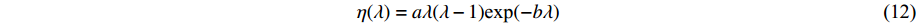
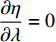
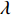
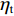
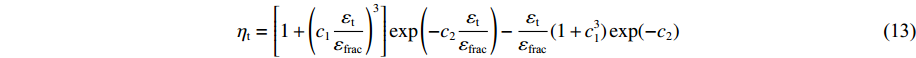
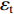
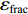
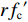
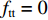
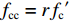
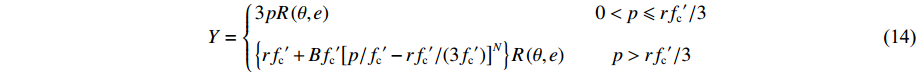
 DownLoad:
DownLoad:



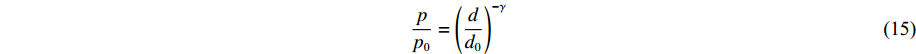
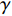







 下载:
下载: