Diffusion of Helium in Calcite and Aragonite:A First-Principles Study
doi: 10.11858/gywlxb.20180698
-
Abstract: Helium diffusion in carbonate minerals is important for studying the physical and chemical properties and dynamic processes of Earth’s degassing. This paper discussed helium incorporation and diffusion mechanism in crystals of calcite and aragonite based on density functional theory calculations. The diffusion pathways, activation energies (Ea), and frequency factors (v) of helium under the surface and mantle condition were calculated. Calculations show an apperant anisotropy of helium diffusion in calcite, with more energetically favorable directions along a(b) axis. The moderate anisotropy of helium diffusion is showed in aragonite, in which the diffusion rate along c axis is slower than that along a axis. Under high pressure conditions, the activation energies of helium diffusion in aragonite increase with pressure. The closure temperature for calcite crystal varies from −54 ℃ to −25 ℃ in the direction [010], and for aragonite varies from −12 ℃ to 23 ℃ in [100]. Aragonite may be more retentive for helium than calcite under surface condition, which agrees well with previous experimental studies.
-
Key words:
- helium diffusion /
- calcite /
- aragonite /
- ab initio /
- pressure effect
摘要: 研究碳酸盐矿物中氦的扩散行为对理解地球脱气过程中的物理化学性质和动力过程具有重要意义。基于密度泛函理论研究了氦在方解石和文石矿物中的扩散机理,计算了氦在地表和地幔条件下的扩散路径、激活能(Ea)和频率因子(v)。计算结果表明:氦在方解石中的扩散具有明显的各向异性,沿a(b)轴方向的扩散更快;文石呈现中等的各向异性,沿c轴的扩散速率低于a轴。在高压条件下,文石的激活能随压力的增大而增大。方解石晶体在[010]方向的封闭温度为–54~–25 ℃,沿[100]方向的封闭温度为 –12~23 ℃。在地表条件下,氦在文石中的滞留能力比在方解石中强,与以往的实验研究结果一致。 -
软质高分子聚合物材料因生产成本低、质量轻、具有良好的抗冲击性能等优点在军工、汽车、航空、电子[1-4]等行业得到广泛应用,如用于汽车碰撞实验的假人皮肤材料[5]、航空航天仿生材料[6]等。在上述领域,构件/材料常受动态冲击载荷作用,明确其动态力学性能,构建描述其黏弹性力学特征的本构模型,可为软质高分子聚合物材料的设计开发、性能优化及可靠性分析提供理论模型和方法支撑。
学者们曾利用分离式霍普金森压杆(Split Hopkinson pressure bar, SHPB)装置对PVC弹性体等软质高分子聚合物材料开展了动态压缩力学性能研究[7-8]。与SHPB相比,分离式霍普金森拉杆(Split Hopkinson tensile bar, SHTB)装置采用拉伸加载形式,便于开展材料的动态冲击损伤、变形、断裂等行为研究。近年来,研究人员已对丁氰橡胶[9]、聚脲[10]、半晶聚合物高密度聚乙烯(HDPE)[11]、猪皮[12]、聚氨酯弹性体[13]等低阻抗软材料进行了静、动态拉伸力学性能测试。同时,自动网格法应变测量[14]、脉冲整形[15]等技术以及高/低温环境箱[16]、扫描电镜[17-18]、高速摄像机[19]等装置也被用于软材料的拉伸变形、裂纹萌生及扩展机理分析。通过静、动态拉伸实验发现:软质高分子聚合物材料的拉伸强度会随着应变率对数的增大而线性增加,且不同分子链结构的软质高分子聚合物在同一高拉伸应变率下有不同的拉伸强度和拉伸应变[20]。
但是,软质高分子聚合物材料动态拉伸实验过程中仍存在试样连接方式难以确定、胶黏剂黏接强度低、加载应变率不恒定等问题。为揭示PVC弹性体材料的静、动态拉伸力学性能,利用Instron-5943万能材料试验机和改进型SHTB实验装置对PVC弹性体进行静、动态直接拉伸实验,分析应变率对PVC弹性体材料静、动态拉伸力学性能的影响规律。
1. 实验材料及测试方法
1.1 准静态拉伸实验
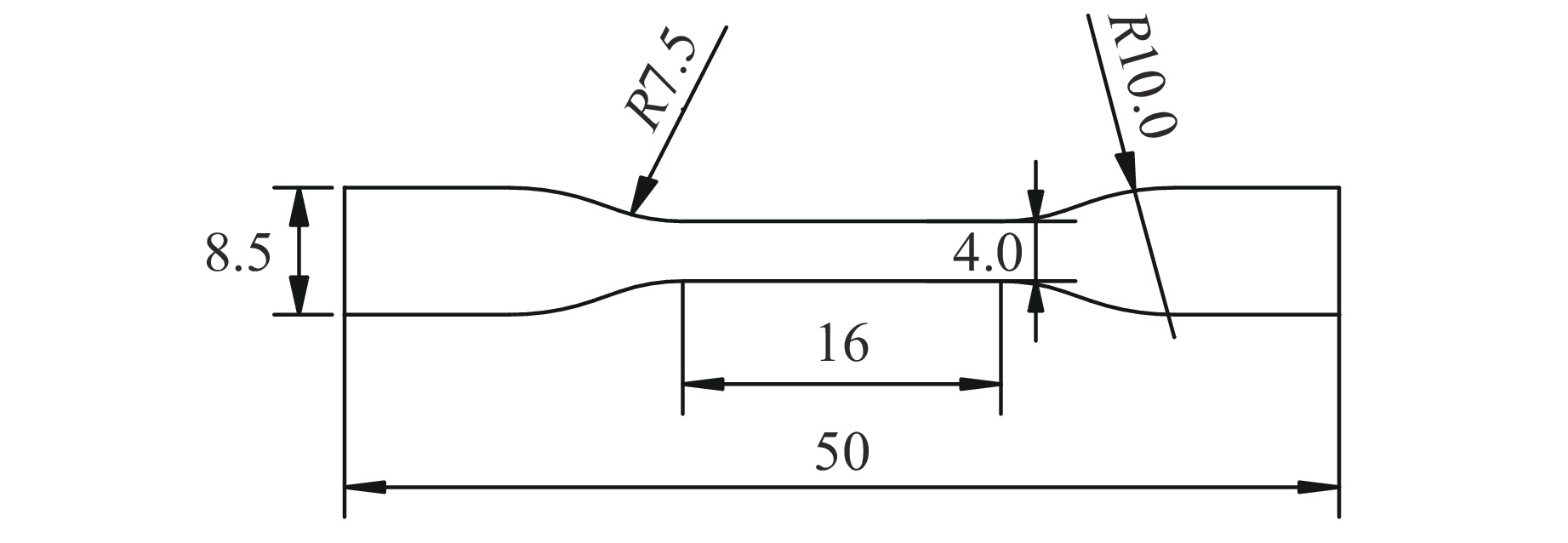
实验材料为PVC弹性体。准静态拉伸试样根据GB/T 528–2009[21]中的3型试样标准设计由模具冲压而成,具体尺寸见图1,试样呈哑铃状,厚度为2 mm。由于弹性体材料动态拉伸实验试样的设计尚无统一标准,因此本研究中动态拉伸实验试样将依据动态拉伸实验的具体效果进行设计并制备,详见2.3.1节。
PVC弹性体的准静态拉伸力学性能实验是在Instron-5943万能材料试验机上进行的。实验时,将试验机的加载速率设置为96 mm/min,使其应变率为0.1 s−1。拉伸过程中的载荷(F)和位移(ΔL)数据通过传感器输入至计算机中,利用式(1)、式(2)将其转化为应力、应变数据
σ=FAs (1) ε=ΔLLs (2) 式中:
σ 为应力,As为试样截面积,ε 为应变,Ls为试样长度。1.2 动态拉伸实验
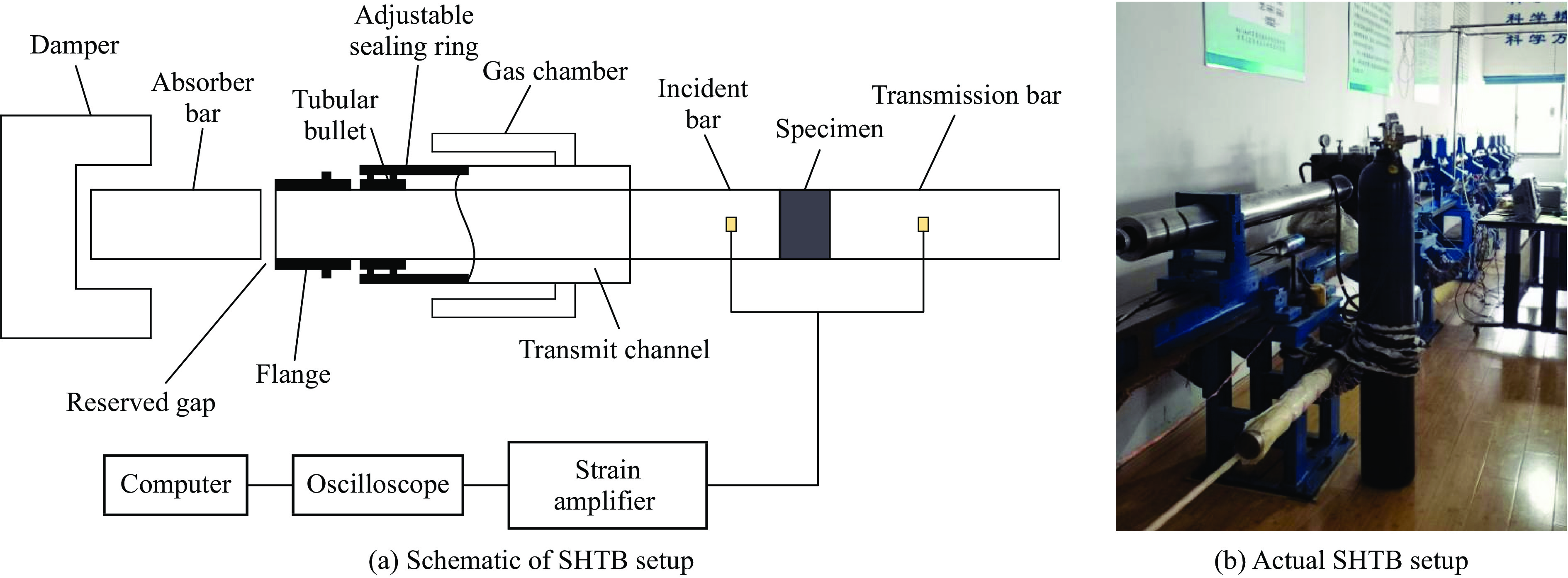
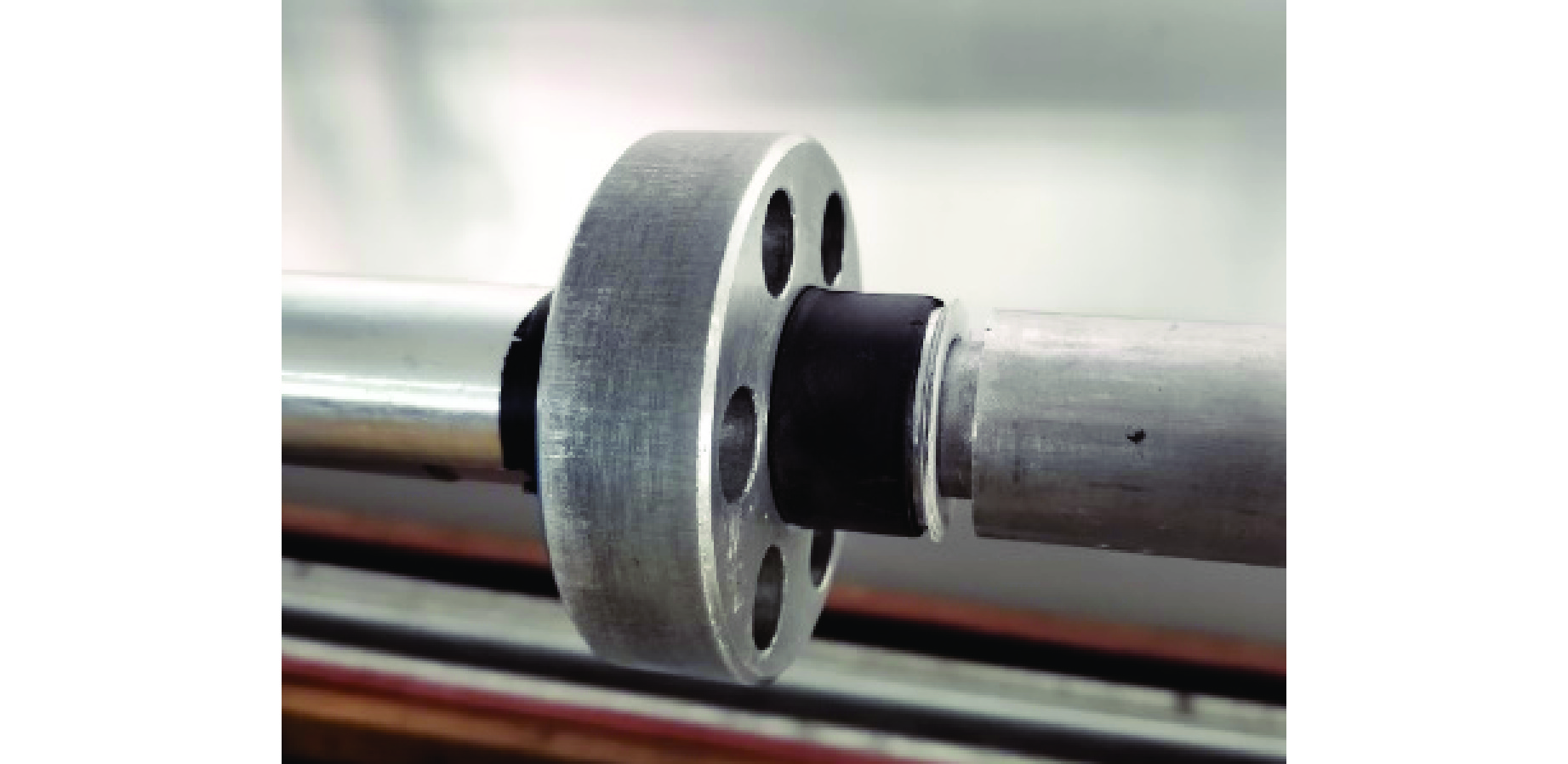
采用合肥姜水动态力学实验技术有限公司第一实验室的分离式霍普金森拉杆(SHTB)实验系统开展聚氯乙烯(PVC)弹性体材料在高应变率下的动态拉伸实验,其装置原理及实物见图2。
调节储气瓶阀向储气室充气,待储气室内气压到达预定值时,关闭储气瓶阀,触发发射按钮,使储气室内高压气体流入发射通道,推动管状套筒子弹在入射杆上加速至一定速度,并以该速度撞击入射杆顶端的法兰盘,在入射杆中产生一个压缩应力波,其持续时间由子弹长度决定。套筒子弹与法兰盘撞击瞬间形成的压缩应力波分为两部分:一部分压缩应力波被吸收杆捕获,最终被能量吸收器(阻尼器)吸收;另一部分压缩应力波在入射杆自由端面处反射形成入射杆中的拉伸应力波。当该波传递至试样端面时,由于杆系与试样间的波阻抗不匹配,一部分拉伸应力波会被反射回入射杆形成反射波,另一部分则会穿过试样并传递至透射杆形成透射波。
根据一维弹性应力波的传播理论,由入射杆和透射杆上的半导体应变片分别获取入射波
εi(t) 、反射波εr(t) 和透射波εt(t) 的应变脉冲信号,可计算出试样两端面的力F1 、F2 及位移u1 、u2 F1=E0A0[εi(t)+εr(t)] (3) F2=E0A0εt(t) (4) u1=C0∫[εi(t)−εr(t)]dt (5) u2=C0∫εt(t)dt (6) 当试样内部满足应力均匀性假设[22]时,材料的应力、应变、应变率具有以下关系
σs=F1+F22As (7) εs=u1−u22Ls (8) ˙εs=dεdt (9) 由于二波法和三波法比较时,三波法的可信度较高,更能反映真实的测试结果,故使用三波法处理实验所得数据。整理式(3)~式(9)可得数据处理公式
˙εs=C0Ls[εi(t)−εr(t)−εt(t)] (10) εs=C0Ls∫t0[εi(t)−εr(t)−εt(t)]dt (11) σs=A0E02As[εi(t)+εr(t)+εt(t)] (12) 式中:
˙εs 、εs 和σs 分别为动态拉伸实验中试样的应变率、应变和应力,εi(t) 、εr(t) 和εt(t) 分别为应变片所获取的电压信号经转换之后的入射应变、反射应变和透射应变,C0 、A0 和E0 分别为杆的弹性波波速、横截面积和弹性模量,t 为应力波在试样内的传播时间。1.3 动态拉伸实验关键问题及解决方法
SHTB实验装置的拉杆均为直径20 mm的实心铝杆,杆系总长度为9500 mm,其中,入射杆长度为5000 mm,透射杆长度为3000 mm,吸收杆长度为1500 mm。法兰盘安装于入射杆上,管状铝质套筒子弹长度为600 mm。软质高分子聚合物材料的SHTB实验与SHPB实验不同,实验过程存在动态拉伸试样连接方式及胶黏剂优选、恒应变率加载与入射波完全卸载等问题,可联合波形分析和高速摄像等方法加以解决。
1.3.1 试样连接方式的确定及胶黏剂优选
目前,SHTB实验中试样的连接方式主要有3种:螺纹连接、挂接以及黏接。由于PVC弹性体材料自身特性不宜采用螺纹连接,因而本研究首先尝试了较常用的挂接方式(使用特殊夹具夹持固定试样)。
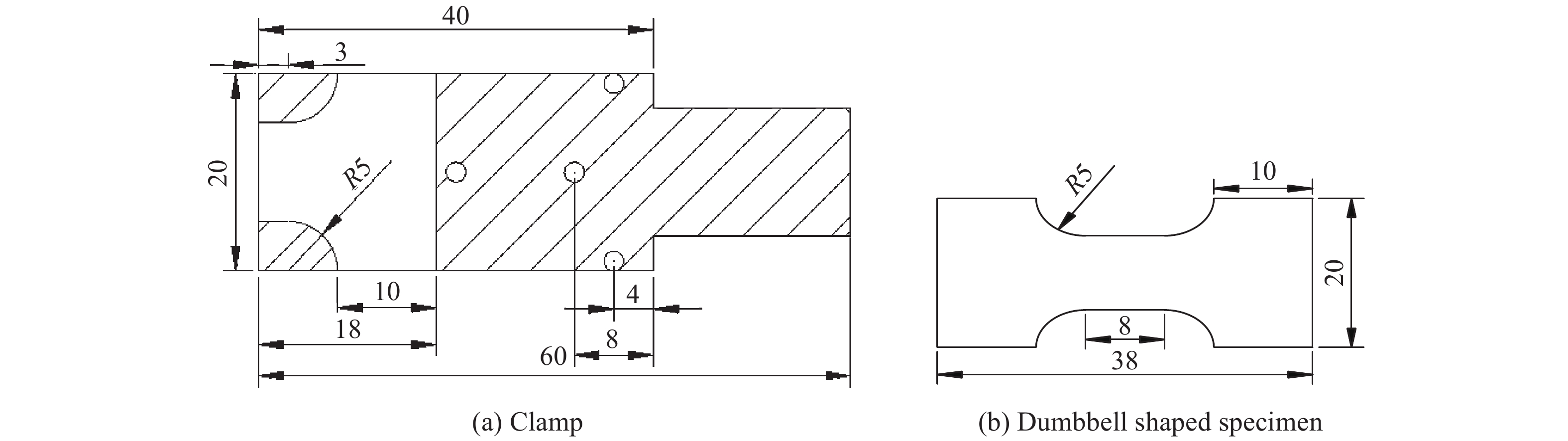
挂接夹具和哑铃状试样尺寸如图3所示,由于聚氯乙烯(PVC)弹性体材料的波速较低,难以达到应力均匀,故试样不可过长;同时若试样长度过短,试样两端夹持部分会影响实验结果的准确性。根据橡胶材料测试经验[23],本研究动态拉伸试样有效拉伸长度设置为2 mm。
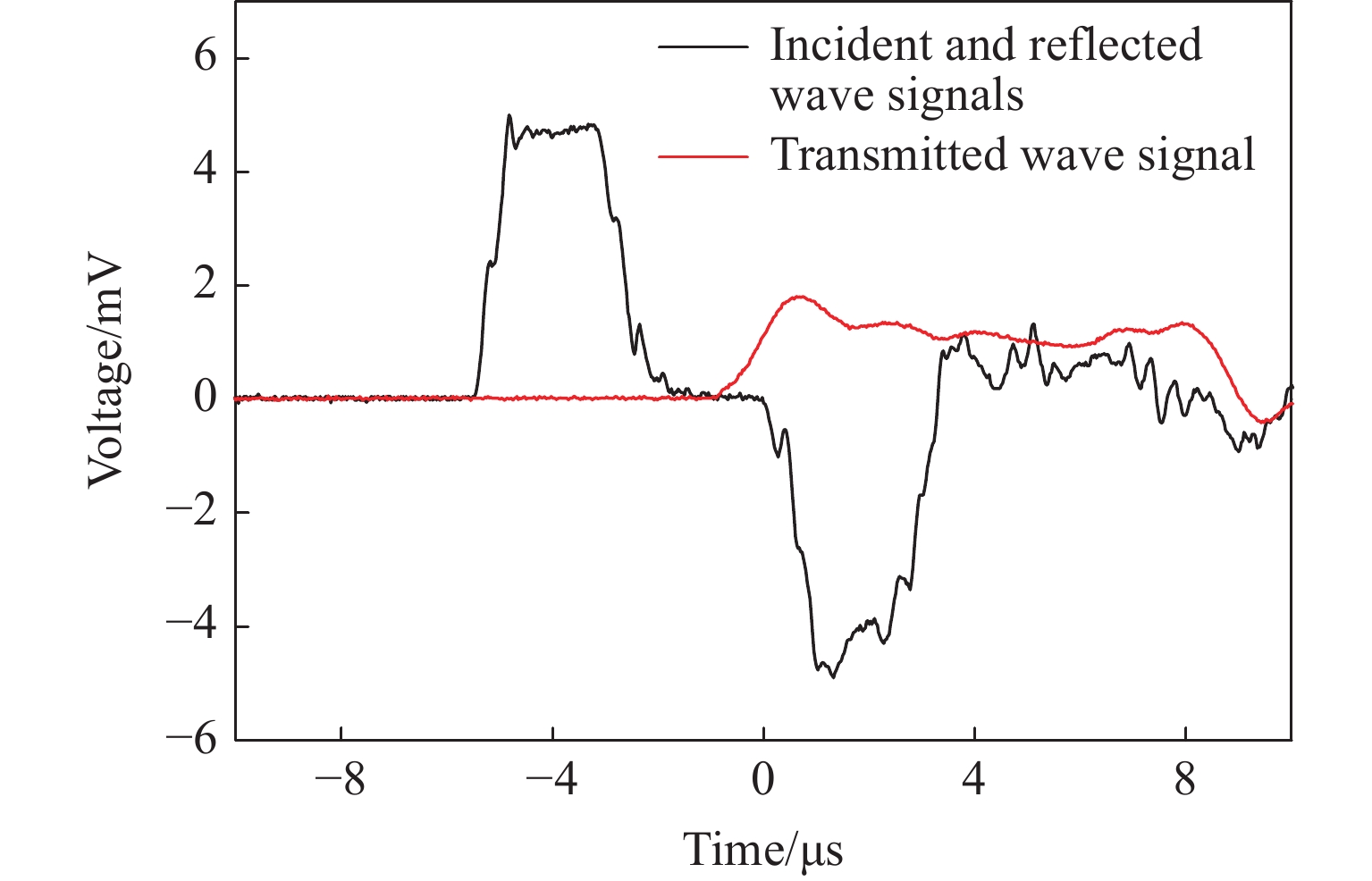
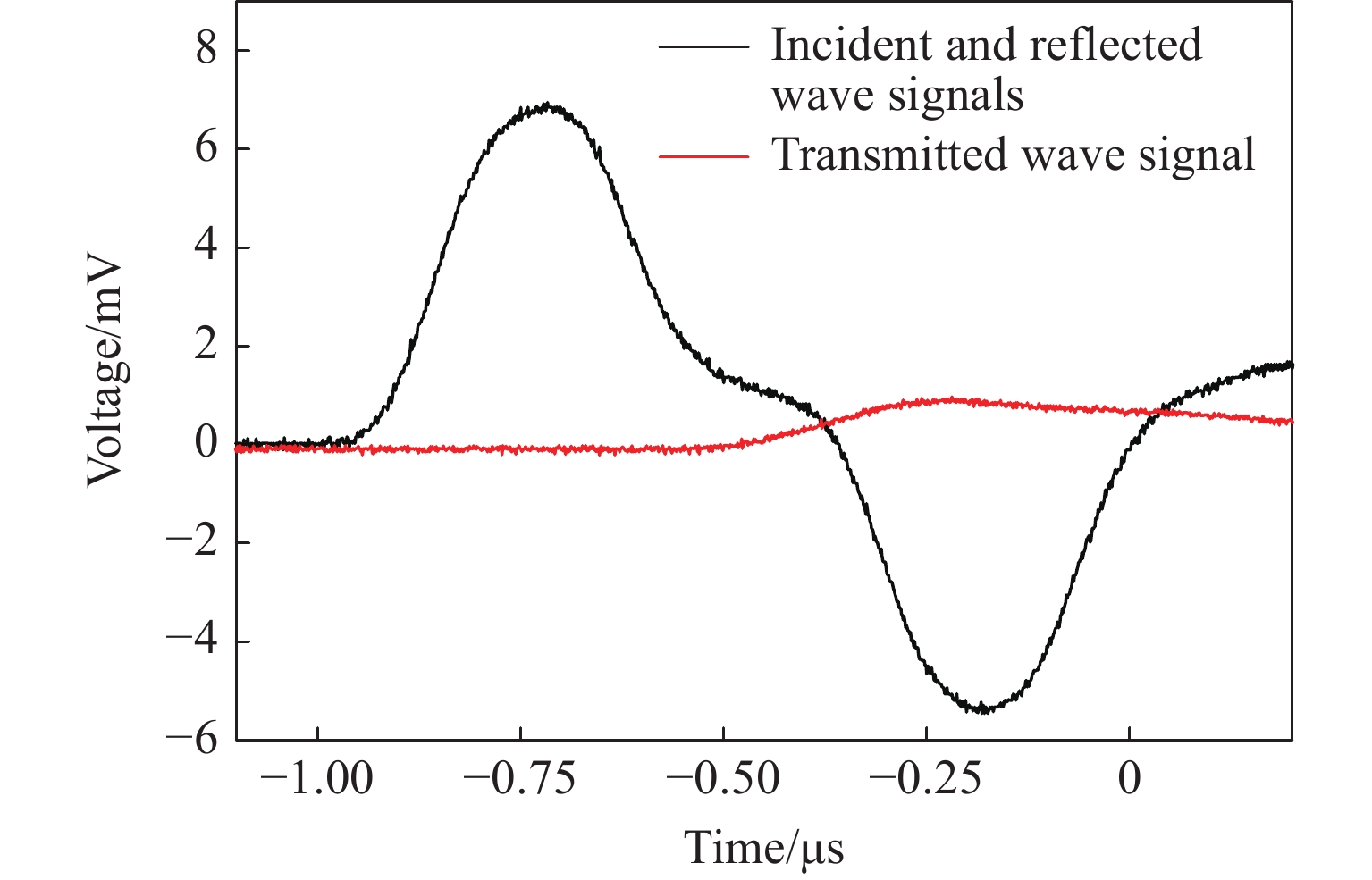
挂接所得波形见图4。由图4可知:入射脉冲为矩形方波,且在100 μs内便可达到最大应变;透射脉冲较宽且透射信号在0~0.2 μs之间有明显下降点,但始终未下降至水平基线位置。
通过分析发现,出现这一现象主要有两方面原因:一是挂接中使用的夹具对波形传播造成了干扰;二是透射杆长度过短,使得通过应变片的透射波沿透射杆返回,与尚未完全通过应变片的透射波在应变片处发生了叠加。
为解决上述问题,对原有SHTB实验装置进行了改进。改进后的SHTB杆系总长度为11500 mm,其中,入射杆长度为5000 mm,透射杆长度为5000 mm,吸收杆长度为1500 mm。试样的连接方式改为黏接(将试样用合适强度的胶黏剂黏接于入射杆与透射杆之间),试样尺寸为
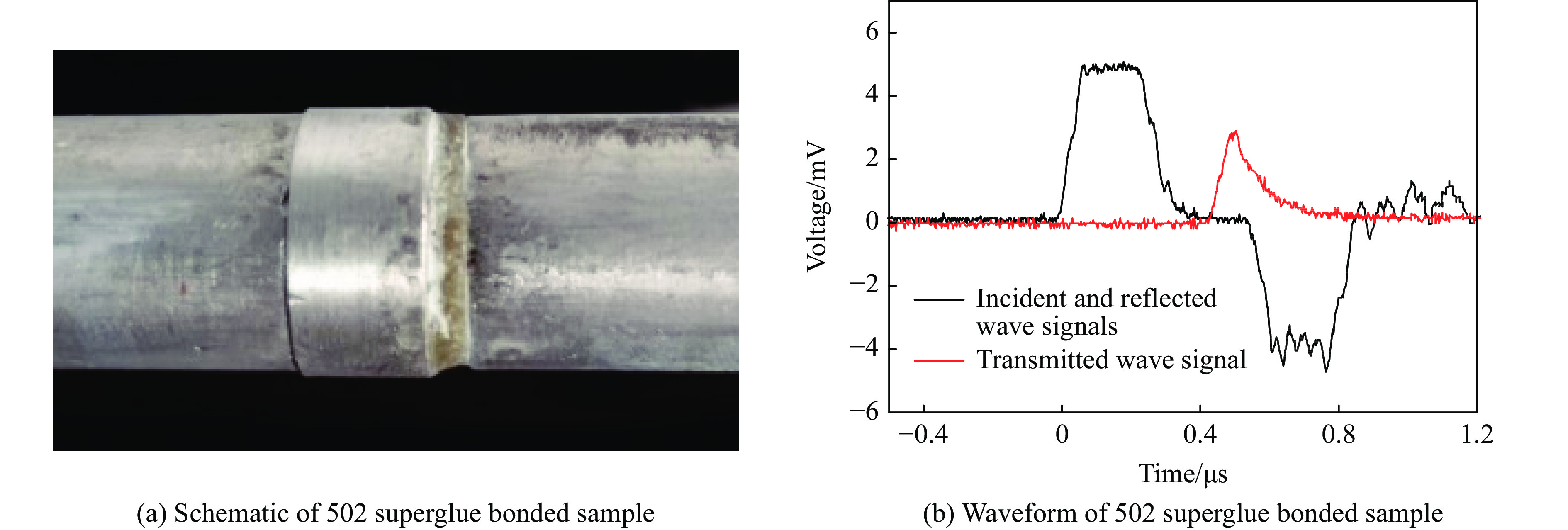
∅ 20 mm× 2 mm。由于胶黏剂完全固化后才可达到最大黏接强度,为加快实验速度,首先选用了强度高、完全固化时间短(约10 min)的502瞬间强力胶进行了实验,试样黏接示意图及加载波形见图5。
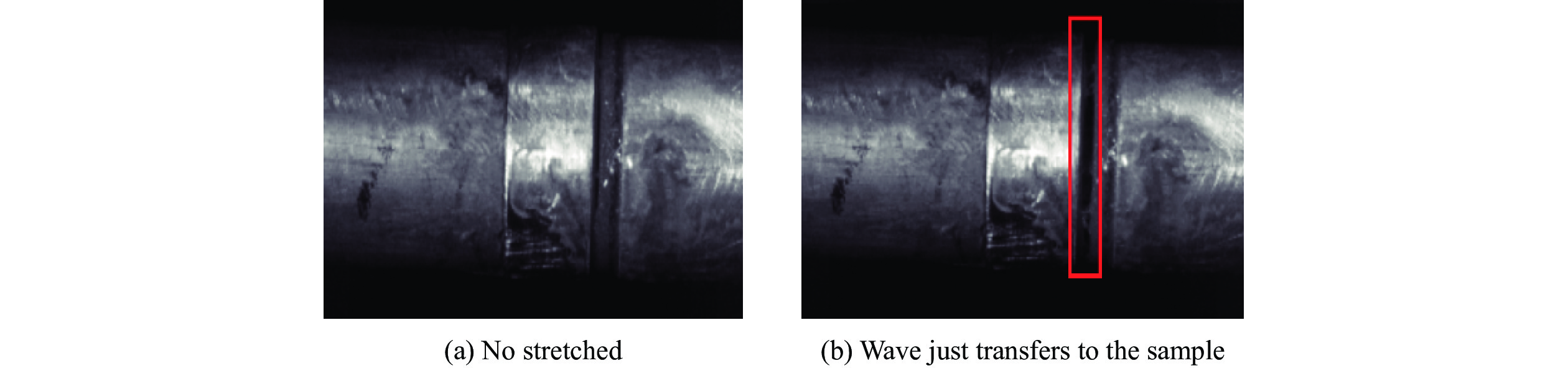
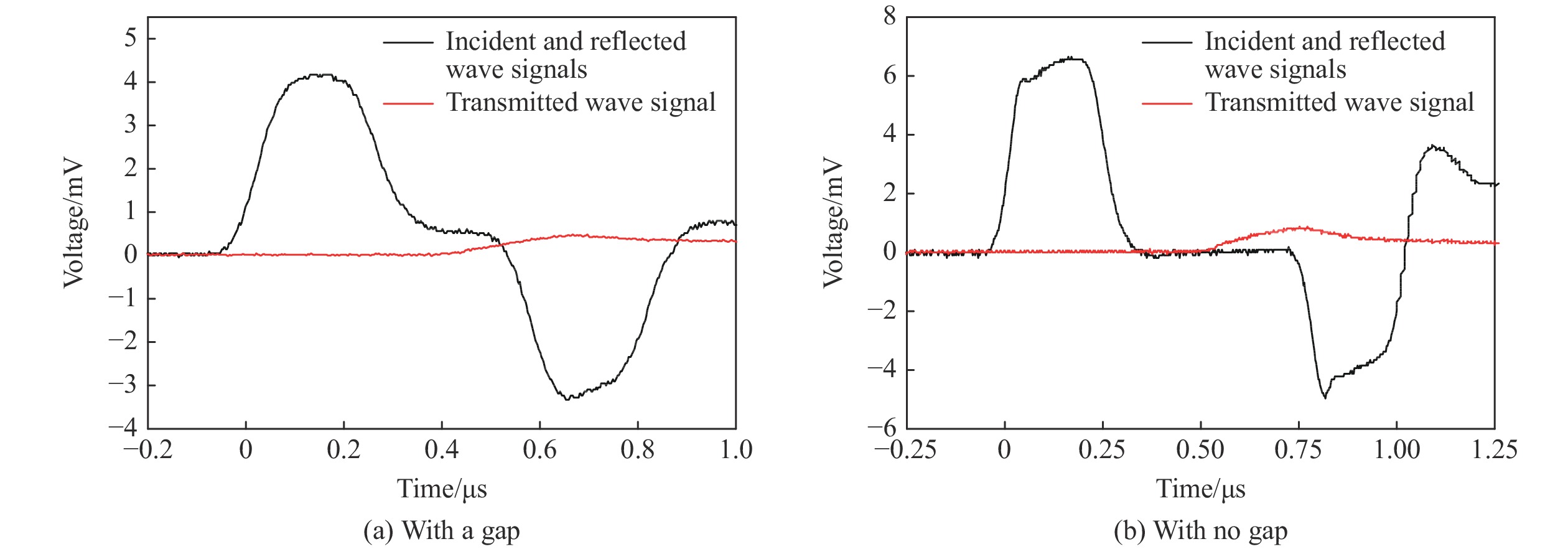
由图5可知,入射波仍为矩形方波,且可在100 μs内达到最大应变;透射脉冲在极短时间内上升至最大应变后迅速下降且回到基线位置。为明确透射波上升至最大应变后立即卸载的原因,采用高速摄像机(V1212,深圳约克科技有限公司)记录了动态拉伸实验过程,发现波传递至试样与入射杆黏接面的瞬间,502瞬间强力胶发生强烈抖动,导致试样与入射杆黏接面脱胶,致使透射波上升至最大应变后立即卸载,高速摄像照片见图6。
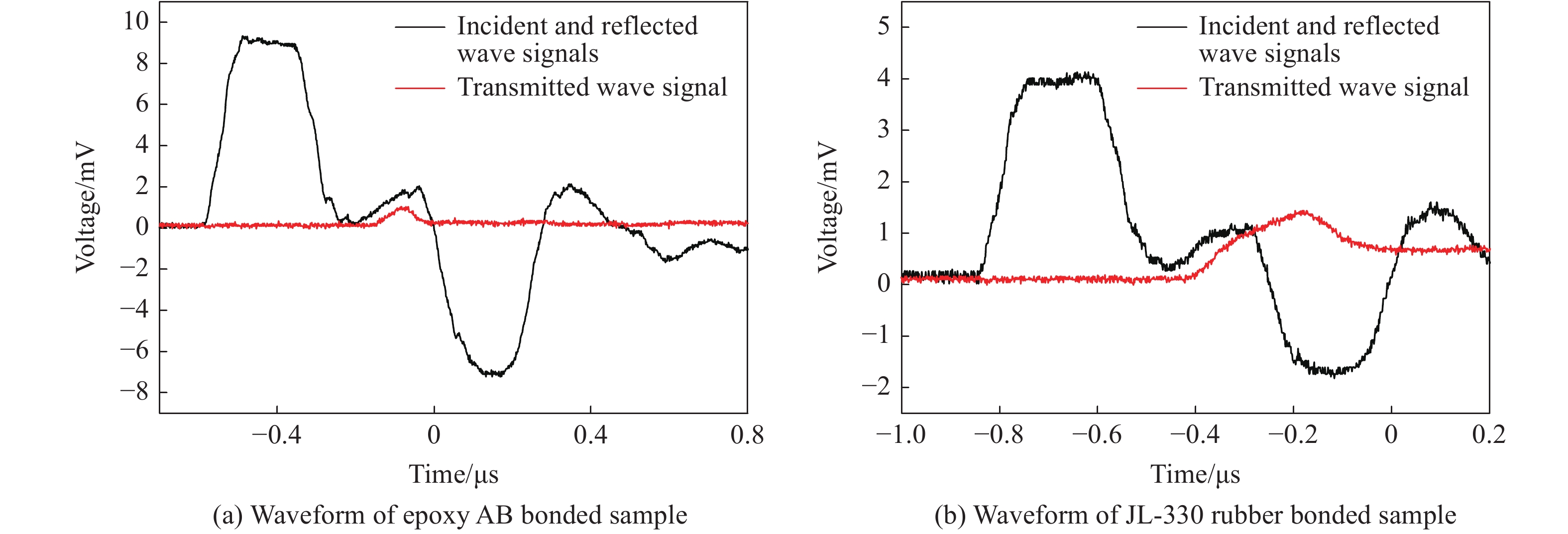
为解决脱胶问题,对比了多种类型的胶黏剂(如YH-818专用橡胶胶水、固特灵401胶水、环氧树脂AB胶、JL-330橡胶专用胶等)并进行了胶黏剂优选。研究发现,环氧树脂AB胶和JL-330橡胶专用胶可以将试样牢固地黏接于入射杆与透射杆之间。利用SHTB装置对这两种胶黏剂黏接的试样进行了动态拉伸实验,以确定这两种胶黏剂的适用性,实验所得波形见图7。
由图7可知,采用两种胶黏剂所得入射脉冲均为矩形方波,且可在100 μs内达到最大应变,其中,环氧树脂AB胶所得透射脉冲上升至最大应变后迅速下降并可回到基线,JL-330橡胶专用胶所得透射波加载时间变长(约在200 μs左右),且达到最大应变后缓慢下降,说明JL-330橡胶专用胶可在一定程度上缓解透射波上升至最大应变后立即卸载的问题,故后续实验将尝试用JL-330橡胶专用胶进行聚氯乙烯弹性体动态拉伸实验。
1.3.2 恒应变率加载
当SHTB实验采用直接加载时,入射波为方波且在极短时间内即可达到最大应变,难以保证整个加载过程为恒应变率加载。为确保SHTB实验结果的准确性,在法兰盘端面(套筒子弹撞击端面)上放置脉冲整形器,以获得所需的入射波特征(通常保证入射波上升沿所对应的加载时间大于加载脉冲在试样中3个往复所需时间)并实现恒应变率加载。在本SHTB实验中,脉冲整形器材料采用铜版纸,并加工成外径为28.4 mm、内径为20 mm的环形片,涂抹少量凡士林将其黏附在法兰盘的端面上,脉冲整形器的安装见图8。
试样中应力波波速约为70 m/s,达到基本均匀需要约240 μs才能使波在试样中传播3个来回。经过多次实验发现,实验中加入整形器后所得入射波的上升沿为270~300 μs,试样达到应力平衡状态的时间充足,其波形如图9所示。
1.3.3 入射波完全卸载
SHTB实验过程中,常将吸收杆与入射杆间留出一定空隙(2~5 mm),以避免入射杆与吸收杆之间发生二次撞击,但在PVC弹性体的SHTB实验过程中发现,入射杆与吸收杆间的空隙会导致入射波偏离基线,即未完全卸载,见图10(a)。对实验装置进行分析发现,法兰盘厚度远小于子弹长度,使得子弹与法兰盘一起运动,这是引发入射波偏离基线的主要原因。为了解决这一问题,在实验时应消除入射杆与吸收杆之间的空隙,以卸载入射波,进而获得理想实验波形,见图10(b)。
2. 实验结果与分析
2.1 准静态拉伸应力-应变曲线分析
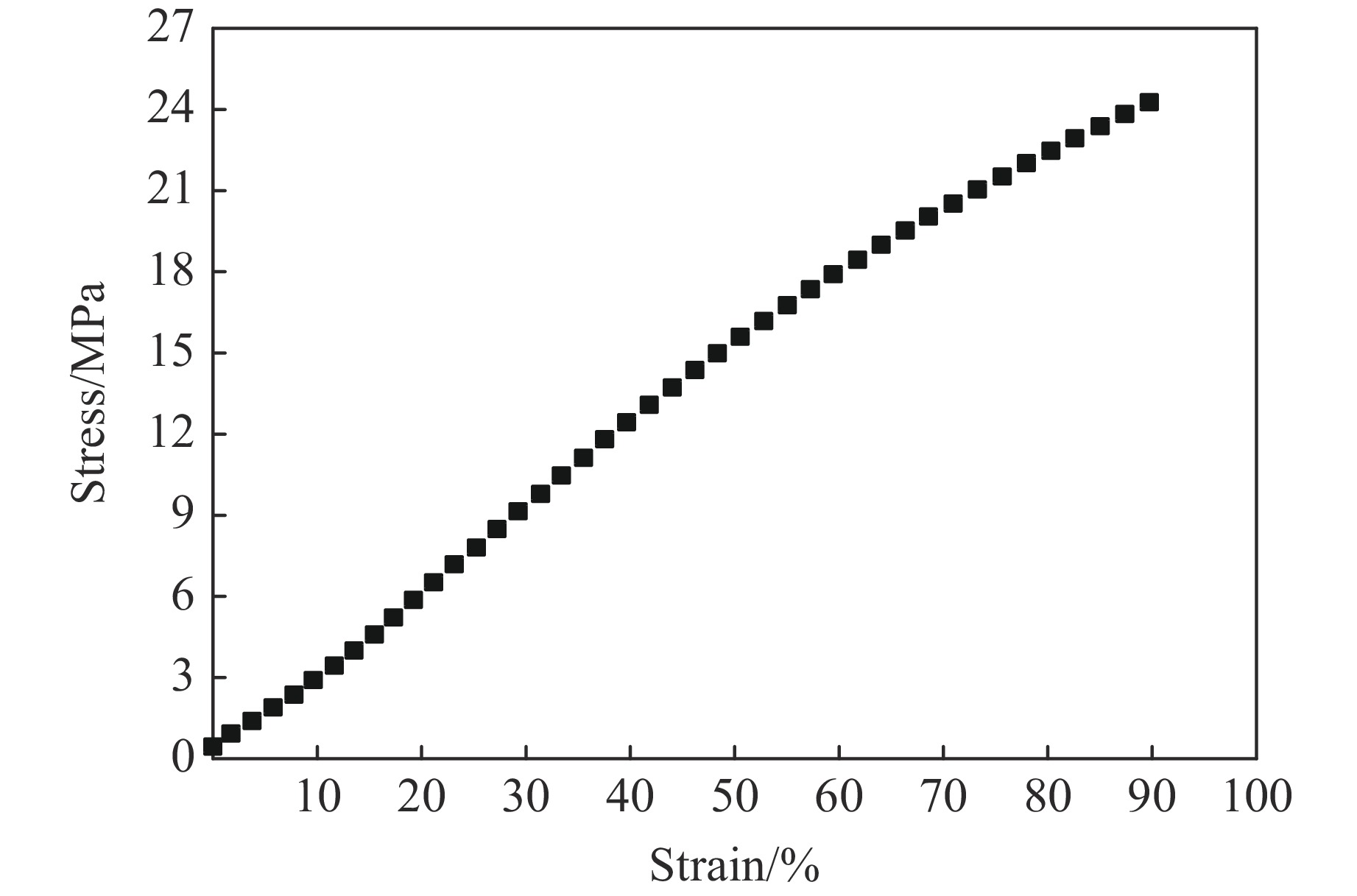
PVC弹性体在准静态加载下的拉伸载荷-位移曲线如图11所示。由图11可以看出,PVC弹性体在准静态下的拉伸力学性能基本呈线性增加趋势。从载荷-位移曲线中可以计算得出,PVC弹性体在0.1 s−1应变率下的拉伸模量为27 MPa。
2.2 动态拉伸应力-应变曲线分析
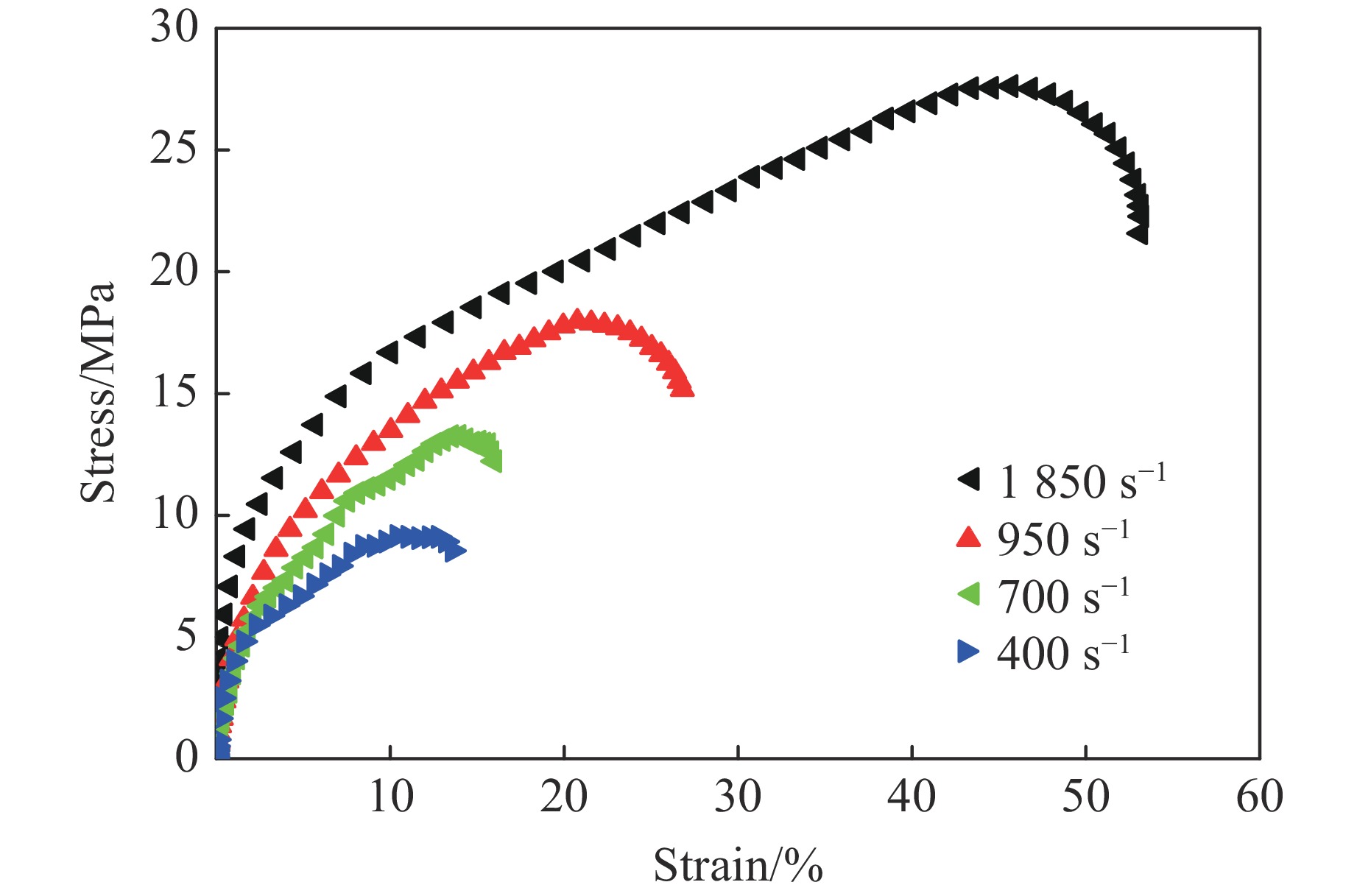
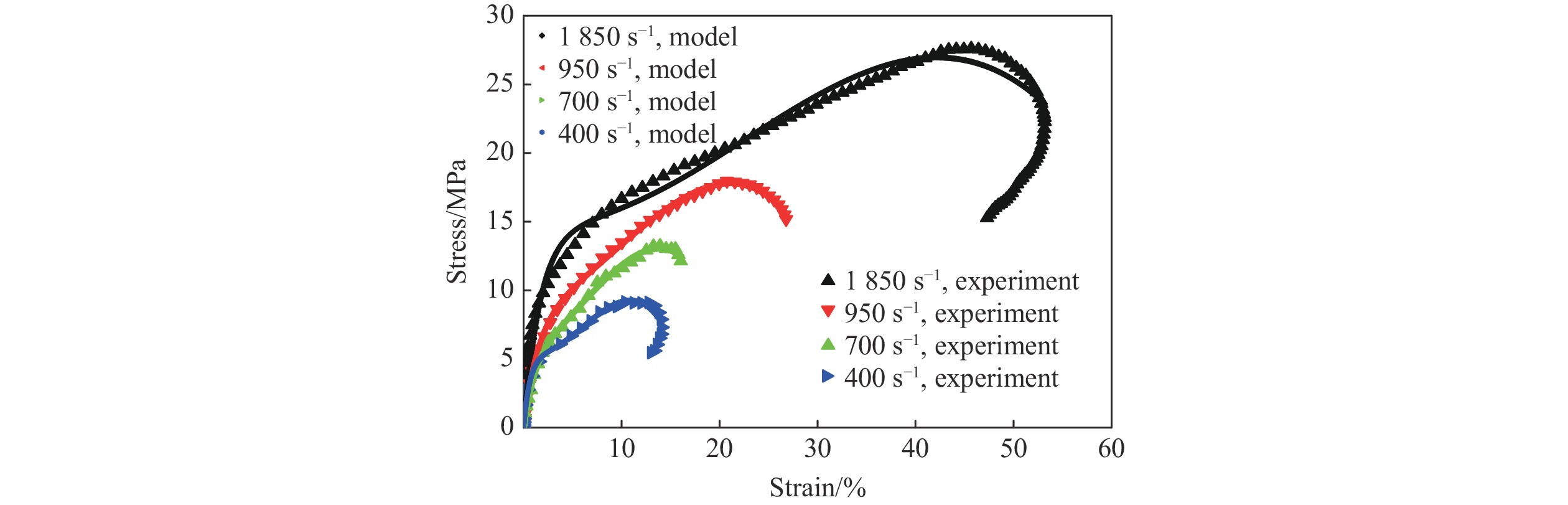
对PVC弹性体动态拉伸实验数据进行处理,得到应变率为400~1850 s−1时的拉伸应力-应变曲线,如图12所示。由图12可以看出,PVC弹性体在高应变率拉伸加载条件下的应力-应变曲线可分为弹性、塑性以及卸载3个阶段,呈现出明显的非线性特征,且在不同的高应变率载荷作用下具有不同的拉伸弹性模量、峰值应力、峰值应变,表现出一定的应变率敏感性。图12中,应变为0.1时的对应点与原点连线,用其斜率表征杨氏模量Eg
,称之为割线模量。为进一步说明PVC弹性体的应变率效应,取图12中应变为0.1处对应的割线模量、峰值应力、峰值应变进行比较,其具体变化情况见表1。 表 1 动态参数变化情况Table 1. Variations of dynamic propertiesStrain rate/s−1 Secant modulus/MPa Peak stress/MPa Peak strain/% 400 89.78 9.19 14.12 700 115.62 13.25 16.07 950 134.82 17.99 26.85 1 850 166.81 27.63 53.19 由表1可知,当应变率范围为400~1850 s−1时,割线模量、峰值应力及峰值应变均随应变率的增加而增大。
3. ZWT非线性黏弹性模型
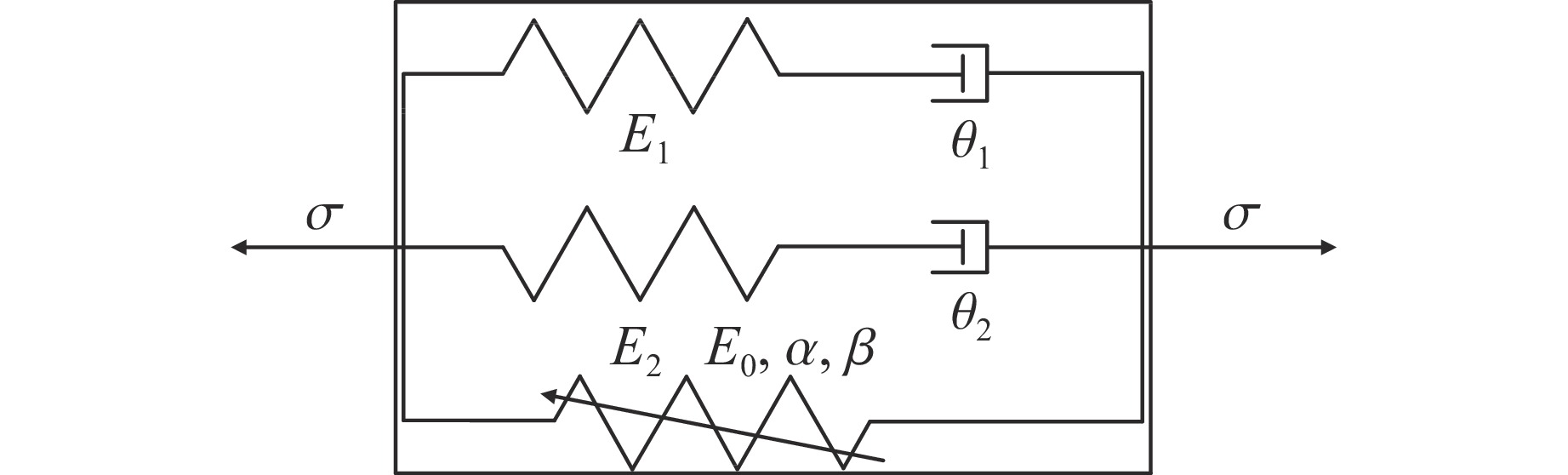
高分子聚合物的动态力学性能可由一个非线性弹簧和两个Maxwell单元组成的朱-王-唐(ZWT)本构模型(见图13)描述,其表达式为
σ=E0ε+αε2+βε3+E1∫t0˙εexp(−t−τθ1)dτ+E2∫t0˙εexp(−t−τθ2)dτ (13) 式中:前3项用于表征材料非线性弹性响应且与应变率无关,后两项分别代表低、高应变率下的黏弹性响应,E0、
α 和β 为试验确定的弹性常数,E1、θ 1和E2、θ 2分别为试验确定的低、高应变率下的弹性常数和松弛时间。当材料受到低应变率加载时,高应变率所对应的Maxwell单元始终处于松弛状态;而当材料受到高应变率加载时,低应变率所对应的Maxwell单元则来不及松弛。相应的模型表达式可简化为σ=E0ε+αε2+βε3+E1∫t0˙εexp(−t−τθ1)dτ (14) σ=(E0+E1)ε+αε2+βε3+E2∫t0˙εexp(−t−τθ2)dτ (15) 本实验的加载率可近似看作恒应变率加载,故式(14)和式(15)可写为
σ=E0ε+αε2+βε3+E1θ1˙ε[1−exp(−εθ1˙ε)] (16) σ=(E0+E1)ε+αε2+βε3+E2θ2˙ε[1−exp(−εθ2˙ε)] (17) 采用式(16)和式(17)拟合PVC弹性体在高应变率下的实验数据,相应的拟合参数见表2。
表 2 ZWT模型拟合参数值Table 2. ZWT model fitting parameter valuesStrain rates/s−1 E0 E1 α β E2 θ2 400 −7.364 6.930 0.166 0.009 8.414 0.002 0 700 −0.350 0.630 0.076 0.004 5.074 0.002 0 950 51.249 −51.151 0.051 0.002 5.482 0.001 7 1 850 2.392 −2.550 0.027 0 8.647 0.001 0 图14为实验结果与本构模型拟合结果的对比。可以看出,两者吻合较好,说明该本构能够较好地描述PVC弹性体在高应变率范围内的动态力学性能。
4. 结 论
开展了PVC弹性体材料的动态拉伸实验,联合波形分析和高速摄像等方法对动态拉伸试样连接方式及胶黏剂进行了优选,实现了恒应变率加载与入射波完全卸载,研究了PVC弹性体在高应变率(400~1850 s−1)拉伸载荷作用下的力学性能,并构建了黏弹性本构模型。通过实验结果分析发现:
(1)提出的基于SHTB装置的动态拉伸技术可用于软质高分子聚合物材料,能满足霍普金森实验的两个基本假设,测试所得结果可反映材料的动态力学性能,且装置结构简单,便于操作和推广;
(2)聚氯乙烯(PVC)弹性体在低应变率(0.1 s−1)拉伸载荷作用下具有明显的非线性弹性特征,PVC弹性体在高应变率(400~1850 s−1)载荷作用下具有不同的拉伸弹性模量、峰值应力、峰值应变,具有明显的应变率敏感性;
(3)PVC弹性体的动态拉伸力学性能的黏弹性特征明显,用ZWT本构模型描述特征时误差较小。
研究结果可为软质高分子聚合物材料的静、动态力学性能研究提供参考。为研究温度对PVC弹性体静、动态拉伸力学性能的影响,后期将用高/低温环境箱开展不同环境温度下的PVC弹性体材料的静、动态拉伸力学性能测试实验。
-
Figure 4. Comparisons of our Arrhenius relations for calcite (a) and aragonite (b) with the data of Cherniak et al.[4] (He diffusion in calcite displays marked anisotropy, while in aragonite shows moderate anisotropy.)
Figure 6. Calculated closure temperature (Tc) as a function of grain radius (a) along different directions in calcite and aragonite (Closure temperature are plotted for assuming spherical geometry (A=55) of the crystals. Helium in each carbonate composition using Dodson’s (1973) equation and a cooling rate of 10 ℃/Ma.)
Table 1. Calculated structural parameters of calcite and aragonite in comparison with previous theoretical and experimental values
Mineral Data source Unit cell volume/nm3 a/nm b/nm c/nm C-O bond distance/nm Ca-O bond distance/nm Calcite This work 379.58 5.05 17.21 1.299 2.383 Calculation[22] 383.20 5.05 17.33 1.291 2.397 Experiment[23] 368.10 4.99 17.06 1.284 2.359 Aragonite This work 232.58 5.01 8.01 5.79 1.291 2.469 Calculation[24] 233.84 5.02 8.04 5.80 1.292 2.440 Experiment[25] 226.65 4.96 7.96 5.74 1.284 2.414 Table 2. Calculated parameters for helium diffusion in calcite and aragonite under ambient and high pressures
Mineral Pressure/GPa Direction Ea/(kJ·mol–1) v/THz l/nm D0/(m2·s–1) Calcite 0 [010] 67.64 4.29 5.05 5.46×10–7 0 [001] 97.36 4.19 4.71 4.65×10–7 0 [100] 82.40 7.71 5.00 9.64×10–7 0 [001] 96.00 6.34 5.79 1.06×10–6 3 [100] 110.57 7.11 4.98 8.82×10–7 3 [001] 125.43 6.57 5.68 1.06×10–6 Aragonite 6 [100] 115.78 7.03 4.95 8.61×10–7 6 [001] 133.63 6.85 5.85 1.17×10–6 10 [100] 139.42 7.54 4.90 9.05×10–7 10 [001] 160.17 7.56 5.47 1.13×10–6 14 [100] 154.38 7.01 4.86 8.28×10–7 14 [001] 174.45 8.41 5.36 1.21×10–6 Table 3. Summary of the characteristic bond distances of activated states in calcite and aragonite under different pressure conditions (All bond distances are the smallest distances.)
Mineral Pressure/GPa Direction Ca-O bond distance/nm C-O bond distance/nm He-O bond distance/nm Calcite 0 [010] 2.261 1.299 2.033 0 [001] 2.241 1.295 1.922 0 [100] 2.356 1.296 2.141 0 [001] 2.412 1.287 2.042 3 [100] 2.358 1.294 2.067 3 [001] 2.379 1.294 2.002 Aragonite 6 [100] 2.351 1.289 2.026 6 [001] 2.317 1.284 1.970 10 [100] 2.340 1.278 1.982 10 [001] 2.290 1.274 1.927 14 [100] 2.334 1.277 1.951 14 [001] 2.265 1.270 1.909 -
[1] CHERNIAK D J, WATSON E B, THOMAS J B. Diffusion of helium in zircon and apatite [J]. Chemical Geology, 2009, 268(1): 155–166. [2] REICH M, EWING R C, EHLERS T A, et al. Low-temperature anisotropic diffusion of helium in zircon: implications for zircon (U–Th)/He thermochronometry [J]. Geochimica et Cosmochimica Acta, 2007, 71(12): 3119–3130. doi: 10.1016/j.gca.2007.03.033 [3] REINERS P W. Zircon (U-Th)/He thermochronometry [J]. Reviews in Mineralogy Geochemistry, 2005, 58(1): 151–179. doi: 10.2138/rmg.2005.58.6 [4] CHERNIAK D J, AMIDON W, HOBBS D, et al. Diffusion of helium in carbonates: effects of mineral structure and composition [J]. Geochimica et Cosmochimica Acta, 2015, 165: 449–465. doi: 10.1016/j.gca.2015.06.033 [5] COPELAND P, WATSON E B, URIZAR S C, et al. Alpha thermochronology of carbonates [J]. Geochimica et Cosmochimica Acta, 2007, 71(18): 4488–4511. doi: 10.1016/j.gca.2007.07.004 [6] COPELAND P, COX K, WATSON E B. The potential of crinoids as (U+Th+Sm) /He thermochronometers [J]. Earth and Planetary Science Letters, 2015, 42: 1–10. [7] CROS A, GAUTHERON C, PAGEL M, et al. 4He behavior in calcite filling viewed by (U-Th)/He dating, 4He diffusion and crystallographic studies [J]. Geochimica et Cosmochimica Acta, 2014, 125: 414–432. doi: 10.1016/j.gca.2013.09.038 [8] AMIDON W H, HOBBS D, HYNEK S A, et al. Retention of cosmogenic 3He in calcite [J]. Quaternary Geochronology, 2015, 27: 172–184. doi: 10.1016/j.quageo.2015.03.004 [9] BENGTSON A, EWING R C, BECKER U. He diffusion and closure temperatures in apatite and zircon: a density functional theory investigation [J]. Geochimica et Cosmochimica Acta, 2012, 86: 228–238. doi: 10.1016/j.gca.2012.03.004 [10] WANG K, BRODHOLT J, LU X. Helium diffusion in olivine based on first principles calculations [J]. Geochimica et Cosmochimica Acta, 2015, 156: 145–153. doi: 10.1016/j.gca.2015.01.023 [11] BALOUT H, ROQUES J, GAUTHERON C, et al. Helium diffusion in pure hematite (α-Fe3O3) for thermochronometric applications: a theoretical multi-scale study [J]. Computational and Theoretical Chemistry, 2017, 1099: 21–28. doi: 10.1016/j.comptc.2016.11.001 [12] SONG Z, WU H, SHU S, et al. A first-principles and experimental study of helium diffusion in periclase MgO [J]. Physics and Chemistry of Minerals, 2018, 45(7): 641–654. doi: 10.1007/s00269-018-0949-y [13] DODSON M H. Closure temperatures in cooling geological and petrological systems [J]. Contributions to Mineralogy Petrology, 1973, 40(3): 259–274. doi: 10.1007/BF00373790 [14] HOHENBERG P, KOHN W. Inhomogenous electron gas [J]. Physical Review, 1964, 136: 864–871. doi: 10.1103/PhysRev.136.B864 [15] KOHN W, SHAM L J. Quantum density oscillations in an inhomogeneous electron gas [J]. Physical Review, 1965, 137: 1697–1705. doi: 10.1103/PhysRev.137.A1697 [16] KRESSE G, FURTHMULLER J. Efficiency of ab-initio total energy calculations for metals and semiconductors using a plane-wave basis set [J]. Computational Materials Science, 1996, 6(1): 15–50. doi: 10.1016/0927-0256(96)00008-0 [17] KRESSE G, HAFNER J. Ab initio molecular dynamics for liquid-metals [J]. Physical Review B, 1993, 47(1): 558–561. doi: 10.1103/PhysRevB.47.558 [18] BLÖCHL P E. Projected augmented-wave method [J]. Physical Review B, 1996, 50(24): 17953–17979. [19] KRESSE G, JOUBERT D. From ultrasoft pseudopotentials to the projector augmented-wave method [J]. Physical Review B, 1999, 59(3): 1758–1775. doi: 10.1103/PhysRevB.59.1758 [20] PERDEW J P, BURKE K, ERNZERHOF M. Generalized gradient approximation made simple [J]. Physical Review Letters, 1996, 77(18): 3865–3868. doi: 10.1103/PhysRevLett.77.3865 [21] CHADI D J. Special points for Brillouin-zone integrations [J]. Physical Review B, 1977, 16(4): 1746–1747. doi: 10.1103/PhysRevB.16.1746 [22] BRIK M G. First-principles calculations of structural, electronic, optical and elastic properties of magnesite MgCO3 and calcite CaCO3 [J]. Physica B: Condensed Matter, 2011, 406(4): 1004–1012. doi: 10.1016/j.physb.2010.12.049 [23] MALSEN E N, STRELTSOV V A, STRELTSOVA N R, et al. X-ray study of the electron density in calcite, CaCO3 [J]. Acta Crystallographica Section B: Structural Science, 1993, 49(4): 636–641. doi: 10.1107/S0108768193002575 [24] OGANOV A R, GLASS C W, ONO S. High-pressure phases of CaCO3: crystal structure prediction and experiment [J]. Earth and Planetary Science Letters, 2006, 241(1): 95–103. [25] DICKENS B, BOWEN J S. Refinement of the crystal of the aragonite phase of CaCO3 [J]. Physics and Chemistry A, 1971, 75(1): 27–32. [26] HENKELMAN G. Improved tangent estimate in the nudged elastic band method for finding minimum energy paths and saddle points [J]. Journal of Chemical Physics, 2000, 113(22): 9978–9985. doi: 10.1063/1.1323224 [27] VINEYARD G H. Frequency factors and isotope effects in solid state rate processes [J]. Journal of Physics and Chemistry of Solids, 1957, 3(1/2): 121–127. [28] BENDER M L. Helium-uranium dating of corals [J]. Geochimica et Cosmochimica Acta, 1973, 37(5): 1229–1247. doi: 10.1016/0016-7037(73)90058-6 -








 下载:
下载:
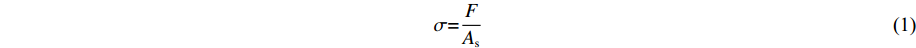
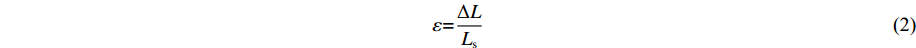
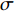
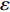

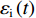
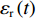
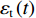
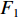

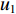
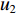
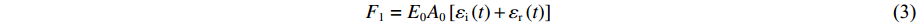
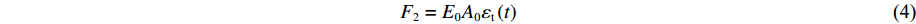
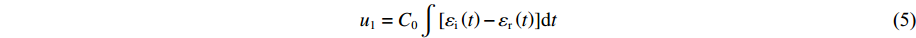
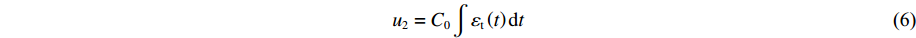
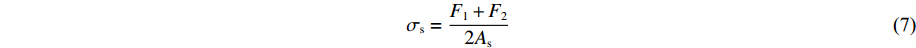
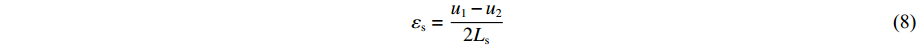
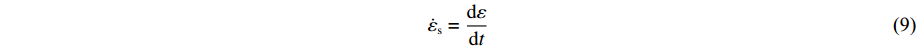
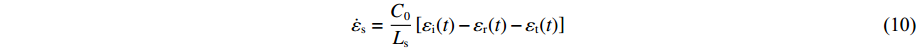
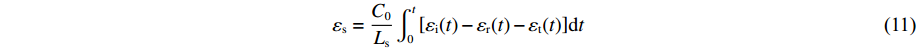
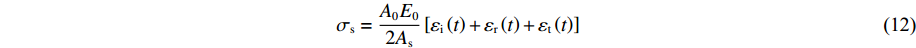

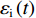


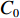
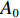
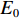
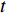












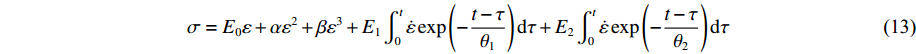

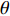
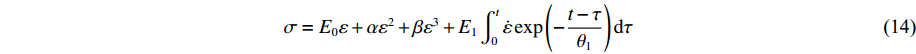
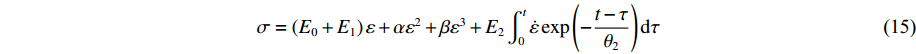
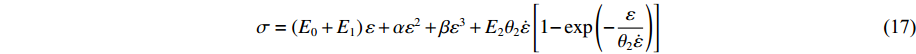


 下载:
下载: