Numerical Analysis of Impact of Shot Hole Spacing on Crack Growth in Rock
-
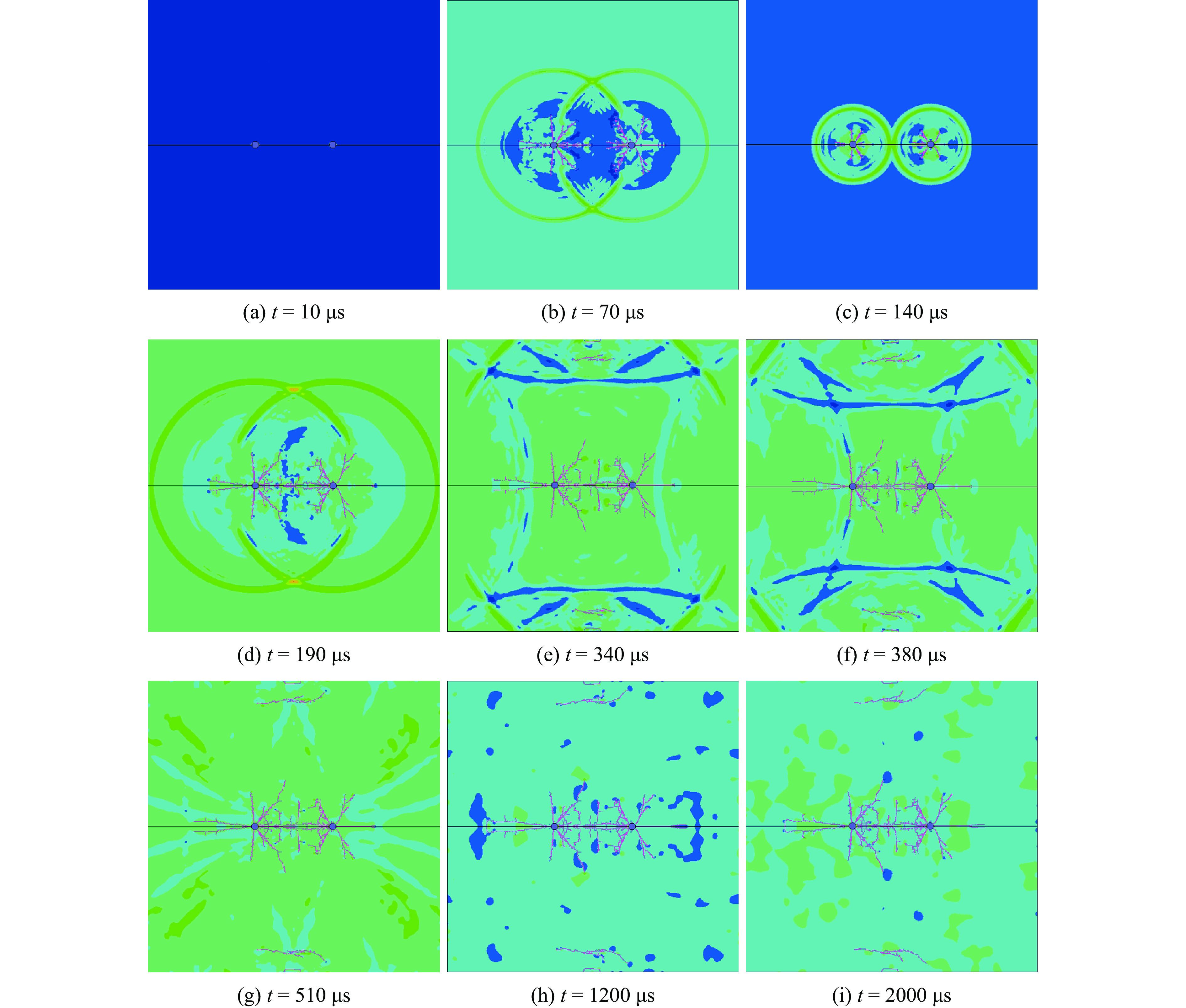
摘要: 为实现对岩石的充分破碎，有效利用炸药能量，基于有限元分析软件ANSYS/LS-DYNA及流固耦合（ALE）算法，研究了不同炮孔间距对岩石（角岩）爆炸裂纹扩展的影响，同时将模拟结果应用在工程实践上加以验证。结果表明：随着两炮孔间距离的增大，单炮孔周围裂纹扩展更加充分，炮孔周围粉碎区增大，萌生的分支小裂纹逐渐减少，主裂纹增多。在两炮孔之间受到相邻炮孔爆炸应力波影响的区域，主裂纹发育扩展较为明显，且随着炮孔间距的增大，主裂纹相互贯通的位置越靠近两炮孔中心连线方向。工程实践表明：数值模拟结果与爆破工程效果具有较好的吻合性，将数值模拟结果用来指导爆破方案设计是可行的，能够为爆破工程提供重要的参考。Abstract: In order to achieve full fragmentation of the rock and effectively use the explosive energy, the impact of different hole spacing on the rock (hornfels) blast crack extension was studied via ANSYS/LS-DYNA software package using fluid-structure interaction (ALE) algorithm. The results showed that with the increase of the distance between the two holes, the crack growth around the single gun hole becomes more sufficient, the comminution area around the gun hole increases, the generated branch small crack gradually decreases, the main crack increases, and the crack growth rate is about 0.42 times that of the longitudinal wave velocity of the rock. In the area between the two holes affected by the explosion stress wave of the adjacent holes, the main crack growth and expansion are more obvious, and with the increase of hole spacing, the position of the main crack interconnection is closer to the direction of connecting the center of the two holes. Engineering practice suggested that the results of numerical simulation has positive effect on blasting engineering, the results of numerical simulation can be used to guide the design of blasting scheme and can provide important reference value for the blasting engineering.
-
Key words:
- finite element /
- fluid-solid coupling /
- crack propagation /
- blasthole spacing /
- spallation
-
图 5 不同孔间距下炮孔中心连线中点处x方向的压力曲线
Figure 5. Pressure curve in the x direction at the center of the connecting line of gun hole with different hole spacing
${\rho}$/(kg·m–3) E0/GPa ${\mu}$ ${{\sigma _0}}$/MPa Etan/GPa ${{\sigma _{\rm{c}}}}$/MPa ${{\sigma _{{\rm{st}}}}}$/MPa C/s–1 P 2700 68.69 0.228 75 40 150 5.6 2.63 3.96 
下载: 导出CSV
${\rho}$/(kg·m–3) D/(m·s–1) pCJ/GPa A/GPa B/GPa R1 R2 ${\omega}$ 1200 4000 4.80 214 0.093 4.15 0.95 0.3
下载: 导出CSV
表 3 岩石裂隙扩展平均速度
Table 3. The average speed of rock crack extension
Speed of rock crack extension/（m·s−1） V/Cp L=40 cm L=60 cm L=80 cm 2051（t=40 ${{\text{μ}}{\rm{s}}}$） 2157（t=60 ${{\text{μ}}{\rm{s}}}$） 2286（t=70 ${{\text{μ}}{\rm{s}}}$） 0.39 2197（t=70 ${{\text{μ}}{\rm{s}}}$） 2083（t=120 ${{\text{μ}}{\rm{s}}}$） 2285（t=140 ${{\text{μ}}{\rm{s}}}$） 0.41 1656（t=230 ${{\text{μ}}{\rm{s}}}$） 1923（t=210 ${{\text{μ}}{\rm{s}}}$） 2140（t=190 ${{\text{μ}}{\rm{s}}}$） 0.44 1968（Average） 2054（Average） 2237（Average） 0.41
下载: 导出CSV
表 4 爆破参数表
Table 4. Blasting parameters
H/m h/m a/m b/m L/m l/m Q/kg 1.7 0 2.0 1.2 0.20 1.50 0.9 2.0 0 2.0 1.2 0.22 1.78 1.0 2.5 0 2.5 1.5 0.45 2.05 2.0 3.0 0 3.0 1.5 0.67 2.33 3.0
下载: 导出CSV
表 5 振动监测表
Table 5. Blasting vibration monitoring
Measuring point Distance/m vx/(cm·s–1) fx/Hz vy/(cm·s–1) fy/Hz vz/(cm·s–1) fz/Hz 1 163 –0.21 9.16 –0.28 9.16 0.27 18.31 2 154 0.04 8.85 0.04 35.71 0.03 9.48 3 339 0.04 12.16 0.05 7.83 0.06 13.29 4 234 0.19 18.31 –0.14 9.16 –0.11 18.31
下载: 导出CSV
-
[1] MA G W, HAO H, WANG F. Simulations of explosion-induced damage to underground rock chambers [J]. Journal of Rock Mechanics and Geotechnical Engineering, 2011, 3(1): 19–29. doi: 10.3724/SP.J.1235.2011.00019 [2] YUE Z W, QIU P, YANG R S, et al. Stress analysis of the interaction of a running crack and blasting waves by caustics method [J]. Engineering Fracture Mechanics, 2017, 184: 339–351. doi: 10.1016/j.engfracmech.2017.08.037 [3] 何成龙, 杨军. 主动围压和爆炸加载作用下岩石动态响应研究 [J]. 兵工学报, 2017, 38(12): 2395–2405. doi: 10.3969/j.issn.1000-1093.2017.12.013HE C L, YANG J. Research on dynamic response of rock under blast loading and active confining pressure [J]. Acta Armamentarii, 2017, 38(12): 2395–2405. doi: 10.3969/j.issn.1000-1093.2017.12.013 [4] ZHAO J J, ZHANG Y, RANJITH P G. Numerical simulation of blasting-induced fracture expansion in coal masses [J]. International Journal of Rock Mechanics and Mining Sciences, 2017, 100: 28–39. doi: 10.1016/j.ijrmms.2017.10.015 [5] ZHANG X L, JIAO Y Y, MA J F. Simulation of rock dynamic failure using discontinuous numerical approach [J]. Computers and Geotechnics, 2018, 96: 160–166. doi: 10.1016/j.compgeo.2017.10.001 [6] 夏祥, 李海波, 李俊如, 等. 岩体爆生裂纹的数值模拟 [J]. 岩土力学, 2006, 27(11): 1987–1991. doi: 10.3969/j.issn.1000-7598.2006.11.025XIA X, LI H B, LI J R, et al. Numerical simulation of blast-induced cracks in rock [J]. Rock and Soil Mechanics, 2006, 27(11): 1987–1991. doi: 10.3969/j.issn.1000-7598.2006.11.025 [7] 周艳, 叶海旺, 易长平, 等. 台阶爆破相邻炮孔间损伤范围的数值模拟 [J]. 工程爆破, 2014, 20(2): 17–20, 28. doi: 10.3969/j.issn.1006-7051.2014.02.005ZHOU Y, YE H W, YI C P, et al. Numerical simulation of damage zone between the adjacent boreholes in bench blasting [J]. Engineering Blasting, 2014, 20(2): 17–20, 28. doi: 10.3969/j.issn.1006-7051.2014.02.005 [8] 郭东明, 闫鹏洋, 杨仁树, 等. 动静荷载下巷道围岩倾斜裂纹的动焦散试验 [J]. 采矿与安全工程学报, 2016, 33(4): 668–675.GUO D M, YAN P Y, YANG R S, et al. Dynamic caustic test of inclined crack in roadwaysurrounding rock under dynamic and static load [J]. Journal of Mining and Safety Engineering, 2016, 33(4): 668–675. [9] 钟波波, 李宏, 张永彬. 爆炸荷载作用下岩石动态裂纹扩展的数值模拟 [J]. 爆炸与冲击, 2016, 36(6): 825–831. doi: 10.11883/1001-1455(2016)06-0825-07ZHONG B B, LI H, ZHANG Y B. Numerical simulation of dynamic cracks propagation of rock under blasting loading [J]. Explosion and Shock Waves, 2016, 36(6): 825–831. doi: 10.11883/1001-1455(2016)06-0825-07 [10] 门建兵, 蒋建伟, 王树有. 爆炸冲击数值模拟技术基础 [M]. 北京: 北京理工大学出版社, 2015.MEN J B, JIANG J W, WANG S Y. Numerical simulation of explosion impact [M]. Beijing: Beijing Institute of Technology Press, 2015. [11] 钮强. 岩石爆破机理[M]. 沈阳: 东北工学院出版社, 1990.NIU Q. Rock blasting mechanism [M]. Shenyang: Northeast Institute of Technology Press, 1990. [12] LI X F, LI H B, ZHAO J. 3D polycrystalline discrete element method (3PDEM) for simulation of crack initiation and propagation in granular rock [J]. Computers and Geotechnics, 2017, 90: 96–112. doi: 10.1016/j.compgeo.2017.05.023 [13] YILMAZ O, UNLU T. Three dimensional numerical rock damage analysis under blasting load [J]. Tunnelling and Underground Space Technology, 2013, 38: 266–278. doi: 10.1016/j.tust.2013.07.007 [14] WANG Z L, KONIETZKY H. Modelling of blast-induced fractures in jointed rock masses [J]. Engineering Fracture Mechanics, 2009, 76(12): 1945–1955. doi: 10.1016/j.engfracmech.2009.05.004 [15] ZHU W C, BAI Y, LI X B. Numerical simulation on rock failure under combined static and dynamic loading during SHPB tests [J]. International Journal of Impact Engineering, 2012, 49: 142–157. doi: 10.1016/j.ijimpeng.2012.04.002 [16] 王礼立, 朱兆祥. 应力波基础 [M]. 2版. 北京: 国防工业出版社, 2005.WANG L L, ZHU Z X. Stress wave foundation [M]. 2nd ed. Beijing: National Defense Industry Press, 2005. [17] 陈宝心, 杨勤荣. 爆破动力学基础 [M]. 武汉: 湖北科学技术出版社, 2005.CHEN B X, YANG Q R. Basis of blasting dynamics [M]. Wuhan: Hubei Science and Technology Press, 2005. -
下载:
点击查看大图
图(7) / 表(5)
计量
- 文章访问数: 6626
- HTML全文浏览量: 2410
- PDF下载量: 37
