Elastic and Mechanical Properties of Rare Earth Boride LaB6 Crystalline Material
-
摘要: 利用密度泛函理论和Birch-Murnaghan物态方程，系统分析了LaB6晶体材料的弹性常数参数、体弹性模量、剪切弹性模量及其他力学性能。结果表明：LaB6晶体具有较大的弹性常数参数C11，说明在此主轴应力方向上具有较大的弹性常数；同时它还具有较大的体弹性模量，并且体弹性模量具有各向同性，剪切弹性模量具有各向异性；LaB6晶体的杨氏模量为227.85 GPa，泊松比为0.26，体剪弹性模量比值达到1.44，表明其脆性较强，不易发生弹性形变；LaB6晶体的硬度达到11.56 GPa，平均弹性波速达4.87 km/s。LaB6的带隙宽度为0.20 eV，呈金属性，内部电子具有较强的局域性，La和B之间具有较强的共价键成分。Abstract: The elastic constants parameters, bulk modulus, shear modulus and mechanical properties of the LaB6 crystalline material have been systematically studied with density functional theory and the Birch-Murnaghan formation. The results show that the LaB6 has larger C11, indicating its larger elastic constant along this direction. The LaB6 also has large bulk modulus, and the bulk modulus is isotropic, whereas the shear modulus is moderately anisotropic. The LaB6 crystalline material has large Young’s modulus of 227.85 GPa, so it is hard for elastic deformation. The Poisson’s ratio is 0.26 and the B/G value is 1.44, these values indicate the moderate brittleness of this material. The hardness of LaB6 is 11.56 GPa and the mean elastic velocity is 4.87 km/s. The LaB6 has narrow band gap of 0.20 eV, showing that it is metallic type material. The electrons of LaB6 have strong localization interaction, and there is covalent bond between La and B.
-
Key words:
- LaB6 /
- elastic properties /
- mechanical properties /
- elastic constant
-
空间碎片是人类航天活动产生的太空垃圾。随着航天活动的日益频繁，空间环境日趋恶化，空间碎片对航天器安全和航天员生命构成了严重的现实威胁，是空间长期可持续发展面临的严重挑战。按照目前空间碎片的增长趋势推算，在未来50年左右空间碎片密度将达到一个临界值，引发碎片间的链式撞击效应(凯斯勒效应)，之后近地空间将彻底不可用[1]。当前已经采用的空间碎片减缓、碰撞规避、防护等措施都不能从根本上遏制空间碎片数量的快速增长。为了彻底扭转空间碎片环境不断恶化的趋势，保持空间碎片环境态势的稳定，乃至达到清洁空间的目的，对空间碎片进行主动移除已经成为国际航天界的当务之急和唯一选择[2]。在众多的空间碎片主动移除技术中，激光烧蚀驱动技术具有操作简便、反应迅速、成本低、可靠性高以及可多次使用的特点，不仅能够高效移除尺寸为1~10cm的危险空间碎片，也能够防止空间碎片与航天器或碎片之间的相互撞击，可有效地减少空间碎片的数量，成为当前空间碎片主动移除的研究热点之一[2-4]。
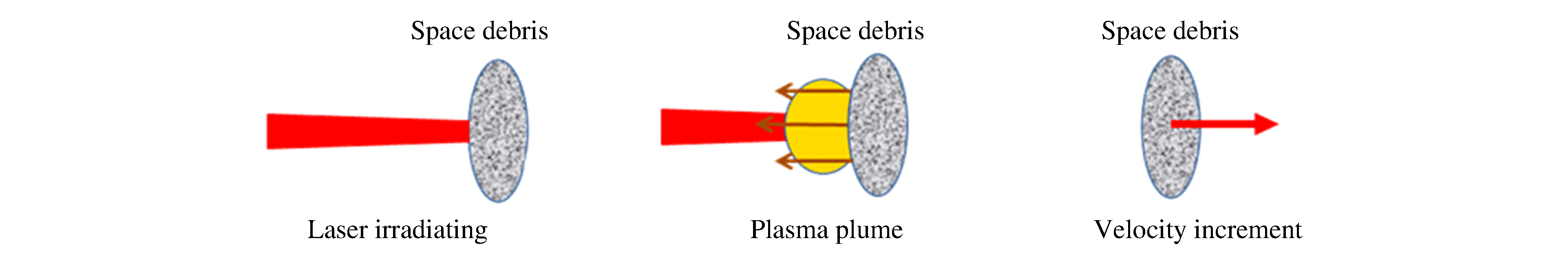
激光烧蚀驱动移除空间碎片技术的原理是：高功率激光辐照碎片表面，使光斑区材料熔化、汽化、等离子体化，高温高压等离子体和气体物质飞散形成反喷等离子体羽流，反喷羽流与空间碎片的动量交换使碎片获得一个与其相反的冲量，从而驱动碎片变轨，使低轨碎片逐步落入大气层烧毁[5]。激光烧蚀驱动碎片原理如图 1所示[2]。
目前，国内外对激光与空间碎片相互作用的冲量耦合规律和冲量耦合系数变化规律都已经进行了大量深入研究[6-7]。但这些研究主要是针对静止状态下理想的平面目标开展的，而真实的空间碎片的几何外形绝大多数都是不规则的，不规则外形会对激光辐照驱动效果造成很大影响。为了实现激光烧蚀对空间碎片的精准驱动，必须研究其不规则的几何外形对激光驱动冲量的影响。目前，由于尚缺乏不规则外形目标冲量耦合系数的实验测量数据，因此普遍采用的方法是将其离散为多个已有冲量耦合系数数据的平面目标，分别计算驱动效果后再进行累加。通过该思路金星等[8]、张品亮等[9]分别研究了覆盖式光斑和点光斑作用于立方体、圆柱体等典型几何外形目标的驱动效果。美国劳伦斯利弗莫尔国家实验室的Liedahl等[10-11]研究了激光作用于外形不规则碎片的一般计算方法，但是该方法不仅对每一特定形状目标都必须根据其外形做针对性的分析计算，而且对目标外形信息的准确度、完整度要求甚高，导致该方法使用起来非常复杂，不具备普适性和通用性，基本无法真正在工程中进行应用。
为解决这一问题，本研究提出了一种基于平面目标实验结果，通过对几何形状不规则目标进行表面三角化重构进而简化计算激光驱动效果的方法。根据复杂外形目标表面的顶点信息，基于在实验中测得的同一平面目标材料的冲量耦合系数，就可对其激光驱动效果进行精确计算。以几种典型的外形规则目标和不规则目标为例，应用该方法，研究目标几何形状对激光驱动冲量的影响规律。
1. 计算方法
理想的激光辐照烧蚀驱动平面目标的基本公式为[12]
mΔv=CmE (1) 式中：m为目标质量(单次脉冲作用过程中，烧蚀质量可忽略不计[13])，Δv为目标产生的速度增量，Cm为冲量耦合系数，E为单次激光脉冲辐照到目标表面的能量。
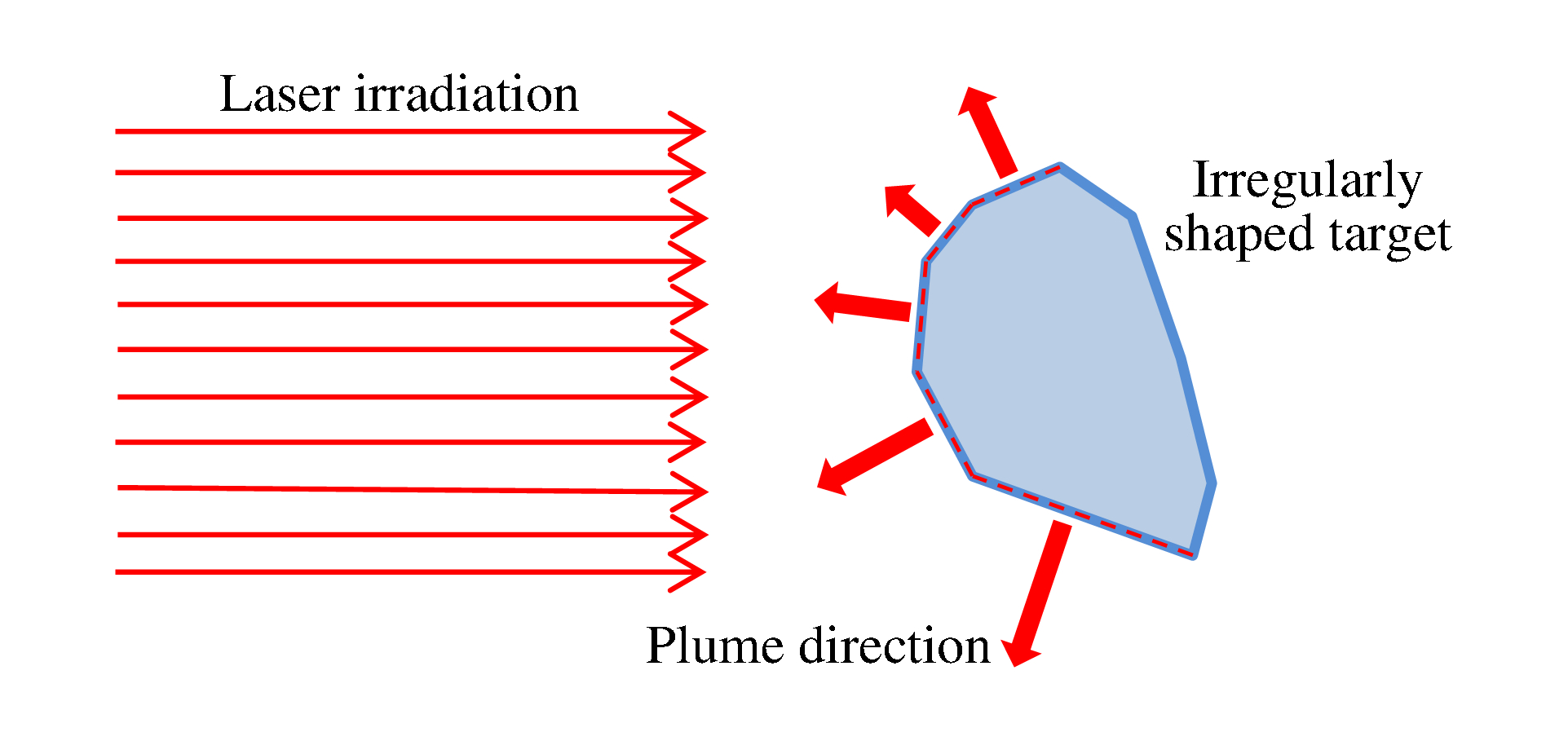
对于外形不规则目标，在计算激光烧蚀驱动行为时，首先需要分别计算每个受照射表面元的冲量，然后相加获得目标总冲量，如图 2所示。根据国内外大量的实验研究，无论激光入射方向如何，等离子体羽流喷射方向始终沿着烧蚀区域法向[10, 14], 因此，有计算公式如下[7]
mΔv=−CmI∑iSi|k⋅ni|ni (2) 
图 2 激光照射外形不规则目标Figure 2. Schematic of irregularly shaped target under laser irradiation式中：Si为第i个面元面积，k为照射激光单位向量，ni为第i个表面元法向量，I为激光能量密度。
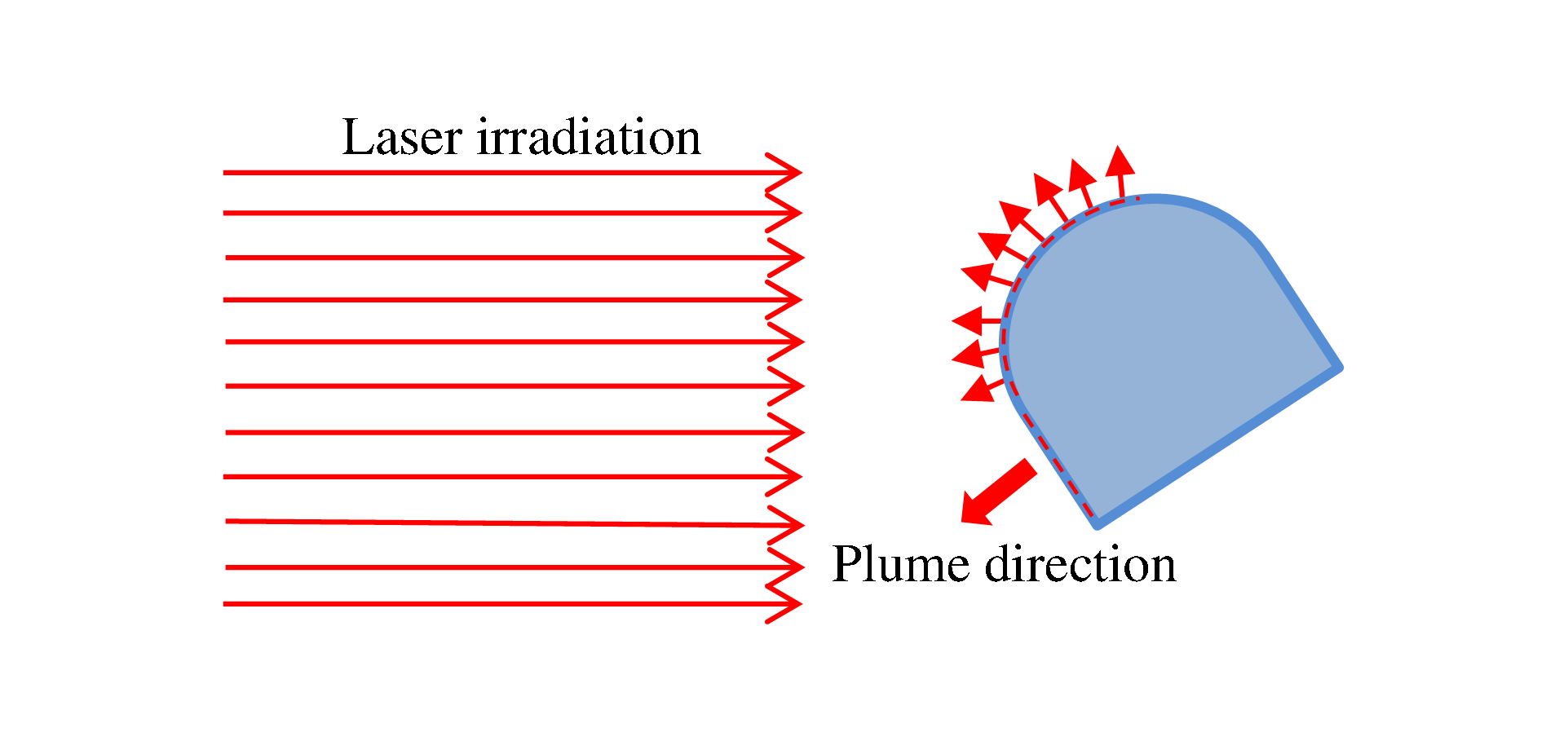
对于存在曲面的外形不规则目标，则需筛选出该曲面被照射部分区域，对其进行积分，获得该面产生的冲量，如图 3所示，计算公式如下：
(mΔv)i=−CmI∬|k⋅ni|nidS (3) 因为不规则目标表面形状各异、大小不一，且平面与曲面混杂，各表面面积和法向量难以计算获取，不规则曲面方程尤其难以得到，因而以上公式在实际中难以应用。为解决这一问题，我们提出的新思路是：根据不规则目标表面顶点坐标信息，将其表面三维重构成多个三角面组合，对每个三角面建立通用化的计算方法进行计算，最后对所有三角面进行求和。具体算法如下。
(1) 不规则目标表面三维重构
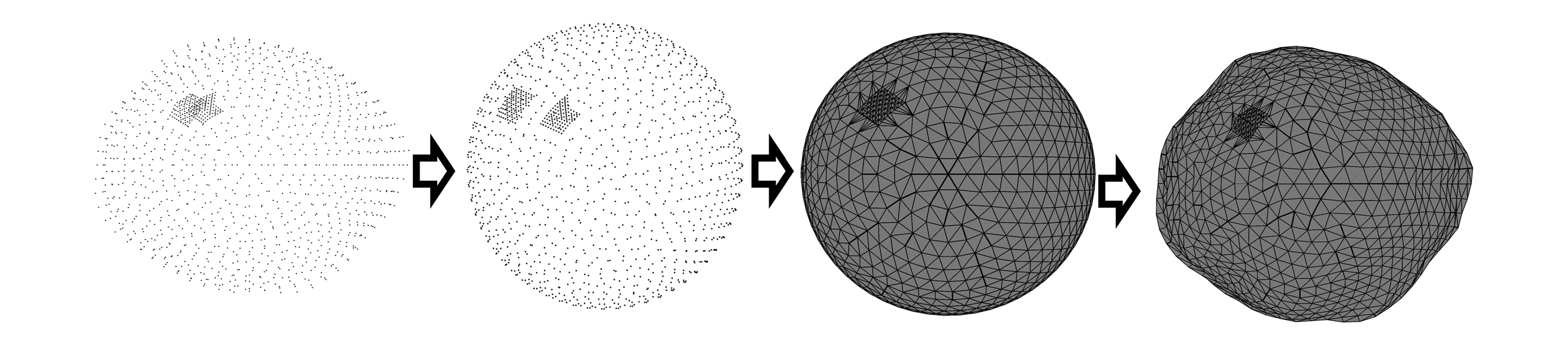
计算过程中输入的目标信息为不规则目标表面顶点信息。根据拓扑学中关于墨卡托投影Delaunay三角剖分的基本理论，对于任何一个凸多面体，经过拓扑变换后都可以等价于球形，其表面点和凸多面体的顶点一一对应，且表面顶点之间的相互位置关系并不随着拓扑变换而改变[15]。因此，对其三角化可以等效为对所对应的球面上的点进行三角化。
具体处理步骤为：第1步，将目标表面所有顶点坐标变换到以其几何中心为原点的直角坐标系下，并投影到单位球面上；第2步，筛选出单位球面的南、北极极点，以最小范围划定南、北极圈，对其内的顶点进行三角化(三角扇形)；第3步，将剩余球面通过墨卡托投影展开成二维平面，并对其内定点采用Bowyer-Watson算法进行Delaunay三角化；第4步，将获得的三角化后的点面关系信息返回到原几何体，即获得其表面三角化重构信息。这一过程如图 4所示。
图 4 三角化三维重构过程Figure 4. Schematic of three-dimensional triangulation reconstruction process(2) 基本参数计算
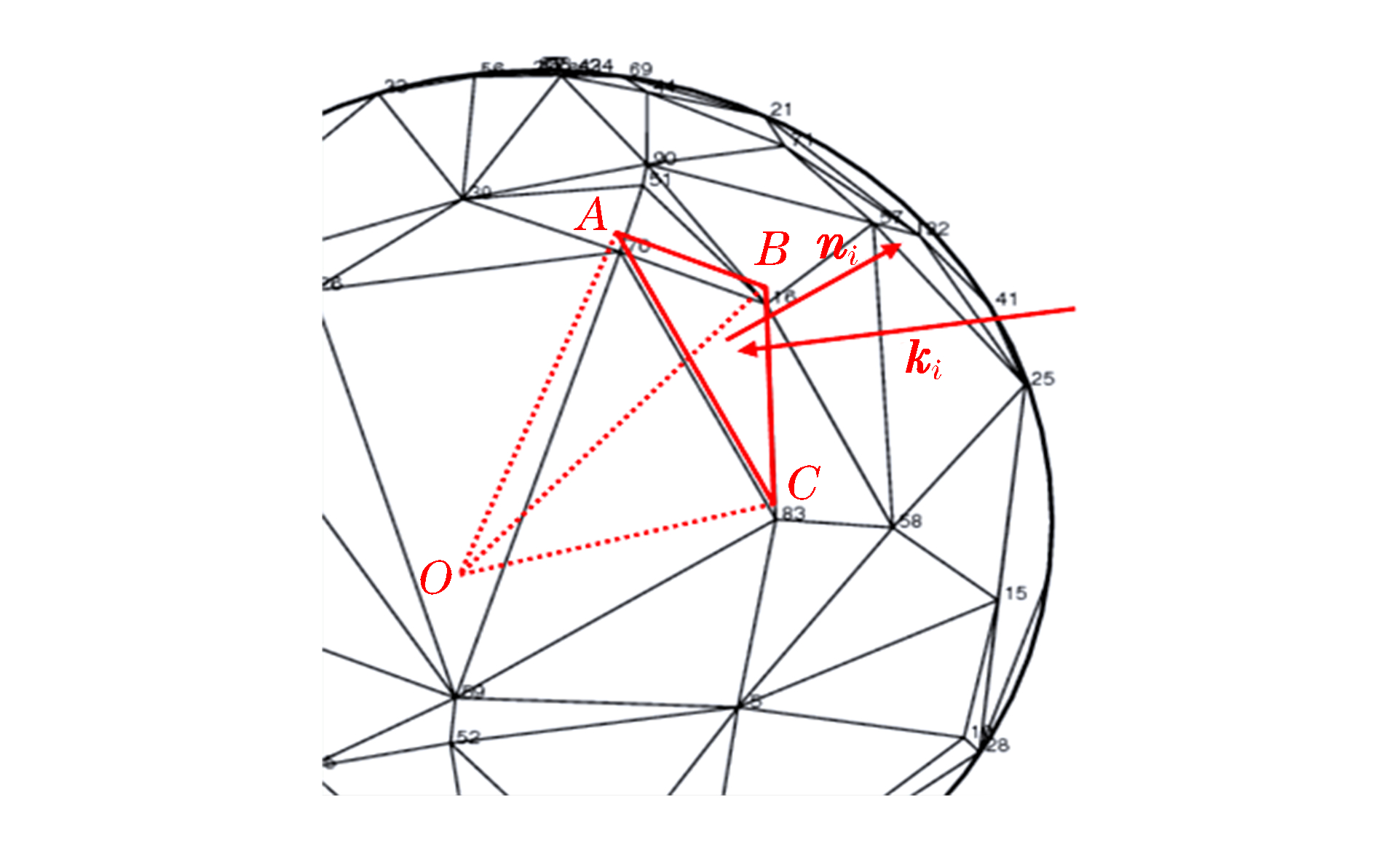
每个三角面的3个顶点和不规则目标质心构成一个四面体，将不规则目标分割为若干个四面体的组合，以每个四面体为一个基本计算单元，如图 5中四面体OABC即为基本计算单元。分别计算每个计算单元的体积、质量及针对质心的转动惯量，并通过累加获得不规则目标的总体积、质量及针对质心的转动惯量。
(3) 驱动效果计算
针对不规则目标的每个三角面，筛选出被激光照射的面，图 5中三角面ABC即为基本激光照射面，ni为该面法向量，k为入射激光向量。根据(1)式及该表面材料的冲量耦合系数实验值，计算每个被照射表面所获得的冲量，并将各表面冲量分解为沿x、y、z 3轴的冲量和以过质心分别与x、y、z 3轴平行的直线为转轴的冲量矩，进一步累加计算后获得该次激光脉冲作用后目标的速度和转动角速度改变量。
本研究提出的方法仅需获得目标表面顶点信息即可通过三维重构对激光照射驱动效果进行计算。在实际中通过雷达光学等探测手段返回的目标不规则表面信息也多为表面顶点坐标形式，因此对于各种复杂的不规则目标，其表面顶点信息较容易获得，便于工程应用。
2. 典型几何形状目标算例
将表面三角化重构计算方法编写为C语言程序，并选取立方体、球体和圆柱体3个典型几何形状目标进行计算，通过对比本研究方法计算结果与已有公式计算结果，验证该方法的准确性。
2.1 立方体
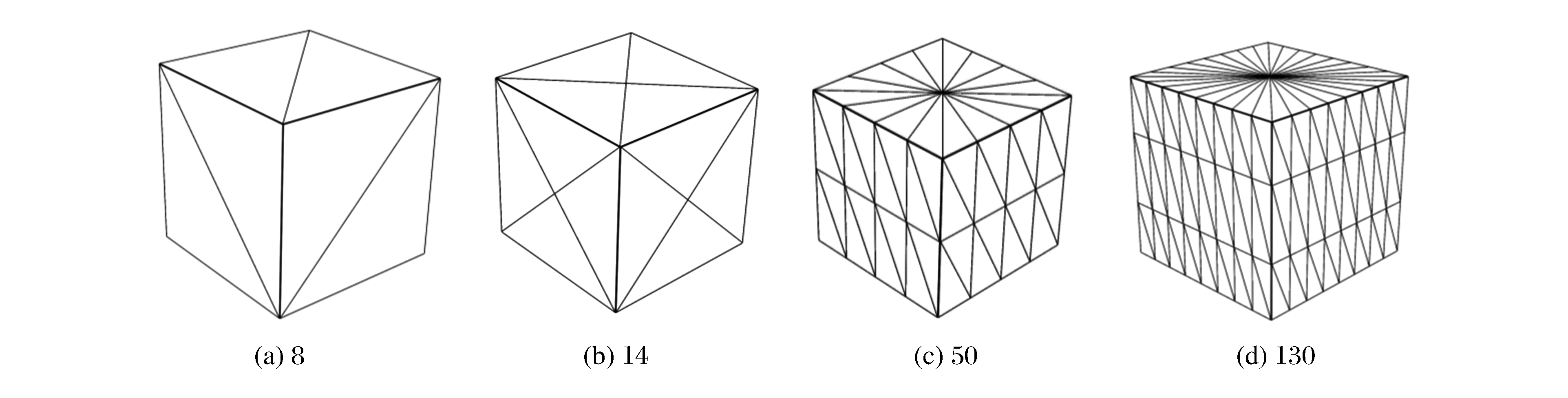
立方体因其定点明确、边界规整，可直接进行三角化，也可在其边界和表面获得插值点后进行计算，其不同三角化效果如图 6所示。
图 6 不同顶点数量下立方体三角化重构Figure 6. Triangulation reconstruction of cube with different vertex numbers对于边长为a的立方体，根据(2)式有
mΔv=−CmIa2∑i|k⋅ni|ni (4) 具体到三维直角坐标系中各边分别与3个坐标轴平行的立方体，在受到单位矢量为(cos θ sin α, cos θ cos α, sin θ)、能量密度为I的激光覆盖照射时，单次脉冲所获得冲量为
{Px=cosθsinαCmIa2Py=cosθsinαCmIa2Pz=sinθCmIa2 (5) 以密度为2.7g/cm3的典型铝合金材料为例，其冲量耦合系数为0.000 06N·s·J-1。对于边长为1cm的铝合金立方体，当其受到方向矢量为(-1，-1，-1)、到靶表面能量密度为5.7J/cm2的激光覆盖照射时，其公式计算结果与三角化计算结果对比如表 1所示。
表 1 不同顶点数量下立方体三角化计算结果Table 1. results of cube with different vertex numbersVertex
numberMass/
gMoment of inertia/
(10-4g·m2)Impulse/
(mN·s)Velocity/
(mm·s-1)Angular velocity/
(rad·s-1)Jx Jy Jz Px Py Pz Δvx Δvy Δvz ωx ωy ωz 8 2.7 1.31 1.31 1.31 1.96 1.96 1.96 -2.493 -2.493 -2.493 0 0 0 14 2.7 1.31 1.31 1.31 1.96 1.96 1.96 -2.493 -2.493 -2.493 0 0 0 50 2.7 1.31 1.31 1.31 1.96 1.96 1.96 -2.493 -2.493 -2.493 0 0 0 130 2.7 1.31 1.31 1.31 1.96 1.96 1.96 -2.493 -2.493 -2.493 0 0 0 Formula 2.7 1.31 1.31 1.31 1.96 1.96 1.96 -2.493 -2.493 -2.493 0 0 0 由表 1中计算结果可看出，对于立方体，三角化计算结果与公式计算的结果完全一致，且不受插值点数量和三角面划分的影响。
2.2 球体
因球体表面都是曲面并没有顶点，所以需先对其表面插值获得顶点信息才能通过三角化进行计算，不同插值点数量下三角化如图 7所示。
图 7 不同顶点数量下球体三角化Figure 7. Triangulation reconstruction of sphere with different vertex numbers对于半径为R的球体，根据(3)式有
mΔv=23CmI π R2k (6) 对密度为2.7g/cm3、半径为2.5cm的铝合金球体，冲量耦合系数为0.000 06N·s·J-1，当其受到方向矢量为(-1，-1，-1)、到靶表面能量密度为5.7J/cm2的激光覆盖照射时，其公式计算结果与三角化计算结果对比如表 2所示。
表 2 不同顶点数量下球体三角化计算结果Table 2. Calculation results of sphere with different vertex numbersVertex
numberMass/
gMoment of inertia/
(g·m2)Impulse/
(mN·s)Velocity/
(mm·s-1)Angular velocity/
(10-7rad·s-1)Jx Jy Jz Px Py Pz Δvx Δvy Δvz ωx ωy ωz 72 158.926 603 1 0.109 0.109 0.105 2.693 2.691 2.534 5.820 5.820 5.477 2 220 121 10 200 222 170.834 427 5 0.122 0.122 0.121 2.744 2.746 2.715 5.518 5.521 5.459 6.74 6.98 3 790 382 172.723 740 5 0.124 0.124 0.123 2.766 2.766 2.718 5.501 5.500 5.406 2.22 4.95 3 250 762 174.891 641 2 0.126 0.126 0.126 2.769 2.768 2.764 5.438 5.437 5.429 2.02 5.55 2 820 1 452 175.755 229 0 0.127 0.127 0.127 2.775 2.775 2.771 5.425 5.424 5.415 1.71 3.79 217 2 452 176.064 045 8 0.128 0.128 0.128 2.779 2.779 2.773 5.422 5.422 5.407 1.42 3.46 192 Formula 176.625 0.129 0.129 0.129 2.780 2.780 2.780 5.406 5.406 5.406 0 0 0 从表 2中可看出，当在球表面插值72点(经线8点、纬线10点)时，计算结果与公式结果有较大偏差。几何体参数方面：如图 7(a)所示，在球表插值72点划分方法下，三角化重构的几何体与球体差距较大，导致计算所得球体质量小于公式结果，相对偏差为10.02%；由于丢失的质量都位于球体表面附近，进一步导致了更大的转动惯量损失，对x(y)轴转动惯量的相对偏差为15.50%，对z轴转动惯量的相对偏差为18.61%；且由于经线和纬线插值点数目不同以及z轴与x、y轴投影方向表面三角化划分方式不同，导致z轴和其他两轴的参数值不同。激光作用效果方面：由于球体表面插值72点三角化重构的表面与原球面的偏差，使激光作用点位置和法向量角度均发生改变，导致计算所得冲量也与公式计算结果不同，x(y)轴方向冲量的相对偏差为3.13%，z轴方向冲量的相对偏差为8.85%；x(y)轴方向速度增量相对偏差为7.66%，z轴方向速度增量相对偏差为1.31%。
对同一球体采用更密集的插值点划分方式：222点(经线13点、纬线20点，见图 7(b))、382点(经线21点、纬线20点，见图 7(c))、762点(经线21点、纬线40点，见图 7(d))、1 452点(经线31点、纬线50点，见图 7(e))、2 452点(经线51点、纬线50点, 见图 7(f))。
从表 2中计算结果可看出，插值点的加密显著降低了与公式计算结果的偏差，随着插值点数的增加，偏差缩小。2 452个插值点时：质量相对偏差为0.32%；转动惯量相对偏差(x、y、z轴)均为0.78%；x(y)轴冲量的相对偏差为0.36%, z轴冲量的相对偏差为0.25%；x(y)速度的相对偏差为0.30%，z轴速度的相对偏差为0.02%。可见，当插值点达到一定数量时，三角化计算值已与公式计算值十分接近。
据此可看出，在对球体采用本研究方法计算时，插值点数量对计算精度有较大影响。插值点过少时，粗糙的三角化将导致较大的计算偏差，插值点的增加能显著提高计算精度。
2.3 圆柱体
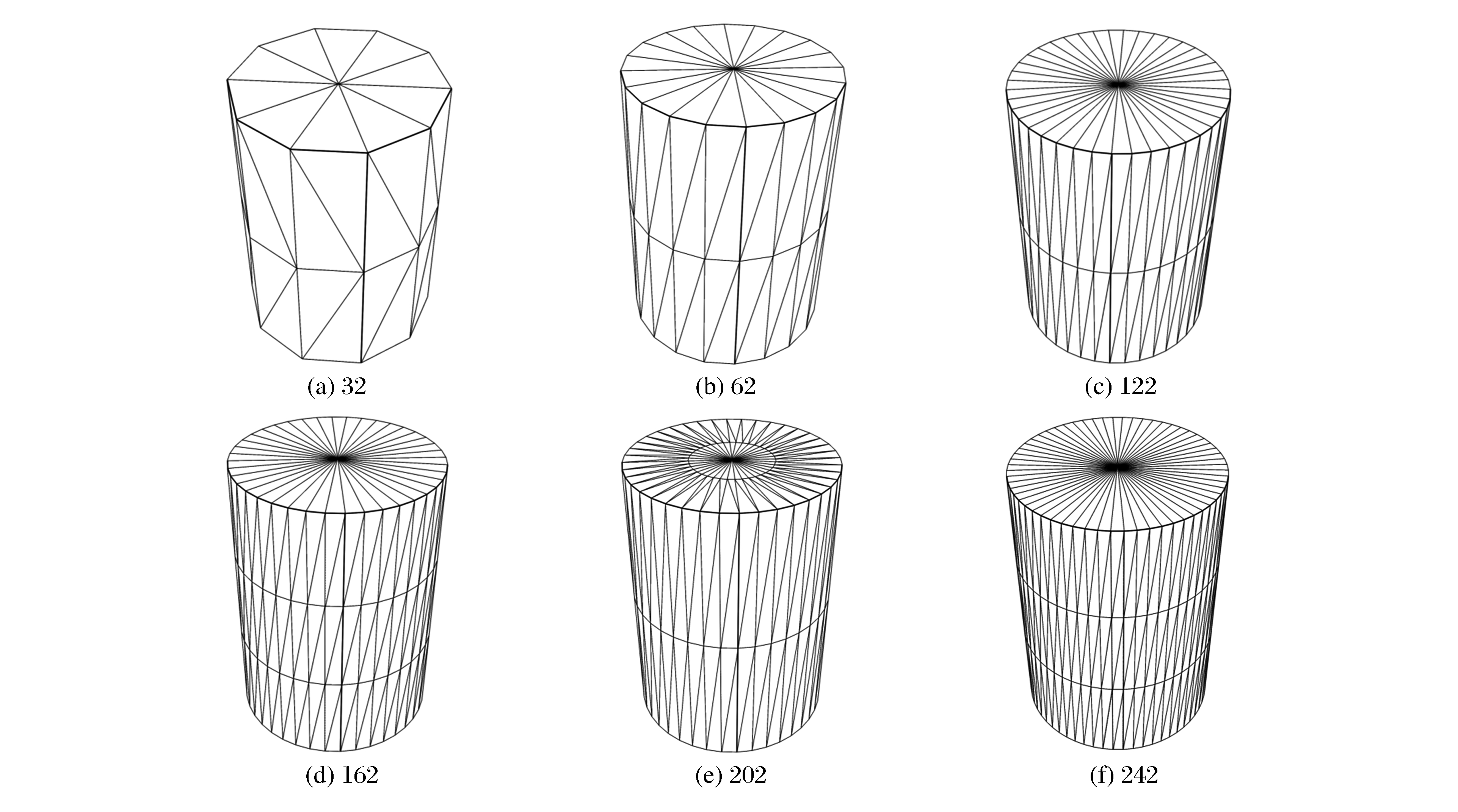
因圆柱体侧面是曲面并没有顶点，所以需先对其底圆和母线插值获得顶点信息才能通过三角化重构进行计算，不同插值点数量下三角化如图 8所示。
图 8 不同顶点数量下圆柱体三角化Figure 8. Triangulation reconstruction of cylinder with different vertex numbers对半径为R、高为H的圆柱体，受到单位矢量为(cos θsin α, cos θcos α, sin θ)、能量密度为I的激光覆盖照射时，根据(2)式、(3)式有
{Px= π 2sinαcosθCmIHRPy= π 2cosαcosθCmIHRPz=sinθCmI π R2 (7) 对底面半径为2.5 cm、高为7 cm的铝合金圆柱体，冲量耦合系数为0.000 06 N·s·J-1，当其受到方向矢量为(-1，-1，-1)、到靶表面能量密度为5.7 J/cm2的激光覆盖照射时，其公式计算结果与三角化计算结果对比如表 3所示。
表 3 不同顶点数量下圆柱体三角化计算结果Table 3. Calculation results of cylinder with different vertex numbersVertex
numberMass/
gMoment of inertia/
(g·m2)Impulse/
(mN·s)Velocity/
(mm·s-1)Angular velocity/
(10-8rad·s-1)Jx Jy Jz Px Py Pz Δvx Δvy Δvz ωx ωy ωz 32 347.290 1 0.560 5 0.560 5 0.295 7 5.305 5.302 3.603 5.249 5.249 3.565 3.72×10-4 4.75×10-4 2.52 62 365.152 7 0.597 2 0.597 2 0.326 7 5.369 5.369 3.788 5.053 5.053 3.565 1.21×10-8 5.23×10-8 2.50 122 369.618 3 0.606 7 0.606 7 0.334 8 5.386 5.386 3.835 5.006 5.006 3.565 3.52×10-16 2.76×10-16 2.39 162 369.618 3 0.606 7 0.606 7 0.334 8 5.386 5.386 3.835 5.006 5.006 3.565 3.52×10-16 2.75×10-16 2.39 202 369.618 3 0.606 7 0.606 7 0.334 8 5.386 5.386 3.835 5.006 5.006 3.565 3.52×10-16 2.78×10-16 2.39 242 370.305 3 0.608 5 0.608 5 0.336 4 5.388 5.388 3.844 4.997 4.997 3.565 2.01×10-16 1.92×10-16 2.40 Formula 370.992 4 0.609 9 0.609 9 0.337 6 5.389 5.389 3.849 4.988 4.988 3.563 0 0 0 从表 3中结果可看出，当在圆柱表面插值32点(底圆10点、母线3点)时，计算结果与已有公式结果有一定差距。几何体参数方面：如图 8(a)所示，该划分方法下，三角化重构的几何体与圆柱体差距较大，导致计算所得圆柱体质量小于公式结果，相对偏差为6.39%；进而导致了更大的转动惯量损失，x、y轴转动惯量的相对偏差为8.10%, z轴转动惯量的相对偏差为12.41%。激光作用效果方面：由于重构后底面与圆柱体底圆差距过大，一方面导致三角化重构表面与原圆柱体侧面曲面产生偏差，使激光作用点位置和法向量角度均发生改变；另一方面导致底面受辐照面积减小，使计算所得x、y、z轴冲量均与公式计算结果不同，x、y轴方向冲量的相对偏差为1.56%、z轴方向冲量的相对偏差为6.39%。在x、y轴方向，由于质量和冲量两者的共同偏差，导致速度增量出现5.23%的相对偏差；z轴方向则由于三角化导致的激光辐照表面损失和质量损失等比例，圆柱体三角化后的面质比保持不变，因而该方向速度相对偏差很小，为0.056%。
对同一圆柱体采用更密集的插值点划分方式：62点(底圆20点、母线3点，见图 8(b))、122点(底圆40点、母线3点，见图 8(c))、162点(底圆40点、母线4点，见图 8(d))、202点(底圆80点、母线3点，见图 8(e))、242点(底圆60点、母线4点，见图 8(f))。其中，62点、122点方案均为细化底圆曲线上的插值，从表 3中可看出，随着插值点的增加，计算精度显著提高。162点和202点分别细化了母线上和底圆平面中的插值，计算结果与122点时完全一致，说明对直线插值点加密以及对平面三角化的细化无法提高计算精度，误差来源于曲线和曲面。242点是在162点的基础上进一步加密了底圆的插值点，偏差进一步缩小：质量相对偏差为0.19%；x、y轴转动惯量相对偏差为0.23%，z轴转动惯量相对偏差为0.36%；x、y轴冲量相对偏差为0.020%，z轴冲量相对偏差为0.13%；x、y轴速度相对偏差为0.18%，z轴速度相对偏差为0.056%。
2.4 讨论
根据以上对立方体、圆柱体、球体计算结果的对比和分析，可见：(1)对于立方体这类直线棱划分的平面包裹的几何体，三角化重构计算方法准确度很高，其结果与公式计算结果完全一致; (2)直线(如立方体的棱、圆柱的母线)上插值点的划分密度对计算结果没有影响，可直接采用几何体天然顶点; (3)对于圆柱体侧面和球体表面等曲面的三角化精度和曲线上插值点的密度会大大影响计算精度，曲线上插值点越密集，曲面三角化后表面越精确，计算结果越准确。
在实际应用中，不规则形状目标的曲面和曲线信息往往是未知的，无法像以上规则几何体一样根据顶点信息和曲线方程进行插值加密，其计算精度就取决于初始获得的目标表面顶点信息。初始获得的目标表面顶点越多、越密集、越准确，三角化重构出来的目标表面就越接近目标真实情况，在此基础上进行的激光驱动计算结果也就越精确。
通过该方法还可以反过来研究激光烧蚀驱动碎片技术中对目标探测系统精度的要求。首先，选取一个已知准确外形信息且包含复杂表面细节的不规则几何体，通过三角化计算获得精确的作用效果。然后，通过逐渐删除顶点逐渐减少信息的完整度和准确度来模拟探测精度下降对目标细节的丢失，并依次将计算结果与精确结果对比，获得不同精度下的误差变化规律。最后，根据碎片移除、主动防御的不同操控精度需求可获得不同的目标信息精度需求，进而提出对探测系统的不同精度要求。
3. 激光辐照外形规则目标产生的冲量规律
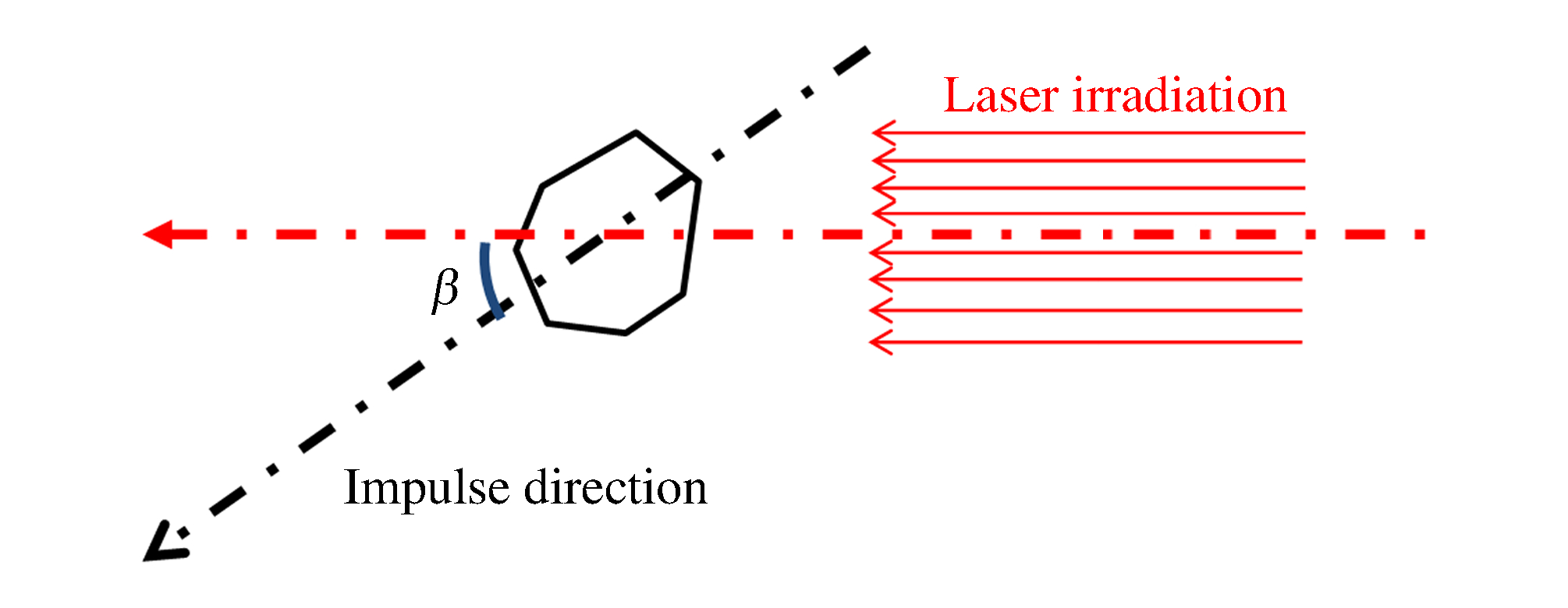
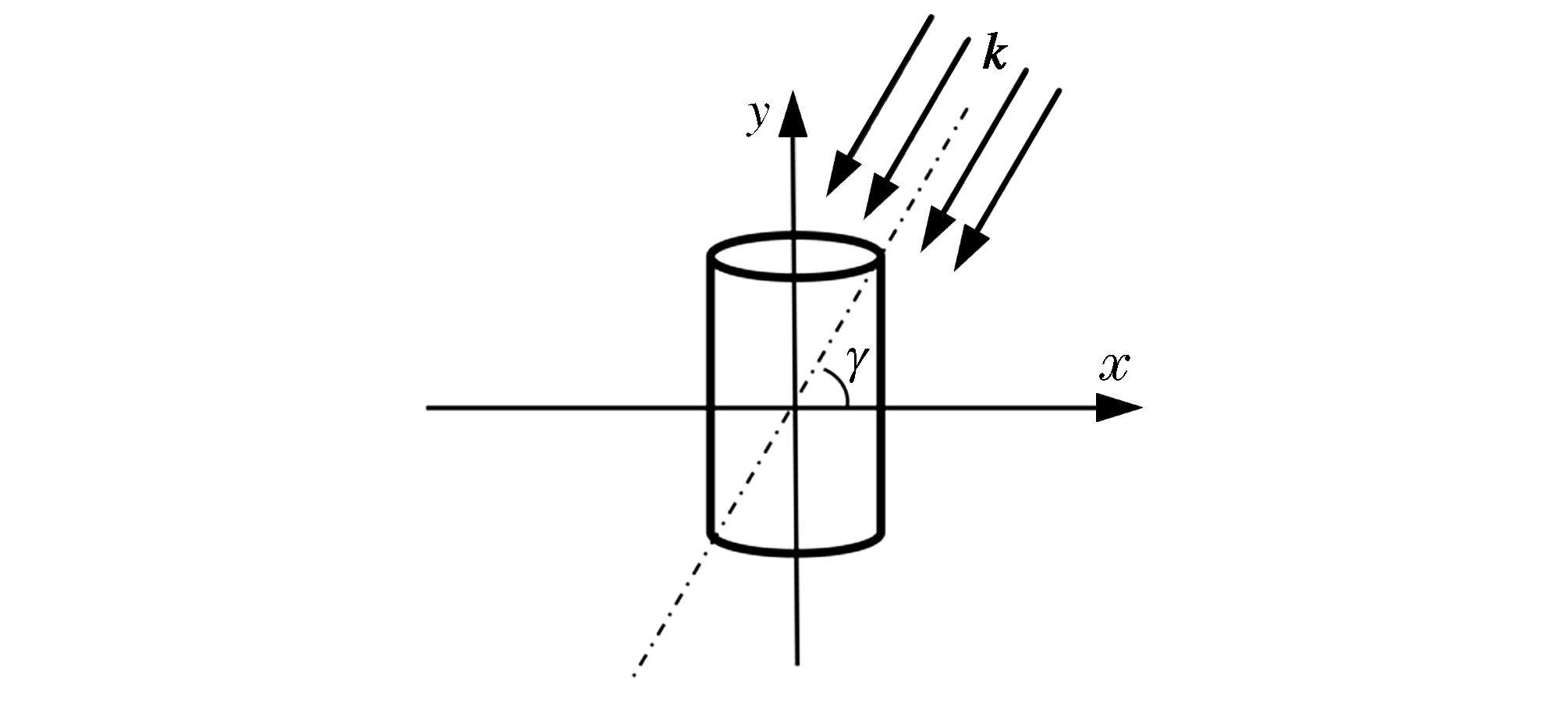
在激光辐照驱动目标过程中，一般关注的驱动效果主要有两个：一是驱动产生冲量的方向，二是驱动产生冲量的大小。一般期待的冲量方向为沿激光辐照方向，而目标外形会导致实际冲量方向偏离激光照射方向，并使沿激光辐照方向的冲量减小。因此对目标外形因素导致的冲量方向与激光方向的偏角β (见图 9)和目标外形对沿激光辐照方向冲量大小的影响进行研究。
3.1 立方体
根据(5)式可知，单位矢量为(cos θsin α, cos θcos α, sin θ)的激光覆盖照射立方体时冲量方向矢量为(cos θsin α, cos θcos α, sin θ)，与作用激光矢量一致，因此其几何外形不影响激光照射后产生冲量的方向。
立方体沿激光作用方向的合冲量大小始终为CmIa2，等于其单个表面接收到同强度激光垂直照射时获得的冲量大小。
3.2 球体
由于球体的对称性，任意角度激光覆盖式辐照方向必然过球心，所以其冲量方向始终和激光照射方向相同，其几何外形不影响激光照射后产生的冲量方向。
球体沿激光作用方向的合冲量大小始终为\frac{2}{3}{C_{\rm{m}}}I{\rm{ \mathsf{ π} }}{R^2}，等于相同半径平面目标受到同等强度激光垂直照射时获得的冲量大小的2/3。
3.3 圆柱体
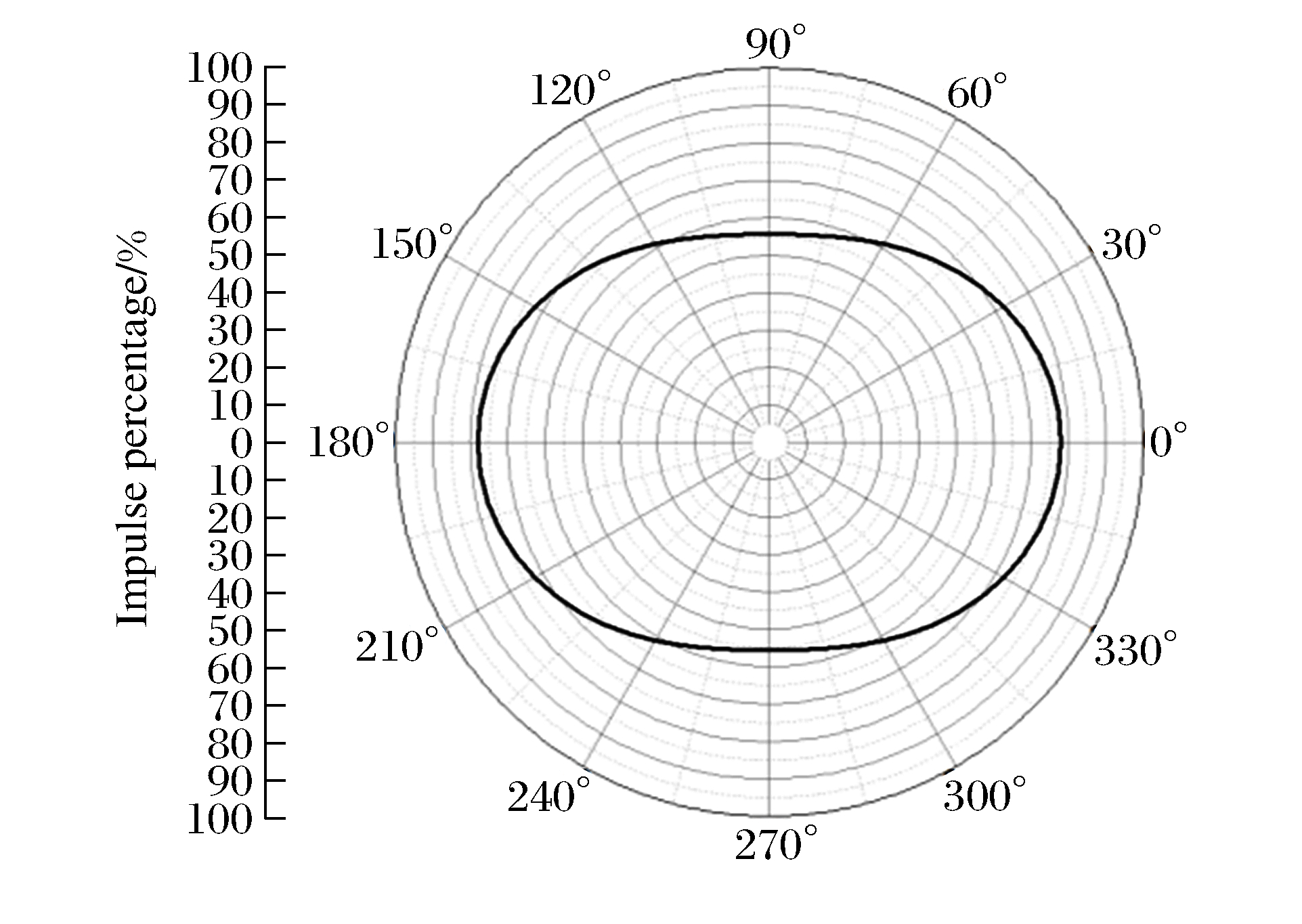
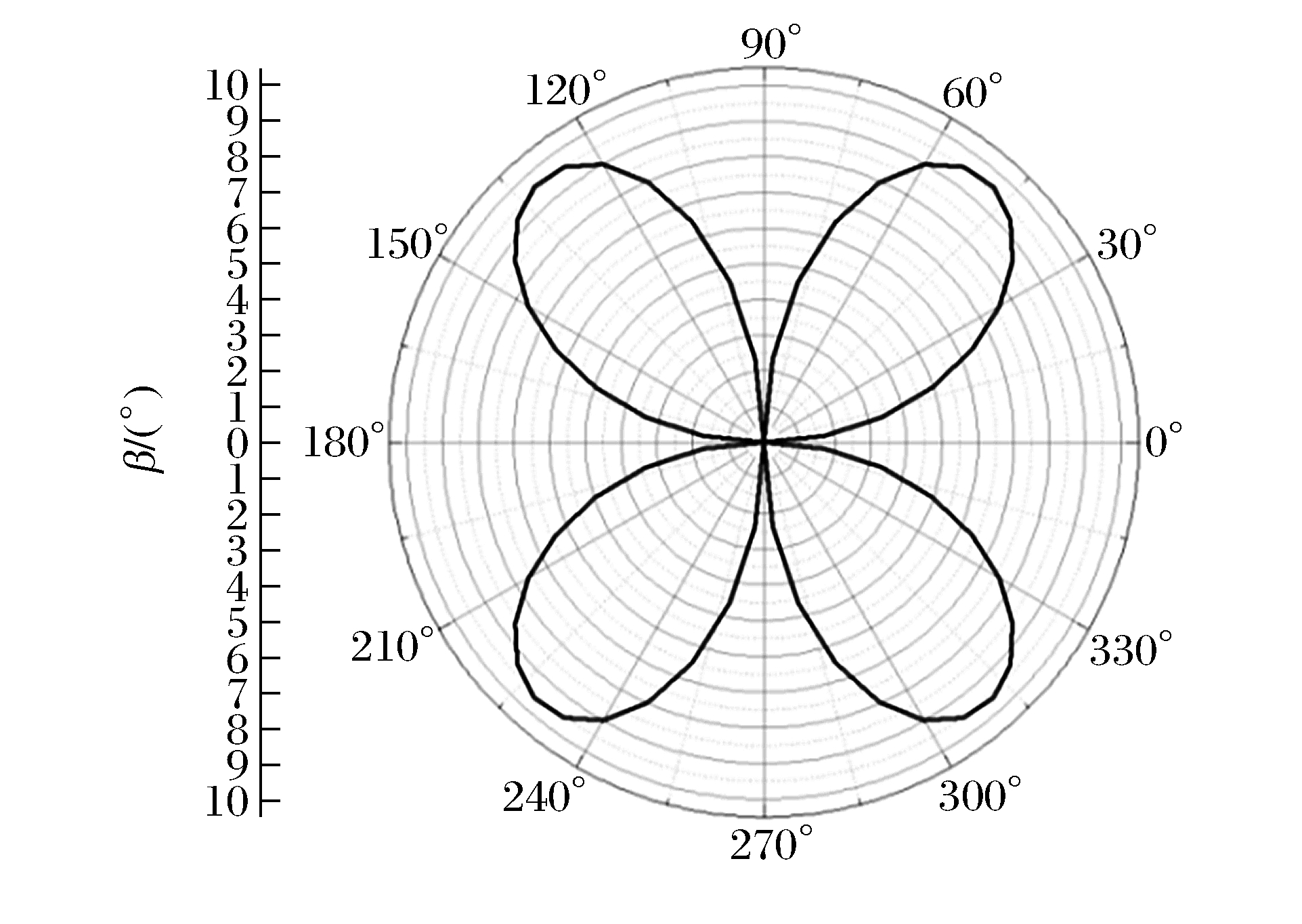
激光照射方向与x轴的夹角为γ，如图 10所示。计算得到不同γ下沿激光方向的冲量占激光照射同截面平面目标冲量的比例如图 11所示，不同γ下冲量与激光方向的偏角β如图 12所示。
图 11 圆柱体在不同角度激光照射下产生的冲量占平面目标冲量的比例Figure 11. Impulse percentage of cylinder to planner target irradiated by laser at different angles
图 12 圆柱体在不同角度激光照射下产生的冲量偏角Figure 12. Impulse angle of cylinder irradiated by laser at different angles从图 11中可看出：激光从正侧面照射圆柱体时冲量损失比例为22%，冲量损失最小；激光从顶部和底部照射时冲量损失比例为为44%，冲量损失最大。从图 12中可看出，激光从圆柱体对称轴方向入射时冲量偏角为0°，离对称轴越远冲量偏角越大，最大为9.6°。综合两者结果，从圆柱体侧边垂直方向照射能在获得最大冲量的同时使冲量方向与激光方向一致。
3.4 圆锥体
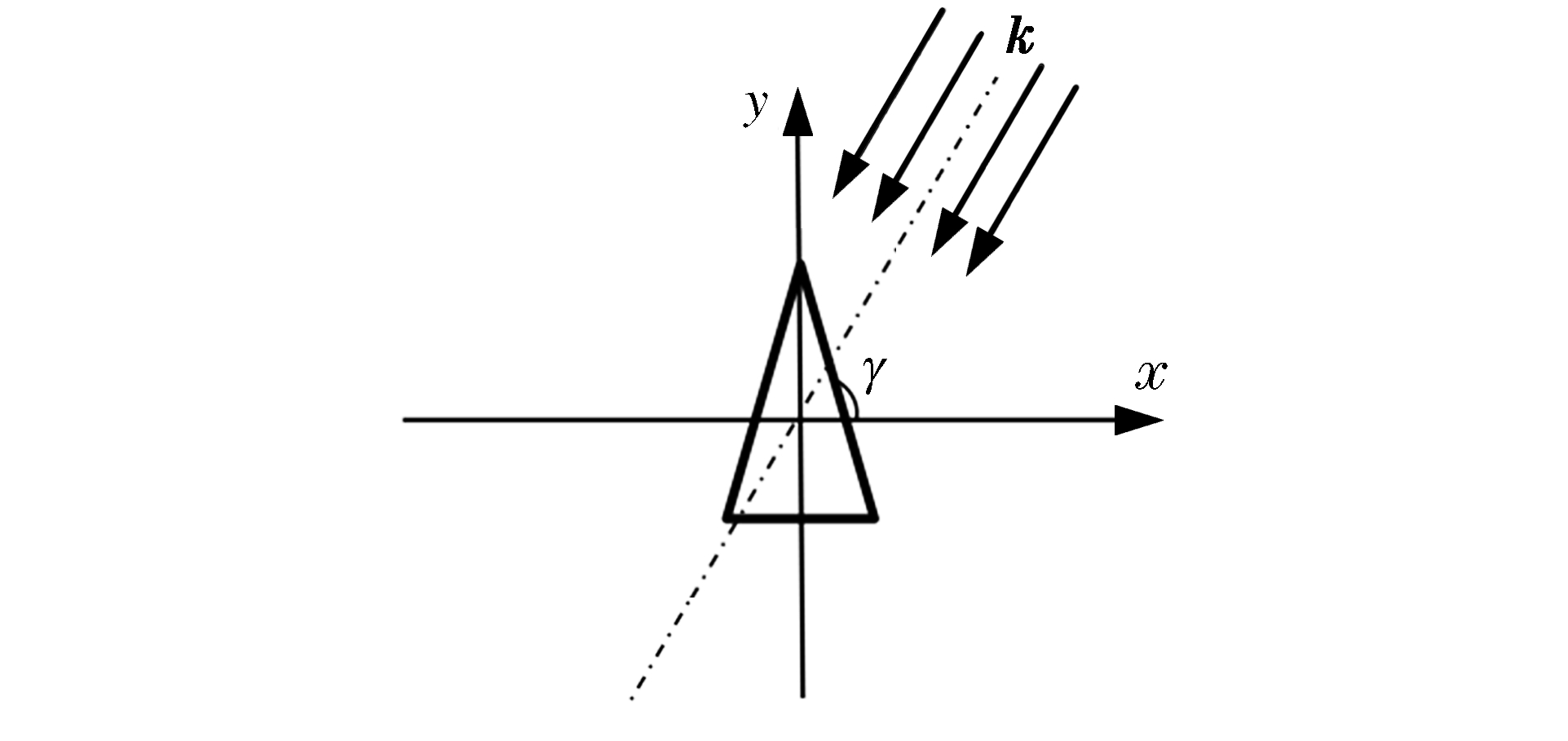
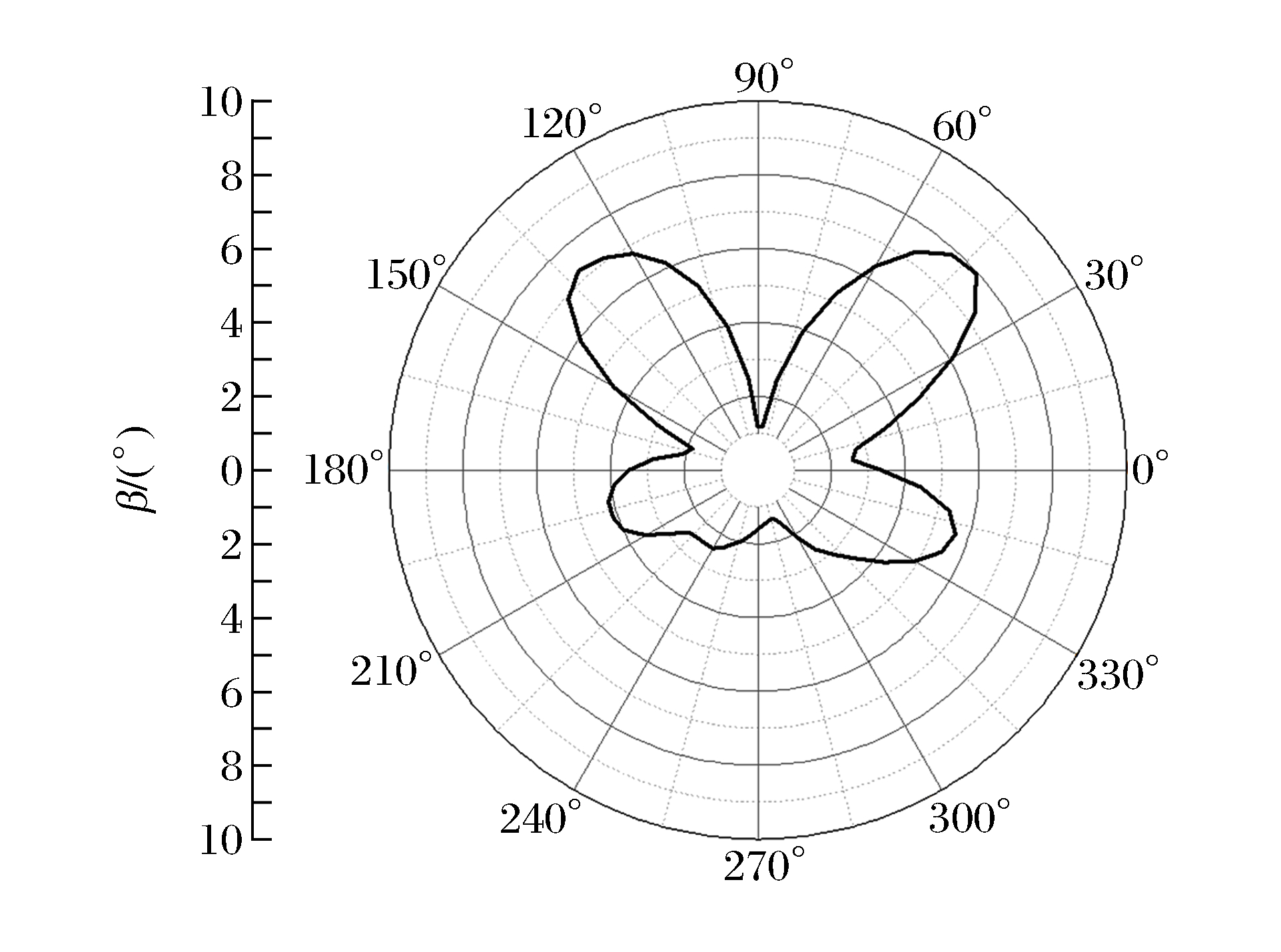
对高10 cm、底面半径2.5 cm的圆锥体，激光照射方向与x轴夹角为γ，如图 13所示。计算得到不同γ下沿激光方向的冲量占激光照射同截面平面目标冲量的比例如图 14所示，不同γ下产生的冲量与激光方向的偏角β如图 15所示。
图 14 圆锥体在不同角度激光照射下产生的冲量占平面目标冲量的比例Figure 14. Impulse percentage of cone to planner target irradiated by laser at different angles
图 15 圆锥体在不同角度激光照射下产生的冲量偏角Figure 15. Impulse angle of cone irradiated by laser at different angles从图 14中可看出：激光从圆锥底部和侧边方向入射时冲量损失比例均较小，分别为22%和16%；激光从圆锥顶点方向入射时冲量损失比例最大，为80%。从图 15中可看出：激光除了从对称轴方向入射以外，从垂直于侧面方向和底角所对方向入射时也有两个偏角接近0°极小值；在两个侧面方向偏角最大，为48.1°。综合两者结果，从圆锥体底面垂直方向照射能在获得最大冲量的同时使冲量方向与激光方向一致。
4. 激光辐照外形不规则目标产生的冲量规律
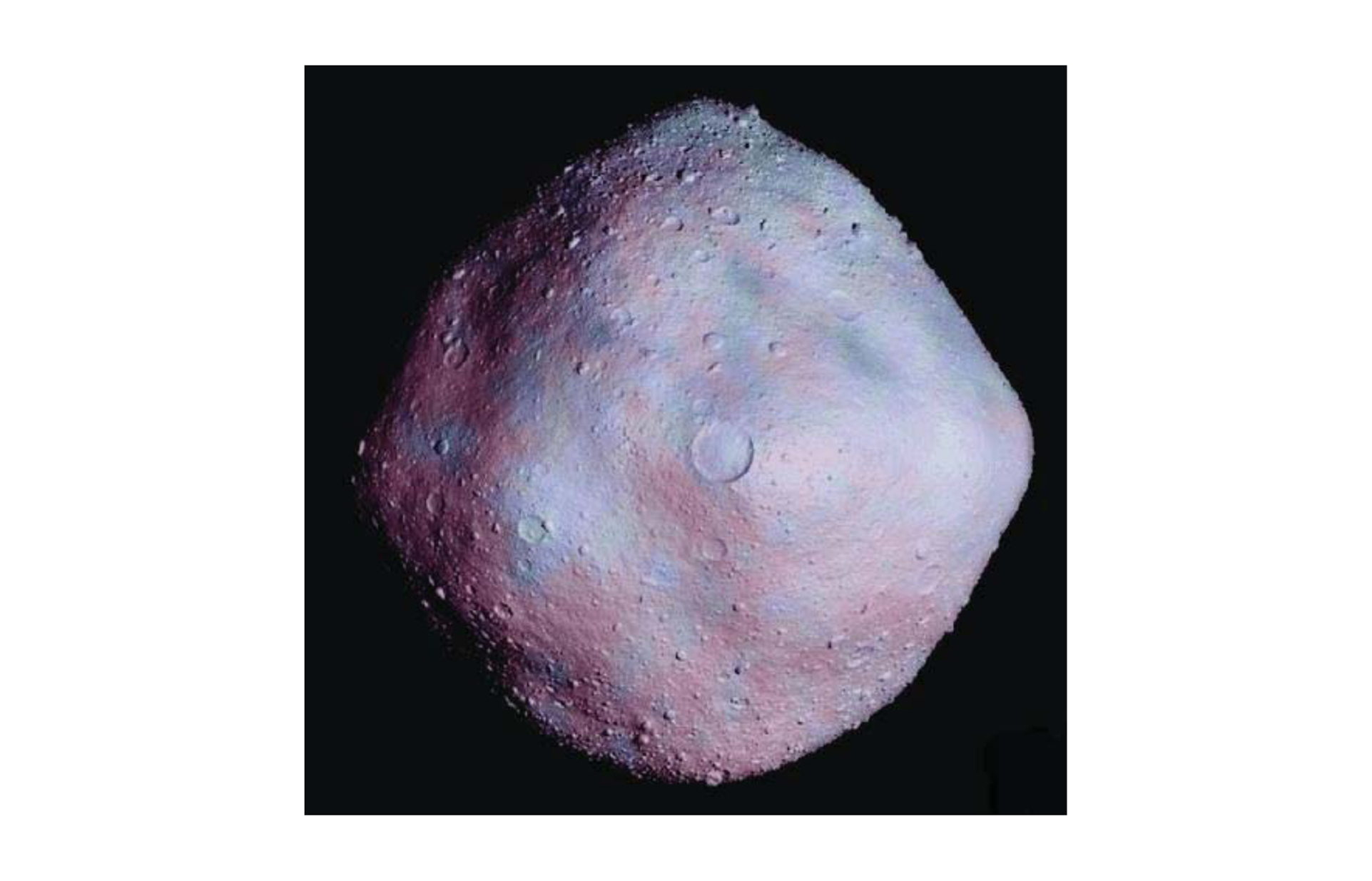
这里以小行星为例。外形不规则目标选择编号为101955的小行星贝努，如图 16所示，是一个典型的外形不规则目标。该小行星发现于1999年9月，NASA对其化学、物理和运动学特性进行了大量的测量，获得了丰富的数据，并于2016年9月8日发射了“奥西里斯”探测器，计划在2019年与其交会期间对其进行环绕探测和接触采样。贝努是一颗对地球有威胁的“潜在危险天体”(Potentially Hazardous Asteroid，PHA)，最小轨道交会距离约0.002 AU(1 AU=1.495 978 7×1011 m)，其撞击地球的危险性目前排在PHA第2位(在2182年)。激光烧蚀驱动是抵御小行星撞击地球的可能手段之一，因此，以贝努为算例除了可以检验本研究所提方法外，同时也有重要的实际价值。
贝努的平均直径为492 m，赤道尺寸为565 m×535 m，自旋周期为4.297 d(角速度1.69×10-5 rad/s)。从NASA网站上的小行星数据库可获得包含其顶点坐标信息的几何外形数据[16]，其三角化后结果如图 17所示。
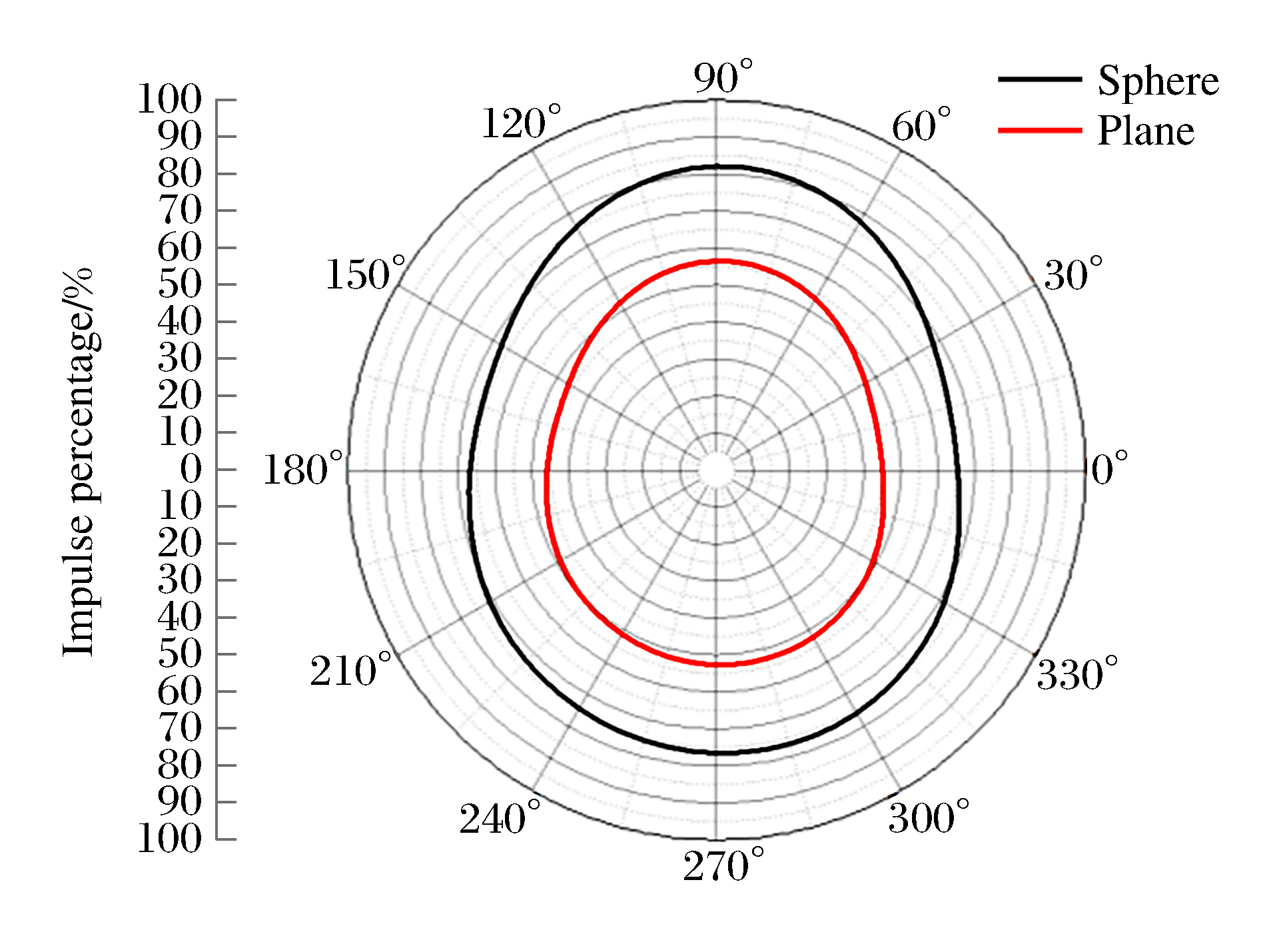
贝努为碳质小行星，表面存在富含碳元素的风化层，因此取其冲量耦合系数为0.000 086 N·s·J-1，据估算贝努密度为1.181 g/cm3。选取激光方向矢量为(-1，-1，-1)，到靶表面能量密度为5.7 J/cm2。在其坐标xy平面内改变激光照射角度γ, 计算得到不同γ下沿激光辐照方向的冲量占激光照射同截面平面目标及同半径球体冲量的比例如图 18所示，不同γ下冲量与激光方向的偏角β如图 19所示。
图 18 贝努在不同角度激光照射下产生的冲量占平面/球体目标的比例Figure 18. Impulse percentage of Bennu to plane/sphere target irradiated by laser at different angles
图 19 贝努在不同角度激光照射下产生的冲量偏角Figure 19. Impulse angle of Bennu irradiated by laser at different angles根据结果可知：由于贝努近似一个扁球体，不同角度激光辐照下产生的冲量大小受外形影响较小，在90°照射角附近冲量损失最小，相比同直径规则球体损失了17%，相比同截面平面目标损失了43%；其外形对冲量偏角的绝对值影响较小，最大值为8°，但不同方向上差别较大，在90°和180°附近冲量偏角达到最小值。
5. 结论
基于目标表面三角化三维重构提出了一种可以精确计算激光辐照外形不规则目标产生的冲量大小及方向的方法，以立方体、球体和圆柱体3个典型的外形规则目标为对象，验证了方法的计算精度。利用该方法研究了激光辐照典型外形规则和不规则目标产生的冲量规律，结果显示：对于立方体和球体，由于其自身的对称性，其外形对激光辐照产生的冲量方向无影响，但是会导致冲量大小发生改变；对于圆柱体和圆锥体，其外形对激光辐照产生的冲量大小和方向均有影响，影响大小随激光入射角度的不同而改变，在特定入射角度下，圆柱体和圆锥体均能在不影响激光作用冲量方向的情况下获得最大冲量。对于小行星“贝努”这种类似球体的不规则目标，其外形会使激光作用冲量的大小产生较大损失，而对冲量方向的影响相对较小，但受照射角度影响明显。这一方法及研究结论对深化激光烧蚀驱动移除空间碎片技术及其工程应用有重要价值。
-
图 2 LaB6晶体材料的能带结构和分态密度
Figure 2. Band structure and partial density of states for the LaB6 crystalline material
表 1 LaB6晶体材料的晶格参数
Table 1. Structural parameters of LaB6 crystalline material
Method a/nm b/nm c/nm α/(°) β/(°) γ/(°) Experiment 0.415 49 0.415 49 0.415 49 90 90 90 Calculation 0.420 205 0.420 205 0.420 205 90 90 90 
下载: 导出CSV
表 2 LaB6晶体材料的体弹性模量和剪切弹性模量
Table 2. Bulk modulus and shear modulus of LaB6 crystalline material
BV/GPa BR/GPa BH/GPa GV/GPa GR/GPa GH/GPa 160.55 160.55 160.55 111.49 68.85 90.17
下载: 导出CSV
表 3 LaB6晶体材料的力学性能参数和弹性波速
Table 3. Mechanical parameters and elastic velocities of LaB6 crystalline material
E/GPa γ λ AB AG H/GPa vl/(km·s–1) vt/(km·s–1) vm/(km·s–1) 227.85 0.26 1.44 0 0.24 11.56 7.72 4.38 4.87
下载: 导出CSV
表 4 LaB6晶体材料的电荷转移
Table 4. Charge distributions of LaB6 crystalline material
Atom Charge distribution/e s orbital p orbital d orbital Total charge B 0.88 2.54 0.00 –0.42 La 1.50 5.46 1.51 2.53
下载: 导出CSV
-
[1] LAFFERTY J M. Boride cathodes [J]. Journal of Applied Physics, 1951, 22(3): 299–309. doi: 10.1063/1.1699946 [2] HOSSAIN F M, RILEY D P, MURCH G E. Ab initio calculations of the electronic structure and bonding characteristics of LaB6 [J]. Physical Review B, 2005, 72(23): 235101. doi: 10.1103/PhysRevB.72.235101 [3] CHEN C H, AIZAWA T, IYI N, et al. Structural refinement and thermal expansion of hexaborides [J]. Journal of Alloys and Compounds, 2004, 366(1/2): L6–L8. [4] 张宁, 张玖兴, 包黎红. 悬浮区域熔炼法制备REB6(LaB6、CeB6)单晶体及其表征 [J]. 功能材料, 2012, 43(2): 178–180 doi: 10.3969/j.issn.1007-4252.2012.02.016ZHANG N, ZHANG J X, BAO L H, et al. Floating zone growth and characterization of single crystal REB3 (LaB6, CeB6) cathode [J]. Chinese Journal of Functional Materials, 2012, 43(2): 178–180 doi: 10.3969/j.issn.1007-4252.2012.02.016 [5] 包黎红, 那仁格日乐, 特古斯, 等. 放电等离子烧结原位合成La xCe1- xB6化合物及性能研究 [J]. 物理学报, 2013, 62: 196105 doi: 10.7498/aps.62.196105BAO L H, NARENGERILE, TEGUS O, et al. Synthesis and properties of LaxCe1–xB6 compounds by in-situ spark plasma sintering [J]. Acta Physica Sinica, 2013, 62: 196105 doi: 10.7498/aps.62.196105 [6] 刘洪亮, 张忻, 王杨, 等. 单晶LaB6阴极材料典型晶面的电子结构和发射性能研究 [J]. 物理学报, 2018, 67: 048101 doi: 10.7498/aps.67.20172187LIU H L, ZHANG X, WANG Y, et al. Surface electronic structures and emission property of single crystal LaB6 typical surfaces [J]. Acta Physica Sinica, 2018, 67: 048101 doi: 10.7498/aps.67.20172187 [7] 高瑞兰, 于化顺, 于普涟, 等. LaB6多晶材料的制备工艺研究 [J]. 山东大学学报(工学版), 2002, 32(6): 593–596 doi: 10.3969/j.issn.1672-3961.2002.06.024GAO R L, YU H S, YU P L, et al. Preparation of LaB6 polycrystalline materials [J]. Journal of Shandong University (Engineering Science), 2002, 32(6): 593–596 doi: 10.3969/j.issn.1672-3961.2002.06.024 [8] NYE J F. Physical properties of crystals: their representation by tensors and matrices [M]. Oxford: Oxford University Press, 1957. [9] HILL R. The elastic behaviour of a crystalline aggregate [J]. Proceedings of the Physical Society Section A, 1952, 65(5): 349. doi: 10.1088/0370-1298/65/5/307 [10] TIAN Y, XU B, ZHAO Z. Microscopic theory of hardness and design of novel superhard crystals [J]. International Journal of Refractory Metals and Hard Materials, 2012, 33: 93–106. doi: 10.1016/j.ijrmhm.2012.02.021 [11] BORN M, HUANG K. Dynamical theory of crystal lattices [M]. Oxford: Clarendon Press, 1968. [12] HAINES J, LEGER J M, BOCQUILLON G. Synthesis and design of superhard materials [J]. Annual Review of Materials Research, 2001, 31(1): 1–23. doi: 10.1146/annurev.matsci.31.1.1 [13] PANDA K B, CHANDRAN K S R. Determination of elastic constants of titanium diboride (TiB2) from first principles using FLAPW implementation of the density functional theory [J]. Computational Materials Science, 2006, 35(2): 134–150. doi: 10.1016/j.commatsci.2005.03.012 [14] ANDERSON O L. A simplified method for calculating the Debye temperature from elastic constants [J]. Journal of Physics and Chemistry of Solids, 1963, 24(7): 909–917. doi: 10.1016/0022-3697(63)90067-2 -
下载:
下载:
点击查看大图
计量
- 文章访问数: 9685
- HTML全文浏览量: 4466
- PDF下载量: 38
