Strain Rate Effect of GFRP-Reinforced Circular Steel Tube under Low-Velocity Impact
-
摘要: 讨论了玻璃纤维/环氧树脂复合材料(Glass Fiber Reinforced Plastic,GFRP)增强Q235圆钢管在低速冲击荷载作用下的应变率效应。通过轴压试验和轴向低速冲击试验获得了试件在准静态和低速冲击状态下的力学响应(轴向荷载及轴向位移),为后续仿真工作提供了依据。编写了可以考虑初始失效、损伤演化及应变率效应的GFRP材料子程序(VUMAT),并基于ABAQUS对构件的轴压及轴向冲击过程进行了仿真再现。通过仿真将不考虑应变率效应、只考虑钢管应变率效应、只考虑GFRP应变率效应、考虑钢管及GFRP应变率效应4种情况下的结果进行了对比分析。
-
关键词:
- 应变率效应 /
- 钢管 /
- 玻璃纤维/环氧树脂复合材料 /
- 低速冲击试验 /
- 有限元模拟
Abstract: This paper discussed the strain rate effect of glass fiber/epoxy resin composite (GFRP)-reinforced Q235 circular steel tube under low-velocity impact load. Firstly, the responses (axial load and displacement) of GFRP-reinforced steel tube under quasi-static and low-velocity impact load were obtained through axial compression tests and low-velocity impact tests respectively, which provided gist for the subsequent simulation. Secondly, a VUMAT subroutine considered initial failure modes, damage evolution and strain rate effect of GFRP was introduced; then the axial compression and low-velocity impact processes were simulated. Finally, the results under 4 situations (ignoring strain rate effect, considering the strain rate effect of steel tube, considering the strain rate effect of GFRP and considering the strain rate effect of both steel tube and GFRP) was compared using simulation. -
红砂岩和石灰岩是比较常见的岩土材料,广泛地分布于我国各大山脉。红砂岩和石灰岩可以作为抵御武器破坏的天然防护屏障,因此研究其在压缩过程中的破坏效应(如裂纹扩展、崩落等)对于弹丸对岩石靶板的侵彻、防御工事的修筑、地下深井的开挖设计等都有重要的现实意义。石灰岩作为大陆的标志性岩石,其动、静态性能的研究成果已相当丰富,近年来不仅发现了损伤后石灰岩单轴再加载的力学特性[1],而且证明了加载速率对三轴压缩时岩石抗压峰值应力的影响显著[2]。此外,岩石的尺寸效应对力学性能的影响研究也日新月异。杜晶[3]根据能量耗散规律,从能量理论的角度对不同长径比的岩石特性机理进行了描述;高富强等[4]、洪亮等[5]研究了加载条件和尺寸效应对岩石抗压性能的耦合影响,得到了动态尺寸效应占主导地位的临界加载速度;平琦等[6]对相同直径、不同长度的石灰岩试件在高应变率下的抗压强度进行了探讨;陈思顺[7]利用正交实验,研究了加载速率、均质度、垫片材质对岩石在单轴压缩下力学特性的影响。上述研究探讨了影响岩石动、静态压缩特性的多种因素,并且结论以大量试验为基础,在针对某一种岩石时可信度较高,然而当试验范围跨越不同种类的岩石时,基于上述结论对结果进行分析难免会产生误差。在岩石的破坏模式方面,Hoerth等[8]通过飞片撞击试验,分析了在高动态加载下应力波在砂岩内部的传播过程;Baranowski等[9]基于JH-2本构模型,通过落锤冲击试验与数值模拟,确定了对岩石断裂影响最显著的参数;高阳等[10]利用声发射监测技术,得到了含有预裂纹的石灰岩在准静态破碎过程中裂纹的演化规律;张盛等[11]采用半圆盘石灰岩试样的三点弯曲断裂试验,考察了试样预裂纹对断裂韧度值的影响。此外,还有很多学者通过系统研究,建立了描述裂纹的断裂损伤模型,但是由于多种原因,在对岩石介质的抗侵彻分析中尚未用到上述结论。
综上所述,不同种类岩石在动态和静态压缩下,不同加载率下裂纹形成及破坏模式有相当大的差异,这种差异会对岩石介质的抗侵彻性能产生显著影响,然而这方面的相关报道很少。为此,本研究拟对相同尺寸的不同岩石试件进行准静态和动态压缩试验,对其破坏模式进行归纳总结,为进一步开展岩石在不同受压状态下的破坏失效模式研究、不同岩石介质的抗侵彻性能研究奠定基础。
1. 岩石材料的力学性能测试及对比
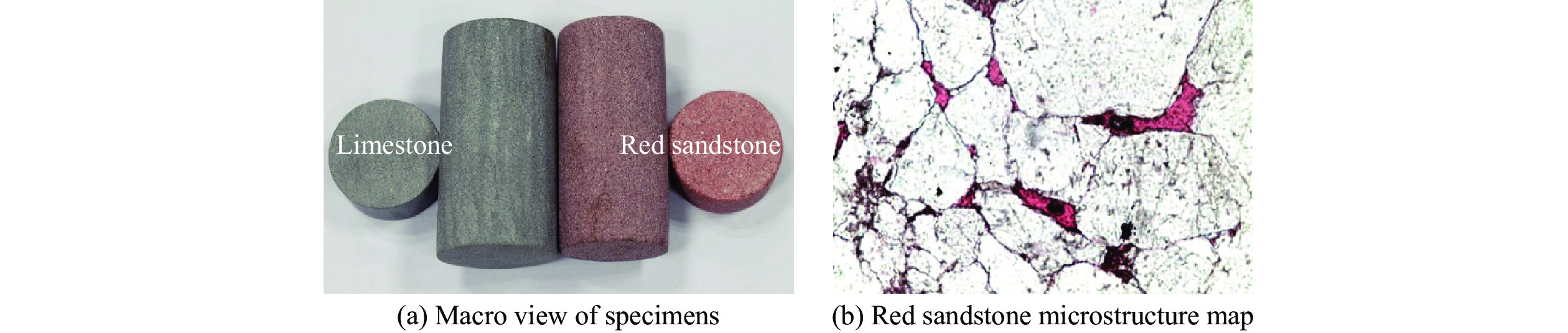
本试验所采用的红砂岩外表呈暗红色,石灰岩呈青灰色。两种岩石均使用钻孔取样机从完整岩块部位取芯,经切割、打磨加工后形成试样。试样表面无破损,无明显裂纹,颗粒分布均匀。根据程浩[12]、冯春迪等[13]的研究,红砂岩的粒径约为0.1 mm,石灰岩粒径在0.01~0.10 mm之间。试件及其微观孔隙、裂纹如图1所示。石灰岩的密度为2600 kg/m3,红砂岩的密度为2460 kg/m3[14-15]。
1.1 准静态压缩试验
采用CSS44300型电子万能材料试验机,分别对两种岩石试样开展两种应变率下的准静态压缩试验,采用高速摄影机记录岩石试件被压溃时的裂纹扩展模式,试验布局如图2所示。
根据标准[16]的要求和杜晶[3]的研究,并考虑万能材料试验机的最大载荷,设计准静态压缩试件的尺寸为
∅40mm×80mm 。设L为试件长度,˙ε 为名义应变率,v为试验机横梁的压缩速度,则˙ε=vL (1) 根据式(1),设定v分别为0.96和9.60 mm/min,计算得到压缩时试件的
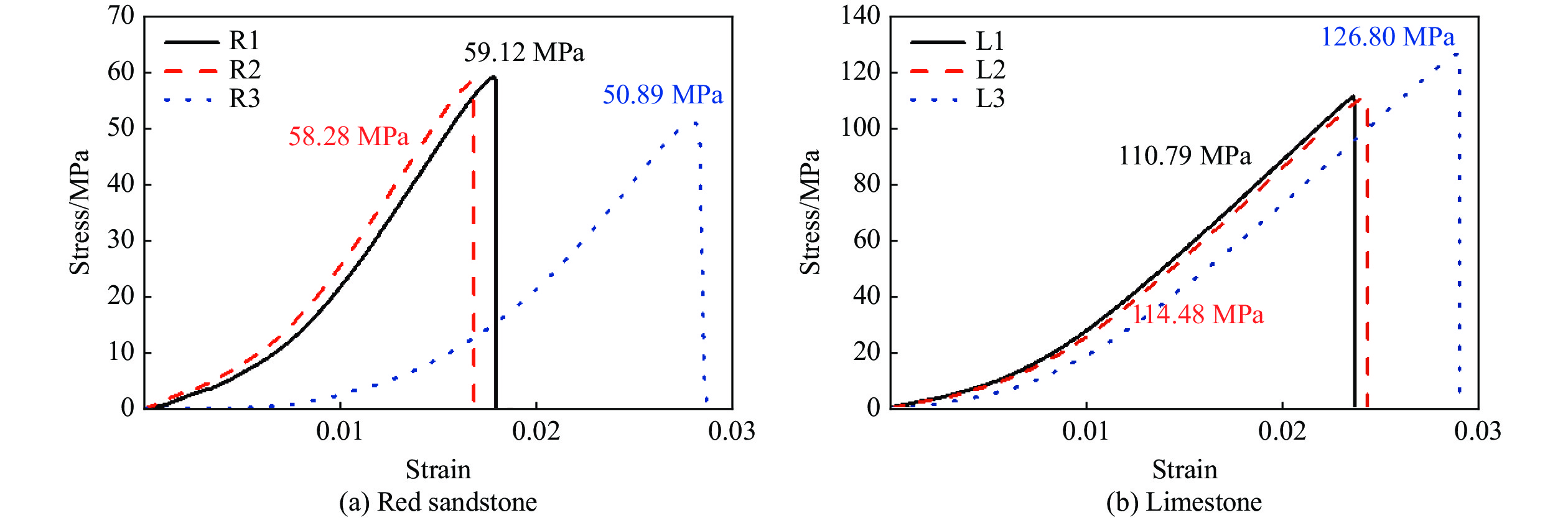
˙ε 分别为2 × 10−4和2 × 10−3 s−1。红砂岩试件R1、R2以及石灰石试件L1、L2对应的应变率为2 × 10−4 s−1,红砂岩试件R3和石灰石试件L3对应的应变率为2 × 10−3 s−1。设
s 为真实应力,e 为真实应变,则s=FA=FA0(1−ε)=σ(1−ε) (2) e=ln(l0l)=ln(11−ε) (3) 式中:F为试验机的加载力,A和A0为岩石试件的初始截面积和瞬时截面积,
σ 为工程应力,ε 为工程应变,l 和l0 为岩石试件的初始长度与瞬时长度。根据式(2)和式(3),可以得到红砂岩和石灰岩试样在两种应变率下的真实应力-应变曲线,如图3所示。由图3可知,红砂岩和石灰岩试件的准静态单轴压缩试验过程可分为4个阶段:压实阶段、弹性阶段、屈服阶段、破坏阶段。试件的破坏模式如图4所示,可以很明显地看出试件以剪切破坏为主,破坏模式为单面剪切。
1.2 霍普金森杆动态压缩试验
为了保证岩石试件的均匀性,参考文献[17-18]的研究,并且考虑压杆直径因素,确定试件的尺寸为
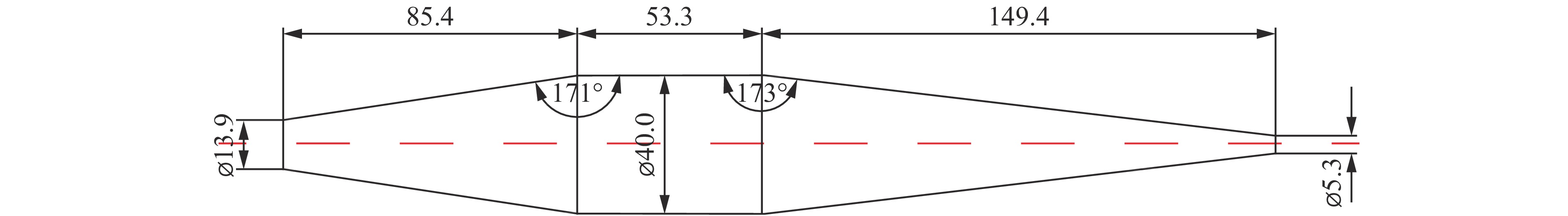
∅40mm×20mm 。由于岩石试件的尺寸相对较大,采用与入射杆等截面的圆柱形子弹时,很难保证应力波在试件中传播到达峰值之前达到平衡,因此需要增大应力波的上升段坡度。为了尽可能保证多次试验的一致性,选择如图5所示的纺锤形子弹。图6显示了整形后的入射波形。原先具有前驱振荡的方波变为半正弦波。此外,试验过程中使用高速摄影机在垂直于杆轴向的方向记录岩石试件的破坏模式。当子弹撞击入射杆时触发高速摄影机,通过控制软件Pcc2.6控制触发记录时间,确保记录到试件的变形和破坏模式。
为了获得不同应变率下的应力-应变曲线,动态压缩试验分组如表1所示。
表 1 动态压缩试验分组Table 1. Runs of dynamic compression testsRock material Test No. Total number of trials Bullet speed/(m·s−1) Rock material Test No. Total number of trials Bullet speed/(m·s−1) Red sandstone H-1 3 14.8 Limestone S-1 3 16.7 H-2 3 16.5 S-2 3 18.6 根据一维弹性应力波假设,有如下公式
σs(t)=AbE2As[εi(XG1,t)+εr(XG1,t)+εt(XG2,t)] εs(t)=C0lS∫t0[εi(XG1,t)−εt(XG2,t)−εr(XG1,t)]dt 式中:
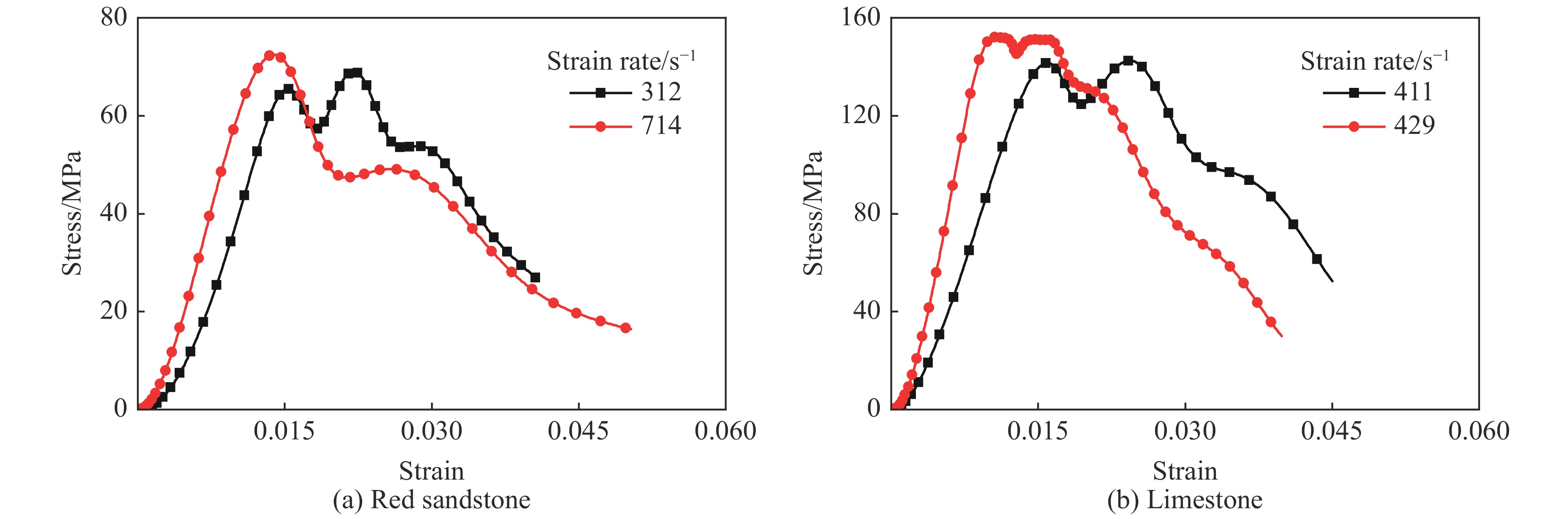
Ab 为入射杆和透射杆的横截面积,Ab =1256mm2 ;E为杆材料高硬铝的弹性模量,E =71GPa ;C0 为杆材料的纵波波速,C0 = 5100 m/s;As 为岩石试件的横截面积,As = 1256 mm2;lS 为试样的长度,lS =20mm ;t 为动态压缩试验中应力波加载时间;εi(XG1,t) 、εr(XG1,t) 、εt(XG2,t) 分别为入射应变、反射应变和透射应变,XG1 和XG2 分别为入射杆和透射杆上所贴应变片传回的超动态应变仪电压信号强度。结合超动态应变仪读取的数据,计算得到红砂岩和石灰岩在高应变率下的应力-应变曲线,如图7所示。需要说明的是,红砂岩和石灰岩的动态压缩试验在两种不同的气缸压力下各重复3组,最终选取红砂岩和石灰岩重复性较好的有效数据各两组。2. 破坏模式分析
岩样在不同加载速率下的破坏模式不同。在准静态单轴压缩条件下,岩石呈现出剪切破坏现象。学者们利用分离式霍普金森压杆(SHPB)装置进行了大量的岩石动态压缩试验[19-22],总结出3种典型的破坏模式:拉应力破坏、剪切破坏和张应变破坏。在实际操作中,上述3种破坏模式往往不会单独出现,因为岩样中或多或少存在微裂纹,而微裂纹在应力作用下成核、扩张的过程会对岩样的破坏模式起到决定性作用。
2.1 准静态压缩
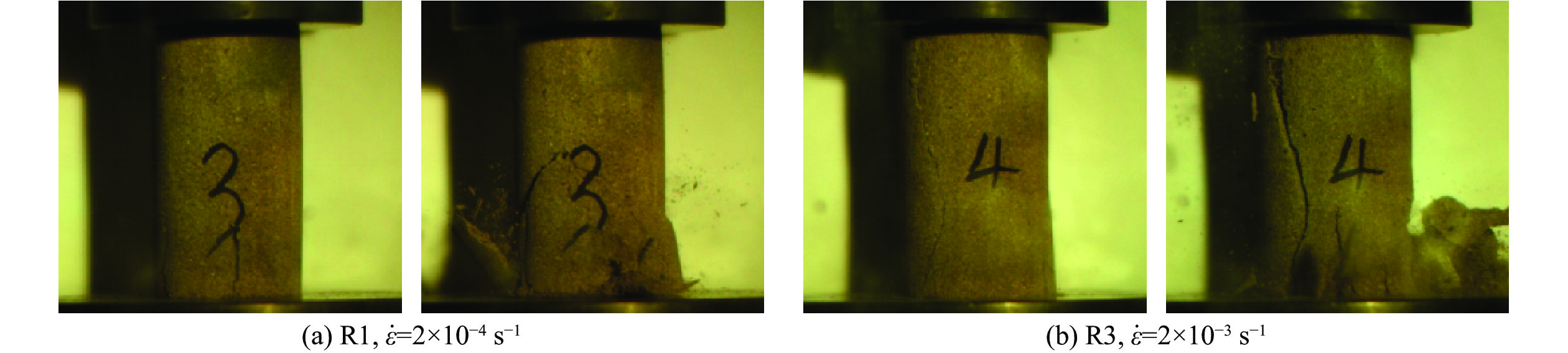
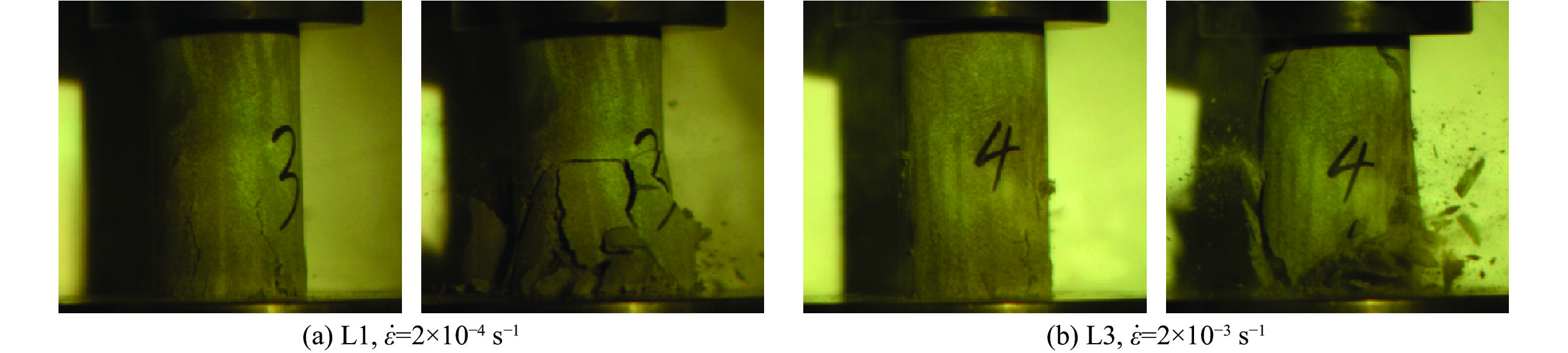
以图8和图9为例说明以两种加载速率进行准静态压缩时两种岩样的破坏模式。图8和图9中试样侧面编号为加工号,与本试验无关。
从图8和图9可以看出:当应变率为2 × 10−4 s−1时,岩样表面的剪切裂纹均从下端产生并且扩展,最后导致岩样单侧被压溃;当应变率为2 × 10−3 s−1时,岩样在拉剪应力作用下产生的裂纹迅速扩展至上下端面,导致整个岩石试件发生破坏。由图3的应力突变可以看出,岩样在无围压条件下表现出明显的脆性特征,当岩样内部应力达到起裂应力阈值时,岩石内部产生除原生微裂纹之外的新生裂隙,并且新生裂隙稳定扩展,整个试件表现出以塑性变形为主的初期膨胀;当应力继续增加至损伤阈值应力时,新生裂隙和原生裂隙迅速扩展并相互贯通,岩样内部累进式破坏,出现扩容现象,即体积加速膨胀;当应力加载至峰值时,已经连接的微裂隙迅速发展为宏观剪切面,试件出现宏观破坏[23]。
结合图3、图8和图9可以得出:当名义应变率为2 × 10−4 s−1时,由于红砂岩试样R1为单侧压溃,即大尺寸留芯破坏,此时压力机判断试验未结束,而红砂岩试样R3为垂直轴线方向的整体破碎,所以当应变率为2 × 10−4 s−1时,峰值压力较小;石灰岩试样L3表现为面向高速摄影机镜头的一侧崩落飞出,其上下端面与压力机仍有接触,且加载速率高于试样L1一个数量级,故其应力-应变曲线的峰值较高,且弹性段较试样L1的弹性段长。
2.2 动态压缩
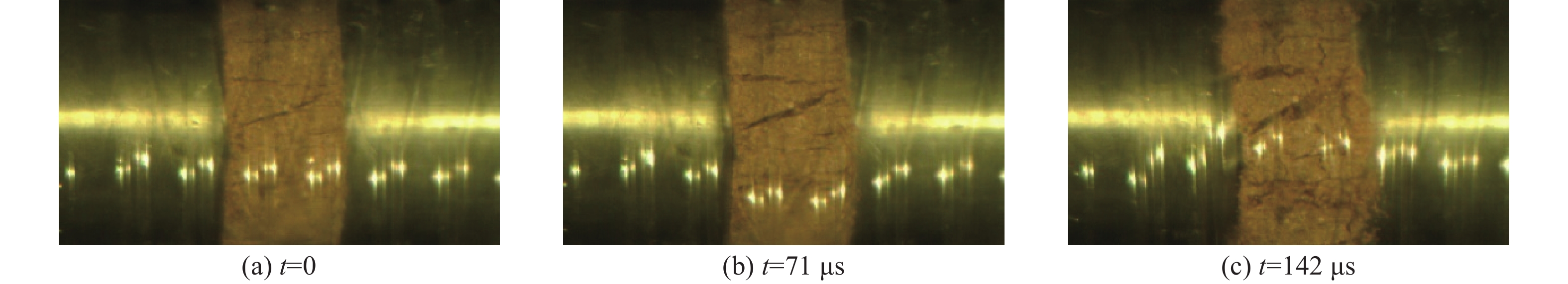
以图10和图11为例说明两种岩样在动态压缩下的破坏模式,取岩石试件出现裂纹的时间为t = 0,高速摄影机的拍摄帧率为14002帧每秒。
从图10和图11可以发现,在冲击载荷作用下被夹持在入射杆和透射杆之间的岩石试件侧面首先出现轴向贯穿裂纹,随着冲击载荷的持续作用,轴向裂纹数量增多且扩展为破裂面,直至整个岩石试件破碎,其中石灰岩破碎成数量非常多的碎块,碎块沿垂直于杆轴线方向飞散出来,而红砂岩则破碎为粉末,没有超过1 cm的岩块。根据一维应力假设,当岩石试件与压杆的截面积相等时,试件在受压时处于一维压缩状态;试件的长径比为0.5,长度较小,试样侧面可视为自由面,由入射杆传入试件的压缩波经反射后形成拉伸波,虽然拉伸波的幅值较小,但是一般脆性岩石材料的抗压强度为抗拉强度的8~10倍,抗拉强度一般小于10 MPa,所以岩石试件易在拉伸波的作用下发生失效破坏;在冲击载荷加载初期,虽然试件与杆的接触面没有发生破坏,但是侧面拉伸裂纹的产生、扩展直至贯穿试件,使得岩石试件迅速破碎失效。
以下将从微观构造与能量传递的角度对动态压缩下岩石试件的破坏模式进行分析。
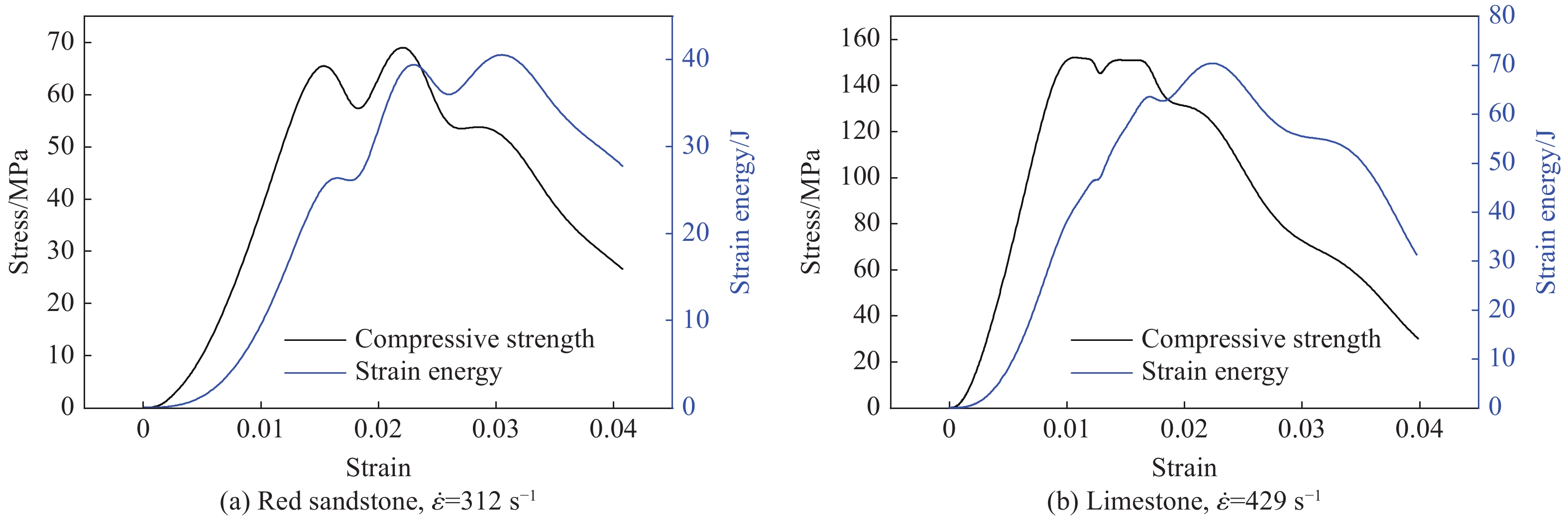
本试验所使用的霍普金森杆直径为40 mm,且冲头为纺锤形,虽然保证了试件在破碎前能够达到应力平衡状态,但是其能量加载相对缓慢。如图12所示,子弹通过入射杆传递到试件上的能量在第一时间不足以使岩石试件发生变形破坏,但是其加载会使岩石内部微裂纹被压实,导致抗压强度曲线出现第一次上下波动(由图7(a)中应变率为714 s−1的红砂岩压缩曲线可知,当冲击速度足够大时,试件会直接达到破碎阈值应力而破碎,并非随能量累积产生累进式破坏);随后试件的应变能继续增加,直至裂纹扩展至整个试件,此时试件未完全破坏,但是由于宏观裂纹的存在,抗压强度曲线开始向下波动;当试件的应变能达到最大时,红砂岩和石灰岩的应变能分别为40.3和70.7 J,试件被完全破坏,崩落飞出,两条曲线同时下降。
2.3 裂纹扩展速度的对比分析
根据图10和图11,已知试件的厚度为20 mm,并在控制软件Pcc2.6中对高速摄影机的测距进行标定,相机帧率为14002帧每秒,即图像之间的时间间隔为71 μs。根据软件的测距功能,可测得每帧之间裂纹宽度的变化,再除以时间,即为裂纹周向扩展速度。红砂岩和石灰岩在动态压缩下的裂纹周向扩展速度如图13所示。
由图13可知,岩石剪切裂纹的周向扩展速度与岩石的动态抗压强度密切相关,即抗压强度越大,周向扩展速度越大。根据岩石受压时能量的演化规律[24]对此现象进行解释。如图12所示,当岩石试件的抗压强度较大时,其储能极限也较大,弹性能除了转化为微观裂纹发展为宏观裂纹以及宏观裂纹扩展所需的能量外,还有大部分能量转化为碎块的动能,表现为裂纹的周向扩展速度更高。此外,因为干燥的岩石不存在自由水表面形成的减缓裂纹扩展速度的黏结力,所以扩展速度在外界能量持续输入的情况下基本呈线性增加。
3. 结 论
(1)加载速率对岩石在准静态压缩下的破坏模式的影响较大。应变率为10−4 s−1量级时,试件易被单侧压溃出现留芯破坏;应变率为10−3 s−1量级时,试件表现为整体的剪切破坏。在准静态压缩范畴,岩石强度的应变率效应并不明显,反而是破坏模式对强度的影响较大。
(2)当SHPB系统中子弹提供的能量较小且无法迅速达到岩样的断裂阈值应力时,岩样会随着能量的不断输入以及自身应变能的不断增加出现累进式破坏现象,这也是动态压缩应力-应变曲线出现起伏的根本原因;当子弹速度足够大时,岩样会直接达到断裂阈值应力,并且动态压缩应力-应变曲线只会出现一个波峰。
(3)由于石灰岩试件的抗压强度大于红砂岩,储能极限也大于红砂岩,故其破碎时会将更多的能量转化为碎块的动能,从而表现为动态压缩下抗压强度大的试件破碎后裂纹的周向扩展速度更快。
-
表 1 试件设计尺寸
Table 1. Design size of tested specimens
Specimen D×t/(mm×mm) [θ/(°)]n tc/mm L/mm Type of test v/(m·s−1) Maximum strain rate/s−1 S-58-1.5-30 58.0×1.5 [30/–30]3 2.0 100.0 Compression S-58-2.0-90 58.0×2.0 [90]6 2.0 100.0 Compression D-58-1.5-30-3.0 58.0×1.5 [30/–30]3 2.0 100.0 Impact 3.0 9.5 D-58-1.5-30-4.5 58.0×1.5 [90]6 2.0 100.0 Impact 4.5 21.1 D-58-2.0-90-4.5 58.0×2.0 [90]6 2.0 100.0 Impact 4.5 21.1 A/MPa B/MPa n m Tm/K Tr/K 272.28 899.7 0.94 0.1515 1795 293 D1 D2 D3 D4 D5 –43.408 44.608 0.016 0.0145 –0.046 Failure modes Initial failure criterions Damage evolution laws Fiber tensile failure Ft1=(σ1Xt)2+(σ4S12)2+(σ6S13)2⩾1 Dt1=εft1εft1−εit1(1−εit1ε1) Fiber compression failure Fc1=(σ1Xc)2⩾1 Dc1=εfc1εfc1−εic1(1−εic1ε1) Resin tensile failure Ft2=(σ2Yt)2+(σ4S12)2+(σ5S23)2⩾1 Dt2=εft2εft2−εit2(1−εit2ε2) Resin compression failure Fc2=(τnt(θ)SA23+μntσn(θ))2+(τnl(θ)S12+μnlσn(θ))2 Dc2=γfrγfr−γir(1−γirγr) Stretch in-layer delamination Ft3=(σ3Zt)2+(σ6S13)2+(σ5S23)2 Dt3=εft3εft3−εit3(1−εit3ε3) Compression in-layer delamination Fc3=(σ3Zc)2+(σ6S13)2+(σ5S23)2 Dc3=εfc3εfc3−εic3(1−εic3ε3) Modulus/GPa Poisson’s ratio Strength/MPa Fracture energy/(N·mm) E1=41.29; E2=E3=4.21; ν12=ν13=0.31; Xt =884.5; Xc =837.17; Yt=Zt=37.38; Γt1=28.25; Γc1=80.1; G12=G13=3.16; G23=3.0 ν23=0.42 Yc =Zc=145; S12=S13=44.765; S23=50.88 Γt2=Γt3=0.36; Γc2=Γc3=7.24 Elastic properties/GPa Strength/MPa Fracture toughness/(N·mm−1) B-K parameter Knn =4210; Ktt=3160; Kll=3160 Tn =37.380; Tt=44.765; Tl=44.765 Gn =0.36; Gt=1.33; Gl=1.33 η=2.6 表 7 GFRP应变率效应模型所需参数
Table 7. Parameters for the model considering the strain rate effect of GFRP
Parameter α β γ Parameter α β γ E11/GPa 41.29 1.139 0.276 Xt/MPa 837.17 7.721 0.886 E22/GPa 4.21 0.437 0.262 Yt/MPa 37.38 13.088 0.131 G12/GPa 3.16 –0.941 0.054 Yc/MPa 145 0.11 1.278 Xt/MPa 881.5 7.721 0.886 S/MPa 44.756 15.656 0.086 表 8 仿真试件设计
Table 8. Design of simulated specimens
Specimen D/mm t/mm [θ/(°)]n tc/mm L/mm Displacement/mm Loading speed/(m·s−1) A-1 58.0 2.0 [90]6 2.0 100 20.0 Quasi-static A-2 58.0 2.0 [90]6 2.0 100 20.0 2.0 A-3 58.0 2.0 [90]6 2.0 100 20.0 4.0 A-4 58.0 2.0 [90]6 2.0 100 20.0 6.0 A-5 58.0 2.0 [90]6 2.0 100 20.0 8.0 B-1 58.0 2.0 [90]6 3.0 100 20.0 Quasi-static B-2 58.0 2.0 [90]6 3.0 100 20.0 2.0 B-3 58.0 2.0 [90]6 3.0 100 20.0 4.0 B-4 58.0 2.0 [90]6 3.0 100 20.0 6.0 B-5 58.0 2.0 [90]6 3.0 100 20.0 8.0 C-1 58.0 3.0 [90]6 2.0 100 20.0 Quasi-static C-2 58.0 3.0 [90]6 2.0 100 20.0 2.0 C-3 58.0 3.0 [90]6 2.0 100 20.0 4.0 C-4 58.0 3.0 [90]6 2.0 100 20.0 6.0 C-5 58.0 3.0 [90]6 2.0 100 20.0 8.0 -
[1] 岳清瑞, 杨勇新. 纤维增强复合材料加固结构耐久性研究综述 [J]. 建筑结构学报, 2009, 30(6): 8–15.YUE Q R, YANG Y X. Introduction to durability of concrete strengthened with fiber reinforced polymers [J]. Journal of Building Structures, 2009, 30(6): 8–15. [2] ZHI X D, WU Q J, WANG C. Experimental and numerical study of GFRP-reinforced steel tube under axial impact loads [J]. International Journal of Impact Engineering, 2018, 122: 23–37. doi: 10.1016/j.ijimpeng.2018.07.018 [3] BATUWITAGE C, FAWZIA S, THAMBIRATNAM D, et al. Impact behaviour of carbon fibre reinforced polymer (CFRP) strengthened square hollow steel tubes: a numerical simulation [J]. Thin-Walled Structures, 2018, 131: 245–257. doi: 10.1016/j.tws.2018.06.033 [4] ALAM M I, FAWZIA S. Numerical studies on CFRP strengthened steel columns under transverse impact [J]. Composite Structures, 2015, 120: 428–441. doi: 10.1016/j.compstruct.2014.10.022 [5] 李洋, 王俊, 刘伟庆. 纤维复合材料-钢组合柱侧向冲击试验和有限元仿真分析 [J]. 钢结构, 2017, 32(2): 21–26.LI Y, WANG J, LIU W Q. Experimental study and FE simulation of the anti-impact performance of GFRP-steel column subjected to transverse impact [J]. Steel Construction, 2017, 32(2): 21–26. [6] KADHIM M M A, WU Z J, LEE S C. Loading rate effects on CFRP strengthened steel square hollow sections under lateral impact [J]. Engineering Structures, 2018, 171: 874–882. doi: 10.1016/j.engstruct.2018.04.066 [7] KADHIM M M A, WU Z J, LEE S C. Experimental study of CFRP strengthened steel columns subject to lateral impact loads [J]. Composite Structures, 2018, 185: 94–104. doi: 10.1016/j.compstruct.2017.10.089 [8] JOHNSON G R, COOK W H. A constitutive model and data for metals subjected to large Strains, high strain rates and high temperatures [C]//Proceedings of the Seventh International Symposium on Ballistics. The Hague, Netherlands, 1983: 1–7. [9] ZERILLI F J, ARMSTRONG R W. Dislocation-mechanics-based constitutive relations for material dynamics calculations [J]. Journal of Applied Physics, 1987, 61(5): 1816–1825. doi: 10.1063/1.338024 [10] LIN L, FAN F, ZHI X D. Dynamic constitutive relation and fracture model of Q235A steel [J]. Applied Mechanics and Materials, 2013, 274: 463–466. doi: 10.4028/www.scientific.net/AMM.274 [11] ZHANG D N, SHANGGUAN Q Q, XIE C J, et al. A modified Johnson-Cook model of dynamic tensile behaviors for 7075-T6 aluminum alloy [J]. Journal of Alloys and Compounds, 2015, 619: 186–194. doi: 10.1016/j.jallcom.2014.09.002 [12] TAN J Q, ZHAN M, LIU S, et al. A modified Johnson-Cook model for tensile flow behaviors of 7050-T7451 aluminum alloy at high strain rates [J]. Materials Science and Engineering:A, 2015, 631: 214–219. doi: 10.1016/j.msea.2015.02.010 [13] HASHIN Z. Failure criterion for unidirectional fiber composite [J]. Journal of Applied Mechanics, 1980, 47: 329–334. doi: 10.1115/1.3153664 [14] PUCK A, SCHURMANN H. Failure analysis of FRP laminates by means of physically based phenomenological models [J]. Composites Science and Technology, 2001, 62: 1633–1662. [15] SINGH H, NAMALA K K, MAHAJAN P. A damage evolution study of E-glass/epoxy composite under low velocity impact [J]. Composites Part B: Engineering, 2015, 76: 235–248. doi: 10.1016/j.compositesb.2015.02.016 [16] LIAO B B, LIU P F. Finite element analysis of dynamic progressive failure of plastic composite laminates under low velocity impact [J]. Composite Structures, 2017, 159: 567–578. doi: 10.1016/j.compstruct.2016.09.099 [17] Dassault Systèmes Simulia Corp. ABAQUS 6.11 user’s manual [Z]. Providence, RI: Dassault Systèmes Simulia Corp, 2011. [18] SHOKRIEH M M, KARAMNEJAD A. Investigation of strain rate effects on the dynamic response of a glass/epoxy composite plate under blast loading by using the finite difference method [J]. Mechanics of Composite Materials, 2014, 50(3): 295–310. doi: 10.1007/s11029-014-9415-1 -








 下载:
下载:


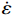
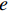
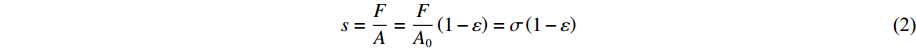
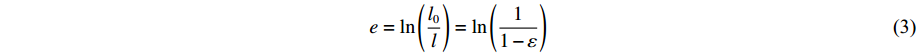

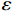



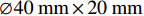


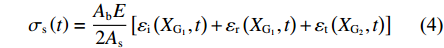
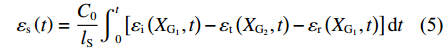
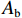
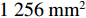
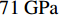
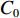
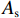

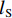
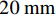


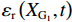
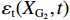
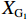
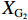




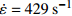


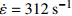
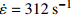



 下载:
下载: