爆炸冲击波在仿桥梁结构内传播的数值模拟

孟祥瑞; 栗建桥; 宁建国; 许香照

doi:10.11858/gywlxb.20180649

爆炸冲击波在仿桥梁结构内传播的数值模拟

doi: 10.11858/gywlxb.20180649

北京理工大学爆炸科学与技术国家重点实验室，北京　100081

基金项目: 国家自然科学基金（11532012，11802026）；中国博士后科学基金（2018M641209）

详细信息

作者简介:
孟祥瑞（1994－），男，硕士研究生，主要从事结构爆炸冲击数值计算研究. E-mail: woo888888@163.com

通讯作者:
许香照（1989－），男，博士，主要从事计算爆炸力学研究. E-mail: xuxiangxuxiangz@bit.edu.cn

中图分类号: O383.2; O389
计量
- 文章访问数: 5699
- HTML全文浏览量: 2549
- PDF下载量: 43
出版历程
- 收稿日期: 2018-10-15
- 修回日期: 2019-01-06
- 刊出日期: 2019-08-25

Numerical Simulation of Explosive Shock Wave Propagation in Imitation Bridge Structure

State Key Laboratory of Explosion Science and Technology, Beijing Institute of Technology, Beijing 100081, China

摘要

摘要: 桥梁作为交通枢纽中的重要关卡，受到强冲击载荷作用后的毁伤效果一直是国内外关注的热点问题。炸药爆炸是对其进行毁伤的最为有效的手段之一，研究爆炸冲击波在桥梁结构中的传播规律对桥梁结构抗爆设计和爆炸事故救援具有至关重要的作用。为此，搭建了桥梁的局部结构并进行爆炸毁伤实验，为数值模拟研究提供数据参考。采用自主开发的三维爆炸与冲击问题仿真软件EXPLOSION-3D对仿桥梁结构的爆炸冲击波传播问题进行了数值模拟研究。将数值模拟结果与实验结果进行对比，验证了数值算法的有效性；进一步通过对比不同位置处的压力时程曲线来分析爆炸冲击波在仿桥梁结构中的传播规律，并分析了炸药在不同位置处爆炸和不同当量炸药爆炸对桥梁结构毁伤的影响规律。基于数值仿真结果，得到了给定工况下炸药对仿桥梁结构内的人体和车辆的毁伤程度。最后，通过对比分析不同工况的数值模拟结果，从仿真的角度给出了安全预防建议。
- 多物质欧拉方法 /
- 并行计算 /
- 桥梁结构 /
- 爆炸冲击波 /
- 破坏毁伤
Abstract: The bridge is an essential part in the transportation system, and its damage effect under the strong impact load is always a hot issue in the world. Currently, the explosive explosion is one of the most effective bridge damage methods. Therefore, the research on the explosive shock wave propagation law in the bridge structure plays an important role in the process of anti-explosion design and explosion accident rescue. In this paper, a local imitation structure of the bridge was constructed, and the experiment of the explosive blasting in the bridge was performed. Then, the self-developed software EXPLOSION-3D was adopted to simulate the propagation process of explosive shock wave inner the imitation bridge structure. The numerical simulation results were compared with the experimental results to verify the effectiveness of the numerical algorithm. Further, the propagation laws were analyzed according to pressure-time history curves at different positions. Besides, the explosion damage effects of the bridge structure at different locations and equivalent explosives were also evaluated. Based on the numerical simulation results, the damage degree towards to the human body and vehicle which reside in the imitation bridge structure were obtained. Finally, some safety preventive suggestions were given in the view of simulation after compared and analyzed the numerical results in the different conditions.
- multi-material Eulerian method /
- parallel computation /
- bridge structure /
- explosive shock wave /
- damage

HTML全文

Dynamic compressive strengths of concrete-like materials are usually obtained by conducting laboratory tests such as split Hopkinson pressure bar (SHPB) tests^[1-5].Many empirical formulae for dynamic increase factor (DIF) based on laboratory test data were reported in the related literature Ref.[6-9].

It has to be mentioned here that the SHPB test data available for concrete materials in the literature were very scattered^[7-10] due to the combined effects of strain rate, inertia and specimen size.More comprehensive investigation and discussion of the possible influencing factors can be also obtained in Ref.[1, 2, 7-11].The inertia (confinement) effect has been widely investigated experimentally^[12], theoretically^[13-14], numerically^{[5, 15]} and an empirical equation for the dynamic increase factor due to inertia (confinement) effect was suggested in Ref.[16], which took no account of the effect of concrete specimen size.As strain rate effects on the compressive strengths of concrete-like materials play an important part in the construction of material constitutive models which, in turn, exert a great deal of influence on the numerical simulations of concrete structures subjected to intense dynamic loadings, it is, therefore necessary to obtain the pure strain rate effect data by eliminating the data due to the effects of inertia (confinement) and specimen size from SHPB tests.

In the present study, numerical simulations with a rate-independent material model are carried out on the influence of specimen size in SHPB tests on concrete and a new empirical equation for the dynamic increase factors due to inertia (confinement) effect is proposed which takes account of specimen size effect through its volume.Comparisons are made between the results from the numerical simulation and those from the new empirical formula and discussed.

1. Material Model

The computational constitutive model for concrete developed in Ref.[1] is used to simulate the SHPB tests on concrete in the present study.This material model consists of equation of state (EOS), strength model including Lode effect, damage criteria and strain rate effects, etc.

1.1 Equation of State

The porous equation of state is used in the present study and can be expressed as^[17]

$\bar \mu = \frac{{\rho \alpha }}{{{\rho _0}{\alpha _0}}} - 1 = \frac{\alpha }{{{\alpha _0}}}\left( {1 + \mu } \right) - 1$

(1)

where μ=ρ/ρ₀-1 is the volumetric strain, in which ρ and ρ₀ are respectively the current and initial densities; α=ρ_s/ρ and α₀=ρ_s0/ρ₀ are the current and initial porosities in which ρ_s and ρ_s0 are the current and initial densities of solid (fully-compacted) material.

For μ > 0, concrete material is under compression condition

$\left\{ \begin{array}{l} \alpha = \max \left\{ {1, \min \left[{{\alpha _0}, 1 + \left( {{\alpha _0}-1} \right){{\left( {\frac{{{p_{{\rm{lock}}}}-p}}{{{p_{{\rm{lock}}}}-{p_{{\rm{crush}}}}}}} \right)}^n}} \right]} \right\}\\ p = {K_1}\bar \mu + {K_2}{{\bar \mu }^2} + {K_3}{{\bar \mu }^3} \end{array} \right.$

(2)

where K₁, K₂ and K₃ are the bulk moduli for fully compacted concrete material, p_crush is the pressure at which pore collapse occurs, p_lock is the pressure beyond which concrete material is fully compacted, n is the compaction exponent.

For μ < 0, concrete material is under tension condition.Hence, the pressure is

$p = {K_1}\bar \mu$

(3)

1.2 Strength Model

The strength surface of concrete can be written in the following form^[1]

$Y = \left\{ \begin{array}{l} 3\left( {p + {f_{{\rm{tt}}}}} \right)r\left( {\theta, e} \right)\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;p \le 0\\ \left[{3{f_{{\rm{tt}}}} + \frac{{3p\left( {{f_{{\rm{cc}}}}-3{f_{{\rm{tt}}}}} \right)}}{{{f_{{\rm{cc}}}}}}} \right]r\left( {\theta, e} \right)\;\;\;\;\;\;\;\;0 < p < \frac{{{f_{{\rm{cc}}}}}}{3}\\ \left[{{f_{{\rm{cc}}}} + B{{f'}_{\rm{c}}}{{\left( {\frac{p}{{{{f'}_{\rm{c}}}}}-\frac{{{f_{{\rm{cc}}}}}}{{3{{f'}_{\rm{c}}}}}} \right)}^N}} \right]r\left( {\theta, e} \right)\;\;\;\;\;\;\;\;p \ge \frac{{{f_{{\rm{cc}}}}}}{3} \end{array} \right.$

(4)

where f_cc=f_c'δ_{m_t}η_c and f_tt=f_tδ_tη_t in which f_c' and f_t are the static compressive and tensile strength, δ_{m_t} and δ_t^[2] are the dynamic increase factors due to strain rate effects only in compression and tension, η_c and η_t^[18-20] are shape functions which represent shear damage and tensile softening of concrete respectively; B and N are empirical constants; r(θ, e)^[21] is the Lode effect with θ and e being the Lode angle, the ratio of the tensile meridian to the compression meridian, respectively.

By setting f_tt=0 and f_cc=f_c'r (residual strength), the residual strength surface for concrete can be obtained from Eq.(4)

$Y = \left\{ \begin{array}{l} 3pr\left( {\theta, e} \right)\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;0 < p < \frac{{{{f'}_{\rm{c}}}r}}{3}\\ \left[{{{f'}_{\rm{c}}}r + B{{f'}_{\rm{c}}}{{\left( {\frac{p}{{{{f'}_{\rm{c}}}}}-\frac{r}{3}} \right)}^N}} \right]r\left( {\theta, e} \right)\;\;\;\;\;p \ge \frac{{{{f'}_{\rm{c}}}r}}{3} \end{array} \right.$

(5)

Other information about shear, tensile damage, Lode effect and strain rate effect can be found in Ref.[1-2].

2. Specimen Size Effect

In this section, the commercial software LS-DYNA3D with user-defined subroutines is used to carry out the numerical study on the influence of specimen size in SHPB tests on concrete using a recently developed constitutive model for concrete^[1] by setting the material DIF (δ) equal to 1.The inertia effect dynamic enhancement factor (R_c) can be obtained by dividing the strength results of simulation by quasi-static strength.The values of various parameters used in the material model are listed in Table 1^[1].In this case, the numerically obtained strength increment of the studied specimen is attributed to the inertia (confinement) effect only.

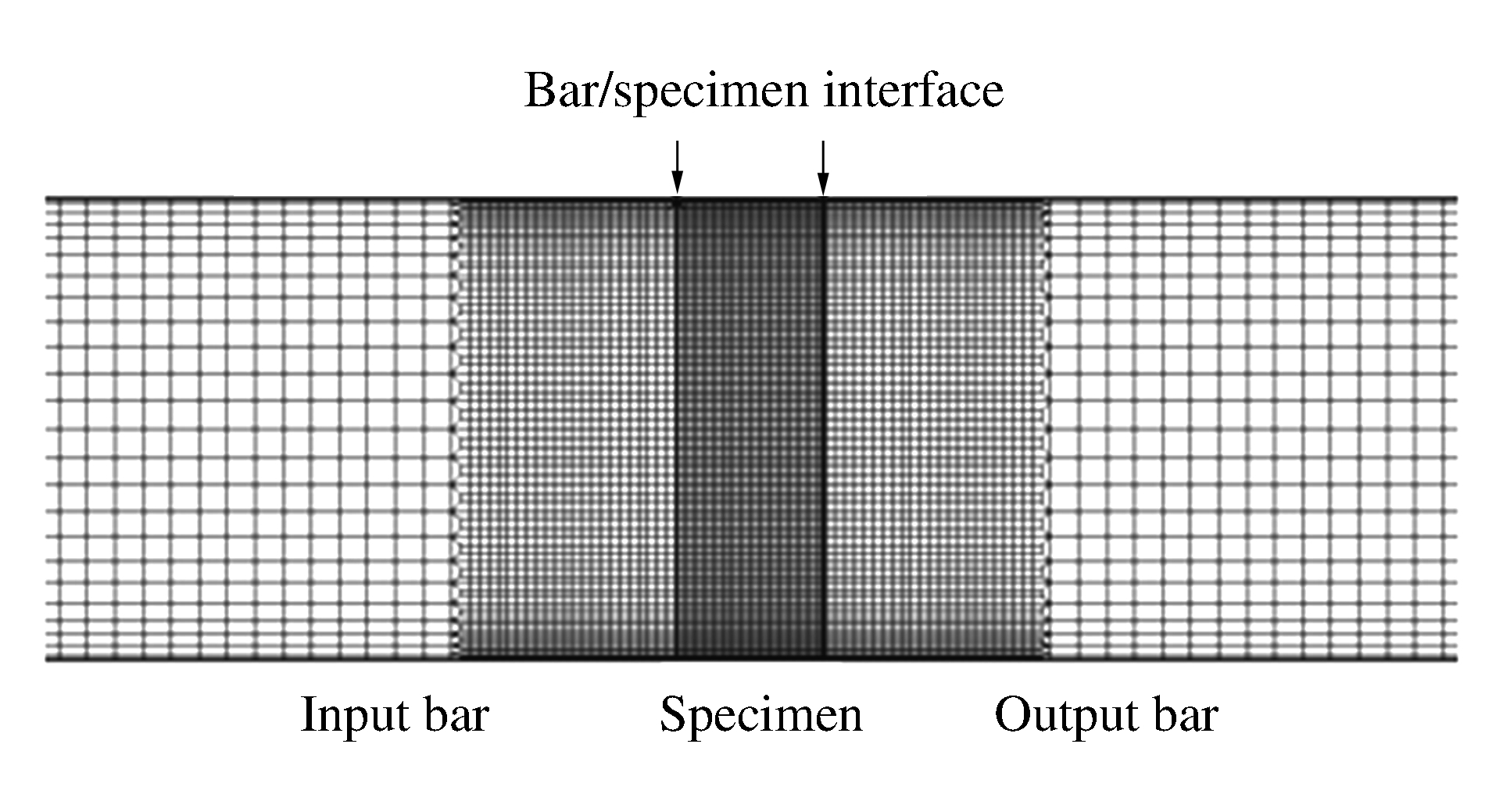
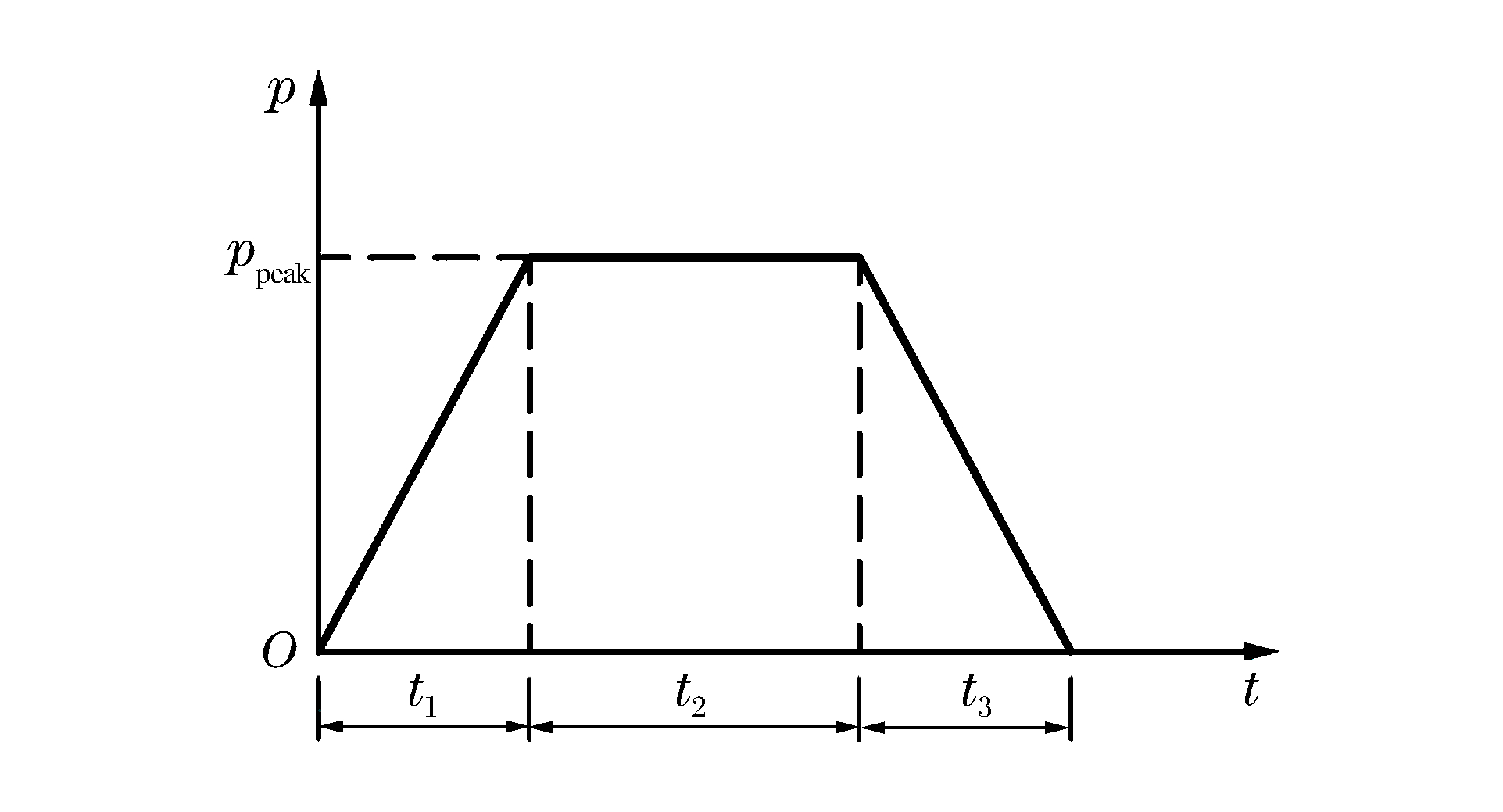
Table 1. Material parameters of concrete^[1]

Parameters of EOS								Parameters of constitutive model
ρ₀/(kg·m^-3)	ρ_s0/(kg·m^-3)	p_crush/MPa	p_lock/GPa	n	K₁/GPa	K₂/GPa	K₃/GPa	Strength surface					Shear damage				Tensile damage			Lode effect
ρ₀/(kg·m^-3)	ρ_s0/(kg·m^-3)	p_crush/MPa	p_lock/GPa	n	K₁/GPa	K₂/GPa	K₃/GPa	f_c'/MPa	f_t/MPa	B	N	G/GPa	λ_s	l	r	λ_m	c₁	c₂	ε_frac	e₁	e₂	e₃
2 400	2 680	15.2	3	3	13.9	30	10	45.6	3.8	1.7	0.7	10.5	4.6	0.45	0.3	0.3	3	6.93	0.007	0.65	0.01	5

| Show Table

DownLoad: CSV

An SHPB system contains incident and transmitter pressure bars with a short specimen between them, as shown in Fig. 1.A stress pulse of trapezium shape is applied to the incident pressure bar as shown schematically in Fig. 2 in which the incident stress starts from 0, quickly rises to the peak value of p_peak at t₁, keeps as a constant for t₂, then drops back to 0 after t₃ and are summarized in Table 2.

Figure 1. Finite element model of SHPB system

DownLoad: Full-Size Img PowerPoint

Figure 2. Loading function used in finite element analysis

DownLoad: Full-Size Img PowerPoint

Table 2. Load function parameters for direct compression analyses

t₁/μs	t₂/μs	t₃/μs	p_peak/MPa
25	200	25	Varies

| Show Table

DownLoad: CSV

Friction is an important factor which needs to be considered.However, in SHPB tests on concrete the effect of friction is negligibly small^[22] in terms of its contribution to the total dynamic increase factor and is usually ignored in numerical simulations^[23].Moreover, in SHPB tests measures are usually taken (i.e. by applying lubricant to both ends of concrete samples) to further reduce the effect of friction.Hence, in the present study the effect of friction is also ignored in the numerical simulations.

In this study, the dynamic strength increase factor is employed to reflect the effect of specimen size in SHPB tests on concrete.If there is no size effect there will be no difference for concrete samples with different sizes or volumes in terms of the dynamic increase factors at the same strain rate.Otherwise there will be size effect in SHPB tests on concrete.

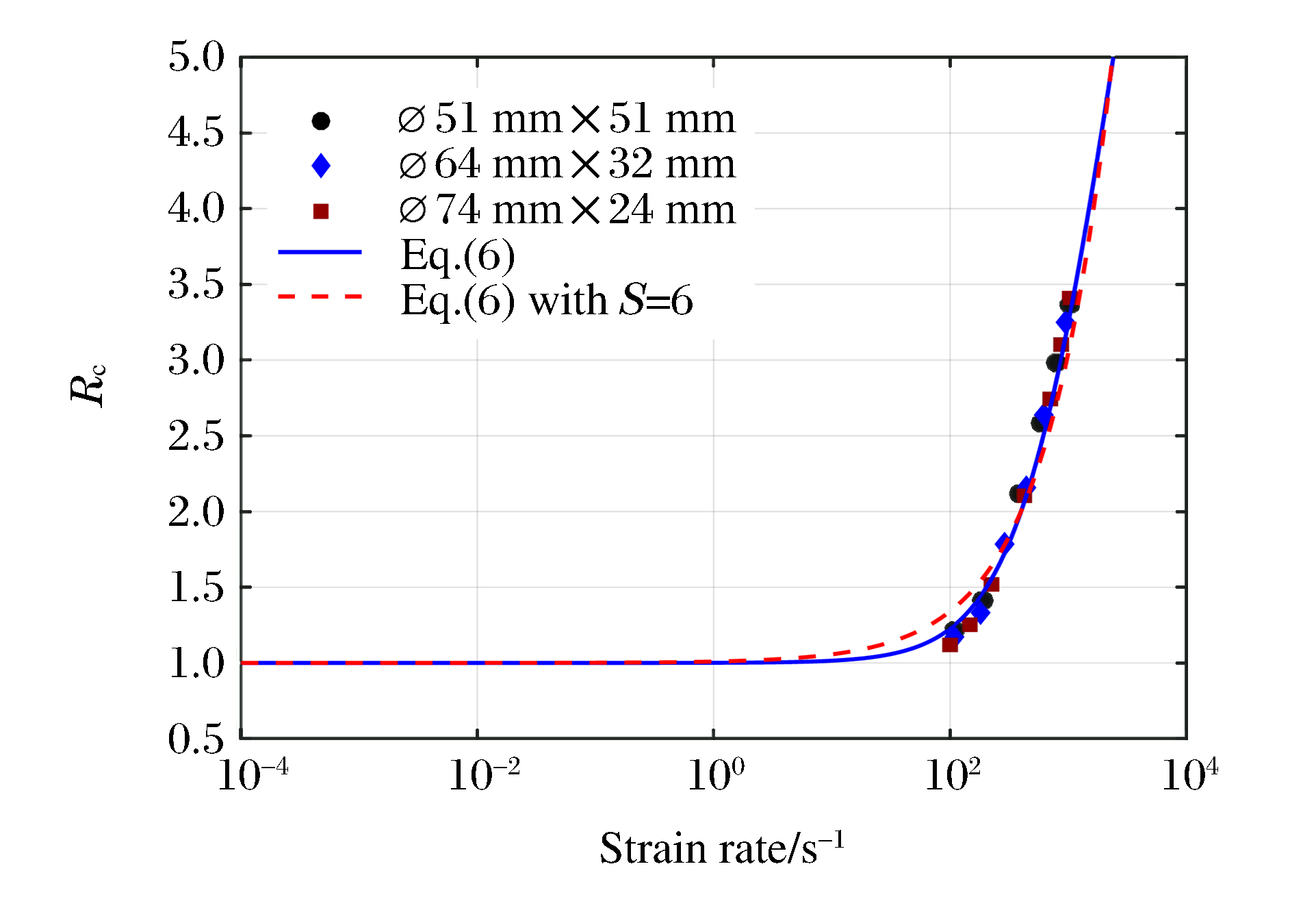
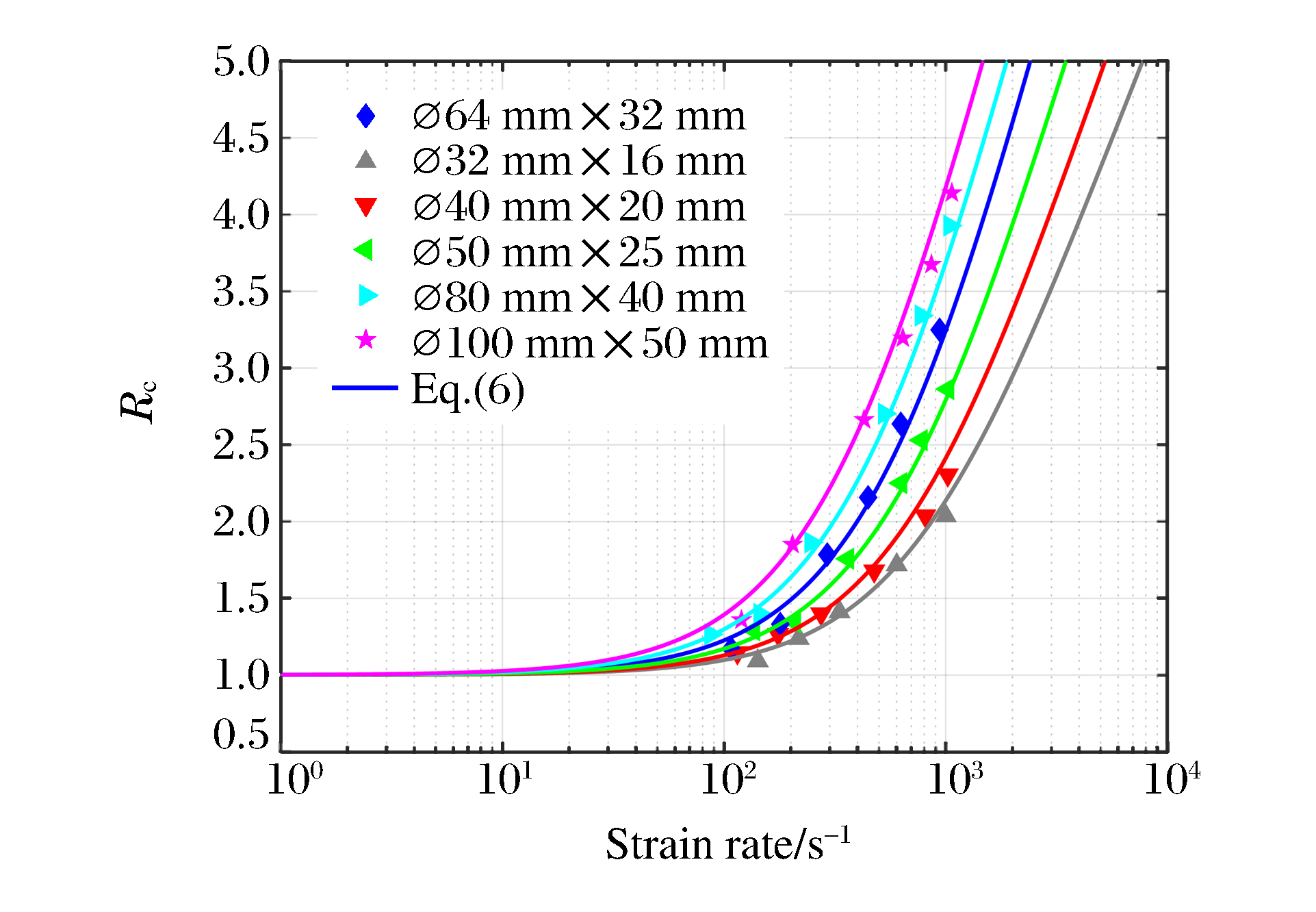
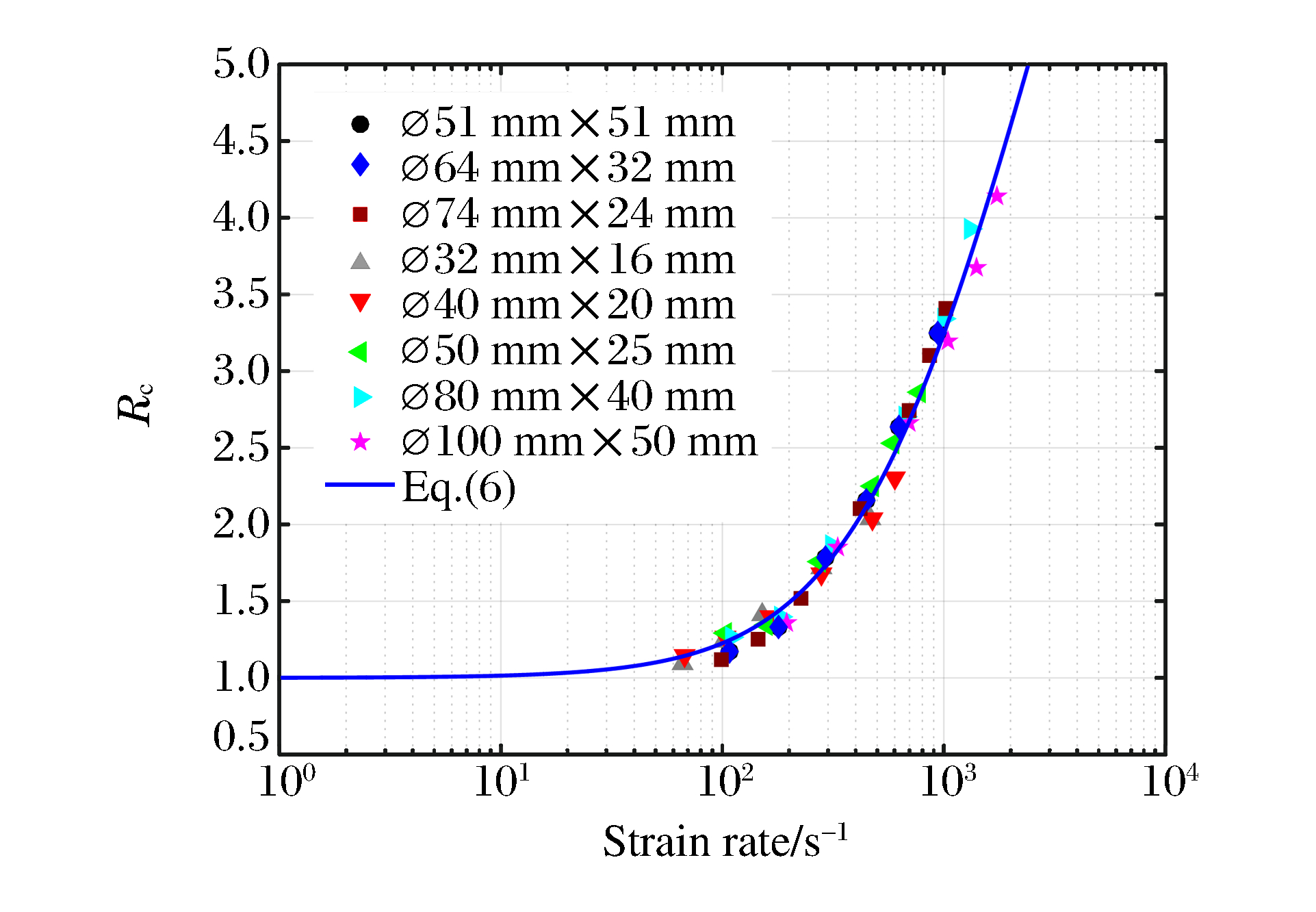
Fig. 3 shows the numerical results for the dynamic increase factor due to inertia (confinement) effect R_c for concrete specimens with the same volume of different length/diameter ratios (i.e., Ø51 mm×51 mm, Ø64 mm×32 mm, Ø74 mm×24 mm) whilst Fig. 4 shows the numerical results for concrete samples with different volume of the same length/diameter ratios (i.e., Ø64 mm×32 mm, Ø80 mm×40 mm).It can be seen from Fig. 3 that the inertia effect is mainly related to the specimen volume and is insensitive to the length/diameter ratio when the volume and the material parameters of the specimens are kept the same.It also can be seen from Fig. 4 that the dynamic increase factor due to inertia (confinement) effect increases with the increase of the concrete specimen volume, which indicates that size effect does exist in SHPB tests on concrete as described above.

Figure 3. Comparison of Eq.(6) with numerical results fordynamic increase factor due to inertia (confinement)effect for concrete specimens with the same volumeof different length/diameter ratios

DownLoad: Full-Size Img PowerPoint

Figure 4. Comparison of Eq.(6) with numerical results fordynamic increase factor due to inertia (confinement)effect for concrete specimens with different volumeof the same length/diameter ratio

DownLoad: Full-Size Img PowerPoint

3. A New Empirical Equation

On the basis of the numerical results discussed in the previous section, a new empirical equation which takes consideration of the specimen size effect in SHPB tests on concrete is suggested here to describe the dynamic increase factor due to inertia (confinement) effect R_c by using the monotonically increasing and continuous properties of exponential functions in a simple and easy to use form, namely

${R_{\rm{c}}} = {S^{\lg }}\left( {\frac{{\dot \varepsilon }}{{{{\dot \varepsilon }_0}}}} \right) + \frac{1}{\beta }\lg {\left( {\frac{V}{{{V_0}}}} \right)^{ - W}} + 1$

(6)

where S, W, β are the constants to be determined numerically, ${\dot \varepsilon }$ is the strain rate, ${{{\dot \varepsilon }_0}}$ is the reference strain rate for a reference specimen usually taken to be ${{{\dot \varepsilon }_0}}$ =1.0 s^-1, V is the volume of a concrete specimen under investigation, V₀ is the volume of a concrete sample with a reference size, say, Ø51 mm×51 mm.Set V=V₀ in Eq.(6), one obtains the dynamic increase factor due to inertia effect for the reference concrete specimen R_c0.

In the following, first, one determines the values of S and W in Eq.(6) using the numerical results for the concrete specimen with the same volume (i.e. V=V₀) as presented in Fig. 3 and, then, the value of β in Eq.(6) using the numerical results for the concrete samples with different sizes/volumes as given in Fig. 4.

Fig. 3 shows the comparison between the numerical results and Eq.(6) with S=6, W=2.8 and V=V₀ as indicated by the dashed line.It can be seen from Fig. 3 that reasonable agreement is obtained.Further examination reveals that the value of S in Eq.(6) should not be a constant but a function of strain rate.The slope of the curve is too small at the low strain rate, and too large at the high strain rate.By using the centrosymmetric properties of the hyperbolic tangent and the x-axis paralleled, S is taken as the following form to increase the slope of the curve at the low strain rate and decrease the slope at the high strain rate, namely

$S = - {F_i}\tan \;{\rm{h}}\left[{\lg \left( {\frac{{\dot \varepsilon }}{{{{\dot \varepsilon }_0}}}-{W_i}} \right){S_i}} \right] + {G_i}$

(7)

where F_i, W_i, S_i, G_i are the constants determined using the numerical results for concrete samples with constant volume as presented in Fig. 3, namely, F_i=6.0, W_i=2.8, S_i=0.8, G_i=8.5.The solid line in Fig. 3 is predicted from Eq.(6) with V=V₀ together with Eq.(7).It is clear from Fig. 3 that good agreement is obtained.

Fig. 4 shows the comparison between Eq.(6) with β=2.7 and the numerical results obtained for the concrete samples with different sizes/volumes.The values of all the other parameters in Eq.(6) are listed in Table 3.It is evident from Fig. 4 that the present model agrees well with the numerical simulations.

Table 3. Values of various parameters in Eq.(6) and Eq.(7)

${{{\dot \varepsilon }_0}}$ /s^-1	F_i	S_i	W_i	G_i	W	β
1.0	6.0	0.8	2.8	8.5	2.8	2.7

| Show Table

DownLoad: CSV

In order to verify the validity of Eq.(6) more numerical simulations are performed on concrete specimens with different sizes. The numerical results are also presented in Fig. 4 and comparisons are also made between Eq.(6) and the numerical results.It can be seen from Fig. 4 that good agreement is obtained.

Fig. 5 shows the normalization of all the numerical results for concrete specimens with different sizes as given in Fig. 3 and Fig. 4 with respect to those for the reference concrete sample, namely, Ø51 mm×51 mm.It is clear from Fig. 5 that all the numerical results collapse into one line.It lends further support to the validity of Eq.(6) for the dynamic increase factor due to inertia (confinement) effect which takes into consideration the influence of specimen size.

Figure 5. Variation of normalized numericallyobtained dynamic increase factor due to inertia(confinement) effect with strain rate

DownLoad: Full-Size Img PowerPoint

4. Conclusions

The influence of specimen size in SHPB tests on concrete has been investigated numerically using a rate-independent material model.A new empirical equation for the dynamic increase factor due to inertia (confinement) effect has also been proposed which takes into account the specimen size effect through its volume.It is demonstrated that the new empirical formula agrees well with the numerical results for SHPB tests on concrete with different specimen sizes.

图 1 PMMIC-3D的程序流程图

Figure 1. Program flow of PMMIC-3D

下载: 全尺寸图片幻灯片

图 2 桥梁设计图

Figure 2. The design of bridge

下载: 全尺寸图片幻灯片

图 3 现场搭建图

Figure 3. Actual construction of bridge

下载: 全尺寸图片幻灯片

图 4 爆炸后模型受损情况

Figure 4. Damage of model after explosion

下载: 全尺寸图片幻灯片

图 5 桥梁三维模型图

Figure 5. 3D model of bridge

下载: 全尺寸图片幻灯片

图 6 前处理网格效果图

Figure 6. Grid meshing

下载: 全尺寸图片幻灯片

图 7 不同时刻爆炸冲击波传播图像

Figure 7. Explosion shock wave propagation images at different time

下载: 全尺寸图片幻灯片

图 8 实验结果与仿真结果对比

Figure 8. Comparision diagram of experimental and simulation results

下载: 全尺寸图片幻灯片

图 9 起爆点与关键点位置图

Figure 9. Explosive position and critical points

下载: 全尺寸图片幻灯片

图 10 部分关键点的压力时程曲线

Figure 10. Pressure-time curves at some reference positions

下载: 全尺寸图片幻灯片

图 11 关键点峰值压力

Figure 11. Peak pressure at different points

下载: 全尺寸图片幻灯片

图 12 不同炸高的炸药位置

Figure 12. Explosive position with different height

下载: 全尺寸图片幻灯片

图 13 不同水平位置的炸药

Figure 13. Explosion positions

下载: 全尺寸图片幻灯片

图 14 不同炸高情况下关键点的峰值压力

Figure 14. Peak pressure at different points with different heights

下载: 全尺寸图片幻灯片

图 15 不同水平位置炸药情况下的峰值压力

Figure 15. Peak pressure at different points with different horizontal positions

下载: 全尺寸图片幻灯片

图 16 关键点在不同当量炸药情况下的峰值压力

Figure 16. Peak pressure at different positions with different explosive mass

下载: 全尺寸图片幻灯片

图 17 桥体为金属介质时部分三维仿真结果

Figure 17. Some 3D simulation results of steel structure

下载: 全尺寸图片幻灯片

图 18 破坏效果对比

Figure 18. Comparison of damage effects

下载: 全尺寸图片幻灯片

图 19 桥体为不同介质时关键点处的峰值压力

Figure 19. Peak pressure at different positions with different structural medium

下载: 全尺寸图片幻灯片

表 1 桥梁事故部分事件

Table 1. Some accidents of bridge

Date	Accident
2004–06–10	Collapse accident of TianZhuangTai bridge in Panjin, Liaoning
2004–06–14	Collapse accident of bridge in Longgang, Shenzhen
2006–08–02	Collapse accident of XiongYue bridge, Yingkou, Liaoning
2006–03–11	“3.11” collapse of bridge in Yangzhou, Jiangsu
2006–11–26	316 national highway Lengshui bridge
2007–04–29	Collapse accident of California expressway to Oakland
2007–06–15	Collapse accident of Jiujiang bridge, Guangdong
2009–07–15	Collapse accident of Tianjin Tanggu ramp bridge
2010–12–03	Collapse accident of Jiaxu river crossing bridge in Haining, Zhejiang
2013–02–01	Collapse accident of Yichang bridge in Henan
2014–08–30	Fujian Shaowu bridge accident
2015–04–02	Jinbao high–speed rail collapses under construction of viaduct
2015–06–19	Collapse accident of Guangdong Jiangxi expressway ramp bridge

下载: 导出CSV

表 2 B炸药性能参数

Table 2. Performance parameters of Explosive B

Density/(g·cm⁻³)	CJ pressure/GPa	CJ detonation velocity/(m·s⁻¹)	Specific energy/(kJ·g⁻¹)
1.67	15.0	8100	9.5

下载: 导出CSV

表 3 45钢的材料参数

Table 3. Material parameters of 45 steel

$\rho$ /(g·cm⁻³)	E/GPa	${c_0}$	${\gamma _0}$	$a$	${s_1}$	${s_2}$	${s_3}$
7.85	206	4600	2.0	0.43	1.33	0.0	0.0

下载: 导出CSV

参考文献(17)

[1]	张钱城, 郝方楠, 李裕春. 爆炸冲击载荷作用下车辆和人员的损伤与防护 [J]. 力学与实践, 2014, 36(5): 527–539. ZHANG Q C, HAO F N, LI Y C. Reserch progress in the injury and protection to vehicle and passengers under explosive shock loading [J]. Mechanics in Engineering, 2014, 36(5): 527–539.
[2]	GANNON J C. Design of bridges for security against terrorist attacks [D]. Austin, TX: The University of Texas at Austin, 2004: 10–125.
[3]	JONES N, BREBBIA C A. Structures under shock and impact VIII [M]. Southampton, UK: WIT Press, 2004: 53–62.
[4]	WINGET D G, MARCHAND K A, WILLIAMSON E B. Analysis and design of critical bridges subjected to blast loads [J]. Journal of Structural Engineering, 2005, 131(8): 1243–1255. doi: 10.1061/(ASCE)0733-9445(2005)131:8(1243)
[5]	WILLIAMSON E B, WINGET D G. Risk management and design of critical bridges for terrorist attacks [J]. Journal of Bridge Engineering, 2005, 10(1): 96–106. doi: 10.1061/(ASCE)1084-0702(2005)10:1(96)
[6]	IBRAHIM A, SALIM H. Numeical prediction of the dynamic response of prestressed concrete box girder bridges under blast loads [C]//11th International LS-DYNA Users Conference, 2006: 13–15.
[7]	魏雪英, 白国良. 爆炸载荷下钢筋混凝土柱的动力响应及破坏形态分析 [J]. 解放军理工大学学报(自然科学版), 2007, 8(5): 525–529. WEI X Y, BAI G L. Dynamic response and failure modes of RC column under blast load [J]. Journal of PLA University of Science and Technology (Natural Science Edition), 2007, 8(5): 525–529.
[8]	SYNGELLAKIS S. Design against blast [M]. Southampton, UK: WIT Press, 2012: 143–151.
[9]	SON J, LEE H J. Performance of cable-stayed bridge pylons subjected to blast loading [J]. Engineering Structures, 2011, 18(33): 1133–1148.
[10]	NING J G, MA T B, FEI G L. Multi-material Eulerian method and parallel computation for 3D explosion and impact problems [J]. International Journal of Computational Methods, 2014, 11(5): 1350079. doi: 10.1142/S0219876213500795
[11]	NING J G, MA T B, LIN G H. A grid generator for 3-D explosion simulations using the staircase boundary approach in Cartesian coordinates based on STL models [J]. Advances in Engineering Software, 2014, 67(1): 148–155.
[12]	FEI G L, MA T B. Large-scale high performance computation on 3D explosion and shock problems [J]. Applied Mathematics and Mechanics (English Edition), 2011, 32(3): 375–382. doi: 10.1007/s10483-011-1422-7
[13]	FEI G L, MA T B, NING J G. Parallel computing of the multi-material eulerian numerical method and hydrocode [J]. International Journal of Nonlinear Sciences & Numerical Simulation, 2010, 11(Suppl): 189–193.
[14]	FEI G L, MA T B, NING J G. Study on the numerical simulation of explosion and impact processes using PC cluster system [J]. Advanced Materials Research, 2012, 433/440: 2892–2898. doi: 10.4028/www.scientific.net/AMR.433-440
[15]	JOHNSON W E, ANDERSON C E. History and application of hydrocodes in hypervelocity impact [J]. International Journal Impact Engineering, 1987, 5(1): 423–439.
[16]	ANDERSON C E. An overview of the theory of hydrocodes [J]. International Journal Impact Engineering, 1987, 5(1): 33–59.
[17]	BENSEN D J. A multi-material Eulerian formulation for the efficient solution of impact and penetration problems [J]. Computational Mechanics, 1994, 15(6): 558–571.