Elastic Properties of ReN2 under High Pressure
-
摘要: 超硬材料在工业上具有广泛的应用前景,如切割器具、研磨材料及耐磨涂层等。作为5d过渡金属双氮化合物之一的ReN2由共价键、离子键及金属键混合而成,因而具有诸多如高硬度、高熔点、耐腐蚀等优异的物理性质,进而具有潜在的研究价值。采用密度泛函理论中的平面波赝势法计算了零温零压下C2/m-ReN2的结构性质,并首次研究了高压下C2/m-ReN2的力学结构稳定性及弹性性质。研究得出了C2/m-ReN2的弹性常数、弹性模量、德拜温度、声速随压强的变化关系,除个别弹性常数,这些物理量皆随压强的增加而增加。还预测了C2/m-ReN2的韧脆性,并估算了C2/m-ReN2的维氏硬度。Abstract: Super hard materials have wide applications in industry, such as cutting tools, abrasive materials, wear resistant coatings. As one of the 5d transition metals double nitrogen compound, ReN2 contains both covalent bond, ionic bond and metallic bonding. In view of its many excellent physical properties, such as high hardness, high melting point and corrosion resistance, ReN2 earns much research interests. This article has calculated the structural properties of C2/m-ReN2 under zero temperature and zero pressure using the plane wave pseudo-potential method of density functional theory, and has studied the mechanical structure stability and elastic properties of C2/m-ReN2 under high pressure for the first time. The relations of the elastic constants, various modulus of elasticity, Debye temperature and the sound speed of C2/m-ReN2 with the pressure have obtained. In addition to the individual elastic constants, these quantities increase with the increase in pressure. In addition, we have also predicted the toughness and brittleness of C2/m-ReN2, and have estimated the Vickers hardness of C2/m-ReN2.
-
Key words:
- ReN2 /
- mechanical structure /
- elastic property /
- hardness
-
随着航天活动的日益增多,空间碎片数量急剧增加,航天器防护问题显得尤为重要,空间碎片主要分布在人类使用最频繁、距地球表面2000 km的低地球轨道,这些空间碎片的运行速度是第一宇宙速度,如果与航天器发生撞击,其平均撞击速度可达10 km/s,对航天器安全和航天员生命造成巨大威胁[1]。由于这些潜在威胁的影响,人们不得不关注航天器的防护问题。1947年Whipple提出的双层板防护结构是应用最广泛的防护结构,其基本原理是空间碎片撞击第一层防护板之后,破碎成大量高速运动的粒子,这些粒子作用于航天器舱壁,将点载荷转化为面载荷,减小了航天器舱壁的损伤。碎片云的形成与弹靶材料的破碎行为息息相关,然而研究材料的破碎过程,首先要从弹靶材料中应力波的传播入手。国内外学者对撞击产生碎片云的分布已有大量的研究成果,但是对于球形弹丸超高速撞击靶板时弹丸材料破碎行为的研究相对较少。哈尔滨工业大学迟润强等[2]将破碎的弹丸材料分为主体部分和后剥落部分,主体部分即为高压影响区域,后剥落部分可以看作层裂区域。弹丸的破碎行为直接影响弹丸形成碎片云在空间的分布规律,有很重要的研究意义。
本研究利用AUTODYN软件进行数值模拟。由于在超高速(尤其是几千米每秒的侵彻速度)下,如果采用Lagrange方法进行模拟很容易引起网格畸变,Euler方法也有类似的问题,因此采用光滑粒子流体动力学(Smoothed Particle Hydrodynamics,SPH)方法。SPH方法是一种无网格方法,可以有效地避免极度大变形时网格扭曲造成的精度破坏等问题,非常适合求解高速碰撞等动态大变形问题[3]。卞梁等[4]采用SPH方法对平板碰撞过程中波的传播和反射等现象进行了数值模拟,通过与解析解的对比,证实了SPH方法能够很好地模拟应力波的传播规律。本研究通过将SPH粒子数量设置为相对较大的数值,模拟得到压力脉冲在弹丸材料中较为细致的传播过程,以及弹丸背表面和侧表面层裂片的厚度,从而细致地描述弹丸材料的破碎过程,通过与层裂理论相结合,获得弹丸材料的破碎过程随碰撞初始条件的变化规律。
1. 数值模拟参数选择及模型合理性验证
数值模拟采用AUTODYN中的SPH方法,球形弹丸和靶板材料选择硬铝合金Al2024-T351,采用Johnson-Cook本构模型[5-6],屈服应力Y的表达式为
Y=(A+BεnP)(1+Cln˙ε∗P)(1−TmH) (1) 式中:A、B、C、n和m为5种材料常数;εP为有效塑性应变;
˙ε∗P 为归一化有效塑性应变率,其中参考应变率˙ε0 =1 s-1;TH=(T–Troom)/(Tmelt–Troom),其中Troom代表室温,Tmelt代表材料熔点。具体参数见表1。表 1 Al2024-T351材料的Johnson-Cook本构模型参数Table 1. Johnson-Cook model parameters for Al2024-T351A/MPa B/MPa C m n Troom/K Tmelt/K 265 426 0.015 1 0.34 300 775 状态方程采用Mie-Grüneisen状态方程,表达式为
p=pH+Γρ(e−eH) (2) 式中:p和e分别为静水压力和比内能;Γρ=Γ0ρ0=C(C为常数),Γ和ρ分别为Grüneisen参数和密度,Γ0和ρ0为初始Grüneisen参数和初始密度。Mie-Grüneisen状态方程的Shock形式定义如下
{pH=ρ0C20μ(1+μ)[1−(S−1)μ]2eH=pH2ρ0(μ1+μ)μ=ρ/ρ0−1 (3) 式中:pH和eH分别为Hugoniot曲线上静水压力和比内能的参考值;S表示冲击波速度U和波后质点速度uP之间线性关系的斜率,通常U=C0+SuP,C0为体积声速。具体参数见表2。
表 2 Al2024-T351材料的Mie-Grüneisen状态方程参数Table 2. Mie-Grüneisen state equation parameters for Al2024-T351ρ0/(g·cm-3) C0/(m·s-1) S Γ0 2.785 5328 1.338 2 由于材料的抗拉能力有限,导致球形弹丸背表面出现层裂现象,本研究采用应力失效判据,即:材料承受的拉应力大于失效应力值时,粒子失效。采用Grady-Spall失效模型,该模型定义的失效应力是随着材料密度、体积声速以及屈服应力变化的,失效应力σs的表达式如下(其中铝合金材料的εc取0.15)
σs=√2ρC20Yεc (4) 数值模拟中,弹靶都采用对称建模方式。本研究的弹丸尺寸相对靶板较小,建立的靶板模型尺寸远远大于弹丸模型尺寸,因此采用无反射边界条件,不考虑靶板直径的影响。为了得到应力波的传播过程,弹丸和靶板的SPH粒子尺寸均设置为0.01 mm。
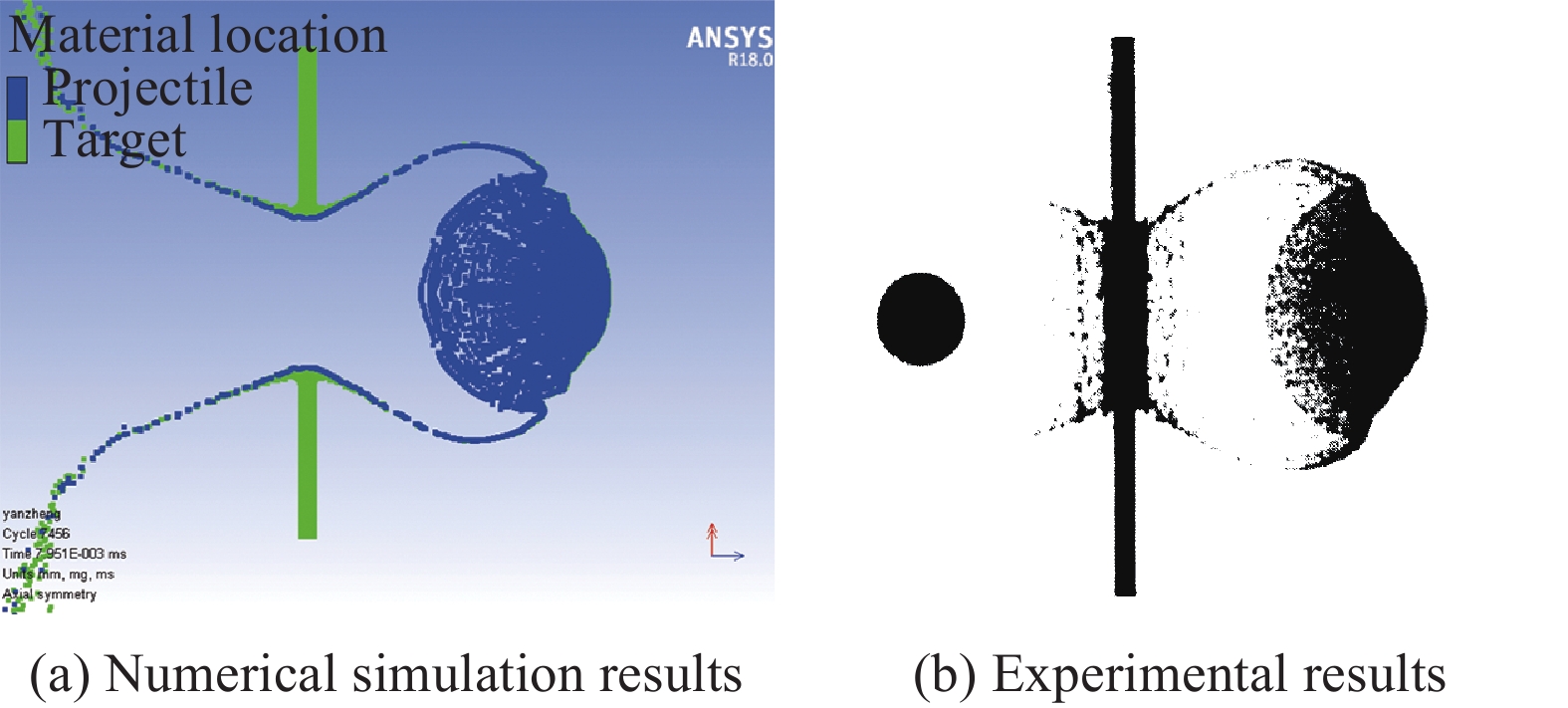
Piekutowski[7]开展了一系列超高速撞击实验,采用X射线照相技术拍摄到一组清晰的碎片云形态图像,本研究选择其中一种工况进行数值模拟的合理性验证。弹丸直径12.70 mm,靶板厚度2.03 mm,弹丸初始撞击速度6.38 km/s,选取与实验一致的弹丸和靶板材料,用硬铝合金撞击硬铝合金。从拍摄图像(见图1)可以看到,前端是一个碎片云密集区域,后边基本可以看作一个球壳结构。图1(a)是通过AUTODYN中的SPH方法得到的碎片云形态,与实验拍摄的碎片云形态吻合较好,证明了数值模拟方法的可靠性。此外在形成碎片云的过程中,材料的拉伸破坏和弹丸背表面的层裂对碎片云形态有非常直接的影响,如果层裂过程与实际过程不符,则数值模拟得到的碎片云与实验必定会有差异,而事实证明两者吻合度很高,侧面说明SPH方法对于层裂现象的数值模拟也是可靠的。从SPH方法的角度来讲,它以核函数近似为基础,将连续介质离散为一系列具有质量的粒子,通过核函数将方程离散,由于本研究设置的SPH粒子尺寸非常小,计算时邻域搜索半径也相对很小,因此提高了数值模拟结果的准确性,即对于裂纹扩展以及弹丸背表面层裂的仿真也是可靠的。
2. 弹丸材料中应力波传播规律
球形弹丸超高速撞击靶板形成碎片云的整个过程主要与两个初始条件有关:弹丸的初始速度以及靶板厚度与弹丸直径的比例。球形弹丸的破碎过程也随初始条件的变化而改变。通过建立不同初始条件的模型,以数值模拟结果为参考,结合应力波传播理论,得到两个初始条件对弹丸中应力波传播的影响规律。
2.1 相同工况下不同时间弹丸中压力曲线分析
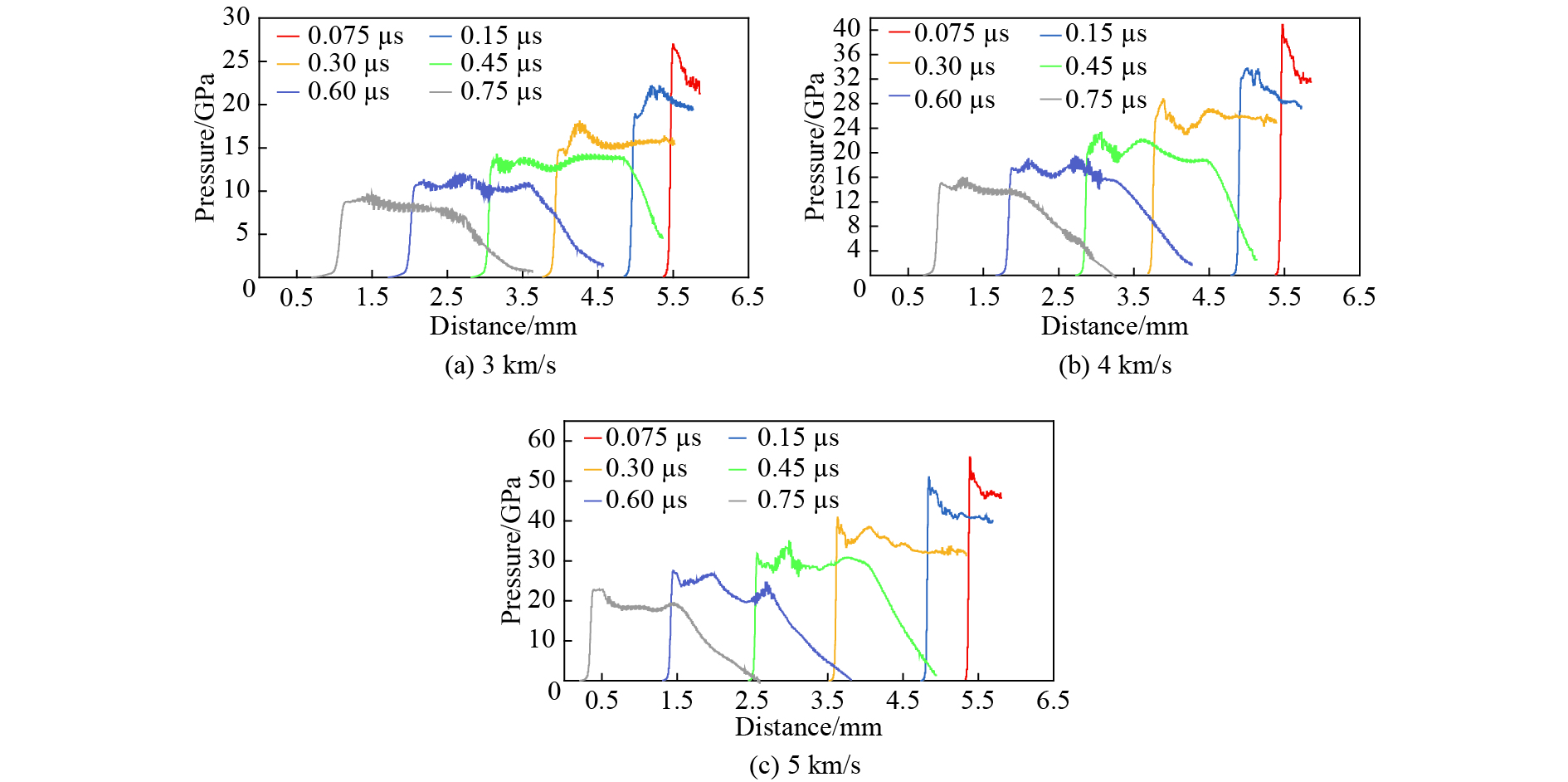
设计3种数值模拟工况,球形弹丸直径和靶板厚度保持不变(弹丸直径6 mm,靶板厚度1.5 mm),弹丸初始速度分别为3、4、5 km/s,对碰撞后不同时间弹丸中传播的压力脉冲进行分析。碰撞开始后不同时间压力曲线如图2所示。横坐标以弹丸远离靶板自由面为基准(零点),可以清晰地看到压力脉冲由碰撞端向背表面传播的过程。
从图2可以看出,碰撞开始后弹丸中压力脉冲逐渐变宽,一定时间后,脉冲宽度保持不变,但从开始碰撞到应力波传播至弹丸背表面的整个过程中,压力脉冲的峰值压力逐渐降低。弹丸速度为3 km/s时,碰撞开始时峰值压力达到26.9 GPa,传播到接近背表面时峰值压力为8.4 GPa;弹丸速度为4 km/s时,碰撞开始时峰值压力达到40.0 GPa,传播到接近背表面时峰值压力为13.5 GPa;弹丸速度为5 km/s时,碰撞开始时峰值压力达到55.9 GPa,传播到接近背表面时峰值压力为22.8 GPa。这主要是靶板反射稀疏波和侧方稀疏波双重作用的结果。提取每一时刻的峰值压力,绘制峰值压力-时间曲线,如图3所示。
从图3中的数据可以看出,弹丸中峰值压力的衰减接近于线性衰减,且衰减速率随着弹丸初始速度的提高有增大的趋势。
2.2 不同弹丸初速下压力曲线分析
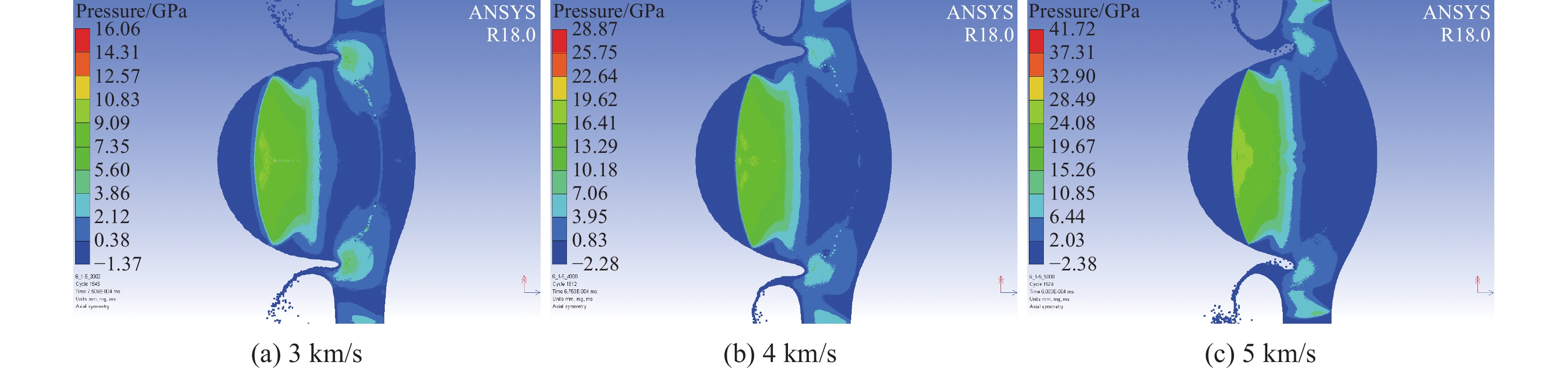
设计3种数值模拟工况,弹丸直径6 mm,靶板厚度1.5 mm,弹丸初始速度分别为3、4、5 km/s。弹丸压力云图如图4所示。
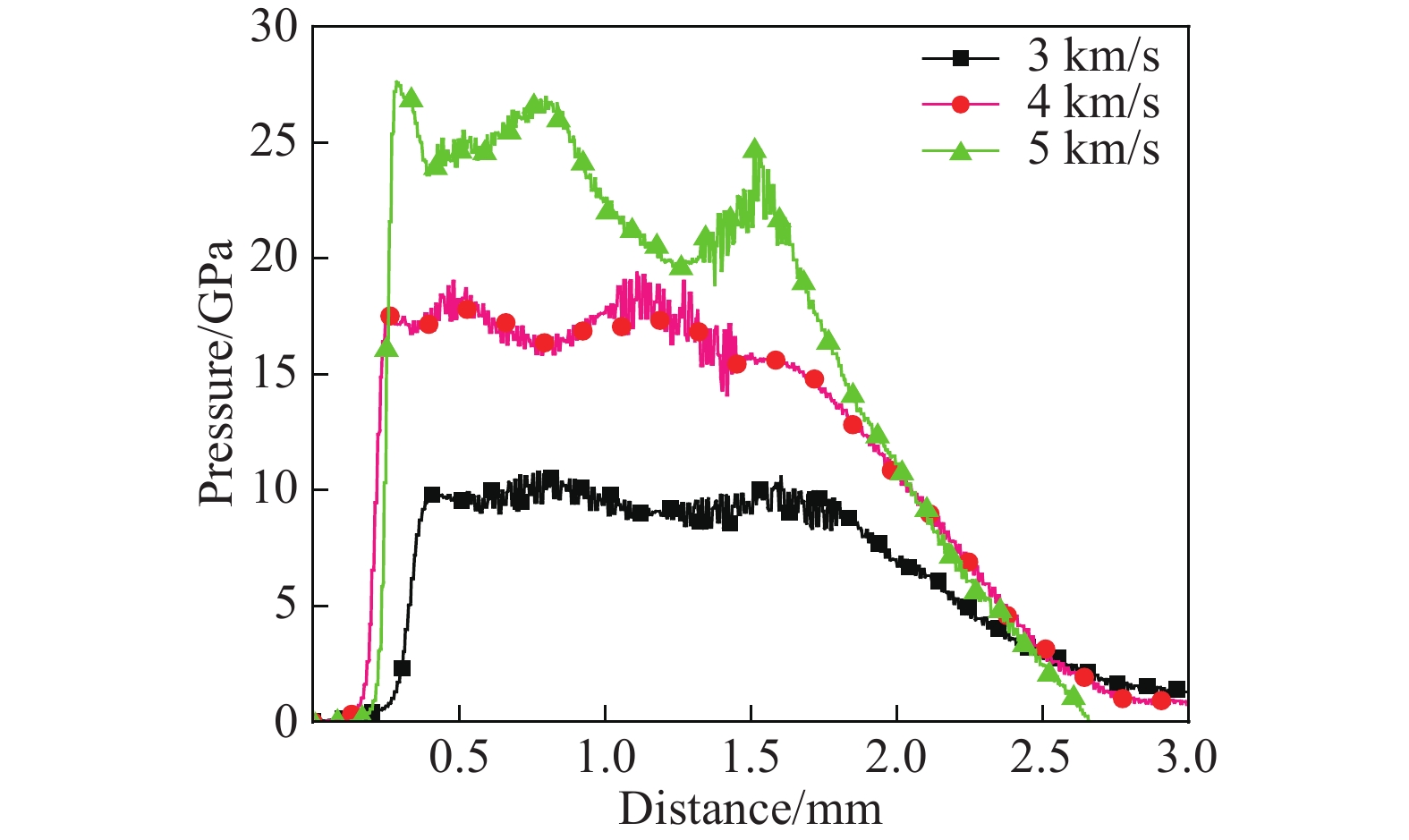
图5为碰撞相同时间(0.6 μs)时,3种工况下弹丸材料中的压力脉冲曲线。弹丸初始速度为3、4、5 km/s时,弹丸中的压力脉宽均为3 mm左右,可以看出,不同弹丸初始速度下,弹丸中压力脉冲的宽度基本保持不变。但是在碰撞后的同一时刻,弹丸中的峰值压力有很大的不同,且随着撞击速度的增大而呈现递增趋势。通过脉冲曲线可以看出,压力脉冲大致可以分为两个阶段,第一阶段的斜率较小,第二阶段的斜率较大[8],这样的脉冲形式将会对之后弹丸背表面的层裂情况产生影响。
2.3 不同靶板厚度下压力曲线分析
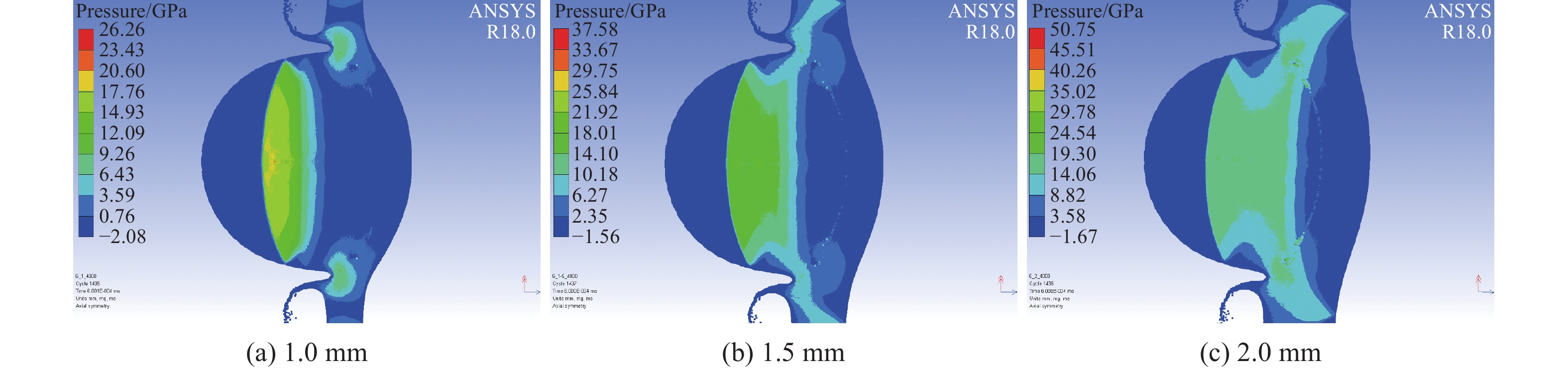
模拟3种工况,保持弹丸直径和弹丸初始速度不变(弹丸直径6 mm,弹丸初始速度4 km/s),靶板厚度分别为1.0、1.5、2.0 mm。压力云图如图6所示。
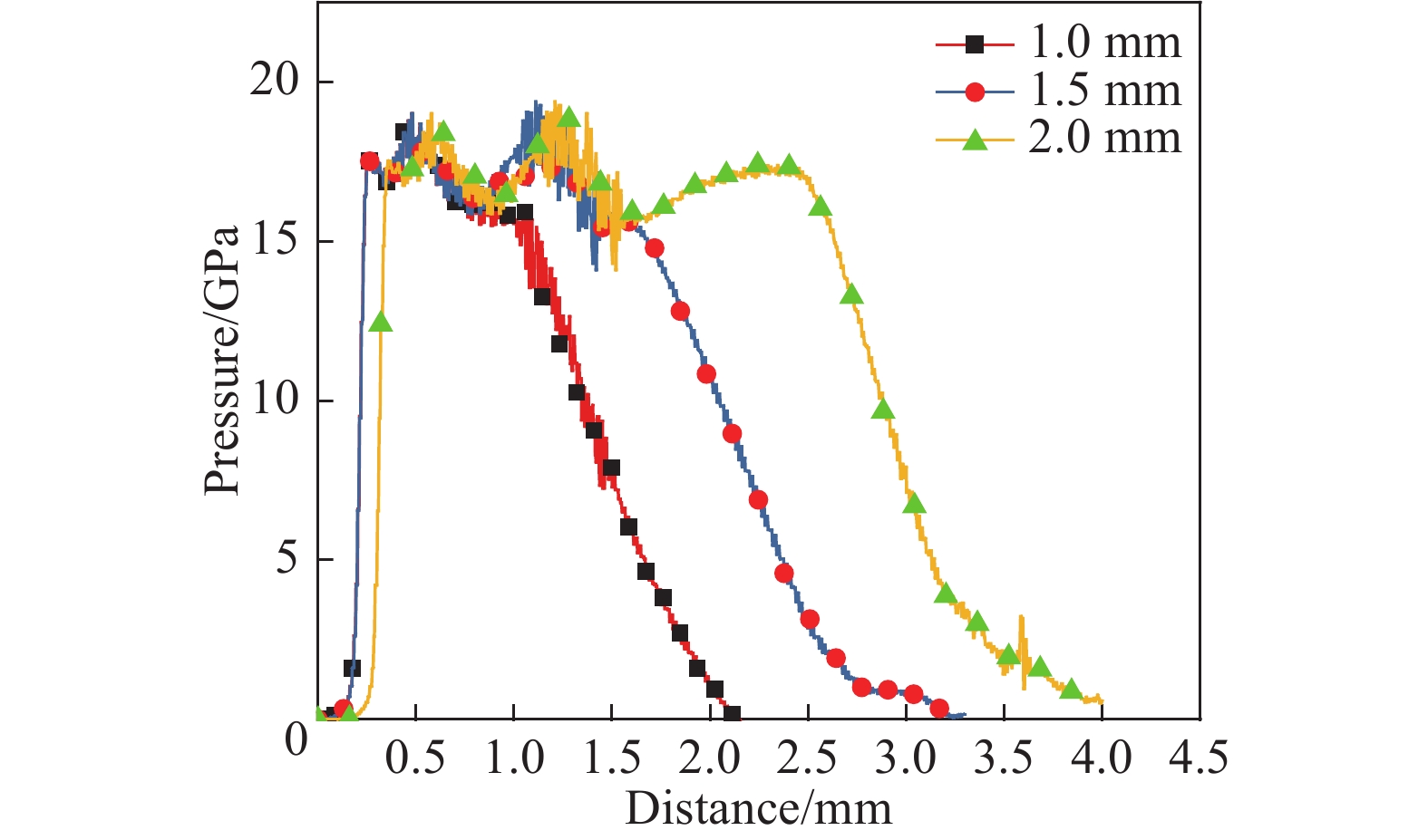
图7为碰撞相同时间(0.6 μs)时,3种工况下弹丸材料中的压力脉冲曲线。靶板厚度为1.0、1.5、2.0 mm时,弹丸中传播的压力脉冲宽度分别为2、3和4 mm。由此可以很直观地看出,弹丸中传播的压力脉冲的宽度随靶板厚度的增加而变宽。由压力脉冲曲线可以得到,虽然脉冲宽度随着靶板厚度的增加而增加,但是峰值压力的强度基本保持不变,也就是说,靶板厚度的增加主要影响弹丸中传播压力脉冲的宽度,对峰值压力的影响不大。
3. 压力脉冲与弹丸破碎的关系
弹丸中压力脉冲形状直接影响弹丸背表面的破碎行为。在弹丸和靶板尺寸相同的情况下,弹丸初始撞击速度直接影响弹丸中传播压力脉冲的峰值压力,而脉冲的宽度基本不变,即:弹丸速度越高,压力脉冲的衰减速度越快。如果把脉冲看作线性衰减,则层裂片厚度δ满足
δ=λ2⋅σcσm (5) 式中:λ为脉冲宽度,σc为弹丸材料动态断裂应力,σm为脉冲压力峰值[9]。
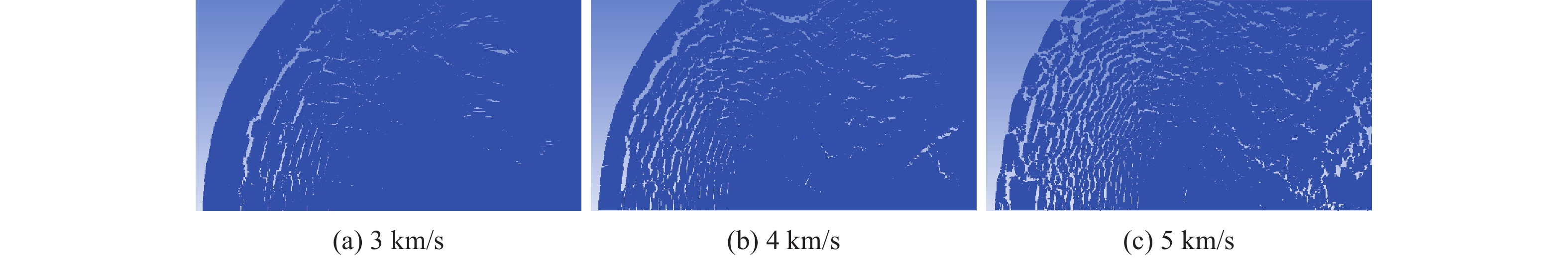
由(5)式可以看出,脉冲峰值越大,层裂厚度越小。此外,弹丸中压力脉冲主要分为两个阶段,第一阶段衰减速率小,第二阶段衰减速率大,因此决定了弹丸背表面的初始层裂片厚度会大于此计算值,后期层裂厚度小于此计算值。图8为弹丸速度分别为3、4、5 km/s时弹丸背表面层裂图,与理论分析一致。弹丸速度为3 km/s时,第一层层裂厚度为0.4 mm,之后层裂厚度趋于稳定,为0.07 mm;弹丸速度为4 km/s时,第一层层裂厚度为0.25 mm,之后层裂厚度趋于稳定,为0.07 mm;弹丸速度为5 km/s时,第一层层裂厚度为0.20 mm,之后层裂厚度趋于稳定,为0.06 mm[10]。
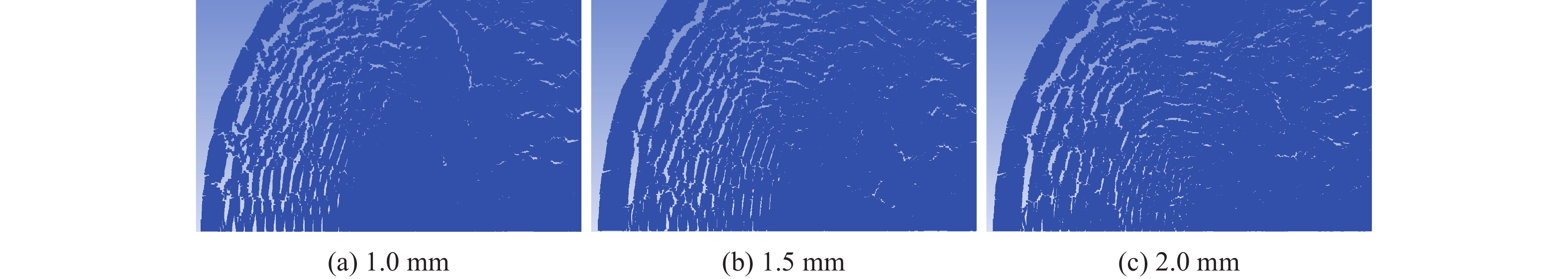
图9是弹丸尺寸6 mm、初始速度4 km/s工况下,靶板厚度分别为1.0、1.5、2.0 mm时弹丸背表面的层裂图。靶板厚度为1.0 mm时,第一层层裂厚度为0.21 mm,之后的稳定层裂厚度为0.065 mm;靶板厚度为1.5 mm时,第一层层裂厚度为0.25 mm,之后的稳定层裂厚度为0.070 mm;靶板厚度为2.0 mm时,第一层层裂厚度为0.26 mm,之后的稳定层裂厚度为0.065 mm。弹丸初始速度相同的情况下,靶板背表面的层裂片厚度基本相同。
靶板越厚,弹丸中压力脉冲的宽度越大,直接影响弹丸背表面在弹丸撞击轴线方向上的层裂深度。靶板厚度为1.0、1.5、2.0 mm时,弹丸背表面层裂沿弹丸撞击轴向的深度分别为1.0、1.3和1.7 mm,即:在弹丸尺寸和弹丸初始速度相同的情况下,靶板厚度越大,弹丸背表面层裂深度越大。
4. 结 论
(1)采用AUTODYN中的SPH方法,对球形弹丸超高速撞击靶板进行数值模拟,结果表明:在弹丸和靶板尺寸不变的情况下,球形弹丸的撞击速度直接影响弹丸中压力脉冲的峰值压力,且撞击速度越高,峰值压力越大;在弹丸尺寸和弹丸初始速度不变的情况下,靶板厚度影响弹丸中传播压力脉冲的宽度,且靶板厚度越大,压力脉冲越宽。
(2)弹丸超高速撞击靶板过程中,弹丸中的峰值压力衰减速率随着撞击速度的增加而变快。
(3)弹丸中传播的压力脉冲形状影响弹丸背表面的层裂:压力脉冲的峰值压力越大,压力的衰减速率越快,则弹丸背表面的层裂厚度越小;压力脉冲的峰值压力越小,衰减速率越慢,则弹丸背表面层裂厚度越大。且弹丸中传播的压力脉冲越宽,弹丸背表面沿弹丸撞击轴线方向上的层裂深度越大。
-
表 1 在p=0 GPa和T=0 K下的平衡晶格参数a、b、c及
β ,平衡体积V0,体模量B0,体模量对压强的一阶导数B′0 及其他理论值[13, 27]Table 1. Equilibrium lattice parameters a, b, c and
β , equilibrium volume V0, bulk modulus B0, and its pressure derivationB′0 at p=0 GPa and T=0 K, together with other theoretical results [13, 27]Method a/nm b/nm c/nm β/(°) V0/nm3 B0/GPa B0′/GPa This work 0.682 0.282 0.939 142.38 0.027 59 361 4.78 Ref. [13] 0.682 0.284 0.936 142.30 0.027 60 Ref. [27] 0.683 0.284 0.939 0.027 77 表 2 在p=0 GPa和T=0 K下的体模量B、剪切模量G、杨氏模量E、泊松比
σ 、维氏硬度HV及其他理论值[13, 27]Table 2. Bulk modulus B, shear modulus G, Young’s modulus E, Poisson’s ratio
σ and Vickers hardness HV at p=0 GPa and T=0 K, together with other theoretical results[13, 27]Method B/GPa G/GPa E/GPa σ Hv /GPa This work 370 242 596 0.23 27.66 Ref. [13] 369 217 Ref. [27] 376 210 531 0.26 -
[1] OYAMA S T. Crystal structure and chemical reactivity of transition metal carbides and nitrides [J]. Journal of Solid State Chemistry, 1992, 96(2): 442–445. doi: 10.1016/S0022-4596(05)80279-8 [2] LÉVY F, HONES P, SCHMID P E, et al. Electronic states and mechanical properties in transition metal nitrides [J]. Surface and Coatings Technology, 1999, 120/121: 284–290. doi: 10.1016/S0257-8972(99)00498-3 [3] IVANOVSKII A L. Platinum group metal nitrides and carbides: synthesis, properties and simulation [J]. Russian Chemical Reviews, 2009, 78(4): 303–318. doi: 10.1070/RC2009v078n04ABEH004036 [4] GILMAN J J, CUMBERLAND R W, KANER R B. Design of hard crystals [J]. International Journal of Refractory Metals and Hard Materials, 2006, 24(1/2): 1–5. [5] HAINES J, LEGER J M, BOCQUILLON G. Synthesis and design of superhard materials [J]. Annual Review of Materials Research, 2001, 31(1): 1–23. doi: 10.1146/annurev.matsci.31.1.1 [6] CROWHURST J C, GONCHAROV A F, SADIGH B, et al. Synthesis and characterization of the nitrides of platinum and iridium [J]. Science, 2006, 311(5765): 1275–1278. doi: 10.1126/science.1121813 [7] GREGORYANZ E, SANLOUP C, SOMAYAZULU M, et al. Synthesis and characterization of a binary noble metal nitride [J]. Nature Materials, 2004, 3(5): 294–297. doi: 10.1038/nmat1115 [8] YOUNG A F, SANLOUP C, GREGORYANZ E, et al. Synthesis of novel transition metal nitrides IrN2 and OsN2 [J]. Physical Review Letters, 2006, 96(15): 155501. doi: 10.1103/PhysRevLett.96.155501 [9] ZHAO E, WU Z. Structural, electronic and mechanical properties of ReN2 from first principles [J]. Computational Materials Science, 2008, 44(2): 531–535. doi: 10.1016/j.commatsci.2008.04.016 [10] LI Y, ZENG Z. New potential super-incompressible phase of ReN2 [J]. Chemical Physics Letters, 2009, 474(1/2/3): 93–96. [11] DU X P, WANG Y X, LO V C. Investigation of tetragonal ReN2 and WN2 with high shear moduli from first-principles calculations [J]. Physics Letters A, 2010, 374(25): 2569–2574. doi: 10.1016/j.physleta.2010.04.020 [12] KAWAMURA F, YUSA H, TANIGUCHI T. Synthesis of rhenium nitride crystals with MoS2 structure [J]. Applied Physics Letters, 2012, 100(25): 251910. doi: 10.1063/1.4729586 [13] WANG Y, YAO T, YAO J L, et al. Does the real ReN2 have the MoS2 structure? [J]. Physical Chemistry Chemical Physics, 2013, 15(1): 183–187. doi: 10.1039/C2CP43010J [14] WANG Y, LV J, ZHU L, et al. Crystal structure prediction via particle-swarm optimization [J]. Physical Review B, 2010, 82(9): 094116. doi: 10.1103/PhysRevB.82.094116 [15] BOUHEMADOU A, KHENATA R. Pseudo-potential calculations of structural and elastic properties of spinel oxides ZnX2O4 (X= Al, Ga, In) under pressure effect [J]. Physics Letters A, 2006, 360(2): 339–343. doi: 10.1016/j.physleta.2006.08.008 [16] LOUAIL L, MAOUCHE D, ROUMILI A, et al. Calculation of elastic constants of 4d transition metals [J]. Materials Letters, 2004, 58(24): 2975–2978. doi: 10.1016/j.matlet.2004.04.033 [17] SEGALL M D, LINDAN P J D, PROBERT M J, et al. First-principles simulation:ideas, illustrations and the CASTEP code [J]. Journal of Physics: Condensed Matter, 2002, 14(11): 2717–2744. doi: 10.1088/0953-8984/14/11/301 [18] VANDERBILT D. Soft self-consistent pseudopotentials in a generalized eigenvalue formalism [J]. Physical Review B, 1990, 41(11): 7892–7895. doi: 10.1103/PhysRevB.41.7892 [19] PERDEW J P, BURKE K, ERNZERHOF M. Generalized gradient approximation made simple [J]. Physical Review Letters, 1996, 77(18): 3865–3868. doi: 10.1103/PhysRevLett.77.3865 [20] PERDEW J P, CHEVARY J A, VOSKO S H, et al. Atoms, molecules, solids, and surfaces:Applications of the generalized gradient approximation for exchange and correlation [J]. Physical Review B, 1992, 46(11): 6671–6687. doi: 10.1103/PhysRevB.46.6671 [21] PFROMMER B G, COTE M, LOUIE S G, et al. Relaxation of crystals with the quasi-Newton method [J]. Journal of Computational Physics, 1997, 131(1): 233–240. doi: 10.1006/jcph.1996.5612 [22] MONKHORST H J, PACK J D. Special points for Brillouin-zone integrations [J]. Physical Review B, 1976, 13(12): 5188–5192. doi: 10.1103/PhysRevB.13.5188 [23] WALLACE D C. Thermodynamics of crystals [M]. New York: Wiley, 1972: 582. [24] WANG J, LI J, YIP S, et al. Mechanical instabilities of homogeneous crystals [J]. Physical Review B, 1995, 52(17): 12627–12635. doi: 10.1103/PhysRevB.52.12627 [25] BARRON T H K, KLEIN M L. Second-order elastic constants of a solid under stress [J]. Proceedings of the Physical Society, 1965, 85(3): 523–532. doi: 10.1088/0370-1328/85/3/313 [26] BIRCH F. Finite elastic strain of cubic crystals [J]. Physical Review, 1947, 71(11): 809–824. doi: 10.1103/PhysRev.71.809 [27] ZHAO Z L, BAO K, LI D, et al. Nitrogen concentration driving the hardness of rhenium nitrides [J]. Scientific Reports, 2014, 4(1): 4797. [28] WATT J P. Hashin-Shtrikman bounds on the effective elastic moduli of polycrystals with orthorhombic symmetry [J]. Journal of Applied Physics, 1979, 50(10): 6290–6295. doi: 10.1063/1.325768 [29] HILL R. The elastic behaviour of a crystalline aggregate [J]. Proceedings of the Physical Society, Section A, 1952, 65(5): 349. doi: 10.1088/0370-1298/65/5/307 [30] PUGH S F.XCII Relations between the elastic moduli and the plastic properties of polycrystalline pure metals [J]. The London, Edinburgh, and Dublin Philosophical Magazine and Journal of Science, 1954, 45(367): 823–843. doi: 10.1080/14786440808520496 [31] FRANTSEVICH I N, VORONOV F F, BOKUTA S A. Elastic constants and elastic moduli of metals and insulators [M]. Kiev: Naukova Dumka, 1983: 60–180. [32] IVANOVSKII A L. Microhardness of compounds of rhenium with boron, carbon, and nitrogen [J]. Journal of Superhard Materials, 2012, 34(2): 75–80. doi: 10.3103/S1063457612020013 [33] TIAN Y J, XU B, ZHAO Z S. Microscopic theory of hardness and design of novel superhard crystals [J]. International Journal of Refractory Metals and Hard Materials, 2012, 33: 93–106. doi: 10.1016/j.ijrmhm.2012.02.021 -







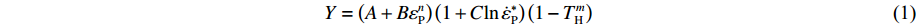

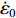
 下载:
下载:
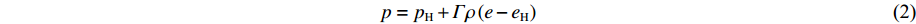
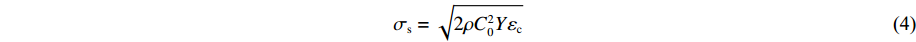







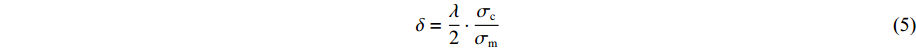



 下载:
下载: