超低密度笼形冰相及其负压相图

黄盈盈; 苏艳; 赵纪军

doi:10.11858/gywlxb.20180643

超低密度笼形冰相及其负压相图

doi: 10.11858/gywlxb.20180643

黄盈盈^{1, 2,},
苏艳^1,,
赵纪军^1,*, ,

1.
大连理工大学，辽宁大连　116024
2.
中国科学院上海应用物理研究所，上海　201800

基金项目: 国家自然科学基金（11674046）

详细信息

作者简介:
黄盈盈（1988－），女，博士，助理研究员，主要从事低密度冰和水合物研究. E-mail: 573050164@qq.com

苏　艳（1983－），女，博士，副教授，主要从事天然气水合物和含能材料理论研究. E-mail: su.yan@dlut.edu.cn

通讯作者:
赵纪军（1973－），男，博士，教授，主要从事低维凝聚态物理和计算材料学研究. E-mail: zhaojj@dlut.edu.cn

中图分类号: O521.2; O642.4; O641.3
计量
- 文章访问数: 8160
- HTML全文浏览量: 4203
- PDF下载量: 76
出版历程
- 收稿日期: 2018-09-25
- 修回日期: 2018-10-29

Ultralow-Density Clathrate Ices and Phase Diagram under Negative Pressure

HUANG Yingying^{1, 2
,},
SU Yan^1
,,
ZHAO Jijun^{1,*
, ,}

1.
Dalian University of Technology, Dalian 116024, China
2.
Shanghai Institute of Applied Physics, Chinese Academy of Science, Shanghai 201800, China

摘要

摘要: 水不仅在地球上无处不在，而且在太阳系中（如彗星、小行星及巨行星的卫星上）也普遍存在。因此，探索存在于不同环境条件下不同形态的水冰晶体对物理学、化学、生物学、地球科学以及行星科学都有着重要意义。根据周围的环境条件（压强和温度），冰呈现出极其丰富和复杂的相图。目前，实验上已合成了18个晶体冰相，分别是ice Ic、ice Ih、ice II 直至ice XVII。此外，还有一些来自于笼形包合物的假想超低密度冰相，分别是I型、II型、H型、K型和T型笼形冰。近期，在实验室中合成的II型笼形冰（即ice XVI）出现在了水的负压相图中，极大地激发了人们去探索其他低密度笼形冰。结合带有色散修正的密度泛函理论计算和经典的蒙特卡罗、分子动力学模拟，我们预测了两个具有超低密度的立方笼形冰相，将其依次命名为s-III笼形冰（ρ=0.593 g/cm³）和s-IV笼形冰（ρ=0.506 g/cm³）。s-III笼形冰的元胞由2个二十六面体的大笼子（8⁶6⁸4¹²）和6个十面体的小笼子（8²4⁸）组成。s-IV笼形冰的元胞中含有8个二十六面体的大笼子（12⁴6⁴4¹⁸）、8个十二面体的中等尺寸笼子（6⁶4⁶）和6个八面体的小笼子（6²4⁶）。对于这两种笼形冰，超大尺寸的二十六面体水笼子以及不同笼子之间的独特堆积方式使它们的密度极低。把所有低密度冰相（其密度小于或者等于ice XI）考虑在内，我们基于TIP4P/2005模型势函数构建了水在负压下的p-T（压强-温度）相图。在s-II笼形冰下方的极低负压区域内，s-III和s-IV笼形冰取代了之前认为的s-H笼形冰，分别占据了高温和低温部分，因此在相图中产生了一个三相点（T=115 K，p=–488.2 MPa）。密度泛函理论计算表明，通过在二十六面体大笼子中添加合适尺寸的客体分子，比如十二面烷（C₂₀H₂₀）和富勒烯（C₆₀），能够分别充分地稳定s-III和s-IV笼形冰晶格。基于实验室中已经制备出的无客体分子填充的s-II笼形冰，且被认定为ice XVI相，那么s-III和s-IV笼形冰很可能是ice XVIII或ice XIX的候选结构。它们一旦在实验室中被合成，则可以作为一种储存气体的材料用来封装气体分子（如H₂、CH₄、CO₂等）。计算表明：s-III笼形冰在低温和室温下的储氢能力均为s-II的两倍左右，达到了美国能源部在海陆运输上制订的储氢目标。
- 笼形冰 /
- 相图 /
- 负压 /
- 超低密度
Abstract: Water is not only omnipresent on the Earth but also ubiquitous in the solar system such as on comets, asteroids, or icy moons of the giant planets. Hence, exploration of different forms of ice in different environment has significant implication to physical science, chemical science, bioscience, geoscience and planetary science. Depending on the surrounding conditions of pressure and temperature, water ice exhibits an exceptionally rich and complicated phase diagram. To date, at least eighteen crystalline ice phases (ice Ih, Ic, ice II to ice XVII) have been identified under laboratory conditions. In addition, there are many hypothetical ultralow-density ice phases from clathrate hydrates, such as structure I (s-I), structure II (s-II), structure H (s-H), structure K (s-K) and structure T (s-T) ices. Recently, the s-II clathrate ice (ice XVI) produced in the laboratory emerges in the negative pressure part of phase diagram, which stimulates greatly people to explore the other low-density clathrate ices. Using extensive Monte Carlo packing algorithm, classical molecular dynamins simulations, and dispersion-corrected density functional theory optimization, we predict two cubic clathrate ices with ultralow densities, and name them as s-III (ρ=0.593 g/cm³) and s-IV (ρ=0.506 g/cm³) clathrate ices. The unit cell of s-III clathrate ice is composed of two large icosihexahedral cavities (8⁶6⁸4¹²) and six small decahedral cavities (8²4⁸), while the unit cell of s-IV clathrate ice is constructed by eight large icosihexahedral cavities (12⁴6⁴4¹⁸), eight intermediate dodecahedral cavities (6⁶4⁶), and six small octahedral cavities (6²4⁶). For these two clathrate ices, the large-sized icosihexahedral cavities and the unique packed patterns among different cavities result in their record low densities. Considering all the low-density (lower than ice XI or equal to ice XI) ices, we construct a new p-T (pressure-temperature) phase diagram of water with TIP4P/2005 model potential under negative pressures. Below the deeply negative-pressure region of s-II clathrate ice, s-III and s-IV clathrate ices replace s-H clathrate ice, arising as the most stable ice phases in the high-temperature part and the low-temperature part, respectively. As a result, a triple point (T = 115 K, p = –488.2 MPa) appears in the phase diagram. The density functional theory calculations suggest that the s-III and s-IV clathrate ices can be fully stabilized by encapsulating an appropriate guest molecule such as dodecahedrane molecule (C ₂₀H₂₀) and fullerene molecule (C₆₀) in the large cavity, respectively. Considering that the guest-free s-II clathrate ice has been produced in the laboratory, which is also recognized as ice XVI, both the s-III and s-IV clathrate ices can be viewed as potential candidates of ice XVIII or ice XIX. Computations show that the hydrogen storage capacities of s-III ice clathrate amount to nearly twice of those for the s-II ice clathrate at low temperature and room temperature, which satisfies the DOE ultimate target for on-board hydrogen storage.
- clathrate ice /
- phase diagram /
- negative pressure /
- ultralow-density

HTML全文

泡沫铝作为一种新型功能与结构材料在近几年被广泛应用。其自身独特的多孔结构决定了它具有低密度、高孔隙率和大的比表面积。这些特性使它具有隔音降噪、缓冲吸能等多种作用，被广泛应用于航空航天、国防军事、汽车防护等领域。已有研究表明：泡沫材料在压缩过程中的应力-应变曲线呈现明显的3个阶段，分别是线弹性阶段、塑性平台阶段和密实阶段^[1]，其中塑性平台阶段是由于胞孔大量坍塌产生的，该过程能够吸收较多的能量，胞孔的破坏模式呈现多样化，因此研究泡沫铝胞孔的破坏模式及微观变形机理对提高泡沫铝的吸能效率有着重要意义。

泡沫铝变形过程中往往呈现出典型的不均匀压缩特性，利用数字散斑和图像相关方法研究其变形特征具有全场性和直观性等优点^[2-3]。魏志强等^[4]利用高速摄影技术对泡沫铝的分离式霍普金森压杆（SHPB）实验进行了跟踪拍摄，发现利用图像处理软件分析所得到的应变结果与SHPB后处理得到的应变结果基本一致。Jung等^[5]利用数字图像相关法对Ni/Al复合开孔泡沫铝的微观变形进行了研究，发现这种方法可以有效地观察到泡沫铝的微观变形。房亮等^[6]通过数字图像相关法研究了闭孔泡沫铝的压缩力学行为，认为闭孔泡沫铝在弹性范围内受压时具有较高的线性度，且发现单个孔的变形特征与孔壁的形态有关。章超等^[7]基于数字图像相关法的原理对泡沫铝的冲击压缩过程进行了拍摄跟踪，结果表明在压缩过程中会随机产生多个变形带，形状主要有斜“I”型和“V”型。Kadkhodapour等^[8]、杨福俊等^[9]在对闭孔泡沫铝变形的研究中发现，泡沫铝的宏观变形受单个胞体变形的影响，且单个胞体的变形模式与胞体的形状以及胞体分布的随机性有关。在泡沫铝研究中以闭孔泡沫铝较多^[10-12]。潘艺等^[13]认为基体材料和相对密度影响泡沫铝的变形特性，且变形特性也与胞孔分布的随机性有关。Mu等^[14]提出胞体的变形与自身的形态有关，且存在4种失效模式。杨宝等^[15]通过观察冲击过程中试件的变形图，发现泡沫铝在动态下的破坏模式与准静态下的类似，变形破坏模式有节点旋转变形、悬臂壁弯曲变形、剪切变形破坏、水平曲壁压弯变形以及斜向细孔壁屈曲变形等。

球形孔开孔泡沫铝由于胞元尺寸和形状统一，在各个方向上的力学性能基本一致，闭孔泡沫铝相对密度较低，且陈永涛等^[16]认为相对密度对吸能效率的极值影响较小，并得出闭孔泡沫铝单位体积吸收的能量低于开孔泡沫铝的结论。对开孔泡沫铝应变率效应的研究结果不一：Deshpande^[17]、Mukai^[18]等的研究表明，开孔泡沫铝对应变率不敏感；程和法等^[19]认为泡沫铝的压缩性能具有明显的应变率效应，且应变率越高，吸能效果越好。球形孔开孔泡沫铝由于存在孔壁，兼具通孔和闭孔泡沫铝的特征，可以在某些特殊应用中发挥缓冲耗能的作用。然而，球形孔开孔泡沫铝在压缩载荷下的力学性能、变形特征和细观机理尚不清楚，传统泡沫铝在由变形集中带演化主导的应力平台阶段内材料整体和胞元孔的变形如何影响球形孔泡沫铝的力学行为也亟需研究。基于此，本研究首先针对球形孔开孔泡沫铝的静-动态力学性能进行实验研究，再利用数字图像相关技术对其在准静态压缩下的介观变形机制进行分析。

1.   静-动态力学性能和行为实验

实验材料选用北京强业泡沫金属公司提供的球形孔开孔泡沫铝，基体材料为纯铝，采用造孔剂渗流法制备，胞孔直径6 mm，壁面连通孔孔径1～2 mm，试样密度0.9～1.0 g/cm³。静态力学性能实验的试样尺寸为ø30 mm×35 mm，采用电子万能试验机测试。动态力学性能实验分别采用落锤试验机和SHPB，试样尺寸分别为ø30 mm×35 mm和ø30 mm×20 mm。落锤质量约40 kg，冲击高度约1.2 m，锤头上安装加速度传感器测量冲击过程中的加速度，并通过积分换算得到工程应力-应变曲线。SHPB装置杆件直径为50 mm，子弹、入射杆和透射杆长度分别为1.0、2.5和2.5 m，考虑到泡沫材料的透射波信号较弱，采用半导体应变计测量透射波。另外，为研究准静态加载下泡沫铝和胞元孔的具体变形模式，采用GOM 5M三维全场动态测量系统拍摄球形开孔泡沫铝的准静态压缩过程，基于ARAMIS软件对采集图片进行图像处理，获得位移场和应变场信息。实验装置见图1。实验所用两部相机的焦距均为400 mm，分辨率为2 448×2 050像素，标定视场尺寸为44 mm×55 mm。考虑到泡沫铝表面不规则，散斑实验采用矩形试样，尺寸为35 mm×35 mm×35 mm，在观测面喷涂黑白相间随机分布的散斑场（见图2）。加载速率1 mm/min，图像采集间隔为2 s。

图 1 三维全场应变测量系统

Figure 1. Three dimensional full-field strain measurement system

下载: 全尺寸图片幻灯片

图 2 泡沫铝试样及喷散斑后试样

Figure 2. Foamed aluminum sample and speckle sample

下载: 全尺寸图片幻灯片

2.   实验结果与讨论

2.1   力学性能分析

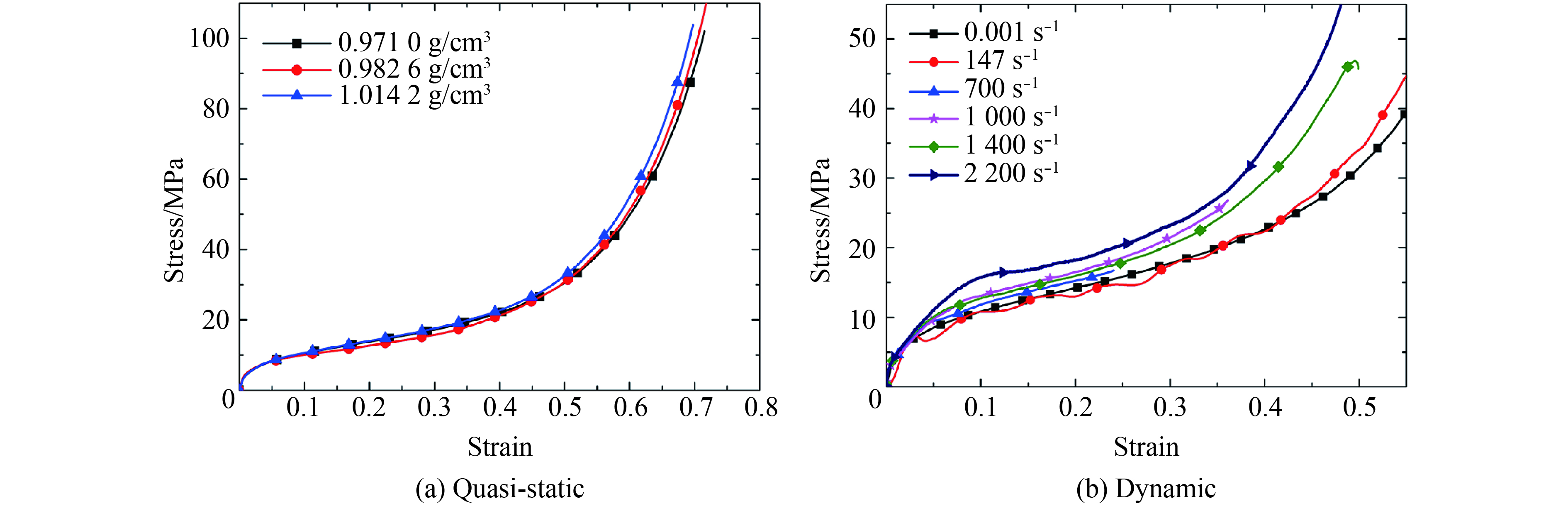
对泡沫铝力学性能进行分析，图3(a)为准静态压缩过程中泡沫铝的应力-应变曲线，可知:曲线较为光滑平缓，与胞元孔结构和尺寸一致性较高有关;平台阶段较为平稳，主要是由于孔壁厚度较大，胞元孔坍塌时承载能力没有突然降低，体现了球形孔泡沫铝的优点。

动态实验曲线由相同应变率下3组实验曲线的平均值获得，且取0.05应变下的应力为屈服应力^[20]。对比不同应变率下的应力-应变曲线（图3(b)）可知，屈服强度在应变率为0.001 s^–1时为8.592 MPa，随着应变率的增大，屈服强度增大，在应变率为2 200 s^–1时为15.387 MPa，增大了80%。为了定量分析能量吸收特性^[20]，对比可知20%应变对应的流动应力从14.205 MPa增大到18.236 MPa，提高了28%，吸收能量从2.03 MJ/m³增大到2.78 MJ/m³，增加了40%。文献[21]指出泡沫铝的平台应力接近应变量为0.2时的流动应力，可见该泡沫铝的静、动态力学性能差异显著，存在明显的应变率效应，且动态冲击下泡沫铝具有更高的屈服强度，能吸收更多能量，动态吸能效率的提高说明球形孔泡沫铝具有优异的力学性能，更有利于其作为高速缓冲吸能结构的芯层。

2.2   变形模式分析

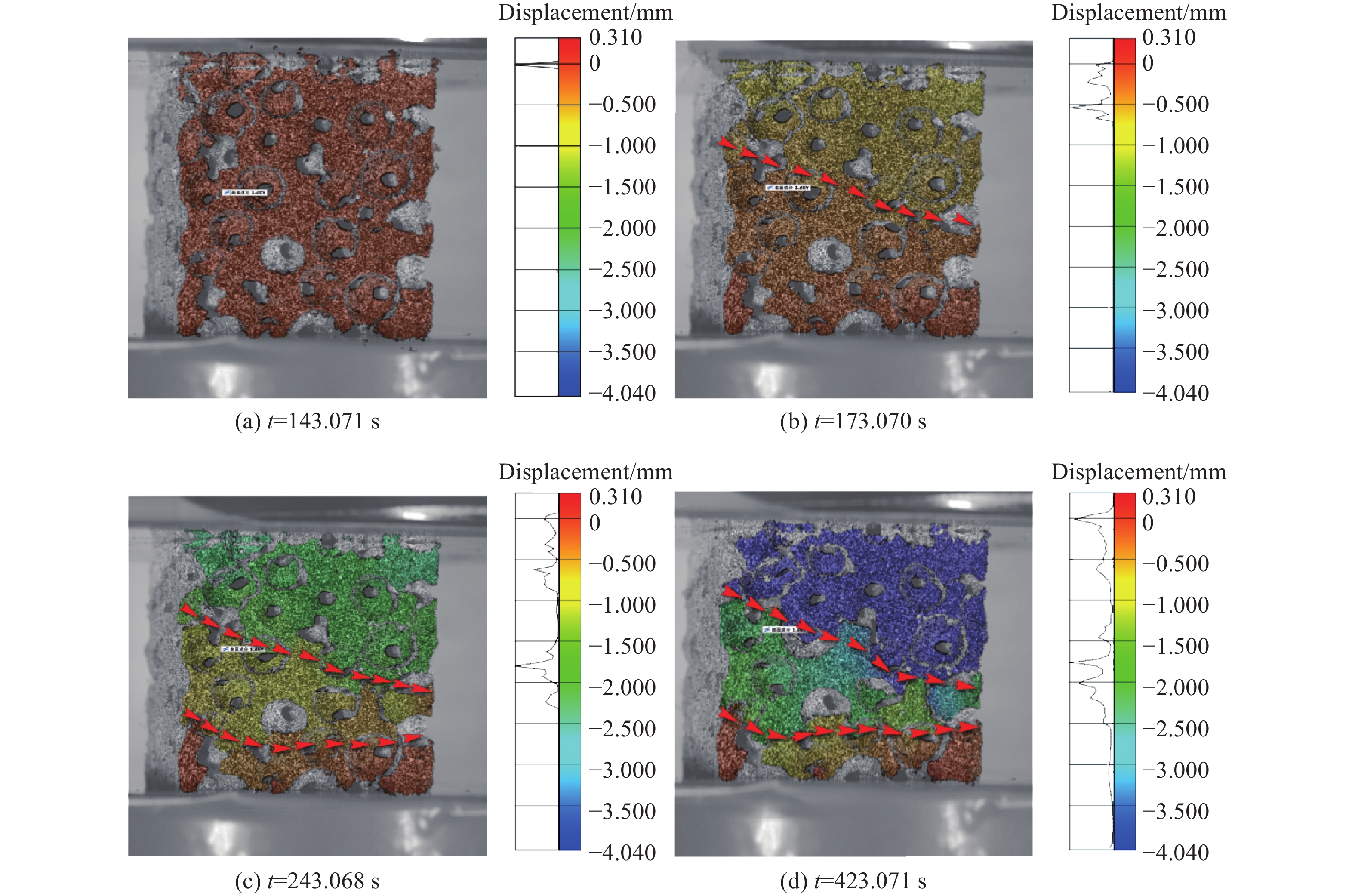
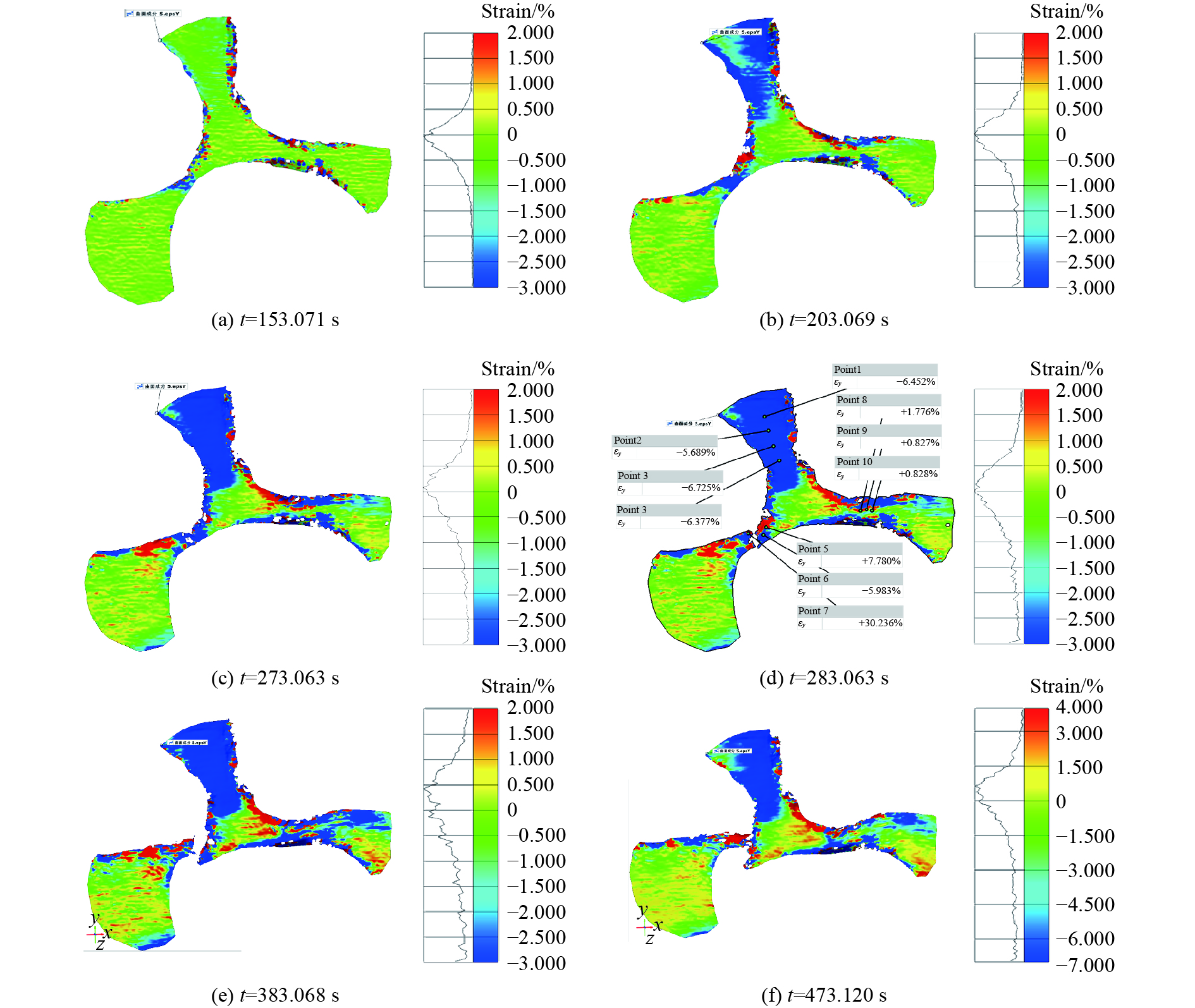
图4为球形开孔泡沫铝压缩过程位移场，可见，在压缩时间t=173.070 s时（见图4（b））虚线位置出现一条局部变形带，随着加载的进行出现第二条变形带（图4（c）、4（d））。局部变形带的产生是泡沫铝胞孔不同形式的坍塌造成的，与胞孔的分布以及孔壁的位置有关，最先发生坍塌的胞孔组成了第一条变形带，这种现象与闭孔泡沫铝相似，都是局部变形带的产生和演化导致材料应力-应变曲线出现典型的平台阶段。由图3中泡沫铝的应力-应变曲线可知，在平台阶段泡沫铝吸收大量能量，这一阶段就是胞孔大量坍塌出现局部变形带的过程。

图 4 压缩过程泡沫铝表面位移场分布

Figure 4. Displacement field distribution of foamed aluminum surface during compression

下载: 全尺寸图片幻灯片

图 3 泡沫铝的压缩应力-工程应变曲线

Figure 3. Compressive stress-engineering strain curve of aluminum foam

下载: 全尺寸图片幻灯片

通过观察与统计胞孔破坏模式，发现胞孔的变形模式主要有3种，如图5所示，其中：图5（a）为孔壁屈曲变形，图5（b）中的孔发生了扭转变形，图5（c）显示在压缩时孔壁既发生扭转变形又存在剪切变形。这与文献[9]中提到的闭孔泡沫铝胞孔的变形模式类似。

图 5 胞孔的变形模式

Figure 5. Deformation mode of cell

下载: 全尺寸图片幻灯片

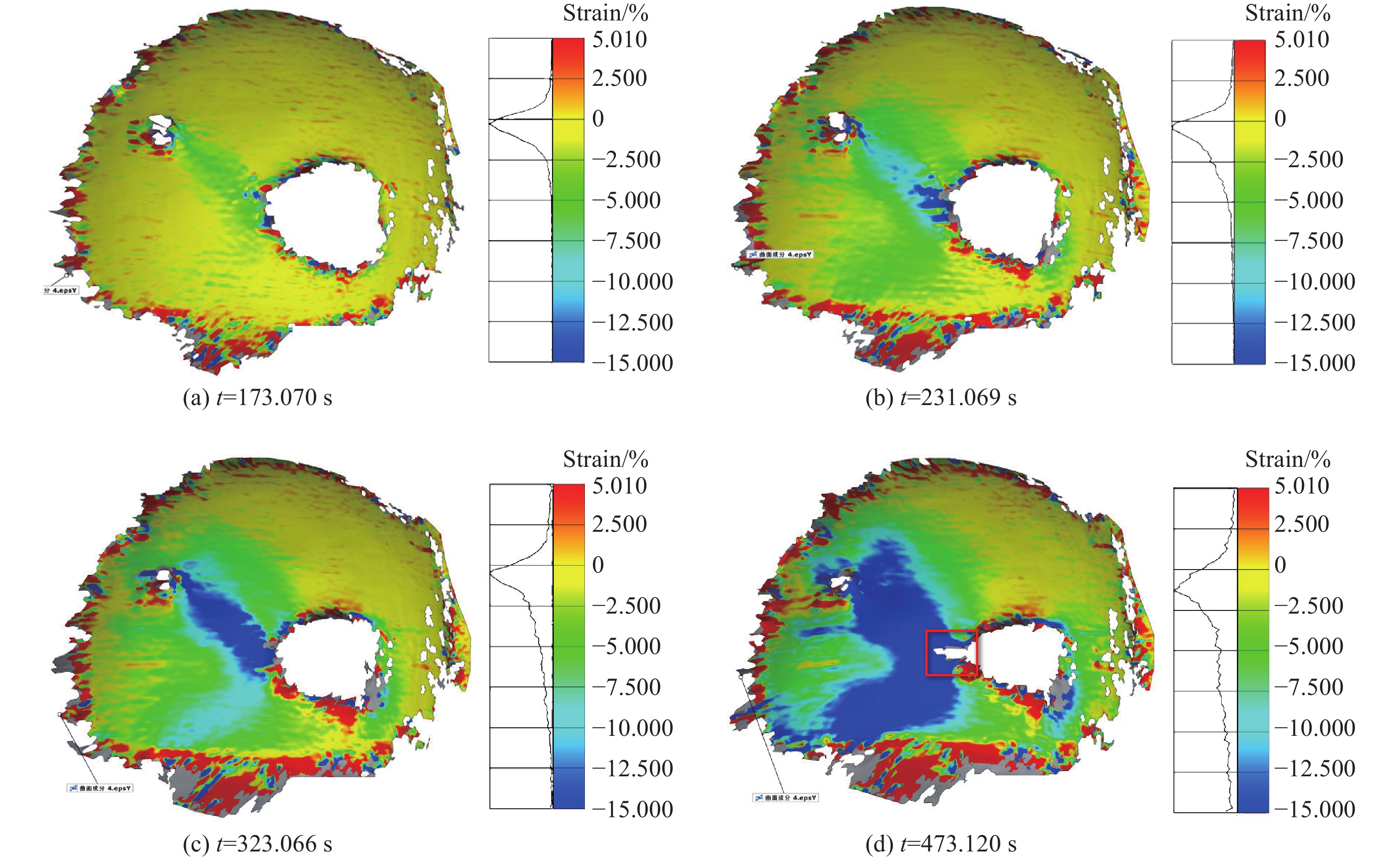
为了分析泡沫铝的介观变形机制，选取单个孔的应变场（图6（a））进行分析。图6（c）为孔的侧面图，可以看出是一个半球形。由该胞孔的应变场（图7）可以看出，在加载时间为173.070 s时，在胞孔壁上的通孔边界处出现一条变形带；继续加载时，在同一起始位置出现第二条变形带，且变形带上应变较大，单个胞孔在压缩变形过程中的应变分布存在很明显的不均匀性。两条变形带的起始位置相同，都是从胞孔上通孔的缺陷处开始，即图6（b）红框中的缺口，且胞孔向后凸起，导致在压缩过程中变形沿着局部变形带发生屈曲；在压缩时间为473.120 s时（见图7（d））缺口变深，胞孔局部变形带就是由于缺口处的应力集中造成的，且多数胞孔情况类似。由此可知，开孔泡沫铝在压缩过程中单个胞体孔壁上由于孔壁缺陷处的应力集中会出现多条变形带，且由于孔壁的凸起，导致胞孔轴向屈曲。

图 6 单胞孔位置与其孔的侧面应变场

Figure 6. Location of the single cell on its surface and lateral strain field map on the side of the celll

下载: 全尺寸图片幻灯片

图 7 单胞压缩过程应变场分布

Figure 7. Distribution of strain field on the surface of a single cell during compression

下载: 全尺寸图片幻灯片

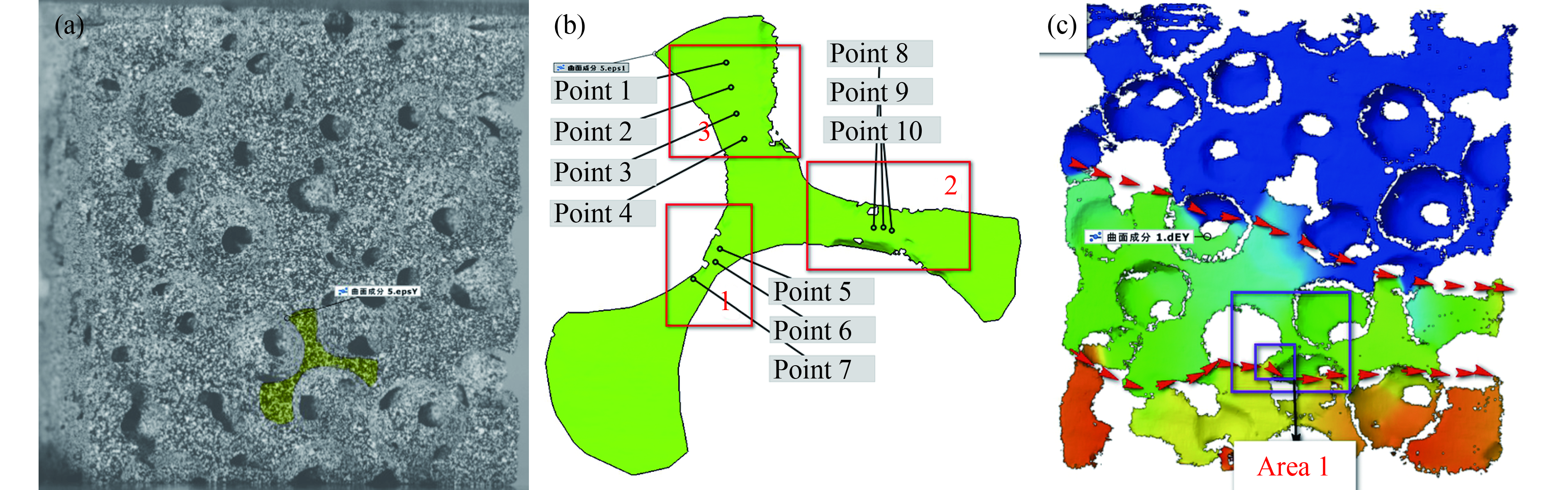
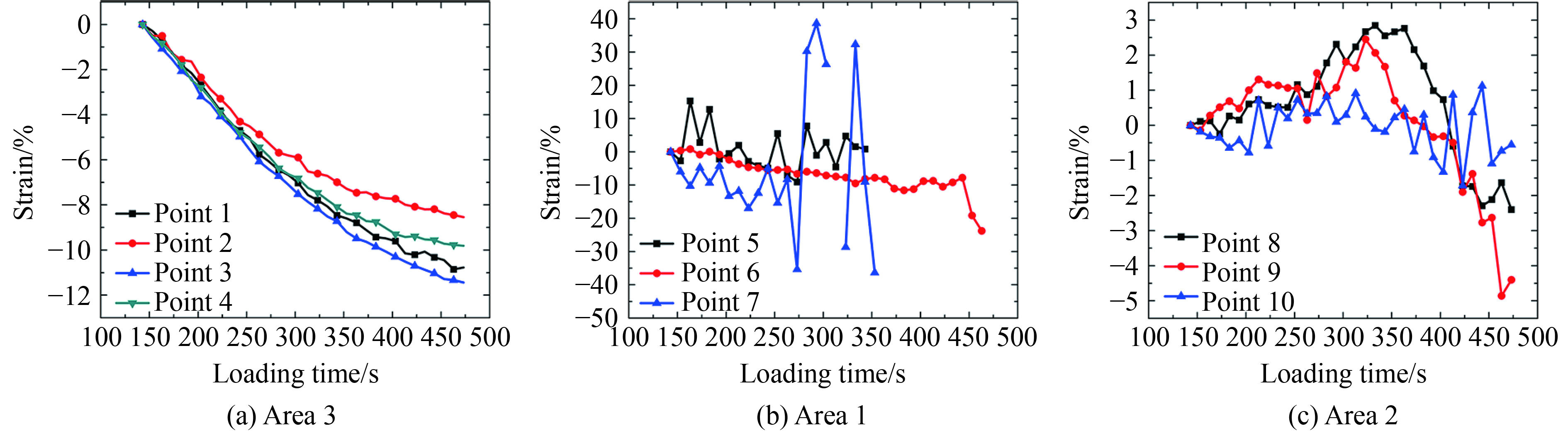
为了分析孔壁的变形模式，选取如图8所示3个厚度不同、方向不同的孔壁组成的区域，单个孔壁呈现“I”型，该结构在泡沫铝中较为普遍，且1区孔壁在变形带处，“I”型孔壁的断裂与破坏直接导致了孔的坍塌变形。在3个孔壁上各选几个点（图8（b）），由分析软件计算出各点的应变-时间曲线，如图9所示。1区上的点既有压应变又有拉应变，在加载时间273.063 s后孔壁有了明显破坏，而在孔壁破坏的过程中，由图9(b)与图10(d)都可以看出此时点7有较大的拉应变，达到30%，而点6上压应变较大，因此1区孔壁在破坏过程中受到过较大拉应力，且最终断裂，过程中存在剪切破坏。在153.071 s时，3区上的点1、2、3、4都为压应变，呈线性增大，即孔壁变形模式为孔壁屈曲变形，2区上的点8、9、10处既存在压应变又存在拉应变，且2区在1区孔壁破坏并最终断裂前变形很小，在1区断裂后其变形明显，孔壁上点的拉应变增大，因此可以判断该孔壁是由于1区孔壁破坏造成的扭转与剪切的复合变形。可见在泡沫铝的压缩过程中胞孔的变形模式是由于孔壁变形的多样化造成的，孔壁的变形模式主要有孔壁屈曲变形、剪切、扭转加剪切复合变形3种，最先发生破坏的孔壁变形模式为剪切变形。

图 8 泡沫铝观测面不同位置孔壁

Figure 8. Hole wall at different positions on the observation surface of aluminum foam

下载: 全尺寸图片幻灯片

图 9 孔壁各点应变-时间曲线

Figure 9. Strain-time curve of each point on the hole wall of foamed aluminum

下载: 全尺寸图片幻灯片

图 10 孔壁不同时刻应变场分布

Figure 10. Strain field distribution of aluminum foam wall at different time

下载: 全尺寸图片幻灯片

经过以上对宏观与介观的分析可以发现，整体变形带的产生与胞孔的变形有关，胞孔的变形模式由孔壁的变形模式决定，孔壁的破坏直接造成了胞孔的坍塌，而胞孔的坍塌又明显地反映出局部变形带的存在。孔壁的3种变形模式决定了胞孔的变形模式，且局部变形带本身由最先发生破坏的孔壁连接而成，对多组实验的统计表明，多条变形带上孔壁的破坏模式以剪切破坏为主。孔壁的变形模式与孔壁的厚度以及方向有关，3种变形模式中剪切变形最不稳定，导致孔壁最先破坏，并出现局部变形带。

3.   结　论

利用三维全场应变测量系统全面分析了球形开孔泡沫铝在准静态压缩下的介观变形，得到以下结论。

（1）球形孔开孔泡沫铝具有明显的应变率效应，随着应变率的增加，屈服强度增加，平台段提高，且从准静态到应变率为2000 s^–1的过程中，应变在0.2时能量吸收增加40%。

（2）球形孔开孔泡沫铝在细观结构和变形行为上接近于传统闭孔泡沫金属，变形集中带的产生和演化主导了材料的屈服平台阶段行为，局部变形带的产生机理与闭孔泡沫铝类似。

（3）单个胞体在压缩过程中会在孔壁缺陷处出现局部变形带，且不止一条，主要是由于缺陷位置经过压缩后出现的应力集中造成的。

（4）胞孔的变形模式主要有3种，屈曲变形、剪切变形、扭转加剪切复合变形；主要由孔壁的3种变形模式决定，孔壁的变形模式与孔壁的厚度以及加载方向有关。

图 1 水冰相图^[2]

Figure 1. Phase diagram of water ice^[2]

下载: 全尺寸图片幻灯片

图 2 证实一个大气压存在(a)和Huygens证实负压存在的实验示意(b)（76 cm的水银柱以上表示负压）^[39]

Figure 2. Schematic representation of experiment to demonstrate the existence of the atmospheric pressure (a) and Huygens’s experiment to generate negative pressure under laboratory condition (b) (Above the approximately 76 cm mercury line the pressure is below zero^[39].)

下载: 全尺寸图片幻灯片

图 3 TIP4P/2005模型下水的负压相图^[25]

Figure 3. Phase diagram of water in negative pressures with the TIP4P/2005 model^[25]

下载: 全尺寸图片幻灯片

图 4 THF+H₂水合物中H₂的含量随THF浓度变化的关系曲线以及相应体系中H₂分布情况的结构示意^[52]

Figure 4. H₂ gas content as a function of THF concentration, and a schematic diagram of H₂ distribution in the cages of THF+H₂ hydrate^[52]

下载: 全尺寸图片幻灯片

图 5 s-III笼形冰的结构示意:(a) 组成s-III相的两种水笼子（下面是由48个水分子形成的8⁶6⁸4¹²笼子, 上面是由16个水分子形成的8²4⁸笼子；只显示了氧原子骨架）, (b)和(c) 分别是1×2超胞和2×2超胞（蓝色虚线表示氢键，红色球表示氧原子，白色棍棒表示氢原子）

Figure 5. Structure of s-III ice clathrate: (a) Two types of building water cages (bottom: 8⁶6⁸4¹², 48-molecule; top: 8²4⁸, 16-molecule; only oxygen frameworks are shown); repeated unit cells (1×2) (b) and 2×2 unit cells (c) (The hydrogen bond network is shown with blue dash line, red ball for oxygen, and white stick for hydrogen.)

下载: 全尺寸图片幻灯片

图 6 ice i、ice XI和笼形冰相s-T、s-I、s-II、s-K、SGT及s-H的晶体结构（2 × 2元胞）(蓝色虚线表示氢键，红球表示氧原子，白球表示氢原子)

Figure 6. Crystal structures (2 × 2 unit cells) of ice i, ice XI, and clathrates of s-T, s-I, s-II, s-K, SGT and s-H (blue dash line for hydrogen bond, red ball for oxygen and white stick for hydrogen)

下载: 全尺寸图片幻灯片

图 7 ice XI、ice i、s-K、s-I、s-II、s-H、s-III、SGT和s-T冰相的晶格结合能（平均到每个分子上）随体积变化的函数曲线（插图是42~48 Å³体积区间内的放大函数曲线。E_latt定义为E_latt = E_w ‒ E_cry/N，其中：N是晶体中水分子的数目，E_cry和E_w分别是晶体的总能和单个水分子的能量。）

Figure 7. Lattice cohesive energies (E_latt) for ice XI, ice i, s-K, s-I, s-II, s-H, s-III, SGT, and s-T clathrates as function of volume per water molecule (Inset is amplification of the region for the volume between 42–48 Å³. E_latt is defined as E_latt = E_w – E_cry/N, where N is the number of water molecules in the crystal, E_cry and E_w are the total energies of the ice/clathrate crystal and an individual water molecule, respectively.)

下载: 全尺寸图片幻灯片

图 8 s-I、s-II、s-H、s-III、SGT、s-K、s-T和ice i的相对焓（以ice XI为参考相）随负压变化的函数曲线

Figure 8. Relative enthalpy versus negative pressure for clathrate phases s-I, s-II, s-H, s-III, SGT, s-K, s-T, and ice i with ice XI as a reference

下载: 全尺寸图片幻灯片

图 9 负压区域内TIP4P/2005水模型的p-T相图(液态水与Ih、s-II相的共存曲线来源于文献[26])

Figure 9. p-T phase diagram of TIP4P/2005 water model in the region of negative pressures (The phase boundaries between liquid water and Ih or s-II ice phases are taken from Ref. [26].)

下载: 全尺寸图片幻灯片

图 10 (a) C₂₀H₂₀分子封装在8⁶6⁸4¹²水笼子中的结构示意；(b) 每个大笼子均被一个C₂₀H₂₀分子占据时s-III笼形冰的结构示意（显示的是2×2的超胞）

Figure 10. (a) Structure of an individual 8⁶6⁸4¹²water cage with a C₂₀H₂₀ molecule encapsulated; (b) Structure of the s-III clathrate with one C₂₀H₂₀ molecule encapsulated in each large cavity (2×2 unit cell is shown for clearer view.)

下载: 全尺寸图片幻灯片

图 11 在温度分别为77、240和298 K时，s-III和s-II笼形冰相在不同氢压下对氢气的吸附函数曲线（中间图形中的黑色方框表示在温度为240 K、压强为300 MPa条件下获取的实验值^[48-49]）

Figure 11. Hydrogen uptake versus hydrogen pressure for empty s-III and s-II ice clathrate lattices at temperatures of 77, 240 and 298 K, respectively (In the middle panel, the corresponding experimental value ^[48-49] for the s-II ice clathrate at 240 K and 300 MPa is marked by a black square.)

下载: 全尺寸图片幻灯片

图 12 s-IV笼形冰的晶体结构：(a) 3种类型的水笼子（只给出氧原子骨架），从左到右依次是具有T对称性的48元12⁴6⁴4¹⁸大笼子、具有T对称性的24元6⁶4⁶中等尺寸笼子、具有S₆对称性的12元6²4⁶小笼子；(b) 和 (c) 是s-IV笼形冰立方元胞示意（蓝色虚线表示氢键，红球代表氧原子，白棍代表氢原子）

Figure 12. Crystalline structure of the s-IV ice clathrate: (a) Three types of cavities (only oxygen frameworks are shown), from left to right they are large cavity—48-member 12⁴6⁴4¹⁸with T symmetry, intermediate cavity—24-member 6⁶4⁶ with T symmetry, and small cavity—12-member 6²4⁶ with S₆ symmetry, respectively; (b) and (c) are the cubic unit cell of the s-IV ice clathrate (The hydrogen-bonding network is shown with blue dash line, red for oxygen, white for hydrogen.)

下载: 全尺寸图片幻灯片

图 13 笼形冰相s-II、s-III和s-IV的相对焓值随压强变化的函数曲线（以ice XI相的焓值为参考）

Figure 13. Relative enthalpy per water molecule as a function of pressure for s-II, s-III, and s-IV ice clathrates, with the ice XI being as a reference

下载: 全尺寸图片幻灯片

图 14 TIP4P/2005模型下的水冰负压相图（s-II、ice XI和液态水的相边界曲线来自于同一水模型下的研究^[26]）

Figure 14. Phase diagram of water ice at negative pressures based on the TIP4P/2005 water model (The phase boundaries among s-II, ice XI, ice Ih, and liquid water are taken from a previous study^[26] with the same TIP4P/2005 model.)

下载: 全尺寸图片幻灯片

表 1 不同冰相和无客体分子填充的笼形冰的元胞内分子数目(Z_cell)、元胞的平衡体积(V_cell)、平均O-O距离(d_O-O)、密度(ρ)以及平均到每个分子上的晶格结合能(E_latt)(括号内的数据源于实验值)

Table 1. Number of water molecules per unit cell (Z_cell), equilibrium volume of unit cell (V_cell), average distance between oxygen atoms in adjacent water molecules (d_O-O), density (ρ), and lattice cohesive energy per water molecule (E_latt) for various ice and guest-free clathrate phases (The values in parenthesis are experimental data.)

Phase	Z_cell	V_cell/Å³	d_O-O/Å	ρ/(g·cm^-3)	E_latt/(kJ·mol^-1)
ice XI	8	266 (257^[17])	2.785 (2.735^[17])	0.900 (0.930^[17])	62.84 (58.86^[74])
ice i	8	280	2.785	0.855	61.31
s-I	46	1 692	2.765	0.813	61.38
s-K	80	2 962	2.765	0.808	60.76
s-II	136	5 059 (5 022^[22])	2.865(2.751^[22])	0.804 (0.81^[22])	61.37
s-T	12	453	2.795	0.792	60.23
s-H	34	1 325	2.785	0.768	60.79
SGT	64	2 650	2.765	0.722	59.27
s-III	48	2 423	2.765	0.593	55.77

下载: 导出CSV

表 2 通过vdW-DF2/DFT计算得到的ice XI、s-II、s-III和s-IV冰相元胞内的分子数目(Z_cell)、元胞的平衡体积(V_cell)、平均O-O距离(d_O-O)、平均氢键键长(d_O···H)、密度(ρ)、平均到每个水分子上的晶格结合能(E_latt)（括号内的数据是实验值）

Table 2. Number of water molecules per unit cell (Z_cell), equilibrium volume of unit cell (V_cell), average distance between oxygen atoms in adjacent water molecules (d_O-O), average length of hydrogen bond (d_O···H), mass density (ρ), and lattice cohesive energy per water molecule (E_latt) from vdW-DF2/DFT calculations for ice XI, s-II, s-III and s-IV ice clathrates (The values in parenthesis are experimental values.)

Phase	Z_cell	V_cell/Å³	d_O-O/Å	d_O…H/Å	ρ/(g·cm^-3)	E_latt/(kJ·mol^-1)
ice XI	8	265 (267^[17])	2.785 (2.735^[17])	1.785	0.903 (0.93^[17])	65.64 (63.86^[74])
s-II	136	5 190 (5 022^[22])	2.785 (2.751^[22])	1.795	0.784 (0.81^[22])	64.08
s-III	48	2 426	2.765	1.795	0.592	58.64
s-IV	192	11 350	2.815	1.855	0.506	58.23

下载: 导出CSV

参考文献(79)

[1]	SALZMANN C G, RADAELLI P G, SLATER B, et al. The polymorphism of ice: five unresolved questions [J]. Physical Chemistry Chemical Physics, 2011, 13(41): 18468–18480. doi: 10.1039/c1cp21712g
[2]	BARTELS-RAUSCH T, BERGERON V, CARTWRIGHT J H E, et al. Ice structures, patterns, and processes: a view across the icefields [J]. Reviews of Modern Physics, 2012, 84(2): 885–944. doi: 10.1103/RevModPhys.84.885
[3]	KUHS W F, LEHMANN M S. The structure of the ice Ih by neutron diffraction [J]. The Journal of Physical Chemistry, 1983, 87(21): 4312–4313. doi: 10.1021/j100244a063
[4]	ANDERSSON O, SUGA H. Thermal conductivity of amorphous ices [J]. Physical Review B, 2002, 65(14): 140201. doi: 10.1103/PhysRevB.65.140201
[5]	HANSEN T C, FALENTY A, KUHS W F. Modelling ice Ic of different origin and stacking-faulted hexagonal ice using neutron powder diffraction data [J]. Special Publication-Royal Society of Chemistry, 2006, 311: 201.
[6]	MURRAY B J, BERTRAM A K. Formation and stability of cubic ice in water droplets [J]. Physical Chemistry Chemical Physics, 2006, 8(1): 186–192. doi: 10.1039/B513480C
[7]	MALKIN T L, MURRAY B J, BRUKHNO A V, et al. Structure of ice crystallized from supercooled water [J]. Proceedings of the National Academy of Sciences, 2012, 109(4): 1041–1045. doi: 10.1073/pnas.1113059109
[8]	KAMB B. Ice II. a proton-ordered form of ice [J]. Acta Crystallographica, 1964, 17(11): 1437–1449. doi: 10.1107/S0365110X64003553
[9]	FORTES A D, WOOD I G, ALFREDSSON M, et al. The incompressibility and thermal expansivity of D₂O ice II determined by powder neutron diffraction [J]. Journal of Applied Crystallography, 2005, 38(4): 612–618. doi: 10.1107/S0021889805014226
[10]	LONDONO J D, KUHS W F, FINNEY J L. Neutron diffraction studies of ices III and IX on under-pressure and recovered samples [J]. The Journal of Chemical Physics, 1993, 98(6): 4878–4888. doi: 10.1063/1.464942
[11]	ENGELHARDT H, KAMB B. Structure of ice IV, a metastable high-pressure phase [J]. The Journal of Chemical Physics, 1981, 75(12): 5887–5899. doi: 10.1063/1.442040
[12]	KAMB B, PRAKASH A, KNOBLER C. Structure of ice V [J]. Acta Crystallographica, 1967, 22(5): 706–715. doi: 10.1107/S0365110X67001409
[13]	KUHS W F, FINNEY J L, VETTIER C, et al. Structure and hydrogen ordering in ices VI, VII, and VIII by neutron powder diffraction [J]. The Journal of Chemical Physics, 1984, 81(8): 3612–3623. doi: 10.1063/1.448109
[14]	JORGENSEN J D, WORLTON T G. Disordered structure of D₂O ice VII from in situ neutron powder diffraction [J]. The Journal of Chemical Physics, 1985, 83(1): 329–333. doi: 10.1063/1.449867
[15]	BESSON J M, PRUZAN P, KLOTZ S, et al. Variation of interatomic distances in ice VIII to 10 GPa [J]. Physical Review B, 1994, 49(18): 12540–12550. doi: 10.1103/PhysRevB.49.12540
[16]	HEMLEY R J, JEPHCOAT A P, MAO H K, et al. Static compression of H₂O-ice to 128 GPa (1.28 Mbar) [J]. Nature, 1987, 330(6150): 737–740. doi: 10.1038/330737a0
[17]	LEADBETTER A J, WARD R C, CLARK J W, et al. The equilibrium low-temperature structure of ice [J]. The Journal of Chemical Physics, 1985, 82(1): 424–428. doi: 10.1063/1.448763
[18]	SALZMANN C G, KOHL I, LOERTING T, et al. Pure ices IV and XII from high-density amorphous ice [J]. Canadian Journal of Physics, 2003, 81(1): 25–32.
[19]	KOZA M, SCHOBER H, T LLE A, et al. Formation of ice XII at different conditions [J]. Nature, 1999, 397(6721): 660–661.
[20]	SALZMANN C G, RADAELLI P G, HALLBRUCKER A, et al. The preparation and structures of hydrogen ordered phases of ice [J]. Science, 2006, 311(5768): 1758–1761. doi: 10.1126/science.1123896
[21]	SALZMANN C G, RADAELLI P G, MAYER E, et al. Ice XⅤ: a new thermodynamically stable phase of ice [J]. Physical Review Letters, 2009, 103(10): 105701. doi: 10.1103/PhysRevLett.103.105701
[22]	FALENTY A, HANSEN T C, KUHS W F. Formation and properties of ice XVI obtained by emptying a type sII clathrate hydrate [J]. Nature, 2014, 516(7530): 231–233. doi: 10.1038/nature14014
[23]	DEL ROSSO L, CELLI M, ULIVI L. New porous water ice metastable at atmospheric pressure obtained by emptying a hydrogen-filled ice [J]. Nature Communications, 2016, 7: 13394. doi: 10.1038/ncomms13394
[24]	RUSSO J, ROMANO F, TANAKA H. New metastable form of ice and its role in the homogeneous crystallization of water [J]. Nature Materials, 2014, 13(7): 733–739. doi: 10.1038/nmat3977
[25]	KOSYAKOV V I, SHESTAKOV V A. On the possibility of the existence of a new ice phase under negative pressures [J]. Doklady Physical Chemistry, 2001, 376(4): 49–51.
[26]	CONDE M M, VEGA C, TRIBELLO G A, et al. The phase diagram of water at negative pressures: virtual ices [J]. The Journal of Chemical Physics, 2009, 131(3): 034510. doi: 10.1063/1.3182727
[27]	HUANG Y, ZHU C, WANG L, et al. A new phase diagram of water under negative pressure: the rise of the lowest-density clathrate s-III [J]. Science Advances, 2016, 2(2): e1501010. doi: 10.1126/sciadv.1501010
[28]	HUANG Y, ZHU C, WANG L, et al. Prediction of a new ice clathrate with record low density: a potential candidate as ice XIX in guest-free form [J]. Chemical Physics Letters, 2017, 671: 186–191. doi: 10.1016/j.cplett.2017.01.035
[29]	MCMAHON J M. Ground-state structures of ice at high pressures from ab initio random structure searching [J]. Physical Review B, 2011, 84(22): 220104. doi: 10.1103/PhysRevB.84.220104
[30]	JI M, UMEMOTO K, WANG C-Z, et al. Ultrahigh-pressure phases of H₂O ice predicted using an adaptive genetic algorithm [J]. Physical Review B, 2011, 84(22): 220105. doi: 10.1103/PhysRevB.84.220105
[31]	WANG Y, LIU H, LV J, et al. High pressure partially ionic phase of water ice [J]. Nature Communications, 2011, 2: 563. doi: 10.1038/ncomms1566
[32]	MILITZER B, WILSON H F. New phases of water ice predicted at megabar pressures [J]. Physical Review Letters, 2010, 105(19): 195701. doi: 10.1103/PhysRevLett.105.195701
[33]	STROBEL T A, SOMAYAZULU M, SINOGEIKIN S V, et al. Hydrogen-stuffed, quartz-like water ice [J]. Journal of the American Chemical Society, 2016, 138(42): 13786–13789. doi: 10.1021/jacs.6b06986
[34]	DEL ROSSO L, GRAZZI F, CELLI M, et al. Refined structure of metastable ice XVII from neutron diffraction measurements [J]. The Journal of Physical Chemistry C, 2016, 120(47): 26955–26959. doi: 10.1021/acs.jpcc.6b10569
[35]	FENNELL C J, GEZELTER J D. Computational free energy studies of a new ice polymorph which exhibits greater stability than ice Ih [J]. Journal of Chemical Theory and Computation, 2005, 1(4): 662–667. doi: 10.1021/ct050005s
[36]	CHOU I-M, SHARMA A, BURRUSS R C, et al. Transformations in methane hydrates [J]. Proceedings of the National Academy of Sciences, 2000, 97(25): 13484–13487. doi: 10.1073/pnas.250466497
[37]	VATAMANU J, KUSALIK P G. Unusual crystalline and polycrystalline structures in methane hydrates [J]. Journal of the American Chemical Society, 2006, 128(49): 15588–15589. doi: 10.1021/ja066515t
[38]	KURNOSOV A, MANAKOV A, YU. KOMAROV V, et al. A new gas hydrate structure [J]. Doklady Physical Chemistry, 2001, 381(5): 303–305.
[39]	IMRE A R. On the existence of negative pressure states [J]. Physica Status Solidi (B), 2007, 244(3): 893–899. doi: 10.1002/(ISSN)1521-3951
[40]	HERBERT E, BALIBAR S, CAUPIN F. Cavitation pressure in water [J]. Physical Review E, 2006, 74(4): 041603.
[41]	DAVITT K, ROLLEY E, CAUPIN F, et al. Equation of state of water under negative pressure [J]. The Journal of Chemical Physics, 2010, 133(17): 174507. doi: 10.1063/1.3495971
[42]	AZOUZI M E M, RAMBOZ C, LENAIN J-F, et al. A coherent picture of water at extreme negative pressure [J]. Nature Physics, 2012, 9(1): 38.
[43]	ZHENG Q, DURBEN D J, WOLF G H, et al. Liquids at large negative pressures: water at the homogeneous nucleation limit [J]. Science, 1991, 254(5033): 829–832. doi: 10.1126/science.254.5033.829
[44]	YANG S H, NOSONOVSKY M, ZHANG H, et al. Nanoscale water capillary bridges under deeply negative pressure [J]. Chemical Physics Letters, 2008, 451(1): 88–92.
[45]	JACOBSON L C, HUJO W, MOLINERO V. Thermodynamic stability and growth of guest-free clathrate hydrates: a low-density crystal phase of water [J]. The Journal of Physical Chemistry B, 2009, 113(30): 10298–10307. doi: 10.1021/jp903439a
[46]	VOS W L, FINGER L W, HEMLEY R J, et al. Novel H₂-H₂O clathrates at high pressures [J]. Physical Review Letters, 1993, 71(19): 3150–3153. doi: 10.1103/PhysRevLett.71.3150
[47]	DYADIN Y A, LARIONOV E G, MANAKOV A Y, et al. Clathrate hydrates of hydrogen and neon [J]. Mendeleev Communications, 1999, 9(5): 209–210. doi: 10.1070/MC1999v009n05ABEH001104
[48]	MAO W L, MAO H-K, GONCHAROV A F, et al. Hydrogen clusters in clathrate hydrate [J]. Science, 2002, 297(5590): 2247–2249. doi: 10.1126/science.1075394
[49]	MAO W L, MAO H-K. Hydrogen storage in molecular compounds [J]. Proceedings of the National Academy of Sciences of the United States of America, 2004, 101(3): 708–710. doi: 10.1073/pnas.0307449100
[50]	LOKSHIN K A, ZHAO Y. Fast synthesis method and phase diagram of hydrogen clathrate hydrate [J]. Applied Physics Letters, 2006, 88(13): 131909. doi: 10.1063/1.2190273
[51]	FLORUSSE L J, PETERS C J, SCHOONMAN J, et al. Stable low-pressure hydrogen clusters stored in a binary clathrate hydrate [J]. Science, 2004, 306(5695): 469–471. doi: 10.1126/science.1102076
[52]	LEE H, LEE J-W, KIM D Y, et al. Tuning clathrate hydrates for hydrogen storage [J]. Nature, 2005, 434(7034): 743. doi: 10.1038/nature03457
[53]	KOMATSU H, YOSHIOKA H, OTA M, et al. Phase equilibrium measurements of hydrogen-tetrahydrofuran and hydrogen-cyclopentane binary clathrate hydrate systems [J]. Journal of Chemical & Engineering Data, 2010, 55(6): 2214–2218.
[54]	STROBEL T A, HESTER K C, SLOAN E D, et al. A hydrogen clathrate hydrate with cyclohexanone: structure and stability [J]. Journal of the American Chemical Society, 2007, 129(31): 9544–9545. doi: 10.1021/ja072074h
[55]	STROBEL T A, KOH C A, SLOAN E D. Water cavities of sH clathrate hydrate stabilized by molecular hydrogen [J]. The Journal of Physical Chemistry B, 2008, 112(7): 1885–1887. doi: 10.1021/jp7110549
[56]	DUARTE A R C, SHARIATI A, ROVETTO L J, et al. Water cavities of sH clathrate hydrate stabilized by molecular hydrogen: phase equilibrium measurements [J]. The Journal of Physical Chemistry B, 2008, 112(7): 1888–1889. doi: 10.1021/jp7110605
[57]	KIM D-Y, LEE H. Spectroscopic identification of the mixed hydrogen and carbon dioxide clathrate hydrate [J]. Journal of the American Chemical Society, 2005, 127(28): 9996–9997. doi: 10.1021/ja0523183
[58]	JIANG X, WU X, ZHENG Z, et al. Ionic and superionic phases in ammonia dihydrate NH₃·2H₂O under high pressure [J]. Physical Review B, 2017, 95(14): 144104. doi: 10.1103/PhysRevB.95.144104
[59]	WILLOW S Y, XANTHEAS S S. Enhancement of hydrogen storage capacity in hydrate lattices [J]. Chemical Physics Letters, 2012, 525/526: 13–18. doi: 10.1016/j.cplett.2011.12.036
[60]	AKKERMANS R L C, SPENLEY N A, ROBERTSON S H. Monte Carlo methods in materials studio [J]. Molecular Simulation, 2013, 39(14/15): 1153–1164. doi: 10.1080/08927022.2013.843775
[61]	KIRCHNER M T, BOESE R, BILLUPS W E, et al. Gas hydrate single-crystal structure analyses [J]. Journal of the American Chemical Society, 2004, 126(30): 9407–9412. doi: 10.1021/ja049247c
[62]	KRESSE G, FURTHM LLER J. Efficient iterative schemes for ab initio total-energy calculations using a plane-wave basis set [J]. Physical Review B, 1996, 54(16): 11169–11186. doi: 10.1103/PhysRevB.54.11169
[63]	KRESSE G, JOUBERT D. From ultrasoft pseudopotentials to the projector augmented-wave method [J]. Physical Review B, 1999, 59(3): 1758–1775. doi: 10.1103/PhysRevB.59.1758
[64]	LEE K, MURRAY É D, KONG L, et al. Higher-accuracy van der Waals density functional [J]. Physical Review B, 2010, 82(8): 081101. doi: 10.1103/PhysRevB.82.081101
[65]	SPOEL D V D, LINDAHL E, HESS B, et al. GROMACS: fast, flexible, and free [J]. Journal of Computational Chemistry, 2005, 26(16): 1701–1718. doi: 10.1002/(ISSN)1096-987X
[66]	MOUSTAFA S G, SCHULTZ A J, KOFKE D A. Effects of finite size and proton disorder on lattice-dynamics estimates of the free energy of clathrate hydrates [J]. Industrial and Engineering Chemistry Research, 2015, 54(16): 4487–4496. doi: 10.1021/ie504008h
[67]	VEGA C, ABASCAL J L F, MCBRIDE C, et al. The fluid–solid equilibrium for a charged hard sphere model revisited [J]. The Journal of Chemical Physics, 2003, 119(2): 964–971. doi: 10.1063/1.1576374
[68]	L SAL M, VACEK V. Direct evaluation of solid–liquid equilibria by molecular dynamics using Gibbs-Duhem integration [J]. Molecular Simulation, 1997, 19(1): 43–61. doi: 10.1080/08927029708024137
[69]	CHALLA S R, SHOLL D S, JOHNSON J K. Adsorption and separation of hydrogen isotopes in carbon nanotubes: multicomponent grand canonical Monte Carlo simulations [J]. The Journal of Chemical Physics, 2002, 116(2): 814–824. doi: 10.1063/1.1423665
[70]	RAPPE A K, CASEWIT C J, COLWELL K S, et al. UFF, a full periodic table force field for molecular mechanics and molecular dynamics simulations [J]. Journal of the American Chemical Society, 1992, 114(25): 10024–10035. doi: 10.1021/ja00051a040
[71]	BARONI S, DE GIRONCOLI S, DAL CORSO A, et al. Phonons and related crystal properties from density-functional perturbation theory [J]. Reviews of Modern Physics, 2001, 73(2): 515–562. doi: 10.1103/RevModPhys.73.515
[72]	TRIBELLO G A, SLATER B, ZWIJNENBURG M A, et al. Isomorphism between ice and silica [J]. Physical Chemistry Chemical Physics, 2010, 12(30): 8597–8606. doi: 10.1039/b916367k
[73]	GIES H, MARKER B. The structure-controlling role of organic templates for the synthesis of porosils in the systems SiO₂/template/H₂O [J]. Zeolites, 1992, 12(1): 42–49. doi: 10.1016/0144-2449(92)90008-D
[74]	WHALLEY E. Energies of the phases of ice at zero temperature and pressure [J]. The Journal of Chemical Physics, 1984, 81(9): 4087–4092. doi: 10.1063/1.448153
[75]	ABASCAL J L F, VEGA C. A general purpose model for the condensed phases of water: TIP4P/2005 [J]. The Journal of Chemical Physics, 2005, 123(23): 234505. doi: 10.1063/1.2121687
[76]	SLOAN JR E D, KOH C. Clathrate hydrates of natural gases [M]. 3rd ed. Boca Raton, FL: CRC press, 2008.
[77]	PAQUETTE L A, TERNANSKY R J, BALOGH D W, et al. Total synthesis of dodecahedrane [J]. Journal of the American Chemical Society, 1983, 105(16): 5446–5450. doi: 10.1021/ja00354a043
[78]	STRUZHKIN V V, MILITZER B, MAO W L, et al. Hydrogen storage in molecular clathrates [J]. Chemical Reviews, 2007, 107(10): 4133–4151. doi: 10.1021/cr050183d
[79]	LIN K, YUAN Q, ZHAO Y-P. Using graphene to simplify the adsorption of methane on shale in MD simulations [J]. Computational Materials Science, 2017, 133: 99–107. doi: 10.1016/j.commatsci.2017.03.010