Numerical Simulation of Penetration in Concrete Sheet Based on SPH Method
-
摘要: 随着混凝土结构强度的不断提高,越来越多的防护工事选择混凝土作为主要建筑材料。在光滑粒子流体动力学方法的基础上,提出了TCK-HJC复合本构模型,对锥形弹刚性侵彻过程中混凝土薄板的变形损伤进行数值模拟,采用拟流体模型处理失效的混凝土碎片,分析了不同侵彻角(0°,60°)下锥形弹侵彻混凝土薄板的变形过程,得到了薄板的压力分布以及失效混凝土碎片的飞散角度,并与实验进行对比。结果表明,数值模拟方法是合理的,为进一步研究脆性材料的力学性能奠定了技术基础。
-
关键词:
- 混凝土 /
- TCK-HJC复合本构模型 /
- 光滑粒子流体动力学方法 /
- 脆性材料
Abstract: As the continuous improvement of the strength of concrete structure, more and more protective fortifications have chosen concrete as the main building material. On the basis of the smooth particle hydrodynamics method, the TCK-HJC composite constitutive model is proposed to simulate the deformation damage of the concrete target during the penetration process of the rigid conical projectile, and the pseudo-fluid model is used to describe the failed concrete fragments. The deformation process of the conical projectile penetrating into the concrete target under different penetration angles (0°, 60°) were analyzed, and the pressure on the surface of the target was also obtained. The scattering angles of the broken concrete fragments were obtained, and the comparison with experiment shows the simulation result is robust and accurate, which lays a technical foundation for further simulation of the mechanical properties of brittle materials. -
长直空间具有横截面尺寸小、长度尺寸远大于截面尺寸、泄爆面积有限等特点,常见于煤矿巷道、隧道洞库、管涵暗渠、城市综合管廊等。在煤矿采挖、隧道施工以及油气管道输运过程中存在燃气泄漏的可能,泄漏的燃气在长直空间内与空气混合后遇点火源极易引发爆炸,进而造成人员伤亡和财产损失。近年来相关事故时有发生,例如:2013年青岛排水暗渠油气爆炸,2015年成都洛带古镇隧道瓦斯爆炸,2016年重庆金山沟煤矿瓦斯爆炸等。燃气爆炸产生的压力波是造成人员及结构损害的主要原因之一[1-2],有学者发现特定条件下长直空间燃气爆炸存在明显的超压振荡现象[3-8],而超压振荡将引起荷载峰值及升压速率的明显升高[9-10]、流场湍流程度增强[11],并可能造成结构高温蠕变[3]以及引发结构共振[12],从而进一步加剧爆炸对人员和结构的危害。考虑到长直空间燃气爆炸事故可能造成的严重后果,开展爆炸超压及其振荡抑制措施研究十分必要。
Xing等[12]利用长度为2 m的圆管进行了甲烷/空气爆炸实验,发现CH4的体积分数为9.5%~12.0%时,密闭管道爆炸出现了剧烈的压力振荡;朱传杰等[13-14]通过实验和数值模拟(AutoReaGas)研究了不同尺寸密闭方管内CH4/空气爆炸超压的振荡特性,认为超压的振荡是压力波在管道两端产生反射的结果;韦世豪等[15-16]进行了小尺寸密闭管道内汽油蒸气/空气爆炸实验,认为前驱波和压缩波在容器内的反射叠加是产生压力振荡的主要原因,而火焰和压力波耦合所形成的正反馈现象进一步加剧了振荡。研究人员在长直尾端泄爆空间内同样观察到了压力振荡现象[6-8];Xing等[12]发现随着泄爆面积的增加,压力振荡相比于密闭管道逐渐衰减,但另外出现了亥姆霍兹振荡;李毅[17]、路长等[18]通过实验发现气体流入流出的交替变化对压力的振荡具有影响;王亚磊等[19]在长0.5 m的尾端管道内开展了燃气爆炸实验,结果表明管内气体的快速泄放和回流造成了压力的振荡。
目前应用较为广泛的抑爆措施主要包括水系抑爆及惰性气体抑爆,二者分别通过在可燃气体中加入一定的水雾/水蒸气和惰性气体对爆炸产生抑制。用于抑爆的惰性气体主要为氮气(N2)和二氧化碳(CO2)[20-21],实验发现随着N2和CO2浓度的增加,惰性气体对甲烷(CH4)爆炸的抑制作用增强[22-23],CO2和N2对甲烷/空气混合气体的抑爆浓度分别为22%~23%和30%~37%[24-26]。李成兵[27]、Xie等[28]认为CO2的比热容更高以及CO2作为燃烧产物能够从化学角度对燃烧产生抑制是CO2抑爆性能优于N2的主要原因。水系抑爆主要包括水雾抑爆和水蒸气抑爆,秦文茜等[29]、康泉胜等[30]分别通过实验研究了超细水雾对瓦斯和丙烷爆炸的抑制效果,发现添加超细水雾能够有效降低爆炸超压及其升压速率,并使爆炸下限大幅提高,随着超细水雾添加量的增加,抑制效果增强。高旭亮[31]利用Fluent软件研究了超细水雾对CH4爆炸的抑制效果,得到了与上述实验相同的结论,此外还发现当施加量达到一定程度时,超细水雾甚至会完全抑制CH4的爆炸。刘丹等[32]通过实验研究了水蒸气对CH4爆炸超压及爆炸极限的影响,发现水蒸气同样能够对爆炸产生抑制,且随着水蒸气浓度的增加,爆炸超压及升压速率降低,爆炸极限范围缩小。
研究人员对长直空间燃气爆炸超压的振荡现象进行了研究,但大多关心超压的振荡特性及其产生原因,鲜有关于超压振荡抑制措施的研究报道。虽然添加N2、CO2和水蒸气能够在一定程度上对燃气爆炸超压及其升压速率产生抑制,但其能否抑制超压的振荡仍有待深入探讨。本研究拟通过FLACS数值模拟软件,建立长直密闭和长直尾端泄爆管道模型,并利用已有实验数据对模型进行验证;基于已验证的数值模型,以化学当量比甲烷/空气混合气体为研究对象,分析不同浓度CO2、N2及水蒸气对长直空间燃气爆炸超压及升压时间的抑制作用,对比3种气体对超压振荡的抑制效果,探究不同气体抑爆效果差异的原因。
1. 数值模拟
1.1 理论模型
甲烷/空气混合气体的爆炸是一个快速释能的化学过程。FLACS描述可压缩流体流动的数学模型包括质量守恒方程、动量守恒方程以及焓的输运方程[33]。
质量守恒方程:
∂∂t(βvρ)+∂∂xj(βjvρuj)=˙mV (1) 动量守恒方程:
∂∂t(βvρui)+∂∂xj(βjρuiuj)=−βv∂p∂xi+∂p∂xi(βjσij)+Fo,i+Fw,i+βv(ρ−ρ0)gi (2) 焓的输运方程:
∂∂t(βvρh)+∂∂xj(βjρujh)=∂∂xj(βjμeffσh∂h∂xj)+βvdpdt+˙QV (3) 式中:t为时间;
βv 为体积孔隙率;ρ 为密度;ρ0为初始密度;m为质量;β 为火焰模型中的转换系数;V为比容;h为比焓;σh为焓对应的Prandtl数,取0.7;μeff 为有效黏度;Q为热量流率;p为压强;ui 、uj 分别为i 方向和j 方向的平均速度;σij 为应力张量分量;gi为i方向的重力加速度;Fw,i 、Fo,i 分别为容器壁和亚网格阻塞引起的流动阻力。FLACS的湍流模型为标准k-
ε 模型[33],属于涡黏模型中的两方程模型,湍动能k的运输方程为∂(ρk)∂t+∂(ρkui)∂xi=∂∂xj[(μ+μtσk)∂k∂xj]+Gk+Gb−ρε−YM+Sk (4) 耗散率
ε 的运输方程为∂(ρε)∂t+∂(ρεui)∂xi=∂∂xj[(μ+μtσε)∂ε∂xj]+C1εεk(Gk+C3εGb)−C2ερε2k+Sε (5) 式中:
μ 为动力黏度;μt 为湍动黏度;Gk 是由平均速度梯度引起的湍动能k 的产生项;Gb 是由浮力引起的湍动能k 的产生项;YM 代表可压湍流中脉动扩张的贡献;σk 为湍动能k 对应的Prandtl数,取为1.0;σε 为耗散率ε 对应的Prandtl数,取为1.3;Sk 、Sε 为用户定义的源项;C1ε 、C2ε 、C3ε 为经验常数,FLACS根据Launder等[34]的研究结果,分别取1.44、1.92、0.80。FLACS燃烧模型由火焰模型和燃烧速度模型组成[33]。火焰模型采用
β 模型,通过定义扩散系数D和反应速率RF 确定燃烧标准和反应速率在数值火焰区域的空间分布。燃烧速度取准层流燃烧速度和湍流燃烧速度中的较大值。1.2 模型建立
建模过程中对FLACS管道数值模型做以下简化:(1)模型管道壁面为刚性,不考虑其变形和损坏;(2)假设爆炸过程为绝热爆炸,不考虑由管道壁面散热引起的能量损失;(3)忽略模型管道壁上的各种可关闭预留孔口、传感器以及管道连接部位等细节,忽略管道壁对压力波的阻碍作用,认为容器内壁光滑。
考虑到长直密闭和尾端泄爆管道内燃气爆炸均可能存在超压的振荡现象,建立如图1所示的完全密闭和尾端泄爆两种管道模型,两种管道内部尺寸均为0.8 m × 0.8 m × 30 m,尾端泄爆管道泄爆口尺寸为0.8 m × 0.8 m,泄爆压力为0 kPa。点火点设置在管道端部中央,分别在每节管道侧壁距点火点1、4、7、···、28 m每隔3 m处布置压力测点,依次编为测点1~测点10。流速尺度(CFLV)和声速尺度(CFLC)分别取为0.5和5.0,初始温度为20 ℃,初始压强100 kPa,相对湍流强度和湍流长度尺度均为零,其余设置均采用默认值。
密闭模型各边界均设置为EULER边界,尾端泄爆管道气体泄放到开敞空间,故将除z轴负方向(地面)外的其他方向均设置为PLAN_WAVE边界。计算域及网格尺寸设置如下:将管道内部设置为核心区,核心区分别采用0.10、0.05、0.03 m 3种尺寸的网格。密闭管道核心区以外0.2 m范围为拉伸区,尾端泄爆管道核心区外x轴正负方向3 m,y轴正方向15 m,y轴负方向0.5 m,z轴正方向10 m,z轴负方向0.2 m为拉伸区,拉伸系数为1.2。
1.3 模型验证
研究人员对FLACS模拟惰性气体抑爆的可靠性进行了验证,陈晓坤等[35]利用FLACS软件对多组分可燃气体添加CO2后的爆炸参数进行了模拟研究,并将模拟结果与已有实验数据进行对比,发现不同配比条件下的最大爆炸超压模拟值与实验值的相对误差均小于16%,说明利用 FLACS 模拟多组分可燃性气体爆炸可行。罗振敏等[36]在内径88 mm、长1600 mm的尾端泄爆管道内进行了CO2抑制甲烷/空气爆炸实验,并通过FLACS软件对实验进行了模拟,结果表明,除CO2添加量为18%时误差较大外,模拟得到的火焰传播时间和火焰传播速度均与实验值基本吻合且变化趋势一致,认为误差主要来源于模型的理想环境假设以及FLACS对小尺寸管道模拟的偏差。张印等[37]利用FLACS软件对20 L密闭容器内添加CO2的CH4/空气预混爆炸进行了数值模拟,发现最大爆炸压力的模拟值与理论计算值的相对误差在6.5%~10.5%之间,爆炸温度的模拟值与计算值的相对误差在3%~13%之间。
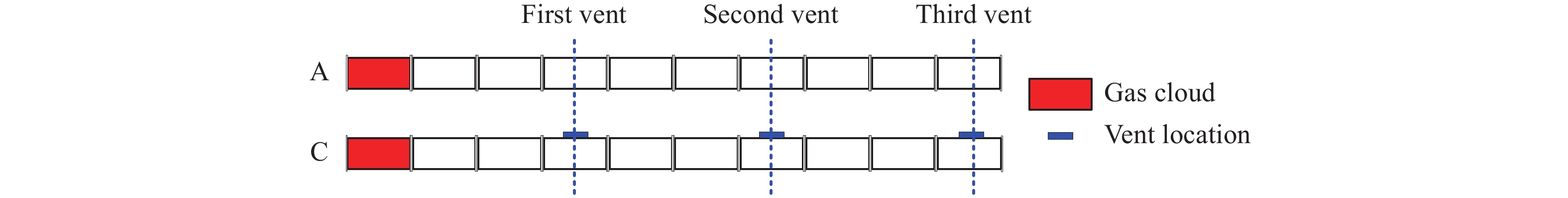
为验证FLACS模拟大尺寸长直管道燃气爆炸的准确性,对Li等[38]开展的长直空间燃气爆炸实验进行了模拟,模拟工况见图2。该实验管道尺寸为0.8 m × 0.8 m × 30 m,由10节长度为3 m的钢制管道组成。工况A和工况B均在管道端部充入3 m长的9.5%甲烷/空气混合气体,点火位置位于管道端部中央,点火能量100 mJ。工况A中管道完全密闭,工况C中第4、7、10节管道顶部泄爆口(尺寸0.6 m × 0.6 m)打开,泄爆压力0 kPa。需要指出的是,工况A的数值模型与完全密闭管道模型(图1(a))相同;工况C的数值模型除泄爆口数量与分布尾端泄爆管道模型(图1(b))不同外,其他设置均与之相同。
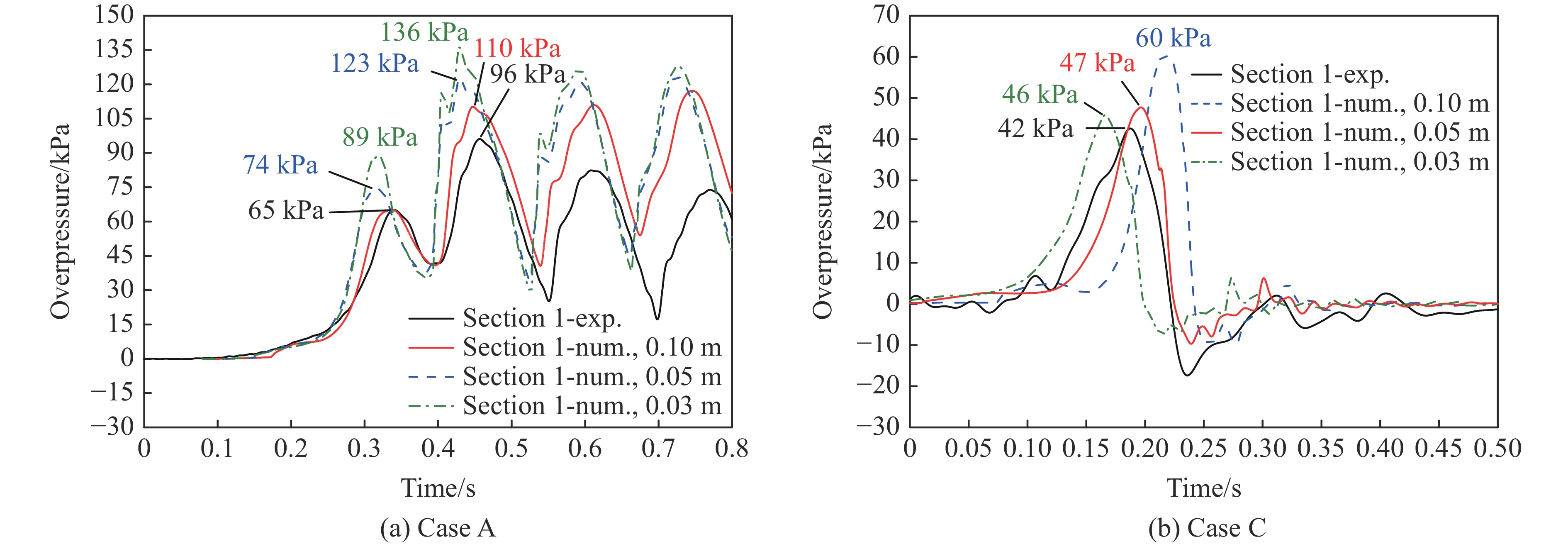
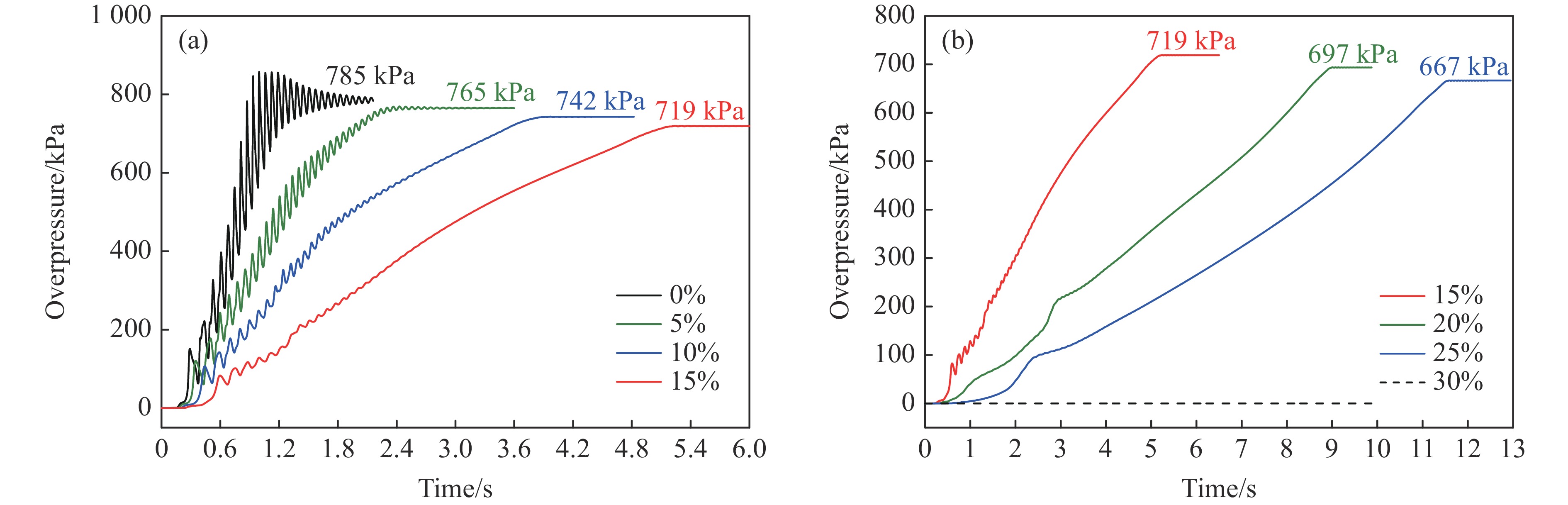
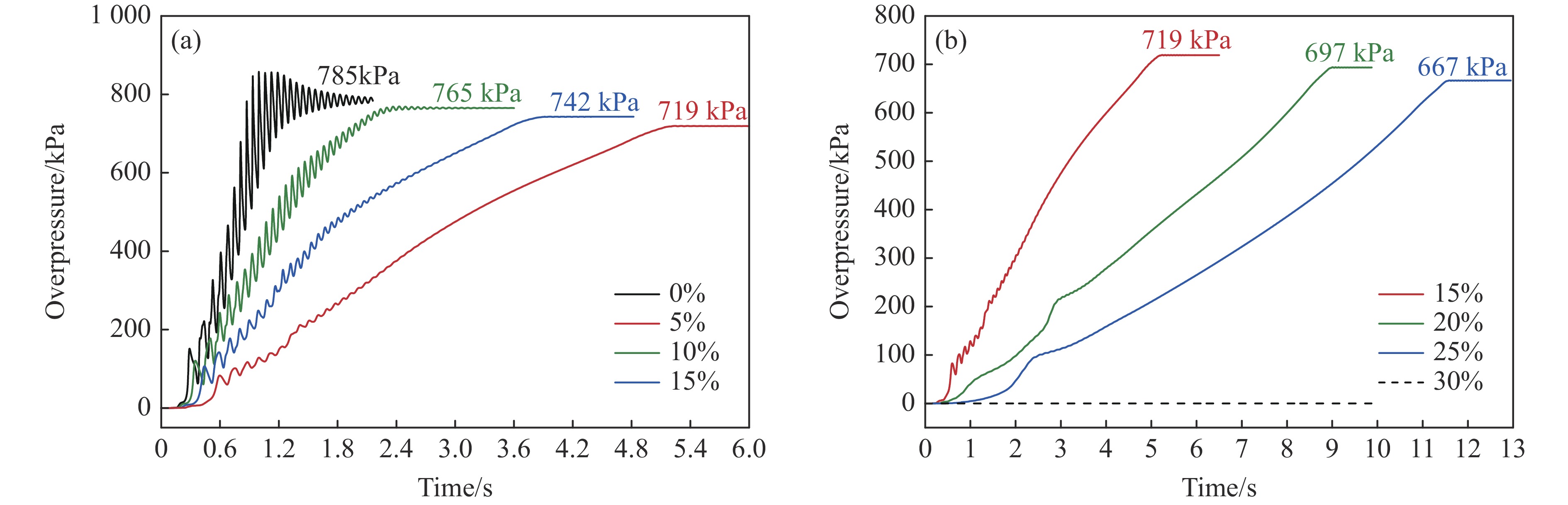
工况A和工况C的模拟结果与实验值的对比如图3所示,以靠近点火端的测点1为例,可以看到密闭和泄爆条件下FLACS均能够正确地模拟爆炸超压随时间的变化规律。对于密闭管道(工况A),网格尺寸为0.10 m时模拟值与实验值最接近,第1个超压峰值(65 kPa)与实验值完全相同,第2个超压峰值(110 kPa)的相对误差为14.6%。对于泄爆管道(工况C),网格尺寸为0.05和0.03 m时误差较小且接近,超压峰值的误差分别为9.5%、11.9%。造成模拟值与实验值差异的主要原因:实验管道壁为散热良好的钢制粗糙壁面,而数值模型做了光滑、绝热的假设。模拟结果表明,数值模型基本可靠,模拟误差在可接受范围内。综合考虑结果的准确性和计算效率,后续密闭管道和泄爆管道分别采用0.10和0.05 m尺寸的网格进行计算。
1.4 模拟工况
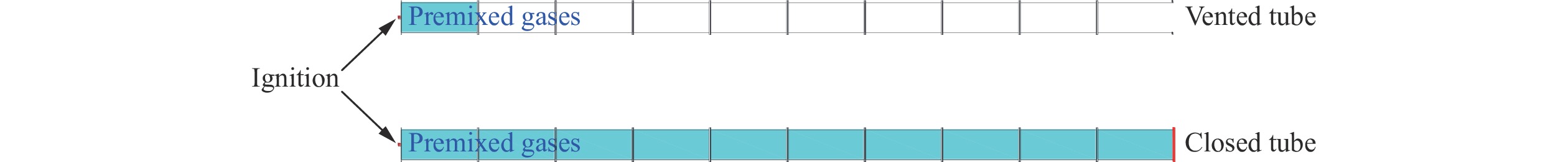
为研究添加惰性气体及水蒸气对长直空间内燃气爆炸超压及其振荡的抑制效果,利用FLACS软件对添加了不同体积分数N2、CO2和水蒸气的化学当量比CH4/空气混合气体进行数值模拟,添加气体的体积分数如表1所示。充气体积如图4所示,在密闭管道内充满混合气体,在尾端泄爆管道点火端充入3 m长的混合气体。由于密闭管道尾端[14]和泄爆管道点火端[19]的爆炸超压及其振荡相较管道其他位置更明显,如未特别说明,本研究所讨论的密闭管道内的超压时程曲线均取自管道尾端测点(测点10),所讨论的尾端泄爆管道内的超压时程曲线均取自管道点火端测点(测点1)。
表 1 添加气体的体积分数(密闭/泄爆)Table 1. Volume fraction of added gas (closed/end-vented)% CO2 N2 Water vapor (H2O) CO2 N2 Water vapor (H2O) 0 0 0 20 25 20 4 5 4 24 30 24 8 10 8 25 31 26 12 15 12 27 16 20 16 2. 模拟结果
2.1 密闭管道
2.1.1 CO2的抑爆效果
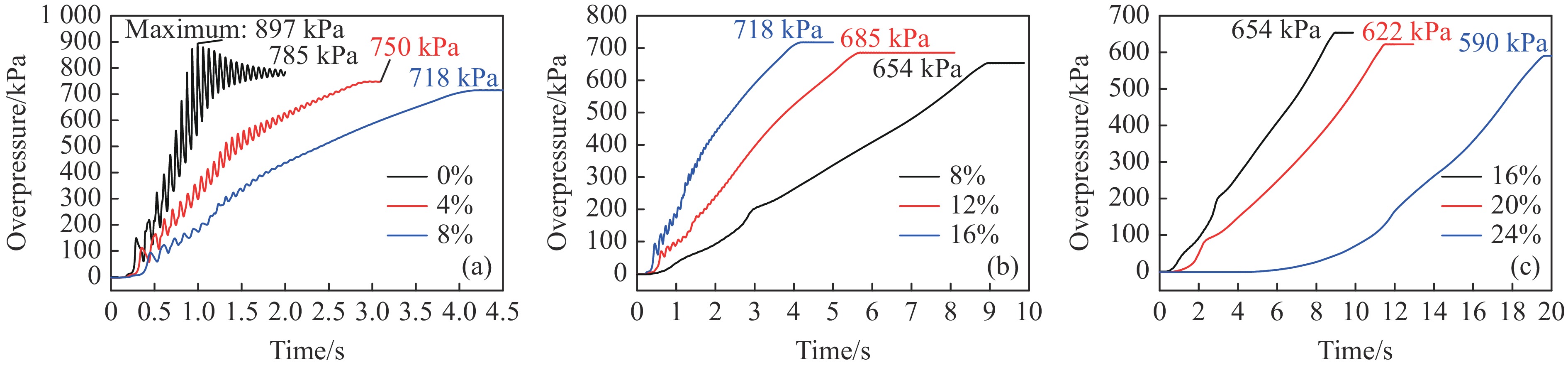
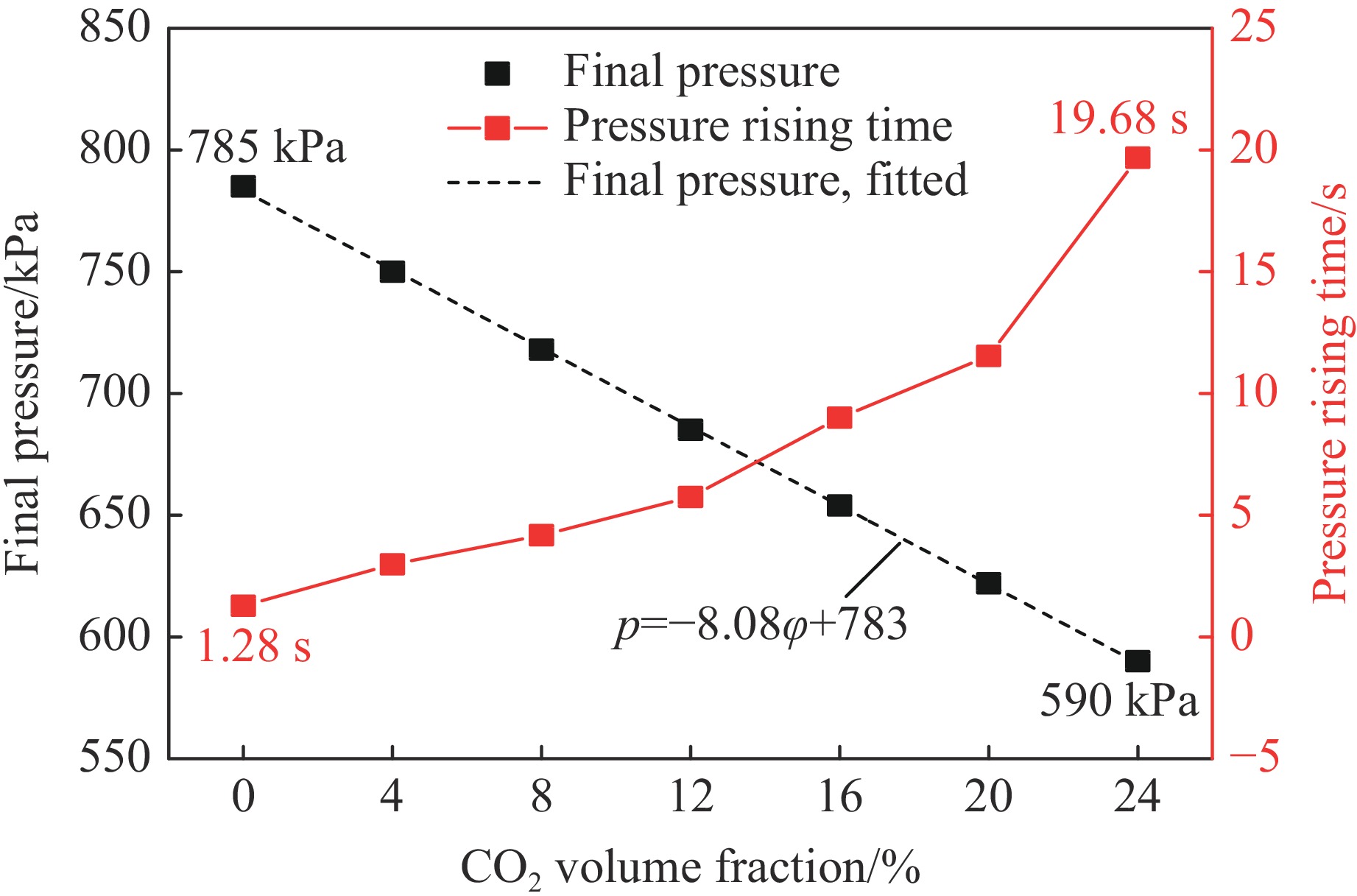
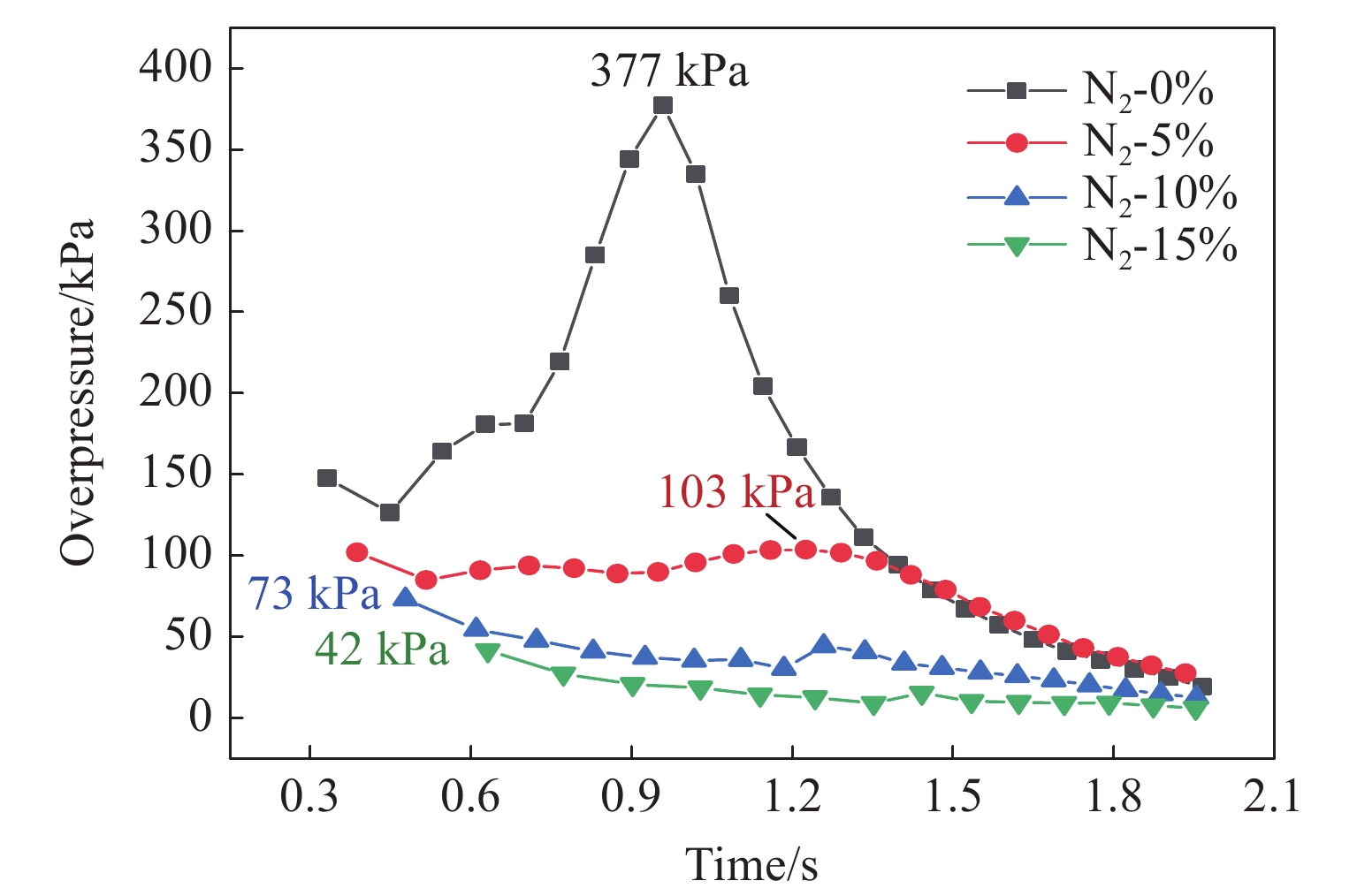
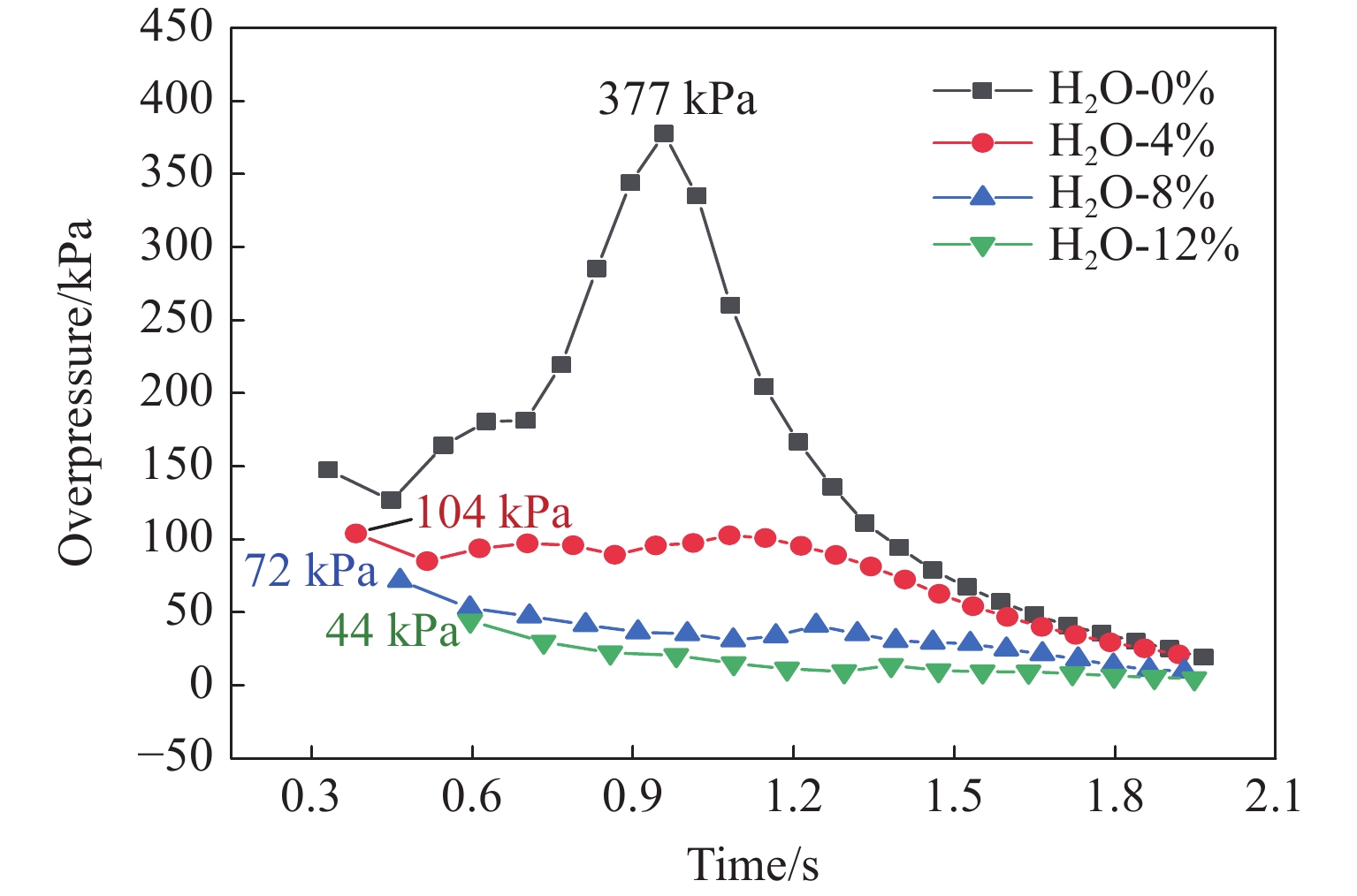
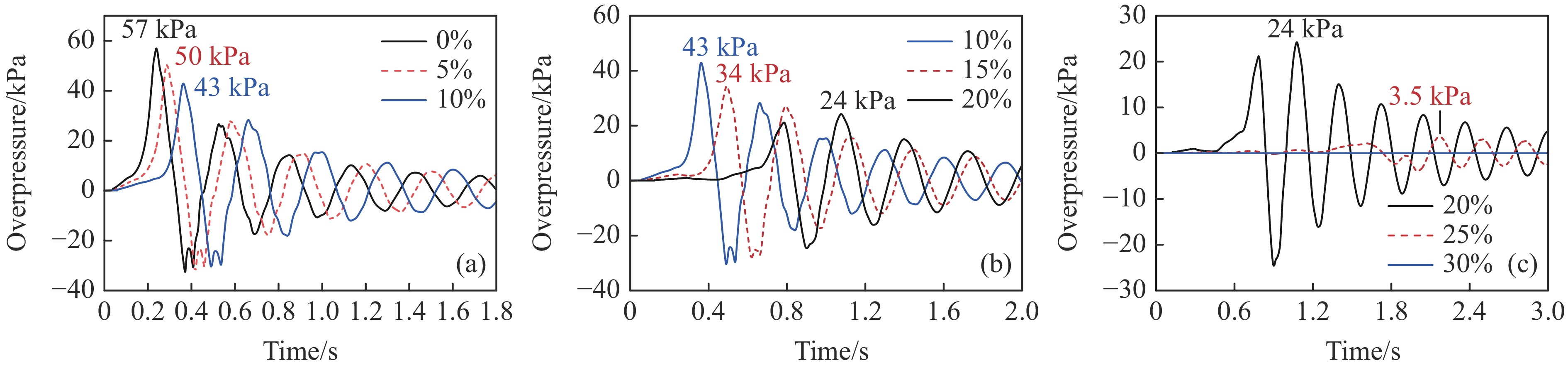
通过模拟发现,密闭管道内CO2的体积分数超过24%时不能点火,爆炸被完全抑制,与前人实验结果[24-26]非常接近。图5为添加不同体积分数CO2预混气体的爆炸超压时程曲线。可以发现,随着CO2体积分数的增加,超压的振荡现象逐渐减弱,振荡持续时间逐渐变短,当CO2添加量达到16%时,振荡完全消失。CO2对超压振荡的抑制可归因于其对混合气体燃烧速率的降低作用[27, 39]:首先,添加CO2稀释了甲烷及氧气,使反应速率降低,燃烧产生的热量减少;其次,CO2将吸收燃烧产生的热量使反应区温度降低,抑制链式反应的进行;最后,CO2作为燃烧产物之一将与燃烧区自由基反应,使自由基浓度降低,爆炸链传递速度减缓。密闭管道内超压的振荡主要是压力波来回反射传播的结果[13-16],燃烧速率的减缓将造成压力波强度(大小及升压速率)下降,使管内压力能够及时分布均匀,所以最终未表现出压力振荡现象。
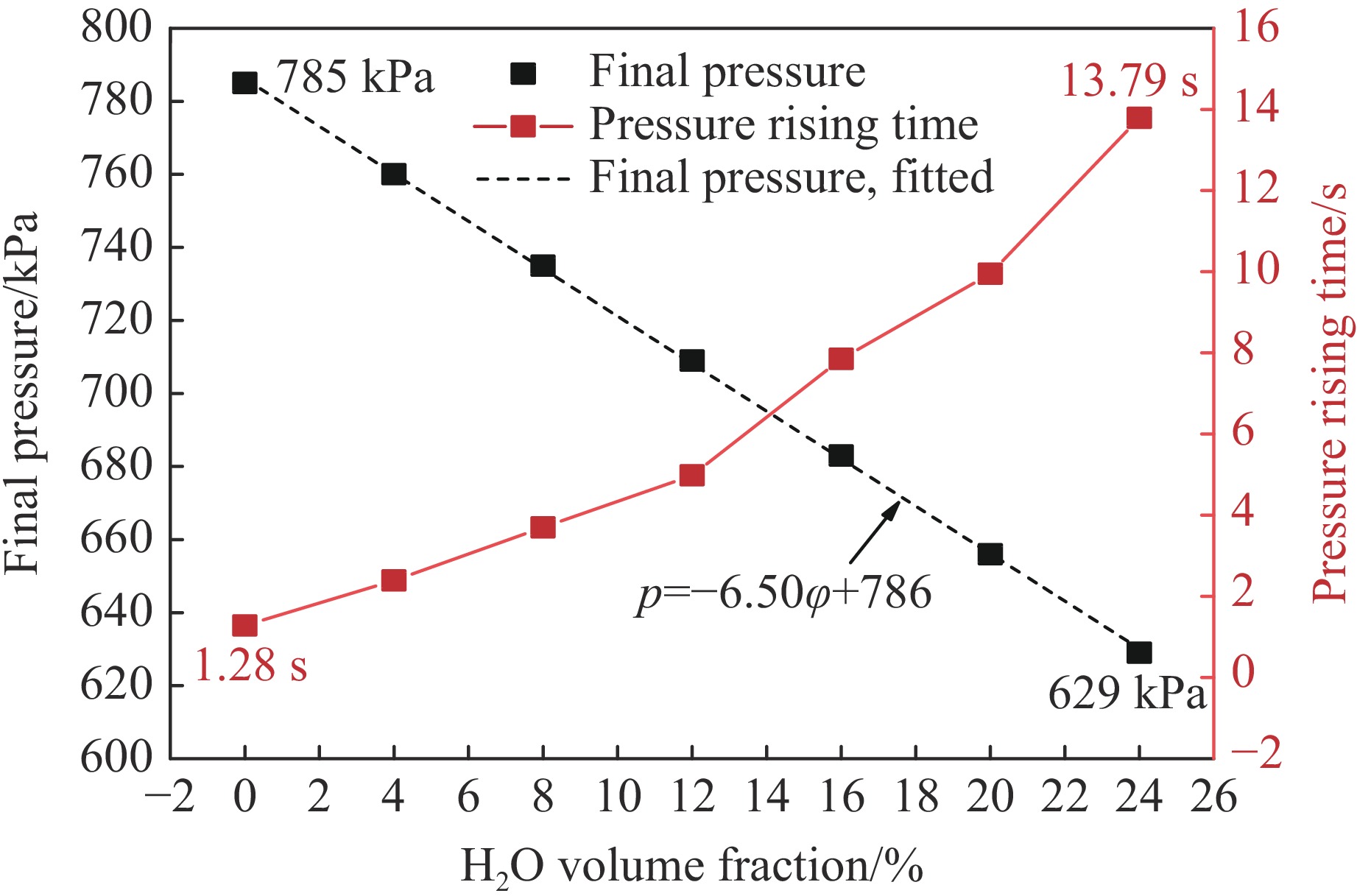
如图6所示,当CO2体积分数(
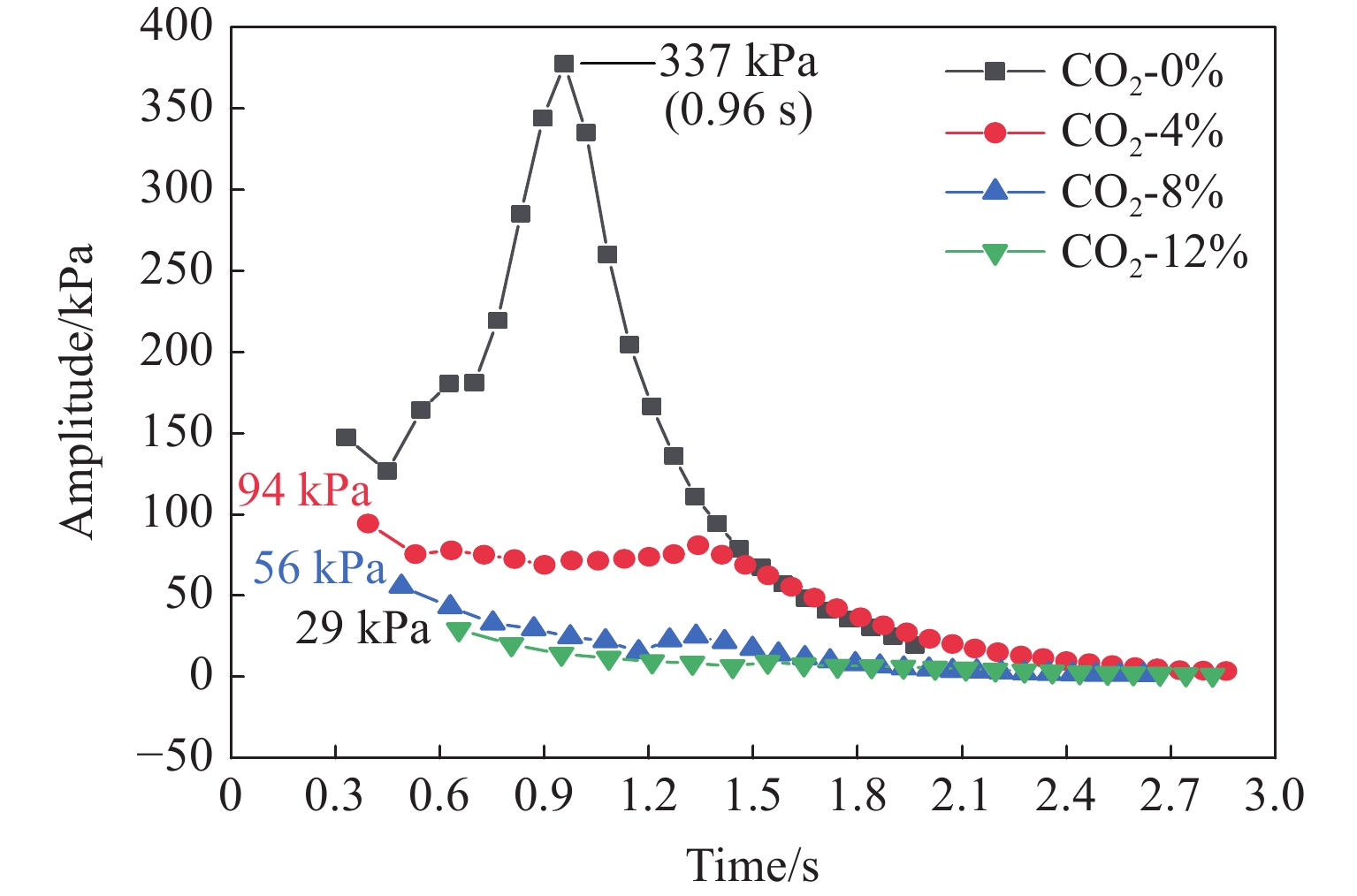
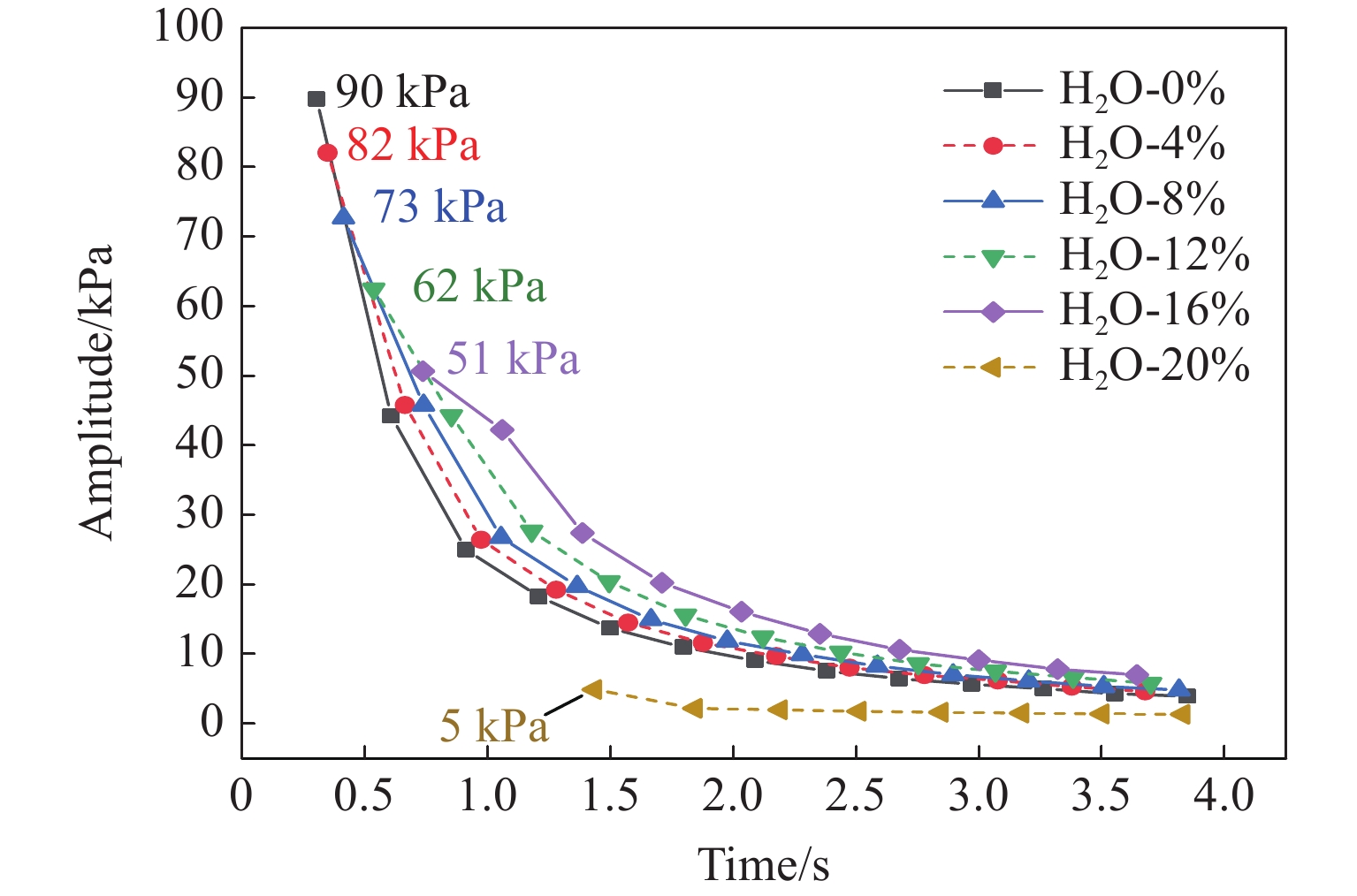
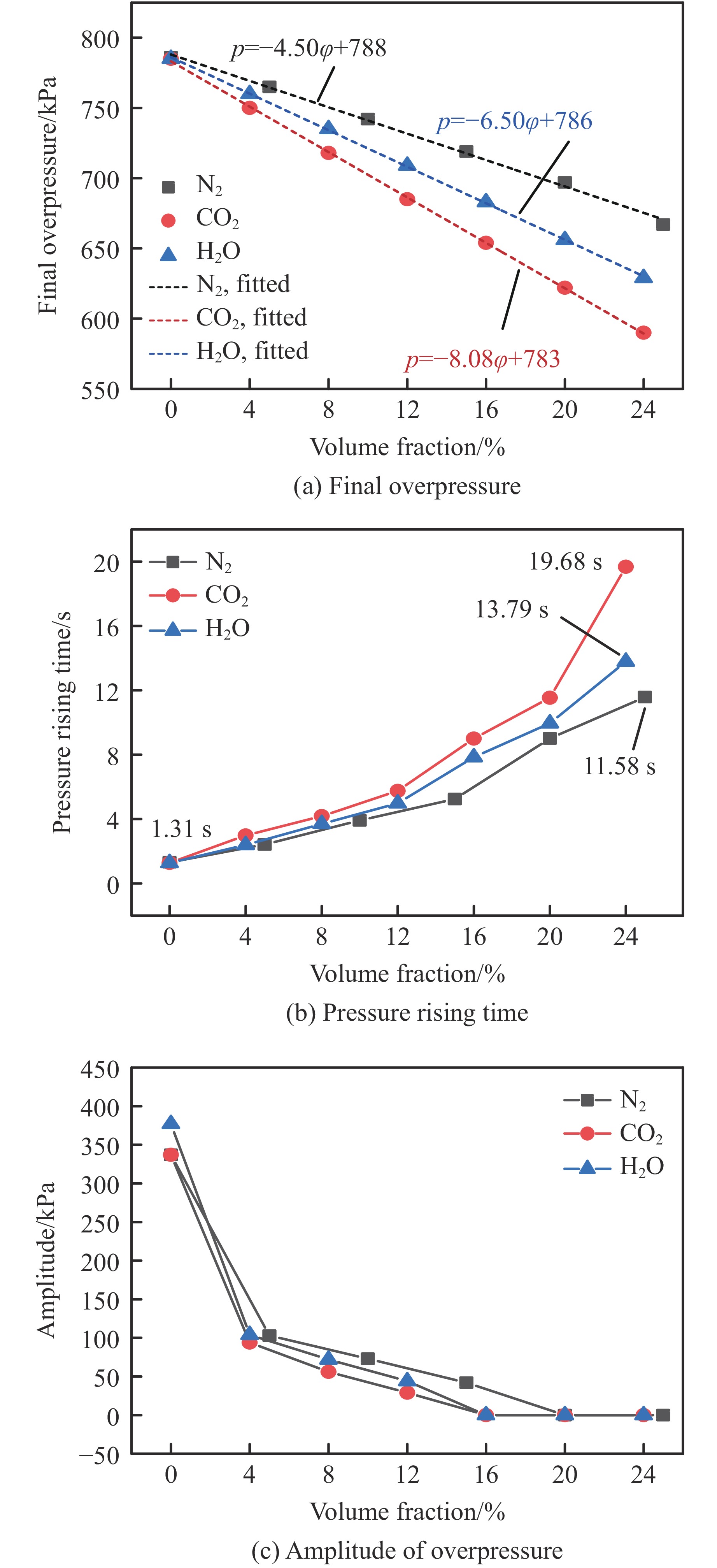
φ )从零逐渐上升到24%时,升压时间(达到压力峰值的时间)从1.28 s逐渐增加到19.60 s,最终爆炸超压由785 kPa呈线性降低到590 kPa,即CO2的体积分数每增加10%,升压时间平均增加7.63 s,爆炸超压下降81 kPa。升压时间的增加是燃烧速率降低的结果,爆炸超压的降低则与添加CO2后管内燃料质量的降低有关。需要指出的是,未添加CO2时,超压峰值(897 kPa)受压力振荡的影响高于最终爆炸超压,但当CO2的体积分数达到4%时,受CO2抑爆作用的影响,超压的振荡减弱并不再使超压峰值升高,此后管内最终压力即为爆炸最大超压。超压振幅随CO2体积分数的变化见图7。可以看到:未添加CO2时压力振幅存在一个明显的上升阶段(0~0.96 s),该阶段点火后气体燃烧产生的压力波在管道内的往复反射形成了超压的振荡;燃烧与压力波的耦合作用使压力振幅不断升高[15-16],并在0.96 s达到最大值(337 kPa);0.96 s之后压力振幅进入下降阶段,此时燃料被大量消耗,预混气体浓度减小,燃烧速率降低,燃烧对压力波的增强逐渐小于压力波的摩擦损耗,振幅呈指数函数下降。需要指出的是,数值模型假设管道壁绝热,所以不考虑散热造成的压力振幅降低。随着CO2添加量的增加,超压振幅逐渐减小,振幅随时间的变化由升高和降低两个阶段逐渐变为仅有降低阶段,这是因为燃烧产生的压力波强度随着CO2体积分数的升高而降低,当CO2的体积分数达到一定值(约8%),燃烧对压力波的增强作用将小于气体摩擦损耗对压力波的衰减作用,振幅上升阶段消失。
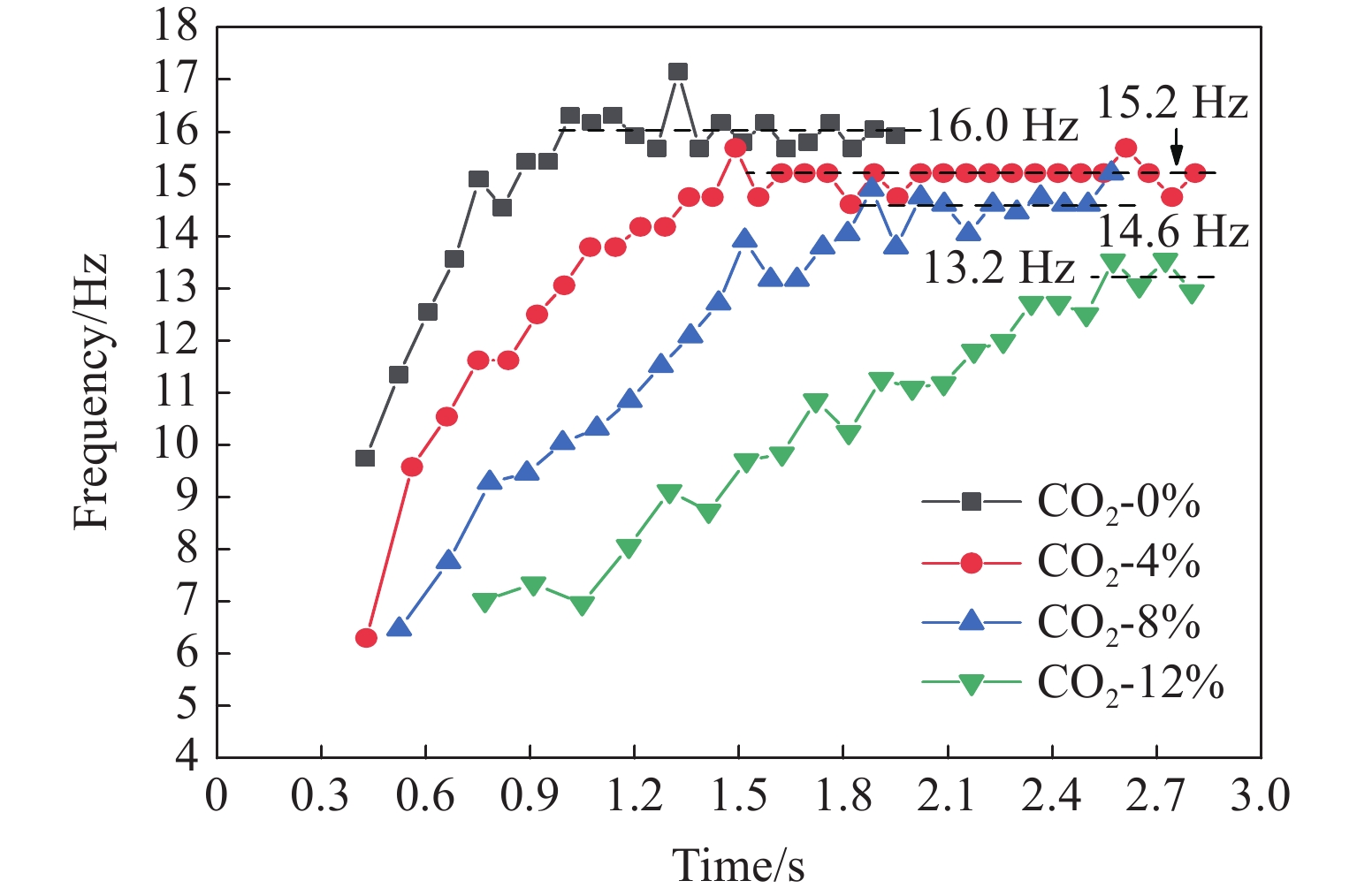
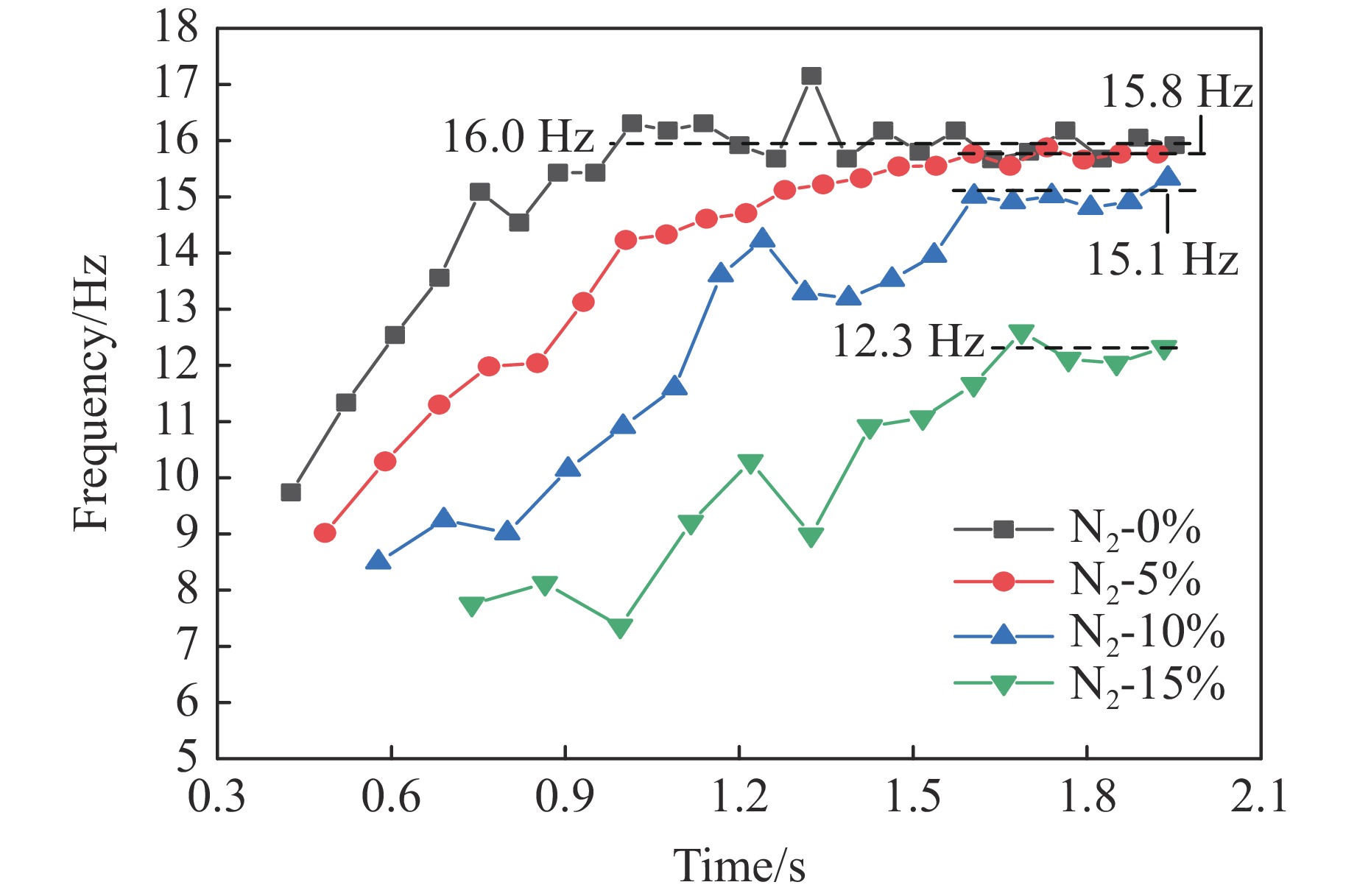
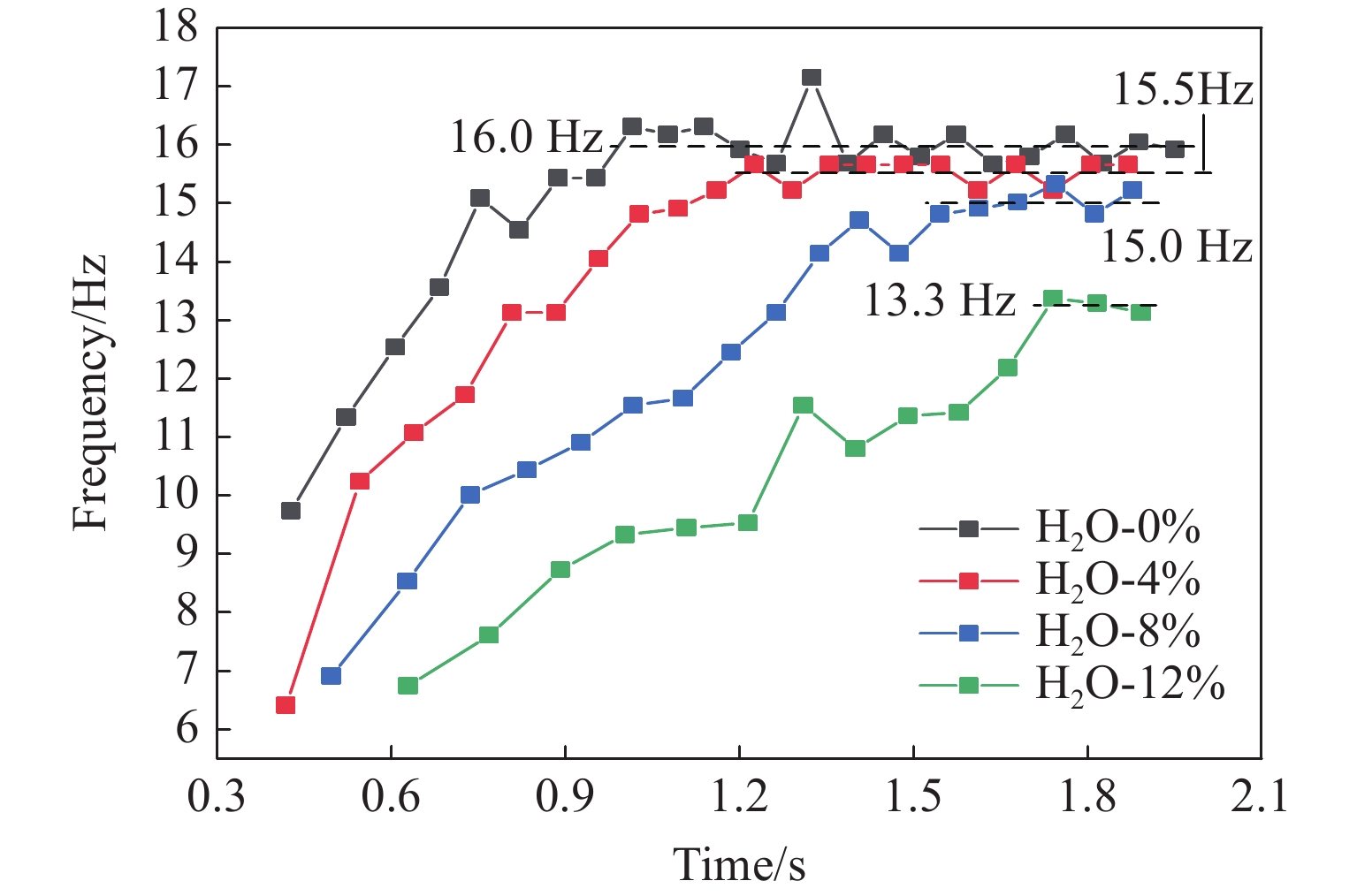
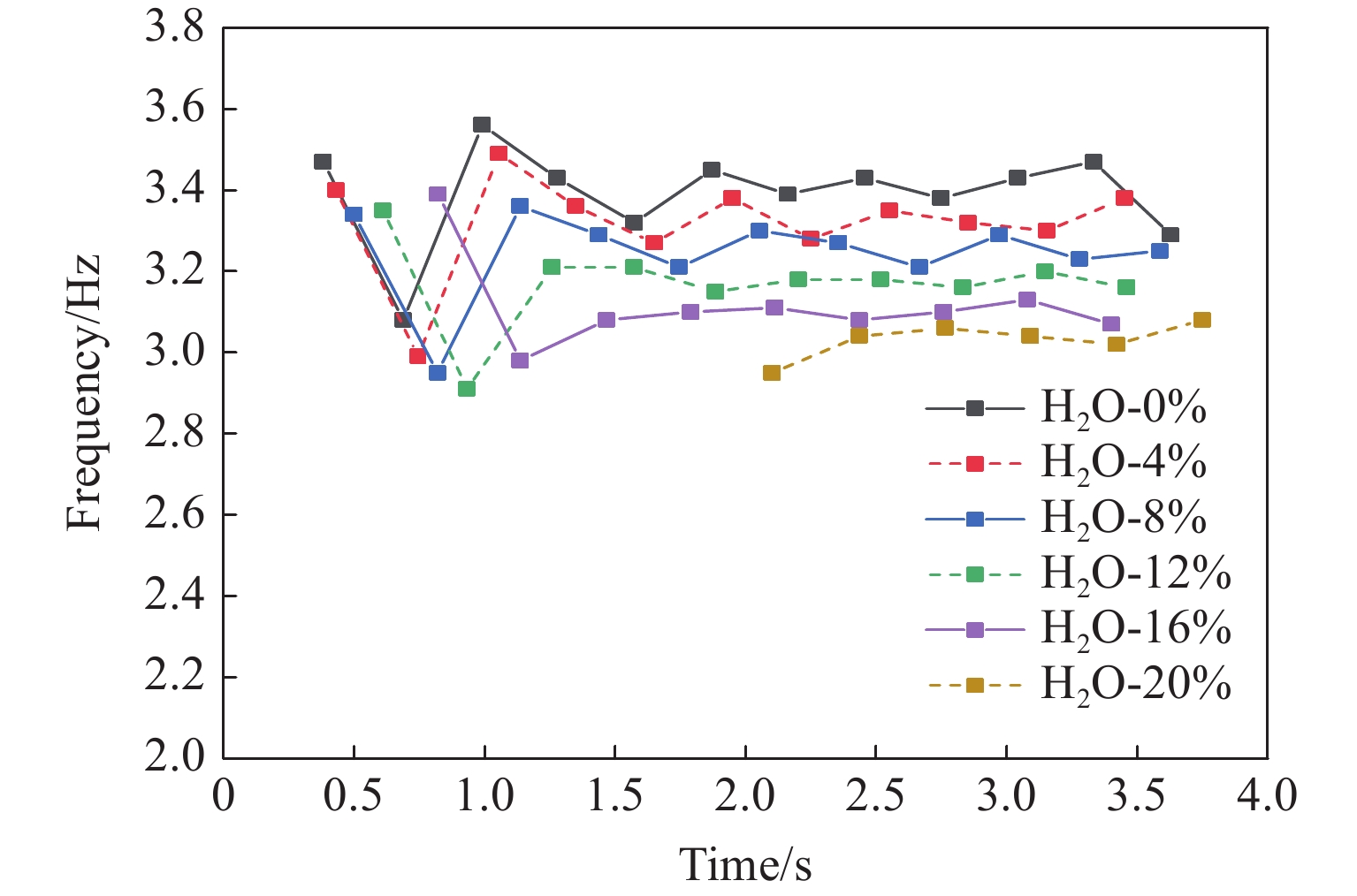
图8为不同CO2体积分数条件下超压振荡频率随时间的变化。可以看到,振荡频率随时间逐渐升高,并最终趋于一定值。此外,可以发现当CO2添加量增加时,超压振荡频率的上升速率和最大超压振荡频率降低。超压振荡归因于压力波的往复反射传播,在管道长度不变的情况下,超压振荡频率的大小主要取决于压力波的传播速度。在管道模型的绝热假设条件下,随着燃烧的进行,管内温度逐渐上升,并在燃烧结束后维持在一定值,而压力波传播速度与气体温度的1/2次方正相关[40],故超压振荡频率表现为先上升后维持的变化规律。另一方面,随着CO2体积分数的增加,甲烷的质量减小,燃烧产生的热量减少,造成管内温度降低,故最大超压振荡频率随CO2体积分数的增加而降低。
2.1.2 N2的抑爆效果
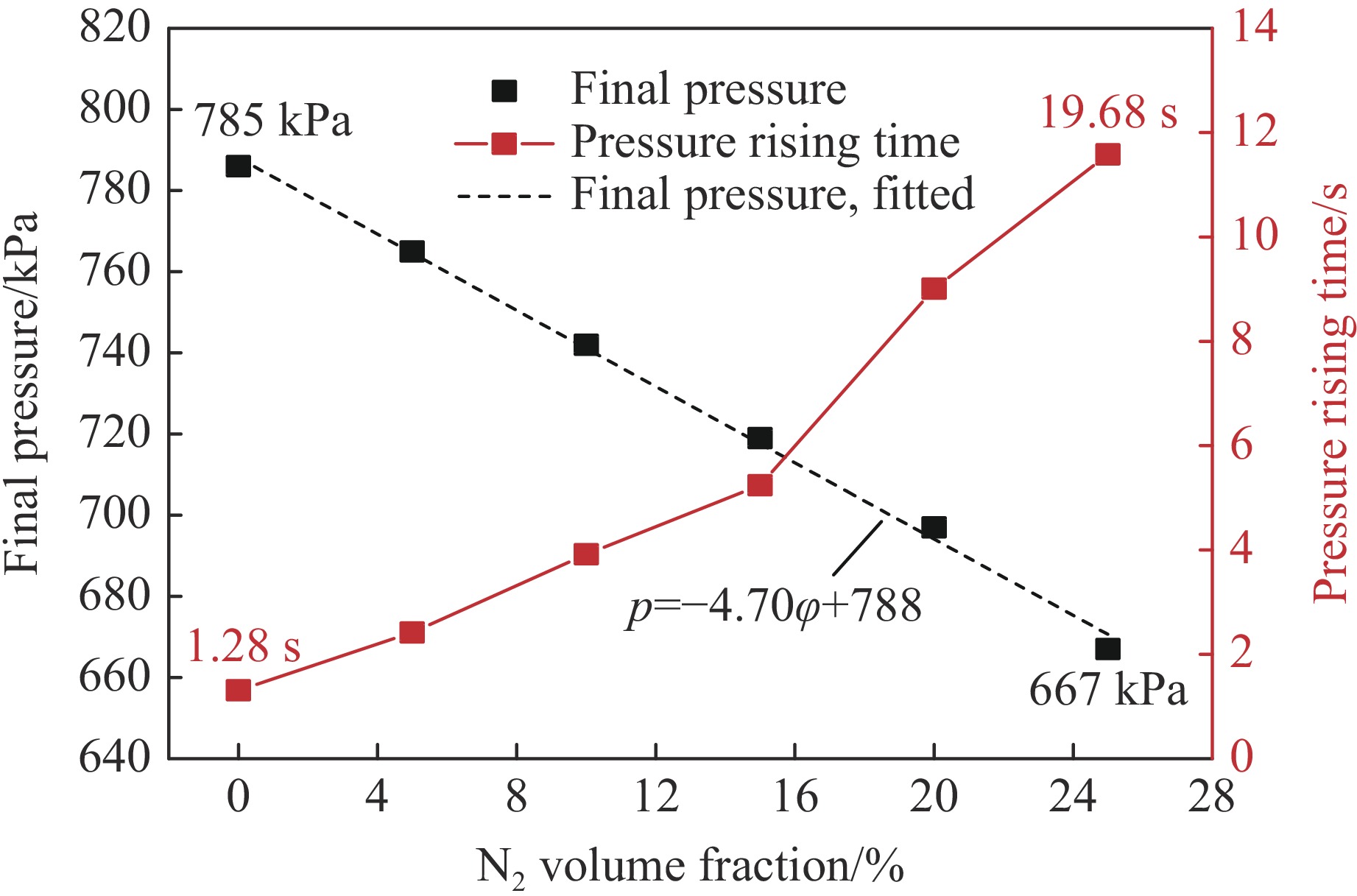
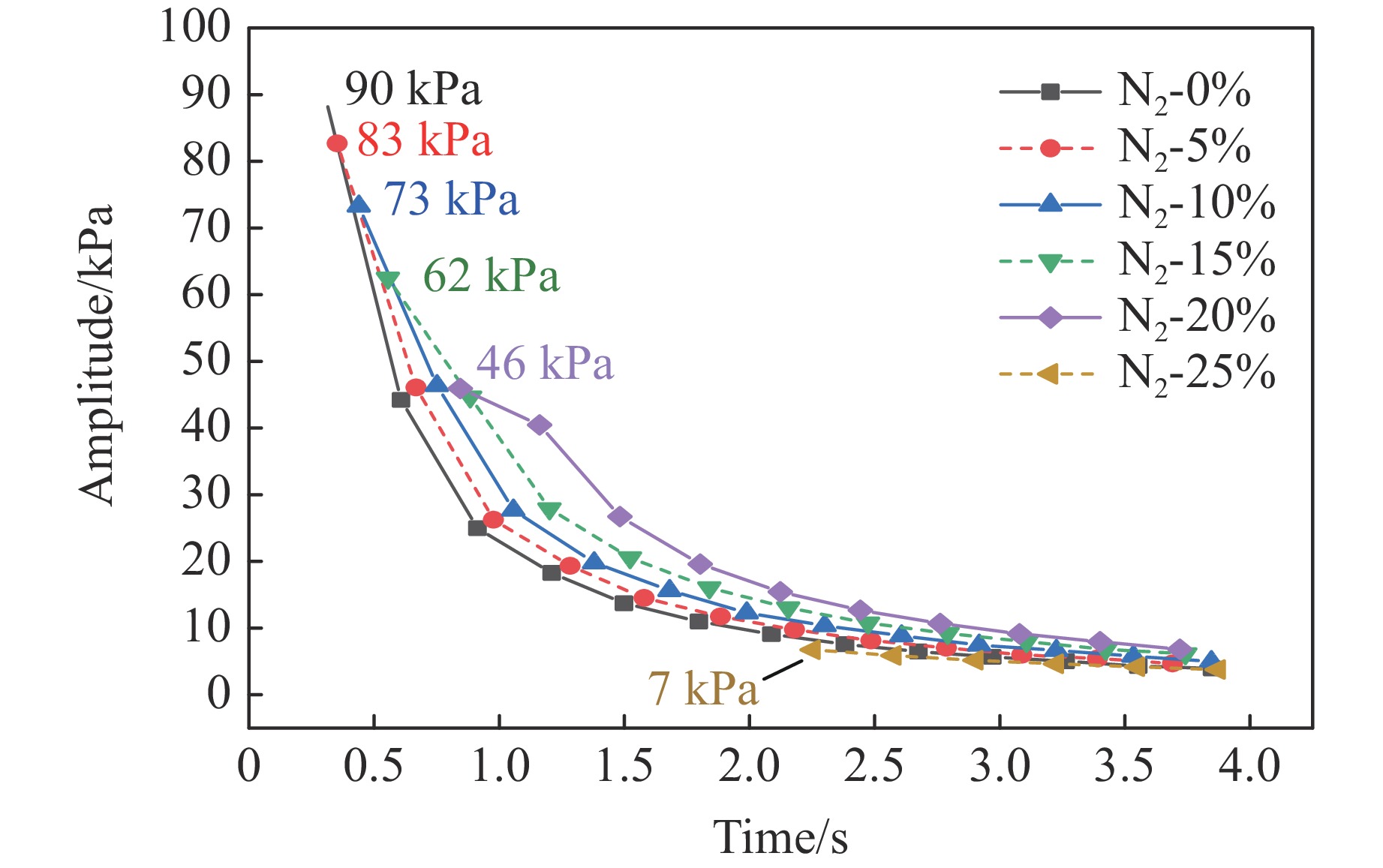
图9为添加不同体积分数N2预混气体的爆炸超压时程曲线。模拟发现:N2的体积分数等于30%时,管内压力在10 s内均无明显变化;当N2的体积分数超过30%时,无法点火,可以认为模拟得到的N2完全抑爆浓度为30%,与已有实验结果[24-26]基本一致。图10显示了最终爆炸超压及升压时间随N2体积分数的变化。当N2的体积分数由零增加到25%时,升压时间从1.28 s逐渐增长到11.58 s,最终爆炸超压由785 kPa线性降低到667 kPa,即N2的体积分数每增加10%,升压时间平均增加4.12 s,爆炸超压下降47 kPa。随着N2体积分数的升高,超压的振荡逐渐减弱,振荡持续时间逐渐变短,N2的体积分数为20%时,振荡被完全抑制。
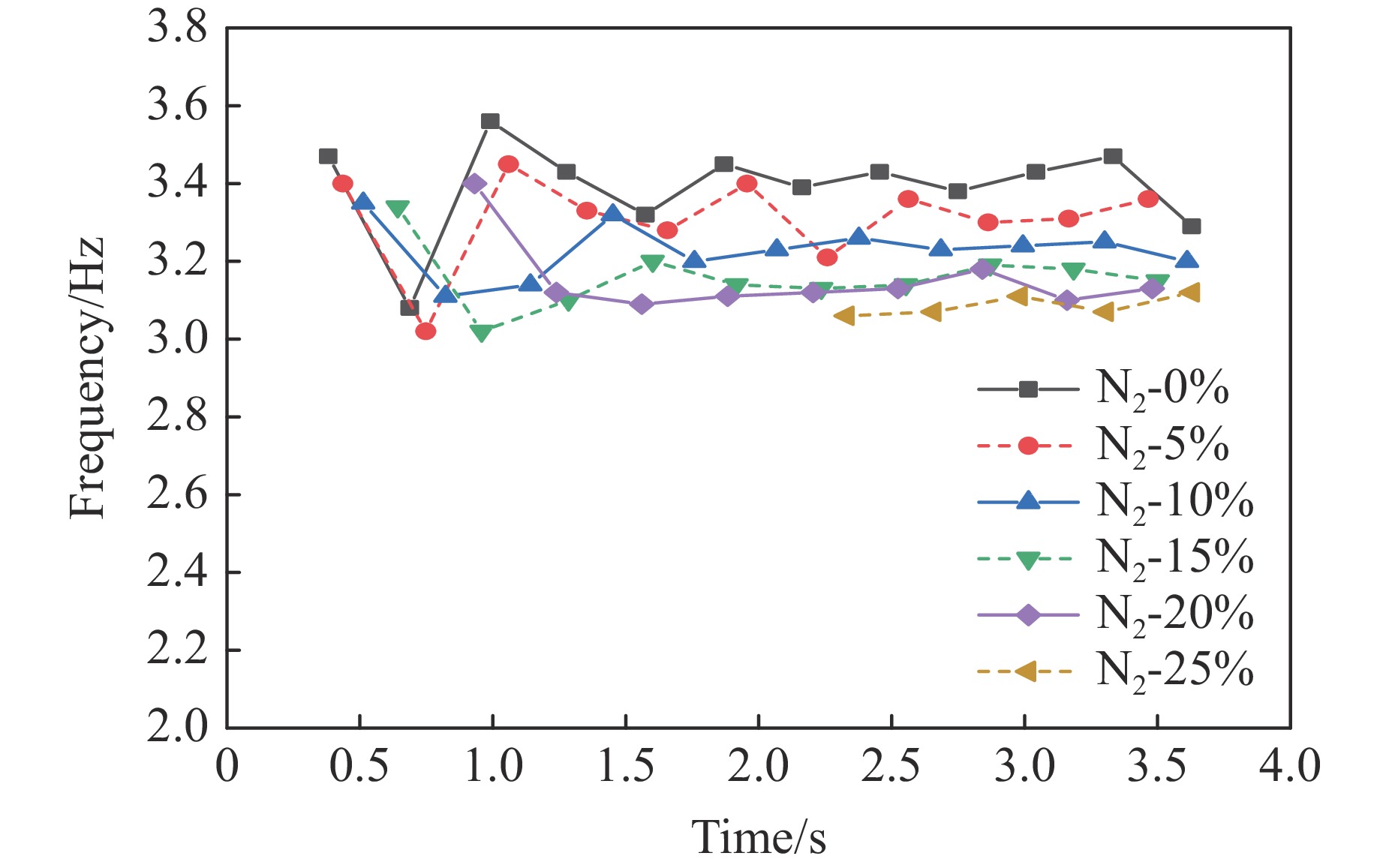
图11为超压振幅随N2体积分数的变化。随着N2添加量的增加,超压振幅逐渐降低,N2的体积分数达到10%时,振幅上升阶段消失,高于CO2(8%)。图12为超压振荡频率随N2体积分数的变化。随着N2添加量的增加,管内温度降低,压力波传播速度降低,超压振荡频率减小。N2主要通过稀释甲烷和氧气浓度抑制爆炸,其次是吸收燃烧产生的热量,相比CO2缺少了化学反应抑制一项,故N2对爆炸超压及其振荡的抑制作用弱于CO2[27, 39]。
2.1.3 水蒸气的抑爆效果
图13为不同水蒸气体积分数下甲烷/空气混合气体的爆炸超压时程曲线。可见,当水蒸气的体积分数达到16%时,超压振荡被完全抑制。此外,当水蒸气的体积分数达到26%时,管内压力在16 s内几乎未上升;当水蒸气的体积分数超过26%时,混合气体无法点火,由此可知模拟得到的水蒸气完全抑爆浓度为26%。
如图14所示,当水蒸气的体积分数由零增加到24%时,升压时间从1.28 s逐渐增长到13.79 s,最终爆炸超压由785 kPa线性降低到629 kPa,即水蒸气的体积分数每增加10%,升压时间平均增加5.21 s,爆炸超压下降65 kPa。
图15和图16分别显示了超压振幅和超压振荡频率随水蒸气体积分数的变化。与添加CO2和N2类似,当水蒸气的体积分数约为8%时,超压振幅的上升阶段消失,随着水蒸气体积分数的继续增大,最大超压振幅减小,超压振荡频率降低。
2.2 尾端泄爆管道
2.2.1 CO2的抑爆效果
图17为不同CO2体积分数条件下混合气体爆炸超压时程曲线。可以发现,当尾端泄爆管道内CO2的体积分数达到24%时,混合气体仅发生缓慢燃烧,管内压力不发生明显变化;当CO2的体积分数为25%时,气体将不能点火,可以认为,尾端泄爆管道CO2的完全抑爆浓度为24%,与密闭管道相同。
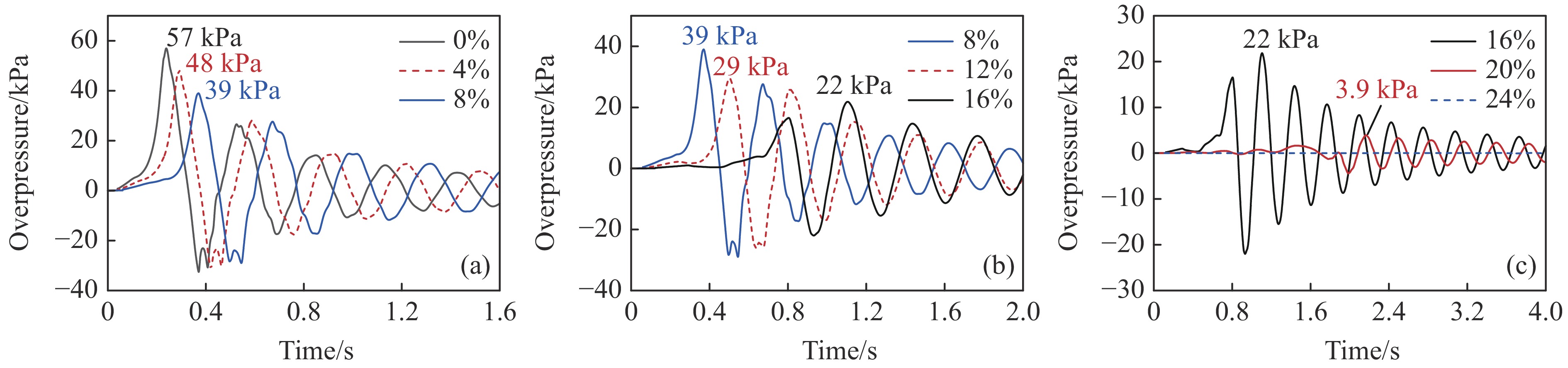
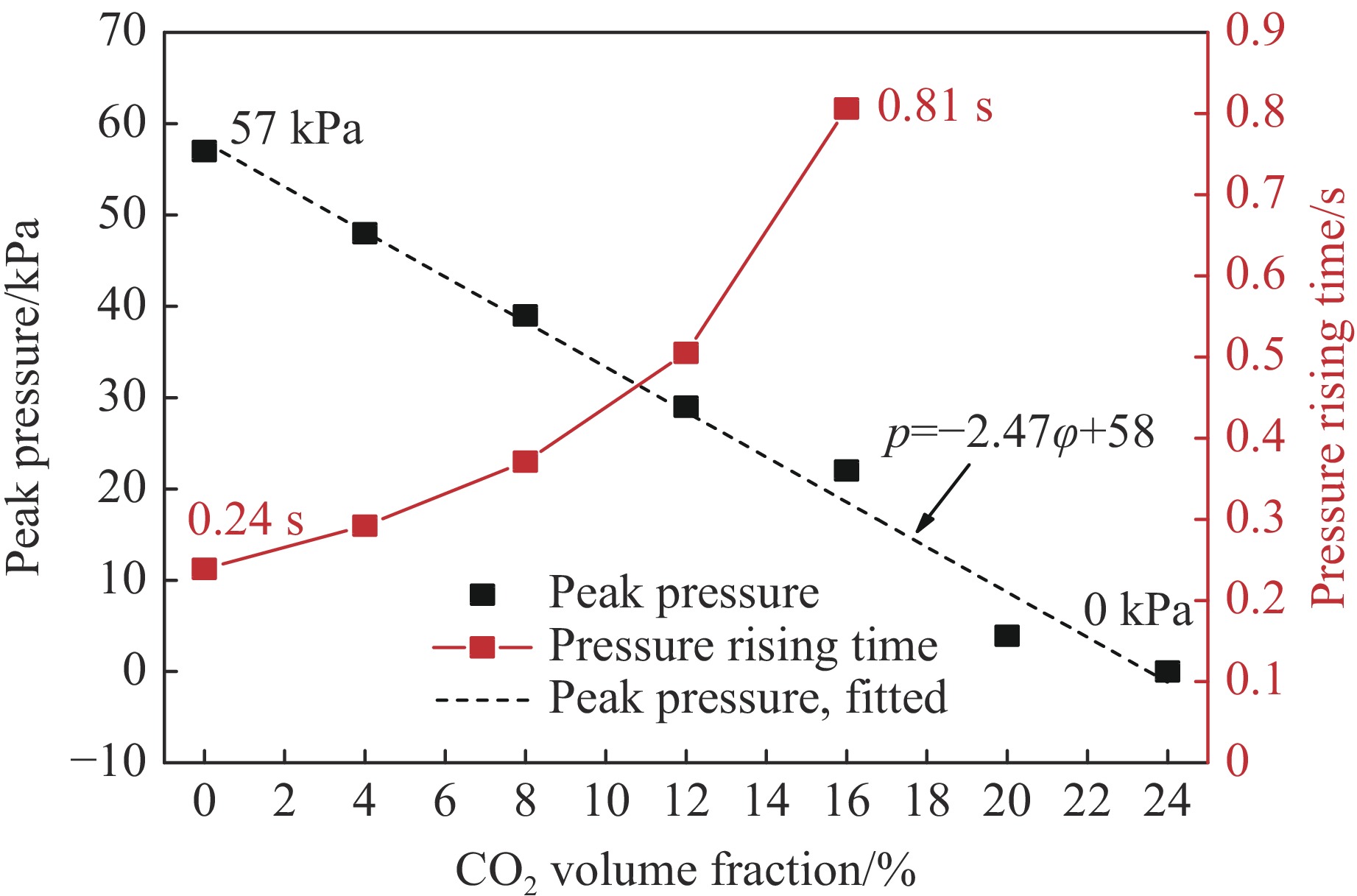
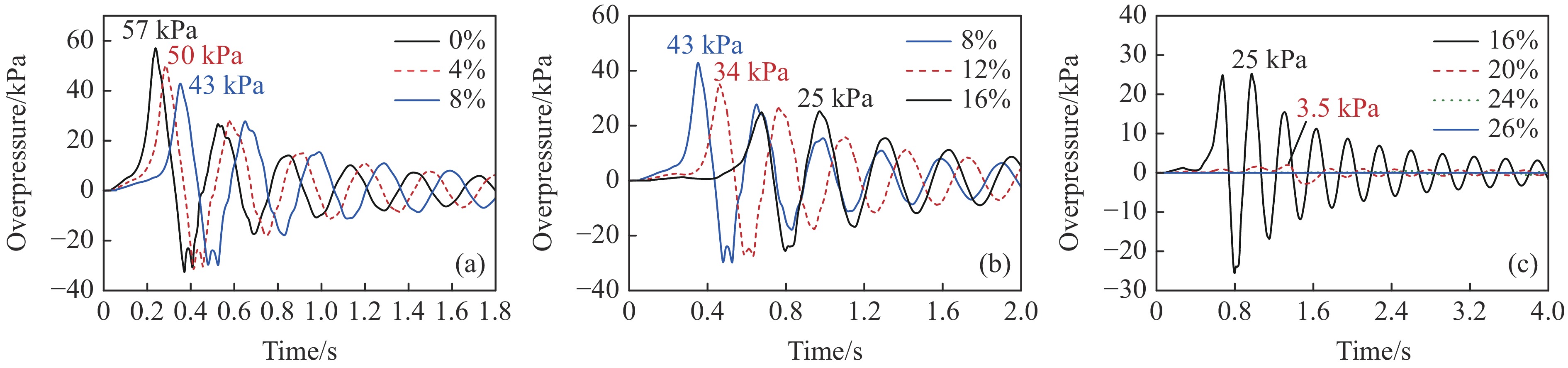
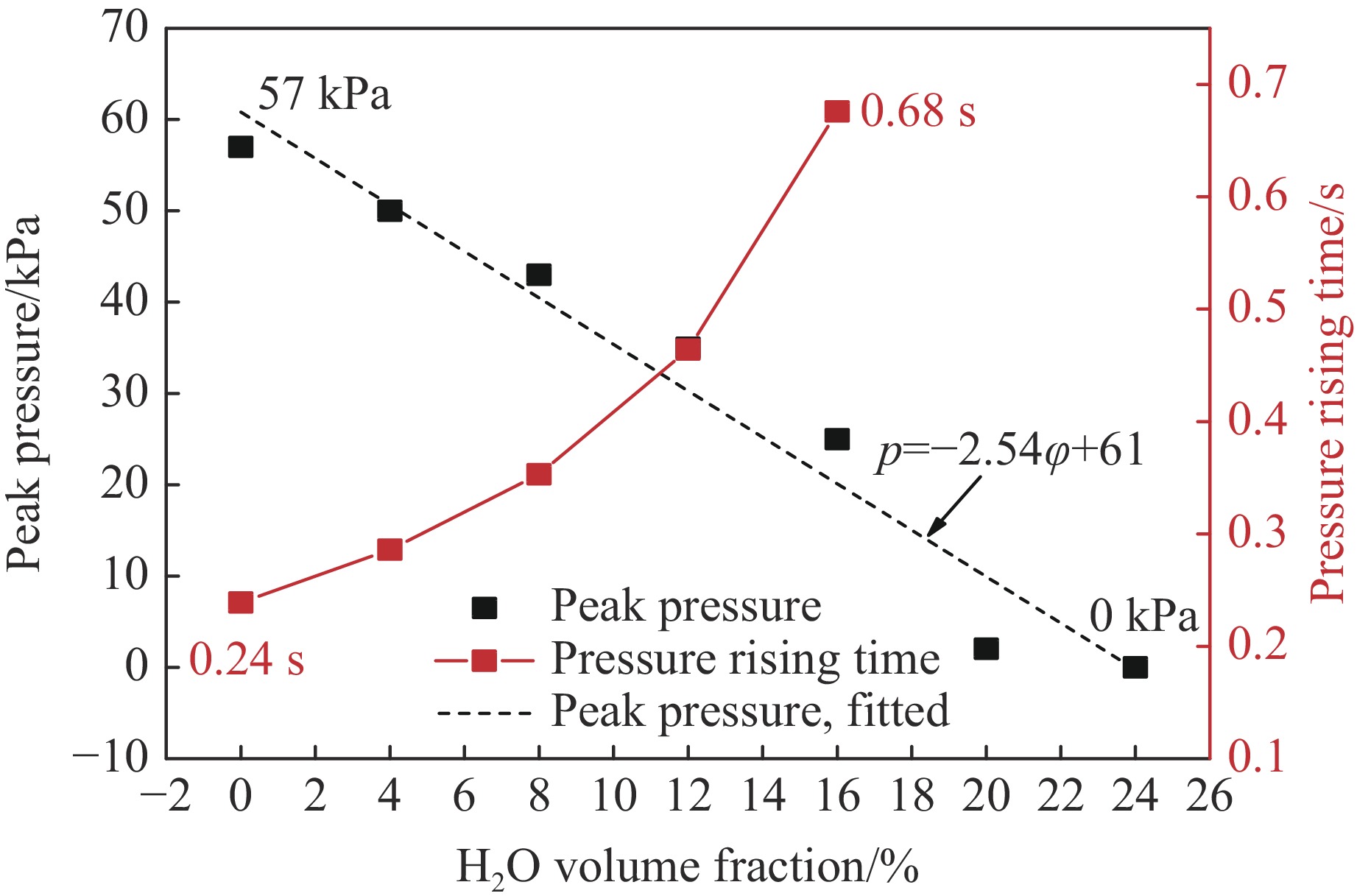
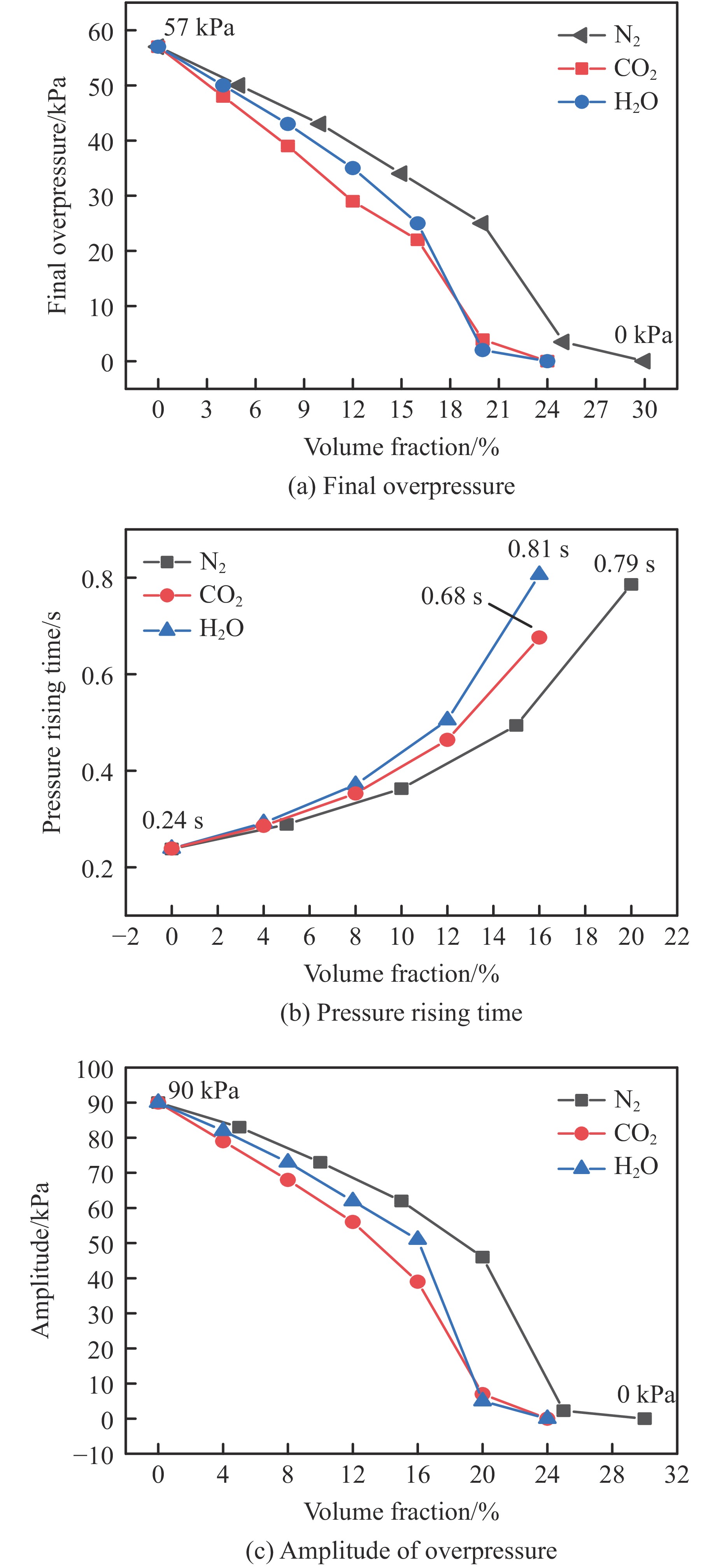
如图18所示,随着CO2的体积分数由零增长到24%,升压时间从0.24 s逐渐增长到0.71 s,超压峰值由57 kPa接近线性降低到0 kPa,即CO2的体积分数每增加10%,爆炸超压下降约24 kPa。
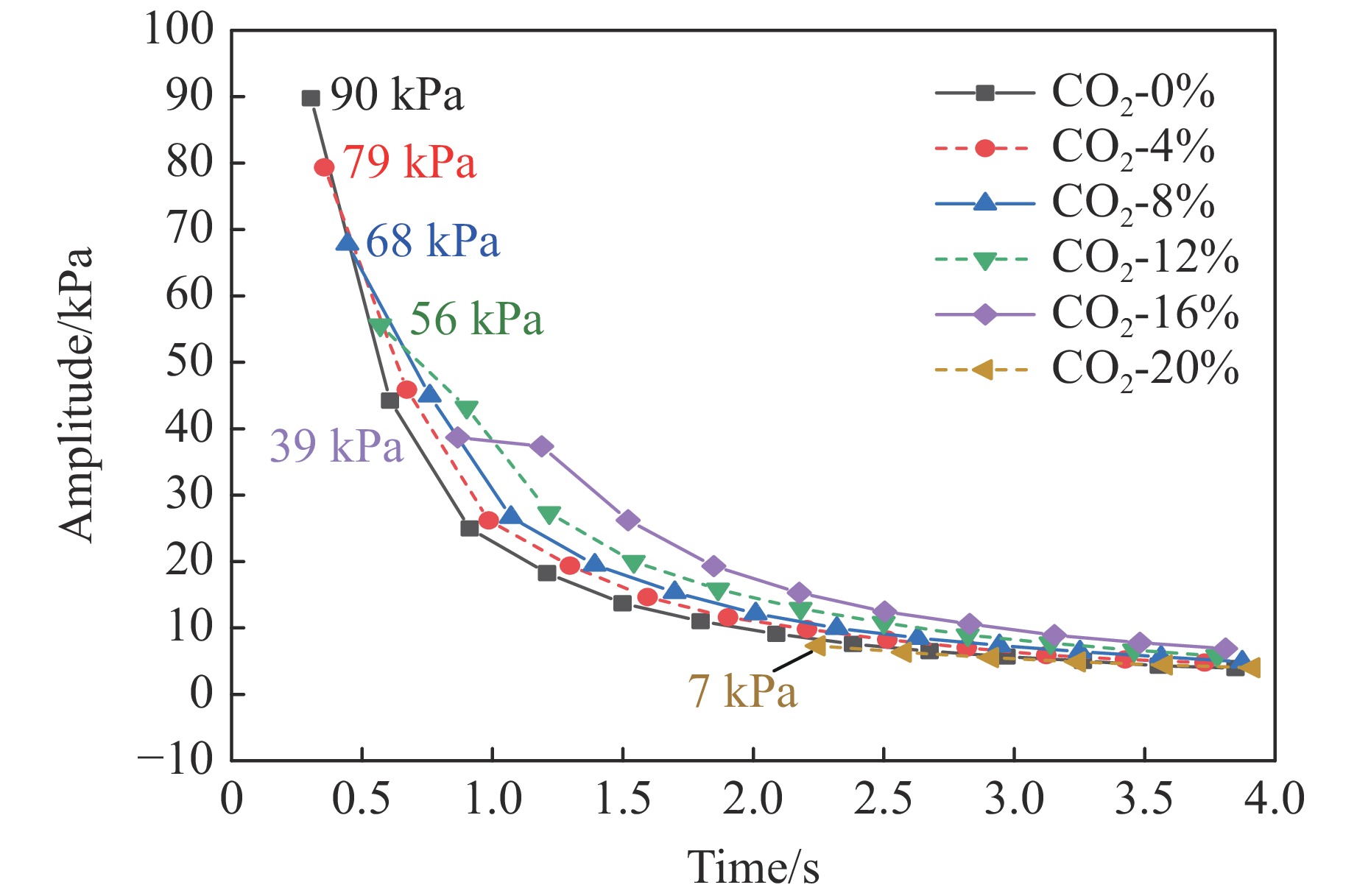
图19为爆炸超压振幅随时间的变化。可见,不同CO2体积分数条件下混合气体的压力振幅均随时间呈指数函数下降。泄爆管道内压力的振荡类似于阻尼振动[41],振荡可归因于管内气体过度泄放引起气体往复的泄出与回流[19],因此可以认为,压力振幅的下降与振荡过程中气体动能被耗散有关。此外,随着CO2的体积分数由零增加到20%,最大压力振幅由90 kPa减小为7 kPa,这是CO2导致的混合气体燃烧速率降低、爆炸超压和管内气体流速减小的结果。
图20给出了压力振荡频率随时间的变化。可以发现,振荡频率随CO2体积分数的增加而降低,可解释为:CO2的体积分数越大,燃烧速率越低,管内气体通过燃烧获取的速度越小,气体往复运动一个周期所需时间更长,最终导致超压振荡频率降低。不考虑由超压时程曲线得到压力振荡频率的误差,可以发现,各工况下的压力振荡频率几乎不随时间而明显上升或下降,如果认为尾端泄爆管道内压力的振荡为阻尼振动,则压力振荡频率的不变可能与阻尼振动的等时性[41]有关。
2.2.2 N2的抑爆效果
图21为不同N2体积分数下混合气体爆炸超压时程曲线。可以发现,当N2的体积分数达到30%时,混合气体仅发生缓慢燃烧,管内压力无明显变化;当N2的体积分数为31%时,爆炸被完全抑制,说明尾端泄爆管道内N2的完全抑爆浓度与密闭管道相同,均为30%。
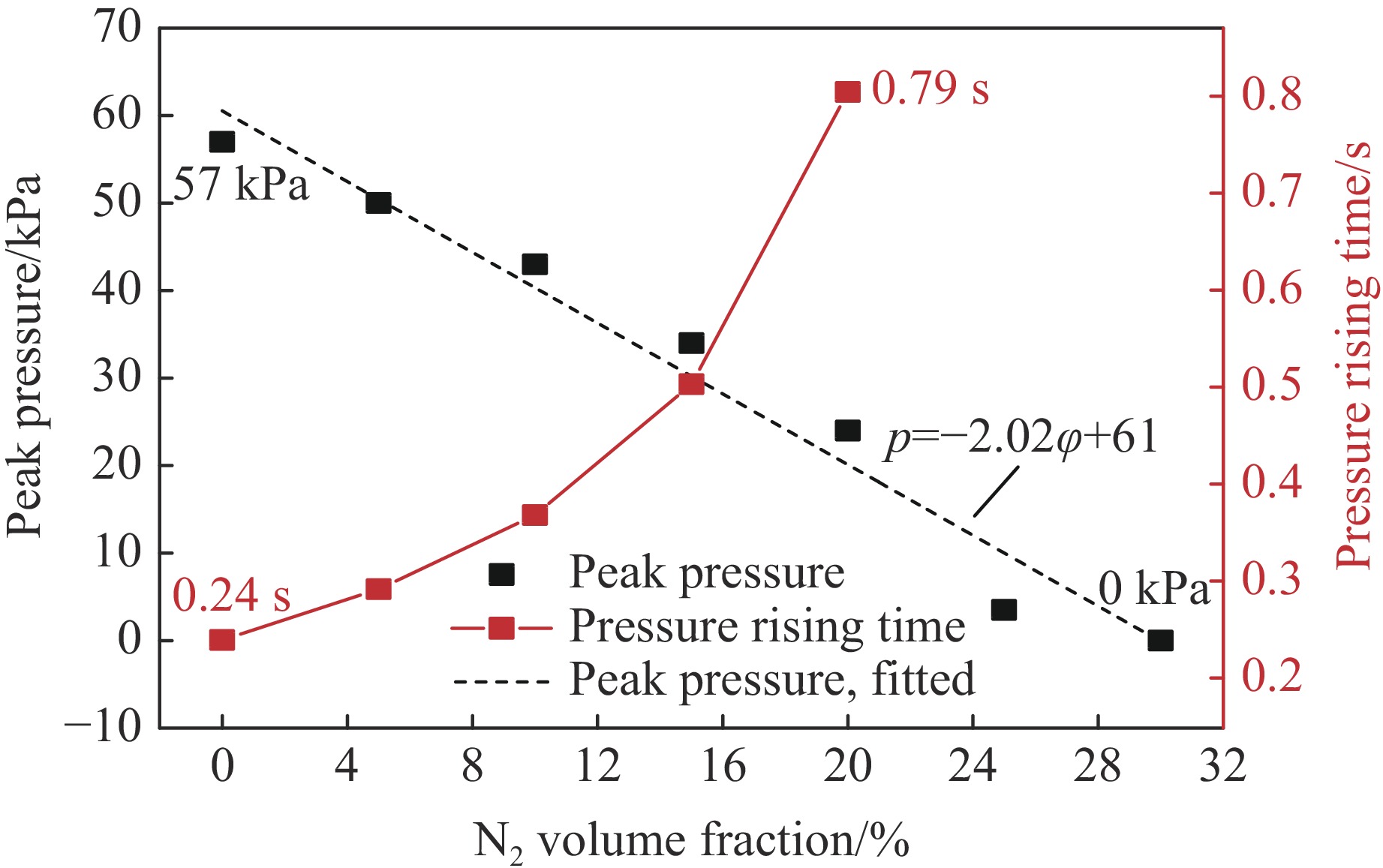
如图22所示,当N2的体积分数由零增加到30%,升压时间由0.24 s增长到0.79 s,超压峰值由57 kPa接近线性降低到0 kPa,即CO2的体积分数每增加10%,爆炸超压下降20 kPa。
压力振幅和压力振荡频率随时间的变化分别如图23和图24所示。可以看到,随着N2体积分数的升高,超压振幅和振荡频率均逐渐减小,说明N2对尾端泄爆管道内爆炸超压的振荡效应具有一定的抑制作用。
2.2.3 水蒸气的抑爆效果
图25为不同水蒸气体积分数下甲烷/空气混合气体的爆炸超压时程曲线。可以发现,尾端泄爆管道内水蒸气完全抑制爆炸的体积分数与密闭管道相同,均为26%。
从图26可以看出,随着水蒸气体积分数由零增加到24%,升压时间从0.24 s逐渐增长到0.68 s,最终爆炸超压由57 kPa接近线性降低到0 kPa,即水蒸气的体积分数每增加10%,爆炸超压下降约25 kPa。
图27和图28分别显示了不同水蒸气体积分数下超压振幅和振荡频率随时间的变化。可以看出,振幅和频率均随着水蒸气含量的增加而减小,说明添加水蒸气同样能够有效抑制尾端泄爆管道内甲烷爆炸超压的振荡效应。
2.3 不同气体抑制效果对比
由2.1、2.2节可知,CO2、水蒸气和N2的完全抑爆浓度分别为25%、26%、30%,且泄爆条件对完全抑爆浓度没有影响。密闭条件下完全抑制超压振荡时CO2、水蒸气和N2的体积分数分别为16%、16%、20%,尾端泄爆条件下压力振荡被完全抑制时的体积分数则与完全抑爆浓度相同,为25%、26%、30%。图29和图30分别为添加CO2、水蒸气和N2对长直空间内甲烷爆炸超压抑制作用的对比。可以发现,当添加气体浓度相同时,密闭和尾端泄爆管道均是添加CO2的爆炸超压及其振幅下降最明显,升压时间增加最长,添加水蒸气次之,添加N2最差,说明CO2对爆炸超压及其振荡的抑制作用最强,N2最弱。
3种气体抑爆效果的差异可解释为[27, 39]:N2仅能从物理角度(稀释、吸热)对超压及其振荡产生抑制,而CO2与水蒸气作为甲烷爆炸的燃烧产物,除物理抑爆外,还能够与燃烧区自由基反应,降低自由基浓度,抑制爆炸链传递速度,进而从化学角度对爆炸产生抑制,故抑爆效果优于N2。水蒸气和CO2抑爆效果的差异可能与二者比热容不同及参与链式反应的种类和程度不同有关。
3. 结 论
利用FLACS软件建立了长直(密闭/尾端泄爆)空间管道模型,并对模型进行了验证。基于已验证的模型研究了添加CO2、N2和水蒸气的化学当量比CH4/空气混合气体的爆炸情况,讨论了添加不同浓度气体对爆炸超压及其振荡的抑制作用,并对比了3种气体的抑爆效果。
(1)管道内气体爆炸超压随添加气体体积分数的增加而降低,CO2、水蒸气和N2的体积分数每增加10%,密闭管道气体爆炸的最终超压分别下降81、65、47 kPa,尾端泄爆管道超压峰值分别下降24、25、20 kPa,3种气体的完全抑爆浓度分别为25%、26%和30%(体积分数)。
(2)CO2、水蒸气和N2对长直空间燃气爆炸超压的振荡具有抑制作用,压力振幅和压力振荡频率均随添加气体体积分数的增加而减小,密闭管道内3种气体完全抑制超压振荡时的体积分数分别为16%、16%、20%,尾端泄爆管道分别为25%、26%、30%。
(3)CO2对爆炸超压及其振荡的抑制效果最好,水蒸气次之,N2最弱。这是因为N2仅能从物理角度对爆炸产生抑制,而CO2与水蒸气作为甲烷爆炸的燃烧产物,还能通过参与链式反应而抑制爆炸。水蒸气和CO2抑爆效果的差异可能与二者比热容以及参与链式反应的种类和程度不同有关。
-
表 1 混凝土的状态方程和本构方程参数
Table 1. Parameters of equation-of-state equation and constitutive equation of concrete
G/GPa ρ/(kg·m–3) A B N ˙ε0/s–1 C f′c/MPa Smax 14.8 2450 0.79 1.6 0.61 1.0 0.007 48 7.0 pl/MPa pc/MPa εf μc D1 D2 μ1 T/MPa K1/GPa 800 16.0 0.01 0.001 0.04 1.0 0.1 5 85 K2/GPa K3/GPa v β K/(1026 m–3) m KIC/(MPa·m1/2) –171 208 0.27 0.5 1.1452 6 2.758 -
[1] HOLMQUIST T J, TEMPLETON D W, BISHNOI K D. Constitutive modeling of aluminum nitride for large strain, high-strain rate, and high-pressure applications [J]. International Journal of Impact Engineering, 2001, 25(3): 211–231. doi: 10.1016/S0734-743X(00)00046-4 [2] 孙炜海, 鞠桂玲, 杨班权. 平头弹丸侵彻B4C陶瓷/金属复合靶板的数值模拟 [J]. 装甲兵工程学院学报, 2014, 28(2): 45–48SUN W H, JU G L, YANG B Q. Numerical simulation on penetration of B4C ceramic/metal composite targets struck by flat-ended projectiles [J]. Journal of Academy of Armored Force Engineering, 2014, 28(2): 45–48 [3] BACKMAN M E, GOLDSMITH W. The mechanics of penetration of projectiles into targets [J]. International Journal of Engineering Science, 1978, 16(1): 1–99. doi: 10.1016/0020-7225(78)90002-2 [4] 钱伟长. 穿甲力学 [M].北京: 高等教育出版社, 1984.QIAN W C. Penetration mechanics [M]. Beijing: High Education Press, 1984. [5] CORBETT G G, REID S R, JOHNSON W. Impact loading of plates and shells by free-flying projectiles: a review [J]. International Journal of Impact Engineering, 1996, 18(2): 141–230. doi: 10.1016/0734-743X(95)00023-4 [6] FORRESTAL M J, FREW D J, HANCHAK S J, et al. Penetration of grout and concrete targets with ogive-nose steel projectiles [J]. International Journal of Impact Engineering, 1996, 18(5): 465–476. doi: 10.1016/0734-743X(95)00048-F [7] LI Q M, REID S R, WEN H M, et al. Local impact effects of hard missiles on concrete targets [J]. International Journal of impact engineering, 2005, 32(1): 224–284. doi: 10.1016/j.ijimpeng.2005.04.005 [8] 陈小伟. 穿甲/侵彻问题的若干工程研究进展 [J]. 力学进展, 2009, 39(3): 316–351 doi: 10.3321/j.issn:1000-0992.2009.03.006CHEN X W. Advances in the penetration/perforation of rigid projectiles [J]. Advances in Mechanics, 2009, 39(3): 316–351 doi: 10.3321/j.issn:1000-0992.2009.03.006 [9] LI Q M, CHEN X W. Dimensionless formulae for penetration depth of concrete target impacted by a non-deformable projectile [J]. International Journal of Impact Engineering, 2003, 28(1): 93–116. doi: 10.1016/S0734-743X(02)00037-4 [10] GEBBEKEN N, GREULICH S, PIETZSCH A. Hugoniot properties for concrete determined by full-scale detonation experiments and flyer-plate-impact tests [J]. International Journal of Impact Engineering, 2006, 32(12): 2017–2031. doi: 10.1016/j.ijimpeng.2005.08.003 [11] 梁斌. 动能攻坚战斗部对混凝土靶侵爆效应研究 [D].绵阳:中国工程物理研究院, 2009.LIANG B. Research on invading explosion effect of concrete target by kinetic energy [D]. Mianyang: China Academy of Engineering Physics, 2009. [12] 黄民荣.刚性弹体对混凝土靶的侵彻与贯穿机理研究 [D].南京:南京理工大学, 2011.HUANG M R. Penetration and perforation mechanism of rigid projectile into the concrete target [D]. Nanjing: Nanjing University of Science & Technology, 2011. [13] 邓佳杰, 张先锋, 葛贤坤, 等. 基于局部相互作用理论的侵彻弹头部形状优化及仿真 [J]. 爆炸与冲击, 2017, 37(4): 611–620 doi: 10.11883/1001-1455(2017)04-0611-10DENG J F, ZHANG X F, GE X K, et al. Nose-shape optimization and simulation of projectiles penetrating into concrete target based on local interaction theory [J]. Explosion and Shock Waves, 2017, 37(4): 611–620 doi: 10.11883/1001-1455(2017)04-0611-10 [14] FORRESTAL M J, FREW D J, HICKERSON J P, et al. Penetration of concrete targets with deceleration-time measurements [J]. International Journal of Impact Engineering, 2003, 28(5): 479–497. doi: 10.1016/S0734-743X(02)00108-2 [15] 王成, 王万军, 宁建国. 聚能装药对混凝土靶板的侵彻研究 [J]. 力学学报, 2015, 47(4): 672–684WANG C, WANG W J, NING J G. Investigation on shaped charge penetrating into concrete targets [J]. Chinese Journal of Theoretical and Applied Mechanics, 2015, 47(4): 672–684 [16] 薛建锋, 沈培辉, 王晓鸣. 弹体斜侵彻混凝土靶的实验研究及其数值模拟 [J]. 爆炸与冲击, 2017, 37(3): 536–543 doi: 10.11883/1001-1455(2017)03-0536-08XUE J F, SHEN P H, WANG X M. Experimental study and numerical simulation of projectile obliquely penetrating into concrete target [J]. Explosion and Shock Waves, 2017, 37(3): 536–543 doi: 10.11883/1001-1455(2017)03-0536-08 [17] KONG X Z, WU H, FANG Q, et al. Projectile penetration into mortar targets with a broad range of steiking velocities: test and analyses [J]. International of Journal of Impact Engineering, 2017, 106: 18–29. doi: 10.1016/j.ijimpeng.2017.02.022 [18] GUPTA N K, AITMAN B S, CARGILE J D, et al. Normalimpact of ogive nosed projectiles on thin plates [J]. International of Journal of Impact Engineering, 2001, 25(7): 641–660. doi: 10.1016/S0734-743X(01)00003-3 [19] WARREN T L, FOSSUM A F, FREW D J. Penetration into low-strength (23 MPa) concrete: target characterization andsimulations [J]. International of Journal of Impact Engineering, 2004, 30(5): 477–503. doi: 10.1016/S0734-743X(03)00092-7 [20] 董军, 邓国强, 杨科之, 等. 弹丸对混凝土薄板的冲击破坏效应 [J]. 岩石力学与工程学报, 2005, 24(4): 713–720 doi: 10.3321/j.issn:1000-6915.2005.04.029DONG J, DENG G Q, YANG K Z, et al. Damage effect of thin concrete slabs subjected to projectile impact [J]. Chinese Journal of Rock Mechanics and Engineering, 2005, 24(4): 713–720 doi: 10.3321/j.issn:1000-6915.2005.04.029 [21] 强洪夫, 范树佳, 陈福振, 等. 基于SPH 方法的聚能射流侵彻混凝土靶板数值模拟 [J]. 爆炸与冲击, 2016, 36(4): 516–524 doi: 10.11883/1001-1455(2016)04-0516-09QIANG H F, FAN S J, CHEN F Z, et al. Numerical simulation on penetration of concrete target by shaped charge jet with SPH method [J]. Explosion and Shock Waves, 2016, 36(4): 516–524 doi: 10.11883/1001-1455(2016)04-0516-09 [22] 张馨予, 吴艳青, 黄风雷. PBX装药弹体侵彻混凝土薄板的数值模拟 [J]. 含能材料, 2018, 26(1): 101–108ZHANG X Y, WU Y Q, HUANG F L. Numerical simulation on the dynamic damage of PBX charges filled in projectiles during penetrating thin concrete targets [J]. Chinese Journal of Energetic Materials, 2018, 26(1): 101–108 [23] 武海军, 黄风雷, 付跃升, 等. 钢筋混凝土中爆炸破坏效应数值模拟分析 [J]. 北京理工大学学报, 2007, 27(3): 200–204 doi: 10.3969/j.issn.1001-0645.2007.03.004WU H J, HUANG F L, FU Y S, et al. Numerical simulation of reinforced concrete breakage under internal blast loading [J]. Transactions of Beijing Institute of Technology, 2007, 27(3): 200–204 doi: 10.3969/j.issn.1001-0645.2007.03.004 [24] SWEGLE J W, ATTAWAY S W. On the feasibility of using smoothed particle hydrodynamics for underwater explosion calculations [J]. Computational Mechanics, 1995, 17(3): 151–168. doi: 10.1007/BF00364078 [25] LIBERSKY L D, PETSCHEK A G, CARNEY T C, et al. High strain Lagrangian hydrodynamics: a three-dimensional SPH code for dynamic material response [J]. Journal of Computational Physics, 1993, 109(1): 67–75. doi: 10.1006/jcph.1993.1199 [26] MONAGHAN J J. Smoothed particle hydrodynamics [J]. Annual Review of Astronomy and Astrophysics, 1992, 30(1): 543–574. doi: 10.1146/annurev.aa.30.090192.002551 [27] JOHNSON G R, COOK W. A constitutive model and data for metals subjected to large strains, high strain rates and high temperatures [C]// Proceedings of the Seventh International Symposium on Ballistics. The Hague, 1983: 541–547. [28] QIANG H, WANG K, GAO W. Numerical simulation of shaped charge jet using multi-phase SPH method [J]. Transactions of Tianjin University, 2008, 14(1): 495–499. [29] 强洪夫, 孙新亚, 陈福振, 等. 钻地弹侵爆多层混凝土建筑物的SPH 数值模拟 [J/OL]. 爆炸与冲击(2018–06–02) [2018–09–14].http://kns.cnki.net/kcms/detail/51.1148.O3.20180531.1019.034.html.QIANG H F, SUN X Y, CHEN F Z, et al. Numerical simulation of earth penetrating weapon penetration and explosion multi-layer concrete structures with SPH method [J]. Explosion and Shock Waves (2018–06–02) [2018–09–14]. http://kns.cnki.net/kcms/detail/51.1148.O3.20180531.1019.034.html. [30] 陈福振, 强洪夫, 高巍然. 气粒两相流传热问题的光滑离散颗粒流体动力学方法数值模拟 [J]. 物理学报, 2014, 63(23): 230206 doi: 10.7498/aps.63.230206CHEN F Z, QIANG H F, GAO W R. Numerical simulation of heat transfer in gas-particle two-phase flow with smoothed discrete particle hydro dynamics [J]. Acta Physica Sinica, 2014, 63(23): 230206 doi: 10.7498/aps.63.230206 [31] 强洪夫, 高巍然. 完全变光滑长度SPH法及其实现 [J]. 计算物理, 2008, 25(5): 569–575 doi: 10.3969/j.issn.1001-246X.2008.05.008QIANG H F, GAO W R. SPH method with fully variable smoothing lengths and implementation [J]. Chinese Journal of Computational Physics, 2008, 25(5): 569–575 doi: 10.3969/j.issn.1001-246X.2008.05.008 [32] 强洪夫.光滑例子流体动力学方法及应用 [M]. 北京: 科学出版社, 2017: 246–287. [33] 金乾坤. 混凝土动态损伤与失效模型 [J]. 兵工学报, 2006, 27(1): 10–14 doi: 10.3321/j.issn:1000-1093.2006.01.003JIN Q K. Dynamic damage and failure model for concrete materials [J]. Acta Armamentarii, 2006, 27(1): 10–14 doi: 10.3321/j.issn:1000-1093.2006.01.003 -







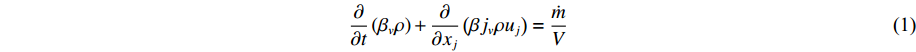
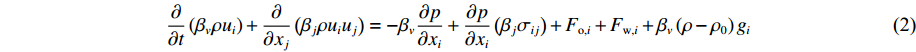
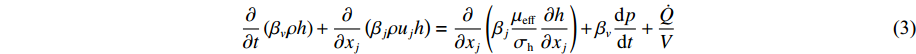
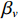
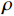

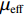
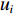
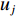
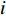
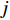
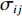

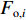
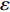
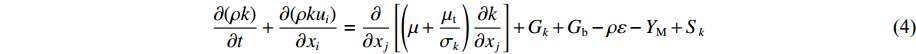
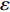
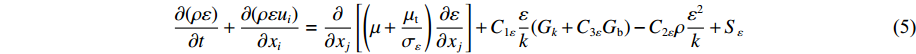
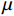
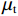

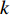
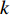
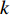

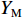

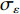
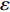
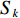
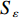
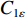
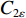
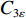
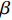
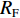

 下载:
下载:




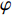


























 下载:
下载: