-
Abstract: A new approach is presented herein to predict the failure of metallic materials. A failure criterion that caters for the effects of stress triaxiality and Lode parameter is proposed and it is applicable not only to metals with ēf > ef but also to metals with ēf ≤ ef , here ēf and ef are the two parameters defined as the true strains at stress triaxiality of η = 1/3 for Lode parameters of ξ = 1 (axisymmetric stress state) and ξ = 0 (plane strain state) respectively. Furthermore, only two laboratory tests such as smooth bar tension test and pure shear test are needed to calibrate the failure criterion. The present failure criterion is proved in good agreement with the test data for various metals under different loading conditions.
-
Key words:
- failure criterion /
- metallic material /
- stress triaxiality /
- Lode parameter /
- smooth bar tension test /
- pure shear test
-
Rapid progress of computer technology and computing technique in recent years has made it possible to apply numerical simulations to the study of response and failure of structures under different loading conditions. As structural failure is usually caused by its material failure, it is, therefore, essential to develop a criterion that can both accurately predict the material failure and be aptly implemented in a numerical model.
Johnson and Cook[1-2] proposed a constitutive model which included a damage function to estimate the fracture of metallic materials. The fracture criterion is a function of hydrostatic pressure (stress triaxiality), strain rate and temperature but does not depend on the third invariant of the deviatoric stress tensor. Furthermore, the influence of stress triaxiality on the material ductility was also investigated by others[3-5]. It was found that the material ductility decreases as stress triaxiality increases.
Wierzbicki et al.[6] assessed the effectiveness and accuracy of 7 fracture criteria for metallic materials. It was demonstrated that the stress triaxiality alone cannot sufficiently characterize the effect of stress state on ductile fracture and that the effect of the Lode angle needs to be taken into account. Xue[7] further considered the joint effects of pressure and Lode angle on the ductile fracture and defined a fracture envelope in principal stress space and, in particular, suggested two kinds of Lode dependence functions. Bai and Wierzbicki[8] proposed an uncoupled material model for metal plasticity and ductile fracture in which the failure criterion was of the asymmetric three dimensional fracture locus as compared to the previously suggested symmetric ones. Later on, Bai and Wierzbicki[9] suggested a general form of asymmetric ductile fracture which represents an extension to the Mohr-Coulomb failure criterion. Meanwhile, other authors[10-12] also investigated the effect of Lode angle on the ductile fracture.
It should be mentioned here that comments made on the general formulation in terms of interdependence on hydrostatic and deviatoric state are valid for any material. However, there are two problems which need to be addressed. The first one is that there are often too many coefficients which need to be determined from laboratory tests and the second is that the average values of stress triaxiality and Lode angle were used in constructing a fracture locus instead of the actual values of these two parameters.
The objective of the present paper is to propose a new approach for the failure of metallic materials which is dependent on both stress triaxiality and Lode angle. It is applicable not only to metals with ēf>ef but also to metals with ēf≤ef, here ēf and ef are the two parameters defined as the true strains at stress triaxiality of η=1/3 for Lode parameters of ξ=1 (axisymmetric stress state) and ξ=0 (plane strain state) respectively. Furthermore, only two laboratory tests such as smooth bar tension test and pure shear test are needed to calibrate the failure criterion. The new failure criterion is compared with available material test data and discussed.
1. Formulation of a New Approach
A new approach is proposed in the following which can be used to predict the failure of metallic materials under different loading conditions by considering effects of both stress triaxiality and Lode angle. Firstly, some parameters are defined and then the new approach is formulated; finally, a failure criterion is given in which only two parameters are needed for determination from two laboratory tests.
1.1 Definition of
ˉef,γf,e∗f andˉγf,ef,γ∗f For a metallic material, a criterion considering the effects of both stress triaxiality
η and Lode angle parameterξ is needed to predict its failure.η andξ are defined by the following equationsη=σmˉσ=I13√3J21/2 (1) ξ=272J3ˉσ3=3√3J32J23/2 (2) where
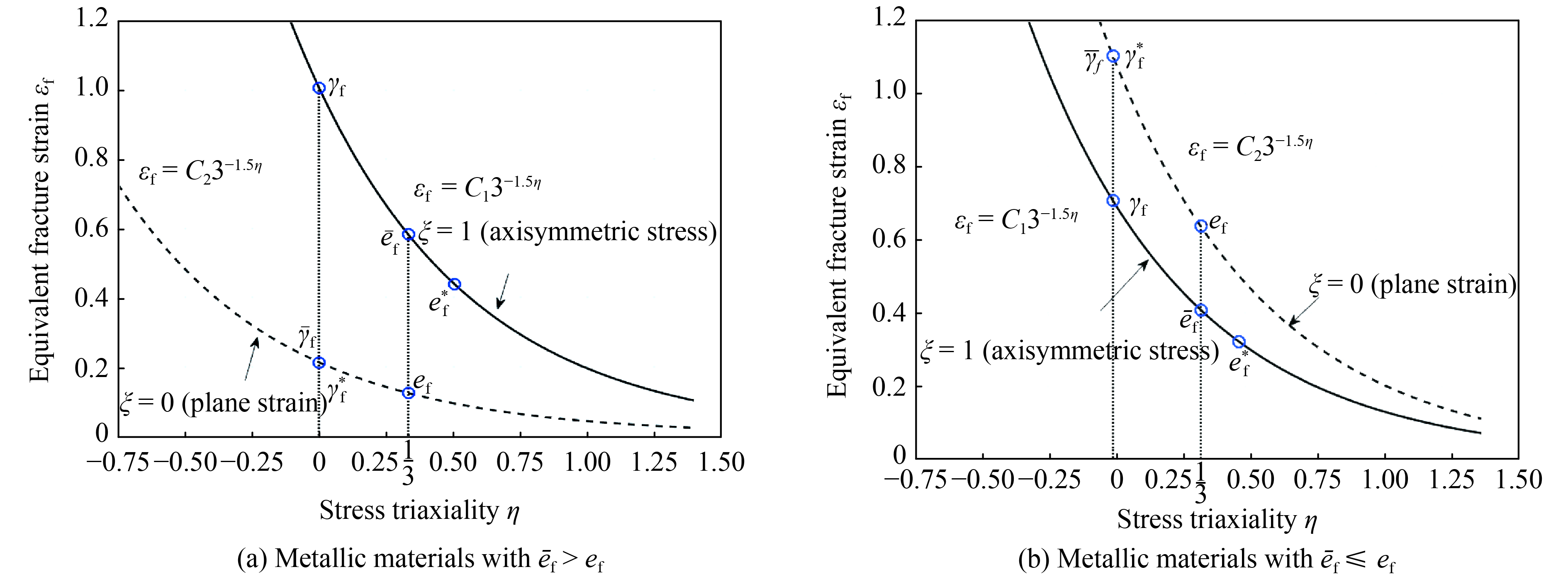
σm=(σ1+σ2+σ3)/3 ,ˉσ=√[(σ1−σ2)2+(σ2−σ3)2+(σ1−σ3)2]/2 are mean and equivalent stresses, respectively, withσ1 ,σ2 andσ3 as the principal stresses;I1=σ1+σ2+σ3 represents the first invariant of the stress tensor;J2=[(σ1−σ2)2+(σ2−σ3)2+(σ3−σ1)2]/6 andJ3=(σ1−σm)(σ2−σm)(σ3−σm) are the second and third invariants of the stress deviator tensor, respectively. The Lode angle parameterξ is related to the Lode angleθ on the π plane throughξ=−sin(3θ) and the range of the Lode angle is−π/6⩽θ⩽π/6 . Hence,ξ takes values of−1⩽ξ⩽1 .Fig.1 shows the definition of various parameters in the space of fracture strain
εf versus stress triaxialityη where two scenarios are considered, namely, materials withˉef>ef as shown schematically in Fig.1(a) and materials withˉef⩽ef as shown schematically in Fig.1(b). Hereˉef designates the theoretical value of axisymmetric stress state (i.e. tension withη=1/3,ξ=1 ),γf represents the theoretical value of axisymmetric stress state (i.e. shear withη=0,ξ=1 ) which can be taken asγf=√3ˉef ;ˉγf represents the theoretical value of plane strain state (i.e. shear withη=0,ξ=0 ),ef indicates the theoretical value of plane strain state (i.e. tension withη=1/3,ξ=0 ) which can be taken asef=ˉγf/√3 ;e∗f is the experimentally obtained true fracture strain in a smooth bar tension test (ξ=1 ) andγ∗f is the experimentally measured shear strain in a torsion test (ξ=0 ).For a constant Lode angle (i.e.
ξ=constant ), the relationship between equivalent fracture strain and stress triaxiality can be expressed as an exponential function[13]. Moreover, the theoretical value of fracture strain in shear (η=0 ) is√3 times that in tension (η=1/3 ) (Johnson and Cook[1]). It can be easily shown that the relationship between equivalent fracture strainεf and stress triaxialityη take the form ofεf=C1e−1.5(ln3)η forξ=1 after usingγf=√3ˉef and forξ=0 it takes the form ofεf=C2e−1.5(ln3)η after usingˉγf=√3ef as shown in Fig.1.1.2 A New Approach
It has been found experimentally that some more ductile metals such as OFHC cooper have a larger equivalent strain to fracture in shear than that in tension whilst some less ductile metals such as 4340 steel and 2024-T351 aluminum alloy have a smaller equivalent strain to fracture in shear than that in tension (see Johnson and Cook[2] and Wierzbicki et al.[6] for more details). In the following, a new approach is suggested for the failure of a metallic material on the basis of the discussion in Section 1.1 where two scenarios are considered depending upon the relative magnitude of
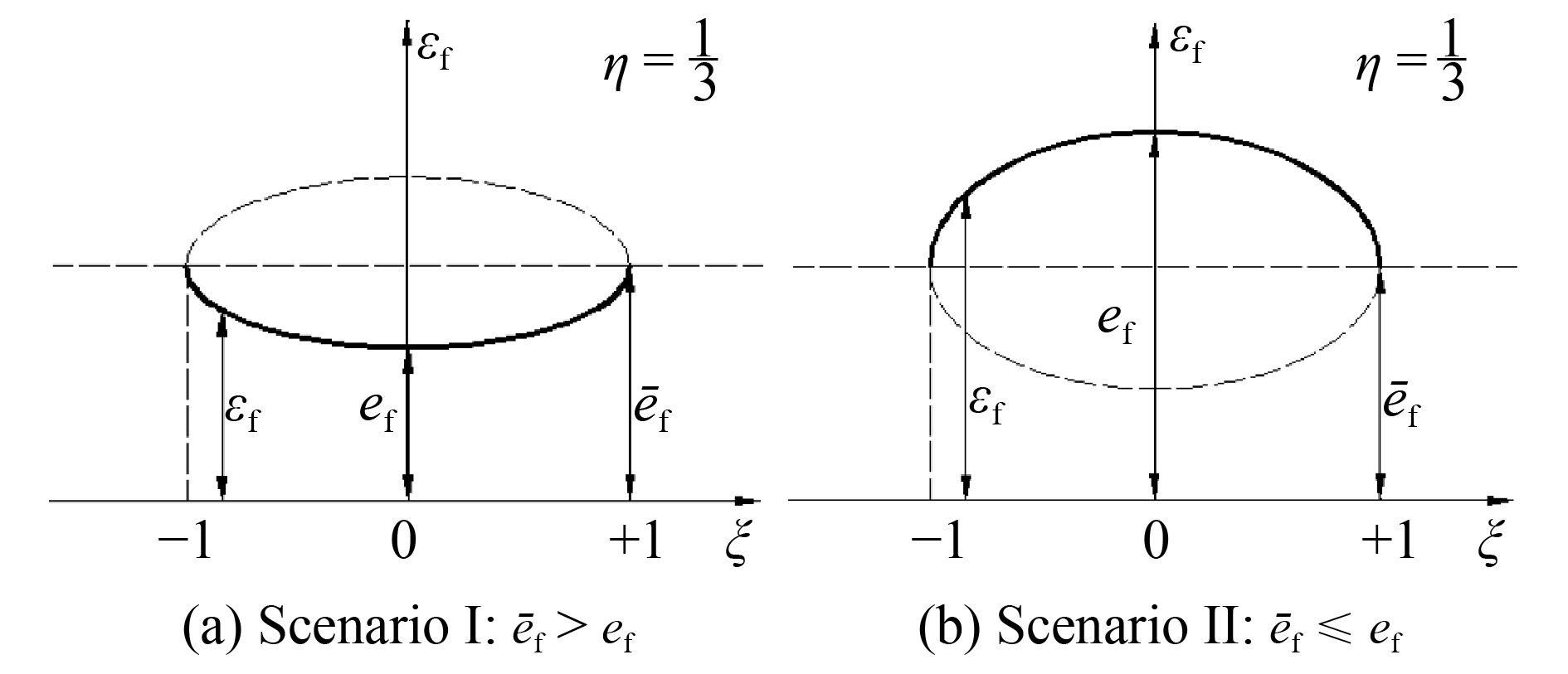
ˉef andef (Fig.2).1.2.1 Scenario I:
ˉef>ef The failure locus for a metallic material with
ˉef>ef in the space ofεf andξ forη=1/3 can be represented by the lower part of an elliptic curve as shown schematically in Fig.2(a), which can be described by the following equation(εf−ˉefˉef−ef)2+ξ2=1 (3) Hence, the equivalent fracture strain can be rewritten as
εf=ˉef−(ˉef−ef)(1−ξ2)1/2 (4) after taking it into consideration that
εf is less thanˉef .1.2.2 Scenario II:
ˉef⩽ef As shown schematically in Fig.2(b), the failure locus for a metallic material with
ˉef⩽ef in the space ofεf andξ forη=1/3 can be represented by the upper part of an elliptic curve, which can be described by the following expression(εf−ˉefef−ˉef)2+ξ2=1 (5) Hence, the equivalent fracture strain can be recast into the following form
εf=ˉef+(ef−ˉef)(1−ξ2)1/2 (6) or
εf=ˉef−(ˉef−ef)(1−ξ2)1/2 (7) It is noted from Eq.(4) and Eq.(7) that no matter what scenario it is the equivalent fracture strain takes the same form. It is also noted here that
ˉef andef are the specific points on the failure curves forξ=1 andξ=0 , respectively and, hence, they must satisfy the corresponding equations, namely,ˉef=C1e−1.5(ln3)η andef=C2e−1.5(ln3)η . Substitutingˉef=C1e−1.5(ln3)η andef=C2e−1.5(ln3)η into Eq.(4) or Eq.(7) and rearranging yieldεf(η,ξ)=[C1−(C1−C2)(1−ξ2)1/2]×3−1.5η (8) where
C1,C2 are constants to be determined from laboratory material tests.1.3 Determination of
C1,C2 A criterion for the failure of a metallic material (Eq.(8)) has been developed which is a function of both stress triaxiality and Lode angle parameter. The two constants, i.e.
C1,C2 can be estimated from two laboratory material tests such as smooth bar tension test and pure shear test.For the smooth bar tension test, the Lode angle parameter
ξ=1 and the experimentally obtained true fracture strain isεf=e∗f and its corresponding stress triaxiality isη=η∗ . Substituting these values into Eq.(8) and rearranging it, we arrive atC1=31.5η∗e∗f . Similarly, for the pure shear test, the Lode angle parameter isξ=0 and the experimentally obtained critical shear strain isεf=γ∗f and its corresponding stress triaxiality can be approximated asη=0 . Substituting these values into Eq.(8) and rearranging it, we haveC2=γ∗f .2. Comparison with Test Data and Discussion
The failure criterion developed in Section 1 is compared with the test data for various metallic materials under different loading conditions as reported in the literature. As discussed above only two laboratory material tests are needed to determine the values of
e∗f (the true fracture strain in tension),γ∗f (the critical rupture strain in shear) andη∗ (the stress triaxiality corresponding toe∗f ). In the following, the procedures for calculating the values of these 3 parameters are first briefly described and then the newly developed failure criterion (Eq.(8)) is compared with the available experimental results for various metallic materials.For a smooth bar tensile test, the equivalent true plastic strain to fracture in the necking cross-section of the smooth round bar can be approximately calculated by the following equation
e∗f=2ln(d0/df) (9) where
d0 is the initial diameter anddf is the minimum cross-section diameter at fracture. For a pure shear (i.e. tubular torsion) test, the equivalent true strain to fracture can be calculated by the following expressionγ∗f=Rφ/L(10) (10) where R is the initial radius of tubular torsion specimens, L is the initial length of the gage section and
φ is the average rotation angle to fracture found in the measured torque-rotation curves.The value of stress triaxiality
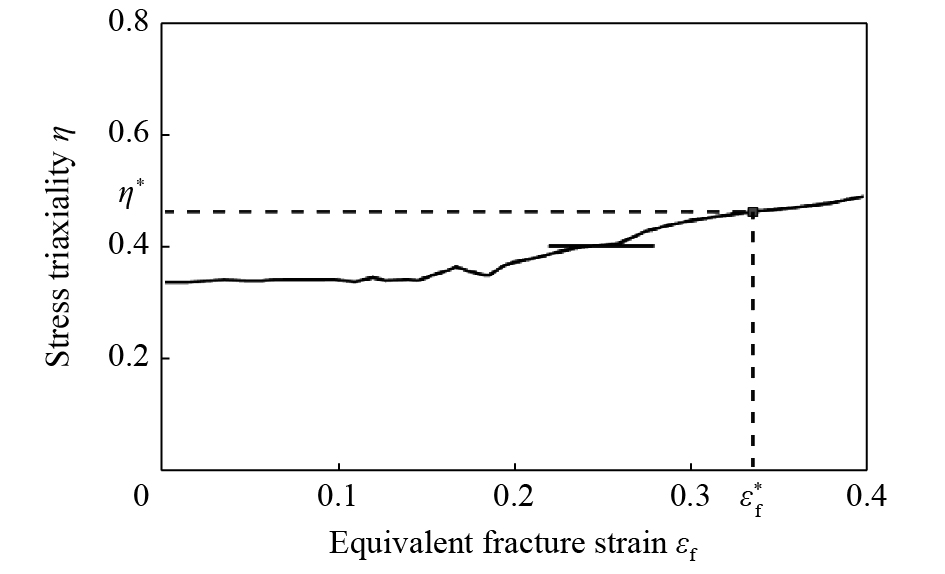
η∗ which corresponds to the equivalent true fracture straine∗f in a smooth bar tension test is obtained by the following method, as shown schematically in Fig.3 which shows variation of stress triaxiality with equivalent true strain in a smooth bar under tensile loading[14]. As can be seen from Fig.3, the stress triaxiality is not a constant during the entire tensile deformations. Before the necking occurs the deformations in the gauge length are nearly uniform and the stress triaxiality remains almost constant, namely, the theoretical value of 1/3; after the necking occurs the stress triaxiality is no longer a constant but varies with the tensile deformations due to a change in stress state in the necking section. As shown in Fig.3, it is straightforward to determine the value ofη∗ sincee∗f has been estimated from the smooth bar tension tests using Eq.(9).Comparisons are made in Fig.4–Fig.9 between the newly developed failure criterion (Eq.(8)) and the test data available for different ductile metals under different loading conditions. In Fig.4–Fig.9, the broken line and the dotted line represent Eq.(8) with
ξ=1 andξ=0 , respectively. The results from the two laboratory tests (i.e. smooth bar tension test and pure shear test) as presented in Fig.4–Fig.9(a) and those (i.e. smooth bar tension test and plane strain flat bar test) as given in Fig.9(b) which are used to determine the values of two constantsC1,C2 in Eq.(8) can be viewed as calibration data and all the other test results as verification data.Fig.4 shows comparison between the newly developed failure criterion (Eq.(8)) and the experimental data for the 4340 steel as reported by Johnson and Cook[2] who conducted 4 types of laboratory material tests including the smooth bar tension test, tubular torsion test and two notched tension tests. In the calculation, the value of
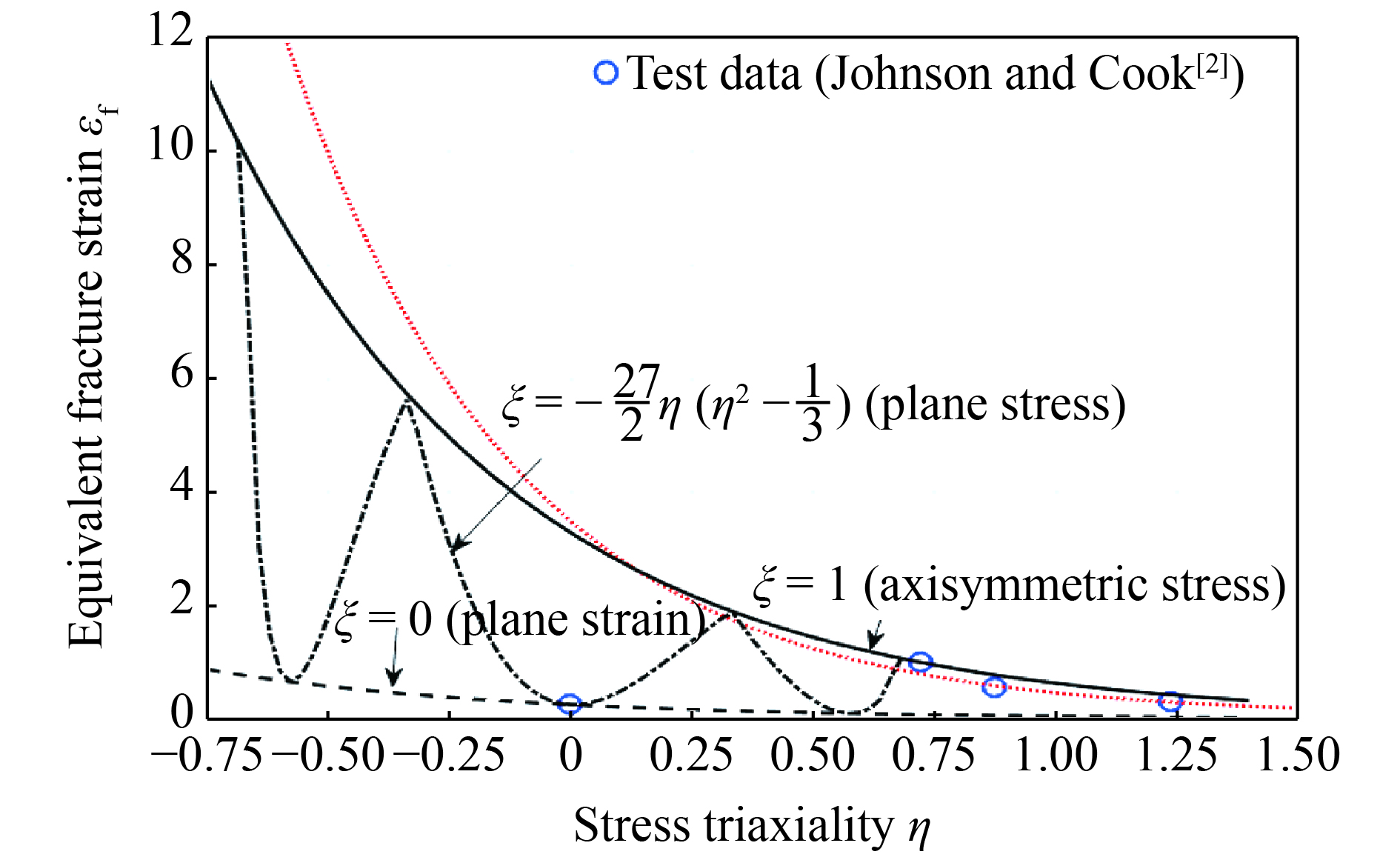
γ∗f is obtained directly from the tubular torsion test data in Fig.12 of Johnson and Cook[2] and it is equal to 0.253 6 (i.e.γ∗f=0.2536 ); Similarly, the value ofe∗f is determined to bee∗f=1 from the smooth bar tension test data in Fig.6 of Johnson and Cook[2] and the value ofη∗ is estimated to beη∗=0.7220 from Fig.11 of Johnson and Cook[2]. It is easy to calculate the values of the constantsC1 andC2 in Eq.(8) and prove them to beC1=31.5η∗e∗f=3.2864 andC2=γ∗f=0.2536 using the values of relevant parameters as obtained above. It can be seen that the present model predictions are in good agreement with the experimental results for the 4340 steel. Furthermore, it is easy to show thatef=0.1464 (Eq.(8) withη=1/3,ξ=0 ) andˉef=1.8974 (Eq.(8) withη=1/3,ξ=1 ). Hence, by definition the 4340 steel as examined in Johnson and Cook[2] belongs to Category I material sinceˉef>ef as discussed in Section 1.1.Fig.5 shows comparison of the present model (Eq.(8)) with the experimental data for the Armco iron as reported by Johnson and Cook[2] who conducted 4 types of laboratory material tests including smooth bar tension test, tubular torsion test and two notched tension tests. In the calculation, the value of
γ∗f is obtained directly from the tubular torsion test data in Fig.12 of Johnson and Cook[2] and it is equal to 2.915 0 (i.e.γ∗f=2.9150 ); Similarly, the value ofe∗f is determined to bee∗f=1.8670 from the smooth bar tension test data in Fig.6 of Johnson and Cook[2] and the value ofη∗ is estimated to beη∗=0.8150 from Fig.11 of Johnson and Cook[2]. It is easy to calculate the values of the constantsC1 andC2 in Eq.(8) to beC1=31.5η∗e∗f=7.1520 andC2=γ∗f=2.9150 using the values of relevant parameters as obtained above. It is evident that the present model predictions are in good agreement with the experimental results for the Armco iron. Moreover, it is easy to show thatef=1.6830 (Eq.(8) withη=1/3,ξ=0 ) andˉef=4.1292 (Eq.(8) withη=1/3,ξ=1 ). By definition the Armco iron as investigated in Johnson and Cook[2] belongs, therefore, to Category I material sinceˉef>ef as discussed in Section 1.1.Fig.6 shows comparison between Eq.(8) and the test results for the OFHC copper as reported by Johnson and Cook[2] who performed 4 types of laboratory material tests including smooth bar tension test, tubular torsion test and two notched tension tests. In the calculation, the value of
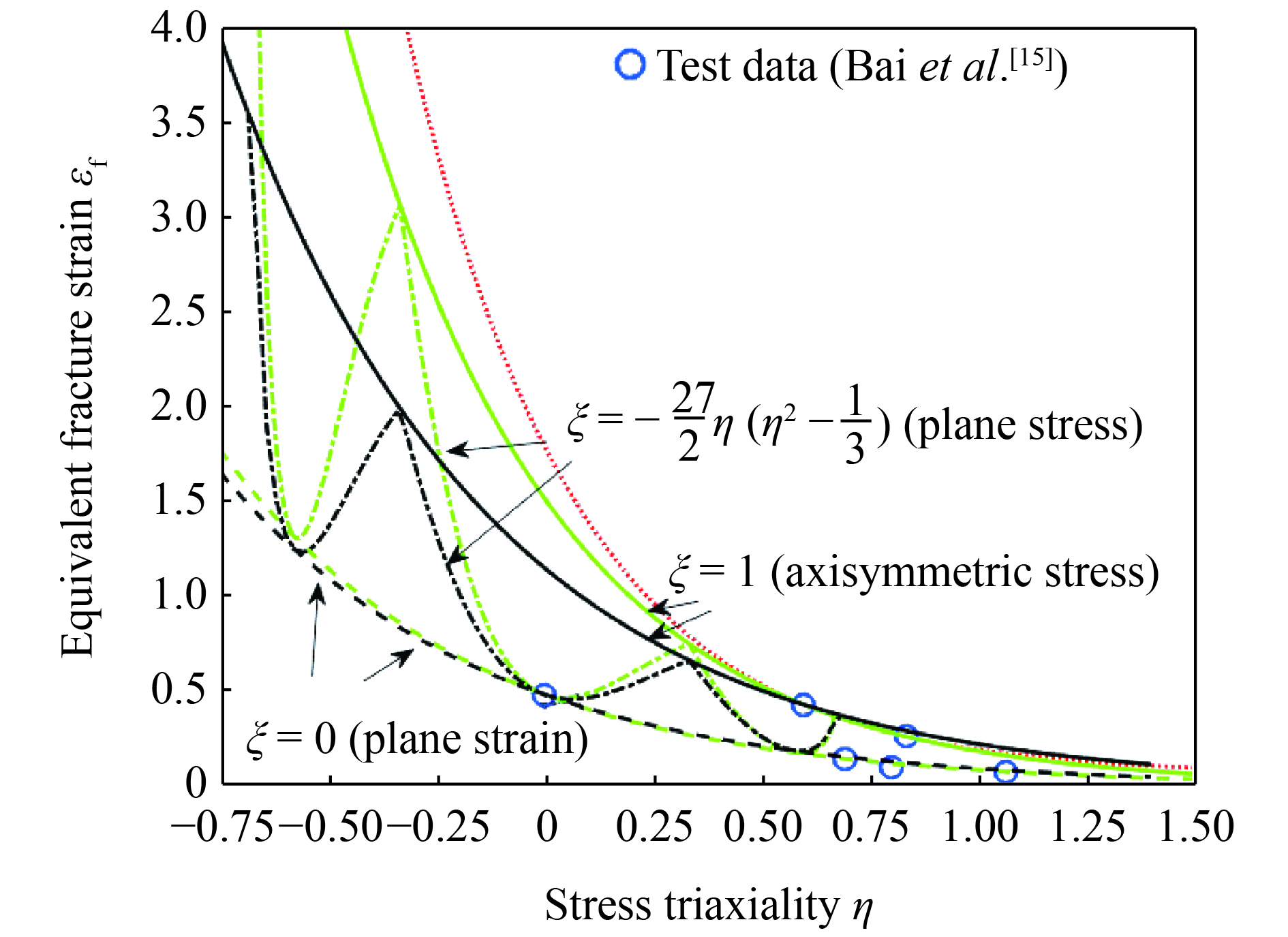
γ∗f is taken to be 8.700 0 (i.e.γ∗f=8.7000 ) which was obtained from the torsion test on another shorter-gage specimen (Johnson and Cook[2]). This is because none of the OFHC copper specimens fractured due to rotational limitations of the torsion tester as pointed out by Johnson and Cook. On the other hand, the value ofe∗f is determined to bee∗f=1.4480 from the smooth bar tension test data in Fig.6 of Johnson and Cook[2] and the value ofη∗ is estimated to beη∗=0.7300 from Fig.11 of Johnson and Cook[2]. It is easy to calculate the values of the constantsC1 andC2 in Eq.(8) to beC1=31.5η∗e∗f=4.8219 andC2=γ∗f=8.7000 using the values of relevant parameters as obtained above. It is shown that the present model predictions are in good agreement with the experimental results for the OFHC copper. Furthermore, it is easy to show thatef=5.0229 (Eq.(8) withη=1/3,ξ=0 ) andˉef=2.7839 (Eq. (8) withη=1/3,ξ=1 ). Hence, by definition the Armco iron as examined in Johnson and Cook[2] belongs to Category II material sinceˉef⩽ef as discussed in Section 1.1.Fig.7 shows comparison of Eq.(8) with the test data for the 1045 steel as studied by Bai et al.[15] who carried out 6 types of laboratory material tests including smooth bar tension test, tubular torsion test, notched bar tension test and 3 flat grooved plane strain tests. In the calculation, the value of
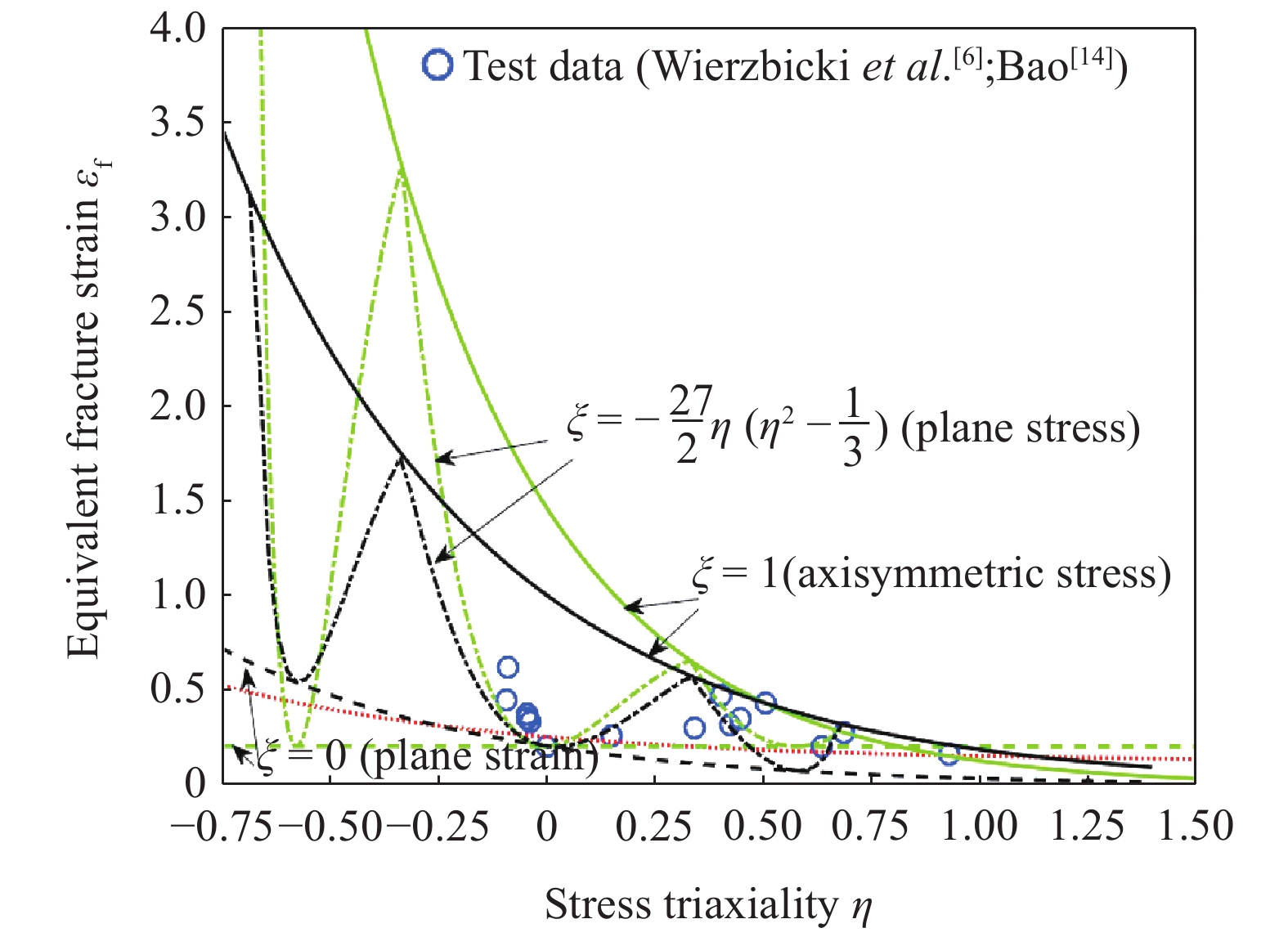
γ∗f is obtained from the tubular torsion test and directly taken from Eq.(24) of Bai et al.[15] to be 0.480 0 (i.e.γ∗f=0.4800 ); the value ofe∗f is taken bee∗f=0.4269 directly from Table 2 of Bai et al.[15] for the smooth bar tension test which was calculated using Eq.(13) of Bai et al.[15] and the value ofη∗ is estimated to beη∗=0.5990 from Fig.18 of Bai et al.[15]. It is easy to calculate the values of the constantsC1 andC2 in Eq.(8) to beC1= 31.5η∗e∗f=1.1456 andC2=γ∗f=0.4800 using the values of relevant parameters as obtained above. It is demonstrated that the present model predictions are in good agreement with the experimental results for the 1045 steel. Furthermore, it is easy to show thatef=0.2771 (Eq.(8) withη=1/3,ξ=0 ) andˉef=0.6614 (Eq.(8) withη=1/3,ξ=1 ). Hence, by definition the 1045 steel as studied in Bai et al.[15] belongs to Category I material sinceˉef>ef as discussed in Section 1.1.Fig.8 shows comparison of the newly developed failure criterion (Eq.(8)) with the test data for 2024-T351 aluminum alloys as reported by Wierzbicki et al.[6] and Bao[14] who conducted 15 types of laboratory material tests. In the calculation, the value of
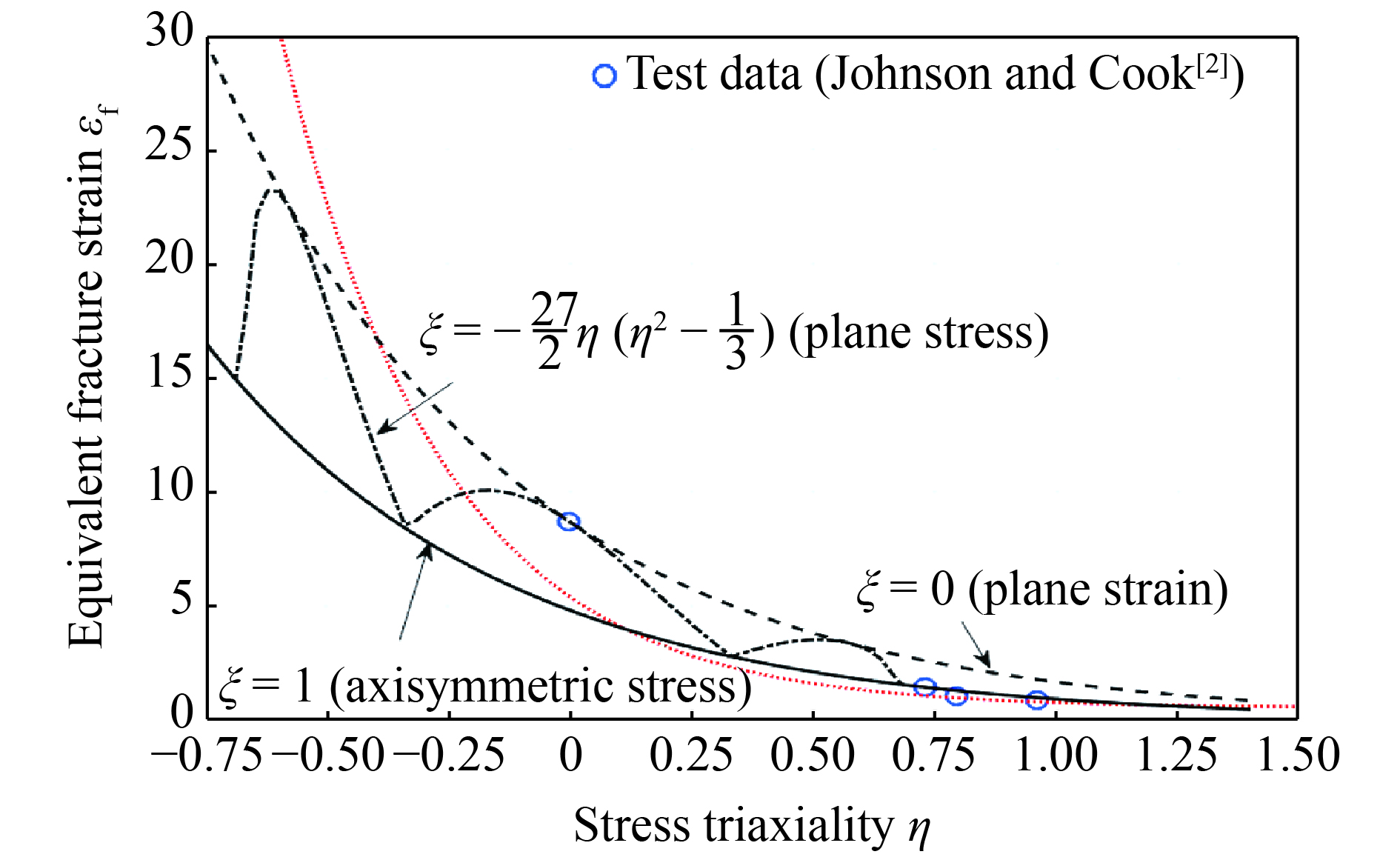
γ∗f is obtained directly from the shear test data in Table 1 of Bai et al.[9] and it is equal to 0.210 7 (i.e.γ∗f=0.2107 ); the value ofe∗f is determined to bee∗f=0.4375 from the smooth bar tension test data in Fig.8 of Wierzbicki et al.[6] and the value ofη∗ is estimated to beη∗=0.5050 from Fig.5.18 of Bao[14]. Hence, the values of the constantsC1 andC2 in Eq. (8) can be easily calculated to beC1=31.5η∗e∗f=1.0055 andC2=γ∗f=0.2107 using the values of relevant parameters as obtained above. It is evident from Fig.8 that the present model predictions are in good agreement with the experimental results for the 2024-T351 aluminum alloy. Moreover, it is easy to show thatef=0.1216 (Eq. (8) withη=1/3,ξ=0 ) andˉef=0.5805 (Eq.(8) withη=1/3,ξ=1 ). By definition the 2024-T351 aluminum as investigated in Wierzbicki et al.[6] and Bao[14] belongs, therefore, to Category I material sinceˉef>ef as discussed in Section 1.1. Figure 8. Comparison of the newly developed failure criterion with the test data for 2024-T351 aluminum alloy (Wierzbicki et al.[6]; Bao[14]) in the space of εf and η. Red line: Johnson-Cook failure criterion[2]; green lines: the model proposed by Xue and Wierzbicki[6]; black lines: the present model (Eq.(8))
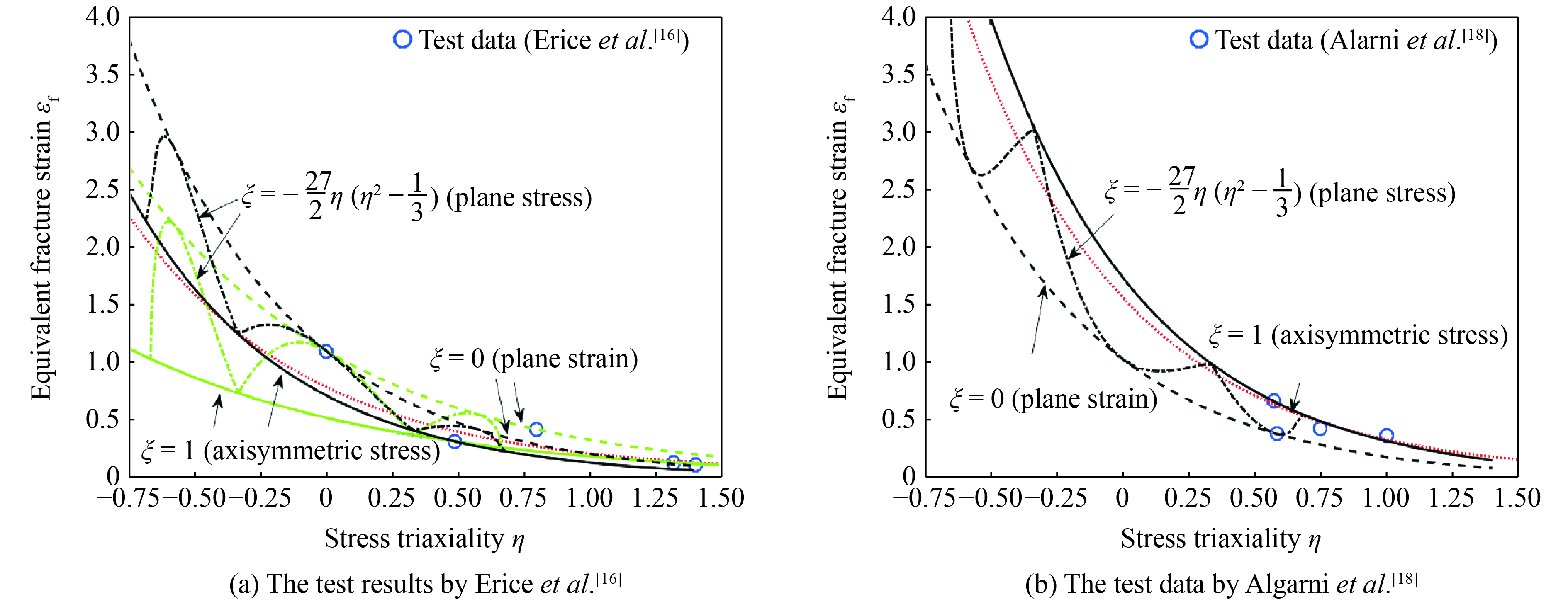
Figure 8. Comparison of the newly developed failure criterion with the test data for 2024-T351 aluminum alloy (Wierzbicki et al.[6]; Bao[14]) in the space of εf and η. Red line: Johnson-Cook failure criterion[2]; green lines: the model proposed by Xue and Wierzbicki[6]; black lines: the present model (Eq.(8))Fig.9(a) shows comparison of the newly developed failure criterion (Eq.(8)) with the test data for Inconel 718 nickel-base superalloy as reported by Erice et al.[16-17] who conducted 5 types of laboratory material tests including smooth bar tension test, shear test, plane strain test and two notched tension tests. In the calculation, the value of
γ∗f is obtained directly from the shear test data in Table 3 of Erice et al.[16] and it is equal to 1.100 0 (i.e.γ∗f=1.1000 ); Similarly, the value ofe∗f is determined to bee∗f=0.3200 from the smooth bar tension test data in Table 3 of Erice et al.[16] and the value ofη∗ is estimated to beη∗=0.4899 from Fig.14(a) of Erice et al.[16]. It is easy to calculate the values of the constantsC1 andC2 in Eq. (8) to beC1=31.5η∗e∗f=0.7174 andC2=γ∗f=1.1000 using the values of relevant parameters as obtained above. It can be seen that the present model predictions (Eq.(8)) are in good agreement with the experimental results for the Inconel 718 nickel-base superalloy as examined by Erice et al.[16]. Furthermore, it is easy to show thatef=0.6351 (Eq.(8) withη=1/3,ξ=0 ) andˉef=0.4142 (Eq.(8) withη=1/3,ξ=1 ). Hence, by definition the Inconel 718 nickel-base superalloy as examined in Erice et al.[16-17] belongs to Category II material sinceˉef⩽ef as discussed in Section 1.1.Comparison is made in Fig.9(b) between the Eq.(8) with the test data for Inconel 718 nickel-base superalloy as reported by Algarni et al.[18] who performed 4 types of laboratory material tests including smooth bar tension test, plane strain flat bar test and two notched bar tension tests. As no pure shear or tubular torsion test was done and the value of the constant
C2 needs to be calibrated by other plane strain (ξ=0 ) flat bar test data as reported in Algarni et al.[18] in the calculation. From Table 8 of Algarni et al.[18] and Fig.10 of Algarni et al.[18], it is easy to get the value of the experimental true fracture strain (0.400 4) and the corresponding computed stress triaxiality (0.583 1). Hence, it is easy to show thatC2=1.0465 ; Similarly, from the smooth bar tension test data in Table 8 of Algarni et al.[18], it is easy to get the value of the true fracture strain (0.680 4) and the corresponding stress triaxiality (0.573 8) from Fig.10 of Algarni et al.[18]. Hence, It is easy to calculate the values of the constantsC1 in Eq.(8) to beC1=1.7516 . It can be seen that the present model predictions are in good agreement with the experimental results for the Inconel 718 nickel-base superalloy as examined by Algarni et al.[18]. Furthermore, it is easy to show thatef=0.6042 (Eq.(8) withη=1/3,ξ=0 ) andˉef=1.0113 (Eq.(8) withη=1/3,ξ=1 ). Hence, by definition the Inconel 718 nickel-base superalloy as examined in Algarni et al.[18] belongs to Category I material sinceˉef>ef as discussed in Section 1.1.It appears from Fig.9(a) and Fig.9(b) that the results obtained for the 718 nickel-base superalloy materials investigated by Erice et al.[16] and Algarni et al.[18] are contradictory to each other. It should be mentioned here that the Inconel 718 nickel-base superalloy employed by Erice et al.[16-17] was much more ductile than that used by Algarni et al.[18]. Closer examination reveals that the discrepancy between these two Inconel 718 nickel-base superalloy materials may be due to different heat treatment (for example, the superalloy used by Erice et al.[16] was precipitation hardened) and different chemical composition (for instance, the superalloy employed by Algarni et al.[18] had Fe).
Also shown in Fig.4–Fig.9 are the fracture loci for the plane stress state (as indicated by the black solid line) which are predicted theoretically by the present model with
ξ being replaced by the following expressionξ=−272η(η2−13) (11) As can be seen, some of the shapes of the fracture loci for the plane stress state as shown in Fig.4, Fig.5, Fig.7, Fig.8 and Fig.9(b) open upward whilst the others as shown in Fig.6 and Fig.9(a) open downward. This is no surprising since the plane stress state is only a special case of the present model which divides materials into two categories, i.e. Category I material with
ˉef>ef and Category II material withˉef⩽ef .The predictions from the Johnson-Cook failure criterion[2] and the model suggested by Xue and Wierzbicki[6] are also shown in Fig.7, Fig.8, Fig.9(a) whilst in Fig.4, Fig.5, Fig.6 and Fig.9(b) only the predictions from the Johnson-Cook failure criterion are given and those from the model proposed by Xue and Wierzbicki are not available due to lack of test data. The values of the empirical constants D1–D3 in the Johnson-Cook failure criterion[2] and c1–c4 in the model proposed by Xue and Wierzbicki[6] are evaluated and listed in Table 1. It is clear from these figures that the Johnson-Cook failure criterion has failed to give consistent results and this is not surprising since it does not taken into account the effect of Lode angle. It is also clear from these figures that the present model (Eq.(8)) and the model proposed by Xue and Wierzbicki[6] produce more or less similar results for stress triaxiality greater than zero approximately whilst for stress triaxiality less than zero the difference between these two model predictions increases with decreasing stress triaxiality. It should be mentioned here that only two standard laboratory material tests (i.e. smooth bar tensile test and pure shear (tubular torsion) test) are needed to calibrate the two empirical constants in the present model whereas at least two axisymmetric tests (i.e., two notched tensile tests) and two plane strain tests (namely, pure shear test and flat dog-bone tensile test) are required to estimate the four empirical constants in the model suggested by Xue and Wierzbicki[6].
Materials Johnson-Cook failure criterion Model proposed by Xue and Wierzbicki D1 D2 D3 c1 c2 c3 c4 4340 steel 0.05[2] 3.44[2] –2.12[2] Armco iron –2.20[2] 5.43[2] –0.47[2] OFHC copper 0.54[2] 4.89[2] –3.03[2] 1045 steel 0.06 1.72 –2.58 1.511 0 2.110 2 0.480 0 1.738 8 2024-T351 0.13[6] 0.13[6] –1.50[6] 1.466 0 2.394 0 0.210 0 0.005 0 Inconel 718[16] 0.04[16] 0.75[16] –1.45[16] 0.525 1 1.010 9 1.100 0 1.188 7 Inconel 718[18] 0.04 1.54 –1.60 3. Conclusions
A new approach has been presented in this paper to predict the failure of metallic materials with the main conclusions achieved.
(1) On the basis of the test data metals can be divided into two categories, namely metals with ēf>ef and metals with ēf≤ef, here ēf and ef are the two parameters defined as the true strains at stress triaxiality of η=1/3 for Lode parameters of ξ=1 (axisymmetric stress state) and ξ=0 (plane strain state), respectively.
(2) A new failure criterion, which takes into account the effects of stress triaxiality and Lode parameter, has been obtained.
(3) This newly developed failure criterion has been shown to accord well with the test data for various metals under different loading conditions.
(4) Only two laboratory tests such as the smooth bar tension test and the pure shear (tubular torsion) test are needed to calibrate the failure criterion.
-
Figure 8. Comparison of the newly developed failure criterion with the test data for 2024-T351 aluminum alloy (Wierzbicki et al.[6]; Bao[14]) in the space of εf and η. Red line: Johnson-Cook failure criterion[2]; green lines: the model proposed by Xue and Wierzbicki[6]; black lines: the present model (Eq.(8))
Table 1. Values of constants in the Johnson-Cook failure criterion[2] and the model proposed by Xue and Wierzbicki[6]
Materials Johnson-Cook failure criterion Model proposed by Xue and Wierzbicki D1 D2 D3 c1 c2 c3 c4 4340 steel 0.05[2] 3.44[2] –2.12[2] Armco iron –2.20[2] 5.43[2] –0.47[2] OFHC copper 0.54[2] 4.89[2] –3.03[2] 1045 steel 0.06 1.72 –2.58 1.511 0 2.110 2 0.480 0 1.738 8 2024-T351 0.13[6] 0.13[6] –1.50[6] 1.466 0 2.394 0 0.210 0 0.005 0 Inconel 718[16] 0.04[16] 0.75[16] –1.45[16] 0.525 1 1.010 9 1.100 0 1.188 7 Inconel 718[18] 0.04 1.54 –1.60 -
[1] JOHNSON G R, COOK W H. A constitutive model and data for metals subjected to large strains, high strain rates and high temperatures [C]//Proceedings of the 7th International Symposium on Ballistics, 1983, 21: 541-547. [2] JOHNSON G R, COOK W H. Fracture characteristics of three metals subjected to various strains, strain rates, temperatures and pressures [J]. Engineering Fracture Mechanics, 1985, 21(1): 31–48. doi: 10.1016/0013-7944(85)90052-9 [3] ALVES M, JONES N. Influence of hydrostatic stress on failure of axisymmetric notched specimens [J]. Journal of the Mechanics & Physics of Solids, 1999, 47(3): 643–667. [4] BØRVIK T, HOPPERSTAD O S, BERSTAD T. On the influence of stress triaxiality and strain rate on the behaviour of a structural steel. Part II. numerical study [J]. European Journal of Mechanics A/Solids, 2003, 22(1): 15–32. doi: 10.1016/S0997-7538(02)00005-0 [5] BAO Y, WIERZBICKI T. On fracture locus in the equivalent strain and stress triaxiality space [J]. International Journal of Mechanical Sciences, 2004, 46(1): 81–98. doi: 10.1016/j.ijmecsci.2004.02.006 [6] WIERZBICKI T, BAO Y, LEE Y W. Calibration and evaluation of seven fracture models [J]. International Journal of Mechanical Sciences, 2005, 47(4): 719–743. [7] XUE L. Damage accumulation and fracture initiation in uncracked ductile solids subject to triaxial loading [J]. International Journal of Solids and Structures, 2007, 44(16): 5163–5181. doi: 10.1016/j.ijsolstr.2006.12.026 [8] BAI Y, WIERZBICKI T. A new model of metal plasticity and fracture with pressure and Lode dependence [J]. International Journal of Plasticity, 2008, 24(6): 1071–1096. doi: 10.1016/j.ijplas.2007.09.004 [9] BAI Y, WIERZBICKI T. Application of extended Mohr-Coulomb criterion to ductile fracture [J]. International Journal of Fracture, 2010, 161(1): 1–20. doi: 10.1007/s10704-009-9422-8 [10] CHOCRON S, ERICE B, ANDERSON C E. A new plasticity and failure model for ballistic application [J]. International Journal of Impact Engineering, 2011, 38(8): 755–764. [11] BRÜNIG M, CHYRA O, ALBRECHT D. A ductile damage criterion at various stress triaxialities [J]. International Journal of Plasticity, 2008, 24: 1731–1755. doi: 10.1016/j.ijplas.2007.12.001 [12] MALCHER L, PIRES F M A, SÁ J M A C D. An extended GTN model for ductile fracture under high and low stress triaxiality [J]. International Journal of Plasticity, 2014, 54(2): 193–228. [13] RICE J R, TRACEY D. On the ductile enlargement of voids in triaxial stress fields [J]. Journal of the Mechanics & Physics of Solids, 1969, 17(3): 201–217. [14] BAO Y. Prediction of ductile crack formation in uncracked bodies [D]. Boston, Massachusetts: Massachusetts Institute of Technology, 2003. [15] BAI Y, TENG X, WIERZBICKI T. On the application of stress triaxiality formula for plane strain fracture testing [J]. Journal of Engineering Materials and Technology, 2009, 131(2): 021002. doi: 10.1115/1.3078390 [16] ERICE B, GÁLVEZ F. A coupled elastoplastic-damage constitutive model with Lode angle dependent failure criterion [J]. International Journal of Solids and Structures, 2014, 51(1): 93–110. doi: 10.1016/j.ijsolstr.2013.09.015 [17] ERICE B, PÉREZ-MARTÍN M, GÁLVEZ F. An experimental and numerical study of ductile failure under quasi-static and impact loadings of Inconel 718 nickel-base superalloy [J]. International Journal of Impact Engineering, 2014, 69: 11–24. doi: 10.1016/j.ijimpeng.2014.02.007 [18] ALGARNI M, BAI Y, YOUNGSIK C. A study of Inconel 718 dependency on stress triaxiality and Lode angle in plastic deformation and ductile fracture [J]. Engineering Fracture Mechanics, 2015, 147: 140–157. doi: 10.1016/j.engfracmech.2015.08.007 -


推荐:氢氘物态方程研究进展(特约综述)
第五届高压科学卓越青年学者评选通知
2024年度《高压物理学报》优秀审稿人评选结果
2024年上海光源同步辐射大压机实验技术培训班通知
《高压物理学报》将于2025年1月由双月刊变更为月刊
动载下材料物性机器学习与高通量研究专刊征稿启事
《高压物理学报》门户网站和投审稿系统7月28日~8月6日期间暂停运行
《高压物理学报》第二届青年编委会招募启事
《高压物理学报》2023年度优秀审稿人和优秀论文评选结果
第十四届全国爆炸力学学术会议 第二轮通知
第九届“从原子到地球”——高压科学研讨会第三轮通知
第九届“从原子到地球”——高压科学研讨会第二轮通知
第四届全国超高速碰撞学术会议通知(第二轮)
第二十一届中国高压科学学术会议第一轮通知
通知
推荐:高压下Fe92.5O2.2S5.3的熔化温度(特约稿件)
推荐:透明陶瓷的超高压制备研究进展(特约综述)
推荐:氢的高压奇异结构与金属化(特约综述)
推荐:第二主族氢化物的高压研究(特约综述)
推荐:氢氘物态方程研究进展(特约综述)
第五届高压科学卓越青年学者评选通知


























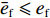
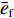
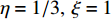
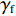
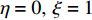
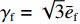
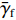
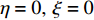
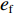
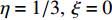
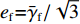
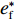
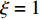
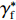
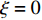

 DownLoad:
DownLoad:
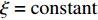
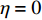

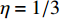
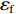
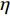
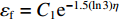
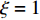
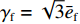
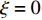
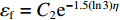
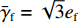
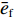
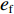

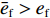
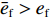
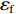
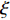
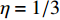

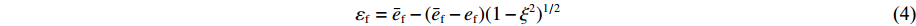

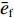

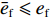
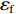
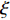
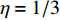
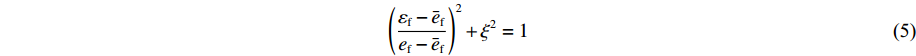

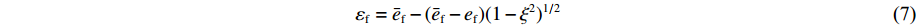
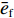
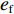
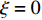
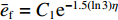

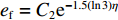
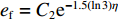
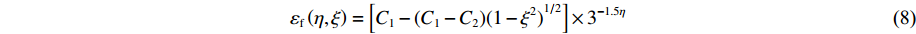



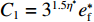
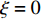







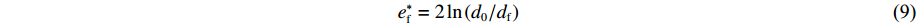


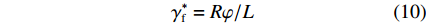






























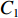
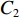
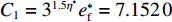
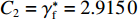
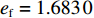
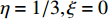
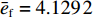













































































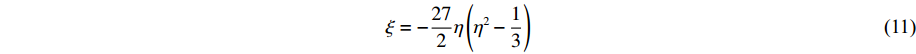


 DownLoad:
DownLoad:



 下载:
下载: