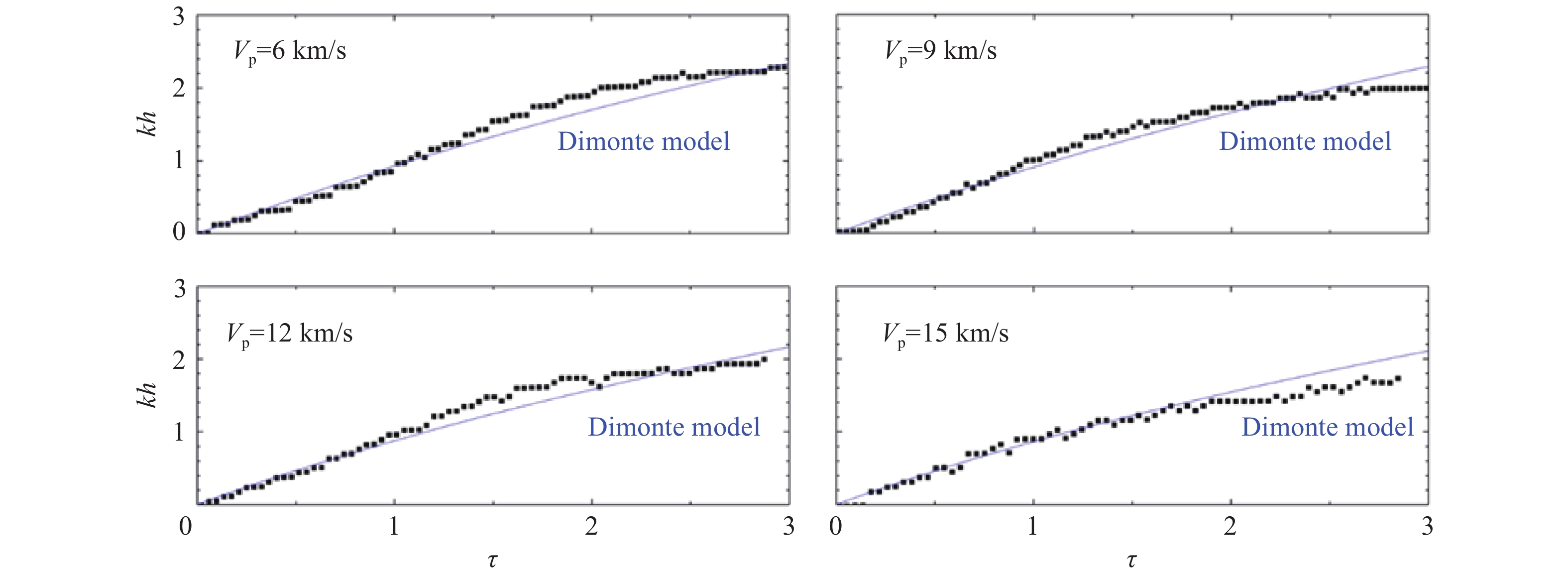
Microscale Self-Similarity Phenomenon of RM Instability on the Copper/Helium Interface with Molecular Dynamics Simulation
-
摘要: 极端冲击加载条件下的RM (Richtmyer-Meshkov)不稳定性在惯性约束核聚变领域有重要的学术价值和工程意义。宏观动力学方法受限于极端条件下的模型和参数准确性而难以直接应用，微观分子动力学方法则受限于计算量而难以直接模拟宏观尺度现象。为了解RM不稳定性微观与宏观规律之间的联系，采用基于嵌入原子多体势（EAM）的分子动力学方法模拟铜-氦微观尺度界面在极端冲击加载条件下（活塞冲击加载速度6～15 km/s）的RM不稳定性现象，对比文献提供的近似条件下宏观模拟结果发现，演化过程在唯象上完全相似。进一步比较了不同尺度（7.3～145.0 nm）、不同冲击加载速度（11.7～20.6 km/s）、不同初始界面扰动（扰动振幅与波长之比0.20～0.05）条件下振幅发展规律，发现在相同冲击动力条件和边界条件下，RM不稳定性的振幅增长规律在计算尺度范围内完全自相似，主要参数的变化特征符合理论预测。尽管理论模型因简化而存在一定偏差，但是微观模拟获得的振幅增长规律与宏观现象有相似的变化特征。Abstract: The Richtmyer-Meshkov instability (RMI) under extreme shock conditions has important academic and engineering significance in the field of inertial confinement fusion (ICF). Present macroscopic hydrodynamic methods are difficult to be directly applied to RMI in extreme conditions due to the lack of proper models and parameters in such states, while the microscopic results obtained by molecular dynamics (MD) are also difficult to be applied directly in macroscopic scale simulation due to computational cost. To understand the connection between macroscopic and microscopic RMI, the RMI evolution on copper-helium interface at different micro scales under different piston shock conditions (6–15 km/s) was simulated by the molecular dynamics method based on embedded-atom potential (EAM) models. Firstly, the RMI evolution obtained by MD was compared with available literature macroscopic results under similar conditions. Phenomenological similarity results between macroscopic and microscopic RMI is confirmed. The evolution histories of initial sinusoidal disturbance (amplitude/wave length ratio 0.20–0.05) at different incident shock wave speeds (11.7–20.6 km/s) and different scales (7.3–145.0 nm) from RMI simulations were further compared and analyzed. It is found that all amplitude evolution curves behave with self-similarity under same shock and boundary conditions, all main parameters vary in accordance with prediction of theoretical model. Although there exists some extent of discrepancy, similar amplitude evolution characteristics results are obtained by microscopic and macroscopic simulations.
-
镁铝合金（MB2）作为一类特殊的合金材料，具有低密度、高强度、易机械加工、耐腐蚀等特点，广泛应用于车辆工程、航空航天等领域，其动态加载下的力学和物理特性对相关结构设计等具有重要意义。国内外对MB2合金的早期研究主要集中于力学特性方面，通过开展低压动态响应特性实验研究，获得了材料的弹塑性响应[1]、动态损伤[2]及层裂行为[3]的初步认识，近年来Millett等[4]通过一维冲击加载研究了MB2合金在早期变形和位错条件下随加载应力、加载脉宽而变化的弹塑性和剪切强度行为。另外，对动态加载下MB2合金的物态方程及相变研究已开展了一系列工作，主要集中于冲击Hugoniot数据测量[5]，然而与动态加载下物理、力学特性紧密相关的相变研究尚处于起步阶段。声速作为应力扰动在材料中传播的定量表征参量，是获知材料动态响应特性（如相变、屈服强度、剪切模量）的主要途径之一[6–7]，然而相关的研究工作却鲜见报道。
本研究拟采用反向碰撞实验技术[8–9]，结合具有高时空分辨率的全光纤激光干涉测速技术DPS（Doppler Pin System）[10]，对MB2合金开展30～73 GPa压力范围内的冲击Hugoniot及声速测量实验，并与早期实验数据进行对比验证，分析MB2合金的冲击熔化行为。
1. 实 验
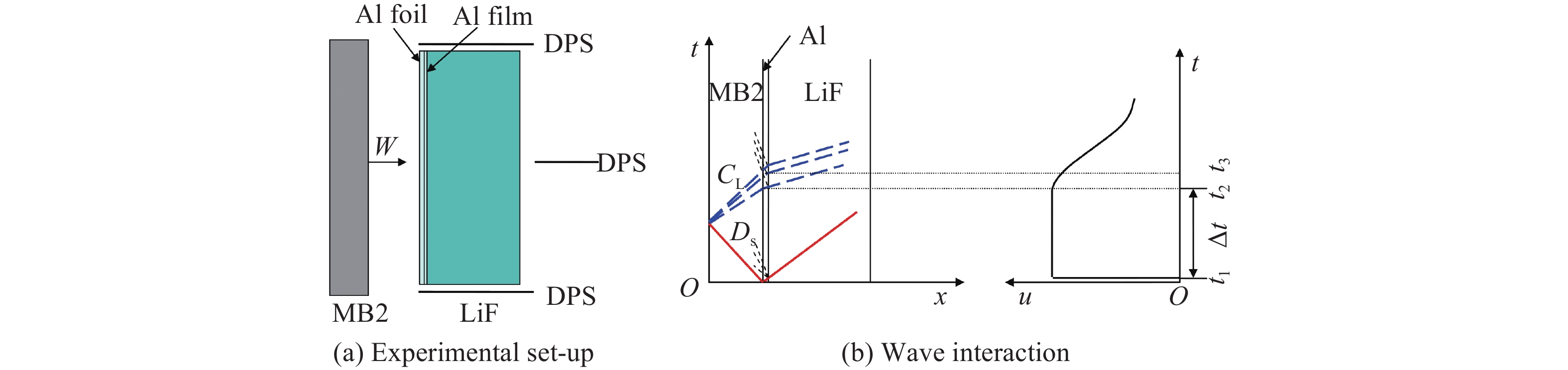
本研究涉及低压力区的声速测量，为此采用反向碰撞实验设计，即将样品材料制作成飞片，撞击LiF透明窗口，其原理如图1所示。在拉氏坐标下，飞片以速度W直接撞击窗口（t=t1），在飞片和窗口中分别产生左行冲击波和右行冲击波，引起飞片/窗口界面粒子速度的突跃。当飞片中的左行冲击波到达后界面时，将反射中心稀疏波，该稀疏波的波速就是材料在冲击压缩下的声速。如果样品材料发生冲击熔化，则中心稀疏波将以单一塑性波的形式在样品内传播，并在飞片/窗口界面处（t=t2）发生卸载，引起粒子速度的下降。如果样品材料没有发生冲击熔化，则中心稀疏波包括传播速度较快的弹性波和传播速度相对较慢的塑性波。当传播速度较快的弹性卸载波到达飞片/窗口界面时（t=t2），界面粒子速度下降，在速度剖面上形成第1个拐点；当塑性卸载波到达飞片/窗口界面时（t=t3），界面粒子速度再次突变，在速度剖面上形成第2个拐点；当后续稀疏波陆续到达飞片/窗口界面时，会导致界面粒子速度的连续下降；如果在卸载过程中样品材料发生相变，则也会在速度剖面上形成拐点。
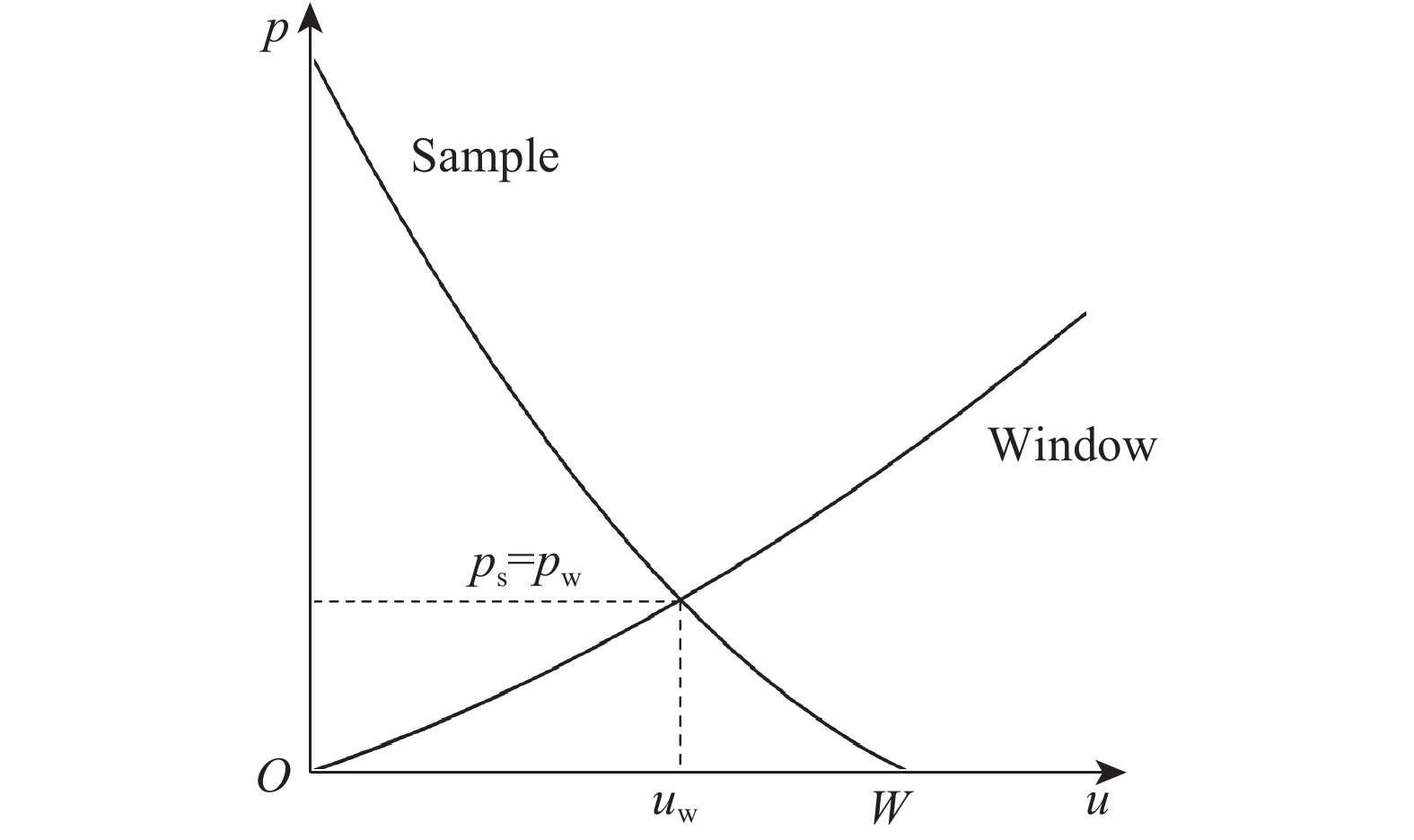
根据图2所示的界面连续性条件（其中p为冲击压力，u为粒子速度），结合Rankine-Hugoniot关系[11]，可以得到样品内的冲击波速度Ds为
Ds=ρ0wDwuwρ0s(W−uw)(1) 式中：D、
ρ 、W分别为冲击波速度、密度和飞片速度，下标s和w分别对应样品和窗口。如果窗口材料的D-u曲线满足线性关系Dw=C0w+λwuw(2) 式中：C0w和
λw 为窗口材料的Hugoniot参数，则（1）式可表示为Ds=ρ0w(C0w+λwuw)uwρ0s(W−uw) (3) 因此，只需要测得飞片（样品）击靶速度W和窗口的波后粒子速度uw，就可以获得样品的粒子速度us（us=W–uw）和对应的冲击波速度Ds。随后，由波系作用（见图1（b））的几何关系可知，样品材料Hugoniot状态的拉格朗日纵波声速CL为
CL=DshsDst12−hs (4) 相应的欧拉纵波声速Cl为
Cl=DshsDst12−hsDs−usDs (5) 式中：hs为样品厚度，下标1和2对应波剖面上不同的时间点。
可以看到，反向碰撞法以波剖面测量为基础，波系作用简单，通过波剖面的粒子速度及时间信息得到高压声速，实验数据具有较高的精度，但是由于可供选择的窗口材料种类较少，目前使用的LiF窗口的阻抗较低，导致实验压力范围有限。
2. 实验结果与分析
实验在中国工程物理研究院流体物理研究所
∅ 30 mm二级轻气炮上进行。将MB2合金飞片安装在弹丸上，将弹丸发射至稳定的弹道速度W，并撞击LiF单晶窗口，通过DPS测量飞片击靶速度以及飞片/窗口界面粒子速度，获得待测材料的冲击波速度和声速。为了提高测试界面对DPS入射光的反射效率，避免靶室残留气体对测试的干扰，窗口击靶面上镀1μm 厚的铝膜并贴8μm 厚铝箔。LiF窗口折射率修正采用Rigg等[12]的公式uw=0.7895×u0.9918 (6) 式中：uw代表修正后的窗口界面粒子速度，u代表实测界面粒子速度，二者单位均为km/s。其中LiF密度为2.638 g/cm3，冲击波速度D和粒子速度u的关系为D=5.150+1.352u（单位km/s）[5]。
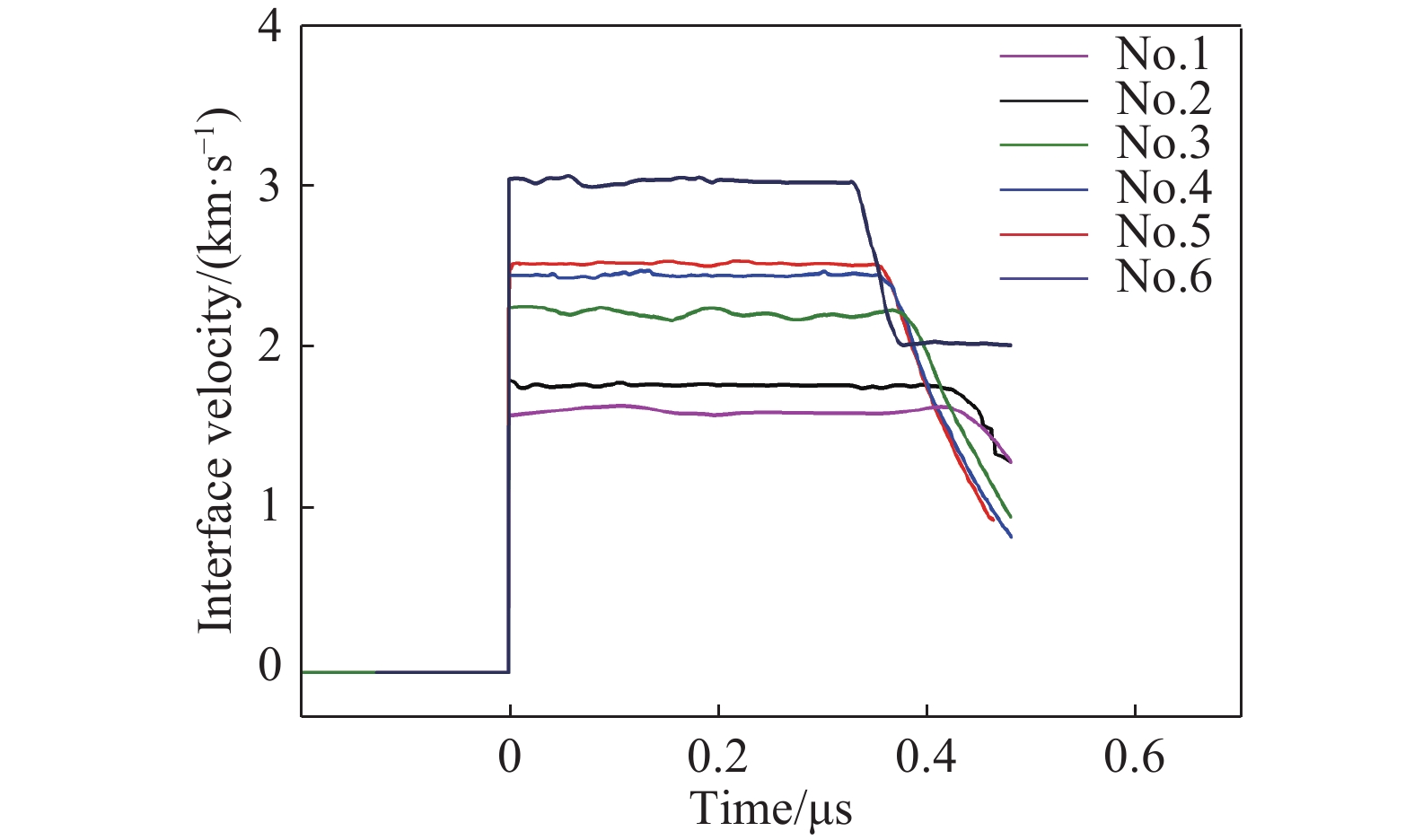
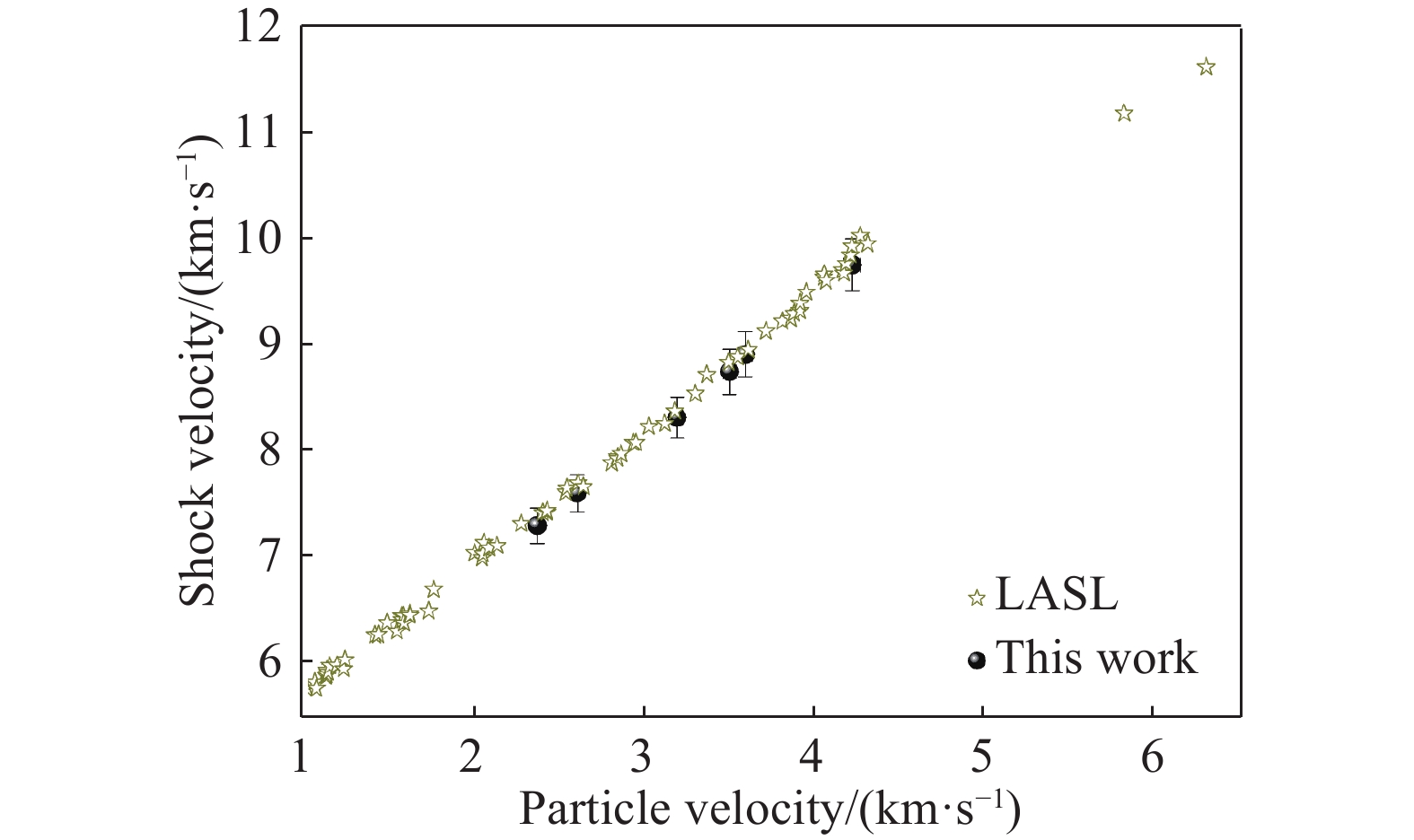
图3给出了6发实验测得的MB2/LiF界面粒子速度剖面（平台高度随加载压力的增大而升高）。通过界面粒子速度剖面，由（1）式～（6）式可得MB2样品在30～73 GPa冲击压力范围内的冲击波速度-粒子速度和声速-压力数据，实验结果列于表1，其中密度采用排水法测量，实测值为(1.775±0.004) g/cm3。冲击波速度-粒子速度数据如图4所示，图中还显示了美国洛斯阿拉莫斯国家实验室（LASL）发表的实验数据[5]。从图4中可以看出，本研究获得的实验数据与已有实验数据具有较好的一致性。
表 1 MB2样品冲击实验参数及结果Table 1. Shock experiment parameters and results of MB2Exp.No. hs/mm W/(km·s–1) uw/(km·s–1) us/(km·s–1) Ds/(km·s–1) p/GPa Cl/(km·s–1) 1 1.980±0.004 3.949±0.020 1.589±0.016 2.360±0.026 7.303±0.166 30.6±0.4 7.983±0.297 2 1.997±0.004 4.358±0.020 1.763±0.018 2.595±0.027 7.607±0.175 35.0±0.5 9.101±0.394 3 1.978±0.004 5.379±0.027 2.195±0.022 3.184±0.030 8.317±0.191 47.0±0.7 9.167±0.402 4 1.981±0.004 5.928±0.030 2.435±0.024 3.493±0.039 8.746±0.214 54.2±0.9 8.912±0.380 5 1.981±0.004 6.100±0.030 2.514±0.025 3.586±0.039 8.907±0.213 56.7±0.9 8.601±0.348 6 1.987±0.004 7.220±0.036 3.003±0.030 4.217±0.050 9.747±0.245 73.0±1.2 9.723±0.463 从图3可以看到：6发实验测得的界面粒子速度剖面质量良好，粒子速度剖面对应的冲击波、卸载波到达样品/窗口界面的特征信号清晰；加载压力为30.6和35.0 GPa时卸载剖面的弹-塑性特征明显，表明MB2合金在该冲击压力下尚未完全熔化；随着加载压力的升高，卸载剖面的弹-塑性特征逐渐消失，当加载压力达到73.0 GPa时，弹-塑性卸载特征完全消失，表明MB2已完全进入熔化相区，与图5所示的不同加载压力下声速转变特征一致。
如图5所示，当加载压力由30.6 GPa增加到35.0 GPa时，纵波声速逐渐增大，由7.983 km/s增大至9.101 km/s；但是，当加载压力增大至47.0 GPa时，纵波声速逐渐向体波声速偏转，跃变为9.167 km/s，预示着随着加载压力的升高，材料内部的剪切效应减小，冲击熔化发生；直至压力达到56.7 GPa时，纵波声速转变为体波声速，由此进一步确认了冲击加载下MB2合金发生熔化。Urtiew等[13]通过理论预测MB2合金在57 GPa附近开始熔化，该结果与本研究根据声速判定的冲击熔化区域基本一致，从而进一步证实了MB2合金在该压力范围内发生冲击熔化，只是Urtiew等预估的理论压力略高。图5中的实线是根据以下公式[11]计算得到的体波声速曲线
C2b=12V2(γV)HpH−V2dpHdV[1−12(γV)H(V0−VH)] (7) 式中：Cb为体波声速；
ρ 、γ 分别为密度和Grüneisen系数，ργ=ρ0γ0 ，ρ0 为材料初始密度，γ0 =1.43[14]。图5中的虚线是基于吴-经方程[15]计算得到的体波声速曲线。可以看出，理论计算的体波声速曲线较冲击加载实验值偏低约10%。这主要是由于合金材料自身成键及结合能的影响，难以采用传统的混合法则或物理模型准确计算物态方程的基本参数，如Grüneisen系数等，由此导致理论预测结果与实验结果出现差异（通常理论值偏低）。根据不确定度传递关系[16]，当全部直接测量量（输入量）Yi彼此独立不相关时，由其确定的间接测量量z的合成不确定度
Δz 由下式确定Δz2=N∑i=1(∂f∂yi)2Δy2i (8) 式中：yi为输入量Yi的直接测量值，z为被测量的测量值，f为z和yi的函数关系，N为输入量的总个数，
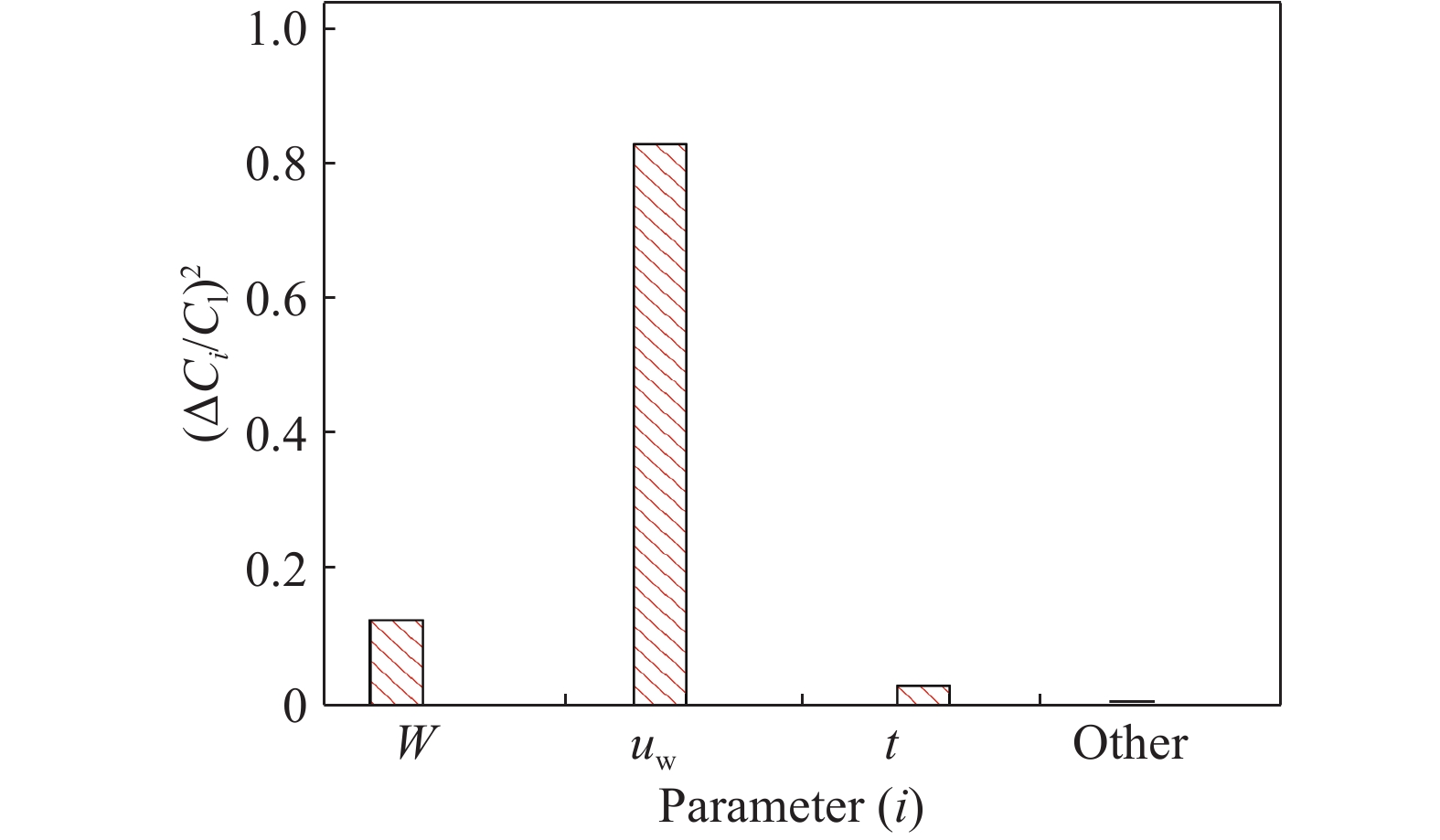
Δyi 为直接测量值的不确定度。因而，当密度为ρ0s 、厚度为hs 的飞片撞击窗口时，样品/窗口界面处粒子速度跳跃，实验测得飞片速度W 、界面粒子速度uw 、时间间隔（剖面平台）t12 ，相应的测量不确定度为Δρ0s 、Δhs 、ΔW 、Δuw 、Δt12 ，而窗口Hugoniot参数（ρ0w 、C0w 、λw ）的不确定度（Δρ0w 、ΔC0w 、Δλw ）已知。由于各测量量是独立测量的，（C0w ，λw ）相互作用项较小，可忽略不计，据此可根据不确定度传递律确定声速测量的不确定度。如图6所示，就该例反碰撞实验（No.2）而言，影响声速测量不确定度的因素很多，包括样品初始密度、厚度、飞片速度、界面粒子速度、追赶时间、窗口材料冲击Hugoniot参数等。样品内部冲击压缩状态（如粒子速度、冲击波速度、冲击压力等）均通过飞片速度、界面粒子速度及窗口Hugoniot参数计算获得，影响声速测量不确定度的主要因素在于飞片速度、界面粒子速度及稀疏波追赶时间（平台时间），所占比例（即对（8）式中各平方项求和，下同）约为声速测量不确定度的99%，其余如初始密度、厚度等参量在当前诊断水平下对声速测量不确定度的贡献较小，约占总体的1%。在现有诊断条件下，飞片速度采用DPS直接测量，测量扩展不确定度不大于0.5%；界面粒子速度剖面的测量不确定度主要受平台区速度及稀疏波追赶时间的影响，影响因素包含干涉信号数据转换精度、窗口折射率修正、起跳及卸载时刻的判断等，综合而言，平台区速度测量的扩展不确定度不大于1%，追赶时间测量的扩展不确定度约6 ns。总体而言，传递至声速的测量扩展不确定度不超过5%。
图 6 反碰撞法测量声速实验中不确定度Figure 6. Uncertainties for backward-impact experiment based on law of propagation3. 结 论
采用反向碰撞实验技术，结合具有高时空分辨率的DPS，获得了MB2合金在30～73 GPa压力范围内的冲击Hugoniot及声速数据。随着加载压力的升高，MB2合金纵波声速呈现出明显的向体波声速转变的趋势，预示着材料内部的剪切效应逐渐减小，冲击熔化发生，其相变压力区间为40～57 GPa。该实验结果与不同加载压力下卸载波剖面对应的弹-塑性转变特征完全一致，由此进一步确认了冲击加载下MB2合金熔化相变的发生。
感谢中国工程物理研究院流体物理研究所黄金、康强、叶素华、方茂林、向曜民、陈志云等在实验过程中给予帮助。
-
图 1 初始Cu-He正弦单模界面模型及参数定义
Figure 1. Initial characteristic parameters of single mode sinusoidal Cu-He interface model
图 2 宏观流体动力学模拟的单模RMI（左）与MD模拟的微观结果（右）的唯象相似性
Figure 2. Macroscopic hydrodynamics and microscopic molecular dynamics simulations of single mode RMI (left) with similar phenomenological results (right)
图 4 微观RMI现象典型时刻的密度分布与原子分布
Figure 4. Density contours and atom arrangements map of RMI simulations by MD at typical time constants
图 6 不同尺度模型的无量纲振幅-时间曲线
Figure 6. Non-dimensional amplitude evolution histories of different scale simulations
图 7 不同尺度、相同无量纲时刻的密度等值图
Figure 7. Density contours of different scale simulations with the same non-dimensional time constants
图 8 不同冲击加载速度下的无量纲振幅-时间曲线
Figure 8. Non-dimensional time histories of amplitude evolution under different Vp
图 9 不同初始振幅波长比条件下的无量纲振幅-时间曲线
Figure 9. Non-dimensional time histories of amplitude evolution with different initial amplitude/wave length ratio
表 1 RMI不同尺度计算结果的参数统计
Table 1. Computed statistical results of RMI parameters at different scales
λ/nm Wi/(km·s–1) δWi/% Wt/(km·s–1) δWt/% U/(km·s–1) δU/% V0/(km·s–1) δV0/% A δA/% 7.3 11.5 0.3 18.1 1.4 11.5 1.8 3.16 2.3 –0.83 1.1 14.5 11.7 2.1 17.6 1.3 11.9 1.5 3.19 1.4 –0.83 1.1 29.0 11.5 0.3 18.2 2.0 11.7 0.1 3.23 0.1 –0.84 0 72.5 11.4 0.5 17.7 0.7 12.1 3.2 3.28 1.3 –0.85 1.1 145.0 11.2 2.2 17.6 1.3 11.4 2.7 3.32 2.5 –0.85 1.1 
下载: 导出CSV
表 2 不同冲击加载速度下RMI参数
Table 2. Parameters of RMI under different Vp
Vp/(km·s–1) Wi/(km·s–1) Wt/(km·s–1) U/(km·s–1) V0/(km·s–1) A 6 11.7 17.6 11.9 3.22 –0.83 9 14.2 24.4 16.6 3.43 –0.84 12 16.5 33.5 21.9 3.57 –0.85 15 20.6 41.7 28.9 3.74 –0.83
下载: 导出CSV
表 3 不同初始振幅波长比条件下RMI参数
Table 3. Parameters of RMI with different initial amplitude/wave length ratio
a Wi/(km·s–1) Wt/(km·s–1) U/(km·s–1) V0/(km·s–1) A 0.20 11.7 17.6 11.9 3.22 –0.83 0.10 11.7 17.8 11.8 2.00 –0.85 0.05 11.6 18.0 11.6 1.03 –0.84
下载: 导出CSV
-
[1] RICHTMYER R D. Taylor instability in shock acceleration of compressible fluids [J]. Communications on Pure and Applied Mathematics, 1960, 13(2): 297–319. doi: 10.1002/(ISSN)1097-0312 [2] MESHKOV E E. Instability of the interface of two gases accelerated by a shock wave [J]. Fluid Dynamics, 1969, 4(5): 101–104. [3] ANDREWS M J. Workshop: research needs for material mixing at extremes: LA-UR-11-02565 [R]. Los Alamos: Los Alamos National Laboratory, 2011. [4] GRAZIANI F R, BATISTA V S, BENEDICT L X, et al. Large-scale molecular dynamics simulations of dense plasmas: the Cimarron Project [J]. High Energy Density Physics, 2012, 8(1): 105–131. doi: 10.1016/j.hedp.2011.06.010 [5] ZHAKHOVSKII V, NISHIHARA K, ABE M. Molecular dynamics simulation on stability of converging shocks [C]// TANAKA K A, MEYERHOFER D D, MEYER-TER-VEHN J. Proceedings of the 2nd International Conference on Inertial Fusion Science and Applications. Paris: Elsevier, 2002: 106-109. [6] KADAU K, GERMANN T C, HADJICONSTANTINOU N G, et al. Nanohydrodynamics simulations: an atomistic view of the Rayleigh-Taylor instability [J]. Proceedings of the National Academy of Sciences, 2004, 101(16): 5851–5855. doi: 10.1073/pnas.0401228101 [7] ZYBIN S V, ZHAKHOVSKII V V, BRINGA E M, et al. Molecular dynamics simulations of the Richtmyer-Meshkov instability in shock loaded solids [J]. AIP Conference Proceedings, 2006, 845(1): 437–441. [8] CHERNE F J, DIMONTE G, GERMANN T C. Richtmyer-Meschov instability examined with large-scale molecular dynamic simulations: LA-UR-11-04503 [R]. Los Alamos: Los Alamos National Laboratory, 2011. [9] 龚新高. 高温及高压下液体镓的结构——第一性原理分子动力学方法研究 [J]. 物理学报, 1995, 44(6): 885–896GONG X G. Structural properties of liquid gallium at high temperature and high pressure—an ab initio molecular dynamics study [J]. Acta Physica Sinica, 1995, 44(6): 885–896 [10] 何以广. 氢和氦高压物性的第一原理分子动力学研究及实验探索 [D]. 北京: 清华大学, 2010. [11] 张玉娟. 温稠密乙烷等流体物性的第一性原理分子动力学研究 [D]. 北京: 中国工程物理研究院, 2013. [12] 刘海, 李启楷, 何远航. 高速冲击压缩梯恩梯的分子动力学模拟 [J]. 力学学报, 2015, 47(1): 174–179LIU H, LI Q K, HE Y H. Molecular dynamics simulations of high velocity shock compressed TNT [J]. Chinese Journal of Theoretical and Applied Mechanics, 2015, 47(1): 174–179 [13] 王裴, 秦承森, 张树道, 等. SPH方法对金属表面微射流的数值模拟 [J]. 高压物理学报, 2004, 18(2): 149–156 doi: 10.3969/j.issn.1000-5773.2004.02.010WANG P, QIN C S, ZHANG S D, et al. Simulated microjet from free surface of aluminum using smoothed particle hydrodynamics [J]. Chinese Journal of High Pressure Physics, 2004, 18(2): 149–156 doi: 10.3969/j.issn.1000-5773.2004.02.010 [14] HUANG S, WANG W, LUO X. Molecular-dynamics simulation of Richtmyer-Meshkov instability on a Li-H2 interface at extreme compressing conditions [J]. Physics of Plasmas, 2018, 25(6): 062705. doi: 10.1063/1.5018845 [15] DAW M S, BASKES M I. Embedded-atom method: derivation and application to impurities, surfaces, and other defects in metals [J]. Physical Review B, 1984, 29(12): 6443. doi: 10.1103/PhysRevB.29.6443 [16] ACKLAND G J, BACON D J, CALDER A F, et al. Computer simulation of point defect properties in dilute Fe-Cu alloy using a many-body interatomic potential [J]. Philosophical Magazine A, 1997, 75(3): 713–732. doi: 10.1080/01418619708207198 [17] GERMANN T C, DIMONTE G, HAMMERBERG J E, et al. Large-scale molecular dynamics simulations of particulate ejection and Richtmyer-Meshkov instability development in shocked copper [C]// DYMAT 2009, EDP Sciences, 2009: 1499-1505. [18] RIKANATI A, ORON D, SADOT O, et al. High initial amplitude and high Mach number effects on the evolution of the single-mode Richtmyer-Meshkov instability [J]. Physical Review E, 2003, 67(2): 026307. doi: 10.1103/PhysRevE.67.026307 [19] DIMONTE G, RAMAPRABHU P. Simulations and model of the nonlinear Richtmyer-Meshkov instability [J]. Physics of Fluids, 2010, 22(1): 014104. doi: 10.1063/1.3276269 [20] HOLMES R L, DIMONTE G, FRYXELL B, et al. Richtmyer-Meshkov instability growth: experiment, simulation and theory [J]. Journal of Fluid Mechanics, 1999, 389: 55–79. doi: 10.1017/S0022112099004838 -
下载:
下载:
点击查看大图
计量
- 文章访问数: 7823
- HTML全文浏览量: 4376
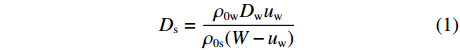
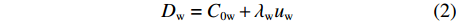
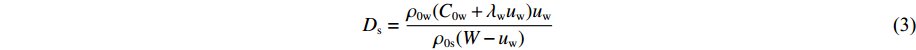
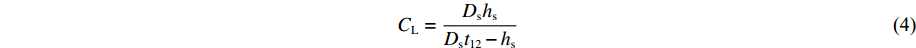
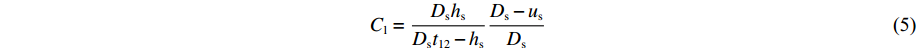
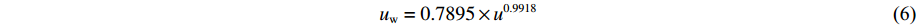
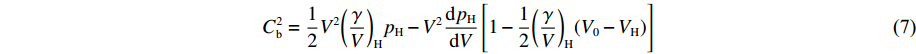
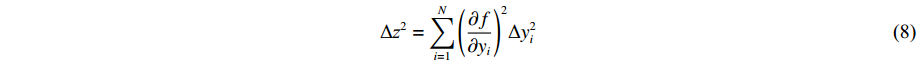
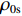
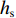
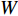
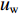
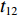
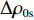
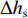
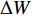
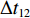
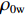
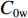
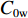
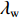
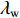
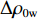
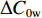
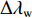
- PDF下载量: 31
